A Study of the Complexity of Problems Posed by Talented Students in Mathematics
Abstract
1. Introduction
“The process of creating problems represents one of the forms of authentic mathematical research. Properly implemented in class activities, it has the potential to go beyond the limitations of word problems—at least as they are typically treated. Promoting creation of problems is one way to achieve development of different student potentialities and stimulate greater mental flexibility.”(p. 53)
Study Variables Related to the Complexity of Mathematical Problems
2. Methodology
2.1. Participants
2.2. Instrument
2.3. Categories of Analysis
2.3.1. Category I: Syntactical Complexity
2.3.2. Category II: Mathematical Complexity
2.4. Data Analysis
3. Results
3.1. General Characteristics of the Problems Posed
3.2. Analysis by Syntactical Complexity
“If the train at its maximum capacity can reach a maximum speed of 500 km/h, in addition the average weight of each person is 75 kg and if a passenger car and three freight cars are added, it can reach a maximum speed of 400 km/h At what time does he arrive in Alajuela if in the middle of the journey he makes a 20-min stop and returns his number of wagons to the first mentioned, knowing that it travels at a maximum speed at all times and that from Cartago to Alajuela there are 5554 km?”
“In a train with six cars for passengers, two for parcels and another two to transport animals, it leaves the Pérez Zeledón station at 7:00 am and arrives in San José at 11:00 am. Find out how long it takes to get there and how many people it carries in each car if there are 253 people in total.”
3.3. Analysis by Mathematical Complexity
“If boy A has run a distance of 560 m and girl B has a total of 480 m, how many laps does boy C need to catch up with boy A, knowing that boy C has run a distance of 160 m?”
“Juan, Carlos and María are doing a race that consists of 5 laps. If while Maria completes the first lap, Carlos goes for the third and Juan for the second, Juan goes at a speed of 15 (m)/s and Carlos goes at a speed of 20 m/s. How fast must Maria go to be the winner?”
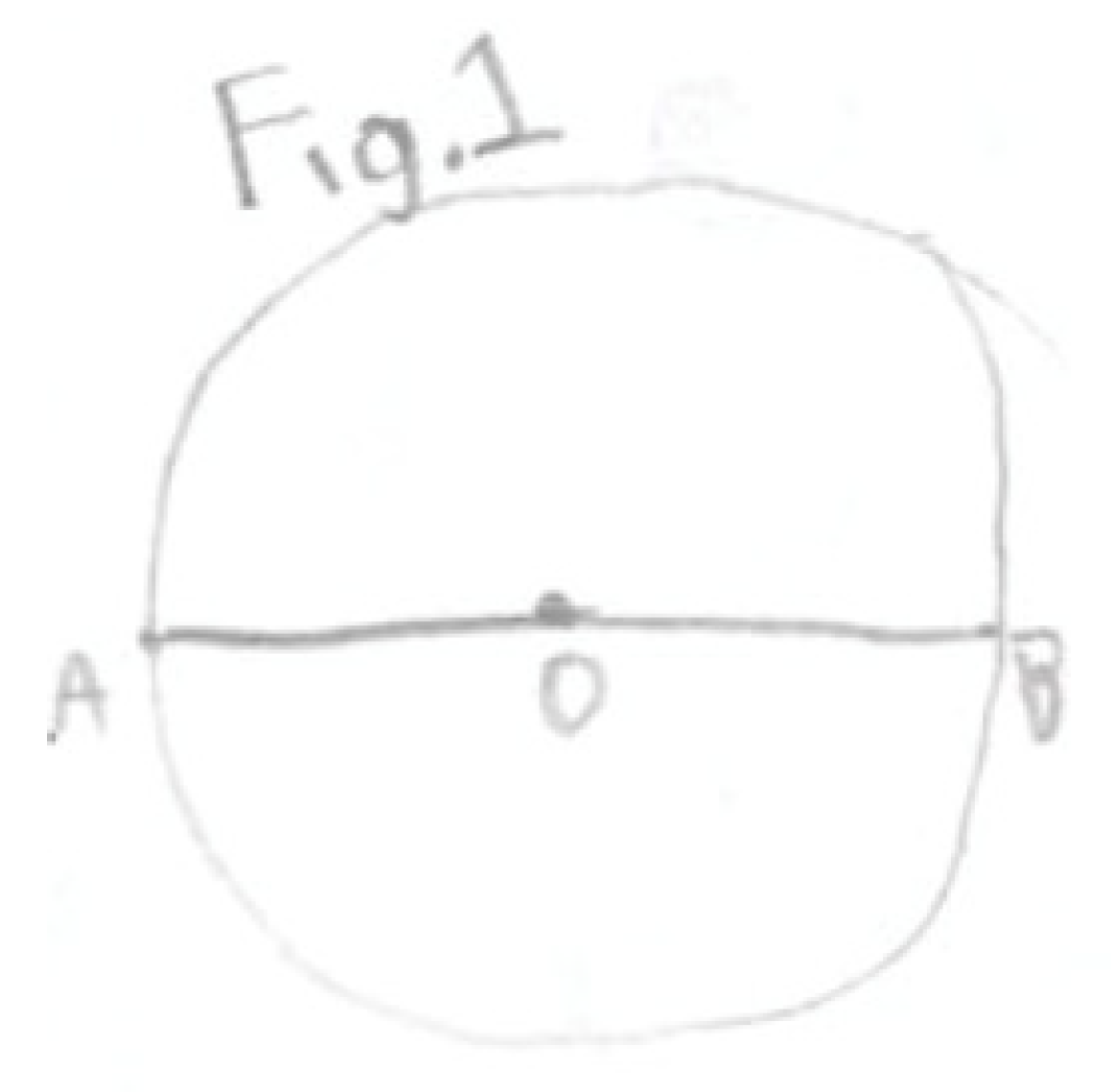
“If I have a hexagonal prism whose volume is of the volume of the sphere in Fig. 1; AB is three units greater than the radius of the hexagon, whose perimeter is equivalent to where and m; and x is equal to the value in degrees of ; what is the area of the hexagonal prism?”
4. Discussion and Conclusions
5. Limitations
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Singer, F.M.; Ellerton, N.; Cai, J. Problem-posing research in mathematics education: New questions and directions. Educ. Stud. Math. 2013, 83, 1–7. [Google Scholar] [CrossRef]
- Singer, F.M.; Sheffield, L.S.; Freiman, V.; Brandl, M. Research on and Activities for Mathematically Gifted Education; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Sánchez, L.; Juárez, E.L.; Juárez, J.A. Análisis de creatividad en el planteamiento de problemas de ecuaciones lineales. Rev. Iber. Ed. Mat. 2020, 16, 119–134. [Google Scholar]
- Ayllón, M.F.; Gómez, I. La invención de problemas como tarea escolar. Esc. Abi. 2014, 17, 29–40. [Google Scholar]
- Mora-Badilla, M.; Gutiérrez, Á. Habilidades de visualización en niños de Primaria con alta capacidad matemática. In Proceedings of the Jornadas Internacionales de Investigación y Práctica Docente en Alta Capacidad Matemática, Logroño, Spain, 19–20 November 2021. [Google Scholar]
- Pelczer, I.; Voica, C.; Gamboa, F. Problem Posing Strategies of first year Mathematics students. In Proceedings of the Joint Meeting of PME 32 and PME-NA XXX, Morelia, México, 17–21 July 2008. [Google Scholar]
- Castro, E. La invención de problemas y sus ámbitos de investigación. In Proceedings of the Investigaciones en Pensamiento Numérico y Algebraico e Historia de la Matemática, Granada, España, 10 February 2011. [Google Scholar]
- Espinoza, J.; Lupiáñez, J.L.; Segovia, I. La invención de problemas aritméticos por estudiantes con talento matemático. Elec. J. Res. Educ. Psych. 2016, 14, 368–392. [Google Scholar] [CrossRef][Green Version]
- Malaspina, U. Creación de problemas y juegos para el aprendizaje de las Matemáticas. Ed. Mat. Inf. 2021, 10, 1–17. [Google Scholar] [CrossRef]
- Kaba, Y.; Şengül, S. Developing the Rubric for Evaluating Problem Posing (REPP). Int. Online J. Educ. Sci. 2016, 8, 8–25. [Google Scholar] [CrossRef]
- Silver, E.; Cai, J. Assessing students’ mathematical problem posing. Teach. Chil. Math. 2005, 12, 129–135. [Google Scholar] [CrossRef]
- Bonotto, C. Artifacts as sources for problem-posing activities. Educ. Stud. Math. 2013, 83, 37–55. [Google Scholar] [CrossRef]
- Ayllón, M.F.; Gallego, J.L.; Gómez, I.A. La actuación de estudiantes de educación primaria en un proceso de invención de problemas. Perf. Educ. 2016, 38, 51–67. [Google Scholar]
- Malaspina, U. Creación de problemas: Sus potencialidades en la enseñanza y aprendizaje de las matemáticas. Cuad. Inv. Form. Educ. Mat. 2016, 11, 321–331. [Google Scholar]
- Fernández, J.A.; Barbarán, J.J. Inventar Problemas Para Desarrollar la Competencia Matemática; Editorial la Muralla: Madrid, Spain, 2015. [Google Scholar]
- Ramírez, R.; Cañadas, M. Nominación y atención del talento matemático por parte del docente. Rev. Did. Mat. 2018, 79, 23–30. [Google Scholar]
- Lupiáñez, J.L.; Espinoza, J. ¿Es la excelencia matemática una prioridad curricular? Cuad. Inv. Form. Educ Mat. 2019, 18, 130–138. [Google Scholar]
- Rodríguez-Naveiras, E.; Verche, E.; Hernández-Lastrini, P.; Montero, R.; Borges, A. Differences in working memory between gifted or talented students and community samples: A meta-analysis. Psicothema 2019, 31, 255–262. [Google Scholar] [PubMed]
- Gutiérrez, M.P.; Maz, A. Educación y Diversidad. In La Educación de Niños con Talento en Iberoamerica, 2nd ed.; Benavides, M., Maz, A., Castro, E., Blanco, R., Eds.; Oreal-Unesco: Santiago, Chile, 2004; Volume 1, pp. 15–21. [Google Scholar]
- Benavides, M. Caracterización de Sujetos con Talento en Resolución de Problemas de Estructura Multiplicativa. Ph.D. Thesis, University of Granada, Granada, Spain, 2008. [Google Scholar]
- García, R. Diseño y Validación de un Instrumento de Evaluación de la Competencia Matemática. Rendimiento Matemático de los Alumnos Más Capaces. Ph.D. Thesis, National University of Distance Education, Madrid, Spain, 2014. [Google Scholar]
- Espinoza, J. Invención de Problemas por Estudiantes con Talento en Matemática: Un Estudio Exploratorio. Master’s Thesis, University of Granada, Granada, Spain, 2011. [Google Scholar]
- Krutetskii, V.A. The Psychology of Mathematical Abilities in School Children; University of Chicago Press: Chicago, IL, USA, 1976. [Google Scholar]
- Ellerton, N. Children’s made-up mathematics problems: A new perspective on talented mathematicians. Educ. Stud. Math. 1986, 17, 261–271. [Google Scholar] [CrossRef]
- Silver, E.; Cai, J. An analysis of arithmetic problem posing by middle school students. J. Res. Math. Ed. 1996, 27, 521–539. [Google Scholar] [CrossRef]
- OCDE. El programa PISA de la OCDE. Qué es y Para Qué Sirve? OCDE: Paris, Francia, 2006. [Google Scholar]
- Stein, M.K.; Smith, M.; Henningsen, M.; Silver, E.A. Implementing Standards-Based Mathematics Instruction: A Casebook for Professional Development, 2nd ed.; Teachers College Press: New York, NY, USA, 2009. [Google Scholar]
- Puig, L.; Cerdan, F. Problemas Aritméticos; Síntesis: Madrid, Spain, 1988. [Google Scholar]
- Espinoza, J.; Segovia, I.; Lupiáñez, J.L. Un esquema para analizar los enunciados de los estudiantes en contextos de invención de problemas. Uniciencia 2015, 29, 58–81. [Google Scholar]
- Gregorio, J.R. La resolución de problemas en primaria. Sigma 2005, 27, 9–34. [Google Scholar]
- Castro, E. Niveles de Comprensión en Problemas Verbales de Comparación Multiplicativa. Ph.D. Thesis, University of Granada, Granada, Spain, 1995. [Google Scholar]
- Cohen, L.; Manion, L.; Morrison, K. Research Methods in Education, 6th ed.; Routledge Falmer: New York, NY, USA, 2007. [Google Scholar]
- Raven, J.C.; Court, J.H.; Raven, J. Test de Matrices Progresivas. Escalas Coloreadas, General y Avanzadas; Paidós: Buenos Aires, Argentina, 1993. [Google Scholar]
- Stoyanova, E. Problem posing in mathematics classrooms. In Research in Mathematics Education: A Contemporary Perspective; McIntosh, A., Ellerton, N., Eds.; Mathematics, Science and Technology Education Centre Edith Cowan University: Perth, Australia, 1998; pp. 164–185. [Google Scholar]
- Reyes-Santander, P.; Karg, A. Una aproximación al trabajo con niños especialmente dotados en matemática. In Proceedings of the SEIEM XIII, Granada, Spain, 10–12 September 2009. [Google Scholar]
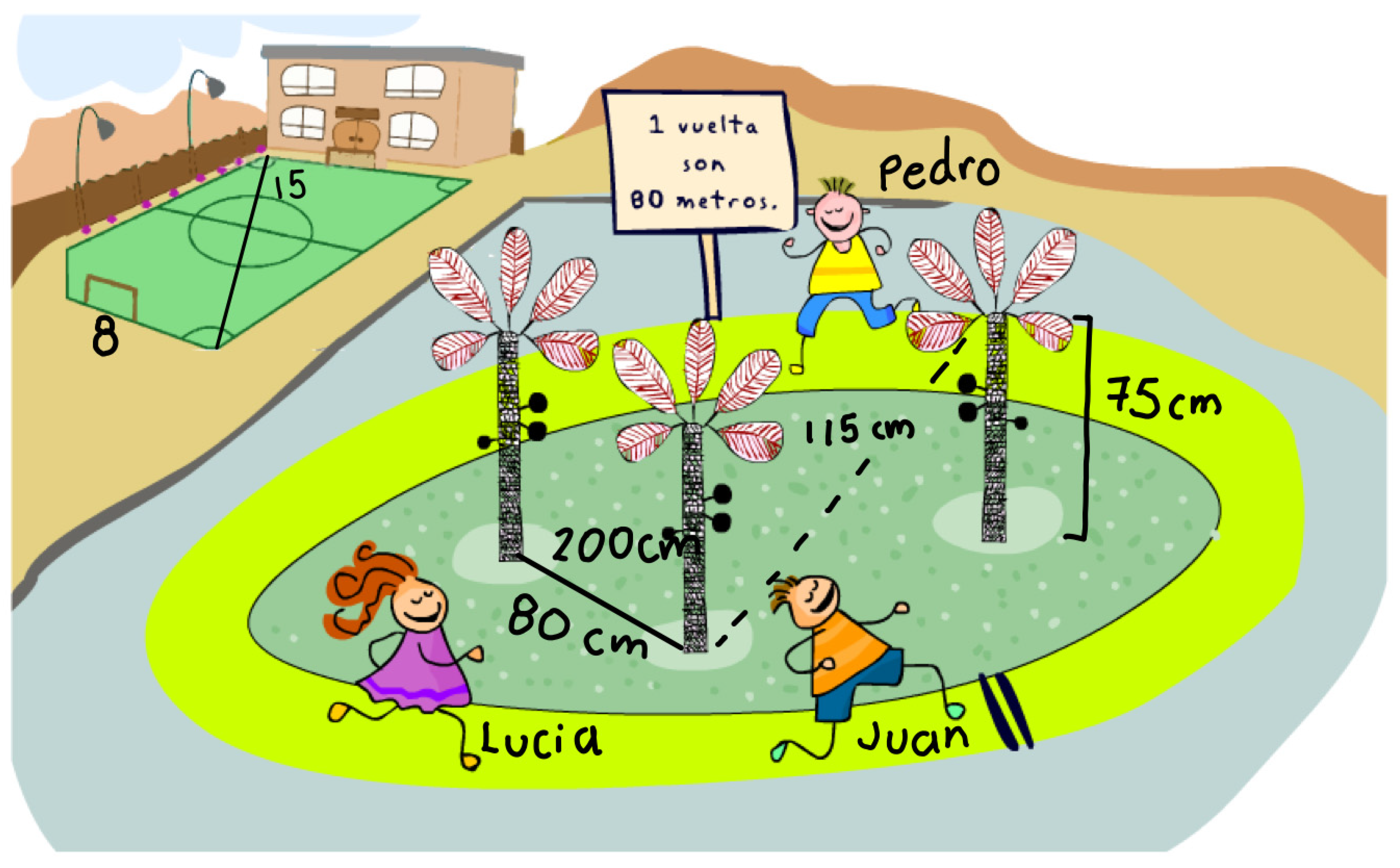
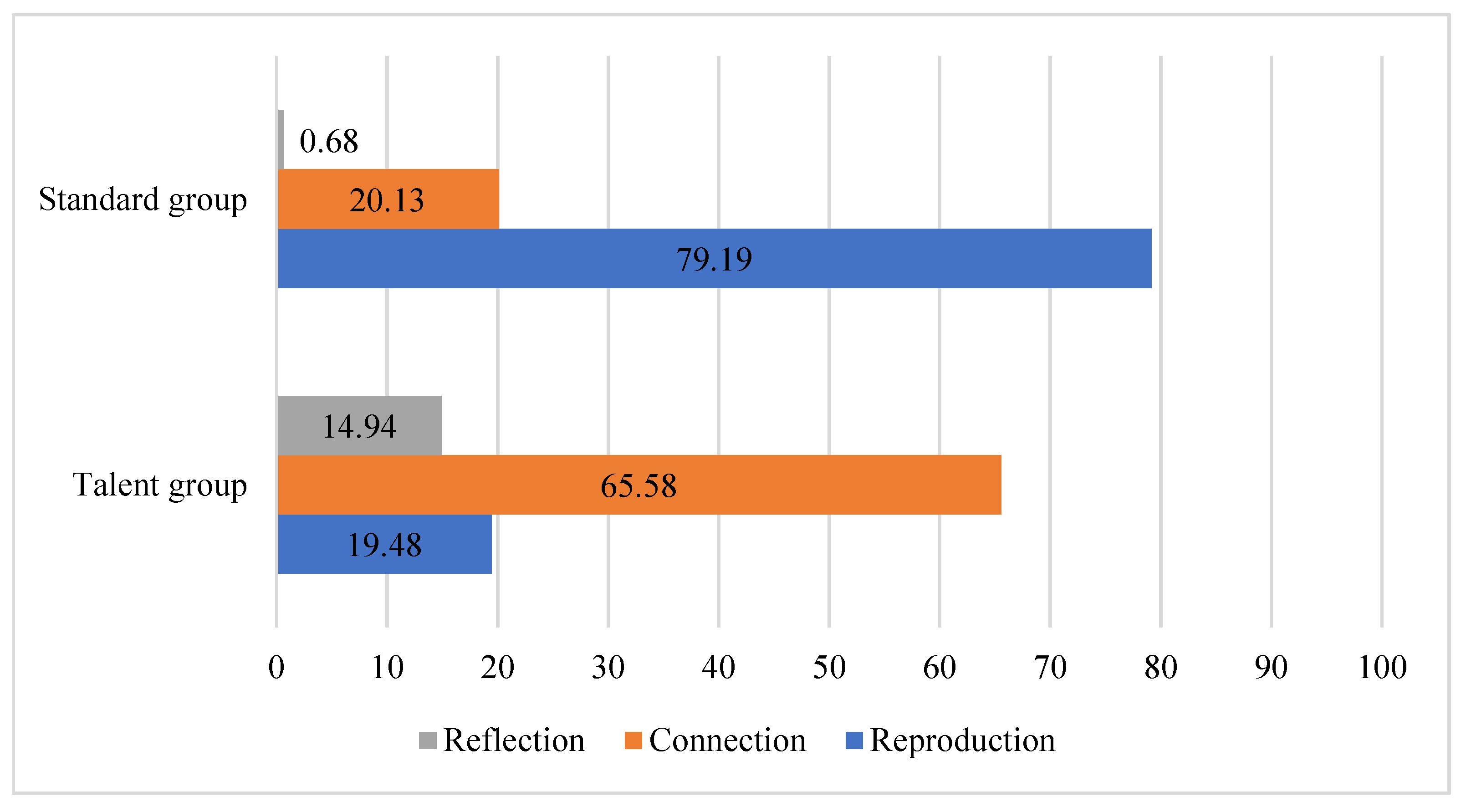
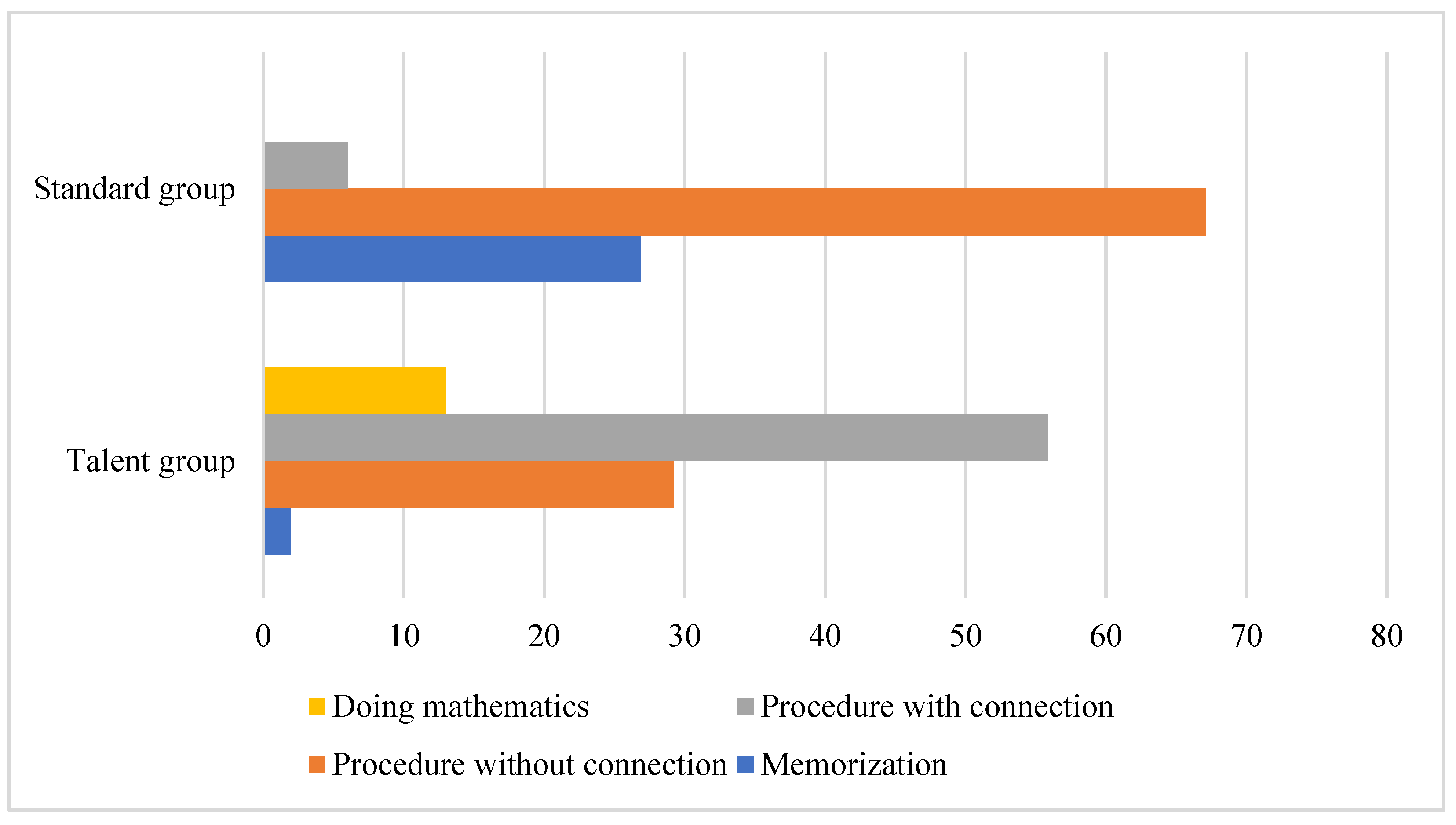
| Number of Propositions | Talent Group | Standard Group |
|---|---|---|
| One proposition | 4 (2.60) 1 | 18 (12.08) |
| Two propositions | 27 (17.53) | 69 (46.31) |
| Three propositions | 48 (31.17) | 47 (31.54) |
| Four propositions | 41 (26.62) | 11 (7.38) |
| Five or more propositions | 31 (22.08) | 4 (2.68) |
| Total | 154 | 149 |
| Number of Steps | Talent Group | Standard Group |
|---|---|---|
| One step | 2 (1.30) 1 | 26 (17.45) |
| Two steps | 24 (15.58) | 68 (45.64) |
| Three steps | 32 (20.78) | 41 (27.52) |
| Four steps | 29 (18.83) | 11 (7.38) |
| Five or more steps | 67 (43.51) | 3 (2.01) |
| Total | 154 | 149 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Espinoza, J.; Lupiáñez, J.L.; Segovia, I. A Study of the Complexity of Problems Posed by Talented Students in Mathematics. Mathematics 2022, 10, 1841. https://doi.org/10.3390/math10111841
Espinoza J, Lupiáñez JL, Segovia I. A Study of the Complexity of Problems Posed by Talented Students in Mathematics. Mathematics. 2022; 10(11):1841. https://doi.org/10.3390/math10111841
Chicago/Turabian StyleEspinoza, Johan, José Luis Lupiáñez, and Isidoro Segovia. 2022. "A Study of the Complexity of Problems Posed by Talented Students in Mathematics" Mathematics 10, no. 11: 1841. https://doi.org/10.3390/math10111841
APA StyleEspinoza, J., Lupiáñez, J. L., & Segovia, I. (2022). A Study of the Complexity of Problems Posed by Talented Students in Mathematics. Mathematics, 10(11), 1841. https://doi.org/10.3390/math10111841







