Domination and Independent Domination in Hexagonal Systems
Abstract
:1. Motivation
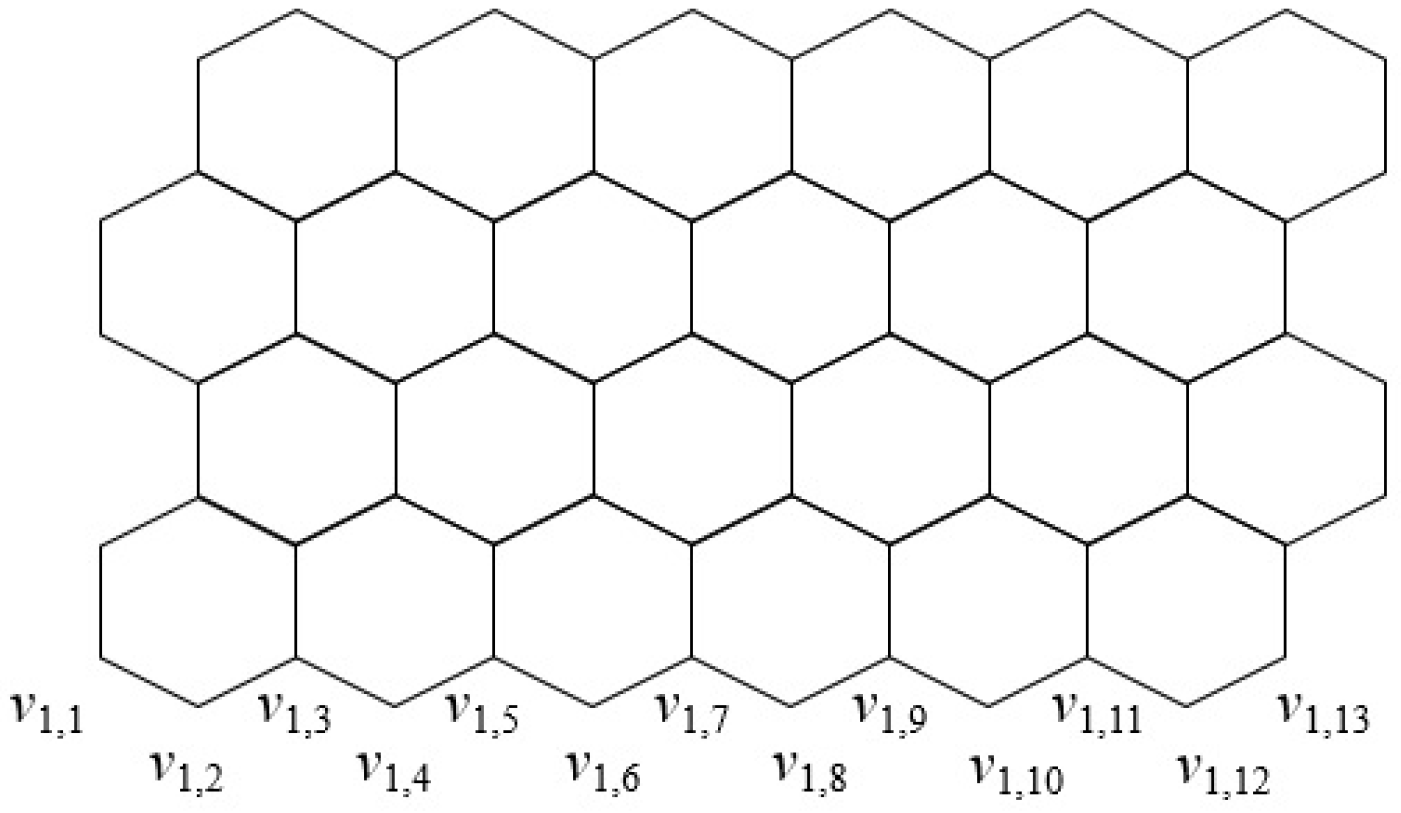
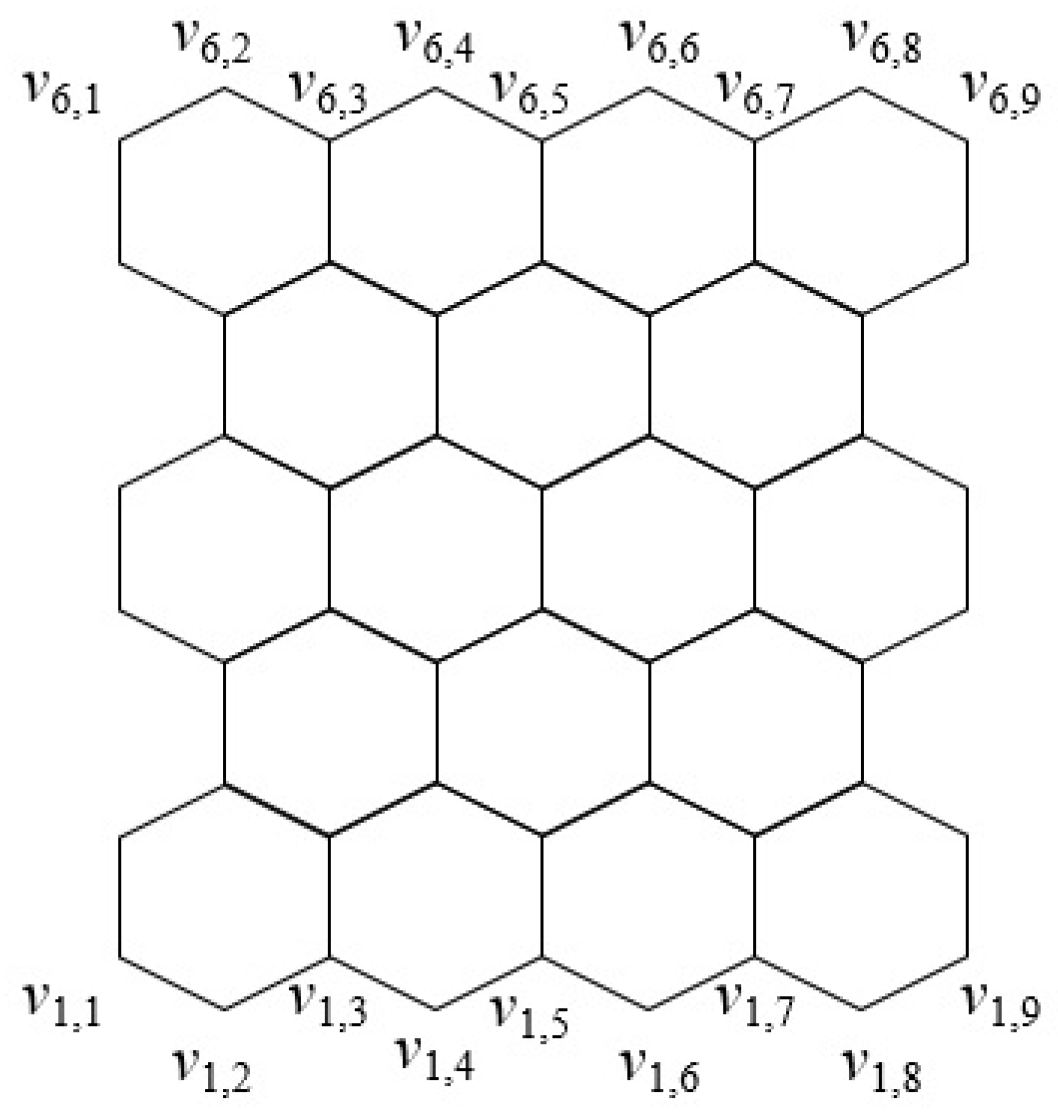
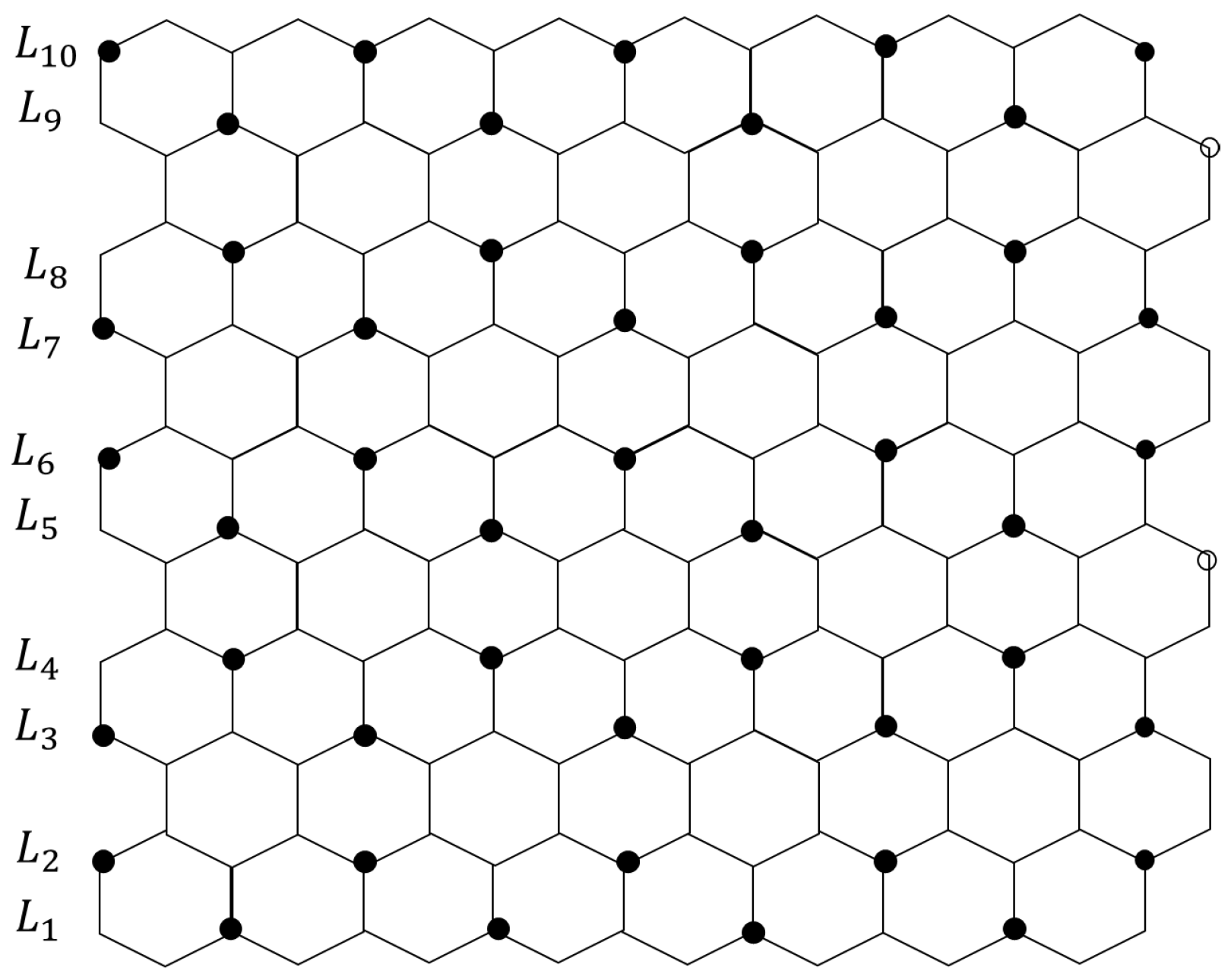
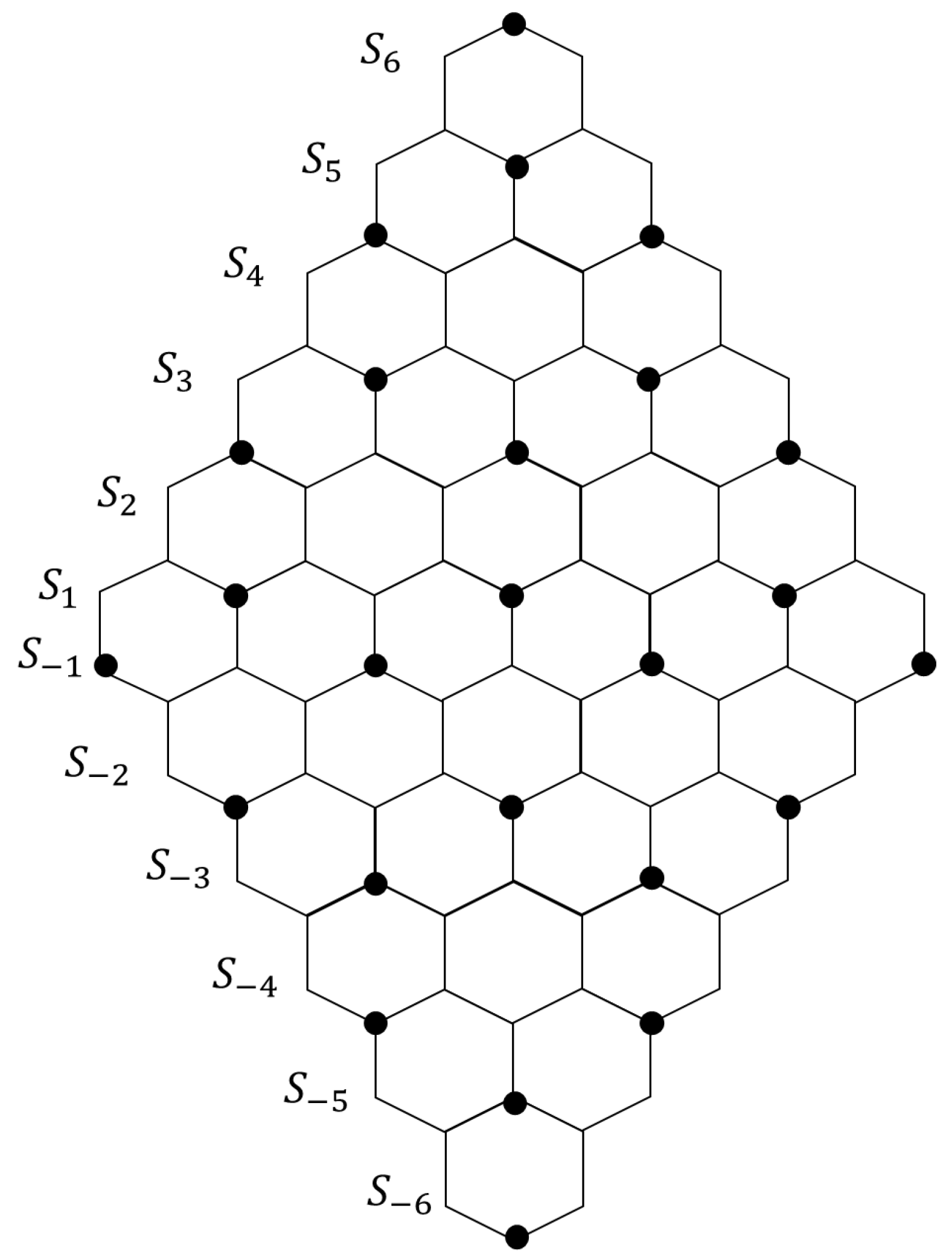
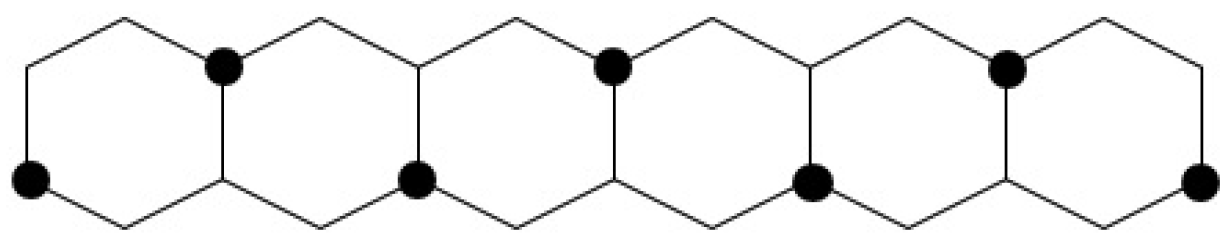
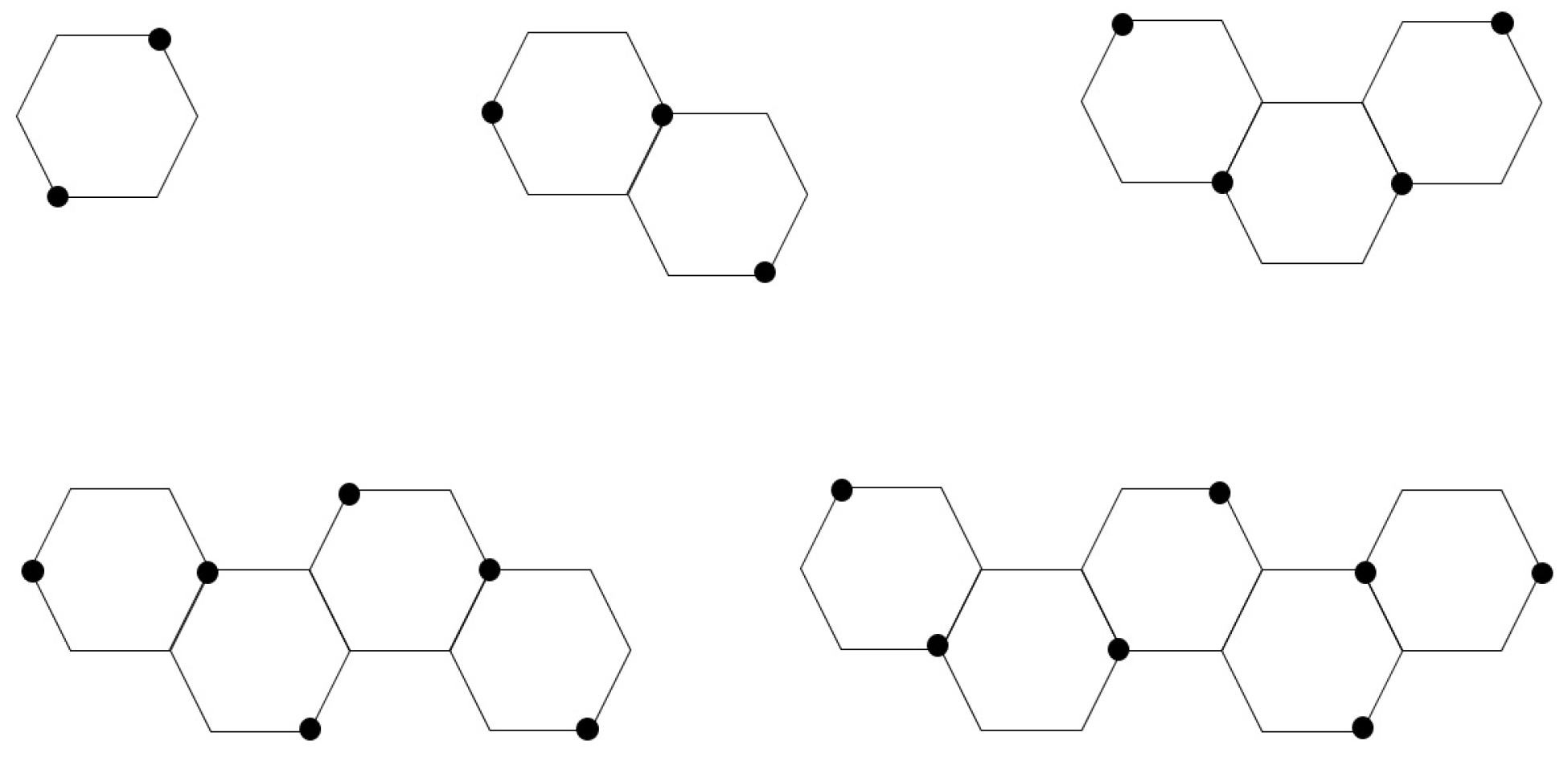
2. Catacondensed and Pericondensed Hexagonal Systems
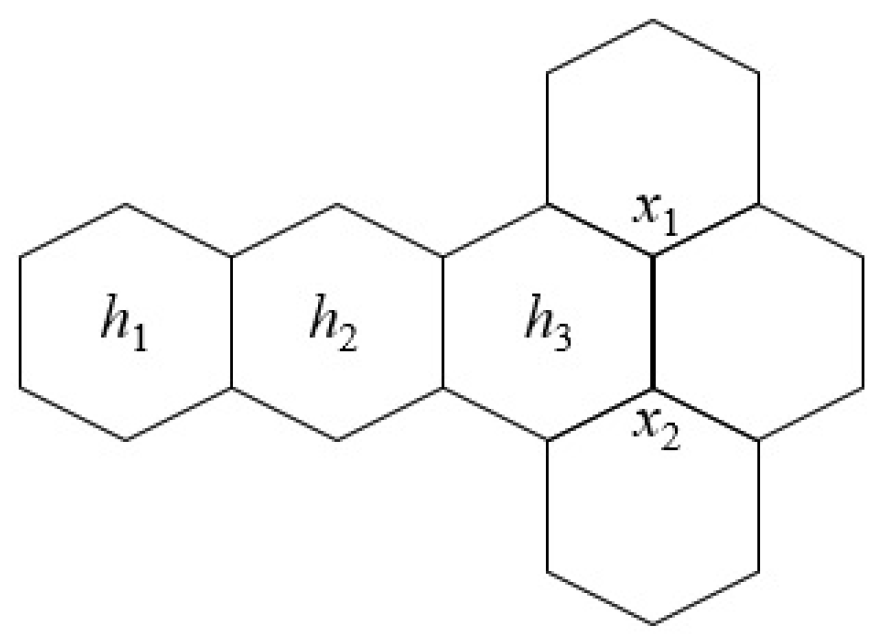
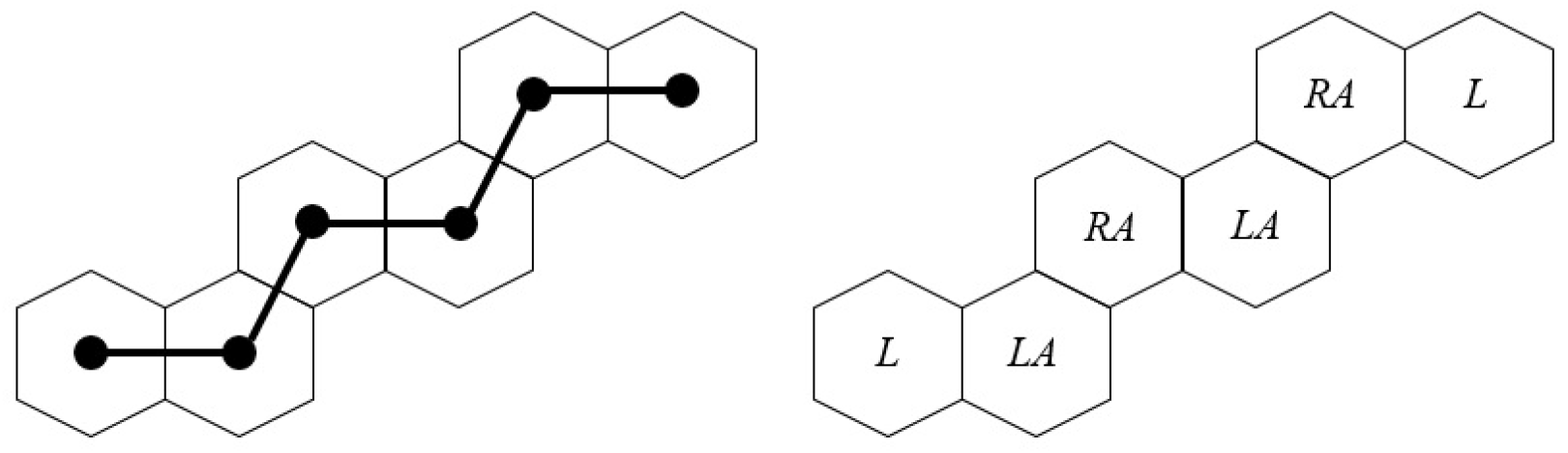
2.1. Catacondensed Hexagonal Systems
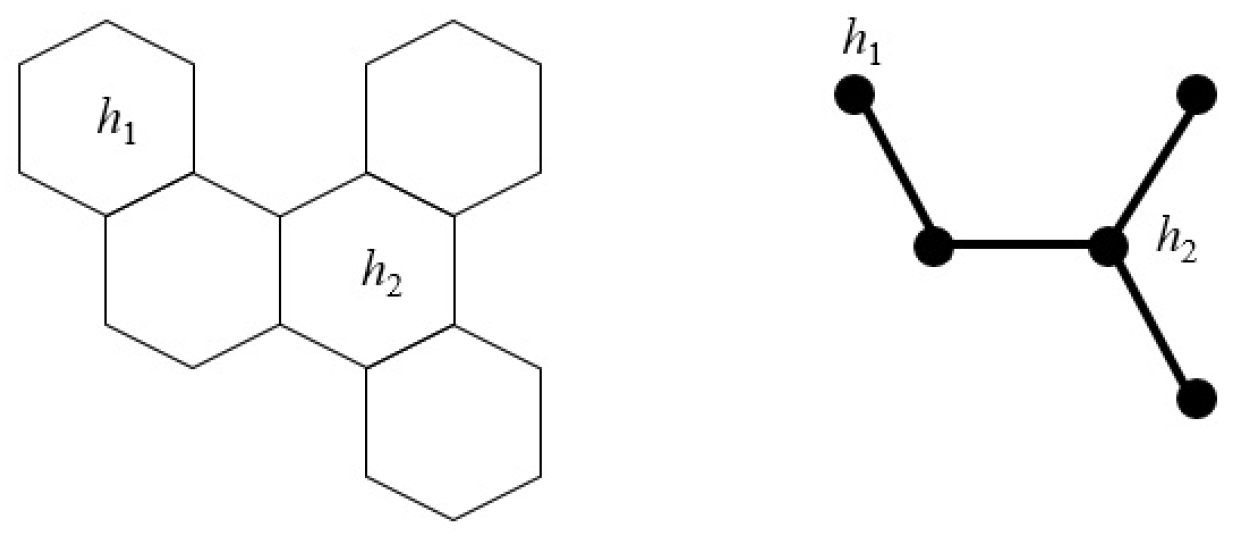
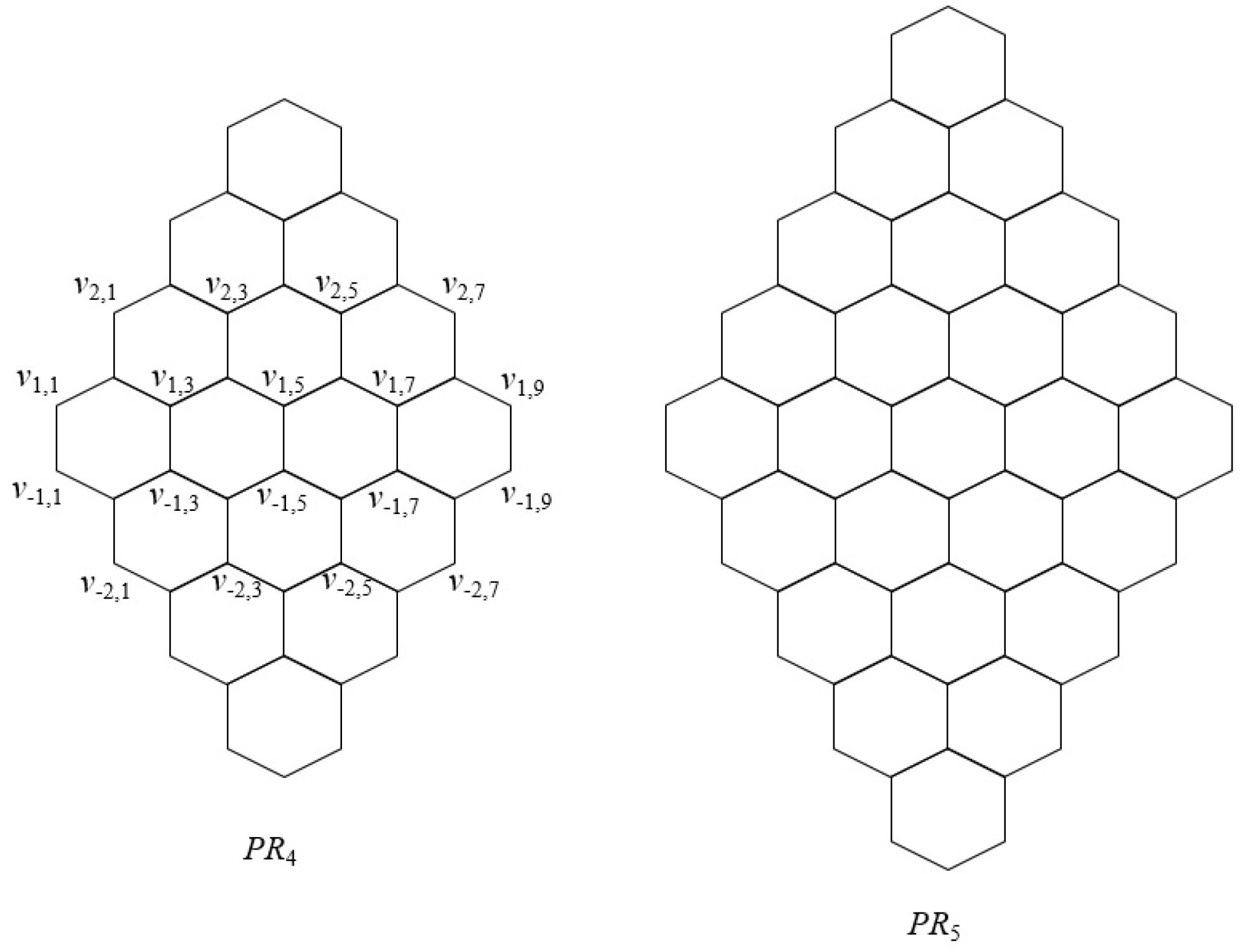
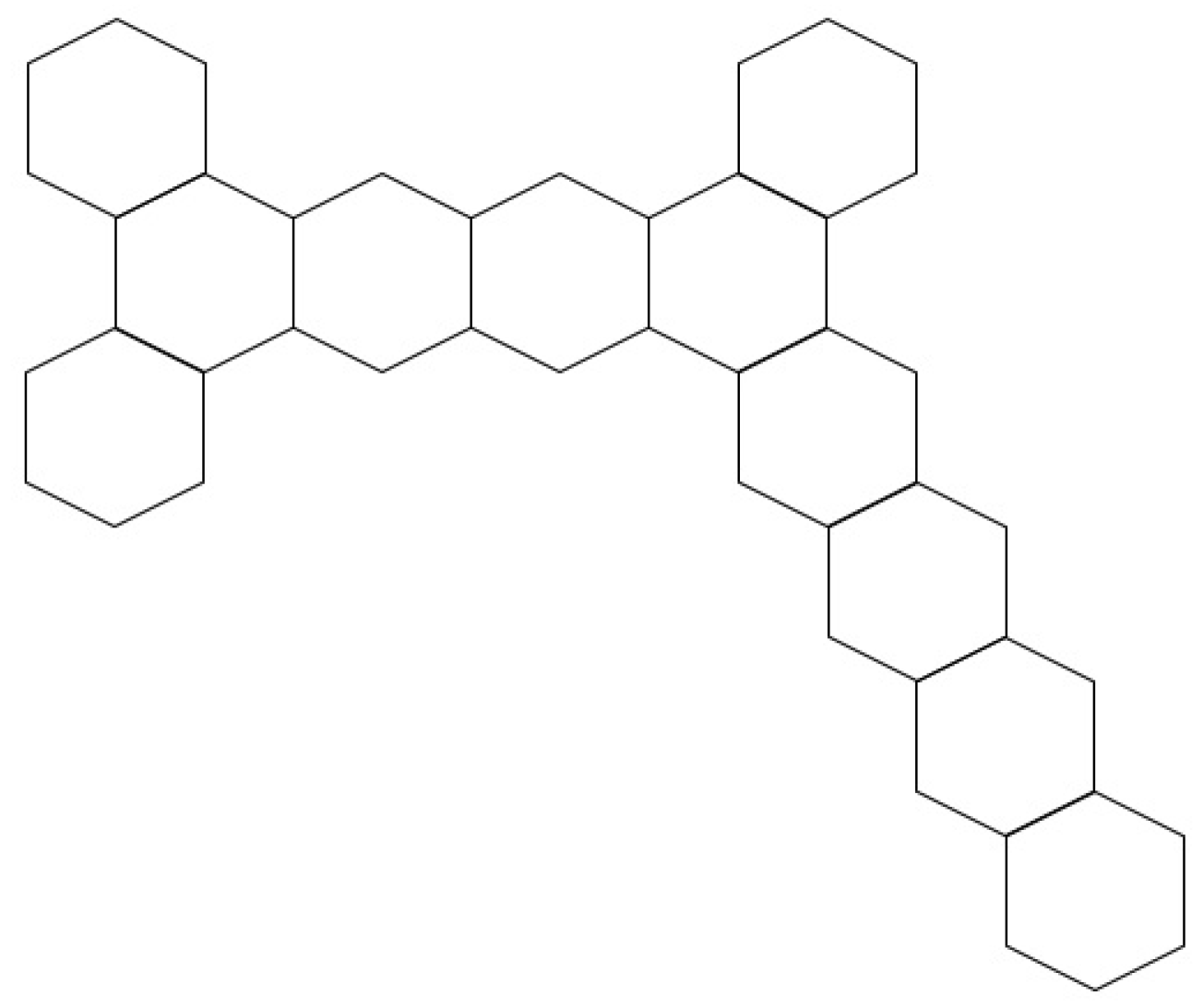
2.2. Pericondensed Hexagonal Systems
3. Main Results
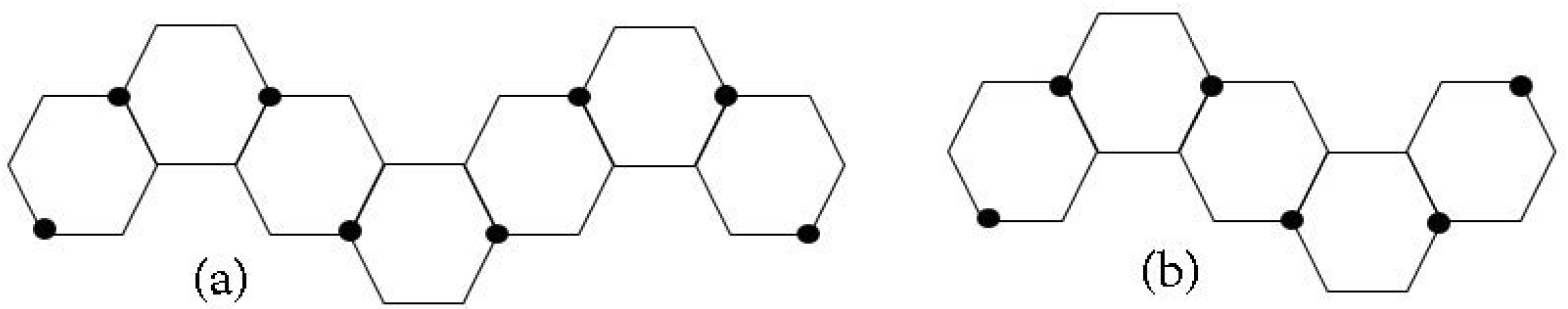
3.1. Pericondensed Hexagonal Systems
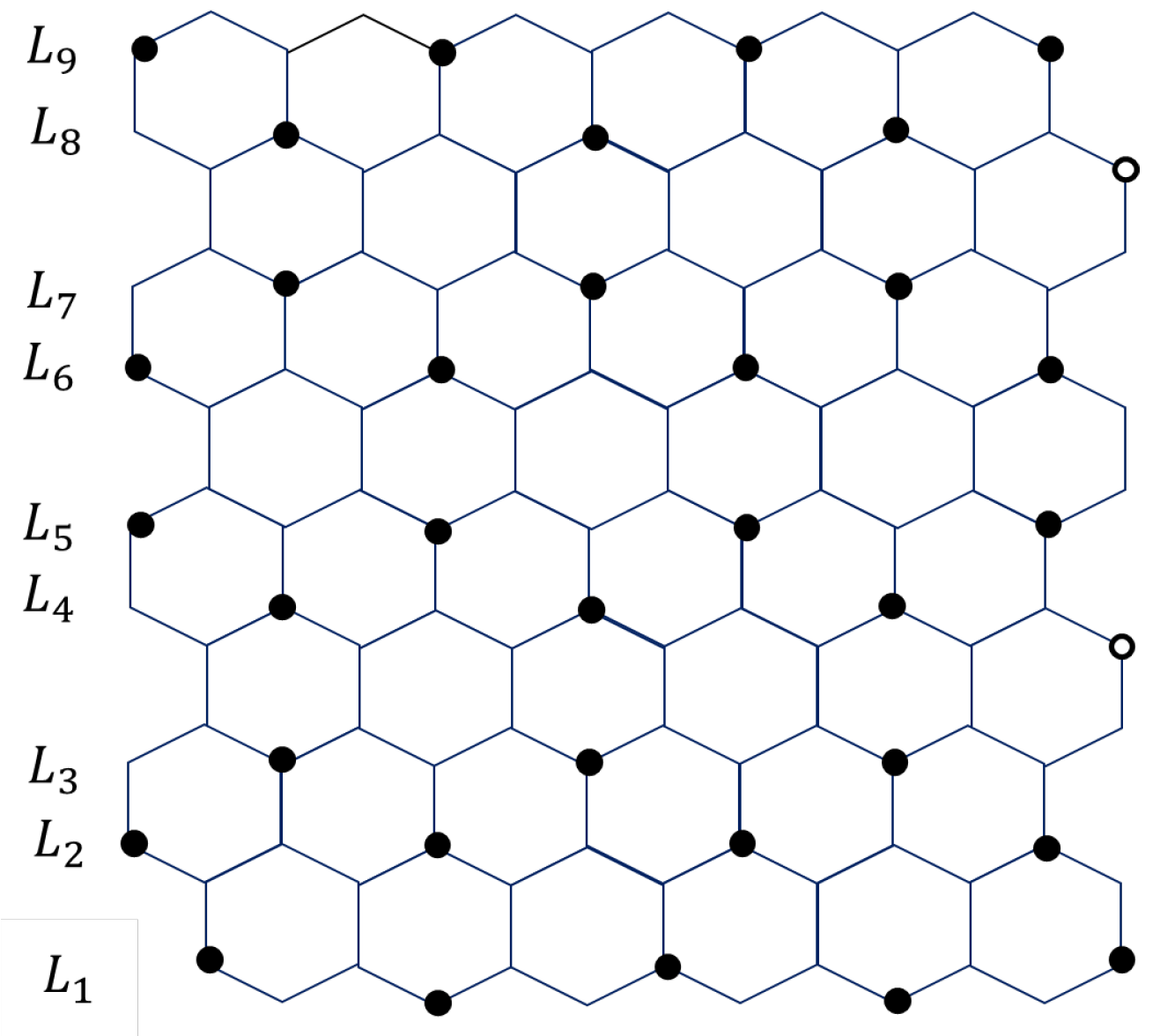
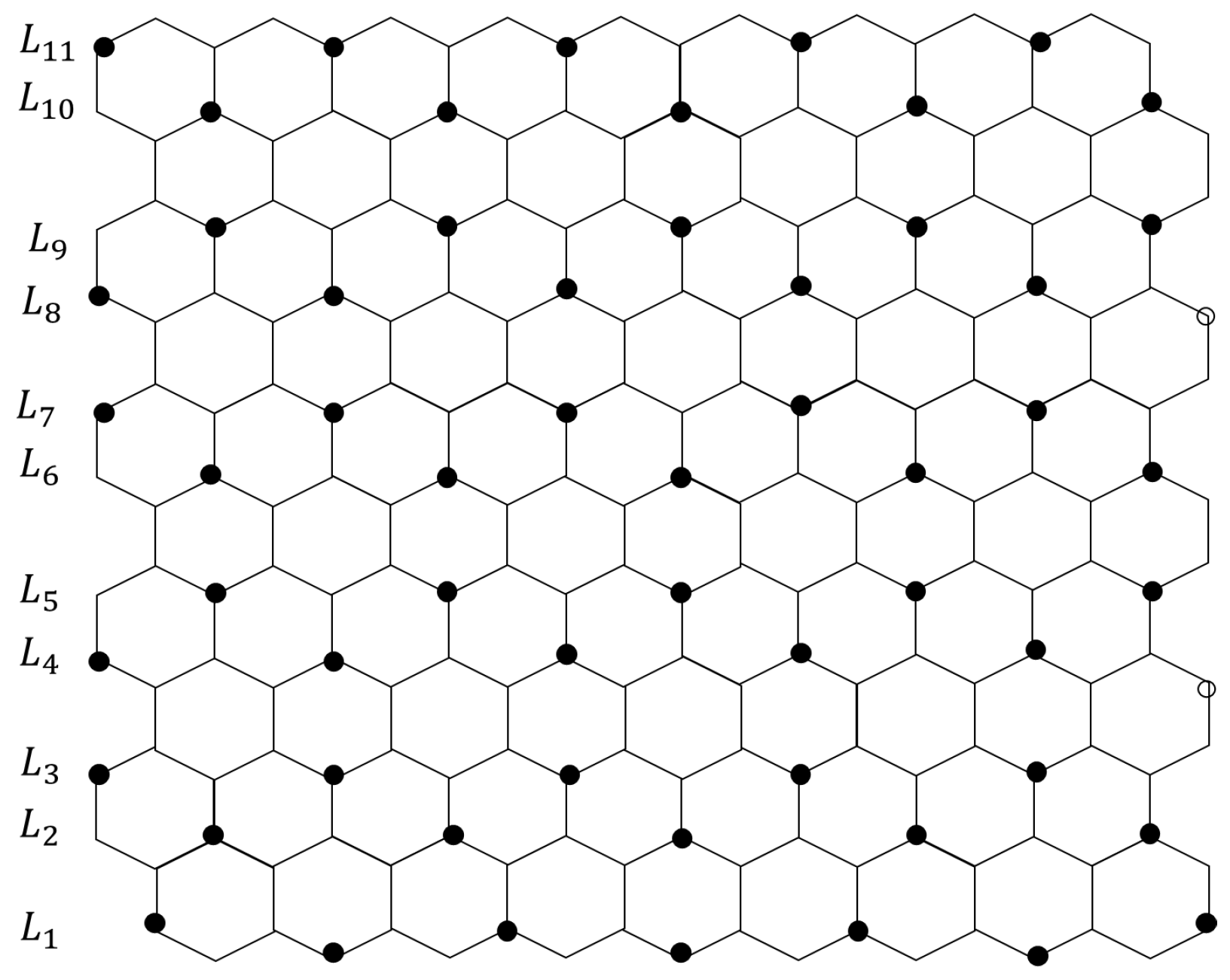
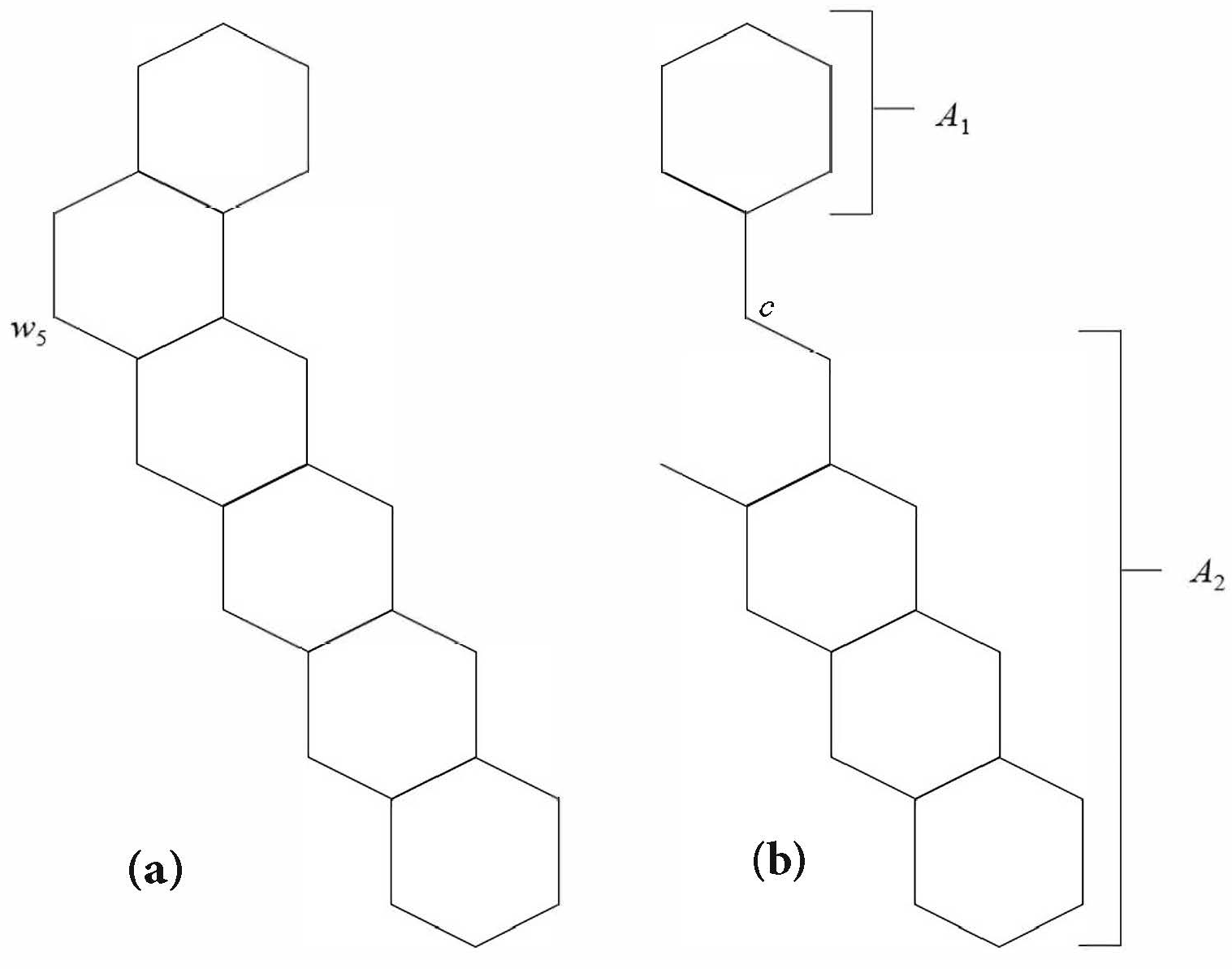
3.2. Catacondensed Hexagonal Systems
- (a)
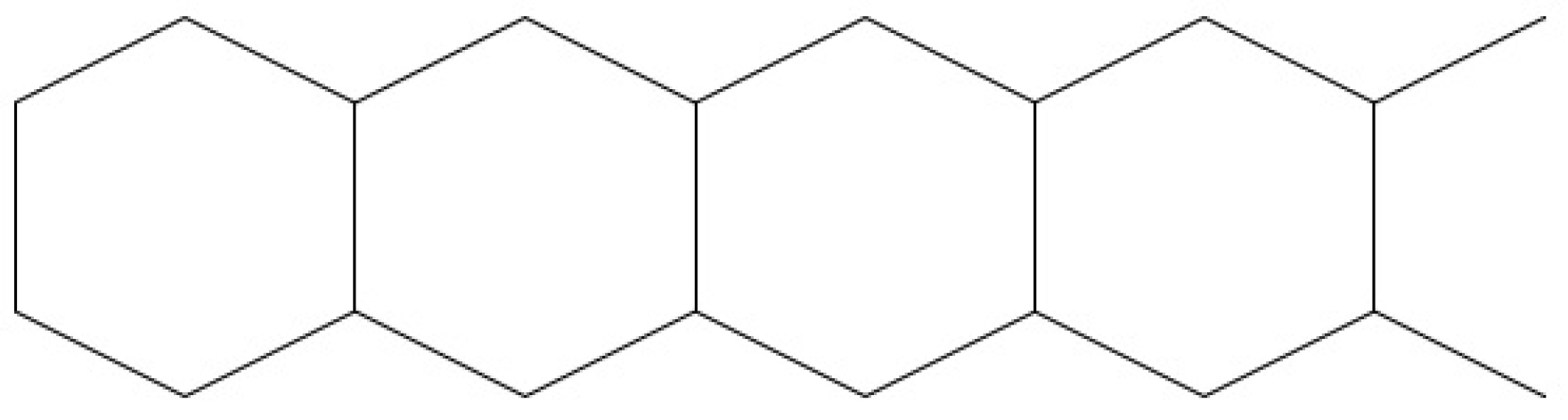
- If H is a linear hexagonal chain, then .
- (b)
- If H is a zigzag, then .
- (c)
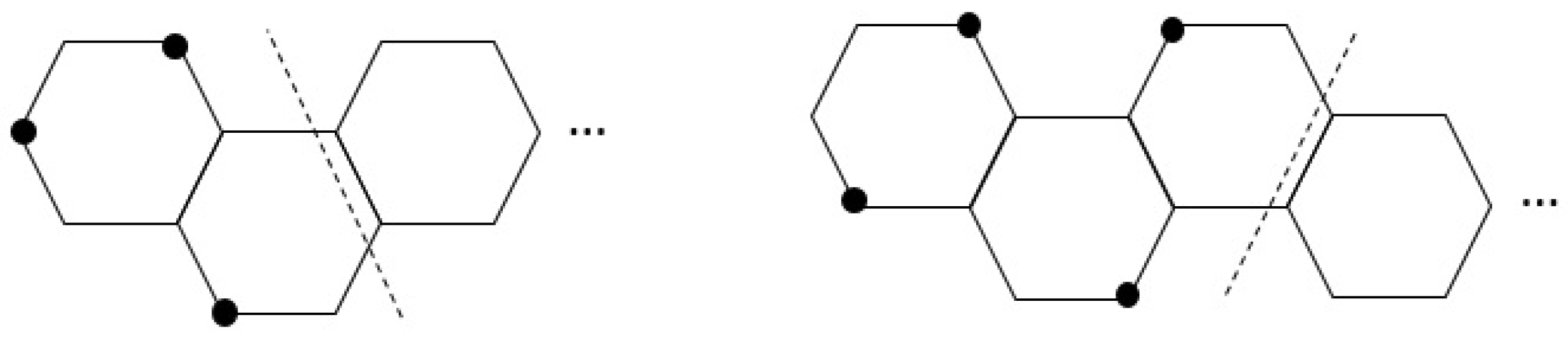
- If H is a relaxed zigzag, then .
4. Proofs
4.1. Proof of Theorem 3
4.2. Proof of Theorem 4
4.3. Proof of Theorem 5
4.4. Proof of Theorem 6
4.5. Proof of Theorem 7
5. Discussion and Conjectures
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hutchinson, L.; Kamat, V.; Larson, C.E.; Mehta, S.; Muncy, D.; Cleemput, N.V. Automated Conjecturing VI: Domination number of benzenoids. MATCH Commun. Math. Comput. Chem. 2018, 80, 821–834. [Google Scholar]
- Bermudo, S.; Higuita, R.A.; Rada, J. Domination number of catacondensed hexagonal systems. J. Math. Chem. 2021, 59, 1348–1367. [Google Scholar] [CrossRef]
- Bermudo, S.; Higuita, R.A.; Rada, J. Domination in hexagonal chains. Appl. Math. Comput. 2000, 369, 124817. [Google Scholar] [CrossRef]
- Gao, Y.; Zhu, E.; Shao, Z.; Gutman, I.; Klobucar, A. Total domination and open packing in some chemical graphs. J. Math. Chem. 2018, 56, 1481–1492. [Google Scholar] [CrossRef]
- Majstorovič, S.; Klobuxcxar, A. Upper bound for total domination number on linear and double hexagonal chains. Int. J. Chem. Model. 2009, 3, 139–145. [Google Scholar]
- Haynes, T.W.; Knisley, D.; Seier, E.; Zou, Y. A quantitive analysis of secondary RNA structure using domination based parameters on trees. BMC Bioinform. 2006, 7, 108. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Allan, R.B.; Laskar, R. On domination and idependent domination numbers of a graph. Discret. Math. 1978, 23, 73–76. [Google Scholar] [CrossRef] [Green Version]
- Topp, J.; Volkmann, L. On graphs with equal domination and independent domination numbers. Discret. Math. 1991, 96, 75–80. [Google Scholar] [CrossRef] [Green Version]
- Favaron, O.; Kaemawichanurat, P. Inequalities between the Kk-isolation number and the independent Kk-isolation number of a graph. Discret. Appl. Math. 2021, 289, 93–97. [Google Scholar] [CrossRef]
- Furuya, M.; Ozeki, K.; Sasaki, A. On the ratio of the domination number and the independent domination number in graphs. Discret. Appl. Math. 2014, 178, 157–159. [Google Scholar] [CrossRef]
- Southey, J.; Henning, M.A. Domination versus independent domination in cubic graphs. Discret. Math. 2013, 313, 1212–1220. [Google Scholar] [CrossRef]
- Klobučar, A.; Klobuxcxar, A. Total and double total domination number on hexagonal grid. Mathematics 2019, 7, 1110. [Google Scholar] [CrossRef] [Green Version]
- Gutman, I.; Cyvin, S. Introduction to the Theory of Benzenoid Hydrocarbons; Springer: New York, NY, USA, 1989. [Google Scholar]
- Quadras, J.; Mahizl, A.S.M.; Rajasingh, I.; Rajan, R.S. Domination in certain chemical graphs. J. Math. Chem. 2015, 53, 207–219. [Google Scholar] [CrossRef]






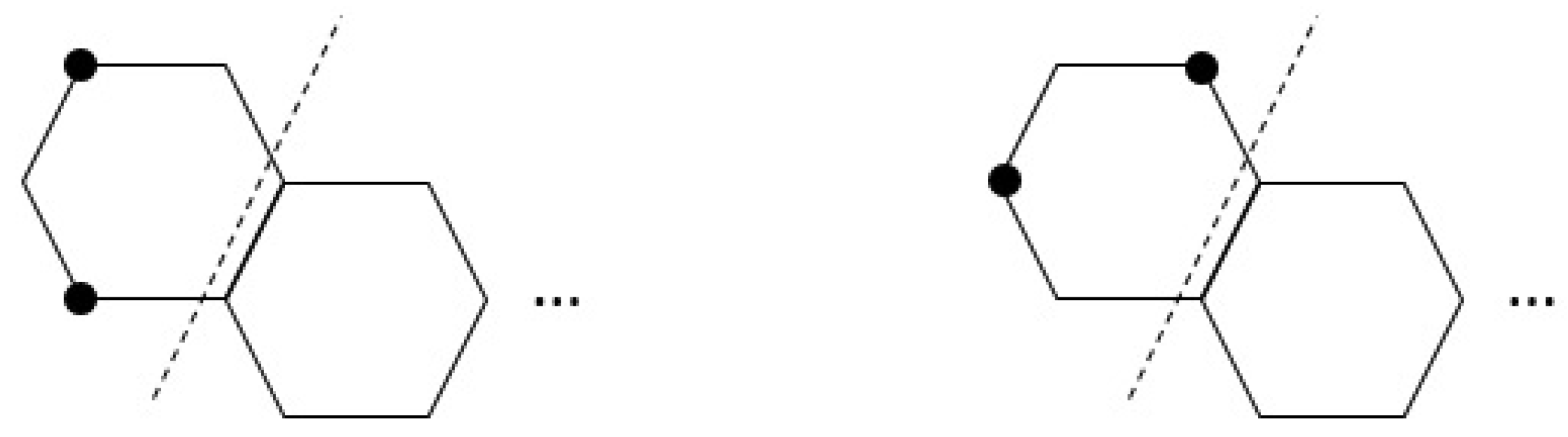






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almalki, N.; Kaemawichanurat, P. Domination and Independent Domination in Hexagonal Systems. Mathematics 2022, 10, 67. https://doi.org/10.3390/math10010067
Almalki N, Kaemawichanurat P. Domination and Independent Domination in Hexagonal Systems. Mathematics. 2022; 10(1):67. https://doi.org/10.3390/math10010067
Chicago/Turabian StyleAlmalki, Norah, and Pawaton Kaemawichanurat. 2022. "Domination and Independent Domination in Hexagonal Systems" Mathematics 10, no. 1: 67. https://doi.org/10.3390/math10010067
APA StyleAlmalki, N., & Kaemawichanurat, P. (2022). Domination and Independent Domination in Hexagonal Systems. Mathematics, 10(1), 67. https://doi.org/10.3390/math10010067





