Rejecting Platonism: Recovering Humanity in Mathematics Education

Abstract

In this paper, I consider a pervasive myth in mathematics education, that of Plato-formalism. I show that this myth is ahistorical, acultural, and harmful, both for mathematics and for society. I argue that, as teachers, we should reject the myth of Plato-formalism and instead understand mathematics as a human activity. This philosophy humanizes mathematics and implies that math education should be active, cultural, historical, social, and critical—helping students learn formal mathematics, while also learning that mathematics shapes their lives, that this shaping is a result of human work and choices, and that students are empowered to shape those choices.

1. Introduction

As a high-school math teacher, my first activity for every class was a discussion exploring the classic question: “was mathematics invented or discovered?” Although I professed to be agnostic in this discussion, I was not. I believed that mathematics existed “out there”—independent of time, space, and culture—and hence, that mathematics was discovered. Such a position is neatly summarized by Martin Gardner [1]:

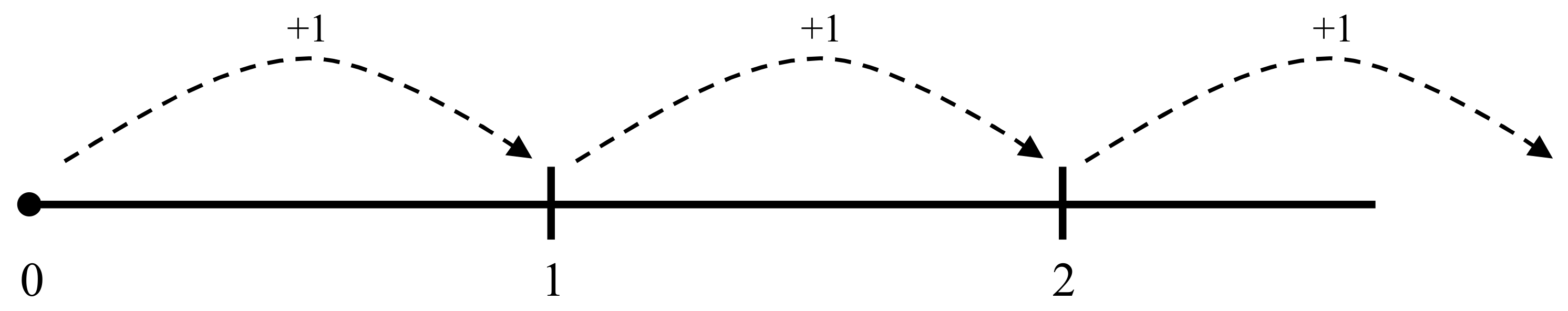
[W]hen two dinosaurs met two dinosaurs there were four dinosaurs. In this prehistoric tableau “2 + 2 = 4” was accurately modeled by the beasts, even though they were too stupid to know it and even though no humans were there to observe it.

This position feels safe to me. Comforting. Yet, I am about to argue that this position is based on a myth, one which is ahistorical, acultural, and ultimately, harmful. In taking this stance, I take up a minority position within Western culture, where the Platonic ideals expressed by Gardner reign supreme [2,3]. In this paper, I will review the literature that supports this conclusion, and I will present an alternative vision for mathematics and mathematics education.

First, however, I would like to frame this paper a bit. This is a paper about a mathematical myth, written by a math teacher, for math teachers. It is also a paper about mathematical philosophy. Why should teachers care about philosophy? Because it turns out that what teachers believe about mathematics is more consequential for their teaching than what they believe about learning [4] (but see [5] for a counter-argument). However, while literature about mathematical philosophy abounds, very little of it is written for teachers and teacher educators [6,7] (at least within the last 30 years, but see [8]). In this paper, I aim to change that by arguing for a philosophy that is more powerful and more faithful to the historical record—and ultimately more human—than the dominant mathematical philosophy in the west.

To begin, I review this dominant mathematical philosophy, which I call the Plato-formalist philosophy. I argue that this philosophy is a myth by showing it is both ahistorical and acultural. I then describe a different approach: mathematics as a human—and therefore a social, cultural, and historical—activity, and I explain how this approach overcomes the shortcomings of Plato-formalism. Finally, I discuss the significance for math education of adopting this approach.

2. The Plato-Formalist Philosophy

Mathematical Platonism is the belief that mathematics exists as a complete structure, somewhere “out there”, just waiting to be discovered. More formally, this belief holds the following three theses: (1) mathematical objects exist; (2) they are neither physical nor mental, and they exist outside of space and time; and (3) they exist independent of any sentient being and the culture thereof [3,9,10]. Such a view is pervasive, both in popular culture and among mathematicians [2,3].

Alongside this philosophy is another belief about mathematics, called “formalism”. Formalism was a movement in the late 19th and early 20th century to show that mathematics was a self-contained system without any relation to the physical world [11]. The key consideration is that the system is consistent (no statement is simultaneously true and false) and complete (every possible statement can be proven to be either true or false). Even though Gödel [12] (translation in [13]) demonstrated that such a program was impossible for any system that is complicated enough to include arithmetic, formalist logic continues to dominate mathematics [14].

Platonism and formalism are not the same, and indeed one could argue that they are ideologically opposed. For our present purposes it suffices to consider them as forming an axis—the Plato-formalist axis—upon which mathematics is often positioned in popular culture, professional work, and mathematics education [15].

3. Plato-Formalism Is a Myth

Plato-formalism is popular, but it is a myth. To understand why, let us first take a short detour to Western science. In the West, we have folk notions of science as a process of pure discovery, a dispassionate study of the objective reality that surrounds us. However, as sociologists and historians of science [16,17,18,19,20] have shown, science is much more than observing and reporting. First, observations are theory-laden [16] and are culturally-conditioned [19]. Additionally, the job of the scientist is to produce facts that fit the world through observation, but also to organize systems of relations in the world such that those facts are valued. As Bruno Latour explains, “Scientific facts are like trains, they do not work off their rails. You can extend the rails and connect them but you cannot drive a locomotive through a field” [17] (p. 155). The work of “extending and connecting the rails” is the human work that is required to produce a world where facts can be accepted as “true”. It is the work of turning an artifact (something produced by humans) into a fact (something that appears to have an objective existence). This is a constructive and social process (documented extensively in [18]), which belies folk notions of science as dispassionate discovery.

Just as the history of science is rife with examples of scientists doing work to create a world in which their facts are accepted as true, so too is the history of mathematics. For example, concepts including zero, negative numbers, complex numbers, infinity, and the calculus (to name but a few) all experienced turbulent introductions into Western mathematics, and their ultimate acceptance was a product of human work to create the conditions under which these ideas could be accepted as true. Today of course, these objects have become so naturalized that the human work required to make them and sustain them as naturalized objects has become largely invisible [21] (for accessible historical treatments that reveal these objects in all of their anthropological strangeness, see: zero: [22]; negative numbers: [23]; complex numbers: [24]; infinity: [25]; the calculus: [26]).

As I write this, I can hear the objections. ‘Okay, maybe infinity required human work to make it true, but 2 + 2 = 4 does not. As the Gardner quote in the beginning of this paper demonstrated, this fact was true for the dinosaurs. It always has been true and always will be true’. But the Gardner quote is deceptive. To understand why, we need to examine the grammatical role of 2.

When Gardner discusses 2 dinosaurs, “2” is an adjective. It is being used to describe dinosaurs. Thus, Gardner’s statement isn not a statement about numbers, it is a statement about dinosaurs. Substitute any object you want for the dinosaurs, and you are left with a statement about those objects: a statement, in other words, about the physical world. In the equation, 2 + 2 = 4, however, things are very different. Here, “2” is a noun. It is not describing an object, it is the object [27,28].

Of course, this is not just an argument about grammar. It’s an argument about the difference between concrete quantity (i.e., 2 dinosaurs) and abstract number (i.e., 2). The difference is profound. Somewhere, “2” became decontextualized and thingified as an object in its own right [29,30]. This is a massive human achievement. Indeed, the eminent Russian psychologist Lev Vygotsky viewed such decontextualization as the principle measure of human sociocultural evolution [31].

Like many human achievements, the thingification of number in the West is an innovation that was driven by material necessity—probably the demands of commerce. In fact, commerce drove many mathematical achievements. For example, surviving evidence suggests that coordinate systems developed from a need to parcel land in ancient Egypt [32]. For communities that have different demands, we would expect to see different innovations in the ways that people interact with quantity and space, and indeed we do [2]. For example, Pinxten, Van Dooren, & Harvey [33] studied a Navajo community, and found that rather than parceling land via boundaries, members of the community “systemically represent the world and every discrete entity as a dynamic and continually changing entity” [33] (p. 36). In this dynamic and relational view of space, “notions of boundary cannot easily be grasped with the Western perspectives; again, the essentially dynamic nature of anything existing has to be taken into account so that boundaries are recognized as extreme variations in process, rather than static positions” [33] (p. 36).

Thus, both the history of Western mathematics as well as contemporary cross-cultural studies belie the folk notions of Plato-formalism. Historically, the introduction of new concepts into mathematics was almost always turbulent and required human work to create the conditions under which they could be accepted. Contemporaneously, when we examine cross-cultural mathematics, we find “various types of mathematics, irreducible to each other” [2] (p. 457). All of this contradicts the Platonic notion that a single mathematics exists in complete form, just waiting to be discovered. Similarly, the tight coupling of mathematics to local practices belies formalist notions that mathematics is a “game of symbols”.

Despite this evidence, widespread belief in the myth of Plato-formalism still underscores many educational practices and this has consequences, both for mathematics education and for society.

4. The Consequences of Plato-Formalism on Mathematics Education and Society

One result of the widespread belief in the myth of Plato-formalism in the West is that mathematics is seen as value-neutral, and it is taught as such. However, mathematics has values. When we teach mathematics as if it were neutral, we are teaching these values [34,35], and this has consequences [36].

One such value is objectism: the systemic decomposition of the environment into discrete objects, to be categorized and abstracted. We can trace the evolution of objectism in mathematics back to Euclid, who built his Elements on three geometric objects (the point, the line, and the plane). But Euclid did not have to follow such a program. During his lifetime, there were two competing philosophies on the nature of the world, embodied by Heraclitus on the one hand, who saw the world in terms of change and flux, and Democritus on the other, who saw the world in terms of ‘atoms’ and objects. In the modern-day West, Heraclitus’s world-view is largely forgotten, and Western mathematics, science, and society have all been structured in the mold of Democritus [34,37].

The consequence of this choice has been the reification of abstraction as the gold standard in mathematics. This has led to staggering progress in developing mathematics as a discipline. However, it has also had the effect of restricting school mathematics for generations of students, who learn that “mathematics” means memorizing formal algorithms and procedures for abstract symbol-manipulation. These procedures are revered for their perceived generality; they can potentially be applied and used in many different situations, often very different from those in which they were learned. However, such transfer is problematic at best [38,39]. Often, people use a variety of situationally-relevant methods for computation—strategies that recruit features of the situation into the computation, rather than strategies that abstract out those features [38,40,41,42,43,44,45]. Mathematics adheres in the relationship between people and setting.

We do not have to look far to find people that see the world in terms of relationships rather than objects. For example, recall how the Navajo community described above represented the world in terms of change and relationships. My point here is not to advocate for such a worldview, but to re-present it so that those of us in the West can see that objectism is a cultural way of perceiving the world [19], rather than “the way the world exists”.

Understanding objectism as a product of human work allows us to explore the consequences for math education and society. For math education, one consequence is the fetishization of abstract procedures, as I discussed above. For Western society, one likely consequence of objectism is our insistence on rigid categorization systems, formalized in the Aristotelian “law of the excluded middle:” the logic proposition that states “that which is not true is false”. It is surely the case that categorization is an inevitable human activity [21]. However, the rigidity with which we categorize in the West is not inevitable; in many cases dialectic notions are more appropriate than Aristotelian rigidity. However, rigid categorization persists, and is highly consequential:

Each standard and each category valorizes some point of view and silences another. This is not inherently a bad thing—indeed it is inescapable. But it is an ethical choice, and as such it is dangerous—not bad, but dangerous.

[21] (pp. 5–6)

The above discussion stands in sharp contrast to the neutrality and objectivity that is often associated with mathematics. But mathematics is neither objective nor neutral: it is imbued with values including objectism. If we teach mathematics as if it were neutral we reify these values and make them invisible, beyond the gaze of critical study [34,35,36]. In the remainder of this paper, I will sketch an alternate vision that humanizes mathematics and math education, a vision that accepts the notion that mathematics is not neutral, and that makes visible the values that have thus far been kept hidden.

5. Mathematics Is a Human Activity

By now, my general claim should be clear: mathematics is a human activity [28,46,47]. It happens as humans mathematize the word, and it leads to the creation of mathematical objects, including concepts, models, tools, strategies, symbols, and algorithms. These objects become reified—thingified [30]—in culture, and thus can become tools that enable new forms of mathematical activity. This set of objects, which is created by humans as they engage in joint activity and reified in culture, is what we in the West now call mathematics.

Let us consider the nature of these mathematical objects. When I say that mathematical objects are cultural, I mean that they are the collected product of human mathematical activity [48]. In this way, mathematical objects exist in the same way that languages exist, or symphonies, or literature. They are neither mental nor physical, but neither do they exist Platonically, independent of humans.

As cultural objects, mathematical objects can be manipulated and extended by humans to create new culture. Just as human authors manipulate existing language to create new works (consider a Shakesperian play), so too do humans manipulate existing mathematics to produce new mathematics. This new mathematics is then subjected to social review, judged by how well it fits with the existing physical and cultural worlds. This review is a social process and the standards for review are simply social agreements [11,49]. For example, the notion of what constitutes a “proof” in mathematics is not universal, neither across social groups [50], nor across time [51].

It has been useful up to this point to draw a parallel between mathematical objects and other cultural objects such as language, music, and literature. However, there is something that separates mathematical objects from these other cultural forms, namely, the stunning regularity that mathematical objects exhibit. To explore this further, let us return to the trope of 2 + 2 = 4.

As I summarized earlier, “2” as a mathematical object is very different from the physical notion of “2 dinosaurs”. Mathematically, 2 is a cultural object, one that exists in Western culture by shared agreement. For example, in certain versions of set theory, 2 is understood as a very particular set that is built recursively from the null set [52]. “2” also exists in the counting sequence, by agreement, between 1 and 3. From this perspective, we might say that 2 is “counted into existence” by humans following an agreed-upon convention [53].

However, 2 exists as more than simply an abstract set, or a symbol in an abstract sequence, it is intertwined with the physical world. Humans most likely invented 2 as an abstraction of the physical world [32], many of us understand 2 in terms of the physical world such as a collection of objects or a distance [54], and we impose 2 on the physical world via counting, measurement, and coordinate systems [55]. Thus, although 2 is a human creation, we are not free to make our own decisions about what 2 is. It is constrained by social agreement to conform to certain aspects of the physical and mathematical world. So invented and constrained, 2 is now very different than other cultural objects: it has a life of its own, with consequences that became inevitable immediately upon its invention and constraint [27,28,56,57]. One of those consequences is that 2 + 2 = 4.

Much mathematical work involves the “discovery” of these consequences. Even here, however, mathematics is more than deductive discovery, it still retains constructive and social elements [58]. For example, as described by Bloor [59], we might explore the consequences of 2 and + in two contexts in which we have agreed upon rules for their application: physical measurement and counting. A common way to conceptualize the coupling of these applications is a number line, where “0” represents the starting point in our measurement and our counting sequence, and increases in length on the number line correspond to successions in the counting sequence (Figure 1). On the number line, 2 + 1 = 3.

However, what if I told you that we were doing this on a wheel, as in Figure 2? Notice that on this wheel, when we move forward one unit from 2, we arrive back at our starting point, which we have agreed is “0”. Thus, following our agreed convention on the wheel, we find that 2 + 1 = 0, and not 3 as shown on the number line. We have a contradiction! Which is it?

First of all, we should ask, why does it have to be one or the other? Why cannot 2 + 1 equal 0 and 3? I admit that this makes me uncomfortable, but I recognize that this is because I am wedded to a mathematics that is built on the Aristotelian law of the excluded middle—that is, the notion that “that which is not true is false.” My purpose is not to argue against the desirability of keeping this notion in Western mathematics (although some have explored this, see [37] for a brief summary), I just want to point out that it is a choice. My discomfort with the notion that 2 + 1 might have two different answers has nothing to do with the “true” existence of 2 + 1, and is instead a historically-contingent consequence of prior human work.

Okay, but let us say that we agree to keep the law of the excluded middle. We are then left with a contradiction. How shall we resolve it? The answer is that we must come to a social agreement about which one we want to keep. There is no “true” value of 2 + 1, just agreed upon meanings and behaviors. If those behaviors come into conflict we must make decisions, and decision-making is a social process. In the West we have decided that “everyday” arithmetic should remain consistent with the number line, and therefore it is excluded from the wheel. However, in doing so-called “wheel” arithmetic, we have invented a whole new arithmetic. Western mathematicians call this “modular” arithmetic, and it exists right alongside everyday arithmetic both in mathematics and in our everyday lives (for example, in the U.S. we use wheel arithmetic—that is, arithmetic modulo 12—to keep track of time during the day, where adding an hour follows the counting sequence 8:00, 9:00, 10:00 … up until 12:00, at which point adding another hour takes us to 1:00, not 13:00; in many other societies, the same principle is used but with 24 as the modulus). Examples of such inventions abound in mathematics, from non-Euclidean geometries that spring from a rethinking of parallel lines, to p-adic number systems that can be understood by rethinking the notion of distance.

When we understand that mathematics is a human activity, the historical controversies that have surrounded the introduction of new mathematics are not difficult to explain. They are the result of situations like wheel arithmetic, where established principles come into conflict. Similarly, the wheel arithmetic example shows us how it is perfectly possible that multiple mathematics can exist across cultures and time: mathematics happens as humans solve problems in their environment, and different problems lead to different mathematics. Hence, mathematics is not a Platonic structure that exists “out there”, waiting to be discovered. Instead, mathematics is a human activity, and what we in the West know today as mathematics is a human—and therefore a social, cultural, and historically-contingent—achievement.

6. Significance for Math Educators

Rejecting the myth of Plato-formalism and adopting the perspective that mathematics is a human activity implies that math education should be: active, cultural, historical, critical, and social.

6.1. Active

The Plato-formalist view of mathematics has led to a view of math education as a two-step process. First, students learn formal skills and algorithms, and then they apply those skills in exercises. When we understand mathematics as a human activity, we see that this is an “anti-didactic inversion,” [46] of teaching the results of an activity rather than the activity itself. Instead, students should engage in mathematical activity first, and through this activity they should invent mathematical objects. In other words, rather than starting with the structure of mathematics, math education should engage students in structuring activities [60,61]. These structuring activities should be “whole activities” [62], which intertwine multiple strands of mathematics [61]. In this way, skills are not separated from the practices that give them meaning [45,63].

None of this is to suggest that students should not learn formal mathematics. Rather, it is a suggestion for how students should learn formal mathematics. The job of the teacher is to engage students in guided reinvention [64], such that students invent mathematical objects by mathematizing their world—including the mathematical world.

Over the past three decades, hundreds of studies have been conducted on active learning. Overwhelmingly, the evidence suggests that students who experience active learning learn more mathematics and develop more positive mathematical identities, as compared to students who experience more passive forms of instruction such as lectures (for reviews and meta-analyses, see [65,66,67,68,69,70,71,72,73]). The evidence is so overwhelming that, after reviewing 225 studies, Freeman and colleagues explained, “If the experiments analyzed here had been conducted as randomized controlled trials of medical interventions, they may have been stopped for benefit—meaning that enrolling patients in the control condition might be discontinued because the treatment being tested was clearly more beneficial” [65] (p. 8413).

6.2. Cultural

An amazing thing happens when mathematical objects are incorporated into activity: they enable new forms of activity and transform mental functioning in the process [74]. An example will help to make this point. Figure 3 shows two representations of the height of an individual on a Ferris Wheel as a function of time. Each representation is a mathematical object. Now, imagine that we want to predict the height of the individual after riding the wheel for 30 s. The task is different depending on the object used.

Analytically, we have to perform algebraic, arithmetic, and trigonometric operations. Graphically, we have to coordinate horizontal and vertical distances. Thus we might ask, where did the algebra go? The arithmetic? The trigonometry? The answer is that these operations were absorbed into the graph. The graphical representation thus transforms a computation task into a spatial coordination task (cf., [75]).

More generally, different mathematical objects transform the mental functioning necessary to solve a problem. Consequently, mental operations cannot exist separately from these objects. The implication is profound: mathematical cognition does not happen solely “between the ears” [76], but rather is distributed across systems of persons and objects [77,78,79]:

If we ascribe to individual minds in isolation the properties of systems that are actually composed of individuals manipulating systems of cultural artifacts, then we have attributed to individual minds a process that they do not necessarily have.

[78]

The key consideration for teachers is the classroom environment. Often, classrooms are organized such that they restrict access to the resources that create cognition (consider, for example, the barren conditions under which students take tests). This might make sense from a perspective in which learning is seen as acquisition of knowledge (e.g., [80]), but it is counter-productive from the cultural perspective that I have outlined here. From a cultural perspective, “humans create their cognitive powers by creating the environments in which they exercise those powers” [78]. Thus, the way to make classrooms powerful places for cognition is to saturate them with cognitive resources and give students the power to manipulate that environment [63,81,82].

6.3. Historical

Engaging students in the history of mathematics helps to make mathematics less mystical by making visible the role of humans, showing students that “mathematics exists and evolves in time and space, [and] human beings have taken part in the evolution” [83]. The goal is to help students see mathematics as historically contingent [6], and to see themselves and their ancestors as historical actors, actively playing a role in the colossal human achievement that is Western mathematics.

6.4. Critical

Western mathematics and Western society have grown together and influenced each other through human decisions. The Plato-formalist view reifies the resulting mathematical and societal structures as natural. However, when we understand mathematics as a historically-contingent result of human work, we can critically examine its consequences.

For instance, imagine a math class that engages in the wheel arithmetic example that I described earlier. As the class struggles with the idea that 2 + 1 might equal both 0 and 3, it would be a great time for the teacher to question why it is such a struggle to accept both. This might lead to a discussion of rigid categorizations, and the real consequences of these categorizations. For example, a class might discuss the consequences of rigid gender categorization in a society that insists on categorizing people before they can use the restroom.

Furthermore, when we see how mathematics is intertwined with society, we can see how Western mathematics can be used as a hegemonic tool. Such has been the case throughout colonial history, as the supposedly “neutral” mathematical practices of the West have been used as a tool of domination and cultural genocide [84]. Even in modern United States (U.S.) schools, mathematics continues to operate as an instrument of colonization [85] that reproduces historical systems of privilege and inequality [86], all while normalizing and obscuring these operations [2]. Students deserve to engage with these ideas [36], and understanding mathematics as a human activity can help to expose mathematics to critical review within the math classroom.

It is not all bad of course. Mathematics is one of humanity’s greatest achievements, and it has been used to shape society in an uncountable number of positive ways. Even if mathematics has had and continues to have pernicious effects, so too can it be used as a tool for social justice [87,88,89]. As students engage in critical study of mathematics and its effect on society, they should do so with an understanding that the effects of mathematics are human effects, and that they, as historical actors, have the power to shape those effects (Gutstein [87] calls this critical agency).

6.5. Social

Mathematics requires social interaction. Social interaction facilitates problem-solving [90,91], and it is through social interaction that mathematical objects are invented and thingified—both historically [58] and in classrooms [92,93].

But social interaction is more than a means to help students do mathematics. It is the requisite background against which the previous four features (active, cultural, historical, and critical) are enacted, and it is the mechanism by which students are produced as cultural people. For Radford [94], this sort of production is more important than technical competencies:

What is important in teaching–learning mathematics is not really to become a good problem solver. Although knowing how to solve problems in a technical sense may be an important goal, more important, I think, is the range of possibilities that mathematics offers to our students to live it as a social, historical, cultural, and esthetic experience. But to be truly meaningful, this experience has to occur in the public space of words, deeds and actions—in the polis, that is to say, the organized space of the people “as it arises out of acting and speaking together”.

(Arendt, 1958b, p. 198) [79] (p. 111)

7. Conclusions

Twenty five years ago, Barbeau [95] summarized the popular perception of mathematics:

Most of the population perceive mathematics as a fixed body of knowledge long set into final form. Its subject matter is the manipulation of numbers and the proving of geometrical deductions. It is a cold and austere discipline which provides no scope for judgment or creativity.

(quoted in [96], p. 432)

Barbeau describes the popular perception of mathematics as a Plato-formalist structure. But Plato-formalism is a myth. That this myth continues to exact such a strong hold on popular perceptions is a tragedy, for our students, for our society, and for our mathematics. As teachers, we can make things right for our students by rejecting Plato-formalism and embracing mathematics as a human activity, engaging students in mathematical experiences that are active, cultural, historical, critical, and social. This will help students to learn formal Western mathematics, as well as empower students to shape and use mathematics in their daily lives, and to see, challenge, and influence the effects that mathematics has on society.

In the past 25 years, mathematics education has made astonishing progress as a field, and this has had material effects for countless students (see e.g., the studies on active learning referenced in Section 6 of this paper). Yet, the situation that Barbeau described is still far too pervasive. We can change that. Rejecting the myth of Plato-formalism is the first step.