Influence of Professional Materials on the Decision-Making of Preservice Secondary Teachers When Noticing Students’ Mathematical Thinking

Abstract

This research aims to investigate the impact of professional materials’ interpretation on the decision-making of preservice secondary teachers when analyzing the mathematical thinking of a 15–16-year-old high school student engaged in arithmetic problem-solving. Our conceptual framework considers the model of curricular noticing and the specific model of noticing students’ mathematical thinking, establishing connections between both. The participants were 20 preservice teachers taking part in a training program in two Spanish universities. They were grouped randomly into five groups. The data were preservice secondary teachers’ written responses to a professional task of noticing students’ mathematical thinking by solving two arithmetic problems. A qualitative analysis was carried out considering the skills that make up the theoretical models considered. Findings show that the various interpretations made by the preservice secondary teachers of the professional materials gave rise to different interpretations of the secondary school student’s error and this led them to propose different instructional responses. There were two groups focused on a relational interpretation and three focused on a procedural interpretation (and response). We conclude that the way in which secondary school student teachers begin to make sense of professional materials is influenced by their experiences, background, and beliefs about mathematics teaching.

1. Introduction

One of the objectives of the training programs for preservice mathematics teachers is to facilitate the acquisition of the teaching skills necessary for their future profession, among which is learning to notice classroom situations, so teacher educators improve the training by incorporating research results and redesigning their programs for that purpose (Jacobs et al., 2010; van Es, 2011). According to Fernández and Choy (2020), “Mathematics teacher noticing has been conceptualized as a skill that allows teachers to recognize important events in a classroom and decide on effective instructional responses” (p. 337). Like this, research focused on moments when (preservice) teachers interacted with students (van Es & Sherin, 2002; Sherin, 2007; Star & Strickland, 2008). For example, van Es and Sherin (2002) studied teachers’ interpretations of classroom interactions, conceptualizing noticing in three skills: (a) identifying what is important in a classroom situation; (b) making connections between specific features of classroom interactions and the teaching–learning principles they represent; and (c) using what is known about the context to reason about these classroom interactions. Later, Sherin (2007) introduced the concept of teachers’ professional vision, partially derived from Goodwin’s (1994) conceptualization (sociocultural perspective), which refers to the ability to notice and interpret significant features of classroom interactions. This concept encompasses two main elements: the teacher’s selective attention, which involves determining what to focus on at any particular moment, and knowledge-based reasoning, which is how the teacher analyses and understands what has been observed based on their own knowledge and comprehension.

Understanding students’ thinking is crucial for (preservice) teachers, as instruction must connect with what students currently understand in order to facilitate their further learning (Carpenter et al., 1988; Philipp et al., 2007). In this context, different conceptualizations have emerged, such as noticing students’ mathematical thinking (Jacobs et al., 2010; Sherin et al., 2011). This has been characterized as a set of three interrelated skills: (a) attending to students’ strategies, (b) interpreting students’ understanding, and (c) deciding how to respond based on inferred understanding. The research results on noticing students’ mathematical thinking have allowed progress in development opportunities for the professional development of (preservice) teachers and increased the quality of their instruction (Borko et al., 2008).

Further, noticing students’ mathematical thinking necessitates that the preservice teachers direct their attention to the nature of student cognition. Specifically, they must discern whether students exhibit procedural thinking, characterized by a focus on algorithmic application—the “how to”—or, conversely, relational thinking. This latter mode of thinking involves an understanding of the salient mathematical properties and relationships (“which”), an articulation of the rationale underpinning their relevance to the problem (“why”), and an awareness of the contexts in which these properties and relationships are applicable (“when”).

Extant research has explored the development of relational thinking in primary and secondary school students. For instance, studies have investigated the integration of arithmetic and algebra in elementary education (Carpenter et al., 2003), the comprehension of numerical equalities within foundational arithmetic (Castro & Molina, 2007), the development of fractional understanding (Empson et al., 2011), and the simplification of algebraic fractions (Vega-Castro et al., 2011, 2012). However, a notable gap persists in the literature regarding how teachers discern the specific type of mathematical thinking—whether relational or procedural—that students employ when engaging with problem-solving tasks (Jacobs et al., 2010; Walkoe, 2015).

Noticing students’ mathematical thinking can occur in various contexts, including the analysis of video recordings of classroom interactions (Roller, 2016; Sherin et al., 2011; van Es, 2011; Star & Strickland, 2008; Schack et al., 2013), the use of online discussions (Fernández et al., 2012), and the examination of student responses to tasks related to different areas of mathematics. These areas include algebraic thinking (Magiera et al., 2013), proportional reasoning (Buforn et al., 2022; Son, 2013), derivatives (Sánchez-Matamoros et al., 2019), limits (Fernández et al., 2024), and numeration and algorithms (Dick, 2017; Schack et al., 2013). Additionally, other methods such as using learning trajectories (Callejo et al., 2022) and conducting lesson studies (Amador & Carter, 2018; Guner & Akyuz, 2020; Murata, 2011) can also facilitate the noticing of students’ mathematical thinking. All of these pieces of research have provided evidence that preservice teachers can improve their noticing practices with support. While some researchers have observed a link between effectively noticing students’ mathematical thinking and teaching experience (Dreher & Kuntze, 2015; Jacobs et al., 2010), this relationship appears to be complex.

It appears that expertise in noticing is more closely associated with specific facets of professional knowledge than with mere teaching experience. This observation has led researchers to investigate how preservice teachers perceive and interpret curricular materials during instructional decision-making and lesson planning. More recently, this research has expanded to examine the dynamic interactions between (preservice) teachers and curricular materials in the context of lesson planning (Amador et al., 2017; Barno & Dietiker, 2022; Choy et al., 2017). Additionally, studies have focused on the interplay of knowledge, perception, and the pedagogical environment (e.g., Dominguez, 2021; Mason, 2021; Scheiner, 2021; van Es & Sherin, 2021).

The Curricular Noticing Framework has provided opportunities to understand how preservice teachers conceptualized mathematical concepts, such as the division of fractions (Males et al., 2015), after engaging with multiple curricular resources. The findings of this research also highlight that preservice teachers developed greater critical capacity and paid closer attention to specific aspects of tasks in order to evaluate the extent to which they enhanced or hindered students’ mathematical reasoning. They subsequently adapted these tasks to improve the quality of instruction. Ultimately, engaging in professional noticing of curricular materials can facilitate instructional decision-making by taking into account students’ mathematical thinking, thereby enhancing teachers’ practices. Results also indicated that preservice teachers faced difficulties in proposing activities to complete a sequence of instruction that were consistent with the provided interpretation, demonstrating a disconnect between what was identified in the curricular material and the design (Fernández et al., 2020; Rotem & Ayalon, 2023; Zorrilla et al., 2024).

This suggests that teacher noticing encompasses dimensions beyond teacher–student and teacher–curriculum interactions, warranting further investigation. This study seeks to contribute empirical evidence that illuminates additional factors influencing teachers’ decision-making. Specifically, it examines how preservice secondary teachers, utilizing materials provided within their training program, attend to and interpret student mathematical thinking as evidenced by student responses to a mathematical task, and subsequently determine appropriate support strategies.

Teacher educators offer preservice teachers learning opportunities during their training programs that enable them to develop their noticing skills for teaching. To accomplish this, they provide various types of resources that help preservice teachers address professional tasks (such as planning a lesson or interpreting student mathematical thinking), which must be used to justify their responses. These resources (henceforth, professional materials) include curricular materials (such as curricula and textbooks, among others) as well as theoretical documents that provide summarized information from research findings (for example, learning trajectories for different concepts, characteristics of inquiry-based teaching models, and relational thinking in mathematics education).

We hypothesize that preservice teachers may interact differently with the professional materials provided to them for noticing students’ mathematical thinking or planning lessons. Therefore, it is essential to investigate the relationship between how preservice teachers use these professional materials for solving professional tasks in their training program and their reflections on their interactions with students. This raises the question of what role these professional materials play in the noticing of preservice teachers: specifically, how they view and interpret these materials and whether such interpretations may influence their teaching decisions. Therefore, the aim of the research is to investigate the impact of professional materials’ interpretation on the decision-making of preservice secondary teachers when analyzing the mathematical thinking of a 15–16-year-old high school student engaged in arithmetic problem-solving.

To respond to the objective set forth in this research, we rely on two conceptual frameworks: noticing students’ mathematical thinking (Jacobs et al., 2010) and curricular noticing (Dietiker et al., 2018; Males et al., 2015).

1.1. Professional Noticing of the Students’ Mathematical Thinking

To improve the teaching and learning of a mathematical topic, we need to make sense of different classroom situations. Mason (2002) considers that noticing intentionally is characteristic of a profession and differentiates the professional from someone who is not. This author maintains that the increase in intentionality reflects professional experience and that experienced individuals can go beyond common reactions. In this way, they respond more professionally to different aspects of a situation. According to Mason, the competency of “noticing professionally” involves two processes: (1) reporting as objectively as possible about phenomena, avoiding interpretation, judgment, or evaluation, and (2) “explaining” what happens in the classroom or explaining and interpreting what is perceived.

A particular aspect of Mason’s noticing is the professional noticing of students’ mathematical thinking conceptualized by Jacobs et al. (2010) as a set of three interrelated skills: (a) attending to students’ strategies, (b) interpreting students’ understanding, and (c) deciding how to respond based on inferred understanding. Understanding and analyzing students’ mathematical reasoning involves “reconstructing and inferring” students’ understanding from what the student writes, says, or does. Interpreting students’ mathematical thinking requires more than simply indicating what is correct or incorrect in their answers; it requires determining how students’ answers are or are not significant from the point of view of mathematical learning (Hines & McMahon, 2005; Wilson et al., 2013).

1.2. Curricular Noticing

Curricular noticing is defined as the process through which teachers make sense of the complexity of the content and pedagogical opportunities from it (Males et al., 2015). It is based on three interrelated skills as an extension of the framework “professional noticing of students’ mathematical thinking”. These skills comprise a set of curricular practices that allow teachers to recognize, make sense of, and strategically use the opportunities offered by curricular materials. These can take various forms, such as mathematical activities (tasks, games, exercises, etc.), mathematical content (a definition or theorem, etc.), and teaching methodology (timing, organization of the classroom class, etc.) (Dietiker et al., 2018).

In the curricular noticing, these skills are defined as:

• Curricular attending: noticing, reading, and recognizing particular aspects of the curricular materials based on the educational objectives set, the purposes of the interaction, how to structure an activity, which questions to ask during the activity, and any reference to possible strategies of the students, etc.
• Curricular interpreting: Making sense of what has been identified, connecting the aspects identified in the materials considering the teachers’ mathematical knowledge for teaching, the knowledge of the subject, and the knowledge of the pedagogical content (including the knowledge of the students, the teaching, and curriculum) and horizon content knowledge (Ball et al., 2008). Interpreting curricular materials by teachers depends on their previous experiences, objectives, and previous knowledge.
• Curricular responding: Making curricular decisions based on interpretation, choosing, manipulating, sequencing, adapting, and deciding. This skill includes both decisions about how to respond and how these responses are enacted in the classroom, taking into account the interpretation of what the curriculum offers and whether it is in line (or not) with the learning objective(s) to be reached. Teachers also use their interpretations to make decisions about what and how to use the curricular materials as they plan.

Research indicates that the development of noticing skills during initial teacher training correlates with changes in pedagogical reasoning (Seidel & Stürmer, 2014; van Es & Sherin, 2002). In this study, we conceptualize curricular reasoning as the diverse ways in which noticing skills are interconnected (Barnhart & van Es, 2015; Rotem & Ayalon, 2023; Scheiner, 2021; van Es & Sherin, 2021).

1.3. Relationship Between Professional Noticing of Students’ Mathematical Thinking and Curricular Noticing

Both professional noticing of students’ mathematical thinking and curricular noticing demonstrate similar cognitive processes, suggesting that the development of one can support the development of the other (Kang & Ellis, 2024).

Focusing on the specific skills within professional and curricular noticing, respectively, we can establish connections between them. In relation to the “attend to” skill in professional noticing, (preservice) teachers should concentrate on students’ mathematical thinking and their use of mathematical strategies (Jacobs et al., 2010). When attending to curricular materials, (preservice) teachers should focus on the underlying design principles, long-term mathematical learning objectives, and pedagogical opportunities (Dietiker et al., 2018). Regarding the “interpret” skill, whether interpreting student mathematical thinking or curricular materials, (preservice) teachers should make sense of their observations by drawing upon their mathematical content knowledge, pedagogical content knowledge, knowledge of students, curriculum knowledge, and knowledge of teaching strategies (Dietiker et al., 2018; Jacobs et al., 2010). Finally, concerning the “decide” skill, both contexts necessitate consideration of students’ mathematical thinking and curricular materials. This involves making adaptations based on student responses whilst also considering the (enacted) curriculum. Consequently, this leads to decisions about which problems to pose next, how to modify a task, or how to sequence tasks in a different order (Dietiker et al., 2018; Jacobs et al., 2010).

Further research appears necessary to investigate how these theoretical frameworks can support preservice teachers in developing the competence to effectively notice mathematics teaching and learning situations within the context of teacher education programs. This is particularly relevant given the limited research that utilizes both frameworks (e.g., Amador et al., 2017), with studies such as Kang and Ellis (2024), in the context of mathematical modeling, representing notable exceptions.

Therefore, the objective of this research is to answer the following research question:

• Does the curricular noticing of preservice secondary teachers influence their professional noticing of the mathematical thinking of a student of the fourth compulsory secondary education?

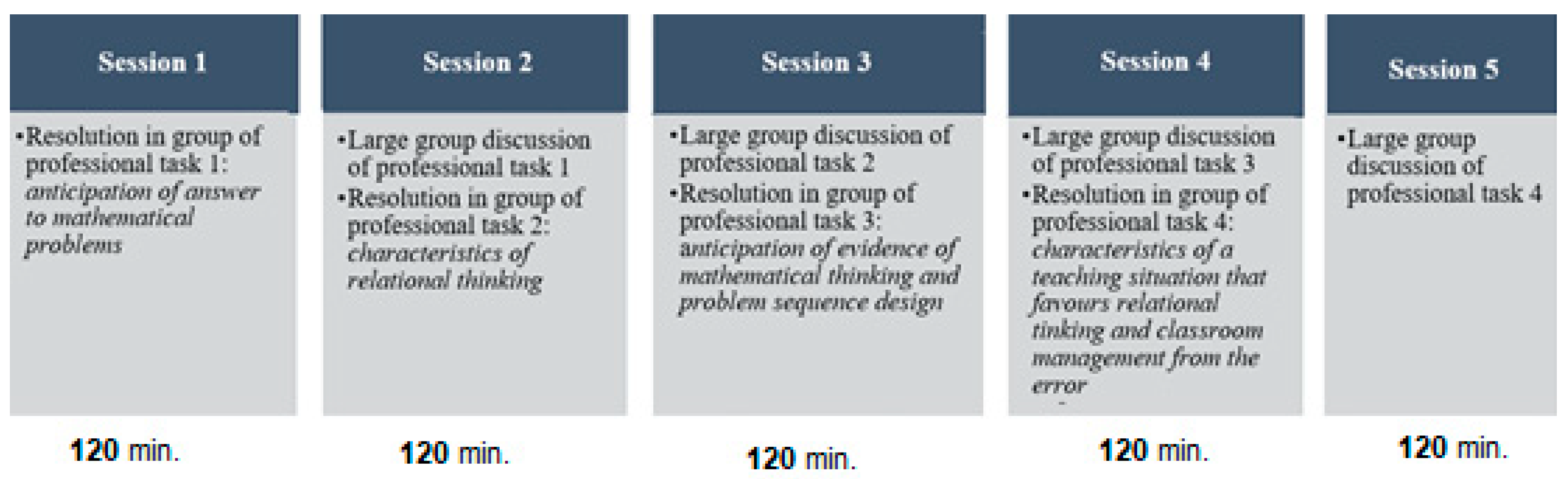
2. Materials and Methods

2.1. Participants and Settings

Twenty secondary preservice teachers (SPTs), with varied undergraduate degrees in engineering, physics, and mathematics, participated in this study. Recruited from two Spanish universities, they were randomly assigned to five groups of four (G1–G5) and were engaged in their respective universities’ Teaching Professional Master’s programs. The training program featured diverse learning environments addressing multiple mathematical domains. All participants were informed about the research, freely agreed to participate, and gave permission to use their written reports as data for the study.

This study centered on a learning environment designed to foster “Relational Thinking” within arithmetic and algebraic contexts. Specifically, two of the three researchers served as instructors within this environment. The SPTs should (a) identify and characterize evidence of relational thinking in high school students when solving arithmetic and algebraic problems and (b) recognize the importance of developing relational understanding (Skemp, 1978) in high school students so that they acquire the mathematical competence established in the high school curriculum.

The environment was composed of five sessions of 120 min each. The SPTs carried out four professional tasks. The professional tasks consisted of two primary elements: practice records, encompassing student mathematical problem responses, teacher–student interactions, and textbook materials, and associated professional questions. In the first session, SPT groups collaboratively completed professional task 1. Sessions two, three, and four commenced with a whole-group discussion, led by the teacher, regarding the professional task completed in the preceding session. Following this discussion, the SPT groups proceeded to solve a new professional task. In the fifth session, SPTs participated in a whole-group discussion of professional task 4 (Figure 1).

The primary objective of the initial three sessions was to enable SPTs to characterize their own and high school students’ solutions to various arithmetic and algebraic tasks, differentiating between relational and procedural thinking, and to interpret student responses. The fourth session focused on developing SPTs’ ability to notice high school students’ mathematical thinking, particularly when errors occurred in arithmetic task solutions, and to determine strategies for promoting relational thinking through mathematical discussions.

To complete the professional tasks, the SPTs were provided with three key professional materials: two ad hoc theoretical documents, DOC.1 addressing relational thinking and DOC.2 focusing on productive mathematical discussions, and the official state Compulsory Secondary Education curriculum document (BOE, 2015).

For the first three professional tasks, in addition to the curriculum, the SPTs had the theoretical document about relational thinking (DOC.1) with information on the characteristics of relational and procedural thinking. This document presents different definitions of relational thinking from other authors. Firstly, it indicates what it means for Mason et al. (2009) that an individual manifests relational thinking. Secondly, the particularization of relational thinking in arithmetic by Castro and Molina (2007) is shown, followed by some examples of resolutions that manifest relational thinking in arithmetic and, finally, the particularization of relational thinking in algebra by Hoch and Dreyfus (2006), contrasting this definition with that of procedural thinking as well as some examples where both thoughts are revealed. To solve professional task 4, the SPTs had the curriculum, the document about relational thinking, and a new document related to productive mathematical discussions (DOC.2). This theoretical document provided information on five practices for orchestrating whole-class discussions using students’ responses to promote relational thinking. It incorporated examples of teacher-proposed scenarios, unexpected classroom situations, and student errors (Shimizu et al., 2015; Skemp, 1978; Stein et al., 2008).

In this study, we concentrate on the analysis of the professional task from the fourth session, as it offers insights into how SPTs utilize the provided professional materials to observe high school students’ mathematical thinking and facilitate relational thinking through productive mathematical discussions.

2.2. Data Collection Instrument

The data collection instrument is the professional task 4 (Figure 2) performed in session 4. The practice register of professional task 4 was created to include (a) two arithmetic problems within the numbers and algebra block (BOE, 2015), aimed at recognizing the notable identity “sum by difference, difference of squares” in a numerical equality; and (b) a resolution by Carlos, high school student (15–16 years old). We note that Carlos’ resolution includes an over-generalization of the multiplication property of powers as applied to the subtraction of powers. The professional questions aimed to guide the SPTs toward a professional notice of Carlos’ mathematical thinking and to the approach of a possible productive mathematical discussion in the classroom to promote relational thinking. For the resolution of the professional task, the SPTs had at their disposal the professional materials about relational thinking (DOC.1), productive mathematical discussions (DOC.2), and the official state Compulsory Secondary Curriculum document (BOE, 2015).

A potential planning task to enhance Carlos’ relational thinking could involve the SPTs first addressing an error (whether it be a nonexistent or misapplied property) to ensure that the student recognizes it. Following this, the SPTs could provide a series of similar tasks with different pairs of numbers, allowing him to identify and apply the “difference of squares” property to various specific cases: 4² − 2²; 7² − 5²; 5² − 2²; …; 60² − 40²; …; etc. Subsequently, Carlos would be able to apply this understanding to algebraic expressions: 4x² − y²; 16x² − 9y². For the final task, the SPTs could suggest solving the following problem: 1001² − 999².

2.3. Analysis

The data consist of the written report from the five SPT groups to professional task 4, carried out in session 4. The qualitative analysis was completed and triangulated by three researchers in accordance with the analysis scheme, which is shown in

Table 1. Scheme of analysis of the professional noticing of students’ mathematical thinking mediated by the analysis of the noticing of the professional materials.

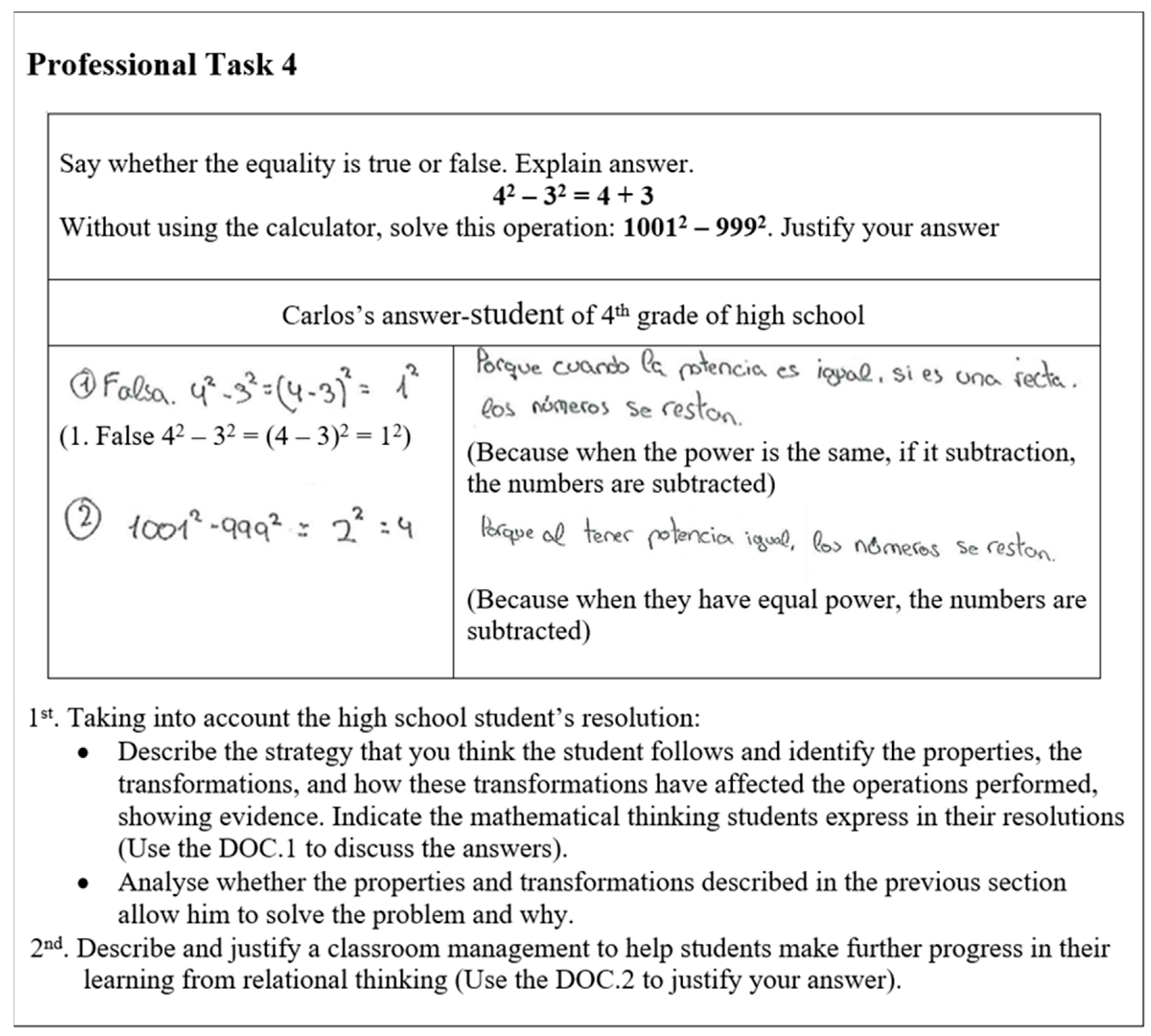
	Skills	Description	Skills		Description
Curricular Noticing	The curricular content required for problem-solving	Problems: power, arithmetic operations, hierarchy of operations, notable identity: “sum by difference”. Curriculum: (Block 2: numbers and algebra)	Attending to	Mathematical content implicit in problems and Carlos’ resolution of the problem	Carlos’ answer: Over-generalization of the power multiplication property, applied to the subtraction of powers: (a − b)n = an − bn. Curriculum	Professional Noticing
Curricular Noticing	The problems and the answer from the mathematical knowledge for teaching	Carlos’ answer: They connect Carlos’ answer with the meaning of relational or procedural thinking. DOC.1	Interpreting	Carlos’ mathematical thinking	Carlos’ answer: they indicate the type of thinking Carlos expresses, justifying it through evidence DOC.1	Professional Noticing
Professional Noticing	About what problems they propose	Coherence of the objective with the problems Whether the problems encourage relational or procedural thinking. DOC.1	Deciding	About how they respond (considered content) on how they carry it out (instructional support)	They consider the contents of powers and their properties and/or notable identities. Curriculum They sequence the problems to be proposed and give methodological indications. DOC.2	Curricular Noticing

. In each group’s written report, expressions were identified that evidenced the use of the curriculum, for instance, in determining the task’s objective, or in detecting the error in student Carlos’ response by relating it to a nonexistent property of powers. We also focused on the use of any of the professional materials. For example, if, when interpreting the students’ thinking, reference was made to the characteristics of relational thinking provided in the professional material (DOC.1):

[…] based on the five ways of paying attention to mathematical objects or structures (Mason et al., 2009), we consider that relational thinking in arithmetic focuses on:

• The use of the fundamental properties of numbers and operations to transform numerical sentences rather than to find the result of operations (Kızıltoprak & Köse, 2017),
• The intellectual activity of examining arithmetic expressions globally (i.e., as wholes) and taking advantage of the relationships identified both to solve a problem and to make a decision or learn more about a situation or a certain concept (Castro & Molina, 2007).

This key role offers two benefits that allow students not only to restructure arithmetic operations to change the calculation but also to transform numerical sentences using fundamental arithmetic properties. Relational thinking helps students realize that both sides of an equation represent the same numbers without doing any calculations.

Furthermore, we identified whether the decision was aligned with the interpretation of the students’ thinking and whether the sequence of tasks, along with the instructional strategies, would promote relational thinking or, conversely, if their decisions merely attempted to resolve the error and, subsequently, the SPTs proposed tasks to reproduce procedures (Table 1).

For example, Group 5, based on its interpretation of DOC.1, considers Carlos’ mathematical thinking to be relational due to the SPTs. Group 5 considered characteristics of relational thinking reflected in DOC.1: they understood the operation as a whole and the use of properties to interpret Carlos’ mathematical thinking (

Table 2. Evidence of how Group 5 interprets document DOC.1 and Carlos’ mathematical thinking.

Evidence (Group 5)
We believe that the student shows evidence of relational thinking since he observes the operation as a whole and tries to apply a property (even if it is wrong). If he had procedural thinking, in the first case, “42 − 32”, he would have calculated the first term 42 = 4·4 = 16 and then the second 32 = 3·3 = 9 and would have subtracted both numbers later straight away.
Curricular Noticing	Interpreting document DOC.1	Interpreting thinking	Professional Noticing
	[…] Observes the operation as a whole and tries to apply a property (even if wrong)	We believe the student shows evidence of relational thinking […], if he had procedural thinking, in the first case, “42 − 32”, he would have calculated the first term 42 = 4·4 = 16 and then the second 32 = 3·3 = 9 and then subtracted it straight away.

).

Meanwhile, Group 4 interprets Carlos’ mathematical thinking to be procedural thinking because they have considered that the characteristics of relational thinking reflected in DOC.1 are not presented in the student’s answer: the recognizing of relationships and properties, the totality of the mathematical structure (

Table 3. Evidence of how the G4 group interprets DOC.1 and Carlos’ mathematical thinking.

Evidence (Group 4)
The student does not know how to use it, or he, perhaps, confuses the properties. Consider that, if the exponents of subtraction are the same, it operates with the bases leaving the same exponent. Therefore, you arrive at a wrong solution. The student has procedural thinking since he is unable to recognize relationships and perceive properties and therefore does not reason from those properties. Furthermore, he has not been able to notice the entire mathematical structure, he has not identified the remarkable identity property.
Curricular Noticing	Interpreting document DOC.1	Interpreting thinking	Professional Noticing
	[…] he is unable to recognize relationships and perceive properties; […] he does not reason based on those properties. Furthermore, he has not been able to notice the totality of the mathematical structure […]	[…] The student has a completely procedural thinking

).

The analysis has enabled us to understand how the SPTs interpreted Carlos’ response through the contents of the curriculum and the theoretical aspects of procedural and relational thinking outlined in DOC.1. Furthermore, their curricular noticing influenced whether Carlos’ answer was deemed significant in terms of relational thinking, ultimately resulting in a variety of decisions (DOC.1 and DOC.2).

3. Results

The results present in two sections that correspond to the two ways of noticing Carlos’ mathematical thinking influenced by their curricular noticing to the professional materials. Some SPTs focused on a relational interpretation (and response) and others focused on a procedural interpretation (and response).

3.1. SPTs Notice Carlos’ Mathematical Thinking as Relational Thinking

The SPTs of groups G2 and G5 prioritized the use of a property as a characteristic of relational thinking (DOC.1), regardless of its validity, considering that relational thinking implies focusing attention on identifying properties and how the operations are transformed to be applied in the resolution of the problems. Consequently, the SPTs of these groups have valued that Carlos manifests relational thinking by having examined the arithmetic expressions given globally (i.e., as wholes) and identified as a property the “over-generalization” of the multiplication of powers applied to the subtraction of powers, although wrong (

Table 4. Evidence of how the G2 group interprets DOC.1 and Carlos’ thinking.

Evidence (Group 2)
The student tries to apply relational thinking because he thinks he has identified a (wrong) property of powers when it has a different base, but the same exponent. The property he is trying to apply, as it is not true leads him to the wrong conclusion. If he had used procedural thinking by performing operations, he would have realized that the equality is true. Even though he could have concluded that the equality is true as a property (a2 − b2 = a + b).
Curricular Noticing	Interpreting document DOC.1	Interpreting thinking	Professional Noticing
	[…] identifies a property of powers (wrong) that have different base, but the same exponent.	The student tries to apply relational thinking […]. If he had made use of procedural thinking by performing operations, he would have realized that the equality was true.

).

The interpretation made by both groups has given rise to two decisions that affect the content to be taken into account (how to respond) and the type of tasks to favor relational thinking. The G2 group focuses on powers and their properties while the G5 group focuses on powers and notable identities. Regarding the type of tasks, the G2 group proposes two tasks whose objective is to identify and apply the properties of powers to simplify expressions (

Table 5. Evidence of how the G2 group makes decisions.

Evidence (G2)
First, Carlos is asked to perform the calculation by expressing the powers as products, that is, 4.4 − 3.3, calculating the result and finding the difference. In this way, he would realize that his proposal is incorrect since 4.4 − 3.3 = 7 ≠ (4 − 3)2 = 12, and he would recognize that the statement is correct. Next, he would be asked if anyone in the class knows which property his classmate attempted to use. Together, we would likely conclude that there has been a confusion between an·bn = (a·b)n which is correct, and an + bn = (a + b)n, which is incorrect. The following question is posed to the class: If 42 − 32 = 7 = 4 +3 is correct, could we identify as a property a2 − b2 = a + b? Assuming that a student responds yes or expresses doubt, we would use the second part to justify that a2 − b2 = a + b cannot be identified as a property. We would operate as follows: 10012 − 9992 = 1001 + 999 = 2000. Now, to verify this, we would calculate the result using another method that we would ask the students about it. Almost certainly, they would suggest using the same approach as in the first part, that is, procedurally 10012 − 9992 = 1001·1001 − 999·999 = 1 002 001· 998 001 = 4 000. This way, we confirm that a2 − b2 = a + b is not a property. Finally, to further develop relational thinking, we would ask if they remember any notable identities and if there are any that could be used in these exercises. We would conclude by recalling that a2 − b2 = (a + b)·(a − b) and solve both exercises: 42 − 32 = (4 + 3)·(4 − 3) = 7·1 = 7; 10012 − 9992 = (1001+ 999)·(1001 − 999) = 2000·2 = 4000. With this lesson, the aim would be to motivate the students to approach the problems in different ways and to verify their results, while also emphasizing that using notable identities leads to simpler operations.
Professional Noticing	Making decisions		Curricular Noticing
	Proposing problem	Strategies for mathematical discussion
	Express the powers of 42 − 32 as products	[…] would realize that what he has proposed is wrong since 4.4 − 3.3 = 7 ≠ (4 − 3)2 = 12 and he would detect that the statement is correct
	If 42 − 32 = 7 = 4 + 3 is correct, can it be identified as a property that a2 − b2 = a + b?	[…] to justify that a2 − b2 = a + b cannot be identified as a property. We would operate as follows: 10012 − 9992 = 1001 + 999 = 2000 […] 10012 − 9992 = 1001·1001 − 999·999 = 1 002 001·998 001 = 4 000
	Solve 42 − 32 and 10012 − 9992	[…] we would ask if they remember the notable identities and if there were any that could be used in these exercises.

).

Meanwhile, the G5 group poses tasks of the type: (a − b)², a² − b², (a + b) · (a − b) to apply properties of powers and notable identities to recognize these simple structures in more complex ones as proposed by Hoch and Dreyfus (2006) in DOC.1 to promote relational thinking in the case of algebraic structures (

Table 6. Evidence of how the G5 group makes decisions.

Evidence (G5)
In the first place, to understand the problem, the student would be presented with two different ways of solving powers. They have a different base and the same power but are expressed differently. In this way, the aim is to make the student see that the result is not the same. For example: (4 − 3)2 = 12 = 1 42 − 32 = 16 − 9 = 7, donned 42 = 16 and 32 = 9 As can be verified (4 − 3)2 ≠ 42 − 32, in this way, the student would understand that the property used to solve the problem is wrong. This is a procedural way of solving the problem, helping to understand the inequality. Investigating further, the student can solve the problem in a relational way, as was his initial purpose, but he must understand the correct property that he can apply for its resolution. This case can be solved by applying the notable identities; specifically, the following: (a + b)·(a − b) = a2 − b2 The next step is to make the student understand that this property was valid for solving the problem, and the first problem is presented inversely, how notable identity is studied, i.e.: (4 + 3)·(4 − 3) = 42 − 32 = 16 − 4 = 12. With this, we guide the student to be able to choose the most appropriate manipulations to make use of the structure in its minimum expression and thus be able to show relational thinking by applying properties correctly. Therefore, once he understands the exercise, the aim is for the student to solve the second problem proposed contrary to how this property is usually studied, to observe whether he has been able to understand these notable identities, which facilitates the resolution of problems of differences of square power with different numerical bases. With what has been learned, the student should now be able to solve it without any difficulty and when solving it, making the necessary transformations to apply the notable identity and, according to Hoch and Dreyfus (2006), he would show relational thinking in this exercise: 10012 − 9992 = (1001 + 999)·(1001 − 999) = 2000·2 = 4000 Once the value of 10002 − 9992 has been correctly calculated through the notable identity, a more complex exercise is proposed to the student in order to see if he/she is able to identify the relationship between the new exercise and the property ‘sum by difference equals difference of squares’ favoring relational thinking. The exercise proposed is: (x − 3)4 − (x + 3)4.
Making decisions
Professional Noticing	Proposing problem	Strategies for mathematical discussion	Curricular Noticing
	[…](4 − 3)2     42 − 32 (4 + 3)·(4 − 3) 10012 − 9992 […] (x − 3)4 − (x + 3)4 They enhance relational thinking	[…] I would present the student with two different ways of solving powers […] […] pose the first problem in an inverse manner, which is how said remarkable identity is studied […] […]identify the relationship between the new exercise and the notable identity

).

Both decision-making processes support relational thinking related to the content to be learned in the training program and they are accompanied by the appropriate strategies for mathematical discussion.

3.2. SPTs Notice Carlos’ Mathematical Thinking as Procedural Thinking

The SPTs of groups G1, G3, and G4 prioritized the use of an erroneous property, which is not a mathematical property, as its characteristic of procedural thinking (DOC.1). The SPTs interpreted that relational thinking implies identifying a mathematical property and that its application, based on the corresponding transformations, allows the problem to be correctly solved. Therefore, these groups of SPTs considered that Carlos manifests procedural thinking by not examining the arithmetic expressions given globally (i.e., as wholes) and, consequently, not identifying the property of the powers that would allow solving the problems, which explains Carlos’ erroneous transformations (

Table 7. Evidence of how the Group G1 of SPTs interprets DOC.1 and Carlos’ thinking.

Evidence (Group 1)
The student knows the power verbally but does not know its concept and confuses the property of multiplication of powers with the same exponent, using it also for subtraction. 1st, he considers that A2 − B2 = (A − B)2, although he does not make this explicit; 2nd, he operates; 3rd, in the last step, (22 = 4), we do not know if the pupil understands the power correctly and therefore does 22, or multiplies the base by the exponent. His procedure indicates that there is a predominance of procedural thinking.
Curricular Noticing	Interpreting document DOC.1	Interpreting thinking	Professional Noticing
	[…] confuses the property of multiplication of powers with the same exponent, using it for subtraction as well […]	[…] 1st, he considers that A2 − B2 = (A − B)2, although this is not made explicit; 2nd, operates; 3rd, in the last step, (22 = 4), we do not know if the pupil knows the power correctly and therefore does 22, or on the contrary multiplies the base by the exponent. His procedure indicates that there is a procedural predominance.

).

Another piece of evidence that characterizes Carlos’ interpretation of mathematical thinking as procedural is the one we present below, coming from the G3 group of SPTs (

Table 8. Evidence of how Group 3 of SPTs interprets document DOC.1 and Carlos’ thinking.

Evidence (Group 3)
We notice that in step 1 [solving the first problem] he performs a subtraction transformation of the form: a2 − b2 = (a − b)2, which is not right. It is a purely procedural resolution, as he has not understood the properties of addition (subtraction), and merely applies a formula he remembers.
Curricular Noticing	Interpreting document DOC.1	Interpreting thinking	Professional Noticing
	[…] performs a subtraction transformation of the form a2 − b2 = (a − b)2, which is not correct […].	[…] It is a purely procedural resolution, as he has not understood the properties of addition (subtraction), and merely applies a formula he remembers.

).

The interpretation carried out by the three groups involved decision-making regarding the contents to be taken into account (how to answer), focused on powers and notable identities. The proposed exercises are intended to recognize the error and then use the notable identity in a numerical identity to solve it (

Table 9. Evidence of how the Group G1 of SPTs makes decisions.

Evidence (G1)
We propose the first exercise to the class 42 − 32 = 4 + 3. After a few minutes, we call on a student, Student A, to write his response on the board: 42 − 32 = (4 − 3)2 = 12. To the question of whether all the students agree, Student B says that 42 − 32 = 16 − 9 = 7 ≠ 12. In response to these answers, we present the following exercises on the board: 42 + 32   42·32   42÷32 We allow a few minutes for the students to solve them. We encounter cases where the answers are like those of Student A and Student B. We collect the solutions from Students A and B in the following table.
		Exercises	Solution from Student A		Solution from Student B
		42 + 32	(4 + 3)2 = 72 = 49		16 + 9 = 25
		42·32	(4·3)2 = 122 = 144		16·9 = 144
		42 ÷ 32	(4 ÷ 3)2 = 1.332 = 1.77		16 ÷ 9 = 1.77
In light of these responses, the students realize that Student A’s method only works in cases of multiplication and division of powers with the same exponent. We conclude by asking the students to solve the exercise 10012 − 9992. After a few minutes, Student E solves the problem by applying what he has learned: (1001)·(1001) − (999)·(999). In response, Student F states that it can be solved using a concept studied in previous topics, the difference of squares: 10012 − 9992 = (1001 + 999)·(1001 − 999) = 2000·2 = 4000
Making decisions
Professional Noticing	Proposing problem			Strategies for mathematical discussion			Curricular Noticing
	State whether the equality is true or false 42 − 32 = 4 + 3 Perform the operations: 42 + 32  42·32  42÷32 Resolve this operation: 10012 − 9992			[…] After a few minutes, we call on Student A to write his response on the board: 42 − 32 = (4 − 3)2 = 12. When asked if all the students agree, Student B says that 42 − 32 = 16 − 9 = 7 ≠ 12. […] […] We collect the solutions from Students A and B in the following table [see evidence] […] We conclude by asking the students to solve the exercise 10012 − 9992

).

4. Discussion and Conclusions

The objective of this research is to investigate the impact of professional material interpretation on the decision-making of preservice secondary teachers when analyzing the mathematical thinking of a 15–16-year-old high school student engaged in arithmetic problem-solving. The findings revealed that SPTs’ engagement with curricular materials significantly shapes their professional noticing of student thinking, leading to varied instructional decisions.

The results highlighted two distinct approaches among SPTs in interpreting student errors. Some groups viewed errors as indicators of procedural thinking, emphasizing the importance of mathematically valid properties. These SPTs proposed tasks focused on correcting errors and reinforcing procedural knowledge. Other groups interpreted the same errors as evidence of relational thinking, valuing the student’s attempt to use properties, albeit incorrectly. These SPTs designed tasks to build on the student’s understanding and promote deeper relational thinking. The importance of this result lies in how the different SPTs have interpreted the same professional document about relational thinking. The difficulty in the interpretation was not in the mathematical content of the task or in the student’s error but in how each group of SPTs interpreted the meaning of the error in terms of a document prepared by the teacher training program’s instructors, which contained pedagogical content that was novel to all of them. These interpretation patterns tell us about how they conceive mathematics teaching and how they value student errors, with some SPTs placing mathematical correctness at the center of their decision-making, while for others, the student’s attempt to use properties was the main factor in deciding subsequent learning. Possibly, in both cases, personal beliefs and experiences are conditioning the interpretation and decision-making (Schoenfeld, 2010).

All secondary preservice teachers have determined the nature of student’s mathematical thinking by considering several characteristics that comprise relational thinking (e.g., examining the entirety of the mathematical structure, recognizing relationships, identifying properties, and reasoning from identified properties) based on the professional materials (DOC.1). This implies that they have engaged in curricular reasoning with the professional materials of the training program. The difference in the two interpretations of the student’s mathematical thinking made by the SPTs lies in how they reasoned with the professional materials to generate knowledge to notice the student’s mathematical thinking.

The study also revealed that SPTs’ interpretations and decisions were influenced by their prior experiences, beliefs about mathematics teaching, and the learning environment. This suggests that teacher education programs should focus on developing SPTs’ ability to critically evaluate and integrate information from professional materials as well as fostering a deeper understanding of relational thinking (Hoch & Dreyfus, 2006; Mason et al., 2009; Castro & Molina, 2007). Although it might not be possible to fully assess the characteristics of a student’s relational or procedural thinking with a single task, we can observe how future teachers analyze and interpret the curriculum and the training program’s theoretical materials to generate information that allows them to examine a student’s thinking. This provides valuable feedback to teacher trainers, enabling them to enhance the training environment based on the identified difficulties.

The curricular reasoning expressed has shaped the preservice teachers’ decisions, leading those who believed that the properties used should be mathematically valid to consider that the student exhibited relational procedural thinking. They proposed tasks aimed at helping secondary education students understand the properties of exponents and apply them in various arithmetic expressions. However, this decision-making does not guarantee the development of relational thinking that would benefit the student, as it fails to introduce new tasks that enable students to recognize simple structures within other more complex ones and to use the appropriate properties in different contexts. This decision-making highlights a conception of mathematics teaching in which students’ errors are not leveraged as learning opportunities to progress toward relational thinking, which would allow for effective problem-solving in various situations. This might stem from their lived experiences, their schooling, their beliefs about teaching mathematics, and other factors.

On the contrary, the groups of secondary preservice teachers who emphasized the importance of the student believing he had identified a property, and thus demonstrated relational thinking, proposed tasks that supported the student’s relational thinking, encouraging the identification of simple structures within more complex ones and the use of the properties of exponents and notable identities (Hoch & Dreyfus, 2006). This decision reveals that these preservice teachers not only correct the student’s error but also leverage it as a learning opportunity for students to deepen their understanding of mathematical relationships and properties in the resolution of arithmetic and algebraic expressions. The mathematics secondary preservice teachers’ noticing of the professional materials and the student’s mathematical thinking could have been mediated by the educational context in which they carried out their professional task and the expectations that the SPTs considered that the teacher educators had (Mason, 2021). We do not have enough information to know whether the decisions were due to the recognition of the learning opportunities offered by the professional materials (Dietiker et al., 2018).

This research provides insights into how preservice teachers begin to make sense of professional materials to interpret students’ mathematical thinking and how they plan to use these materials to anticipate instruction (Brown & Edelson, 2003), ultimately proposing strategies for mathematical discussion determined by their curricular reasoning. The empirical results obtained show that preservice teachers have followed a process entails several key stages:

• Preservice teachers initially attend to the mathematical content of the problem statement, aligning it with the curriculum (curricular noticing).
• Subsequently, they analyze the student’s response to identify errors and recognize utilized mathematical properties (professional noticing), connecting these observations to both the curriculum and relevant theoretical documents (professional noticing and curricular noticing).
• Preservice teachers then interpret all available professional materials, generating knowledge (curricular noticing) that facilitates the interpretation of the student’s mathematical thinking (professional noticing).
• Finally, based on their interpretations, they determine the most appropriate task to address the student’s difficulties (professional noticing) and design a sequence of tasks to promote relational thinking (curricular noticing), for which the preservice teachers interact with all available professional materials to plan a lesson (professional noticing and curricular noticing).

This process provides empirical evidence for the relationship between curricular noticing and professional noticing and demonstrates how preservice teachers, when examining students’ mathematical thinking; integrate these two forms of noticing. This integration suggests that professional and curricular noticing can be viewed as complementary. This finding aligns with Kang and Ellis (2024), who suggest that curricular noticing is evident when educators observe and analyze students’ mathematical thinking, drawing upon professional noticing. The incorporation of curricular noticing into professional noticing underscores the consistent nature of the work of teaching (Males et al., 2015).

Nevertheless, it remains important to investigate the mechanisms by which preservice teachers make instructional decisions and implement effective classroom management strategies to foster student learning (Stein et al., 2007) within the context of lesson planning and implementation. Whole-class discussions conducted at the beginning of the sessions with the instructor may have facilitated understanding of the theoretical documents. However, it is necessary for the instructor to be able to interpret the SPTs’ thinking during the training sessions in order to make decisions that align with the learning objectives and support the development of competencies in future teachers

This research topic seems promising because, in studies on interactions with materials (Amador et al., 2017; Barno & Dietiker, 2022; Choy et al., 2017) as well as those focused on observing student thinking (Callejo et al., 2022; Fernández et al., 2024; Sánchez-Matamoros et al., 2019), future teachers, either explicitly or implicitly, handle documents and theoretical information that they must internalize. Their interpretation influences instructional decisions. This way of making the interrelationship between these two theoretical models explicit can be the starting point to answer the question of what other aspects condition the difficulty of teachers (future teachers) in decision-making and to understand decision-making conditioned by the future teachers’ curricular perspective on the material provided in the training program, among other aspects. For example, in studies where a consistent interpretation of the teaching situation and student thinking was shown, but disconnected from the curricular material, from the design, and from the interpretation of student thinking (Fernández et al., 2020; Rotem & Ayalon, 2023; Zorrilla et al., 2024).

In conclusion, this research contributes to the growing body of literature on teacher noticing by highlighting the crucial role of curricular materials in shaping preservice teachers’ instructional decisions. The findings suggest that teacher education programs should provide opportunities for SPTs to engage with a variety of professional materials and develop their skills in curricular noticing. Future research could explore the long-term impact of these interventions on SPTs’ teaching practices and student learning outcomes.