Redesigning and Evaluating a Science Activity to Foster Mathematical Problem Solving
Abstract
1. Introduction
2. Theoretical Frameworks as the Basis for the Research Questions and Analysis
2.1. Problem Solving
2.1.1. Problem Solving Process
2.1.2. Aspects of Problem Solving
2.1.3. Fostering (Mathematical) Problem Solving
- Learning problem solving through problem solving
- Reflecting on the problem-solving process
- Teaching problem-solving strategies (heuristics)
- Collaboration
2.1.4. Analysis Tool—Descriptive Phase Model of the Problem-Solving Process
2.2. Black Box
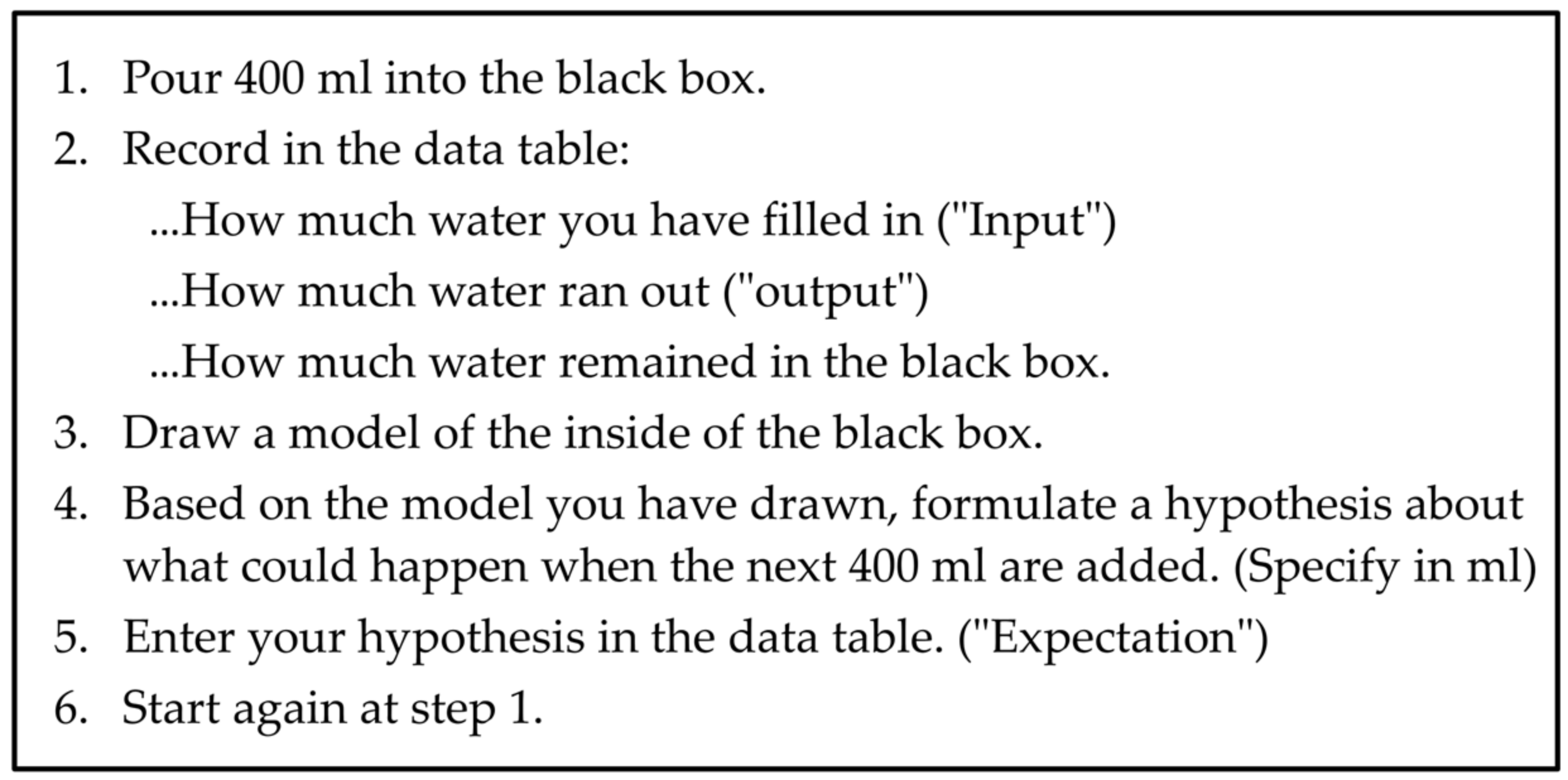
2.2.1. The Water Black Box and Its Simulation
2.2.2. STEM-Task—Description of the Original Biology Task
3. Aims and Research Questions
- What structures of students’ problem solving are identified in the (redesigned) task?
- What sub-processes, such as routine or non-routine, of problem-solving are realized in working on the (redesigned) task?
4. Research Design
4.1. Redesigning the Water Black Box Activity
- Look for regularities in the properties of the water box!
- What is the minimum amount of water that must be put into the empty box for water to flow out again?
- Does the partition of the water quantity influence how much water is in the measuring cylinder at the end?
- Check all the series of measurements!
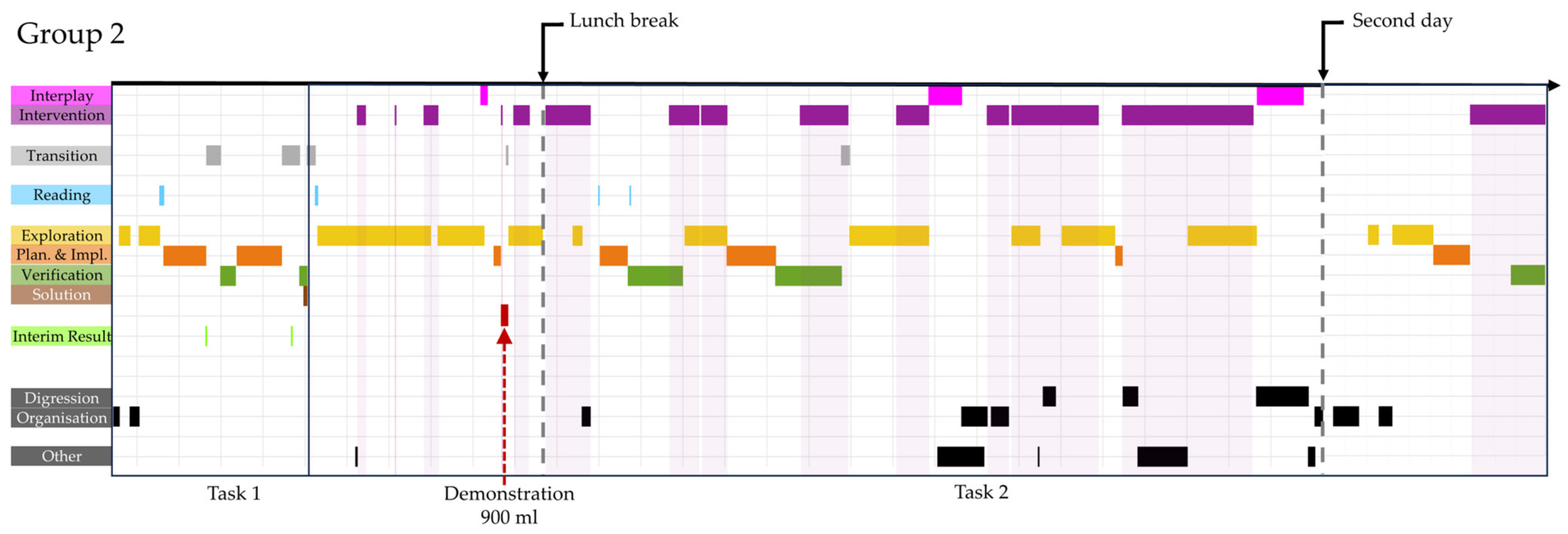
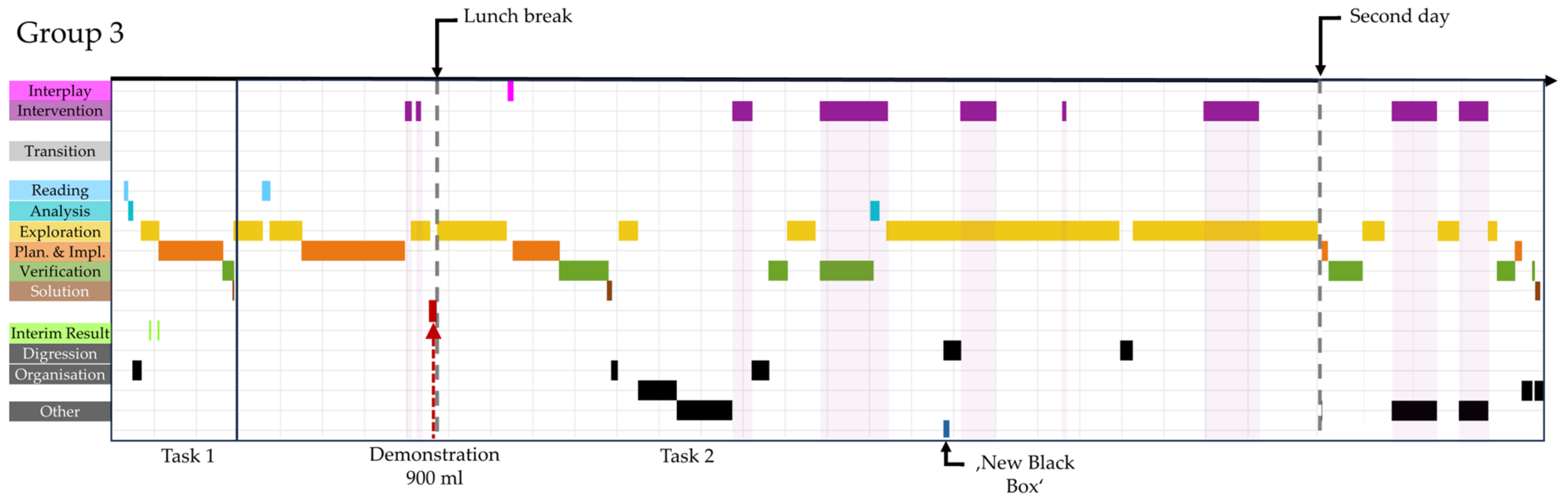
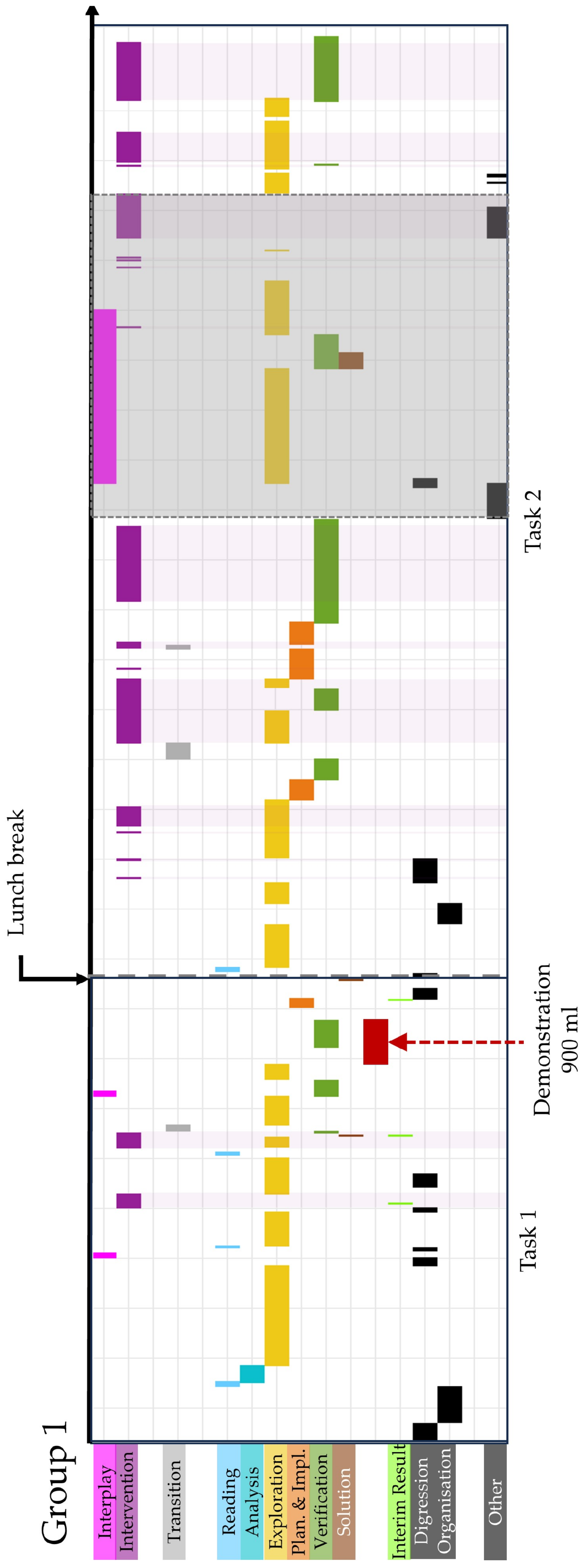
4.2. Evaluation of the Redesigned Learning Sequence according to Mathematical Problem Solving
4.2.1. Data Collection—Sample
4.2.2. Data Analysis—Categorization System
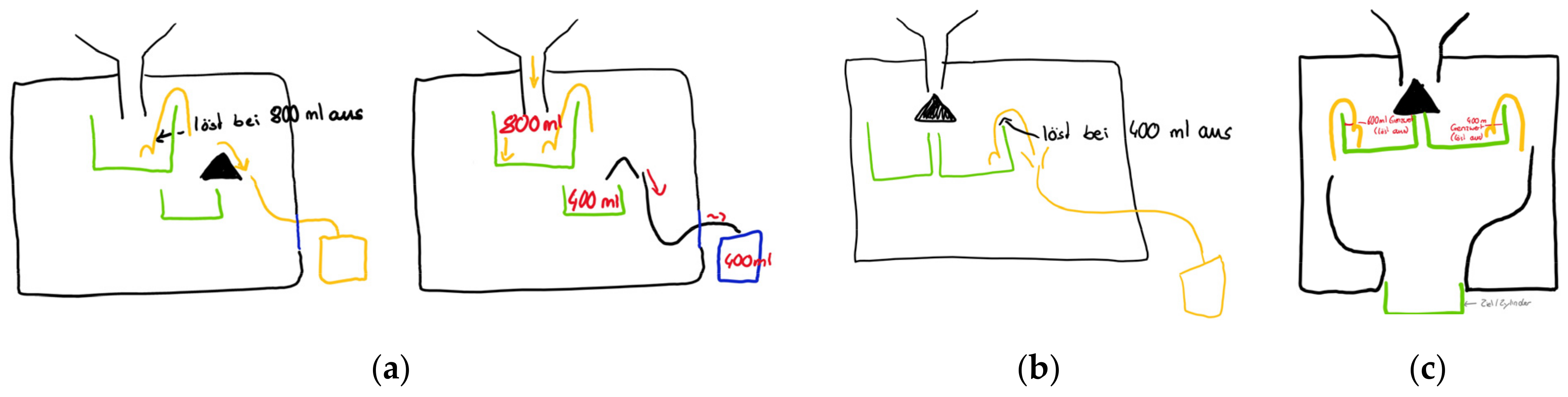
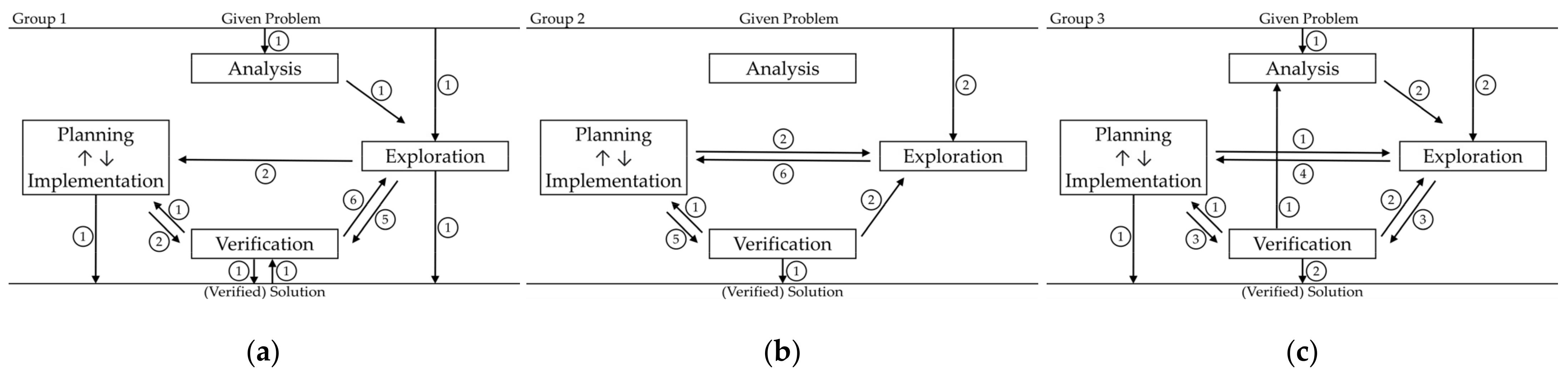
5. Results
6. Discussion
7. Limitations
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Details on the Qualitative Analysis
| Phase/Code | Description of the Code ** | Example of the Coding (Extracts of Episodes) |
|---|---|---|
| Analysis | Understanding the problem, including selecting certain perspectives and reformulating the problem | S5: What is our task actually? S6: Yes, okay. Determine how much water would flow into the measuring cylinder in the real water box if a-No, no, I can’t do that now (laughs) S5: Determine how much- S6: S5, that’s asking too much. S5: Determine how much water would flow in the real water box- S5: I don’t understand. S6: Determine how much water would flow into the measuring cylinder in the real water box if 900 milliliters were added in one step. Huh? Ah, we’re supposed to find out now if we put 900 milliliters into this funnel? S5: Yes. S6: How much then somehow- S5: It flows in there. S6: Exactly. 100 milliliters maybe. |
| Exploration | Unstructured approach to solve the problem, including exploration of the problem space and gathering relevant information. | S4: Okay, and on we go. S5: Guys, 400 are in. 400 is in, 400 is not out. Another 400 is 800. What runs out? S6: OK, eh? S5: 400 runs out when 800 is in. S4: That means there are 400 left. S5: There’s 400 left, now I‘ll add another 400. S6: That’s 1600 now. S5: Oh no. S6: Yes. No, it can’t be. S4: Wait a minute, yes, yes, yes. S6: No, we have 400. |
| Planning and Implementation | Structured approach to planning to solve the problem and its implementation to the problem | S8: Then step 2. 200 milliliters remain in the box. S7: Now we just have to do step 3 and step 4, I think, right? S8: Exactly, you can start at step-Okay, but we have to check that again. |
| Verification | Verification and testing of the solution or solution assumptions. | S1: Let’s see if our pattern is correct, that at least 800 are needed the first time. S2: Yes, wait a minute. S3: That was the case. That was already here- S1: Yes, yes, but we need more than 2. S2: Shall I do it with 200? S3: Yes. S1: Then 300. S1: Then 400. S3: No, then we’re at 900. S1: Yes? S3: Yes, but—yes, okay. S2: Yes, but then we can’t say #00:32:10# S1: Although, yes, yes, then do another 300. S3: That should be 400 now. (6 sec.) Yes. S1: Yes, that fits. |
| Solution | Students’ solutions to tasks, including solutions that the students have named as the final solution, regardless of whether the students have further engaged with the task after an intervention. | Solution followed by this conversation. S9: So, we have to contact the teacher now. So, no. S8: Let’s just say: We’re done. […] H1: Have you finished yet? S8: Yes. |
| Organization | Task-related work, but no content-related work | S8: No, but you’d better not take my sketch, I scribbled on it a bit. S9: Yes, otherwise I would have copied it in now and put #00:21:59# on it again. S8: Oh, we still have a bit of time. S9: I can draw it again. That’s not bad. |
| Digression | Conversations that are not related to problem solving | S5: Wait a minute (.) Let’s chill. |
References
- Martín-Páez, T.; Aguilera, D.; Perales-Palacios, F.J.; Vílchez-González, J.M. What are we talking about when we talk about STEM education? A review of literature. Sci. Ed. 2019, 103, 799–822. [Google Scholar] [CrossRef]
- Li, Y.; Wang, K.; Xiao, Y.; Froyd, J.E. Research and trends in STEM education: A systematic review of journal publications. Int. J. STEM Educ. 2020, 7, 11. [Google Scholar] [CrossRef]
- Kelley, T.R.; Knowles, J.G. A conceptual framework for integrated STEM education. Int. J. STEM Educ. 2016, 3, 11. [Google Scholar] [CrossRef]
- Aydin Gunbatar, S.; Ekiz Kiran, B.; Boz, Y.; Roehrig, G.H. A closer examination of the STEM characteristics of the STEM activities published in NSTA journals. Res. Sci. Technol. Educ. 2022, 1–26. [Google Scholar] [CrossRef]
- Just, J.; Siller, H.-S. The Role of Mathematics in STEM Secondary Classrooms: A Systematic Literature Review. Educ. Sci. 2022, 12, 629. [Google Scholar] [CrossRef]
- Galili, I. Physics and Mathematics as Interwoven Disciplines in Science Education. Sci. Educ. 2018, 27, 7–37. [Google Scholar] [CrossRef]
- van den Eynde, S.; van Kampen, P.; van Dooren, W.; de Cock, M. Translating between graphs and equations: The influence of context, direction of translation, and function type. Phys. Rev. Phys. Educ. Res. 2019, 15, 020113. [Google Scholar] [CrossRef]
- Siller, H.-S.; Günster, S.M.; Geiger, V. Mathematics as a Central Focus in STEM—Theoretical and Practical Insights from a Special Study Program Within Pre-service (Prospective) Teacher Education. In Disciplinary and Interdisciplinary Education in STEM; Li, Y., Zeng, Z., Song, N., Eds.; Springer Nature: Cham, Switzerland, 2024; pp. 317–343. ISBN 978-3-031-52923-8. [Google Scholar]
- Fitzallen, N. STEM Education: What Does Mathematics Have to Offer? In Mathematics education in the margins. In Proceedings of the 38th Annual Conference of the Mathematics Education Research Group of Australasia, Sunshine Coast, Australia, 13–18 July 2015; pp. 237–244. [Google Scholar]
- Braber, N.; Krüger, J.; Mazereeuw, M.; Kuiper, W. Reflecting on the Value of Mathematics in an Interdisciplinary STEM Course. In Proceedings of the Eleventh Congress of the European Society for Research in Mathematics Education, Utrecht, The Netherlands, 5–10 February 2019. [Google Scholar]
- Li, Y.; Schoenfeld, A.H. Problematizing teaching and learning mathematics as “given” in STEM education. Int. J. STEM Educ. 2019, 6, 44. [Google Scholar] [CrossRef]
- Stohlmann, M. Three modes of STEM integration for middle school mathematics teachers. Sch. Sci. Math. 2019, 119, 287–296. [Google Scholar] [CrossRef]
- Maass, K.; Geiger, V.; Ariza, M.R.; Goos, M. The Role of Mathematics in interdisciplinary STEM education. ZDM Math. Educ. 2019, 51, 869–884. [Google Scholar] [CrossRef]
- Forde, E.N.; Robinson, L.; Ellis, J.A.; Dare, E.A. Investigating the presence of mathematics and the levels of cognitively demanding mathematical tasks in integrated STEM units. Discip. Interdiscip. Sci. Educ. Res. 2023, 5, 3. [Google Scholar] [CrossRef]
- Arnone, K.A.; Hanuscin, D. An Exploratory Cross-Sectional Survey Study of Elementary Teachers’ Conceptions and Methods of STEM Integration. J.-STEM 2018, 4, 159–178. [Google Scholar] [CrossRef]
- Shriki, A.; Lavy, I. Mathematics and sciences teachers collaboratively design interdisciplinary lesson plans: A possible reality or wishful thinking? In Proceedings of the 41st Conference of the International Group for the Psychology of Mathematics Education, Singapore, 17–22 July 2017; pp. 201–208. [Google Scholar]
- Krell, M.; Hergert, S. The Black Box Approach: Analyzing Modeling Strategies. In Towards a Competence-Based View on Models and Modeling in Science Education; Upmeier zu Belzen, A., Krüger, D., van Driel, J., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 147–160. ISBN 978-3-030-30254-2. [Google Scholar]
- Rott, B.; Specht, B.; Knipping, C. A descriptive phase model of problem-solving processes. ZDM Math. Educ. 2021, 53, 737–752. [Google Scholar] [CrossRef]
- Liljedahl, P.; Santos-Trigo, M.; Malaspina, U.; Bruder, R. Problem Solving in Mathematics Education; Springer International Publishing: Cham, Switzerland, 2016; ISBN 978-3-319-40729-6. [Google Scholar]
- OECD. PISA 2022 Assessment and Analytical Framework; PISA, OECD Publishing: Paris, France, 2023. [Google Scholar] [CrossRef]
- Lambdin, D.V. Benefits of teaching through problem solving. Teach. Math. Through Probl. Solving Grades PreK 2003, 6, 3–14. [Google Scholar]
- Cai, J.; Hall, E.; Cifarelli, V.; Hiebert, J.; Lester, F.; Zawojewski, J. What research tells us about teaching mathematics through problem solving. Teach. Math. Through Probl. Solving PreK 2003, 6, 241–254. [Google Scholar]
- Hiebert, J.; Carpenter, T.P.; Fennema, E.; Fuson, K.; Human, P.; Murray, H.; Olivier, A.; Wearne, D. Problem Solving as a Basis for Reform in Curriculum and Instruction: The Case of Mathematics. Educ. Res. 1996, 25, 12. [Google Scholar] [CrossRef]
- Rott, B. Rethinking heuristics—Characterizations and vignettes. LUMAT 2015, 3, 122–126. [Google Scholar] [CrossRef][Green Version]
- Mayer, R.E.; Wittrock, M.C. Problem solving. Handb. Educ. Psychol. 2006, 2, 287–303. [Google Scholar]
- Lester, F.K.; Cai, J. Can Mathematical Problem Solving Be Taught? Preliminary Answers from 30 Years of Research. In Posing and Solving Mathematical Problems; Felmer, P., Pehkonen, E., Kilpatrick, J., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 117–135. ISBN 978-3-319-28021-9. [Google Scholar]
- Santos-Trigo, M. Problem Solving in Mathematics Education. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014; pp. 496–501. ISBN 978-94-007-4978-8. [Google Scholar]
- Philipp, K. Experimentelles Denken: Theoretische und Empirische Konkretisierung einer Mathematischen Kompetenz; [Experimental Thinking: Theoretical and Empirical Concretization of a Mathematical Competence]; Springer Fachmedien Wiesbaden: Wiesbaden, Germany, 2013; ISBN 978-3-658-01119-2. [Google Scholar]
- Polya, G. How to Solve It: A New Aspect of Mathematical Method, 2nd ed.; Princeton University Press: Princeton, NJ, USA, 2004; ISBN 978-0-691-11966-3. First published 1945. [Google Scholar]
- Schoenfeld, A.H. Mathematical Problem Solving; Academic Press: London, UK, 1985; ISBN 978-012-628-870-4. [Google Scholar]
- Schoenfeld, A.H. Learning to Think Mathematically: Problem Solving, Metacognition, and Sense Making in Mathematics (Reprint). J. Educ. 2016, 196, 1–38. [Google Scholar] [CrossRef]
- Liljedahl, P.; Cai, J. Empirical research on problem solving and problem posing: A look at the state of the art. ZDM Math. Educ. 2021, 53, 723–735. [Google Scholar] [CrossRef]
- Amalina, I.K.; Vidákovich, T. Cognitive and socioeconomic factors that influence the mathematical problem-solving skills of students. Heliyon 2023, 9, e19539. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Y.; Gong, T.; Saldivia, L.E.; Cayton-Hodges, G.; Agard, C. Using process data to understand problem-solving strategies and processes for drag-and-drop items in a large-scale mathematics assessment. Large-Scale Assess. Educ. 2021, 9, 2. [Google Scholar] [CrossRef]
- Tian, J.; Braithwaite, D.W.; Siegler, R.S. Distributions of textbook problems predict student learning: Data from decimal arithmetic. J. Educ. Psychol. 2021, 113, 516–529. [Google Scholar] [CrossRef]
- Koichu, B.; Parasha, R.; Tabach, M. Who-Is-Right tasks as a means for supporting collective looking-back practices. ZDM Math. Educ. 2021, 53, 831–846. [Google Scholar] [CrossRef]
- Bruder, R.; Collet, C. Problemlösen Lernen im Mathematikunterricht: [mit Kopiervorlagen]; [Learning to solve problems in math lessons, 1st ed.]; Cornelsen Scriptor: Berlin, Germany, 2011; ISBN 978-3589230747. [Google Scholar]
- Rott, B. Mathematische Problembearbeitungsprozesse von Fünftklässlern—Entwicklung eines deskriptiven Phasenmodells. [Problem Solving Processes of Fifth Graders: Developing a Descriptive Phase Model]. J. Math.-Didakt. 2014, 35, 251–282. [Google Scholar] [CrossRef]
- Williams, J.; Wake, G. Black Boxes in Workplace Mathematics. Educ. Stud. Math. 2007, 64, 317–343. [Google Scholar] [CrossRef]
- Bissell, C. Models and «black boxes»: Mathematics as an enabling technology in the history of communications and control engineering. Evue D’histoire Sci. 2004, 57, 305–338. [Google Scholar]
- Straesser, R. Didactics of mathematics: More than mathematics and school! Zent. Didakt. Math. 2007, 39, 165–171. [Google Scholar] [CrossRef]
- Glanville, R. Inside every white box there are two black boxes trying to get out. Behav. Sci. 1982, 27, 1–11. [Google Scholar] [CrossRef]
- Just, J.; Siller, H.-S. Towards implementing Black Boxes in Mathematical Modeling an epistemological Approach Adapting models from science and science education. In Proceedings of the Twelfth Congress of the European Society for Research in Mathematics Education (CERME12), Bolzano, Italy, 2–6 February 2022; pp. 4605–4612. [Google Scholar]
- Ruebush, L.; Sulikowski, M.; North, S. A Simple Exercise Reveals the Way Students Think about Scientific Modeling. J. Coll. Sci. Teach. 2009, 38, 18. [Google Scholar]
- Koch, S.; Krell, M.; Krüger, D. Förderung von Modellkompetenz durch den Einsatz einer Blackbox. [Promotion of model competence through the use of a black box]. Erkenn. Biol. 2015, 14, 93–108. [Google Scholar]
- Greefrath, G.; Siller, H.-S. Digitale Werkzeuge, Simulationen und Mathematisches Modellieren; [Digital Tools, Simulations and Mathematical Modeling]; Springer: Wiesbaden, Germany, 2018; ISBN 978-3-658-21939-0. [Google Scholar]
- Kuckartz, U. Qualitative Text Analysis: A Systematic Approach. In Compendium for Early Career Researchers in Mathematics Education; Kaiser, G., Presmeg, N., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 181–197. ISBN 978-3-030-15635-0. [Google Scholar]
- Rott, B. Mathematisches Problemlösen: Ergebnisse Einer Empirischen Studie; Mathematical Problem Solving: Results of an Empirical Study; WTM-STEIN: Münster, Germany, 2015; ISBN 978-3-942197-67-0. [Google Scholar]
- Johnson, C.C. Handbook of Research on STEM Education; Taylor & Francis Group: Milton, UK, 2020; ISBN 978-0-367-07560-6. [Google Scholar]
- Ortiz-Laso, Z.; Diego-Mantecón, J.-M.; Lavicza, Z.; Blanco, T.F. Teacher growth in exploiting mathematics competencies through STEAM projects. ZDM Math. Educ. 2023, 55, 1283–1297. [Google Scholar] [CrossRef]



| Task | |
|---|---|
| Task 1 | Imagine that you pour 900 mL of water into the funnel all at once. Predict how much water would then flow into the measuring cylinder. Write down your estimation and provide a justification. NOTE: You have space for taking notes on the following page. We assume that, after adding 900 mL of water, _____ mL will flow into the measuring cylinder. |
| Task 2 | Let us now consider the amount of water that flows out of the water box when an arbitrary amount is poured into it. |
| Task 2.1 | First, note down which elements (connections, vessels, …) could potentially be inside the box. Compare your ideas with the suggestions on the “Explanations for task 2.1” card. Add additional elements if needed. |
| Task 2.2 | Now, consider how the interior of the water box could be constructed. Create a sketch and explain the rationale behind the structure you have chosen. Be sure to refer to your results from task 2.1! NOTE: There is space for notes on the next page. |
| Task 2.3 | Make three predictions with your model of the internal structure and check whether you can confirm them with the simulation. Prediction 1: “If 300 mL + 100 mL + 200 mL + 300 mL are added, ____ ml will flow out of the box.” Result in the simulation: _____ ml Our prediction was ☐ correct ☐ incorrect. |
| (a) | Input | Output | (b) | Input | Output |
|---|---|---|---|---|---|
| 100 mL | - | 400 mL | - | ||
| 200 mL | - | 400 mL | 400 mL | ||
| 400 mL | - | 100 mL | - | ||
| 200 mL | 450 mL | ||||
| Total | 900 mL | 450 mL | Total | 900 mL | 400 mL |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Just, J.; Siller, H.-S. Redesigning and Evaluating a Science Activity to Foster Mathematical Problem Solving. Educ. Sci. 2024, 14, 464. https://doi.org/10.3390/educsci14050464
Just J, Siller H-S. Redesigning and Evaluating a Science Activity to Foster Mathematical Problem Solving. Education Sciences. 2024; 14(5):464. https://doi.org/10.3390/educsci14050464
Chicago/Turabian StyleJust, Janina, and Hans-Stefan Siller. 2024. "Redesigning and Evaluating a Science Activity to Foster Mathematical Problem Solving" Education Sciences 14, no. 5: 464. https://doi.org/10.3390/educsci14050464
APA StyleJust, J., & Siller, H.-S. (2024). Redesigning and Evaluating a Science Activity to Foster Mathematical Problem Solving. Education Sciences, 14(5), 464. https://doi.org/10.3390/educsci14050464






