Resources and Praxeologies Involved in Teachers’ Design of an Interdisciplinary STEAM Activity
Abstract
1. Introduction
2. Theoretical Frameworks
2.1. Documentational Approach to Didactics
- Active involvement of the teacher in the reflective collection of resources.
- Observation of the teacher’s activities both in and out of the classroom.
- Collection of the resources utilized and produced by the teacher.
- Organization of follow-up observations over an extended period to discern stabilities and evolutions.
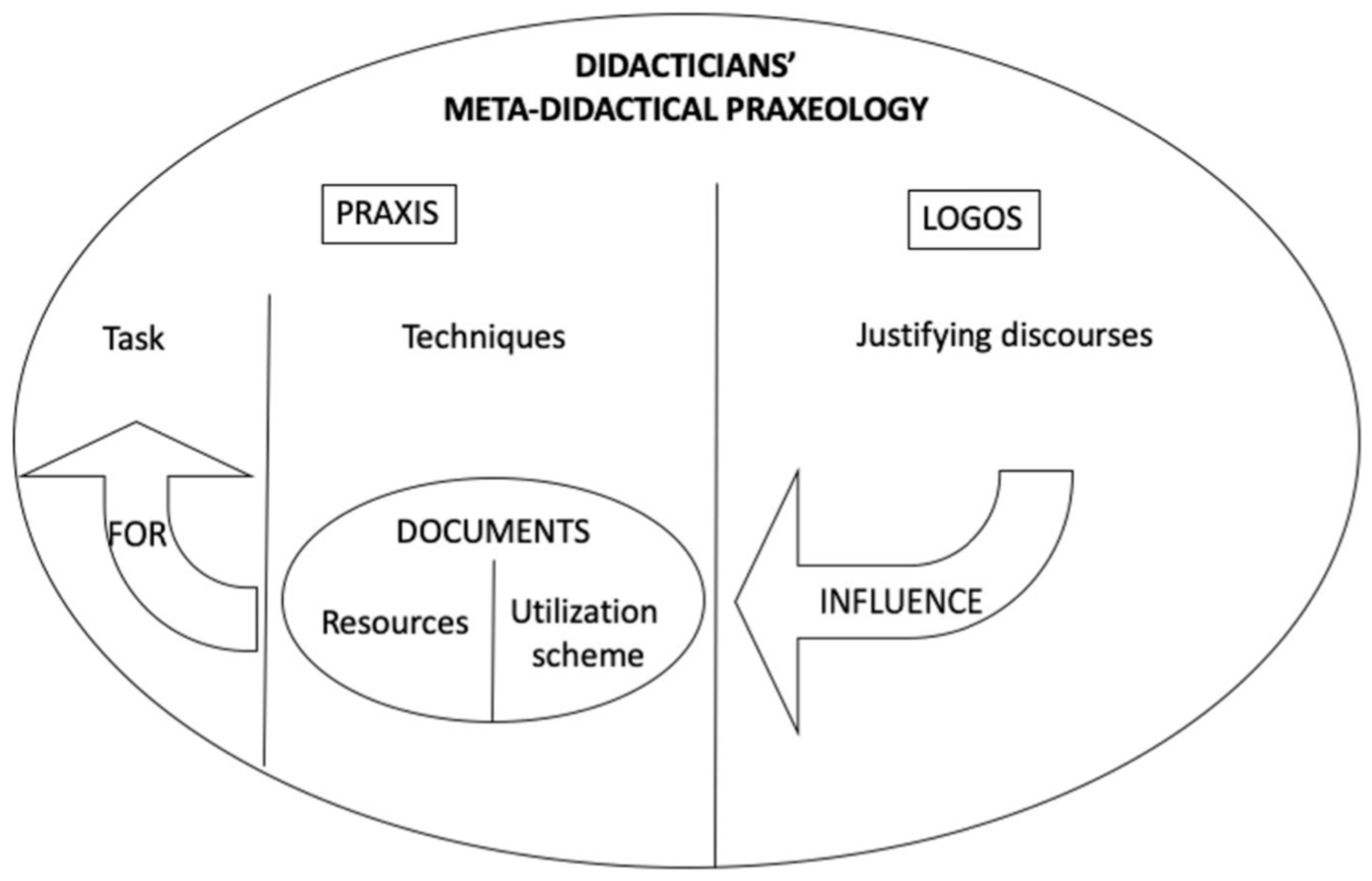
2.2. Meta-Didactical Transposition
2.3. A model Combining DAD and MDT
3. Methodology
3.1. Institutional and Experimental Context
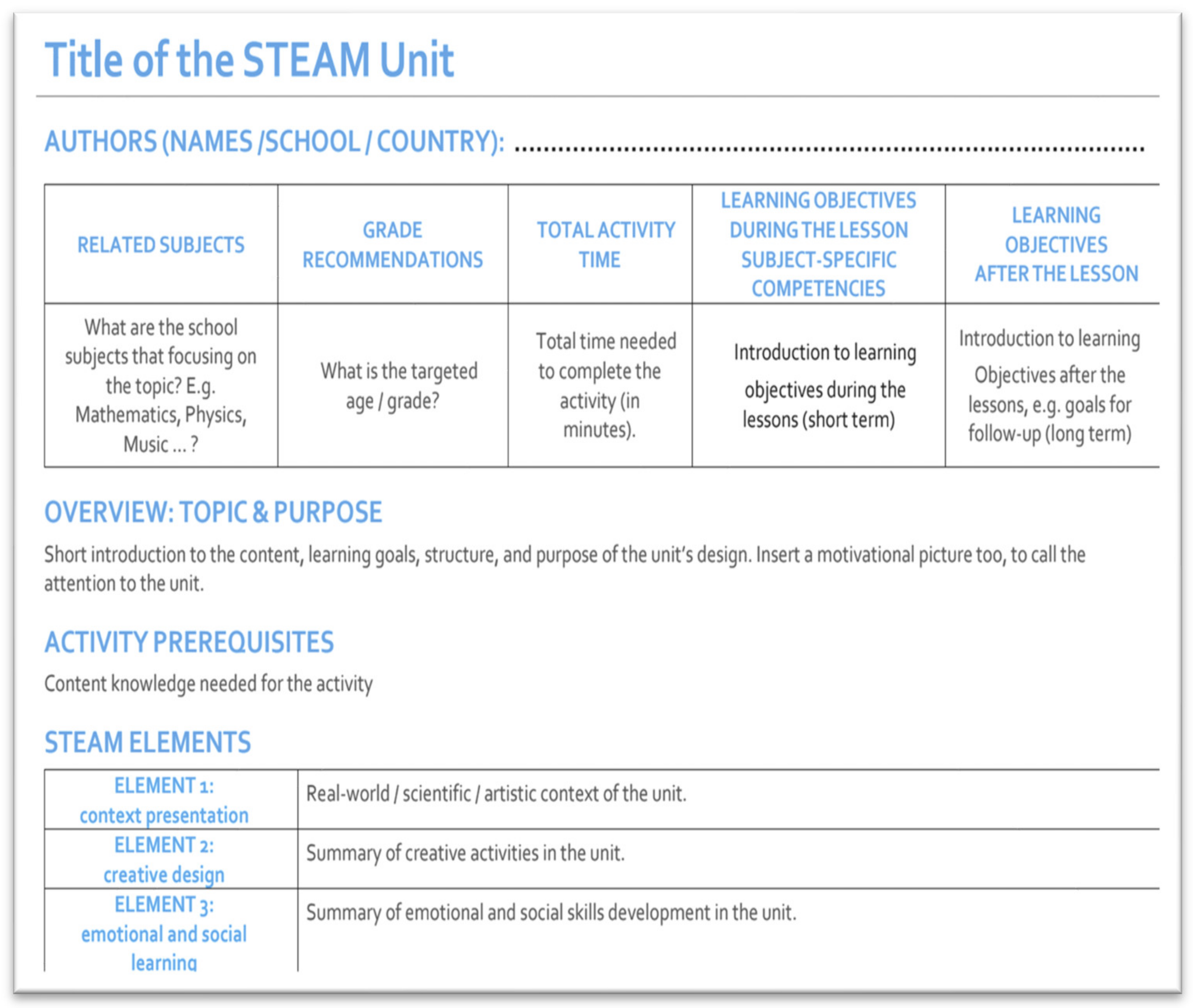
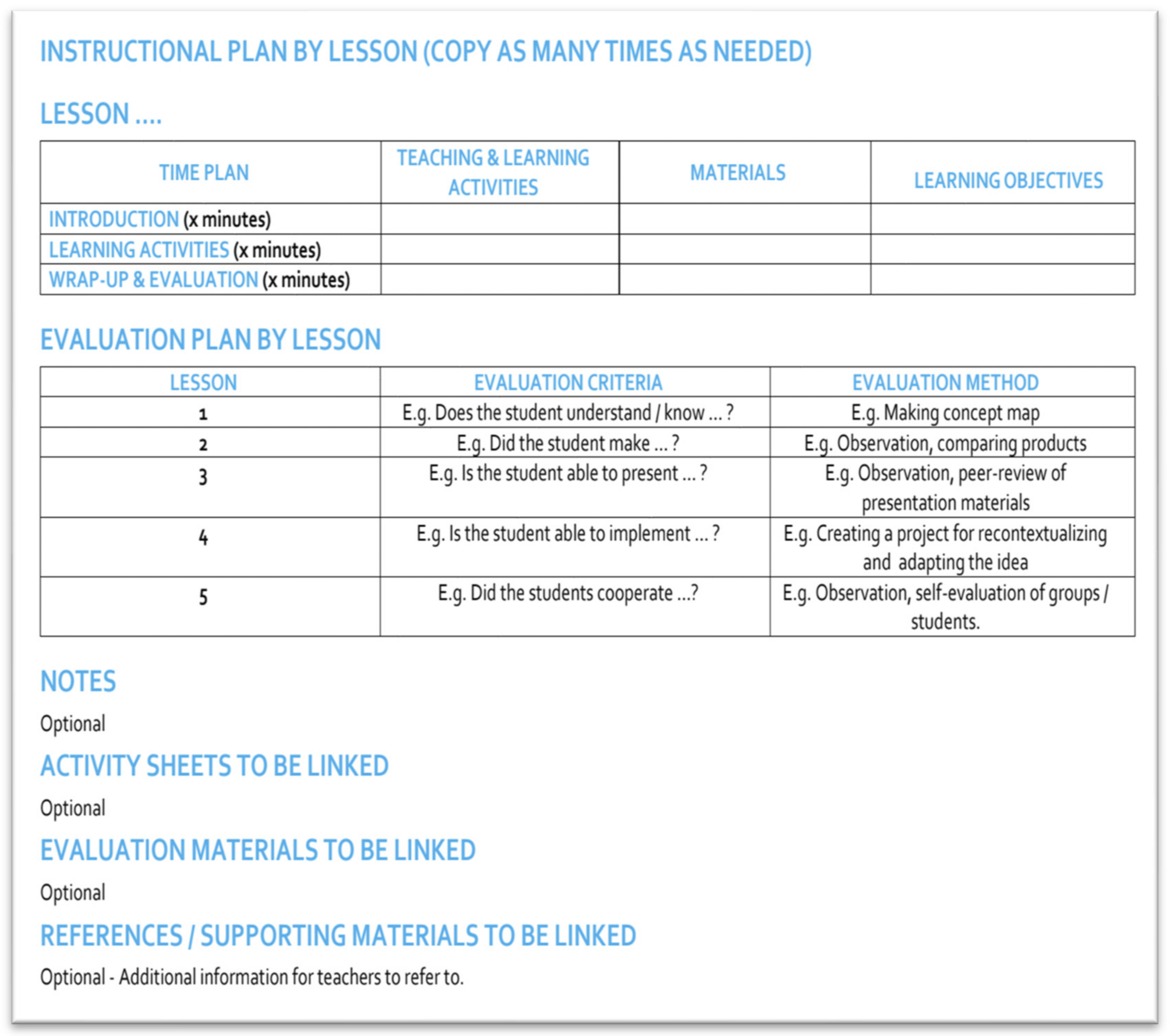
3.2. Data Collection
- -
- The activity template developed within the project (Figure 2), filled out by the teachers;
- -
- Oral interviews with the four teachers, conducted in four different sessions by the third author. The questions related to the teachers’ previous experiences and backgrounds, their relationship with teachers of different disciplines in the context of the STEAM-Connect project, the resources they rely on to design the STEAM activity in their school, and the role that they attribute to mathematics in the context of the STEAM activity;
- -
- A schematic representation of the resource system (SRRS) provided by each of the four teachers.
3.3. Data Analysis
4. Results
4.1. Overview of the STEAM Activity
4.2. Teachers’ Documentation Work
4.2.1. Art Teacher
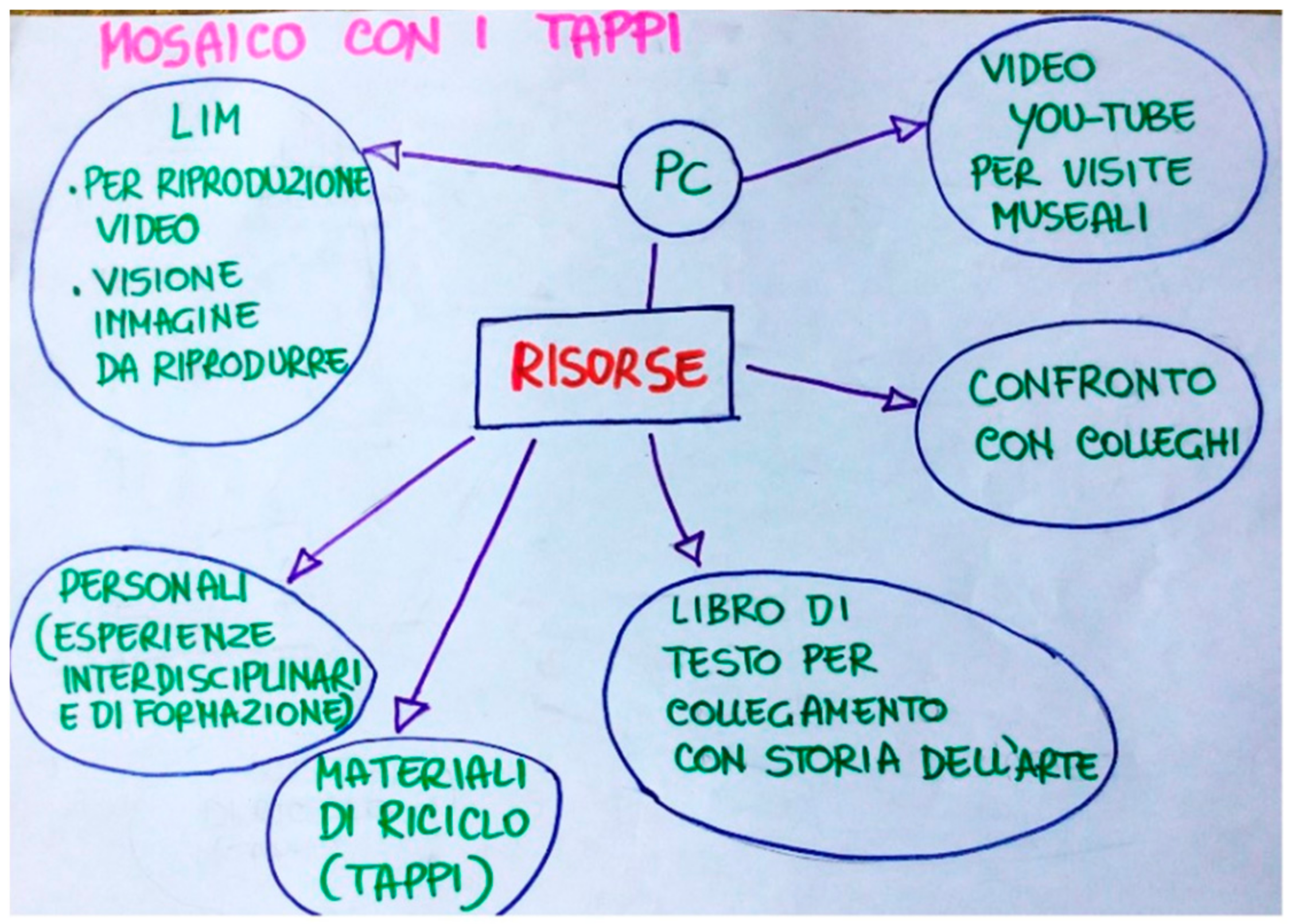
- -
- Technological resources, like the IWB for the reproduction of videos, projection of the image of the module, and projection of the YouTube video with instructions for students;
- -
- Material resources, like caps, sheets, glue, and the art textbook;
- -
- Human resources, like previous professional experiences as well as exchanges and sharing with colleagues.
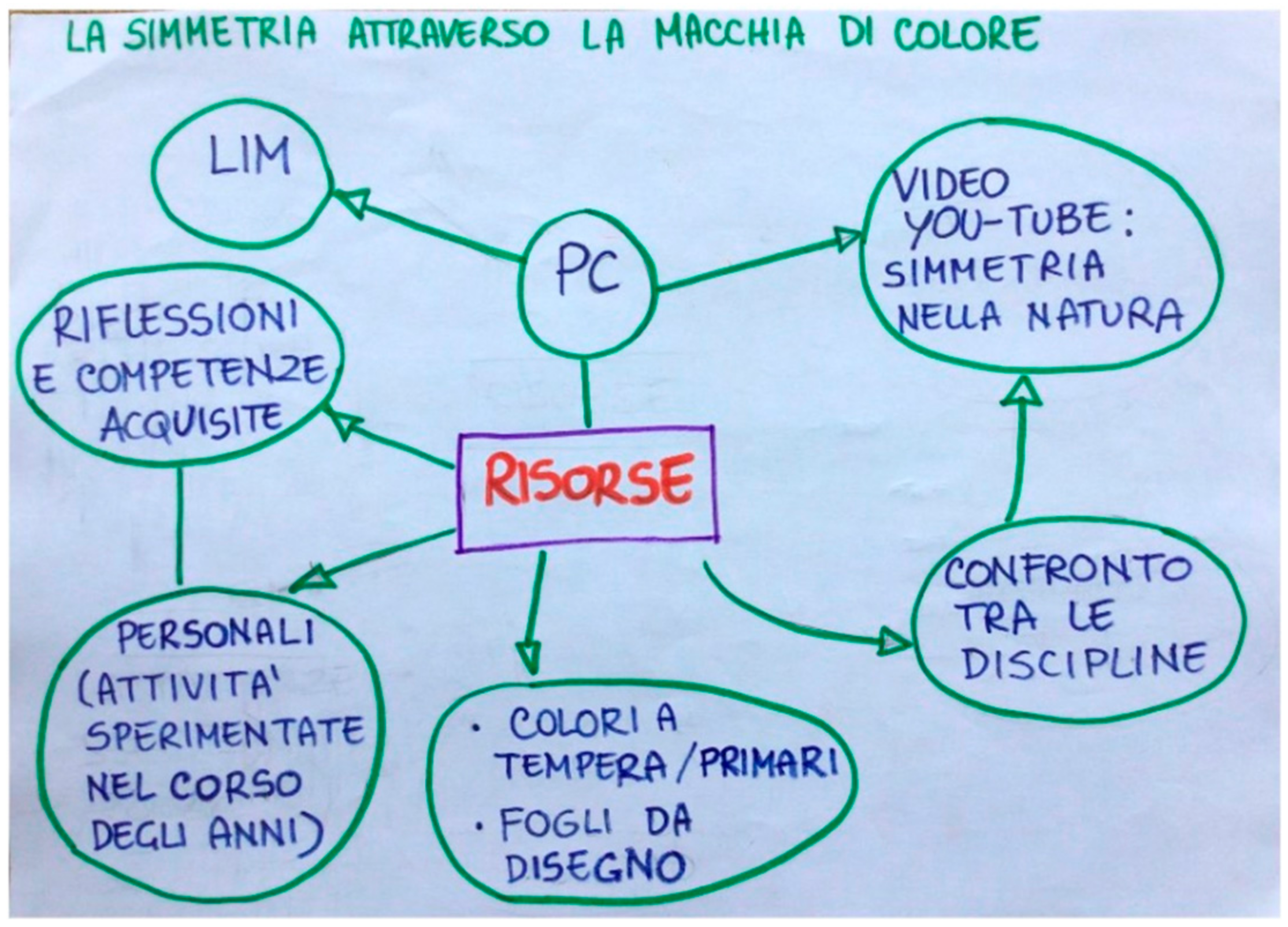
- -
- Technological resources, like the IWB and the YouTube video on symmetries in nature;
- -
- Material resources, like tempera colors and drawing sheets;
- -
- Human resources, like previous professional experiences and acquired skills as well as exchanges and sharing with colleagues.
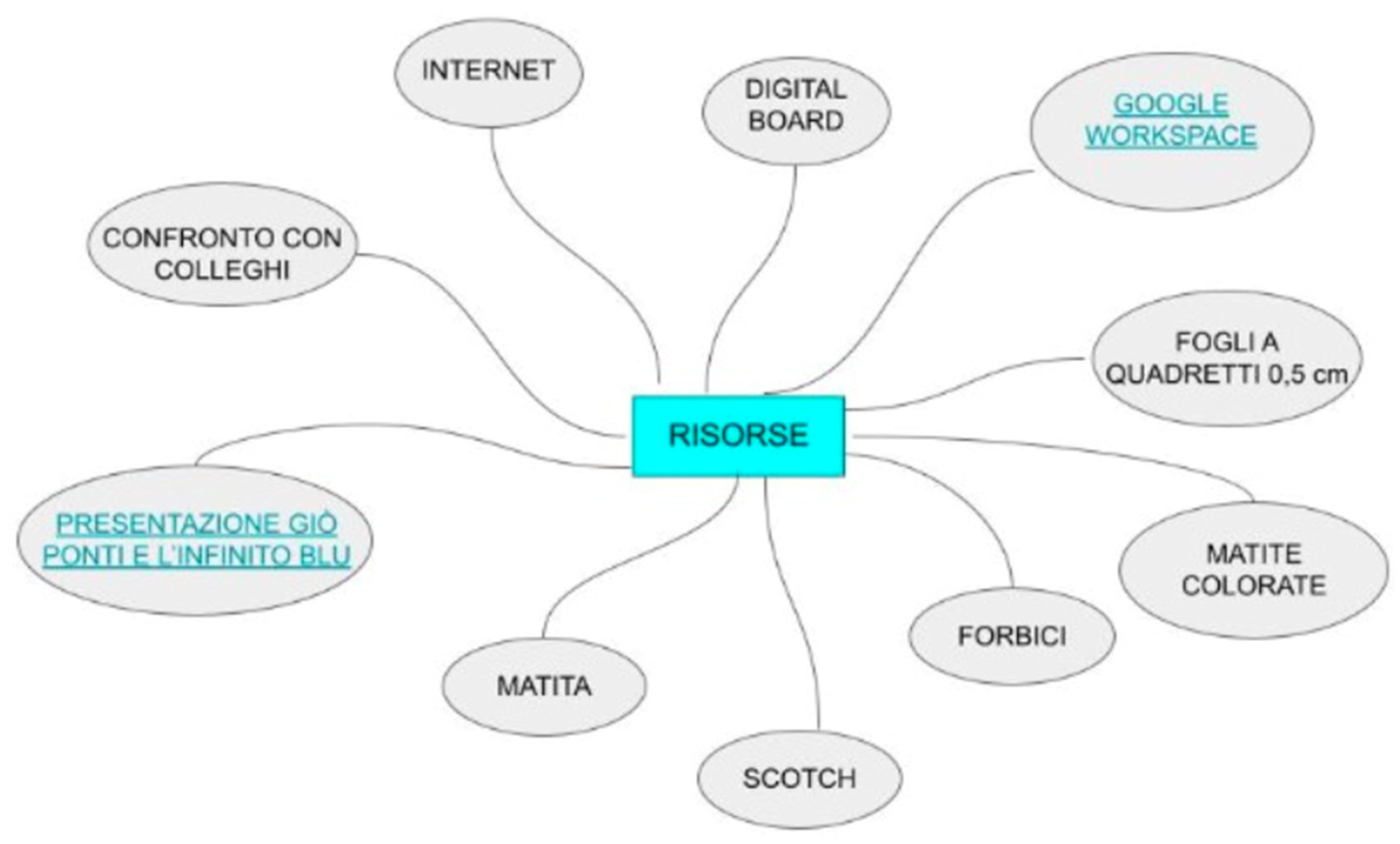
4.2.2. Technology Teacher
- -
- Technological resources, like the IWB, the Internet, Google Workspace, and a slideshow about the work of Giovanni Ponti;
- -
- Material resources, like pencils, colored pencils, scissors, adhesive tape, and graph paper;
- -
- Human resources, like exchanges and sharing with colleagues.
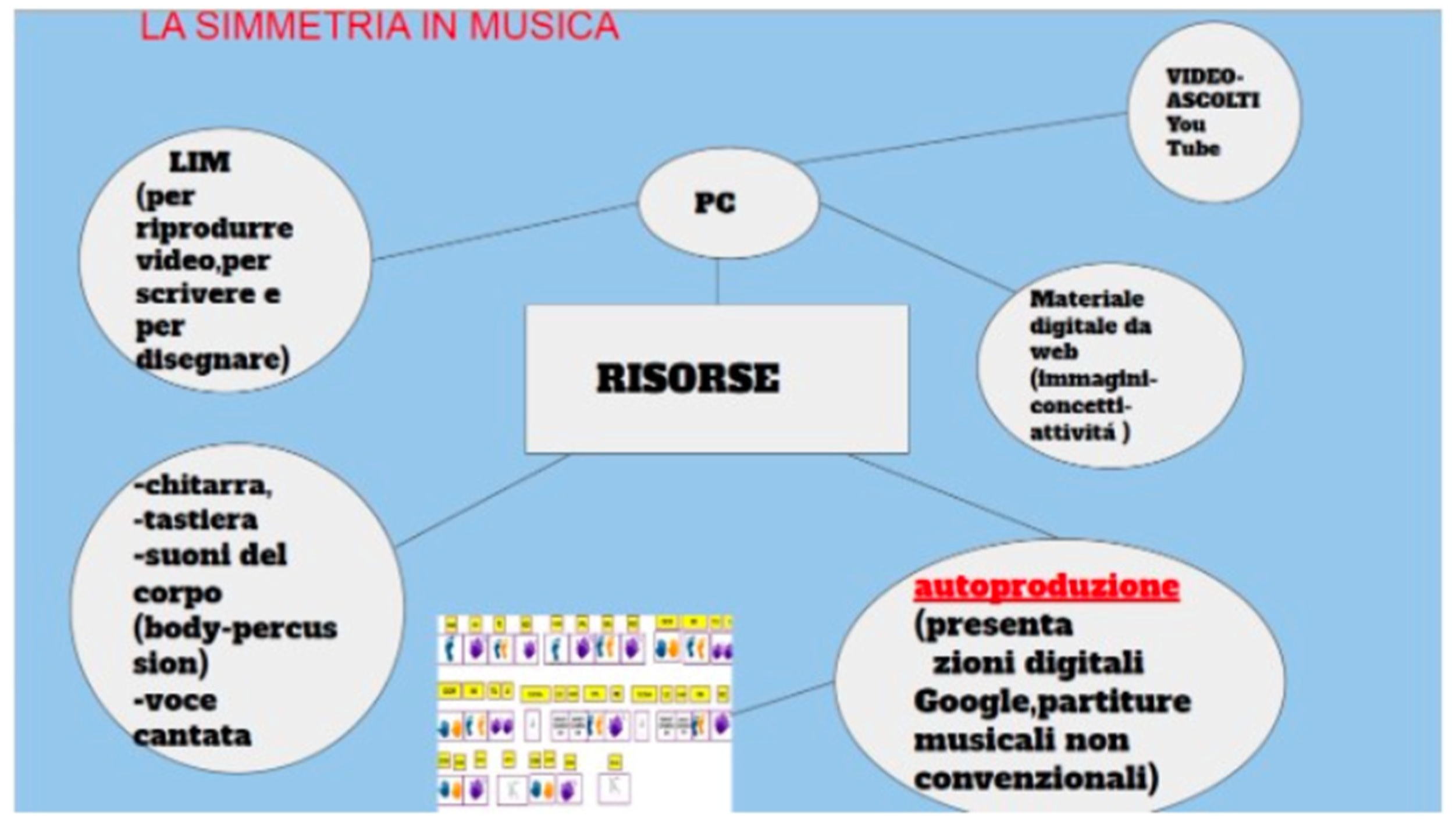
4.2.3. Music Teacher
- -
- Technological resources, like the IWB, PC, YouTube videos, slideshows about unconventional music scores, and digital materials retrieved from the Web;
- -
- Material resources, like musical instruments and pencils;
- -
- Human resources, like students’ voices.
4.2.4. Mathematics Teacher
- -
- Technological resources, like the Internet and Google Drive;
- -
- Material resources, like textbooks;
- -
- Human resources, like previous professional experiences and exchanges with colleagues.

4.3. Teachers’ Shared Meta-Didactical Praxeology
Diana: At the core of the project, there is the exploration of a path that leads students to understand that mathematics models the underlying reality. The intention is to convey the message that mathematics becomes a key to interpreting reality.
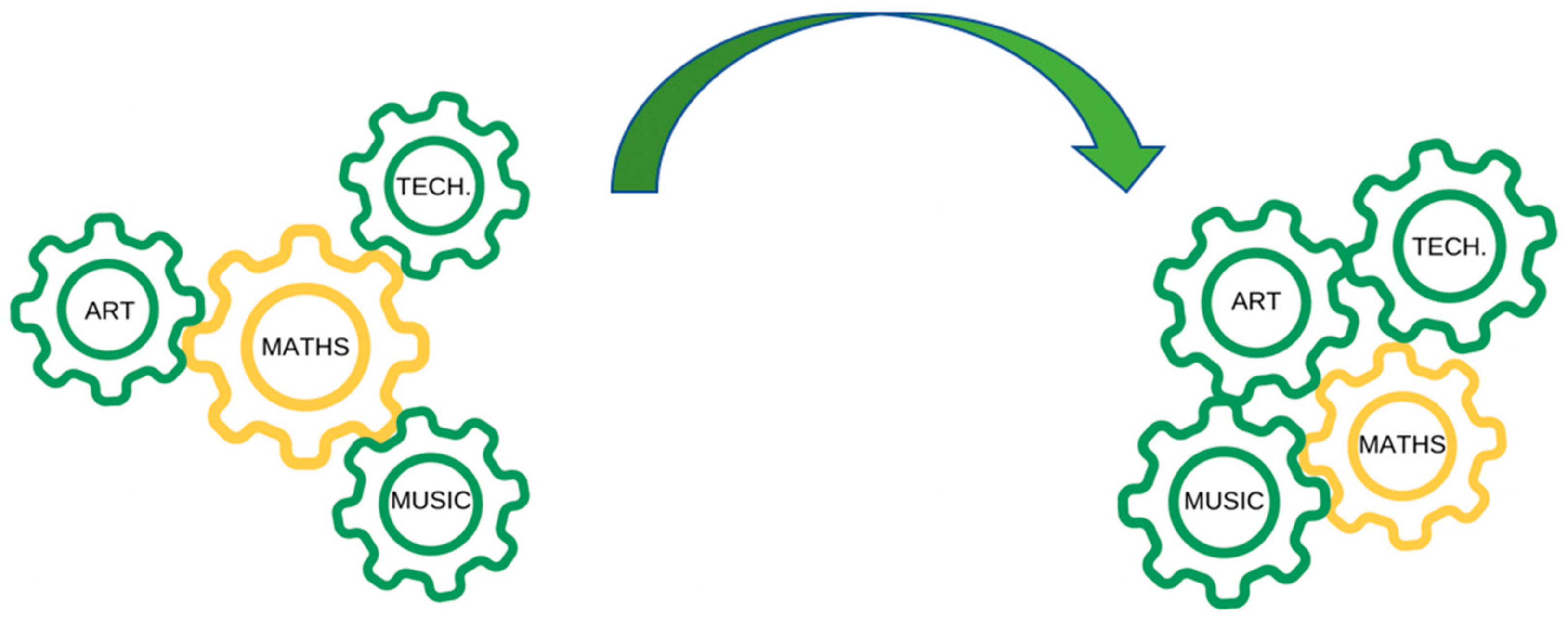
Diana: If I look around in a room or in any context, I see symmetries and translations everywhere.Diana: Mathematics serves as a unifying force in everything, it is a perspective on reality seen through mathematical eyes, but at the end of the activity.Diana: To try to describe with a metaphor the design of this activity, one can see mathematics at the center, like the largest gear of a clock, and the other disciplines as the other little gears that turn independently, not connected to each other. In the following, it happens that the central gear of mathematics shrinks, and the lateral gears enlarge, causing the other disciplines to connect with each other. In this way, the gear no longer has gaps, but it self-feeds each other.
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Henriksen, D.; Mehta, R.; Mehta, S. Design Thinking Gives STEAM to Teaching: A Framework That Breaks Disciplinary Boundaries. In STEAM Education: Theory and Practice; Khine, M.S., Areepattamannil, S., Eds.; Springer: Berlin/Heidelberg, Germany, 2019; pp. 57–78. [Google Scholar] [CrossRef]
- Maass, K.; Geiger, V.; Ariza, M.R.; Goos, M. The Role of Mathematics in interdisciplinary STEM education. ZDM Math. Educ. 2019, 51, 869–884. [Google Scholar] [CrossRef]
- Capone, R. Interdisciplinarity in Mathematics Education: From Semiotic to Educational Processes. EURASIA J. Math. Sci. Technol. Educ. 2022, 18, em2071. [Google Scholar] [CrossRef] [PubMed]
- Apostel, L.; Berger, G.; Briggs, A. (Eds.) Interdisciplinarity: Problems of Teaching and Research in Universities; OECD Publishing: Washington, DC, USA, 1972. [Google Scholar]
- Doig, B.; Williams, J.; Swanson, D.; Borromeo Ferri, R.; Drake, P. (Eds.) Interdisciplinary Mathematics Education. In ICME-13 Monographs; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Khine, M.S.; Areepattamannil, S. (Eds.) STEAM Education: Theory and Practice; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar] [CrossRef]
- Piaget, J. L’ épistémologie des relations interdisciplinaires. In Band 1 Wissenschaft als Interdisziplinäres Problem, Teil 1; Foundation Jean Piaget: Geneva, Switzerland, 2020; pp. 154–171. [Google Scholar] [CrossRef]
- Diego-Mantecon, J.M.; Prodromou, T.; Lavicza, Z.; Blanco, T.F.; Ortiz-Laso, Z. An attempt to evaluate STEAM project-based instruction from a school mathematics perspective. ZDM Math. Educ. 2021, 53, 1137–1148. [Google Scholar] [CrossRef] [PubMed]
- Williams, J.S.; Roth, W.M. Theoretical Perspectives on Interdisciplinary Mathematics Education. In Interdisciplinary Mathematics Education. ICME-13 Monographs; Doig, B., Williams, J., Swanson, D., Borromeo Ferri, R., Drake, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Williams, J.S.; Wake, G.D. Metaphors and models in translation between college and workplace mathematics. Educ. Stud. Math. 2007, 64, 345–371. [Google Scholar] [CrossRef]
- Breiner, J.M.; Harkness, S.S.; Johnson, C.C.; Koehler, C.M. What is STEM? A discussion about conceptions of STEM in education and partnerships. Sch. Sci. Math. 2012, 112, 3–11. [Google Scholar] [CrossRef]
- Wang, H.; Charoenmuang, M.; Knobloch, N.A.; Tormoehlen, R.L. Defining interdisciplinary collaboration based on high school teachers’ beliefs and practices of STEM integration using a complex designed system. Int. J. STEM Educ. 2020, 7, 3. [Google Scholar] [CrossRef]
- Pocalana, G.; Bini, G.; Robutti, O. A meta-disciplinary reflection on a STEAM school activity: The role of mathematics. In Proceedings of the 46th Conference of the International Group for the Psychology of Mathematics Education (Vol. 4), Haifa, Israel, 16–21 July 2023; Ayalon, M., Koichu, B., Leikin, R., Rubel, L., Eds.; PME: Haifa, Israel, 2023. [Google Scholar]
- Cusi, A.; Robutti, O.; Panero, M.; Taranto, E.; Aldon, G. Meta-didactical transposition.2: The evolution of a framework to analyse teachers’ collaborative work with researchers in technological settings. In Mathematics Teacher in the Digital Era, 2nd ed.; Clark-Wilson, A., Robutti, O., Eds.; Springer: Berlin/Heidelberg, Germany, 2023. [Google Scholar]
- Prediger, S.; Bikner-Ahsbahs, A.; Arzarello, F. Networking strategies and methods for connecting theoretical approaches: First steps towards a conceptual framework. ZDM Math. Educ. 2008, 40, 165–178. [Google Scholar] [CrossRef]
- Pocalana, G.; Robutti, O. Evolution of didacticians’ meta- didactical praxeologies and documentation work. Int. J. Sci. Math. Educ. 2023, 22, 211–233. [Google Scholar] [CrossRef] [PubMed]
- Gueudet, G.; Trouche, L. Towards new documentation systems for mathematics teachers? Educ. Stud. Math. 2009, 71, 199–218. [Google Scholar] [CrossRef]
- Gueudet, G.; Trouche, L. (Eds.) Resources vives. In Le Travail Documentaire des Professeurs en Mathématiques; Presses Universitaires de Rennes/INRP, Paideia: Rennes, France, 2010. [Google Scholar]
- Gueudet, G.; Trouche, L. Teachers’ work with resources: Documentational geneses and professional geneses. In From Text to ‘Lived’ Resources: Mathematics Curriculum Materials and Teacher Development; Gueudet, G., Pepin, B., Trouche, L., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 23–41. [Google Scholar] [CrossRef]
- Arzarello, F.; Robutti, O.; Sabena, C.; Cusi, A.; Garuti, R.; Malara, N.; Martignone, F. Meta-didactical transposition: A theore-tical model for teacher education programmes. In The Mathematics Teacher in the Digital Era; Clark-Wilson, A., Robutti, O., Sinclair, N., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 347–372. [Google Scholar] [CrossRef]
- Chevallard, Y. La transposition didactique du savoir savant au savoir enseigné; La Pensée Sauvage: Grenoble, France, 1985. [Google Scholar]
- Chevallard, Y. L’analyse des pratiques enseignantes en théorie anthropologique du didactique. Rech. Didact. Math. 1999, 19, 221–266. [Google Scholar]
- Aldon, G.; Arzarello, F.; Cusi, A.; Garuti, R.; Martignone, F.; Robutti, O.; Soury- Lavergne, S. The meta-didactical transposition: A model for analysing teachers education programs. In Proceedings of the 37th Conference of the International Group for the Psychology of Mathematics Education—Mathematics Learning across the Life Span, Haifa, Israel, 28 July–2 August 2013; Lindmeier, A.M., Heinz, A., Eds.; PME: Haifa, Israel, 2013; Volume 1, pp. 97–124. [Google Scholar]
- Pocalana, G.; Robutti, O. Evolution of teachers’ and researchers’ praxeologies for designing inquiry mathematics tasks: The role of teachers’ beliefs. J. Math. Teach. Educ. 2024. [Google Scholar] [CrossRef]
- Pocalana, G.; Robutti, O.; Liljedahl, P. Inquiry activities are not for everyone: Teachers’ beliefs and professional development. Int. J. Math. Educ. Sci. Technol. 2023, 54, 1557–1580. [Google Scholar] [CrossRef]
- Jaworski, B. Theory and practice in mathematics teaching development: Critical inquiry as a mode of learning in teaching. J. Math. Teach. Educ. 2006, 9, 187–211. [Google Scholar] [CrossRef]
- Pocalana, G.; Robutti, O. Mathematics teacher educators work to foster an inquiry community. In Proceedings of the 45th Conference of the International Group for the Psychology of Mathematics Education, Alicante, Spain, 18–23 July 2022; Fernández, C., Llinares, S., Gutiérrez, A., Planas, N., Eds.; PME: Haifa, Israel, 2022; Volume 3, pp. 323–330. [Google Scholar]
- Falls, Z. Beyond boundaries: Pre-service teachers’ experiences of transdisciplinary education via STEAM making projects. In Proceedings of the Society for Information Technology & Teacher Education International Conference, Las Vegas, NV, USA, 18 March 2019; Graziano, K., Ed.; Association for the Advancement of Computing in Education (AACE): Waynesville, NC, USA, 2019; pp. 1556–1562. [Google Scholar]
- Quigley, C.F.; Herro, D. An Educator’s Guide to STEAM: Engaging Students Using Real-World Problems; Teachers College Press: New York, NY, USA, 2019. [Google Scholar]
- Shashidhar, B.; Bhesh Raj, M.; Wandee, K.; Hassan, T.; Munkhjargal, G.; Adeeb, J. At the dawn of science, technology, engineer-ing, arts, and mathematics (STEAM) education: Prospects, priorities, processes, and problems. Int. J. Math. Educ. Sci. Technol. 2022, 53, 2919–2955. [Google Scholar] [CrossRef]

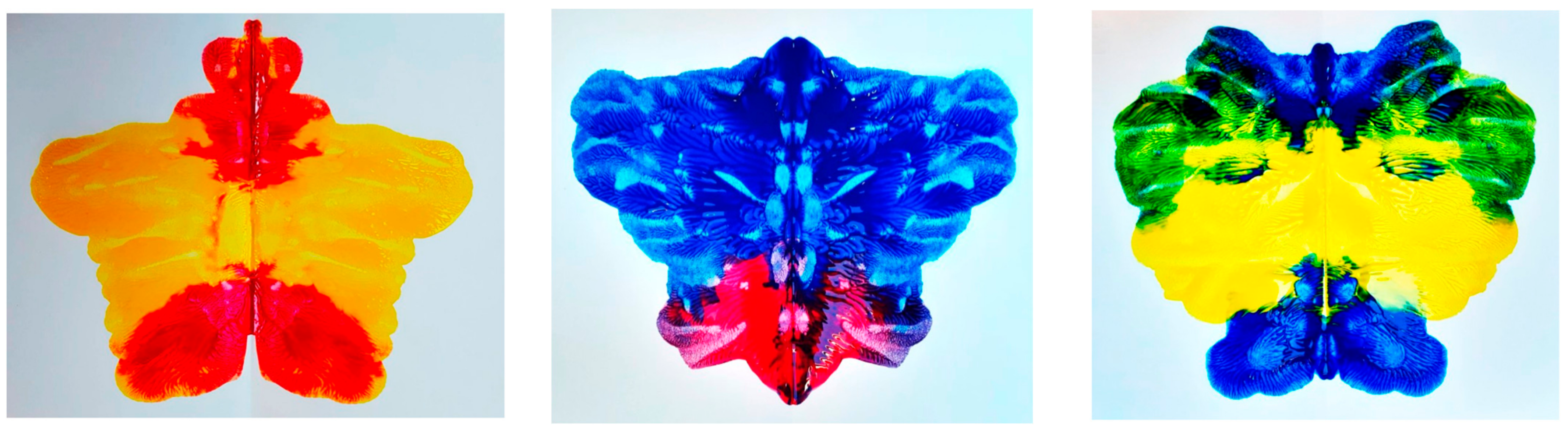





| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
| Classroom situation in which the resources are employed. | Resources utilized by the teacher. | Way in which the teacher utilizes the resources. | Guiding principles underlying the rules of action. |
| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
| Creation of a material module and modular composition. | IWB, YouTube video, and digital image projected onto the IWB. | Project onto the IWB a video with the instructions and the image of the module to reproduce. | Students are facilitated by always having a guide to follow in front of them. |
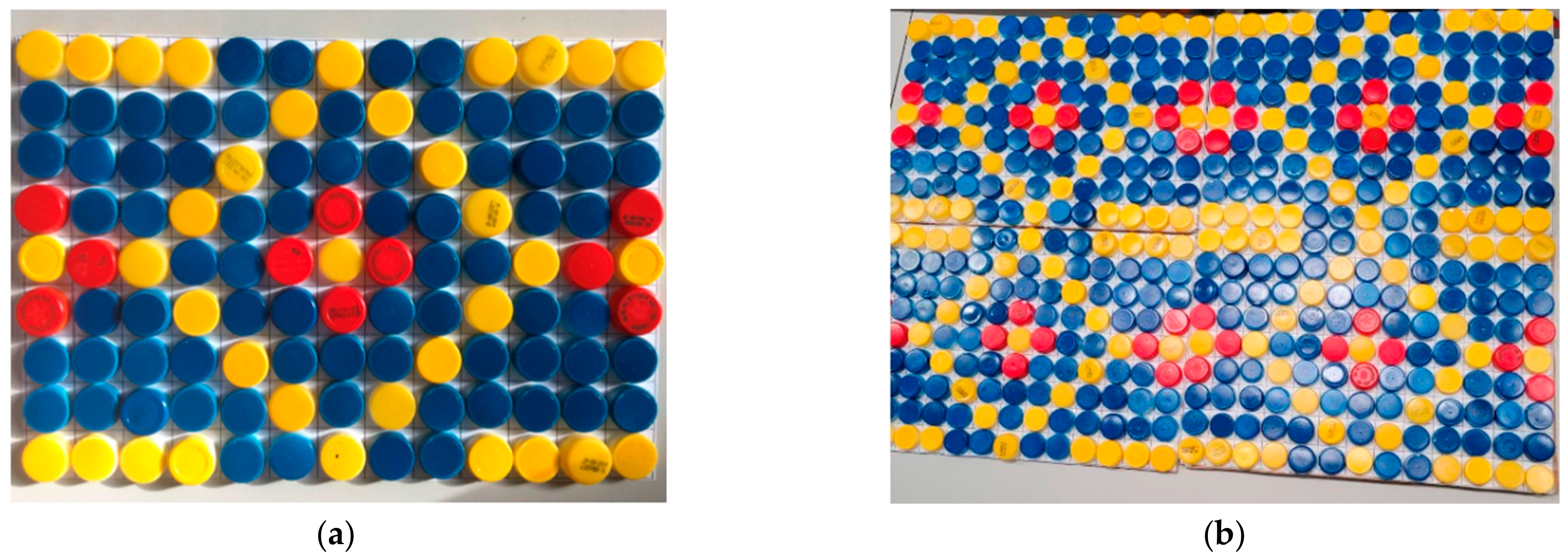
| Plastic bottle caps in three different colors, cardboard sheets, gridded sheets, and glue. | Have the students reproduce the displayed module by gluing the caps onto the grid in the right position. | With hands-on activities, students get to experience geometric shapes that they usually only see in books. | |
| Modules created by the students. | Have the students create different modular compositions by juxtaposing the modules in different ways. | Students gain insight into the characteristics of the modular compositions by noting the differences between the various arrangements. | |
| Teachers’ previous experience. | Rely on personal past experience related to activities where students have to physically create something. | Manual activities involve the students in active learning. | |
| Exchanges with colleagues. | Agree upon the design of this part of the activity with the colleagues teaching technology, music, and mathematics. | The objectives of this part of the activity must align with the broader goal of the STEAM activity in which it is integrated. |
| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
| Creation of a symmetric figure. | IWB, YouTube video. | Project onto the IWB a short video with examples of symmetry in natural objects. | Viewing images related to symmetries found in nature helps students grasp the concept. |
| Tempera colors, drawing sheets. | Have the students fold the sheet in half, choose two colors, place two drops of paint along the fold line of the sheet, fold the sheet, and press to spread the colors. | By folding the sheet and spreading the colors, students can clearly recognize the axis of symmetry of the figure they are creating. | |
| Teachers’ previous experience. | Rely on personal past experience related to activities where students have to physically create something. | Manual activities involve the students in active learning. | |
| Exchanges with colleagues. | Agree upon the design of this part of the activity with the colleagues teaching technology, music, and mathematics. | The objectives of this part of the activity must align with the broader goal of the STEAM activity in which it is integrated. |
| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
| Creation of tiles and compositions with geometric patterns. | IWB, the Internet, Google Workspace, and a slideshow about the tiles designed by Giovanni Ponti. | Show the students the tiles designed by Giovanni Ponti that they will have to reproduce and the different kinds of compositions made with these tiles. | The students need to get a general idea of what they will have to create. |
| Pencils, colored pencils, scissors, rulers, adhesive tape, and graph paper. | Have the students reproduce four identical copies of one of the tiles seen, coloring all four of them in the same way. | Manual activities involve the students in active learning. | |
| Tiles created by the students. | Have the students create different compositions by juxtaposing the four tiles in different ways. | Students gain insight into the characteristics of the different compositions by noting the differences between them. | |
| Exchanges with colleagues. | Agree upon the design of this part of the activity with the colleagues teaching technology, music, and mathematics. | The objectives of this part of the activity must align with the broader goal of the STEAM activity in which it is integrated. |
| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
| Performance of a canon song. | IWB, digital images. | Project symmetric and translated images on the IWB. | It is important to begin by recalling what the students have learned in the previous parts of the activity. |
| Keyboards, pencils. | Distribute a keyboard to each group of students. Ask them to identify any keys that could serve as axes of symmetry, then place a pencil on them. | It is important for students to concretely visualize the axis of symmetry and be able to identify it in new situations. | |
| Students’ voices and “Fra’ Martino” canon score. | Have students sing the canon of “Fra’ Martino” in four voices. | Students need to perceive in music the modular structure they have already encountered in art and technology. | |
| Exchanges with colleagues. | Agree upon the design of this part of the activity with the colleagues teaching technology, music, and mathematics. | The objectives of this part of the activity must align with the broader goal of the STEAM activity in which it is integrated. |
| Situation | Resources | Rules of Action | Operational Invariants |
|---|---|---|---|
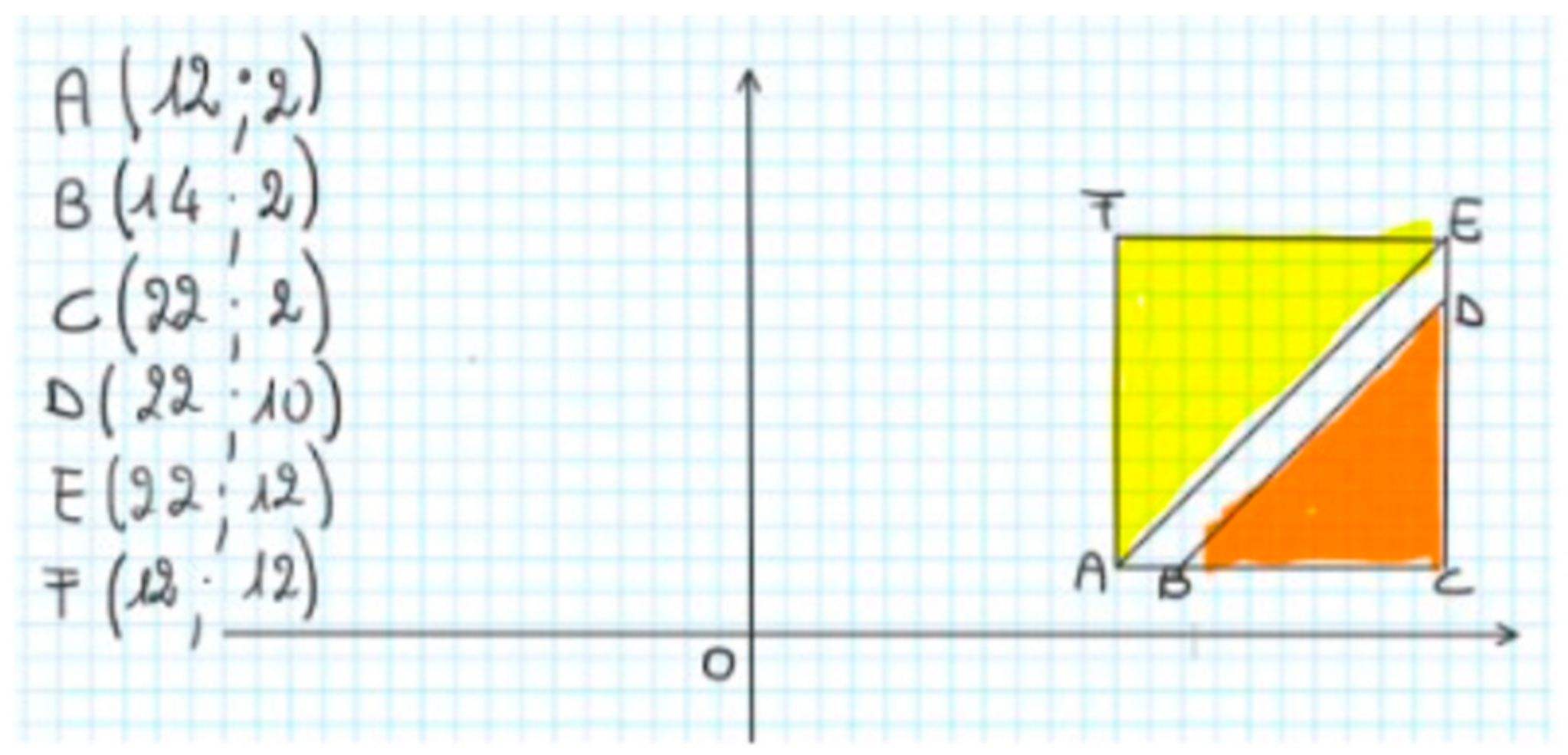
| Formally express mathematically the concepts of axial symmetry and translations using the Cartesian plane. | IWB, pencils, notebooks. | Have students construct symmetric and translated images on the Cartesian plane following what is being constructed on the IWB. | A certain degree of mathematical formalization is necessary to consolidate what has been seen in other disciplines. |
| Artifacts created in previous parts of the activity. | Encourage connections between mathematical formalization and the artifacts created in the previous parts of the activity. | It is important for students to see the connection between mathematics and other disciplines. | |
| Students’ questions and comments. | Promote and value students’ questions and comments. | The questions and comments from students are important for the entire class and for the teacher herself, who can leverage the doubts and beliefs that arise. | |
| Teachers’ previous experience. | Rely on personal past experiences related to interdisciplinary activities. | The teacher is advantaged by having already experienced interdisciplinary activities on similar topics and is aware of what can motivate or hinder students. | |
| Exchanges with colleagues | Agree upon the design of this part of the activity with the colleagues teaching technology, music, and mathematics. | The objectives of this part of the activity must align with the broader goal of the STEAM activity in which it is integrated. |
| Teachers’ Shared Meta-Didactical Praxeology | |
|---|---|
| Praxis | Logos |
| Task: Design of a STEAM activity for lower-secondary students. Techniques: Propose, in the early stages, embodied experiences (like singing) and hands-on activities where students create tangible artifacts. Leave the mathematical formalization of the topic at the end of the activity to interpret what has been done before. |
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pocalana, G.; Robutti, O.; Ciartano, E. Resources and Praxeologies Involved in Teachers’ Design of an Interdisciplinary STEAM Activity. Educ. Sci. 2024, 14, 333. https://doi.org/10.3390/educsci14030333
Pocalana G, Robutti O, Ciartano E. Resources and Praxeologies Involved in Teachers’ Design of an Interdisciplinary STEAM Activity. Education Sciences. 2024; 14(3):333. https://doi.org/10.3390/educsci14030333
Chicago/Turabian StylePocalana, Gabriella, Ornella Robutti, and Elena Ciartano. 2024. "Resources and Praxeologies Involved in Teachers’ Design of an Interdisciplinary STEAM Activity" Education Sciences 14, no. 3: 333. https://doi.org/10.3390/educsci14030333
APA StylePocalana, G., Robutti, O., & Ciartano, E. (2024). Resources and Praxeologies Involved in Teachers’ Design of an Interdisciplinary STEAM Activity. Education Sciences, 14(3), 333. https://doi.org/10.3390/educsci14030333






