Explorative Study of Developing a Mathematical Model for Evaluating HOTS in the Mathematics Curriculum Operating in the KZN TVET Colleges

Abstract

This study developed a mathematical evaluation model, attempting to evaluate the capability of the curriculum (N1 to N2 mathematics) to equip students with higher-order thinking skills (HOTS) in the Technical and Vocational Education and Training (TVET) Colleges. The data set was collected at eMnambithi TVET College during March 2022 and October 2022. The two most crucial elements contributing to students attaining HOTS during teaching and learning are the lecturer’s content delivery ability and the curriculum. In that regard, this study began by partially evaluating the lecturer’s content delivery ability using students’ perspectives through a questionnaire. It was found that N1 and N2 mathematics lecturers’ content delivery abilities from the perspective of the students might be adequate. That left the curriculum as the only major contributing factor in a case where students were found to have poor HOTS at eMnambithi TVET College. Then, the SVHIR model (susceptible S(t), vaccinated V(t), healthy H(t), infected I(t), and recovered R(t)) was successfully developed to attempt the evaluation of students’ HOTS. The model indicated poor HOTS in students at eMnambithi TVET College. That ultimately meant that the curriculum might be incapable of equipping students with HOTS, since the lecturers’ content delivery abilities were deemed to be adequate by the participants of this study.

1. Introduction

In the 1980s to early 1990s in South Africa, the Technical and Vocational Education and Training (TVET) national accredited technical education diploma (NATED) programs (in Report 191) were combined with apprenticeships through the Manpower Training Act (No. 56 of 1981) [1]. The programs were offered as pre-grade 12 (N1–N3) and post-grade 12 (N4–N6) programs; for the formal school system, grade 12 is the exit grade in South Africa. In N1 to N6, the programs in engineering were offered on a trimester basis and the business side programs were offered on a semester basis. As the old government’s system was coming to an end in South Africa, the system of apprenticeship was also declining. The programs started to open for the pre-employed students instead of only students in apprenticeships [2]. Then, beginning in the year 2007, the public South African TVET Colleges have offered numerous NATED programs (N1 to N6) to all the students (employed and pre-employed). The programs of engineering prepare artisans for disciplines such as metalwork, electricity, construction, and mechanics. However, at the end of these programs, students still need to undergo a trade-test preparation and testing before they become entry-level artisans.

Currently in South Africa, a student who acquires N2 TVET training is permitted to undergo trade-test preparation and testing to become an entry-level artisan. This presumes that the N1 and N2 curriculum is sufficient to equip students with the necessary thinking skills required by the industry or real-world problems space. Among those required thinking skills is the higher-order thinking skills (HOTS). HOTS are defined to be the three highest thinking levels of cognitive skills that includes analyzing, evaluating, and creating [3,4]. Students with HOTS are not just remembering the formulas, they understand them and then apply them to problems. The student can analyze (decompose the problem into several parts, then determine the parts that relate to each other and the whole), evaluate (assess which includes checking and criticizing), and create (making something new from the existing). According to Pratama and Retnawati [5], students with HOTS will tend to use logic rather than simply memorize formulas; hence, they will master the concepts and can solve more complex mathematics problems. Some scholars state that students’ confidence level in mathematics increases as higher-order thinking skills increase [6]. This is because the three skills that defines HOTS are the same skills required for mathematics. In that regard, mathematics is one of the contributing subjects/modules to the improvement of HOTS.

Therefore, the current study aimed to find an optimal mathematical model which could be used to evaluate the capability of the curriculum (N1 to N2 mathematics) to equip students with higher-order thinking skills (HOTS) in the TVET Colleges. In that regard, we will discover how efficient the SIR model is (susceptible (S), infected (I), and recovered (R)) in evaluating the curriculum. This will be achieved by applying the SIR model and assuming that low-ability students can affect negatively the HOTS students directly or indirectly. However, we are not nullifying that the opposite case is also true—where HOTS students can impact low-ability students positively. According to Budsankom et al. [7], elements of the classroom environment affecting HOTS are classroom climate, teaching and learning methods, and teacher’s behavior. Since low-ability students are a part of a class, they also contribute in the classroom climate. In the same way, they have an impact in the teaching and learning methods operating in the classroom, given the teaching approach to be used for HOTS and low-ability students is different. For instance, if a large portion of the class are low-ability students, the classroom teaching and learning methods will accommodate mostly the low-ability students, which ultimately affects the HOTS students. However, the study is not suggesting that HOTS students must disengage themselves from low-ability students; rather, we suggest students and teachers must be aware of this possible case to avoid it.

2. Methodology

As the topic of HOTS has become more dominant in teaching and learning, an effective valid and efficient learning model to enhance students’ HOTS has become a necessity. Hence, there are several scholars who have adopted a modeling approach into the HOTS evaluation concept. In Indonesia, two scholars conducted research to develop a HOTS learning model [8]. Their research was a developmental study using Richey and Klein methods of a Type II Model conducted in two phases. The results showed that the developed model passed the validity, effectiveness, and efficiency criteria. The application of the model showed that students’ HOTS and electronics competences can be improved. The other scholars Husamah et al. [9] proposed orientation, identify, discussion, decision, and engage (OIDDE) as a model that can stimulate HOTS in students. They suggested that this can be achieved by assessing three aspects, namely critical thinking, self-regulated thinking, and creative thinking. Their study adopted a classroom action research (CAR) approach conducted in two cycles. The study was conducted on 45 biology education students during their fifth semester. The findings confirmed that OIDDE model improved the following three attributes of HOTS to a very good level: critical thinking (81%), self-regulated thinking (82%), and creative thinking (80%). However, the study suggests that the implementation of the model should be conducted in other subjects/courses during different semesters to support the conclusion that the OIDDE model is applicable. In Malaysia, a particular study focused on examining the effectiveness of the bar model method to understand and answer HOTS questions for primary school learners [10]. They collected their data from 35 learners from Year 6 dual language program (DLP) class at SK Bukit Bandaraya. The findings were analyzed by the statistical package for the social science (SPSS) version 22, and they were based on the pre- and post-test result from the learners. The results revealed that the bar model method showed HOTS effectiveness in those students. They found that with bar model kits, learners pay more attention to the subject discussed. However, in earlier stages, learners had challenges in estimating the size of the bar in accordance with its value but later they were able to estimate the value. This allows engineers and operators to understand and predict how a system will behave under different conditions.

The approach of involving models in higher-order thinking skills has been around for more than two decades [11]. Hence, one would expect good research progress in this area during that period. However, according to the literature reviewed for the current study, such an approach has not received much attention from researchers [8, 9 and 10]. Nevertheless, there is an unpredictable trend where now and again there will be those few researchers showing interest in the approach [12]. This infers that there is more room for scholars to explore their new skills and ideas in this approach, especially mathematics. In the current study, we are of the view that mathematical modeling enables a systematic understanding of the system modeled; hence, we are taking a mathematical modeling approach to evaluate HOTS. Therefore, this section details the approach of the current work towards the evaluation of HOTS in the TVET colleges’ N1 to N2 mathematics curriculum.

2.1. Formulation of the Data Collection Instrument

The data collection instrument was developed by the applying the work of Brookhart [3] and Maharaj and Wagh [13]. Also, some researchers indicate that educational evaluation should at least involve two appraisals, since one cannot draw a conclusion by only assessing students at the end of the program without knowing where they were at beginning of the program [14,15,16]. Hence, the formulated data collection instruments are two similar tests in Appendix A and Appendix B, which consist of the five questions based on the following five HOTS components, respectively [3,13]:

➢

Working systematically through cases in an exhaustive way;

➢

Interpret and extend solutions of problems;

➢

Identifying possible applications of mathematics in the surroundings;

➢

Translate a worded or graphically represented situation to relevant mathematical formalisms;

➢

Use with reasonable skill the available tools for mathematical exploration.

The HOTS questions in the instruments were selected from the curriculum under investigation. However, one may be concerned about using existing knowledge to evaluate HOTS. According to Maharaj and Wagh [13], the three abilities (transfer, critical thinking, and problem solving) are identified as generic to mathematics. They then used those generic abilities to document the expected outcomes for HOTS in the context of first-year students. Also, the current work adopted their idea in the context of a TVET college. Considering that in South Africa the standard of a TVET college education is far lower than of the standard at a university, we focused on applications of the existing knowledge to suit a TVET college standard and chose problems similar to those indicated in the relevant curriculum document. Therefore, we did not forget the components of HOTS when looking at the questions that such students are exposed to in the curriculum. For instance, one of the components of HOTS is creativity which is under critical thinking in the three abilities [13]. Therefore, even though the questions used are from existing knowledge, we assessed the presentation of the solutions by students, whether they had a creativity element or not. Given that there is a common way of answering particular problems but that a creative person will present a different approach to the solution of a known problem, we identify students with HOTS using existing questions in that way.

According to some scholars, there are many variables that can contribute to students attaining HOTS but the most critical are lecturers’ content delivery ability and the curriculum [17,18,19,20,21]. Therefore, prior to curriculum evaluation, the current study is also concerned about the state of the lecturers’ content delivery ability variable. Therefore, students’ responses in Question 6 of Appendix B form a partial evaluation of the lecturers’ content delivery ability. Hence, based on the students’ perspective, the current study will build up an assumption about the N1 and N2 lecturers’ content delivery ability at eMnambithi TVET College.

2.2. Evaluation Model Development

This sub-section focuses on the development of the new mathematical model that can be used to evaluate the capability of the TVET curriculum to equip students with HOTS. Hence, it is structured as a review of the SIR (susceptible, infected, and recovered) model; SVHIR model development for the current study, determination of the new model’s parameters; validation of the SVHIR model; derivation of the basic reproduction ratio, association of the actual data with the SVHIR model; and the SVHIR model application instructions.

2.2.1. Review of the SIR Model

A major assumption of many mathematical models of pandemics is that the population can be divided into a set of distinct compartments. These compartments are defined with respect to disease status. The simplest model is the SIR model described by Kermack and McKendrick [22], which consists of the three compartments which are susceptible (S), infected (I), and recovered (R), where β is the transmission rate and γ is the recovery rate as shown in Figure 1.

Susceptible: Individuals that were never infected, and they can catch the disease. Once they have it, they move into the infected compartment.

Infected: Individuals that can spread the disease to susceptible individuals. The time they spend in the infected compartment is the infectious period, after which they enter the recovered compartment.

Recovered: Individuals in the recovered compartment are assumed to be immune for life.

Note that this study only considers the SIR model without demography, which means the model excludes the births and deaths of the population’s individuals. Hence, the three compartments form a closed system. That simply means the total size (N) of the population does not change and is given as follows [23]:

$$N=S+I+R$$

(1)

The SIR model is easily written using ordinary differential equations (ODEs), which implies a deterministic model (no randomness is involved, the same starting conditions give the same output). Analogous to the principles of reaction kinetics, the model assumes that encounters between infected and susceptible individuals occur at a rate proportional to their respective numbers in the population. The SIR model is given as follows [22]:

$$\left.\begin{array}{c}\frac{dS}{dt}=-\beta SI \\ \frac{dI}{dt}=\beta SI-\gamma I\\ \frac{dR}{dt}=\gamma I \end{array}\right\}$$

(2)

The SIR model possesses the following quantities, parameters, and rates of change [22,24,25]:

• S(t): number of susceptible individuals;
• S′(t): rate of change in S;
• I(t): number of infected individuals;
• I′(t): rate of change in I;
• R(t): number of recovered individuals;
• R′(t): rate of change in R;
• β: disease transmission rate;
• γ: recovery rate.

The SIR model also has the following assumptions about the nature of the disease [22,24,25]:

• The duration of infection is the same for everyone;
• Once recovered, you are immune, and can no longer infect anyone;
• Only a fraction of contacts with the disease cause infection;
• The units of S, I, and R are persons;
• The units of time are days;
• The units of S′, I′, and R′ are persons per day, written person/day.

One of the most vital parameters in epidemiology is the basic reproductive ratio ($R_0$). It is defined as the average number of secondary cases transmitted by a single infected individual that is placed into a fully susceptible population. If $R_0>1$, there will be a pandemic, or the pandemic will continue. If $R_0<1$, the introduced infected people will recover (or die). Ultimately, there will be no pandemic, or the pandemic will stop. The basic reproductive ratio is given as in [23,24]:

$$R_0=\frac{\beta }{\gamma }$$

(3)

The concept of the SIR model has been a center of attention for many scholars to model pandemic diseases. In 2021, a study using the SIR model determined the dynamics of tuberculosis (TB) in Turkey as to how much it will affect the future and the impact of vaccine therapy on the disease. The obtained results revealed that the basic reproduction ratio for the model is less than 1. Also, recently some went as far as to improve the SIR model to best suit the situation of the COVID-19 outbreak. For instance, Diagne et al. [25] recently extended the concept of the SIR model by adding another four compartments. They divided the total population N(t) into several epidemiological states, depending on individuals’ health status as follows: susceptible S(t), vaccinated V(t), exposed E(t), symptomatic infected I(t), infected asymptomatic A(t), hospitalized H(t), and recovered R(t) individuals. On the other hand, Xinxin Zhang [26] analyzes the risk contagion in the banking system using a variation of the SIR model. They found that the bank risk contagion needs to be considered both partially and systemically.

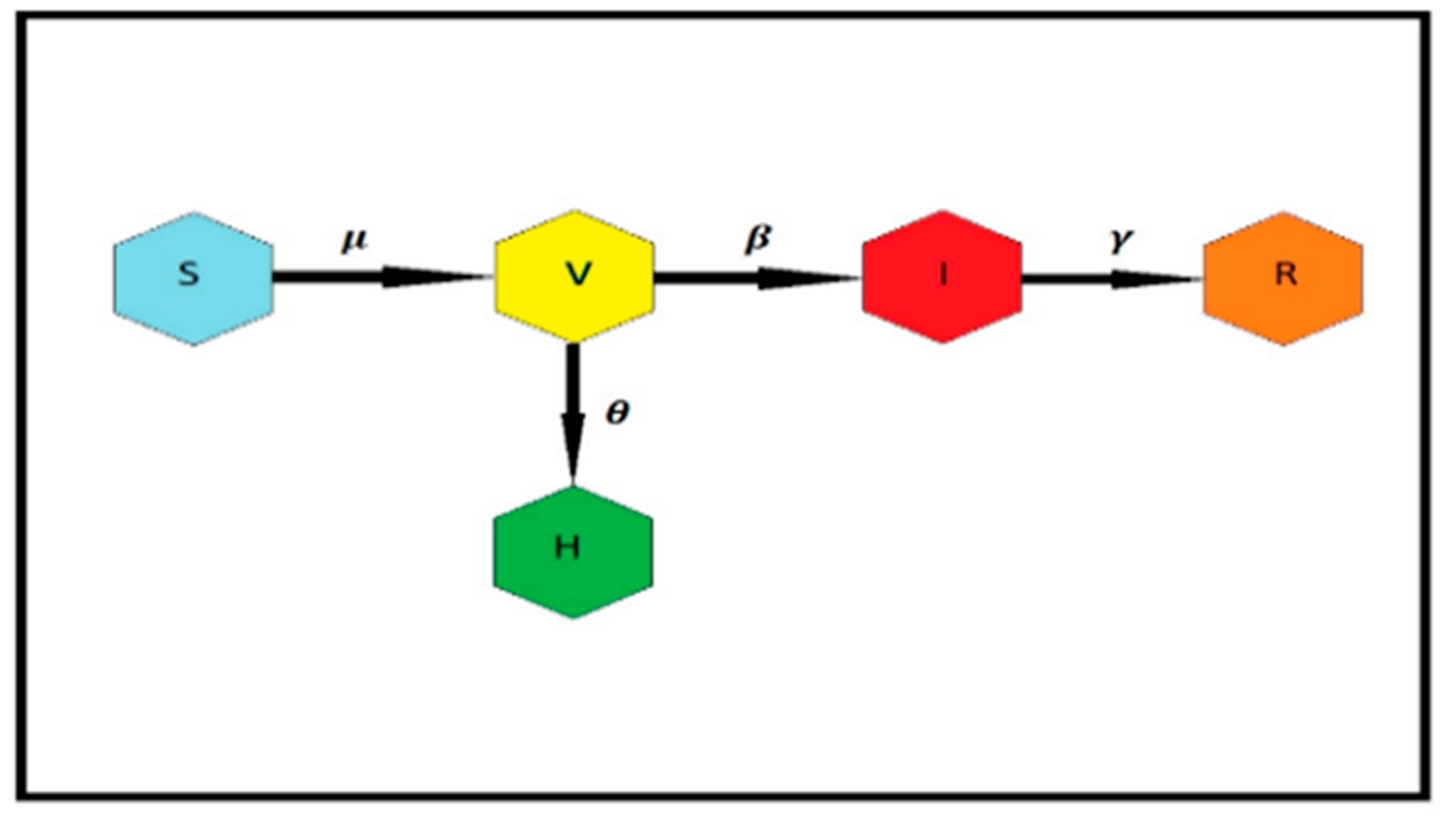
2.2.2. Development of the SVHIR Model

The current study adopted the notion of model development from the SIR model [22]. However, the context of the SIR model is only adopted theoretically to interpret a different context in our scenario practically. It is by no means that the parameters of the SIR model are considered literal for our scenario in the practical sense. For instance, the SIR model parameter describes a variation process of a disease on living organisms. In the current study, our scenario focuses on students, and it is by no means that in a practical sense we consider students being sick or having a disease. Hence, the current study attempted to eliminate the disease part during the adoption of the SIR model and did not succeed. That was because the foundation of the SIR model is based on the disease and when that is eliminated, the model loses its significance. Therefore, the context of the SIR model is adopted as it is for the purpose of developing and applying the model. However, when it comes to the interpretation of the model results, we do not use the same context of the SIR model. For instance, in our conclusions for those students who lacks HOTS, we do not consider them sick but they require improvement.

Some studies revealed that study peer groups have an influence on students, and such an influence could be positive or negative on their academic achievement [27,28]. On the other hand, Mirani [29] focused on the mixed ability classroom; where there are low-ability, average-ability, and high-ability students. One of the findings is that the division of students on the basis of academic performance would cause the quick learners to think that they are very intelligent and thus stop putting in greater effort. In other words, the division can cause low-ability students to negatively impact high-ability students to some extent. Given that division will always exist, whether physically or mentally, for instance scaling of marks or scores (A+, A, B, etc.), there is a form of division which could be necessary but unintentional. Therefore, low-ability students could always negatively affect high-ability students to some extent. In that regard, in this study we assume that the thinking skills of high-ability students can be influenced negatively when they interact or share the same environment with low-ability students. By thinking skills, we also refer to HOTS. In epidemiological terms, in this study we assume that the degradation of higher-order thinking skills (DHOTS) to some extent can be contagious through interaction between high-ability and low-ability students.

To investigate the ability of the N1 to N2 mathematics curriculum to equip students with HOTS, we will use the above assumption to develop a mathematical model. The curriculum is learned in six months (180 days), in which it is also expected to equip students with HOTS during that period. During this period, we will assume there is a pandemic called DHOTS within students enrolled for the N1 to N2 curriculum, and the curriculum acts as a vaccine against the pandemic. In that context, we use the help of Kermack and McKendrick [22] and Diagne et al. [25] to model the behavior of the DHOTS pandemic. Our model called SVHIR has five compartments which are susceptible S(t), vaccinated V(t), healthy H(t), infected I(t), and recovered R(t) as shown in Figure 2. Where parameters such as μ, θ, β, and γ are the vaccination rate, healthy individuals’ discovery rate, disease transmission rate, and recovery rate, respectively. The first compartment is susceptible S(t), which is the stage where all the students first arrive at the N1 level before being exposed to the curriculum. At this compartment, the fact is a certain percentage of students might be already infected by DHOTS and the other portion is not infected (is healthy). That might also be shown by the scores/marks of the first HOTS test (Appendix A) that students obtained: some students passed the test without being exposed to the curriculum. However, according to some researchers, one assessment is not enough to conclude on the state of the student being assessed [14,15,16]. Hence, in this study, we assume all the students at this stage are neither healthy nor infected but are at risk of being infected. The first HOTS test students’ marks do not indicate the students’ status or model compartment but indicate the symptoms of the students in the susceptible compartment. To clarify, in this compartment, it does not mean students have no status but rather we do not have enough evidence to conclude on their status; hence, their status is inconclusive. Second is the vaccinated compartment V(t), the stage where students begin to learn the curriculum. The vaccination compartment has two functions which are to expose the status of the student and cure the disease. The third compartment is healthy H(t): these are students who had never been infected by DHOTS and are not at risk of being infected during the time span of N1 and N2. The fourth compartment is infected I(t); these are students who are infected by DHOTS during the time span of N1 and N2. The last compartment is the recovered R(t), which are students who have recovered from the DHOTS infection through the vaccine (curriculum). There is a criterion that will be explained later, which is used to determine the compartment of each student at time t between the five mentioned compartments.

With the help of Kermack and McKendrick [22] and Diagne et al. [25], the SVHIR model is easily written using ordinary differential equations (ODEs) as follows:

$$\left.\begin{array}{c}\frac{dS}{dt}=-\mu S\left(t\right), \left(a\right)\\ \frac{dV}{dt}=\mu S\left(t\right)-\theta H\left(t\right)-\beta V\left(t\right), \left(b\right)\\ \frac{dH}{dt}=\theta V\left(t\right), \left(c\right)\\ \frac{dI}{dt}=\beta V\left(t\right)-\gamma I\left(t\right), \left(d\right)\\ \frac{dR}{dt}=\gamma I\left(t\right). \left(e\right)\end{array}\right\}$$

(4)

where,

$$V\left(t\right)=H\left(t\right)+I\left(t\right)+R\left(t\right),$$

(5)

and

$$N\left(t\right)=S\left(t\right)+V\left(t\right); N\left(0\right)=S\left(0\right).$$

(6)

All the parameters in the SVHIR model which have not been described before are described in

Table 1. SVHIR model parameters and their descriptions.

Parameters	Description
$\mu$	Vaccination rate
$\theta$	Healthy individuals’ discovery rate
$\beta$	Disease transmission rate
$\gamma$	Recovery rate
$t_0$	Initial or 1st day $t_0=$ 1
$t_f$	Final or 180th day $\left(t_f=180\right)$.
$S_0$	Susceptible individuals on the 1st day/ Initial susceptible individuals
$S_f$	Susceptible individuals on the 180th day/ Final susceptible individuals
$I_0$	Infected individuals on the 1st day/ Initial infected individuals
$I_f$	Infected individuals on the 180th day/ Final infected individuals
$H_0$	Healthy individuals on the 1st day/ Initial healthy individuals
$H_f$	Healthy individuals on the 180th day/ Final healthy individuals
$R_0$	Recovered individuals on the 1st day/ Initial recovered individuals
$R_f$	Recovered individuals on the 180th day/ Final recovered individuals
$V_f$	Vaccinated individuals on the 180th day/ Final vaccinated individuals
$N_0$	Total number of individuals on the 1st day/ Initial total number of individuals

.

2.2.3. Determination of the SVHIR Model Parameters

Since we know the time interval (1 to 180 days), we can determine the finite parameters as follows:

From (4)(a), we obtain

$$\frac{dS}{dt}=-\mu S\left(t\right),$$

$$\frac{dS}{S}=-\mu dt,$$

$$\underset{S_0}{\overset{S_f}{\int }}\frac{dS}{S}=-\mu \underset{t_0}{\overset{t_f}{\int }}dt,$$

$$\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)=-\mu \left(t_f-t_0\right)$$

$$\mu =\frac{\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)}{t_0-t_f}; t_0\ne t_f$$

(7)

Dividing (4)(c) by (4)(a), we obtain

$$\frac{\raisebox{1ex}{$dH$}\!\left/ \!\raisebox{-1ex}{$dt$}\right.}{\raisebox{1ex}{$dS$}\!\left/ \!\raisebox{-1ex}{$dt$}\right.}=\frac{\theta V}{-\mu S},$$

$$\frac{dH}{dS}=\left(\frac{\theta V}{-\mu }\right)\frac{1}{S},$$

$$\underset{H_0}{\overset{H_f}{\int }}dH=\left(\frac{\theta V}{-\mu }\right)\underset{S_0}{\overset{S_f}{\int }}\frac{1}{S}dS,$$

$$H_f-H_0=\frac{-\theta V}{\mu }\left(\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)\right),$$

$$\theta =-\left[\frac{ H_f-H_0}{ \left(\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)\right)}\right]\frac{\mu }{V}$$

Substituting (7) in the above, we obtain

$$\theta =\frac{ H_f-H_0}{ V\left(t_f-t_0\right)}; V\ne 0 and t_f\ne t_0$$

(8)

Dividing (4)(e) by (4)(a), we obtain

$$\frac{dR}{dS}=\frac{\gamma I}{-\mu S},$$

$$\underset{R_0}{\overset{R_f}{\int }}dR=\frac{\gamma I}{-\mu }\underset{S_0}{\overset{S_f}{\int }}\frac{1}{S}dS,$$

$$R_f-R_0 =\frac{-\gamma I}{\mu }\left[\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right) \right],$$

$$\gamma =\frac{\mu }{I}\left[\frac{R_0-R_f}{\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)}\right]$$

Substitute (7) in the above and solving, we obtain

$$\gamma =\left[\frac{\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)}{I(t_0-t_f)}\right]\left[\frac{R_0-R_f}{\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right) }\right]$$

$$\gamma =\frac{R_f-R_0}{I(t_f-t_0)}; I\ne 0 \mathrm{and} t_f\ne t_0$$

(9)

From (4)(c),

$$\begin{gathered} \frac{dH}{dt}=\theta V, \\ \underset{H_0}{\overset{H_f}{\int }}dH=\theta \underset{t_0}{\overset{t_f}{\int }}Vdt, \\ \underset{t_0}{\overset{t_f}{\int }}Vdt=\frac{H_f-H_0}{\theta }. \end{gathered}$$

(10)

Substituting (8) into (10), we obtain

$$\underset{t_0}{\overset{t_f}{\int }}Vdt=V(t_f-t_0).$$

(11)

Add (4)(d) and (4)(e), we obtain

$$\begin{gathered} \frac{dI}{dt}+\frac{dR}{dt}=\beta V, \\ \underset{I_0}{\overset{I_f}{\int }}dI+\underset{R_0}{\overset{R_f}{\int }}dR=\beta \underset{t_0}{\overset{t_f}{\int }}Vdt, \end{gathered}$$

(12)

Substituting (11) into (12), we obtain

$$\begin{gathered} I_f-I_0+R_f-R_0={\beta V(t}_f-t_0), \\ \beta =\frac{I_f+R_f-(I_0+R_0)}{{V(t}_f-t_0) }, \end{gathered}$$

(13)

where $V\ne 0 \mathrm{and} t_f\ne t_0$.

2.2.4. Validation of the SVHIR Model

It is part of the model development procedure to validate the model before its first application. The general notion to validate any model is to compare it with the actual data. Unfortunately, in this study we have very limited data in terms of data points of different times. The current study’s data will not allow us to apply that notion into our model in (4), given that we only have data at two points ($t=1$ and $t=180$). Nonetheless, that does not mean we cannot validate the model; it only means we cannot use the general notion. But nonetheless, this study only needs the model to be valid at $t=180$; given that this is the point where we want to apply the model to investigate whether students benefited from the curriculum or not. Therefore, in our view the resulting functions after integrating (4) are predictions of the respective compartments at time $t$. Hence, if those prediction functions are valid at $t=180=t_f$, we conclude that (4) is also valid at $t_f$.

Development of the General Prediction Functions and Four Parameters (μ, θ, γ, and β)

Since we intend to find general prediction functions, indefinite integrals will be applied; hence, the four parameters will also be general in this subsection.

From (4)(a), we obtain

$$\begin{gathered} \frac{dS}{dt}=-\mu S, \\ \frac{dS}{S}=-\mu dt, \\ \int \frac{dS}{S}=-\mu \int dt, \\ \mathit{ln}\left(S\right)=-\mu t+c_1, \\ {S}_p=S_0{e}^{-\mu t};\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}{S}_0=e^{c_1}. \end{gathered}$$

(14)

Then solving (14), we obtain

$$\mu =\frac{ln\left(\frac{S_0}{S_p}\right)}{t}; t\ne 0 \mathrm{and} S_0,S_p>0$$

(15)

where $S\left(t\right)=S, S\left(1\right)=S_0$, $S_p\left(t\right){=S}_p$, and $c_1$ are respectively defined as the susceptible individuals at time t, susceptible individuals at $t=1$, the SVHIR model predicted number of susceptible individuals at time $t$, and the first constant of integration.

Dividing (4)(c) by (4)(a), we obtain

$$\begin{gathered} \frac{\raisebox{1ex}{$dH$}\!\left/ \!\raisebox{-1ex}{$dt$}\right.}{\raisebox{1ex}{$dS$}\!\left/ \!\raisebox{-1ex}{$dt$}\right.}=\frac{\theta V}{-\mu S}, \\ \frac{dH}{dS}=\left(\frac{\theta V}{-\mu }\right)\frac{1}{S}, \\ \int dH=\left(\frac{\theta V}{-\mu }\right)\int \frac{1}{S}dS, \\ {H}_p=\frac{-\theta V}{\mu }\mathit{ln}\left(S_p\right)+c_2, \end{gathered}$$

(16)

where $\mu \ne 0 \mathrm{and} S_p>0$.

Substituting (15) into (16) and solving, we obtain

$$\theta =\frac{ln\left(\frac{S_0}{S_p}\right)\left(c_2-H_p\right)}{t V\mathit{ln}\left(S_p\right)} ;$$

(17)

where $\left\{c_2\ge H_p\left|S_0,S_p,t,V>0and\theta \ge 0\right.\right\}$.

In the above, $V\left(t\right)=V$, $H_p\left(t\right){=H}_p$, and $c_2$ are respectively defined as the number of the vaccinated individuals at time t (given by (5)), the SVHIR model predicted number of healthy individuals at time $t$, and the second constant of integration. Each resulting lower or upper bound of a constant of integration produced in each equation will be retained throughout the study for simplicity. Then, starting from the constant of integration $c_2$, its lower bound found in (17) will be retained throughout the study.

Dividing (4)(e) by (4)(a), we obtain

$$\begin{gathered} \frac{dR}{dS}=\frac{\gamma I}{-\mu S}, \\ \int dR=\frac{\gamma I}{-\mu }\int \frac{1}{S}dS, \\ {R}_p=\frac{-\gamma I}{\mu }\mathit{ln}\left(S_p\right)+c_3. \end{gathered}$$

(18)

Substituting (15) into (18) and solving, we obtain

$$\gamma =\frac{ln\left(\frac{S_0}{S_p}\right)\left({c_3-R}_p\right)}{t\mathit{ln}\left(S_p\right)I};$$

(19)

where $\left\{c_3\ge R_p\left|I,S_0,S_p,t>0 \mathrm{and} \gamma \ge 0\right.\right\}.$

Then, $I\left(t\right)=I$, $R_p\left(t\right){=R}_p$, and $c_3$ are respectively defined as the number of the infected individuals at time t, the SVHIR model predicted number of recovered individuals at time $t$, and the third constant of integration.

From (4)(c),

$$\begin{gathered} \frac{dH}{dt}=\theta V, \\ \int dH=\theta \int Vdt, \\ \int Vdt=\frac{H_p+c_4}{\theta }, \end{gathered}$$

(20)

When adding (4)(d) and (4)(e), we obtain

$$\begin{gathered} \frac{dI}{dt}+\frac{dR}{dt}=\beta V, \\ \int dI+\int dR=\beta \int Vdt, \\ {I}_p+R_p+c_5=\beta \int Vdt. \end{gathered}$$

(21)

Substitute (20) into (21), we obtain

$$\begin{gathered} I_p+R_p+c_5=\beta \left(\frac{H_p+c_4}{\theta }\right), \\ {I}_p=\beta \left(\frac{H_p+c_4}{\theta }\right)-\left(R_p+c_5\right); \theta \ne 0 \end{gathered}$$

(22)

$$\beta =\frac{\theta (I_p+R_p+c_5)}{H_p+c_4};$$

(23)

Substitute (17) into (23), we obtain

$$\beta =\frac{ln\left(\frac{S_0}{S_p}\right)\left(c_2-H_p\right)(I_p+R_p+c_5)}{t V\mathit{ln}\left(S_p\right)\left(H_p+c_4\right)}.$$

(24)

In the above, $I_p\left(t\right)=I_p$, $c_4$, and $c_5$ are respectively defined as the SVHIR model predicted number of the infected individuals at time t and the fourth and fifth constant of integration. The constant $c_5$ is one of the most influential parameters in (24), since as it increases it brings a drastic change that will require more attention and in our view that is a separate study on its own. Therefore, since this study was more focused on the development side of the new approach (SVHIR model) than advancing it, we will keep $c_5=0$ for this current study and investigate other possibilities of this constant in the future if need be. Therefore, by retaining the lower bound of $c_2$ as mentioned in (17), we obtain (24) to be

$$\beta =\frac{ln\left(\frac{S_0}{S_p}\right)\left(c_2-H_p\right)(I_p+R_p)}{t V\mathit{ln}\left(S_p\right)\left(H_p+c_4\right)};$$

(25)

where $S_0,S_p,t>0 \mathrm{and} \left\{c_4>{-H}_p,c_2\ge H_p\left|\beta \ge 0\right.\right\}$.

Determination of the Constants of Integration (c₂, c₃, and c₄)

In the previous sub-section, we arrived at four different constants of integration but three of them remain partially unknown, given we only know their lower limits. Determining these constants is crucial for this study since they play a very important role towards achieving the goal of the study on HOTS, as it will be seen towards the end of this chapter.

From Equation (17) to (25), we have been able to find the lower bounds or limits of the constants given as follows:

$$\left.\begin{array}{c}{c}_2\ge H_p \left(a\right)\\ c_3\ge R_p \left(b\right)\\ c_4>{-H}_p \left(c\right)\end{array}\right\}$$

(26)

If we refer to the above sub-sections, S′, H′, I′, and R′ are rate of change according to their respective compartments, where their units are persons per day. It is true that each compartment rate of change cannot be greater than the total number of the population (N given by (6)), hence

$$\left.\begin{array}{c}\frac{dH}{dt}\le N, \left(a\right) \\ \frac{dR}{dt}\le N, \left(b\right)\\ \frac{dI}{dt}+\frac{dR}{dt}\le N. \left(c\right)\end{array}\right\}$$

(27)

In (27)(a), we have

$$\frac{dH}{dt}\le N.$$

(28)

Substituting (4)(c) into (28), we obtain

$$\begin{gathered} \theta V\le N, \\ \theta \le \frac{N}{V}; V>0 \end{gathered}$$

(29)

Substituting (17) into (29), we obtain

$$\begin{gathered} \frac{ln\left(\frac{S_0}{S_p}\right)\left(c_2-H_p\right)}{t V\mathit{ln}\left(S_p\right)}\le \frac{N}{V}, \\ {c}_2-H_p\le \frac{ t N\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}, \\ {c}_2\le \frac{ t N\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+H_p; S_0, S_p>0 \end{gathered}$$

(30)

Therefore, combining (26)(a) and (30), we obtain

$$\left\{H_p\le c_2\le \frac{ t N\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+H_p\right\}.$$

(31)

Again, in (27)(b), we also have

$$\frac{dR}{dt}\le N.$$

(32)

Substituting (4)(e) into (32), we obtain

$$\begin{gathered} \gamma I\le N, \\ \gamma \le \frac{N}{I}. I\ne 0 \end{gathered}$$

(33)

Substituting (19) into (33), we obtain

$$\begin{gathered} \frac{ln\left(\frac{S_0}{S_p}\right)\left({c_3-R}_p\right)}{t\mathit{ln}\left(S_p\right)I}\le \frac{N}{I}, \\ {c}_3\le \frac{Nt\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+R_p. S_0, S_p>0 \end{gathered}$$

(34)

Therefore, combining what (26)(b) and (34), we obtain

$$\left\{R_p\le c_3\le \frac{Nt\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+R_p\right\}.$$

(35)

where we differentiate (5), we obtain

$$\frac{dV}{dt}=\frac{dH}{dt}+\frac{dI}{dt}+\frac{dR}{dt}.$$

(36)

Substituting (4)(c), (4)(d) and (4)(e) into (36), we obtain

$$\frac{dV}{dt}=\theta V+\beta V.$$

(37)

Equating (4)(b) and (37), we obtain

$$2\beta V=\mu S-\theta \left(H+V\right).$$

(38)

Substituting (15), (17) and (25) into (38), we obtain

$$\begin{gathered} \frac{2\left(c_2-H_p\right)\left(I_p+R_p\right)}{\ln \left(S_p\right)\left(H_p+c_4\right)} \\ = S-\frac{\left(c_2-H_p\right)(H+V)}{V\mathit{ln}\left(S_p\right)} \end{gathered}$$

(39)

Solving (39), we obtain

$$c_4= \frac{2(I_p+R_p)}{\frac{S ln\left(S_p\right)}{c_2-H_p}-\left(\frac{H+V}{V}\right)}-H_p.$$

(40)

Substituting (30) into (40), we obtain

$$c_4\le \frac{2(I_p+R_p)}{\frac{Sln\left(\frac{S_0}{S_p}\right)}{tN}-\left(\frac{H+V}{V}\right)}-H_p.$$

(41)

Hence,

$$c_4\le \frac{2tNV\left(I_p+R_p\right)}{S ln\left(\frac{S_0}{S_p}\right)V-tN\left(H+V\right)}-H_p;$$

(42)

where $Sln\left(\frac{S_0}{S_p}\right)V\ne tN\left(H+V\right)$.

Therefore from (26)–(42), we obtain the range of the constants of integrations as

$$\left.\begin{array}{l}{H}_p\le c_2\le \frac{ t N\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+H_p\\ R_p\le c_3\le \frac{Nt\mathit{ln}\left(S_p\right)}{ln\left(\frac{S_0}{S_p}\right)}+R_{p }\\ c_4>{-H}_p\\ c_4\le \frac{2NtV\left(I_p+R_p\right)}{S ln\left(\frac{S_0}{S_p}\right)V-Nt\left(H+V\right)}-H_p\end{array} \right\}$$

(43)

Validation

Note that from (14) to (22), it can be observed that we only produced four compartment prediction functions, whereas we have five compartments. We purposefully left out the vaccinated prediction function ($V_p$), because of the following reason:

It has no influence at all in the final conclusions of this study. If all other prediction functions are valid except the vaccinated prediction function, the SVHIR model will still be effective for this current study. However, it should be clear that only the prediction function is insignificant not the compartment itself, given that the vaccinated compartment plays a vital role to other compartments more especially on the healthy and infected.

Therefore, the four resulted prediction functions from (14) to (22) that represent our SVHIR model are as follows:

$$\left.\begin{array}{l}{S}_p=S_0{e}^{-\mu t}, \\ H_p=\frac{-\theta V}{\mu }\mathit{ln}\left(S_p\right)+c_2 \\ R_p=\frac{-\gamma I}{\mu }\mathit{ln}\left(S_p\right)+c_3 \\ I_p=\beta \left(\frac{H_p+c_4}{\theta }\right)-R_p.\end{array}\right\}$$

(44)

Let us consider the model (44) to be valid at $t=180=t_f$. In that case, it will accurately predict all the compartments from the actual data. Hence $S_p=S_f$, $H_p=H_f$, $R_p=R_f$, and $I_p=I_f$ at $t=t_f$, where the $S_f$, $H_f$, $R_f$, and $I_f$ are from the actual data at time $t=t_f$. Since the validation is performed at $t=t_f$, this implies ${N=N}_f$, ${V=V}_f$, and ${S=S}_f$. Then, the constants of integration from (4.43) range as follows:

$$\left.\begin{array}{c}{H}_f\le c_2\le \frac{t_f{N}_f\mathit{ln}\left(S_f\right)}{\mathit{ln}\left(\frac{S_0}{S_f}\right)}+H_f,\hfill \\ R_f\le c_3\le \frac{N_f{t}_f\mathit{ln}\left(S_f\right)}{\mathit{ln}\left(\frac{S_0}{S_f}\right)}+R_f,\hfill \\ c_4>-H_f,\hfill \\ c_4\le \frac{2N_f{t}_f{V}_f\left(I_f+R_f\right)}{S_f\mathit{ln}\left(\frac{S_0}{S_f}\right)V_f-N_f{t}_f\left(H_f+V_f\right)}-H_f\hfill \end{array}\right\}$$

(45)

Note that there are two types of the four parameters derived in this study. Firstly, there is the indefinite type found from (15) to (25), which was used to estimate the lower limits of the constants of integration. Secondly, there is the finite type found from (7) to (13). Both of those types serve the same purpose when the model is valid; hence, either one of them will be suitable. In our case, we choose the finite type given that it is less complex; hence, at $t=180$, the four parameters in (7)–(9) and (13) become

$$\left.\begin{array}{l}\mu =\frac{\mathit{ln}\left(S_f\right)-\mathit{ln}\left(S_0\right)}{t_0-t_f}, (a) \\ \theta =\frac{ H_f-H_0}{ V_f\left(t_f-t_0\right)}, (b)\\ \gamma =\frac{R_f-R_0}{I_f\left(t_f-t_0\right)}, (c)\\ \beta =\frac{I_f+R_f-\left(I_0+R_0\right)}{{V_f(t}_f-t_0) }. (d)\end{array}\right\}$$

(46)

If we can accurately predict the respective compartments in the actual data by using (44)–(46), then we conclude that the SVHIR model is valid at $t=180$.

2.2.5. Basic Reproductive Ratio

The basic reproductive ratio of the SVHIR model is given as follows:

$$R_0=\frac{\beta }{\gamma }.$$

(47)

The basic reproductive ratio at $t=180=t_f$ is found by substituting (46)(c) and (46)(d) into (46), hence

$$\begin{gathered} R_0=\frac{\frac{I_f+R_f-\left(I_0+R_0\right)}{{V_f(t}_f-t_0) }}{\frac{R_f-R_0}{I_f\left(t_f-t_0\right)}}, \\ {R}_0=\frac{I_f\left[I_f+R_f-\left(I_0+R_0\right)\right]}{V_f(R_f-R_0) }. \end{gathered}$$

(48)

According to our model, at $t=1$ all students are just arriving and susceptible. None is infected or recovered, hence $I_0=0$ and $R_0=0$. Then, (48) becomes

$$R_0=\frac{I_f\left(I_f+R_f\right)}{V_f{R}_f },$$

(49)

where $V_f=I_f+H_f+R_f$ and $R_f\ne 0$.

2.2.6. Association of the Actual Data with the SVHIR Model

In this study, we have two HOTS tests (Appendix A and Appendix B), and we use their test scores or marks ($M_i$) to categorize students according to the SVHIR model compartments. However, before detailing the compartments, we first define the test scores ranges, respectively, as shown in

Table 2. Description and compartmental categorization of students based on HOTS tests scores range.

Order	Scores	Description	Compartment
1.	$0\le M_i\le 5\%$	Nil	susceptible
2.	$5\%<M_i<50\%$	fail	infection
3.	$M_i\ge 50\%$	pass	healthy or recovery

. Note the following when interpreting the table:

• A score of less than or equal to 5% cannot be used to define the status of a student: it is a nil, given that this score is highly possible to be obtained by a person who guessed the answers without being exposed to the curriculum. Therefore, we equivalate this person as someone who never took the test; hence, this score is associated with the susceptible compartment;
• A student with a score between 5% and 50% counts as a failed; hence, this score is associated with the infection compartment;
• A student with a score at 50% and above counts as a pass; hence, this score is associated with the healthy or recovery compartment.

There are 15 possible combination outcomes if a student takes the two HOTS tests (Appendix A and Appendix B), and each outcome defines the SVHIR model compartment as shown in

Table 3. Compartmental categorization of students based on HOTS tests scores.

Outcome	Test 1 Marks (t = 0)	Test 2 Marks (t = 180)	Resultant Compartment (t = 180)
1	$0\le M_i\le 5\%$	$0\le M_i\le 5\%$	Susceptible
2	$0\le M_i\le 5\%$	$5\%<M_i<50\%$	Infected
3	$0\le M_i\le 5\%$	$M_i\ge 50\%$	Recovered
4	$5\%<M_i<50\%$	$0\le M_i\le 5\%$	Infected
5	$5\%<M_i<50\%$	$5\%<M_i<50\%$	Infected
6	$5\%<M_i<50\%$	$M_i\ge 50\%$	Recovered
7	$M_i\ge 50\%$	$0\le M_i\le 5\%$	Infected
8	$M_i\ge 50\%$	$5\%<M_i<50\%$	Infected
9	$M_i\ge 50\%$	$M_i\ge 50\%$	Healthy
10	$0\le M_i\le 5\%$	None	Excluded $N_f<S_0$
11	$5\%<M_i<50\%$	None	Excluded $N_f<S_0$
12	$M_i\ge 50\%$	None	Excluded $N_f<S_0$
13	None	$0\le M_i\le 5\%$	Excluded $N_f>S_0$
14	None	$5\%<M_i<50\%$	Infected $N_f>S_0$
15	None	$M_i\ge 50\%$	Excluded $N_f>S_0$

$M_i$—Student’s HOTS test marks/score, t—Days.

. Those outcomes are explained as follows:

• A student who received nil in the first test and nil in the second test is considered susceptible. The first test shows symptoms of susceptibility (neither infected nor healthy but at risk of infection), and towards the end of the curriculum the second test confirms the symptoms remained the same, which means the student did not move to the vaccine compartment. Hence, the student stays in the susceptible compartment. Nonetheless, this does not mean the curriculum was not presented to the student but rather means it was presented and did not make any significant impact or sink in for the student. Therefore, the student is the same as the time of arrival, which happens at the susceptible stage.
• A student who received nil in the first test and failed in the second test is considered infected. The first test show symptoms of susceptibility, and towards the end of the curriculum the second test confirms that the student is infected. Hence the student will move from susceptible S(t), vaccinated V(t) and to infected I(t) compartment. In this case, the curriculum was presented and did make an impact to the student but not enough.
• A student who received nil in the first test and passed in the second test is considered recovered. The first test show symptoms of susceptibility, and towards the end of the curriculum the second test confirms the symptoms have improved. Hence, the student will move from the susceptible S(t) or vaccinated V(t) and to the healthy I(t) compartment. When the curriculum is presented to this student, it is very impactful.
• A student who failed in the first test and received nil in the second test is considered infected. The first test shows symptoms of infection, and towards the end of the curriculum the second test confirms the symptoms of being at risk of infection. This student is considered infected. In the model, this student will move from the susceptible S(t) or vaccinated V(t) and to the infected I(t) compartment. In this case, the curriculum was presented and did make an impact on the student but not enough.
• A student who failed in the first test and failed in the second test is considered infected. The first test shows symptoms of infection, and towards the end of the curriculum the second test confirms the symptoms remained the same. Hence, the student will move from the susceptible S(t) or vaccinated V(t) and to the infected I(t) compartment. In this case, the curriculum was presented and did make an impact on the student but not enough.
• A student who failed in the first test and passed in the second test is considered in recovery. The first test shows symptoms of infection, towards the end of the curriculum the second test confirms the symptoms have gotten better. Hence, the student will move from the susceptible S(t), vaccinated V(t) or infected I(t) and to the recovered R(t) compartment. In this case, the curriculum was presented and did make an impact on the student.
• A student who passed in the first test and received nil in the second test is considered infected. The first test shows symptoms of being healthy, and towards the end of the curriculum the second test confirms the symptoms of being susceptible. For a student to be moved from healthy to susceptible is the indication of degradation of the skill; and that can only happen when someone is infected. Hence, the student will move from the susceptible S(t) or vaccinated V(t) and to the infected I(t) compartment. In this case, the curriculum was presented and did make an impact on the student but not enough.
• A student who passed in the first test and failed in the second test is considered infected. The first test shows symptoms of being healthy, and towards the end of the curriculum the second test confirms the symptoms of infection. For a student to be moved from healthy to susceptible is an indication of degradation of the skill; and that can only happen when someone is infected. Hence, the student will move from the susceptible S(t) or vaccinated V(t) and to the infected I(t) compartment. In this case, the curriculum was presented and did make an impact on the student but not enough.
• A student who passed in the first test and passed in the second test is considered healthy. The first test shows symptoms of being healthy, and towards the end of the curriculum the second test confirms the symptoms have remained the same. Hence, the student will move from the susceptible S(t) or vaccinated V(t) and to the healthy H(t) compartment. This student is presumed to have arrived already equipped with HOTS; hence, when the curriculum is presented to them, it is very impactful.
• A student who received nil in the first test and did not get a chance to participate in the second test is excluded in the current study. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the second test, the student could either be Outcome 1 or 2 or 3 in Table 3, which are three different compartments (susceptible, infected, and recovered) the student could possibly belong to, and the study is unable to conclude about the student’s compartment between the three in the absence of the second test score. Hence, the student is excluded.
• A student who failed in the first test and did not get a chance to participate in the second test is excluded in the current study. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the second test, the student could either be Outcome 4 or 5 or 6 in Table 3, which are two different compartments (infected and recovered) the student could possibly belong to, and the study is unable to conclude about the student’s compartment between the two in the absence of the second test score in that case. Hence, the student is excluded.
• A student who passed in the first test and did not get a chance to participate in the second test is excluded in the current study. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the second test, the student could either be Outcome 7 or 8 or 9 in Table 3, which are two different compartments (infected and healthy) the student could possibly belong to, and the study is unable to conclude about the student’s compartment between the two in the absence of the second test score in that case. Hence, the student is excluded.
• A student who did not participate in the first test and received nil in the second test is excluded in the current study. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the first test, the student could either be Outcome 1 or 4 or 7 in Table 3, which are two different compartments (susceptible and infected) the student could possibly belong to, and the study is unable to conclude about the student’s compartment between the two in the absence of the first test score in that case. Hence, the student is excluded.
• A student who did not participate in the first test and failed in the second test is considered infected. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the first test, the student could either be Outcome 2 or 5 or 8 in Table 3, which are all the infected compartments.
• Lastly, this is a student who only participated in the second test and passed. This student will also be excluded in the current study. The reason is that with the three possible scores (nil, fail, and pass) the student could have obtained in the first test, the student could either be Outcome 3 or 6 or 9 in Table 3, which are two different compartments (recovered and healthy) the student could possibly belong to, and the study is unable to conclude about the student’s compartment between the two in the absence of the first test score in that case. Hence, the student is excluded.

2.2.7. Application of the SVHIR Model Instruction

From the SVHIR model, we produced the basic reproduction in (49) expressed as

$$R_0=\frac{I_f\left(I_f+R_f\right)}{V_f{R}_f };$$

(50)

where $V_f=I_f+H_f+R_f$ and $R_f\ne 0$.

For this study, the data were collected at a TVET college and categorized according to Table 3. For the SVHIR model application, (50) is applied into the actual data to determine which of the following two cases result:

Case 1 ($R_0>1)$

This means the curriculum has failed to equip students with HOTS.

Case 2 $(R_0<1)$

This means the curriculum has equipped students with HOTS.

2.3. Data Collection and Participants

We collected our data at eMnambithi TVET College, Ezakheni E-section campus. It was collected from the 47 students using Appendix A as the pre-assessment and Appendix B as the post-assessment. Then, Appendix C was used to score the students’ responses. The final processed data of the current study are presented in Appendix D. The first part of data collection took place in March 2022 and the second part in October 2022. Normally, N1 classes start in January but, due to COVID-19, classes were disturbed. N1 ended up starting in March 2022. Nonetheless, students were given an equal opportunity of 180 days to complete the N1 to N2 curriculum and all of them received the same teaching approaches.

3. Results of the Research

This section presents the results of the partial evaluation of lecturers’ content delivery ability and the SVHR model application results from the data under investigation.

3.1. Partial Evaluation of the Content Delivery

As mentioned, there are many variables that can contribute to students attaining HOTS but the most critical are lecturers’ content delivery ability and the curriculum [17,18,19,20,21]. For the approach of the current study to work, the status of the content delivery needs be known. If that is not possible, at least a partial investigation of the content delivery must be conducted for the assumption to be built upon.

The current study used Question 6 in Appendix B to partially investigate the N1 and N2 mathematics lecturers’ content delivery ability at eMnambithi TVET College and the results are presented in

Table 4. Validation of the evaluation instrument with Appendix B Question 6.

Students’ Response	Number of Students	Percentage
Yes	26	55.6%
No	2	4.3%
No comment	19	40.4%

. Note that we used the word “partially”, given that it takes more than what we conducted to evaluate the lecturers’ content delivery ability. However, what we performed is the beginning of that evaluation, but it has the potential to indicate the possible outcome of the overall evaluation. Hence, we will build our assumption about the lecturers’ content delivery ability upon it.

3.2. Evaluation of HOTS

This sub-section presents the validation and application of the SVHIR model by using the actual data in Appendix D. Note that the categorization of students according to the SVHIR model compartments in Appendix D was accomplished by applying Table 3. From those categorizations,

Table 5. SVHIR compartment values from the actual data in Appendix D.

Compartment Parameters	Actual Data Values
Initial time (in days)	$t_0=1$
Final time (in days)	$t_f=180$
Initial susceptible individuals	$S_0=47$
Final susceptible individuals	$S_f=2$
Initial infected individuals	$I_0=0$
Final infected individuals	$I_f=36$
Initial healthy individuals	$H_0=0$
Final healthy individuals	$H_f=6$
Initial recovered individuals	$R_0=0$
Final recovered individuals	$R_f=3$
Final vaccinated individuals	$V_f=H_f+I_f+R_f=45$
Initial total number of individuals	$N_0=47$
Final total number of individuals	$N_f=47$

was produced.

3.2.1. Validation of SVHIR Model

In the validation section, our validation notion deduces that the SVHIR model is valid if and only if (44) can predict the compartments with the integration constants satisfying (45). Substituting all the necessary values into (45), the integration constants’ intervals for the current study’s actual data are as follows:

$$\left.\begin{array}{c}6\le c_2\le 1 863. 46,\\ 3\le c_3\le 1 860.47,\\ -6<c_4\le -0.03. \end{array}\right\}$$

(51)

The compartment rates calculated using (46) and the actual data are presented in

Table 6. SVHIR compartment rates calculated from the actual data.

Compartment Rate Names	Calculated Values
Vaccination rate	$\mu =0.0180$
Healthy individuals’ discovery rate	$\theta =0.0007$
Disease transmission rate	$\gamma =0.0005$
Recovery rate	$\beta =0.0050$

. Those rates together with the actual data were applied in the SVHIR model to predict the compartments presented in

Table 7. Predicted versus actual SVHIR compartments.

Integration Constant	Predicted Compartment at ${\mathit{t}}_{\mathit{f}}$	Actual Compartment at ${\mathit{t}}_{\mathit{f}}$
-	$S_p=1.84\approx 2$	$S_f=2$
$c_2=7.21$	$H_p=6$	$H_f=6$
$c_3=3.69$	$R_p=2.71\approx 3$	$R_f=3$
$c_4=-0.54$	$I_p=36$	$I_f=36$

, where the integration constants satisfy the stipulated intervals. Therefore, this study concludes that the SVHIR model is valid at $t=t_f=180$.

3.2.2. Application of SVHR Model

As mentioned in Section 2.2.7, the HOTS is investigated by applying the extension of the SVHIR model called the basic reproductive ratio. Substituting all necessary variables taken from Table 5 into (4.50), we obtain

$$R_0=10.4$$

(52)

The basic reproductive ratio relates to Case 1 ${(R}_0>1)$ according to Section 2.2.7.

4. Results Discussion

As a partial evaluation of the lecturer’s content delivery ability at eMnambithi TVET College, students were asked if they recognize the questions in Appendix A and Appendix B from the class lessons. The results indicate that the first portion of 55.6% responded yes, the second portion of 4.3% responded no, and the last portion of 40.4% did not answer the question. The last portion (40.4%) will be excluded since their stand is unclear. Therefore, when we only consider the first and second portion, we find that the majority of the students considered the questions in Appendix A and Appendix B to be relevant to what they have learnt. That indicates a possible adequate N1 and N2 mathematics lecturers’ content delivery ability. In that regard, the current work establishes an assumption that the lecturers’ content delivery ability might be adequate at eMnambithi TVET College. That leaves the curriculum as the only major contributing factor in the case where students are found to have a poor HOTS. Hence, in that case, the impact of the curriculum remains the only variable to be evaluated in Appendix A and Appendix B regarding students’ responses.

We found the SIR model to be inefficient to evaluate the curriculum. However, after modifying the SIR model to an SVHIR model, it was found to be efficient. Therefore, to investigate the capability of the curriculum to equip students with HOTS, an extension of the SVHIR model called the basic reproductive ratio was applied. The resulting basic reproductive ratio in Section 3.2.2 indicated that students were not helped to improve their HOTS during the period of 6 months that they were exposed to the N1 to N2 mathematics curriculum. In other words, the SVHIR model indicates that the curriculum might be incapable of equipping students with HOTS at eMnambithi TVET College.

5. Conclusions

The study reported on was an exploratory study, where the authors explored a new potential approach of evaluating HOTS in the TVET college mathematics curriculum. Mostly, the study was focused on the development of the evaluation model and less on the application. This was because the application of the model is a separate study on its own. Also, the data were very limited because of the reason mentioned in the next section: the limitations. Hence, all the findings of the study indicate a possible situation rather than a definite one.

Looking at Appendix D or Table 5, at the end of the curriculum about 77% of students were indicated to possibly remain with DHOTS. That is a huge portion of the population, which will make the basic reproductive ratio to be expected far beyond 1, as a confirmation of a large portion of population remain with DHOTS. Indeed, the basic reproductive ratio from the SVHIR model was as anticipated. Hence, the N1 to N2 mathematics curriculum was found as being potentially incapable of equipping students with HOTS.

A further study should be conducted in the future, where the model could be applied to larger-scale data, given that in the current study the model development was a priority rather than its application. Hence, the application was performed on smaller-scale data so as to test the model. However, the model produced a sound conclusion within the context of the available data. Also, in a further study we will have an opportunity to eliminate some of the assumptions. Currently, the study has significant assumptions which has the possibility of affecting the study to some extent.

6. Limitations

During the period of the current study’s execution, we identified a common challenge in most KZN TVET colleges at a distance. There was a lack of proper leadership, which ultimately affects many areas including teaching and learning within each TVET college. Some of the gatekeepers were aware of the challenge, but instead of rectifying it they shielded that challenge. Hence, they prevented any research focusing on teaching and learning from taking place in their institutions. That resulted in the current study having limited data from TVET colleges, given that we were only able to obtain eMnambithi TVET College data. In that regard, the study changed to an exploratory study. Hence, the developed model was only tested to be valid on limited data.

South Africa has nine provinces, where KZN is only one of them. Therefore, the next study should mostly focus on the application of the developed model to a wider scale of data, to be based on the other eight provinces.