Similarities in Procedures Used to Solve Mathematical Problems and Video Games

Abstract

Video game use is widespread among all age groups, from young children to older adults. The wide variety of video game genres, which are adapted to all tastes and needs, is one of the factors that makes them so attractive. In many cases, video games function as an outlet for stress associated with everyday life by providing an escape from reality. We took advantage of this recreational aspect of video games when investigating whether there are similarities between the procedures used to pass a video game level and those used to solve a mathematical problem. Moreover, we also questioned whether the use of video games can reduce the negative emotions generated by mathematical problems and logical–mathematical knowledge in general. To verify this, we used the Portal 2 video game as a research method or tool. This video game features concepts from the spatial–geometric field that the students must identify and relate in order to carry out the procedures required to solve challenges in each level. The procedures were recorded in a questionnaire that was separated into two blocks of content in order to compare them with the procedures used to solve mathematical problems. The first block pertains to the procedures employed and the second block to the emotions that the students experienced when playing the video game and when solving a mathematical problem. The results reveal that the recreational aspect of video games is more important than the educational aspect. However, the students were not aware of using the problem-solving procedures they learned at school to solve different challenges in the video games. Furthermore, overcoming video game challenges stimulates positive emotions as opposed to the negative emotions generated when solving mathematical problems.

1. Introduction

Current technological developments emerge in all social, cultural, and educational contexts. Among these developments, digital whiteboards or didactic software are examples of applications and hardware designed for the educational context. However, there are also digital elements that, despite not being designed for the teaching–learning process, have been used for this purpose. In light of this, video games could be considered based on the same essence as traditional games. McGonigal [1] states that a video game must be based on the premise of overcoming a challenge and being motivated to do so. Therefore, when interacting with these recreational applications, the individual must: (a) analyse the challenge that appears before them and determine what its purpose is; (b) analyse which elements in the game represent support (power-ups) or which elements are negative (enemies, traps, or penalties); (c) discover how to progress or gain experience; (d) consider action sequences by trial-and-error exercises; and (e) put decision-making skills into practice [2]. A careful analysis of the previously mentioned skills reveals that they are similar to those used in problem-solving.

Based on this, problem-solving is one of the most relevant areas in logical–mathematical knowledge. In fact, problem-solving can be applied to the field of mathematics as well as to aspects of daily life: when people encounter situations that require a solution in their daily lives, they unconsciously apply the problem-solving method they learned in school. In this manner, mathematical competence is developed through problem-solving exercises. According to Gorgorió and Albarracín [3]:

Mathematical competence is the ability to use mathematical knowledge in a cross-cutting manner in mathematical and non-mathematical situations and contexts. Mathematical competence goes beyond procedural knowledge; it is manifested in the use of conceptual knowledge in different practical situations.

(pp. 116–117)

In view of this definition of mathematical competence, it could be stated that video games are included in these non-mathematical contexts. However, the question would be whether video games can be used in mathematical contexts, such as classrooms, during mathematics or science classes. According to the literature on this topic, the answer is yes. Various studies describe the use of these elements in the classroom—for example, using the Angry Birds video game to develop mathematical knowledge [4,5,6] or physical knowledge [7,8,9,10,11].

1.1. Problem-Solving

Problem-solving could be considered one of the most important curricular activities in all the stages of a country’s educational system. Analysing the current legislation, one can see that, in all cases, problem-solving is oriented towards problems in children’s daily lives. Focusing on Spain (whose legislation stipulates that problem-solving be present from the earliest stages of education), self-confidence, the capacity for initiative, and problem-solving are developed from early childhood education onwards [12]. In primary education, problem-solving competencies are also developed within the field of mathematics, together with others, such as reading, reflection, planning processes, establishing resolution strategies, and designing and evaluating procedures [13]. In both stages, problem-solving is based on the development of different skills that allow students to address the situation and/or problem while developing skills related to personal development, personal autonomy, confidence, and motivation to overcome situations in their daily lives.

The logical–mathematical skills to be developed are established sequentially through a series of phases. As a result of these phases, a methodology for solving mathematical problems that is applicable to any situation is established. One of the most well-described and frequently used methodologies is that of Polya [14], which outlines four phases to pose and solve a problem through a series of questions set out in a method (

Table 1. Polya’s problem-solving phases.

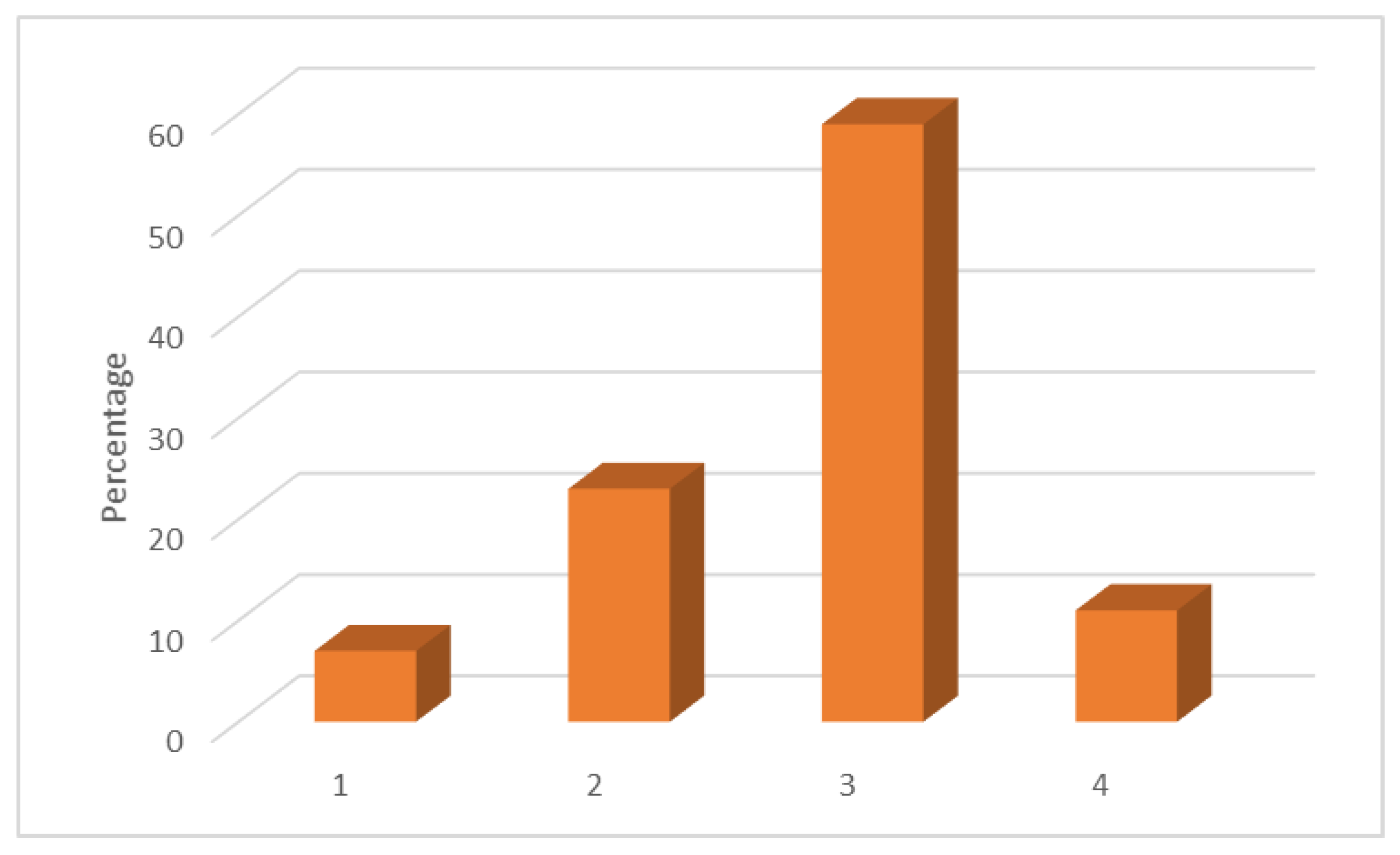
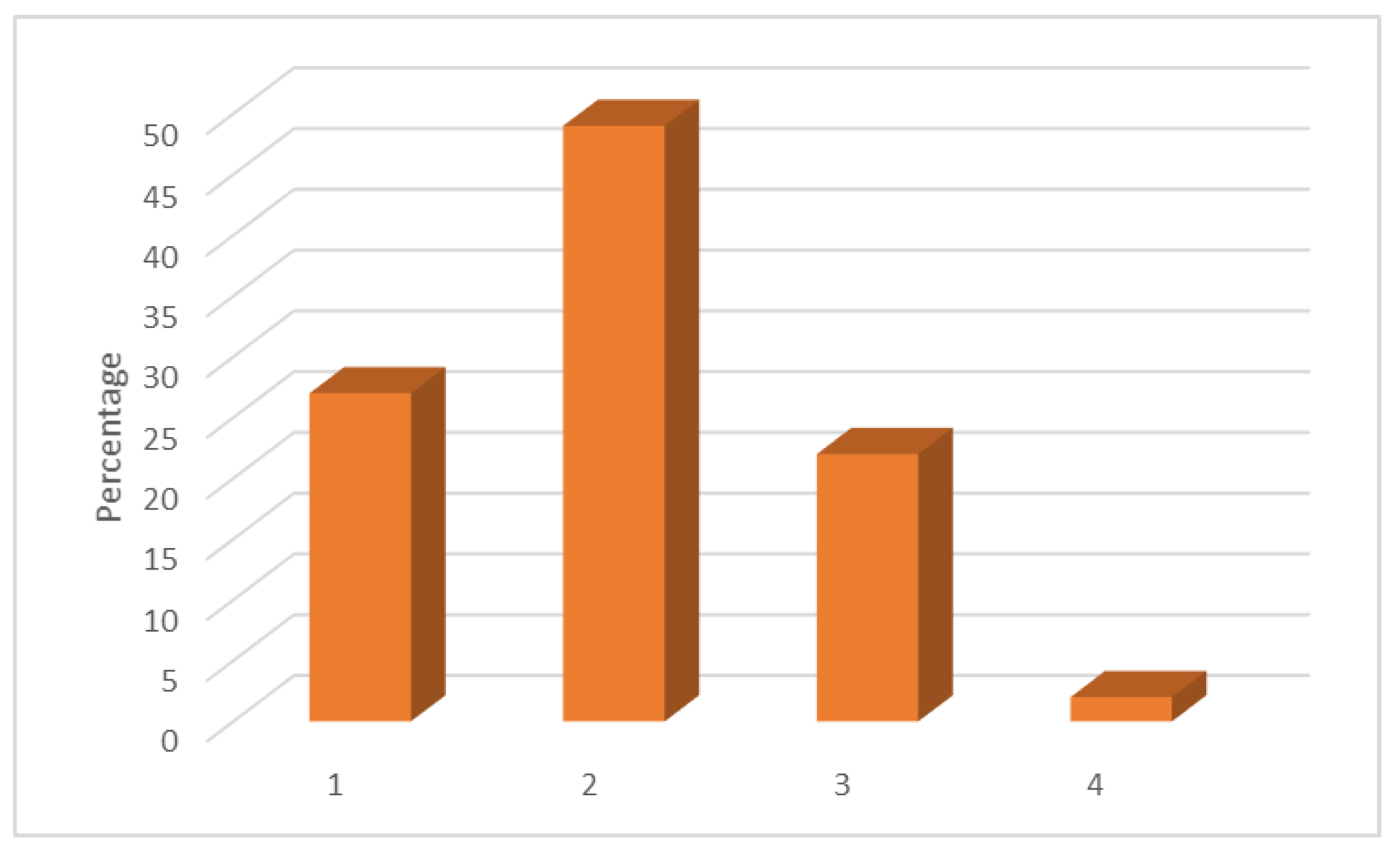
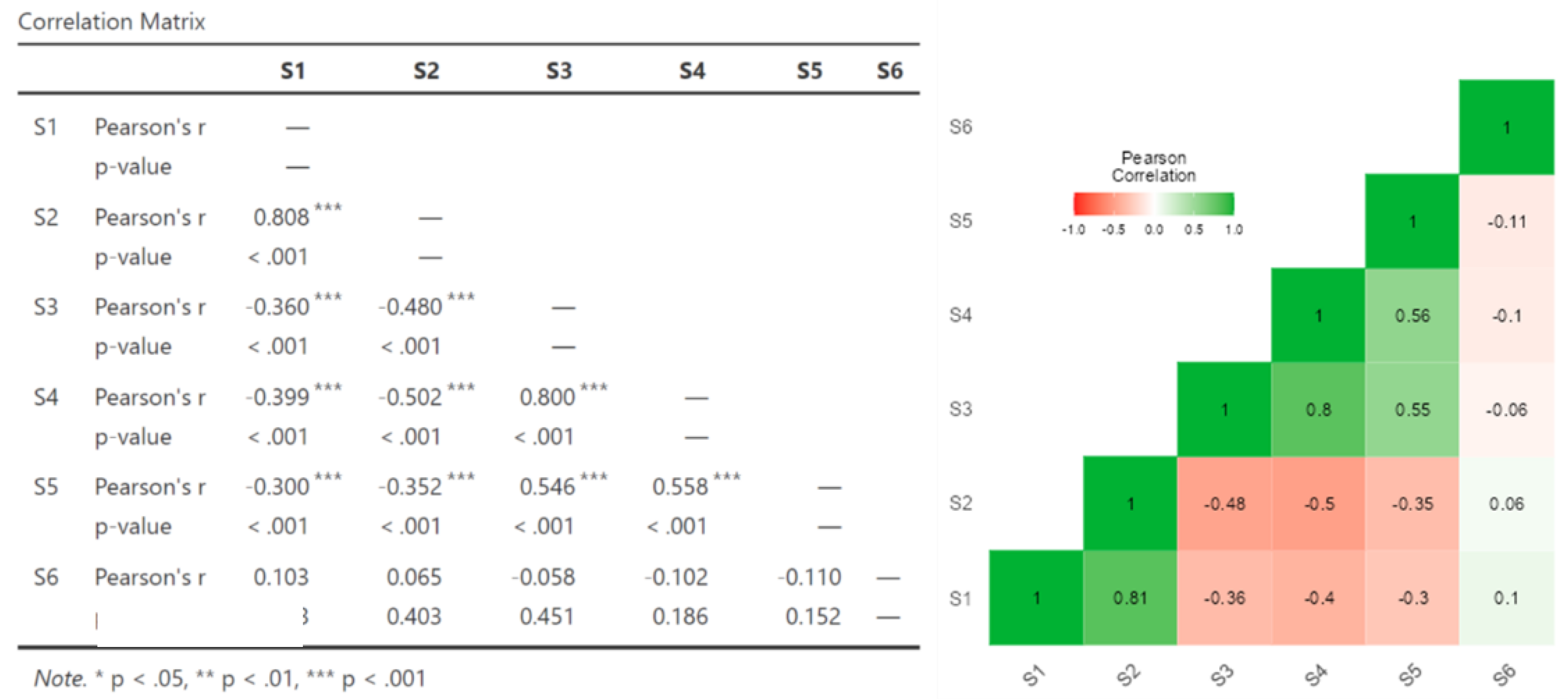
Phases	Questions
Understanding the problem	What is the unknown? What data do I have? What is the condition? Is it enough to find the unknown? Is it redundant, contradictory, or insufficient?
Devising a plan	Have I seen this problem before? Do I know of any similar problems?
Carrying out the plan	Am I sure that each step is correct? Can I prove that the step is correct?
Looking back	Can I check the result and the reasoning? Can I derive the solution differently?

Source: own elaboration based on Polya [14].

).

Mason, Burton, and Stacey [15] described another method of phased problem-solving, which is divided into three phases—entry, attack, and review. As with the previous method, in each of its phases, a series of questions are posed that allow the individual to progress (

Table 2. Summary of Mason, Burton, and Stacey’s problem-solving phases.

Phases	Processes	Issues or Propositions	States
Entry	Specialising	What do I KNOW? What do I WANT? What can I INTRODUCE?	STUCK!
Attack		CONJECTURE
	Generalising	Try (Attempt) Check and distrust (Maybe) But why?	AHA!
Review		CHECK the resolution REFLECT on the key ideas and key moments GENERALISE to a wider context

Source: own elaboration based on Mason et al. [15].

).

Within the description of the method presented by Mason et al. [15], as well as the phases, there are processes such as specialising—typical of the entry and attack phases—and generalising—typical of the attack and review phases. The method introduces the concepts of STUCK! and AHA!—concepts related to the manner of dealing with problem-solving and the learning possibilities that can be extracted from solving the problem.

Being in the STUCK! phase leads to many cases of frustration and a lack of motivation to move forward. Recent studies [16] introduce a new phase in problem-solving methods, in which the identification and control of emotions that arise when solving a problem play an important role. Di Leo et al. [17] indicate that the main emotions that students experience when solving a mathematical problem are frustration and confusion, which are negative emotions. Managing negative emotions, such as confusion, can lead to positive emotions that help with solving the problem. According to Caballero, Blanco, and Guerrero [18], it is necessary to introduce emotional aspects as well as cognitive aspects in mathematical problem-solving. By doing so, we can develop techniques, such as relaxation or breathing techniques, that allow us to transform negative emotions, such as anxiety, into positive emotions. Hannin and Nieuwenhoven [19] found a reduction in negative emotions in students who had developed cognitive and emotional aspects versus those who had only received training in problem-solving, although the cognitive levels were equivalent. Therefore, it is necessary to take into account cognitive and emotional changes as a whole, rather than individually, to understand students’ performance when solving mathematical problems [20]. These changes move students from the STUCK! phase to the AHA! phase.

1.2. Video Games for Problem-Solving

A series of logical–mathematical skills are employed when solving a mathematical problem. These skills can be used to overcome the challenges posed by the different phases of a video game, thus providing a number of opportunities to put mathematical knowledge into practice [21]. Among these skills are observing the elements of the screen or level, differentiating useful elements or accessories, designing strategies, and anticipating results from the objects [22,23,24]. Visuospatial and spatial–geographical skills are also required to interpret plans or areas of the screen. As such, video games provide an opportunity to develop mathematical logic and to establish processes of observation, relation, and operation or transformation.

1.3. Research Questions and Objectives

Considering the relationship that exists between the use of video games and logical–mathematical knowledge, we have posed the following research questions and their corresponding objectives.

Research Question 1. Are the procedures that students use to pass a level in a video game and to solve a mathematical problem comparable?

Objective 1. To verify if the mathematical problem-solving procedures used by students are similar to those they use to pass a level of a video game.

Research Question 2. Do students experience similar feelings when passing a level in a video game and solving a mathematical problem?

Objective 2. To compare the feelings that students experience while playing a video game with those they experience when solving a mathematical problem.

Based on the previous paragraphs, the aim of this study is to discover whether the procedures used to complete video game levels are similar to those used in problem-solving, and to compare whether there are any similarities between the main characteristics of a video game and the characteristics of a mathematical problem. Furthermore, we also aim to observe the emotions students experience when playing video games and compare them with the emotions they experience when solving a mathematical problem.

2. Materials and Methods

2.1. Population and Sample

This study was carried out at the University of Cadiz, in the Faculty of Education Sciences. The participants were 170 trainee teachers taking the subject “Mathematical Knowledge in Early Childhood Education” of the bachelor’s degree in early childhood education (n = 170). We chose to select students taking this subject because it involves developing the first of the three pillars that constitute didactics—that is, logical–mathematical knowledge, in which they develop their own discourse on the construction of this knowledge.

2.2. Method

In order to answer the research questions posed, we decided to use a video game that we know as a research method or tool. We chose the Portal 2 video game, developed by the Valve Corporation, to work on problem-solving with our students. We chose this video game because we were aware of its potential to impart logical–mathematical and spatial–geometric knowledge, which allows students to improve visuospatial competence, and, therefore, to identify shapes or objects that appear in the scene. By looking for the relationship between the shapes and objects that appear on the screen, students obtain information and develop a strategy to pass the level. Portal 2 is a platform/action game with puzzles that appear in the form of a series of riddles on the walls and objects to solve in order to pass to the next level. Hence, we considered it an interesting option to compare the students’ perception of both the video game and solving a mathematical problem, in accordance with Shute et al. [25,26] and Avry et al. [27].

Chorianopoulos and Giannakos [28] highlight the existence of four basic principles in video games that relate them to mathematical knowledge. The following table (

Table 3. Relationship between the basic principles of video games and problem-solving.

Principles	Video Game	Problem-Solving
1st. Hero or heroine. Their story	The video game’s main character is trapped in a futuristic laboratory, controlled by an artificial intelligence called GLaDOS, from which she must escape to save her life.	Statement. Understand the problem, the objectives, and the challenges posed.
2nd. Use of known techniques in the video game	The main character must identify the elements that appear on the screen that can help her pass the level. In order to do so, she can use a portal gun to move objects and open portals in search of items that will allow her to accomplish the mission.	Find known mathematical procedures from the data provided by the statement.
3rd. Involve people in the trial-and-error method.	The completion of different tests forces the main character to open portals in different walls that make up the room to find the evidence.	Find the most appropriate solution to the problem, facilitating its resolution.
4th. Collaborative learning	The video game provides a multiplayer option, with which collaborative learning is developed to pass the different logic games posed.	Analysis and critical-reflective debate that lead to the peers solving the problem amongst themselves.

Source: own elaboration based on the principles of Ref. [28].

) shows the principles and their relationship with the chosen video game, Portal 2, and mathematical problem-solving.

Once the video game had been selected, the students were given a brief presentation on the video game, its context, how to install it, its controls, and the instructions in order to carry out the task correctly. Then, the class was divided into groups of four or five students, and the furniture was rearranged so that the students could work collaboratively. This facilitated both the development of the activity individually and, at a later stage, the sharing of findings and the discussion of relevant questions or doubts that the students had encountered during the activity. This configuration was chosen because group work favours dialogue, critical reflection, and sharing ideas through negotiation. It also allows the teacher to intervene as a dialogue guide or advisor, sharing reflections or doubts with the students and enriching the activity and its result.

The implementation of the task was divided into three parts. The first part consisted of a period of individual free play so that the students could set up the controls to their liking and get used to the dynamics of the game in the first levels, which had a tutorial function. Once the students understood the dynamics of the game, the second part of the task focused on passing the different levels by looking for the procedures required to solve them. At this part, a dialogue was established on various occasions between the students as doubts arose about how to solve the puzzles, the clues, or the handling of the main character. The third part was carried out as a way of closing the activity. At this stage, the students completed a questionnaire that was divided into different blocks. In the first block, descriptive data were collected, such as sex, age, previous studies, if they were a video game player, and the number of hours they spent playing video games. In the second block, they were asked to describe the procedure they followed to pass the different levels. They were asked to describe, step by step, what they had done, what they had looked at, and what decisions they had made in order to solve the problem. In another session, the answers given were analysed and compared with the problem-solving models of Polya and Mason et al., providing an opportunity for the students to analyse and reflect on their findings as a group. Finally, in the last block, they had to express their impressions, feelings, or emotions regarding working with logical–mathematical knowledge in this way. In order to do so, they used a Likert scale and recorded their degree of agreement or disagreement with the statements shown. The statements used in the questionnaire were written according to both the opinions expressed by the students and the objectives set out in this task. The students were also asked to include a brief comment justifying their answer to each of the statements in the questionnaire.

Table 4. Timetable of sessions.

Session	Activity	Duration (Minutes)
Session 0	1. Presentation of the activity	15
	2. Video game installation	20
	3. Video game configuration	5
	4. Free play	30
	5. Sharing of views	20
Session 1	1. Introduction to the session	5
	2. Free play	25
	3. Discussion and analysis of the video game	25
	4. Blocks I and II of the questionnaire	25
Session 2	1. Introduction to the session	5
	2. Presentation of the two problem-solving models	15
	3. Discussion and analysis of the answers given in Block II of Session 1 in terms of the two models	30
	4. Block III of the questionnaire	25
	5. Finishing the activity	5

Source: own elaboration.

, with the distribution of the work during the various sessions conducted, is presented below.

2.3. Instruments

The instrument used was a questionnaire prepared for the study with blocks relating to descriptive data, video game consumption and typology, general knowledge about video games, questions about the activity carried out, and the didactic possibilities of Portal 2 (Figure 1).

Questionnaire: Teach with Portals: This questionnaire has been designed for the students of the bachelor’s degree in early childhood education at the University of Cadiz in order to analyse their experience of using logical –mathematical knowledge when playing the Portal 2 video game.

2.4. Data Analysis

Data collection was carried out following a mixed methodology approach—both quantitative and qualitative—in order to observe the data and thus gain a better understanding of the usefulness of the activity from different perspectives, as indicated by Creswell [29] (p. 18). Qualitative aspects were employed when analysing the problem-solving phases used by the students to pass the levels and comparing them with those indicated by Polya [14] and Mason, Burton, and Stacey [15]. Quantitative aspects were employed when analysing the students’ emotions or feelings towards this logical–mathematical knowledge. Employing both analyses allowed us to provide more in-depth answers to the research questions posed and to fulfil the objectives of this study. Furthermore, to show the validity or internal consistency of our analysis, we carried out a study of the correlations between the different answers our students gave to the statements shown in the third block of the questionnaire. In order to do so, the statistical software Jamovi v.1.8.4 was used.

3. Results and Discussion

The distribution of students by sex shows that the majority of our students were women, 95%, and the rest were men. In terms of their ages, they were between 19 and 24 years old, although there was one 50-year-old student (

Table 5. Distribution of the students in the 2nd year of the bachelor’s degree in early childhood education by sex.

	Frequency	Percentage (%)
Female	161	95
Male	9	5
Total	170	100

Source: own elaboration.

).

One of the questions the students responded to in the questionnaire was related to what Novak and Tassell [30]—citing Stevens and Bavelier (2012)—indicate regarding whether video game players of action games exhibit greater memory, spatial, and geometric skills than non-video game players. These players focus their attention on relevant facts or data and ignore irrelevant information, which is a characteristic that is important when solving mathematical problems. Novak and Tassell [30] also indicate that players improve this characteristic after several hours of gameplay regardless of whether or not the game is an action one. In the case of this study, 46 individuals considered themselves to be video game players. The rest either did not define themselves or did not consider themselves to be video game players because they did not have an established playing routine (

Table 6. Distribution of the students in the 2nd year of the bachelor’s degree in early childhood education according to whether or not they consider themselves to be video game players.

	Female		Male
	Frequency	Percentage (%)	Frequency	Percentage (%)
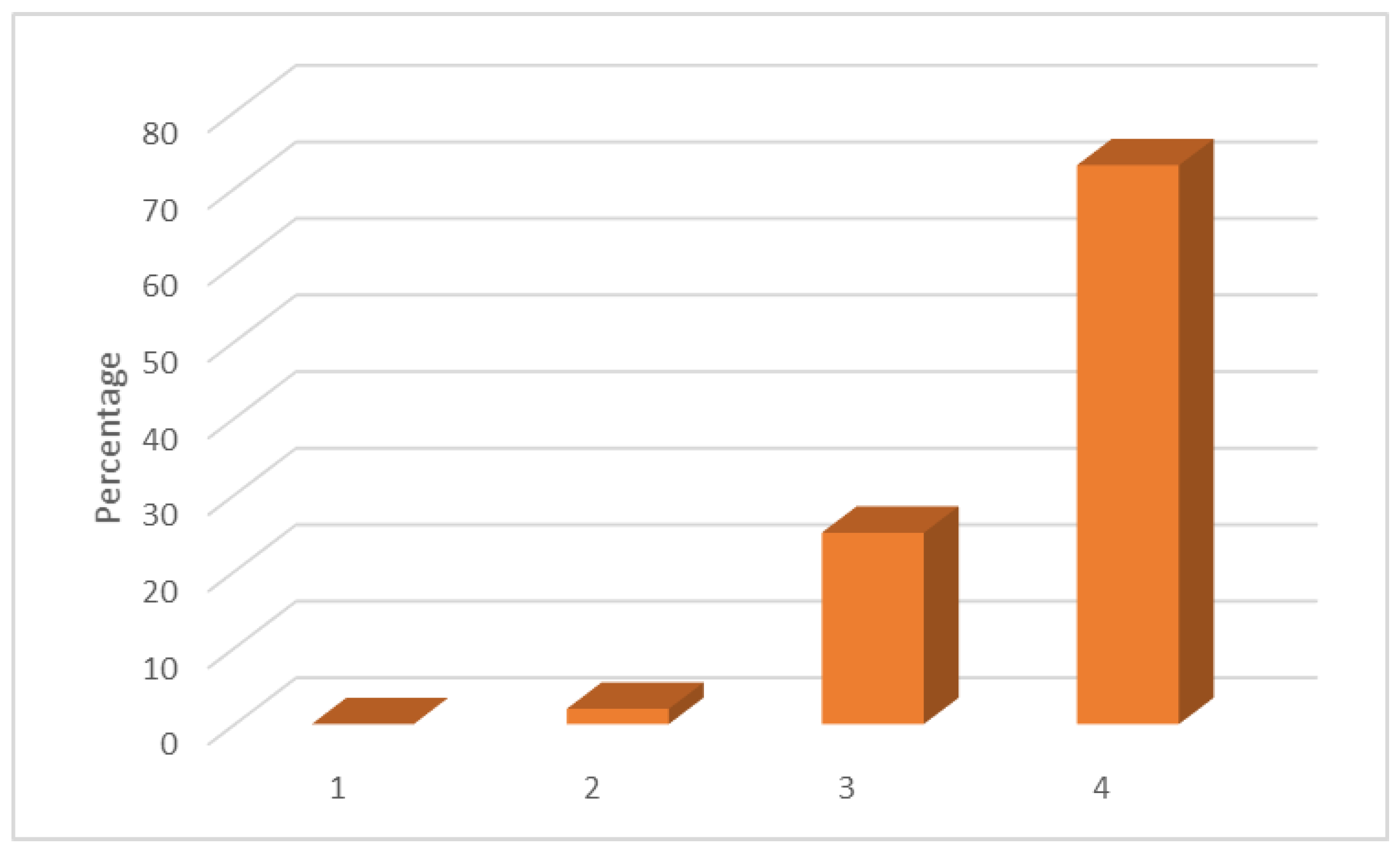
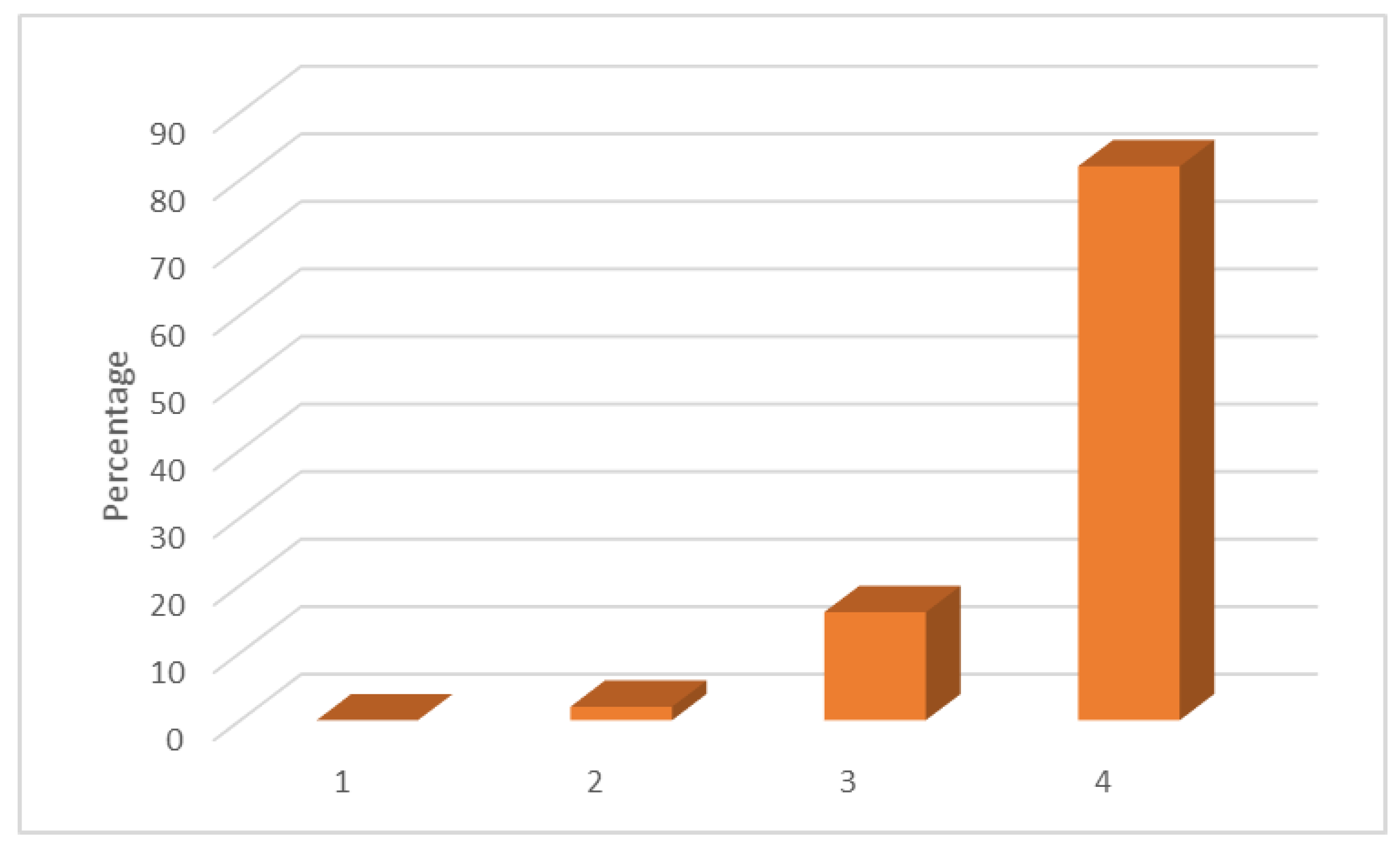
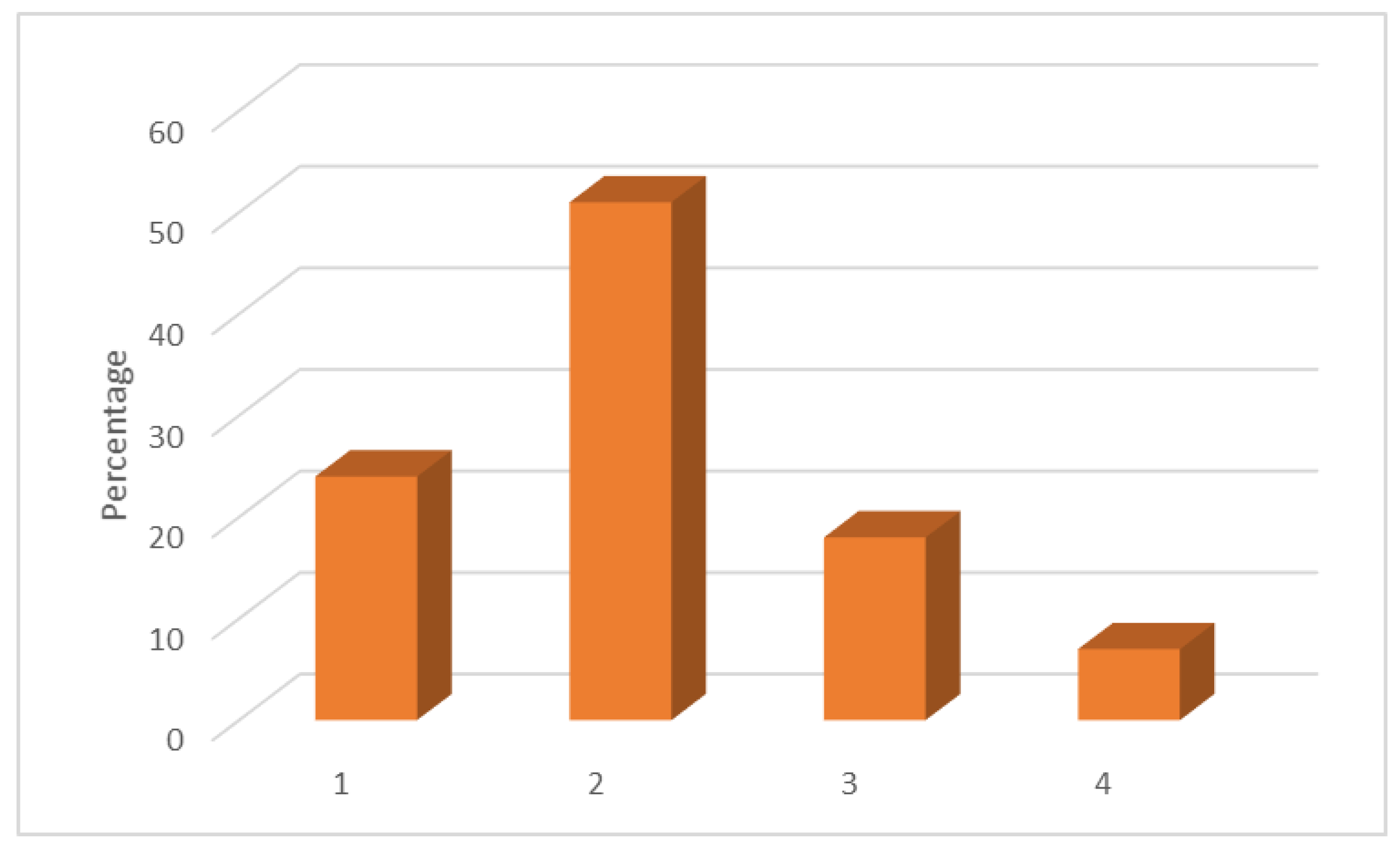
Yes	41	26	5	56
No	114	71	4	44
DK/NR	6	3	0	0
Total	161	100	9	100

Source: own elaboration.

).

In light of this response, one might think that students have the logical–mathematical skills to solve the challenges or problems posed, as Novak and Tassell [30] commented. In the second block of the questionnaire, the students had to reflect on the procedures they followed to pass different levels of the Portal 2 video game. Considering the procedures described by the students, we were able to distinguish or codify three types of players. The first type of player (J1) passes the levels without difficulty, with (J11) or without (J12) requiring external help. The second type of player (J2) is stuck because of not being able to find the clues. This type of player is further divided into two types—those who managed to continue the game despite being stuck (J21) and those who required some kind of help to continue playing (J22). Finally, there are those players who declined to continue playing regardless of whether they refused to continue without help (J31) or with help (J32).

Below are the answers given by our students,

Table 7. Procedures followed by students to pass the level following Polya’s model (1989).

Phase	Explanation
Understanding the problem	“I must leave this level. The objective is to open the exit door. I have to look for the clues on the screen and match them. The clues can be in this room or in the rooms next door.”
Devising a plan	“The clues tell me that I must first perform an action to get to the push button to open the door. I will follow the clues to see what I have to do.”
Carrying out a plan	“I will open the necessary portals to follow the clues. If some portals do not work for me, I can always go back. I have to be careful, as some portals can confuse me when I see the main character moving. In addition, I will move objects that may be in the way or that need to be moved to open the level’s exit door.”
Looking back	“I have managed to open this level’s exit door. I can pass it, but I have opened more portals than necessary. My peers have passed before me with fewer moves. I have learned techniques to move around the level.”

Source: own elaboration.

,

Table 8. Procedures followed by students to pass the level following the model of Mason et al. (1992).

Phase	Explanation
Entry	“I know I have to exit the level through the door that appears and is locked. In order to do this, I have to look at the clues on the walls and objects on the screen. To do this, I can use the portal gun to search for clues or objects that are in other rooms of the level.”
Attack	“When I see the clues, I have to relate them to the actions I have to perform. I will open the necessary portals and move objects to block push buttons. If my decisions are right, I will get close to the exit door, and I will be able to open it. I must check the portals, so I do not get lost in the game.”
Review	“I have passed the level. I was able to match the clues that appeared in the level. I have seen actions that will help me for the following levels.”

Source: own elaboration.

,

Table 9. Procedures followed by students to pass the level following Polya’s model (1989).

Phase	Explanation
Understanding the problem	“I have to open the door that appears on the screen to pass to the next level. There are enough clues in the level to pass. I must follow them.”
Devising a plan	“I must follow the clues and match them. I have to go through the necessary walls or move objects with the portal gun in order to solve the puzzles.”
Carrying out a plan	“I recognised the clues and tried to follow them. I opened portals, but I got lost in them and did not know how to get out. I saw a character running and tried to follow her, thinking it was a clue. I ended up getting disoriented. I had to stop and look at the clues again and realised that the character I was chasing was myself.”
Looking back	“I know I need to find the best place to open the portals so that they do not become more of a problem in the end. I need to look carefully at the clues and think them through before opening a portal or moving an object”.

Source: own elaboration.

,

Table 10. Procedures followed by students to pass the level following the model of Mason et al. (1992).

Phase	Explanation
Entry	“The objective is to open the exit door to pass the level. I have to look at the clues that appear and match them. The portals will help me find objects and new clues.”
Attack	“I had to follow clues and open portals. In some cases, the portals led me to new clues and, in other cases, to twists and turns. I got disoriented. I had to stop playing for a while because I did not understand anything. In some cases, I had to restart the level.”
Review	“I have to check the clues and not open portals for the sake of opening them, as I will eventually lose perspective of the game and not know where I am or what I am doing.”

Source: own elaboration.

,

Table 11. Procedures followed by students to pass the level following Polya’s model (1989).

Phase	Explanation
Understanding the problem	“I have to open the exit door that will allow me to pass the level. To do this, I have to follow some clues, looking for them on the walls, objects, and in other rooms that make up the level.”
Devising a plan	“I have to follow the clues and open the necessary portals to get to the exit door and open it.”
Carrying out a plan	“After opening portals for a while, I do not quite know where I am anymore. I am disoriented and I do not know what to do anymore because I do not even know where the clues are.”
Looking back	(Students do nothing)

Source: own elaboration.

and

Table 12. Procedures followed by students to pass the level following the model of Mason et al. (1992).

Phase	Explanation
Entry	“I must reach the exit door and open it to pass the level. I have to follow the clues that appear in the level.”
Attack	“I opened portals so I could search for clues in the other rooms of the level. At the end, I had many portals opened and I saw someone moving, so to follow her I opened more portals and I did not know how to return. It made me disoriented, I got lost and did not know what to do.”
Review	(Students don’t write anything)

Source: own elaboration.

, analysed from the point of view of the two problem-solving models.

3.1. J11-Type Player. Player Who Does Not Need External Help to Pass a Level

The section below shows the J11- type player, the one who does not need external help to pass a level.

3.2. J21-Type Player. Player Who Is Stuck but Passes the Level without External Help

The section below shows the J21- Type Player, the one who despite being stuck, manages to pass the level without external help.

Two concepts described by Mason et al. [15] appear in this type of player: STUCK! when they start going around in circles opening portals following themselves, and AHA! when they return to solving the problem after getting lost between portals.

3.3. J31-Type Player. Player Who Gets Stuck on a Level and Does Not Continue

The section below presents the J31 type player, the one gets stuck on a level and doesn’t continue.

Responses from the types of students who needed help (J12, J22, or J32) or who relied on their peers to advance in the video game have not been included. The answers they offered were very similar to those presented in Table 7, Table 8, Table 9, Table 10, Table 11 and Table 12, except for the fact that they indicated they required help from their classmates in order to continue to advance in the video game.

Furthermore, by analysing the students’ answers in Table 7, Table 8, Table 9, Table 10, Table 11 and Table 12, we can see not only how the answers conform to the different phases described by Polya [14] and Mason et al. [15] but also how aspects related to mathematical problems appear, such as the statement of the problem, the data that appear, the unknown data, and the possible procedures to link the known and the unknown in order to pass the level, i.e., to overcome the challenge posed. These aspects are in line with the principles indicated by Chorianopoulos and Giannakos [28] that link video games and problem-solving. In the last block of the questionnaire, the students were given a series of statements where they were asked to indicate their degree of agreement (1 = Strongly disagree, 2 = Somewhat disagree, 3 = Somewhat agree, and 4 = Strongly agree) after having played and passed the different levels. The first statements were related to their feelings or emotions towards mathematical knowledge. The following statements were related to the video game and its use with respect to the resolution of mathematical problems. Finally, there were statements related to the emotions experienced during the activity.

The first statement (S1) they had to respond to was: ‘Everything related to mathematical knowledge makes me feel overwhelmed or stressed’. Figure 2 shows that the majority of our students responded ‘Strongly agree’ to the statement (3.71 ± 0.25). This result was linked to the second statement (S2): ‘When I do a task that involves mathematical knowledge, I feel nervous or afraid’. The percentages were very similar in both statements. Figure 3 shows that, once again, the students responded ‘Strongly agree’ (3.81 ± 0.26) to the statement about negative feelings that arise when solving any task involving mathematical knowledge. These behaviours, as Gómez-Chacón [31] indicates—citing different authors—are due to two fundamental aspects: beliefs and emotions; indicating that an important factor is how students learn and use mathematics, or how they see themselves as learners.

The following statements from the questionnaire were related to the video game itself and its relation to problem-solving. The first statement (S3) was: ‘To pass a level of the video game, I must apply the same phases as in problem-solving’. In this case, the students answered mostly ‘Somewhat disagree’ or ‘Somewhat agree’ (2.61 ± 0.17), as can be seen in Figure 4. The students indicated that the main aspect they saw in video games was the recreational aspect or that of diverting from reality, and that they did not think about whether or not the procedures were mathematical when passing a level. The procedures they used were those they knew to be effective in passing the level regardless of the type of game they were playing.

The fourth statement (S4) was: ‘There is a relationship between the situation presented in the video game and solving mathematical problems’. The answers given by the students (Figure 5) show that they do not believe that there is a relationship between playing video games and solving mathematical problems. The students mostly disagreed with the statement, with the most popular response being ‘Somewhat disagree’ (2.08 ± 0.18). Similar to their answer to the previous statement, they justified this by saying that they viewed video games as a distraction to be used for recreational purposes rather than educational purposes. Few students found or justified relationships such as those shown by Chorianopoulos and Giannakos [28]. The students recognised that a problem arose that they had to solve, but it did not correspond to the type of problems they are used to solving in the different educational stages they have gone through.

The final statements that were put forward concerned the emotions or feelings that the students experienced during the game and compared them with those they experienced when solving a mathematical problem. The first of the statements (S5) was related to their emotional state when playing the video game: ‘I felt good when playing the video game’. The majority answered ‘Somewhat agree’ (2.74 ± 0.17), as can be seen in Figure 6. Most of our students found playing the video game to be a pleasant experience that broke from the usual routine of the class. Once again, they highlighted that the recreational aspect of the video game lacked the pressure that accompanies regular classroom activities. However, there was a small number of students that responded ‘Strongly disagree’ to the statement. These students argued that they did not understand the game, that they got disoriented, that they did not manage to pass the level, and that, when they did, it was with the help of their classmates. The argument regarding the disorientation caused by the video game was also put forward by those who answered ‘Somewhat disagree’ as they felt it was easy to get lost and slightly difficult to refocus on the game.

The last statement (S6) was: ‘The emotions I have experienced while playing the video game are the same as those I experience when solving a mathematical problem’. The students mostly disagreed with the statement (1.99 ± 0.19)—which was expected given their responses to the previous statement on the comparison of mathematical problem-solving and passing a level of the video game, as can be seen in Figure 7. Once again, the recreational aspect took precedence over the educational aspect. The students believed that the stress they suffered when carrying out any mathematical activity was not comparable to playing a video game.

Based on the data obtained from the Likert scale for each of the statements, we carried out a correlation analysis on the different statements, shown in Figure 2, Figure 3, Figure 4, Figure 5, Figure 6 and Figure 7. As can be seen in Figure 8, the correlation between statements 1 (S1) and 2 (S2) shows a strong Pearson’s correlation coefficient (0.81, p < 0.001), indicating that the students’ negative feelings towards mathematical knowledge are transferred to any task that involves the use of such knowledge. This result could be justified by students’ opinions such as “I am not good at mathematics” or “I do not like mathematics”. Focusing on the second block of statements related to the use of video games and their relationship to problem-solving (S3 and S4), we also observe a strong Pearson’s correlation coefficient (0.80, p < 0.001). Although both statements S3 and S4 relate video games and problem-solving procedures, when relating them to statements S1 and S2 from the previous block, we discovered that the relationship is no longer direct; instead, we observed an inverse relationship with a negative Pearson’s correlation coefficient (S1 with S3, r2 = −0.36, p < 0.001; S1 with S4, r2 = −0.40, p < 0.001; S2 with S3, r2 = −0.48, p < 0.001, and S2 with S4, r2 = −0.50). As indicated above, the students mainly consider video games to be something fun, separate from mathematics, whose recreational aspect takes precedence over other aspects. Analysing the answers given to the last two statements (S5 and S6), we see that S5 shows a good correlation with S3 (0.55, p < 0.001) and with S4 (0.56, p < 0.001). This exhibits a direct relationship, as would be expected, since the recreational aspect of video games takes precedence over any other aspect, hence the positive emotions they elicit. However, when comparing S5 with S1 (−0.30, p < 0.001) and S2 (−0.35, p < 0.001), we see that there is an inverse relationship as the emotions related to mathematical knowledge are negative, while those related to the use of video games are positive, with the recreational and relaxing aspects of video games taking precedence. Statement 6 (S6), however, exhibits differences to all the previous statements. It presents very weak correlation values with a significance (p-value) greater than 0.1. This could be due to the fact that emotions are highly conditioned by the type of video game chosen and by the interests of the students themselves when playing a video game. Video game choice preferences manifest themselves as more complex relations, according to Ref. [32], and even vary from one time period to another [33]. The possible impact on video game players, their benefits, or their effects on behaviour and emotions must also be considered, as indicated by Ref. [34].

4. Conclusions

Since they became a recreational–cultural element, video games have had a strong presence in people’s daily lives. This means that video games can be used as a medium through which to build didactic experiences, or to be implemented as support tools in the classroom in order to generate learning. Although they were not conceived as a curricular tool, they can be used as a didactic element following a previous treatment and adaptation with respect to the teaching–learning process in which they will be employed.

The objective set out in this study involved aiming to take advantage of the potential provided by video games when analysing whether the techniques or procedures used to overcome a level in a video game are analogous to those used to solve a mathematical problem. We also aimed to analyse whether the situations posed by a video game can be equivalent to those described in a mathematical problem. Based on the results obtained in the answers given by our students, we can state that the students were not sure whether or not they were really using such procedures or whether they are comparable situations. That is, the students were not able to determine their applicability and theoretical transposition to a virtual context and vice versa. However, when describing the procedures they used to pass a level, they conformed to the procedures learned at school. They described in detail each of the phases they went through, which are equivalent to those described for problem-solving in the methods of both Polya [14] and Mason et al. [15]. These seemingly contradictory results lead us to believe that video games are perceived in a purely recreational sense, but the students were not able to discern their didactic potential. Moreover, from their answers, we observed that the feeling of stress or fear that any activity related to mathematical knowledge produces is still present during the problem-solving process.

Our second objective was related to the emotions that students experience when playing a video game and when solving a mathematical problem. We found that, in particular, the Portal 2 video game elicits mixed feelings. We found that there were students who had been challenged, which led them to become more involved in passing the levels despite the different tests and perspectives presented by the video game. That is, it provided extra motivation when facing the proposed challenge. However, other students stated that the movement through the levels of the video game—with recurrent changes of perspective—seemed quite complex to them as they were unable to orient themselves and even felt disoriented.

In conclusion, we can state that the procedures for solving mathematical problems and for passing a level in a video game are the same. However, unlike mathematical activities—which cause students to experience negative feelings—video games promote positive emotions. Video games are considered to be recreational, relaxing, and can provide a means of diverting from academic aspects as they are unrelated to the mathematical knowledge that causes students so much stress or feelings of fear.

The world of video games allows us to take advantage of all their potential for educational purposes by orienting them to work on knowledge that—despite being part of students’ lives—causes them stress and uncertainty when using traditional methodologies and tools. For future lines of research, we could implement the use of video games as a tool to facilitate knowledge by creating a gamified environment in the classroom, as indicated in Ref. [35], in such a way as to encourage students’ commitment and motivation towards mathematical knowledge.

Similarly, taking advantage of video games as a tool for working on logical–mathematical knowledge, we could gain a deeper understanding of the emotions that students experience when faced with logical–mathematical knowledge and whether the use of the video games modifies these feelings.