A Study of Primary Students’ Technology Acceptance and Flow State When Using a Technology-Enhanced Board Game in Mathematics Education

Abstract

Mathematics is a major subject for primary students. However, in mathematics education, many abstract concepts may lead students to lose learning motivation and further affect their learning performance. Therefore, several papers have proposed various board games to support traditional mathematics education. However, teachers have applied traditional board games to support mathematics education in a way that makes it difficult to monitor or capture each student’s learning status and problems during game processes. As mentioned above, this study proposed an educational mathematics board game with mobile and sensor technologies to assist students in learning the concept of prime factorization. The proposed board game can guide students to conduct the game and support teachers to capture the students’ learning status during the game process. To evaluate students’ acceptance and flow state regarding the proposed board game, thirty-nine primary students were invited to participate in an experiment. The experimental results indicated that the students had a high technology acceptance with regard to the proposed board game and high flow state when playing the proposed board game. For high-achieving students, the proposed board game was more entertaining, while low-achieving students might feel a little challenged. In addition, boys were more engaged than girls when playing this board game.

1. Introduction

Mathematics is an important foundation for the development of science, technology, and engineering. Subjects of natural numbers and operations on these numbers which starts to be taught in primary school expand with the concepts of prime numbers, multiples, divisors, common multiples, and common divisors in secondary school [1]. However, students usually have a low learning performance in traditional mathematics classes since the abstract mathematical concepts make them easily confused during the learning process. The learning situation also possibly leads students to learn mathematics with low learning motivation, feeling further frustration [2].

Therefore, several researchers have suggested board-game-based learning approaches to help facilitate a traditional mathematics education [3,4]. It is inferred that board games can situate students in flow state when they are engaged [5]. Moreover, board games usually support more than two students playing together. Previous investigations have also reported that educational board games can enhance the learning interest of students [6,7]. However, during traditional board game processes, students’ learning statuses are very difficult for teachers to monitor [8]. In other words, teachers have difficulty in capturing and analyzing students’ learning problems during and after conducting traditional board games.

In recent years, with the advancement of technology, mobile devices with sensor technologies could play an important role in education [9,10]. In terms of board games, board games with mobile technologies can obviously improve the disadvantages of traditional board games, such as the recording of game play, the immediate guidance or feedback of game play, and so on. Moreover, board games with mobile technologies also retain the advantages of traditional board games, such as the physical interactions with peers. Therefore, technology-enhanced board games are a possible solution to support traditional mathematics education.

As mentioned above, this study proposed an educational mathematics board game with mobile and radio frequency identification (RFID) technologies to assist students in learning and practicing the concept of prime factorization. The proposed board game can guide students to conduct the game and support teachers to monitor and capture the students’ learning status during and after the game process. Before adopting the proposed board game in formal mathematics classes, the literature indicated that it is important to investigate students’ technology acceptance and flow state when using a new technology learning tool in a class [11]. In the educational field, technology acceptance is considered as a prerequisite for teachers and students to adopt technology learning tools to promote learning. Perttula et al. found that flow state is one of the major predictors of an intention to play games in the future [12]. Therefore, the aim of this study is to conduct an experiment for evaluating students’ acceptance and flow state regarding the use of the proposed board game.

2. Literature Review

2.1. Prime Factorization Education

In mathematics education, prime factorization is an important concept to students since it is an essential foundation for them to further learn algebra or cryptography. Prime factorization is related to the concepts of factors, composite numbers, prime numbers, and so on. The concepts with regard to prime factorization are abstract to students who may learn the concepts with poor learning motivation and learning performance. To address the problem, several studies proposed various approaches to enhance students’ learning performance on prime factorization. Russo [13] adopted a board game to engage students in applying the concepts of prime factorization during playing the board game and further practicing the concepts. Oral [14] developed a prime factors chart to teach the concepts of prime factorization. The investigated findings indicated that the prime factors chart positively contributed to the teaching process. Yağmur [15] created a game-based learning activity that can be played for learning the concepts of prime factorization. The research result indicated that the game could support teachers to teach students prime factorization in an effective, enjoying, and enriched learning context.

Although game-based learning approaches can facilitate prime factorization education, Kovalchick and Dawson [16] mentioned some problems with traditional game-based learning. For example, teachers may have to facilitate and guide students to engage in each game. It is difficult to teachers due to there are usually twenty to thirty students in a class. In addition, it is also difficult to teachers to monitor and capture each student’s learning status and misconceptions during and after playing each game [8].

2.2. Flow Theory

Flow theory was firstly introduced by Csikszentmihályi in the 1970s [17]. Flow theory is a mental state attained when a person is fully engaged in an activity and immersed in a feeling of energized focus and enjoyment in the process of the activity. In flow state, people pay full attention to the activity they are engaging in, and their focus of awareness is narrowed down to only that activity [17]. Flow experience refers to the state of complete absorption or engagement in an activity. The state is one of dynamic equilibrium. Entering flow depends on establishing a balance between challenges and skills [17,18]. Massimini and Carli [19] found that when the challenge and skill are both low, apathy rather than flow should ensue.

Froebel [5] pointed out that games can help students get into flow state and present their abilities and knowledge during the game processes. When students are engaged in game-based learning activities, they temporarily forget about anxiety or frustration [20]. The study has shown that game-based learning not only allows students to achieve specific learning goals, but also increases students’ internal motivation to learn mathematics if they get into flow state [21]. Chang, Warden, Liang, and Lin [22] showed that learning achievement and flow exhibit a positive correlation. Moreover, the results indicated that digital game-based learning can enhance flow and learning achievement.

2.3. User Acceptance

The purpose of using educational learning tools in classrooms is to facilitate students’ learning performance. Nevertheless, past studies indicated that before using new learning tools in classrooms, the acceptance of learning tools by students is a necessary consideration for teachers [23,24]. Therefore, understanding the acceptance of educational learning tools by students is a prior step for teachers to conduct meaningful learning activities. In other words, if students do not accept the use of the learning tools, the purpose of using the learning tools in classrooms is difficult to achieve.

In this study, a technology-enhanced board game was proposed to support students in learning prime factorization in games. To understand users’ acceptance of technology-based tools, previous research has mentioned that perceived ease of use and usefulness are critical precursor influencing user acceptance and sustained usage of such tools directly. To measure the two factors, the technology acceptance model (TAM) is a suitable tool. TAM was developed by Davis in 1989 [25], and it contains reliable items to measure perceived ease of use and usefulness scales of users. Furthermore, in game design, fun is a core factor that affects user acceptance of games [26]. Player interaction and competition have been suggested as design elements in games for enhancing fun. An appropriate design of game challenges in games can also help players to have fun [27]. Well-made game mechanics makes a game more fun for players [28]. Chen indicated that game score mechanics and player choice mechanics are suitable for designing games in order to enhance fun [29].

2.4. Board Game in Education

In the past decade, several studies have applied board games to support classroom learning and investigated the impact of board games on students’ learning performance [30,31,32,33]. Cohrssen and Niklas [30] used mathematical board games in preschool settings to support the development of children’s numeracy skills. The research results indicated that children’s mathematical competencies are improved after learning with board games. Nuzulia and Kepirianto [31] applied a board game to improve students’ English dialogue skills. The results pointed out that the approach can reduce students’ language anxiety in dialogue activity. Tsarava, Moeller, and Ninaus [32] adopted a board game to deliver the concepts of basic coding to students and foster their computational thinking ability. Tsai, Chen, Chang, and Liu [33] used a chemical board game to support students to learn chemical knowledge. The investigated results showed that the use of the board game can enhance students’ willingness and learning attitudes. Moreover, the use of the board game can also improve students’ understanding of chemistry concepts.

As noted in the literature, board games are a useful educational tool for teachers to increase students’ engagement in traditional classrooms. However, the literature pointed out that although board games are a possible solution to improve students’ learning engagement, such traditional board games may also lead students to a negative learning state [34,35]. When using traditional board games, teachers have difficulties in observing and guiding every student at the same time [36]. In other words, students could not obtain teachers’ help immediately while encountering problems in games. Cheng and Tsai indicated that teachers’ guidance and assistance significantly enhances students’ learning performance [37]. In addition, teachers cannot monitor and capture every student’s learning status when adopting traditional board games in classes. For teachers, it is important to understand students’ learning status in order to improve the quality of their education [38].

Based on the rationale and purpose of this study, the research questions of this study were proposed as follow.

• What are the students’ perceptions of the technology-enhanced board game in terms of technology acceptance and flow state?
• Will students with low- and high-learning achievements have different technology acceptance and flow state in the technology-enhanced board game?
• Will different genders have different technology acceptance and flow state in the technology-enhanced board game?

3. System Development

3.1. System Architecture

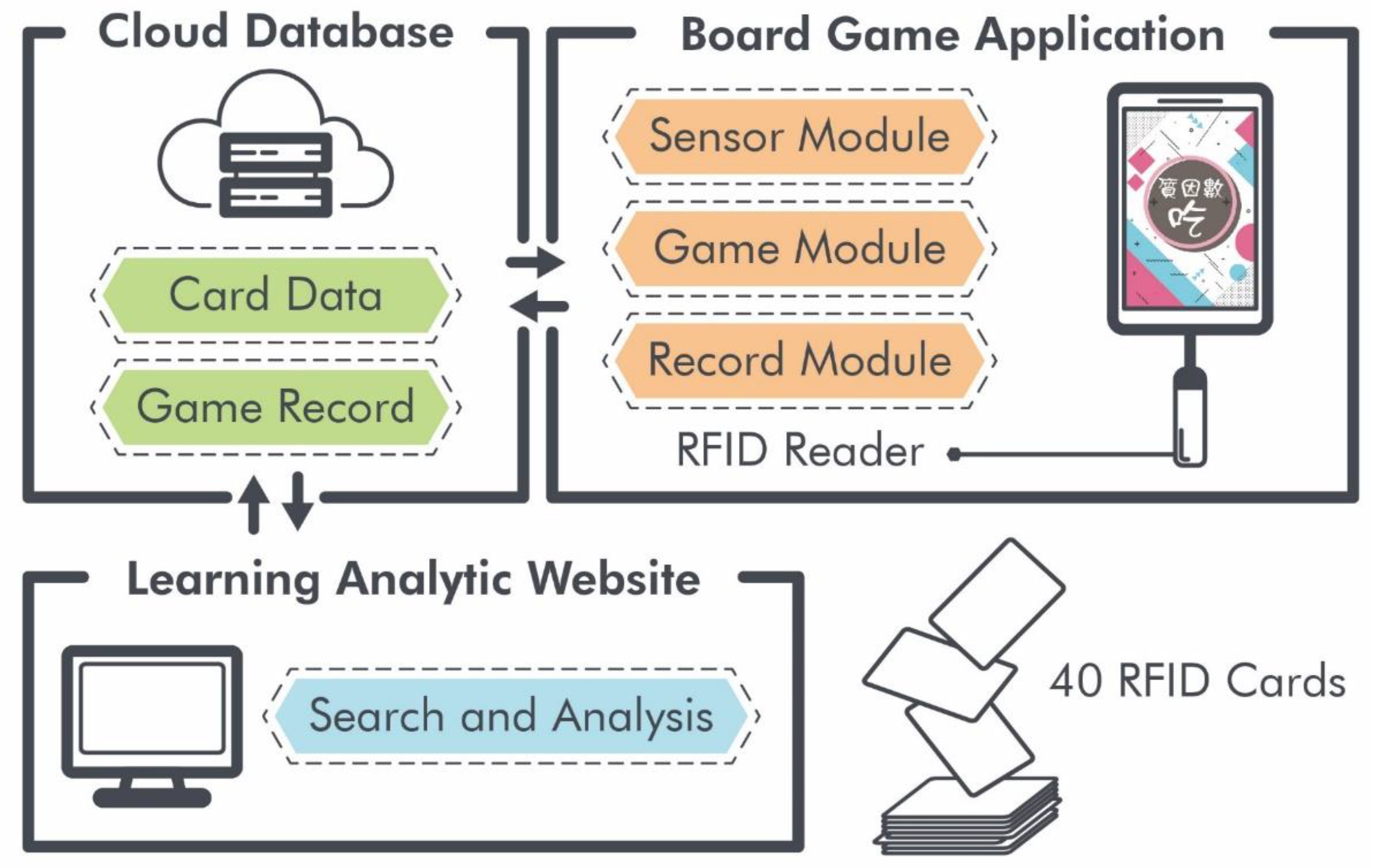
In this study, a technology-enhanced board game was developed and applied to facilitate prime factorization learning in primary mathematics education. The proposed board game is a card game that supports two to three students playing together. The board game is set by a deck of forty RFID cards, a board game application, and a RFID reader. Furthermore, a cloud database was built to work with the board game application for recording game processes derived from each student. In addition, a teacher-side website was deployed for helping teachers to check and analyze students’ game records. The architecture of the proposed board game is shown in Figure 1.

The board game application is a native mobile application which can be installed on a mobile device. The board game application is consisted of a sensor module, a game module, and a record module. The sensor module is used to receive the information of the RFID cards detected by the RFID reader, as illustrated in Figure 2. The game module is designed for guiding students to play the game and refereeing the game for teachers. The record module is used to capture game records and store them to the cloud database.

3.2. System Development

The proposed board game supports two to three students to play together in each game. As shown in Figure 3, each game is set by a deck of forty RFID cards and a mobile device with the board game application and a RFID reader. The play rules of the board game are similar to a card game named Chinese ten, or pick-up red spots.

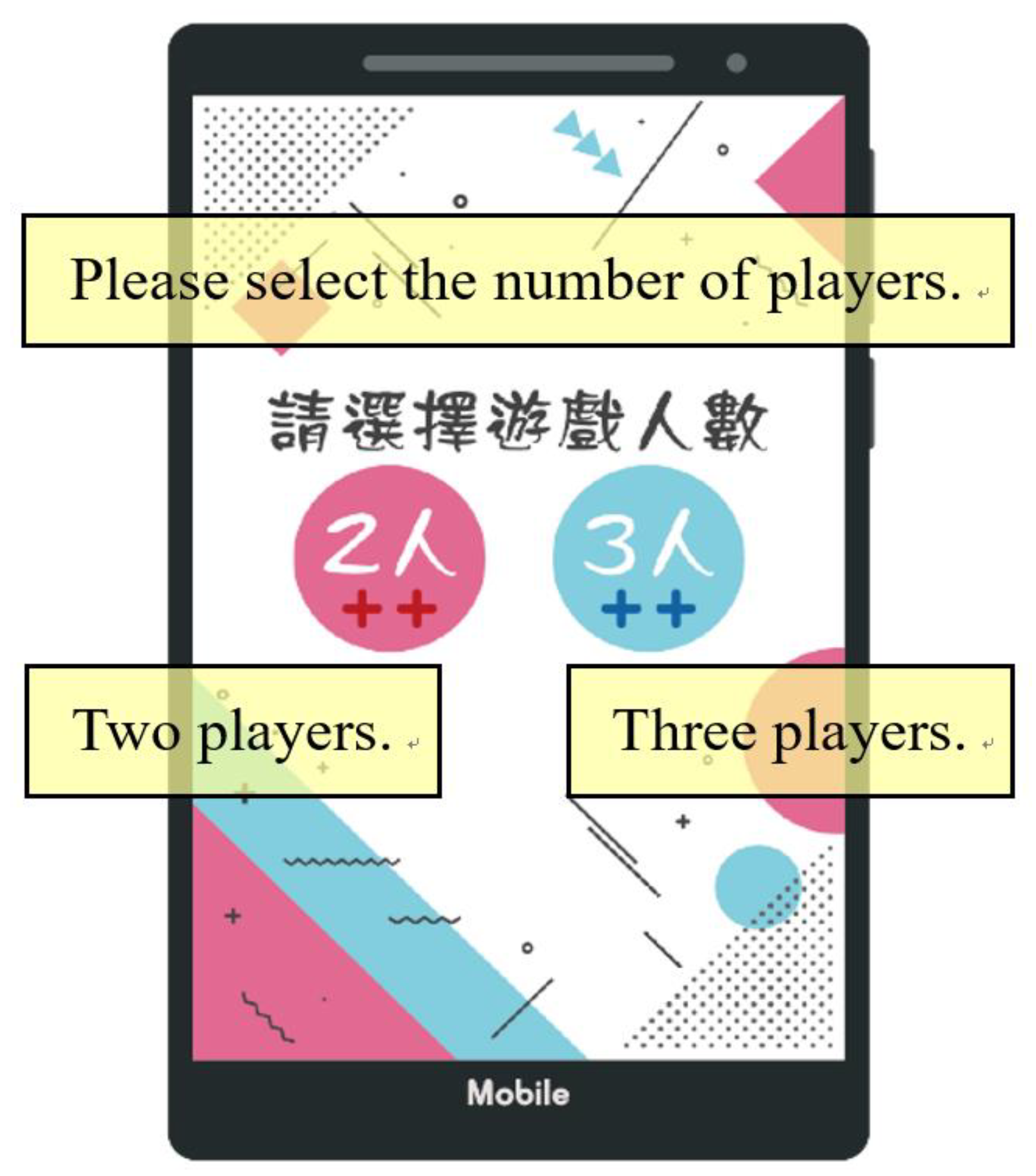
To play the game, students have to initially set the number of players through the board game application, as shown in Figure 4. Following a shuffle of the RFID cards, play begins with each player being dealt six or nine RFID cards depending on whether there are two or three students in the game. Four cards are turned over face up from the remaining deck and placed in a layout. During the game process, students have to play one card from individual hand to capture one card from the layout in rotation. The capture rule is whether the two cards are the same or are a relation between composite number and prime factor. If a capture is successful, the student scores and wins both cards. Otherwise, the student cannot get scores and has to leave a card as a new layout card. Whether the student captured a card successfully or not, the turn passes to the next student. During the game process, students have to use the board game application to detect each card for completing one capture. 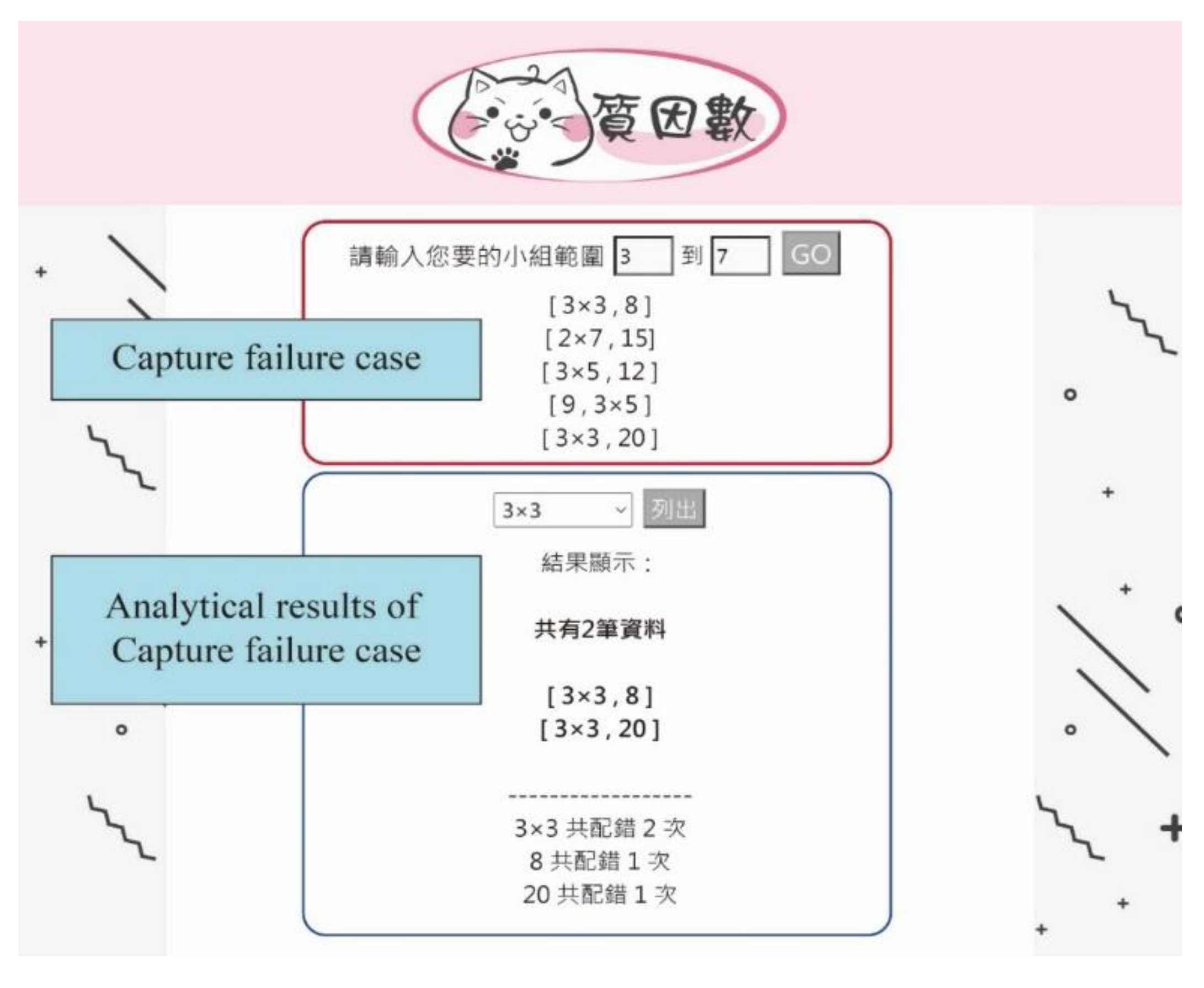In other words, the board game application can assist teachers and students in checking and recording the result of each capture, as show in Figure 5. Finally, teachers can use the teacher-side website to catch students’ failed captures in games in order to analyze students’ misconceptions and conduct remedial teaching, as shown in Figure 6.

4. Experiment

4.1. Procedure

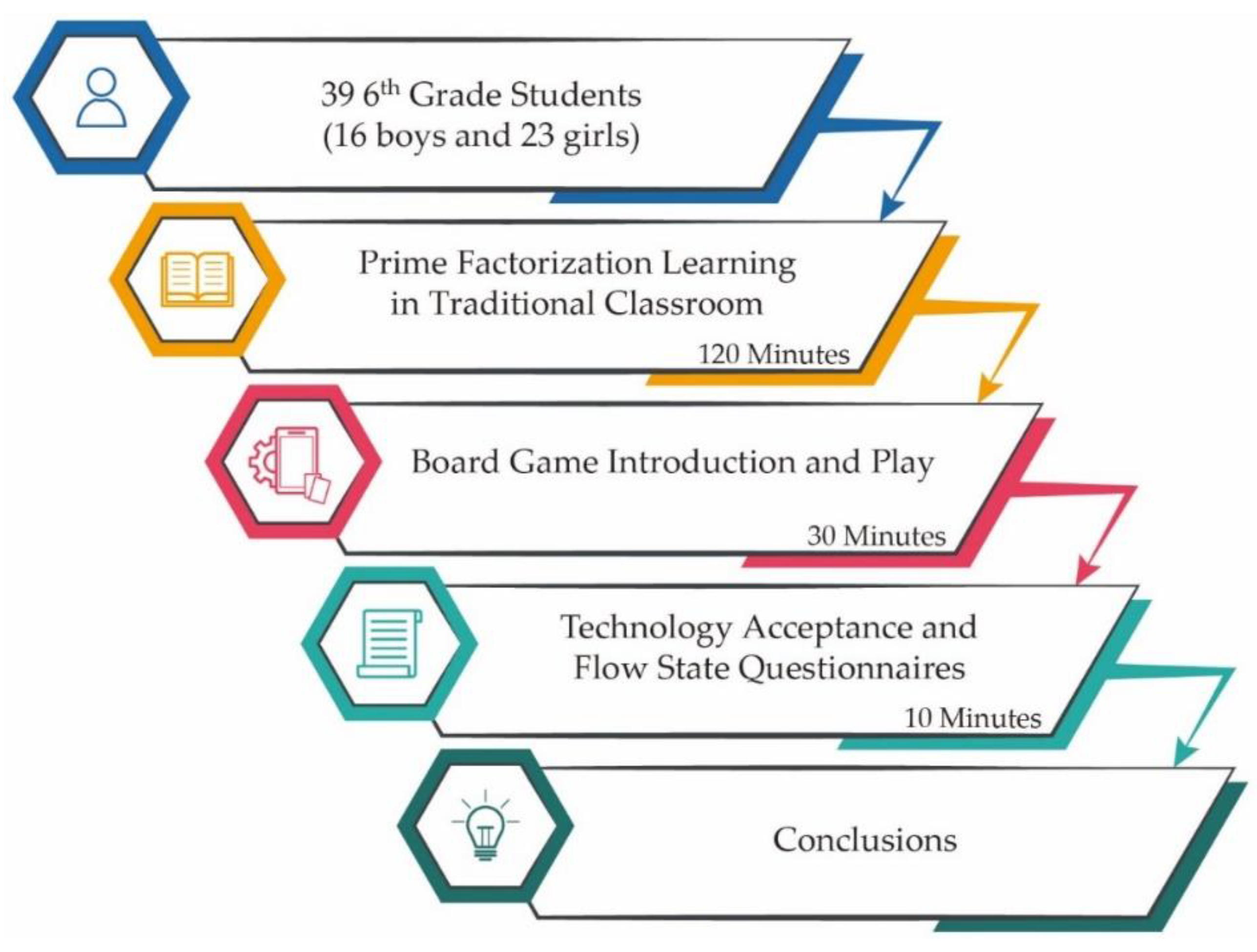
To evaluate students’ technology acceptance and flow state while playing the proposed board game, an experiment was conducted in a primary school, and a teacher and 39 sixth-grade students (16 boys and 23 girls) were invited to participate in the experiment. The procedure of this experiment is described in Figure 7. The experiment had a length of 160 min. In the experiment, the teacher firstly taught prime factorization concepts to the students in three classes comprising 120 min in total. After the prime factorization learning, the teacher used 10 min to explain the rules and operations of the proposed board game to the students. Following that, the students were put into groups of two to three to play the board game. Each game was about 20 min and all students had played at least once. After playing the board game, the students were asked to spend 10 min to take a technology acceptance questionnaire and a flow state questionnaire for measuring their perceptions with regard to the proposed board game.

4.2. Instrument

This study adopted measuring tools including a technology acceptance questionnaire and a flow questionnaire to evaluate the students’ technology acceptance with regard to the technology-enhanced board game and flow state while playing the proposed board game.

The technology acceptance questionnaire contains 16 items in which 12 items were proposed by Davis [25] to evaluate the students’ perceived usefulness and perceived ease of use of the proposed board game. On the other hand, four items were proposed by Chou, Hou, Su, and Chang [39] to measure the students’ perceptions with regards to game elements of the proposed board game from the perspectives of fun, challenge, and game mechanics. The technology acceptance questionnaire was adopted with a five-point Likert rating scale and its Cronbach’s alpha value was 0.961.

The flow questionnaire was developed by Kiili [40]. It consists of 22 items with five-point Likert rating scale ranging from 1 (totally disagree) to 5 (totally agree). The flow questionnaire is divided into two scales which are flow antecedents and flow experience. The scale of flow antecedents is consisted of five sub-scales which are challenge-skill balance, action-awareness, goals of an activity, unambiguous feedback, and control. The scale of flow experience is divided into four sub-scales which are concentration, loss of self-consciousness, the transformation of time, and autotelic experience. The Cronbach’s alpha value of the flow questionnaire was 0.981.

In addition, in order to evaluate the acceptance and flow state of students with high- and low-learning achievements regarding using the proposed board game, each participant’s latest math semester grades, as measured by the primary school, were adopted in this study.

5. Results

In this study, 37 questionnaires completed by the students (15 boys and 22 girls) were collected after the conclusion of the experiment since there were 2 invalid questionnaires. The mean mathematics semester grade of the 37 students was 78.01; the lowest grade was 41.00 and highest grade was 99.33.

5.1. Students’ Perceptions of Technology Acceptance and Game Elements

With regard to the technology acceptance evaluation, the mean and standard deviation of the technology acceptance were 4.48 and 0.58. Moreover, the mean and standard deviation were 4.50 and 0.66 for the perceived usefulness scale, and 4.46 and 0.60 for the perceived ease of use scale. The descriptive statistical results of the technology acceptance showed that the students have positive acceptance to the technology-enhanced board game.

Regarding the game elements of the proposed board game, the mean and standard deviation were 4.46 and 0.66. Furthermore, the mean and standard deviation were 4.59 and 0.72 for the fun scale, 4.53 and 0.79 for the challenge scale, and 4.45 and 0.70 for the game scale. The descriptive statistical results of the game elements indicated that the students felt entertained by the proposed board game.

5.1.1. Analysis of the Technology Acceptance and Game Elements between High and Low Achievers for the Proposed Digital Board Game

To further evaluate the technology acceptance and game elements of the proposed board game by students with different learning achievements, this study used the mean mathematics semester grade (78.01) of the 37 students to divide the students into a high-learning achievement group and a low-learning achievement group. If a student’s mathematics semester grade was higher than 78.01, the student was assigned to the high-learning achievement group. Otherwise, the student was assigned to the low-learning achievement group.

An independent sample t-test was used to analyze the technology acceptance between the high- and low-learning achievement groups. As shown in

Table 1. Analysis of the results of the technology acceptance of high and low achievers by independent sample t-test.

	High Achievers (n = 21)		Low Achievers (n = 16)
	M	SD	M	SD	t(35)	p	Levene’s Test
Technology acceptance	4.49	0.54	4.47	0.64	0.120	0.905	0.319
Perceived usefulness	4.47	0.63	4.56	0.72	−0.377	0.708	0.442
Perceived ease of use	4.52	0.52	4.39	0.70	0.651	0.519	0.293

, the statistical result indicates that the difference in the technology acceptance and each scale between the two groups was not significant. Moreover, the descriptive statistical result reveals that the students of the two groups were able to accept the proposed board game since the mean values of the technology acceptance and each scale were above the median three.

To evaluate the difference in the game elements between the high- and low-learning achievement groups, an independent sample t-test was also used to conduct the analysis. As shown in

Table 2. Analysis of the results of the gameplay of high and low achievers by independent sample t-test.

	High Achievers (n = 21)		Low Achievers (n = 16)
	M	SD	M	SD	t(35)	p	Levene’s Test
Elements of game	4.48	0.64	4.44	0.72	0.351	0.727	0.285
Fun	4.67	0.66	4.50	0.82	0.688	0.496	0.374
Challenge	4.33	0.80	4.38	0.81	−0.157	0.876	0.781
Game mechanics	4.45	0.69	4.44	0.73	0.064	0.950	0.572

, there is no significant difference between the two groups with regards to the game elements and each scale. In addition, the descriptive statistical result showed that the students of both groups felt entertained by the proposed board game, since the mean values of the game elements and each scale were close to five.

5.1.2. Analysis of the Technology Acceptance and Game Elements between Boys and Girls for the Proposed Digital Board Game

In addition to analyzing the differences in the technology acceptance and game elements of the proposed board game between the students with difference learning achievements, this study further conducted the analyses from the view of gender. An independent sample t-test was used to conduct the analyses. As tabulated in

Table 3. Analysis of the results of the technology acceptance by gender by independent sample t-test.

	Boys (n = 15)		Girls (n = 22)
	M	SD	M	SD	t(35)	p	Levene’s Test
Technology acceptance	4.70	0.58	4.32	0.54	2.026	0.050	0.631
Perceived usefulness	4.63	0.69	4.41	0.64	0.978	0.335	0.600
Perceived ease of use	4.78	0.49	4.24	0.58	2.939	0.006 **	0.304

** p < 0.01.

, the difference between boys and girls was not significant from the view of the technology acceptance. However, with regard to the scale of perceived ease of use, the mean and standard deviation were 4.78 and 0.49 responded by the boys, and 4.24 and 0.58 responded by the girls. Moreover, there was a significant difference between boys and the girls (p = 0.006 < 0.01). The result implies that the boys’ perceived ease of use to the proposed board game was higher than the girls.

Furthermore, regarding the evaluation of the game elements, an independent sample t-test was used to analyze the difference between boys and girls. As shown in

Table 4. Analysis of the results of the gameplay by gender by independent sample t-test.

	Boys (n = 15)		Girls (n = 22)
	M	SD	M	SD	t(35)	p	Levene’s Test
Elements of game	4.75	0.57	4.26	0.66	2.334	0.025 *	0.114
Fun	4.87	0.52	4.41	0.80	2.120	0.041 *	0.002
Challenge	4.60	0.83	4.18	0.73	1.617	0.115	0.765
Game mechanics	4.77	0.56	4.23	0.70	2.477	0.018 *	0.055

* p < 0.05.

, there was a significant difference in boys and girls from the view of the game elements (p = 0.025 < 0.05). Moreover, there were also significant differences in the fun scale and game mechanics scale between boys and girls. The above results indicate that the boys had more fun than the girls while playing the proposed board game (p = 0.041 < 0.05). Furthermore, the boys also understood the game mechanics of the proposed board game better than the girls (p = 0.018 < 0.05).

5.2. Flow State

With regard to the flow state evaluation, the mean and standard deviation of the flow state were 4.33 and 0.71. Moreover, the mean and standard deviation were 4.33 and 0.70 for the flow antecedents scale, and 4.30 and 0.75 for the flow experience scale. The descriptive statistical results of the flow state showed that the students have positive engagements while playing the proposed board game.

5.2.1. Analysis of the Flow State between High and Low Achievers

To further evaluate the flow state of the students with different learning achievements while playing the proposed board game, an independent sample t-test was used to analyze the flow state between the high and low learning achievement groups. As shown in

Table 5. Analysis of the results of the flow states of high and low achievers by independent sample t-test.

	High Achievers (n = 21)		Low Achievers (n = 16)
	M	SD	M	SD	t(35)	p	Levene’s Test
Flow state	4.31	0.74	4.34	0.69	−0.125	0.901	0.366
Flow antecedents	4.35	0.71	4.31	0.72	0.175	0.862	0.900
Flow experience	4.26	0.81	4.36	0.67	−0.385	0.702	0.058

, the statistical result indicates that the differences in the flow state and each scale between the two groups were not significant. Moreover, the descriptive statistical result reveals that the students of the two groups were able to engage in the flow state while playing the proposed board game since the mean values of the flow state and each scale were over four.

5.2.2. Analysis of the Flow States between Boys and Girls

In addition to analyzing the difference in the flow state of the students with different learning achievements while playing the proposed board game, this study further conducted the analyses from the view of gender. An independent sample t-test was used to conduct the analyses. As tabulated in

Table 6. Analysis of the results of the flow state of boys and girls playing digital board game by independent sample t-test.

	Boys (n = 15)		Girls (n = 22)
	M	SD	M	SD	t(35)	p	Levene’s Test
Flow state	4.65	0.63	4.10	0.68	2.485	0.018 *	0.353
Flow antecedents	4.69	0.58	4.09	0.68	2.787	0.009 **	0.114
Flow experience	4.61	0.73	4.09	0.69	2.176	0.036*	0.760

* p < 0.05, ** p < 0.01.

, there was a significant difference between boys and girls with regard to the flow state (p = 0.018 < 0.05). Moreover, there were significant differences between boys and girls regarding the scales of flow antecedents (p = 0.009 < 0.01) and flow experience (p = 0.036 < 0.05). The result implies that the boys had better flow state than the girls while playing the proposed board game.

6. Conclusions

This study proposed a technology-enhanced board game to support prime factorization learning in primary mathematical education. Through using mobile and sensor technologies, the proposed board game can guide students to conduct the game and assist teachers in monitoring and capturing the students’ learning status during the game process. To explore students’ perceptions regarding the proposed board game, in this study 39 primary students were invited to play the board game after engaging in traditional prime factorization education, and their technology acceptance and flow state were further investigated. The results of this study indicate that the students had a positive acceptance and flow state with regards to the proposed board game.

In summary, the main contribution of this study is to propose attractive learning tools to assist teachers and students in conducting the practice of prime factorization concepts. In addition, some limitations have to be mentioned. First, as a result of primary school semester considerations in Taiwan, this study did not conduct a random selection to select the students to participate in the experiment. Second, the lack of generalizability is also a limitation of the experimental data as the sample size was not large. Third, the proposed board game can only support two or three students play together. If teachers use the board game in a large-scale class, they have to consider how to set the board game for all student. Fourth, since the rules of proposed board game are similar to Chinese ten, which is familiar to Taiwanese students, the acceptance and flow state evaluated in this study may not be directly transferable to students in other countries. Furthermore, all students participated in the experiment have the experience of playing cards, which may be a reason why the students started faster. However, since the board game rules are simple, students can get started after practicing a few times, even if they have never played a similar board game. Therefore, this study concludes that this problem can be overcome as long as teachers arrange more practice time for students. Future studies can further investigate the correlation between flow and learning gains with regard to the proposed board game. In addition, future studies can consider real-time measurement of students’ flow state instead of post-questionnaire measurement.