Prospective Teachers’ Representations on the Concept of Force
Abstract
:1. Introduction
1.1. Background
1.2. Theoretical Framework from Physics Education Perspective
1.3. Theoretical Framework from General Didactics Perspective
2. Materials and Methods
2.1. The Phases of the Research
2.2. The Investigation Tool
- (A)
- Personal data section
- (B)
- Request section of three “representations that favor the understanding of the concept of force” and, for each of them, a description of the representation and illustration of the didactic and disciplinary motivations on why this representation is effective:
- (C)
- Section of the free “observations and comments”.
2.3. Analysis Methods
- applied vectors representing forces (vectors whose point of application is on one of the systems represented and in which there are clear indications that the arrow actually represents a force and not simply a displacement)
- free vectors (vectors that explicitly represent forces, but which do not have a point of application on a system; vectors which appear more to represent a force field than a force acting on a system, such as the gravitational field)
- arrows (arrows that do not indicate forces, but more often displacements, trajectories).
- Arrows that connect two images that represent different phases of the same situation, that is representing a temporal hierarchy, have not been included in any of the indicated categories.
2.4. The Context of the Research and the Sample
3. Results
3.1. Typology of Situations Represented
3.2. Number of Systems Represented
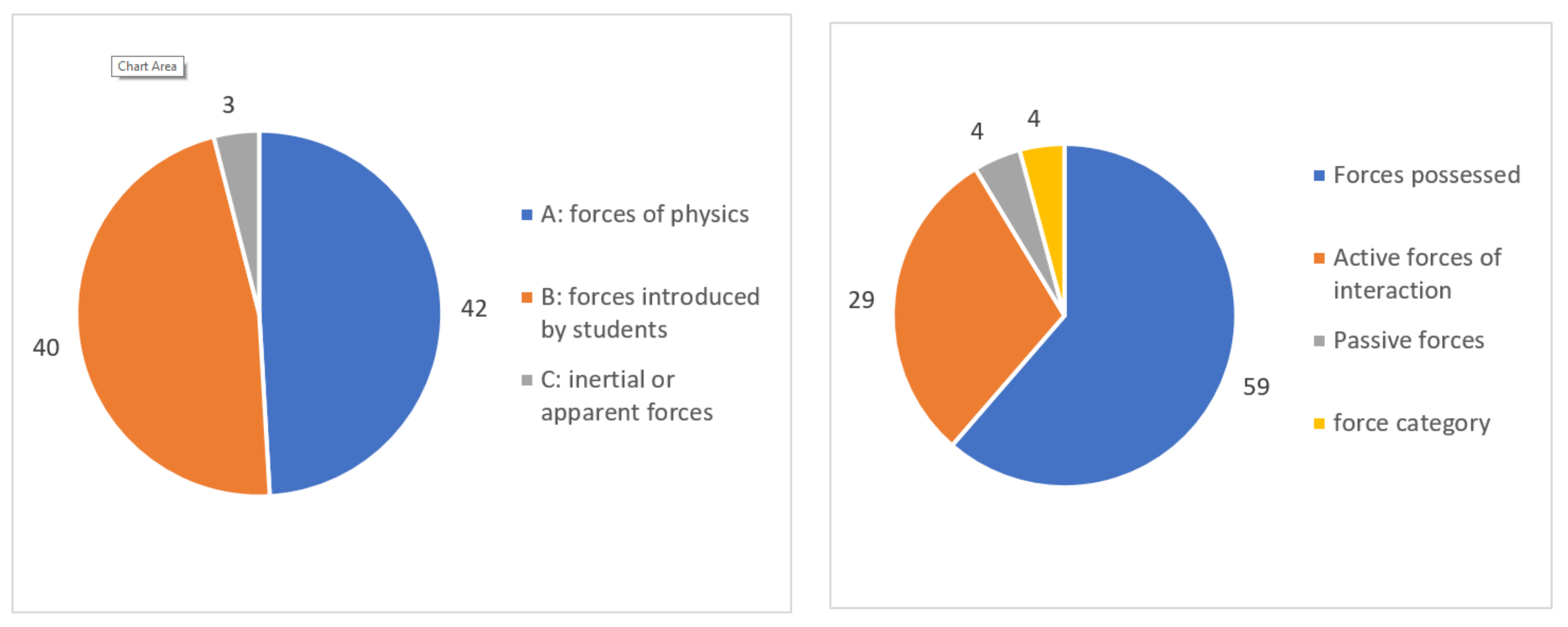
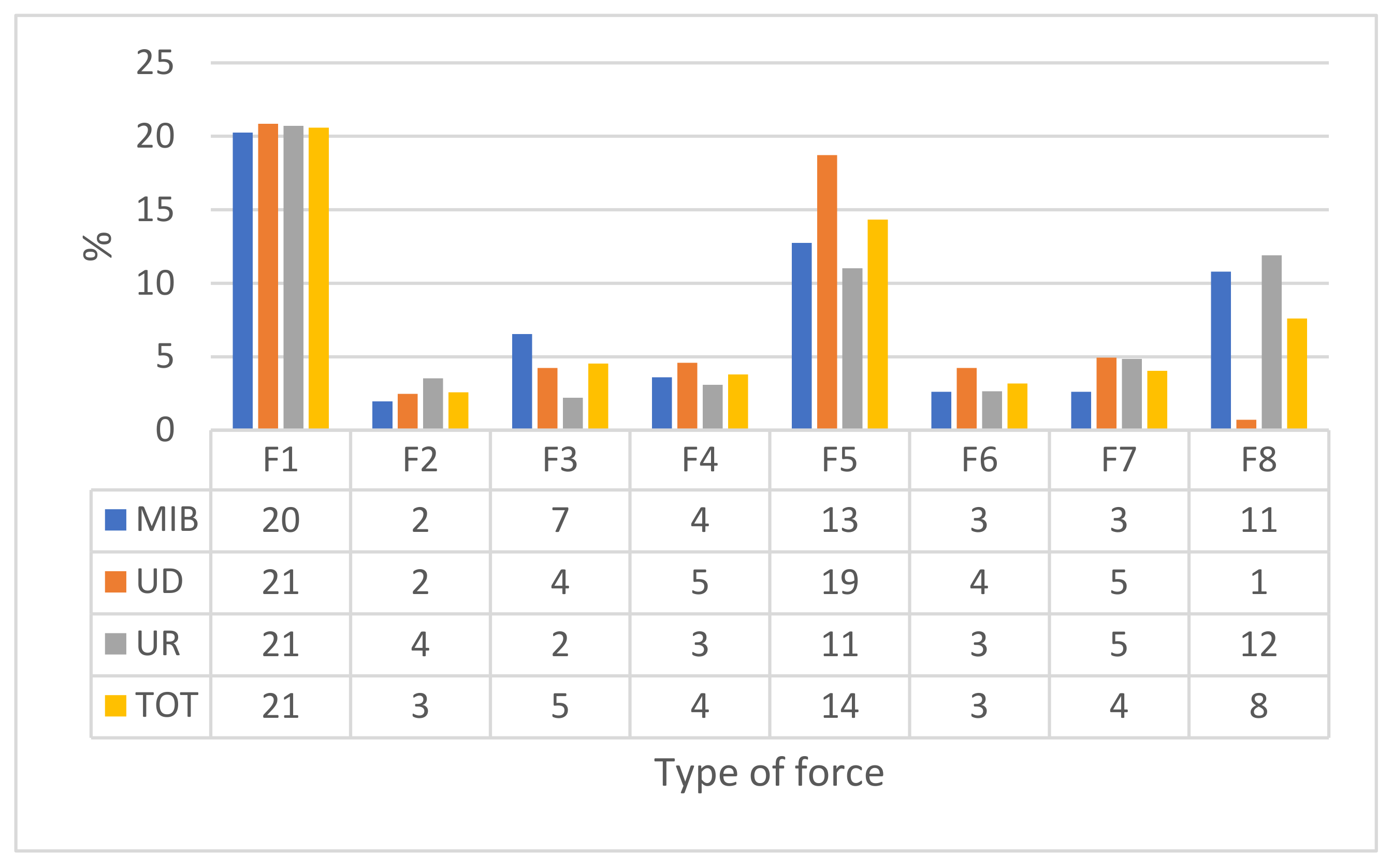
3.3. Types of Force
3.4. Effects of a Force
- -
- “a moving object can exert its own force”
- -
- “example in which forces in a certain sense cause a movement of bodies”
- -
- “in physics to move or push an object a force is applied”
- -
- “to produce a displacement you need a force”
- -
- “the representation [a person lifting an object] allows us to understand that force concerns the displacement and mass of objects. Applying a force therefore implies the displacement of an object that has a certain mass “
- -
- “Highlights that an object that was previously a rest moves as a result of the action of a subject
3.5. Vectors and Forces
3.6. Other Aspects
3.7. Disciplinary Motivation
3.8. Didactic Motivation
4. Discussion
4.1. Discussion on Aspects of Disciplinary Didactics
4.2. Discussion on Aspects of General Didactics
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Form to Collect the Representations on Force

Appendix B
| Founding Nucleus | Conceptual Aspects |
|---|---|
| Force is the formal descriptor of the interaction | Need for two interacting systems (not just one system) Pairs of forces, each acting on one of the two interacting systems (not a single force acting on a single system) |
| Effects of a force on a system | System identification Effect: Deformation—see cases of elasticity Effect: Acceleration—see the three laws of dynamics |
| Center of mass |
|
| Formalization 1: vectorial nature | Representation with an applied vector (originates in a well-defined physical point) |
| Measure of a force in static conditions | Dinamometer: elastic deformation defines the force indicator (operational force def) [dynamic force measurement: no] |
| Formalization 2: composition | Vectorial sum (parallelogram rule) |
| The three principles of dynamics: 1. principle of inertia 2. F = ma 3. Action reaction |
|
| Force measure in dynamical conditions | Inertial mass Inertial balance |
| Formalization 3: application F to a system and Δp (change in quantity of motion): Impulse and change in momentum | Impulse (FΔt) and variation of momentum (Δp): FΔt = Δp
Concepts to reanalyze
|
Types of force:
| Which are indicated and in which contexts (what do they put ?: drawing, graph, formula) Formal expressions
|
Types of forces organization:
| Which are indicated and in which contexts Origin of friction Origin of normal force to the constraint |
| Equilibrium of a body or particles system under static conditions: | Equilibrium of the material point. Equilibrium of forces acting on the same system (v = 0 case) Dynamical equilibrium of forces acting on the same system (v = vo constant case) |
| Equilibrium in static conditions: moment of forces and rigid body | Equilibrated moments (and Forces) acting on the same system Applicazions
|
| Action of a moment of forces and rigid body | Balanced moments (and forces) acting on the same system (no rotations: ω = 0) Unbalanced forces acting on the same system (the system rotates: ω ≠ 0) and we look at the conservation of the angular momentum (see dancer, diver, etc.) |
Appendix C
| Common Sense Conception | References | |
|---|---|---|
| Nature of the Concept of Force | ||
| A1-Force Identified with the action of a system on another at a contact (not at a distance) | [33,34,35,36] | |
| A1.1 Force identified with the action of a system on another at a contact [non reaction] | [33,37,38,39,40,41] | |
| A2. Force as property of a system (force of the wind, force of a person, force of a projectile, weight of a body) | [33,35,42] | |
| A2.1. Force associated to living beings or some machinery | [33,34,43,44,45] | |
| A3. A force produces movement or other changes (explosion, collision) | [33,34,35,43,46,47,48,49,50] | |
| Indicators of the existence of a force—effect produced by a force | ||
| Acceleration deformazione | B1—Displacement, moviment, velocity | [33,34,35,43,46,47,48,49,50,51,52] |
| B2. Weight of an object [indicator of the force needed for displace it] | [43,53,54,55,56,57] | |
| Types of force | ||
| Weight force | C1.1 Weight as property of a body C1.2 On the way up, the push of the hand acts on the descent of the weight C1.3. Weight distinct from gravitational force | [35,53,54,55] |
| Magnetic force | C2.1. Magnetic force acting only on a ferromagnetic object (not on the magnet) | [41] |
| Dipole moment as source | C2.2 Magnetic force as effect of charge | [41] |
| Elastic force | C3. Force of a spring | [54,55,56,57,58] |
References
- Duit, R.; Gropengießer, H.; Kattmann, U.; Komorek, M.; Parchmann, I. The Model of Educational Reconstruction—A Framework for Improving Teaching and Learning Science. In Science Education Research and Practice in Europe. Cultural Perspectives in Science Education; Jorde, D., Dillon, J., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2012; Volume 5. [Google Scholar] [CrossRef]
- Halliday, M.A.K. Language as Social Semiotic. The Social Interpretation of Language and Meaning; Edward Arnold Publishers: London, UK, 1978. [Google Scholar]
- Bailey, J.M.; Slater, T.F. A review of astronomy education research. Astron. Educ. Rev. 2004, 2, 20–45. [Google Scholar] [CrossRef]
- Ojala, J. The third planet. Int. J. Sci. Educ. 1992, 14, 191–200. [Google Scholar] [CrossRef]
- Kikas, E. Pupils’ explanations of seasonal changes: Age differences and the influence of teaching. Br. J. Ed. Psych. 1998, 68, 505–516. [Google Scholar] [CrossRef]
- Mishra, P. The role of abstraction in scientific illustration: Implications for pedagogy. J. Vis. Lit. 1999, 19, 139–158. [Google Scholar] [CrossRef]
- Pena, B.M.; Quilez, M.J.G. The importance of images in astronomy education. Int. J. Sci. Educ. 2001, 23, 1125–1135. [Google Scholar] [CrossRef]
- Vosniadou, S. Instructional considerations in the use of external representations: The distinction between perceptually based depictions, and pictures that represent conceptual models. In Use of Representations in Reasoning and Problem Solving; Verschaffel, L., de Corte, E., de Jong, T., Elen, J., Eds.; Routledge: New York, NY, USA; Abingdon, VA, USA, 2010; pp. 36–54. [Google Scholar] [CrossRef]
- Kress, G.; van Leeuwen, T. Reading Images: The Grammar of Visual Design; Routledge: London, UK; New York, NY, USA, 1996. [Google Scholar]
- Damiano, E. Il Dilemma del Centauro. Stato dell’arte della Ricerca su Didattica Generale e Didattiche Disciplinari; Vita e Pensiero: Milano, Italy, 1996. [Google Scholar]
- Damiano, E. Il Sapere Dell’insegnare; Angeli: Milano, Italy, 2007. [Google Scholar]
- Martini, B. Didattica e didattiche: La dialettica generale/specifico nell’articolazione del campo della didattica. Pedagog. Più Didatt. 2014, 1, 79–87. [Google Scholar]
- Chevallard, Y. Concepts fondamentaux de la didactique: Perspectives apportées par une approche anthropologique. Rech. Didact. Mathématiques 1992, 12, 73–112. [Google Scholar]
- Ligozat, F.; Coquidé, M.; Marlot, C.; Verscheure, I.; Sensevy, G. Didactiques et/ou didactique. Poursuivre le travail de problématisation. Éducation Didact. 2014, 8, 101–116. [Google Scholar] [CrossRef] [Green Version]
- Martinand, J.-L. Point de vue V. Didactique des sciences et Techniques, didactique du curriculum. Éducation Didact. 2014, 8, 65–76. [Google Scholar] [CrossRef]
- Reuter, Y. Didactiques et disciplines: Une relation structurelle. Éducation Didact. 2014, 8, 53–64. [Google Scholar] [CrossRef]
- Vollmer, H.J. Didactique disciplinaire en Allemagne: Développement et perspectives. In Didactique en Construction. Constructions des Didactiques; Dorier, J.-L., Leutenegger, F., Schneuwly, B., Eds.; De Boeck: Bruxelles, Belgium, 2013; pp. 39–62. [Google Scholar]
- Chevallard, Y. La Transposition Didactique. Du Savoir Savant au Savoir Enseigné; La Pensée Sauvage: Grenoble, France, 1991. [Google Scholar]
- Brousseau, G. Théorie des Situations Didactiques; La Pensée Sauvage: Grenoble, France, 1998. [Google Scholar]
- Anning, A. Teachers’ theories about children’s learning. In Teachers’ Professional Learning; Calderhead, J., Ed.; Farmer Press: London, UK, 1988; pp. 128–144. [Google Scholar]
- Antonietti, A. Apprendimento: Che cosa se ne pensa oggi. Scuola Italiana Moderna 1998, 105, 11–13. [Google Scholar]
- Calderhead, J. Teachers: Beliefs and knowledge. In Handbook of Educational Psychology; Berliner, D.C., Calfee, R.C., Eds.; MacMillan: New York, NY, USA, 1996; pp. 88–101. [Google Scholar]
- Putnam, R.T.; Borko, H. Teacher learning: Implications of new views of cognition. In International Handbook of Teachers and Teaching; Biddle, B.J., Good, T.L., Goodson, I.F., Eds.; Kluwer: Dordrecht, The Nederland, 1997; pp. 21–36. [Google Scholar] [CrossRef]
- Antonietti, A.; Liverta Sempio, O.; Marchetti, A.; Pérez-Tello, S. Che cos’è l’apprendimento? Le Concezioni Degli Studenti; Carocci: Roma, Italy, 2005. [Google Scholar]
- Damiano, E. La Mediazione Didattica; Franco Angeli: Milano, Italy, 2016. [Google Scholar]
- Landriscina, F. Modelli di riferimento per l’uso didattico della comunicazione visiva. In Principi di Comunicazione Visiva e Multimediale. Fare Didattica con le Immagini; Calvani, A., Ed.; Carocci: Roma, Italy, 2011; pp. 43–74. [Google Scholar]
- Calvani, A. Principi di Comunicazione Visiva e Multimediale. Fare Didattica con le Immagini; Carocci: Roma, Italy, 2011. [Google Scholar]
- Fischbein, E. The Theory of Figural Concepts. Educ. Stud. Math. 1993, 24, 139–162. [Google Scholar] [CrossRef]
- Shulman, S. Those Who Understand. Educ. Res. 1986, 15, 4–14. [Google Scholar] [CrossRef]
- Shulman, L.S. Knowledge and teaching: Foundations of the new reform. Harv. Educ. Rev. 1987, 57, 1–22. [Google Scholar] [CrossRef]
- Duit, R. Bibliography—STCS. 2009. Available online: https://archiv.ipn.uni-kiel.de/stcse/ (accessed on 31 August 2021).
- Demirci, N. A study about students’ misconceptions in force and motion concepts by incorporating a web-assisted physics program. Turk. Online J. Educ. Technol. TOJET 2005, 4, 40–48. [Google Scholar]
- Halloun, I.A.; Hestenes, D. Common sense concepts about motion. Am. J. Phys. 1985, 53, 1056–1065. [Google Scholar] [CrossRef]
- Minstrell, J. Facets of Students’ Thinking. In Research in Physics Learning: Theoretical Issues and Empirical Studies; Duit, R., Goldberg, F., Niedderer, H., Eds.; IPN Kiel: Kiel, Germany, 1992; pp. 110–128. Available online: https://www.researchgate.net/profile/Hans-Niedderer/publication/330993365_Research_in_Physics_Learning_Theoretical_Issues_and_Empirical_Studies/links/5c5f8abea6fdccb608b40ca2/Research-in-Physics-Learning-Theoretical-Issues-and-Empirical-Studies.pdf#page=110 (accessed on 31 August 2021).
- Ioannides, C.; Vosniadou, S. The changing meanings of force: From coherence to fragmentation. Cogn. Sci. Q. 2002, 2, 5–62. [Google Scholar]
- Watts, M. A study of schoolchildren’s alternative frameworks of the concept of force. Eur. J. Sci. Educ. 1983, 5, 217–230. [Google Scholar] [CrossRef]
- Brown, D.E. Students’ concept of force: The importance of understanding Newton’s third law. Phys. Educ. 1989, 24, 353–358. [Google Scholar] [CrossRef]
- Thornton, R.K.; Sokoloff, D.R. Assessing student learning of Newton’s laws: The Force and Motion Conceptual Evaluation and the Evaluation of Active Learning Laboratory and Lecture Curricula. Am. J. Phys. 1998, 66, 338–352. [Google Scholar] [CrossRef] [Green Version]
- Bao, L.; Hogg, K.; Zollman, D. Model analysis of fine student models: An example with Newton’s third law. Am. J. Phys. 2002, 70, 766–778. [Google Scholar] [CrossRef] [Green Version]
- Kücüközer, A. Evolution of the students’ conceptual understanding in the case of a teaching sequence in mechanics: Concept of interaction. Eurasia J. Math. Sci. Technol. Educ. 2006, 2, 30–40. [Google Scholar] [CrossRef]
- Galili, I. Mechanics background influences students’ conceptions in electromagnetism. Int. J. Sci. Educ. 1995, 17, 371–387. [Google Scholar] [CrossRef]
- Reiner, M.; Slotta, J.D.; Chi, M.T.H.; Resnick, L.B. Naïve physics reasoning: A commitment to substance based conceptions. Cogn. Instr. 2000, 18, 1–34. [Google Scholar] [CrossRef]
- Gilbert, J.; Watts, M. Misconceptions and alternative conceptions: Changing perspectives in science education. Stud. Sci. Educ. 1983, 10, 61–98. [Google Scholar] [CrossRef]
- Kolokotronis, D.; Solomonidou, C. Students’ conceptions about mechanical interaction and design of appropriate educational software for constructive teaching. In Science Education Research, in the knowledge Education Society; Psillos, D., Kariotoglou, P., Tselfes, V., Eds.; Aristothelean University: Thessaloniki, Greece, 2001; pp. 480–482. [Google Scholar]
- Tytler, R.; Darby, L.; Peterson, S. Movement and force. In Teaching Primary Science Constructively; Skamp, K., Ed.; Cengage: Melbourne, Australia, 2012; pp. 99–142. [Google Scholar]
- Viennot, L. Spontaneous reasoning in elementary dynamics. Eur. J. Sci. Educ. 1979, 1, 205–225. [Google Scholar] [CrossRef]
- Clement, J. Students’ preconceptions in introductory mechanics. Am. J. Phys. 1982, 50, 66–71. [Google Scholar] [CrossRef]
- Osborne, R.; Freyberg, P. Learning in Science: The Implications of Children’ Scienc; Heineman: London, UK, 1985. [Google Scholar]
- Chee, C.T. Misconceptions concerning laws of motion, frictional force and work done among gifted, above-average and average achieving students at upper secondary level. In Proceedings of the Workshop on Research for Students’ Conceptual Structures and Changes in Learning Physics; Pak, S., Ed.; National University: Seoul, Korea, 1988; pp. 165–190. [Google Scholar]
- Chee, C.T. Common Misconceptions in Frictional Force among University Physics Students. Teach. Learn. 1996, 16, 107–116. [Google Scholar]
- Bryce, T.; Mac Millan, K. Encouraging conceptual change: The use of bridging analogies in the teaching of action-reaction forces and the ‘at rest’ condition in physics. Int. J. Sci. Educ. 2005, 27, 737–763. [Google Scholar] [CrossRef]
- Low, D.; Wilson, K. Weight, the Normal force and Newton’s Third Law: Dislodging a deeply embedded misconception. J. Aust. Sci. Teach. Assoc. 2017, 63, 17–26. [Google Scholar]
- DiSessa, A. Phenomenology and the evolution of intuition. In Mental Models; Gentner, D., Stevens, A.L., Eds.; Erlbaum: Hillsdale, NJ, USA, 1983; pp. 15–33. [Google Scholar]
- Trumper, R. A longitudinal study of physics students’ conceptions of force in pre-service training for high school teachers. In Research in Science Education-Past, Present, and Future; Komorek, M., Behrendt, H., Dahncke, H., Duit, R., Graeber, W., Kross, A., Eds.; IPN Kiel: Kiel, Germany, 1999; Volume 1, pp. 253–255. [Google Scholar]
- Trumper, R.; Gorsky, P. A cross-college age study about physics students’ conceptions of force in pre-service training for high school teachers. Phys. Educ. 1996, 31, 227–235. [Google Scholar] [CrossRef]
- Bliss, J.; Ogborn, J. Force and motion from the beginning. Learn. Instr. 1994, 4, 7–25. [Google Scholar] [CrossRef]
- DiSessa, A. How should we go about attributing knowledge to students? In Research on Physics Education; Redish, E.F., Vicentini, M., Eds.; Società Italiana di Fisica: Bologna, Italy, 2004; pp. 117–135. [Google Scholar]
- Hestenes, D.; Wells, M.; Swackhamer, G. Force concept inventory. Phys. Teach. 1992, 30, 141–166. [Google Scholar] [CrossRef]



| A4.1—A single isolated stationary or in motion system (without any force) | A4.4 Compound systems (i.e., systems that require the use of the rigid body model) |
| A4.1.1—A single system and a row representing a force acting on it | |
| A4.2—Two interacting systems, without representation of forces | A4.5 Situations involving forces produced by continuous systems (fluids) |
| A4.2.1—Two systems with only a force acting only on one of the two | |
| A4.3—Two systems in which the representation of both acting forces is explicit | A4.6 Metaphor (imagine representing a metaphorical use of of the word force) |
| Types of Force | (A) | (B) | (C) | * | Δ | • | // |
|---|---|---|---|---|---|---|---|
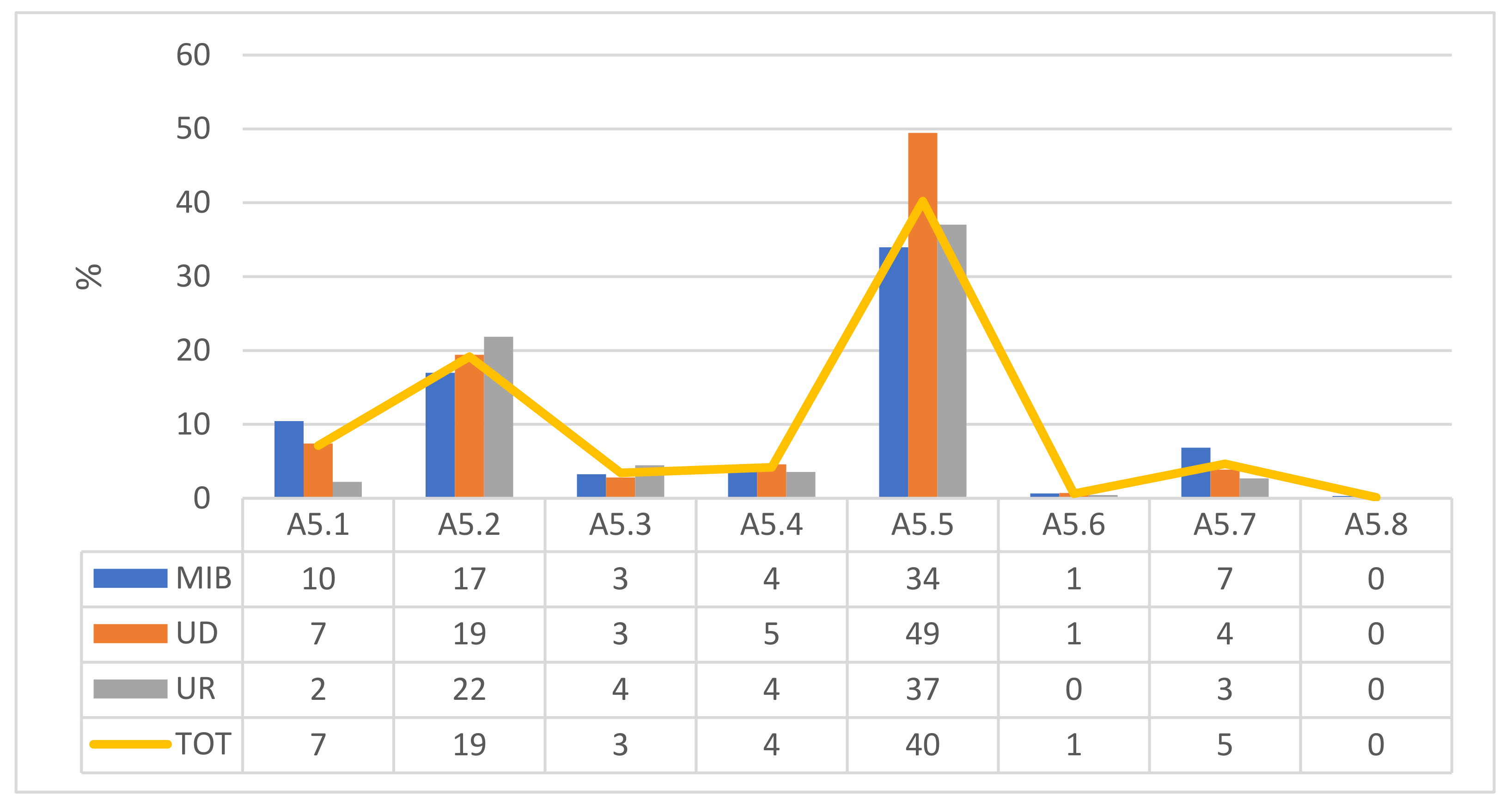
| A5.1 Weight f. A5.2 Gravitazional f. A5.3 Elastic f. A5.4 Sliding, volvent, viscous friction f. A5.5 Contact f. (push/pull) A5.6 Elettric f. A5.7 Magnetic f. A5.8 Nuclear f. A5.9 Archimede/hydrostatic f. A5.10 Centrifugal f. 5.11 Centripetal f. A5.12 Apparent/fictitious/inertial f. A5.13 Muscular/physical f.—physical effort. A5.14 F. possessed by an object/system/entity | (A) (A) (A) (A) (A) (A) (A) (A) (A) ° | (B) (B) (B) | (C) (C) | * * * * * * * | Δ Δ | • • • • | // // // |
| University | Images | Students |
|---|---|---|
| MIB | nMIB = 306 | NMBI = 102 |
| UD | nUD =283 | NUD = 95 |
| UR | nUR = 227 | NUR = 77 |
| TOT | n = 816 | N = 274 |
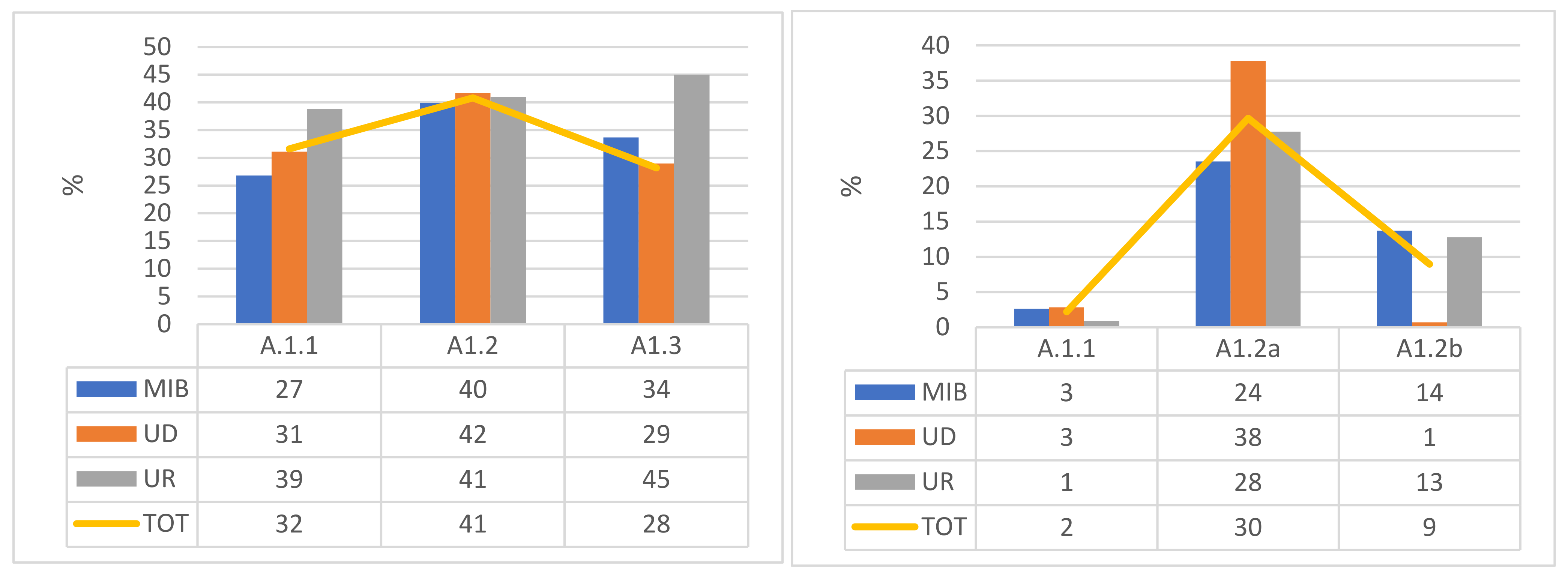
| A1.1 | A1.2 Concept Situations | A1.3 | ||||
|---|---|---|---|---|---|---|
| University | Common Situation/Events Sequences | (a) Model System (i.e., Solar System) | (b) Common Situation with Abstract Iconic Elements (i.e., Arrows, Formula) | (c) Metaphor System | Tot | Situation with Specific Formal Symbols (Forces Vectors) |
| MIB | 82 | 8 | 72 | 42 | 122 | 103 |
| UD | 88 | 8 | 107 | 2 | 118 | 82 |
| UR | 85 | 2 | 63 | 28 | 92 | 45 |
| TOT | 255 | 18 | 242 | 72 | 332 | 230 |
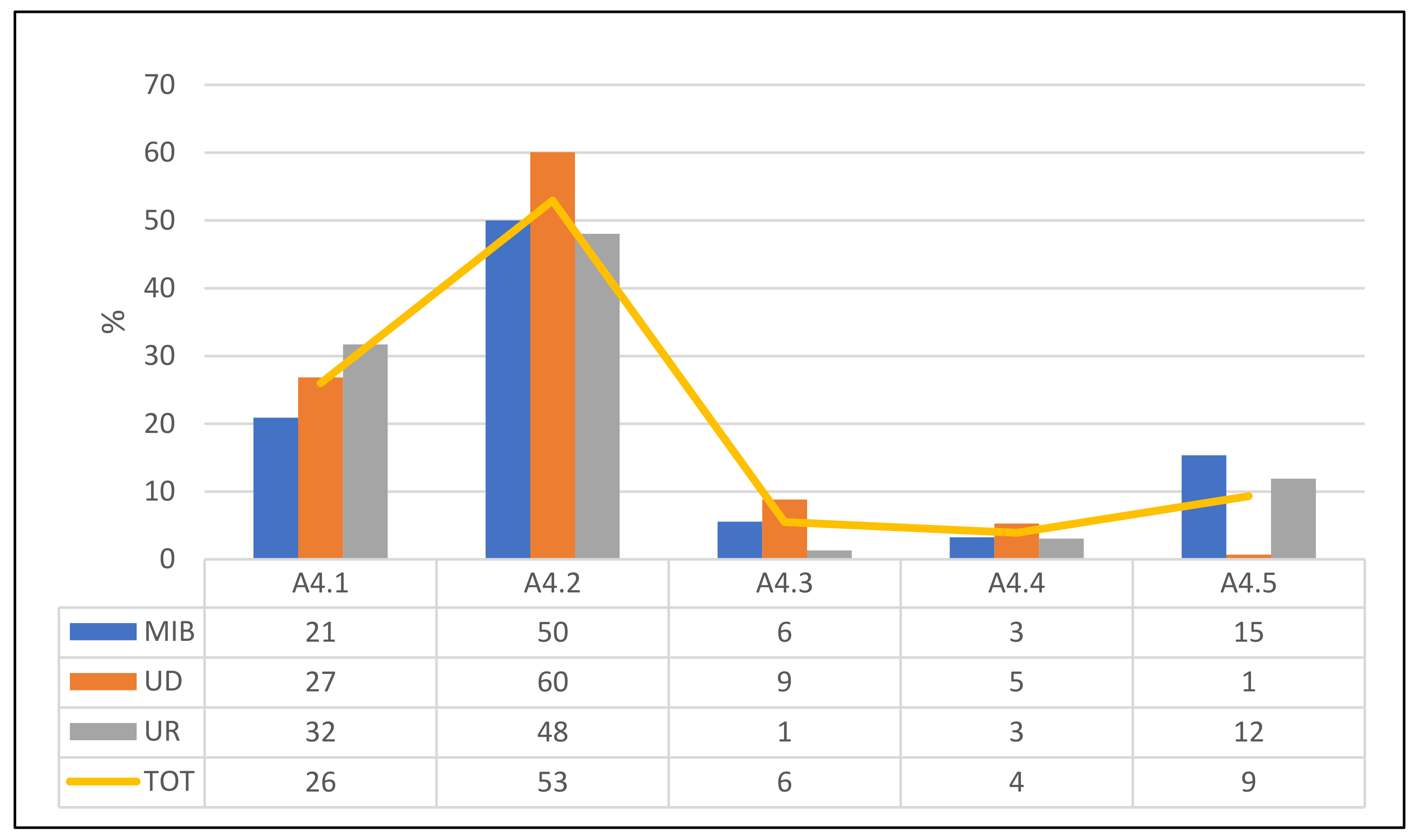
| Category | MIB | UD | UR | Tot | ||||
|---|---|---|---|---|---|---|---|---|
| N | % | N | % | N | % | N | % | |
| A4.1—A single isolated system | 47 | 15 | 49 | 17 | 63 | 28 | 159 | 26 |
| A4.1.1—A single isolated system and one or more forces | 17 | 6 | 27 | 10 | 9 | 4 | 53 | 6 |
| A4.2—Two interacting systems | 153 | 52 | 170 | 61 | 109 | 47 | 422 | 53 |
| A4.2.1—Two interacting systems (without force) | 108 | 38 | 129 | 46 | 87 | 38 | 324 | 40 |
| A4.2.2—Two interacting systems and a force | 32 | 10 | 27 | 10 | 10 | 4 | 69 | 8 |
| A4.2.3—Two interacting systems and two forces | 13 | 4 | 14 | 5 | 12 | 5 | 39 | 5 |
| A4.3 Compound systems (rigid body) | 17 | 6 | 25 | 9 | 3 | 1 | 45 | 6 |
| A4.4 continuous systema (fluids) | 10 | 3 | 15 | 5 | 7 | 3 | 32 | 4 |
| A4.5 Metaphoric representation | 47 | 15 | 2 | 1 | 27 | 12 | 76 | 9 |
| % (N = 321) | A6.1 Deformation | A6.2 Acceleration | A6.3 Displacement Movement | A6.4 Other Effects |
|---|---|---|---|---|
| MIB | 2 | 2 | 10 | 2 |
| UD | 6 | 2 | 53 | 7 |
| UR | 1 | 2 | 12 | 1 |
| TOT | 10 | 5 | 75 | 10 |
| % (N = 394) | A7.1 Applied Vectors | A7.2—Free Vectors | A7.3—Arrows |
|---|---|---|---|
| MIB | 9 | 15 | 13 |
| UD | 7 | 14 | 22 |
| UR | 4 | 7 | 9 |
| TOT | 20 | 36 | 44 |
| % (N = 813) | A9—Forces Composition | A10—Connection Force -Momentum | A11—Moment of a Force | A12—Measure of a Force | A13—Formulas | A14. Graphs |
|---|---|---|---|---|---|---|
| MIB | 8 | 0 | 0 | 1 | 1 | 0 |
| UD | 11 | 0 | 0 | 5 | 2 | 1 |
| UR | 2 | 0 | 0 | 0 | 1 | 0 |
| TOT | 7.3 | 0 | 0.2 | 2.1 | 1.5 | 0.4 |
| A15.1 Energy/Work | A15.2 Power | A15. 3 Magnetic Field | |
|---|---|---|---|
| MIB | 4 | 4 | 1 |
| UD | 9 | 0 | 0 |
| UR | 3 | 3 | 0 |
| TOT | 16 | 7 | 1 |
| Category | MIB (n) | UD(n) | UR (n) | TOT (n) |
|---|---|---|---|---|
| Force as interaction | 4 | 3 | 7 | 17 |
| Force as action (of a body on another one) | 11 | 11 | 9 | 31 |
| Force as transmission of something | 4 | 2 | 6 | |
| Connection force—movement | 13 | 53 | 22 | 88 |
| To put in motion a body is needed a force | 1 | 6 | 8 | 15 |
| Connection force acceleration | 5 | 6 | 3 | 14 |
| Recognition of the existence of a forca | 3 | 25 | 3 | 31 |
| Recognition of the application of a force | 1 | 1 | 5 | 7 |
| Cat | Subcat | MIB | UD | UR | TOT | % TOT |
|---|---|---|---|---|---|---|
| Aspects correlated to the vectorial nature of force | 7 | 57 | 3 | 67 | 8 | |
| Intensity | 9 | 48 | 22 | 79 | 10 | |
| Direction | 7 | 18 | 3 | 28 | 4 | |
| Versus | 2 | 7 | 5 | 14 | 2 | |
| Composition-Sum of forces | 10 | 19 | 12 | 61 | 8 | |
| Equilibrium | 12 | 11 | 10 | 33 | 4 | |
| Disequilibrium | 17 | 88 | 16 | 121 | 15 | |
| Effects of forces | ||||||
| Displacement/movement | 17 | 88 | 16 | 121 | 15 | |
| Effect of a force as an evaluation of its intensity | 1 | 7 | 4 | 12 | 2 | |
| Property of force (Attraction/repulsion) | 11 | 19 | 13 | 43 | 6 | |
| Comprehension that to an action correspond a reaction | 1 | 0 | 2 | 3 | ||
| Coexistence of multiple forces | 3 | 8 | 2 | 13 | ||
| Distinction weight–mass | 2 | 3 | 1 | 6 | ||
| Existence of attractive and repulsive forces | 1 | 3 | 4 | |||
| Role of mass in the force | 27 | 157 | 25 | 209 | 26 | |
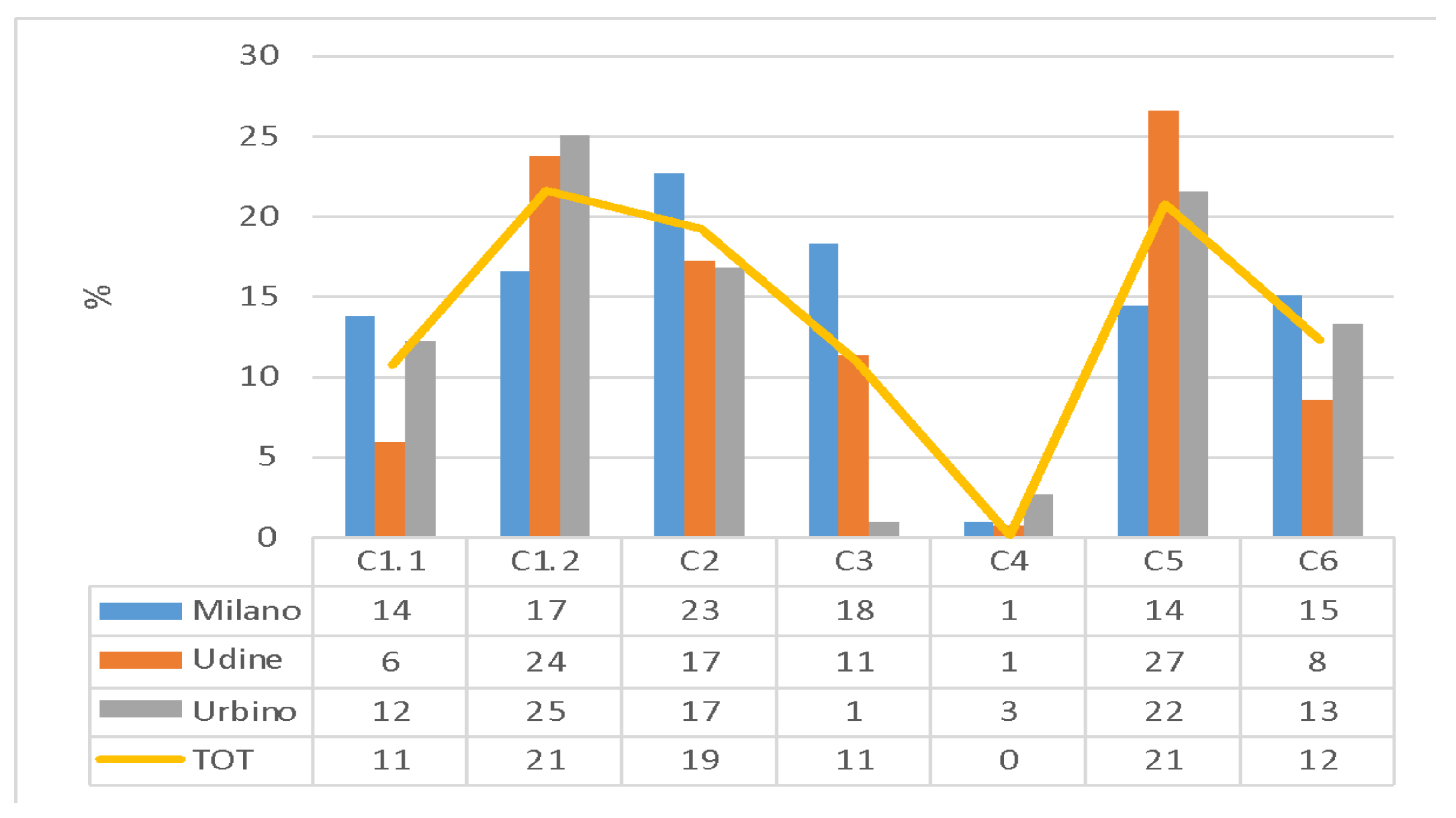
| C1.1 | C1.2 | C2 | C3 | C4 | C5 | C6 | TOT | |
|---|---|---|---|---|---|---|---|---|
| MIB | 42 | 51 | 69 | 56 | 3 | 44 | 46 | 311 |
| UD | 17 | 67 | 49 | 32 | 2 | 75 | 24 | 266 |
| UR | 28 | 57 | 38 | 2 | 6 | 49 | 30 | 210 |
| TOT | 87 | 175 | 156 | 90 | 11 | 168 | 100 | 787 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martini, B.; Michelini, M.; Stefanel, A.; Tombolato, M. Prospective Teachers’ Representations on the Concept of Force. Educ. Sci. 2021, 11, 614. https://doi.org/10.3390/educsci11100614
Martini B, Michelini M, Stefanel A, Tombolato M. Prospective Teachers’ Representations on the Concept of Force. Education Sciences. 2021; 11(10):614. https://doi.org/10.3390/educsci11100614
Chicago/Turabian StyleMartini, Berta, Marisa Michelini, Alberto Stefanel, and Monica Tombolato. 2021. "Prospective Teachers’ Representations on the Concept of Force" Education Sciences 11, no. 10: 614. https://doi.org/10.3390/educsci11100614
APA StyleMartini, B., Michelini, M., Stefanel, A., & Tombolato, M. (2021). Prospective Teachers’ Representations on the Concept of Force. Education Sciences, 11(10), 614. https://doi.org/10.3390/educsci11100614






