Magnetic Resonance Lithography with Nanometer Resolution
Abstract
:1. Introduction
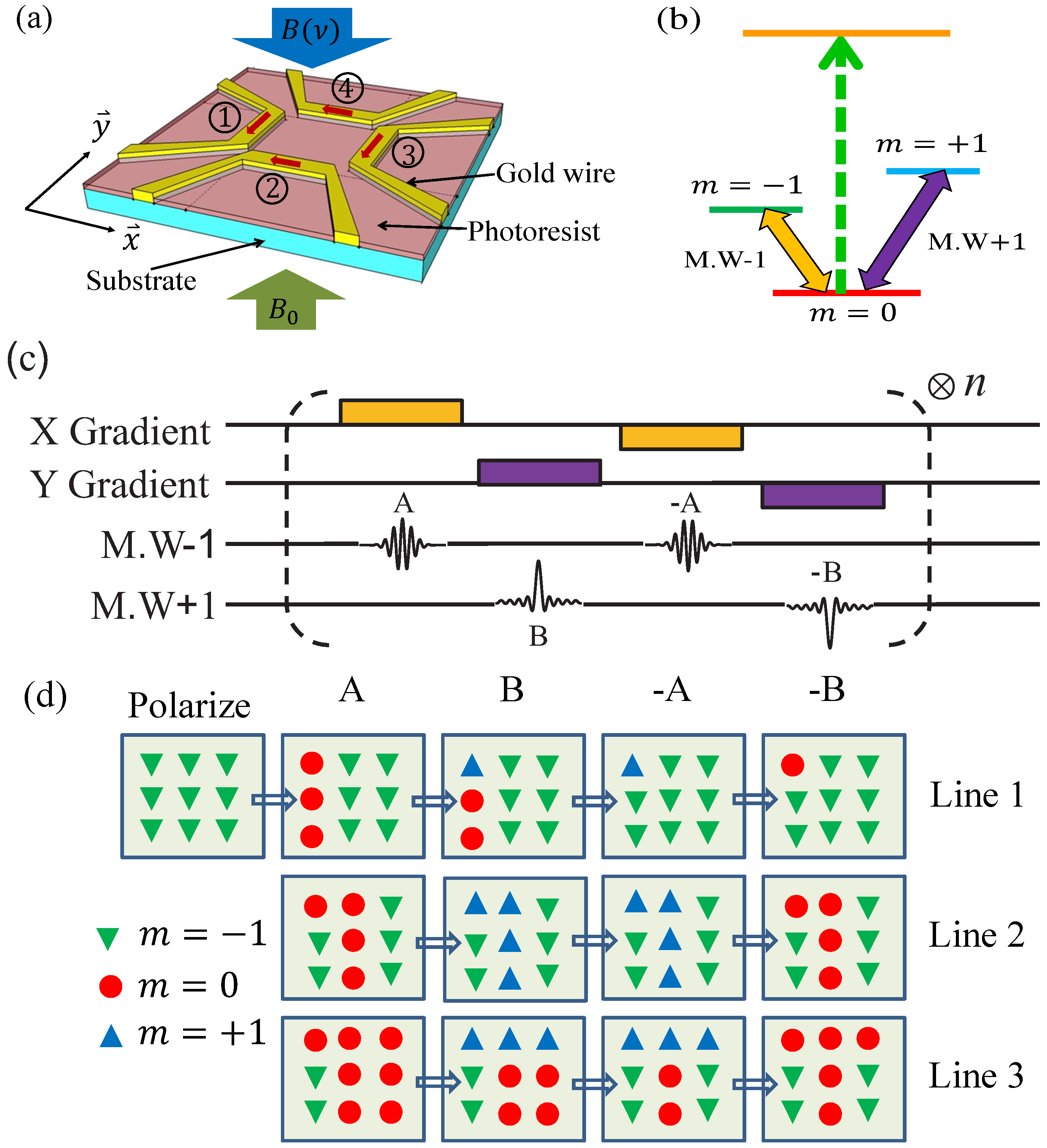
2. Basic Principle and Proposed Experiment
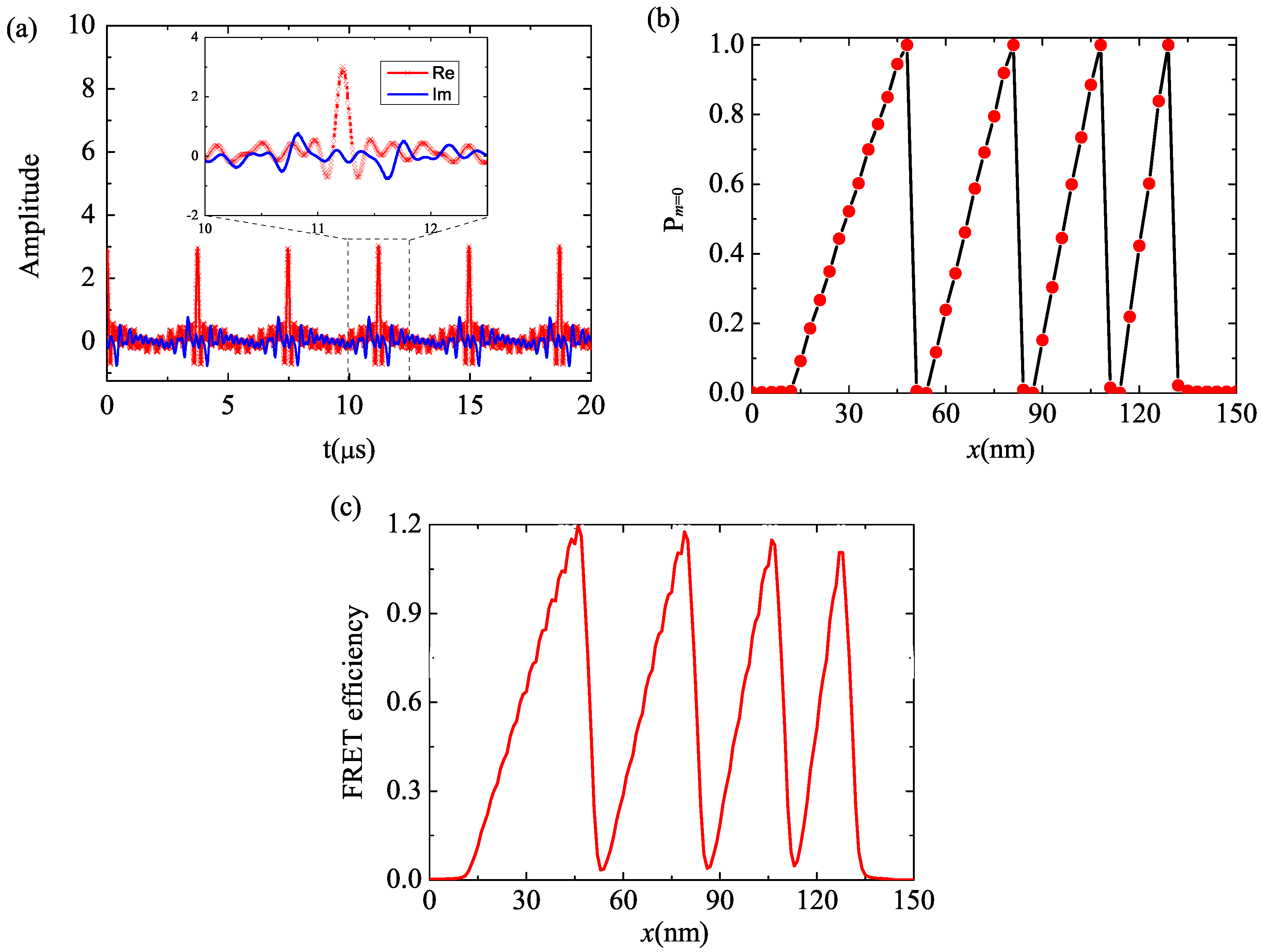
3. Numerical Simulation
4. Possible materials for MRL
5. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| MRI | Magnetic resonance imaging |
| MRL | Magnetic resonance lithography |
| GSD | Ground state depletion |
| FRET | Föster resonance energy transfer |
| AWG | Arbitray waveform generator |
| NV | Nitrogen Vacancy |
| ODMR | Optically detected magnetic resonance |
| SiV | Silicon Vacancy |
| Pr:YAG | Praseodymium-doped yttrium aluminum garnet |
References
- Rayleigh, L. Investigations in Optics, with Special Reference to the Spectroscope. Philos. Mag. Ser. 1880, 9, 40–55. [Google Scholar] [CrossRef]
- Abbe, E. Beitrge zur Theorie des Mikroskops und der Mikroskopischen. Arch. Mikr. Anat. 1873, 9, 413–418. [Google Scholar] [CrossRef]
- Al-Amri, M.; Liao, Z.; Zubairy, M.S. Beyond the Rayleigh Limit in optical Lithography. In Advances in Atomic, Molecular, and Optical Physics; Arimondo, E., Berman, P.R., Lin, C.C., Eds.; Academic Press: Cambridge, MA, USA, 2012; Volume 61, pp. 409–466. [Google Scholar]
- Hemmer, P.R.; Zapata, T. The universal scaling laws that determine the achievable resolution in diffraction schemes for super-resolution imaging. J. Opt. 2012, 14, 083002. [Google Scholar] [CrossRef]
- Gwyn, C.W.; Stulen, R.; Sweeney, D.; Attwood, D. Extreme ultraviolet lithography. J. Vac. Sci. Technol. B 1998, 16, 3142–3149. [Google Scholar] [CrossRef]
- Heuberger, A. X-ray lithography. J. Vac. Sci. Technol. B 1988, 6, 107–121. [Google Scholar] [CrossRef]
- Williams, C.; Kok, P.; Lee, H.; Dowling, J.P. Quantum lithography: A non-computing application of quantum information. Inform. Forsch. Entw. 2006, 21, 73–82. [Google Scholar] [CrossRef]
- Yablonovitch, E.; Vrijen, R.B. Optical Projection Lithography at Half the Rayleigh Resolution Limit by Two-photon Exposure. Opt. Eng. 1999, 38, 334–338. [Google Scholar] [CrossRef]
- Pe’er, A.; Dayan, B.; Vucelja, M.; Silberberg, Y.; Friesem, A.A. Quantum lithography by coherent control of classical light pulses. Opt. Express 2014, 12, 6600–6605. [Google Scholar] [CrossRef]
- Bentley, S.J.; Boyd, R.W. Nonlinear optical lithography with ultra-high sub-Rayleigh resolution. Opt. Express 2004, 12, 5735–5740. [Google Scholar] [CrossRef] [PubMed]
- Boto, A.N.; Kok, P.; Abrams, D.S.; Braunstein, S.L.; Williams, C.P.; Dowling, J.P. Quantum interferometric optical lithography: Exploiting entanglement to beat the diffraction limit. Phys. Rev. Lett. 2000, 85. [Google Scholar] [CrossRef] [PubMed]
- D’Angelo, M.; Chekhova, M.V.; Shih, Y. Two-Photon Diffraction and Quantum Lithography. Phys. Rev. Lett. 2001, 87, 013602. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, G.S.; Boyd, R.W.; Nagasako, E.M.; Bentley, S.J. Quantum interferometric optical lithography: Exploiting entanglement to beat the diffraction limit. Phys. Rev. Lett. 2001, 86, 1389. [Google Scholar] [CrossRef] [PubMed]
- Hemmer, P.R.; Muthukrishnan, A.; Scully, M.O.; Zubairy, M.S. Quantum lithography with classical light. Phys. Rev. Lett. 2006, 96, 163603. [Google Scholar] [CrossRef] [PubMed]
- Sun, Q.; Hemmer, P.R.; Zubairy, M.S. Quantum lithography with classical light: Generation of arbitrary patterns. Phys. Rev. A 2007, 75, 065803. [Google Scholar] [CrossRef]
- Ge, W.; Hemmer, P.R.; Zubairy, M.S. Quantum lithography with classical light. Phys. Rev. A 2013, 87, 023818. [Google Scholar] [CrossRef]
- Kiffner, M.; Evers, J.; Zubairy, M.S. Resonant Interferometric Lithography Beyond the Diffraction Limit. Phys. Rev. Lett. 2008, 100, 073602. [Google Scholar] [CrossRef] [PubMed]
- Liao, Z.; Al-Amri, M.; Zubairy, M.S. Quantum Lithography beyond the Diffraction Limit via Rabi Oscillations. Phys. Rev. Lett. 2010, 105, 183601. [Google Scholar] [CrossRef] [PubMed]
- Liao, Z.; Al-Amri, M.; Becker, T.; Schleich, W.P.; Scully, M.O.; Zubairy, M.S. Atomic Lithography with Subwavelength Resolution via Rabi Oscillations. Phys. Rev. A 2013, 87, 023405. [Google Scholar] [CrossRef]
- Liao, Z.; Al-Amri, M.; Zubairy, M.S. Coherent Atom Lithography with Nanometer Spacing. Phys. Rev. A. 2013, 88, 053809. [Google Scholar] [CrossRef]
- Brimhall, N.; Andrew, T.L.; Manthena, R.V.; Menon, R. Breaking the Far-Field Diffraction Limit in Optical Nanopatterning via Repeated Photochemical and Electrochemical Transitions in Photochromic Molecules. Phys. Rev. Lett. 2011, 107, 205501. [Google Scholar] [CrossRef] [PubMed]
- Gan, Z.; Cao, Y.; Evans, R.A.; Gu, M. Three-dimensional deep sub-diffraction optical beam lithography with 9 nm feature size. Nat. Commun. 2013, 4. [Google Scholar] [CrossRef] [PubMed]
- Hell, S.W.; Wichmann, J. Breaking the diffraction resolution limit by stimulated emission: Stimulated-emission-depletion fluorescence microscopy. Opt. Lett. 1994, 19, 780–782. [Google Scholar] [CrossRef] [PubMed]
- Hell, S.W.; Kroug, M. Ground-state-depletion fluorscence microscopy: A concept for breaking the diffraction resolution limit. Appl. Phys. B Lasers Optics 1995, 60, 495–497. [Google Scholar] [CrossRef]
- Thywissen, J.H.; Prentiss, M. Demonstration of frequency encoding in neutral atom lithography. New J. Phys. 2005, 7. [Google Scholar] [CrossRef]
- Haacke, E.M.; Brown, R.W.; Thompson, M.R.; Venkatesan, R. Magnetic Resonance Imaging: Physical Principles and Sequence Design; John Wiley and Sons: New York, NY, USA, 1999. [Google Scholar]
- Hemmer, P.; AlGhannam, F.; Zubairy, M.S. Toward sub-wavelength lithography with atomic coherence. Proc. SPIE 2013, 8875. [Google Scholar] [CrossRef]
- McFarland, E.W. Time-independent point-spread function for NMR microscopy. Magn. Reson. Imaging 1992, 10, 269–278. [Google Scholar] [CrossRef]
- Ofori-Okai, B.K.; Pezzagna, S.; Chang, K.; Loretz, M.; Schirhagl, R.; Tao, Y.; Moores, B.A.; Groot-Berning, K.; Meijer, J.; Degen, C.L. Spin properties of very shallow nitrogen vacancy defects in diamond. Phys. Rev. B 2012, 86, 081406. [Google Scholar] [CrossRef]
- Scully, M.O.; Zubairy, M.S. Quantum Optics; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Clegg, R.M. Laboratory Techniques in Biochemistry and Molecular Biology; Gadella, T.W.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2009; Volume 33, pp. 1–57. [Google Scholar]
- Tisler, J.; Reuter, R.; Lämmle, A.; Jelezko, F.; Balasubramanian, G.; Hemmer, P.R.; Reinhard, F.; Wrachtrup, J. Highly Efficient FRET from a Single Nitrogen-Vacancy Center in Nanodiamonds to a Single Organic Molecule. ACS Nano 2011, 5, 7893–7898. [Google Scholar] [CrossRef] [PubMed]
- Spectra Group Limited Inc. H-Nu Blue 640, 660 Visible Light Photoinitiators. Available online: http://www.sglinc.com/Portals/0/Images/limited/PDFS/photoinitiators/H-Nu20Blue-IR20TDS.pdf (accessed on 20 April 2012).
- Dunin-Borkowski, R.E.; Kasama, T.; Wei, A.; Tripp, S.L.; Hytch, M.J.; Snoeck, E.; Harrison, R.J.; Putnis, A. Off-axis electron holography of magnetic nanowires and chains, rings, and planar arrays of magnetic nanoparticles. Microsc. Res. Tech. 2004, 64, 390–402. [Google Scholar] [CrossRef] [PubMed]
- Cheung, C.L.; Nikolić, R.J.; Reinhardt, C.E.; Wang, T.F. Fabrication of nanopillars by nanosphere lithography. Nanotechnology 2006, 17. [Google Scholar] [CrossRef]
- Ohno, K.; Heremans, F.J.; Bassett, L.C.; Myers, B.A.; Toyli, D.M.; Bleszynski Jayich, A.C.; Palmstrøm, C.J.; Awschalom, D.D. Engineering shallow spins in diamond with nitrogen delta-doping. Appl. Phys. Lett. 2012, 101, 082413. [Google Scholar] [CrossRef]
- Gaebel, T.; Domhan, M.; Popa, I.; Wittmann, C.; Neumann, P.; Jelezko, F.; Rabeau, J.R.; Stavrias, N.; Greentree, A.D.; Prawer, S.; et al. Room-temperature coherent coupling of single spins in diamond. Nat. Phys. 2006, 2, 408–413. [Google Scholar] [CrossRef]
- Childress, L.; Gurudev Dutt, M.V.; Taylor, J.M.; Zibrov, A.S.; Jelezko, F.; Wrachtrup, J.; Hemmer, P.R.; Lukin, M.D. Coherent Dynamics of Coupled Electron and Nuclear Spin Qubits in Diamond. Science 2006, 314, 281–285. [Google Scholar] [CrossRef] [PubMed]
- Wrachtrup, J.; Jelezko, F. Processing quantum information in diamond. J. Phys. Condens. Matter 2006, 18. [Google Scholar] [CrossRef]
- Balasubramanian, G.; Neumann, P.; Twitchen, D.; Markham, M.; Kolesov, R.; Mizuochi, N.; Isoya, J.; Achard, J.; Beck, J.; Tissler, J.; et al. Ultralong spin coherence time in isotopically engineered diamond. Nat. Matter 2009, 8, 383–387. [Google Scholar] [CrossRef] [PubMed]
- Müller, T.; Hepp, C.; Pingault, B.; Neu, E.; Gsell, S.; Schreck, M.; Sternschulte, H.; Steinmller-Nethl, D.; Becher, C.; Atatüre, M. Optical signatures of silicon-vacancy spins in diamond. Nat. Commun. 2014, 5. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.H.; Green, A.M.; Taylor, R.A.; Sharp, D.N.; Turberfield, A.J.; Brossard, F.S.F.; Williams, D.A.; Briggs, G.A.D. Cryogenic two-photon laser photolithography with SU-8. Appl. Phys. Lett. 2006, 88, 143123. [Google Scholar] [CrossRef]
- Gardner, J.R.; Marable, M.L.; Welch, G.R.; Thomas, J.E. Suboptical wavelength position measurement of moving atoms using optical fields. Phys. Rev. Lett. 1993, 70. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.-Y.; Widmann, M.; Rendler, T.; Doherty, M.W.; Babinec, T.M.; Yang, S.; Eyer, M.; Siyushev, P.; Hausmann, B.J.M.; Loncar, M.; et al. Readout and control of a single nuclear spin with a metastable electron spin ancilla. Nat. Nanotechnol. 2013, 8, 487–492. [Google Scholar] [CrossRef] [PubMed]
- Kolesov, R. Coherent population trapping in a crystalline solid at room temperature. Phys. Rev. A 2005, 72, 051801. [Google Scholar] [CrossRef]
- Kolesov, R.; Reuter, R.; Xia, K.; Stöhr, R.; Zappe, A.; Wrachtrup, J. Super-resolution upconversion microscopy of praseodymium-doped yttrium aluminum garnet nanoparticles. Phys. Rev. B 2011, 84, 153413. [Google Scholar] [CrossRef]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
AlGhannam, F.; Hemmer, P.; Liao, Z.; Zubairy, M.S. Magnetic Resonance Lithography with Nanometer Resolution. Technologies 2016, 4, 12. https://doi.org/10.3390/technologies4020012
AlGhannam F, Hemmer P, Liao Z, Zubairy MS. Magnetic Resonance Lithography with Nanometer Resolution. Technologies. 2016; 4(2):12. https://doi.org/10.3390/technologies4020012
Chicago/Turabian StyleAlGhannam, Fahad, Philip Hemmer, Zeyang Liao, and M. Suhail Zubairy. 2016. "Magnetic Resonance Lithography with Nanometer Resolution" Technologies 4, no. 2: 12. https://doi.org/10.3390/technologies4020012
APA StyleAlGhannam, F., Hemmer, P., Liao, Z., & Zubairy, M. S. (2016). Magnetic Resonance Lithography with Nanometer Resolution. Technologies, 4(2), 12. https://doi.org/10.3390/technologies4020012




