Adsorption of HFO-1234ze(E) onto Steam-Activated Carbon Derived from Sawmill Waste Wood
Abstract
1. Introduction
2. Experimental Methods
2.1. Raw Materials and Chemicals
2.2. Activated Carbon Production Process
2.3. Characterization of Raw Materials and Activated Carbons
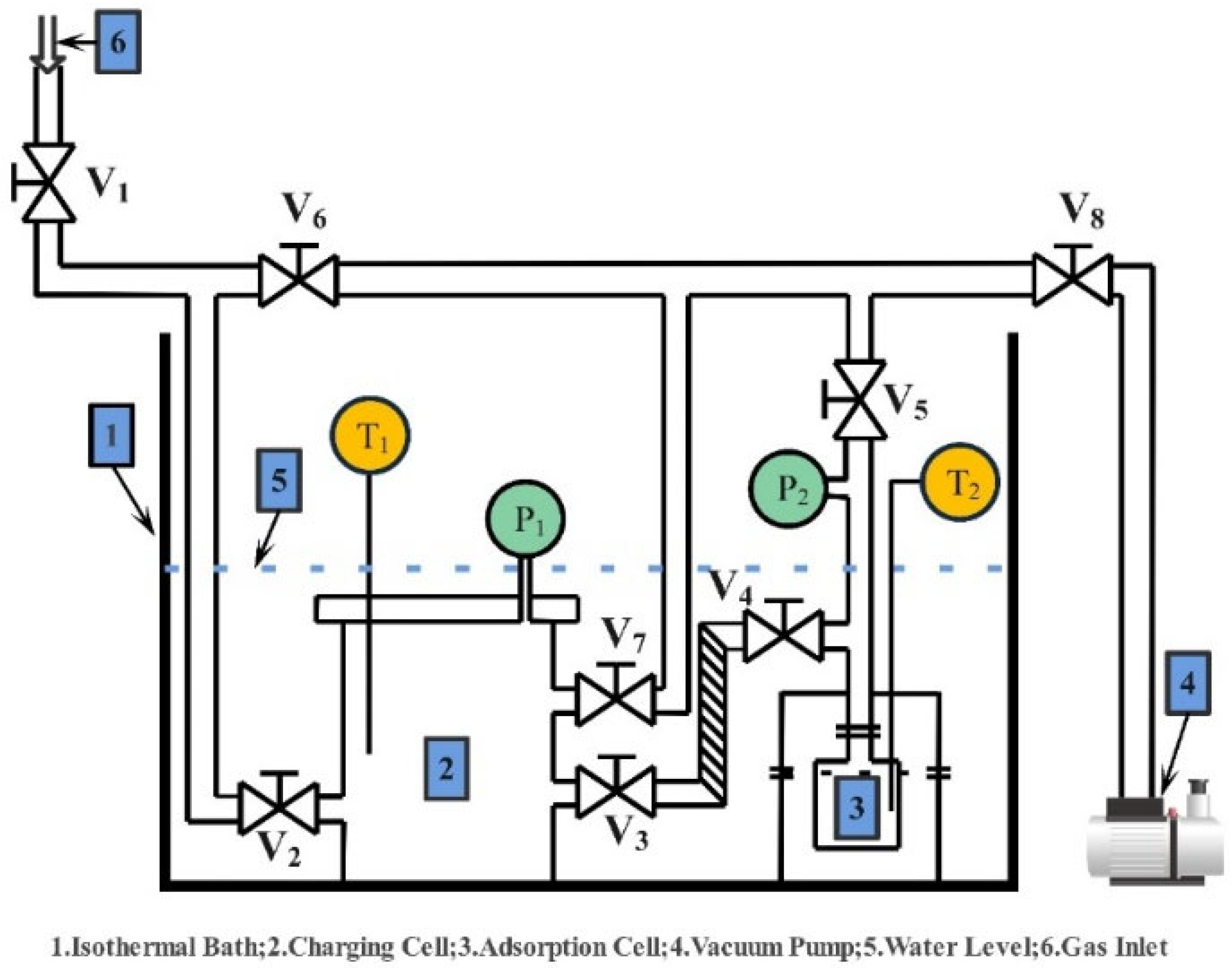
2.4. HFO-1234ze(E) Adsorption Isotherms’ Measurement
- Load the adsorption cell with approximately 1.0 g of the synthetic activated carbon sample;
- Vacuum the entire setup at 85 °C for more than 8 h to guarantee that no gas is adsorbed onto the sample within the system;
- Upon completion of the vacuuming process, seal all valves and allow the system to idle for 24 h to conduct a leak test;
- Upon a successful leak test, proceed with the adsorption experiment in the following manner:
- Maintain the heater and water circulator operation for an extended period to achieve a constant temperature for adsorption;
- Open the inlet valves (V1 and V2) to fill the charging unit and pressurize the cell to the desired pressure (e.g., beginning at around 20 kPa at Tads = 30 °C). Then, close the valves;
- Once pressure and temperature stabilize, note the values of pressure sensor P1 and temperature sensor T1. Input these values into the REFPROP database to compute the density of HFO-1234ze(E) denoted as (1, n), which will be utilized later for calculating the adsorption quantity;
- Open the valves (V3 and V4) between the charging and adsorption cells to let the gas flow into the adsorption unit. Wait until pressure and temperature stabilize, indicating the completion of adsorption. Record the values of pressure sensor P2 and temperature sensor T2. Input these values into the REFPROP database to obtain the density of HFO-1234ze(E) denoted as (2, n);
- Refill the charging unit with HFO-1234ze(E) gas until the subsequent target pressure is attained;
- Repeat steps (b) through (e) until the target pressure reaches the final pressure (which, in this study, is set at 500 kPa).
3. Results and Discussion
3.1. Characterization
3.1.1. Thermal Stability Analysis
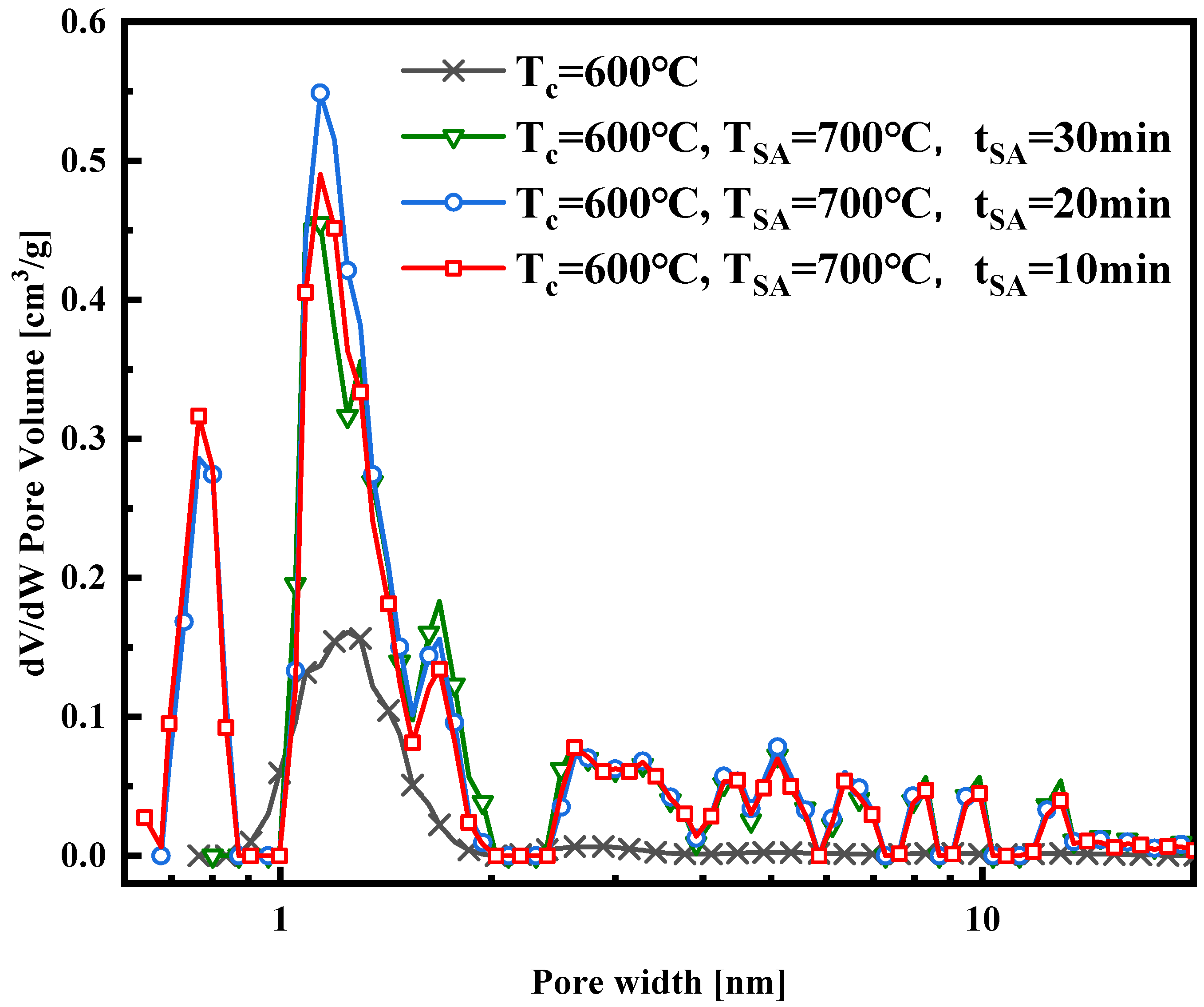
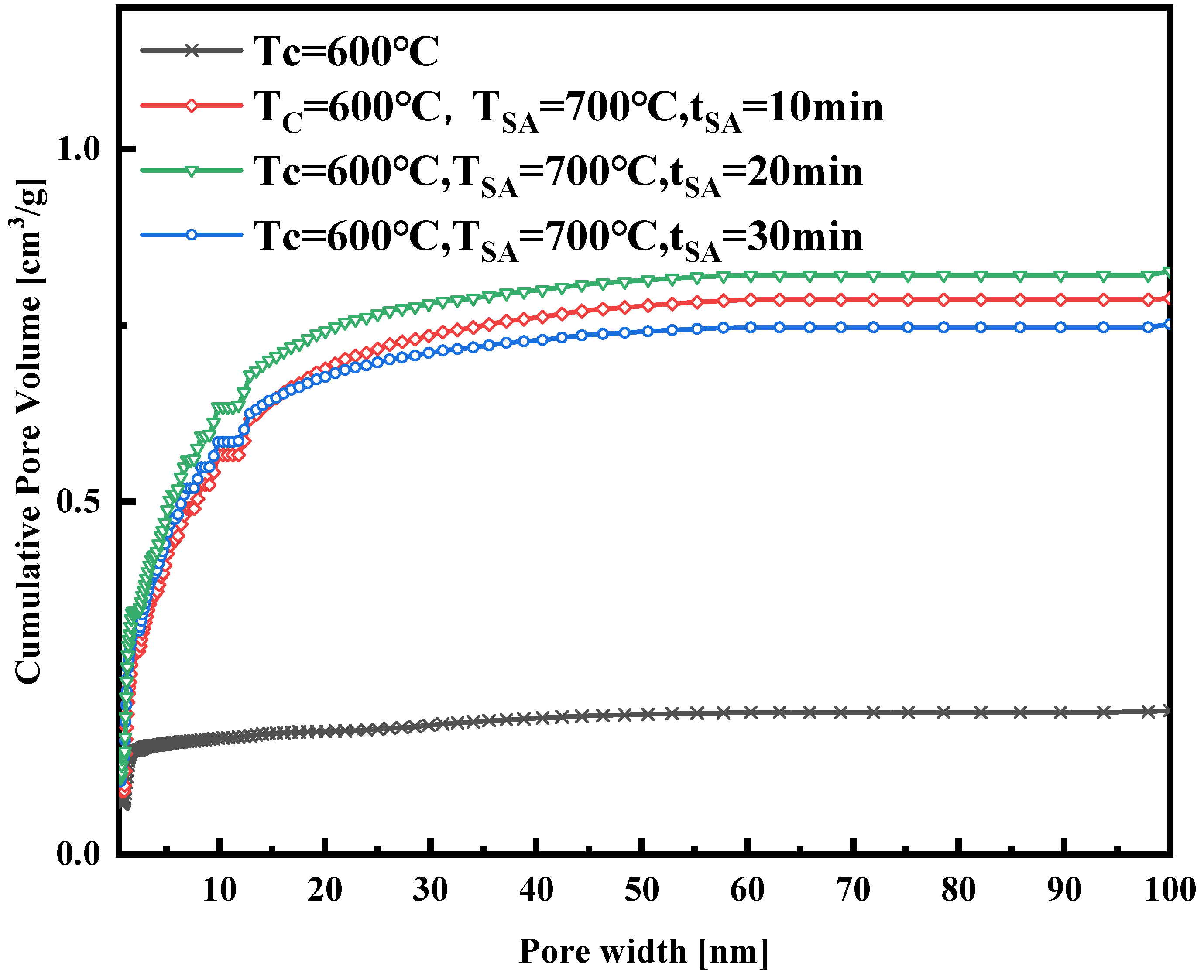
3.1.2. Nitrogen Adsorption Characterization
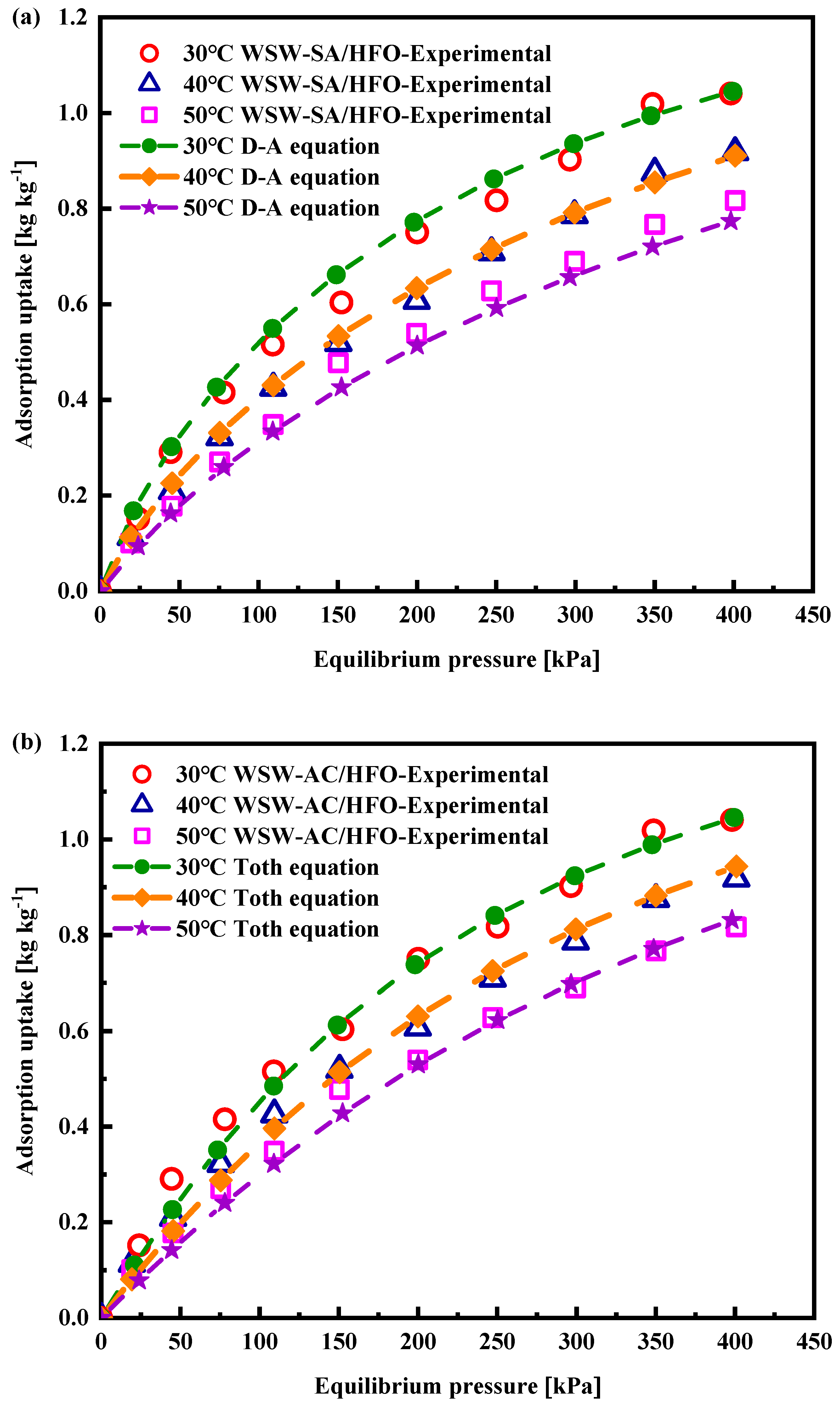
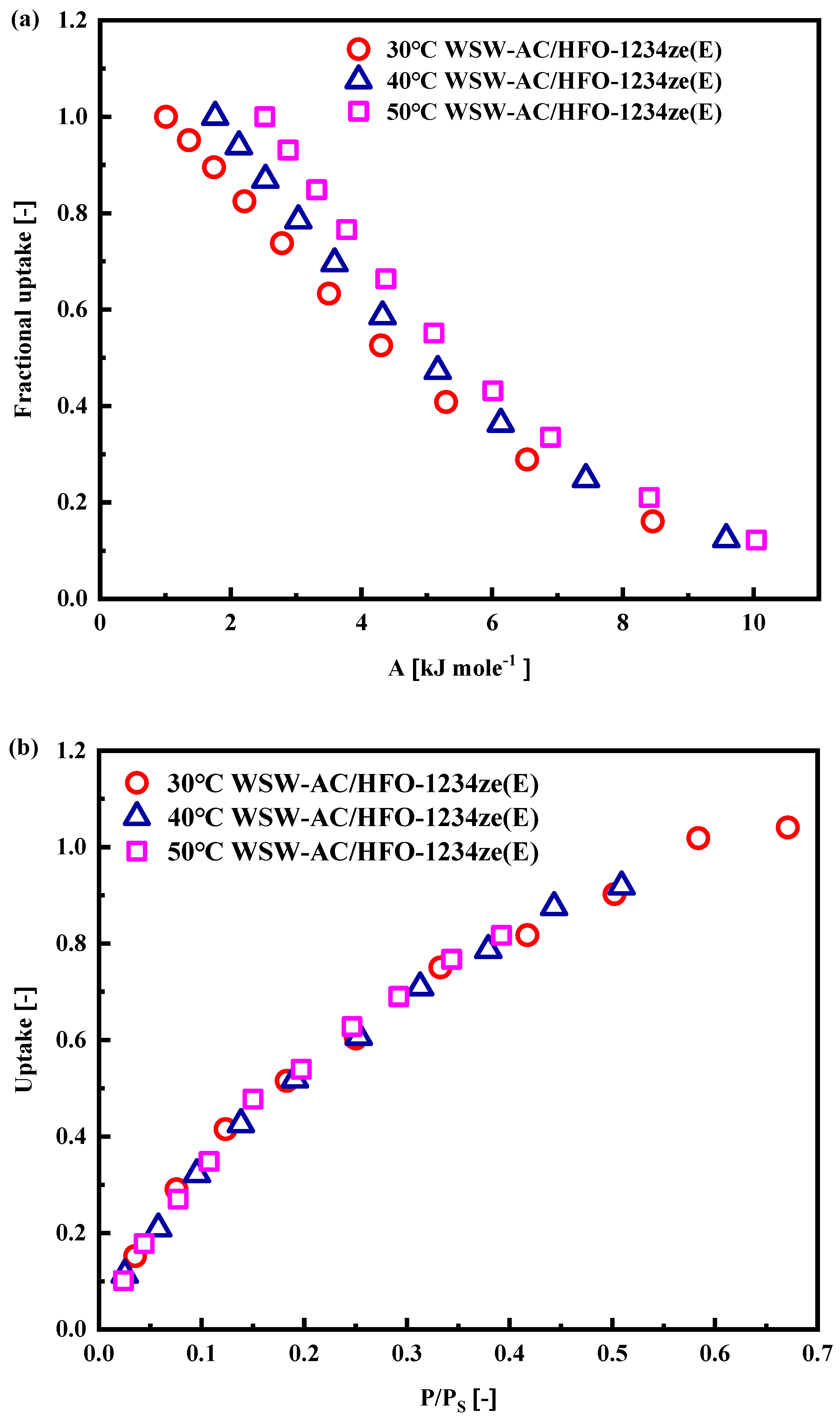
3.2. Adsorption Isotherms
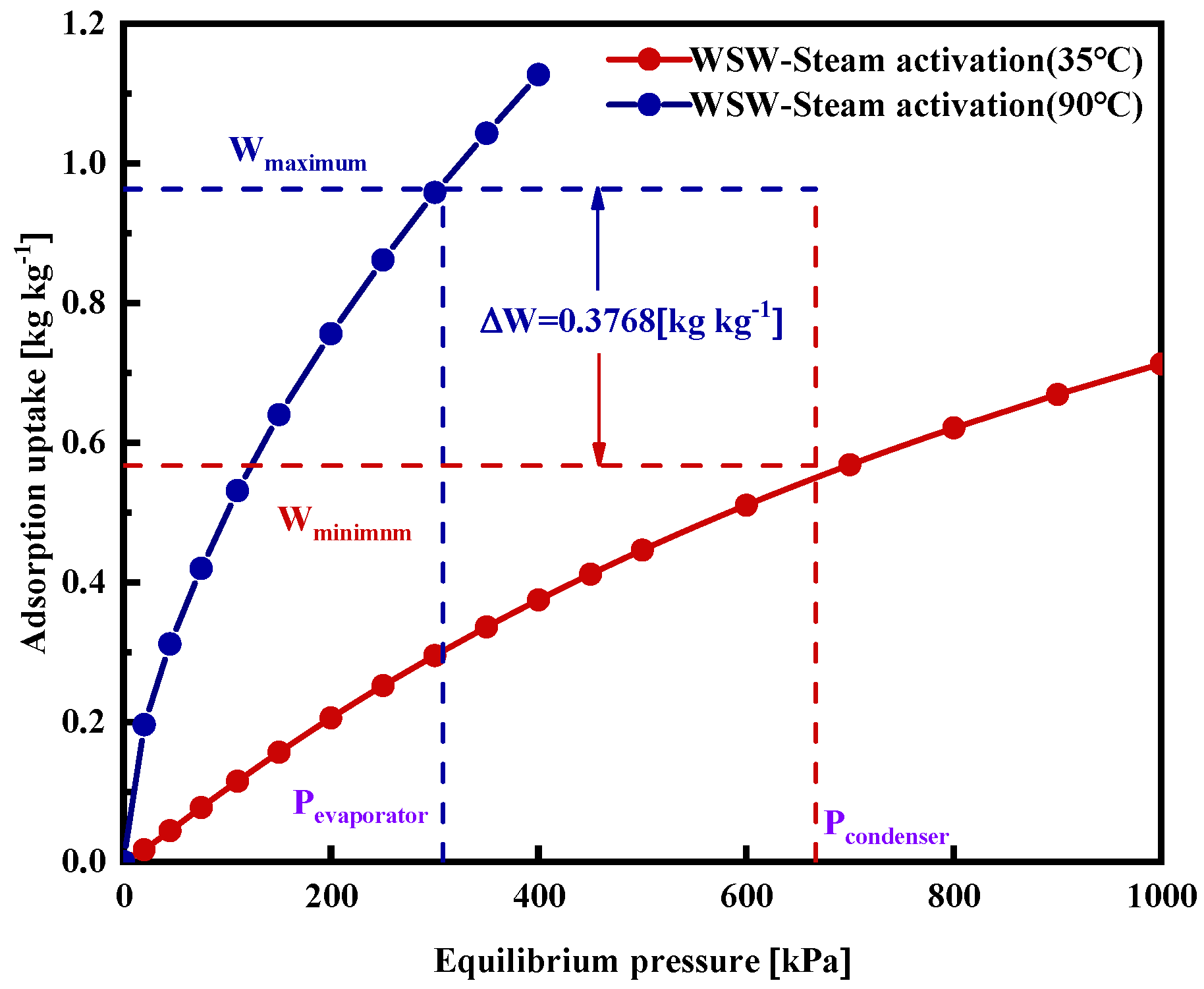
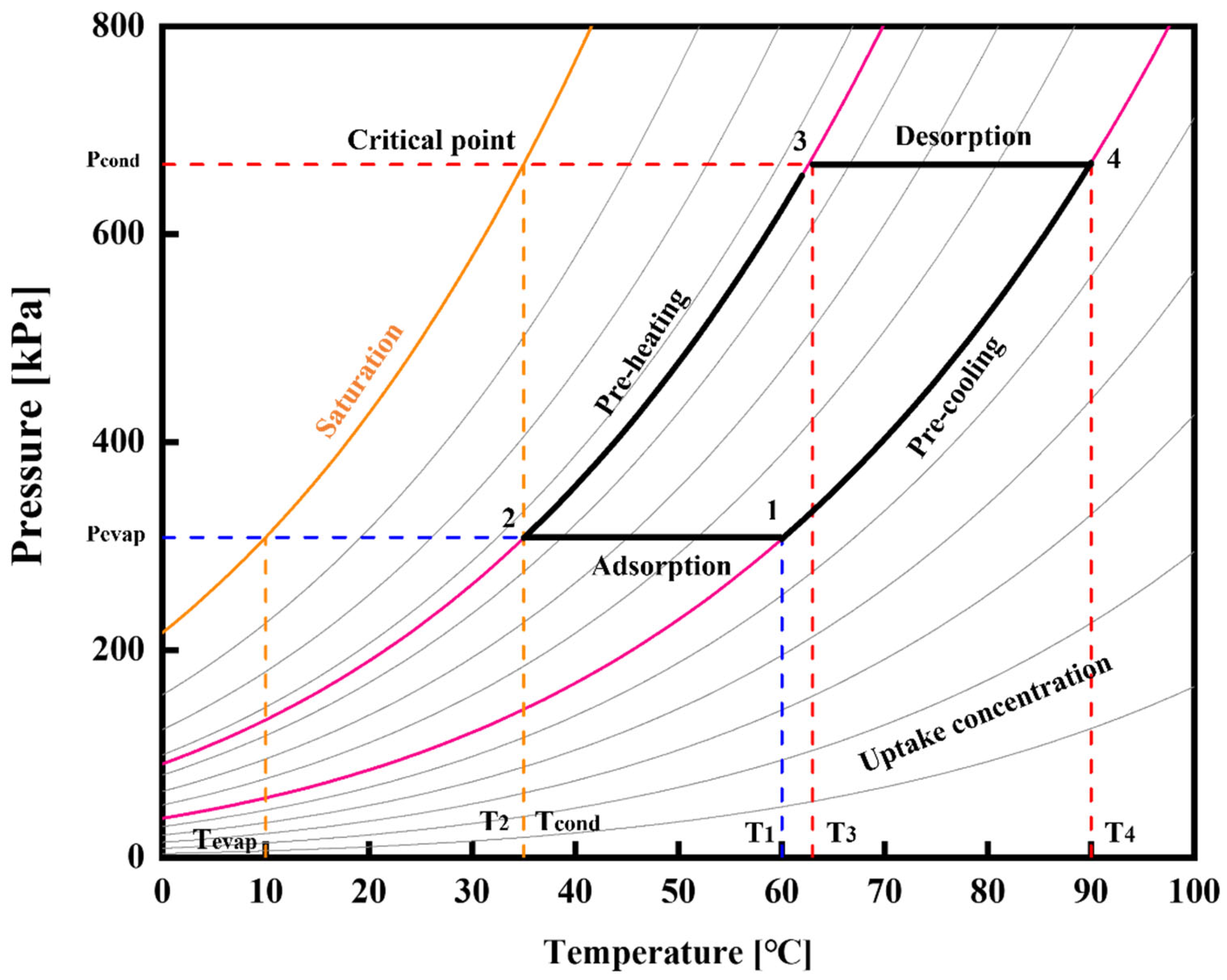
4. Thermodynamic Performances
4.1. Isosteric Heat of HFO-1234ze(E) Adsorption
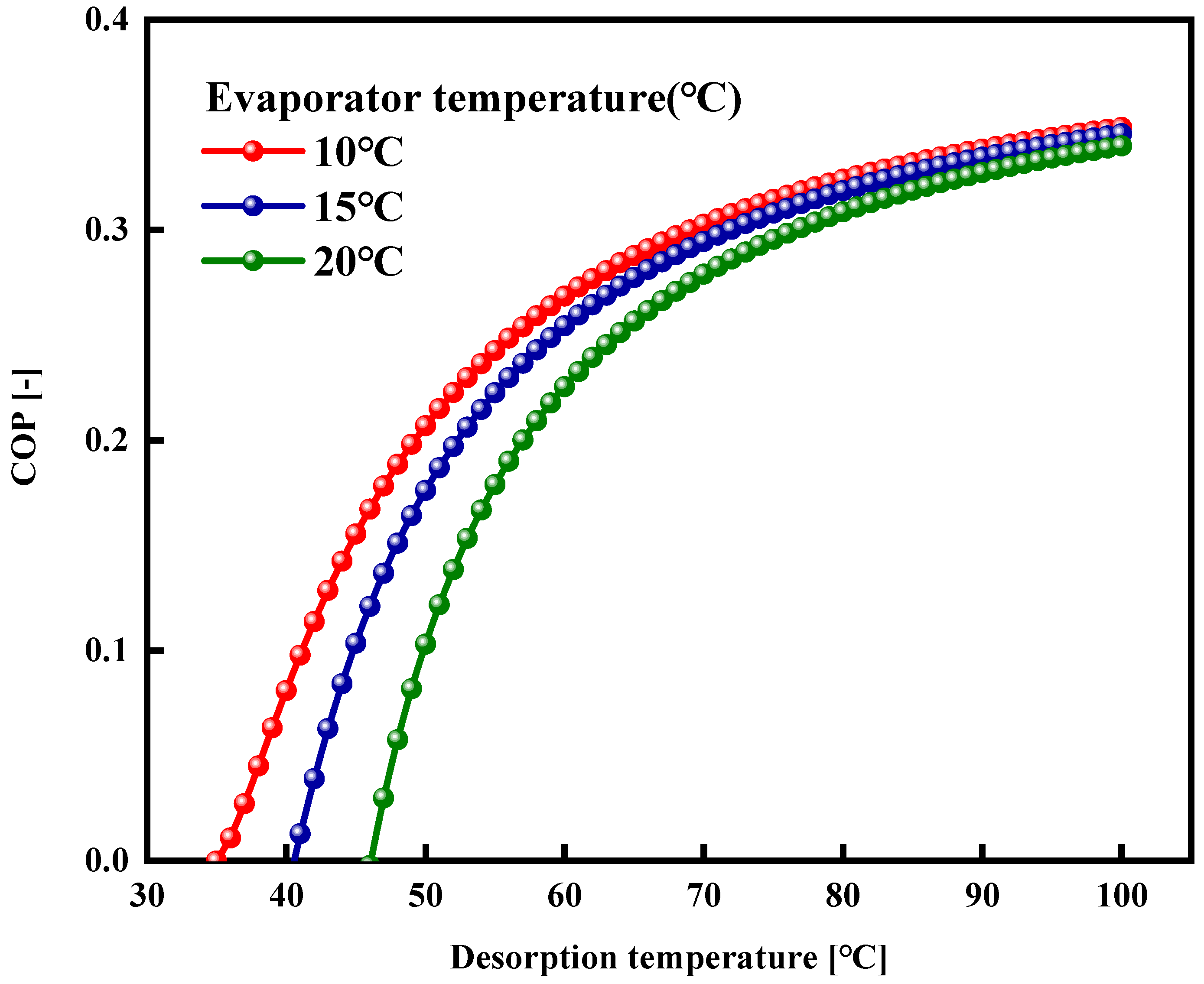
4.2. SCE and COP
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| adsorption potential ) | |
| adsorption characteristic energy ) | |
| equilibrium constant | |
| molar mass ) | |
| adsorption uptake ) | |
| 0 | saturated adsorption uptake ) |
| liquid enthalpy of HFO-1234ze(E) ) | |
| saturated vapor enthalpy of HFO-1234ze(E) ) | |
| evaporator enthalpy of HFO-1234ze(E) vaporization ) | |
| structural heterogeneity parameter ) | |
| equilibrium pressure ) | |
| saturated pressure | |
| isosteric heat of adsorption ) | |
| heterogeneity factor ) | |
| temperature ) | |
| universal gas constant ) | |
| Abbreviation | |
| ads | Adsorption |
| des | Desorption |
| evap | Evaporator |
| cond | Condenser |
| Acronyms | |
| COP | Co-efficient of performance |
| GWP | Global warming potential |
| ODP | Ozone depletion potential |
| SCE | Specific cooling effect |
References
- Muttakin, M.; Pal, A.; Rupa, M.J.; Ito, K.; Saha, B.B. A critical overview of adsorption kinetics for cooling and refrigeration systems. Adv. Colloid Interface Sci. 2021, 294, 102468. [Google Scholar] [CrossRef] [PubMed]
- Rocky, K.A.; Pal, A.; Rupam, T.H.; Nasruddin; Saha, B.B. Zeolite-graphene composite adsorbents for next generation adsorption heat pumps. Microporous Mesoporous Mater. 2021, 313, 110839. [Google Scholar] [CrossRef]
- Pan, Q.; Peng, J.; Wang, H.; Sun, H.; Wang, R. Experimental investigation of an adsorption air-conditioner using silica gel-water working pair. Sol. Energy 2019, 185, 64–71. [Google Scholar] [CrossRef]
- Saha, B.B.; Habib, K.; El-Sharkawy, I.I.; Koyama, S. Adsorption characteristics and heat of adsorption measurements of R-134a on activated carbon. Int. J. Refrig. 2009, 32, 1563–1569. [Google Scholar] [CrossRef]
- Askalany, A.A.; Saha, B.B.; Ismail, I.M. Adsorption isotherms and kinetics of HFC410A onto activated carbons. Appl. Therm. Eng. 2014, 72, 237–243. [Google Scholar] [CrossRef]
- Zheng, J.; Barpaga, D.; Gutiérrez, O.Y.; Browning, N.D.; Mehdi, B.L.; Farha, O.K.; Lercher, J.A.; McGrail, B.P.; Motkuri, R.K. Exceptional Fluorocarbon Uptake with Mesoporous Metal–Organic Frameworks for Adsorption-Based Cooling Systems. ACS Appl. Energy Mater. 2018, 1, 5853–5858. [Google Scholar] [CrossRef]
- Pal, A.; Shahrom, M.S.R.; Moniruzzaman, M.; Wilfred, C.D.; Mitra, S.; Thu, K.; Saha, B.B. Ionic liquid as a new binder for activated carbon based consolidated composite adsorbents. Chem. Eng. J. 2017, 326, 980–986. [Google Scholar] [CrossRef]
- Pal, A.; Thu, K.; Mitra, S.; El-Sharkawy, I.I.; Saha, B.B.; Kil, H.-S.; Yoon, S.-H.; Miyawaki, J. Study on biomass derived activated carbons for adsorptive heat pump application. Int. J. Heat Mass Transf. 2017, 110, 7–19. [Google Scholar] [CrossRef]
- Sun, Y.; Shi, X.-L.; Yang, Y.-L.; Suo, G.; Zhang, L.; Lu, S.; Chen, Z.-G. Biomass-Derived Carbon for High-Performance Batteries: From Structure to Properties. Adv. Funct. Mater. 2022, 32, 2201584. [Google Scholar] [CrossRef]
- Pal, A.; Uddin, K.; Saha, B.B.; Thu, K.; Kil, H.-S.; Yoon, S.-H.; Miyawaki, J. A benchmark for CO2 uptake onto newly synthesized biomass-derived activated carbons. Appl. Energy 2020, 264, 114720. [Google Scholar] [CrossRef]
- Muruganantham, R.; Wang, F.-M.; Yuwono, R.A.; Sabugaa, M.; Liu, W.-R. Biomass Feedstock of Waste Mango-Peel-Derived Porous Hard Carbon for Sustainable High-Performance Lithium-Ion Energy Storage Devices. Energy Fuels 2021, 35, 10878–10889. [Google Scholar] [CrossRef]
- Wang, J.; Nie, P.; Ding, B.; Dong, S.; Hao, X.; Dou, H.; Zhang, X. Biomass derived carbon for energy storage devices. J. Mater. Chem. A 2017, 5, 2411–2428. [Google Scholar] [CrossRef]
- Wang, C.; Wang, X.; Wang, H.; Zhang, L.; Wang, Y.; Dong, C.-L.; Huang, Y.-C.; Guo, P.; Cai, R.; Haigh, S.J.; et al. Low-coordinated Co-N3 sites induce peroxymonosulfate activation for norfloxacin degradation via high-valent cobalt-oxo species and electron transfer. J. Hazard. Mater. 2023, 455, 131622. [Google Scholar] [CrossRef]
- Ahmed, M.J.; Theydan, S.K. Optimization of microwave preparation conditions for activated carbon from Albizia lebbeck seed pods for methylene blue dye adsorption. J. Anal. Appl. Pyrolysis 2014, 105, 199–208. [Google Scholar] [CrossRef]
- Fu, K.; Yue, Q.; Gao, B.; Sun, Y.; Zhu, L. Preparation, characterization and application of lignin-based activated carbon from black liquor lignin by steam activation. Chem. Eng. J. 2013, 228, 1074–1082. [Google Scholar] [CrossRef]
- Jahan, I.; Rupam, T.H.; Palash, M.; Rocky, K.A.; Saha, B.B. Energy efficient green synthesized MOF-801 for adsorption cooling applications. J. Mol. Liq. 2022, 345, 117760. [Google Scholar] [CrossRef]
- Li, W.; Xia, X.; Cao, M.; Li, S. Structure–property relationship of metal–organic frameworks for alcohol-based adsorption-driven heat pumps via high-throughput computational screening. J. Mater. Chem. A 2019, 7, 7470–7479. [Google Scholar] [CrossRef]
- Thu, K.; Takeda, N.; Miyazaki, T.; Saha, B.B.; Koyama, S.; Maruyama, T.; Maeda, S.; Kawamata, T. Experimental investigation on the performance of an adsorption system using Maxsorb III + ethanol pair. Int. J. Refrig. 2019, 105, 148–157. [Google Scholar] [CrossRef]
- Jribi, S.; Saha, B.B.; Koyama, S.; Chakraborty, A.; Ng, K.C. Study on activated carbon/HFO-1234ze(E) based adsorption cooling cycle. Appl. Therm. Eng. 2013, 50, 1570–1575. [Google Scholar] [CrossRef]
- Higashi, Y.; Tanaka, K.; Ichikawa, T. Critical Parameters and Saturated Densities in the Critical Region for trans-1,3,3,3-Tetrafluoropropene (HFO-1234ze(E)). J. Chem. Eng. Data 2010, 55, 1594–1597. [Google Scholar] [CrossRef]
- An, B.; Yang, F.; Duan, Y.; Yang, Z. Measurements and New Vapor Pressure Correlation for HFO-1234ze(E). J. Chem. Eng. Data 2017, 62, 328–332. [Google Scholar] [CrossRef]
- Tanaka, K.; Takahashi, G.; Higashi, Y. Measurements of the Isobaric Specific Heat Capacities for trans -1,3,3,3-Tetrafluoropropene (HFO-1234ze(E)) in the Liquid Phase. J. Chem. Eng. Data 2010, 55, 2267–2270. [Google Scholar] [CrossRef]
- Ye, L.; Islam, M.A.; Rupam, T.H.; Jahan, I.; Saha, B.B. Study on the adsorption characteristics of Maxsorb III/HFO-1234ze(E) pair for adsorption refrigeration applications. Int. J. Refrig. 2023, 146, 248–260. [Google Scholar] [CrossRef]
- Daud, W.M.A.W.; Ali, W.S.W.; Sulaiman, M.Z. The effects of carbonization temperature on pore development in palm-shell-based activated carbon. Carbon 2000, 38, 1925–1932. [Google Scholar] [CrossRef]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the use and interpretation of adsorption isotherm models: A review. J. Hazard. Mater. 2020, 393, 122383. [Google Scholar] [CrossRef] [PubMed]
- Pal, A.; Uddin, K.; Thu, K.; Saha, B.B. Activated carbon and graphene nanoplatelets based novel composite for performance enhancement of adsorption cooling cycle. Energy Convers. Manag. 2019, 180, 134–148. [Google Scholar] [CrossRef]
- Rocky, K.A.; Pal, A.; Moniruzzaman, M.; Saha, B.B. Adsorption characteristics and thermodynamic property fields of polymerized ionic liquid and polyvinyl alcohol based composite/CO2 pairs. J. Mol. Liq. 2019, 294, 111555. [Google Scholar] [CrossRef]
- Shen, D.; Bülow, M.; Siperstein, F.; Engelhard, M.; Myers, A.L. Comparison of Experimental Techniques for Measuring Isosteric Heat of Adsorption. Adsorption 2000, 6, 275–286. [Google Scholar] [CrossRef]
- Saha, B.B.; Koyama, S.; Kashiwagi, T.; Akisawa, A.; Ng, K.C.; Chua, H.T. Waste heat driven dual-mode, multi-stage, multi-bed regenerative adsorption system. Int. J. Refrig. 2003, 26, 749–757. [Google Scholar] [CrossRef]
- Rahman, K.A.; Chakraborty, A.; Saha, B.B.; Ng, K.C. On thermodynamics of methane+carbonaceous materials adsorption. Int. J. Heat Mass Transf. 2012, 55, 565–573. [Google Scholar] [CrossRef]








| Characteristics | Value |
|---|---|
| Chemical formula | C3H2F4 |
| Molar mass | 114.04 g/mol |
| Normal boiling point | 254.2 K |
| Ozone depletion potential | 0 |
| Global warming potential | 6 |
| Critical state pressure | 3636.3 kPa |
| Critical state temperature | 382.52 K |
| Security category | A2L |
| Symbols | Value |
|---|---|
| Samples | Yield (%) | SBET (m2/g) | Vtotal (cm3/g) | Vmicro (cm3/g) | Vmeso (cm3/g) |
|---|---|---|---|---|---|
| WSW–C600 | 100 | 315.9 | 0.204 | 0.143 | 0.061 |
| WSW–A600–30 | 45.5 | 688.3 | 0.506 | 0.225 | 0.281 |
| WSW–A650–30 | 35.2 | 872.9 | 0.748 | 0.252 | 0.496 |
| WSW–A700–30 | 18.5 | 799.9 | 0.794 | 0.222 | 0.572 |
| WSW–A700–20 | 27.6 | 946.8 | 0.843 | 0.279 | 0.564 |
| WSW–A700–10 | 39.8 | 855.6 | 0.830 | 0.249 | 0.581 |
| Model | Fitting Parameters | Values |
|---|---|---|
| D–A | W0 (kg kg−1) | 1.067 |
| E (kJ kg−1) | 46.736 | |
| n (−) | 1.42 | |
| RMSD [%) | 2.67 | |
| R2 | 0.99713 | |
| Tóth | W0 (kg kg−1) | 1.73 |
| Qst (kJ mol−1) | 22.59 | |
| t (−) | 0.70 | |
| (−) | 9.03 | |
| RMSD (%) | 2.14 | |
| R2 | 0.99953 |
| Evaporator Temperature (°C) | Condenser Temperature (°C) | Evaporator Pressure (kPa) | Condenser Pressure (kPa) | Desorption Temperature (°C) | Net Uptake (kg kg−1) | Specific Cooling Effect (kJ kg−1) | Coefficient of Performance (−) |
|---|---|---|---|---|---|---|---|
| 10 | 35 | 308 | 667 | 90 | 0.3768 | 82.99 | 0.355 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bao, H.; Islam, M.A.; Saha, B.B. Adsorption of HFO-1234ze(E) onto Steam-Activated Carbon Derived from Sawmill Waste Wood. Technologies 2024, 12, 104. https://doi.org/10.3390/technologies12070104
Bao H, Islam MA, Saha BB. Adsorption of HFO-1234ze(E) onto Steam-Activated Carbon Derived from Sawmill Waste Wood. Technologies. 2024; 12(7):104. https://doi.org/10.3390/technologies12070104
Chicago/Turabian StyleBao, Huiyuan, Md. Amirul Islam, and Bidyut Baran Saha. 2024. "Adsorption of HFO-1234ze(E) onto Steam-Activated Carbon Derived from Sawmill Waste Wood" Technologies 12, no. 7: 104. https://doi.org/10.3390/technologies12070104
APA StyleBao, H., Islam, M. A., & Saha, B. B. (2024). Adsorption of HFO-1234ze(E) onto Steam-Activated Carbon Derived from Sawmill Waste Wood. Technologies, 12(7), 104. https://doi.org/10.3390/technologies12070104







