Relativistic Option Pricing
Abstract
1. Introduction
2. State of the Art
2.1. Interplanetary Trade
2.2. High Speed Trading
2.3. Other
3. Spacetime Finance
3.1. Concepts
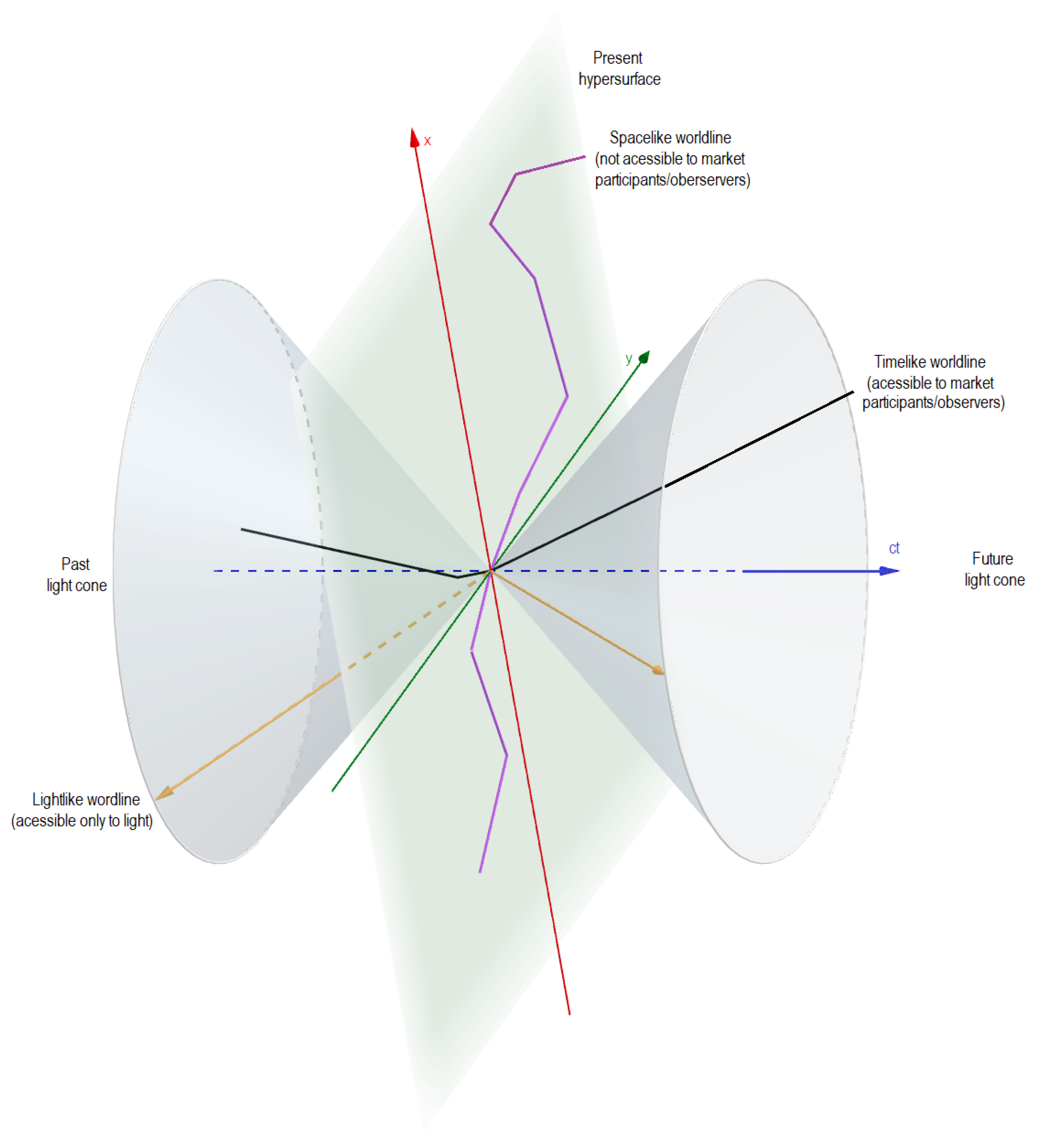
- SpacetimeSpacetime is a space concept where time and spatial dimensions are intertwined and undissociated and where a reference frame is defined. Its dimensions can be interpreted as “degrees of freedom”, which theoretically provide an infinite set of coordinates available to the event.However, spacetime dimensions are isotropic, which means the relation between different reference frames must be deterministic. Thus, they cannot be modelled using stochastic processes. Furthermore, the isotropy of the time dimension does not mean that a "back-in-time" occurrence is possible, it only states that the time flow direction does not matter. Taking a finance perspective, this means that we may calculate future values or present values—i.e., the time flow direction can be what better suits us—however, of course, there is no "back-in-time" possibility. These are the most common mistakes identified in the literature. A stock exchange from a specific city may be defined in a spacetime concept with its spatial coordinates and time dimension.
- Market participants (and observers)The term “observer” is widely used in physics and relativity literature. It intends to describes someone—e.g., a researcher—that does not interfere with what is being studied, nor with the fundamental laws of physics. When taking a financial perspective, it is difficult conceive such a person or entity who only observes the market without playing a role in it. Therefore, the term ”market participant” () appears to be a better fit for financial applications.A can have a more direct intervention in the market—e.g., issuer, broker, investor or analyst—or a lesser one, but still cannot disobey the fundamental laws of physics. We save the term “observer” to refer to an outside person or entity that we can guarantee does not interfere in the market (e.g., a researcher or supervision authority).
- RelativityIn the present study, the term relativity is used in the context of relativity that is not Euclidean and is gravity free, under STR. It affects the spacetime metric and produces market measurable effects. This implies very high velocities and an exact definition, which may depend on the concrete application.When an is moving relative to the stock exchange, relativity is involved, even if its effects are negligible.
- Event and objectObject and event terms commonly have different meanings. An , may interpret a nickel mine as an object that is inanimate. Another can interpret it as a set of material points travelling through the cosmos, at thousands of meters per second. The latter description is more frequently called an event. The term “event” is also more suitable to refer to a deal between two .3 Thus, throughout, we refer only to events (E), instead of events and objects.
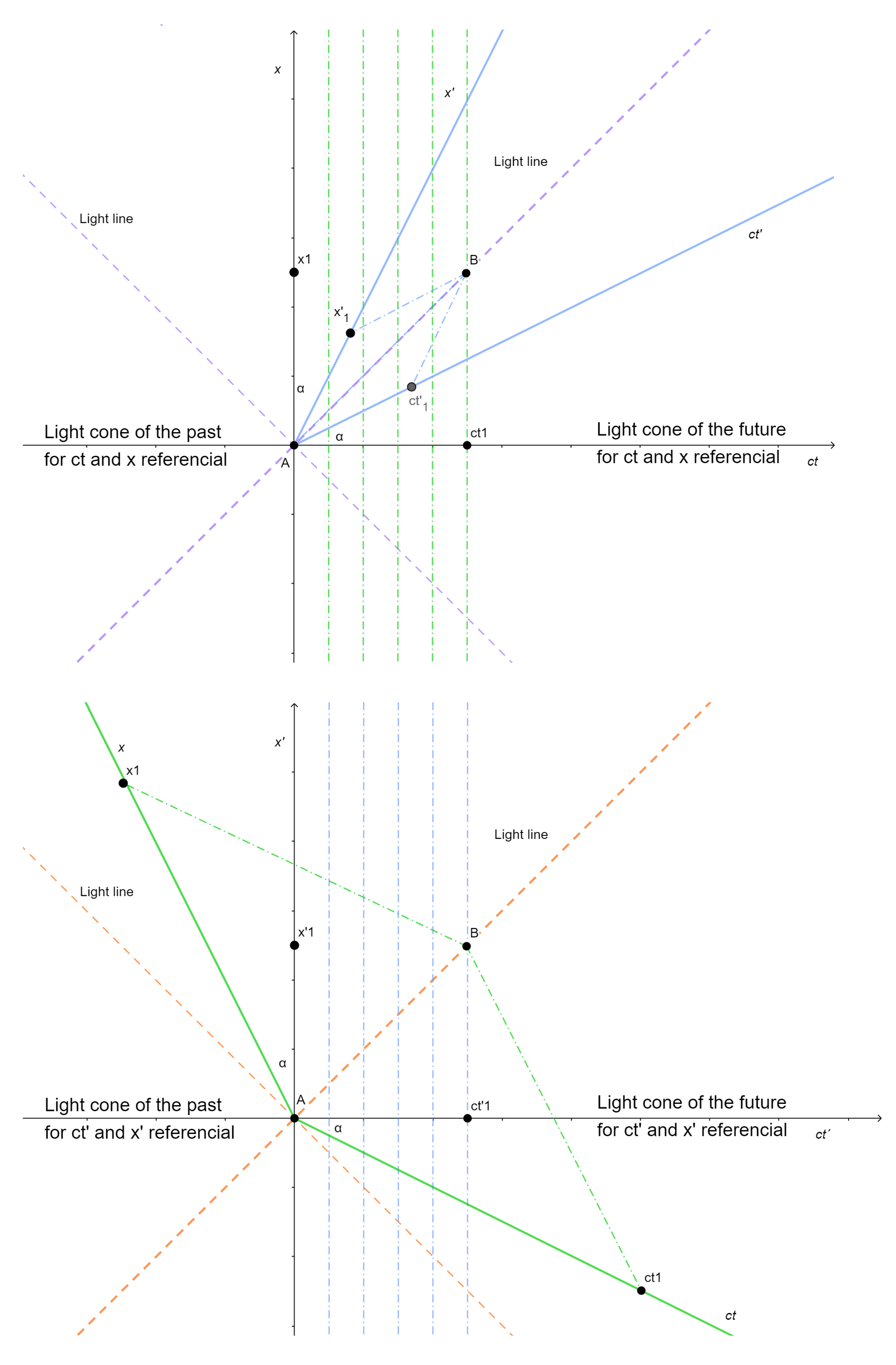
3.2. Minkowski Spacetime
3.3. Lorentz Transformations
3.3.1. Space Contraction and Time Dilation
3.3.2. Proper Time
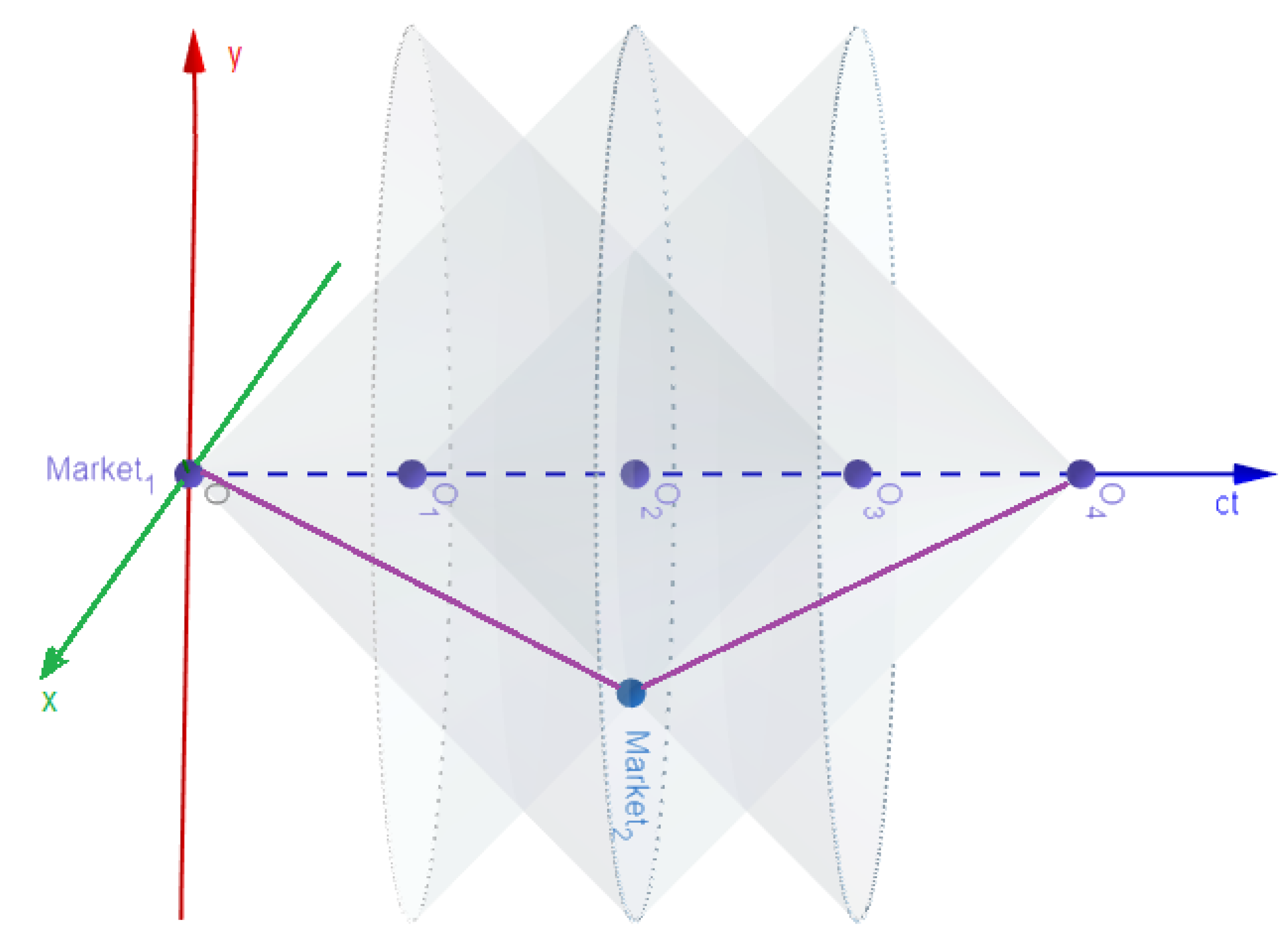
3.3.3. Example
- At point in Market, (point O), MP and MP, agree on the price of an asset12.
- Then MP initiates a journey to Market.
- Exactly the moment when MP reaches Market is a simultaneous moment for MP. MP is at point and measures an elapsed time of T.
- Although from MP perspective, MP is at point . Thus, the elapsed time measured by MP is .
- From the Market perspective, the elapsed time is . Thus, when MP reaches Market, he sees the asset price for time (point ).
- According to MP, when MP reaches Market, he sees the asset price for T (point ).
- Now, let us consider if MP turns around and goes back to Market. While performing the turn, MP is not in an inertial reference frame, because MP has to slow down, turn and accelerate again.
- In this case, from MP’s perspective, while MP is turning back, the reality of MP shifts rapidly (from point to ).
- Although both meet back at point , in Market, MP spent time units, while for MP, it took longer—.
- Both MP and MP agree again on the asset price when they meet again at point (the law of one price holds)13. However one of them has experienced the possible gains or losses in less time than the other, which may be understood as some sort of “spacetime arbitrage”.
- Axiom 1: For all financial events and market participants, when different inertial reference frames are involved, a settlement spacetime reference frame must be considered to serve as a benchmark.
- Axiom 2: When only time incorporates the relativity effects then proper time is the time measure that makes the asset or financial instrument pricing model invariant to all inertial reference frames. All market participants should follow the financial event proper time—i.e., deal or asset duration—to evaluate the asset or financial instrument pricing conditions.
4. Options
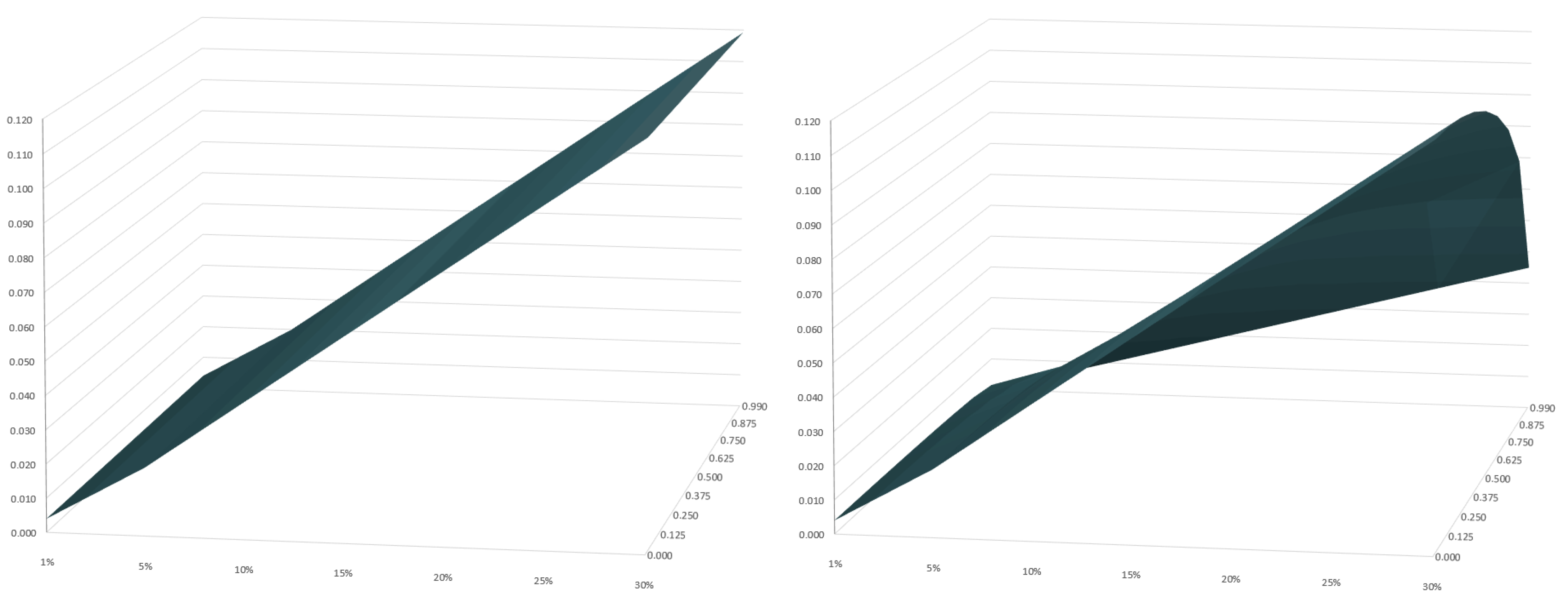
4.1. Pricing
4.2. Some Greeks
4.2.1. Theta
4.2.2. Vega
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Angel, James J. 2014. When finance meets physics: The impact of the speed of light on financial markets and their regulation. Financial Review 49: 271–81. [Google Scholar] [CrossRef]
- Auer, Markus P. 2015. Stranger Times: The Impact of Relativistic Time Concepts on the Time Value of Money. SSRN Electronic Journal, 1–16. [Google Scholar] [CrossRef]
- Bachelier, Louis. 1900. Théorie de la spéculation. Annales Scientifiques de l’École Normale Supérieure 17: 21–86. [Google Scholar] [CrossRef]
- Black, Fischer, and Myron Scholes. 1973. The Pricing of Options and Corporate Liabilities. The Journal of Political Economy 81: 637–54. [Google Scholar] [CrossRef]
- Buchanan, Mark. 2015. Physics in finance: Trading at the speed of light. Nature 518: 161–63. [Google Scholar] [CrossRef]
- Courtault, Jean-Michel, Youri Kabanov, Bernard Bru, Pierre Crépel, Isabelle Lebon, and Arnaud Le Marchand. 2000. Louis Bachelier on the Centenary of Théorie de la Spéculation. Mathematical Finance 10: 339–53. [Google Scholar] [CrossRef]
- de Area Leão Pereira, Eder Johnson, Marcus Fernandes da Silva, and H.B.B. Pereira. 2017. Econophysics: Past and present. Physica A: Statistical Mechanics and Its Applications 473: 251–61. [Google Scholar] [CrossRef]
- de Paula, João Antonio. 2002. Walras no Journal des Économistes: 1860–65. Revista Brasileira de Economia 56: 121–46. [Google Scholar] [CrossRef]
- Dunkel, Jörn, and Peter Hänggi. 2009. Relativistic Brownian motion. Physics Reports 471: 1–73. [Google Scholar] [CrossRef]
- Einstein, Albert. 1905. On the motion of small particles suspended in liquids at rest required by the molecular-kinetic theory of heat. Annalen der Physik 17: 208. [Google Scholar]
- Einstein, Albert. 1905. Zur Elektrodynamik bewegter Körper. Annalen der Physik 322: 891–921. [Google Scholar] [CrossRef]
- Einstein, Albert. 1916. Die Grundlage der allgemeinen Relativitätstheorie. Annalen der Physik 354: 769–822. [Google Scholar] [CrossRef]
- Eugene, Fama. 1970. Efficient Capital Market: A Re-view of Theory and Empirical Work. The Journal of Finance 25: 383–417. [Google Scholar]
- Fama, Eugene. 1991. Efficient capital markets II. The Journal of Finance XLVI: 1575–617. [Google Scholar] [CrossRef]
- Ferreira, Paulo, Éder J. A. L. Pereira, and Hernane B. B. Pereira. 2020. From Big Data to Econophysics and Its Use to Explain Complex Phenomena. Journal of Risk and Financial Management 13: 153. [Google Scholar] [CrossRef]
- Haug, Espen Gaarder. 2004. Space-time Finance. The relativity theory’s implications for mathematical finance. Willmott, July 2–15. [Google Scholar]
- Haug, Espen Gaarder. 2018. Double Light Speed: History, Confusion, and Recent Applications to High Speed Trading. SSRN Electronic Journal, 1–13. [Google Scholar] [CrossRef]
- Heisenberg, Werner. 1927. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Zeitschrift für Physik 43: 172–98. [Google Scholar] [CrossRef]
- Hetherington, Norriss S. 1983. Isaac Newton’s Influence on Adam Smith’s Natural Laws in Economics. Journal of the History of Ideas 44: 497–505. [Google Scholar] [CrossRef]
- Jacobson, Theodore, and Lawrence S. Schulman. 1984. Quantum stochastics: The passage from a relativistic to a non-relativistic path integral. Journal of Physics A: Mathematical and General 17: 375–83. [Google Scholar] [CrossRef][Green Version]
- Kakushadze, Zura. 2017. Volatility smile as relativistic effect. Physica A: Statistical Mechanics and Its Applications 475: 59–76. [Google Scholar] [CrossRef]
- Krugman, Paul. 2010. The theory of interstellar trade. Economic Inquiry 48: 1119–23. [Google Scholar] [CrossRef]
- Laughlin, Gregory, Anthony Aguirre, and Joseph Grundfest. 2014. Information Transmission between Financial Markets in Chicago and New York. Financial Review 49: 283–312. [Google Scholar] [CrossRef]
- Mannix, Brian. 2016. Space-Time Trading: Special Relativity and Financial Market Microstructure. Columbian College of Arts & Sciences. [Google Scholar]
- Mantegna, Rosario N., and H.Eugene Stanley. 1999. Introduction to Econophysics: Correlations and Complexity in Finance. New York: Cambridge University Press, p. 148. [Google Scholar]
- Merton, Robert C. 1973. Theory of rational option pricing. The Bell Journal of Economics and Management Science 4: 141–83. [Google Scholar] [CrossRef]
- Minkowski, Hermann. 1908. Die Grundgleichungen für die elektromagnetischen Vorgänge in bewegten Körpern. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse, 53–111. [Google Scholar]
- Mohajan, Haradhan. 2013. Minkowski geometry and space-time manifold in relativity. Journal of Environmental Treatment Techniques 1: 101–9. [Google Scholar]
- Morton, Jason. 2016. Relativistic Finance. Available online: Medium.com (accessed on 14 June 2021).
- Naber, Gregory L. 2012. The Geometry of Minkowski Spacetime. In Applied Mathematical Sciences. New York: Springer, vol. 92. [Google Scholar] [CrossRef]
- NASA. 2018. Mars Fact Sheet; Washington, DC: NASA.
- Pincak, Richard, and Kabin Kanjamapornkul. 2018. GARCH(1,1) Model of the Financial Market with the Minkowski Metric. Zeitschrift für Naturforschung A 73: 669–84. [Google Scholar] [CrossRef]
- Rindler, Wolfgang. 1982. Introduction to Special Relativity. Oxford: Oxford University Press. [Google Scholar]
- Romero, Juan M., and Ilse B. Zubieta-Martínez. 2016. Relativistic Quantum Finance. arXiv arXiv:1604.01447. [Google Scholar]
- Romero, Juan M., Ulises Lavana, and Elio Martínez. 2013. Schrödinger group and quantum finance. arXiv arXiv:1304.4995. [Google Scholar]
- Samuelson, Paul A. 1965. Proof That Properly Anticipated Prices Fluctuate Randomly. Management Review 6: 41–49. [Google Scholar]
- Saptsin, Vladimir, and Vladimir Soloviev. 2009. Relativistic quantum econophysics—New paradigms in complex systems modelling. arXiv arXiv:0907.1142. [Google Scholar]
- Savoiu, Gheorghe, and Ion Iorga Siman. 2013. History and role of econophysics in scientific research. In Econophysics Background and Applications in Economics, Finance, and Sociophysics. Edited by Gheorghe Săvoiu. Oxford: Academic Press, Chapter 1. pp. 3–16. [Google Scholar]
- Schinkus, Christophe. 2010. Is Econophysics a new discipline. Physica A 389: 3814–21. [Google Scholar] [CrossRef]
- Siklos, Stephen. 2011. Special Relativity. Cambridge: University of Cambridge. [Google Scholar]
- Stanley, H. Eugene, Viktor Afanasyev, Luis A. Nunes Amaral, Serguei V. Buldyrev, Ary L. Goldberger, Steve Havlin, Harry Leschhorn, Philipp H. Maass, Rosario N. Mantegna, C.-K. Peng, and et al. 1996. Anomalous fluctuations in the dynamics of complex systems: From DNA and physiology to econophysics. Physica A: Statistical Mechanics and Its Applications 224: 302–21. [Google Scholar] [CrossRef]
- Tenreiro Machado, J. A. 2014. Relativistic time effects in financial dynamics. Nonlinear Dynamics 75: 735–44. [Google Scholar] [CrossRef]
- Trzetrzelewski, Maciej. 2017. Relativistic Black-Scholes model. Europhysics Letters 117: 1–18. [Google Scholar] [CrossRef][Green Version]
- Wissner-Gross, Alexander D., and Cameron E. Freer. 2010. Relativistic statistical arbitrage. Physical Review E-Statistical, Nonlinear, and Soft Matter Physics 82: 1–7. [Google Scholar] [CrossRef] [PubMed]
- Zumbach, Gilles O. 2007. Time Reversal Invariance in Finance. SSRN Electronic Journal. [Google Scholar] [CrossRef][Green Version]
| 1 | The notion of spacetime is explained in Section 3. |
| 2 | The distance between the planets is not always the same. Planets have elliptical orbits around the Sun. All planets have different elliptics; therefore, the distance between them is not constant. |
| 3 | The term “event” also has a wider meaning—it can define a happening or an object. |
| 4 | It allows to create a metric tensor to perform coordinate transformations between different inertial reference frames. |
| 5 | Only three-dimensions are represented in Figure 1. |
| 6 | Recall m/s in a vacuum. |
| 7 | |
| 8 | The extension of this setup to other spacetime formulations is possible. For the purpose of this paper, the simplest Minkowski spacetime definition suffices. |
| 9 | |
| 10 | That is why, in some of the literature, proper time is also referred to as proper distance or a Minkowski interval. |
| 11 | This example can be understood as an adaptation, to a financial setting, of the well-known “Twin Paradox” (Siklos 2011). |
| 12 | Or other characteristic of the asset. For illustration purpose, we consider the price. |
| 13 | For this to happen MP and MP must have different pricing models for the asset price, as they experienced different time spans between their meetings. For instance, travelling in space for MP may be modelled using price jumps to account to for the time dilation experience, particularly when MP turns back and sees MP passing from to . |
| 14 | |
| 15 | Assuming only time dilation effects and not proper time. |
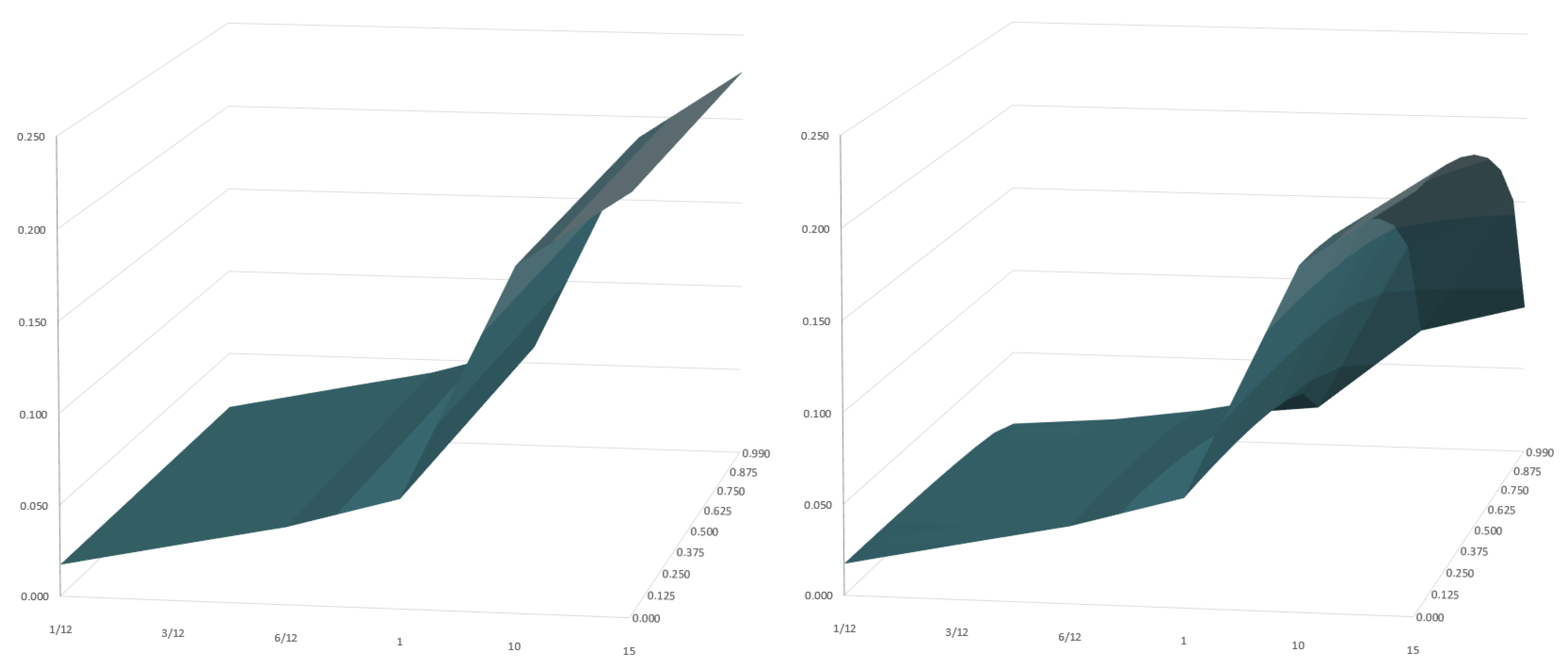



| Velocity 0.0% of c | Velocity 12.5% of c | Velocity 25.0% of c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| T | Call | Call’ | Call/Call’ | T | Call | Call’ | Call/Call’ | T | Call | Call’ | Call/Call’ |
| 1/12 | 1.73% | 1.73% | 1.00000 | 1/12 | 1.73% | 1.72% | 1.00394 | 1/12 | 1.73% | 1.70% | 1.01626 |
| 3/12 | 2.99% | 2.99% | 1.00000 | 3/12 | 2.99% | 2.98% | 1.00394 | 3/12 | 2.99% | 2.94% | 1.01626 |
| 6/12 | 4.23% | 4.23% | 1.00000 | 6/12 | 4.23% | 4.21% | 1.00394 | 6/12 | 4.23% | 4.16% | 1.01625 |
| 1 | 5.98% | 5.98% | 1.00000 | 1 | 5.98% | 5.96% | 1.00394 | 1 | 5.98% | 5.88% | 1.01624 |
| 10 | 18.75% | 18.75% | 1.00000 | 10 | 18.75% | 18.68% | 1.00387 | 10 | 18.75% | 18.45% | 1.01597 |
| 15 | 22.85% | 22.85% | 1.00000 | 15 | 22.85% | 22.77% | 1.00384 | 15 | 22.85% | 22.50% | 1.01582 |
| Velocity 37.5% of c | Velocity 50.0% of c | Velocity 62.5% of c | |||||||||
| T | Call | Call’ | Call/Call | T | Call | Call’ | Call/Call’ | T | Call | Call’ | Call/Call’ |
| 1/12 | 1.73% | 1.66% | 1.03861 | 1/12 | 1.73% | 1.61% | 1.07456 | 1/12 | 1.73% | 1.53% | 1.13180 |
| 3/12 | 2.99% | 2.88% | 1.03860 | 3/12 | 2.99% | 2.78% | 1.07454 | 3/12 | 2.99% | 2.64% | 1.13177 |
| 6/12 | 4.23% | 4.07% | 1.03858 | 6/12 | 4.23% | 3.94% | 1.07450 | 6/12 | 4.23% | 3.74% | 1.13171 |
| 1 | 5.98% | 5.76% | 1.03854 | 1 | 5.98% | 5.56% | 1.07444 | 1 | 5.98% | 5.28% | 1.13159 |
| 10 | 18.75% | 18.06% | 1.03791 | 10 | 18.75% | 17.47% | 1.07323 | 10 | 18.75% | 16.60% | 1.12951 |
| 15 | 22.85% | 22.03% | 1.03756 | 15 | 22.85% | 21.31% | 1.07257 | 15 | 22.85% | 20.25% | 1.12837 |
| Velocity 75.0% of c | Velocity 87.5% of c | Velocity 99.0% of c | |||||||||
| T | Call | Call’ | Call/Call’ | T | Call | Call’ | Call/Call’ | T | Call | Call’ | Call/Call’ |
| 1/12 | 1.73% | 1.40% | 1.22954 | 1/12 | 1.73% | 1.20% | 1.43716 | 1/12 | 1.73% | 0.65% | 2.66230 |
| 3/12 | 2.99% | 2.43% | 1.22948 | 3/12 | 2.99% | 2.08% | 1.43704 | 3/12 | 2.99% | 1.12% | 2.66195 |
| 6/12 | 4.23% | 3.44% | 1.22938 | 6/12 | 4.23% | 2.94% | 1.43687 | 6/12 | 4.23% | 1.59% | 2.66141 |
| 1 | 5.98% | 4.86% | 1.22919 | 1 | 5.98% | 4.16% | 1.43652 | 1 | 5.98% | 2.25% | 2.66034 |
| 10 | 18.75% | 15.30% | 1.22570 | 10 | 18.75% | 13.11% | 1.43032 | 10 | 18.75% | 7.10% | 2.64122 |
| 15 | 22.85% | 18.68% | 1.22379 | 15 | 22.85% | 16.02% | 1.42691 | 15 | 22.85% | 8.69% | 2.63072 |
| Velocity 0.0% of c | Velocity 12.5% of c | Velocity 25.0% of c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | |||
| 1% | 0.40% | 0.40% | 1.0000 | 1% | 0.40% | 0.40% | 1.0039 | 1% | 0.40% | 0.39% | 1.0163 |
| 5% | 1.99% | 1.99% | 1.0000 | 5% | 1.99% | 1.99% | 1.0039 | 5% | 1.99% | 1.96% | 1.0163 |
| 10% | 3.99% | 3.99% | 1.0000 | 10% | 3.99% | 3.97% | 1.0039 | 10% | 3.99% | 3.92% | 1.0163 |
| 15% | 5.98% | 5.98% | 1.0000 | 15% | 5.98% | 5.96% | 1.0039 | 15% | 5.98% | 5.88% | 1.0162 |
| 20% | 7.97% | 7.97% | 1.0000 | 20% | 7.97% | 7.93% | 1.0039 | 20% | 7.97% | 7.84% | 1.0162 |
| 25% | 9.95% | 9.95% | 1.0000 | 25% | 9.95% | 9.91% | 1.0039 | 25% | 9.95% | 9.79% | 1.0162 |
| 30% | 11.92% | 11.92% | 1.0000 | 30% | 11.92% | 11.88% | 1.0039 | 30% | 11.92% | 11.73% | 1.0161 |
| Velocity 37.5% of c | Velocity 50.0% of c | Velocity 62.5% of c | |||||||||
| Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | |||
| 1% | 0.40% | 0.38% | 1.0386 | 1% | 0.40% | 0.37% | 1.0476 | 1% | 0.40% | 0.35% | 1.1318 |
| 5% | 1.99% | 1.92% | 1.0386 | 5% | 1.99% | 1.86% | 1.0746 | 5% | 1.99% | 1.76% | 1.1318 |
| 10% | 3.99% | 3.84% | 1.0386 | 10% | 3.99% | 3.71% | 1.0745 | 10% | 3.99% | 3.52% | 1.1317 |
| 15% | 5.98% | 5.76% | 1.0385 | 15% | 5.98% | 5.56% | 1.0744 | 15% | 5.98% | 5.28% | 1.1316 |
| 20% | 7.97% | 7.67% | 1.0385 | 20% | 7.97% | 7.41% | 1.0743 | 20% | 7.97% | 7.04% | 1.1314 |
| 25% | 9.95% | 9.58% | 1.0384 | 25% | 9.95% | 9.26% | 1.0742 | 25% | 9.95% | 8.79% | 1.1312 |
| 30% | 11.92% | 11.48% | 1.0383 | 30% | 11.92% | 11.10% | 1.0740 | 30% | 11.92% | 10.54% | 1.1309 |
| Velocity 75.0% of c | Velocity 87.5% of c | Velocity 99.0% of c | |||||||||
| Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | Call | Call’ | Call/Call’ | |||
| 1% | 0.40% | 0.28% | 1.2296 | 1% | 0.40% | 0.28% | 1.4372 | 1% | 0.40% | 0.15% | 2.6625 |
| 5% | 1.99% | 1.39% | 1.2295 | 5% | 1.99% | 1.39% | 1.4371 | 5% | 1.99% | 0.75% | 2.6622 |
| 10% | 3.99% | 2.78% | 1.2294 | 10% | 3.99% | 2.78% | 1.4369 | 10% | 3.99% | 1.50% | 2.6615 |
| 15% | 5.98% | 4.16% | 1.2292 | 15% | 5.98% | 4.16% | 1.4365 | 15% | 5.98% | 2.25% | 2.6603 |
| 20% | 7.97% | 5.55% | 1.2289 | 20% | 7.97% | 5.55% | 1.4360 | 20% | 7.97% | 3.00% | 2.6587 |
| 25% | 9.95% | 6.93% | 1.2285 | 25% | 9.95% | 6.93% | 1.4353 | 25% | 9.95% | 3.74% | 2.6565 |
| 30% | 11.92% | 8.31% | 1.2280 | 30% | 11.92% | 8.31% | 1.4344 | 30% | 11.92% | 4.49% | 2.6539 |
| Velocity 0.0% of c | Velocity 12.5% of c | Velocity 25.0% of c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | |||
| 1% | −0.001995 | −0.001995 | 1.000000 | 1% | −0.001995 | −0.001987 | 1.003945 | 1% | −0.001995 | −0.001963 | 1.016265 |
| 5% | −0.009970 | −0.009970 | 1.000000 | 5% | −0.009970 | −0.009931 | 1.003942 | 5% | −0.009970 | −0.009811 | 1.016255 |
| 10% | −0.019922 | −0.019922 | 1.000000 | 10% | −0.019922 | −0.019844 | 1.003935 | 10% | −0.019922 | −0.019604 | 1.016225 |
| 15% | −0.029837 | −0.029837 | 1.000000 | 15% | −0.029837 | −0.029720 | 1.003923 | 15% | −0.029837 | −0.029362 | 1.016175 |
| 20% | −0.039695 | −0.039695 | 1.000000 | 20% | −0.039695 | −0.039541 | 1.003905 | 20% | −0.039695 | −0.039066 | 1.016104 |
| 25% | −0.049480 | −0.049480 | 1.000000 | 25% | −0.049480 | −0.049288 | 1.003883 | 25% | −0.049480 | −0.048700 | 1.016013 |
| 30% | −0.059172 | −0.059172 | 1.000000 | 30% | −0.059172 | −0.058945 | 1.003856 | 30% | −0.059172 | −0.058246 | 1.015903 |
| Velocity 37.5% of c | Velocity 50.0% of c | Velocity 62.5% of c | |||||||||
| Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | |||
| 1% | −0.001995 | −0.001921 | 1.038613 | 1% | −0.001995 | −0.001856 | 1.074568 | 1% | −0.001995 | −0.001762 | 1.131821 |
| 5% | −0.009970 | −0.009600 | 1.038591 | 5% | −0.009970 | −0.009279 | 1.074525 | 5% | −0.009970 | −0.008810 | 1.131746 |
| 10% | −0.019922 | −0.019183 | 1.038520 | 10% | −0.019922 | −0.018543 | 1.074390 | 10% | −0.019922 | −0.017607 | 1.131514 |
| 15% | −0.029837 | −0.028733 | 1.038401 | 15% | −0.029837 | −0.027777 | 1.074165 | 15% | −0.029837 | −0.026378 | 1.131126 |
| 20% | −0.039695 | −0.038233 | 1.038235 | 20% | −0.039695 | −0.036965 | 1.073850 | 20% | −0.039695 | −0.035110 | 1.130583 |
| 25% | −0.049480 | −0.047667 | 1.038022 | 25% | −0.049480 | −0.046094 | 1.073446 | 25% | −0.049480 | −0.043792 | 1.129886 |
| 30% | −0.059172 | −0.057019 | 1.037762 | 30% | −0.059172 | −0.055149 | 1.072952 | 30% | −0.059172 | −0.052409 | 1.129034 |
| Velocity 75.0% of c | Velocity 87.5% of c | Velocity 99.0% of c | |||||||||
| Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | Theta | Theta’ | Theta/Theta’ | |||
| 1% | −0.001995 | −0.001622 | 1.229571 | 1% | −0.001995 | −0.001388 | 1.437207 | 1% | −0.001995 | −0.000749 | 2.662454 |
| 5% | −0.009970 | −0.008110 | 1.229446 | 5% | −0.009970 | −0.006938 | 1.436985 | 5% | −0.009970 | −0.003746 | 2.661768 |
| 10% | −0.019922 | −0.016209 | 1.229056 | 10% | −0.019922 | −0.013871 | 1.436290 | 10% | −0.019922 | −0.007491 | 2.659625 |
| 15% | −0.029837 | −0.024289 | 1.228406 | 15% | −0.029837 | −0.020790 | 1.435133 | 15% | −0.029837 | −0.011233 | 2.656058 |
| 20% | −0.039695 | −0.032338 | 1.227497 | 20% | −0.039695 | −0.027691 | 1.433514 | 20% | −0.039695 | −0.014973 | 2.651072 |
| 25% | −0.049480 | −0.040348 | 1.226328 | 25% | −0.049480 | −0.034566 | 1.431436 | 25% | −0.049480 | −0.018709 | 2.644676 |
| 30% | −0.059172 | −0.048307 | 1.224902 | 30% | −0.059172 | −0.041411 | 1.428900 | 30% | −0.059172 | −0.022440 | 2.636879 |
| Velocity 0.0% of c | Velocity 12.5% of c | Velocity 25.0% of c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | |||
| 1% | 0.398937 | 0.398937 | 1.000000 | 1% | 0.398937 | 0.397370 | 1.003945 | 1% | 0.398937 | 0.392552 | 1.016265 |
| 5% | 0.398818 | 0.398818 | 1.000000 | 5% | 0.398818 | 0.397252 | 1.003942 | 5% | 0.398818 | 0.392438 | 1.016255 |
| 10% | 0.398444 | 0.398444 | 1.000000 | 10% | 0.398444 | 0.396882 | 1.003935 | 10% | 0.398444 | 0.392082 | 1.016225 |
| 15% | 0.397822 | 0.397822 | 1.000000 | 15% | 0.397822 | 0.396267 | 1.003923 | 15% | 0.397822 | 0.391490 | 1.016175 |
| 20% | 0.396953 | 0.396953 | 1.000000 | 20% | 0.396953 | 0.395408 | 1.003905 | 20% | 0.396953 | 0.390661 | 1.016104 |
| 25% | 0.395838 | 0.395838 | 1.000000 | 25% | 0.395838 | 0.394306 | 1.003883 | 25% | 0.395838 | 0.389599 | 1.016013 |
| 30% | 0.394479 | 0.394479 | 1.000000 | 30% | 0.394479 | 0.392964 | 1.003856 | 30% | 0.394479 | 0.388304 | 1.015903 |
| Velocity 37.5% of c | Velocity 50.0% of c | Velocity 62.5% of c | |||||||||
| Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | |||
| 1% | 0.398937 | 0.384106 | 1.038613 | 1% | 0.398937 | 0.371254 | 1.074568 | 1% | 0.398937 | 0.324452 | 1.131821 |
| 5% | 0.398818 | 0.383999 | 1.038591 | 5% | 0.398818 | 0.371157 | 1.074525 | 5% | 0.398818 | 0.324388 | 1.131746 |
| 10% | 0.398444 | 0.383665 | 1.038520 | 10% | 0.398444 | 0.370856 | 1.074390 | 10% | 0.398444 | 0.324187 | 1.131514 |
| 15% | 0.397822 | 0.383110 | 1.038401 | 15% | 0.397822 | 0.370354 | 1.074165 | 15% | 0.397822 | 0.323852 | 1.131126 |
| 20% | 0.396953 | 0.382334 | 1.038235 | 20% | 0.396953 | 0.369654 | 1.073850 | 20% | 0.396953 | 0.323384 | 1.130583 |
| 25% | 0.395838 | 0.381338 | 1.038022 | 25% | 0.395838 | 0.368754 | 1.073446 | 25% | 0.395838 | 0.322783 | 1.129886 |
| 30% | 0.394479 | 0.380125 | 1.037762 | 30% | 0.394479 | 0.367658 | 1.072952 | 30% | 0.394479 | 0.322050 | 1.129034 |
| Velocity 75.0% of c | Velocity 87.5% of c | Velocity 99.0% of c | |||||||||
| Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | Vega | Vega’ | Vega/Vega’ | |||
| 1% | 0.398937 | 0.324452 | 1.229571 | 1% | 0.398937 | 0.277578 | 1.437207 | 1% | 0.398937 | 0.149838 | 2.662454 |
| 5% | 0.398818 | 0.324388 | 1.229446 | 5% | 0.398818 | 0.277538 | 1.436985 | 5% | 0.398818 | 0.149832 | 2.661768 |
| 10% | 0.398444 | 0.324187 | 1.229056 | 10% | 0.398444 | 0.277412 | 1.436290 | 10% | 0.398444 | 0.149812 | 2.659625 |
| 15% | 0.397822 | 0.323852 | 1.228406 | 15% | 0.397822 | 0.277202 | 1.435133 | 15% | 0.397822 | 0.149779 | 2.656058 |
| 20% | 0.396953 | 0.323384 | 1.227497 | 20% | 0.396953 | 0.276909 | 1.433514 | 20% | 0.396953 | 0.149733 | 2.651072 |
| 25% | 0.395838 | 0.322783 | 1.226328 | 25% | 0.395838 | 0.276532 | 1.431436 | 25% | 0.395838 | 0.149673 | 2.644676 |
| 30% | 0.394479 | 0.322050 | 1.224902 | 30% | 0.394479 | 0.276072 | 1.428900 | 30% | 0.394479 | 0.149601 | 2.636879 |
| Velocity 0.0% of c | Velocity 12.5% of c | Velocity 25.0% of c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | |||
| 1% | 0.40% | 0.40% | 0.000% | 1% | 0.40% | 0.40% | 0.002% | 1% | 0.40% | 0.39% | 0.006% |
| 5% | 1.99% | 1.99% | 0.000% | 5% | 1.99% | 1.99% | 0.008% | 5% | 1.99% | 1.96% | 0.032% |
| 10% | 3.99% | 3.99% | 0.000% | 10% | 3.99% | 3.97% | 0.016% | 10% | 3.99% | 3.92% | 0.064% |
| 15% | 5.98% | 5.98% | 0.000% | 15% | 5.98% | 5.96% | 0.023% | 15% | 5.98% | 5.88% | 0.096% |
| 20% | 7.97% | 7.97% | 0.000% | 20% | 7.97% | 7.93% | 0.031% | 20% | 7.97% | 7.84% | 0.127% |
| 25% | 9.95% | 9.95% | 0.000% | 25% | 9.95% | 9.91% | 0.039% | 25% | 9.95% | 9.79% | 0.158% |
| 30% | 11.92% | 11.92% | 0.000% | 30% | 11.92% | 11.88% | 0.047% | 30% | 11.92% | 11.73% | 0.189% |
| Velocity 37.5% of c | Velocity 50.0% of c | Velocity 62.5% of c | |||||||||
| Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | |||
| 1% | 0.40% | 0.38% | 0.015% | 1% | 0.40% | 0.37% | 0.028% | 1% | 0.40% | 0.35% | 0.046% |
| 5% | 1.99% | 1.92% | 0.074% | 5% | 1.99% | 1.86% | 0.138% | 5% | 1.99% | 1.76% | 0.232% |
| 10% | 3.99% | 3.84% | 0.148% | 10% | 3.99% | 3.71% | 0.277% | 10% | 3.99% | 3.52% | 0.464% |
| 15% | 5.98% | 5.76% | 0.222% | 15% | 5.98% | 5.56% | 0.414% | 15% | 5.98% | 5.28% | 0.695% |
| 20% | 7.97% | 7.67% | 0.295% | 20% | 7.97% | 7.41% | 0.551% | 20% | 7.97% | 7.04% | 0.925% |
| 25% | 9.95% | 9.58% | 0.368% | 25% | 9.95% | 9.26% | 0.687% | 25% | 9.95% | 8.79% | 1.154% |
| 30% | 11.92% | 11.48% | 0.440% | 30% | 11.92% | 11.10% | 0.822% | 30% | 11.92% | 10.54% | 1.380% |
| Velocity 75.0% of c | Velocity 87.5% of c | Velocity 99.0% of c | |||||||||
| Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | Call | Call’ | Call-Put’ = Put-Call’ | |||
| 1% | 0.40% | 0.28% | 0.121% | 1% | 0.40% | 0.28% | 0.121% | 1% | 0.40% | 0.15% | 0.249% |
| 5% | 1.99% | 1.39% | 0.607% | 5% | 1.99% | 1.39% | 0.607% | 5% | 1.99% | 0.75% | 1.245% |
| 10% | 3.99% | 2.78% | 1.213% | 10% | 3.99% | 2.78% | 1.213% | 10% | 3.99% | 1.50% | 2.489% |
| 15% | 5.98% | 4.16% | 1.817% | 15% | 5.98% | 4.16% | 1.817% | 15% | 5.98% | 2.25% | 3.731% |
| 20% | 7.97% | 5.55% | 2.418% | 20% | 7.97% | 5.55% | 2.418% | 20% | 7.97% | 3.00% | 4.970% |
| 25% | 9.95% | 6.93% | 3.017% | 25% | 9.95% | 6.93% | 3.017% | 25% | 9.95% | 3.74% | 6.203% |
| 30% | 11.92% | 8.31% | 3.611% | 30% | 11.92% | 8.31% | 3.611% | 30% | 11.92% | 4.49% | 7.431% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carvalho, V.H.; Gaspar, R.M. Relativistic Option Pricing. Int. J. Financial Stud. 2021, 9, 32. https://doi.org/10.3390/ijfs9020032
Carvalho VH, Gaspar RM. Relativistic Option Pricing. International Journal of Financial Studies. 2021; 9(2):32. https://doi.org/10.3390/ijfs9020032
Chicago/Turabian StyleCarvalho, Vitor H., and Raquel M. Gaspar. 2021. "Relativistic Option Pricing" International Journal of Financial Studies 9, no. 2: 32. https://doi.org/10.3390/ijfs9020032
APA StyleCarvalho, V. H., & Gaspar, R. M. (2021). Relativistic Option Pricing. International Journal of Financial Studies, 9(2), 32. https://doi.org/10.3390/ijfs9020032







