Robust Optimization-Based Commodity Portfolio Performance
Abstract
1. Introduction
2. Data and Methodology
2.1. Commodity Sample and Returns Construction
2.2. Robust Optimization
2.3. Algorithms for Robust Optimization under Uncertainty
2.4. Performance Metrics
3. Results
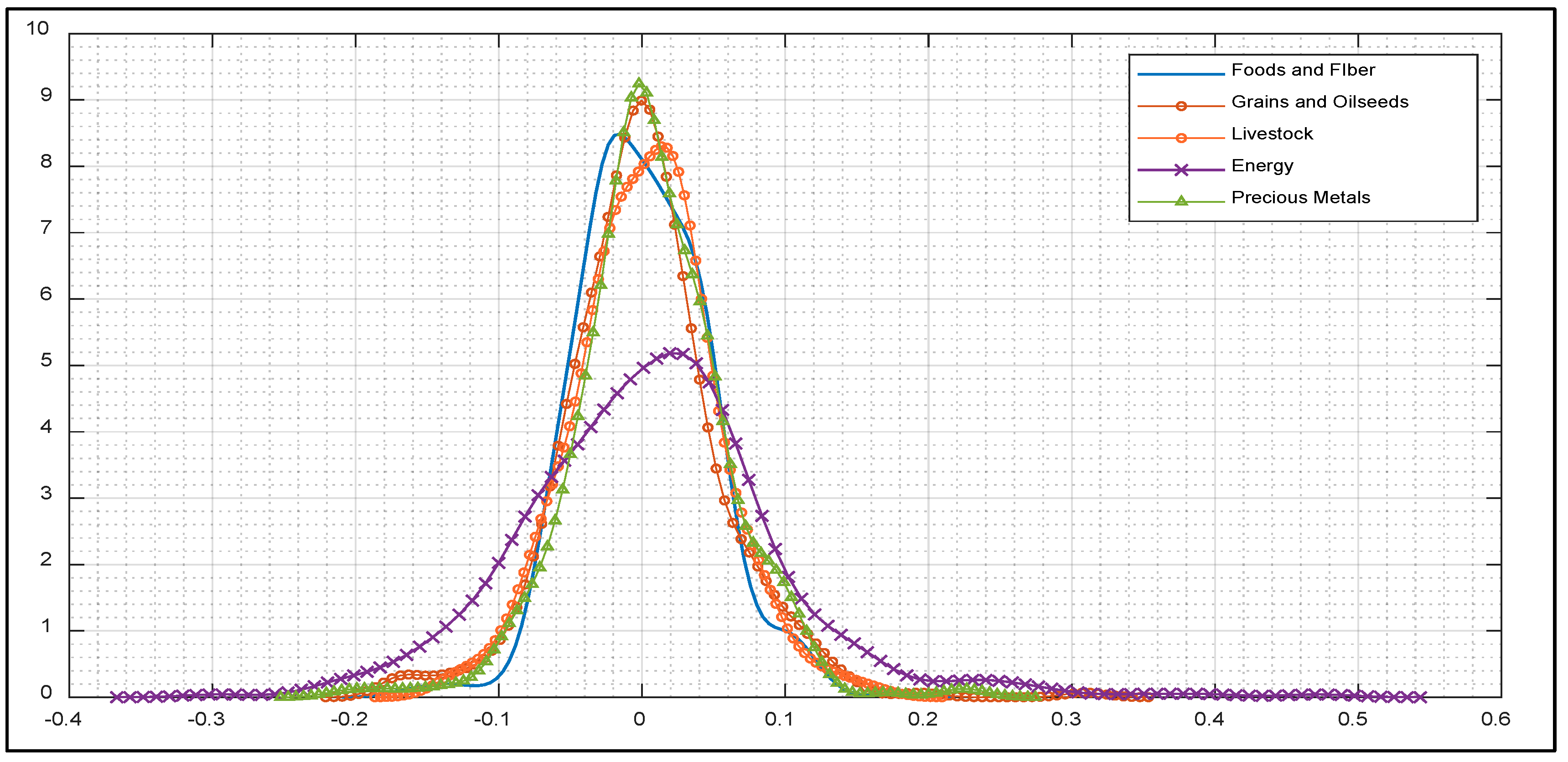
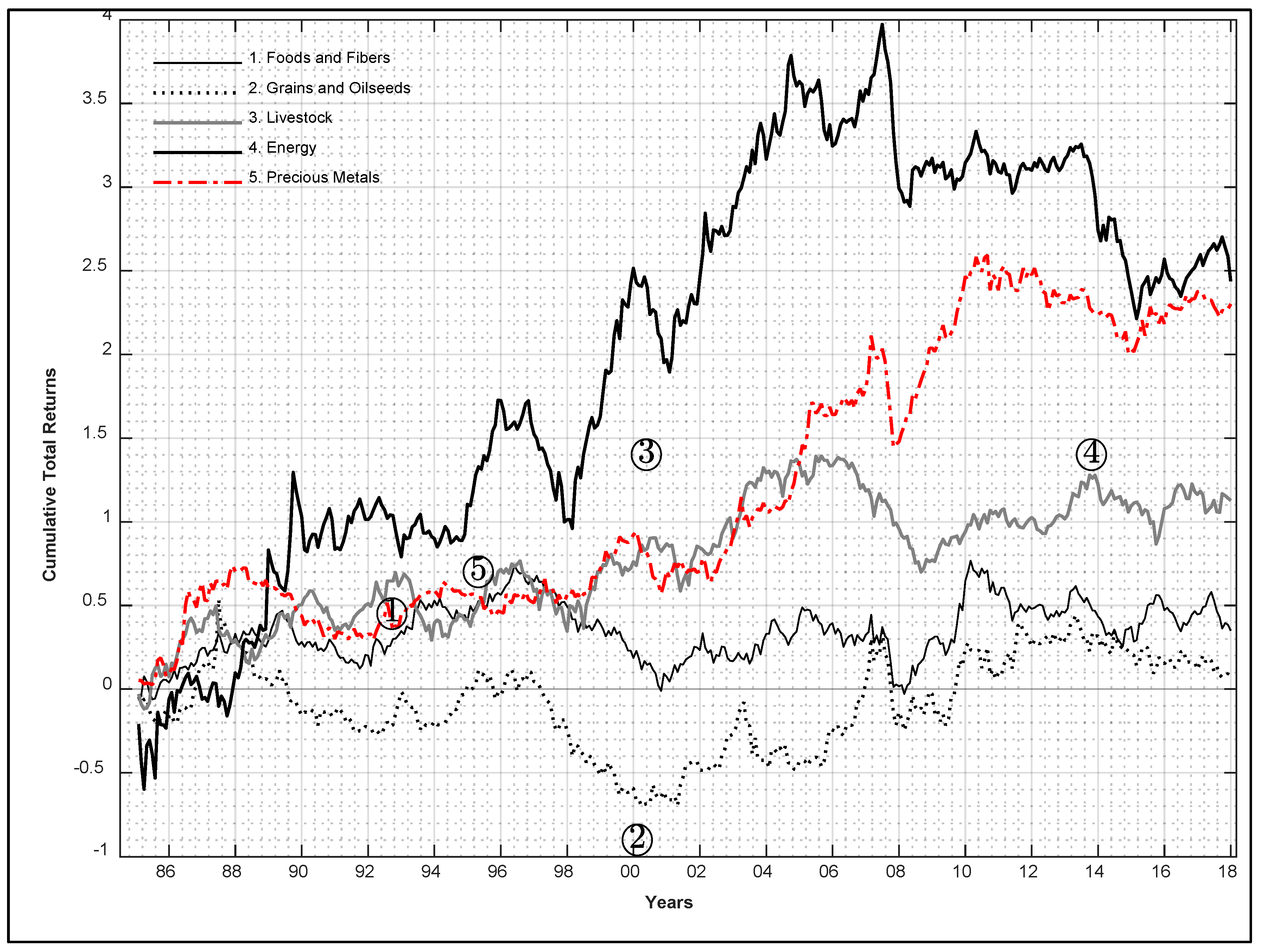
3.1. Sectoral Risk and Returns Analysis
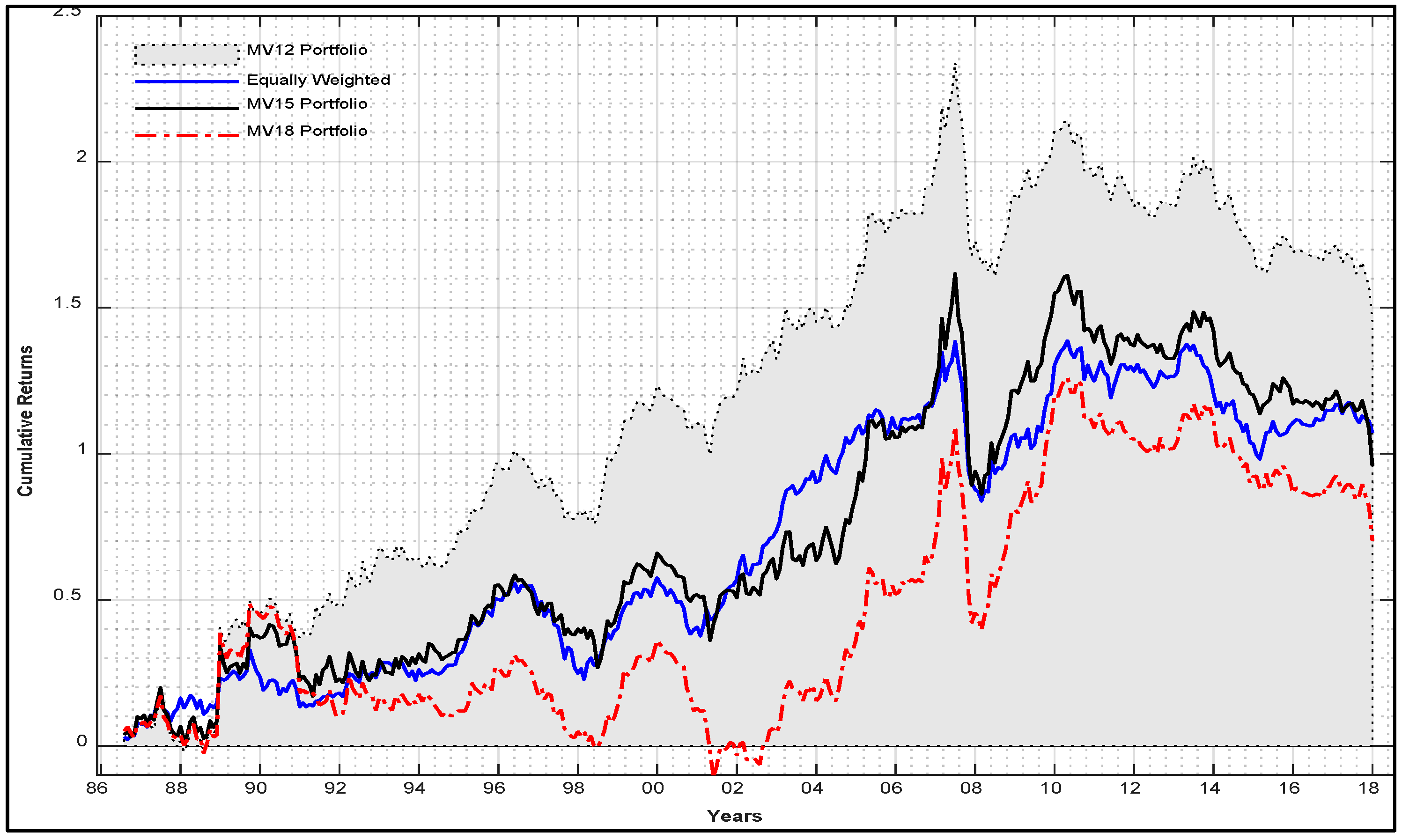
3.2. Portfolio Risk and Returns
3.3. Optimal Performance by Holding Period
4. Concluding Remarks
Author Contributions
Funding
Conflicts of Interest
References
- Adhikari, Ramesh, and Kyle J. Putnam. 2020. Comovement in the Commodity Futures Markets: An Analysis of the Energy, Grains, and Livestock Sectors. Journal of Commodity Markets 18: 100090. [Google Scholar] [CrossRef]
- Asness, Clifford S., Tobias J. Moskowitz, and Lasse Heje Pedersen. 2013. Value and Momentum Everywhere. Journal of Finance 68: 929–85. [Google Scholar] [CrossRef]
- Bakshi, Gurdip, Xiaohui Gao, and Alberto G. Rossi. 2017. Understanding the Sources of Risk Underlying the Cross Section of Commodity Returns. Management Science 65: 619–41. [Google Scholar] [CrossRef]
- Best, Michael J., and Robert R. Grauer. 1991. On the Sensitivity of Mean-Variance-Efficient Portfolios to Changes in Asset Means: Some Analytical and Computational Results. Review of Financial Studies 4: 315–432. [Google Scholar] [CrossRef]
- Bhardwaj, Geetesh, Gary Gorton, and K. Geert Rouwenhorst. 2015. Facts and Fantasies about Commodity Futures Ten Years Later. NBER Working Paper Series; New York: National Bureau of Economic Research, Inc., pp. 1–29. [Google Scholar]
- Bi, Junna, Hanqing Jin, and Qingbin Meng. 2018. Behavioral Mean-Variance Portfolio Selection. European Journal of Operational Research 271: 644–63. [Google Scholar] [CrossRef]
- Black, Fischer, and Robert Litterman. 1992. Global Portfolio Optimization. Financial Analysts Journal 48: 28–43. [Google Scholar] [CrossRef]
- Calafiore, Giuseppe C. 2007. Ambiguous Risk Measures and Optimal Robust Portfolios. SIAM Journal on Optimization 18: 853–77. [Google Scholar] [CrossRef]
- Ceria, Sebastián, and Robert A. Stubbs. 2006. Incorporating Estimation Errors into Portfolio Selection: Robust Portfolio Construction. Journal of Asset Management 7: 109–27. [Google Scholar] [CrossRef]
- DeMiguel, Victor, Lorenzo Garlappi, and Raman Uppal. 2009. Optimal versus Naive Diversification: How Inefficient Is the 1/N Portfolio Strategy? Review of Financial Studies 22: 1915–53. [Google Scholar] [CrossRef]
- Elliott, Robert J., and Tak Kuen Siu. 2010. On Risk Minimizing Portfolios under a Markovian Regime-Switching Black-Scholes Economy. Annals of Operations Research 176: 271–91. [Google Scholar] [CrossRef]
- Erb, Claude B., and Campbell R. Harvey. 2006. The Strategic and Tactical Value of Commodity Futures. Financial Analysts Journal 62: 125–78. [Google Scholar] [CrossRef]
- Fan, Jianqing, Yingying Fan, and Jinchi Lv. 2008. High Dimensional Covariance Matrix Estimation Using a Factor Model. Journal of Econometrics 147: 186–97. [Google Scholar] [CrossRef]
- Goldfarb, Donald, and Garud Iyengar. 2003. Robust Portfolio Selection Problems. Mathematics of Operations Research 28: 1–38. [Google Scholar] [CrossRef]
- Gorton, Gary, and K. Geert Rouwenhorst. 2006. Facts and Fantasies about Commodity Futures. Financial Analysts Journal 62: 47–68. [Google Scholar] [CrossRef]
- Grauer, Robert R., and Frederick C. Shen. 2000. Do Constraints Improve Portfolio Performance? Journal of Banking and Finance 24: 1253–74. [Google Scholar] [CrossRef]
- Green, Richard C., and Burton Hollifield. 1992. When Will Mean-Variance Efficient Portfolios Be Well Diversified? The Journal of Finance 47: 1785–809. [Google Scholar] [CrossRef]
- Huang, Xiaoxia, and Tingting Yang. 2020. How Does Background Risk Affect Portfolio Choice: An Analysis Based on Uncertain Mean-Variance Model with Background Risk. Journal of Banking and Finance 111: 105726. [Google Scholar] [CrossRef]
- Huo, Lijuan, Tae-Hwan Kim, and Yunmi Kim. 2012. Robust Estimation of Covariance and Its Application to Portfolio Optimization. Finance Research Letters 9: 121–34. [Google Scholar] [CrossRef]
- Jensen, Michael C. 1968. Problems in the Selection of Security Portfolios—The Performance of Mutual Funds in the Period 1945–1964. Journal of Finance 23: 389–416. [Google Scholar] [CrossRef]
- Jorion, Philippe. 1986. Bayes-Stein Estimation for Portfolio Analysis. The Journal of Financial and Quantitative Analysis 21: 279–92. [Google Scholar] [CrossRef]
- Kim, Tae-Hwan, and Halbert White. 2004. On More Robust Estimation of Skewness and Kurtosis. Finance Research Letters 1: 56–73. [Google Scholar] [CrossRef]
- Kim, Jang Ho, Woo Chang Kim, and Frank J. Fabozzi. 2013. Composition of Robust Equity Portfolios. Finance Research Letters 10: 72–81. [Google Scholar] [CrossRef]
- Kim, Woo Chang, Jang Ho Kim, and Frank J. Fabozzi. 2016. Robust Equity Portfolio Management + Website: Formulations, Implementations and Properties Using MATLAB, 1st ed. Hoboken: John Wiley & Sons, Inc. [Google Scholar]
- Kim, Jang Ho, Woo Chang Kim, Do-Gyun Kwon, and Frank J. Fabozzi. 2017. Robust Equity Portfolio Performance. Annals of Operations Research 266: 293–312. [Google Scholar] [CrossRef]
- Lim, Andrew E. B., Jeyaveerasingam George Shanthikumar, and Gah-Yi Vahn. 2011. Conditional Value-at-Risk in Portfolio Optimization: Coherent but Fragile. Operations Research Letters 39: 163–71. [Google Scholar] [CrossRef]
- Lwin, Khin T., Rong Qu, and Bart L. MacCarthy. 2017. Mean-VaR Portfolio Optimization: A Nonparametric Approach. European Journal of Operational Research 260: 751–66. [Google Scholar] [CrossRef]
- Markowitz, Harry. 1952. Portfolio Selection. The Journal of Finance 7: 77–91. [Google Scholar]
- Michaud, Richard O. 1998. Efficient Asset Management: A Practical Guide to Stock Portfolio Optimization and Asset Allocation. Boston: Oxford University Press. [Google Scholar]
- Michaud, Richard O., and Robert Michaud. 2008. Estimation Error and Portfolio Optimization: A Resampling Solution. Journal of Investment Management 6: 8–28. [Google Scholar] [CrossRef]
- Miffre, Joëlle, and Georgios Rallis. 2007. Momentum Strategies in Commodity Futures Markets. Journal of Banking and Finance 31: 1863–86. [Google Scholar] [CrossRef]
- Moskowitz, Tobias J., Yao Hua Ooi, and Lasse Heje Pedersen. 2012. Time Series Momentum. Journal of Financial Economics 104: 228–50. [Google Scholar] [CrossRef]
- Natarajan, Karthik, Dessislava Pachamanova, and Melvyn Sim. 2009. Constructing Risk Measures from Uncertainty Sets. Operations Research 57: 1129–41. [Google Scholar] [CrossRef]
- Post, Thierry, Selçuk Karabatı, and Stelios Arvanitis. 2019. Robust Optimization of Forecast Combinations. International Journal of Forecasting 35: 910–26. [Google Scholar] [CrossRef]
- Rad, Hossein, Rand Kwong Yew Low, Joëlle Miffre, and Robert Faff. 2020. Does Sophistication of the Weighting Scheme Enhance the Performance of Long-Short Commodity Portfolios? Journal of Empirical Finance 58: 164–80. [Google Scholar] [CrossRef]
- Rockafellar, Ralph Tyrrell, and Stanislav Uryasev. 2000. Optimization of Conditional Value-at-Risk. The Journal of Risk 2: 21–41. [Google Scholar] [CrossRef]
- Scherer, Bernd. 2007. Can Robust Portfolio Optimisation Help to Build Better Portfolios? Journal of Asset Management 7: 374–87. [Google Scholar] [CrossRef]
- Sharpe, William F. 1964. Capital Asset Prices: A Theory of Market Equilibrium Under Conditions of Risk. The Journal of Finance 19: 425–42. [Google Scholar]
- Shen, Ruijun, and Shuzhong Zhang. 2008. Robust Portfolio Selection Based on a Multi-Stage Scenario Tree. European Journal of Operational Research 191: 864–87. [Google Scholar] [CrossRef]
- Szymanowska, Marta, Frans de Roon, Theo Nijman, and Rob van den Goorbergh. 2014. An Anatomy of Commodity Futures Risk Premia. Journal of Finance 69: 453–82. [Google Scholar] [CrossRef]
- Tütüncü, Reha H., and Mark Koenig. 2004. Robust Asset Allocation. Annals of Operations Research 132: 157–87. [Google Scholar] [CrossRef]
- Zakamulin, Valeriy. 2017. Superiority of Optimized Portfolios to Naive Diversification: Fact or Fiction? Finance Research Letters 22: 122–28. [Google Scholar] [CrossRef]
- Zhang, Jinqing, Zeyu Jin, and Yunbi An. 2017. Dynamic Portfolio Optimization with Ambiguity Aversion. Journal of Banking and Finance 79: 95–109. [Google Scholar] [CrossRef]
- Zhu, Shushang, and Masao Fukushima. 2009. Worst-Case Conditional Value-at-Risk with Application to Robust Portfolio Management. Operations Research 57: 1155–68. [Google Scholar] [CrossRef]
| 1 | In practice, there are many different definitions of robustness based on various mathematical formulations. See Kim et al. (2016) for an overview of several optimization methodologies. |
| 2 | It is worth noting that there is an elliptical constraint on the standard deviation of returns in Kim et al. (2017) that is not assumed in our robust estimation process which may impact on the discrepancy in findings. |
| 3 | Returns are always calculated on the same contract and we do not include the return on collateral associated with the futures contract in the calculation. |
| 4 | Asness et al. (2013) and Moskowitz et al. (2012) create a monthly series with the same procedure; specifically, to convert the daily returns to monthly returns the following formula is applied: . |
| 5 | In fact, when the past 24 months of return data are used in the robust portfolio optimization process, both the MV and CVaR portfolios further underperform the naïve buy-and-hold benchmark. |

| Sector | Commodities |
|---|---|
| Foods and Fibers | Cocoa, Coffee, Orange Juice, Sugar #11, Cotton, Lumber |
| Grains and Oilseeds | Corn #2, Oats, Rough Rice #2, Soybeans, Soybean Meal, Soybean Oil, Wheat, Barley, Canola |
| Livestock | Feeder Cattle, Live Cattle, Lean Hogs, Pork Bellies |
| Energy | Crude Oil, Heating Oil #2, Unleaded Gas, Natural Gas, Propane |
| Precious Metals | Copper, Gold, Palladium, Platinum, Silver |
| Performance Metric | Description |
|---|---|
| Arithmetic mean | Reported as the average monthly return expressed as an annualized percentage. |
| Standard deviation | Reported as the average monthly standard deviation expressed as an annualized percentage. |
| Geometric mean | Reported as the average monthly return expressed as an annualized percentage. |
| Cumulative return | Reported as the portfolio return over the full sample period. |
| Sample skewness | Reported as a monthly average. |
| Sample excess kurtosis | Reported as a monthly average. |
| Sharpe ratio | Reported as the average excess monthly return divided by the monthly standard deviation, where the risk-free rate is obtained from Ken French’s website. |
| Tracking error | Reported as the monthly average standard deviation of the difference between a commodity portfolio returns and the value-weighted market index of returns from the Center for Research in Security Prices (CRSP). The CRSP market index return is obtained from Ken French’s website. |
| Information ratio | Reported as the excess monthly return of a portfolio in excess of the CRSP value-weighted market index of returns divided by the tracking error. |
| CAPM alpha | Reported as the average monthly alpha computed following Jensen (1968) expressed as an annualized percentage, where the market factor is obtained from Ken French’s website. |
| CAPM beta | Reported as the average monthly beta computed following Sharpe (1964), where the market factor is obtained from Ken French’s website. |
| Treynor ratio | Reported as the average excess monthly return divided by the monthly portfolio beta, where the risk-free rate is obtained from Ken French’s website. |
| Sortino ratio | Reported as the average excess monthly return divided by the monthly standard deviation of negative asset returns, where the risk-free rate is obtained from Ken French’s website. |
| Historical 95% VaR | Reported as the average expected 1-month loss with 95% certainty, based on historical returns. |
| Normal 95% VaR | Reported as the average expected 1-month loss with 95% certainty, under normality. |
| Historical 95% CVaR | Reported as the average expected 1-month loss beyond the VaR with 95% certainty, based on normality. |
| Normal 95% CVaR | Reported as the average expected 1-month loss beyond the VaR with 95% certainty, based on historical returns. |
| M-square | Reported as the average monthly return of a portfolio plus the product of the average monthly Sharpe ratio of the equally weighted benchmark and the average deviation of the standard deviation of the portfolio under consideration from the benchmark portfolio. |
| Statistics | Foods | Grains | Livestock | Energy | Metals |
|---|---|---|---|---|---|
| Arithmetic Mean (%) | 1.0662 | 0.1996 | 3.4703 | 7.6406 | 7.2003 |
| Standard Deviation (%) | 15.7008 | 19.1108 | 17.0492 | 31.3845 | 18.2431 |
| Geometric Mean (%) | −0.1667 | −1.5985 | 1.9863 | 2.6406 | 5.4434 |
| Cumulative Returns (%) | 35.0131 | 6.5806 | 112.7373 | 243.7192 | 230.1109 |
| Sample Skewness | 0.1328 | 0.3862 | 0.0607 | 0.6909 | 0.0068 |
| Sample Excess Kurtosis | 0.9356 | 3.1046 | 0.4567 | 3.0975 | 2.3497 |
| Sharpe Ratio (%) | −0.0384 | −0.0446 | 0.0045 | 0.0390 | 0.0605 |
| Tracking Error (%) | 0.0560 | 0.0648 | 0.0646 | 0.0971 | 0.0607 |
| Information Ratio (%) | −0.1466 | −0.1377 | −0.0966 | −0.0302 | −0.0539 |
| CAPM Alpha (%) | 0.2201 | 0.2008 | 0.0442 | 0.1818 | 0.2621 |
| CAPM Beta | 0.0040 | 0.0008 | 0.0645 | 0.0339 | 0.0222 |
| Treynor Ratio (%) | −0.1460 | −0.1362 | −0.0964 | −0.0301 | −0.0539 |
| Sortino Ratio (%) | −0.0539 | −0.0630 | 0.0064 | 0.0605 | 0.0900 |
| Historical 95% VaR | 6.5260 | 8.6736 | 7.9052 | 13.5137 | 8.0517 |
| Normal 95% VaR | 7.3668 | 9.0577 | 7.8108 | 14.2868 | 8.0812 |
| Historical 95% CVaR | 9.0241 | 12.3231 | 10.1816 | 17.8220 | 11.4665 |
| Normal 95% CVaR | 9.2607 | 11.3630 | 9.8673 | 18.0726 | 10.2818 |
| M-Square (%) | −0.0081 | −0.0084 | −0.0063 | −0.0048 | −0.0038 |
| Statistics | EW | MV12 | MV15 | MV18 | CVaR12 | CVAR15 | CVaR18 |
|---|---|---|---|---|---|---|---|
| Arithmetic Mean (%) | 3.4571 | 4.7156 | 3.0853 | 2.2482 | 4.6876 | 2.3093 | 2.3355 |
| Standard Deviation (%) | 11.4143 | 15.5863 | 15.8840 | 16.0667 | 15.5513 | 15.6294 | 16.0739 |
| Geometric Mean (%) | 2.7800 | 3.4714 | 1.7792 | 0.9629 | 3.4493 | 1.0537 | 1.0478 |
| Cumulative Returns (%) | 107.2094 | 145.4249 | 95.8390 | 70.1003 | 144.5779 | 71.9832 | 72.7921 |
| Sample Skewness | −0.5860 | 0.6603 | −0.2534 | 0.8613 | 0.6664 | −0.2570 | 0.8595 |
| Sample Excess Kurtosis | 3.1129 | 12.0746 | 6.7088 | 10.6254 | 12.1978 | 6.1192 | 10.7345 |
| Sharpe Ratio (%) | 0.0095 | 0.0294 | 0.0003 | −0.0144 | 0.0290 | −0.0137 | −0.0129 |
| Tracking Error (%) | 0.0477 | 0.0555 | 0.0561 | 0.0573 | 0.0555 | 0.0560 | 0.0573 |
| Information Ratio (%) | −0.1181 | −0.0834 | −0.1057 | −0.1155 | −0.0838 | −0.1172 | −0.1142 |
| CAPM Alpha (%) | 0.1852 | 0.2218 | 0.2231 | 0.2014 | 0.2205 | 0.2084 | 0.2014 |
| CAPM Beta | 0.0153 | 0.0173 | 0.0114 | 0.0092 | 0.0173 | 0.0091 | 0.0096 |
| Treynor Ratio (%) | −0.1192 | −0.0823 | −0.1059 | −0.1129 | −0.0827 | −0.1174 | −0.1117 |
| Sortino Ratio (%) | 0.0129 | 0.0444 | 0.0004 | −0.0213 | 0.0437 | −0.0189 | −0.0190 |
| Historical 95% VaR | 5.0998 | 5.8583 | 6.7128 | 6.4342 | 5.8908 | 6.6751 | 6.3910 |
| Normal 95% VaR | 5.1362 | 7.0161 | 7.2886 | 7.4435 | 7.0017 | 7.2309 | 7.4398 |
| Historical 95% CVaR | 7.7301 | 9.2222 | 10.6792 | 10.2852 | 9.2187 | 10.7611 | 10.2213 |
| Normal 95% CVaR | 6.5130 | 8.8962 | 9.2046 | 9.3815 | 8.8776 | 9.1162 | 9.3787 |
| M-Square (%) | −0.0055 | −0.0047 | −0.0059 | −0.0066 | −0.0047 | −0.0065 | −0.0065 |
| Statistics | HP1 | HP3 | HP6 | HP9 | HP12 |
|---|---|---|---|---|---|
| Arithmetic Mean (%) | 4.7156 | 3.2670 | 3.2149 | 2.9777 | 2.8339 |
| Standard Deviation (%) | 15.5863 | 16.2129 | 16.1022 | 15.9408 | 16.4617 |
| Geometric Mean (%) | 3.4714 | 1.9352 | 1.9033 | 1.6893 | 1.4789 |
| Cumulative Returns (%) | 145.4249 | 101.3997 | 99.8060 | 92.5416 | 88.1293 |
| Sample Skewness | 0.6603 | 0.5784 | 0.5969 | 0.4159 | 0.7399 |
| Sample Excess Kurtosis | 12.0746 | 10.4462 | 10.0213 | 7.6699 | 6.2305 |
| Sharpe Ratio (%) | 0.0294 | 0.0034 | 0.0025 | −0.0016 | −0.0040 |
| Tracking Error (%) | 0.0555 | 0.0566 | 0.0561 | 0.0582 | 0.0589 |
| Information Ratio (%) | −0.0834 | −0.1023 | −0.1040 | −0.1035 | −0.1042 |
| CAPM Alpha (%) | 0.2218 | 0.2333 | 0.2404 | 0.1644 | 0.1804 |
| CAPM Beta | 0.0173 | 0.0115 | 0.0110 | 0.0149 | 0.0129 |
| Treynor Ratio (%) | −0.0823 | −0.1008 | −0.1025 | −0.1024 | −0.1026 |
| Sortino Ratio (%) | 0.0444 | 0.0050 | 0.0037 | −0.0024 | −0.0061 |
| Historical 95% VaR | 5.8583 | 6.5522 | 6.9092 | 6.5516 | 6.7393 |
| Normal 95% VaR | 7.0161 | 7.4301 | 7.3817 | 7.3243 | 7.5833 |
| Historical 95% CVaR | 9.2222 | 9.9578 | 10.2224 | 9.9530 | 9.9973 |
| Normal 95% CVaR | 8.8962 | 9.3858 | 9.3241 | 9.2472 | 9.5690 |
| M-Square (%) | −0.0047 | −0.0058 | −0.0058 | −0.0060 | −0.0061 |
| Statistics | HP1 | HP3 | HP6 | HP9 | HP12 |
|---|---|---|---|---|---|
| Arithmetic Mean (%) | 4.6878 | 3.1452 | 3.1313 | 2.9732 | 2.8119 |
| Standard Deviation (%) | 15.5513 | 16.1615 | 16.0486 | 15.9299 | 16.3438 |
| Geometric Mean (%) | 3.4495 | 1.8229 | 1.8290 | 1.6876 | 1.4750 |
| Cumulative Returns (%) | 144.5836 | 97.6744 | 97.2473 | 92.4031 | 87.4524 |
| Sample Skewness | 0.6665 | 0.5738 | 0.5911 | 0.4486 | 0.6995 |
| Sample Excess Kurtosis | 12.1978 | 10.5528 | 10.1023 | 7.8684 | 5.8570 |
| Sharpe Ratio (%) | 0.0290 | 0.0013 | 0.0011 | −0.0017 | −0.0044 |
| Tracking Error (%) | 0.0555 | 0.0564 | 0.0559 | 0.0582 | 0.0586 |
| Information Ratio (%) | −0.0838 | −0.1044 | −0.1055 | −0.1036 | −0.1051 |
| CAPM Alpha (%) | 0.2205 | 0.2345 | 0.2417 | 0.1643 | 0.1807 |
| CAPM Beta | 0.0173 | 0.0110 | 0.0106 | 0.0149 | 0.0128 |
| Treynor Ratio (%) | −0.0827 | −0.1028 | −0.1039 | −0.1024 | −0.1035 |
| Sortino Ratio (%) | 0.0437 | 0.0019 | 0.0016 | −0.0025 | −0.0067 |
| Historical 95% VaR | 5.8908 | 6.5674 | 6.9211 | 6.5630 | 6.7529 |
| Normal 95% VaR | 7.0017 | 7.4156 | 7.3631 | 7.3195 | 7.5291 |
| Historical 95% CVaR | 9.2186 | 9.9688 | 10.2255 | 9.9368 | 9.9135 |
| Normal 95% CVaR | 8.8776 | 9.3651 | 9.2990 | 9.2411 | 9.5006 |
| M–Square (%) | −0.0047 | −0.0059 | −0.0059 | −0.0060 | −0.0061 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adhikari, R.; Putnam, K.J.; Panta, H. Robust Optimization-Based Commodity Portfolio Performance. Int. J. Financial Stud. 2020, 8, 54. https://doi.org/10.3390/ijfs8030054
Adhikari R, Putnam KJ, Panta H. Robust Optimization-Based Commodity Portfolio Performance. International Journal of Financial Studies. 2020; 8(3):54. https://doi.org/10.3390/ijfs8030054
Chicago/Turabian StyleAdhikari, Ramesh, Kyle J. Putnam, and Humnath Panta. 2020. "Robust Optimization-Based Commodity Portfolio Performance" International Journal of Financial Studies 8, no. 3: 54. https://doi.org/10.3390/ijfs8030054
APA StyleAdhikari, R., Putnam, K. J., & Panta, H. (2020). Robust Optimization-Based Commodity Portfolio Performance. International Journal of Financial Studies, 8(3), 54. https://doi.org/10.3390/ijfs8030054





