Multi-Factor Asset-Pricing Models under Markov Regime Switches: Evidence from the Chinese Stock Market
Abstract
:1. Introduction
2. Data and Methodology
2.1. Data
2.1.1. Benchmark Portfolios
2.1.2. Constructing Risk Factors
2.2. Methodology and Models
2.2.1. The Framework of a Markov Regime-Switching Model
2.2.2. CAPM with Markov Switching (MR–CAPM)
2.2.3. Fama–French Three-Factor Model with Markov Switching (MR-FF3 Model)
3. Empirical Results
3.1. Estimation of MR-CAPM
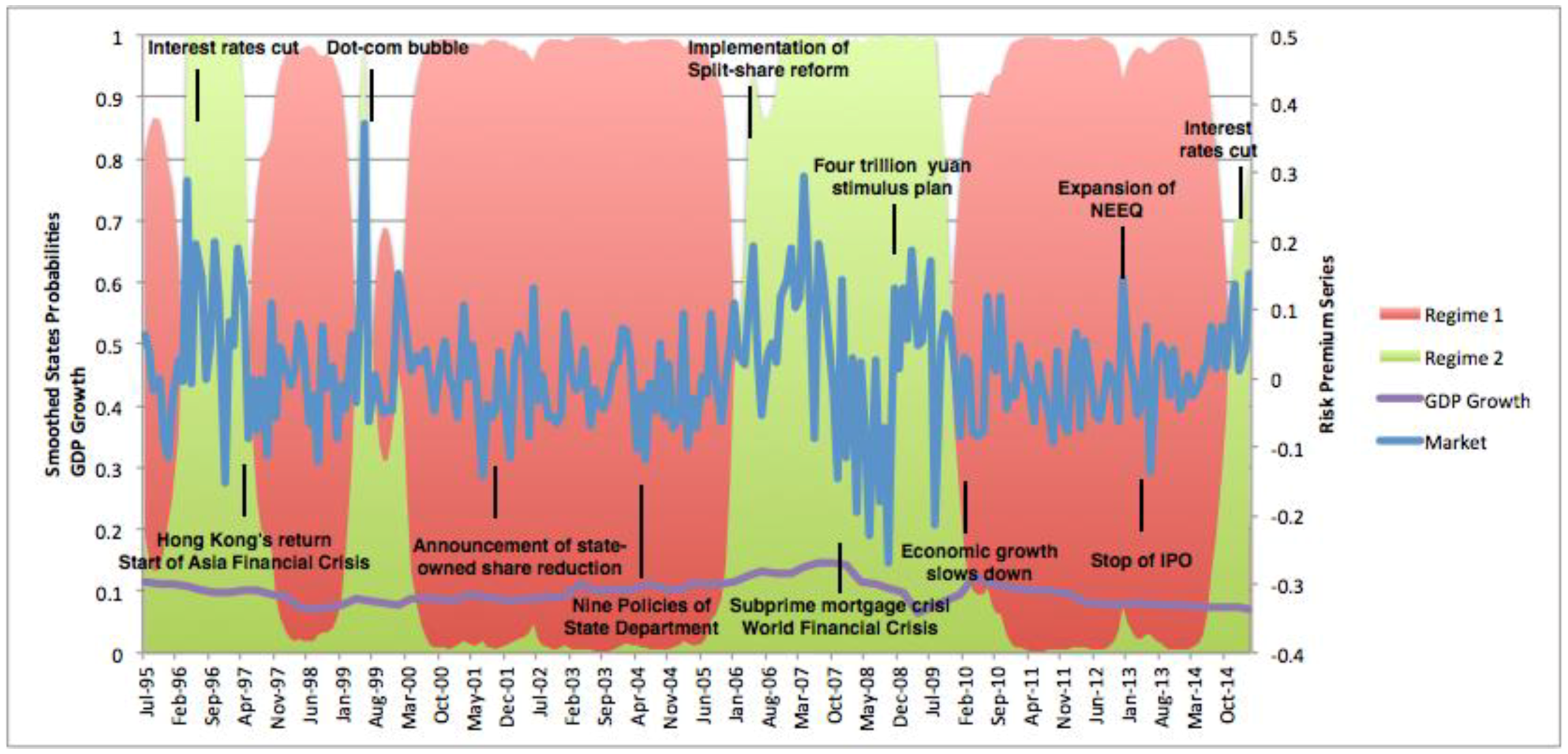
3.1.1. Risk Factor Variations
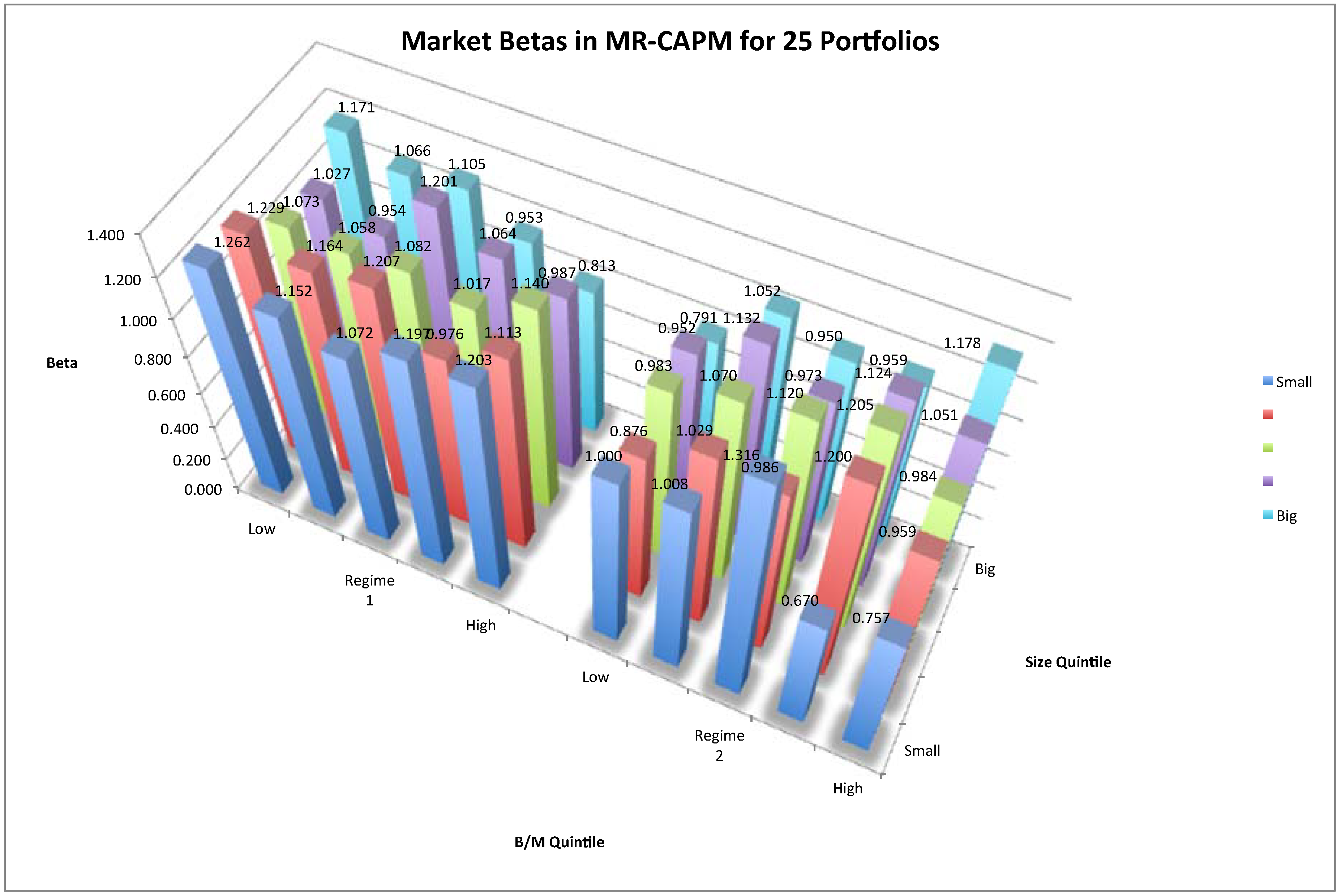
3.1.2. Risk Loading Variations
3.2. Estimation of MR-FF3 Model
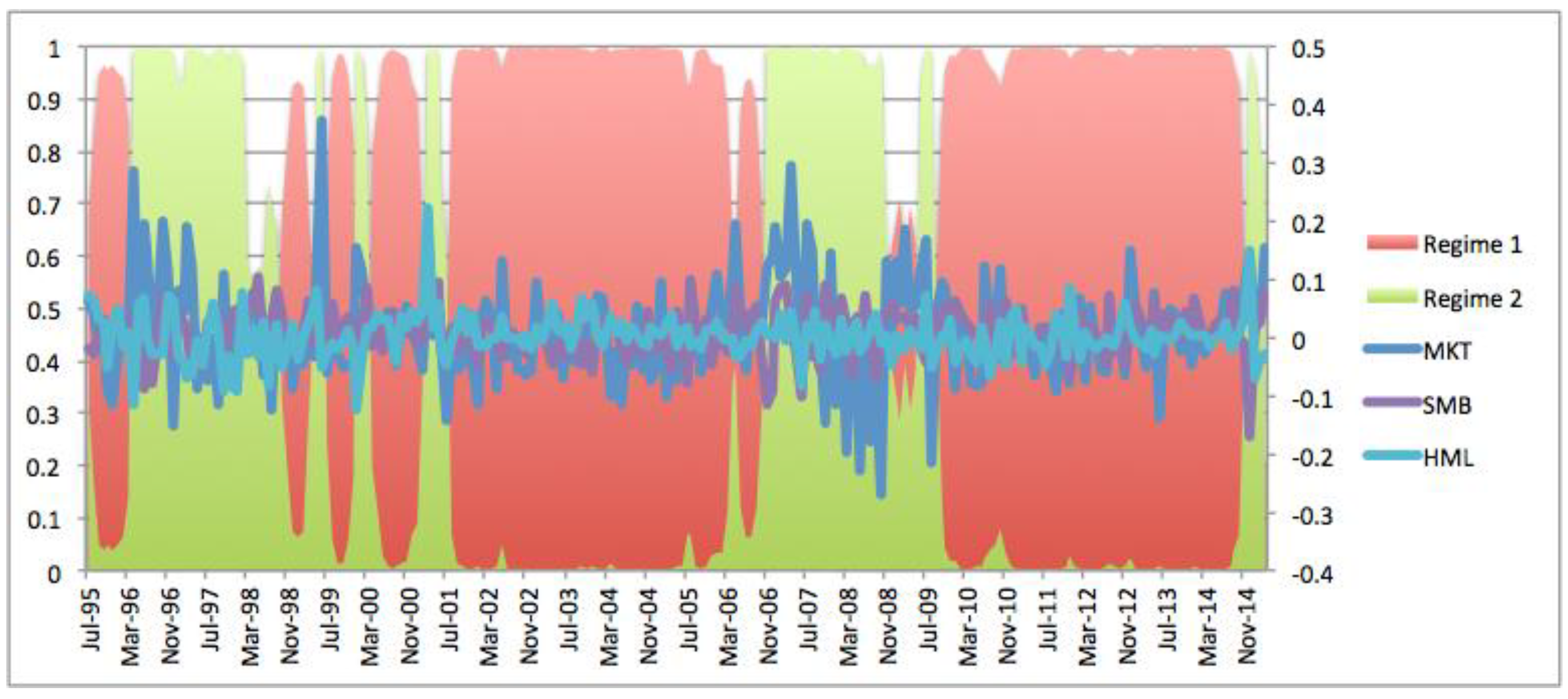
3.2.1. Risk Factor Variations
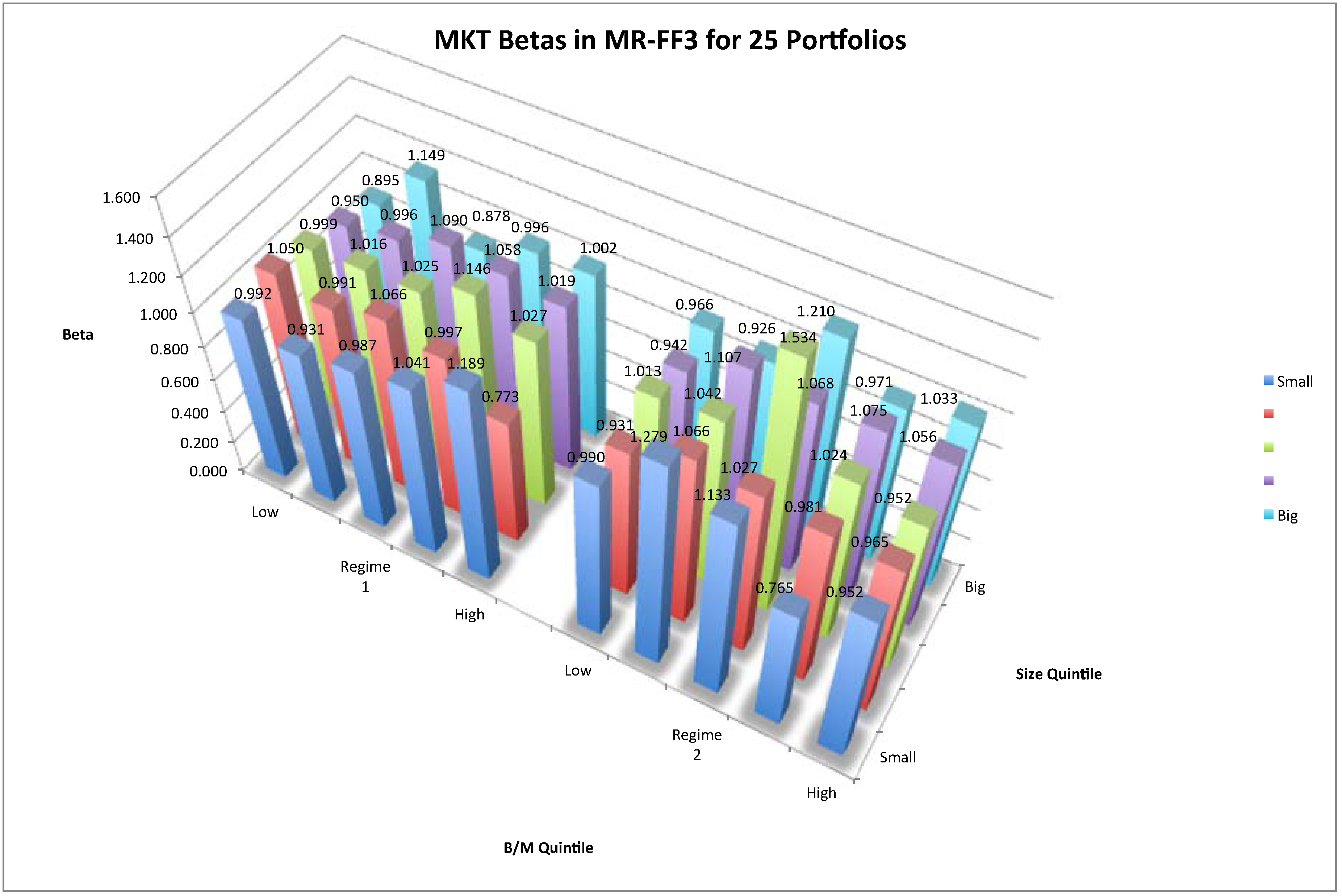
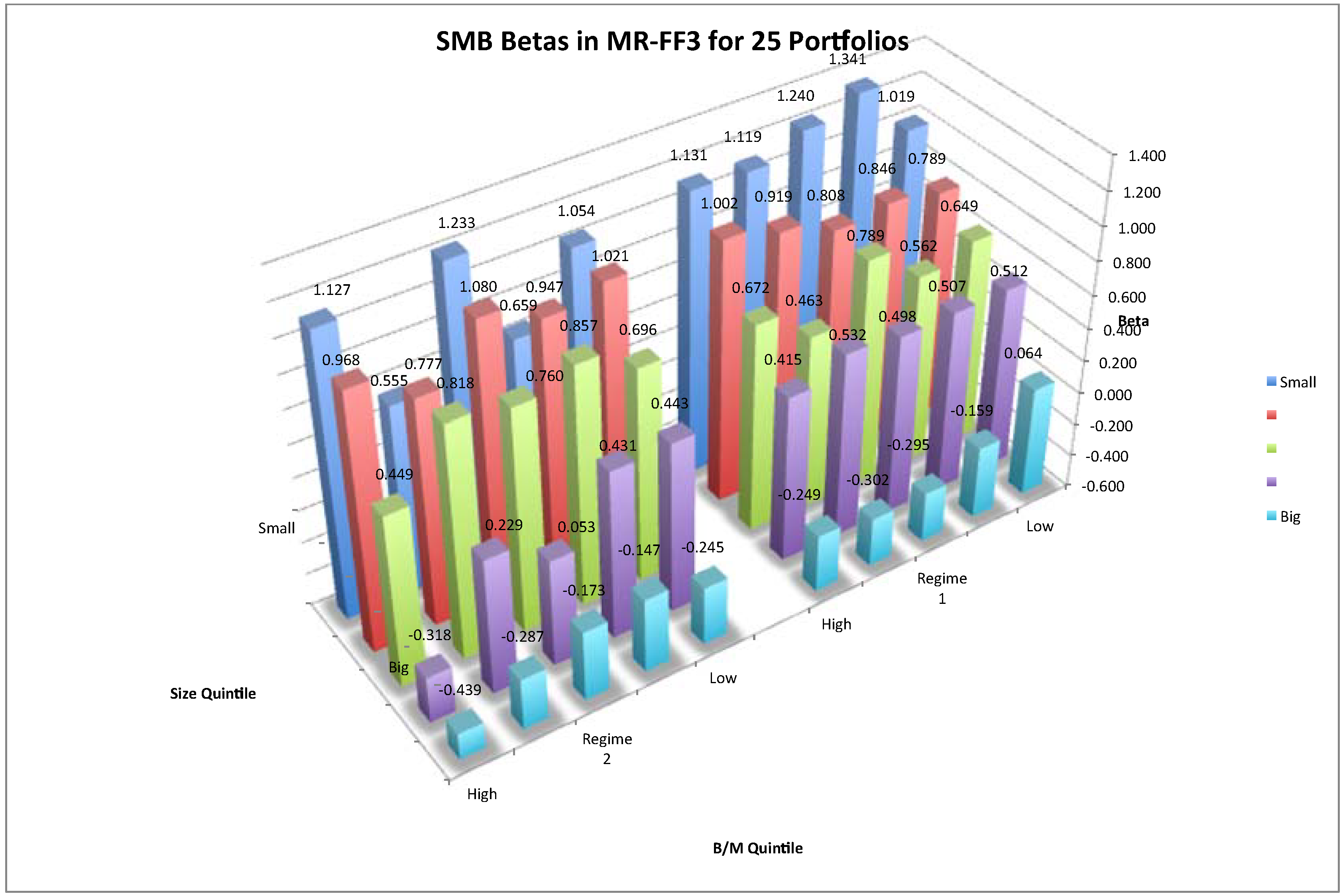
3.2.2. Risk Loading Variations
3.2.3. Robustness Test Using a Hedging Portfolio
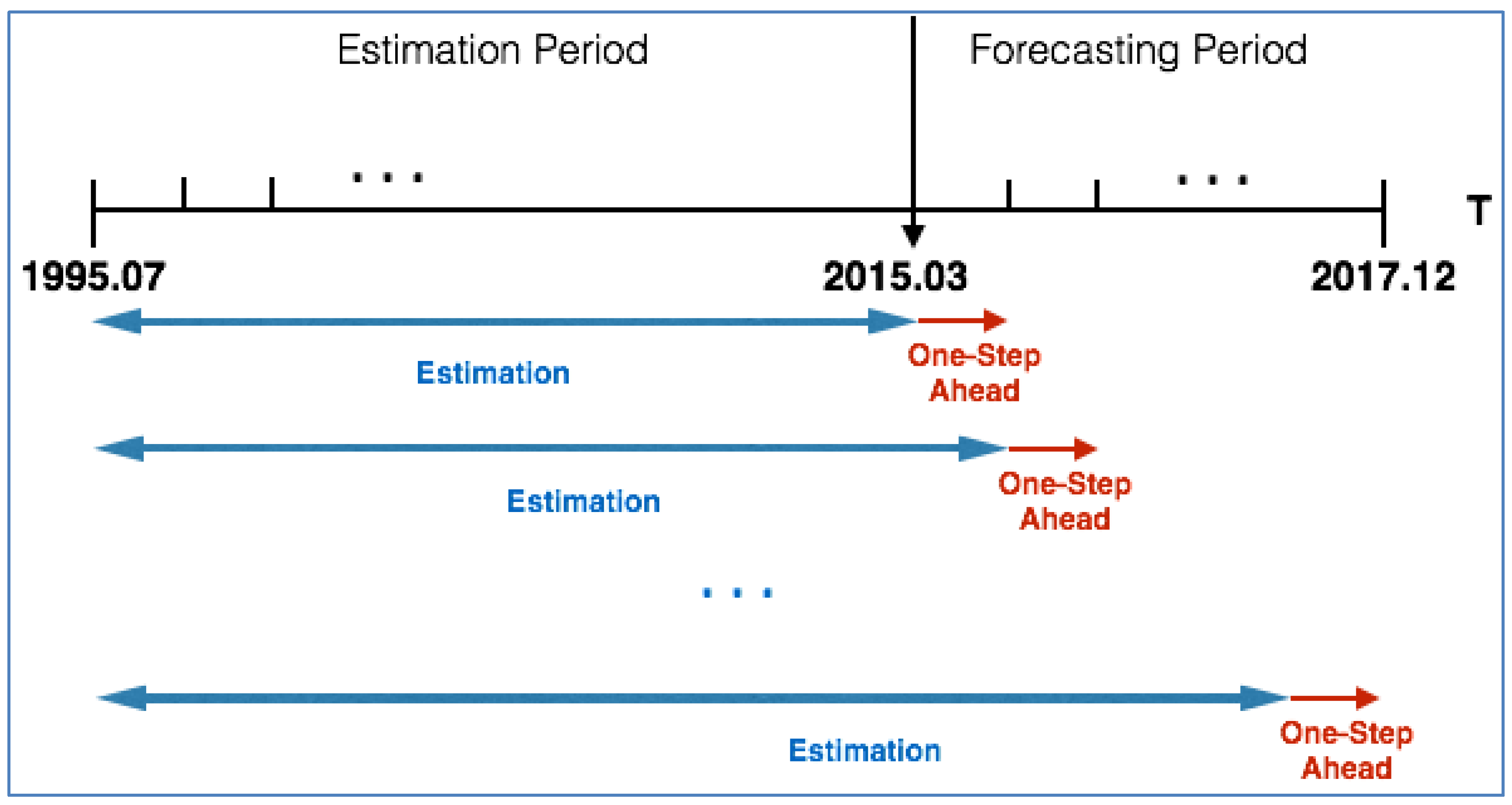
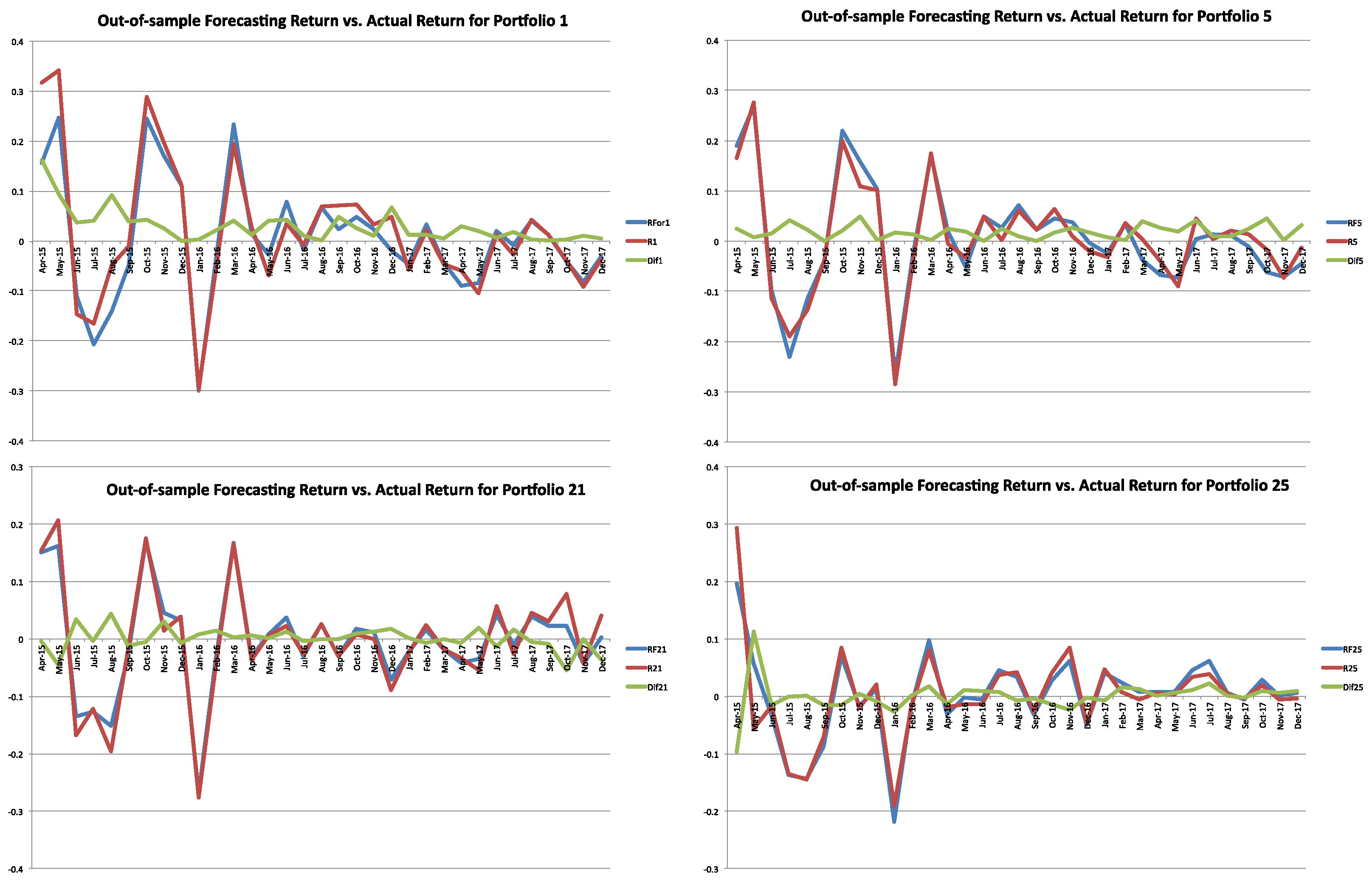
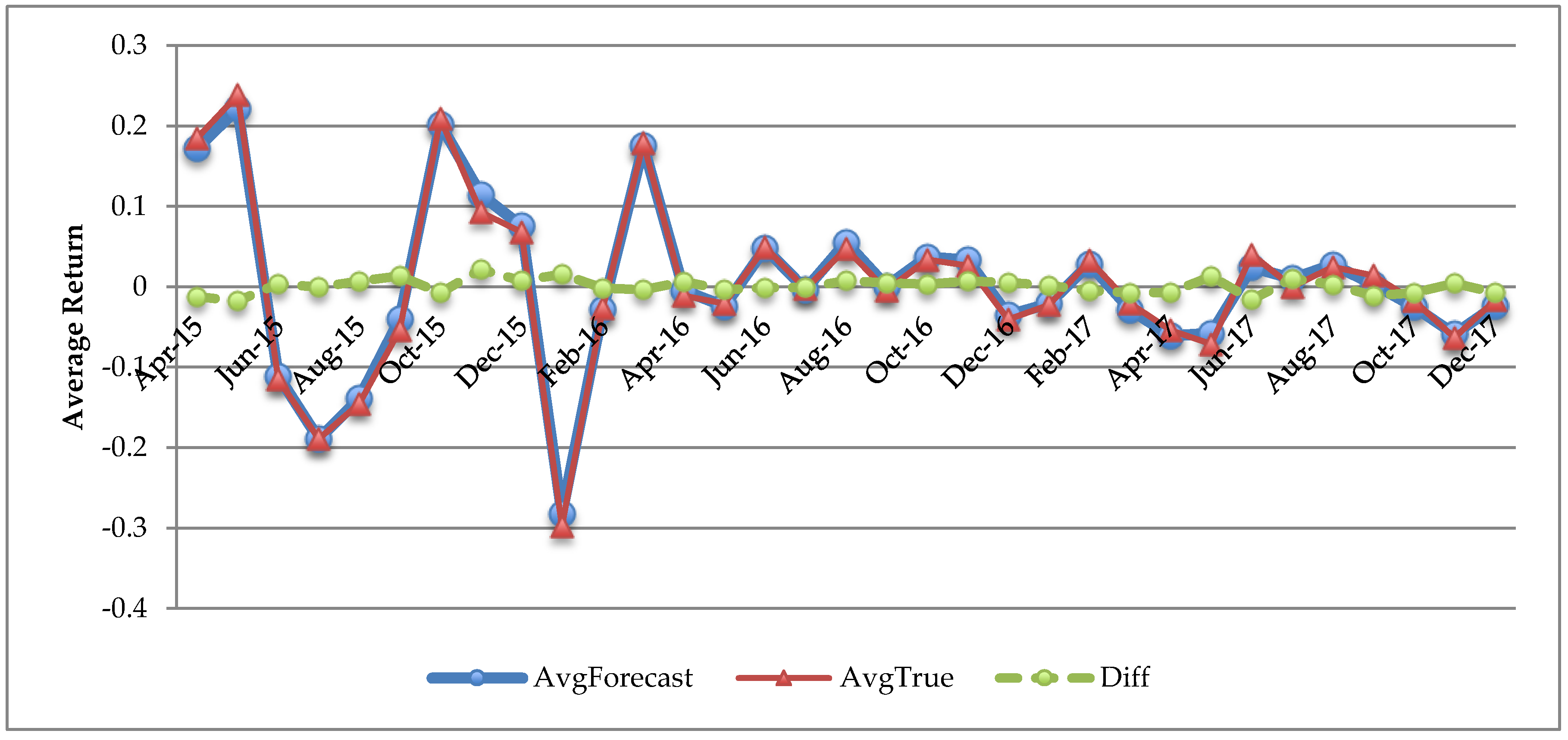
3.3. Out-of-Sample Analysis on MR-FF3 Model
4. Discussion
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Statement 1
References
- Abdymomunov, Azamat, and James Morley. 2011. Time variation of CAPM betas across market volatility regimes. Applied Financial Economics 21: 1463–78. [Google Scholar] [CrossRef]
- Ammann, Manuel, and Michael Verhofen. 2009. The effect of market regimes on style allocation. Financial Markets and Portfolio Management 20: 309–37. [Google Scholar] [CrossRef]
- Brunnermeier, Markus, Michael Sockin, and Wei Xiong. 2017. China’s model of managing the financial system. Work. Pap. Available online: https://www.princeton.edu/~wxiong/papers/ChinaTrading.pdf (accessed on 18 May 2018).
- Campbell, John Y., Andrew. W. Lo, and A. Craig MacKinlay. 1997. The Econometrics of Financial Markets. Princeton: Princeton University Press. [Google Scholar]
- Chen, Jieting. 2017. What explains the investment anomaly in the Chinese stock market? Nankai Business Review International 8: 495–520. [Google Scholar] [CrossRef]
- Cochrane, John. 2005. Asset Pricing, rev. ed. Princeton: Princeton University Press. [Google Scholar]
- Coggi, Patrick, and Bogdan Manescu. 2004. A multifactor model of stock returns with endogeneous regime switching. Discussion Paper No. 2004-01, St. Gallen University. Available online: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.197.3511&rep=rep1&type=pdf (accessed on 18 May 2018).
- Carhart, Mark M. 1997. On persistence in mutual fund performance. The Journal of Finance 52: 57–82. [Google Scholar] [CrossRef]
- Cotter, John, and Enrique Salvador. 2014. The non-linear trade-off between return and risk: A regime-switching multi-factor framework. SSRN Work. Pap. Available online: http://dx.doi.org/10.2139/ssrn.2513282 (accessed on 18 May 2018).
- Fama, Eugene, and Kenneth French. 1992. The cross-section of expected stock returns. The Journal of Finance 47: 427–65. [Google Scholar] [CrossRef]
- Fama, Eugene, and Kenneth French. 1993. Common risk factors in the returns on stocks and bonds. Journal of Financial Economics 33: 3–56. [Google Scholar] [CrossRef]
- Fama, Eugene, and Kenneth French. 1996. Multifactor explanations of asset pricing anomalies. The Journal of Finance 51: 55–84. [Google Scholar] [CrossRef]
- Fama, Eugene, and Kenneth French. 2006. Dissecting anomalies. The Journal of Finance 63: 1653–78. [Google Scholar] [CrossRef]
- Fama, Eugene, and Kenneth French. 2015. A five-factor asset pricing model. Journal of Financial Economics 116: 1–22. [Google Scholar] [CrossRef]
- Fama, Eugene, and Kenneth French. 2016. Dissecting anomalies with a five-factor model. The Review of Financial Studies 29: 69–103. [Google Scholar] [CrossRef]
- Ferson, Wayne, and Campbell Harvey. 1999. Conditioning variables and the cross-section of stock returns. Journal of Finance 54: 1325–60. [Google Scholar] [CrossRef]
- Ghysels, Eric. 1998. On stable factor structures in the pricing of risk: Do time-varying betas help or hurt? The Journal of Finance 53: 549–73. [Google Scholar] [CrossRef]
- Guidolin, Massimo, and Allan Timmermann. 2007. Asset allocation under multivariate regime switching. Journal of Economic Dynamics & Control 31: 3503–44. [Google Scholar] [CrossRef]
- Guidolin, Massimo, and Allan Timmermann. 2008. Size and value anomalies under regime shifts. Journal of Financial Econometrics 6: 1–48. [Google Scholar] [CrossRef]
- Guo, Bin, Wei Zhang, Yongjie Zhang, and Han Zhang. 2017. The five-factor asset pricing model tests for the Chinese stock market. Pacific-Basin Finance Journal 43: 84–106. [Google Scholar] [CrossRef]
- Hamilton, James D. 1989. A new approach to the economic analysis of nonstationary time series and the business cycle. Econometrica 57: 357–84. [Google Scholar] [CrossRef]
- Hamilton, James D. 1994. Time Series Analysis. Princeton: Princeton University Press. [Google Scholar]
- He, Jia, Raymond Kan, Lilian Ng, and Chu Zhang. 1996. Tests of the relations among marketwide factors, firm-specific variables, and stock returns using a conditional asset pricing model. The Journal of Finance 51: 1891–908. [Google Scholar] [CrossRef]
- Lin, Jianhao, Meijin Wang, and Linfeng Cai. 2012. Are the Fama–French factors good proxies for latent risk factors? Evidence from the data of SHSE in China. Economics Letters 116: 265–68. [Google Scholar] [CrossRef]
- Lintner, John. 1965a. The valuation of risk assets and the selection of risky investments in stock portfolios and capital budgets. The Review of Economics and Statistics 47: 13–37. [Google Scholar] [CrossRef]
- Lintner, John. 1965b. Securities prices, risk, and maximal gains from diversification. The Journal of Finance 20: 587–615. [Google Scholar] [CrossRef]
- Markowitz, Harry. 1952. Portfolio selection. The Journal of Finance 7: 77–91. Available online: http://links.jstor.org/sici?sici=0022-1082%28195203%297%3A1%3C77%3APS%3E2.0.CO%3B2-1 (accessed on 18 May 2018).
- Merton, Robert C. 1969. Lifetime portfolio selection under uncertainty: The continuous-time case. Review of Economics and Statistics 51: 247–57. [Google Scholar] [CrossRef]
- Merton, Robert C. 1971. Optimum consumption and portfolio rules in a continuous-time model. Journal of Economic Theory 3: 373–413. [Google Scholar] [CrossRef]
- Merton, Robert C. 1973. An intertemporal capital asset pricing model. Econometrica 41: 867–87. [Google Scholar] [CrossRef]
- Mossin, Jan. 1966. Equilibrium in a capital asset market. Econometrica 34: 768–83. [Google Scholar] [CrossRef]
- Mulvey, John M., and Yonggan Zhao. 2011. An Investment Model via Regime-Switching Economic Indicators. Technical Report. Research Report. Princeton: Bendheim Center for Finance and Operations Research and Financial Engineering Department, Princeton University. [Google Scholar]
- Ozoguz, Arzu. 2008. Good times or bad times? Investors’ uncertainty and stock returns. The Review of Financial Studies 22: 4377–22. [Google Scholar] [CrossRef]
- Perlin, Marcelo. 2014. MS regress—The MATLAB package for Markov regime switching models. Available online: http://ssrn.com/abstract=1714016 (accessed on 18 May 2018).
- Rossi, Alberto G. P., and Allan Timmermann. 2010. What is the shape of the risk-return relation? AFA 2010 Atlanta Meetings Paper. Available online: http://dx.doi.org/10.2139/ssrn.1364750 (accessed on 18 May 2018).
- Sharpe, William F. 1964. Capital Asset Prices: A theory of market equilibrium under conditions of risk. The Journal of Finance 19: 425–42. [Google Scholar]
- Treynor, Jack L. 1961. Market value, time, and risk. SSRN Work. Pap. Available online: http://dx.doi.org/10.2139/ssrn.2600356 (accessed on 18 May 2018).
- Treynor, Jack L. 1962. Toward a theory of market value of risky assets. SSRN Work. Pap. Available online: http://dx.doi.org/10.2139/ssrn.628187 (accessed on 18 May 2018).
| 1 | Proof of this statement is shown in Appendix A. |

| Size | Book-to-Market (B/M) | ||
|---|---|---|---|
| Growth | Medium | Value | |
| (30%) | (40%) | (30%) | |
| Small (50%) | S/L | S/M | S/H |
| Big (50%) | B/L | B/M | B/H |
| Regime | St = 1 (Bear) | St = 2 (Bull) | |
|---|---|---|---|
| Transition matrix Π | St = 1 (Bear) | 0.97 (0.00) | 0.06 (0.00) |
| St = 2 (Bull) | 0.03 (0.00) | 0.94 (0.00) | |
| Expected Duration | 34.05 | 18.01 | |
| MKT | μm | −0.005 (0.38) | 0.048 (0.00) |
| σm | 0.059 (0.00) | 0.122 (0.00) | |
| Regime | St = 1 (Bear) | St = 2 (Bull) | |
|---|---|---|---|
| Transition matrix Π | St = 1 (Bear) | 0.95 (0.00) | 0.11 (0.00) |
| St = 2 (Bull) | 0.05 (0.00) | 0.89 (0.00) | |
| Expected Duration | 18.89 | 9.36 | |
| MKT | μm | −0.001 (0.85) | 0.037 (0.02) |
| σm | 0.060 (0.00) | 0.123 (0.00) | |
| SMB | μs | 0.009 (0.01) | 0.008 (0.28) |
| σs | 0.031 (0.00) | 0.057 (0.00) | |
| HML | μh | 0.004 (0.28) | 0.003 (0.65) |
| σh | 0.027 (0.00) | 0.003 (0.00) | |
| Panel A: Estimates for the Four Models | |||||
| Model | Regime | Intercept | MKT | SMB | HML |
| Unconditional CAPM | 0.011 | 0.061 | |||
| (0.038) | (0.303) | ||||
| MR-CAPM | St = 1 | 0.010 | 0.161 | ||
| (0.006) | (0.000) | ||||
| St = 2 | 0.017 | −0.052 | |||
| (0.223) | (0.698) | ||||
| Unconditional FF3 | −0.002 | −0.004 | 1.436 | 1.437 | |
| (0.301) | (0.868) | (0.000) | (0.000) | ||
| MR-FF3 | St = 1 | −0.004 | 0.031 | 1.439 | 1.235 |
| (0.010) | (0.091) | (0.000) | (0.000) | ||
| St = 2 | 0.018 | −0.183 | 1.042 | 2.105 | |
| (0.076) | (0.067) | (0.000) | (0.000) | ||
| Panel B: Statistics for the Four Models | |||||
| Model | SSR | Log Likelihood | AIC | BIC | HQC |
| Unconditional CAPM | 1.470 | 266.017 | −2.228 | −2.199 | −2.216 |
| MR-CAPM | 1.472 | 313.237 | −2.576 | −2.459 | −2.529 |
| Unconditional FF3 | 0.222 | 489.909 | −4.100 | −4.042 | −4.077 |
| MR-FF3 | 0.179 | 544.315 | −4.492 | −4.317 | −4.421 |
| MR-FF3 | In-Sample RMSE | Out-of-Sample RMSE |
|---|---|---|
| 1995.07–2015.03 | 2015.04–2017.12 | |
| Portfolio 1 | 0.045 | 0.039 |
| Portfolio 5 | 0.034 | 0.019 |
| Portfolio 21 | 0.027 | 0.020 |
| Portfolio 25 | 0.027 | 0.020 |
| Hedging Portfolio of “5–21” | 0.027 | 0.016 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, J.; Kawaguchi, Y. Multi-Factor Asset-Pricing Models under Markov Regime Switches: Evidence from the Chinese Stock Market. Int. J. Financial Stud. 2018, 6, 54. https://doi.org/10.3390/ijfs6020054
Chen J, Kawaguchi Y. Multi-Factor Asset-Pricing Models under Markov Regime Switches: Evidence from the Chinese Stock Market. International Journal of Financial Studies. 2018; 6(2):54. https://doi.org/10.3390/ijfs6020054
Chicago/Turabian StyleChen, Jieting, and Yuichiro Kawaguchi. 2018. "Multi-Factor Asset-Pricing Models under Markov Regime Switches: Evidence from the Chinese Stock Market" International Journal of Financial Studies 6, no. 2: 54. https://doi.org/10.3390/ijfs6020054
APA StyleChen, J., & Kawaguchi, Y. (2018). Multi-Factor Asset-Pricing Models under Markov Regime Switches: Evidence from the Chinese Stock Market. International Journal of Financial Studies, 6(2), 54. https://doi.org/10.3390/ijfs6020054





