Capital Asset Pricing Model Testing at Warsaw Stock Exchange: Are Family Businesses the Remedy for Economic Recessions?

Abstract

In this article, we test the capital asset pricing model (CAPM) on the Warsaw Stock Exchange (WSE) by measuring the performance of two portfolios composed of construction firms: family-controlled and nonfamily controlled. These portfolios were selected from the WIG-Construction (WIG—Warszawski Indeks Giełdowy—Warsaw Stock Exchange Index). The performance of both portfolios was measured in the period from 2006 to 2012 with respect to three sub-periods: (1) pre-crisis period: 2006–2007; (2) crisis period: 2008–2009; and (3) post-crisis period: 2010–2012. This division was constructed in this way to find out how family firms performed in crisis times in relation to nonfamily firms. In addition, the construction portfolio was chosen due to its sensitivity to recessions. When an economy faces a downturn, construction firms are among the first to be exposed to risk. The performance was measured by using the capital asset pricing model with statistical inference. We find that public family firms significantly outperformed non-family peers in the crisis times.

1. Introduction

The most recent U.S. domestic housing subprime crisis in 2007–2008 predominantly impacted the construction sector. Its negative consequences spread out to the rest of the U.S. economy and eventually expanded worldwide (International Labour Organization [1]). According to The Organisation for Economic Co-operation and Development (OECD) data, almost all countries tracked by the OECD reported a fall in GDP of 2.1 percentage points in the first quarter of 2009 and a rise in unemployment of 2.4 percentage points in May, 2009 (OECD [2]). This was the largest decline reported by the OECD since 1960, which predominantly affected the construction and automobile sectors. In the U.S., this impact was more devastating and led to an almost tripling of the unemployment rate, which reached 20.6% in 2010 (Current Population Survey (CPS) [3]). In response, the U.S. launched the American Recovery and Reinvestment Act of 2009. This crisis also had a very deep and negative impact on EU countries and the Euro area, where the construction sector accounted for 6.0% of EU GDP in 2010. The deepest decline of −8.5% in the EU-27 and −7.9% in the Euro area hit in 2009 (see Table 1).

Table 1. Annual growth, index of production for construction, EU-27 and Euro area, 2000–2010 (¹) (% growth; comparison with the previous year).

		2000	2001	2002	2003	2004	2005	2006	2007	2008	2009	2010
EU-27	Total construction	4.0	0.5	1.1	1.8	0.8	1.9	3.6	2.0	−3.8	−8.5	−4.1
	Building	5.2	0.4	0.7	2.7	1.8	2.2	4.3	1.9	−4.3	−10.9	−3.4
	Civil engineering	0.2	1.1	1.7	−1.5	−3.2	1.9	−1.2	2.9	−1.4	2.3	−7.1
EA-17	Total construction	4.1	0.6	0.4	0.9	0.0	2.2	3.7	1.3	−5.5	−7.9	−7.7
	Building	5.3	0.4	−0.1	1.6	0.8	2.6	4.7	1.2	−6.1	−10.1	−6.7
	Civil engineering	1.4	0.8	0.5	−0.8	−2.1	2.7	−1.8	2.1	−4.2	0.6	−12.0

(¹) Estimates; working day adjusted series. Source: Eurostat.

Table 1. Annual growth, index of production for construction, EU-27 and Euro area, 2000–2010 (¹) (% growth; comparison with the previous year).

		2000	2001	2002	2003	2004	2005	2006	2007	2008	2009	2010
EU-27	Total construction	4.0	0.5	1.1	1.8	0.8	1.9	3.6	2.0	−3.8	−8.5	−4.1
	Building	5.2	0.4	0.7	2.7	1.8	2.2	4.3	1.9	−4.3	−10.9	−3.4
	Civil engineering	0.2	1.1	1.7	−1.5	−3.2	1.9	−1.2	2.9	−1.4	2.3	−7.1
EA-17	Total construction	4.1	0.6	0.4	0.9	0.0	2.2	3.7	1.3	−5.5	−7.9	−7.7
	Building	5.3	0.4	−0.1	1.6	0.8	2.6	4.7	1.2	−6.1	−10.1	−6.7
	Civil engineering	1.4	0.8	0.5	−0.8	−2.1	2.7	−1.8	2.1	−4.2	0.6	−12.0

(¹) Estimates; working day adjusted series. Source: Eurostat.

Only one EU country reported positive growth in the construction sector during the crisis period: Poland with a growth of 10.2% in 2008 and 4.5% in the following year (see Table 2).

Table 2. Annual growth, index of production for total construction, 2000–2010 (¹) (% growth; comparison with the previous year).

	Weight in 2005 (% of EU-27)	2000	2001	2002	2003	2004	2005	2006	2007	2008	2009	2010
EU-27	100.0	4.0	0.5	1.1	1.8	0.8	1.9	3.6	2.0	−3.8	−8.5	−4.1
EA-17	71.3	4.1	0.6	0.4	0.9	0.0	2.2	3.7	1.3	−5.5	−7.9	−7.7
Belgium	2.0	:	−2.4	−2.6	−0.2	2.9	0.6	3.3	1.5	−0.4	−3.3	−2.1
Bulgaria	0.1	:	13.4	3.8	4.6	35.3	31.7	24.8	26.8	12.6	−14.2	−17.9
Czech Republic	0.8	0.8	10.3	2.9	9.5	8.6	5.2	6.4	6.8	−0.3	−0.6	−7.3
Denmark	1.7	1.7	−6.7	−1.2	2.1	−0.2	3.1	3.8	−4.2	−5.7	−10.8	−8.4
Germany	11.1	−3.5	−7.6	−4.3	−4.2	−5.3	−5.3	6.3	2.9	−0.7	0.1	0.2
Estonia	0.1	18.6	4.2	22.6	6.1	12.5	22.4	26.9	13.5	−13.3	−29.8	−12.4
Ireland	2.8	:	3.4	2.0	5.7	25.3	10.0	3.8	−13.5	−29.2	−36.9	−30.1
Greece	1.1	:	6.6	39.1	−5.7	−15.9	−38.7	3.6	14.3	7.7	−17.5	−31.6
Spain	18.8	10.7	3.0	0.6	7.2	2.3	10.9	2.2	−4.3	−16.3	−11.3	−20.2
France	12.5	6.0	1.2	−2.3	−0.8	−1.2	2.7	4.2	2.3	−3.7	−5.9	−3.4
Italy	11.5	6.2	6.2	5.1	2.8	1.6	1.3	3.9	6.4	−1.1	−11.5	−3.4
Cyprus	0.3	:	3.7	3.2	6.5	4.4	2.9	4.1	6.8	2.3	−10.6	−8.0
Latvia	0.1	7.2	6.2	12.1	13.1	13.1	15.5	13.3	13.6	−3.1	−34.9	−23.4
Lithuania	0.2	−18.1	7.4	21.7	27.8	6.8	9.9	21.7	22.2	4.0	−48.5	−7.7
Luxembourg	0.3	:	4.2	1.9	0.9	−1.1	−0.9	2.6	2.6	−1.8	0.8	0.1
Hungary	0.5	7.7	9.3	18.0	2.7	4.3	15.7	−0.7	−14.0	−5.2	−4.4	−10.4
Malta	0.0	:	−4.0	23.4	−5.7	8.0	18.5	4.4	7.2	6.6	−7.9	0.2
Netherlands	4.9	:	1.9	−3.1	−4.9	−2.6	3.2	2.3	5.6	3.2	−3.0	−11.0
Austria	2.2	−0.6	−0.6	0.5	12.3	5.0	4.9	5.9	3.9	−0.9	−1.6	−4.3
Poland	1.7	1.2	−10.9	−10.1	−7.2	−1.9	9.2	15.6	16.3	10.2	4.5	3.7
Portugal	1.8	:	4.7	−1.1	−8.6	−4.4	−4.5	−6.3	−4.0	−1.2	−6.6	−8.5
Romania	0.4	:	11.5	4.5	3.2	1.4	6.6	15.6	33.1	26.7	−15.2	−13.4
Slovenia	0.2	2.9	−10.5	7.5	9.6	0.7	2.0	15.7	18.5	15.5	−20.9	−16.9
Slovakia	0.2	0.2	0.7	4.1	5.7	5.9	14.5	15.4	5.5	11.5	−11.2	−4.3
Finland	1.3	8.0	−0.1	1.4	4.2	4.4	5.2	7.8	10.2	4.1	−13.2	11.9
Sweden	2.1	4.4	5.4	0.3	0.0	0.1	3.0	8.0	6.2	4.2	−3.5	5.9
United Kingdom	21.2	4.2	1.1	4.6	5.6	3.5	−0.5	1.4	2.3	−1.3	−11.6	7.3
Norway	-	−2.1	1.2	−0.1	2.1	7.4	8.9	6.0	5.8	1.1	−8.3	−0.1
Switzerland	-	2.7	−2.7	0.9	0.1	3.1	2.6	2.0	1.2	2.4	1.4	1.9
Montenegro	-	:	5.3	0.3	−8.5	−5.8	7.6	46.1	−1.7	20.7	−19.3	−0.6
Croatia	-	−8.9	3.7	13.0	22.3	1.6	−0.2	9.3	2.6	11.8	−6.9	−15.9
FYR of Macedonia	-	:	:	:	:	:	:	−12.3	7.5	25.5	13.7	15.2
Turkey	-	:	:	:	:	:	:	18.4	5.5	−7.6	−16.3	17.5

(¹) Estimates; working day adjusted series. Source: Eurostat.

Table 2. Annual growth, index of production for total construction, 2000–2010 (¹) (% growth; comparison with the previous year).

	Weight in 2005 (% of EU-27)	2000	2001	2002	2003	2004	2005	2006	2007	2008	2009	2010
EU-27	100.0	4.0	0.5	1.1	1.8	0.8	1.9	3.6	2.0	−3.8	−8.5	−4.1
EA-17	71.3	4.1	0.6	0.4	0.9	0.0	2.2	3.7	1.3	−5.5	−7.9	−7.7
Belgium	2.0	:	−2.4	−2.6	−0.2	2.9	0.6	3.3	1.5	−0.4	−3.3	−2.1
Bulgaria	0.1	:	13.4	3.8	4.6	35.3	31.7	24.8	26.8	12.6	−14.2	−17.9
Czech Republic	0.8	0.8	10.3	2.9	9.5	8.6	5.2	6.4	6.8	−0.3	−0.6	−7.3
Denmark	1.7	1.7	−6.7	−1.2	2.1	−0.2	3.1	3.8	−4.2	−5.7	−10.8	−8.4
Germany	11.1	−3.5	−7.6	−4.3	−4.2	−5.3	−5.3	6.3	2.9	−0.7	0.1	0.2
Estonia	0.1	18.6	4.2	22.6	6.1	12.5	22.4	26.9	13.5	−13.3	−29.8	−12.4
Ireland	2.8	:	3.4	2.0	5.7	25.3	10.0	3.8	−13.5	−29.2	−36.9	−30.1
Greece	1.1	:	6.6	39.1	−5.7	−15.9	−38.7	3.6	14.3	7.7	−17.5	−31.6
Spain	18.8	10.7	3.0	0.6	7.2	2.3	10.9	2.2	−4.3	−16.3	−11.3	−20.2
France	12.5	6.0	1.2	−2.3	−0.8	−1.2	2.7	4.2	2.3	−3.7	−5.9	−3.4
Italy	11.5	6.2	6.2	5.1	2.8	1.6	1.3	3.9	6.4	−1.1	−11.5	−3.4
Cyprus	0.3	:	3.7	3.2	6.5	4.4	2.9	4.1	6.8	2.3	−10.6	−8.0
Latvia	0.1	7.2	6.2	12.1	13.1	13.1	15.5	13.3	13.6	−3.1	−34.9	−23.4
Lithuania	0.2	−18.1	7.4	21.7	27.8	6.8	9.9	21.7	22.2	4.0	−48.5	−7.7
Luxembourg	0.3	:	4.2	1.9	0.9	−1.1	−0.9	2.6	2.6	−1.8	0.8	0.1
Hungary	0.5	7.7	9.3	18.0	2.7	4.3	15.7	−0.7	−14.0	−5.2	−4.4	−10.4
Malta	0.0	:	−4.0	23.4	−5.7	8.0	18.5	4.4	7.2	6.6	−7.9	0.2
Netherlands	4.9	:	1.9	−3.1	−4.9	−2.6	3.2	2.3	5.6	3.2	−3.0	−11.0
Austria	2.2	−0.6	−0.6	0.5	12.3	5.0	4.9	5.9	3.9	−0.9	−1.6	−4.3
Poland	1.7	1.2	−10.9	−10.1	−7.2	−1.9	9.2	15.6	16.3	10.2	4.5	3.7
Portugal	1.8	:	4.7	−1.1	−8.6	−4.4	−4.5	−6.3	−4.0	−1.2	−6.6	−8.5
Romania	0.4	:	11.5	4.5	3.2	1.4	6.6	15.6	33.1	26.7	−15.2	−13.4
Slovenia	0.2	2.9	−10.5	7.5	9.6	0.7	2.0	15.7	18.5	15.5	−20.9	−16.9
Slovakia	0.2	0.2	0.7	4.1	5.7	5.9	14.5	15.4	5.5	11.5	−11.2	−4.3
Finland	1.3	8.0	−0.1	1.4	4.2	4.4	5.2	7.8	10.2	4.1	−13.2	11.9
Sweden	2.1	4.4	5.4	0.3	0.0	0.1	3.0	8.0	6.2	4.2	−3.5	5.9
United Kingdom	21.2	4.2	1.1	4.6	5.6	3.5	−0.5	1.4	2.3	−1.3	−11.6	7.3
Norway	-	−2.1	1.2	−0.1	2.1	7.4	8.9	6.0	5.8	1.1	−8.3	−0.1
Switzerland	-	2.7	−2.7	0.9	0.1	3.1	2.6	2.0	1.2	2.4	1.4	1.9
Montenegro	-	:	5.3	0.3	−8.5	−5.8	7.6	46.1	−1.7	20.7	−19.3	−0.6
Croatia	-	−8.9	3.7	13.0	22.3	1.6	−0.2	9.3	2.6	11.8	−6.9	−15.9
FYR of Macedonia	-	:	:	:	:	:	:	−12.3	7.5	25.5	13.7	15.2
Turkey	-	:	:	:	:	:	:	18.4	5.5	−7.6	−16.3	17.5

(¹) Estimates; working day adjusted series. Source: Eurostat.

Due to its market vulnerability, the construction sector may be used as a litmus test for company performance and in a particular crises resistance.

It seems interesting to measure the behavior of family firms exposed to crises. Some researchers argue that during periods of economic meltdown, family businesses exposed to increased risk situations may perform differently than non-family business by prioritizing the security of family over firm interests (Lee [4]; Van Gils et al. [5]; Rosenblatt [6]). In opposition to this opinion, some researchers have found that family firms may withstand crisis exposure, because of having strong family and stakeholder relations (Sirmon et al. [7]; Corbetta and Salvato [8]; Anderson and Reeb [9]). These contradictory views call for further research of the behavior of family firms in crises.

The objective of this paper is to measure the performance of construction companies listed on the Warsaw Stock Exchange with respect to two portfolios: family- and non-family controlled. The performance of these portfolios was measures in three sub-periods: (1) pre-crisis period: 2006–2007; (2) crisis period: 2008–2009; (3) post-crisis period: 2010–2012. The capital asset pricing model was used to conclude whether family-controlled firms underperformed or outperformed their non-family peers in terms of expected returns and risk.

Since the seminal findings of Sharpe [10], Lintner [11] and Mossin [12], CAPM has gained the immense interest of researchers. Some questioned, while others supported, the positive and linear relation between the risk and return of securities that CAPM predicted. In addition, over the past few decades, researchers have tried to relax CAPM assumptions and apply this model in a wide array of contexts. The cross-sectional behavior of asset returns has been researched voluminously in U.S., though with limited interest in Poland (Lyn and Zychowicz [13]; Kompa and Matuszewska [14]; Witkowska [15]; Gębka [16]; Kowalewski et al. [17]; or Waszczuk [18]).

Risk and return are two basic and important financial indicators for investors. In general, investors expect more returns for taking more risk. This linear relationship is reflected in the CAPM model and is still perceived as being powerful. Its components as beta or as systematic risk allow the prediction of rates on returns of securities portfolios.

This paper is structured as follows: The next section provides the theoretical background of CAPM with an outline of its evolution and supportive and contradictory views. In the methodological part, we present portfolio construction, address the public family firms and test data retrieved from the Warsaw Stock Exchange. The final section discusses the results and limitations.

2. Theoretical Background

The capital asset pricing model was developed almost simultaneously by Sharpe [10], Lintner [11] and Mossin [12] based on Harry Markovitz’s modern portfolio theory [19]. This model assumes the mean variance as the investment allocation predictor to one-period utility maximizing investors. Five decades later, this model and its variants are still widely used in finance to explain and predict the performance of asset portfolios, the cost of capital and the relation between asset risk and expected return, among others things. In particular, this theory assumes that investors should be rewarded by higher asset returns for taking higher risks. In essence, CAPM assumes two types of risk: systematic (non-diversifiable), which is related to the market, and specific and diversifiable (or unsystematic), which are related to individual securities that may be diversified. Unsystematic risk may be minimalized by effectively diversifying and increasing the portfolio size. On the other hand, systematic risk relates to the overall movement of the market or economy and cannot be diversified. As a consequence, CAPM helps investors in measuring a security risk with respect to the market. Testing CAPM over the long term, researchers have replicated this model in many contexts and countries and are still divided regarding its viability. Some find supportive and some contradictory evidence against CAPM.

Jensen et al. [20] proved the validity of CAPM on the New York Stock Exchange by testing linearity in a cross-section of expected monthly excess returns and betas on ten portfolios between 1931 and 1965. Fama and McBeth [21] found a positive linear relationship between average returns and the beta of assets on the NYSE between 1926 and 1968. Isakov [22], in his examination of the Swiss stock market between 1983 and 1991, proved the viability of CAPM. Zhang and Wihlborg [23] tested a monthly time series of equity prices by analyzing 753 firms quoted in six European emerging stock markets: Cyprus, Czech Republic, Greece, Hungary, Russia and Turkey between 1995 and 2002. They found a positive correlation between beta and returns for domestic CAPM. Litzenberger and Ramaswamy [24] found a positive relationship between dividend yield and stock return for the 1936–1977 period. Durack et al. [25] found supportive evidence for the CAPM model by testing Australian companies. Köseoğlu and Mercangöz [26] proved the validity of the zero beta capital asset pricing model by analyzing stocks within the ISE 100 on the Istanbul Stock Exchange.

However there is another stream of research against CAPM. Ibbotson and Sinquefield [27] revised the CAPM formula by ruling out beta and arguing that returns are better explained by the size of company and its book to market ratio. Roll [28] proved CAPM misspecification as the result of not finding an adequate measure of market portfolio. Banz [29] challenged CAPM by analyzing NYSE stocks between 1936 and 1975 and found that small firms had higher risk adjusted returns than larger firms. He argued that the size effect had existed for at least forty decades, which stood against the CAPM model. However, he argued that this effect was not linear and not stable; thus, the conclusions may not be supportive. Fama and French [30] analyzed a hundred portfolios on NYSE, AMEX and NASDAQ between July 1963, and December 1990. Their findings proved that size mattered, but not the relationship between beta and returns. Michailidis et al. [31] tested weekly stock returns of 100 firms listed in Greece between January 1998, and December 2002, and arrived at conclusions that higher risk is correlated with higher return, but the reverse correlation does not hold. Adedokun and Olakojo [32] arrived at the same results by testing monthly stock returns from a hundred Nigerian firms between January 2008, and December,2009. Hasan et al. [33] tested CAPM for the Bangladesh stock market and found out that this model does not prove its validity.

Mixed results of testing CAPM encouraged researchers to adjust this model by other variables than just using beta to explain returns. Merton [34] introduced intertemporal beta to differentiate investor and market beta. In the first case, investors bear greater risk (cash flow) than the market overall (discount beta equal to the variance of the market return), which should be reflected in higher returns. Breeden [35] proposed adjusting Merton’s beta by the consumption aggregate in a single equation. Basu [36] argued that the relationship between price-earnings ratios is stronger than CAPM predicts. Bhandari [37] found a positive relationship between debt to equity and expected stock returns. This relation holds true, even with different controlling variables, such as firm size, beta and the January effect. In addition, the relationship holds, even without the January effect, though it is weaker. Some researchers found that leverage and the book-to-market ratio better explain higher asset returns than CAPM (Rosenberg et al., [38]; Chan et al. [39]). In addition, Chan et al. [39] measured the relation between stock returns and four variables: earnings yield, size, book to market ratio and cash flow yield in Japan. They found that all four of these variables correlate with market return, out of which, the book to market ratio and cash flow yield correlate the most with returns. In line with these vast contributions, Fama and French [40] revised their model by analyzing the two variables of size and book-to-market ratio in addition to excess market return. Javid and Ahmad [41] argued that conditional co-skewness explains non-normality in stock prices. Akbari and Mohammadi [42] found no relation between beta and the leverage ratios of 115 firms quoted at the Tehran Stock Market between 2005 and 2012.

There is very limited interest in research testing CAPM on family firms. Zellweger [43] pointed out the revision of CAPM to address the time horizon inherent in family firms. Zellweger argues that by extending the time horizon for investments, the marginal risk of an investment is reduced.

Some research studies have focused on testing CAPM on the Warsaw Stock Exchange. Lyn and Zychowicz [13] found book to market and dividend yield ratios in some Eastern European markets to be predictors of returns over six to 12 months. Kompa and Matuszewska [14] researched returns of five companies with the highest percentage shares listed under the Warsaw Stock Exchange Index 20 that characterize the largest and the most liquid companies. Witkowska [15] analyzed five portfolios composed of securities between 2002 and 2005 and found proof for the viability of the CAPM on the WSE. Gębka [16] found, for example, cross-autocorrelation between size and volume in portfolio returns during the 1996–2000 period. Waszczuk [18] showed that momentum trading may not be taken into account to generate profits beyond a one-month holding period between 2002 and 2011. In addition, she found proof for applying size and value strategies; however, their relevance varies over time. Almost none of the analyses of quoted Polish securities focused on measuring the performance of family firms. The only study that addressed family firms was performed by Kowalewski et al. [17]. They analyzed public family firms and found a relationship between the share of ownership and firm performance by analyzing 217 Polish companies from 1997 to 2005.

This study aims at contributing to the field of CAPM testing by analyzing securities of family-controlled vs. non-family peers, in particular measuring their performance in the crisis period.

3. Methodology

3.1. Definition

Chua et al. [44] analyzed the literature on the definition of family businesses, where they referred to Alcorn’s definition of a public family firm as follows: “if part of the stock is publicly owned, the family must also operate the business”. This rests in line with the principles of the Wallenbergs, the owners of the group of family businesses termed as the Wallenberg sphere. The Wallenbergs use the term “active ownership” to express family engagement in the businesses (Lindgren et al. [45]). This does not rely on quantification of ownership shares, but rather, controlling firms both in formal and informal ways. The minimum degree of active ownership means taking an active part in management tasks in the sense of influencing managerial and supervisory functions. Jaskiewicz et al. [46] define family businesses by numbers, i.e., having “family ownership of more than 25%, as well as family control and management participation”. Blondel et al. [47] analyzed family firms quoted on the Partis Stock Exchange defined as: “a business where one or several individuals or families are identified as the major ultimate owner or owners”. Leach and Bogod [48] classified a firm “as family controlled when at least 25% of shares are in the hands of family shareholders (provided the other 75% shareholding is distributed across smaller minority shareholders) and the business has experienced generational change”. Villalonga and Amit [49] examine their research findings and assume a family firm with “a minimum control threshold of 20% of the votes, being the largest shareholder or voteholder, having family officers or directors, or being in second or later generation”. Sraer and Thesmar [50] followed the definition of Villalonga and Amit [49] to measure the performance of public family firms in France. Miller et al. [51] stress the importance of family involvement in the ownership from the time perspective. They define “a family firm as one in which multiple members of the same family are involved as major owners or managers, either contemporaneously or over time”. We follow the definition of Villalonga and Amit [49].

3.2. Data Description

We analyzed monthly weighted portfolio returns consisting of 24 public construction firms that belong to the Warsaw Stock Exchange Construction Index (WIG-Construction) (see Table 3 and Table 4).

Table 3. WIG-Construction portfolio in PLN (family-controlled firms in italics).

Company	Market Cap		Family
	Market Cap	%	Shares %	Votes %
Budimex	1,303,762,250	36.62%	-	-
Elektrobudowa	498,435,000	14.00%	-	-
Trakcja PRKII	322,712,640	9.06%	-	-
Mostostal Zabrze	190,973,650	5.36%	-	-
Erbud	163,726,800	4.60%	-	-
Polimex-Mostostal	134,416,620	3.78%	-	-
Ulma-Construccion Polska	121,458,400	3.41%	-	-
Instal Kraków	117,900,450	3.31%	-	-
Unibep	101,347,200	2.85%	26.98% *17.83%	26.98% 17.83%
P.A. Nova	94,367,000	2.65%	18.19% 9.13%	20.80% 10.88%
Prochem	66,588,900	1.87%	-	-
Mirbud	63,918,020	1.80%	43.19%	43.19%;
Herkules	62,118,000	1.74%	18.99%	18.99%; 93.31% **
Elektrotim	61,625,740	1.73%	-	-
ZUE	58,560,000	1.64%	72.75% 0.01%	72.75% 0.01%
Projprzem	50,534,820	1.42%	-	-
Mostostal Warszawa	39,325,140	1.10%	-	-
Centrum Nowoczesnych Technologii	34,203,650	0.96%	-	-
Tesgas	20,029,750	0.56%	40.58%	55.66%
Mostostal Płock	14,261,400	0.40%	-	-
Energoaparatura	13,069,400	0.37%	-	-
Bipromet	12,009,900	0.34%	-	-
Mostostal Export	7,941,450	0.22%	-	-
Awbud	7,413,450	0.21%	-	-
Non-family cap	3,160,359,660	88.76%	-	-
Family-controlled cap	400,339,970	11.24%	-	-
TOTAL CAP	3,560,699,630	100.00%	-	-

* Two owning families; ** % in the Extraordinary General Meeting. Source: Warsaw Stock Exchange [52].

Table 3. WIG-Construction portfolio in PLN (family-controlled firms in italics).

Company	Market Cap		Family
	Market Cap	%	Shares %	Votes %
Budimex	1,303,762,250	36.62%	-	-
Elektrobudowa	498,435,000	14.00%	-	-
Trakcja PRKII	322,712,640	9.06%	-	-
Mostostal Zabrze	190,973,650	5.36%	-	-
Erbud	163,726,800	4.60%	-	-
Polimex-Mostostal	134,416,620	3.78%	-	-
Ulma-Construccion Polska	121,458,400	3.41%	-	-
Instal Kraków	117,900,450	3.31%	-	-
Unibep	101,347,200	2.85%	26.98% *17.83%	26.98% 17.83%
P.A. Nova	94,367,000	2.65%	18.19% 9.13%	20.80% 10.88%
Prochem	66,588,900	1.87%	-	-
Mirbud	63,918,020	1.80%	43.19%	43.19%;
Herkules	62,118,000	1.74%	18.99%	18.99%; 93.31% **
Elektrotim	61,625,740	1.73%	-	-
ZUE	58,560,000	1.64%	72.75% 0.01%	72.75% 0.01%
Projprzem	50,534,820	1.42%	-	-
Mostostal Warszawa	39,325,140	1.10%	-	-
Centrum Nowoczesnych Technologii	34,203,650	0.96%	-	-
Tesgas	20,029,750	0.56%	40.58%	55.66%
Mostostal Płock	14,261,400	0.40%	-	-
Energoaparatura	13,069,400	0.37%	-	-
Bipromet	12,009,900	0.34%	-	-
Mostostal Export	7,941,450	0.22%	-	-
Awbud	7,413,450	0.21%	-	-
Non-family cap	3,160,359,660	88.76%	-	-
Family-controlled cap	400,339,970	11.24%	-	-
TOTAL CAP	3,560,699,630	100.00%	-	-

* Two owning families; ** % in the Extraordinary General Meeting. Source: Warsaw Stock Exchange [52].

Table 4. WIG-Construction companies by expected returns and betas (not adjusted for size).

Company	2006–2007		2008–2009		2010–2011
	Expected Return	Beta	Expected Return	Beta	Expected Return	Beta
Budimex	0.04	1.12	0.00	0.48	0.01	1.34
Elektrobudowa	0.08	1.23	−0.01	0.38	−0.02	0.88
Trakcja PRKII	-	-	−0.01	0.59	−0.06	2.12
Mostostal Zabrze	0.11	2.11	−0.01	1.31	−0.04	2.16
Erbud	0.03	0.51	0.00	1.16	−0.04	0.82
Polimex-Mostostal	0.02	1.45	−0.03	1.17	−0.03	1.50
Ulma-Construccion Polska	0.11	0.70	−0.02	1.40	−0.01	0.31
Instal Kraków	0.07	0.98	0.00	1.00	−0.01	0.85
Unibep	-	-	0.30	1.19	0.01	0.48
P.A. Nova	0.01	0.31	0.28	0.71	0.01	1.04
Prochem	0.02	1.17	−0.01	1.36	−0.01	1.64
Mirbud	-	-	0.19	0.61	−0.01	0.59
Herkules	0.09	3.04	0.18	1.40	−0.06	1.40
Elektrotim	−0.07	1.20	0.00	0.38	−0.02	1.39
ZUE	-	-	-	-	−0.07	−0.05
Projprzem	0.05	2.09	−0.03	0.69	−0.02	1.18
Mostostal Warszawa	0.09	0.73	0.01	0.52	−0.04	1.31
Centrum Nowoczesnych Technologii	0.18	3.30	0.00	1.40	0.02	0.18
Tesgas	-	-	0.06	0.02	−0.03	0.78
Mostostal Płock	0.08	1.69	−0.01	0.99	−0.04	1.81
Energoaparatura	0.05	1.90	−0.01	0.65	−0.03	1.21
Bipromet	-	-	−0.02	0.39	0.01	−0.09
Mostostal Export	0.08	3.59	−0.02	0.53	−0.01	1.36
Awbud	0.10	2.76	−0.04	0.58	0.01	0.74

Source: own computations based on the Warsaw Stock Exchange data [52].

Table 4. WIG-Construction companies by expected returns and betas (not adjusted for size).

Company	2006–2007		2008–2009		2010–2011
	Expected Return	Beta	Expected Return	Beta	Expected Return	Beta
Budimex	0.04	1.12	0.00	0.48	0.01	1.34
Elektrobudowa	0.08	1.23	−0.01	0.38	−0.02	0.88
Trakcja PRKII	-	-	−0.01	0.59	−0.06	2.12
Mostostal Zabrze	0.11	2.11	−0.01	1.31	−0.04	2.16
Erbud	0.03	0.51	0.00	1.16	−0.04	0.82
Polimex-Mostostal	0.02	1.45	−0.03	1.17	−0.03	1.50
Ulma-Construccion Polska	0.11	0.70	−0.02	1.40	−0.01	0.31
Instal Kraków	0.07	0.98	0.00	1.00	−0.01	0.85
Unibep	-	-	0.30	1.19	0.01	0.48
P.A. Nova	0.01	0.31	0.28	0.71	0.01	1.04
Prochem	0.02	1.17	−0.01	1.36	−0.01	1.64
Mirbud	-	-	0.19	0.61	−0.01	0.59
Herkules	0.09	3.04	0.18	1.40	−0.06	1.40
Elektrotim	−0.07	1.20	0.00	0.38	−0.02	1.39
ZUE	-	-	-	-	−0.07	−0.05
Projprzem	0.05	2.09	−0.03	0.69	−0.02	1.18
Mostostal Warszawa	0.09	0.73	0.01	0.52	−0.04	1.31
Centrum Nowoczesnych Technologii	0.18	3.30	0.00	1.40	0.02	0.18
Tesgas	-	-	0.06	0.02	−0.03	0.78
Mostostal Płock	0.08	1.69	−0.01	0.99	−0.04	1.81
Energoaparatura	0.05	1.90	−0.01	0.65	−0.03	1.21
Bipromet	-	-	−0.02	0.39	0.01	−0.09
Mostostal Export	0.08	3.59	−0.02	0.53	−0.01	1.36
Awbud	0.10	2.76	−0.04	0.58	0.01	0.74

Source: own computations based on the Warsaw Stock Exchange data [52].

Next, these firms were separated to form two sub-portfolios: family-controlled firms (6 firms) and nonfamily-controlled peers (18 firms). The firm size was used as weight in the portfolio formation. We calculated beta estimates and portfolio expected returns followed by Blume [53], Friend and Blume [54] and Black et al. [55]:

(1)

where x_iconstr denotes weights i = 1,2,…, N for assets in the construction portfolio (constr), E(R_constr) expected return on the construction portfolio and E(R_i) expected return on security i.

The financial data for both of these types of portfolios were analyzed from 2006 to 2012 and divided into three sub-periods: (1) before the crisis: 2006–2007; (2) in crisis: 2008–2009; and (3) after the crisis 2010–2012 (see Table 5).

Table 5. Portfolio population.

	Before The Crisis	In Crisis	After The Crisis
Portfolio formation period	2006–2007	2008–2009	2010–2012
No. of family firms	2	5	6
No. of nonfamily firms	16	18	18

Table 5. Portfolio population.

	Before The Crisis	In Crisis	After The Crisis
Portfolio formation period	2006–2007	2008–2009	2010–2012
No. of family firms	2	5	6
No. of nonfamily firms	16	18	18

3.3. Methodology and Results

The CAPM model assumes a linear dependency between expected return on any asset with the expected return on the market portfolio. Jagannathan and McGratten [56] reflected that CAPM explains differences in the risk premium across assets, which helps investors make decisions. The risk is equivalent with beta. The slope of the CAPM line expresses the market risk premium, while the intercept represents the risk-free rate. Black et al. [55] and Fama and MacBeth [21] proposed a two-pass methodology to test the viability of CAPM. The first pass estimates portfolio betas, while the second pass regresses average returns based on the first-pass estimated portfolio betas. Sharpe [10], Lintner [11] and Mossin [12] provided the formula that explains the excess return of assets by risk premium:

E(R_i) = R_f + (E(R_m) − R_f)

(2)

where E(R_i) is the expected return on security i, R_f the return of the risk-free security, β_i the systematic risk of the security i calculated as Cov(R_i,R_m)/Var(R_m) and E(R_m) the expected return on the market portfolio.

Jensen [20] concludes that the correlation between beta and expected returns requires a time-series regression formulated as follows:

R_i − R_f = α_i + β_i(R_m – R_f) + ε_i

(3)

Jensen’s alpha defines the pricing error (abnormal performance). When alpha increases, the reliability of return estimates may deteriorate. Jensen’s alpha shall equal zero to hold the CAPM model. We followed Formulas (4) and (5) to measure the performance of construction portfolios on the Warsaw Stock Exchange. We calculated the total risk as the variance of returns:

(4)

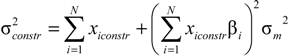and unsystematic risk as the transformation of the Equation (4):

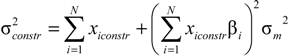
σ_εi² = σ_constr² − β_i² σ_m²

(5)

We used the Warsaw Stock Exchange Index as the proxy for the construction market portfolio and the reference rates (minimum money market intervention rate) published by the National Bank of Poland as the proxy for the risk-free rate. Then, we tested stock returns for the normal distribution by using the Jarque–Bera test. The Jarque–Bera test relies on the third and fourth momentum and follows χ2 distribution with two degrees of freedom:

(6)

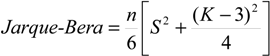where S is the skewness, K the kurtosis and n the number of observations. The null hypothesis of returns following the normal distribution was tested at a p-value of 95%. The results are presented in Table 6.

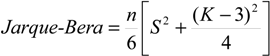
Table 6. Tests of two construction portfolios on the Warsaw Stock Exchange (WSE): non-family controlled firms (NFF) and family-controlled firms (FF).

	2006–2007		2008–2009		2010–2012
	NFF	FF	NFF	FF	NFF	FF
Expected Return	5.35%	4.13%	−0.76%	23.56%	−1.56%	−1.72%
Variance	0.093	0.071	0.022	0.031	0.011	0.010
Standard Deviation	0.306	0.267	0.150	0.175	0.107	0.103
Coefficient of variation	5.719	6.467	−19.816	0.742	−6.816	−5.963
Beta	1.223	1.392	0.680	0.921	1.305	0.711
Systematic Risk	0.005	0.006	0.004	0.007	0.004	0.001
Specific Risk	0.089	0.065	0.018	0.023	0.008	0.009
Specific Risk/Variance	0.948	0.912	0.819	0.757	0.661	0.892
Jensen’s alpha	0.032	0.018	−0.008	0.237	−0.018	−0.020
Skewness	6.142	1.231	0.438	−0.623	0.175	0.212
Kurtosis	72.109	0.471	2.210	5.022	2.206	1.722
Jarque–Bera	3284.634	1.039	1.043	1.175	0.564	0.453

Table 6. Tests of two construction portfolios on the Warsaw Stock Exchange (WSE): non-family controlled firms (NFF) and family-controlled firms (FF).

	2006–2007		2008–2009		2010–2012
	NFF	FF	NFF	FF	NFF	FF
Expected Return	5.35%	4.13%	−0.76%	23.56%	−1.56%	−1.72%
Variance	0.093	0.071	0.022	0.031	0.011	0.010
Standard Deviation	0.306	0.267	0.150	0.175	0.107	0.103
Coefficient of variation	5.719	6.467	−19.816	0.742	−6.816	−5.963
Beta	1.223	1.392	0.680	0.921	1.305	0.711
Systematic Risk	0.005	0.006	0.004	0.007	0.004	0.001
Specific Risk	0.089	0.065	0.018	0.023	0.008	0.009
Specific Risk/Variance	0.948	0.912	0.819	0.757	0.661	0.892
Jensen’s alpha	0.032	0.018	−0.008	0.237	−0.018	−0.020
Skewness	6.142	1.231	0.438	−0.623	0.175	0.212
Kurtosis	72.109	0.471	2.210	5.022	2.206	1.722
Jarque–Bera	3284.634	1.039	1.043	1.175	0.564	0.453

The Jarque–Bera test proved that the normal distribution has to be rejected for the period from 2006 to 2007 (χ²_0.05;2 > 5.991). However, the normal distribution test may not be rejected for all remaining portfolios between 2008 and 2012 (χ²_0.05;2 ≤ 5.991), and therefore, the CAPM model may be used to explain the relationship between the market returns and risk of construction portfolios. Before the crisis, both portfolios reported positive returns, and non-family firms outperformed family-controlled ones by 1.22%. The returns were opposite in the crisis period, when family firms significantly outperformed non-family peers. In addition, the latter firms reported negative returns in the crisis. The data shows that tolerance for risk has declined since the crisis hit. This behavior seems to be rational, as invoked by the reaction to the global recession. In addition, public construction companies exhibited high proportions of specific, rather than systematic, risk. In periods before and after the crisis, all construction portfolios were oversensitive with respect to the market (β > 1). During the crisis, both portfolios were less sensitive to the market (β < 1). When the crisis hit, all public construction companies were overvalued, except for family-controlled ones, which were undervalued.

4. Conclusions

This research focused on testing the validity of CAPM on the Warsaw Stock Exchange across two portfolios of family- and non-family-controlled companies. These portfolios were constructed on the basis of the complete data retrieved from the Warsaw Stock Exchange. As a consequence, we made calculations on the whole population (not sample) data. In this respect, even the small amount of data does not exclude an inference. These two portfolios were then adjusted for the size, i.e., market capitalization, to avoid the dominance of large companies in the population.

The assumption of normal distribution may not be rejected according to the Jarque–Bera test for the period from 2008 to 2012; therefore, the relation between return and risk holds in the case of the WSE. However, for the period from 2006 to 2007, the Jarque–Bera yielded outlying numbers; therefore, we did not draw conclusions.

By assuming that market portfolios were efficient, returns of family construction companies significantly outperformed their nonfamily peers in the latest crisis. Investors who invested in family-controlled portfolios during that time might have expected exceptional returns of 23.56%. In addition, these securities were undervalued, which does not prove CAPM invalidity. As Jensen argued [55], “deficiencies as measurement and model specification error due to proxies for variables do not reject CAPM”.

Although the capital asset pricing model was substantially developed since its inception (see Theoretical Background), we decided to prove its validity as developed by Sharpe [10], Lintner [11] and Mossin [12], because of scant testing in the case of family firms. However it would be tempting to move beyond this version of CAPM to get more insight into the performance of family firms on stock exchanges.

This research was limited to public companies. In addition to the extraordinary returns of family-controlled firms, the results suggest that a vast amount of risk is specific for all construction portfolios. It would be interesting to find out the sources of this risk. As mentioned in the Introduction, Poland was the only EU country in which the construction industry withstood the crisis and reported positive returns. However, as this research is limited to public companies, it would be interesting to analyze in-depth factors that allowed family-controlled firms operating in the construction sector to outperform their non-family peers. In addition, this paper used the capital asset pricing model to explain the performance of two portfolios that used a family-ownership as the distinction factor. The outperformance of the family-owned firms during the crisis may be either attributed to family-ownership or non-family-related factors and being the result of portfolio composition. This issue also needs to be further analyzed, i.e., by conducting a qualitative research.