Nonprofits and C Corporations: Performance Comparison

Abstract

We extend the performance comparison study of nonprofits (NPs) and pass-throughs by examining large NPs and large C corporations (CCs). Unlike that study, we also examine performance outcomes under two different tax shield policies. We use the Capital Structure Model as our main methodology. Our purpose is to compare large NPs with large CCs in terms of debt choice, valuation, leverage gain, and growth-related outcomes. All tests considered, NPs (compared to CCs) have a 34.90% valuation advantage; achieve a 78.12% greater increase in value when going from nongrowth to growth (using a 12.34% lower plowback ratio and 10.97% less in retained earnings); attain a 2.56% greater optimal leverage ratio; and, realize 10.97% less in dollars added from debt. We show that switching from an interest tax shield to a retained earnings tax shield increases CC value between 1.35% and 3.28%. The NP value limit is only 0.42% since NPs pay little taxes. Our findings are value-additive for the comparative ownership form research.

1. Introduction

Earlier Capital Structure Model (CSM) research (Hull and Price 2015; Hull 2020b) examines the performance of the for-profit (FP) ownership types, namely, C corporations (CCs) and pass-through (PTs). These studies focus on US ownership types. The most recent CSM research investigates and compares the performance of the US nonprofit (NP) ownership form and the US FP ownership form consisting of the PT ownership type (Hull and Van Dalsem 2021). This paper extends the comparative ownership performance research by being the first CSM study to compare the performances of NPs and CCs as measured by debt choice, valuation, leverage gain, and growth-related outcomes. More specifically, we compare the largest NPs and the largest CCs as the largest enterprises dominate these two ownership categories in terms of revenue and expenses. Comparative ownership performance research is important given that organizations migrate from one ownership form to another looking for the best fit to maximize their sales and services. For example, hospitals change between NP and FP status. In addition, NPs can contain both profit and nonprofit centers. Thus, NPs can resemble FPs by paying business and personal taxes on their for-profit business ventures.

This paper’s purpose can be expressed as the comparison of the performance of large NPs with large CCs under different scenarios involving tax rates, legislative tax environments, nongrowth versus growth, and different tax policies. In regard to the latter, we test two tax policies. First, we examine a tax policy (common throughout the world) that allows an interest tax shield (ITS) where interest paid on debt is a tax deductible expenses. Second, we replace an ITS tax policy with a retained earnings tax shield (RTS) tax policy that allows for a direct tax deduction on retained earnings (or internal equity funds) that are used for growth. To our knowledge, there is no widespread use of an RTS, albeit there are policies similar to an RTS such as a federal government policy that grants tax credit or subsidies for retained earnings used for research and development (R&D). Tax credits and subsidies related to R&D tend to be used as a temporary solution for countries with failing economies.

As noted by researchers who examine organization structures such as Anheier (2014), organizations switch ownership forms. One reason to switch concerns profitability. Unlike FPs, NPs are not viewed as profit-driven. Regardless, they share features similar to the FP ownership types of PT and CC. To illustrate, both NPs and FPs consist largely of small enterprises. In addition, most NP and FP revenue comes from small subsets of the largest NPs and the largest FPs. As noted by Hull and Van Dalsem (2021), since NPs depend on FP earnings for funding, NPs have their welfare influenced by same market forces as FPs. They also point out that NPs and FPs compete within the same industries. For example, consider the healthcare industry where NPs and FPs (be they PTs or CCs) supply the same services and compete for the same customers. NPs can also compete with FPs when they sponsor for-profit side ventures. Finally, NPs and FPs are alike in having payroll taxes and in producing financial statements that report assets, liabilities, revenues, and expenses.

Hull and Van Dalsem (2021) write that NPs have three major tax benefits (albeit these benefits would not apply for any for-profit activity by an NP). First, NPs are tax-exempt, which means they can avoid the FP forms of taxes such as federal, state, county, municipal, property, and sales. Second, NPs do not have payouts to equity owners in the form of taxable dividends and capital appreciation. Third, since NPs issue tax-exempt debt, its debtholders can avoid paying taxes on interest income. To illustrate the nature of tax-exempt debt holdings, NPs pursue financing through tax-free municipal bonds that are issued by states and municipalities. As noted by Hull and Van Dalsem, these bonds are tax-exempt for the bondholders unless more than 5% of proceeds are used for profitable activities. In conclusion, NPs can circumvent forms of taxes paid by FPs with the major exception being payroll taxes used to fund Social Security and Medicare.

Given the nature of this paper’s extension of recent CSM research on comparing the performance of ownership forms (Hull 2020b; Hull and Van Dalsem 2021), its design and contents resemble this research. Due to our focus on CC tax rates (as opposed to PT tax rates), we only apply the CSM of Hull (2018) that focuses on CCs and so we use this version of the CSM as our method to determine optimal outcomes for NPs and CCs. The use of the CC version of the CSM is consistent with the notion that NPs with taxable side-ventures are more likely to be taxed as CCs than PTs. The CSM research of Hull (2019) also covers CCs while deriving unique CSM equations applicable to PTs and the tax shield policies used in this paper (namely, ITS and RTS). The CSM enables us to calculate firm value for increasing debt-for-equity choices associated with credit ratings of diminishing quality. From these calculations, we can pinpoint the maximum firm value (max V_L) and thus detect the optimal debt-to-firm value ratio (ODV) that accompanies the optimal credit rating (OCR). As noted by prior ownership comparison research, the key to these calculations is the assumption of parallel risk classes. Because NPs draw large sources of funding from FPs, their revenue streams rely on the same economic factors as FPs. Thus, both NPs and FPs are capable of having parallel risk classes.

Parallel risk classes imply that NPs and FPs can experience similar costs of borrowing. To generate borrowing costs, we use data given by Damodaran (2021) for 2020, which are the most recent data at the time this study originated. These costs of borrowing are based on Damodaran’s credit spreads that he matches to bond ratings and interest coverage ratios (ICRs). The same borrowing costs for NPs and FPs enable the CSM to compute outcomes so that comparisons between ownership forms can be made and conclusions can be drawn that are based on contrasting tax rates assigned to different ownership forms.

Hull and Van Dalsem (2021) offer four arguments that support the notion that the outcomes for NPs and PTs can be based on parallel risk classes. Their arguments also apply to this study of NPs and CCs. Thus, for what follows, while using the Hull and Van Dalsem NP arguments, we adapt their PT arguments to CCs. First, there exists businesses (such as the healthcare industry) that can choose between being an NP or CC and for which they compete for producing the same goods and services. Second, NPs and CCs are similar in that both consist largely of small organizations while having large entities that dominate in terms of revenue and expenses. Third, NPs and CCs are influenced by the same economic and market conditions in that NPs are dependent on funding provided by CCs and their workforce. As noted by Hull and Van Dalsem, even government funding for NPs is dependent on FPs (and thus CCs) paying taxes. Fourth, charitable contributions received by NPs (such as revenue streams from CCs) fall during recessionary periods of financial distress.

Our findings are new as the comparative research on NPs versus CCs is (to our knowledge) nonexistent in terms of the outcomes we examine. For our growth tests, we use the historical growth rate of 3.12% and two sets of higher growth rates projected by tax experts under the Tax Cuts and Jobs Act (TCJA). We also test two sets of tax rates for a pre-TCJA tax law environment and two sets for TCJA tax law environment. These sets of tax rates (described in Section 2.3) are consistent with those suggested by tax experts. Unlike prior ownership performance comparison research, our findings cover two different tax policies used by Hull and Hull (2021), namely, an ITS policy and an RTS policy. For an ITS policy, interest lowers taxable income. For an RTS policy, retained earnings (RE) lowers taxable income. For the CSM, RE refers to all internally generated cash flows set aside for growth before taxes are paid. For an RTS policy, taxes are not paid on RE if used for growth. In this study, we use an RTS policy, which as developed by Hull (2019) refers to a “100%” or “full” RTS policy. This policy differs from a partial-RTS policy, which was a focus of the taxpayer wealth and federal tax revenue study of Hull and Hull (2021), where partial refers to using only part of RE for growth as a tax deduction.

This study’s array of tests (involving different growth rates, different tax rate environment, different tax rate sets, and different tax policies) help to further establish the scientific value of our findings. Our findings are based on 48 tests consisting of 24 NP tests and 24 CC tests where NPs refer to large NPs and CCs refer to large CCs. Since this study is the first ownership performance comparison study that considers two tax policies, the presentation of our findings will include (when applicable) which tax policy drives our overall findings from our 48 tests; otherwise, our findings are to be taken as being strongly driven by both ITS and RTS tests.

With the above in mind, we summarize some of our key findings below in a manner used by prior research (Hull 2020b; Hull and Van Dalsem 2021). However, first we should point out that there is no expectation that similarities should exist in our key findings with those of prior research. For example, Hull and Van Dalsem tests focus on smaller PTs and smaller NPs, while this study focuses on larger NPs and larger CCs. Thus, besides the different ownership types that can be involved, there are different size factors. In addition, our study is the only study to include RTS policy tests. In brief, we do not attempt to make any comparisons between this study and prior research in their key findings as that as that may not be possible due too significant differences.

First, we find that ODVs are 2.56% higher for NPs compared to CCs revealing that NPs utilize slightly more leverage to reach maximum firm value (max V_L). These results are driven by the RTS tests where ODV is 6.69% higher for NPs compared to 1.56% lower for ITS tests. Second, we show that max V_L for NPs is 34.90% higher than CCs. This finding reveals how tax avoidance impacts value. Third, we discover that NPs achieve a 78.12% greater increase in value compared to CCs when going from their nongrowth max V_L to their growth max V_L. This finding is largely explained by the ITS tests where NPs achieve a 132.42% greater increase compared to 23.82% for RTS tests.

Fourth, NPs achieve their increase in value from growth with a before-tax plowback ratio (PBR_BT) that is 12.34% smaller than CCs and an unlevered growth rate (g_U) that is 8.40% lower than CCs. The latter two results are largely explained by the ITS tests. The smaller values for NPs occur because PBR_BT and g_U are positively related to RE and CCs can only use RE after business taxes are paid on it while NPs are capable of avoiding business taxes. Thus, a dollar of RE goes further for NPs. Fifth, in terms of the maximum gain to leverage (max G_L), NPs gain 10.97% less in absolute dollars by issuing debt compared to CCs. This can be explained by the ITS tests where NPs gain 24.29% less compared to 2.36% more for RTS tests. The ITS finding that NPs gain less compared to CCs can be explained by not having a large interest tax shield due to paying lower taxes.

Sixth, in terms of the maximum percent change in unlevered equity (max %∆E_U) from a debt-for-equity transaction, we find that NPs increase 6.02% beyond their unlevered equity (E_U) value with leverage compared to 10.13% for CCs. In addition, we discover that max %∆E_U is 50.46% lower for NPs compared to CCs. These results are driven by the ITS tests where max %∆E_U is 65.79% lower for NPs compared to 35.13% lower for RTS tests. Seventh, with regard to the net benefit from leverage (NB), we find that E_U for NPs rises 17.06% for every dollar of debt while E_U for CCs increases 29.33% (which indicates that NB is 12.27% lower for NPs compared to CCs). The lower value of 12.27% is explained by both ITS and RTS tests. While these percentages suggest that NPs are less efficient in their use of each dollar of debt, they also reflect the fact that NPs have higher firm valuations and so they must issue more debt to attain the same ODVs and same optimal credit ratings (OCRs). For both NPs and CCs, OCR is Moody’s rating of A3 except for pre-TCJA tests for CCs where OCR is Baa2 (which is a rating that is one notch less in quality).

2. Background and Literature Review

This section largely follows the NP and PT study of Hull and Van Dalsem (2021) but is updated to replace PTs with CCs. This section begins by providing background information on the ownership forms of nonprofit (NP) and for-profit (FP); sources of equity and debt financing for NPs and CCs; and, tax rates applicable to NPs and CCs. We then provide a literature review of capital structure models.

2.1. Key Features of Nonprofits (NPs) and C Corporations (CCs)

Table 1. Main Features for Nonprofits (NPs) and C Corps (CCs).

Nonprofit	C Corp
Owned By: No individuals	Owned By: Individual investors
Ownership categories: Corporation (501c3), association, trust	Ownership categories: C corp (files its own tax return using Form 1120)
Board Members: Nominated	Board Members: Elected by equity owners
Official obligations: Fulfill duties related to goals involving service, education, and research	Official obligations: Increase ownership wealth by providing profitable services and products
Major Goal: Maximize value in terms of service distributions	Major Goal: Maximize value in terms of monetary distributions
Mission: Described largely in terms of service, education, research, and growth	Mission: Described largely in terms of profit, efficiency, service, and growth
Decision-making and implementation: Cautiously proceeds to satisfy mission and constituencies	Decision-making and implementation: Quickly responds to profitable opportunities
Sources of Revenues: Services, contributions, grants, and investments (including endowment income)	Sources of Revenues: Business activities (sales and services) and investments in other businesses
Equity Distributions: Non-monetary distributions in form of services rendered to those in need	Equity Distributions: Monetary distributions to owners in form of cash payouts and capital gains
Sources of Equity Financing: Internal equity (eligible revenues, investment/endowment income); External equity (contributions, grants, government)	Sources of Equity Financing: Internal equity (retained earnings); External equity (new seasoned equity offerings or equity-like offerings such as warrants)
Sources of Debt Financing: Personal tax-exempt debt, nonfinancial debt (mortgages), short-term debt (trade credit, bank borrowings avoided as interest not exempt from taxes)	Sources of Debt Financing: Bond issues (various types such as senior, subordinate, callable, convertible, etc.), short-term debt (trade credit, bank borrowings)
Corporate Taxes: Only apply to profitable ventures	Corporate Taxes: Apply to taxable corporate earnings
Personal Equity Taxes: Zero unless there are taxable for-profit ventures that have distributions	Personal Equity Taxes: Personal taxes paid on dividends and capital gains
Personal Debt Taxes: Zero or minor due to issuing mostly tax-exempt debt	Personal Debt Taxes: Personal taxes paid on interest from debt
Interest or Retained Earnings Tax Shield: Zero or small tax shields for side ventures that are for-profit	Interest or Retained Earnings Tax Shield: Full business tax shields

Table 1 provides key features for nonprofits (NPs) and C corps (CCs) for fifteen categories so that similarities and differences among these two ownership types can be compared.

provides an overview of the main features for the ownership categories of NPs and CCs in a fashion similarly performed by Hull and Van Dalsem (2021) for NPs and PTs. The Major Goal row of the Nonprofit column reports that NPs have a major goal of maximizing service distributions. In contrast, the C Corp column for this row states that CCs strive to maximize monetary distributions. While these goals appear to be dissimilar, they have the same intertwined theme of value be it monetized or unmonetized. The Equity Distributions row portrays the same theme. NP equity distributions are services performed, while CC equity distributions are monetarized as cash payouts and capital gains.

The topic of taxes is discussed in the last five rows of Table 1. The row on corporate taxes and the two rows on personal taxes furnish an indicator of the favorable after-tax valuation of NPs compared to CCs. As found in the last row, only CCs generate a large ITS and/or RTS. However, NPs can counterbalance this tax shield shortcoming (as found in the Sources of Debt Financing row) by issuing local and state government bonds that are exempt from personal taxes. Because of the ability to issue tax-exempt debt, NPs will avoid taxable debt (such as bank debt). Hull and Van Dalsem (2021) write that CCs pay both corporate taxes on earnings and personal taxes on distributions and capital gains. With the ratification in December 2017 of the Tax Cuts and Jobs Act (TCJA), the maximum CC tax rate of 0.35 fell to a flat rate of 0.21 while that for PTs and personal taxpayers declined much less from 0.396 to 0.37. However, the personal tax rate typically paid by CC owners is below the maximum statutory tax rate of 0.37, which is a rate that applies to PTs and salaried workers. The maximum personal tax rate paid by most CC owners on qualified dividends and capital gains is 0.20.

Notwithstanding their dissimilarities in tax liabilities, NPs and CCs have many of the same key features. Below we discuss five of these.

First, some enterprises can elect to be either an NP or a CC. As noted by Hull and Van Dalsem (2021), an ownership form choice indicates an enterprise can take on substitute ownership forms. Furthermore, NPs can take on for-profit activities with these activities typically taxed at CC tax rates. In addition, within industries like the health care industry, NPs coexist with CCs with each supplying the same goods and services.

Second, like most CCs (that are small), NPs are not publicly traded. Thus, as noted by Hull and Van Dalsem (2021), market risk for NPs cannot be directly computed from empirical data when using the CAPM beta (whereas betas can be computed for large CCs). Regardless, all NPs and all CCs are influenced by market conditions as their well-beings are altered by factors that shape the economic environment for business, investing and employment change. For example, large NP donors give through incomes tied to their for-profit businesses, which depend on favorable market conditions. Furthermore, as pointed out by Hull and Van Dalsem, another major source of income for NPs is their investment portfolios that can be significantly invested in the stock market and so involve assets subject to market risk. The holding of large stock portfolios should be especially applicable to the largest NPs that we study.

Third, since market risk influences NPs and CCs, they are both vulnerable to risk types that together embody market risk. As discussed by Hull and Van Dalsem (2021), these risk types include interest rate, exchange rate, geopolitical events, and recessions. In regard to recessionary risk, they contend that charitable contributions received by NPs decline when businesses falter during an economic recession such as is caused by a financial crisis, an external trade shock, an hostile supply shock, the bursting of an economic bubble, or a large-scale natural or anthropogenic disaster. It follows that NPs and CCs partake in risk categories that all enterprises face including operational risk and financial risk as well as other less common risk categories. Given the similar exposure of NPs and CCs to all forms of risk, our tests assume that NPs and CCs can inhabit similar risk classes and thus can share in the same costs of borrowing. This assumption enables us to compare these two firm types based on their different tax situations.

Fourth, while both NPs and CCs consist primarily of smaller enterprises, a small subset of the largest NPs and CCs dominate. For example, Brookings (2017) states that 1% of FPs (e.g., CCs and PTs combined) have more than USD 10 million in revenues. As described by Hull and Van Dalsem (2021) similar statistics exist for the largest NPs. They note that the National Center for Charitable Statistics (2018) writes that even after excluding NPs with gross receipts below the USD 50,000 filing threshold, the remaining two-thirds of NPs in 2015 contain 210,670 public charities with less than USD 500,000 in expenses (revenues average about 7.5% greater than expenses). Hull and Van Dalsem state that these 210,670 NPs compose less than two percent of total public charity expenses of USD 32.3 billion. Finally, they report that NPs with USD 10 million or more in expenses include about 5% of total public charities but account for nearly 88% of public charity expenses of USD 1.6 trillion.

2.2. Equity and Debt Financing for Nonprofits (NPs) and C Corporations (CCs)

Hull and Van Dalsem (2021) report that NP researchers (Bowman 2002; C. Miller 2003; Jegers and Verschueren 2006; Calabrese 2011) describe NP borrowings in the same manner as the two general FP types of financing: equity and debt. In this section, we borrow from Hull and Van Dalsem when discussing NP and CC financing except, instead of contrasting NP and PT financing (as done by Hull and Van Dalsem), we will once again contrast the NP and CC ownership types focusing on large NPs and large CC.

For the key feature labeled Sources of Equity Financing in Table 1, we find that internal equity and external equity are given as the two major sources of equity financing for both NPs and CCs. For this paper that uses the Capital Structure Model (CSM), the CSM refers to internal equity as the cash flows generated by an enterprise and set aside for growth before any applicable taxes are supplied. As noted by Hull and Van Dalsem (2021), internal equity for NPs include unrestricted cash flows or cash flows earmarked for growth in the form of eligible revenues and investment/endowment income while external equity for NPs include cash inflows for growth from contributions, grants, and government sources. For CCs, internal equity includes retained earnings while external equity consists largely of money raised from investors, which for large CCs include proceeds from seasoned equity offerings (or derivatives tied to equity warrants).

For the key feature labeled Sources of Debt Financing, Table 1 reports that debt for NPs can be classified as (i) tax-exempt for which no personal taxes exist, (ii) nonfinancial debt (consisting of mortgages that serves the same purpose as long-term financial debt,) and (iii) short-term debt (mostly trade credit as bank borrowings are avoided). Researchers (Bowman 2015; Calabrese and Ely 2016) suggest that not only does tax-exempt debt dominate the long-term debt for NPs but it has been on the rise since the 1990s. Because NPs can issue tax exempt debt, they avoid the more expensive taxable debt such as bank debt. As noted by Hull and Van Dalsem (2021), NPs (like CCs) will use trade credit and a line of credit with banks to cover short-term needs. Akin to trade credit is a line of credit with a bank that NPs (like CCs) can utilize to cover the holes in uneven monthly revenue. Unlike large NPs, large CCs undertake large issues of taxable corporate bonds. Table 1 reports that these bond issues include various types (senior subordinate, callable, convertible, and so forth).

In terms of comparing financing forms between NPs and FPs, Hull and Van Dalsem (2021) indicate that the literature is sparce with one exception being the heathcare industry where NPs coexist with FPs (both PTs and CCs). Noting that the NP and FP ownership forms have unique financing mechanisms, Trussell (2012) argues that these differences do not impact the relative amount of debt and equity in their capital structures. The latter is consistent with both this paper’s findings and those of Hull and Van Dalsem.

2.3. Assignment of Unlevered and Levered Tax Rates for Nonprofits (NPs) and C Corporations (CCs)

In addition to federal taxes, CCs (like all FPs) pay non-federal taxes such as excise, state, county, municipal, property, sales, and payroll. With the exception of payroll taxes, NPs can avoid forms of taxes paid by FPs unless the NP is staffed by all volunteers. NPs can also take on taxable FP ventures for which significant taxes can be paid. However, these FP ventures for NPs are limited in that a major problem develops if FP ventures begin overtaking NP activities. The end result is that an NP will risk losing its tax-exempt status as too many revenue-producing FP ventures are not consistent with an NP’s mission. Simply put, profitable business activities unrelated to an NP’s purpose should not absorb a substantial amount of NP employee time or produce greater income than generated by their NP activities. For states without taxes, CCs (like all FPs) do not pay state taxes and so can resemble NPs by avoiding state taxes. While NPs may not typically have taxable revenue to shield, profitable CCs do. However, CCs can avoid taxes through loopholes, especially at the federal level. For our tests, we only consider federal taxes as one major goal of our paper is to show how federal revenue is influenced by a country’s federal (or national government) tax shield policy.

For this paper’s methodology, we use the CSM which is a model that requires the assignment of tax rates for its application. As described in Section 3.1 this model, as extended by Hull (2014) and corrected by Hull (2018), includes the use of unlevered and levered tax rates where the subscript “1” indicates an unlevered tax rate and the subscript “2” indicates a levered tax rate. For the CSM research (Hull 2005; Hull and Price 2015) that does not allow for a change in tax rates, the same tax rate is used for all leverage choices. For this research, the corporate, personal equity, and debt tax rates are simply referred to as T_C, T_E, and T_D, respectively.

As first defined by Hull (2014), T_C1, T_E1, and T_D1 are the respective unlevered corporate, personal equity, and personal debt tax rates while T_C2, T_E2, and T_D2 are the corresponding levered tax rates that are modified with changes in debt. Since unlevered refers to no debt, the unlevered debt tax rate of T_D1 is only used for the practical purpose as a starting point from which the debt tax rate can change once debt is first issued. Because we begin with an unlevered firm and allow tax rates to change when debt increases, Hull argues that T_D1 < T_D2, T_C2 < T_C1, and T_E2 < T_E1 hold. For our tests, each tax rate changes by 0.03 in its predicted direction, which means that the corporate and personal equity tax rates fall, while the personal debt tax rate rises with each increasing debt-for-equity choice. Each of our fifteen increasing debt-for-equity choice corresponds to a fall in the quality for one of the fifteen credit ratings given by Damodaran (2021).

Like the PT and NP performance comparison study of Hull and Van Dalsem (2021), a key tax rate for this CC and NP study is the business tax rate. Not only does it influence the ability of a firm to grow, but it also impacts the amount of its tax shield. For this study, the business tax rate is arguably more important since this study uses two tax shields (namely, ITS and RTS) with each shield affected by the business tax rate. By testing two tax shields, this study significantly differs from the study by Hull and Van Dalsem that only uses an ITS. For CCs and NPs (with taxable business ventures), the business tax rate is the corporate tax rate. Thus, unlike a study of PTs that has no corporate tax rate but only personal tax rates, this study of CCs deals with double taxation as both corporate and personal taxes apply. Of further importance, this study uses large NPs where significant taxable business ventures are more prevalent as they can be needed to attain wide-ranging growth goals. Large NPs with taxable ventures will (like CCs) experience double taxation since NPs are primarily classified (from a tax point) as paying both corporate and personal taxes. In the remainder of the section, we will provide the tax rates used in our tests. At the end of this section, these tax rates will be summarized in table format.

2.3.1. Low “L” Tax Rate Tests

After introducing the topic of tax rates, this subsection describes the “low” (L) tax rate set for NPs and CCs. The next subsection discusses the “high” (H) tax rate set for NPs and CCs. Both of these sets use CC tax rates since NPs are generally taxed as CCs when they have taxable side businesses. Because some NPs are PTs, we make adjustments, where applicable, when assigning personal tax rates to account for that fact PTs have higher personal tax rates than CCs. For CCs, we use tax rates similar to prior CSM research that tests CCs (Hull and Hull 2021; Hull 2020a, 2020b). This prior research documents the sources used when setting targeted levered tax rates.

Historically, tax rates often change and some tax experts expect that the current corporate tax rate can rise in the future returning to pre-TCJA tax levels. Thus, besides using current TCJA rates in tests, we also use pre-TCJA rates where the corporate tax rate is much higher. Given the above, we apply tax rates within two tax law environments: a pre-TCJA tax law environment and a TCJA tax law environment. The latter began 1 January 2018. From our study of the tax sources, for which we find median tax rates that are smaller than mean tax rates, one can argue that L tax rates give a greater weight to median tax rate values whereas H tax rates give a greater weight to mean tax rate values.

For our low (L) tax rate tests for a pre-TCJA tax law environment, we use zero tax rates for NPs under the assumption there are no FP activities. With no owners for NPs, there are no payouts and so T_E1 is zero. Since zero tax rates cannot change with leverage, the levered tax rate T_E2 is also zero at ODV. Bowman (2015) states that NP debt is mostly tax-exempt and suggests more and more NPs are issuing only tax-exempt debt. Since NPs can issue debt that is exempt from personal taxes, it is likely that debtholders attain a personal tax rate of zero on its debt ownership. Thus, for our L tests we use zero for T_D1 and T_D2. With no corporate taxes from FP activities, T_C1 and T_C2 are also zero. These zero tax rates are also used for our TCJA tests. If there are no FP activities, then different tax laws such as ITS and RTS have no influence as each depends on a positive business tax rate. In addition, without FP activities, changes in tax brackets and ownership types under which NPs might be taxed are irrelevant as corporate and personal taxes are all zero. In brief, all L tests for NPs use zero tax rates.

For CC tax rates for L tests under a pre-TCJA tax law environment, we assign the following three unlevered and targeted levered tax rates. First, for CC equity taxpaying owners, we use an unlevered T_E1 of 0.11. This CC usage enables us to achieve a levered T_E2 that is near our desired target of 0.094 at ODV. Second, for personal taxes on debt where the debt tax rate increases with leverage, we begin with a T_D1 of 0.15 to achieve a levered T_D2 that is near our target of 0.175 at ODV. Third, for corporate taxes, we use an unlevered T_C1 of 0.30 to achieve a levered T_C2 that is near our target of 0.254 at ODV.

For CC tax rates for L tests under a TCJA tax law environment, personal equity tax rates on dividends and capital gains are the same as their pre-TCJA rates as tax laws governing dividends and capital gains are unchanged. Thus, T_E1 and T_E2 remain at 0.11 and 0.094, respectively. However, TCJA slightly lowered the tax rate on interest income. Thus, we slightly decrease the personal tax rates on debt so that T_D1 is now 0.14 with a target of 0.16 for T_D2 at ODV. Because corporate tax rates dropped from a maximum tax rate of 0.35 before TCJA to a flat rate of 0.21 for TCJA, we set T_C1 at 0.19 to achieve our target of 0.165 for T_C2 at ODV.

2.3.2. High “H” Tax Rate Tests

For our high (H) tax rate tests under pre-TCJA, we use non-zero tax rates for NPs under the assumption that a number of large NPs will engage in taxable adventures to support their nonprofit activities. Non-zero tax rates are consistent with the notion that large NPs are more likely to have the resources to successfully support taxable business ventures. This paper’s high tax rates differ from Hull and Van Dalsem (2021) who tests smaller NPs that would have smaller for-profit business ventures and less capacity to issue tax-exempt debt. For NP tests utilizing H tax rates under a pre-TCJA tax law environment, we use a T_E1 of 0.05 to achieve our target of 0.045 for T_E2 at ODV. The personal tax rates are tweaked upward since some NPs may be PTs and so pay a much higher personal tax rate. Otherwise, personal taxes are largely governed by the lower capital gains and qualified dividends rate but even here can also be influenced in some situations by the higher personal income tax rates. Since large NPs have greater sources of funds, we continue to use zero tax rates for T_D1 and T_D2 under the assumption that the largest NPs are better equipped to avoid taxable debt. In addition, the latest borrowing trend for NPs is “impact financing” where NPs lend money to other NPs. Profits from these lending endeavors do not create taxable interest because the interest goes back into the lending NP for more lending to other NPs that need financing. This is further evidence that zero debt tax rates may hold for NPs regardless of FP activities. For NPs, we use pre-TCJA values for T_C1 and T_C2 that are 0.06 and 0.05, respectively.

For H tests for NPs under TCJA, we use values for T_E1, T_D1 and T_C1 of 0.04, 0, and 0.04 to achieve respective levered targeted values for T_E2, T_D2, and T_C2 of 0.035, 0, and 0.035 at ODV. The T_C1 fall from a pre-TCJA of 0.06 to a TCJA of 0.04 is justified due to the large fall of 0.14 in the maximum corporate tax rate under TCJA. The decrease in the personal income tax bracket is only 0.026 less (but tax brackets are also favorable in lowering taxes paid) so we assign the fall in T_E1 from 0.05 to 0.04 when going from pre-TCJA tests to TCJA tests. The same rate of 0.04 for T_E1 and T_C1 can be justified given the number of tax loopholes that FPs have when it comes to lowering corporate taxes. Our set of tax rates for NPs assumes that nearly one-fifth of NP activities are FP activities. This can be accomplished in a number of ways ranging from small side ventures to actually owning and operating for-profit subsidiaries that serve as a source of income to finance the NP’s non-profit activities.

CC values that we use for H tests in a pre-TCJA tax law environment are as follows: The unlevered tax rate values for T_E1, T_D1 and T_C1 are set at 0.165, 0.18, and 0.21, respectively, to achieve corresponding targeted levered values for T_E2, T_D2, and T_C2 of 0.14, 0.224, and 0.295 at ODV. Tax rates for CC utilized for H tests in a TCJA tax rate setting are as follows. The unlevered tax rate values for T_E1, T_D1 and T_C1 of 0.165, 0.180, and 0.21 are fixed to attain respective targeted levered values for T_E2, T_D2, and T_C2 of 0.14, 0.21, and 0.18 at ODV.

Finally, it can be noted that unlevered tax rates and targeted levered tax rates are the same for both an ITS policy and RTS policy. For all tests, we achieve effective levered tax rates that are similar to what was targeted. This is discussed below where we show the actual differences between targeted levered tax rates and the effective levered tax rates that actually occur for our tests.

Table 2. Two Tax Rate Sets for Large Nonprofits and Large C Corporations.

Nonprofit (unlevered, levered, and target tax rates)	C Corporation (unlevered, levered, and target tax rates)
Panel A: Low (L) Tax Rates
Pre-TCJA (target levered rate in parenthesis)
Nonprofit (NP): Tax rates are the same for ITS and RTS	C Corp (CC): Levered tax rates differ for ITS and RTS
	ITS
TC1 = 0 and TC2 = 0 (0)	TC1 = 0.30 and TC2 = 0.2499 (0.254)
TE1 = 0 and TE2 = 0 (0)	TE1 = 0.11 and TE2 = 0. 0916 (0.094)
TD1 = 0 and TD2 = 0 (0)	TD1 = 0.15 and TD2 = 0.1791 (0.175)
	RTS
	TC1 = 0.30 and TC2 = 0.2577 (0.254)
	TE1 = 0.11 and TE2 = 0. 0945 (0.094)
	TD1 = 0.15 and TD2 = 0.1739 (0.175)
TCJA (target levered rate in parenthesis)
Nonprofit (NP): Tax rates are the same for ITS and RTS	C Corp (CC): Tax rates are the same for ITS and RTS
TC1 = 0 and TC2 = 0 (0)	TC1 = 0.19 and TC2 = 0.1632 (0.165)
TE1 = 0 and TE2 = 0 (0)	TE1 = 0.11 and TE2 = 0. 0945 (0.094)
TD1 = 0 and TD2 = 0 (0)	TD1 = 0.14 and TD2 = 0.1623 (0.16)
Panel B: High (H) Tax Rates
Pre-TCJA (target levered rate in parenthesis)
Nonprofit (NP): Tax rates are the same for ITS and RTS	C Corp (CC): Levered tax rates differ for ITS and RTS
	ITS
TC1 = 0.06 and TC2 = 0.0515 (0.05)	TC1 = 0.35 and TC2 = 0.2915 (0.295)
TE1 = 0.05 and TE2 = 0.0429 (0.045)	TE1 = 0.165 and TE2 = 0.1374 (0.14)
TD1 = 0.0 and TD2 = 0 (0)	TD1 = 0.19 and TD2 = 0.2269 (0.224)
	RTS
	TC1 = 0.35 and TC2 = 0.3006 (0.295)
	TE1 = 0.165 and TE2 = 0.1417 (0.14)
	TD1 = 0.19 and TD2 = 0.2203 (0.224)
TCJA (target levered rate in parenthesis)
Nonprofit (NP): Tax rates are the same for ITS and RTS	C Corp (CC): Tax rates are the same for ITS and RTS
TC1 = 0.04 and TC2 = 0.0343 (0.035)	TC1 = 0.21 and TC2 = 0.1803 (0.18)
TE1 = 0.04 and TE2 = 0.0343 (0.035)	TE1 = 0.165 and TE2 = 0.1417 (0.14)
TD1 = 0 and TD2 = 0 (0)	TD1 = 0.180 and TD2 = 0.2087 (0.21)

Table 2 reports tax rates for two tax sets based on two tax rate scenarios: a low (L) tax rate set and a high (H) tax rate set. Each of these two tax rate sets are tested under two different tax law environments (pre-TCJA and TCJA) and two different tax shield laws (ITS and RTS). Unlevered tax rates are given first and are followed by levered tax rates where the levered rate is the effective (or actual) tax rate achieved in our tests. The tax rate in the parentheses is the targeted levered tax rate that is suggested by prior CSM research (Hull and Price 2015; Hull 2019; Hull and Van Dalsem 2021). It is this research that documents tax sources for the targeted levered rates.

summarizes the tax rates just discussed. For each row, the unlevered tax rate is given first in both the Nonprofit and C Corp columns. This rate is followed by the levered tax rate that occurs for our tests at ODV. This levered tax rate is the effective tax rate that is actually achieved in our tests. The targeted tax rate is reported last and is in parentheses. The target rate is suggested by prior CSM research (Hull and Price 2015; Hull 2019; Hull and Van Dalsem 2021). It is this research that documents tax sources consistent with our targeted levered rates. As seen when comparing what our tests achieve and what we targeted, Table 2 reveals that all targets are reasonably realized. This means that we have set the unlevered rates with enough precision to achieve tax rates at ODV that are consistent with what tax experts suggest.

Panel A contains the L tax rates and Panel B provides the H tax rates. The top half of each panel gives the pre-TCJA tax rates and the bottom half reports the TCJA tax rates. For a pre-TCJA tax law environment, the ITS and RTS tests for CC generate different effective levered tax rates due to attaining different optimal credit ratings (OCRs). Thus, Table 2 reports the ITS and RTS results separately. When looking at Table 2, we can see the large change when going from an L tax rate to an H tax rate for the effective corporate business tax rate of T_C2. This rate affects both growth (through RE) and the tax shield policy, be it ITS where interest paid on debt is deductible expense or RTS where RE is a deductible expense. This can also be seen from the definitions where ITS = T_C2 × I and RTS = T_C2 × RE where I is the interest paid and RE is the before-tax earnings retained for growth.

2.4. Capital Structure Theory

Since prior research that compares ownership performance (Hull 2020b; Hull and Van Dalsem 2021) has overviewed capital structure theory, we will only summarize this research while also interjecting our own thoughts. This will be done below by briefly discussing the three primary capital structure theories: trade-off, agency, and pecking order.

First, trade-off theory (Baxter 1967; DeAngelo and Masulis 1980; Hackbarth et al. 2007; Berk et al. 2010; Hull 2018) contends that there is an optimal debt-to-firm value ratio (ODV) that maximizes firm value. Trade-off theory has been validated by empirical studies (Graham 2000; Korteweg 2010; Van Binsbergen et al. 2010) by showing that firm value increases from 4% to 10% when an ODV is attained. This range from 4% to 10% can arguably be broadened if the same tests were conducted under a different tax bracket system as the prior research was done pre-TCJA and even long before TCJA with different tax brackets. Regardless, a major feature of trade-off theory is the role of taxes and tax policy that typically favors debt over equity. In conclusion, if taxes are a primary determinant of an enterprise’s capital structure, we would expect differences in ODVs when comparing NPs and FPs since NPs have no taxes or small taxes.

Second, agency theory (Jensen and Meckling 1976; Jensen 1986; Manne 1999), like trade-off theory, advocates an optimal mix of debt-equity but contends that taxes are not necessary. Maximum valuation occurs at ODV simply from principal-agent valuation effects. For large FPs with many individual owners, there is greater separation between owners (principals) and managers (agents), thus leading to greater conflicts of interest between owners who supply capital and agents who manage the capital. For large NPs, capital is primarily provided by donors who lack the power given to the owners of large FPs. Thus, agency conflicts between donors and management for NPs can be diminished since NP donors do not have a profit incentive (like FP owners). However, donors can often sit on NP board of directors (granting them extra power).

In conclusion, if agency effects for NPs are less than CCs, we would expect differences in ODVs when comparing NPs and FPs. This would be similar to the expectation that taxes drive ODVs. However, if agency theory drives capital structure decision-making for both NPs and CCs and is found equally in both, we would expect fewer differences in ODVs.

Third, pecking order theory (Donaldson 1961; Myers 1977; Myers and Majluf 1984; Bowman 2002; Calabrese 2011) contends that the preference in financing for FPs begins with internal equity (e.g., RE for growth) followed by debt. New equity issuances is the last preference due to asymmetric information costs such as is reflected in the drop in stock price when a seasoned equity offering is announced. Researchers (Bowman 2002; Calabrese 2011) argue the same financing preferences for FPs apply to NPs. For example, the equity forms of financing (such as charitable contributions) take precedence over debt financing. Bowman (2002) writes that the actual cost of NP debt relates to the concern about default because of the difficulties involved with NPs selling off their assets. For Bowman, debt would be preferred over asset conversion (e.g., selling a portion of the endowment). Calabrese (2011) writes that NPs prefer internal equity (in the form of accumulated unrestricted residual revenues) over external financing. The information asymmetries triggering the preference for internal equity for NPs diverge from those present for FPs. This is because of fewer visible benefits to outsiders and variation in NP donor abilities. In conclusion, an argument can be offered that the impact on capital structure for NPs can be similar to FPs.

3. Methodology

In this section, we describe our methodology that consists largely of the Capital Structure Model (CSM). Of importance, the CSM addresses the missing research gap that this study focuses on, namely, the performance comparisons between large NPs and large CCs. The CSM is able to address this gap due to its advantages that include the following benefits. First, the CSM is a perpetuity model and this paper seeks to compare ownership types based on their long-term after-tax valuation. Second, the CSM is able to use all relevant factors needed for long-term valuation including costs of borrowing, growth rates, tax rates, and tax shield policies. Third, compared to other models, the CSM is more practical in terms of its application that entails novel approaches to using measurable inputs that supply precise outputs for ownership type comparisons. Fourth, the CSM is the only capital structure valuation model that uses unlevered and levered growth rates that that tie together the plowback-payout and debt-equity choices.

We also briefly overview prior research that details how the CSM is used to identify optimal outcomes such as maximum firm value (max V_L) and the optimal credit rating (OCR). We then present the method to get costs of borrowing using credit spreads that are matched to interest coverage ratios. This procedure holds a key to discovering max V_L and OCR.

3.1. Capital Structure Model (CSM)

The gain to leverage (G_L) is defined as V_L minus V_U where V_L and V_U are the levered and unlevered firm values with V_U often referred to as unlevered equity (E_U) since an unlevered firm is all equity. V_L is composed of levered equity (E_L) and debt (D). Hull (2014) expands on the earlier CSM research on C corps (CCs) under an ITS tax policy by incorporating changes in tax rates and shows that the nongrowth CC equation for G_L is

G_L(nongrowth) = (1 − α_Ir_D/r_L)D + (1 − α₂r_U/r_L)E_U

(1)

where

α_I is the M. Miller (1977) alpha first identified by Farrar and Selwyn (1967) and equals (1−T_E2)(1−T_C2)/(1−T_D2) while α₂ is the Hull (2014) alpha and equals (1−T_E2)(1−T_C2)/(1−T_E1)(1−T_C1) with T_E1 and T_E2 as the respective unlevered and levered personal equity tax rates, T_C1 and T_C2 as the respective unlevered and levered corporate equity tax rates, and T_D2 as the debt tax rate (which by definition is only a levered rate);

r_D, r_L, and r_U, are the respective costs of debt, levered equity, and unlevered equity;

D is the debt issuance that retires a portion of E_U with D = (1 − T_D2)I/r_D with I as the interest; and,

E_U(nongrowth) = (1 − T_E1)(1 − T_C1)C/r_U where C is the before-tax payout with C = (1 − PBR_BT)(CF_BT) where PBR_BT is the before-tax plowback ratio with PBR_BT = RE/CF_BT where PBR_BT = 0 since RE is the earnings retained for growth before taxes are paid where for Equation (1) we have RE (or RE_BT) = 0 as this is a nongrowth equation with no internal equity used for growth and CF_BT is the perpetual before-tax cash flow equal to C for nongrowth since PBR_BT = 0. The perpetual levered equity value (E_L) is influential in the derivational process for CSM equations. For a CC nongrowth situation, E_L = (1 − T_E2)(1 − T_C2)(C − I)/r_L.

As shown by Hull (2019) for a tax policy where RTS replaces ITS, Equation (1) maintains the same expression but two definitions for two variables are modified. First, the Miller alpha is now α₁ = (1 − T_E2)/(1 − T_D) as (1 − T_C2) falls out since I is no longer deductible. Second, the definition for debt is changed to D = (1 − T_D)(1 − T_C2)I/r_D where (1 − T_C2) indicates I is now taxed at the corporate level.

For this study that focuses on federal tax rates, it is more precise to say that CF_BT refers to cash flows before federal corporate taxes. CF_BT are the cash flows that can be used for paying federal taxes, interest income on debt, and dividends as well as supplying funds for RE. From an accounting standpoint, CF_BT resembles earnings before interest and taxes (EBIT) so that all expenses including non-federal taxes would have already been recorded as expenses when computing EBIT.

Hull (2018) expands on the CSM research with an ITS tax policy by correcting the levered equity growth rate (g_L) equation given by Hull (2010) and providing nongrowth and growth constraints. Since the CSM assumes growth through earnings that are retained from internal operations, the growth constraint is also called the retained earnings (RE) constraint. For CCs, the CSM growth equation for G_L with tax rate changes is

G_{L (growth)} = (1 − α_Ir_D/r_Lg)D + (1 − α₂r_Ug/r_Lg)E_U

(2)

where

α_I, α₂, r_D, and D are the same as defined when describing Equation (1);

r_Lg and r_Ug are the growth-adjusted cost of levered equity (defined as r_L minus the levered equity growth rate, g_L) and the growth-adjusted cost of unlevered equity (defined as r_U minus the unlevered equity growth rate, g_U); and,

E_{U (growth)} = (1 − T_E1)(1 − T_C1)C/r_Ug where C = (1 − PBR_BT)(CF_BT) with PBR_BT = RE/CF_BT > 0 since RE > 0 as RE is used for growth.

Equation (2) uses two growth rates first given by Hull (2010). They are an unlevered growth rate (g_U) defined as g_U = r_U(1 − T_C1)RE/C and a levered equity growth rate (g_L). The latter was modified by Hull (2018) who argued that g_L = r_L(1 − T_C2)RE/[C + G − (1 − T_C2)I] for levered CCs where G is the perpetual before-tax cash flow arising from G_L with G = r_LgG_L/(1 − T_E2)(1 − T_C2). While g_U is determined by the plowback-payout decision through RE, g_L is shaped by both the plowback-payout decision through RE and the debt-equity decision through I. It follows that Equation (2) ties together the growth (and thus plowback-payout choice) decision and the leverage (debt-equity) decision through g_L. For a CC growth situation, we substitute r_L for r_Lg in the nongrowth E_L to get growth E_L = (1 − T_E2)(1 − T_C2)(C − I)/r_Lg.

As shown by Hull (2019) for a tax policy where RTS replaces ITS, Equation (2) maintains the same expression but definitions are modified. Besides the modifications given above for (1) caused by switching from an ITS to an RTS where there were changes in the definitions for α₁ and D, Hull now modifies the growth rate definitions used in (2). We have: g_U = r_U(RE)/C where (1 − T_C1) falls out of the numerator since there is no longer corporate taxation on RE; g_L = r_L(RE)/(C + G − I) where (1 − T_C2) falls out of both the numerator (no tax on RE) and also the denominator (I is no longer deductible).

Under the existing tax policy of an ITS, I is an expense that generates an interest tax shield (ITS) for CCs. In equation form, we have ITS = T_C2(I). With RE set by the CC’s plowback-payout choice, the denominator in the equation of g_L = r_L(1 − T_C2)RE/[C + G − (1 − T_C2)I] reveals that C + G > (1 − T_C2)I must hold if the amount of RE from the growth choice and the amount of debt from the leverage choice are both adequate. Based on the definition of g_L for CCs, Hull (2018) posits that the growth (or RE) constraint of C + G − (1 − T_C2)I ≥ RE must hold for a leverage choice to be possible. While we might say it is possible or feasible there are caveats. For example, the growth rate should not exceed rates that are unreasonable. Thus, even if growth constraint is not violated, the P choice may still not be feasible. For example, if the historical growth rate is 3.12%, then a growth rate of 5% might suggest the choice is not feasible (at least not for a typical or average firm). If the growth constraint does not hold, a firm no longer has sufficient RE to achieve growth with internal funds belonging to equity owners. Since RE is zero for nongrowth, the nongrowth constraint for CCs can be expressed as C + G − (1 − T_C2)I ≥ 0. For the CSM, there is a cost to use RE (CRE) under an ITS policy. CRE is simply the business taxes paid on RE. For CCs, we have: CRE = T_C2(RE).

For an RTS with growth, the RE constraint is C + G − I ≥ RE as (1 − T_C2) drops out since I is no longer deductible. Since RE = 0 for nongrowth, the RTS nongrowth constraint is C + G − I ≥ 0, which reduces to C + G ≥ I.

3.2. Identifying the Optimal P Choice for Growth and Nongrowth Situations

P refers to the proportion of unlevered equity (E_U) retired with debt (D). Identifying the optimal P choice for nongrowth tests using Equation (1) is straightforward as the maximum firm value (max V_L) is the P choice that produces the largest firm value when conducting tests for all possible P choices. An unfeasible P choice for a nongrowth test can occur for two situations. First, the P choice violates the nongrowth constraint such as occurs when there are not enough cash flows to cover the interest payments. This violation occurs for high leverage choices, especially those past the optimal P choice. For all of our nongrowth tests, max V_L occurs before there is violation of the nongrowth constraint. Second, the P choice can give the largest V_L for a very high investment grade credit rating that is not attainable except for a very small percent of enterprises. For our tests, we find this situation occurs for NP tests with zero tax rates. The credit rating that occurs is Moody’s Aa2 accompanied by a low DV that is under 0.19. The V_L that occurs is virtually the same as what occurs for a rating of Moody’s A3, which is the OCR found for other NP tests. In fact, there is less than 0.7% difference in V_L between Aa2 and A3. Thus, we argue that the more common rating of A3 is OCR and not Aa2. This argument is consistent with Morningstar (2019) that finds the highest quality grade ratings are not only rare but have become even more scarce over time. For our growth tests, the V_L that occurs at A3 is always greater than that at Aa2 and so this V_L is the only real candidate for max V_L that identifies ODV and OCR.

As discussed by prior CSM research (Hull 2019, 2020b; Hull and Van Dalsem 2021), identifying max V_L, using Equation (2) for a growth situation, is not as simple as using Equation (1) for a nongrowth situation. Given the complexities, they argue (after exhaustive testing) that the nongrowth OCR should be used to identify the growth OCR. Using the nongrowth OCR when applying the growth equation, e.g., Equation (2), we change PBR_BT through trial and error until the historical growth rate of 3.12% is attained at this OCR. Since greater growth rates are expected under TCJA, we repeat our TCJA tests using growth rates of 3.90% and 4.50%. The growth rates of 3.12%, 3.90%, and 4.50% are the same rates used by Hull and Van Dalsem and similar to that used by the growth CSM research under TCJA. As presented by this research, the rate of 3.12% is consistent with long-run historical growth in GDP and the rate of 3.90% is the rate suggested by tax experts for a TCJA tax law environment. The rate of 4.50% is the maximum rate that tax experts estimate can occur given the drop in tax rates ushered in TCJA. Its use is considered a candidate for the best outcome scenario for a TCJA setting while 3.12% would be considered a worst outcome candidate. For our tests using data for 2020 given by Damodaran (2021), we find that OCR is Moody’s A3 for six nongrowth CC tests (four are TCJA tests and two are pre-TCJA tests) and all eight nongrowth NP tests (four TCJA tests and four pre-TCJA tests). For the two pre-TCJA tests for CCs using L and H tax rates, we find that OCR is Moody’s Baa2, which is the rating of lower quality (but nearest to) Moody’s A3. Moody’s A3 and Baa2 are similar as both are medium grade ratings except Baa2 is a notch below in quality. These two rating are the two most common ratings assigned by Moody’s.

3.3. P Choices, Costs of Borrowing, and Betas

Table 3. Costs of Borrowing.

P Choice	T C2	ICR	Moody’s Rating	Credit Spread	rD	rL	βD	βL
0.09222051	0.0582	16.000	Aaa	0.69%	5.64%	8.44%	0.1426	0.7211
0.19166453	0.0565	7.500	Aa2	0.85%	5.80%	8.60%	0.1756	0.7541
0.23123954	0.0548	6.000	A1	1.07%	6.02%	8.82%	0.2211	0.7996
0.27998121	0.0531	4.875	A2	1.18%	6.13%	8.93%	0.2438	0.8223
0.36815152	0.0515	3.625	A3	1.33%	6.28%	9.08%	0.2748	0.8533
0.45834713	0.0500	2.750	Baa2	1.71%	6.66%	9.46%	0.3533	0.9318
0.48762513	0.0485	2.375	Ba1	2.31%	7.26%	10.06%	0.4773	1.0558
0.51330250	0.0470	2.125	Ba2	2.77%	7.72%	10.52%	0.5723	1.1508
0.49974477	0.0456	1.875	B1	4.05%	9.00%	11.80%	0.8368	1.4153
0.52977557	0.0442	1.625	B2	4.86%	9.81%	12.61%	1.0041	1.5826
0.56478929	0.0429	1.375	B3	5.94%	10.89%	13.69%	1.2273	1.8058
0.57334105	0.0416	1.025	Caa	9.46%	14.41%	17.21%	1.9545	2.5331
0.78389816	0.0404	0.725	Ca2	9.97%	14.92%	17.72%	2.0599	2.6384
1.10736026	0.0392	0.425	C2	13.09%	18.04%	20.84%	2.7045	3.2831
1.89828374	0.0380	0.200	D2	17.44%	22.39%	25.19%	3.6033	4.1818

Table 3 reports the outcomes from computing P choices, costs of borrowing, and betas given interest coverage ratios (ICRs) matched to both Moody’s ratings and also credit spreads for the year 2020 as provided by Damodaran (2021). These outcomes use high (H) tax rates for nonprofit (NPs) prior to Tax Cuts and Jobs Act (TCJA) under an ITS tax policy with growth of 3.12%. These NP tax rates used in the table correspond to unlevered, levered, and targeted rates found in top half of Panel B in Table 2 for pre-TCJA tax rate environment with an ITS tax policy. ICR ranges are given by Damodaran for large, small, and financial service firms. We use Damodaran’s large ICRs as we test large NPs and large CCs for which large ICRs are a better fit. The fifteen ICRs given in Table 3 are computed as the average of the fifteen large ICR ranges supplied by Damodaran with the first and last ranges treated differently as described by Hull (2020a). Using Damodaran’s definition for ICR, Hull computes interest (I) as I = (1 − T_C2)CF_BT/ICR where T_C2 is the effective (average) corporate tax rate on taxable business income and CF_BT is the before-tax cash flows that, for our tests, equal USD 1,000,000 (with CF_BT analogous to EBIT). The fifteen T_C2 values and ICR values are given in the second and third columns, respectively. From the fifteen ICR values, we compute fifteen I values. From these fifteen I values, we use their corresponding levered personal debt rate (T_D2) and cost of debt (r_D) values to calculate fifteen debt (D) values where D = (1 − T_D2)I/r_D. Since we assume all large NPs issue tax exempt debt, we have D = I/r_D as all T_D2 values are zero. We next compute the fifteen P choices where P is the proportion of unlevered equity (E_U) retired by D. In equation form, we have P = D/E_U. This table reports P choices for NPs in the first column along with corresponding Moody’s ratings and credit spreads in the third and fourth columns. The P choices provided in this table for NPs are not the same as those used for CCs. This is because P choices can differ for a number of reasons including differences in tax rates. To get fifteen r_D values (reported in the r_D column) we add each credit spread (CS) to the risk-free rate (r_F) of 4.95% so that we have: r_D = r_F + CS. The value of 4.95% is given by Damodaran (2021) as the average 3-month T-bill rate for 1928–2020. If we factor in the recent downward trend (at the time this research began), an r_F of 4.95% is also consistent with the 30-year government bonds as given by Federal Reserve Economic Data (2020) for the past fifteen years. To get a cost of levered equity (r_L) matched to each r_D, we add an equity risk premium over a corporate bond portfolio (EPB) to each r_D. Following Damodaran (2021), we use EPB = 2.80%, which is given as the difference between the geometric average stock return for 1928–2020 and the average corporate bond return for 1928–2020. By adding 2.80% to our fifteen increasing r_D values, we get fifteen increasing r_L values. In equation form, we have: r_L = r_D + EPB. From each r_D and r_L we compute respective debt beta (β_D) and levered equity beta (β_L) using the CAPM where β_D = (r_D − r_F)/(r_M − r_F) and β_L = (r_L − r_F)/(r_M − r_F). Optimal values in this table are given in the bold print row where the optimal P choice is 0.36815152.

contains the procedure to get the costs of borrowing for each P choice. Table 3 reports P choices using H tax rates for NPs for a pre-TCJA tax rate environment with an ITS and growth of 3.12%. Prior CSM research computes (in a fashion similar to Table 3) P choices using tax rates for CCs (Hull 2020a), PTs (Hull 2020b), and NPs with zero tax rates (Hull and Van Dalsem 2021). This table’s procedure for NPs differs from that of Hull and Van Dalsem on two fronts. First, Table 3 uses NP tax rates that are not zero. Second, this table also uses more recent data on credit spreads to determine costs of borrowing matched to P choices. As shown later, the credit spreads we use are consistent with the average of credit spreads for a recent eight-year period. As noted by prior CSM research, using spreads to get costs of borrowing is consistent with researchers (Graham and Harvey 2001; Kisgen 2006) who suggest credit ratings influence a firm’s ODV. By using credit spreads, the CSM produces a sequence of increasing borrowing costs that can be used to compute increasing debt-to-firm value ratios (DVs). This sequence is needed to identify which DV corresponds to the maximum firm value as this DV is the ODV.

To estimate the costs of borrowing provided in Table 3, we begin by retrieving fifteen credit spreads corresponding to fifteen credit ratings given by Damodaran (2021) for 2020. Damodaran supplies a range of interest coverage ratios (ICRs) for each spread and its corresponding rating for three groups of firms: large, small, and financial service. For this study, the best fit is the large group given that we are examining the largest NPs and CCs. We follow Hull (2020a) in choosing a feasible point within each range of the large group when identifying an ICR to represent its range. We can point out that the 2020 ICRs that we use are the same as the 2019 ICRs provided by Damodaran with his 2019 spreads and ratings. To our knowledge, Damodaran first began reporting ICRs for the year 2018. His data are updated each January and includes data for the prior year.

While ICR is conventionally defined as EBIT/I, Damodaran defines ICR as (1 − T_BL)EBIT/I where T_BL is the effective tax rate on income at the business level. Given Damodaran’s ICRs and noting EBIT is analogous to CF_BT, we use the following equation to get the interest (I) paid on debt (D) per USD 1,000,000 in CF_BT: I = (1 − T_BL)CF_BT/ICR. For our tests, we compute I using the CSM’s T_C2 for T_BL where T_C2 is the business tax rate for CCs. The assignment of T_C2 values is described in Section 2.3. Given Damodaran’s fifteen ICR values, we compute fifteen I values. Given the fifteen I values and their corresponding T_D2 values (that are all zero for our tests) and cost of debt (r_D) values, we calculate fifteen D values where D = (1 − T_D2)I/r_D. We then compute fifteen P choices using P = D/E_U. Table 3 reports these P choices in the first column along with their corresponding T_C2, ICRs, Moody’s ratings and credit spreads in the next four columns.

We add each of the fifteen credit spread to a risk-free rate (r_F) of 4.95%, as provided by Damodaran (2021), to get fifteen values for r_D. To illustrate, for the Moody’s credit rating of A3 where the credit spread (CS) is 1.33%, we have: r_D = r_F + CS = 4.95% + 1.33% = 6.28%. This value is reported in the fifth row of the r_D column in Table 3. The fifth row is in bold print to indicate it is the optimal row as maximum firm value corresponds to this row. Using an equity risk premium of stocks over bonds (EPB) of 2.80%, as also provided by Damodaran, we compute costs of levered equity (r_L). We have r_L = r_D + EPB = 6.28% + 2.80% = 9.08%. This value of 9.08% is reported in the bold print row of the r_L column. Finally, we compute debt and equity betas using the CAPM with these values given in the last two columns. These computations reveal two required results. First, the first debt beta (β_D) of 0.1426 is greater than the risk-free beta of zero. Second, the first levered equity beta (β_L) of 0.7211 is greater than the unlevered beta (β_U) of 0.7 (given later). The β_L at the OCR of Moody’s A3 is 0.8533 and thus below the market beta (β_M) of 1. However, a β_M of 1 is also associated with firms of all sizes and our sample contains the largest enterprises that are less risky than smaller enterprises. While not shown in Table 3 (which is an NP table), the β_L for a large CC (for the corresponding test) is 0.9318 as its OCR of Moody’s Baa2. This beta value is closer to a β_M of 1 indicating that our large CCs capture what we would expect as large firms have less market risk than the average firm.

3.4. Credit Spreads over Time

Table 4. Credit Spreads: 2013 through 2020.

Credit Ratings Moody’s/S&P	Credit Spread Statistics by Years/Period (Means for the Eight Years in the Last Column)
	2013	2014	2015	2016	2017	2018	2019	2020	2013–2020
Aaa/AAA	0.4000%	0.4000%	0.7500%	0.6000%	0.5400%	0.7500%	0.6300%	0.6900%	0.5950%
Aa2/AA	0.7000%	0.7000%	1.0000%	0.8000%	0.7200%	1.0000%	0.7800%	0.8500%	0.8188%
A1/A+	0.8500%	0.9000%	1.1000%	1.0000%	0.9000%	1.2500%	0.9750%	1.0700%	1.0056%
A2/A	1.0000%	1.0000%	1.2500%	1.1000%	0.9900%	1.3750%	1.0764%	1.1800%	1.1214%
A3/A−	1.3000%	1.2000%	1.7500%	1.2500%	1.1250%	1.5625%	1.2168%	1.3300%	1.3418%
Baa2/BBB	2.0000%	1.7500%	2.2500%	1.6000%	1.2700%	2.0000%	1.5600%	1.7100%	1.7675%
Ba1/BB+	3.0000%	2.7500%	3.2500%	2.5000%	1.9844%	3.0000%	2.0000%	2.3100%	2.5993%
Ba2/BB	4.0000%	3.2500%	4.2500%	3.0000%	2.3813%	3.6000%	2.4000%	2.7700%	3.2064%
B1/B+	5.5000%	4.0000%	5.5000%	3.7500%	2.9766%	4.5000%	3.5100%	4.0500%	4.2233%
B2/B	6.5000%	5.0000%	6.5000%	4.5000%	3.5719%	5.4000%	4.2120%	4.8600%	5.0680%
B3/B−	7.2500%	6.0000%	7.5000%	5.5000%	4.3656%	6.6000%	5.1480%	5.9400%	6.0380%
Caa/CCC	8.7500%	7.0000%	9.0000%	6.5000%	8.6369%	9.0000%	8.2000%	9.4600%	8.3184%
Ca2/CC	9.5000%	8.0000%	12.0000%	8.0000%	10.6300%	11.0769%	8.6424%	9.9700%	9.7274%
C2/C	10.5000%	10.0000%	16.0000%	10.5000%	13.9519%	14.5385%	11.3412%	13.0900%	12.4902%
D2/D	12.0000%	12.0000%	20.0000%	14.0000%	18.6025%	19.3846%	15.1164%	17.4400%	16.0679%
Mean	4.8833%	4.2633%	6.1400%	4.3067%	4.8431%	5.6692%	4.4539%	5.1147%	4.9593%
StDev	3.9775%	3.6493%	5.8879%	3.9770%	5.5564%	5.5750%	4.4309%	5.1321%	4.7383%

Table 4 reports statistics for credit spreads for years 2013 through 2020 and for the eight-year period of 2013–2020. This table shows the similarity between the 2020 credit spreads we use in this paper’s tests and the averages for the credit spreads for 2013–2020. Data are supplied by Damodaran at https://pages.stern.nyu.edu/~adamodar/New_Home_Page/data.html. Accessed 15 January 2021.

reports the fifteen credit spreads given by Damodaran (2021) for the years from 2013 through 2020 and for the average of these years in the last or “2013–2020” column. The last two rows of Table 4 supplies means and standard deviations for the fifteen credit spreads and their averages in the last column. The “2020” column reveal that the mean and standard deviation for the fifteen credit spreads for the year 2020 are 5.1147% and 5.123%, respectively. Among all years, the year 2020 most resembles the mean of 4.9593% and the standard deviation of 4.7383% for the eight-year period. These latter two values are found in the “2013–2020” column in the last two rows.

While not shown in Table 4, if we take the difference in mean and standard deviation for all eight individual years from mean and standard deviation for the eight-year period, we get an average of 0.5115% for the eight mean differences and an average of 0.7646% for the eight standard deviation differences. In contrast, the respective differences are only 0.1554% and 0.3938% for the mean and standard deviation for year 2020 compared with the mean and standard deviation for the eight-year average. In conclusion, the year 2020 that provides our credit spread data shows strong resemblance to average credit spreads for the period from 2013 to 2020. Thus, were we to test other years, we would expect deviations for individual years but, on average, these results can reasonably be expected to resemble those found in this paper.

4. Results Using High (H) Tax Rates under TCJA with Historical Growth

Section 4 describes introductory variables used in the CSM followed by illustrations that contain key outcomes from nonprofit (NP) and C corporations (CC) tests. For these illustrations, we use a historical growth rate of 3.12% and high (H) tax rates under a pre-TCJA tax law environment with an ITS tax policy. Section 5 will incorporate low (L) tax rates, TCJA tax rates, an RTS tax policy, and two growth rates predicted under TCJA.

In addition to OCR, ten other outcomes are highlighted including the seven major outcomes reported by the prior CSM research that includes CCs (Hull and Price 2015; Hull 2020a, 2020b). These seven outcomes are: the debt choice outcomes of P and DV; the valuation outcomes of E_U and max V_L; and, leverage gain outcomes of max G_L, max %∆E_U, and NB. In Section 5, we will add to these seven outcomes by including the three growth-related outcomes from Hull and Van Dalsem (2021). These outcomes are PBR_BT, g_U, and DGN (max V_L with growth minus max V_L with nongrowth). Tables 8 and 9 include these ten outcomes and OCR that is added as a third debt choice outcome.

4.1. Variables and Computations

Table 5. Introductory Variables and Computations.

Panel A. Alpha Computations for High (H) Tax Rates Pre-TCJA

Large Nonprofit (NP) Alpha Computations:

For an unlevered situation for the high (H) tax rate scenario for a pre-TCJA tax rate environment and an ITS tax policy, the unlevered personal equity tax rate (TE1) is 0.05, the unlevered corporate tax rate (TC1) = 0.06, and the unlevered personal debt tax rate (TD1) = 0. The latter only exists hypothetically (since unlevered means no debt) but is assigned a beginning value to achieve an effective levered personal tax rate on debt (TD2) at the optimal P choice. TD1 and TD2 are zero because we assume large NPs have enough clout to avoid issuing taxable debt, which is to say all debt they issue can be exempt from personal taxes. Following prior CSM research originating in Hull (2014) and using a 0.03 change in tax rates between P choices, the levered personal equity tax rate (TE2) is less than TE1 since taxes paid by equity owners decrease by 0.03 with each increasing P choice. Similarly, the levered corporate tax rate (TC2) is less than TC1 since corporate taxes decrease by 0.03 with each increasing P choice. If TD1 was not assumed to be zero, TD2 would be greater than TD1 since TD2 increases by 0.03 with each increasing P choice.     For the first debt-for-equity choice using TD1 = 0, we have: TD2 = TD1(1 + ΔTD1)1 = 0(1 − 0.03)1 = 0. Using TE1 = 0.05, we have: TE2 = TE1(1 − ΔTE1)1 = 0.05(1 − 0.03)1 = 0.0485. Using TC1 = 0.06, we have: TC2 = TC2(1 − ΔTC2)1 = 0.06(1 − 0.03)1 = 0.0582. Computing the alphas to ten digits (so later computations can minimize rounding off errors), we have:     α1 = (1 − TE2)(1 − TC2)/(1 − TD2) = (1 − 0.0485)(1 − 0.0582)/(1 − 0) = 0.8961227000.     α2 = (1 − TE2)(1 − TC2)/(1 − TE1)(1 − TC1) = (1 − 0.0485)(1 − 0.0582)/(1 − 0.05)(1 − 0.06) = 1.0034968645.     For the fifth (and optimal) debt-for-equity choice using TD2 = TD1(1 + 0.03)5 = 0(0.8587340257) = 0, TE2 = TE1(1 − 0.03)5 = 0.05(0.8587340257) = 0.0429367013, and TC2 = TC1(1 − 0.03)5 = 0.06(0.8587340257) = 0.0515240415, we have:     α1 = (1 − TE2)(1 − TC2)/(1 − TD2) = (1 − 0.0429367013)(1 − 0.0515240415)/(1 − 0) = 0.9077515296.     α2 = (1 − TE2)(1 − TC2)/(1 − TE1)(1 − TC1) = (1 − 0.0429367013)(1 − 0.0515240415)/(1 − 0.05)(1 − 0.06) = 1.0165190700.

Large C Corporation (CC) Alpha Computations:

For an unlevered situation for the high (H) tax rate scenario for a pre-TCJA tax rate environment and an ITS tax policy, the unlevered personal equity tax rate (TE1) is 0.165, the unlevered corporate tax rate (TC1) = 0.35, and the beginning personal tax rate on debt income is TD1 = 0.19.     For the first debt-for-equity choice using TD1 = 0.19, we have: TD2 = TD1(1 + ΔTD1)1 = 0.19(1 − 0.03)1 = 0.1957. Using TE1 = 0.165, we have: TE2 = TE1(1 + ΔTE1)1 = 0.165(1 − 0.03)1 = 0.16005. Using TC1 = 0.35, we have: TC2 = TC2(1 − ΔTC2)1 = 0.35(1 − 0.03)1 = 0.3395, we have (to ten digits so later computations can minimize rounding off errors):     α1 = (1 − TE2)(1 − TC2)/(1 − TD2) = (1 − 0.16005)(1 − 0.3395)/(1 − 0.1957) = 0.6897761718.     α2 = (1 − TE2)(1 − TC2)/(1 − TE1)(1 − TC1) = (1 − 0.16005)(1 − 0.3395)/(1 − 0.165)(1 − 0.35) = 1.0221777522.     For the sixth (and optimal) debt-for-equity choice using TD2 = TD1(1 + 0.03)6 = 0.19(0.8329720049) = 0.2268699363, TE2 = TE1(1 − 0.03)6 = 0.165(0.8329720049) = 0.1374403808, and TC2 = TC1(1 − 0.03)6 = 0.35(0.8329720049) = 0.2915402017, we have:     α1 = (1 − TE2)(1 − TC2)/(1 − TD2) = (1 − 0.1374403808)(1 − 0.2915402017)/(1 − 0.1582646809) = 0.7904088103.     α2 = (1 − TE2)(1 − TC2)/(1 − TE1)(1-TC1) = (1 − 0.1374403808)(1 − 0.2915402017)/(1 − 0.165)(1 − 0.35) = 1.1259121397.

Panel B. Unlevered Firm Value (EU) Computations

NP example using CC definitions given in Section 3.1:   PBRBT = 0.2144; CFBT = USD 1,000,000; RE = PBRBT(CFBT) = 0.2144(USD 1,000,000) = USD 214,400.     CRE = TC2(RE) = 0.0515240415(USD 214,400) = USD 11,046.75.     %CRE per USD 1,000,000 of CFBT = USD 11,046.75/USD 1,000,000 = 0.01104675 or 1.104675% or about 1.10%.     C = (1 − PBRBT)(CFBT) = (1 − 0.2144)(USD 1,000,000) = USD 785,600.     GU = rU(1 − TC1)RE/C = 0.08338(1 − 0.06)USD 214,400/USD 785,600 = 0.021390112 or 2.1390112%.     rUg = rU − gU = 0.08338 − 0.0213901116 = 0.0619898884.     EU = (1 − PBRBT)(1 − TE1)(1 − TC1)CFBT/rUg = (1 − 0.2144)(1 − 0.05)(1 − 0.06)USD 1,000,000/0.0619898884 = USD 11,317,020.

CC example using CC definitions given in Section 3.1:   PBRBT = 0.2933; CFBT = USD 1,000,000; RE = PBRBT(CFBT) = 0.2933(USD 1,000,000) = USD 293,300.     CRE = TC2(RE) = 0.2915402017(USD 293,300) = USD 85,508.74.     %CRE per USD 1,000,000 of CFBT = USD 85,508.74/USD 1,000,000 = 0.08550874 or 8.55087% or about 8.55%.     C = (1 − PBRBT)(CFBT) = (1 − 0.2933)(USD 1,000,000) = USD 706,700.     GU = rU(1 − TE1)RE/C = 0.08338(1 − 0.165)USD 293,300/USD 706,700 = 0.0224932505 or 2.249325046%.     rUg = rU − gU = 0.065 − 0.0224932505 = 0.0608867495.     EU = (1 − PBRBT)(1 − TE1)CFBT/rUg = (1 − 0.2933)(1 − 0.165)USD 1,000,000/0.0608867495 = USD 6,299,588.

Table 5 has two panels. Panel A provides NP and CC examples for computing the two alpha coefficients (α₁ and α₂) presented in Section 3.1. These coefficients capture the impact of tax rates in G_L equations. This panel provides sample computations for α₁ and α₂ for the two ownership categories of nonprofits (NPs) and C corps (CCs). For the illustration in this table, we use the high (H) tax rates under pre-TCJA in the top half of Panel B in Table 2. An ITS tax policy holds for computations. The values for α₁ and α₂ rise for increasing P choices as long as at least one tax rate is positive. For our tests, tax rates change by 0.03 for each subsequent P in the directions discussed by Hull (2014) as described in Section 2.3. Panel B provides NP and CC examples for computing the unlevered firm value (E_U). E_U is important since each debt choice retires a fraction of E_U. Consistent with the data from Damodaran (2021), we use 0.7 for the unlevered equity beta (β_U). Additionally, consistent with the geometric average return from 1928 through 2021 as given by Damodaran, we use 9.79% for the market return (r_M). Given these values and r_F = 4.95% from Table 3, the unlevered equity rate of return (r_U) given by the CAPM is r_U = r_F + β_U(r_M − r_F) = 4.95% + 0.7000(9.79% − 4.95%) = 8.338%. When applying the CSM with growth, the before-tax plowback ratio (PBR_BT) is set by trial and error until g_L = 3.12% is achieved at the optimal credit rating (OCR) where the latter is determined by the nongrowth test as described in Section 3.2.

presents introductory variables and performs preliminary computations needed to begin the process that uses CSM equations to investigate optimal financing for NPs and CCs (where the use of NPs and CCs refer to large NPs and CCs even if not designated as such). Panel A focuses on the computations for the CSM’s two alpha tax rate variables, α₁ and α₂, using (i) the H tax rates in a pre-TCJA tax law environment (as given in top half of Panel B of Table 2) and under an ITS tax policy and (ii) the historical growth rate of 3.12% discussed in Section 3.2. As argued by Hull (2014), α₁ and α₂ can exercise a key valuation function in the first and second components, respectively, of CSM G_L equations. However, for NPs with L tax rates that are all zero, α₁ and α₂ values do not exercise an influence because α₁ = 1 and α₂ = 1 for all P choices where P refers to the proportion of unlevered equity (E_U) retired with debt (D).

For an alpha variable to change with leverage, at least one tax rate has to be greater than zero. As computed in Panel A (and ignoring the P = 0 choice), the first P choice for NPs gives (rounding off to four digits) α₁ = 0.8961 and α₂ = 1.0035 while the fifth P choice gives α₁ = 0.9078 and α₂ = 1.0165. The fifth P choice is also the optimal choice and coincides with optimal outcomes including the optimal credit rating (OCR), which is Moody’s rating of A3. For CCs, the alpha values in Panel A are (rounding off to four digits): α₁ = 0.6900 and α₂ = 1.0220 for the first P choice and α₁ = 0.7904 and α₂ = 1.1259 for the sixth P choice. The latter P choice is also the optimal choice and coincides with optimal outcomes including the optimal credit rating (OCR), which is Moody’s rating of Baa2. When comparing alphas, we find that CCs have a greater variation in its values indicating the potential for a greater valuation impact when computing V_L.

In Panel B, we offer NP and CC examples when computing the following six variables where each ownership form has USD 1,000,000 in CF_BT. These six variables are: retained earnings (RE); cost to use RE (CRE); %CRE is CRE per USD 1,000,000 of CF_BT (expressed in percentage terms); before-tax payout I; unlevered equity growth rate (g_U); and, E_U. From the E_U values in this panel, we can see just how much advantage an NP has over a CC due to not paying federal taxes. As computed in Panel B, the advantage is USD 5,017,432 as E_U is USD 11,317,020 for an NP but only USD 6,299,588 for a CC. From this panel, we can also discover that CRE is USD 11,046.75 for an NP. This compares to USD 85,508.74 for a CC. CRE per USD 1,000,000 of CF_BT is about 1.10% for an NP and about 8.55% for a CC. This difference casts light on the before-tax plowback ratio (PBR_BT) outcomes in Panel B where NPs achieve 3.12% growth with a PBR_BT of 0.2144 at its OCR of A3 compared to 0.2933 for CCs at its OCR of Baa2. We observe that as the cost of using RE increases, the value for PBR_BT also increases.

From the results in Table 5, we conclude that, ceteris paribus, lower costs (in the form of lower tax rates) allow OCR to be attained with a lower PBR_BT and thus less RE is needed to achieve the same growth rate of 3.12%. If less RE is needed with lower tax rates, then growth using RE becomes more affordable as the cost of using RE falls. This is seen in the much lower CRE value for NPs compared to CCs. This conclusion is consistent with the empirical research (as summarized by McBride 2012) and tax experts at the Tax Foundation (2018) and Tax Policy Center (2020) who argue that higher tax rates inhibit growth. With higher taxes, more before-tax RE is needed to grow and the CRE per USD 1,000,000 of CF_BT becomes too costly. This explains our later results where we find that using a high business tax rate yields a nongrowth firm value greater than its growth value. The question then becomes: “How can we change tax policy so that firms can grow with less impediments to growth while also providing taxes to fund needed government expenditures?” As we will shown in this paper, tax policy governing retained earnings and interest expenses can make a difference in firm value. If so, there are widespread implications in other areas such as taxpayer wealth and federal tax revenue.

4.2. Illustrations of Large NP and Large CC Outcomes

Table 6. Illustration for Large Nonprofits.

Panel A. Key Outcomes for P Choices (Optimal Outcomes in Bold Print)
	P Choice = Proportion of Unlevered Firm Value (EU) Retired by Debt (D)
Outcomes	0.0000	0.0922	0.1917	0.2312	0.2800	0.3682	0.4583	0.4876	0.5133
Moody’s Rating	n.a.	Aaa	Aa2	A1	A2	A3	Baa2	Ba1	Ba2
Debt (D)	0.000	1.044	2.169	2.617	3.169	4.166	5.187	5.518	5.809
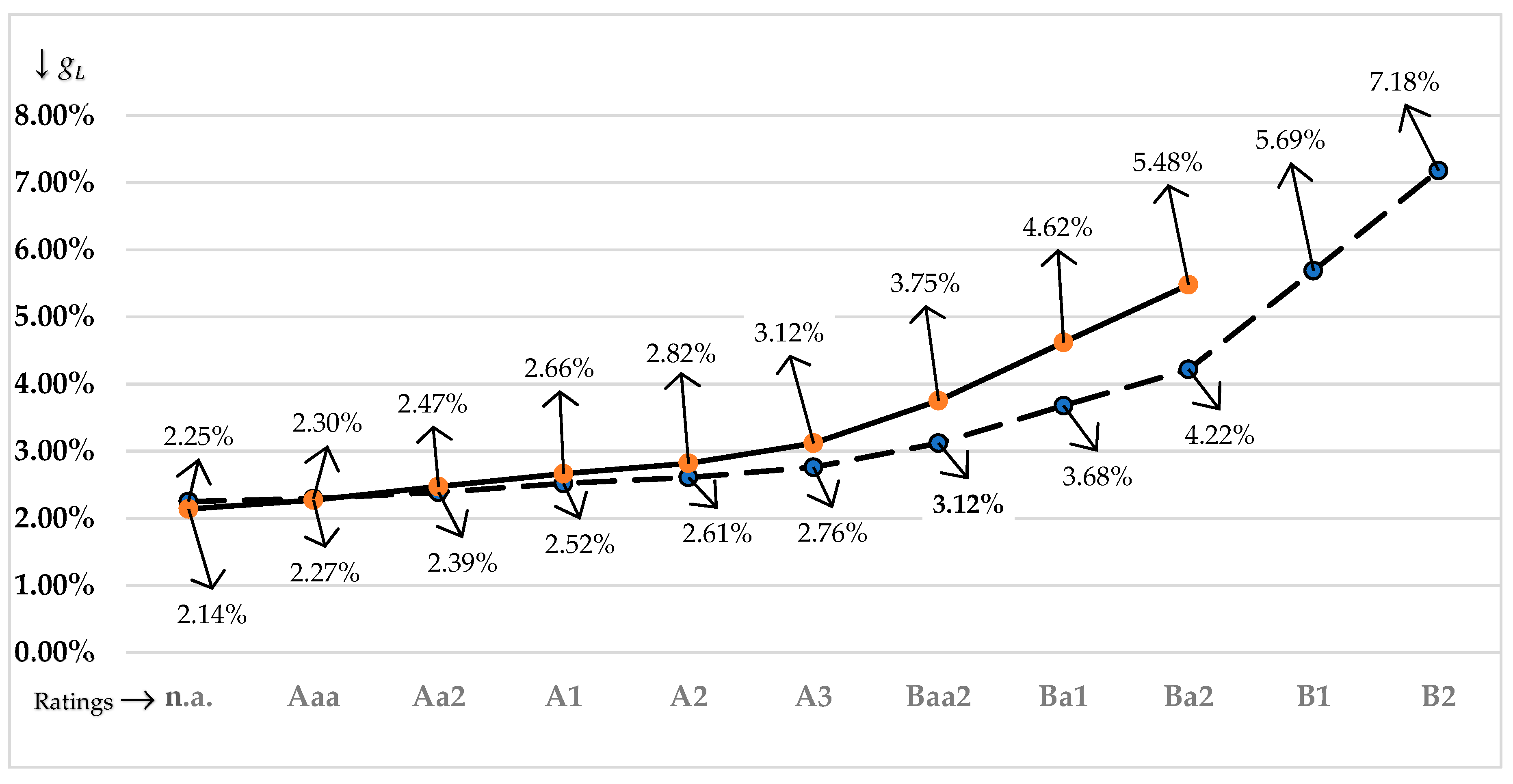
Equity growth rate: gL	2.14%	2.27%	2.47%	2.66%	2.82%	3.12%	3.75%	4.62%	5.48%
Growth adjusted: rLg	6.20%	6.17%	6.13%	6.16%	6.11%	5.96%	5.71%	5.44%	5.04%
1st component of GL	0.000	0.188	0.323	0.308	0.292	0.181	−0.325	−1.209	−2.339
2nd component of GL	0.000	0.098	0.213	0.195	0.319	0.648	1.217	1.874	2.956
Gain to leverage: GL	0.000	0.286	0.535	0.503	0.610	0.829	0.892	0.665	0.617
Firm value: VL	11.317	11.603	11.852	11.820	11.927	12.146	12.209	11.982	11.934
Equity value: EL	11.317	10.560	9.683	9.203	8.759	7.980	7.022	6.464	6.125
%∆EU	0.00%	2.53%	4.73%	4.45%	5.39%	7.33%	7.89%	5.88%	5.46%
NB	0.0%	27.4%	24.7%	19.2%	19.3%	19.9%	17.2%	12.1%	10.6%
DV	0.0000	0.0899	0.1830	0.2214	0.2657	0.3430	0.4248	0.4605	0.4868
Panel B. Computations for Optimal Outcomes at P = 0.36815152
D = P(EU) = 0.36815152(USD 11,317,020) = USD 4,166,378 or D = −(1 − TD2)I/rD = −(1 − 0)USD 261,648.54/0.0628 = USD 4,166,378.   gL = r − (1 − TC2)RE/[C − G − −(1 − TC2)I] = 0.090 − (1 − 0.0515240415)USD 214,400/[USD 785,600 + USD 54,438 − 37 − −(1 − 0.0515240415)USD 261,648.54] = 0.0311967879 or about 3.12%. Thus, rLg = −rL − gL = 0.0–08 − 0.0311967879 = 0.0596032121 or about 5.96%.   Max GL = −(1 − αIrD/rLg)D + –(1 − α2rUg/rLg)EU = −[1 − 0.9077515296(0.0628)/0.0596032121]USD 4,166,378 + –[1 − 1.01651907(0.0619898884)/0.0596032121]USD 11,317,020 = USD 181,494 + USD 647,597 = USD 829,091.   Max VL = EU + Max GL = USD 11,317,020 + USD 829,091 = USD 12,146,111.   EL = −VL − D = USD 12,146,111 − USD 4,166,378 = USD 7,979,733.   Max %∆EU = Max GL/EU = USD 829,091/USD 11,317,020 = 0.0733 or 7.33%.   NB = Max GL/D = USD 829,091/USD 4,166,378 = 0.1990 or 19.90%.   ODV = D/Max VL = USD 4,166,378/USD 12,146,111 = 0.3430.

Table 6 has two panels. Panel A uses Equation (2) to provide outcomes for the unlevered P choice and the eight feasible levered P choices for a nonprofit (NP) where P is the proportion of unlevered equity (E_U) retired by debt (D). This table uses 2020 data (including spreads) given by Damodaran (2021). Violations of the growth (RE) constraint, described in Section 3.1, do not hold for this table as the first violation does not occur until the ninth levered P choice with a Moody’s credit rating of B1. When using Equation (2), we follow Hull (2014) and allow positive tax rates to change as described in Section 2.3. This table uses high (H) tax rates for pre-TCJA tests under an ITS tax policy. (If we use low tax rates that are all zero, then tax rates could not change as P choices change). The pre-TCJA tax rates are given in the nonprofit column of the top half of Panel B in Table 2. Outcomes are based on before-tax cash flows (CF_BT) of USD 1,000,000 with a historical growth rate of 3.12% that is achieved when a Moody’s A3 is the optimal credit rating (OCR). OCR is determined by the nongrowth test described in Section 3.2. Dollar values for key outcomes in Panel A are in millions (USD M). Firm value (V_L) = E_U + gain to leverage (G_L). Levered equity (E_L) = −V_L − D. %∆E_U is G_L as a percent of E_U. Net benefit from leverage (NB) is G_L as a percent of D. DV is the debt-to-firm value ratio. The optimal DV is ODV and is identified from the maximum gain to leverage (max G_L) that coincides with the maximum firm value (max V_L) since max V_L = E_U + max G_L. Panel B provides computation for the optimal outcomes. The bold print column in Panel A indicates optimal outcomes. As seen in the “DV” or last row, these occur when ODV = 0.3430. Optimal outcomes are designated by the bold print column where the optimal P = 0.3682. To avoid rounding offer errors, we use values up to eleven decimal points in computations. For example, we use P = 0.36815152 instead of 0.3682. From the optimal bold print row in Table 3, we have ICR = 3.625, r_D = 6.28% and r_L = 9.08% when Moody’s rating is A3, which is the OCR. From Table 5, we have T_E1 = 0.05, T_E2 = 0.0429367013, T_D1 = 0; T_D2 = 0; T_C1 = 0.06; T_C2 = 0.0515240415, g_U = 0.021390112, r_U = 0.08338, CF_BT = USD 1,000,000, RE = USD 214,400, C = USD 785,600, E_U (or V_U) = USD 11,317,020 and the before-tax plowback ratio plowback ratio (PBR_BT) = 0.2144. For the optimal choice of P = 0.3682 where debt retires 0.3682 of E_U, and I = (1 − T_C2)CF_BT/ICR = (1 − 0.0515240415)USD 1,000,000/3.625 = USD 261,648.54, we have D = USD 4,166,378. G is determined by iterative process due to its interdependence with G_L and g_L. In equation form, we have: G = r_LgG_L/(1 − T_E2)(1 − T_C2) = 0.0596032121(USD 829,091)/(1 − 0.0429367013)(1 − 0.0515240415) = USD 54,438.37. Given G, we compute g_L as 0.0311967879. Using Equation (2) with α₁ = 0.9077515296, α₂ = 1.01651907, r_Ug = r_U − g_U = 0.08338 − 0.0213901116 = 0.0619898884, r_Lg = r_L − g_L = 0.0908 − 0.0311967879 = 0.0596032121, and above values for E_U, r_D, and D, we compute Max G_L.

uses Equation (2) to generate NP outcomes using a 3.12% growth rate at the OCR of A3 identified by the nongrowth test. Like the prior two tables, we use the high (H) tax rates for nonprofit (NPs) prior to Tax Cuts and Jobs Act (TCJA) as given in the top half of Panel B in Table 2. As before, we use credit spread data for 2020 from Damodaran (2021). In the columns of Panel A in Table 6, we provide outcomes for the unlevered P choice of 0 and the eight feasible levered P choices ranging from 0.0922 to 0.5133 where the growth constraiIt is not violated. However, since 3.12% is the historical average growth rate, choices beyond that rate are not deemed acceptable. If we set P choices past the OCR of A3 at a 3.12% growth rate, then G_L and V_L values would be smaller than given in Table 6 and below those found for A3 at 3.12% growth. The debt-to-firm value ratio (DV) that is optimal is ODV and it is identified from the maximum gain to leverage (max G_L) that coincides with the maximum firm value (max V_L) since max V_L = E_U + max G_L. The process to identify max V_L was described in Section 3.2. In Panel A, ODV, max V_L, max G_L and other optimal outcomes are in bold print column. Panel B provides computations for these optimal outcomes.

In Panel A of Table 6, the bold print column reveals that the optimal P is 0.3682, OCR is a Moody’s rating of A3 (identified by the nongrowth test in Section 3.2), and a levered growth rate of 3.12% under a pre-TCJA tax law environment with an ITS. As noted in Section 3.1, a rate of 3.12% is suggested by data for annual growth in U.S. real GDP for a recent seventy-year period as supplied by the US Bureau of Economic Analysis (2020). The last row of the bold print column discloses that ODV is 0.3430. The reported debt-to-firm value ratios (DVs) in Table 6 are slightly smaller than P choices because the denominator in DV computations consider the gain to leverage (G_L) that is positive. However, with larger DVs and lower quality credit ratings, G_L will decline reversing the trend where DV is smaller than P choices. Besides differences in the denominators (e.g., P = D/E_U while DV = D/V_L), the differences between P and DV in Table 6 can also be explained by the low sensitivity of DVs to situations like the change in tax rates and the value of certain variables (such as β_U). For example, there is less deviation between P and ODV if tax rates are not allowed to change by 0.03 for increasing P choices. Furthermore, P and ODVs converge for slightly lower β_U values. Finally, there no violations of the growth constraint in Table 6 for the P choices used as a violation does not occur until a Moody’s rating of B1 is reached (which is a notch in quality below Ba2).

Panel A reveals that the first component of G_L is strictly concave downward becoming negative when P = 0.4583, while the second component is positive and increasing for all feasible P choices up to P = 0.4876 except for the small downward bump that occurs at P = 0.2312. Together these two components (that represent G_L) are strictly concave downward except for the minor downward bump at P = 0.2312. The OCR of Moody’s A3, given by the nongrowth test, yields the optimal P choice of 0.3682 where we find that max G_L is USD 0.829M (M = million) and max V_L is USD 12.146M. The values for G_L and V_L at P = 0.4583 are USD 892M and USD 12.209M and thus slightly greater than max G_L of USD 0.829M and max V_L of USD 12.146M. However, a growth rate of 3.75% is needed to achieve G_L and V_L values of USD 892M and USD 12.209M and such a growth rate is not sustainable, on average, for a perpetuity situation given that the long-run historical growth rate is only 3.12%. Suppose a 3.75% growth rate is just as achievable as 3.12% at the OCR of A3. If so, a growth of 3.75% at A3 would have greater G_L and V_L values than USD 0.892M and USD 12.909M. For example, a V_L of USD 12.572M would occur at a rating of A3 with 3.75% growth and this is greater than USD 12.209M achieved at a rating of Baa2 when growth is 3.75%.

Panel B of Table 6 continues the NP computations began in Table 3 and Table 5. This panel (and its details below) shows how I, D, G, g_L, r_Lg, max G_L, max V_L, E_L, max %∆E_U, NB, and ODV are calculated for the optimal choice of P = 0.3682. For the latter five variables, we use the following definitions: V_L = G_L + E_U, which only holds when we begin with an unlevered situation and not like the levered situation used by Hull (2012) who derives incremental G_L equations that allow for a wealth transfer); E_L = V_L − D; percent change in E_U (%∆E_U) = G_L/E_U in percentage form; net benefit from leverage (NB) = G_L/D in percentage form; and, debt-to-firm value ratio (DV) = D/V_L. The max %∆E_U of 7.33% found in the bold print column agrees with the for-profit (FP) research (Graham 2000; Korteweg 2010; Van Binsbergen et al. 2010) that collectively suggests that leverage increases firm value between 4% and 10% at ODV. Thus, it appears that large NPs, like for-profits (FPs), can attain ODVs similar to those found for FPs. If we consider the results of our tests using both our H and L tax rates presented in Table 2, the NP values reported in this paper for max %∆E_U range from 3.123% to 9.081% with a mean of 6.129% and a median of 6.541%. Finally, as seen in the next to last row of Panel B, NB is reported as 19.90%. Thus, every dollar of debt adds 19.9 cents to E_U at the ODV of 0.3430.

Table 7. Illustration for Large C Corporations.

Panel A. Key Outcomes for P Choices (Optimal Outcomes in Bold Print)
	P Choice = Proportion of Unlevered Firm Value (EU) Retired by Debt (D)
Outcomes	0.0000	0.0935	0.1954	0.2370	0.2882	0.3803	0.4747	0.5060	0.5332
Moody’s Rating	n.a.	Aaa	Aa2	A1	A2	A3	Baa2	Ba1	Ba2
Debt (D)	0.000	0.589	1.231	1.493	1.816	2.396	2.991	3.188	3.359
Equity growth rate: gL	2.25%	2.30%	2.39%	2.52%	2.61%	2.76%	3.12%	3.68%	4.22%
Growth adjusted: rLg	6.09%	6.14%	6.21%	6.30%	6.32%	6.32%	6.34%	6.38%	6.30%
1st component of GL	0.000	0.216	0.415	0.452	0.496	0.563	0.507	0.246	−0.065
2nd component of GL	0.000	0.081	0.146	0.184	0.288	0.415	0.512	0.584	0.786
Gain to leverage: GL	0.000	0.297	0.562	0.636	0.784	0.977	1.020	0.830	0.721
Firm value: VL	6.300	6.597	6.861	6.936	7.084	7.277	7.319	7.130	7.021
Equity value: EL	6.300	6.008	5.630	5.443	5.268	4.881	4.329	3.942	3.662
%∆EU	0.00%	4.71%	8.92%	10.10%	12.45%	15.51%	16.19%	13.17%	11.45%
NB	0.0%	50.5%	45.6%	42.6%	43.2%	40.8%	34.10%	26.0%	21.5%
DV	0.0000	0.0892	0.1794	0.2153	0.2563	0.3292	0.4086	0.4471	0.4784
Panel B. Computations for Optimal Outcomes at P = 0.47473223
D = P(EU) = 0.47473223(USD 6,299,588) = USD 2,990,617 or D = −(1 − TD2)I/rD = −(1 − 0.2268699363)USD 257,621.74/0.0666 = USD 2,990,617. gL = r − (1 − TC2)RE/[C − G − −(1 − TC2)I] = 0.0946(1 − 0.2915402017)USD 293,300/[USD 706,700 + USD 105,790 − 59 − −(1 − 0.2915402017)USD 257,621.74] = 0.0312028632 or about 3.12%. Thus, rLg = −rL − gL = 0.0–46 − 0.0312028632 = 0.0633971368. Max GL = −(1 − αIrD/rLg)D +–(1 − α2rUg/rLg)EU = −[1 − 0.7904088103(0.0666)/0.0633971368]USD 2,990,617 + –[1 − 1.1259121397(0.0608867495)/0.0633971368]USD 6,299,588 = USD 507,386 + USD 512,336 = USD 1,019,722. Max VL = EU + Max GL = USD 6,299,588 + USD 1,019,722 = USD 7,319,310. EL = −VL − D = USD 7,319,310 − USD 2,990,617 = USD 4,328,693. Max %∆EU = Max GL/EU = USD 1,019,722/USD 6,299,588 = 0.161871 or about 16.19%. NB = Max GL/D = USD 1,019,722/USD 2,990,617 = 0.340974 or about 34.10%. ODV = D/Max VL = USD 2,990,617/USD 7,319,310 = 0.4086.

Table 7 has two panels. Panel A uses Equation (2) to provide outcomes for the unlevered P choice and ten levered P choices for a C corp (CC) where P is the proportion of unlevered equity (E_U) retired by debt (D). This table uses 2020 credit spreads from Damodaran (2021). Violations of the growth (RE) constraint, described in Section 3.1, do not hold for this table as the first violation does not occur until the eleventh levered P choice with a Moody’s credit rating of B3. When using Equation (2), we follow Hull (2014) and allow tax rates to be a function of debt causing α₁ and α₂ to increase as debt rises as described in Section 2.3. The pre-TCJA tax rates are given in the C corp column of the top half of Panel B in Table 2 for an ITS. Outcomes are based on before-tax cash flows (CF_BT) of USD 1,000,000 with a historical growth rate of 3.12% that is achieved when a Moody’s Baa2 is the optimal credit rating (OCR). OCR is determined by the nongrowth test described in Section 3.2. Dollar values for key outcomes in Panel A are in millions (USD M). Firm value (V_L) = E_U + gain to leverage (G_L). Levered equity (E_L) = V_L − D. %∆E_U is G_L as a percent of E_U. Net benefit from leverage (NB) is G_L as a percent of D. DV is the debt-to-firm value ratio. The optimal DV is ODV and is identified from the maximum gain to leverage (max G_L) that coincides with the maximum firm value (max V_L) since max V_L = E_U + max G_L. Optimal outcomes are designated by the bold print column where the optimal P = 0.47473223. Panel B computes outcomes at ODV as designated in the bold print column. To minimize rounding off errors, we use values up to eleven decimal points. From Table 3, we have ICR = 2.750, r_D = 6.6600% and r_L = 9.4600% when Moody’s rating is Baa2, which is the OCR for CCs. From Table 5, we have T_E1 = 0.165, T_E2 = 0.1374403808, T_D2 = 0.2268699363, g_U = 2.249325046%, r_U = 8.338%, CF_BT = USD 1,000,000, RE = USD 293,300, C = USD 706,700, E_U (or V_U) = USD 6,299,588 and the before-tax plowback ratio (PBR_BT) = 0.2933. For the optimal choice of P = 0.47473223 where debt retires 0.47473223 of E_U, and I = −(1 − T_C2)CF_BT/ICR = −(1 − 0.2915402017)USD 1,000,000/2.750 = USD 257,621.74, we compute D. Given G (as determined by iterative process due G’s interdependence with G_L and g_L) = r_LgG_L − (1 − T_E2) = 0.0633971368(USD 1,019,722.37) − (1 − 0.1374403808) = USD 105,790.59, we compute g_L. With α₁ = 0.7904088103, α₂ = 1.1259121397 and above values for E_U, r_D, and D, we compute Max G_L.

repeats Table 6 but is for a large CC instead of a large NP. The optimal P choice of 0.4747 in Table 7 for a large CC is greater than that of 0.3682 in Table 6 for a large NP. Once again, the column in Panel A with the optimal P choice is in bold print to indicate optimal values achieved when firm value is maximize. In the columns of Panel A in Table 7, we provide outcomes for the unlevered P choice of 0 and the nine levered P choices ranging from 0.0935 to 0.5501 where the growth constraint is not violated. This indicates there is enough RE to achieve the growth designated by the plowback-payout policy for ratings from Aaa to B2. Table 7 has an OCR of Baa2. This OCR was identified by the nongrowth test and is a notch below the OCR of A3 found in Table 6. Unlike Table 6, the G_L and V_L values for the rating just past OCR is not greater that the max V_L and max G_L values at the OCR of Baa2 even though the growth rate is greater. For example, the g_L of 3.68% at the lower rating of Ba1 yields G_L and V_L values below that attained with a g_L of 3.12% at the OCR of Baa2.

Panel A of Table 7 reveals that the first component of G_L is positive for the first seven levered P choices for a large CC. This is different from the results in Table 6 for an NP where only the first five levered P choices were positive. The second component for large CCs is increasing. This is similar to NPs where its second components was also increasing except for a downward bump at the P choice corresponding to Moody’s rating of A1. Similar to the generally increasing G_L for NPs in Panel A of Table 6, Panel A of Table 7 reveals that G_L is strictly increasing for CCs until we go past the OCR of Baa2. At OCR, the large CC’s max G_L is USD 1.020M and max V_L is USD 7.319M. The corresponding NP values in Table 7 for NPs were max G_L is USD 0.829M and max V_L is USD 12.146M. Thus, compared to large NPs, large CCs have greater max G_L values despite having much smaller max V_L values.

Panel B of Table 6 continues the computations began in Table 3 and Table 5 that are applicable to large CCs. Panel B reports that %∆E_U is 16.19% for a large CC and this is greater than 7.33% for a large NP while the ODV of 0.4086 for a large CC is greater than the ODV of 0.3430 for a large NP. In addition to the smaller max G_L value for an NP (compared to a CC), its smaller %∆E_U can be explained by the fact that it has a much greater E_U value than a CC. Like %∆E_U, the NB of 19.90% for an NP is less than 34.10% reported for a CC. The smaller NB for an NP can be explained by its larger E_U for which it takes more debt to retire greater amounts of E_U to achieve its OCR of A3. At ODV, Table 7 shows that that a CC issues only USD 2.991M in debt compared to USD 4.116M in debt for an NP in Table 6. More debt issued for NPs relative to their lower max G_L translates into a smaller NB so that NPs can be said to be relatively less efficient in its use of debt by getting less value per dollar of debt that is issued.

In conclusion, noticeable differences in Table 6 and Table 7 occur between large NPs and large CCs. First, for D and max V_L, NPs have much greater values. Second, for max G_L, %∆E_U, and NB, NPs have much smaller values. These dissimilarities can be explained in terms of differences in tax rates since we are comparing NPs and CCs with an identical risk class as captured by the same costs of capital.

4.3. Five Illustrative Figures Using TCJA Tax Rates and Growth of 3.12%

We now offer five illustrative figures that plot values for five outcomes against Moody’s credit ratings where these values are from Table 6 and Table 7. The five outcomes are P, G_L, V_L, %∆E_U, and g_L and each is plotted along the vertical axis with ratings along the horizontal axis where ratings are decreasing in quality (from the highest quality of Aaa to the lowest quality of B2). These outcomes include both NP values (in solid line trajectories) and CC values (in dashed line trajectories). These two trajectories enable us to contrast NP and CC outcomes. These figures only show plot points where the growth constraint is not violated. As first described in Section 4.2, the growth constraint sets in for NPs at a Moody’s credit rating of B1 (highly speculative rating), which is two notches in quality above that for CCs where the growth constraint occurs at B3 (also highly speculative rating and more speculative than B1). For these five figures, like Table 6 and Table 7, we use the high (H) tax rates given in the top half of Panel B in Table 2 for a pre-TCJA tax rate environment. The tax policy is an ITS. As before, any usage of NP and CC refers to a large NP and a large CC.

Figure 1 illustrates the extent that P choices increase as credit ratings decrease in quality where P is the proportion of unlevered equity (E_U) retired by debt (D) and is computed as D/E_U. The one exception where the P value does not increase is for the CC trajectory (dashed line) at the plot point for a B1 rating. The NP trajectory (solid line) undergoes slightly smaller increases in P values at Moody’s ratings decrease in quality. This trajectory reaches the Moody’s rating of Ba2 before the growth constraint sets in at B1. At this plot point of Ba2, P is 0.5133. The CC trajectory continues to a rating of B2 where P is 0.5501. Because the CC trajectory has more P choices, it reaches a higher height compared to NPs. Figure 1 reveals that, for any rating that might be targeted, large NPs will issue less debt compared to its unlevered equity value to achieve the same target rating. However, as can be seen in Table 6 and Table 7, NPs must issue much greater amounts of debt to achieve the same credit rating because they have much greater unlevered firm values.

Figure 2 plots the gain to leverage (G_L) along the vertical axis against credit ratings along the horizontal axis. For the CC trajectory (dashed line), G_L peaks at its OCR of Baa2 with a value of USD 1020M. All points past Baa2 have smaller G_L values as well as growth rates that are greater than 3.12% and so not deemed possible if outcomes are limited to the historically growth rate of 3.12%. For NP trajectory (solid line), G_L is USD 0.829M at the OCR of A3. However, G_L peaks at Baa2 with a value of USD 0.892M. However, this value of USD 0.892M is achieved with a growth rate of 3.75% and so is not deemed possible for a GDP environment where growth is limited to 3.12%. Furthermore, if 3.75% is attainable, then an NP would still target A3 at its OCR as G_L would be higher at USD 0.939M at this target with a growth rate of 3.75%. For a growth rate of 3.12% at Baa2, an NP would only achieve a G_L of USD 0.787M, which is below USD 0.829M achieved at A3 with 3.12% growth. Thus, an NP is better off with a 3.12% growth at A3. In brief, no matter what the growth rate, the NP will always be better off at A3 than other credit rating choices. Thus, we conclude that A3 is the OCR, which is consistent with the procedure in Section 3.2 that argues that the nongrowth OCR should be used as the growth OCR.

Figure 2 illustrates the downward concave relation between G_L when plotted against increasing leverage choices. Consistent with trade-off theory that posits an optimal debt-to-firm value ratio (ODV) exists, Figure 2 reveals full (two-sided) concave trajectories except for the NP trajectory where there is a downward bump for a Moody’s rating of A1 (upper medium grade rating). The next plot point with A2 is where the G_L differences in the NP trajectory and CC trajectory peak at USD 0.784M − USD 0.610M = USD 0.174M (which is a 28.48% difference in terms of the lower NP value of USD 0.610M). By the time we get to the last credit rating of Ba2 for which both NPs and CCs have plot points, we find a smaller difference of 16.81%.

The lower NP max G_L of USD 0.829M (compared to the CC max G_L of USD 1.020M) is consistent with the notion that the advantage from an ITS should be greater for CCs compared to NPs. This should be especially true for pre-TCJA tests where the corporate tax rate is much higher that its TCJA counterpart so that the small ITS for NPs is dominated by the large ITS for CCs. However, debt still adds value for NPs due to other influences such as those posited by agency models. To illustrate, the percentage increase in unlevered value from issuing debt (%∆E_U) is 7.33% for large NPs as given in Table 6. This percentage is much lower that the %∆E_U of 16.19% for large CCs found in Table 7. Thus, not only do CCs have a greater absolute gain (as seen in its greater max G_L value), CCs also increase relatively more in unlevered firm value from debt and this latter result is consistent with NPs having greater unlevered firm values as well as less of an ITS. However, any CC advantage from having a much greater ITS is offset since NP debt owners pay less personal tax on debt compared to CC debt owners. To illustrate the offsetting nature for the tests represented in Figure 2, the difference in business tax of 0.2400 favoring CCs at its OCR is substantially neutralized by the difference in the personal tax on debt of 0.2269 favoring NPs at its OCR. Thus, the greater max G_L value for CCs (which is an after-tax value) compared to that for NPs is probably only partially explained by differences in ITS.

In Figure 3, we duplicate Figure 2 by replacing G_L with V_L. Since our valuations are after all taxes are considered, this figure visually shows the tremendous V_L advantage that an NP (solid line trajectory) has over a CC (dashed line trajectory) from not paying taxes when everything else is equal (such as same before-tax cash flows, same costs of borrowing, and same growth rate). Thus, Figure 3 serves to depict, from a strictly tax standpoint, the vast differences in V_L that occur when everything is the same except tax rates. In fact, Figure 3 reveals that the NP max V_L of USD 12.146M is USD 4.827M greater than the CC max V_L of USD 7.319M. None of this difference can explained by the gain to leverage since the NP max G_L of USD 0.829M is less than the CC max G_L of USD 1.046M.

Figure 4 shows the relative gain to leverage in terms of the percentage change in unlevered firm value (%∆E_U). This figure illustrates the greater relative gain to leverage for CCs (dashed line trajectory) compared to NPs (solid line trajectory) by showing the superiority of CCs over NPs for all leverage choices up to the Moody’s rating of Ba2 at which point the NP trajectory ends. The differences in values for %∆E_U increase up to Baa2 and then declines for Ba1 and Ba2. The decline is short-lived because the growth constraint is violated after Ba2 for NPs. Finally, the difference in %∆E_U between CCs and NPs at their OCRs is 16.19% − 7.33% = 8.86% in favor of CCs.

Figure 5 plots the levered equity growth rate (g_L) against ratings. In examining values for the levered equity growth rates (e.g., g_L values) past the 3.12% target, Figure 5 reveals that g_L values for NPs (solid line) are greater until the NP growth constraint sets in at B1, at which point g_L values for NPs cease to exist while those for CCs (dashed line) continue to rise until the CC growth constraints sets in at B3. While an NP achieves a growth rate of 5.48% at its last credit rating (which is Ba2), a CC attains a growth rate of 7.18% at its last rating (which is B2). Of interest (and as seen in Figure 2, Figure 3 and Figure 4), higher growth rates for CCs do not lead to greater values for G_L, V_L, and %∆E_U when past OCR. As alluded to previously, while NPs can achieve higher values past OCR, these are achieved with a growth rate at Baa2 that is not possible historically (at least for the average company). If possible, then values for G_L, V_L, and %∆E_U would be greater with that growth rate at A3.

5. Results Incorporating Low Tax Rates, TCJA, RTS, and Increased Growth

The tests in this section consider the high (H) and low (L) tax rate scenarios discussed in Section 2.3, two different tax law environments (pre-TCJA and TCJA), two tax policies (ITS and RTS), and two growth rates projected under TCJA (3.90% and 4.50%) discussed in Section 3.2. As before, we perform tests that take credit spreads matched to credit ratings provided by Damodaran (2021) for the year 2020.

The results from this section are reported in Table 8 and Table 9. Twelve outcomes are highlighted. First, we include the seven outcomes reported by the prior C corp (CC) and/or pass-through (PT) research (Hull and Price 2015; Hull 2020a, 2020b) These seven outcomes are: the debt choice outcomes of P and DV; the valuation outcomes of E_U and max V_L; and, leverage gain outcomes of max G_L, max %∆E_U, and NB. Second, we now include the three growth-related outcomes from Hull and Van Dalsem (2021). These three outcomes are PBR_BT, g_U, and DGN. Third, we will formally report on OCRs, which is a third debt choice outcome.

5.1. ITS Results

Table 8 reports values under an ITS tax policy for eleven outcomes from 24 tests using credit spreads for the year from 2020 as given by Damodaran (2021). The tests include large NPs and large CCs and use the low (L) and high (H) sets of tax rates for both nongrowth and growth situations within a pre-TCJA and TCJA tax law environments. For pre-TCJA tests, we use a growth rate of 3.12%. For TCJA tests, we also use 3.12% along with two other growth rates consistent with increases in growth caused by lower taxes under TCJA. As described in Section 3.2, these rates are 3.90% and 4.50%.

The high (H) set of tax rates were the focus of Table 3, Table 5, Table 6 and Table 7 and Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5. Besides H tax rates, Table 8 also incorporates L tax rates. While both sets of tax rates were given in Section 2.3 and summarized in Table 1, we briefly overview their use in Table 8 noting that some rates do not change with TCJA (such as capital gains and dividend rates) and so rates dominated by these categories do not necessarily change. For L results for NPs use an unlevered corporate business tax rate (T_C1) of 0 for both pre-TCJA and TCJA tests, while the H results use a T_C1 of 0.06 for pre-TCJA tests and 0.04 for TCJA tests. The L results for NPs use an unlevered personal equity tax rate (T_E1) of 0, while the H results use a T_E1 of 0.05 for pre-TCJA tests and 0.04 for TCJA tests. The L results for CCs use an unlevered personal tax rate (T_E1) of 0.11 for both pre-TCJA and TCJA tests, while the H results use a T_E1 of 0.165 for both pre-TCJA tests and TCJA tests. Both the L and H results for NPs use an unlevered personal debt tax rate (T_D1) of 0 for pre-TCJA and TCJA tests. The L results for CCs use an unlevered personal debt tax rate (T_D1) of 0.15 for pre-TCJA tests and 0.14 for TCJA tests, while the H results use a T_D1 of 0.19 for pre-TCJA tests and 0.18 for TCJA tests. For Table 8, we once again use the ICRs given by Damodaran (2021) as associated with his 2020 spreads and ratings that were reported in January 2021. As noted earlier, the credit spreads we use are consistent with the historical averages from 2013 through 2020. The acronym DGN (in the last column) refers to the difference of max V_L with growth minus max V_L with nongrowth. The variable demonstrates how growth adds more value when tax rates are lower. While DGN is a new outcome added to Table 8, the outcomes in the other ten columns have the same definitions given in previous tables.

Table 8 contains results under an ITS tax policy and are reported in six panels that are partitioned in order to capture outcomes based on nongrowth versus growth, ownership type (NP and CC), tax rate set (L and H), and tax legislation (pre-TCJA and TCJA). Panel A has nongrowth, pre-TCJA results. Panel B has nongrowth, TCJA results. Panel C has pre-TCJA results when growth is 3.12%. Panel D has TCJA results when growth is 3.12%. Panel E has TCJA results when growth is 3.90%. Panel F has TCJA results when growth is 4.50%.

Table 8. Optimal Outcomes for Large NPs and Large CCs under ITS.

	P	PBRBT	gU	EU	Max VL	Max GL	Max %∆EU	NB	ODV	OCR	DGN
Panel A. Nongrowth: Pre-TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.13%	8.53%	0.3552	A3	n.a.
NP-H	0.3890	0.0000	0.00%	10.710	11.548	0.838	7.82%	20.11%	0.3608	A3	n.a.
CC-L	0.4500	0.0000	0.00%	7.472	8.600	1.128	15.10%	33.56%	0.3909	Baa2	n.a.
CC-H	0.4594	0.0000	0.00%	6.509	7.786	1.277	19.62%	42.69%	0.3841	Baa2	n.a.
Ave	0.4162	0.0000	0.00%	9.171	10.075	0.904	11.42%	26.22%	0.3727	Baa2/A3	n.a.
Panel B. Nongrowth: TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.13%	8.53%	0.3552	A3	n.a.
NP-H	0.3838	0.0000	0.00%	11.053	11.776	0.723	6.54%	17.04%	0.3602	A3	n.a.
CC-L	0.3562	0.0000	0.00%	8.646	9.498	0.853	9.86%	27.68%	0.3242	A3	n.a.
CC-H	0.3601	0.0000	0.00%	7.911	8.845	0.934	11.81%	32.78%	0.3221	A3	n.a.
Ave	0.3666	0.0000	0.00%	9.901	10.622	0.721	7.83%	21.51%	0.3404	A3	n.a.
Panel C. 3.12% Growth: Pre-TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3682	0.2144	2.14%	11.317	12.146	0.829	7.33%	19.90%	0.3430	A3	0.598
CC-L	0.4569	0.2692	2.15%	7.358	8.284	0.926	12.59%	27.56%	0.4058	Baa2	−0.316
CC-H	0.4747	0.2933	2.25%	6.300	7.319	1.020	16.19%	34.10%	0.4086	Baa2	−0.467
Ave	0.4112	0.2427	2.14%	9.426	10.260	0.835	10.13%	23.59%	0.3720	Baa2/A3	0.185
Panel D. 3.12% Growth: TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3624	0.2078	2.10%	11.703	12.469	0.766	6.54%	18.05%	0.3402	A3	0.693
CC-L	0.3462	0.2522	2.28%	8.895	9.653	0.757	8.51%	24.58%	0.3190	A3	0.154
CC-H	0.3514	0.2607	2.32%	8.107	8.908	0.800	9.87%	28.09%	0.3199	A3	0.062
Ave	0.3513	0.2286	2.18%	10.359	11.080	0.722	7.34%	20.89%	0.3274	A3	0.458
Panel E. 3.90% Growth: TCJA
NP-L	0.3337	0.2297	2.49%	13.164	13.940	0.776	5.90%	17.67%	0.3151	A3	1.572
NP-H	0.3501	0.2446	2.59%	12.116	13.046	0.930	7.68%	21.93%	0.3251	A3	1.270
CC-L	0.3346	0.2931	2.80%	9.202	10.043	0.840	9.13%	27.29%	0.3066	A3	0.544
CC-H	0.3396	0.3021	2.85%	8.391	9.258	0.867	10.33%	30.43%	0.3078	A3	0.412
Ave	0.3395	0.2674	2.68%	10.718	11.572	0.853	8.26%	24.33%	0.3137	A3	0.950
Panel F. 4.50% Growth: TCJA
NP-L	0.3231	0.2556	2.86%	13.596	14.625	1.029	7.57%	23.42%	0.3004	A3	2.257
NP-H	0.3386	0.2709	2.97%	12.527	13.665	1.138	9.08%	26.82%	0.3104	A3	1.889
CC-L	0.3234	0.3217	3.20%	9.523	10.483	0.960	10.09%	31.19%	0.2937	A3	0.985
CC-H	0.3280	0.3308	3.26%	8.686	9.657	0.970	11.17%	34.05%	0.2951	A3	0.811
Ave	0.3283	0.2948	3.07%	11.083	12.107	1.024	9.48%	28.87%	0.2999	A3	1.486

Table 8 report values under an ITS tax policy for eleven outcomes from 24 tests using credit spreads for the year from 2020 as given by Damodaran (2021). The tests include large NPs and large CCs and use the low (L) and high (H) sets of tax rates for nongrowth and growth situations within a pre-TCJA and TCJA tax law environments. For pre-TCJA tests, we use a growth rate of 3.12%. For TCJA tests, we also use 3.12% along with two other growth rates consistent with increases in growth caused by lower taxes under TCJA. As described in Section 3.2, these rates are 3.90% and 4.50%. Tax rates used in ITS tests were described in Section 2.3 and summarized in Table 2. Values for E_U, Max V_L, Max G_L, and DGN are in millions of USD. The abbreviation n.a. refers to not applicable.

Table 8. Optimal Outcomes for Large NPs and Large CCs under ITS.

	P	PBRBT	gU	EU	Max VL	Max GL	Max %∆EU	NB	ODV	OCR	DGN
Panel A. Nongrowth: Pre-TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.13%	8.53%	0.3552	A3	n.a.
NP-H	0.3890	0.0000	0.00%	10.710	11.548	0.838	7.82%	20.11%	0.3608	A3	n.a.
CC-L	0.4500	0.0000	0.00%	7.472	8.600	1.128	15.10%	33.56%	0.3909	Baa2	n.a.
CC-H	0.4594	0.0000	0.00%	6.509	7.786	1.277	19.62%	42.69%	0.3841	Baa2	n.a.
Ave	0.4162	0.0000	0.00%	9.171	10.075	0.904	11.42%	26.22%	0.3727	Baa2/A3	n.a.
Panel B. Nongrowth: TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.13%	8.53%	0.3552	A3	n.a.
NP-H	0.3838	0.0000	0.00%	11.053	11.776	0.723	6.54%	17.04%	0.3602	A3	n.a.
CC-L	0.3562	0.0000	0.00%	8.646	9.498	0.853	9.86%	27.68%	0.3242	A3	n.a.
CC-H	0.3601	0.0000	0.00%	7.911	8.845	0.934	11.81%	32.78%	0.3221	A3	n.a.
Ave	0.3666	0.0000	0.00%	9.901	10.622	0.721	7.83%	21.51%	0.3404	A3	n.a.
Panel C. 3.12% Growth: Pre-TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3682	0.2144	2.14%	11.317	12.146	0.829	7.33%	19.90%	0.3430	A3	0.598
CC-L	0.4569	0.2692	2.15%	7.358	8.284	0.926	12.59%	27.56%	0.4058	Baa2	−0.316
CC-H	0.4747	0.2933	2.25%	6.300	7.319	1.020	16.19%	34.10%	0.4086	Baa2	−0.467
Ave	0.4112	0.2427	2.14%	9.426	10.260	0.835	10.13%	23.59%	0.3720	Baa2/A3	0.185
Panel D. 3.12% Growth: TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3624	0.2078	2.10%	11.703	12.469	0.766	6.54%	18.05%	0.3402	A3	0.693
CC-L	0.3462	0.2522	2.28%	8.895	9.653	0.757	8.51%	24.58%	0.3190	A3	0.154
CC-H	0.3514	0.2607	2.32%	8.107	8.908	0.800	9.87%	28.09%	0.3199	A3	0.062
Ave	0.3513	0.2286	2.18%	10.359	11.080	0.722	7.34%	20.89%	0.3274	A3	0.458
Panel E. 3.90% Growth: TCJA
NP-L	0.3337	0.2297	2.49%	13.164	13.940	0.776	5.90%	17.67%	0.3151	A3	1.572
NP-H	0.3501	0.2446	2.59%	12.116	13.046	0.930	7.68%	21.93%	0.3251	A3	1.270
CC-L	0.3346	0.2931	2.80%	9.202	10.043	0.840	9.13%	27.29%	0.3066	A3	0.544
CC-H	0.3396	0.3021	2.85%	8.391	9.258	0.867	10.33%	30.43%	0.3078	A3	0.412
Ave	0.3395	0.2674	2.68%	10.718	11.572	0.853	8.26%	24.33%	0.3137	A3	0.950
Panel F. 4.50% Growth: TCJA
NP-L	0.3231	0.2556	2.86%	13.596	14.625	1.029	7.57%	23.42%	0.3004	A3	2.257
NP-H	0.3386	0.2709	2.97%	12.527	13.665	1.138	9.08%	26.82%	0.3104	A3	1.889
CC-L	0.3234	0.3217	3.20%	9.523	10.483	0.960	10.09%	31.19%	0.2937	A3	0.985
CC-H	0.3280	0.3308	3.26%	8.686	9.657	0.970	11.17%	34.05%	0.2951	A3	0.811
Ave	0.3283	0.2948	3.07%	11.083	12.107	1.024	9.48%	28.87%	0.2999	A3	1.486

Table 8 report values under an ITS tax policy for eleven outcomes from 24 tests using credit spreads for the year from 2020 as given by Damodaran (2021). The tests include large NPs and large CCs and use the low (L) and high (H) sets of tax rates for nongrowth and growth situations within a pre-TCJA and TCJA tax law environments. For pre-TCJA tests, we use a growth rate of 3.12%. For TCJA tests, we also use 3.12% along with two other growth rates consistent with increases in growth caused by lower taxes under TCJA. As described in Section 3.2, these rates are 3.90% and 4.50%. Tax rates used in ITS tests were described in Section 2.3 and summarized in Table 2. Values for E_U, Max V_L, Max G_L, and DGN are in millions of USD. The abbreviation n.a. refers to not applicable.

Table 8 includes the eleven key outcomes consisting of three debt choice outcomes (P, ODV, and OCR), two valuation outcomes (E_U and max V_L), three leverage gain outcomes (max G_L, max, %∆E_U, and NB), and three growth-related outcomes (PBR_BT, g_U, and DGN). One way of understanding differences in outcomes based on categories such as ownership types, tax rate sets, legislative tax environments, and growth versus nongrowth is to focus on extreme values associated with these categories. Thus, in our analysis (given below), we focus on minimums and maximums for (i) for all values for each outcome and (ii) averages for each panel’s four tests for each outcome (where applicable). Minimums are in italics and maximums are in bold. For example, consider the outcome ODV. The italicized 0.2937 for the CC-L test in Panel F is the minimum of all values for this outcome, while the bold print 0.4086 for the CC-H test in Panel C is the maximum of all values. In terms of the panel averages for ODV, the italicized 0.2999 in the “Ave” row of Panel F is the minimum of the six panel averages, while the bold print 0.3727 in the “Ave” row of Panel A is the maximum of the six panel averages.

In terms of the debt choice outcomes, Table 8 reveals the following from the 24 tests (consisting of four tests for each of the six panels) per outcome. The minimum P choice of 0.3231 occurs in Panel F (4.50% growth, TCJA) for the NP-L test. The minimum ODV of 0.2937 also occurs in Panel F but for the CC-L test. Among all panels, Panel F has the lowest averages for its four values for P and ODV where the respective averages are 0.3283 and 0.2999. The maximum P choice and maximum ODV of 0.4747 and 0.4086, respectively, occur in Panel C (growth 3.12%, pre-TCJA) for the CC-H test. Among panels, Panel A (nongrowth, pre-TCJA) has the highest averages for P and ODV of 0.4162 and 0.3727, respectively. These Panel A averages are slightly higher than those of 0.4112 and 0.3720 that occur in Panel C (growth 3.12%, pre-TCJA). The higher debt choice outcomes for Panels A and C reflect the four OCR outcomes of Baa2 for CC tests. These four OCRs are of lower quality credit ratings that are associated with greater leverage and thus more credit risk. Other than these four occurrences for CC tests (two nongrowth tests in Panel A and two 3.12% growth tests in Panel C), Table 9 reveals that the OCR is Moody’s A3, which is a higher quality rating than Baa2. In conclusion, the debt choice results indicate that greater growth leads to issuing and maintaining relatively less amount of debt while higher amounts of debt and lower quality credit ratings occur during the pre-TCJA period when tax rates are higher especially for CCs.

For the valuation outcomes, Table 8 reports that the smallest E_U and max V_L values of USD 6.300M and USD 7.319M, respectively, occur for the CC-H test in Panel C (3.12% growth, pre-TCJA). The CC-H results in Panel A (nongrowth, pre-TCJA) are also small. The four E_U and max V_L outcomes in Panel A (nongrowth, pre-TCJA) have the lowest averages among panels with respective averages of USD 9.171M and USD 10.075M. The largest E_U of USD 13.596M and the largest max V_L of USD 14.625M occur for the NP-L tests in Panel F (4.50% growth, TCJA). The four E_U and max V_L outcomes in Panel F also have the highest averages among panels with respective averages of USD 11.083M and USD 12.107M. In conclusion (and as might be expected), the smallest valuation outcomes occur for pre-TCJA tests for CCs where tax rates are higher causing after-tax values to be lower and growth to be more costly, while the greatest valuation outcomes occur for NPs when they are not taxed and experience the highest level of growth due to small tax rates.

In terms of the leverage gain outcomes, Table 8 reveals that the lowest values for max G_L, max %∆E_U, and NB of USD 0.375M, 3.13%, and 8.53%, respectively, occur for the NP-L test in both Panel A (nongrowth, pre-TCJA) and Panel B (nongrowth, TCJA). The identical outcomes occur because L tax rates for NPs are zero and so the tax rate environment (pre-TCJA or TCJA) is irrelevant as all nongrowth tax rate environments produce the same outcomes when tax rates are zero. Panel B has the lowest max G_L average of USD 0.721M while Panel D (3.12% growth, TCJA) has the lowest averages for max %∆E_U and NB at 7.34% and 20.89%, respectively. The highest values for max G_L, max %∆E_U, and NB of USD 1.277M, 19.62%, and 42.69%, respectively, occur in Panel A (nongrowth, pre-TCJA) for the CC-H test. Thus, Panel A has both the lowest and highest values and this explains why this panel does not contain all of the lowest or highest panel averages. The lowest and highest values in Panel A are attributed to the widespread differences caused by ownership type where NPs have low leverage gain outcome values and CCs have high values. Panel F (4.50% growth, TCJA) has the highest max G_L average. While Panel A (nongrowth, pre-TCJA) has the highest max %∆E_U average, Panel F (4.50% growth, TCJA) has the highest max G_L and NB averages. The average max %∆E_U for all tests in Table 8 is 9.08% (median is 8.80%). This percentage is consistent with the empirical pre-TCJA ITS research (Graham 2000; Korteweg 2010; Van Binsbergen et al. 2010). This research suggests that debt can increase firm value by as much as 10% (with an average of 7%). In conclusion, nongrowth and low growth NP tests generally have the lowest leverage gain outcomes, while nongrowth and high growth CC tests generally have the highest leverage gain outcomes.

For the growth-related outcomes, we will only focus on growth tests since nongrowth tests provide values that are either zero or not applicable (n.a.). Ignoring these latter tests, Table 8 shows that the smallest PBR_BT and g_U of 0.1938 and 2.00%, respectively, occur in Panel D (3.12% growth, TCJA) for the NP-L test. The smallest DGN is -USD 0.467M and is found in Panel C (3.12% growth, pre-TCJA) for the CC-H test. The negative value indicates that nongrowth offers greater wealth than growth by USD 0.467M. According to the CSM and as noted by Hull and Van Dalsem (2021), this can be explained in terms of the high business tax rate on earnings retained for growth as these funds are subject to taxes before they can be used for growth. The CC-H test with 3.12% growth in Panel C is a pre-TCJA test with much higher business taxes compared to a TCJA test. Among all panels, the smallest average for PBR_BT is 0.2286, which occurs in Panel D (3.12% growth, TCJA). The smallest g_U and DGN averages of 2.14% and USD 0.185M, respectively, are found in Panel C (3.12% growth, pre-TCJA). Once again, this can be explained by greater taxes for pre-TCJA tests that makes growth costly. The largest growth-related outcomes all take place in Panel F (4.50% growth, TCJA) where the largest PBR_BT and g_U values of 0.3308 and 3.26%, respectively, occur for the CC-H test, while the largest DGN of USD 2.257M occurs for the NP-L test. Among all panels, Panel F reports the greatest PBR_BT, g_U, and DGN averages of 0.2948, 3.07%, and USD 1.486M, respectively. In conclusion (and ignoring nongrowth tests), lower growth-related outcomes occur for CC tests in a pre-TCJA tax law environment and for smaller growth rates, while the higher growth-related outcomes occur when larger growth rates are present such as is found in a TCJA tax law environment.

By reporting the L and H results, we demonstrate how growth adds more value when tax rates are lower. This is seen in the last column for the outcome of DGN that captures the difference of max V_L with growth minus max V_L with nongrowth. The lower values in this last column occur for the H tax rate tests and reflect the difficulty of growth adding values when the business tax rate is high making the after-tax usage of retained earnings (RE) more expensive. As noted by Hull and Van Dalsem (2021) in their ownership comparison study of NPs and PTs, the lower values for DGN are consistent with the proof given by Hull (2010) where the minimum g_U implies that the business tax rate on RE must be less than the before-tax plowback ratio (PBR_BT) to make growth valuable for an unlevered firm. Thus, PBR_BT must rise whenever the business tax rate on RE rises or growth becomes less valuable. Lower values for DGN are also consistent with empirical research, as reviewed by McBride (2012), that indicates growth decreases when tax rates increase.

5.2. RTS Results

Table 9 repeats Table 8 but reports values under an RTS tax policy for eleven outcomes based on 24 tests. Below we examine these outcomes in a fashion performed when we examined Table 8 while focusing on a comparison of the similarities and differences between Table 8 and Table 9 based on tax policies. We will also report overall statistics from both tables along with ITS and RTS comparison.

For the debt choice outcomes for RTS tests, Table 9 reveals the following. The minimums for P choice and ODV of 0.3128 and 0.2856, respectively, occur in Panel F (4.50% growth, TCJA) for the CC-L test. While slightly lower than the corresponding Table 8 values, these RTS results for the two debt choice outcomes are still similar to the ITS results in Table 8. One difference (when comparing Panel F in Table 8 with Panel F in Table 9) is that the minimum P choice of 0.3231 for an ITS policy occurs for the NP-L test instead of the CC-L test. Among all panels, Panel F has the lowest averages for its four values for P and ODV where the respective averages are 0.3221 and 0.2952. These RTS values in Table 9 are like the ITS values in Table 8 except (once again) are slightly lower. The maximum P choice and maximum ODV of 0.3890 and 0.3653, respectively, occur in Panel A (nongrowth, pre-TCJA) for the NP-H test. In contrast to these maximum RTS values, the maximum ITS values are noticeably higher with maximums for P choice and ODV of 0.4747 and 0.4086, respectively, with their occurrence in Panel C (growth 3.12%, pre-TCJA) for the CC-H test. Among panels in Table 9, Panel A (nongrowth, pre-TCJA) has the highest average for P choice of 0.3710, while Panel B (nongrowth, TCJA) has highest average for ODV of 0.3482. Once again, RTS results in Table 9 are lower than the ITS results in Table 8. In addition, the RTS results differ from the ITS results where Panel A in Table 8 has the highest average for ODV of 0.3727. The higher debt choice outcomes for Panels A and C in Table 8 for the ITS tests reflect the four OCR occurrences of Baa2. RTS tests do not generate an OCR of Baa2 for any of their tests as all OCRs in Table 9 are A3, which is a higher quality rating than Baa2 and thus would be expected to embody lower leverage outcomes.

Table 9. Optimal Outcomes for Large NPs and Large CCs under RTS.

	P	PBRBT	gU	EU	Max VL	Max GL	Max %∆EU	NB	ODV	OCR	DGN
Panel A. Nongrowth: Pre-TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.12%	8.53%	0.3552	A3	n.a.
NP-H	0.3890	0.0000	0.00%	10.710	11.406	0.696	6.50%	16.70%	0.3653	A3	n.a.
CC-L	0.3606	0.0000	0.00%	7.472	8.055	0.583	7.81%	21.66%	0.3344	A3	n.a.
CC-H	0.3680	0.0000	0.00%	6.509	7.183	0.674	10.36%	28.14%	0.3335	A3	n.a.
Ave	0.3710	0.0000	0.00%	9.171	9.753	0.582	6.95%	18.76%	0.3471	A3	n.a.
Panel B. Nongrowth: TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.12%	8.53%	0.3552	A3	n.a.
NP-H	0.3838	0.0000	0.00%	11.053	11.678	0.625	5.66%	14.74%	0.3632	A3	n.a.
CC-L	0.3562	0.0000	0.00%	8.646	9.123	0.477	5.52%	15.49%	0.3375	A3	n.a.
CC-H	0.3601	0.0000	0.00%	7.911	8.460	0.549	6.93%	19.25%	0.3368	A3	n.a.
Ave	0.3666	0.0000	0.00%	9.901	10.407	0.506	5.31%	14.50%	0.3482	A3	n.a.
Panel C. 3.12% Growth: Pre-TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3653	0.1981	2.06%	11.406	12.177	0.771	6.76%	18.51%	0.3421	A3	0.771
CC-L	0.3381	0.1997	2.08%	8.362	9.034	0.673	8.04%	23.79%	0.3129	A3	0.979
CC-H	0.3443	0.2027	2.12%	7.303	8.026	0.723	9.90%	28.77%	0.3132	A3	0.843
Ave	0.3482	0.1986	2.07%	9.950	10.632	0.683	7.28%	20.97%	0.3247	A3	0.879
Panel D. 3.12% Growth: TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3606	0.1971	2.05%	11.762	12.489	0.727	6.18%	17.13%	0.3397	A3	0.810
CC-L	0.3348	0.1969	2.04%	9.199	9.782	0.583	6.34%	18.95%	0.3148	A3	0.660
CC-H	0.3380	0.1987	2.07%	8.430	9.052	0.622	7.38%	21.85%	0.3148	A3	0.592
Ave	0.3446	0.1966	2.04%	10.530	11.154	0.624	6.08%	17.69%	0.3249	A3	0.747
Panel E. 3.90% Growth: TCJA
NP-L	0.3337	0.2297	2.49%	13.164	13.940	0.776	5.90%	17.67%	0.3151	A3	1.572
NP-H	0.3484	0.2328	2.53%	12.174	13.086	0.912	7.50%	21.51%	0.3241	A3	1.408
CC-L	0.3234	0.2328	2.53%	9.523	10.266	0.743	7.80%	24.13%	0.3000	A3	1.143
CC-H	0.3263	0.2346	2.56%	8.732	9.499	0.768	8.79%	26.94%	0.2999	A3	1.039
Ave	0.3330	0.2325	2.53%	10.898	11.698	0.800	7.50%	22.56%	0.3098	A3	1.291
Panel F. 4.50% Growth: TCJA
NP-L	0.3231	0.2556	2.86%	13.596	14.625	1.029	7.57%	23.42%	0.3004	A3	2.257
NP-H	0.3371	0.2585	2.91%	12.582	13.722	1.140	9.06%	26.87%	0.3091	A3	2.043
CC-L	0.3128	0.2587	2.91%	9.845	10.781	0.936	9.50%	30.38%	0.2856	A3	1.658
CC-H	0.3155	0.2603	2.93%	9.030	9.973	0.944	10.45%	33.13%	0.2857	A3	1.513
Ave	0.3221	0.2583	2.90%	11.263	12.275	1.012	9.14%	28.45%	0.295	A3	1.868

Table 9 report values under an RTS tax policy for eleven outcomes from 24 tests using credit spreads for the year from 2020 as given by Damodaran (2021). The tests include large NPs and large CCs and use low (L) and high (H) sets of tax rates for both nongrowth and growth situations within a pre-TCJA and TCJA tax law environments. For pre-TCJA tests, we use a growth rate of 3.12%. For TCJA tests, we also use 3.12% along with two other growth rates consistent with increases in growth caused by lower taxes under TCJA. As described in Section 3.2, these rates are 3.90% and 4.50%. Tax rates used in RTS tests were described in Section 2.3 and summarized in Table 2. Values for E_U, Max V_L, Max G_L, and DGN are in millions of dollars (USD USD). The abbreviation n.a. refers to not applicable.

Table 9. Optimal Outcomes for Large NPs and Large CCs under RTS.

	P	PBRBT	gU	EU	Max VL	Max GL	Max %∆EU	NB	ODV	OCR	DGN
Panel A. Nongrowth: Pre-TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.12%	8.53%	0.3552	A3	n.a.
NP-H	0.3890	0.0000	0.00%	10.710	11.406	0.696	6.50%	16.70%	0.3653	A3	n.a.
CC-L	0.3606	0.0000	0.00%	7.472	8.055	0.583	7.81%	21.66%	0.3344	A3	n.a.
CC-H	0.3680	0.0000	0.00%	6.509	7.183	0.674	10.36%	28.14%	0.3335	A3	n.a.
Ave	0.3710	0.0000	0.00%	9.171	9.753	0.582	6.95%	18.76%	0.3471	A3	n.a.
Panel B. Nongrowth: TCJA
NP-L	0.3663	0.0000	0.00%	11.993	12.368	0.375	3.12%	8.53%	0.3552	A3	n.a.
NP-H	0.3838	0.0000	0.00%	11.053	11.678	0.625	5.66%	14.74%	0.3632	A3	n.a.
CC-L	0.3562	0.0000	0.00%	8.646	9.123	0.477	5.52%	15.49%	0.3375	A3	n.a.
CC-H	0.3601	0.0000	0.00%	7.911	8.460	0.549	6.93%	19.25%	0.3368	A3	n.a.
Ave	0.3666	0.0000	0.00%	9.901	10.407	0.506	5.31%	14.50%	0.3482	A3	n.a.
Panel C. 3.12% Growth: Pre-TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3653	0.1981	2.06%	11.406	12.177	0.771	6.76%	18.51%	0.3421	A3	0.771
CC-L	0.3381	0.1997	2.08%	8.362	9.034	0.673	8.04%	23.79%	0.3129	A3	0.979
CC-H	0.3443	0.2027	2.12%	7.303	8.026	0.723	9.90%	28.77%	0.3132	A3	0.843
Ave	0.3482	0.1986	2.07%	9.950	10.632	0.683	7.28%	20.97%	0.3247	A3	0.879
Panel D. 3.12% Growth: TCJA
NP-L	0.3451	0.1938	2.00%	12.729	13.292	0.563	4.42%	12.82%	0.3305	A3	0.924
NP-H	0.3606	0.1971	2.05%	11.762	12.489	0.727	6.18%	17.13%	0.3397	A3	0.810
CC-L	0.3348	0.1969	2.04%	9.199	9.782	0.583	6.34%	18.95%	0.3148	A3	0.660
CC-H	0.3380	0.1987	2.07%	8.430	9.052	0.622	7.38%	21.85%	0.3148	A3	0.592
Ave	0.3446	0.1966	2.04%	10.530	11.154	0.624	6.08%	17.69%	0.3249	A3	0.747
Panel E. 3.90% Growth: TCJA
NP-L	0.3337	0.2297	2.49%	13.164	13.940	0.776	5.90%	17.67%	0.3151	A3	1.572
NP-H	0.3484	0.2328	2.53%	12.174	13.086	0.912	7.50%	21.51%	0.3241	A3	1.408
CC-L	0.3234	0.2328	2.53%	9.523	10.266	0.743	7.80%	24.13%	0.3000	A3	1.143
CC-H	0.3263	0.2346	2.56%	8.732	9.499	0.768	8.79%	26.94%	0.2999	A3	1.039
Ave	0.3330	0.2325	2.53%	10.898	11.698	0.800	7.50%	22.56%	0.3098	A3	1.291
Panel F. 4.50% Growth: TCJA
NP-L	0.3231	0.2556	2.86%	13.596	14.625	1.029	7.57%	23.42%	0.3004	A3	2.257
NP-H	0.3371	0.2585	2.91%	12.582	13.722	1.140	9.06%	26.87%	0.3091	A3	2.043
CC-L	0.3128	0.2587	2.91%	9.845	10.781	0.936	9.50%	30.38%	0.2856	A3	1.658
CC-H	0.3155	0.2603	2.93%	9.030	9.973	0.944	10.45%	33.13%	0.2857	A3	1.513
Ave	0.3221	0.2583	2.90%	11.263	12.275	1.012	9.14%	28.45%	0.295	A3	1.868

Table 9 report values under an RTS tax policy for eleven outcomes from 24 tests using credit spreads for the year from 2020 as given by Damodaran (2021). The tests include large NPs and large CCs and use low (L) and high (H) sets of tax rates for both nongrowth and growth situations within a pre-TCJA and TCJA tax law environments. For pre-TCJA tests, we use a growth rate of 3.12%. For TCJA tests, we also use 3.12% along with two other growth rates consistent with increases in growth caused by lower taxes under TCJA. As described in Section 3.2, these rates are 3.90% and 4.50%. Tax rates used in RTS tests were described in Section 2.3 and summarized in Table 2. Values for E_U, Max V_L, Max G_L, and DGN are in millions of dollars (USD USD). The abbreviation n.a. refers to not applicable.

In conclusion, like the ITS results in Table 8, the RTS debt choice results in Table 9 indicate that greater growth leads to issuing and maintaining relatively less amount of debt while higher amounts of debt occur during the pre-TCJA period when tax rates are higher especially for CCs. An examination of Table 8 and Table 9 reveal that ODVs are 2.56% higher for NPs compared to CCs indicating that NPs utilize slightly more leverage to reach max V_L. These results are driven by the RTS tests where ODV is 6.69% higher for NPs compared to 1.56% lower for ITS tests.

In terms of the valuation outcomes, Table 9 reports that the smallest E_U and max V_L values of USD 6.509M and USD 7.183M, respectively, occur for the CC-H test in Panel A (nongrowth, pre-TCJA). These RTS results in Table 9 for the two valuation outcomes differ somewhat from the ITS results in Table 8. While the smallest values still occur for the CC-H test in Table 8, these values occur in Panel A (nongrowth, pre-TCJA) and not Panel C (growth, pre-TCJA) for Table 9 results. Regardless, Table 8 and Table 9 report small values for Panel A and Panel C as both panels have the highest tax rates. The four E_U and max V_L outcomes in Panel A (nongrowth, pre-TCJA) have the lowest averages among panels with respective averages of USD 9.171M and USD 9.753M. These RTS results are similar to the ITS results. In fact, the value of USD 9.171M for E_U average is identical for both ITS and RTS tests. This is because both tax shield policies have no impact on unlevered equity for a nongrowth situation. For example, ITS is zero with no debt and RTS is zero with no growth. The largest E_U of USD 13.596M and the largest max V_L of USD 14.625M occur for the NP-L tests in Panel F (4.50% growth, TCJA) of Table 9. These RTS values are identical to the ITS values in Table 8. The identical values of USD 13.596M for E_U was just described. The identical values of USD 14.625M for max V_L occur because NP-L tests have zero tax rates so that both ITS and RTS have zero tax shields causing identical max V_L values. The four E_U and max V_L outcomes in Panel F also have the highest averages among panels with respective averages of USD 11.263M and USD 12.275M. These RTS results in Table 9, like the ITS results in Table 8, have their highest averages in Panel F. The valuation outcomes for RTS are a bit higher than those for ITS indicating that a tax policy favoring growth is best for business growth and wealth.

In conclusion (and like ITS results in Table 8), the smallest valuation outcomes in Table 9 for RTS results occur for pre-TCJA tests for CCs where tax rates are higher making after-tax values lower and also causing growth to be more costly, while the greatest valuation outcomes occur for NPs when they are not taxed and experience the highest level of growth. In examining Table 8 and Table 9, we find that max V_L for NPs is 34.90% higher than CCs. This finding holds for both ITS test where NPs are 35.46% higher and RTS tests where NPs are 34.33% higher. This finding reveals that tax avoidance impacts value regardless of the tax shield policy.

For the leverage gain outcomes, Table 9 reveals that the lowest values for max G_L, max %∆E_U, and NB of USD 0.375M, 3.13%, and 8.53%, respectively, occur in Panel A (nongrowth, pre-TCJA) for the NP-L test where tax rates are zero. These same values also occur in Panel B (nongrowth, TCJA) for the NP-L test. They are the same because both tax rate environments (pre-TCJA and TCJA) produce the same nongrowth results when tax rates are zero. The same nongrowth results when tax rates are zero also hold regardless of the tax shield policy (ITS or RTS). This is because all tax shields that are dependent on positive tax rates are zero when tax rates are zero. For the latter reason, the RTS values in Table 9 are the same values found in Table 8 for the nongrowth, ITS and NP-L tests in Panels A and B. Like Panel A in Table 8, Panel A in Table 9 does not have the lowest averages among all panels for the three leverage gain outcomes as Panel B (nongrowth TCJA) has the lowest max G_L, max %∆E_U, and NB averages of USD 0.506M, 5.31%, and 14.50%, respectively. There are two differences when comparing the latter RTS results in Table 9 with the ITS results in Table 8. First, Table 9 results for max G_L, max %∆E_U and NB have noticeably lower values compared to Table 8. Second, while Table 9 results are like Table 8 results in that Panel B (nongrowth, TCJA) has the lowest average for max G_L (USD 0.506M for Table 9 versus USD 0.721M for Table 8), the lowest averages for max %∆E_U and NB differ in terms of the panel of occurrence. For example, instead of occurring in Panel B, the ITS results for the lowest averages of 7.34% and 20.89% for max %∆E_U and NB, respectively, occur in Panel D (3.12% growth, TCJA). The highest leverage gain values in Table 9 are found in Panel F where the highest max G_L of USD 1.140M occurs for the NP-H test, while the highest values for max %∆E_U and NB of 10.45% and 33.13%, respectively, occur for the CC-H tests. These RTS results differ from the ITS results. First, ITS values are higher. Second, the highest ITS values for max G_L, max %∆E_U, and NB of USD 1.277M, 19.62%, and 42.69%, respectively, all occur in Panel A (nongrowth, pre-TCJA) for the CC-H test. The overall average max %∆E_U for all tests in Table 9 is 7.04% (median is 7.16%). Like the results in Table 8, this percentage is consistent with the empirical research cited there.

In conclusion, like the ITS results in Table 8, nongrowth and low growth NP tests in Table 9 generally have the lowest leverage gain outcomes, while nongrowth and high growth CC tests generally have the highest leverage gain outcomes. In analyzing Table 8 and Table 9, we find that max G_L is 10.97% less compared to CCs. This can be explained by the ITS tests where NPs gain 24.29% less compared to 2.36% more for RTS tests. The ITS finding that NPs gain less compared to CCs can be explained by not having a large interest tax shield due to paying lower taxes. In terms of max %∆E_U, we discover that NPs rise 6.02% beyond their E_U value with debt issuance while CCs rise 10.13% beyond E_U with debt issuance. In addition, we find that max %∆E_U is 50.46% lower for NPs compared to CCs. These results are mostly driven by the ITS tests where max %∆E_U is 65.79% lower for NPs compared to 35.13% lower for RTS tests. With regard to NB, we ascertain that E_U for NPs rises 17.06% for every dollar of debt while E_U for CCs increases 29.33%. These two percentages reveal that NB is 12.27% lower for NPs compared to CCs. While these percentages suggest that NPs are less efficient in their use of each dollar of debt, they also reflect the fact that NPs have higher firm valuations and so they must issue more debt to attain the same ODVs and same optimal credit ratings (OCRs).

In terms of the growth-related outcomes, Table 9 shows that the smallest PBR_BT and g_U values of 0.1938 and 2.00%, respectively, occur in Panel D (3.12% growth, TCJA) for the NP-L test. These are the same results found in Table 8 for reasons stated previously related to zero tax rates for the NP-L test. The smallest DGN is 0.592 and is found in Panel D (3.12% growth, TCJA) for the CC-H test. This positive DGN value contrasts with the negative value found in Panel C (3.12% growth, pre-TCJA) of Table 8 for the CC-H test under an ITS tax policy. We interpret the difference in DGN values as consistent with the growth friendly tax policy of an RTS as a negative DGN indicates that growth is too costly as the nongrowth firm value is greater than the growth firm value. Among all panels, the smallest average for PBR_BT is 0.1966, which occurs in Panel D (3.12% growth, TCJA). The smallest g_U and DGN averages of 2.04% and USD 0.747M, respectively, are found in Panel C (3.12% growth, pre-TCJA). Once again, these smaller values can be explained by greater taxes for pre-TCJA tests that makes growth costly. The RTS values of 0.1966 and 2.04% for PBR_BT and g_U are smaller than the corresponding values in Table 8 that are 0.2286 and 2.14%. This is consistent an RTS policy causing greater efficiency by needing less RE to grow. Likewise, the larger value USD 0.747M in Table 9, compared to the corresponding value of USD 0.185M in Table 8, indicates that an RTS policy causes nongrowth firm value to increase much more under an RTS tax policy. The largest growth-related outcomes all take place in Panel F (4.50% growth, TCJA) where the largest PBR_BT and g_U values of 0.2603 and 2.93%, respectively, occur for the CC-H test, while the largest DGN of USD 2.257M occurs for the NP-L test where the latter is the same value found in Table 8 for the corresponding test. Once again the same value reflects the fact that the NP-L test has zero tax rates. The values for PBR_BT and g_U of 0.2603 and 2.93% in Table 9 are noticeably smaller than the corresponding values of 0.3308 and 3.26% in Table 8. Once again, this indicates the pro-growth dynamic of an RTS tax policy by needing less RE to grow when it is not taxed. Among all panels, Panel F reports the greatest PBR_BT, g_U, and DGN averages of 0.2583, 2.90%, and USD 1.868M, respectively. These compare with the respective ITS values in Table 8 of 0.2948, 3.07%, and USD 1.486M. The smaller plowback ratio and unlevered growth rates and the larger DGN value in Table 9 are all consistent with the premise that tax shields on RE makes growth less costly and add to firm value.

In conclusion, the results in Table 9, compared to those in Table 8, indicate more efficient use of RE with growth adding more value; otherwise, it can be similarly stated for both tax shield policies that lower growth-related outcomes occur for CC tests in a pre-TCJA tax law environment and for smaller growth rates, while the higher growth-related outcomes occur when larger growth rates are present such as is found in a TCJA tax law environment. In examining Table 8 and Table 9, we find that NPs achieve their increase in value from growth with a PBR_BT that is 12.34% smaller than CCs and a g_U that is 8.40% lower than CCs. The PBR_BT results are largely explained by the ITS tests where the percentages are 23.26% lower compared to 1.41% for RTS tests. Similarly, for g_U, where the respective percentages are 15.00% and 1.79%. The smaller values for NPs occur because PBR_BT and g_U are positively related to RE and CCs can only use RE after business taxes are paid on it while NPs are capable of avoiding business taxes. Thus, a dollar of RE goes further for NPs.

5.3. Limitations and Future Research

As noted by prior CSM research, a study of performance comparison is limited by its assumption that the same general risk classes can exist for ownership forms and types. The rationale for this assumption was examined in Section 1.

Another limitation is our choice of growth rates where we use the historical growth rate of 3.12% and two projected growth rates under TCJA of 3.90% and 4.50% where the latter can be viewed as an optimistic estimation. Justification for 3.12% has been provided by prior CSM research that points out that this rate holds for a recent 70 year period. However, even during this period there have been ups and down as annual growth rates can significantly change from year to year. It remains to be seen how the projected rate of 3.90% will hold up over time. Since the time of this projection, some pessimism has resulted over achieving 3.90%. For example, the greater growth was projected based on CCs using their extra profit (from lower taxes) to increase worker wages thus spurring greater demand and investment. However, companies did not respond as expected as they used the extra funds to buy back shares. In addition, there has been talk about returning to pre-TCJA tax rates for CCs or at least raising them.

Furthermore, a study of this nature can be very dependent on the year for which credit spreads are used. For this reason, this study (or any study depending on data for a specific period of time) is always ongoing as data changes can cause changes in results and conclusions. Finally, there are limitations regarding the choice of tax rates because sources for tax rates can disagree as to what an effective tax rate should be causing uncertainty as to its true value. In conclusion, there are limitations in our research due to the uncertainty involving the values for variables used as inputs in our model.

In terms of future research, we offer the following potentials projects. First, a future project can use this study’s methods and insight to perform a case study on NP and FP enterprises. For example, the healthcare industry is an area where NPs, PTs, and CCs compete. Second, given the dependence of this study’s findings based on the most recent credit spreads at the time this research began, a future project can be undertaken to examine different sets of credit spreads for multiple years. Third, given the uncertainty surrounding the projected TCJA growth rates at the time that TCJA was enacted, further research can test different projected growth rates based on how the economy has responded to TCJA after five or more years. Fourth, different sets of tax rates can be examined. This becomes more important whenever there are significant changes in tax rates such as was experienced with TCJA. Fifth, while extant research has compared different ownership types, these studies have not been uniform differing on years for credit spreads, firm sizes, and tax shield policies. This makes comparisons of ownership performance studies difficult. Thus, future research should be conducted to compare all three ownership types of NPs, PTs, and CCs when credit spreads, firm sizes, and tax policies are all similar.

6. Discussion

The findings of this study have broad implications as we have shown the advantages of an RTS tax policy in terms of aiding growth and firm value. The recent study of Hull and Hull (2021) has already tested one implication by applying a retained earnings tax shield (RTS) policy to taxpayer wealth and federal tax revenue. The findings of that paper detail the advantage of an RTS in solving debt problems. This is consistent with the results of this paper where we show greater valuation results from the use of an RTS especially for CCs. In contrast to this paper’s findings and that of Hull and Hull, the widespread tax policy of most countries only allow for a tax shield where interest payments are a taxable deduction, e.g., an ITS tax policy. While countries may also allow for tax deductions for R&D expenditures through various types of government subsidies, we rarely, if ever, find a permanent and long-standing reduction for retained earnings used for growth. Thus, we fail to find an RTS tax policy.

Economists, business-minded leaders, and academic researchers often comment on the problems associated with allowing a tax shield associated with interest payments while failing to allow a comparable tax reduction to promote growth. Thus, even in the face of research that shows the value of lowering taxes to enable growth, political representatives fail to enact laws that would promote growth and thereby arguably increase both business wealth and government tax revenues. This paper’s findings should further bolster claims that government tax policies need to change by finding ways to support growth through more efficient tax policies that consider tax shields for internal equity funds invested in growth.

Finally, this paper’s NP results can serve to show what can happens if governments stop taxing retained earnings used for growth. As seen in our results for NPs, they are able to achieve a 78.12% greater increase in value when going from nongrowth to growth compared to CCs.

7. Materials and Methods

All materials and methods have been described in this paper so that researchers can replicate and build on published results. The cited sources provide for all data (where applicable) and these data are free to the public for use. As noted, we used sources at the time this research started. To our knowledge, these sources are still available and so no restrictions on this availability should exist. All methods used to produce this paper’s results are established and have been described and cited.

8. Conclusions

This study builds on the recent break-through NP and PT research of Hull and Van Dalsem (2021) by investigating large NPs and large CCs. We do this by computing optimal outcomes for nonprofits (NPs) and C corporations (CCs) using the Capital Structure Model (CSM). We argue that we can assume there are analogous risk classes for different ownership forms as they are intricately interwoven in their activities sharing in the same economic and business forces. With the same before-tax cash flows, costs of borrowing, and growth rates, the main difference in outcomes between nonprofits and C corps can be explained by their differences in tax paid and the tax policy adopted to shield earnings. Using data (credit ratings, credit spreads, and interest coverage ratios) for the most recent year of 2020, we offer the following findings.

First, we find that optimal debt-to-firm value ratios (ODVs) are 2.56% higher for NPs compared to CCs, revealing that NPs utilize slightly more leverage to reach maximum firm value (max V_L). These results are driven by the RTS tests. Second, we show that max V_L for NPs is 34.90% higher than CCs. These finding are driven by both ITS and RTS tests and thus are invariance to the tax policy that governs the choice of tax shields. Third, we discover that NPs achieve a 78.12% greater increase in value compared to CCs when going from their nongrowth max V_L to their growth max V_L. This finding is largely explained by the ITS tests but there is also a substantial increase for RTS tests.

Fourth, NPs achieve their increase in value from growth with a before-tax plowback ratio (PBR_BT) that is 12.34% smaller than CCs and an unlevered growth rate (g_U) that is 8.40% lower than CCs. The latter two results are largely explained by the ITS tests. Fifth, in terms of the maximum gain to leverage (max G_L), NPs gain 10.97% less in absolute dollars by issuing debt compared to CCs. This is entirely explained by the ITS tests as NPs gain 2.36% more in max G_L for RTS tests.

Sixth, in terms of the maximum percent change in unlevered equity (max %∆E_U) from a debt-for-equity transaction, we discover that NPs rise 6.02% beyond their unlevered equity (E_U) value with leverage compared to 10.13% for CCs. In addition, we show that max %∆E_U is 50.46% lower for NPs compared to CCs. These results are driven by both RTS and ITS tests but mostly from the latter. Seventh, with regard to the net benefit from leverage (NB), we find that NB is 12.27% lower for NPs compared to CCs where ITS and RTS can both explain the lower value. Finally, both NPs and CCs have optimal credit ratings (OCRs) dominated by a Moody’s rating of A3. The lone exceptions occur for pre-TCJA tests for CCs where OCR is Baa2 (which is a rating that is one notch less in quality).