Refined Beam Theory for Geometrically Nonlinear Pre-Twisted Structures
Abstract
:1. Introduction
2. Kinematic Description of Pre-Twisted Structures
3. Nonlinear Green Lagrange Strain
4. Constitutive Law
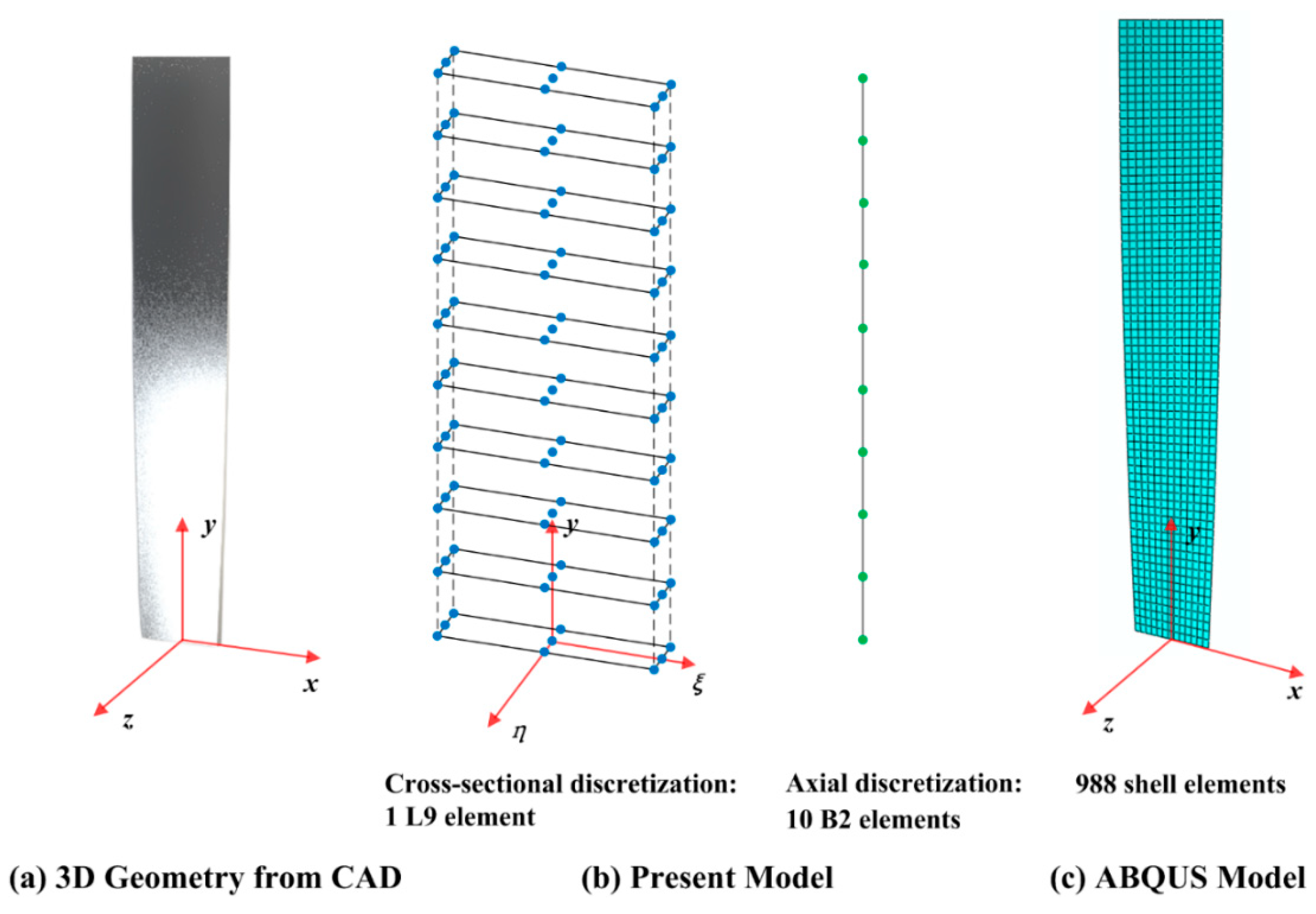
5. Carrera Unified Formulation
6. Equations of Motion
7. Arc-Length Method
8. Results
8.1. Pretwisted Cantilever with Rectangular Cross Section
8.2. Pretwisted Cantilever with arc Profile Cross Section
8.3. Pretwisted Cantilever with Airfoil Profile Cross Section
9. Discussion
- L9 elements have advanced local mapping capabilities to describe pre-twisted structures with different cross-sectional geometries. One L9 element is suitable for constructing the cross-sectional displacement field of pre-twisted structures with arc profile sections, while only eight L9 elements can describe the sharp curvature change and the displacement field within the cross sections of airfoil profile pre-twisted structures. The maximum difference between the present deformation results and those from commercial simulations is 6%.
- The stiffness of a pre-twisted structure can be enhanced by increasing its cross-sectional curvature. For pre-twisted structures with arc profile cross sections, the structural stiffness can be increased by up to 30% as the arc angle increases from 30° to 90°. However, this enhancement is reduced for structures with larger pretwist.
- The untwisted center of the pre-twisted structure coincides with its pre-twisted center. Moreover, the free-end cross section of a thin-walled, pre-twisted structure keeps its original profile.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Rosen, A. Structural and Dynamic Behavior of Pretwisted Rods and Beams. Appl. Mech. Rev. 1991, 44, 483–515. [Google Scholar] [CrossRef]
- Roy, P.A.; Hu, Y.; Meguid, S.A. Dynamic Behaviour of Pretwisted Metal Matrix Composite Blades. Compos. Struct. 2021, 268, 113947. [Google Scholar] [CrossRef]
- Sinha, S.K. Combined Torsional-Bending-Axial Dynamics of a Twisted Rotating Cantilever Timoshenko Beam with Contact-Impact Loads at the Free End. J. Appl. Mech. 2007, 74, 505–522. [Google Scholar] [CrossRef]
- Giavotto, V.; Borri, M.; Mantegazza, P.; Ghiringhelli, G.; Carmaschi, V.; Maffioli, G.; Mussi, F. Anisotropic Beam Theory and Applications. Comput. Struct. 1983, 16, 403–413. [Google Scholar] [CrossRef]
- Borri, M.; Merlini, T. A Large Displacement Formulation for Anisotropic Beam Analysis. Meccanica 1986, 21, 30–37. [Google Scholar] [CrossRef]
- Borri, M.; Ghiringhelli, G.L.; Merlini, T. Linear Analysis of Naturally Curved and Twisted Anisotropic Beams. Compos. Eng. 1992, 2, 433–456. [Google Scholar] [CrossRef]
- Simo, J.C.; Vu-Quoc, L. A Geometrically-Exact Rod Model Incorporating Shear and Torsion-Warping Deformation. Int. J. Solids Struct. 1991, 27, 371–393. [Google Scholar] [CrossRef]
- Gruttmann, F.; Sauer, R.; Wagner, W. A Geometrical Nonlinear Eccentric 3D-Beam Element with Arbitrary Cross-Sections. Comput. Methods Appl. Mech. Eng. 1998, 160, 383–400. [Google Scholar] [CrossRef]
- Atluri, S.N.; Iura, M.; Vasudevan, S. A Consistent Theory of Finite Stretches and Finite Rotations, in Space-Curved Beams of Arbitrary Cross-Section. Comput. Mech. 2001, 27, 271–281. [Google Scholar] [CrossRef]
- Battini, J.-M.; Pacoste, C. Co-Rotational Beam Elements with Warping Effects in Instability Problems. Comput. Methods Appl. Mech. Eng. 2002, 191, 1755–1789. [Google Scholar] [CrossRef]
- Alsafadie, R.; Hjiaj, M.; Battini, J.-M. Corotational Mixed Finite Element Formulation for Thin-Walled Beams with Generic Cross-Section. Comput. Methods Appl. Mech. Eng. 2010, 199, 3197–3212. [Google Scholar] [CrossRef]
- Rong, J.; Wu, Z.; Liu, C.; Brüls, O. Geometrically Exact Thin-Walled Beam Including Warping Formulated on the Special Euclidean Group S E (3). Comput. Methods Appl. Mech. Eng. 2020, 369, 113062. [Google Scholar] [CrossRef]
- Petrov, E.; Géradin, M. Finite Element Theory for Curved and Twisted Beams Based on Exact Solutions for Three-Dimensional Solids Part 1: Beam Concept and Geometrically Exact Nonlinear Formulation. Comput. Methods Appl. Mech. Eng. 1998, 165, 43–92. [Google Scholar] [CrossRef]
- Petrov, E.; Géradin, M. Finite Element Theory for Curved and Twisted Beams Based on Exact Solutions for Three-Dimensional Solids Part 2: Anisotropic and Advanced Beam Models. Comput. Methods Appl. Mech. Eng. 1998, 165, 93–127. [Google Scholar] [CrossRef]
- Klinkel, S.; Govindjee, S. Anisotropic Bending-Torsion Coupling for Warping in a Non-Linear Beam. Comput. Mech. 2003, 31, 78–87. [Google Scholar] [CrossRef]
- Silvestre, N.; Camotim, D. Nonlinear Generalized Beam Theory for Cold-Formed Steel Members. Int. J. Str. Stab. Dyn. 2003, 03, 461–490. [Google Scholar] [CrossRef]
- Basaglia, C.; Camotim, D.; Silvestre, N. Non-Linear GBT Formulation for Open-Section Thin-Walled Members with Arbitrary Support Conditions. Comput. Struct. 2011, 89, 1906–1919. [Google Scholar] [CrossRef]
- Gonçalves, R.; Camotim, D. Geometrically Non-Linear Generalised Beam Theory for Elastoplastic Thin-Walled Metal Members. Thin-Walled Struct. 2012, 51, 121–129. [Google Scholar] [CrossRef]
- Carrera, E.; Pagani, A.; Petrolo, M.; Zappino, E. Recent Developments on Refined Theories for Beams with Applications. Mech. Eng. Rev. 2015, 2, 14–00298. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Zhao, Y.; Wang, N.; Chen, X. Dynamic Analysis of Varying Speed Rotating Pretwisted Structures Using Refined Beam Theories. Int. J. Solids Struct. 2020, 185, 292–310. [Google Scholar] [CrossRef]
- Pagani, A.; Carrera, E. Unified Formulation of Geometrically Nonlinear Refined Beam Theories. Mech. Adv. Mater. Struct. 2018, 25, 15–31. [Google Scholar] [CrossRef] [Green Version]
- Pagani, A.; Carrera, E. Large-Deflection and Post-Buckling Analyses of Laminated Composite Beams by Carrera Unified Formulation. Compos. Struct. 2017, 170, 40–52. [Google Scholar] [CrossRef] [Green Version]
- Carrera, E.; Pagani, A.; Augello, R. Evaluation of Geometrically Nonlinear Effects Due to Large Cross-Sectional Deformations of Compact and Shell-like Structures. Mech. Adv. Mater. Struct. 2020, 27, 1269–1277. [Google Scholar] [CrossRef]
- Farsadi, T.; Rahmanian, M.; Kayran, A. Geometrically Nonlinear Aeroelastic Behavior of Pretwisted Composite Wings Modeled as Thin Walled Beams. J. Fluids Struct. 2018, 83, 259–292. [Google Scholar] [CrossRef]
- Ghorbani Shenas, A.; Ziaee, S.; Malekzadeh, P. Nonlinear Vibration Analysis of Pre-Twisted Functionally Graded Microbeams in Thermal Environment. Thin-Walled Struct. 2017, 118, 87–104. [Google Scholar] [CrossRef]
- Pelliciari, M.; Pasca, D.P.; Aloisio, A.; Tarantino, A.M. Size Effect in Single Layer Graphene Sheets and Transition from Molecular Mechanics to Continuum Theory. Int. J. Mech. Sci. 2022, 214, 106895. [Google Scholar] [CrossRef]
- Kloda, L.; Warminski, J. Nonlinear Longitudinal–Bending–Twisting Vibrations of Extensible Slowly Rotating Beam with Tip Mass. Int. J. Mech. Sci. 2022, 220, 107153. [Google Scholar] [CrossRef]
- Treyssède, F. Mode Propagation in Curved Waveguides and Scattering by Inhomogeneities: Application to the Elastodynamics of Helical Structures. J. Acoust. Soc. Am. 2011, 129, 1857–1868. [Google Scholar] [CrossRef] [Green Version]
- Carrera, E.; Cinefra, M.; Zappino, E.; Petrolo, M. Finite Element Analysis of Structures Through Unified Formulation: Carrera/Finite; John Wiley & Sons, Ltd.: Chichester, UK, 2014; ISBN 978-1-118-53664-3. [Google Scholar]
- Wu, B.; Pagani, A.; Chen, W.Q.; Carrera, E. Geometrically Nonlinear Refined Shell Theories by Carrera Unified Formulation. Mech. Adv. Mater. Struct. 2021, 28, 1721–1741. [Google Scholar] [CrossRef]
- Hu, X.X.; Tsuiji, T. Free Vibration Analysis of Curved and Twisted Cylindrical Thin Panels. J. Sound Vib. 1999, 219, 63–88. [Google Scholar] [CrossRef]
- Boubolt, J.C.; Brooks, W. Differential Equations of Motion of Combined Flapwise Bending, Chordwise Bending, and Torsion of Twisted, Nonuniform Rotor Blades; NACA Technical Note 3905; National Advisory Committee For Aeronautics: Washington, DC, USA, 1957.
- Hodges, D.H. Torsion of Pretwisted Beams Due to Axial Loading. J. Appl. Mech. 1980, 47, 393–397. [Google Scholar] [CrossRef]
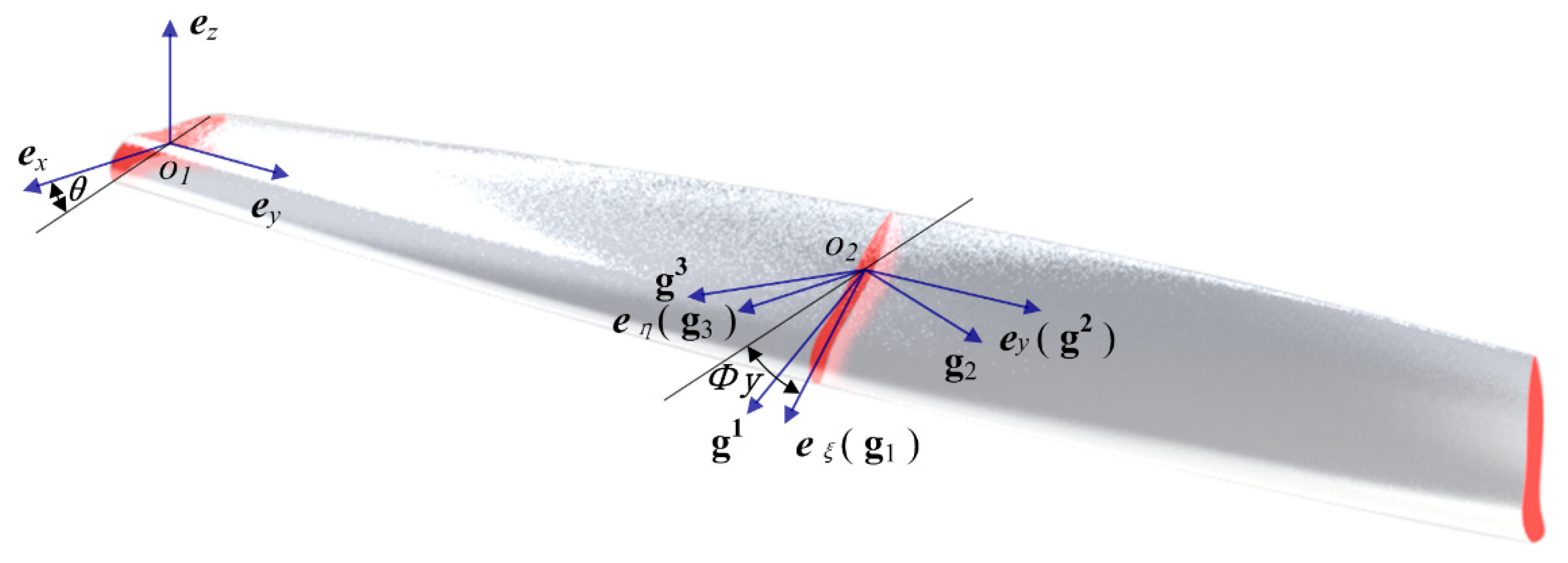
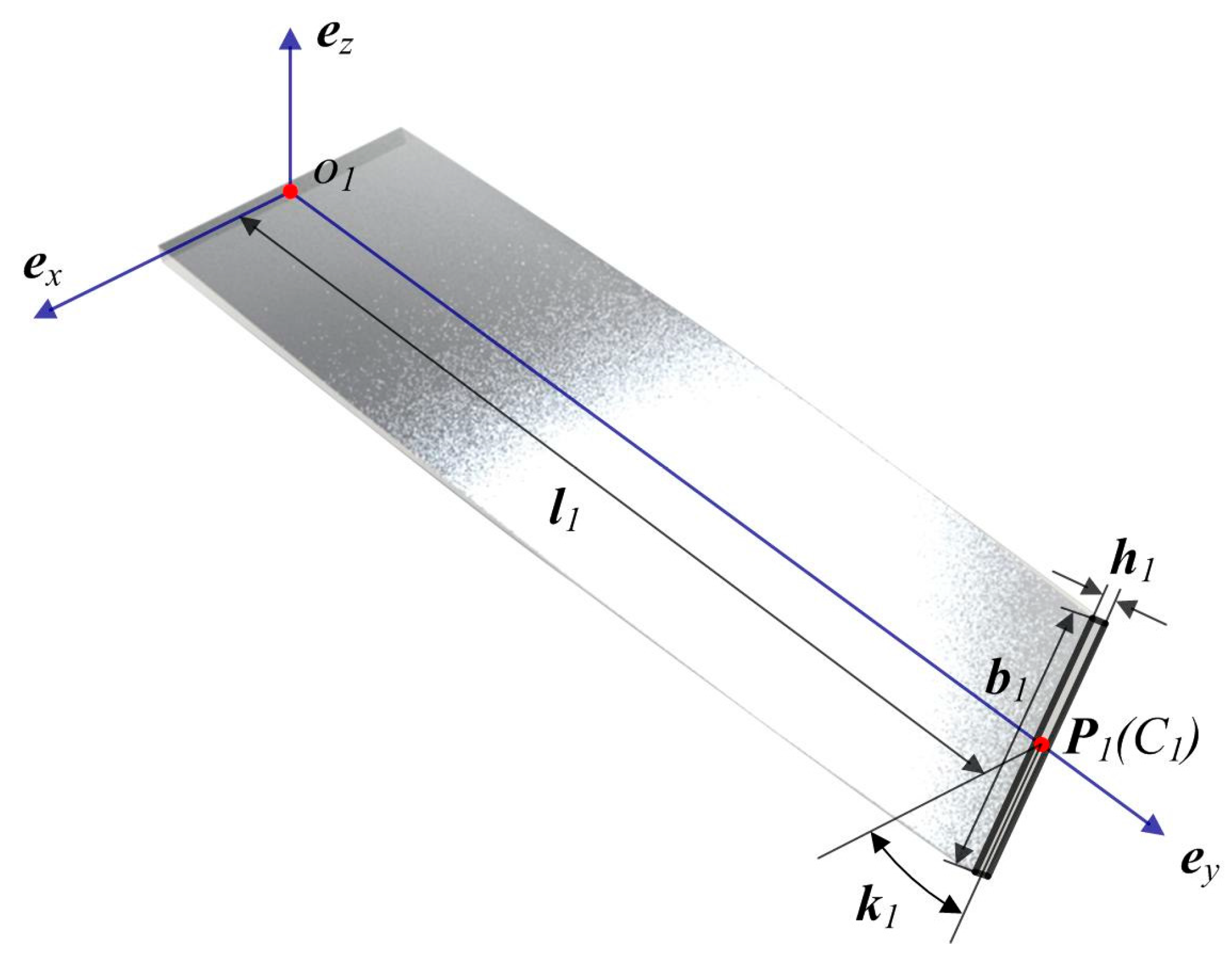

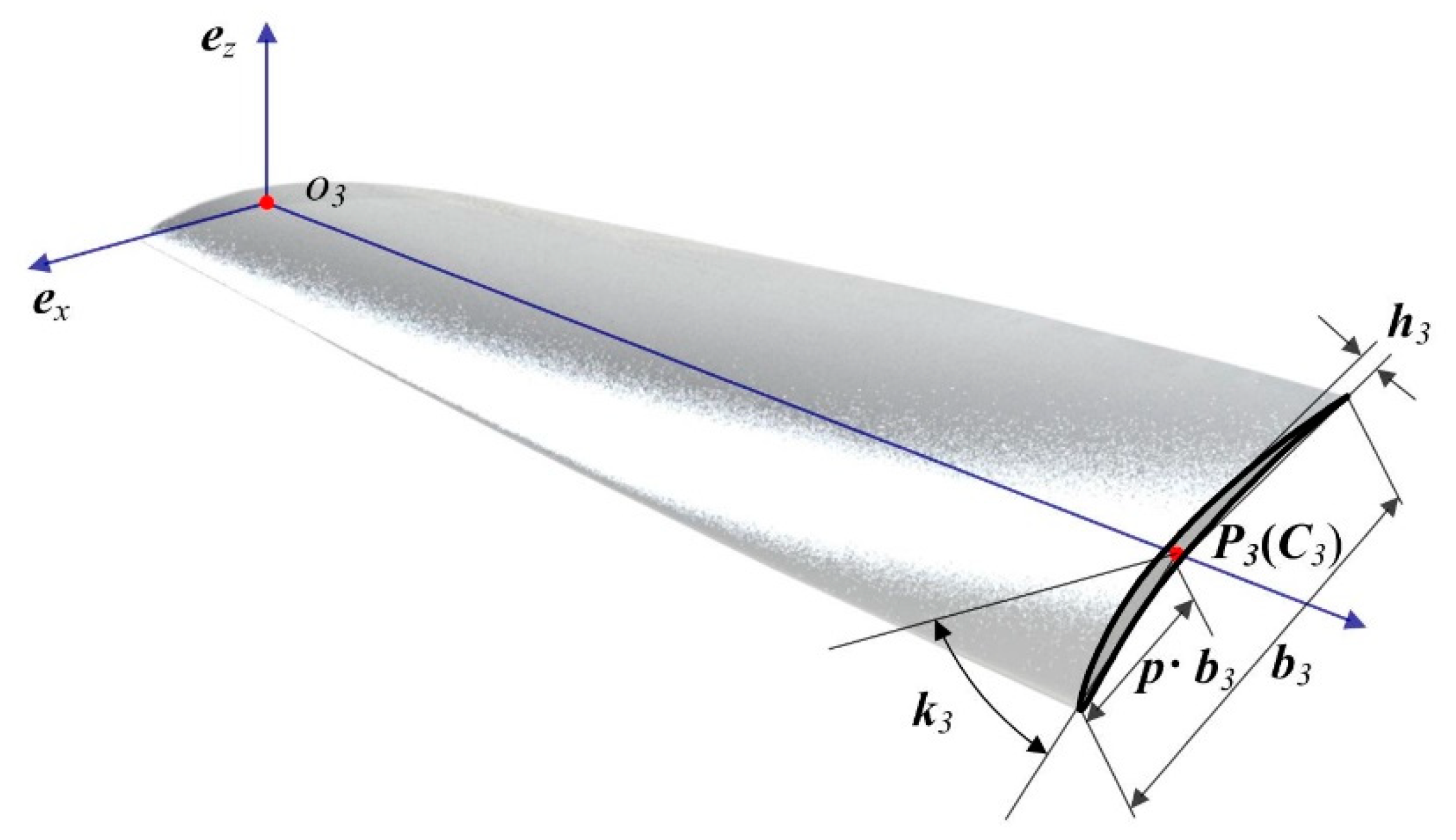
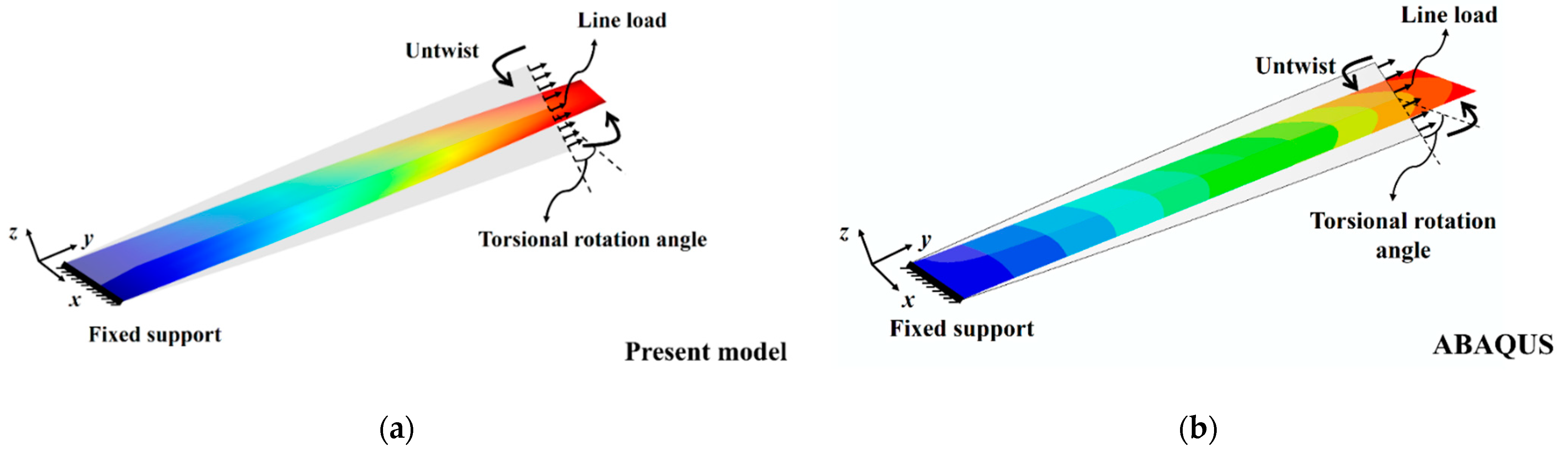

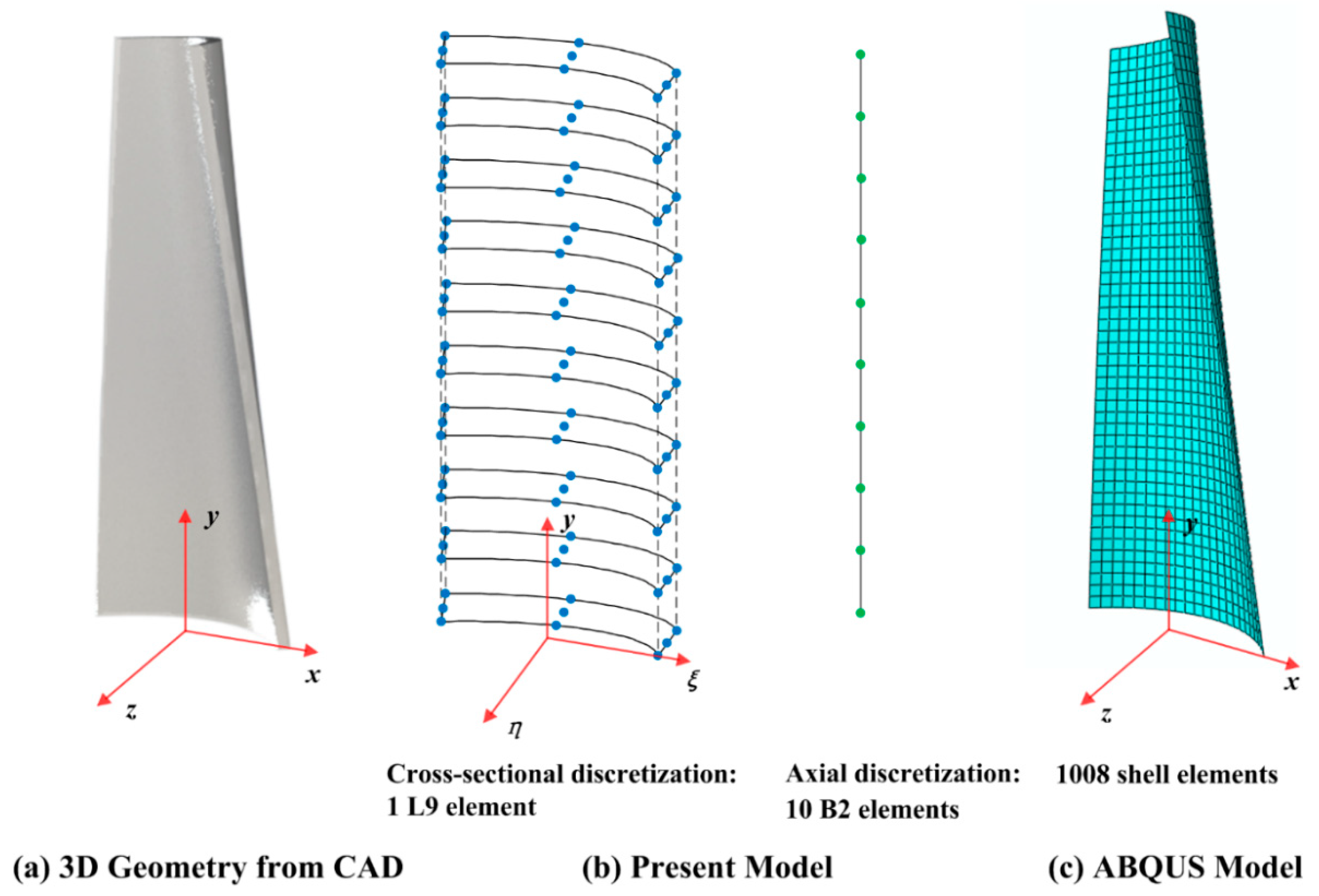
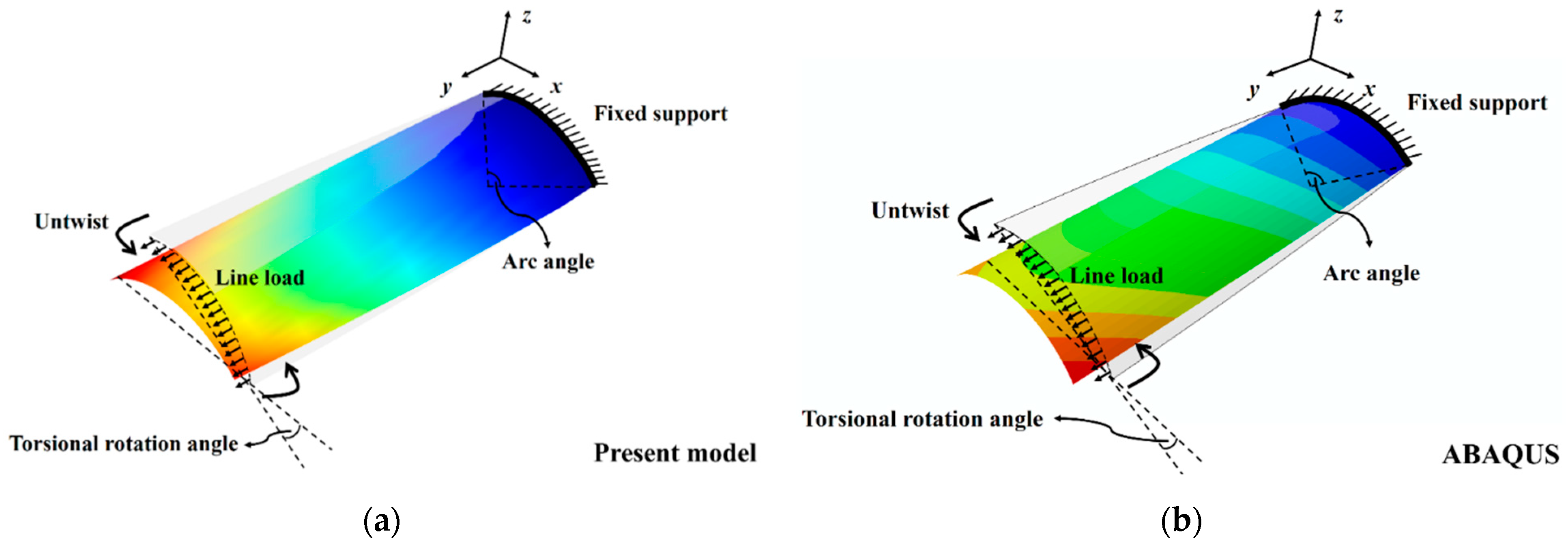


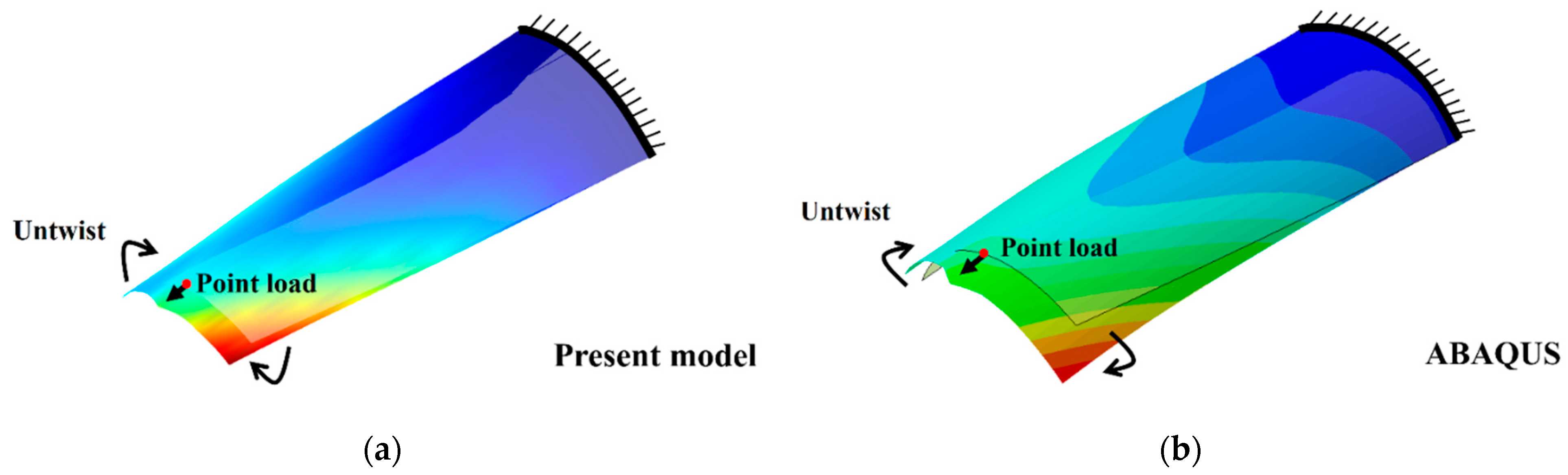

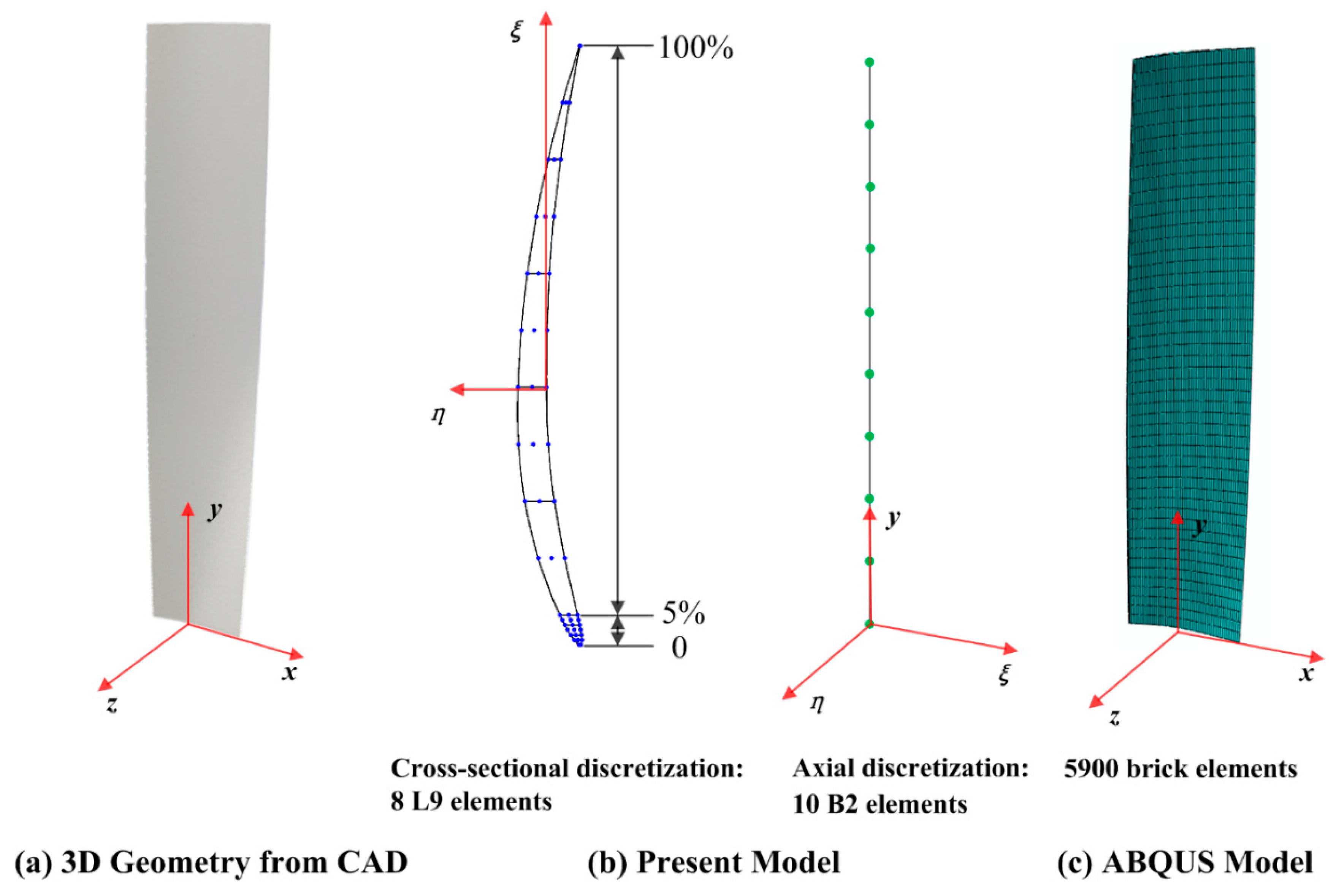
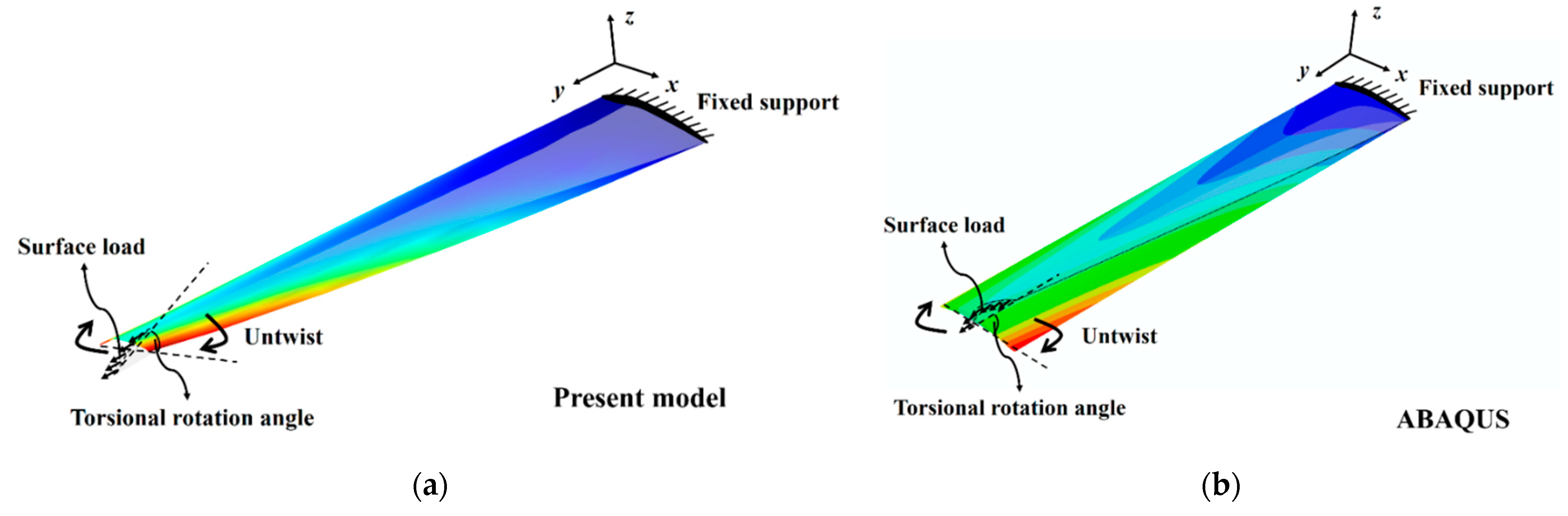
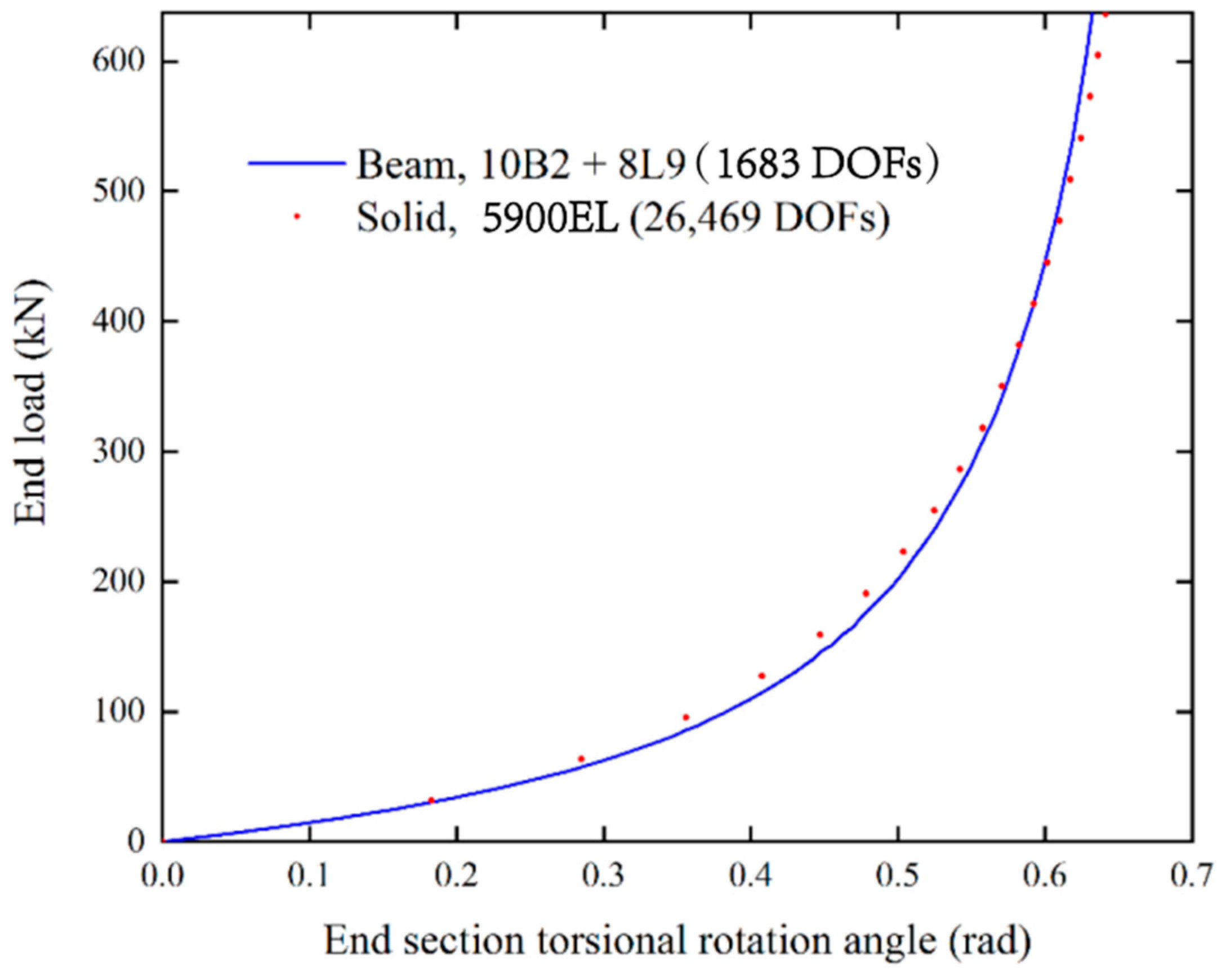
| Properties | Rectangular Cross-Sectional Structure | Arc profile Cross-Sectional Structures | Airfoil Profile Cross-Sectional Structure |
|---|---|---|---|
| Length Ɩ | 152.4 mm | 710 mm | 500 mm |
| Width b | 25.4 mm | 305 mm | 100 mm |
| Thickness h | 1.7272 mm | 3.05 mm | 5 mm (maximum) |
| Arc angle α | - | 30°, 60°, 90° | - |
| Pretwist angle k | 45° | 30°, 60° | 45° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Y.; Zhao, Y.; Liang, H. Refined Beam Theory for Geometrically Nonlinear Pre-Twisted Structures. Aerospace 2022, 9, 360. https://doi.org/10.3390/aerospace9070360
Hu Y, Zhao Y, Liang H. Refined Beam Theory for Geometrically Nonlinear Pre-Twisted Structures. Aerospace. 2022; 9(7):360. https://doi.org/10.3390/aerospace9070360
Chicago/Turabian StyleHu, Yi, Yong Zhao, and Haopeng Liang. 2022. "Refined Beam Theory for Geometrically Nonlinear Pre-Twisted Structures" Aerospace 9, no. 7: 360. https://doi.org/10.3390/aerospace9070360
APA StyleHu, Y., Zhao, Y., & Liang, H. (2022). Refined Beam Theory for Geometrically Nonlinear Pre-Twisted Structures. Aerospace, 9(7), 360. https://doi.org/10.3390/aerospace9070360





