Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material
Abstract
:1. Introduction
2. Materials and Methods
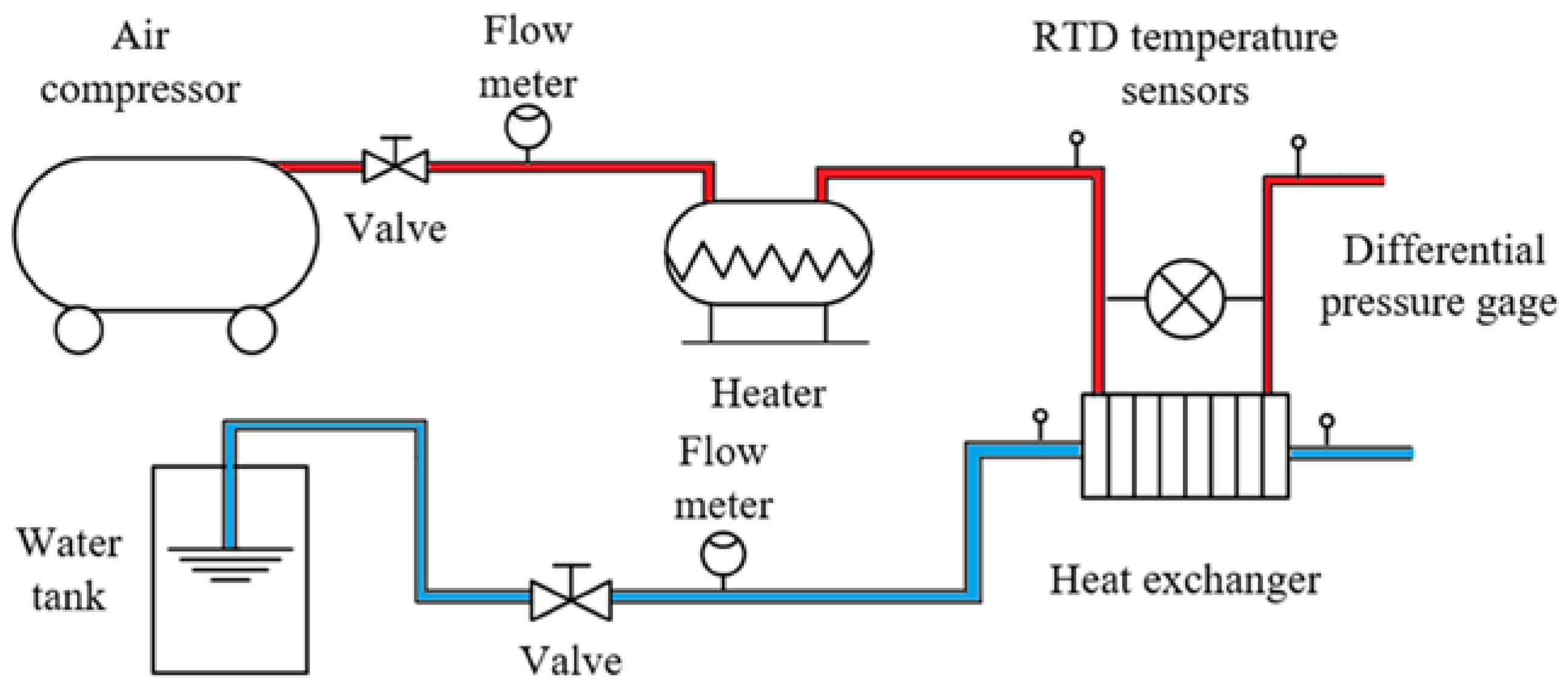
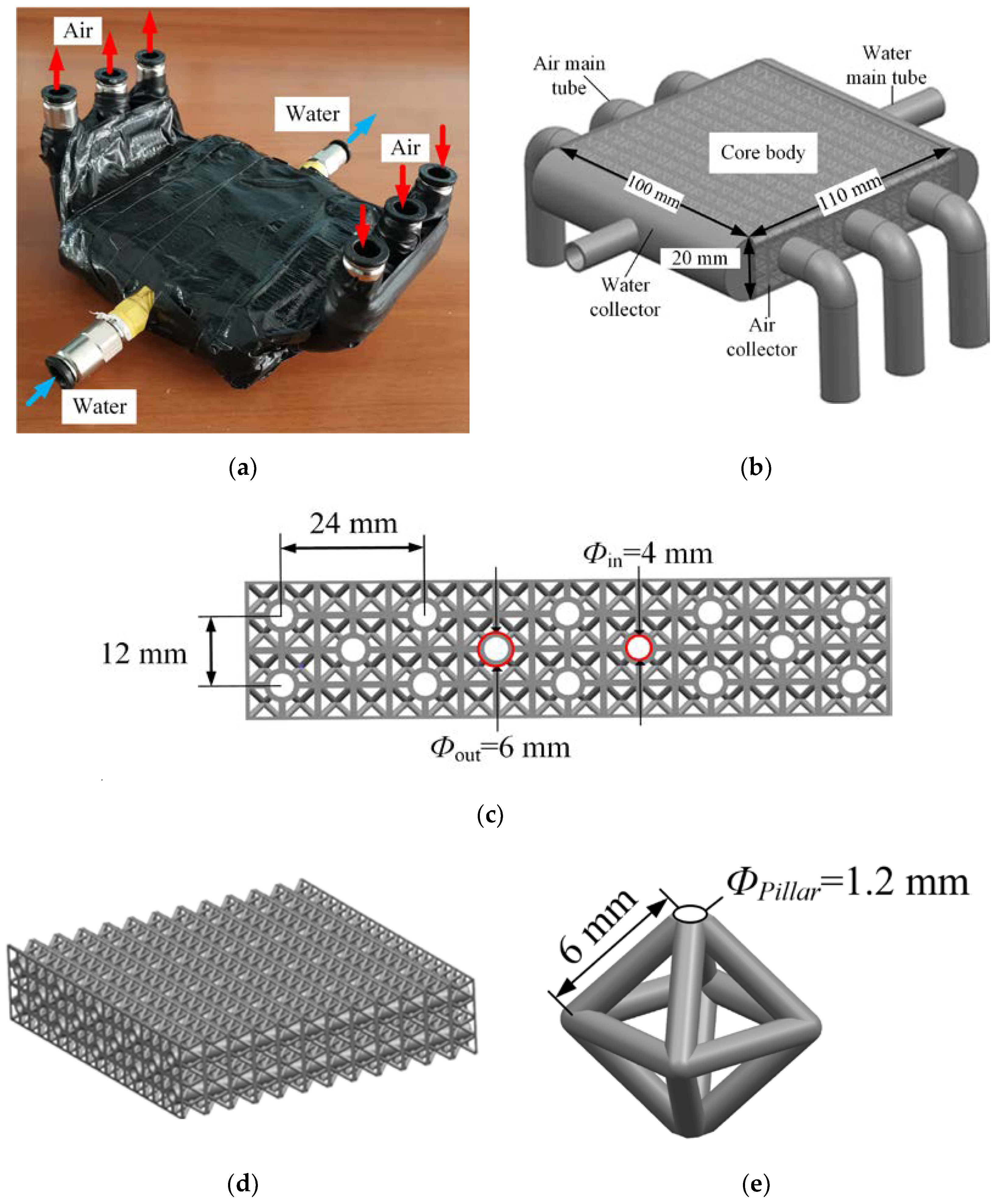
2.1. Experimental Setup
2.2. Computational Modeling
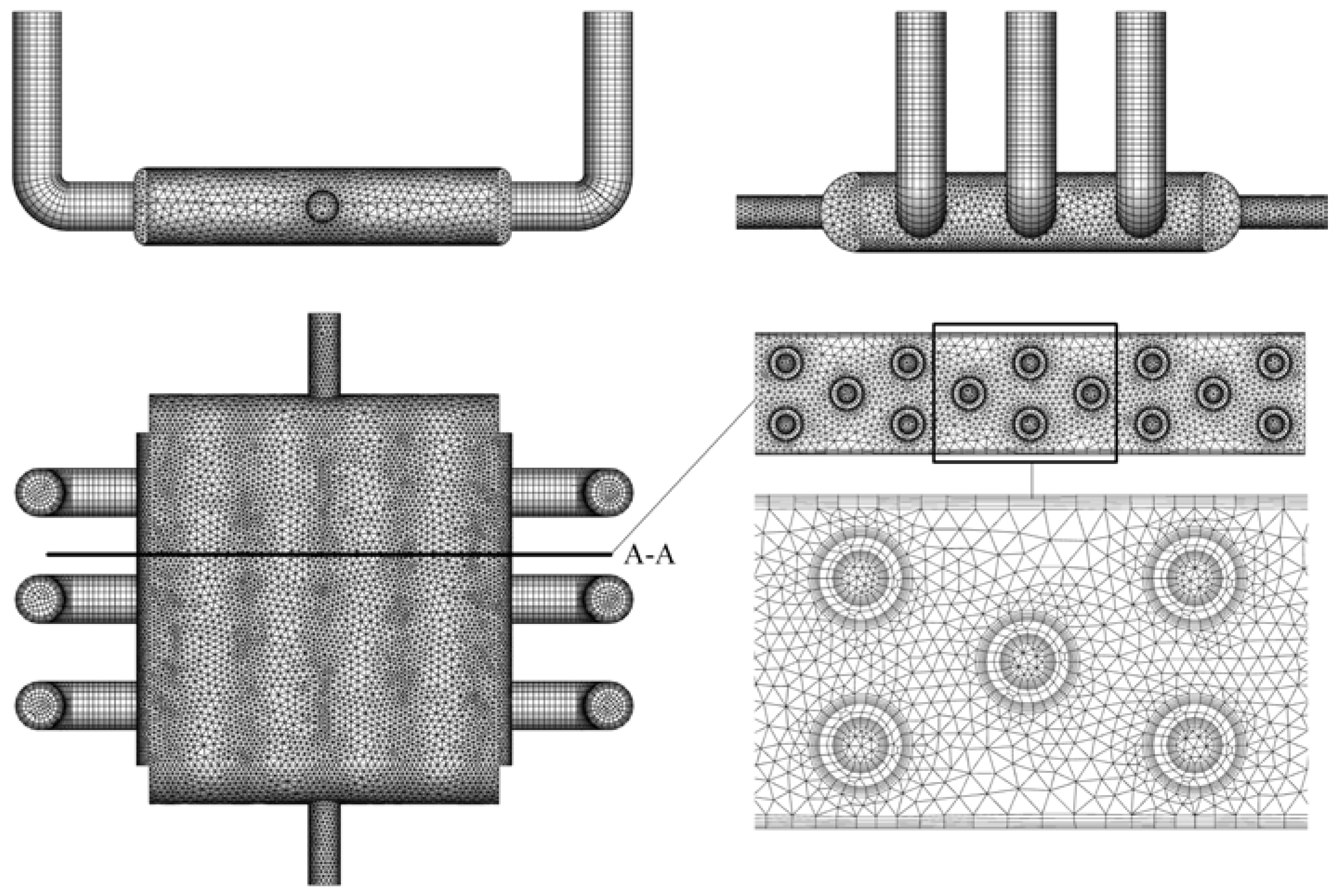
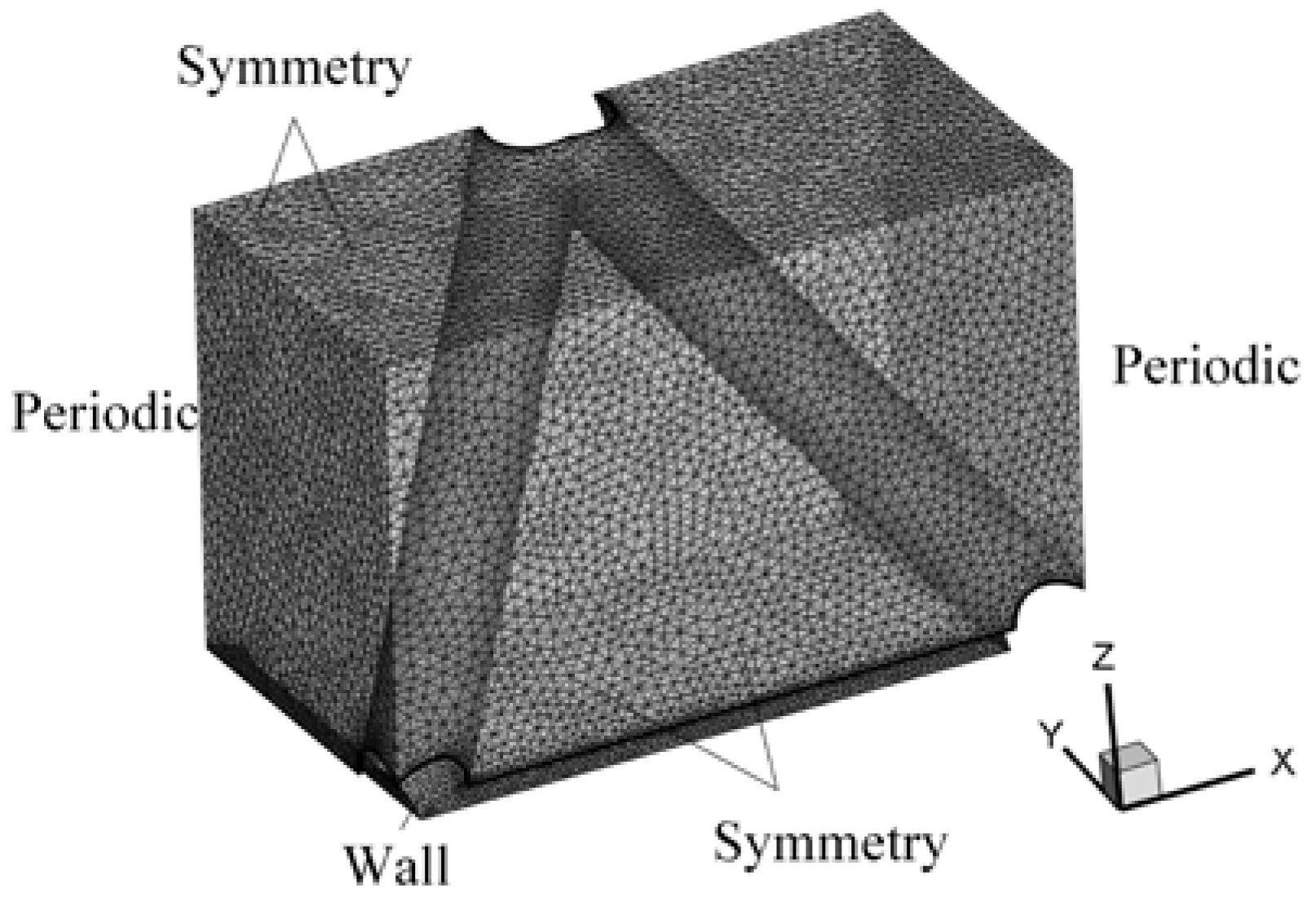
2.2.1. Grid Generation and Boundary Conditions
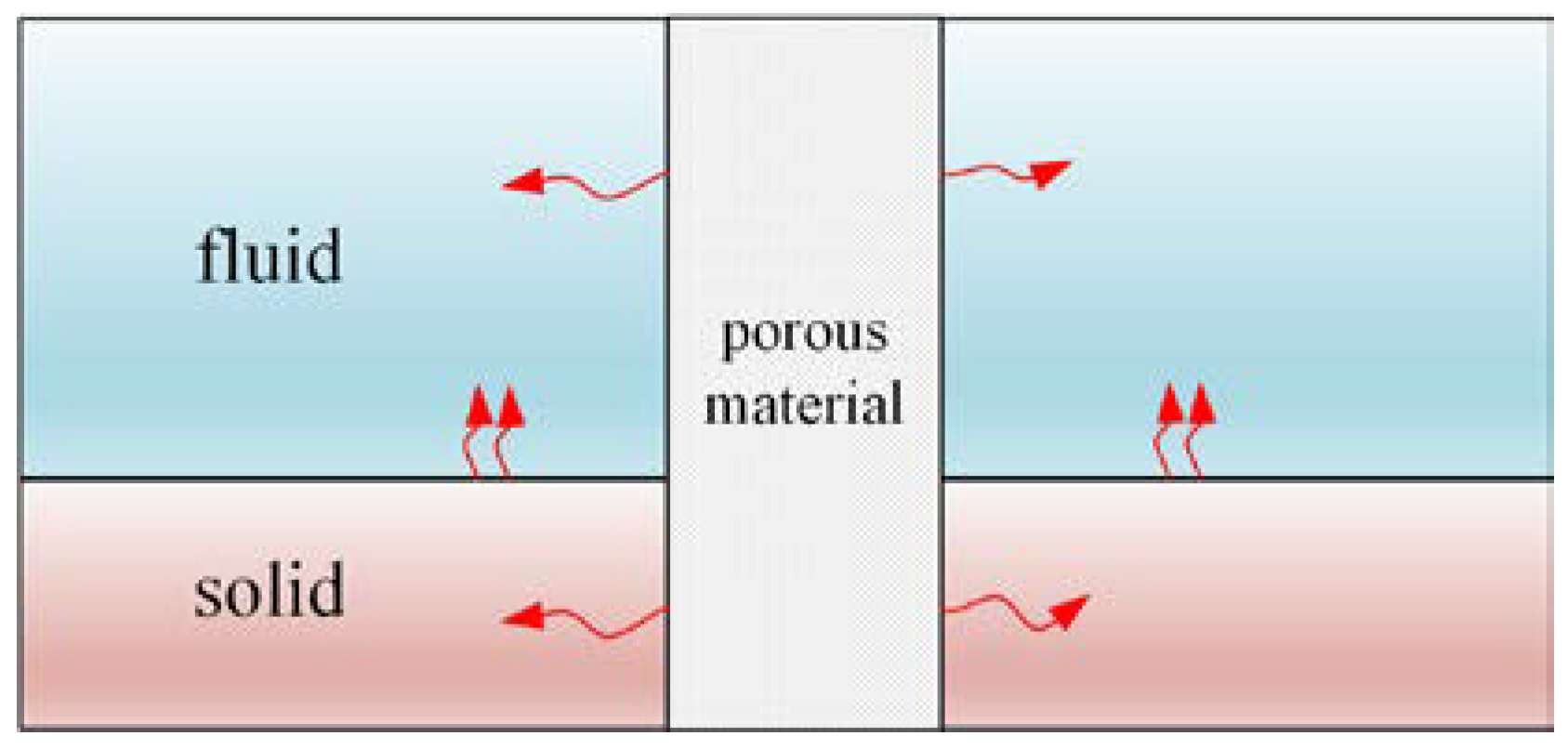
2.2.2. Flow and Heat Transfer Modeling
2.2.3. Parameters Needed for Porous Media Modeling
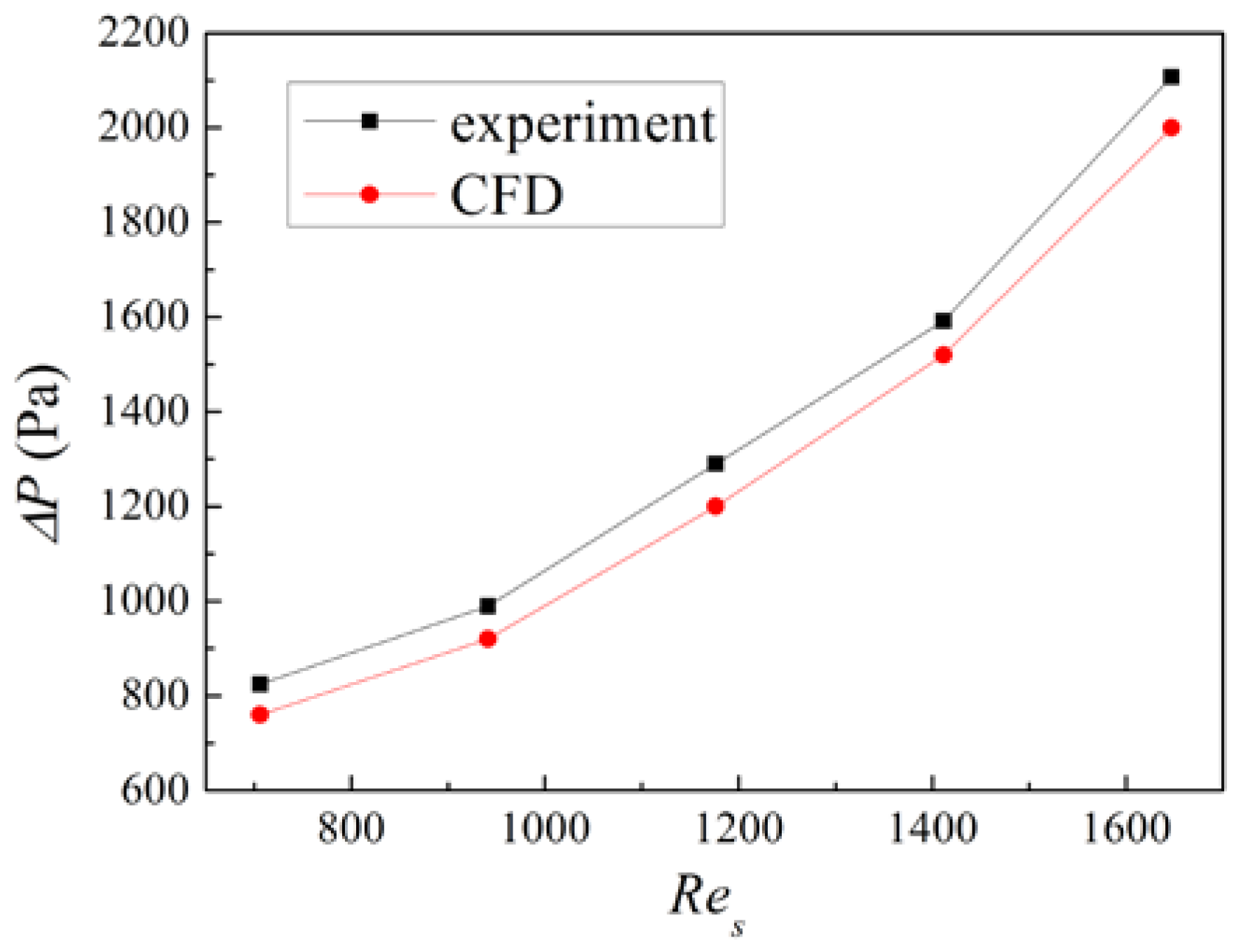
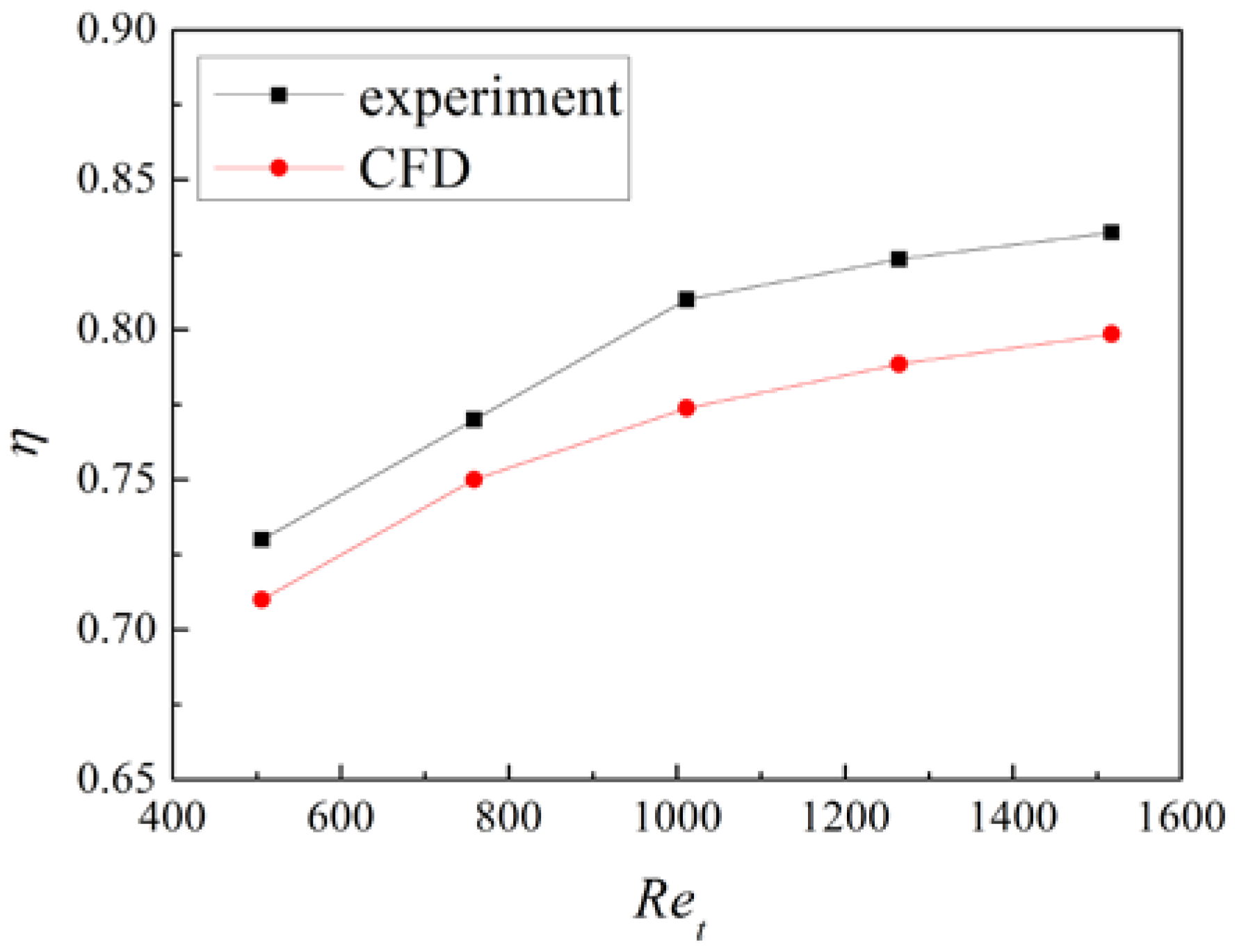
3. Validation of the Method
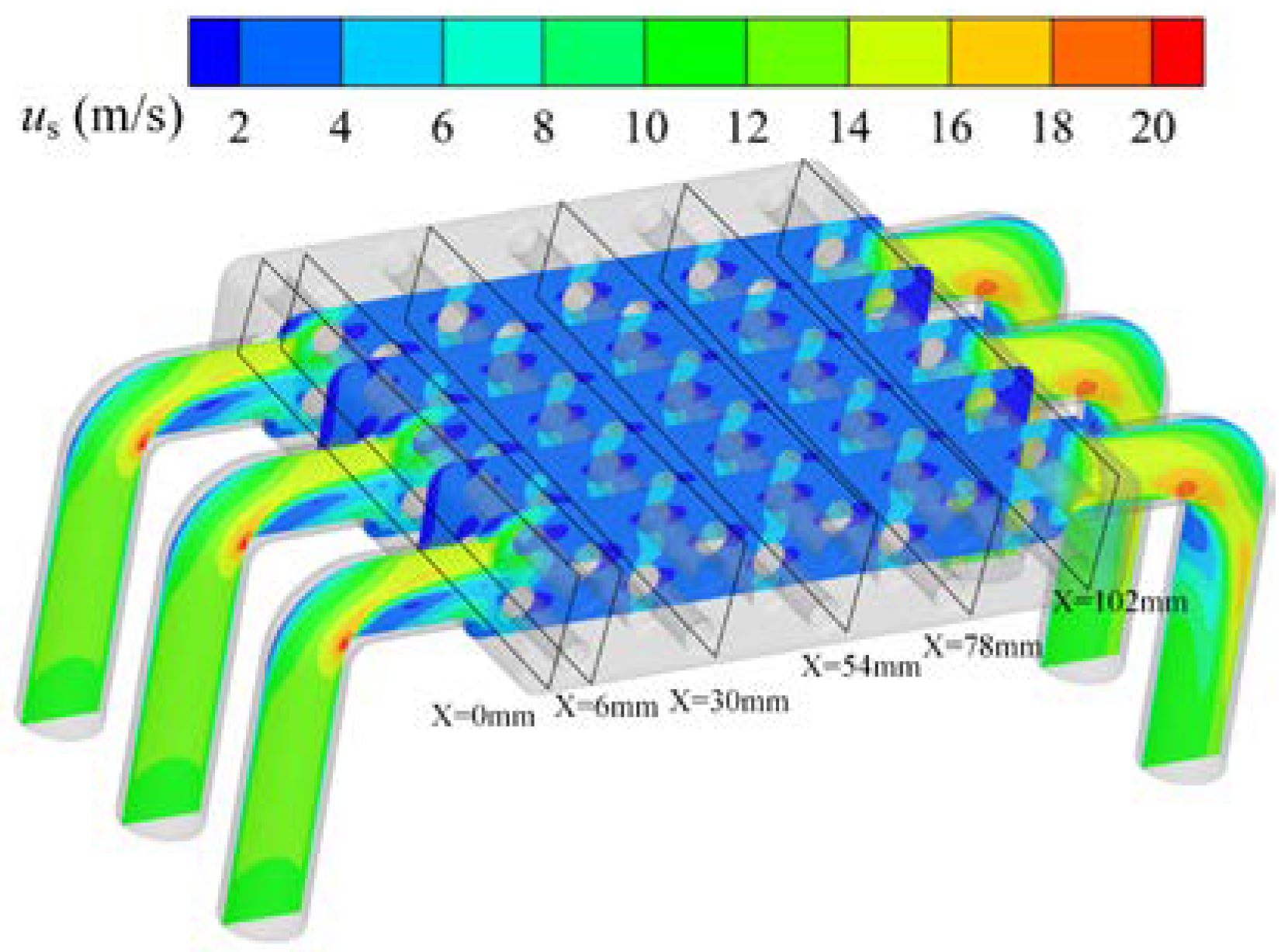
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Williams, M.; Muley, A.; Bolla, J.; Strumpf, H. Advanced Heat Exchanger Technology for Aerospace Applications; SAE International: Warrendale, PA, USA, 2008; pp. 2688–3627. [Google Scholar] [CrossRef]
- Han, X.H.; Wang, Q.; Park, Y.G.; T’Joen, C.; Sommers, A.; Jacobi, A. A Review of Metal Foam and Metal Matrix Composites for Heat Exchangers and Heat Sinks. Heat Transf. Eng. 2012, 33, 991–1009. [Google Scholar] [CrossRef] [Green Version]
- Smith, B.H.; Szyniszewski, S.; Hajjar, J.F.; Schafer, B.W.; Arwade, S.R. Steel Foam for Structures: A Review of Applications, Manufacturing and Material Properties. J. Constr. Steel Res. 2012, 71, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Hung, T.C.; Yan, W.M. Optimization of Design Parameters for a Sandwich Distribution Porous Microchannel Heat Sink. Numer. Heat Transf. Part A Appl. 2014, 66, 29–251. [Google Scholar] [CrossRef]
- Son, K.N.; Weibel, J.A.; Kumaresan, V.; Garimella, S.V. Design of Multifunctional Lattice-Frame Materials for Compact Heat Exchangers. Int. J. Heat Mass Transf. 2017, 115, 619–629. [Google Scholar] [CrossRef] [Green Version]
- Krishnan, S.; Hernon, D.; Hodes, M.; Mullins, J.; Lyons, A.M. Design of Complex Structured Monolithic Heat Sinks for Enhanced Air Cooling. IEEE Trans. Components Packag. Manuf. Technol. 2012, 2, 266–277. [Google Scholar] [CrossRef]
- Ren, Y.X.; Yu, X.; Zhang, X.Z.; Cao, M.G.; Duo, L.; Qiu, Q.G. Flow and Heat Transfer Characteristics of a Ultra lighted and Efficient Porous Media Heat Exchanger. J. Nanjing Univ. Aeronaut. Astronaut. 2019, 4, 449–455. [Google Scholar] [CrossRef]
- Prithiviraj, M.; Andrews, M.J. Three-dimensional Numerical Simulation of Shell-and-tube Heat Exchangers. Part II: Heat transfer. Numer. Heat Transf. Part A Appl. 1998, 33, 817–828. [Google Scholar] [CrossRef]
- Boomsma, K.; Poulikakos, D.; Ventikos, Y. Simulations of Flow through Open Cell Metal Foams Using an Idealized Periodic Cell Structure. International. J. Heat Fluid Flow 2003, 24, 825–834. [Google Scholar] [CrossRef]
- Heltzel, A. Simulation of Emerging Heat Exchanger Technologies for Progressive Aerospace Platforms. In Proceedings of the 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010; American Institute of Aeronautics and Astronautics: Orlando, FL, USA, 2010. [Google Scholar] [CrossRef]
- Dong, M.; Xie, M.Z. Numerical Investigation on Mass Dispersion in Turbulent Flows through Porous Media with High Porosity. Numer. Heat Transf. Part A Appl. 2015, 67, 293–312. [Google Scholar] [CrossRef]
- Wang, X.; Thauvin, F.; Mohanty, K.K. Non-Darcy Flow through Anisotropic Porous Media. Chem. Eng. Sci. 1999, 54, 1859–1869. [Google Scholar] [CrossRef]
- Prithiviraj, M.; Andrews, M.J. Three-dimensional Numerical Simulation of Shell-and-tube Heat Exchangers. Part I: Foundation and fluid mechanics. Numer. Heat Transf. Part A Appl. 1998, 33, 799–816. [Google Scholar] [CrossRef]
- Missirlis, D.; Yakinthos, K.; Storm, P.; Goulas, A. Modeling Pressure Drop of Inclined Flow through a Heat Exchanger for Aero-Engine Applications. Int. J. Heat Fluid Flow 2007, 28, 512–515. [Google Scholar] [CrossRef]
- Yakinthos, K.; Donnerhack, S.; Missirlis, D.; Seite, O.; Storm, P. Derivation of an Anisotropic Model for the Pressure Loss Through a Heat Exchanger for Aero Engine Applications. In Proceedings of the Volume 5: Microturbines and Small Turbomachinery, Orlando, FL, USA, 8–12 June 2009; ASME: Orlando, FL, USA, 2009; pp. 221–229. [Google Scholar] [CrossRef]
- Da Silva Miranda, B.M.; Anand, N.K. Convective Heat Transfer in a Channel with Porous Baffles. Numer. Heat Transf. Part A Appl. 2004, 46, 425–452. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer: New York, NY, USA, 2013; ISBN 978-1-4614-5541-7. [Google Scholar]
- Lin, W.; Xie, G.; Yuan, J.; Sundén, B. Comparison and Analysis of Heat Transfer in Aluminum Foam Using Local Thermal Equilibrium or Nonequilibrium Model. Heat Transf. Eng. 2016, 37, 314–322. [Google Scholar] [CrossRef]
- Wang, H.; Guo, L. Volumetric Convective Heat Transfer Coefficient Model for Metal Foams. Heat Transf. Eng. 2019, 40, 464–475. [Google Scholar] [CrossRef]
- Alazmi, B.; Vafai, K. Constant Wall Heat Flux Boundary Conditions in Porous Media under Local Thermal Non-Equilibrium Conditions. Int. J. Heat Mass Transf. 2002, 45, 3071–3087. [Google Scholar] [CrossRef]
- Ouyang, X.L.; Jiang, P.X.; Xu, R.N. Thermal Boundary Conditions of Local Thermal Non-Equilibrium Model for Convection Heat Transfer in Porous Media. Int. J. Heat Mass Transf. 2013, 60, 31–40. [Google Scholar] [CrossRef]
- Trilok, G.; Kumar, K.K.; Gnanasekaran, N.; Mobedi, M. Numerical Assessment of Thermal Characteristics of Metal Foams of Orderly Varied Pore Density and Porosity under Different Convection Regimes. Int. J. Therm. Sci. 2022, 172, 107288. [Google Scholar] [CrossRef]
- Jadhav, P.H.; Trilok, G.; Gnanasekaran, N.; Mobedi, M. Performance Score Based Multi-Objective Optimization for Thermal Design of Partially Filled High Porosity Metal Foam Pipes under Forced Convection. Int. J. Heat Mass Transf. 2022, 182, 121911. [Google Scholar] [CrossRef]
- ANSYS. Ansys Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]

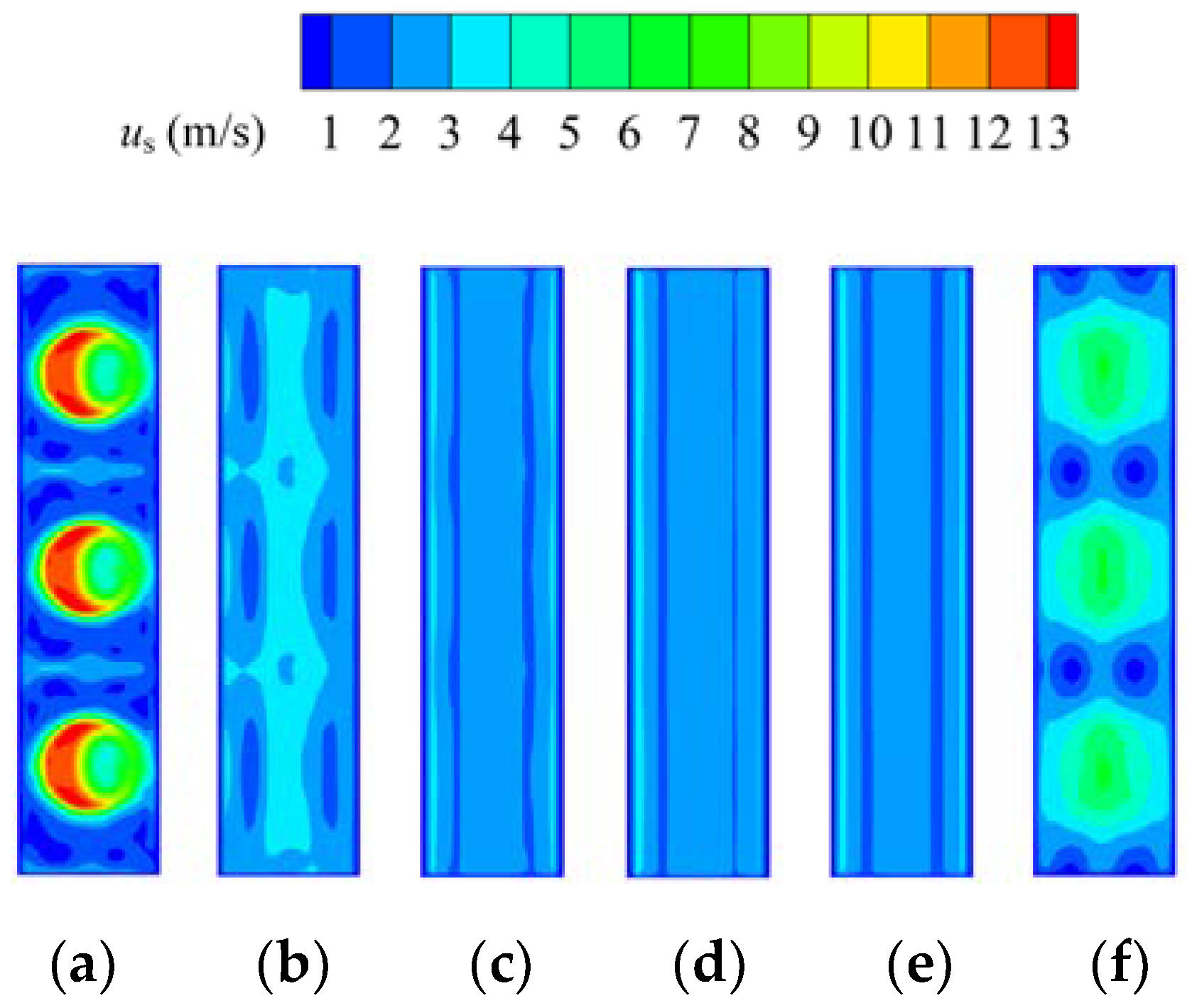

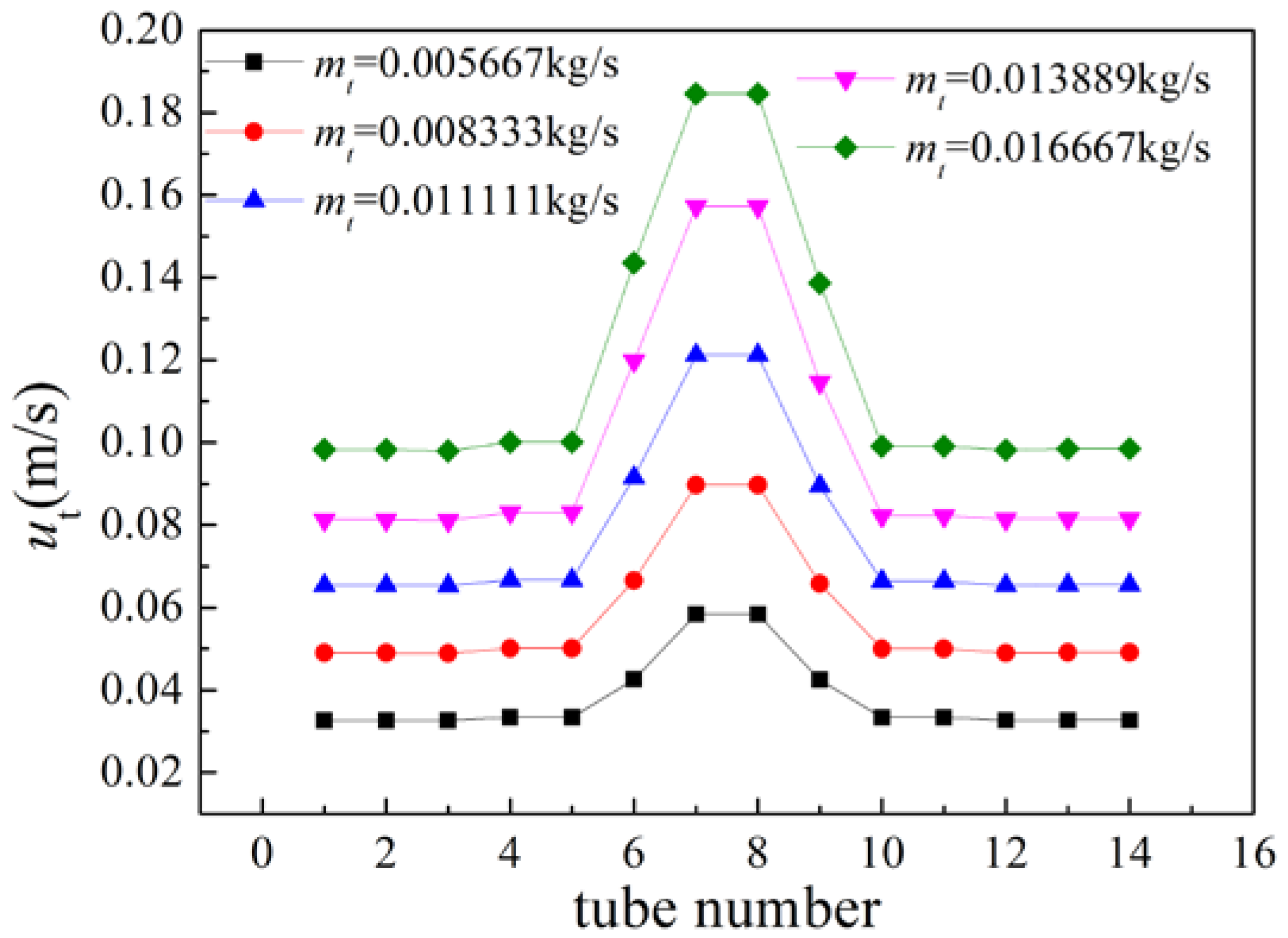

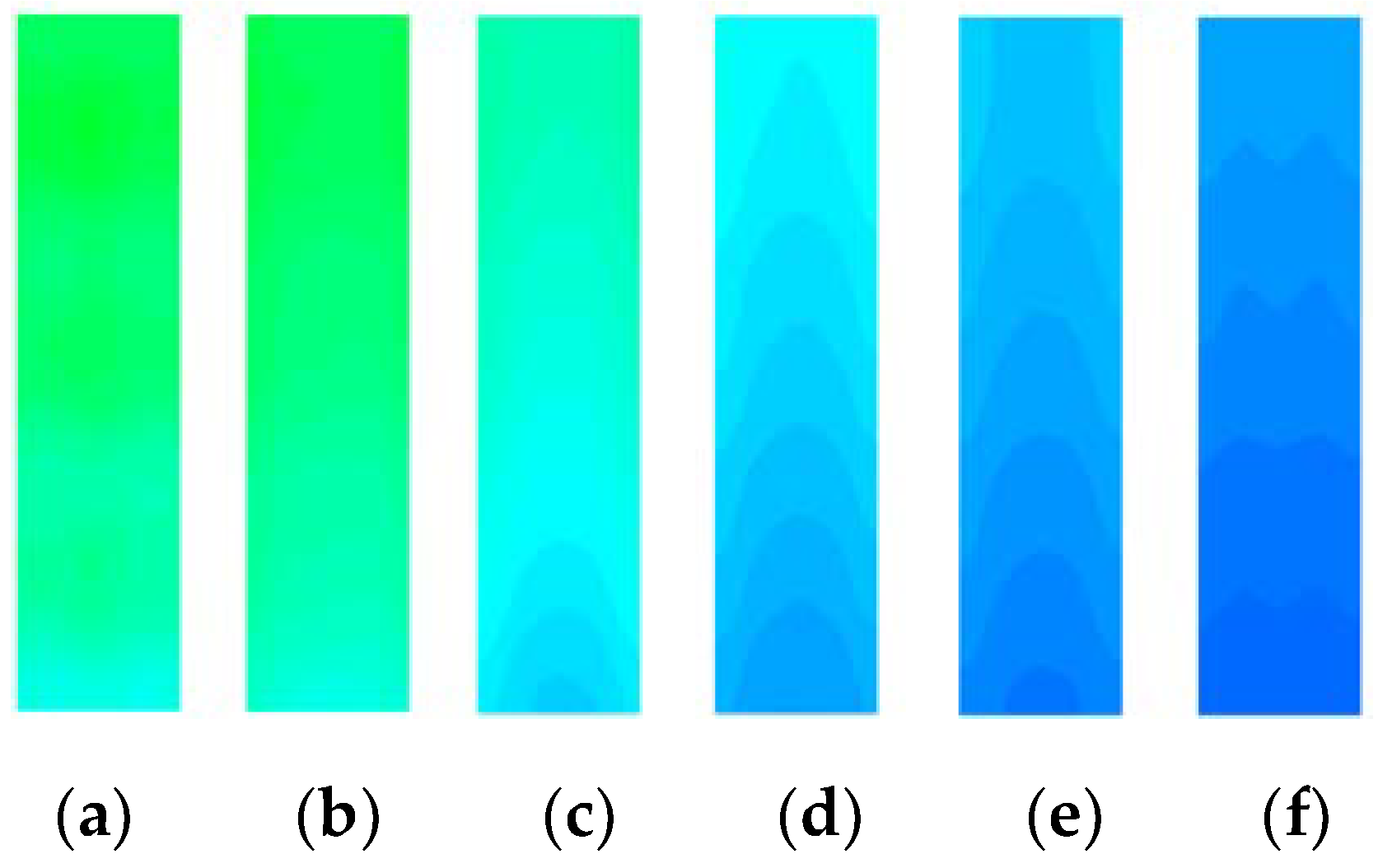

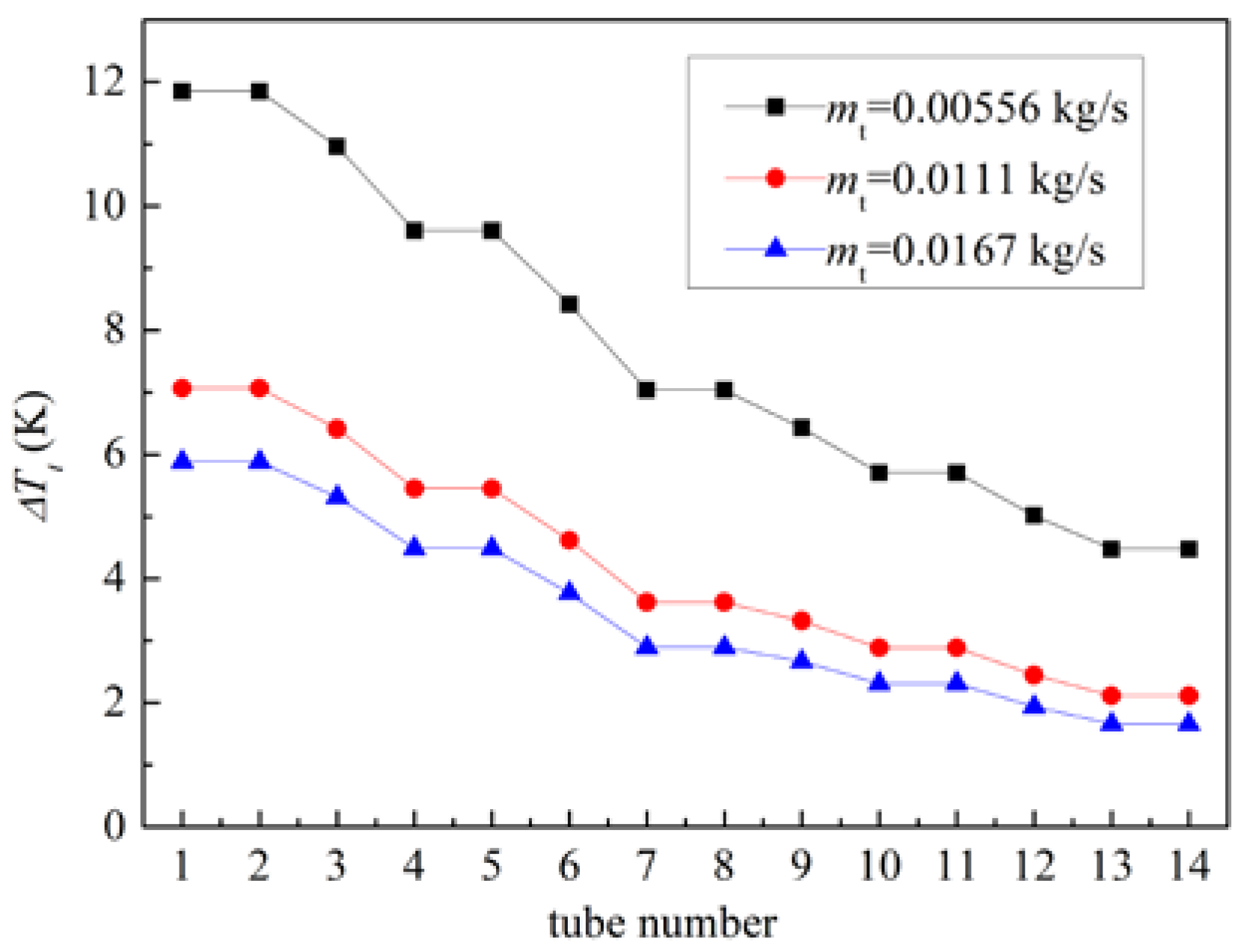


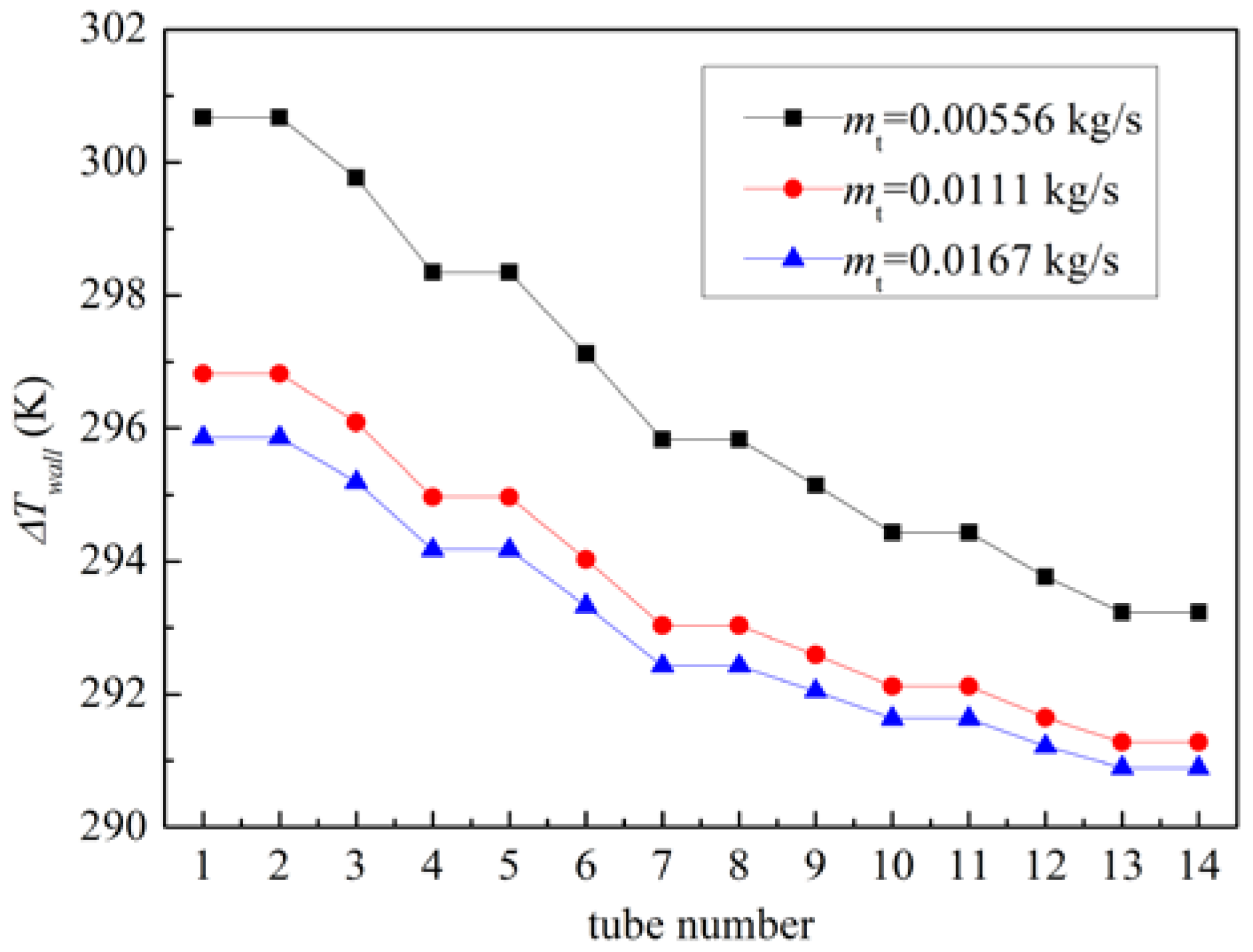
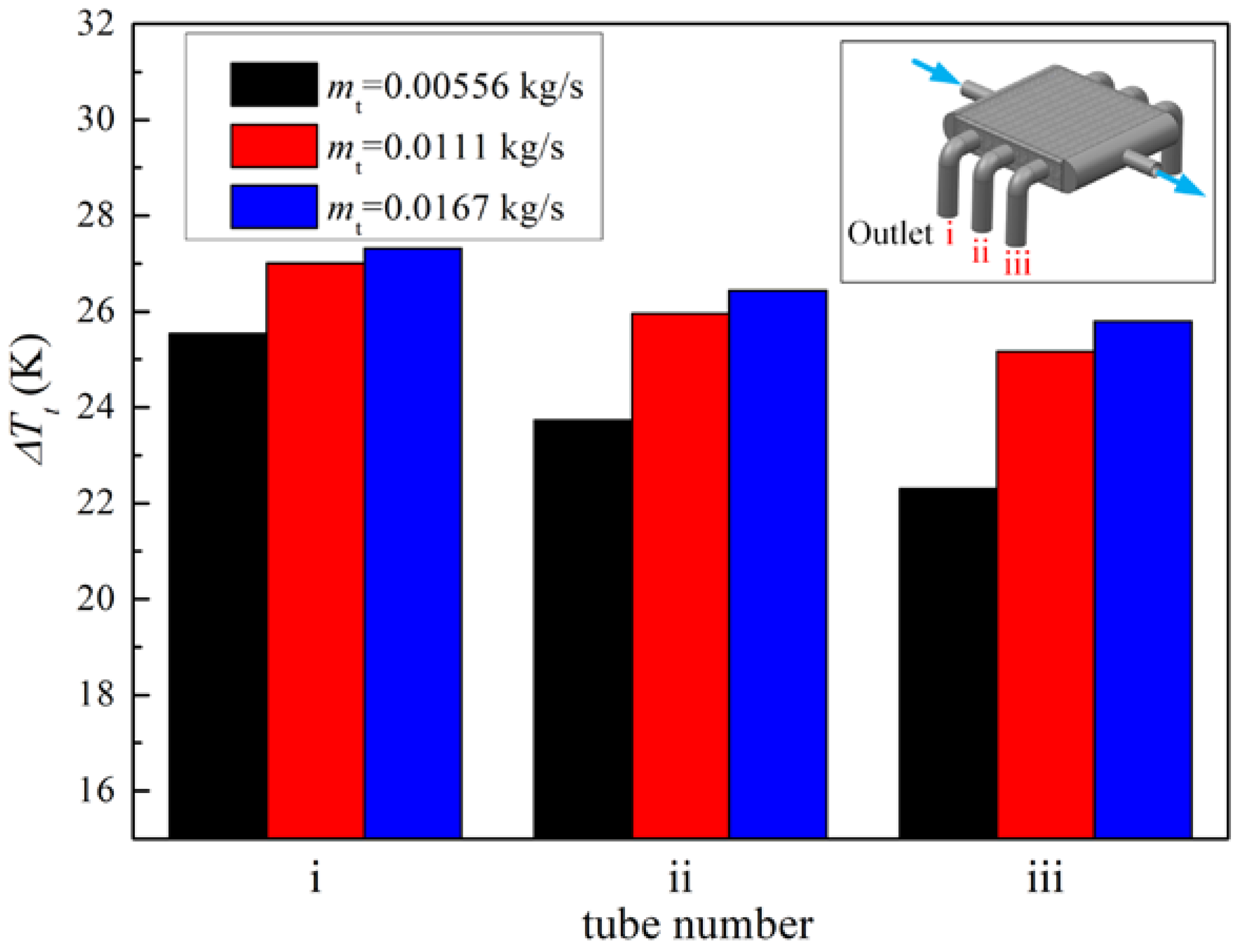
| Boundary Condition Type | Value | |
|---|---|---|
| Shell side walls | Adiabatic wall | none |
| Main tubes walls | ||
| Tube walls | Coupled wall | none |
| Water inlet | Mass flow inlet | 289 K, 0.00556 to 0.01667 kg/s |
| Air inlet | Mass flow inlet | 323 K, 0.00833 kg/s (hot condition) 293 K, 0.005 to 0.0116 kg/s (cold condition) |
| Water outlet | Pressure outlet | 101,325 Pa |
| Air outlet | Pressure outlet | 101,325 Pa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, B.; Zhang, J.; Lian, W. Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material. Aerospace 2022, 9, 238. https://doi.org/10.3390/aerospace9050238
Zhao B, Zhang J, Lian W. Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material. Aerospace. 2022; 9(5):238. https://doi.org/10.3390/aerospace9050238
Chicago/Turabian StyleZhao, Bi, Jingzhou Zhang, and Wenlei Lian. 2022. "Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material" Aerospace 9, no. 5: 238. https://doi.org/10.3390/aerospace9050238
APA StyleZhao, B., Zhang, J., & Lian, W. (2022). Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material. Aerospace, 9(5), 238. https://doi.org/10.3390/aerospace9050238





