Evaluation of Series and Parallel Hybrid Propulsion Systems for UAVs Implementing Distributed Propulsion Architectures
Abstract
:1. Introduction
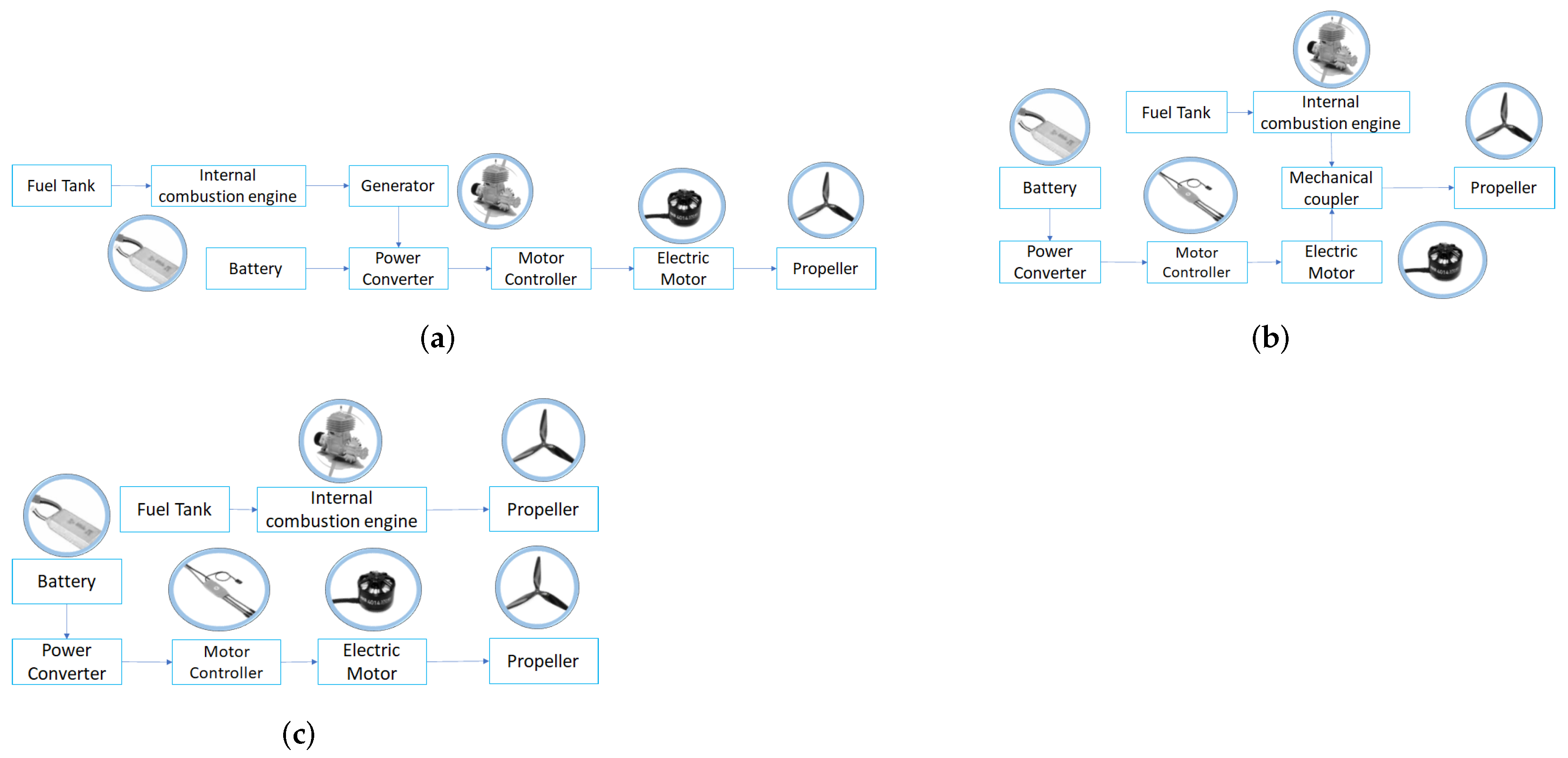
Hybrid Architectures
- Higher MTOM due to the use of a generator.
- Energy losses as the ICE mechanical energy is transformed into electric energy.
- Higher fuel consumption than parallel powertrain.
- Complex powertrain controllers.
- In some cases, a mechanical coupler is needed (see Figure 1b).
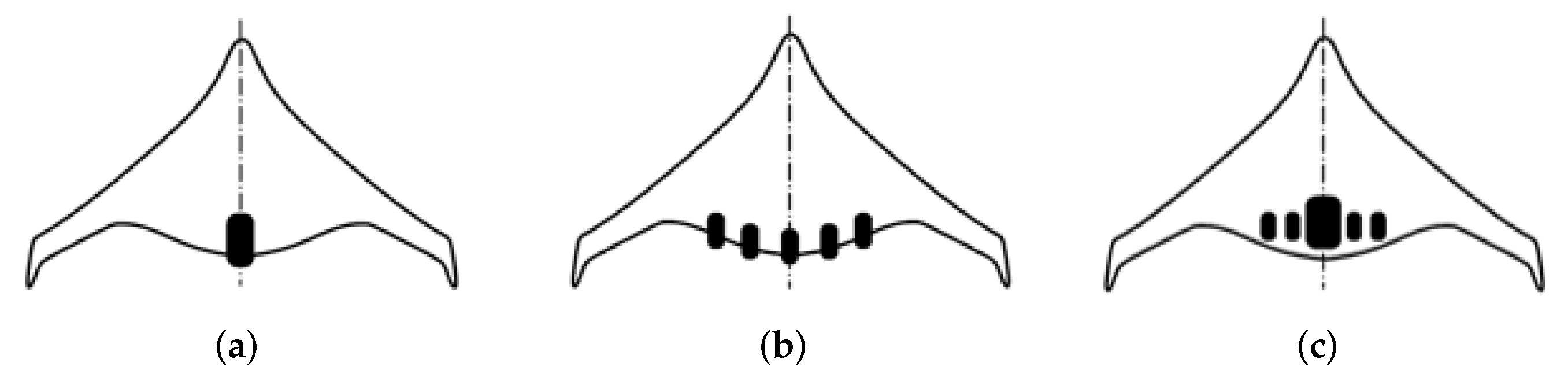
- Higher complexity of distributed propulsion implementation than series powertrain.
2. Methodology
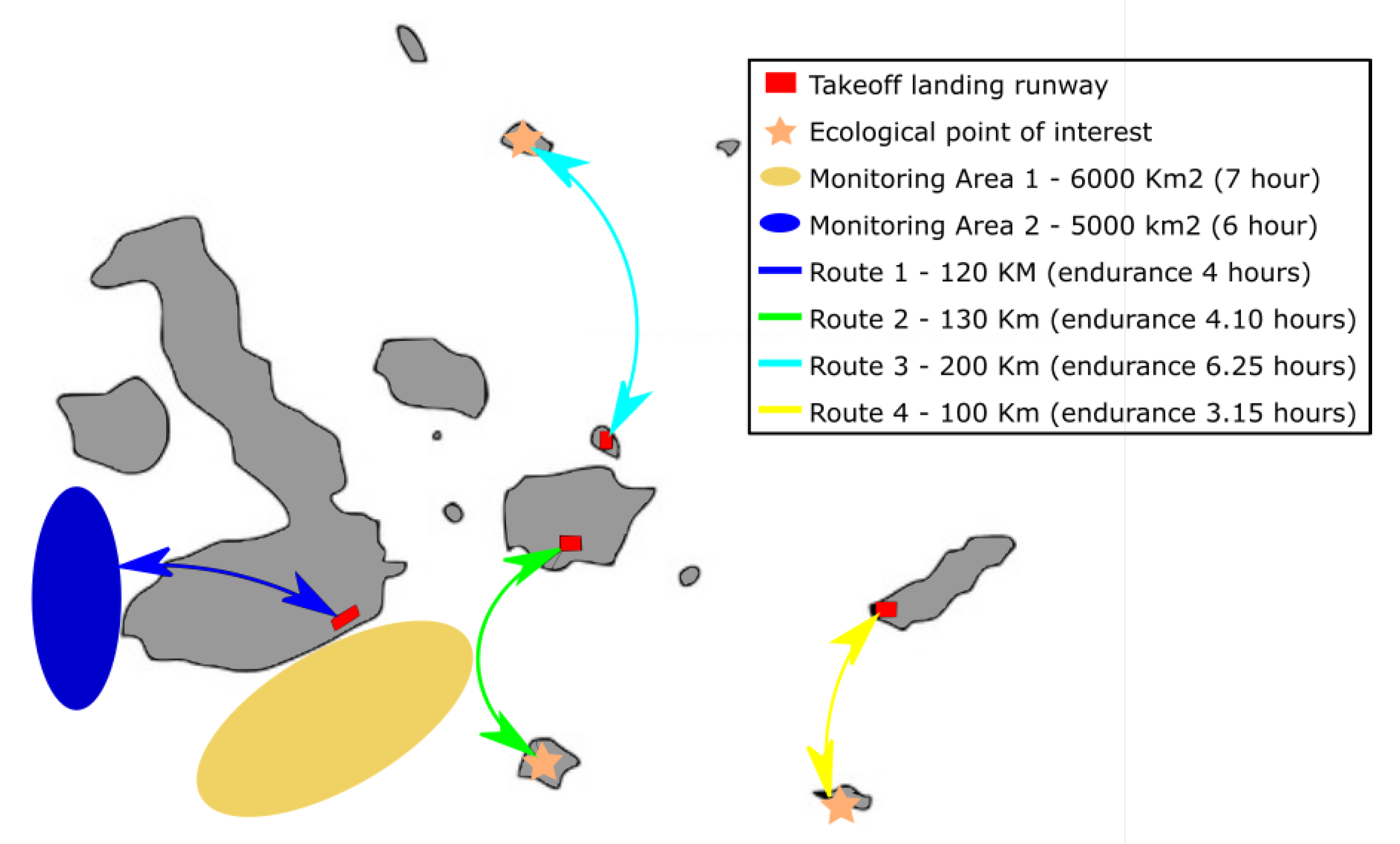
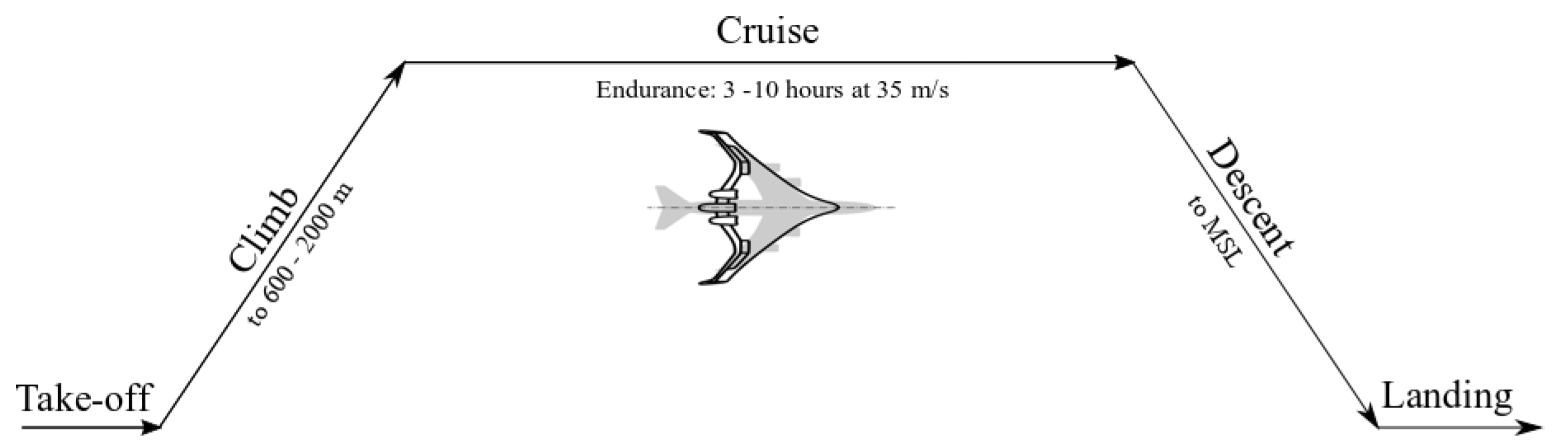
2.1. Design Requirements: Mission Analysis
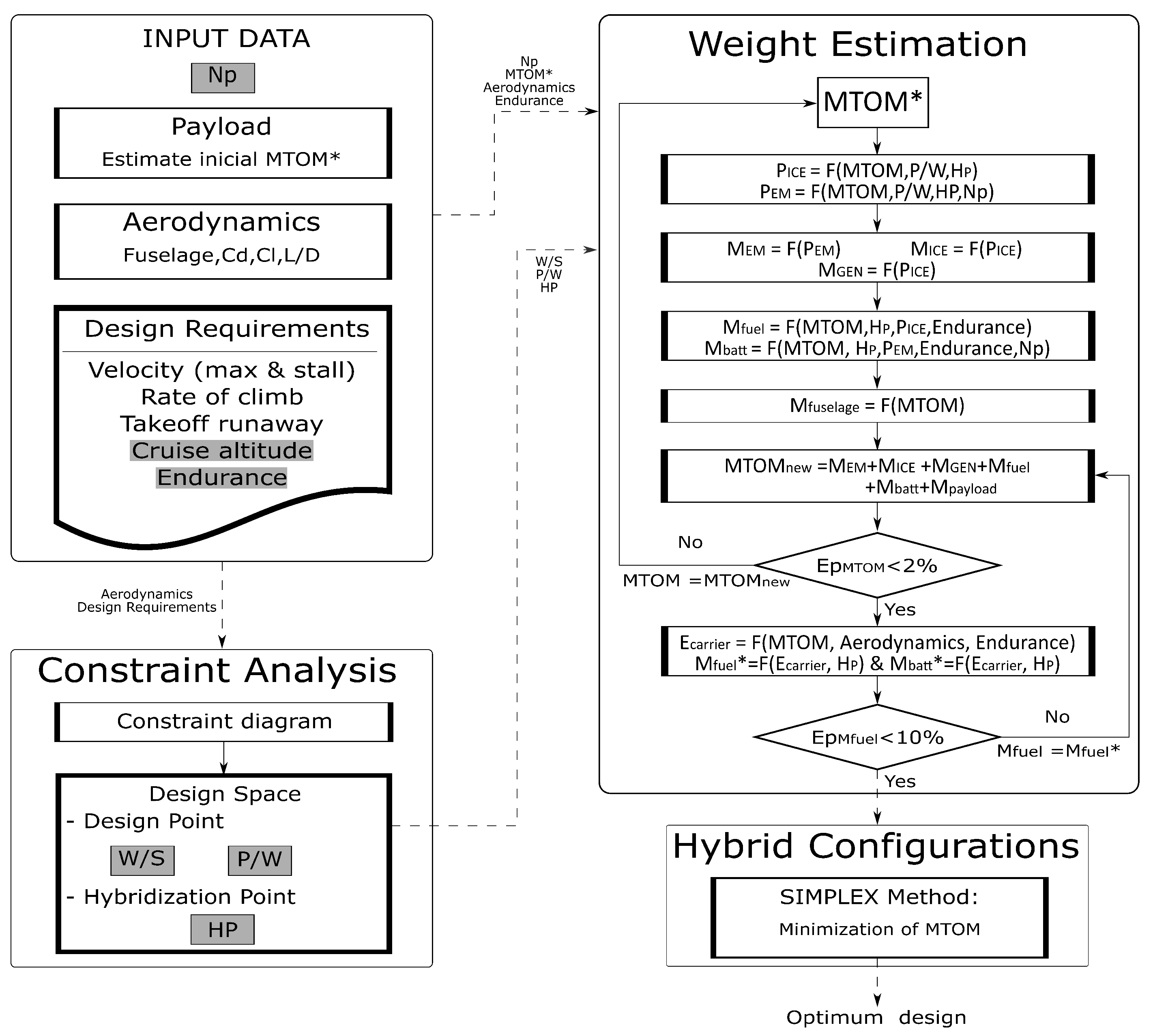
2.2. Framework of Analysis
2.2.1. Conceptual Design of the Hybrid-Electric UAV
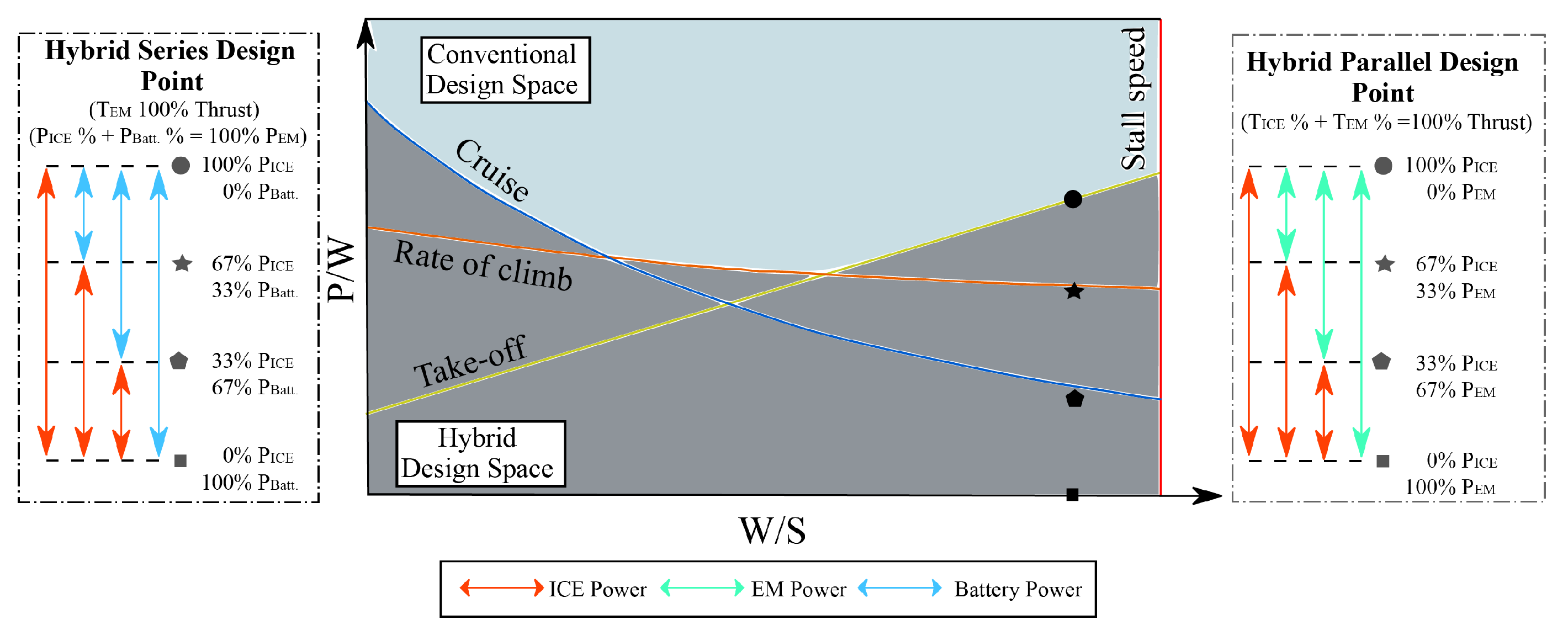
Constraint Analysis
Degree of Hybridization
2.2.2. Preliminary Weight Analysis of the Hybrid-Electric UAV
Electric motor and Generator
Battery Package
Power Converter
ICE Selection
- ICE performance: In order to obtain more accurate results due to the ICE performance decrease with the increase in the operation altitude, the Gagg and Ferrar equation, which is used for engines driving a constant-speed propeller, was employed to determine the engine’s power fall-off as a function of density [38,39].
- Benchmarking analysis: The study considered an Otto’s cycle ICE of two strokes. These types of ICE have high power density, low weight, low costs, compact dimensions, high efficiency, and great reliability, which are the key factors in designing and sizing propulsion systems [40]. In this context, the model used to size the propulsion system is based on a semi-empirical model presented in ref. [34] (see Equation (7)) and consists of an empirical correlation based on a database of 114 two stroke engines.where is the ICE mass (Kg), is the output ICE power required (W), and A and B stand for coefficients of ICE engines weight presented in Table 3.
Empty Weight Fraction
2.2.3. Mission Energy Consumption Schedule
Fuel Consumption
2.2.4. Propulsion Schemes
3. Results and Discussion
3.1. Baseline Reference Model
3.2. Constraint Analysis
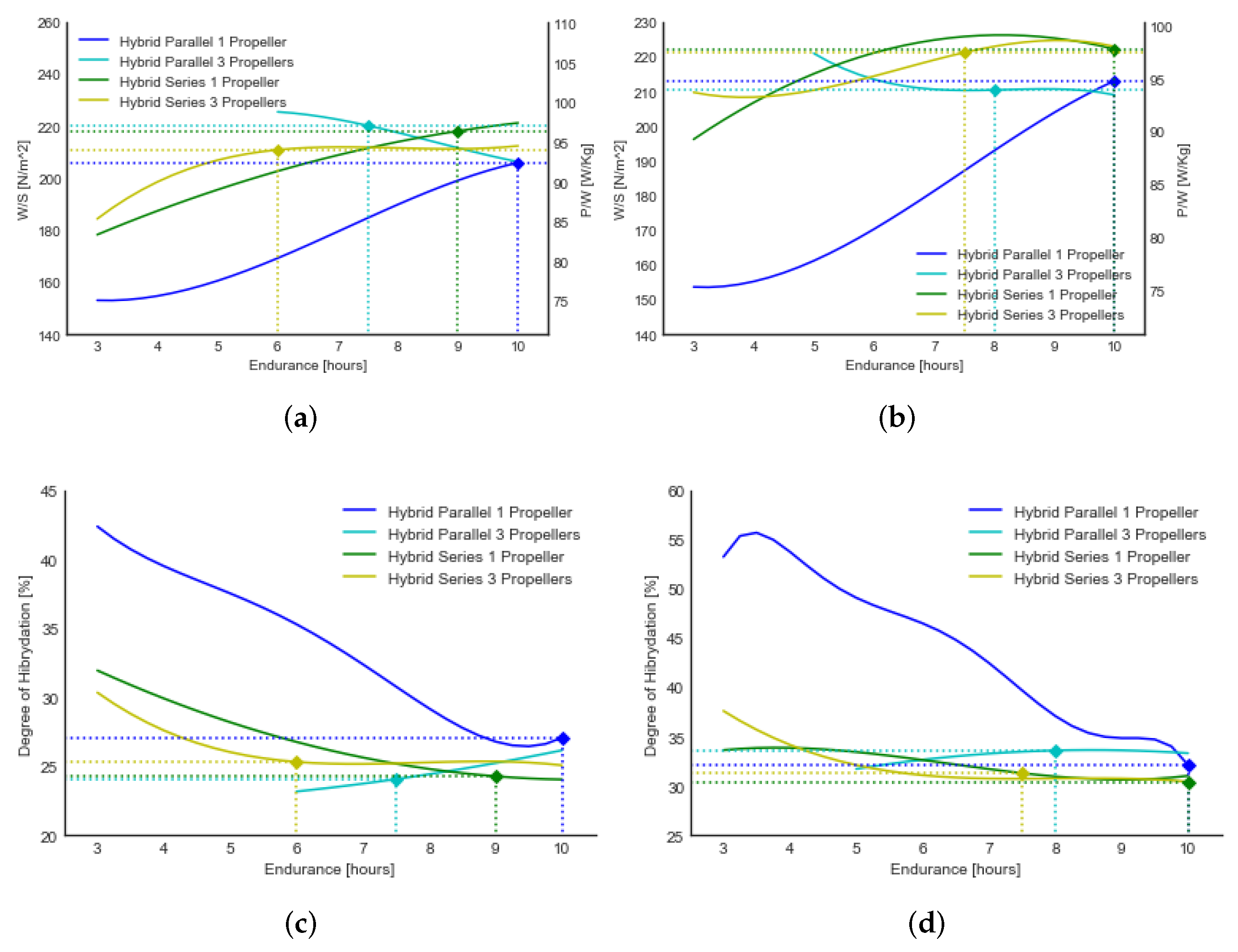
3.3. Hybrid Configurations Performance
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AR | Aspect Ratio |
| BWB | Blended Wing Body |
| EM | Electric Motor |
| Cruise altitude | |
| HP | Hybrid point |
| ICE | Internal Combustion Engine |
| Batteries mass | |
| Electric motor mass | |
| Fuselage mass | |
| Fuel mass | |
| Generator mass | |
| Internal combustion engine mass | |
| MTOM | Maximum take-off mass |
| Np | Number of propellers |
| Power electric motor | |
| Power internal combustion engine | |
| P/W | Power to weight ratio |
| ROC | Rate of climb |
| SFC | Specific fuel consumption |
| STO | Take-off runaway |
| Wing reference Area | |
| UAV | Unmanned Aerial Vehicle |
| Cruise Speed | |
| Stall Speed | |
| W/S | Wing loading |
References
- Panagiotou, P.; Tsavlidis, I.; Yakinthos, K. Conceptual design of a hybrid solar MALE UAV. Aerosp. Sci. Technol. 2016, 53, 207–219. [Google Scholar] [CrossRef]
- Austin, R. Unmanned Aircraft Systems: UAVS Design, Development and Deployment; John Wiley & Sons Ltd.: Chichester, UK, 2011; Volume 54. [Google Scholar]
- Ashcraft, S.W.; Padron, A.S.; Pascioni, K.A.; Stout, G.W., Jr.; Huff, D.L. Review of Propulsion Technologies for N+ 3 Subsonic Vehicle Concepts; NASA Glenn Research Center: Cleveland, OH, USA, 2011.
- Riboldi, C.E.; Gualdoni, F.; Trainelli, L. Preliminary weight sizing of light pure-electric and hybrid-electric aircraft. Transp. Res. Procedia 2018, 29, 376–389. [Google Scholar] [CrossRef]
- Hiserote, R.; Harmon, F. Analysis of hybrid-electric propulsion system designs for small unmanned aircraft systems. In Proceedings of the 8th Annual International Energy Conversion Engineering Conference, Nashville, TN, USA, 25–28 July 2010; p. 6687. [Google Scholar]
- Friedrich, C.; Robertson, P.A. Hybrid-electric propulsion for aircraft. J. Aircr. 2015, 52, 176–189. [Google Scholar] [CrossRef]
- Cinar, G.; Mavris, D.N.; Emeneth, M.; Schneegans, A.; Riediger, C.; Fefermann, Y.; Isikveren, A. Sizing, integration and performance evaluation of hybrid electric propulsion subsystem architectures. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017; p. 1183. [Google Scholar]
- Finger, D.F.; Braun, C.; Bil, C. An initial sizing methodology for hybrid-electric light aircraft. In Proceedings of the 2018 Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 25–29 June 2018; p. 4229. [Google Scholar]
- Xie, Y.; Savvaris, A.; Tsourdos, A.; Laycock, J.; Farmer, A. Modelling and control of a hybrid electric propulsion system for unmanned aerial vehicles. In Proceedings of the 2018 IEEE Aerospace Conference, Big Sky, MT, USA, 3–10 March 2018; pp. 1–13. [Google Scholar]
- De Vries, R.; Brown, M.; Vos, R. Preliminary sizing method for hybrid-electric distributed-propulsion aircraft. J. Aircr. 2019, 56, 2172–2188. [Google Scholar] [CrossRef]
- Rohacs, J.; Rohacs, D. Conceptual design method adapted to electric/hybrid aircraft developments. Int. J. Sustain. Aviat. 2019, 5, 175–189. [Google Scholar] [CrossRef]
- Finger, D.F.; de Vries, R.; Vos, R.; Braun, C.; Bil, C. A comparison of hybrid-electric aircraft sizing methods. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; p. 1006. [Google Scholar]
- Bravo, G.M.; Praliyev, N.; Veress, Á. Performance analysis of hybrid electric and distributed propulsion system applied on a light aircraft. Energy 2021, 214, 118823. [Google Scholar] [CrossRef]
- Zhao, H.; Burke, A. Modelling and Analysis of Plug-in Series-Parallel Hybrid Medium-Duty Vehicles; ITS UC: Davis, CA, USA, 2015. [Google Scholar]
- Finger, D.F.; Braurr, C.; Bil, C. Case studies in initial sizing for hybrid-electric general aviation aircraft. In Proceedings of the 2018 AIAA/IEEE Electric Aircraft Technologies Symposium (EATS), Cincinnati, OH, USA, 12–14 July 2018; pp. 1–22. [Google Scholar]
- Hung, J.; Gonzalez, L.F. On parallel hybrid-electric propulsion system for unmanned aerial vehicles. Prog. Aerosp. Sci. 2012, 51, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Olsen, J.; Page, J.R. Hybrid powertrain for light aircraft. Int. J. Sustain. Aviat. 15 2014, 1, 85–102. [Google Scholar] [CrossRef]
- Valencia, E.A.; Jimenez, D.; Alulema, V.H.; Roumeliotis, I.; Montalvan, J.; Pozo, M.; Cando, E. Modeling of a series hybrid propulsion UAV used for monitoring in the Galapagos Islands. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, virtual event, 24–28 August 2020; p. 3960. [Google Scholar]
- Friedrich, C.; Robertson, P.A. Design of hybrid-electric propulsion systems for light aircraft. In Proceedings of the 14th AIAA Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 16–20 June 2014; p. 3008. [Google Scholar]
- Kim, H.D. Distributed propulsion vehicles. In Proceedings of the 27th International Congress of the Aeronautical Sciences, Nice, France, 19–24 September 2010; pp. 2010–2011. [Google Scholar]
- Carr, L.A.; Stier, A.C.; Fietz, K.; Montero, I.; Gallagher, A.J.; Bruno, J.F. Illegal shark fishing in the Galapagos Marine Reserve. Mar. Policy 2013, 39, 317–321. [Google Scholar] [CrossRef]
- Schiller, L.; Alava, J.J.; Grove, J.; Reck, G.; Pauly, D. The demise of Darwin’s fishes: Evidence of fishing down and illegal shark finning in the Galapagos Islands. Aquat. Conserv. Mar. Freshw. Ecosyst. 2015, 25, 431–446. [Google Scholar] [CrossRef]
- Traveset, A.; Heleno, R.; Chamorro, S.; Vargas, P.; McMullen, C.K.; Castro-Urgal, R.; Nogales, M.; Herrera, H.W.; Olesen, J.M. Invaders of pollination networks in the Galápagos Islands: Emergence of novel communities. Proc. R. Soc. B Biol. Sci. 2013, 280, 20123040. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Weimerskirch, H.; Bishop, C.; Jeanniard-du Dot, T.; Prudor, A.; Sachs, G. Frigate birds track atmospheric conditions over months-long transoceanic flights. Science 2016, 353, 74–78. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jackson, M.H. Galapagos, a Natural History; University of Calgary Press: Calgary, AB, USA, 1993. [Google Scholar]
- Harris, M.P.; Mackay, B.K. A Field Guide to the Birds of Galápagos; Collins: London, UK, 1974. [Google Scholar]
- Sadraey, M.H. Aircraft Design: A Systems Engineering Approach; John Wiley & Sons Ltd.: Chichester, UK, 2012. [Google Scholar]
- Fitzgerald, A.E.; Kingsley, C.; Umans, S.D.; James, B. Electric Machinery; McGraw-Hill: New York, NY, USA, 2003; Volume 5. [Google Scholar]
- Cirigliano, D.; Frisch, A.M.; Liu, F.; Sirignano, W.A. Diesel, spark-ignition, and turboprop engines for long-duration unmanned air flights. J. Propuls. Power 2018, 34, 878–892. [Google Scholar] [CrossRef]
- Fotouhi, A.; Auger, D.J.; Propp, K.; Longo, S.; Wild, M. A review on electric vehicle battery modelling: From Lithium-ion toward Lithium–Sulphur. Renew. Sustain. Energy Rev. 2016, 56, 1008–1021. [Google Scholar] [CrossRef] [Green Version]
- Kuhn, E.; Forgez, C.; Lagonotte, P.; Friedrich, G. Modelling Ni-mH battery using Cauer and Foster structures. J. Power Sources 2006, 158, 1490–1497. [Google Scholar] [CrossRef]
- Gao, X.P.; Yang, H.X. Multi-electron reaction materials for high energy density batteries. Energy Environ. Sci. 2010, 3, 174–189. [Google Scholar] [CrossRef]
- Lieh, J.; Spahr, E.; Behbahani, A.; Hoying, J. Design of hybrid propulsion systems for unmanned aerial vehicles. In Proceedings of the 47th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, San Diego, CA, USA, 31 July–3 August 2011; p. 6146. [Google Scholar]
- Alulema, V.; Valencia, E.; Cando, E.; Hidalgo, V.; Rodriguez, D. Propulsion sizing correlations for electrical and fuel powered Unmanned Aerial Vehicles. Aerospace 2021, 8, 171. [Google Scholar] [CrossRef]
- Brombach, J.; Schröter, T.; Luecken, A.; Schulz, D. Optimizing the weight of an aircraft power supply system through a+/-270 VDC main voltage. Gen 2012, 360, 800. [Google Scholar]
- Hooper, P.R.; Al-Shemmeri, T.; Goodwin, M.J. An experimental and analytical investigation of a multi-fuel stepped piston engine. Appl. Therm. Eng. 2012, 48, 32–40. [Google Scholar] [CrossRef]
- Sadraey, M.H. Aircraft Performance: An Engineering Approach; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Miralles, J.; Giménez, A.; Domenech, L.; García, V. Efecto de la Relación de Compresión en el Rendimiento de Motores de Combustión Interna a diferentes Altitudes. Inf. Tecnol. 2015, 26, 63–74. [Google Scholar] [CrossRef] [Green Version]
- Gudmundsson, S. General Aviation Aircraft Design: Applied Methods and Procedures; Butterworth-Heinemann: Waltham, MA, USA, 2013. [Google Scholar]
- Cantore, G.; Mattarelli, E.; Rinaldini, C.A. A new design concept for 2-Stroke aircraft Diesel engines. Energy Procedia 2014, 45, 739–748. [Google Scholar] [CrossRef] [Green Version]
- Daniel, P.R. Aircraft Design: A Conceptual Approach; American Institute of Aeronautics and Astronautics Inc.: Reston, VA, USA, 2018; pp. 515–552. [Google Scholar]
- Ludowicy, J.; Rings, R.; Finger, D.; Braun, C. Sizing Studies of Light Aircraft with Serial Hybrid Propulsion Systems. Deutscher Luft-und Raumfahrtkongress DLRK 2018; DGLR: Friedrichshafen, Germany, 2018. [Google Scholar]
- Friedrich, C.; Robertson, P. Hybrid-electric propulsion for automotive and aviation applications. CEAS Aeronaut. J. 2015, 6, 279–290. [Google Scholar] [CrossRef] [Green Version]
- Wittmann, W. Flying with Siemens Integrated Drive System; IDT2013064084e; Siemens: Nuremberg, Germany, 2013. [Google Scholar]
- Martini, F. World’s First Serial Hybrid Electric Aircraft to Fly at Le Bourget; AXX20110666e; Siemens: Munich, Germany, 2011. [Google Scholar]

| Parameter | Min. Value | Max. Value |
|---|---|---|
| Cruise altitude (m) | 600 | 2000 |
| Take-off runaway (m) | 110 | 150 |
| Endurance (hours) | 3 | 10 |
| Cruise speed (m/s) | 20 | 40 |
| Payload (Kg) | 32 | 40 |
| • Cameras (Kg) | 2 | 3 |
| • Radar (Kg) | 16 | 20 |
| • Lidar (Kg) | 2 | 3 |
| • Others (Kg) * | 12 | 14 |
| Requirements | Aerodynamics | ||
|---|---|---|---|
| Stall Speed (m/s) | 15 | Efficiency Factor, e | 0.8 |
| Cruise Speed (m/s) | 35 | 1.63 | |
| Max. Cruise Altitude (m) | 2000 | CDo | 0.015 |
| Rate of Climb (m/s) | 4.5 | Aspect Ratio, AR | 4.15 |
| Take-off Runway (m) | 110 | ||
| A | B | R2 | n | |
|---|---|---|---|---|
| Two stroke | 0.0003 | 1.0530 | 0.8959 | 114 |
| Requirements | Aerodynamics | ||
|---|---|---|---|
| Stall Speed (m/s) | 25 | Efficiency Factor, e | 0.8 |
| Cruise Speed (m/s) | 55 | 2 | |
| Max. Cruise Altitude (m) | 1500 | CDo | 0.027 |
| Rate of Climb (m/s) | 4.5 | Aspect Ratio, AR | 8 |
| Take-off Runway (m) | 200 | ||
| 400 Km Mission | ||||||
| P-Hybrid | S-Hybrid | |||||
| Parameter | FH Model | U. Model | Diff. (%) | FH Model | U. Model | Diff. (%) |
| W/S (N/m2) | 748 | 728.08 | 2.7% | 748 | 708.92 | 5.2% |
| P/W (W/kg) | 139.7 | 134.46 | 3.8% | 139.7 | 131.50 | 5.9% |
| MTOM (kg) | 677 | 637.94 | 5.8% | 750 | 734.51 | 2.1% |
| MICE (kg) | 36.9 | 37.48 | 1.6% | 47.3 | 56.04 | 18.5% |
| MFuel (kg) | 25.3 | 27.23 | 7.6% | 32.3 | 33.74 | 4.5% |
| Sref (m2) | 8.88 | 8.59 | 3.3% | 9.84 | 10.16 | 3.3% |
| 1350 Km Mission | ||||||
| P-Hybrid | S-Hybrid | |||||
| Parameter | FH Model | U. Model | Diff. (%) | FH Model | U. Model | Diff. (%) |
| W/S (N/m2) | 750 | 758.73 | 1.2% | 750 | 763.33 | 1.8% |
| P/W (W/kg) | 140.2 | 139.18 | 0.7% | 140.2 | 139.89 | 0.2% |
| MTOM (kg) | 806 | 811.52 | 0.7% | 940 | 910.41 | 3.1% |
| MICE (kg) | 44 | 46.44 | 5.5% | 59.2 | 64.90 | 9.6% |
| MFuel (kg) | 82.1 | 86.41 | 5.2% | 110.3 | 118.12 | 7.1% |
| Sref (m2) | 10.54 | 10.49 | 0.5% | 12.3 | 11.70 | 4.9% |
| P-Hybrid | S-Hybrid | |||||
|---|---|---|---|---|---|---|
| U Model | HEPA Soul | Diff (%) | U Model | Da36 e Star | Diff (%) | |
| WS (N/m2) | 216 | 223.6 | 3.40% | 462.95 | 476 | 2.74% |
| PW (W/kg) | 79.22 | 85.1 | 6.91% | 90.20 | 90.91 | 0.78% |
| HP (%) | 47.27 | 40 | 18.18% | 47.12 | 42.86 | 9.94% |
| MTOM (kg) | 236.70 | 235 | 0.72% | 778.89 | 770 | 1.15% |
| PICE (KW) | 8.98 | 8 | 12.26% | 32.86 | 30 | 9.53% |
| PEM (KW) | 10.02 | 12 | 16.52% | 69.74 | 70 | 0.37% |
| Sref (m2) | 10.75 | 10.3 | 4.37% | 16.50 | 15.85 | 4.11% |
| b (m) | 11.44 | 11.2 | 2.17% | 16.65 | 16 | 4.07% |
| 600 M | 2000 M | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| One Propeller | Three Propellers | One Propeller | Three Propellers | ||||||
| Parameter | Units | Series | Parallel | Series | Parallel | Series | Parallel | Series | Parallel |
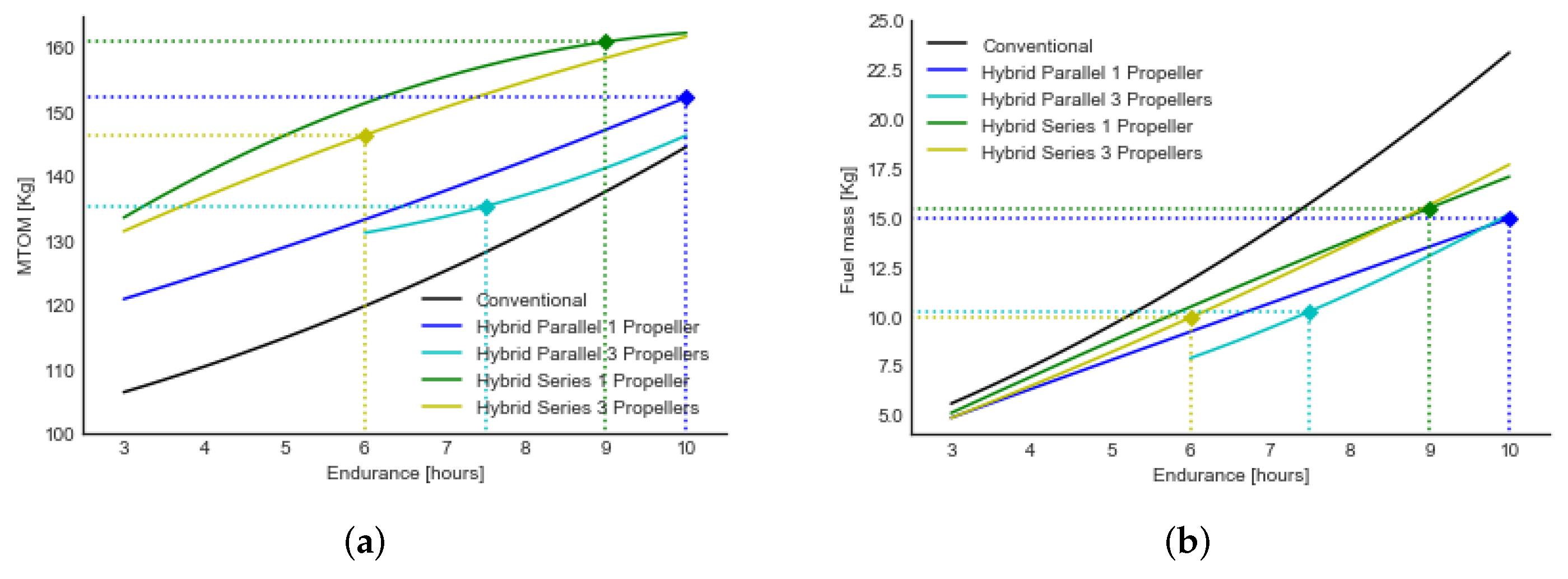
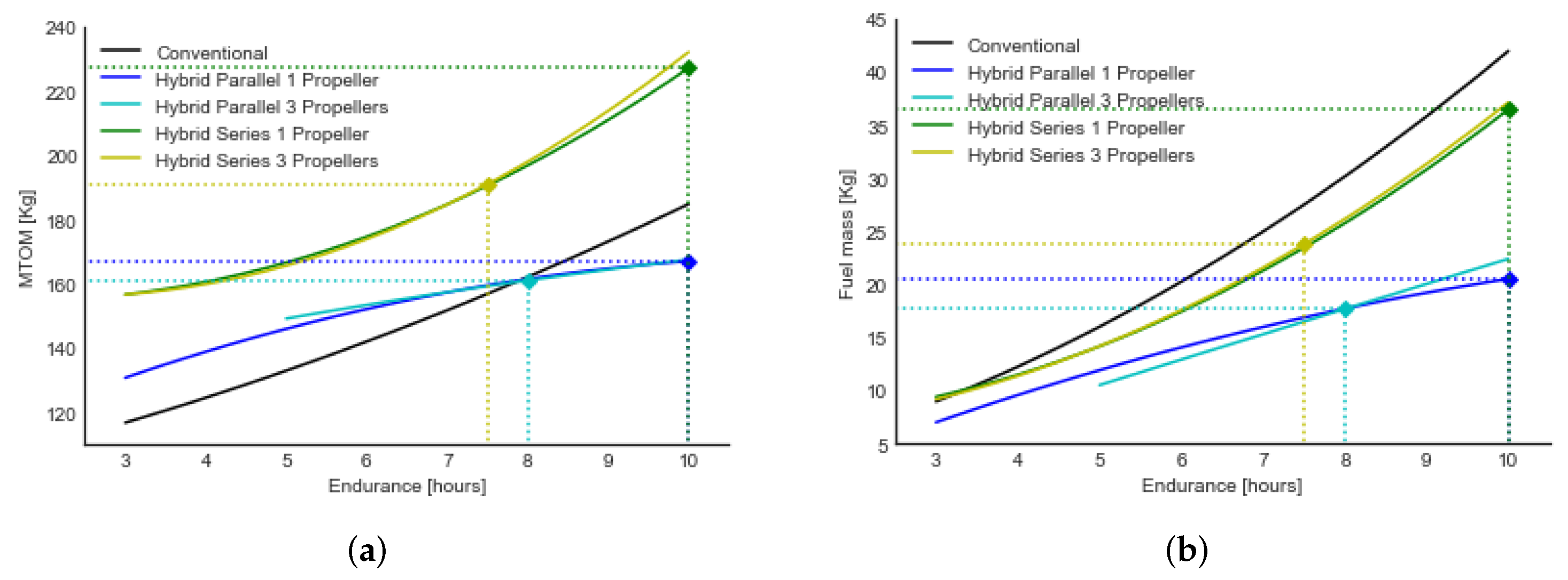
| Endurance | hours | 9 | 10 | 6 | 7.5 | 10 | 10 | 7.5 | 8 |
| Power Loading | W/Kg | 96.46 | 92.14 | 94.09 | 97.15 | 97.80 | 94.80 | 97.47 | 93.92 |
| Wing Loading | N/m2 | 218.13 | 205.98 | 210.92 | 220.23 | 222.19 | 213.05 | 221.20 | 210.41 |
| Hybridization | % | 24.27% | 27.07% | 25.32% | 24.07% | 30.43% | 32.13% | 31.30% | 33.60% |
| MTOM increase | % | 16.91% | 5.33% | 22.17% | 5.57% | 22.87% | 9.63% | 21.75% | 0.67% |
| Fuel saving | % | 23.14% | 36.03% | 16.12% | 34.88% | 13.10% | 51.11% | 13.48% | 41.37% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jimenez, D.; Valencia, E.; Herrera, A.; Cando, E.; Pozo, M. Evaluation of Series and Parallel Hybrid Propulsion Systems for UAVs Implementing Distributed Propulsion Architectures. Aerospace 2022, 9, 63. https://doi.org/10.3390/aerospace9020063
Jimenez D, Valencia E, Herrera A, Cando E, Pozo M. Evaluation of Series and Parallel Hybrid Propulsion Systems for UAVs Implementing Distributed Propulsion Architectures. Aerospace. 2022; 9(2):63. https://doi.org/10.3390/aerospace9020063
Chicago/Turabian StyleJimenez, Darwin, Esteban Valencia, Ariel Herrera, Edgar Cando, and Marcelo Pozo. 2022. "Evaluation of Series and Parallel Hybrid Propulsion Systems for UAVs Implementing Distributed Propulsion Architectures" Aerospace 9, no. 2: 63. https://doi.org/10.3390/aerospace9020063
APA StyleJimenez, D., Valencia, E., Herrera, A., Cando, E., & Pozo, M. (2022). Evaluation of Series and Parallel Hybrid Propulsion Systems for UAVs Implementing Distributed Propulsion Architectures. Aerospace, 9(2), 63. https://doi.org/10.3390/aerospace9020063







