Study on the Leading Edge of a Hypersonic Vehicle Using the Aero-Thermoelastic Coupling Method
Abstract
1. Introduction
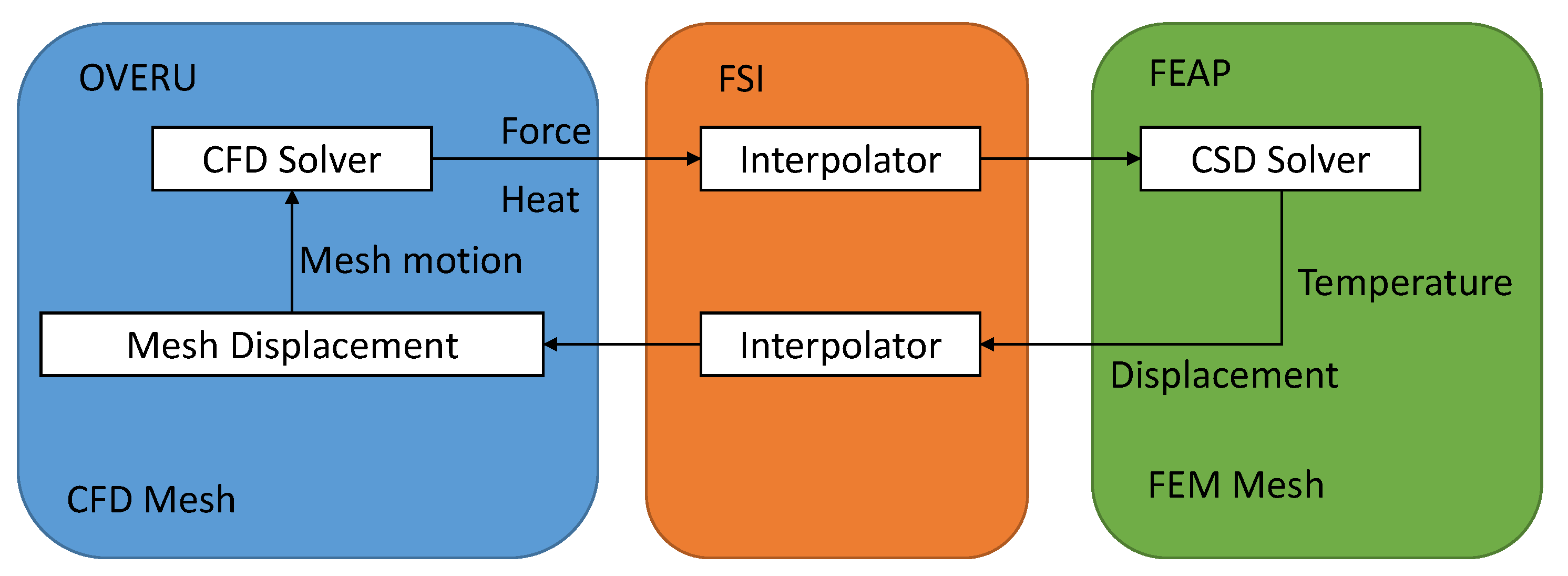
2. Aero-Thermoelastic Coupling Methods
2.1. Flow Solver
2.2. Structural Solver
2.3. Fluid–Structure Interaction
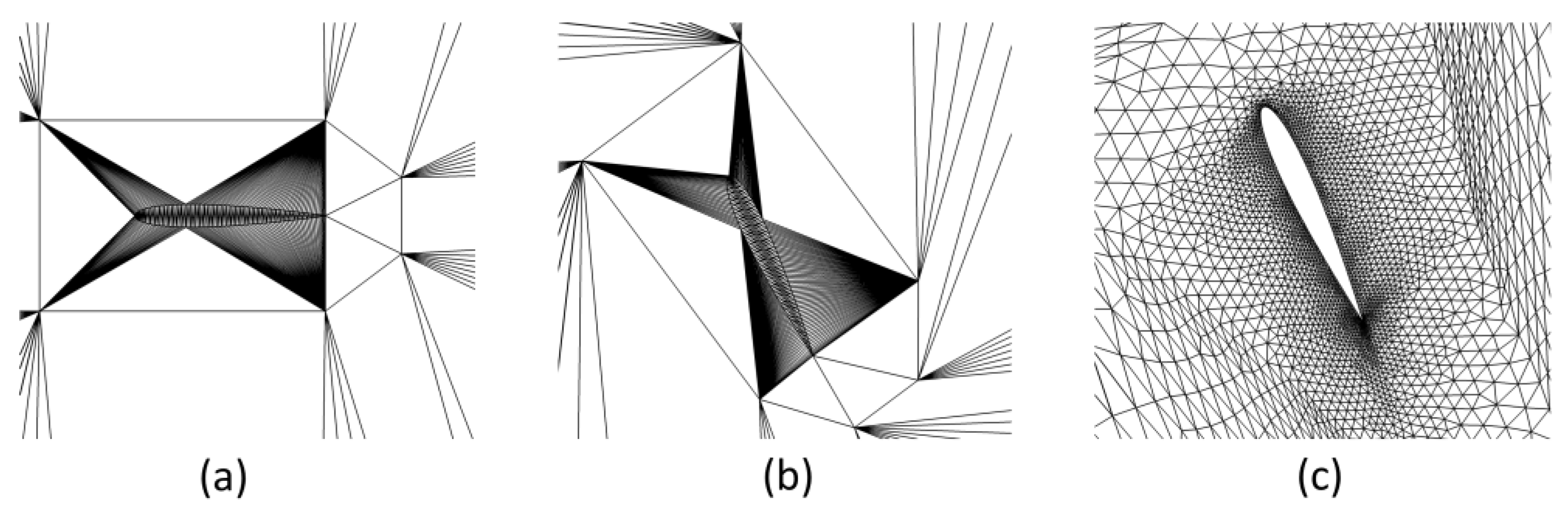
2.4. Grid Deformation
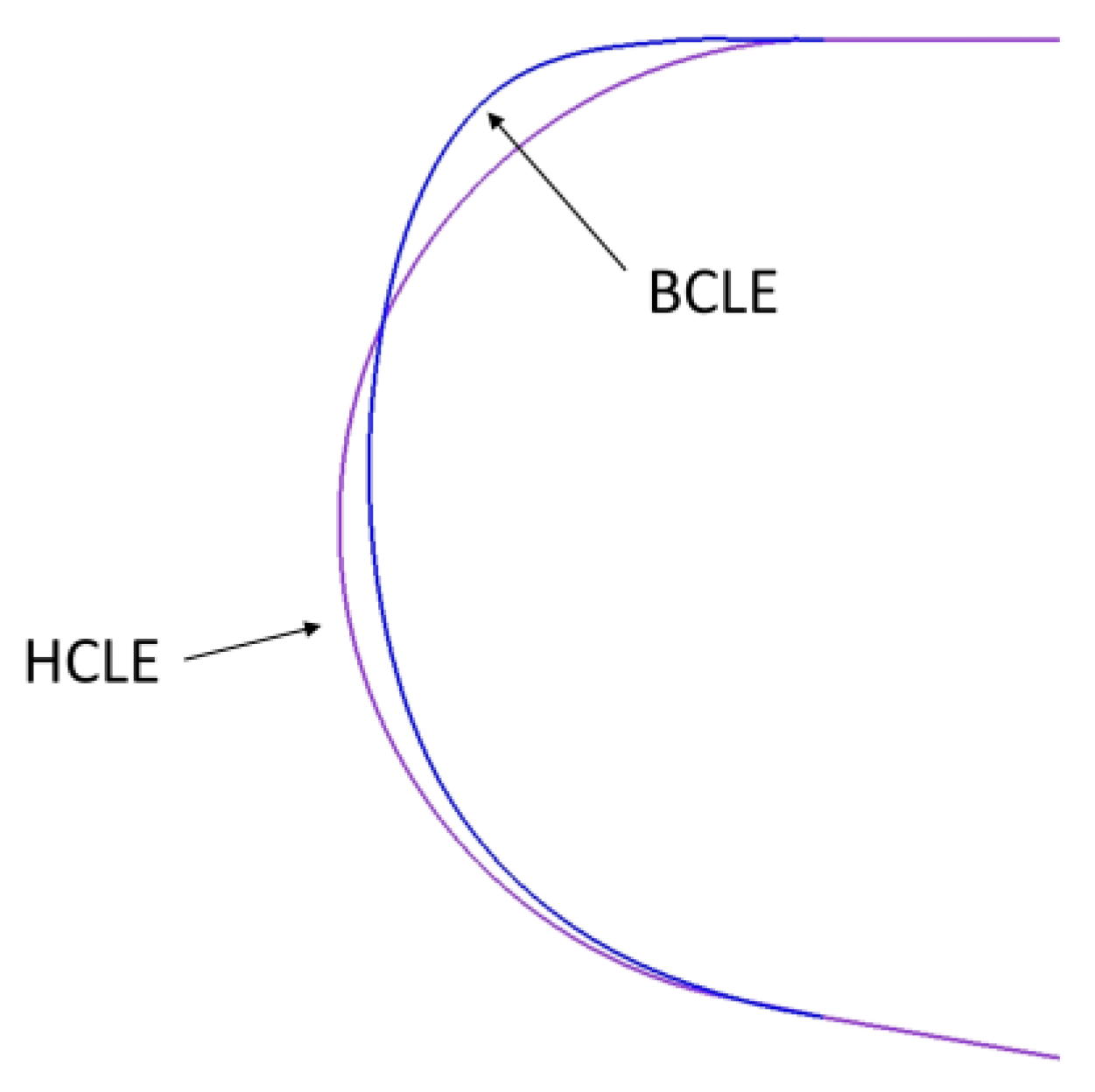
3. Optimization of the Hypersonic Leading Edge
4. Results and Discussion
4.1. Finite Leading Edge
4.1.1. Geometry and Grids
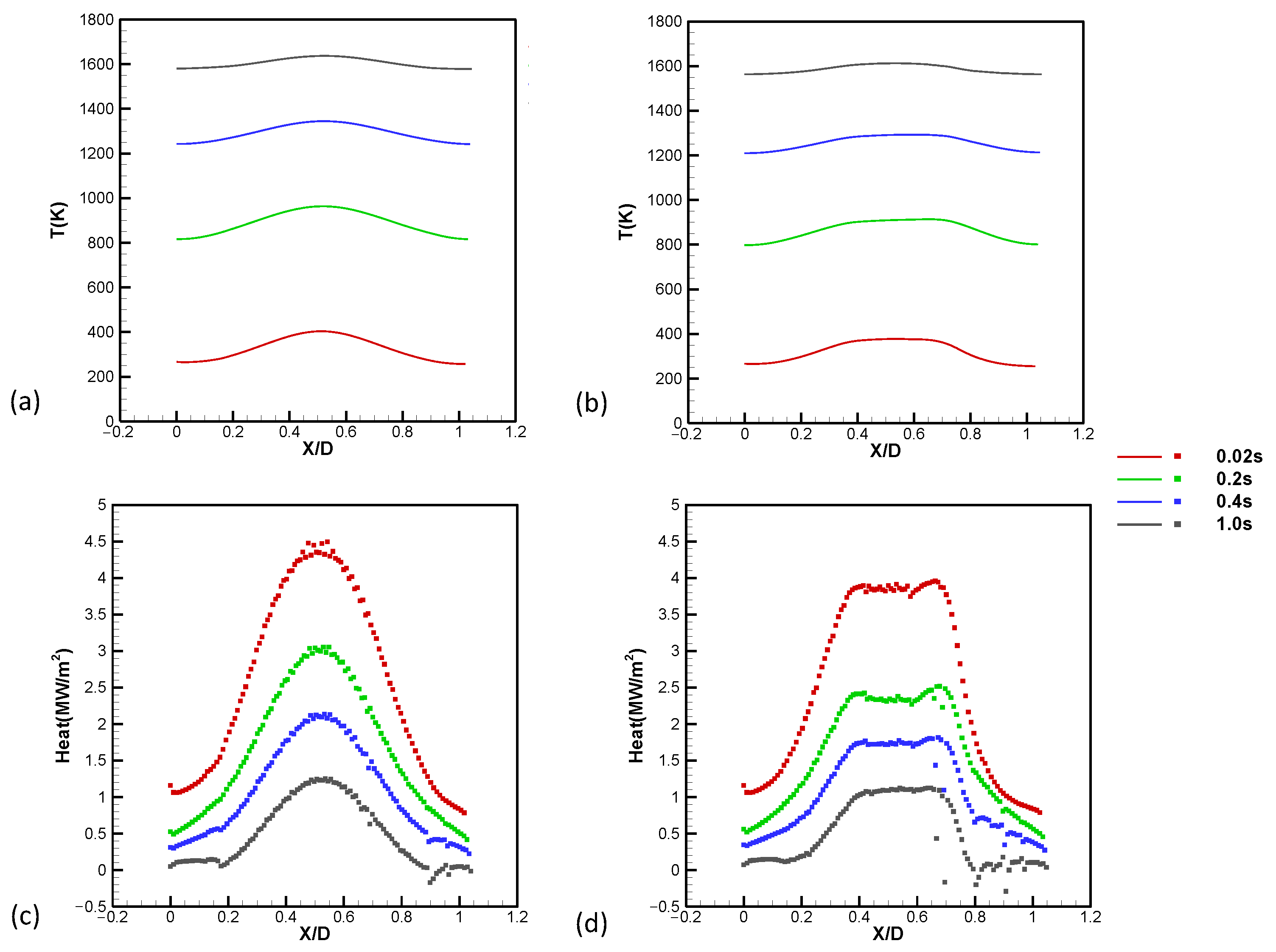
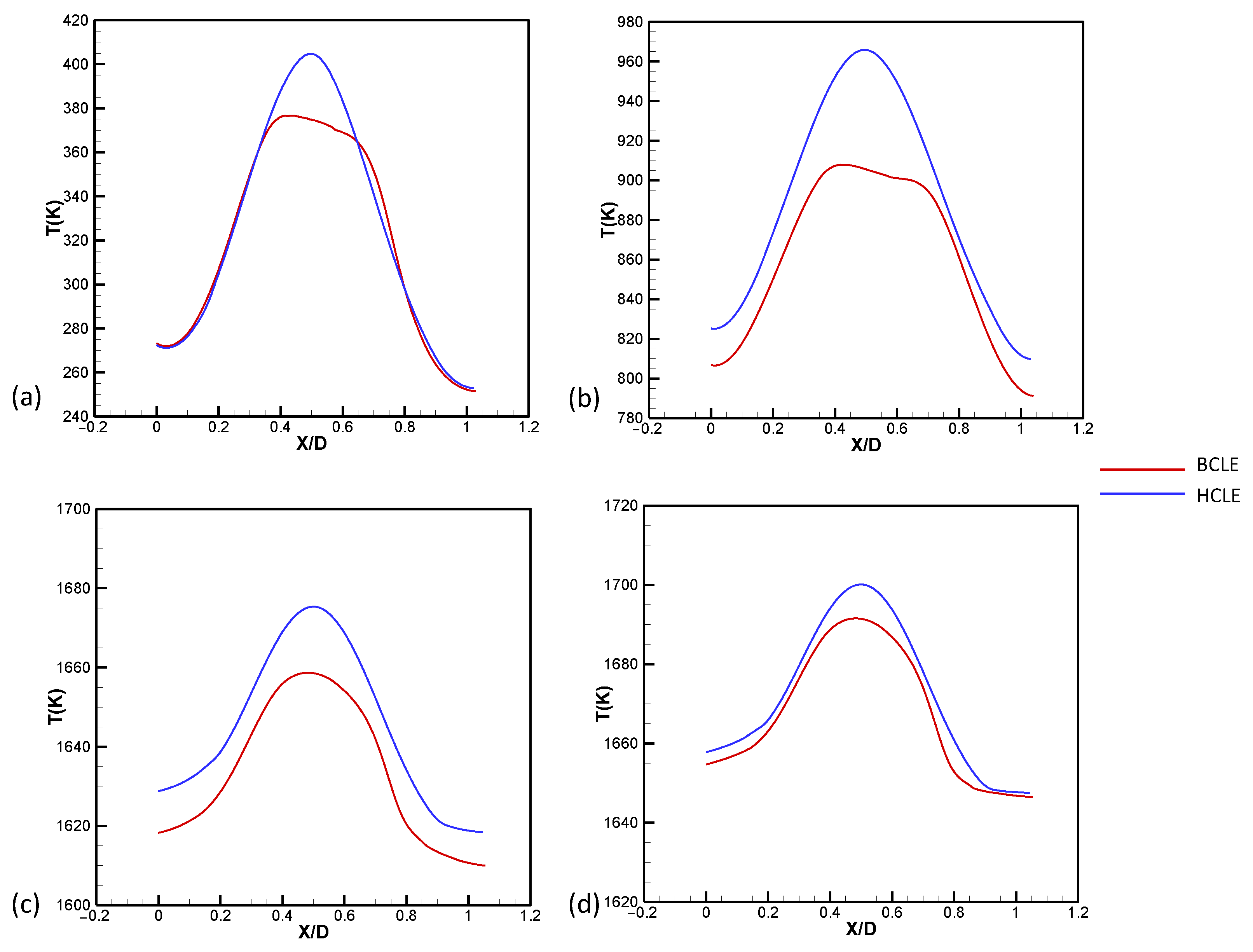
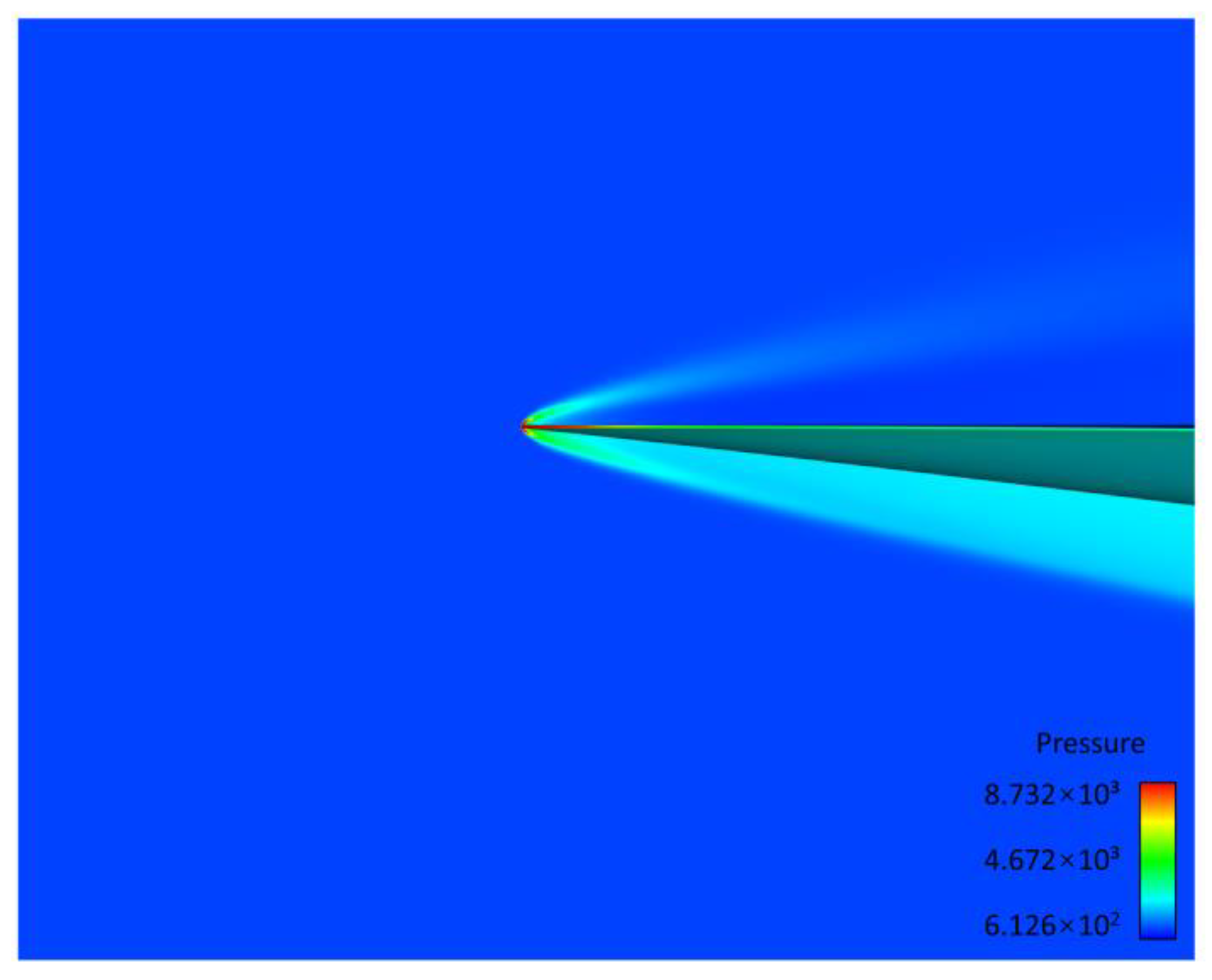
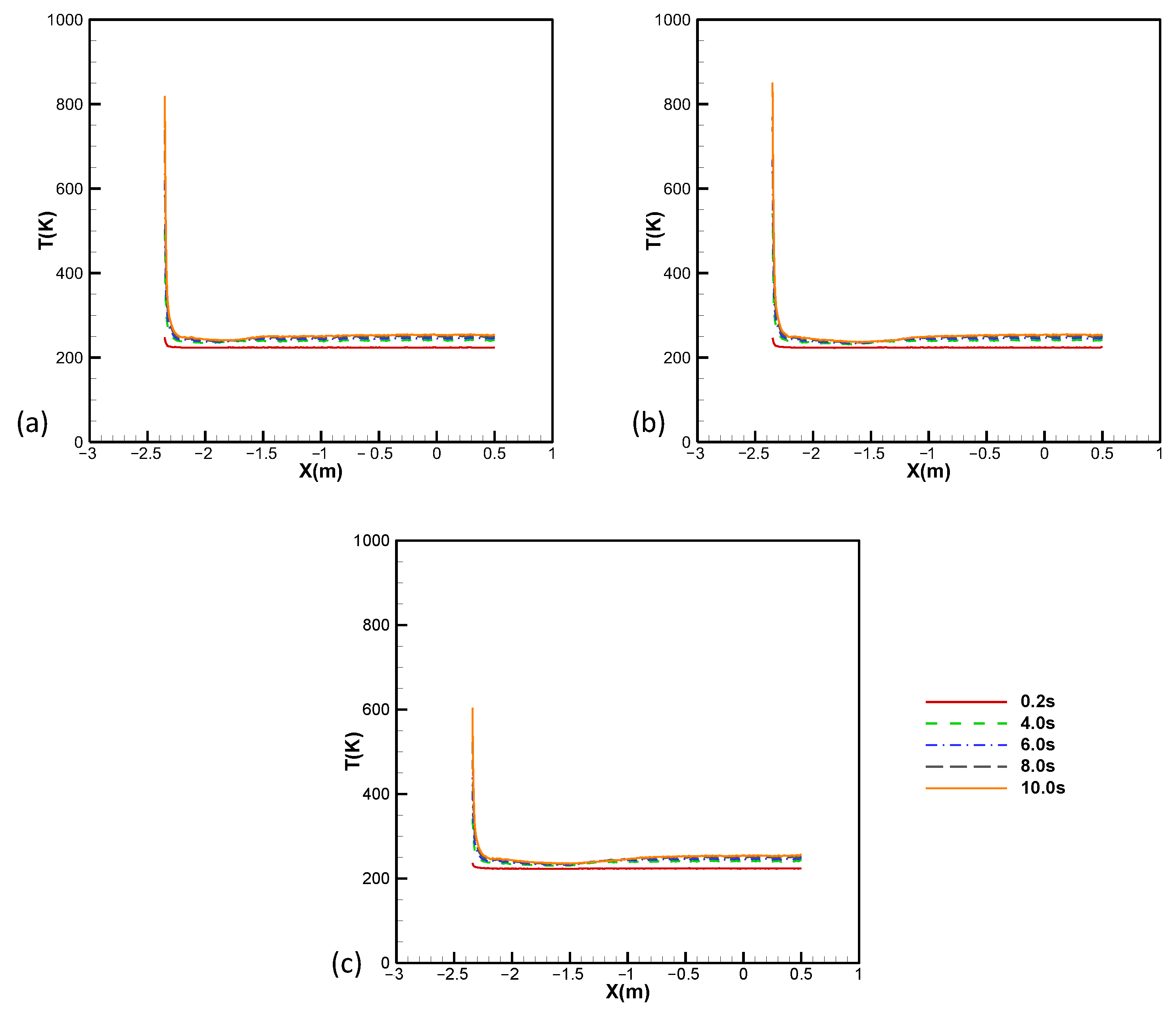
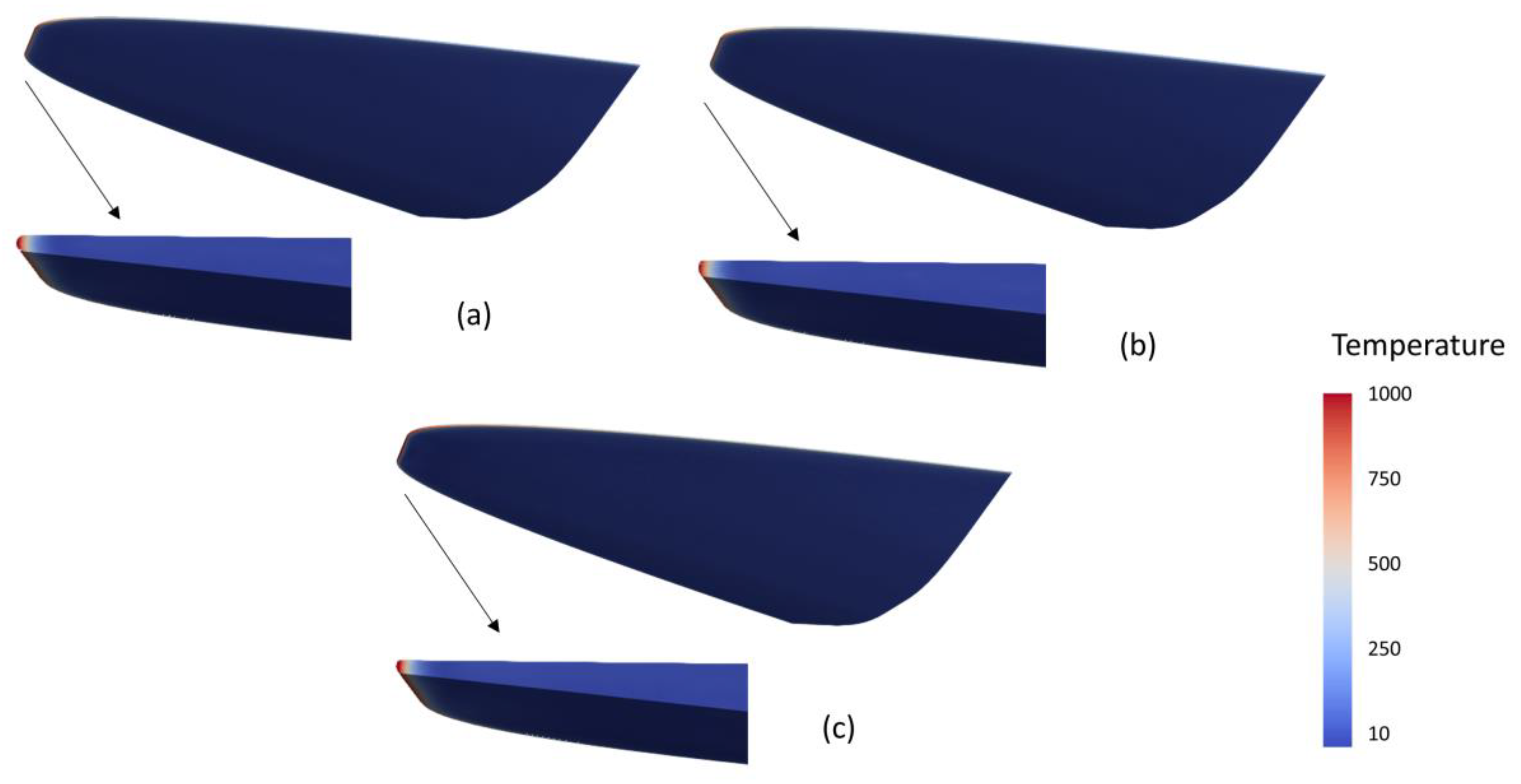
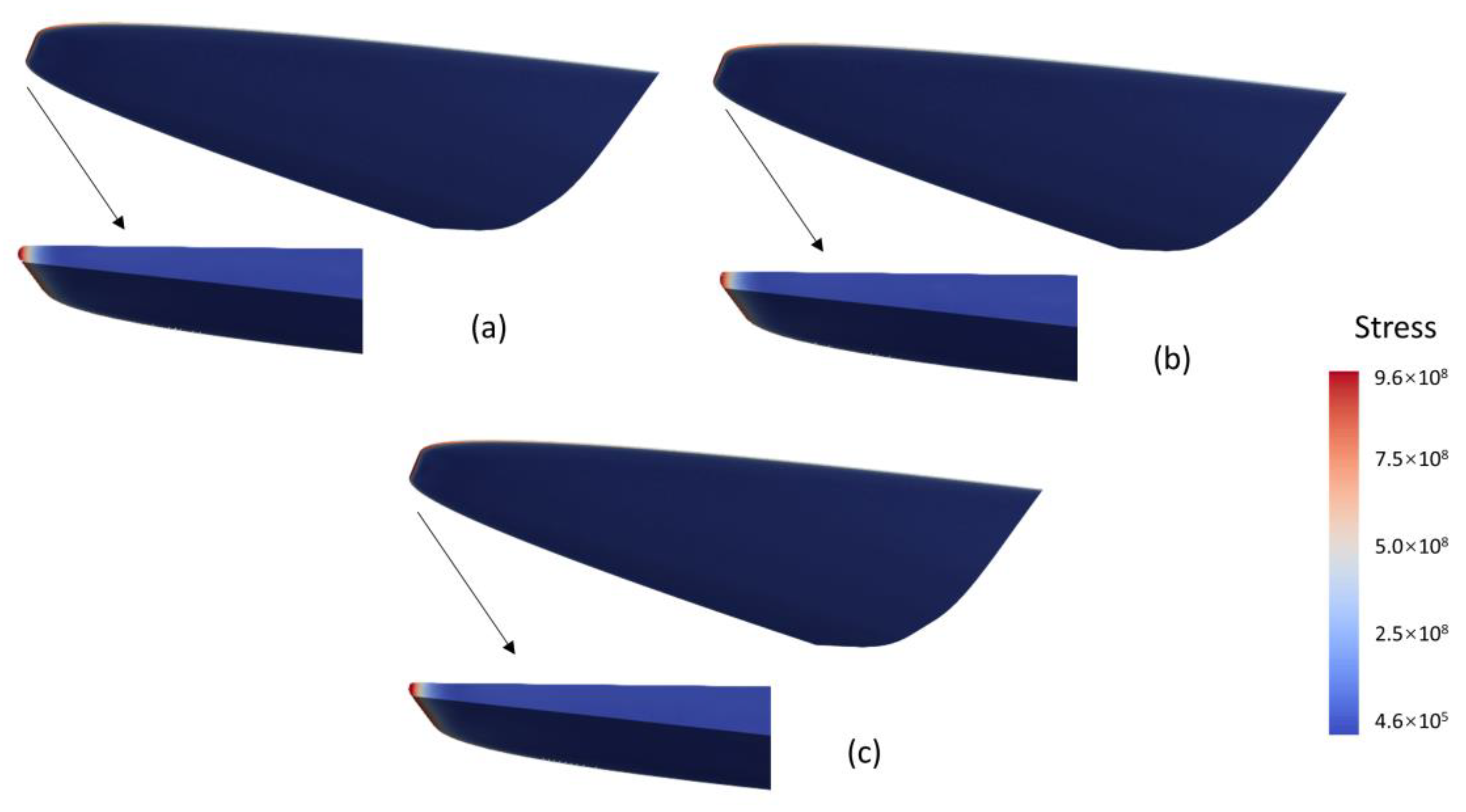
4.1.2. Results
4.2. Hypersonic Vehicle
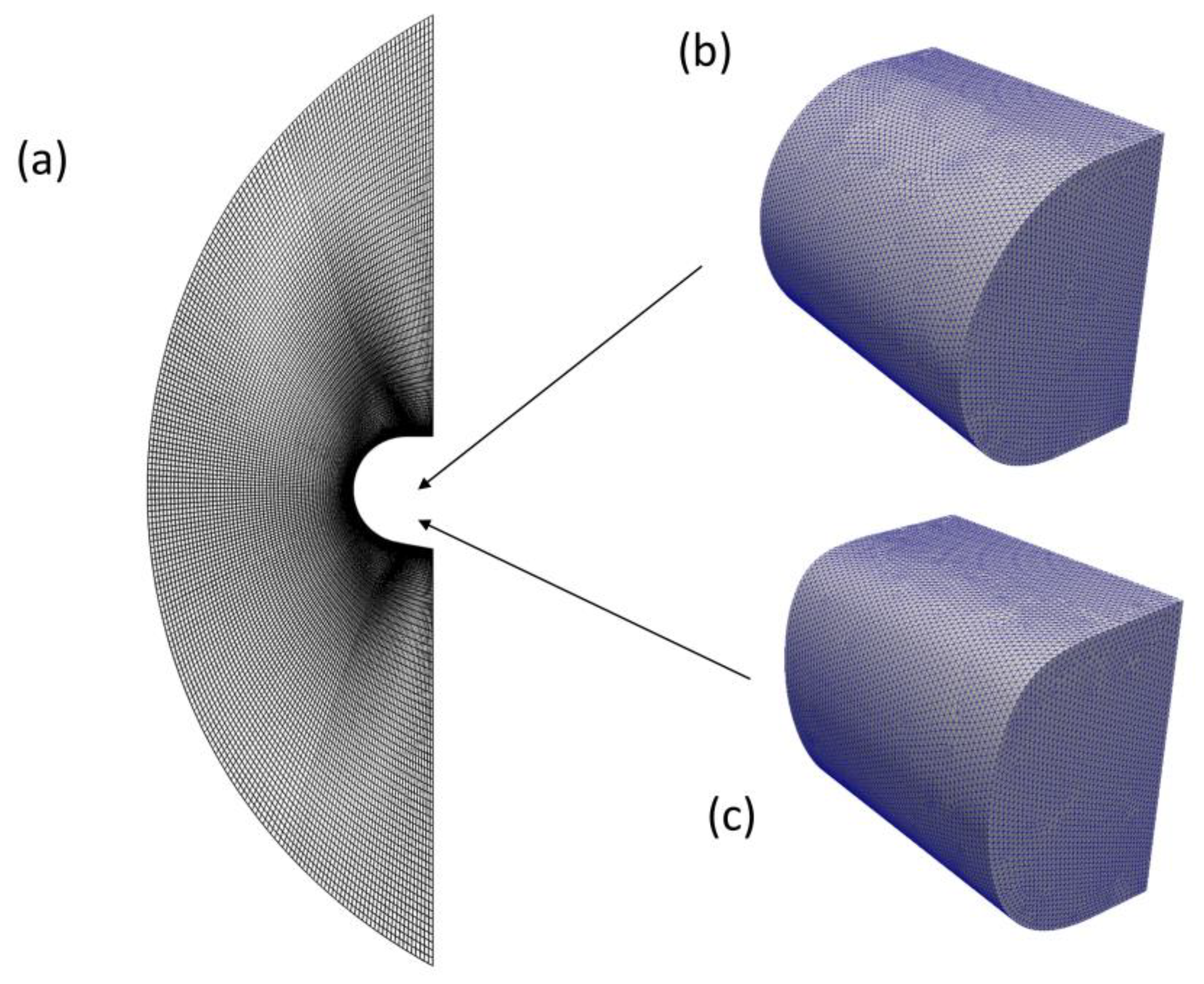
4.2.1. Geometry and Grids
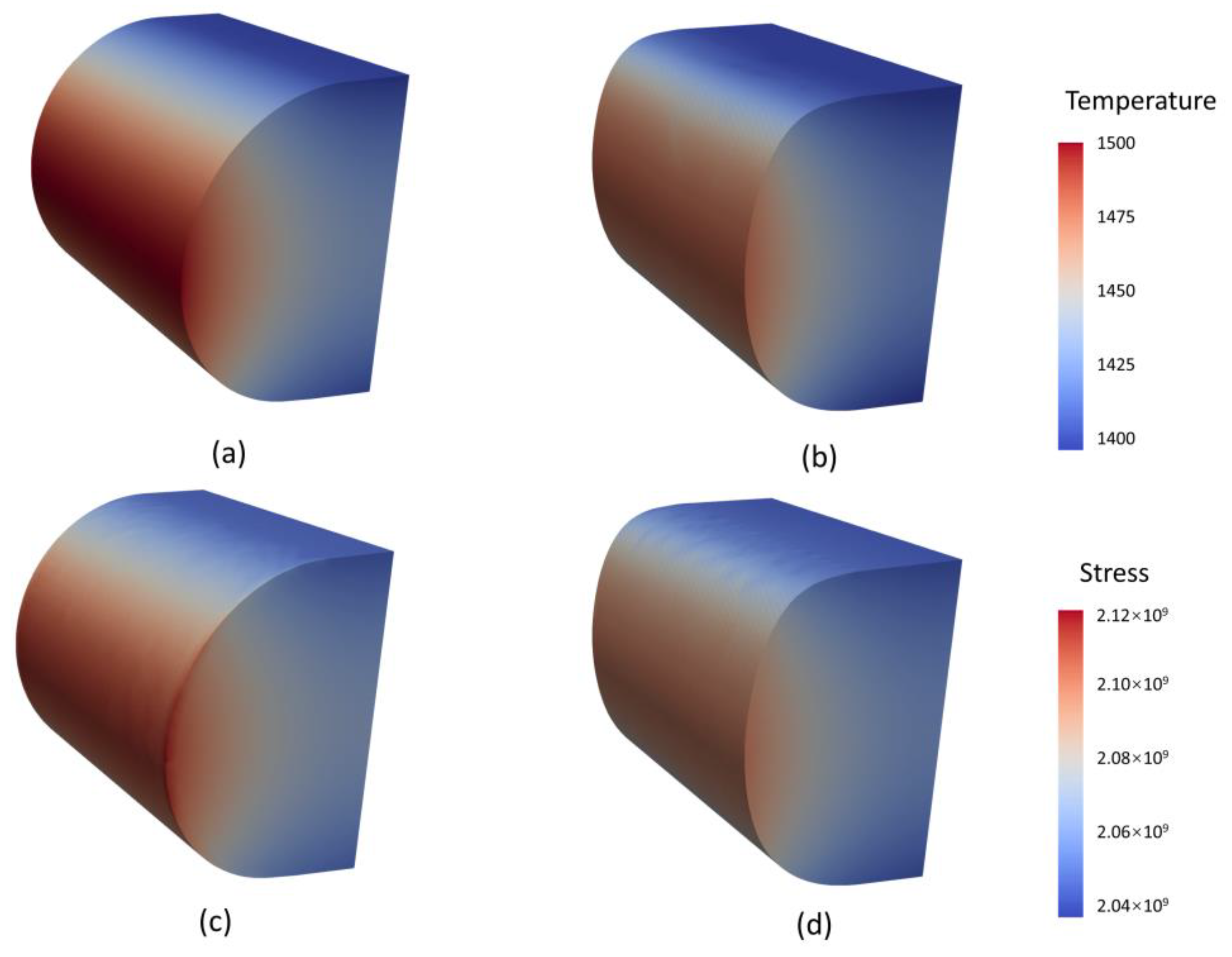
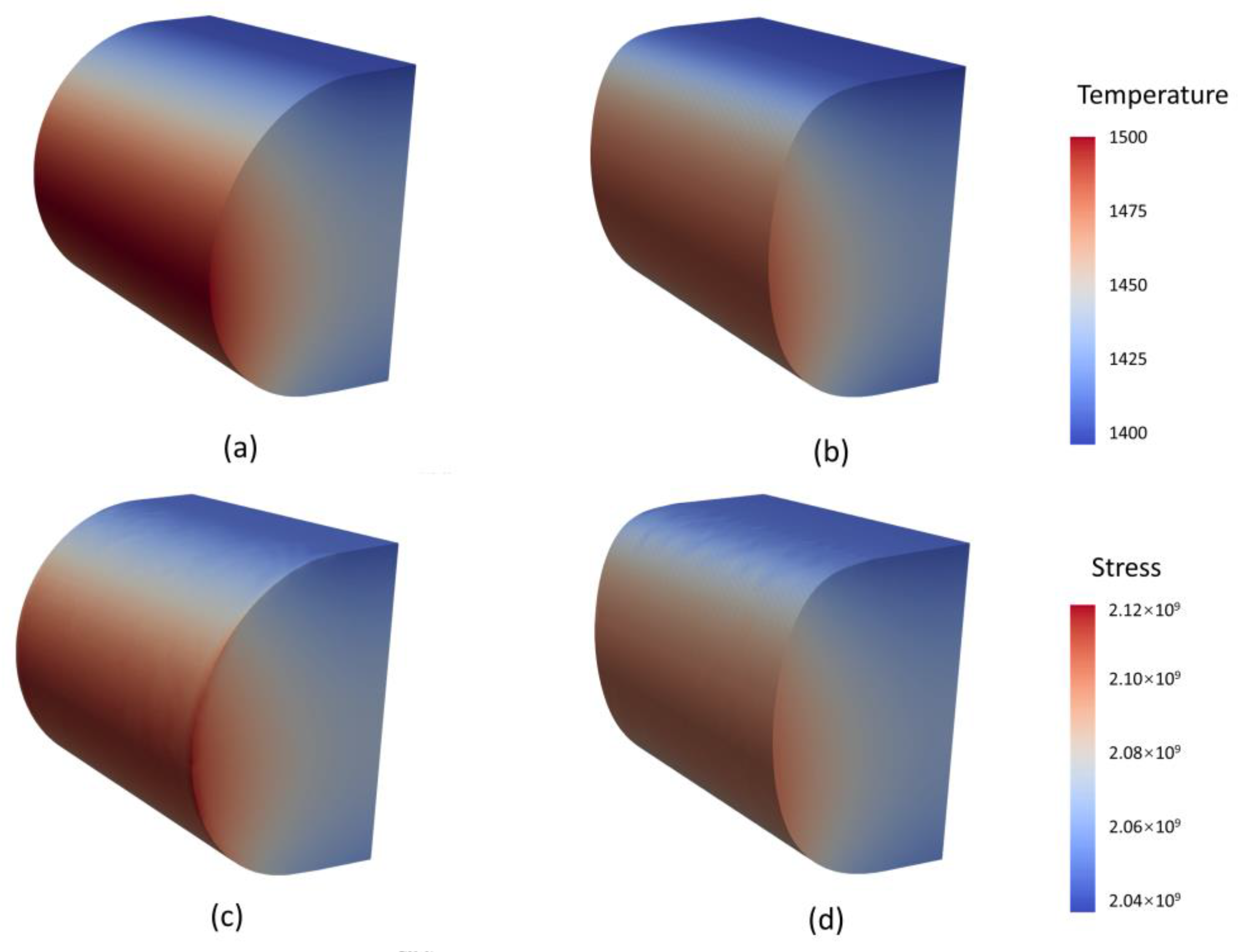
4.2.2. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ohlhorst, C.W.; Glass, D.E.; Bruce, W.E.; Lindell, M.C.; Vaughn, W.L.; Smith, R.W.; Dirling, R.B.; Hogenson, P.A.; Nichols, J.M.; Risner, N.W.; et al. Development of X-43A Mach 10 Leading Edges. In Proceedings of the 56th International Astronautical Congress of the International Astronautical Federation, the International Academy of Astronautics, and the International Institute of Space Law, American Institute of Aeronautics and Astronautics, Fukuoka, Japan, 17 October 2005. [Google Scholar]
- Seager, C.; Agarwal, R.K. Shape Optimization of Axisymmetric Bodies in Hypersonic Flow for Reducing Drag and Heat Transfer. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting, AIAA SciTech Forum; American Institute of Aeronautics and Astronautics, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar]
- Rodi, P.E. Integration of Optimized Leading Edge Geometries Onto Waverider Configurations. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting; AIAA SciTech Forum; American Institute of Aeronautics and Astronautics, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar]
- Hinman, W.S.; Johansen, C.T.; Rodi, P.E. Optimization and Analysis of Hypersonic Leading Edge Geometries. Aerosp. Sci. Technol. 2017, 70, 549–558. [Google Scholar] [CrossRef]
- Santos, W.F.N. Bluntness Impact on Lift-to-Drag Ratio of Hypersonic Wedge Flow. J. Spacecr. Rockets 2009, 46, 329–339. [Google Scholar] [CrossRef]
- Liu, J.; Hou, Z.; Ding, G.; Chen, X.; Chen, X. Numerical and Experimental Study on Waverider with Blunt Leading Edge. Comput. Fluids 2013, 84, 203–217. [Google Scholar] [CrossRef]
- Fu, X.; Liu, J.; Ding, F.; Wang, S.; Dou, T. A Novel Method for Blunting the Leading Edge of Waverider with Specified Curvature. Int. J. Aerosp. Eng. 2020, 2020, 8885062. [Google Scholar] [CrossRef]
- Kamali, S.; Mavriplis, D.J.; Anderson, E.M. Development and Validation of a High-Fidelity Aero-Thermo-Elastic Analysis Capability. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; Volume 34. [Google Scholar]
- Kadivar, M.; Tormey, D.; McGranaghan, G. A Review on Turbulent Flow over Rough Surfaces: Fundamentals and Theories. Int. J. Thermofluids 2021, 10, 100077. [Google Scholar] [CrossRef]
- Culler, A.; Crowell, A.; McNamara, J. Studies on Fluid-Structural Coupling for Aerothermoelasticity in Hypersonic Flow. In Proceedings of the 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference; American Institute of Aeronautics and Astronautics, Palm Springs, CA, USA, 4 May 2009. [Google Scholar]
- McNamara, J.; Culler, A.; Crowell, A. Aerothermoelastic Modeling Considerations for Hypersonic Vehicles. In Proceedings of the 16th AIAA/DLR/DGLR International Space Planes and Hypersonic Systems and Technologies Conference; American Institute of Aeronautics and Astronautics, Bremen, Germany, 19 October 2009. [Google Scholar]
- Chen, L.; Zhou, Z.; Xia, J. One-Parameter Optimal Flux Reconstruction Schemes for Adaptive Mesh Refinement. AIAA J. 2022, 60, 1440–1450. [Google Scholar] [CrossRef]
- Xia, J.; Fu, H.; Tian, S.-L.; Chen, L. Highly Efficient Wall-Distance-Based Parallel Unstructured Overset Grid Assembly. AIAA J. 2021, 59, 5347–5359. [Google Scholar] [CrossRef]
- Nastac, G.; Tramel, R.W.; Nielsen, E.J. Improved Heat Transfer Prediction for High-Speed Flows over Blunt Bodies Using Adaptive Mixed-Element Unstructured Grids. In Proceedings of the AIAA SCITECH 2022 Forum, AIAA SciTech Forum, San Diego, CA, USA, 3–7 January 2022; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2022. [Google Scholar]
- Cui, P.; Zhou, G.; Zhang, Y.; Jia, H.; Wu, X.; Ma, M.; Li, H.; Chen, B. Improved Delayed Detached-Eddy Investigations on the Flow Control of the Leading-Edge Flat Spoiler of the Cavity in the Low-Aspect-Ratio Aircraft. Aerospace 2022, 9, 526. [Google Scholar] [CrossRef]
- Jameson, A.; Yoon, S. LU Implicit Schemes with Multiple Grids for the Euler Equations. In Proceedings of the 24th Aerospace Sciences Meeting; American Institute of Aeronautics and Astronautics, Reno, NV, USA, 6 January 1986. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method, 7th ed.; Elsevier: Oxford, UK, 2013. [Google Scholar]
- Li, X.S.; Demmel, J.W. SuperLU_DIST: A Scalable Distributed-Memory Sparse Direct Solver for Unsymmetric Linear Systems. ACM Trans Math. Softw. 2003, 29, 110–140. [Google Scholar] [CrossRef]
- Chen, L.; Zhao, X. Characterization of Air Flow and Lung Function in the Pulmonary Acinus by Fluid-Structure Interaction in Idiopathic Interstitial Pneumonias. PLoS ONE 2019, 14, e0214441. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Zhao, X. Surface Tension Effects on Pulmonary Acinus Mechanics in Idiopathic Pulmonary Fibrosis Patients. BASIC Clin. Pharmacol. Toxicol. 2019, 125, 36–37. [Google Scholar]
- Sheng, C.; Allen, C.B. Efficient Mesh Deformation Using Radial Basis Functions on Unstructured Meshes. AIAA J. 2013, 51, 707–720. [Google Scholar] [CrossRef]
- Liu, X.; Qin, N.; Xia, H. Fast Dynamic Grid Deformation Based on Delaunay Graph Mapping. J. Comput. Phys. 2006, 211, 405–423. [Google Scholar] [CrossRef]



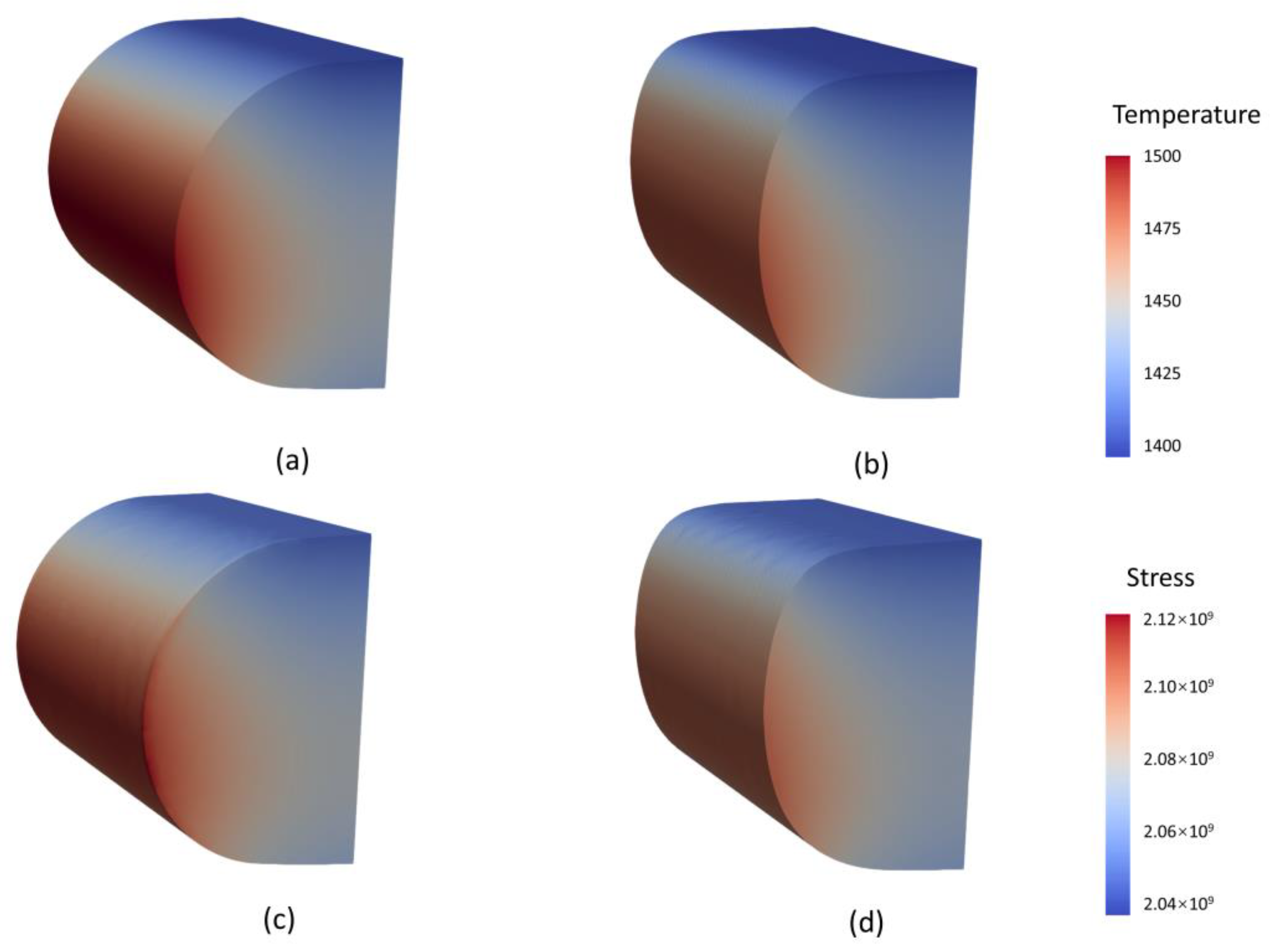
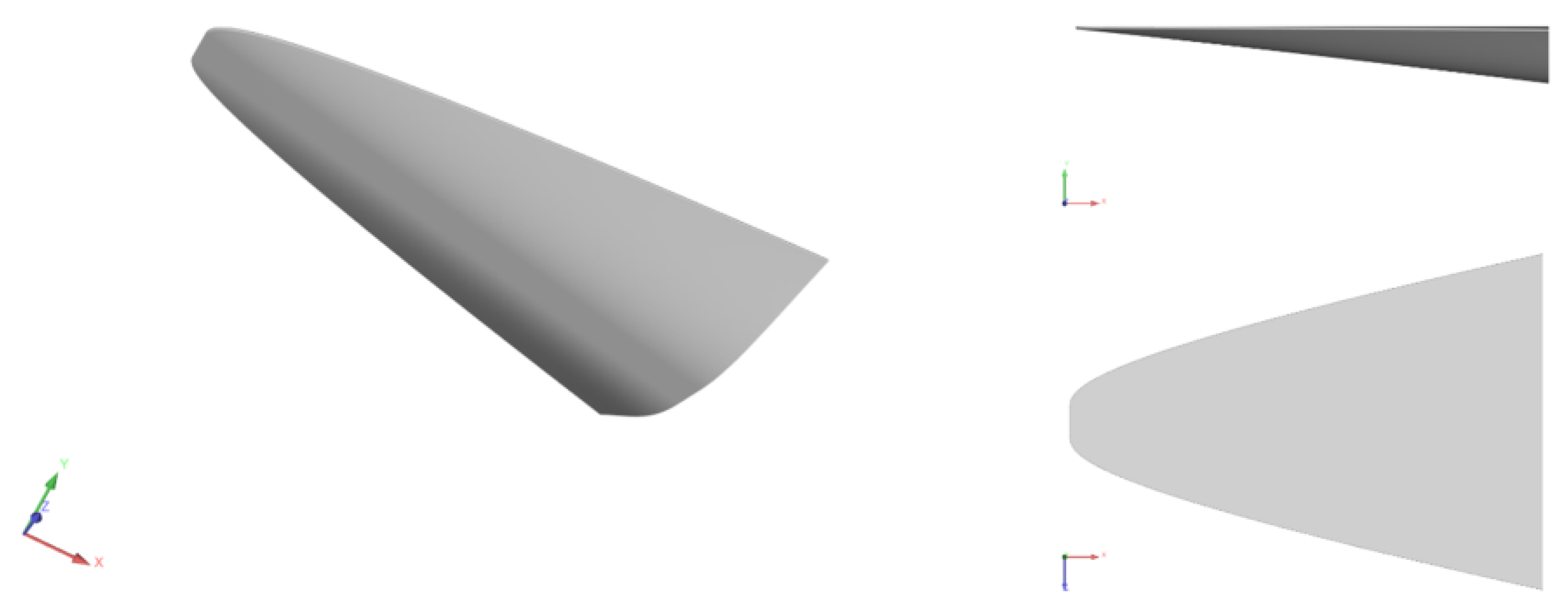
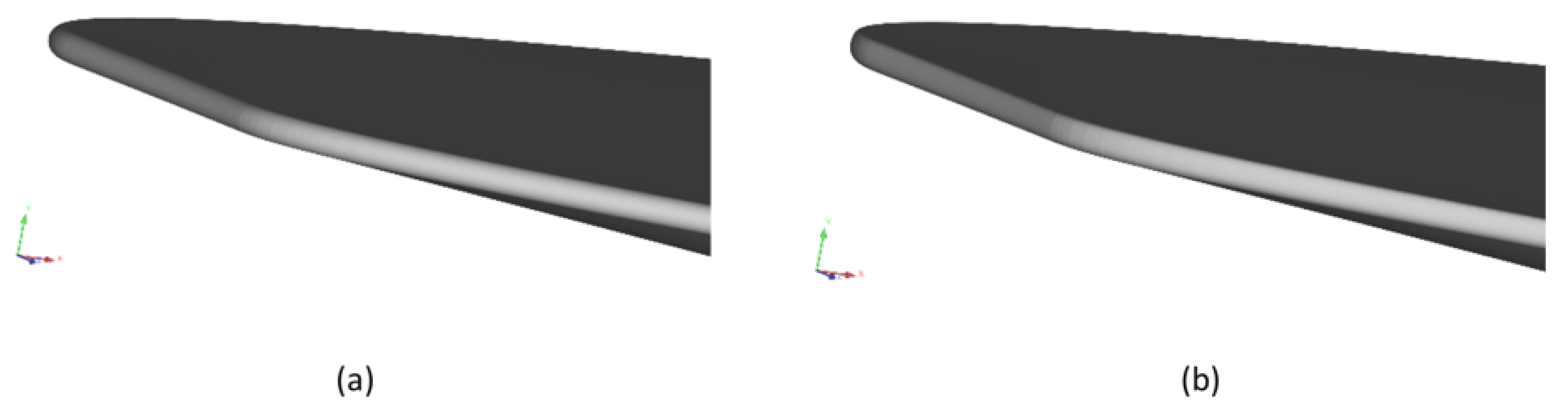
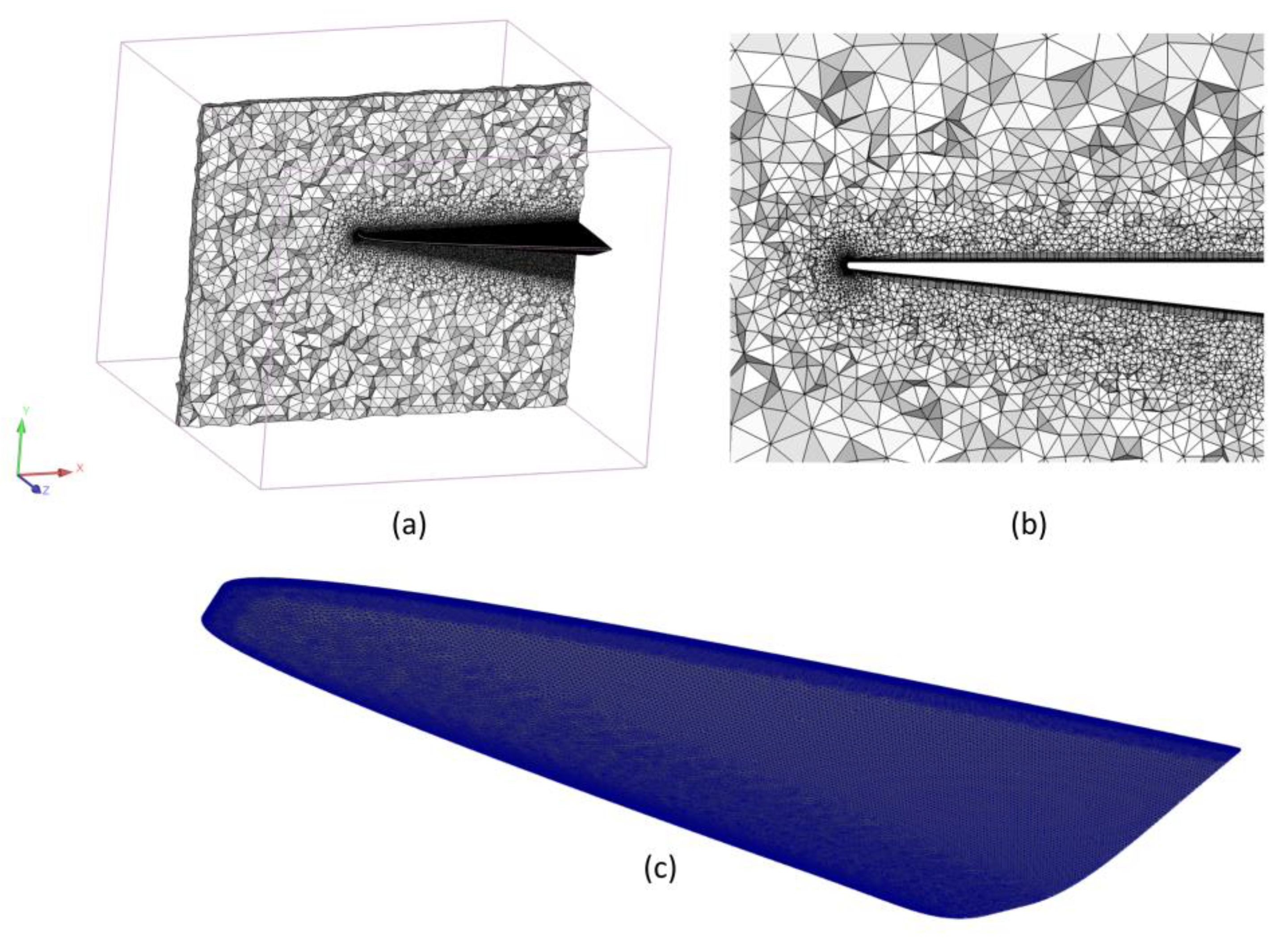

| Mesh | CFD Mesh Elements | CSD Mesh Elements | Maximum Temperature (103 K) |
|---|---|---|---|
| Coarse | 2.3 M | 0.1 M | 1.581 |
| Medium | 3.1 M | 0.2 M | 1.699 |
| Fine | 4.4 M | 0.3 M | 1.727 |
| Mesh | CFD Mesh Elements | CSD Mesh Elements | Maximum Temperature (103 K) |
|---|---|---|---|
| Coarse | 12.6 M | 3.8 M | 1.202 |
| Medium | 17.4 M | 5.2 M | 1.267 |
| Fine | 24.3 M | 7.3 M | 1.274 |
| Model | L/D | Maximum Heat Flux (105 W/m2) | Maximum Temperature (103 K) |
|---|---|---|---|
| HCLE | 4.629 | 7.250 | 1.267 |
| BCLE | 4.641 | 6.608 | 1.201 |
| BCLE2 | 4.886 | 7.140 | 1.224 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Xia, J. Study on the Leading Edge of a Hypersonic Vehicle Using the Aero-Thermoelastic Coupling Method. Aerospace 2022, 9, 835. https://doi.org/10.3390/aerospace9120835
Chen L, Xia J. Study on the Leading Edge of a Hypersonic Vehicle Using the Aero-Thermoelastic Coupling Method. Aerospace. 2022; 9(12):835. https://doi.org/10.3390/aerospace9120835
Chicago/Turabian StyleChen, Long, and Jian Xia. 2022. "Study on the Leading Edge of a Hypersonic Vehicle Using the Aero-Thermoelastic Coupling Method" Aerospace 9, no. 12: 835. https://doi.org/10.3390/aerospace9120835
APA StyleChen, L., & Xia, J. (2022). Study on the Leading Edge of a Hypersonic Vehicle Using the Aero-Thermoelastic Coupling Method. Aerospace, 9(12), 835. https://doi.org/10.3390/aerospace9120835






