1. Introduction
Surviving extreme heating through the atmosphere during high-speed flight and guarding the crew and equipment until they safely reach Earth are the prime objectives of a re-entry vehicle. Although this technology has changed quite significantly in recent years, re-entry vehicles have used the same basic design concept. A re-entry capsule comprises two major components: a blunt front body succeeded by a conical aft body with a rounded or straight base. Re-entry vehicles are subjected to aerodynamic heating during the atmospheric descent. This generates excessive heat; subsequently, the external surface of the capsule gets heated. Hence, to protect the vehicle and its internal structure from such high temperatures, re-entry vehicles are furnished with ablative thermal protection systems. Thermal loads play a major role during the re-entry phase. High temperature change produces in-plane compressive loads, which causes the structure to deform and buckle. When the structure begins to deform from its equilibrium state due to thermal loads, thermal buckling occurs due to the critical buckling temperature, which is the in-plane thermal load. Thermal stresses and deflections will be produced, and the structure will eventually fail if the buckling temperature is further increased. Thin-walled structures, such as shells, undergo buckling when their membrane strain energy is converted to bending energy. Thermal stability characteristics of functionally graded shells are relevant areas of research and hence, in-depth studies are needed. Vibration analysis is also necessary to obtain natural frequency, the knowledge of which will help to prevent failure of structures due to the resonance condition.
Functionally graded materials (FGMs) are inhomogeneous in nature, comprised of two or more different components, and are designed in such a way that the material composition in the direction of thickness is varied gradually [
1]. In the present work, FGMs are designed by varying material composition from metal-rich to ceramic-rich [
2]. Their advantages include better thermal stress reduction, high heat and wear resistance, breakage resistance, etc. Since the variation is gradual, it eliminates stress concentration followed by delamination occurring from composition discontinuities. Various industries, such as automobile, aircraft, aerospace, biomedical, steel and nuclear reactor industries, have paid serious attention to FGMs as heat-protecting advanced materials. In the last decade, several studies emerged on FGM panels. Finite element formulation was developed to explore static structural characteristics of the FGM panels [
3,
4]. The impact of geometrical constraints and temperature fields on the buckling temperature of FGM plates has been examined in [
5,
6,
7]. FGM plates have a higher critical thermal gradient under non-linear temperature distribution than linear distribution. Buckling, as well as dynamic characteristics of axisymmetric FGM circular panels [
8] and elliptical panels [
9] under a thermal environment, have been examined. Zhao et al. [
10] and Kumar et al. [
11] evaluated the dynamic characteristics for FGM panels and the influence of the thermal field in a dynamic analysis was presented in [
12,
13,
14]. They highlighted the influence of boundary constraints, geometrical constraints, material compositions and temperature rise. Other works by Kim [
15] and Huang and Shen [
16] explored linear, as well as non-linear dynamic responses of FGM panels exposed to thermal field and parametric studies for the dynamic nature of the panels. The influence of temperature-reliant material properties on the structural behavior of a thin-walled box column assembled by FGM plates has been studied by Ramkumar and Ganesan [
17].
Shell-type structures are commonly used in aerospace structures due to their free-form shape. Thin shells are highly preferred due to their efficient ways of achieving a greater strength-to-weight ratio. In comparison with other structure types, the most critical part of the shell is the strength of the material because shells tend to deform in buckling and, consequently, exhibit yielding. Rosario et al. [
18] used ultra-high temperature ceramics for the nose of a re-entry vehicle and carried out FEM-based thermo-structural analysis. It has been noted that the studies on FGM shells for the nose structures of re-entry vehicles are highly significant. Buckling, as well as dynamic analysis of FGM shells [
19,
20], have been studied by employing classical thin shell theory, as well as meshless method, respectively. Neves et al. [
21] evaluated the frequencies of clamped, as well as simply supported spherical and cylindrical FGM shells. Hajlaoui et al. [
22] examined the thermal buckling of FGM shells by employing a modified FSDT-based shell element. The proposed formulation has a strong advantage compared to other shear deformation theories. Moita et al. [
23] and Das et al. [
24] inspected the buckling nature of FGM axisymmetric shells exposed to mechanical and thermal environments. Reddy and Chin [
25] and Trabelsi et al. [
26] analyzed the thermal buckling nature of FGM cylinder-shaped shells, as well as plates, by adopting a modified FSDT-based shell element. Sun et al. [
27] derived a novel analytical formulation for the thermal buckling characteristics of FGM cylinder-shaped shells by using temperature-reliant properties. The findings indicate that there exists a critical power law exponent, above which the thermal buckling starts to occur. Torabi et al. [
28] and Sofiyev [
29] conducted buckling analysis of an FGM conical shell under non-linear temperature distribution. Kumar and Kumar [
30] presented a new analytical model to examine the vibration features of FGM-stiffened shallow shells exposed to thermo-mechanical loads. Das and Karmakar [
31] and Jooybar et al. [
32] evaluated the thermal influences on the vibration behavior of an FGM conical shell using FEM and found that the natural frequency reduces because of the incremental increase in temperature, but it has higher circumferential modes. Thermal stresses and semi-vertex angle have a substantial effect on the frequency parameter.
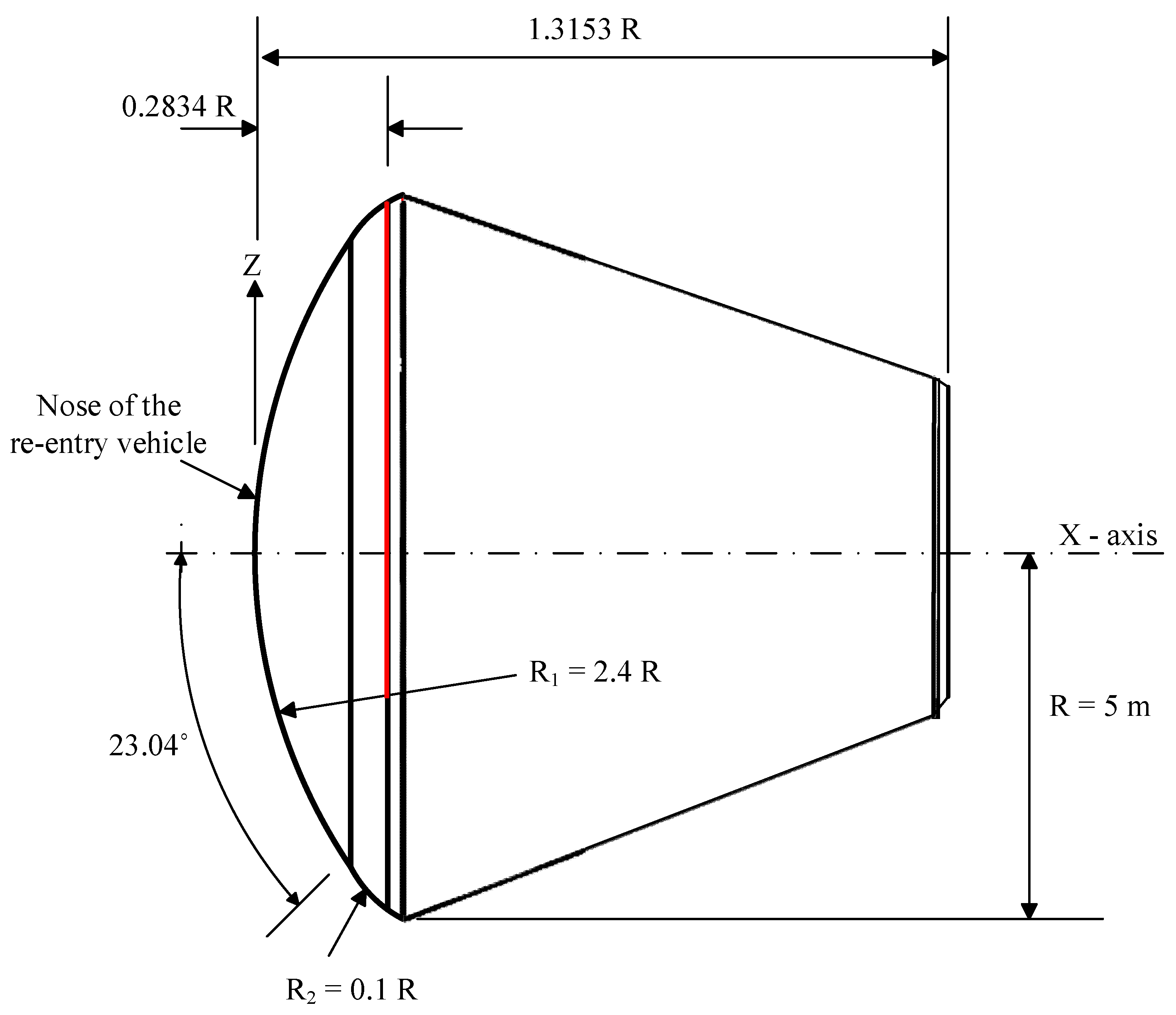
It is noted from the literature mentioned above that it is desirable to explore the application of FGM as nose structures in re-entry vehicles. The base re-entry vehicle model considered for the present study is the Orion model [
33]. The rationale of the study is to understand the thermo-structural behaviour of different FGM shell structures, which are of the size suiting re-entry vehicles. The research intends to determine the thickness required for such thin and large shells to withstand in the re-entry thermal conditions. In the present work, large and thin FGM shell structures with 20 layers made of aluminum/silicon carbide, aluminum/aluminum oxide and Ti-6Al-4V/silicon carbide, as well as Ti-6Al-4V/aluminum oxide, are considered instead of ceramic tiles in Orion to perform the structural and thermal analysis. Thus, the objective of present research is to explore the static, as well as dynamic characteristics of the nose of the re-entry vehicle model made of four different FGM exposed to various temperature fields.
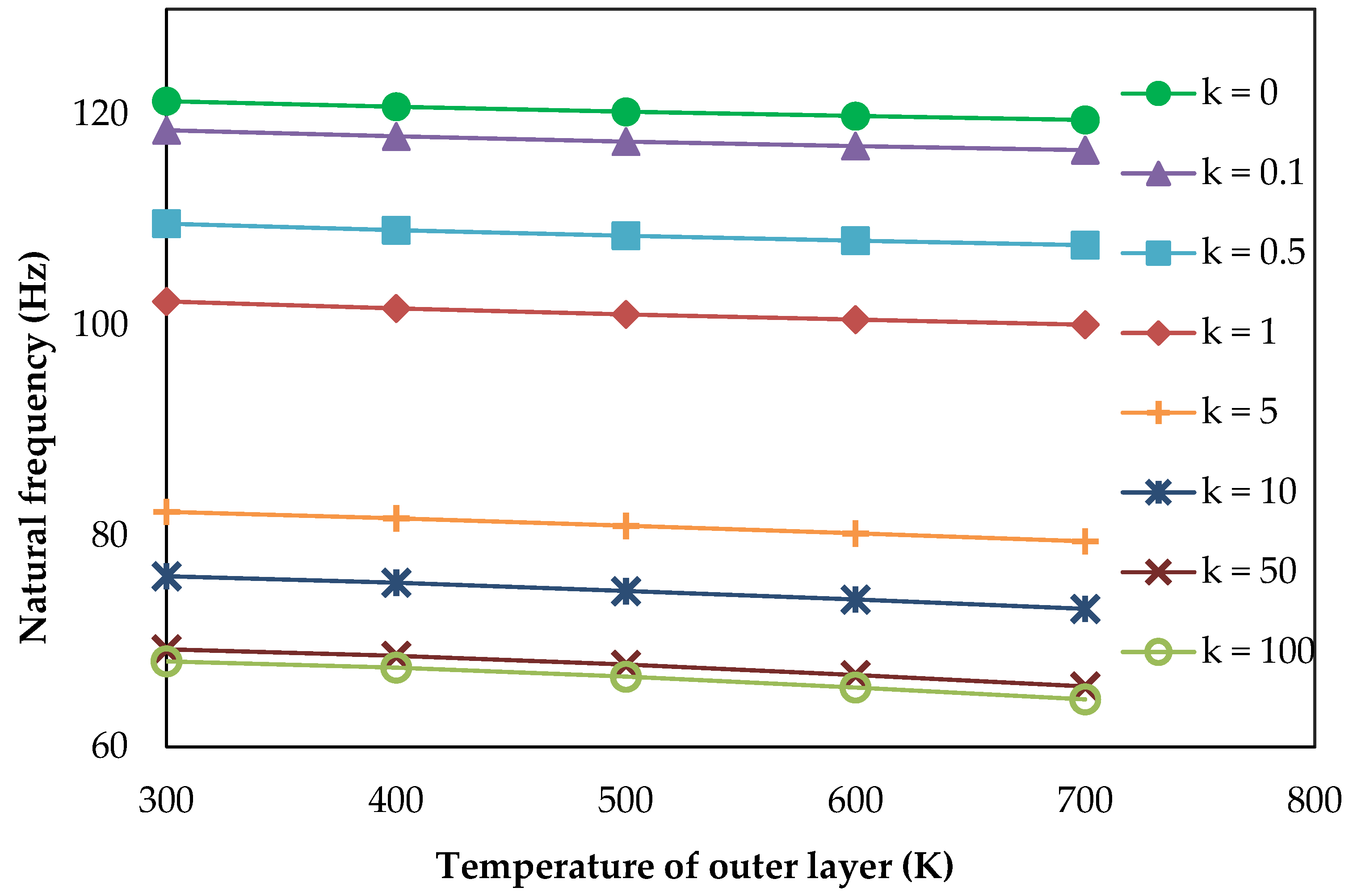
4. Conclusions
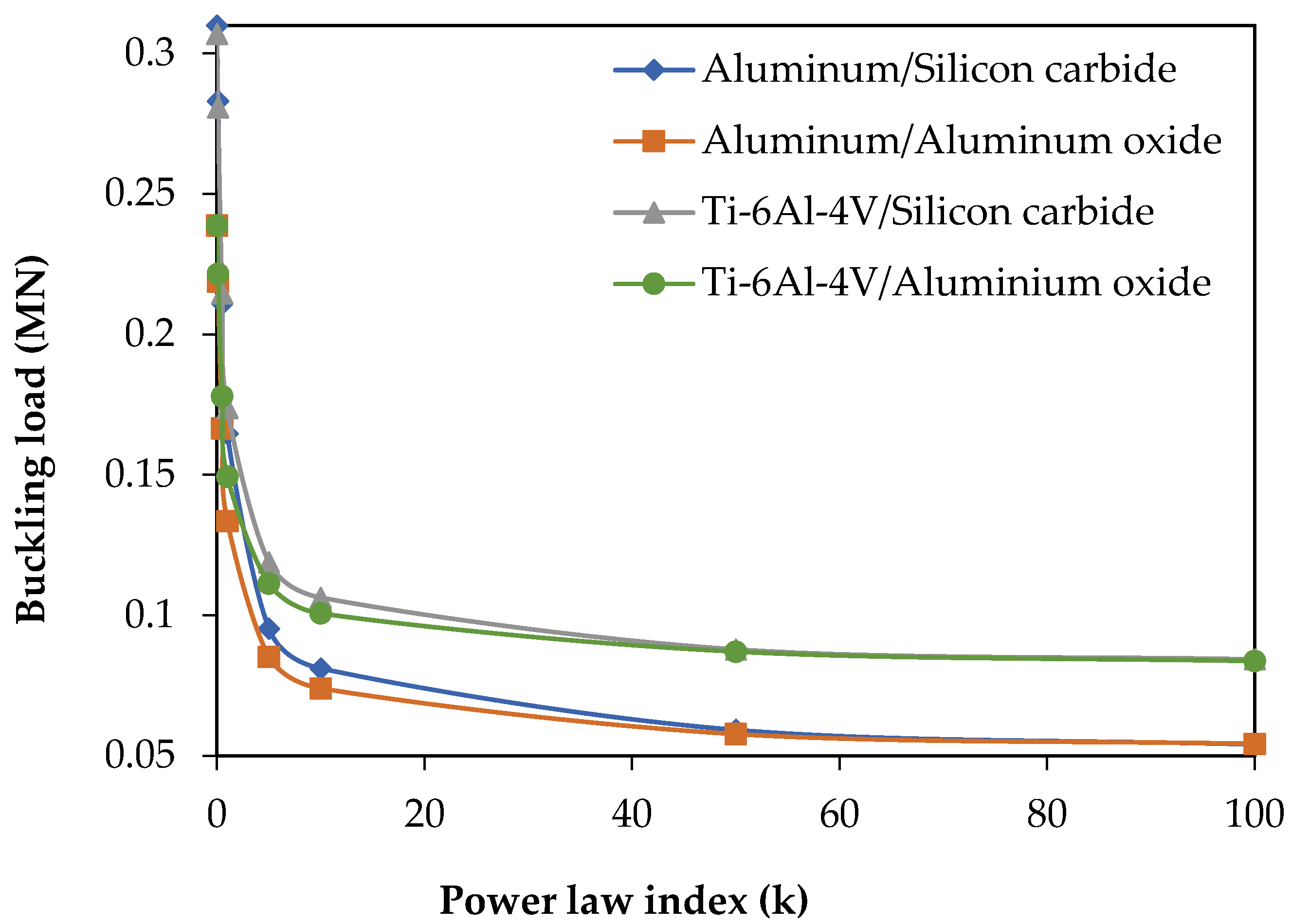
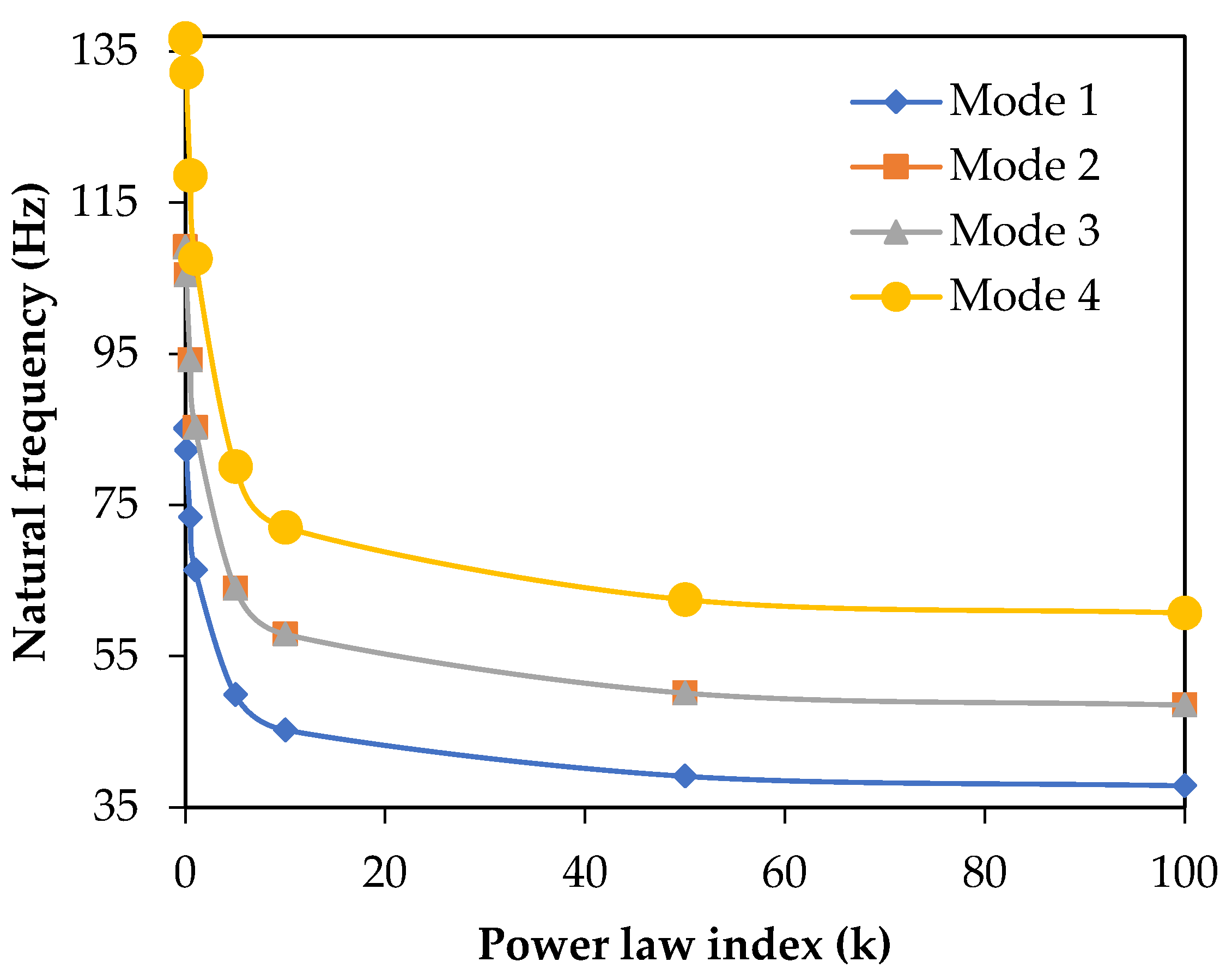
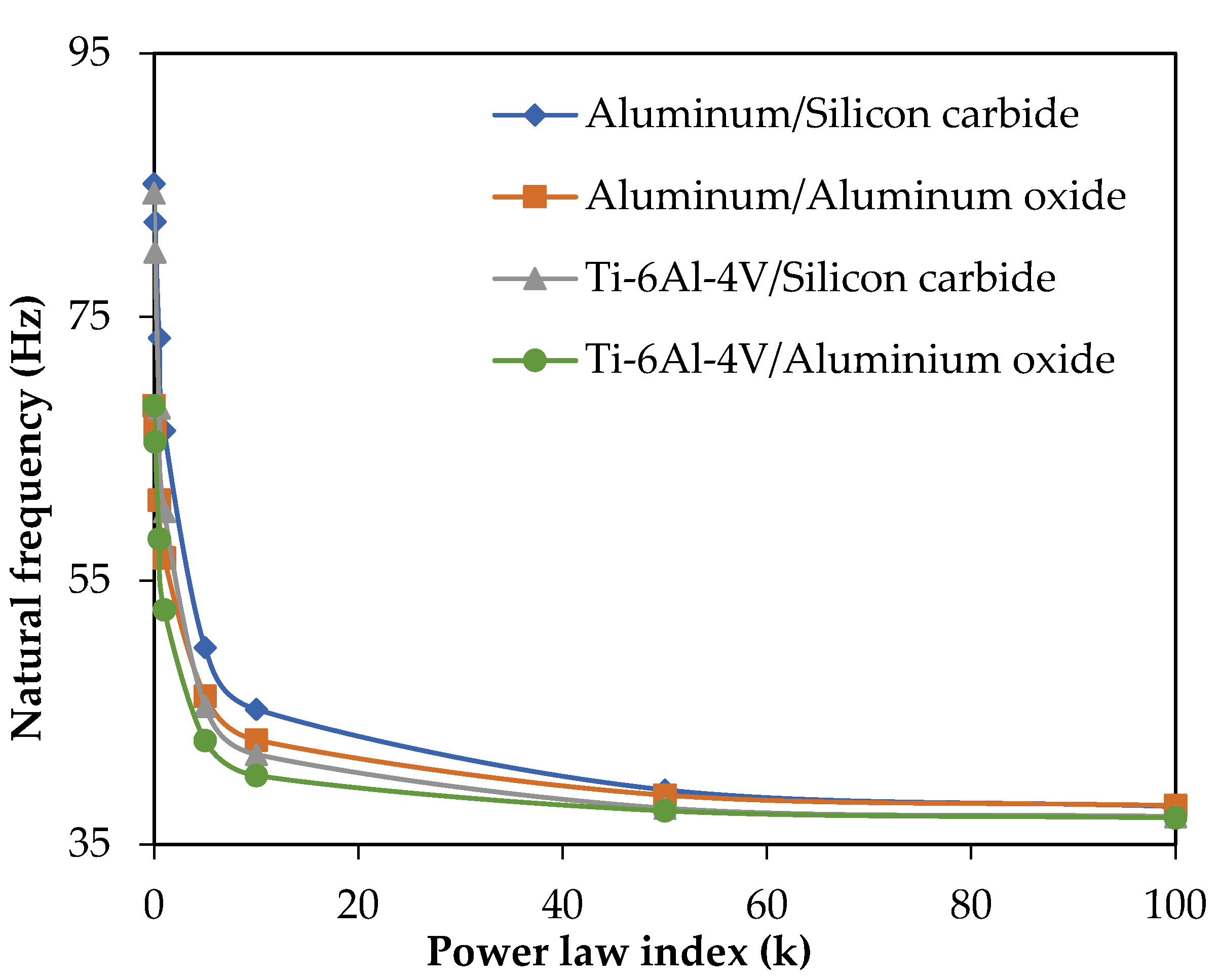
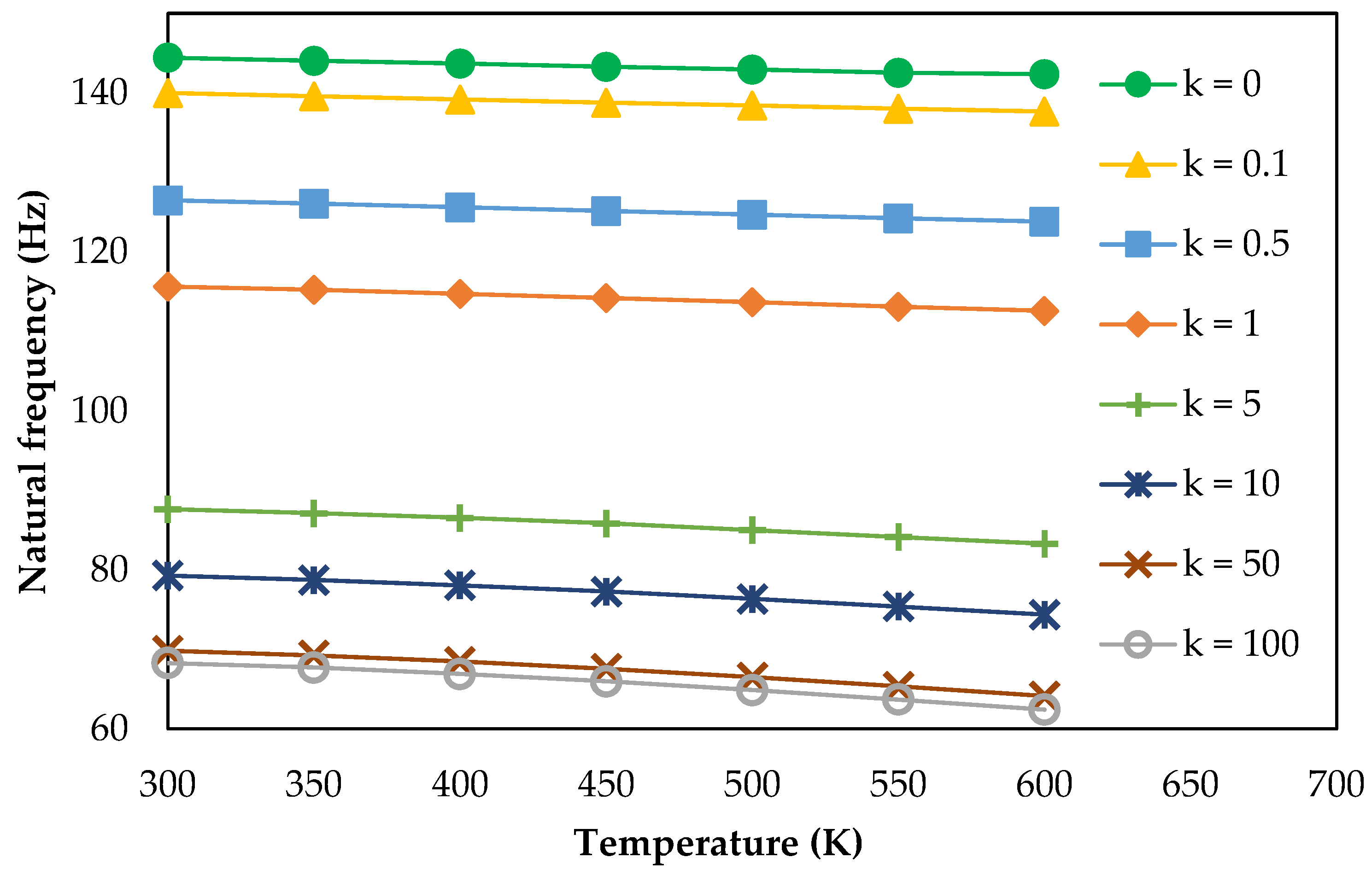
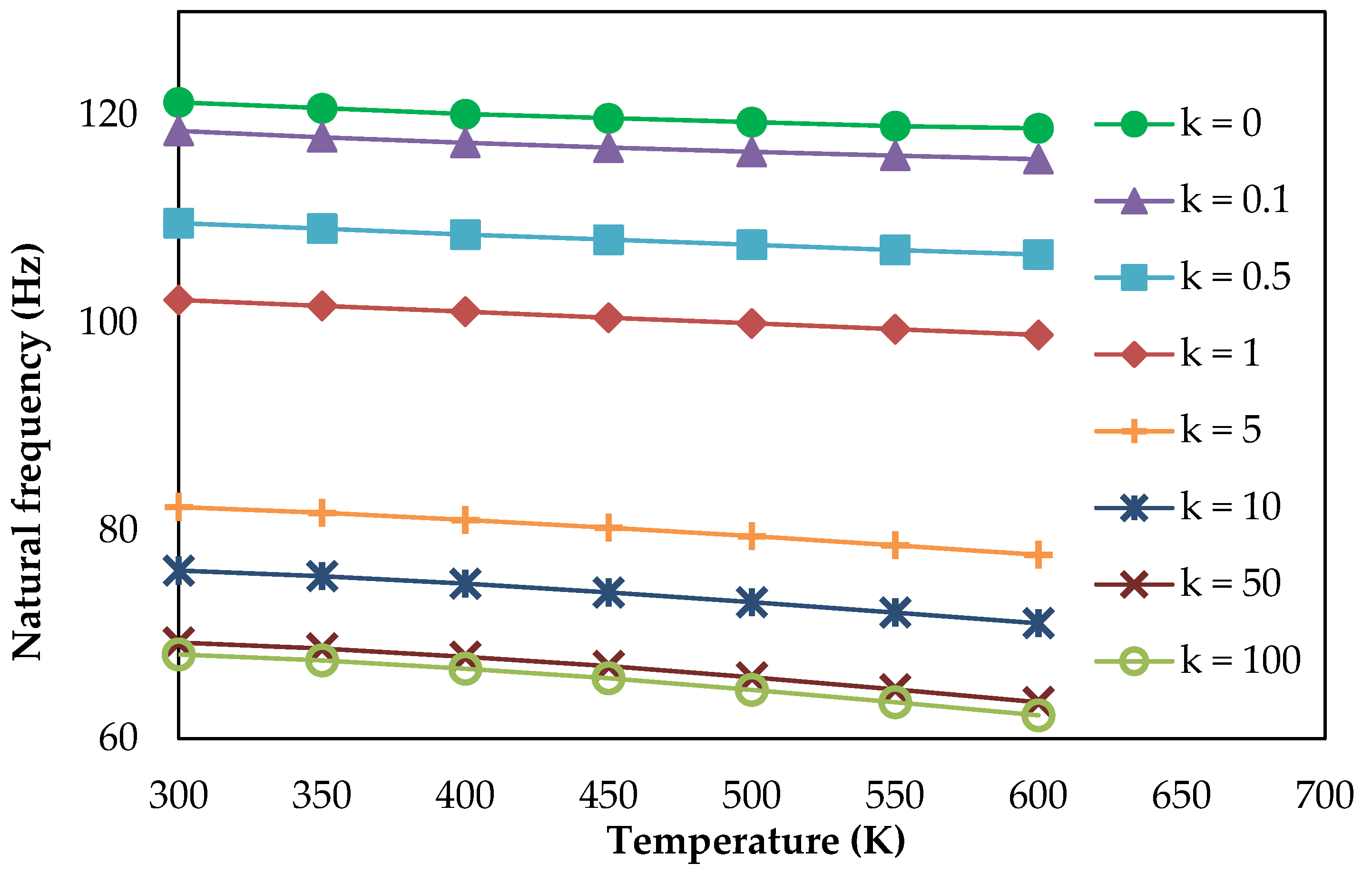
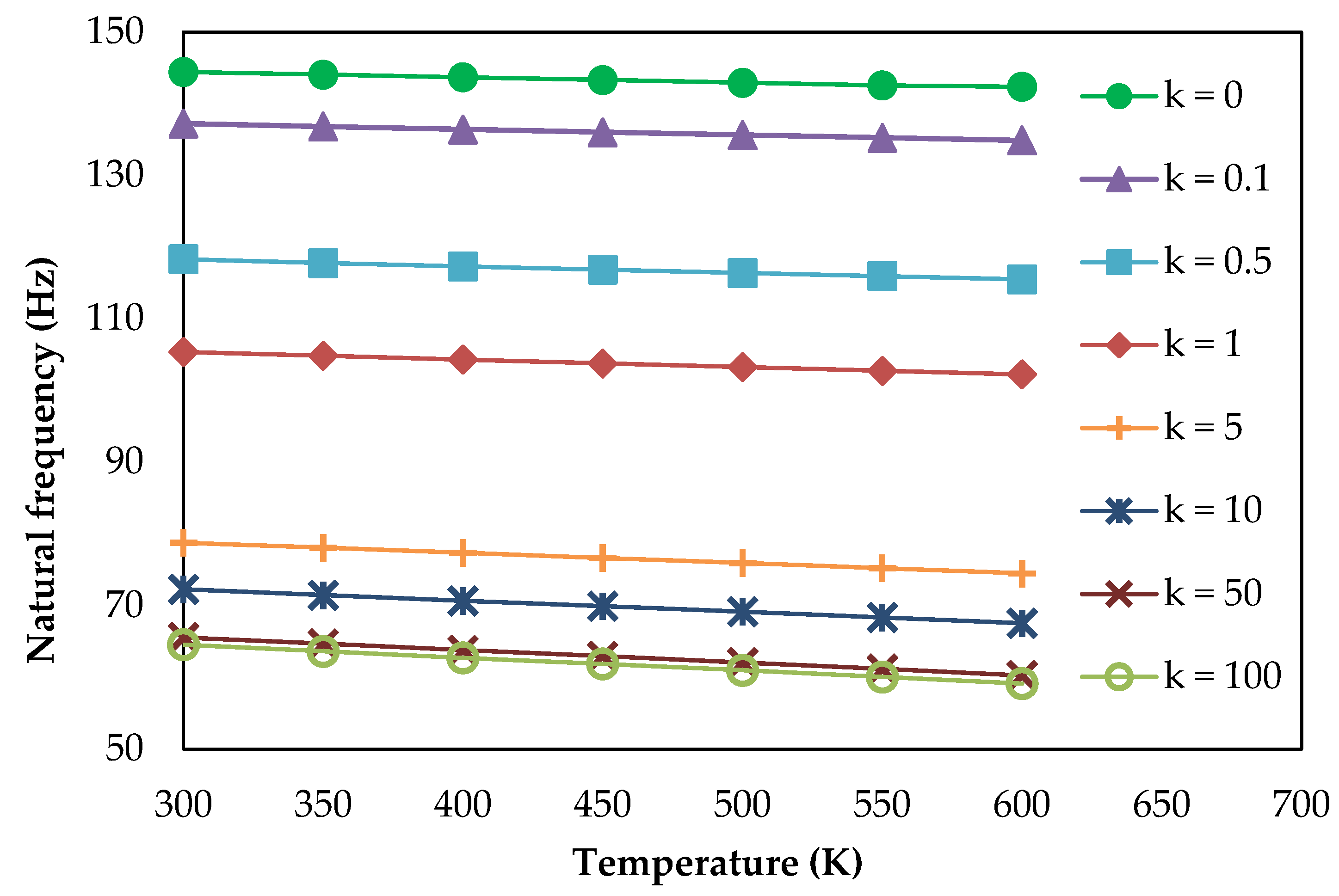
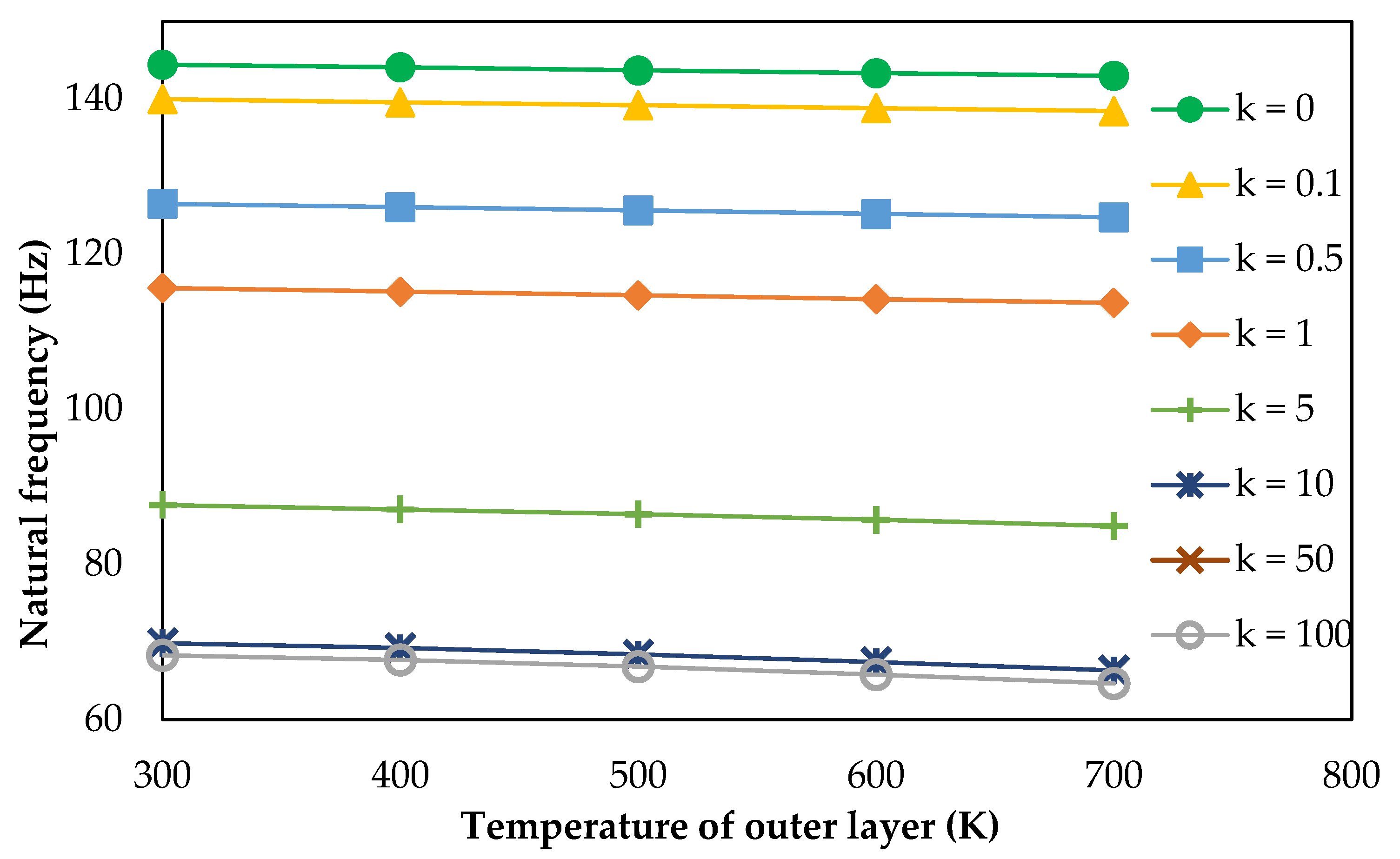
The structural and thermal analysis of the re-entry vehicle nose model made of FGM has been studied and the numerical results for the bending and buckling, as well as the vibration responses have been computed. Four FGM shell structures made of aluminum/silicon carbide, aluminum/aluminum oxide, Ti-6Al-4V/silicon carbide and Ti-6Al-4V/aluminum oxide were considered. Convergence and validation studies were performed to confirm the adopted methodology. The FGM shell with k = 1 gives uniform and linear distribution of material composition in the direction of thickness. Structural analysis of the re-entry vehicle nose model made of FGM shows that an increase in the maximum central displacement and a decrease in the critical buckling load and the natural frequency occurs due to the increase in the power law index. The bending, buckling and vibration characteristics of FGM shell are enhanced when Ti-6Al-4V is considered as a metal constituent due to the high modulus of elasticity and stiffness. Thermal buckling analysis shows that the critical buckling temperature drops with an increase in the thermal environment and the linear temperature rise of the outer layer. It was also observed that when k < 1, it causes the composition of ceramic to be more dominant, which leads to an increase in the compressive strength and the critical buckling temperature. An FGM shell with Ti-6Al-4V/silicon carbide shows good buckling characteristics in thermal applications. From vibration analysis under a thermal environment, it was concluded that the natural frequency reduces with a uniform increase in temperature, as well as in linear a temperature rise. Under various linear temperature rises of outer layer, it was concluded that the increase in shell thickness increases the critical buckling temperature and natural frequency. An FGM shell with a thickness of 0.05 m and k = 0.1 can be used for re-entry vehicle nose structures due to an enhanced critical buckling temperature. Thus, a detailed thermo-structural analysis on thin, large FGM shells for different material combinations was carried out and quantified several significant parametric results. Multiscale analysis can be performed in the future, including the wavelength and amplitude of the long strip inclusion.