Cooperation of Trailing-Edge Flap and Shock Control Bump for Robust Buffet Control and Drag Reduction
Abstract
1. Introduction
2. Problem Definition
2.1. Baseline Airfoil
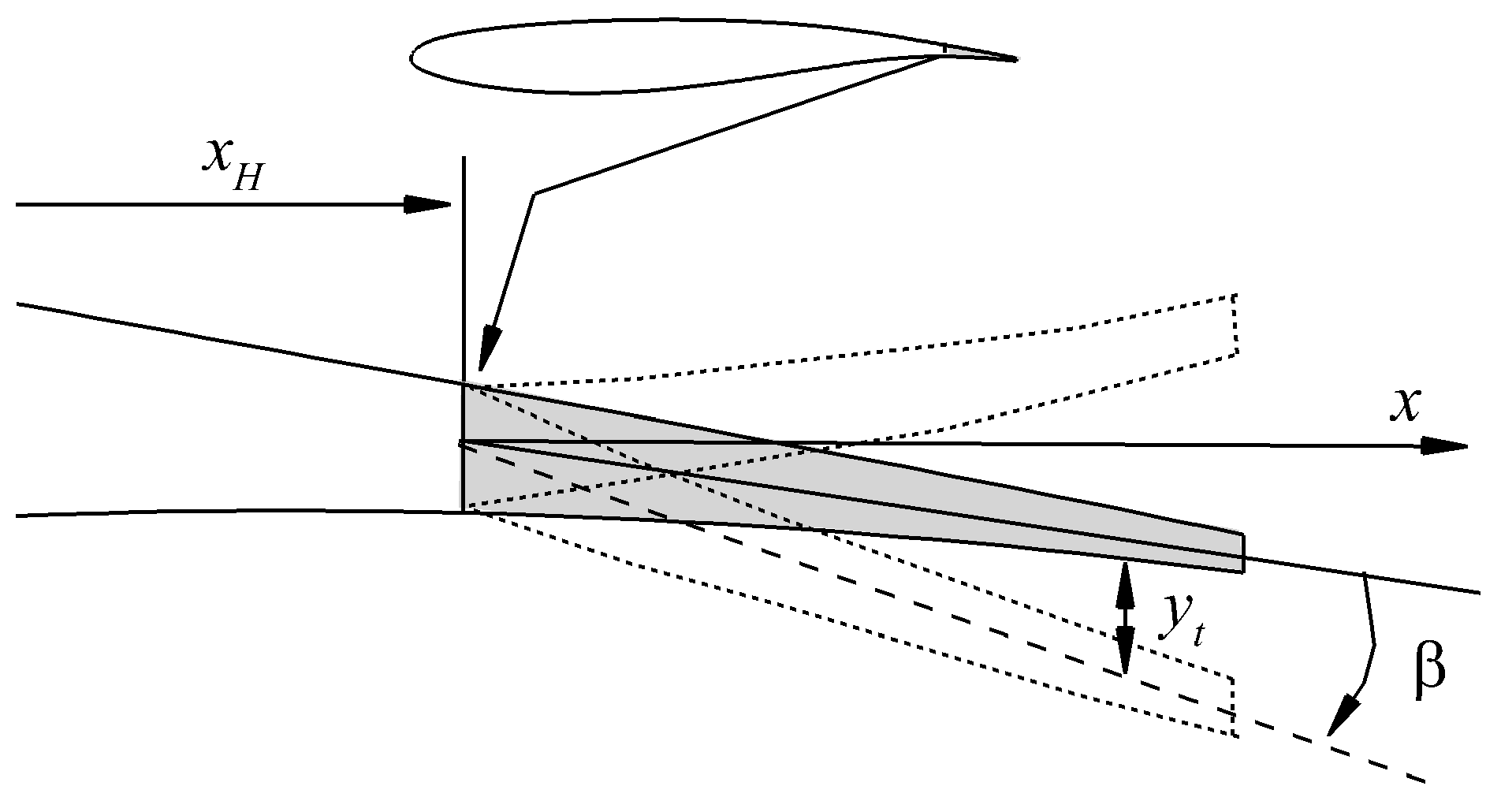
2.2. Definition of Trailing-Edge Flap
2.3. Definition of Shock Control Bump
3. Numerical Methods
3.1. Numerical Algorithm
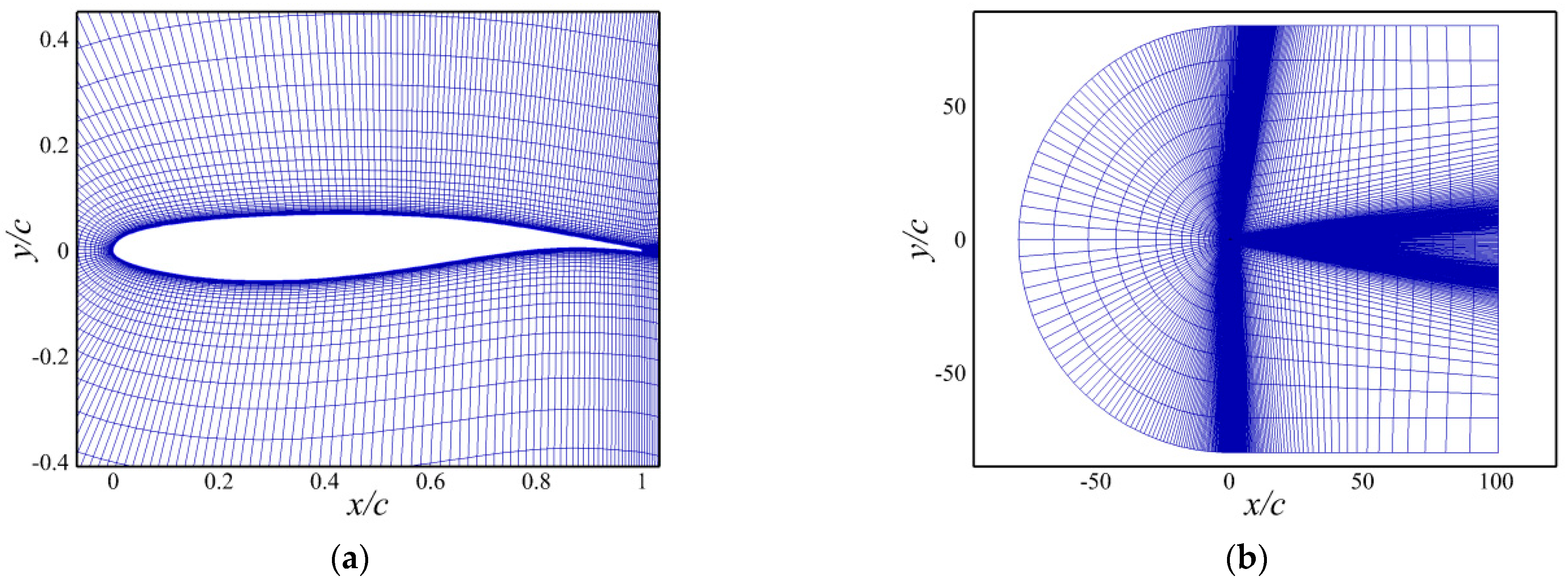
3.2. Grid Convergence Study
3.3. Numerical Validation
4. Results and Discussion
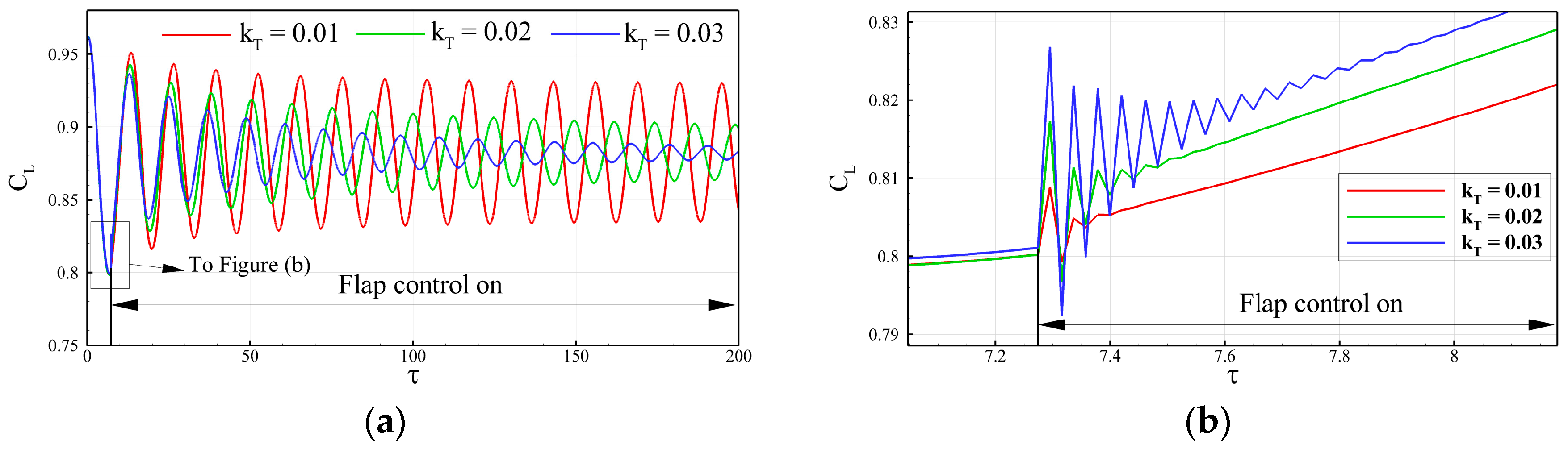
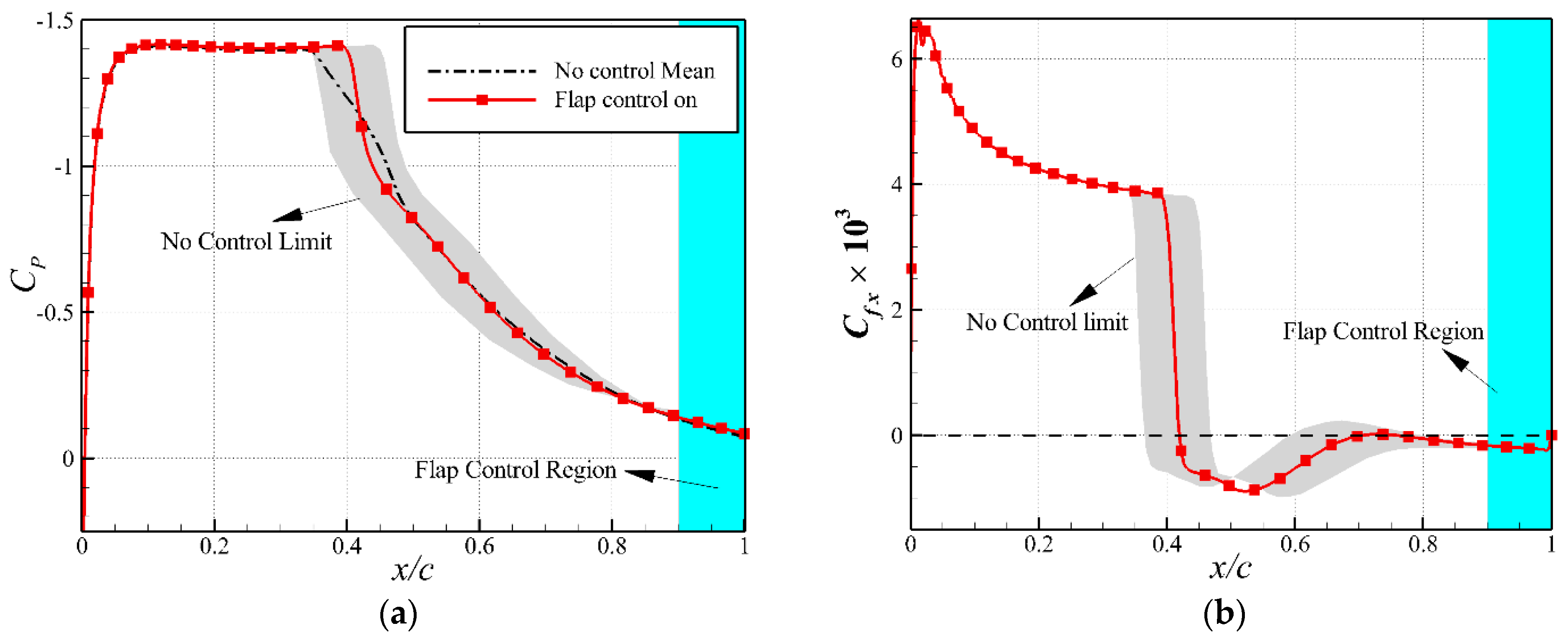
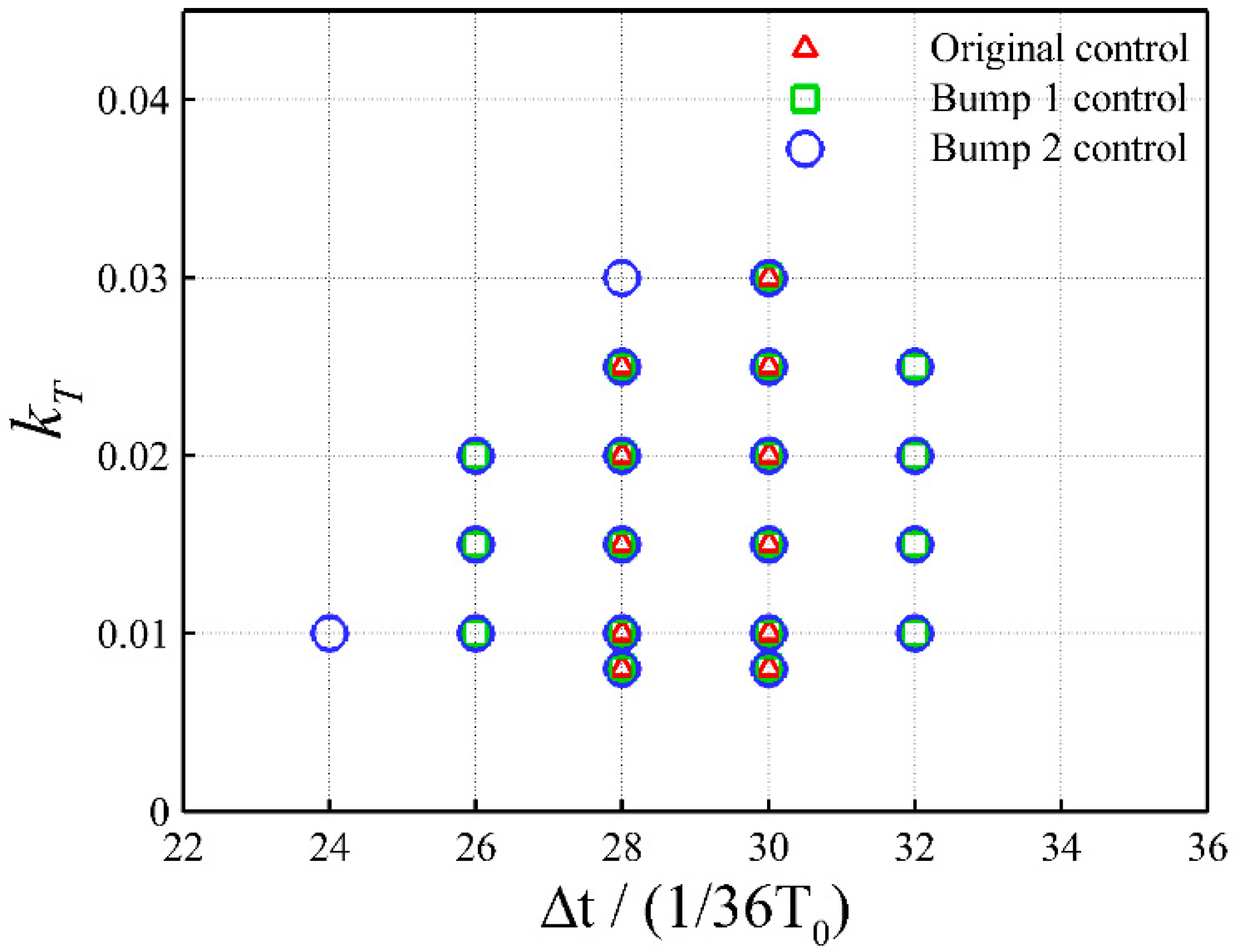
4.1. Closed-Loop Buffet Control Using the Trailing-Edge Flap
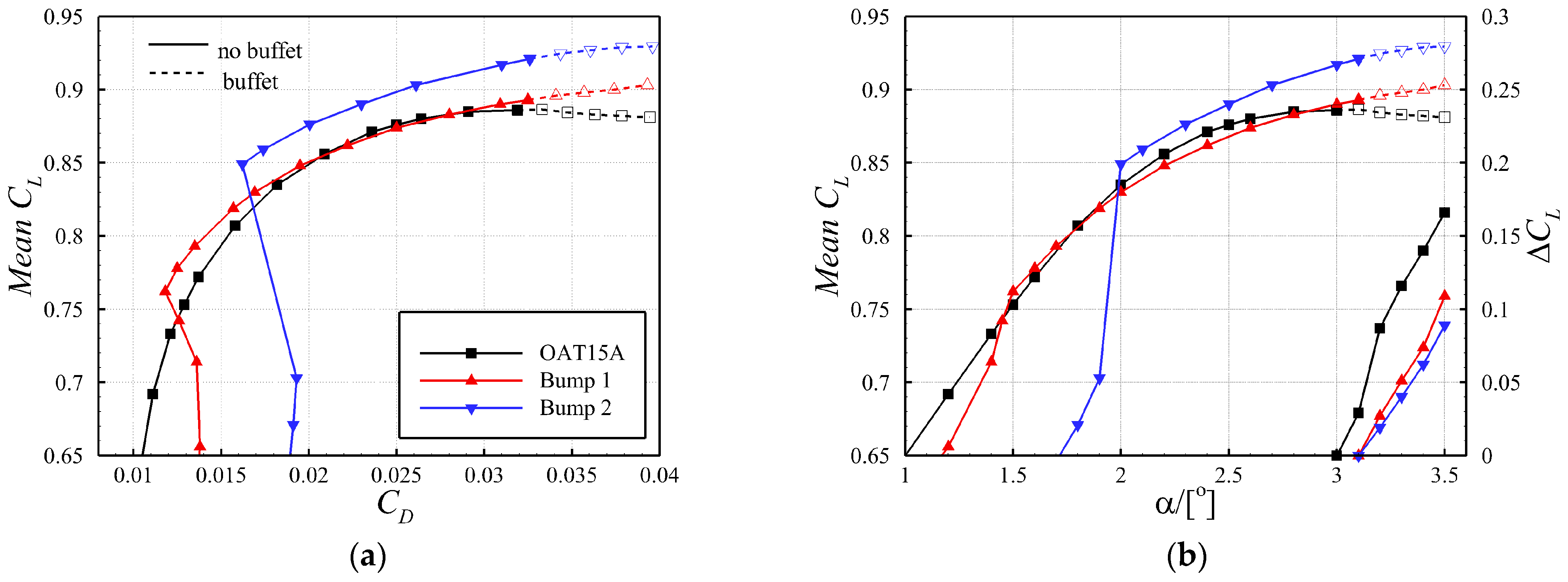
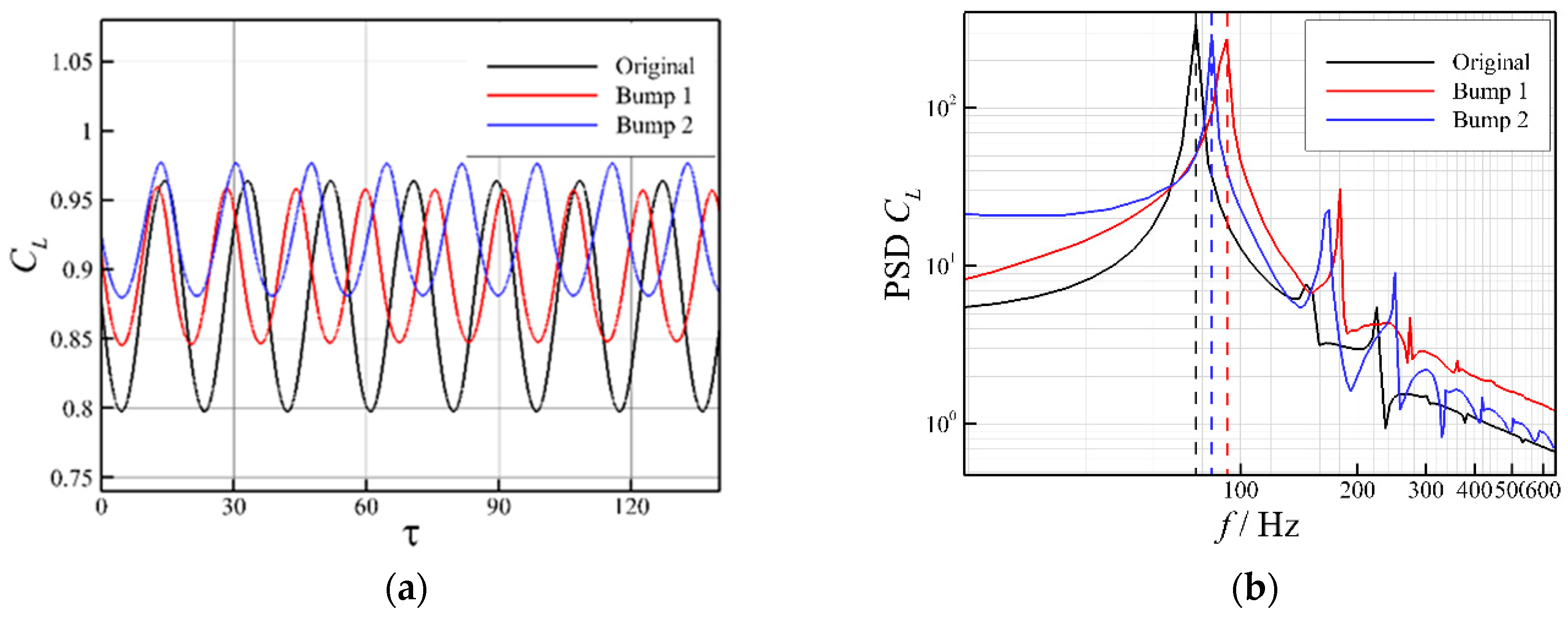
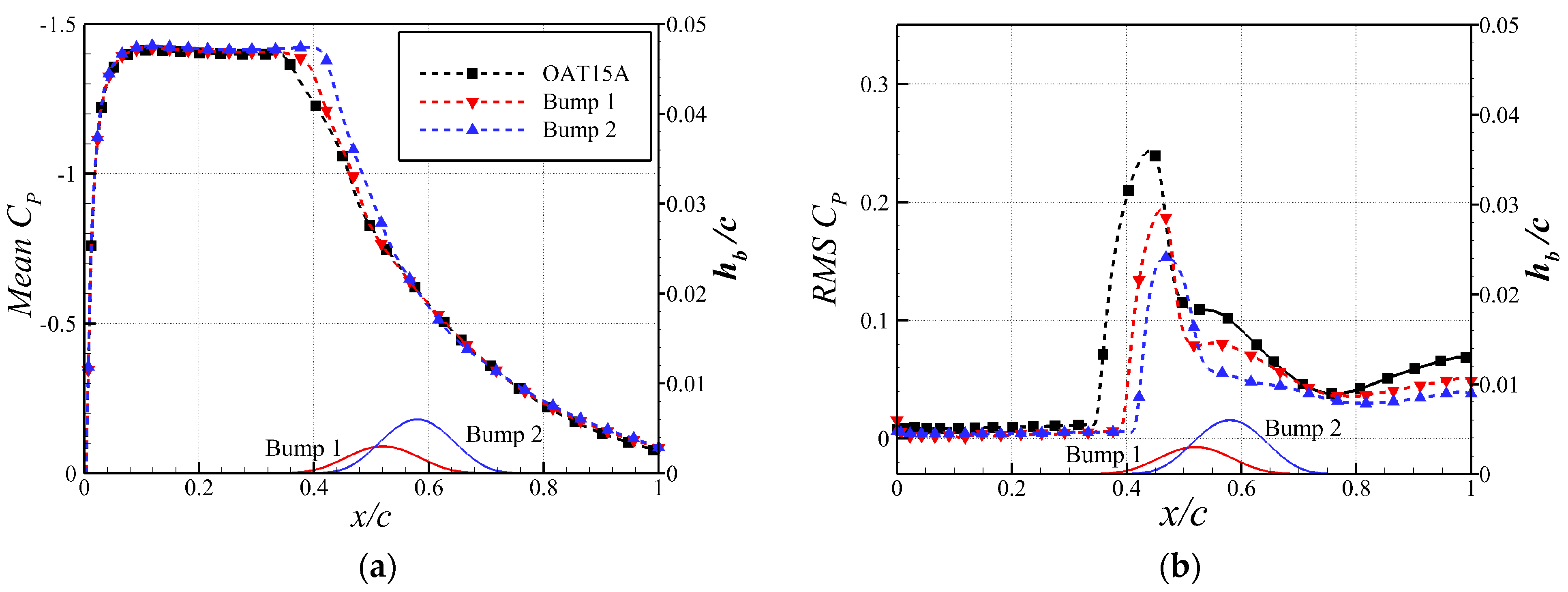
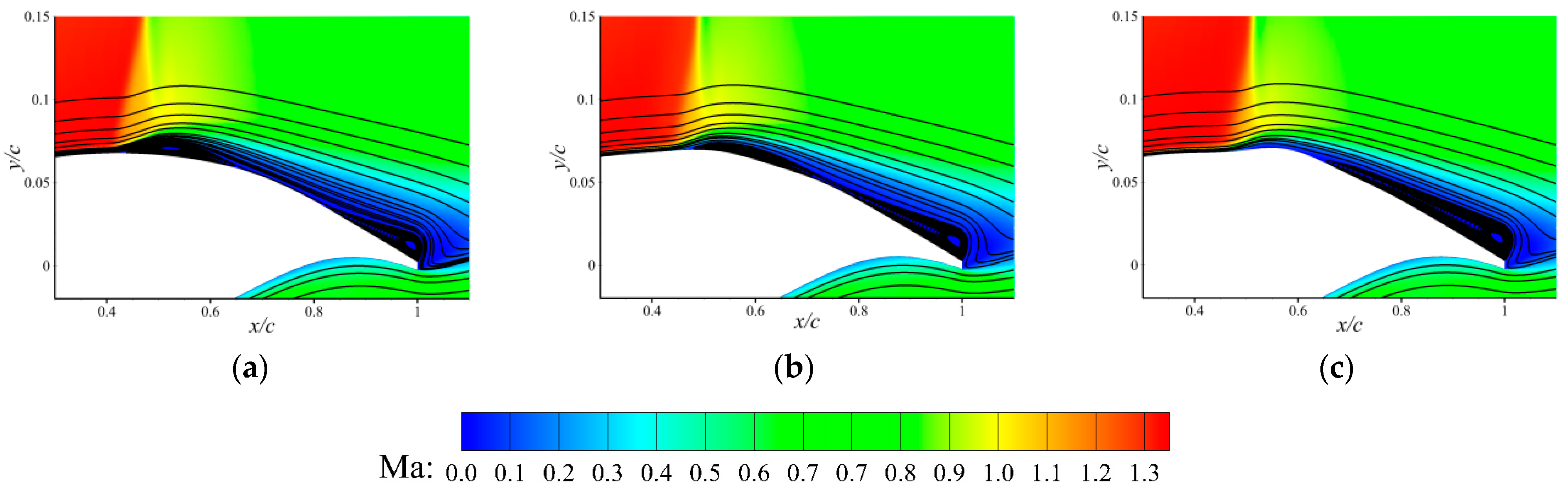
4.2. Shock Control Bumps for Drag Reduction
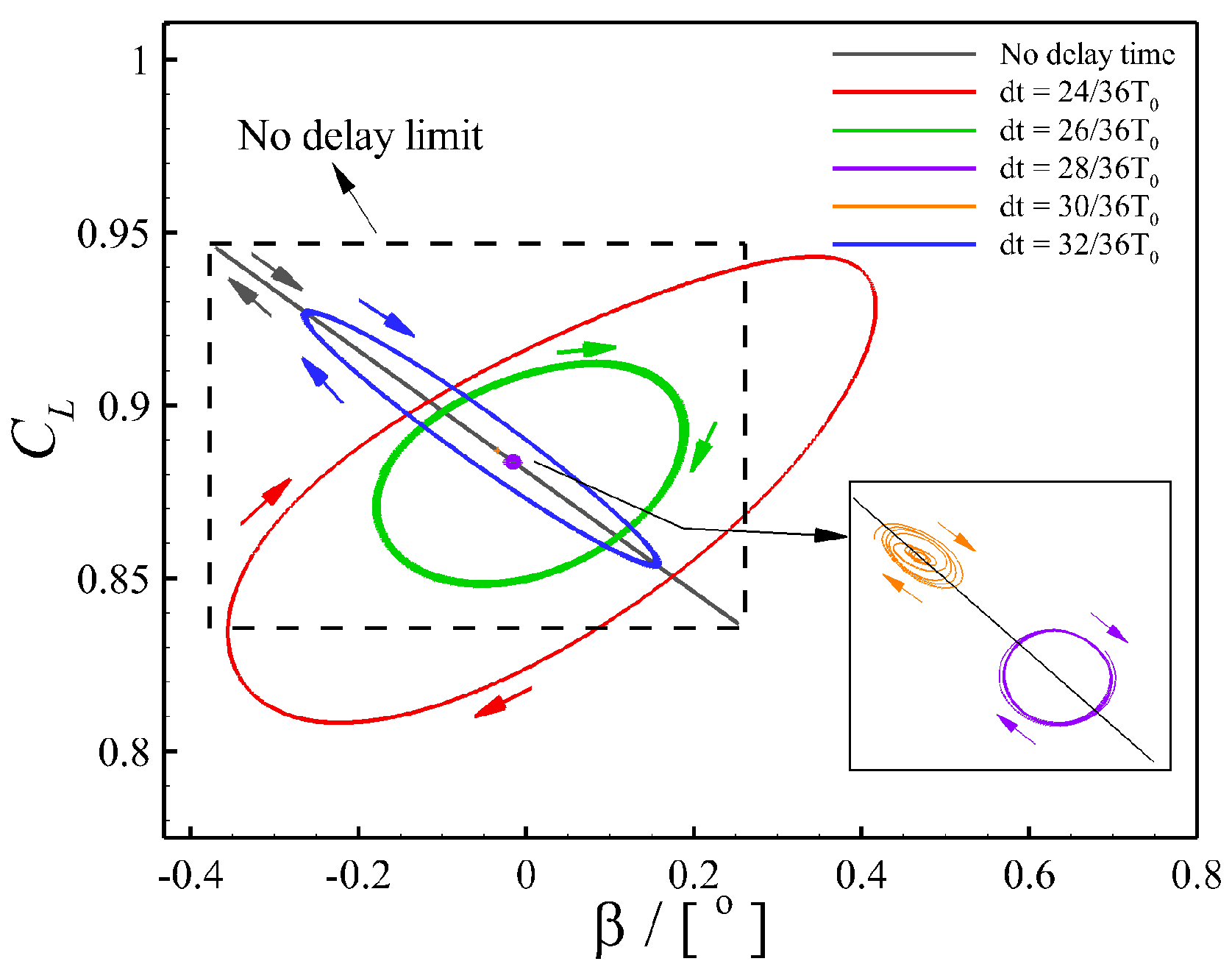
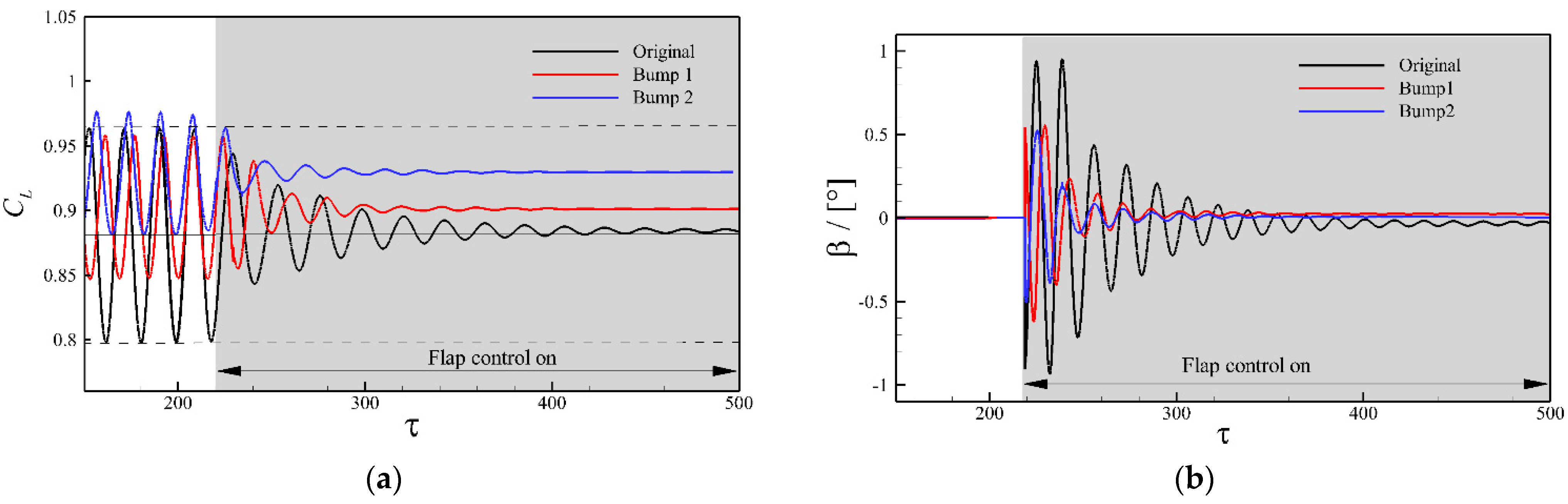
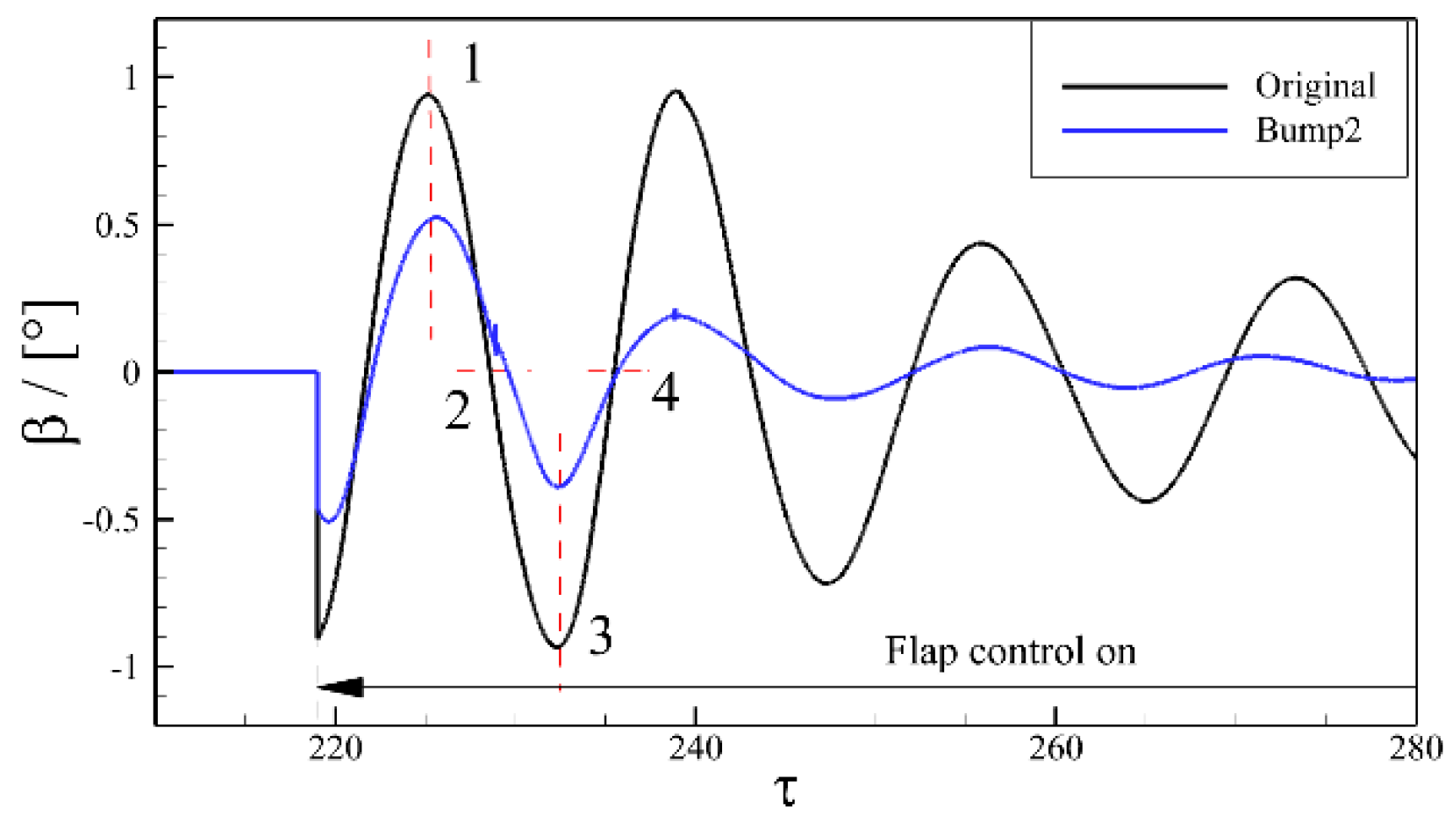
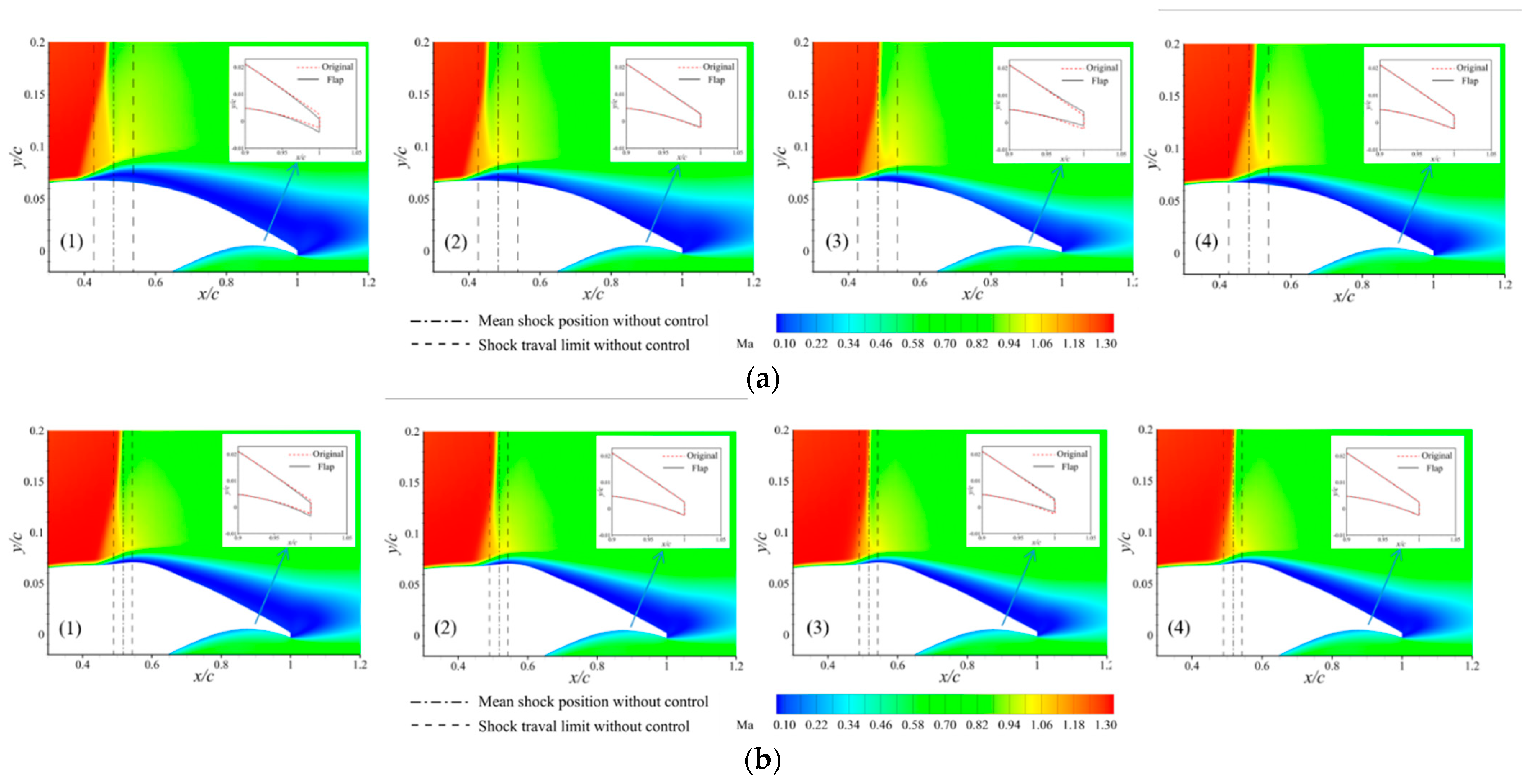
4.3. Closed-Loop Flap Buffet Control Enhanced by Shock Control Bumps
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| c | Airfoil chord length |
| Lift coefficient | |
| Drag coefficient | |
| Pressure coefficient | |
| x-component of skin friction coefficient | |
| fsb | Shock buffet frequency |
| hb | Bump height |
| kT | Gain of the closed-loop control |
| lb | Bump length |
| M∞ | Freestream Mach number |
| p | Pressure |
| Dynamic pressure | |
| Rec | Reynolds number based on the airfoil chord length |
| Shock buffet period | |
| xs | Distance between the bump crest and the mean shock location |
| xsh | Mean shock location |
| α | Freestream angle of attack |
| β | Flap deflection angle |
| τ | Non-dimensional time step |
| Delay time of the closed-loop control | |
| PSD | Power spectral density |
| RMS | Root mean square |
References
- Runze, L.I.; Kaiwen, D.; Zhang, Y.; Haixin, C. Pressure Distribution Guided Supercritical Wing Optimization. Chin. J. Aeronaut. 2018, 31, 1842–1854. [Google Scholar]
- Crouch, J.D.; Garbaruk, A.; Mmagidov, D.; Travin, A. Origin of transonic buffet on aerofoils. J. Fluid Mech. 2009, 628, 357–369. [Google Scholar] [CrossRef]
- Lee, B.H.K. Self-sustained shock oscillations on airfoils at transonic speeds. Prog. Aerosp. Sci. 2001, 37, 147–196. [Google Scholar] [CrossRef]
- Lee, B.H.K. Effects of trailing-edge flap on buffet characteristics of a supercritical airfoil. J. Aircr. 1992, 29, 93–100. [Google Scholar] [CrossRef]
- Lee, B.H.K. Oscillatory shock motion caused by transonic shock boundary-layer interaction. AIAA J. 1990, 28, 942–944. [Google Scholar] [CrossRef]
- Xiao, Q.; Tsai, H.-M.; Liu, F. Numerical Study of Transonic Buffet on a Supercritical Airfoil. AIAA J. 2006, 44, 620–628. [Google Scholar] [CrossRef]
- Deck, S. Zonal-Detached-Eddy Simulation of the Flow Around a High-Lift Configuration. AIAA J. 2005, 43, 2372–2384. [Google Scholar] [CrossRef]
- Deck, S. Numerical Simulation of Transonic Buffet over a Supercritical Airfoil. AIAA J. 2005, 43, 1556–1566. [Google Scholar] [CrossRef]
- Levy, L.L. Experimental and Computational Steady and Unsteady Transonic Flows about a Thick Airfoil. AIAA J. 1978, 16, 564–572. [Google Scholar] [CrossRef]
- Iovnovich, M.; Raveh, D.E. Reynolds-Averaged Navier-Stokes Study of the Shock-Buffet Instability Mechanism. AIAA J. 2012, 50, 880–890. [Google Scholar] [CrossRef]
- Goncalves, E.; Houdeville, R. Turbulence model and numerical scheme assessment for buffet computations. Int. J. Numer. Methods Fluids 2004, 46, 1127–1152. [Google Scholar] [CrossRef]
- Barakos, G.; Drikakis, D. Numerical simulation of transonic buffet flows using various turbulence closures. Int. J. Heat Fluid Flow 2000, 21, 620–626. [Google Scholar] [CrossRef]
- Thiery, M.; Coustols, E. URANS Computations of Shock-Induced Oscillations Over 2D Rigid Airfoils: Influence of Test Section Geometry. Flow Turbul. Combust. 2005, 74, 331–354. [Google Scholar] [CrossRef]
- Thiery, M.; Coustols, E. Numerical prediction of shock induced oscillations over a 2D airfoil: Influence of turbulence modelling and test section walls. Int. J. Heat Fluid Flow 2006, 27, 661–670. [Google Scholar] [CrossRef]
- Illi, S.; Lutz, T.; Krämer, E. On the Capability of Unsteady RANS to Predict Transonic Buffet. In Proceedings of the Third Symposium Simulation of Wing and Nacelle Stall, Braunschweig, Germany, 21–22 June 2012. [Google Scholar]
- Rumsey, C.L.; Sanetrik, M.D.; Biedron, R.T.; Melson, N.D.; Parlette, E.B. Efficiency and accuracy of time-accurate turbulent Navier-Stokes computations. Comput. Fluids 1996, 25, 217–236. [Google Scholar] [CrossRef]
- Bhamidipati, K.K.; Reasor, D.A.; Pasiliao, C.L. Unstructured Grid Simulations of Transonic Shockwave-Boundary Layer Interaction-Induced Oscillations. In Proceedings of the 22nd AIAA Computational Fluid Dynamics Conference, Reston, VA, USA, 22–26 June 2015. [Google Scholar]
- Giannelis, N.F.; Levinski, O.; Vio, G.A. Influence of Mach number and angle of attack on the two-dimensional transonic buffet phenomenon. Aerosp. Sci. Technol. 2018, 78, 89–101. [Google Scholar] [CrossRef]
- Giannelis, N.F.; Levinski, O.; Vio, G.A. Origins of atypical shock buffet motions on a supercritical aerofoil. Aerosp. Sci. Technol. 2020, 107, 106304. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Caruana, D.; Mignosi, A.; Robitaillié, C.; Corrège, M. Separated Flow and Buffeting Control. Flow Turbul. Combust. (Former. Appl. Sci. Res.) 2003, 71, 221–245. [Google Scholar] [CrossRef]
- Caruana, D.; Mignosi, A.; Corrège, M.; Le Pourhiet, A.; Rodde, A.M. “Buffet and buffeting control in transonic flow. Aerosp. Sci. Technol. 2005, 9, 605–616. [Google Scholar] [CrossRef]
- Gao, C.; Zhang, W.; Ye, Z. Numerical study on closed-loop control of transonic buffet suppression by trailing edge flap. Comput. Fluids 2016, 132, 32–45. [Google Scholar] [CrossRef]
- Dandois, J.; Lepage, A.; Dor, J.-B.; Molton, P.; Ternoy, F.; Geeraert, A.; Brunet, V.; Coustols, É. Open and Closed-Loop Control of Transonic Buffet on 3D Turbulent Wings Using Fluidic Devices. Comptes Rendus Mec. 2014, 342, 425–436. [Google Scholar] [CrossRef]
- Tian, Y.; Li, Z.; Liu, P.Q. “Upper Trailing-Edge Flap for Transonic Buffet Control. J. Aircr. 2018, 55, 382–389. [Google Scholar] [CrossRef]
- Smith, A.N.; Babinsky, H.; Fulker, J.L.; Ashill, P.R. Normal Shock Wave-Turbulent Boundary-Layer Interactions in the Presence of Streamwise Slots and Grooves. Aeronaut. J. 2002, 106, 493–500. [Google Scholar] [CrossRef]
- Holden, H.A.; Babinsky, H. Separated Shock-Boundary-Layer Interaction Control Using Streamwise Slots. J. Aircr. 2005, 42, 166–171. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Jarrett, J.P. Toward Designing with Three-Dimensional Bumps for Lift/Drag Improvement and Buffet Alleviation. AIAA J. 2012, 50, 2882–2898. [Google Scholar] [CrossRef]
- Mayer, R.; Lutz, T.; Krämer, E. Numerical Study on the Ability of Shock Control Bumps for Buffet Control. AIAA J. 2018, 56, 1978–1987. [Google Scholar] [CrossRef]
- Holden, H.; Babinsky, H. Effect of Microvortex Generators on Seperated Normal Shock/Boundary Layer Interactions. J. Aircr. 2007, 44, 170–174. [Google Scholar] [CrossRef]
- Rybalko, M.; Babinsky, H.; Loth, E. Vortex Generators for a Normal Shock/Boundary Layer Interaction with a Downstream Diffuser. J. Propuls. Power 2012, 28, 71–82. [Google Scholar] [CrossRef]
- Ashill, P.R.; Fulker, J.L.; Shires, J.L. A Novel Technique for Controlling Shock Strength of Laminar-Flow Airfoil Sections. In Proceedings of the 1st European Forum on Laminar Flow Technology, Hamburg, Germany, 16–18 March 1992; pp. 175–183. [Google Scholar]
- Birkemeyer, J.; Rosemann, H.; Stanewsky, E. Shock control on a swept wing. Aerosp. Sci. Technol. 2000, 4, 147–156. [Google Scholar] [CrossRef]
- Stanewsky, E.; Délery, J.; Fulker, J.; De Matteis, P. Synopsis of the Project EUROSHOCK II. Notes on Numerical Fluid Mechanics and Multidisciplinary Design: Drag Reduction by Shock and Boundary Layer Control-Results of the Project EUROSHOCK II; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Qin, N.; Zhu, Y.; Shaw, S. Numerical study of active shock control for transonic aerodynamics. Int. J. Numer. Methods Heat Fluid Flow 2004, 14, 444–466. [Google Scholar] [CrossRef]
- Qin, N.; Wong, W.S.; Le Moigne, A. Three-Dimensional Contour Bumps for Transonic Wing Drag Reduction. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2008, 222, 619–629. [Google Scholar] [CrossRef]
- Tian, Y.; Gao, S.; Liu, P.; Wang, J. Transonic buffet control research with two types of shock control bump based on RAE2822 airfoil. Chin. J. Aeronaut. 2017, 30, 1681–1696. [Google Scholar] [CrossRef]
- Geoghegan, J.A.; Giannelis, N.F.; Vio, G.A. A Numerical Investigation of the Geometric Parametrisation of Shock Control Bumps for Transonic Shock Oscillation Control. Fluids 2020, 5, 46. [Google Scholar] [CrossRef]
- Mayer, R.; Lutz, T.; Krämer, E.; Dandois, J. Control of Transonic Buffet by Shock Control Bumps on Wing-Body Configuration. J. Aircr. 2019, 56, 556–568. [Google Scholar] [CrossRef]
- Jacquin, L.; Molton, P.; Deck, S.; Maury, B.; Soulevant, D. Experimental Study of Shock Oscillation over a Transonic Supercritical Profile. AIAA J. 2009, 47, 1985–1994. [Google Scholar] [CrossRef]
- Grossi, F.; Braza, M.; Hoarau, Y. Prediction of Transonic Buffet by Delayed Detached-Eddy Simulation. AIAA J. 2014, 52, 2300–2312. [Google Scholar] [CrossRef]
- Huang, J.B.; Xiao, Z.X.; Liu, J.; Fu, S. Simulation of shock wave buffet and its suppression on an OAT15A supercritical airfoil by IDDES. Sci. China Ser. G Physics Mech. Astron. 2012, 55, 260–271. [Google Scholar] [CrossRef]
- Fukushima, Y.; Kawai, S. Wall-Modeled Large-Eddy Simulation of Transonic Airfoil Buffet at High Reynolds Number. AIAA J. 2018, 56, 2372–2388. [Google Scholar] [CrossRef]
- Deng, F.; Qin, N. Quantitative comparison of 2D and 3D shock control bumps for drag reduction on transonic wings. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 2344–2359. [Google Scholar] [CrossRef]
- Geoghegan, J.A.; Giannelis, N.F.; Vio, G.A. Parametric Study of Active Shock Control Bumps for Transonic Shock Buffet Alleviation. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; pp. 1–16. [Google Scholar]
- Sclafani, A.J.; DeHaan, M.A.; Vassberg, J.C.; Rumsey, C.L.; Pulliam, T.H. Drag Prediction for the Common Research Model Using CFL3D and OVERFLOW. J. Aircr. 2014, 51, 1101–1117. [Google Scholar] [CrossRef]
- Vos, R.; Farokhi, S. Introduction to Transonic Aerodynamics; Springer: Dordrecht, The Netherlands, 2015. [Google Scholar]



| Grid | Airfoil Nodes | First Layer | Cell Count | |
|---|---|---|---|---|
| L1 | 417 | 1.5 × 10−6 | 0.75 | 55,000 |
| L2 | 517 | 1.2 × 10−6 | 0.70 | 83,000 |
| L3 | 647 | 1.0 × 10−6 | 0.50 | 106,000 |
| Grid | |||
|---|---|---|---|
| L1 | 0.050 | 0.896 | 76 |
| L2 | 0.166 | 0.881 | 75 |
| L3 | 0.159 | 0.882 | 75 |
| Experiment [40] | 0.220 | - | 69 |
| Parameter | xr | hb/c | lb/c |
|---|---|---|---|
| Bump 1 | 0.52 | 0.003 | 0.4 |
| Bump 2 | 0.58 | 0.006 | 0.4 |
| Shape | ||||||
|---|---|---|---|---|---|---|
| Flap control | Off | On | Off | On | Off | On |
| Original | 0.881 | 0.883 | 0.0395 | 0.0389 | 22.3 | 22.7 |
| Bump 1 | 0.903 | 0.901 | 0.0393 | 0.0388 | 23.0 | 23.2 |
| Bump 2 | 0.930 | 0.930 | 0.0396 | 0.0392 | 23.5 | 23.7 |
| Force Coefficient | Original | Bump 1 | Bump 2 | |||
|---|---|---|---|---|---|---|
| Flap control | Off | On | Off | On | Off | On |
| CL | - | +0.0020 | +0.0120 | −0.0020 | +0.0490 | −0.0000 |
| CD | - | −0.0006 | −0.0002 | −0.0005 | +0.0001 | −0.0004 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Deng, F.; Qin, N. Cooperation of Trailing-Edge Flap and Shock Control Bump for Robust Buffet Control and Drag Reduction. Aerospace 2022, 9, 657. https://doi.org/10.3390/aerospace9110657
Zhang S, Deng F, Qin N. Cooperation of Trailing-Edge Flap and Shock Control Bump for Robust Buffet Control and Drag Reduction. Aerospace. 2022; 9(11):657. https://doi.org/10.3390/aerospace9110657
Chicago/Turabian StyleZhang, Shenghua, Feng Deng, and Ning Qin. 2022. "Cooperation of Trailing-Edge Flap and Shock Control Bump for Robust Buffet Control and Drag Reduction" Aerospace 9, no. 11: 657. https://doi.org/10.3390/aerospace9110657
APA StyleZhang, S., Deng, F., & Qin, N. (2022). Cooperation of Trailing-Edge Flap and Shock Control Bump for Robust Buffet Control and Drag Reduction. Aerospace, 9(11), 657. https://doi.org/10.3390/aerospace9110657








