Backstepping- and Sliding Mode-Based Automatic Carrier Landing System with Deck Motion Estimation and Compensation
Abstract
1. Introduction
- Considering both the airwake and the wind type disturbances. Unlike most studies dealing with automatic carrier landing affected only by the carrier airwake, in this paper, the aircraft dynamics additionally take into account the three most important wind type disturbances, i.e., the wind shears, the wind gusts, and the atmospheric turbulences. Since the aircraft attack and sideslip angles are influenced both by the airwake and the wind type disturbances, the new dynamics reflect better the motion of the airplane.
- Treating separately the prediction and the compensation problems via two independent blocks: (1) a block for the prediction of the deck motion and (2) a block for the compensation of the deck motion. The compensation of the deck motion is achieved by using the signal provided by the deck motion prediction block. This way, the aircraft can correct the position of the ideal touchdown point by using fewer signals from the ship, while the landing accuracy is improved by considering both the airplane-ship relative landing geometry and the configuration of the landing spot on the carrier. Compared to the existing literature, the prediction of the deck motion is achieved here with a recursive-least squares algorithm-based filter, while a tracking differentiator-based deck motion compensation block (TD-DMC) is used to solve the deck motion compensation problem. The TD-DMC blocks have been used so far only for obtaining the imposed values of the aircraft altitude [3]; compared to the classical DMCs, TD-DMC has a simplified structure and an easier parameter tuning.
- Obtaining anovel6-DOFdynamicswithangleofattackcontrolledbythrust. Considering the three wind type disturbances, we mathematically deduced the expressions of the new resultant disturbance type terms, and we included them in the 6-DOF dynamics of the airplane. Additionally, we considered a complete deck motion involving both maneuvering and seakeeping equations; a deterministic form is associated to the maneuvering part, while the seakeeping random motion refers to the motion affected by the wave excitation.
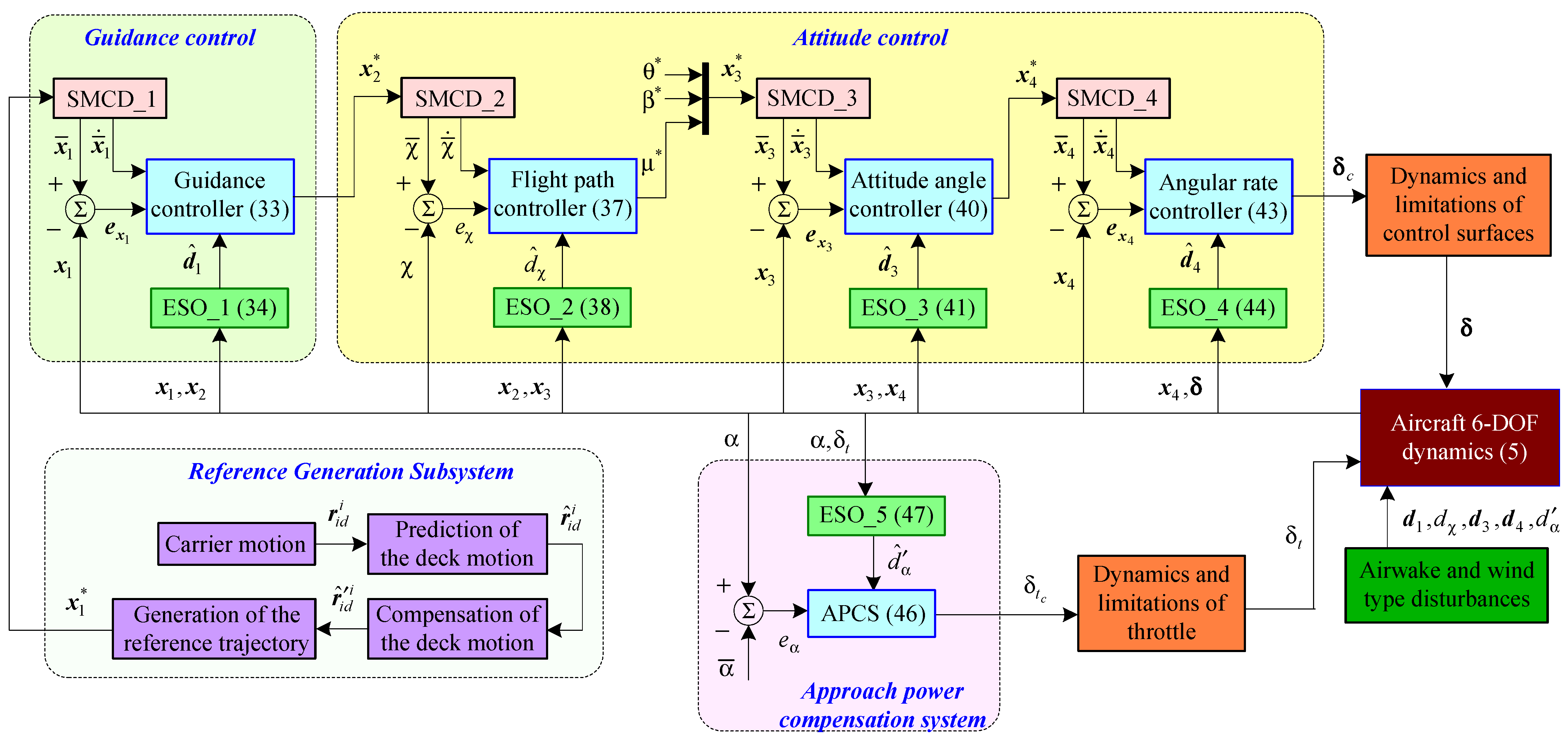
- Designing a control architecture mainly based on innovative combinations between sliding mode-based command differentiators, extended state observers, and backstepping-based controllers. Considering the influence of deck motion, airwake, and wind type disturbances, our novel ACLS has a classical configuration consisting of five control loops, i.e., guidance control, flight path angle control, control of the attitude angles, control of the angular rates, and approach power compensation subsystem. In four of the five loops, the sliding mode-based command differentiators are used to compute the virtual commands and their derivatives; then, five controllers are designed to track the generated commands. The novel ACLS is characterized by trajectory tracking capability, as well as excellent adaptability to the unexpected and even sudden changes in the state of the sea.
- Enhancing the robustness of the controllers via extended state observers. The disturbances depending on the airwake, the wind shears, the atmospheric turbulences, and the wind gusts are successfully estimated by using ESOs and then suppressed by means of the backstepping-based controllers.
2. Aircraft Dynamics during Landing
2.1. Aircraft Model
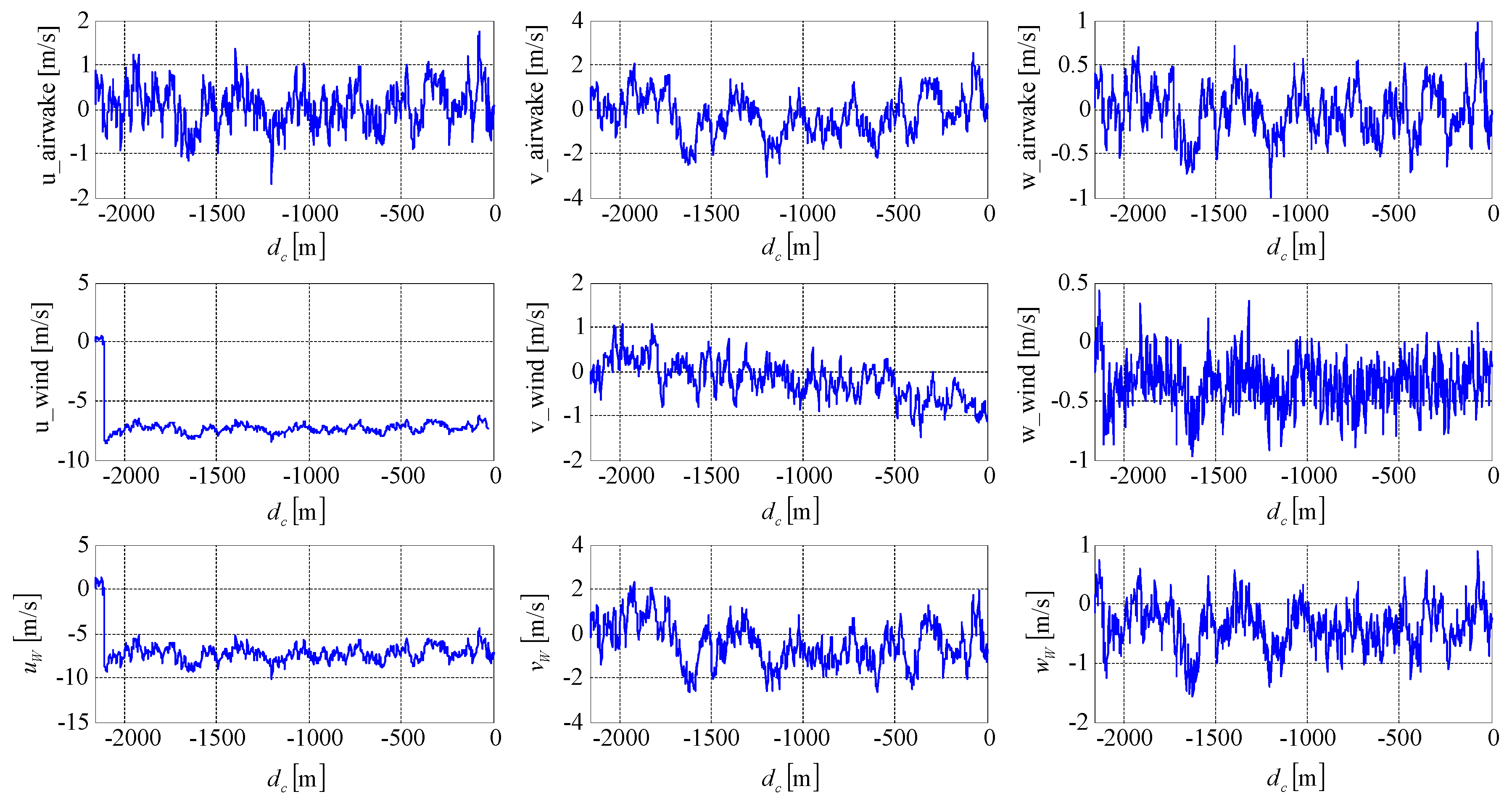
2.2. Models of Airwake and Wind Type Disturbances
2.3. Mathematical Expressions of the External Disturbances
3. Model of the Deck Motion
3.1. Deck Motion Dynamics
3.2. Prediction of the Deck Motion
3.3. Compensation of the Deck Motion
4. Automatic Carrier Landing System Design
4.1. Reference Trajectory of the Aircraft
4.2. Design of the Sliding Mode-Based Command Differentiator
4.3. Design of the Controllers
4.4. Stability Analysis
5. Numerical Simulations
5.1. Numerical Simulation Setup
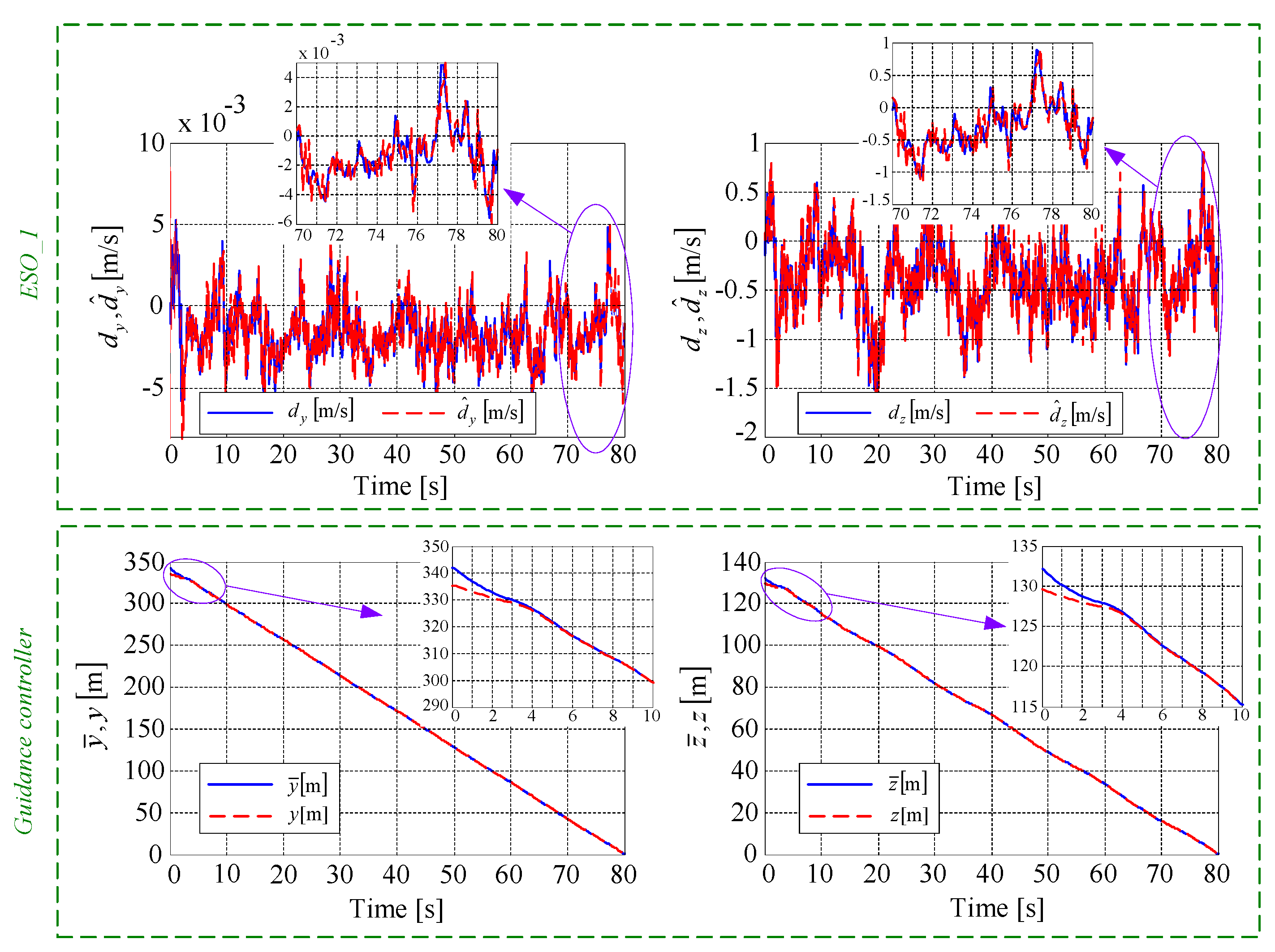
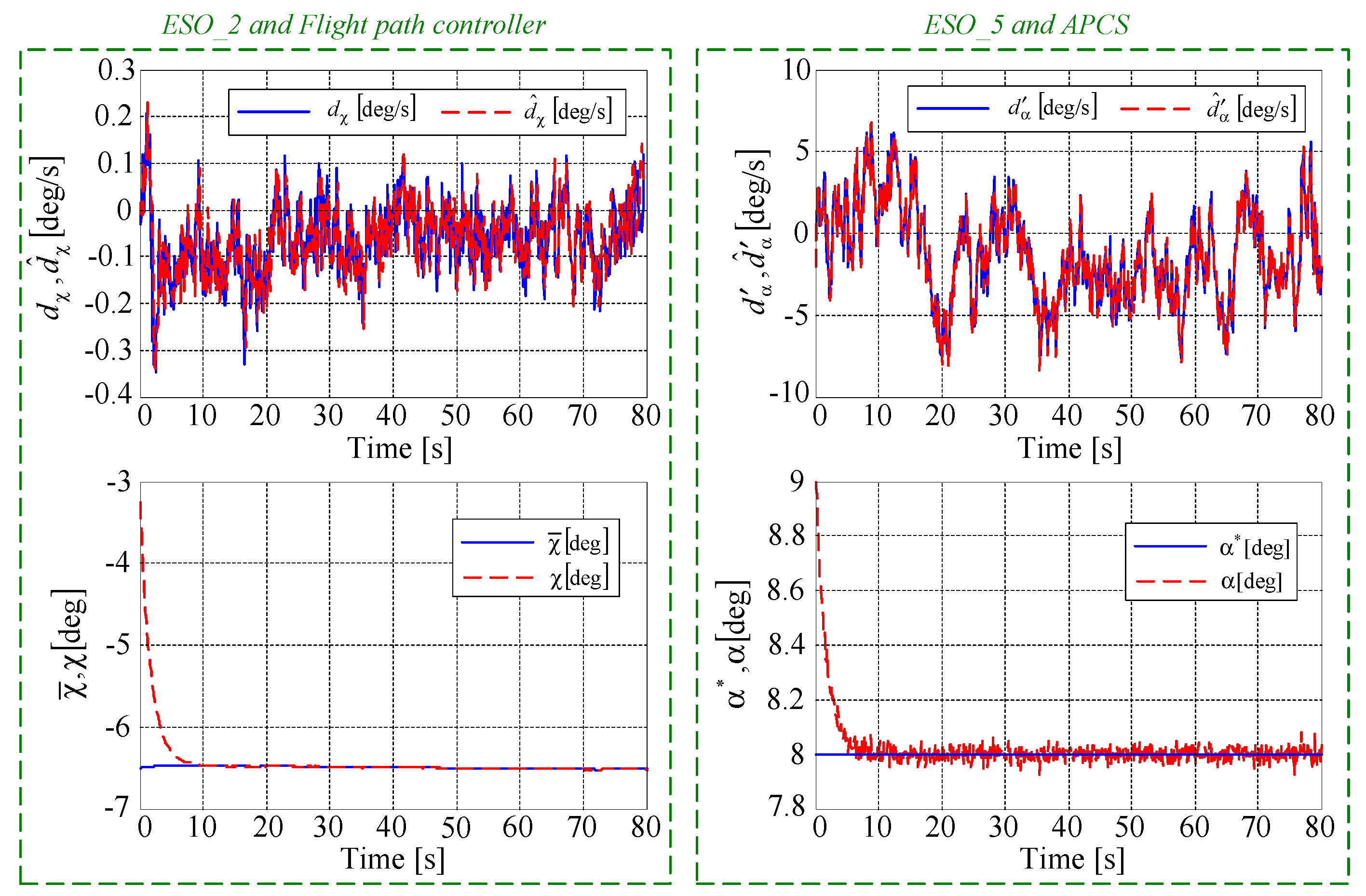
5.2. Simulation Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Aerodynamic and Geometric Parameters [32]
| Meaning | Symbol | Value | Meaning | Symbol | Value |
| Aircraft mass | m | 1587.59 kg | Roll moment of inertia | Ix | 1016.863 kg·m2 |
| Wing area | S | 12.5348 m2 | Pitch moment of inertia | Iy | 6236.762 kg·m2 |
| Wingspan | b | 8.016 m | Yaw moment of inertia | Iz | 6779.089 kg·m2 |
| Aerodynamic mean chord | 1.6459 m | Product moment of inertia | Ixz | 271.164 kg·m2 |
| Meaning | Rolling moment coefficients | Meaning | Yawing moment coefficients | ||||||||
| Symbol | Symbol | ||||||||||
| Value | 0 | −0.14 | −0.35 | 0.56 | 0.03 | 0.11 | Value | −0.07 | −0.6 | −15.7 | −0.9 |
| Meaning | Pitching moment coefficients | Meaning | Lift force coefficients | ||||||||
| Symbol | Symbol | ||||||||||
| Value | 0 | 0.16 | −0.03 | −0.31 | −0.11 | −0.03 | Value | 0.65 | 5 | 9 | 0.39 |
| Meaning | Lateral force coefficients | Meaning | Drag force coefficients | ||||||||
| Symbol | Symbol | ||||||||||
| Value | 0 | −0.94 | 0.01 | 0.59 | 0.26 | 0 | Value | 0.09 | 1.14 | 0 | 0 |
Appendix B. Variables and Vectors for Aircraft Dynamics
References
- Zhen, Z.; Yu, C.; Jiang, S.; Jiang, J. Adaptive super-twisting control for automatic carrier landing of aircraft. IEEE Trans. Aerosp. Elec. Syst. 2020, 56, 984–997. [Google Scholar] [CrossRef]
- Guan, Z.; Liu, H.; Zheng, Z.; Ma, Y.; Zhu, T. Moving path following with integrated direct lift control for carrier landing. Aerosp. Sci. Technol. 2022, 120, 107247. [Google Scholar] [CrossRef]
- Yu, Y.; Wang, H.; Li, N.; Su, Z.; Wu, J. Automatic carrier landing system based on active disturbance rejection control with a novel parameters optimizer. Aerosp. Sci. Technol. 2017, 69, 149–160. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Z.; Zhu, Q.; Wen, Z. Longitudinal automatic carrier-landing control law rejecting disturbances and coupling based on adaptive dynamic inversion. Bull. Pol. Acad. Tech. Sci. 2021, 69, 1. [Google Scholar]
- Bian, Q.; Nener, B.; Wang, J.; Liu, X.; Ma, J. A fitness sharing based ant clustering method for multimodal optimization of the aircraft longitudinal automatic carrier landing system. Aerosp. Sci. Technol. 2022, 122, 107392. [Google Scholar] [CrossRef]
- Yuan, S.; Yang, Y. Design of automatic carrier landing system using H∞ control. In Proceedings of the 3rd IEEE World Congress on Intelligent Control and Automation, Hefei, China, 26 June–2 July 2000; pp. 3449–3451. [Google Scholar]
- Zhen, Z.; Tao, G.; Yu, C.; Xue, Y. A multivariable adaptive control scheme for automatic carrier landing of UAV. Aerosp. Sci. Technol. 2019, 92, 714–721. [Google Scholar] [CrossRef]
- Zhu, Q.; Yang, Z. Design of air-wake rejection control for longitudinal automatic carrier landing cyber-physical system. Comp. Electr. Eng. 2020, 84, 106637. [Google Scholar] [CrossRef]
- Singh, S.; Padhi, R. Automatic path planning and control design for autonomous landing of UAVs using dynamic inversion. In Proceedings of the IEEE American Control Conference, Seattle, WA, USA, 11–13 June 2008; pp. 2409–2414. [Google Scholar]
- Li, J.; Duan, H. Simplified brain storm optimization approach to control parameter optimization in F/A-18 automatic carrier landing system. Aerosp. Sci. Technol. 2015, 42, 187–195. [Google Scholar] [CrossRef]
- Deng, Y.; Duan, H. Control parameter design for automatic carrier landing system via pigeon-inspired optimization. Nonlin. Dyn. 2016, 85, 97–106. [Google Scholar] [CrossRef]
- Dou, R.; Duan, H. Lévy flight based pigeon-inspired optimization for control parameters optimization in automatic carrier landing system. Aerosp. Sci. Technol. 2017, 61, 11–20. [Google Scholar] [CrossRef]
- Zhen, Z.; Jiang, S.; Jiang, J. Automatic carrier landing control for unmanned aerial vehicles based on preview control and particle filtering. Aerosp. Sci. Technol. 2018, 81, 99–107. [Google Scholar] [CrossRef]
- Li, P.; Yu, X.; Zhang, Y.; Peng, X.Y. Adaptive multivariable integral TSMC of a hypersonic gliding vehicle with actuator faults and model uncertainties. IEEE/ASME Trans. Mechatr. 2017, 22, 2723–2735. [Google Scholar] [CrossRef]
- Koo, S.; Kim, S.; Suk, J.; Kim, Y.; Shin, J. Improvement of shipboard landing performance of fixed-wing UAV using model predictive control. Int. J. Contr. Autom. Syst. 2018, 16, 2697–2708. [Google Scholar] [CrossRef]
- Lorenzetti, J.; McClellan, A.R.; Farhat, C.; Pavone, M. UAV aircraft carrier landing using CFD-based model predictive control. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; p. 1721. [Google Scholar]
- Misra, G.; Bai, X. Output-feedback stochastic model predictive control for glideslope tracking during aircraft carrier landing. J. Guid. Con. Dyn. 2019, 42, 2098–2105. [Google Scholar] [CrossRef]
- Lungu, M. Auto-landing of UAVs with variable centre of mass using the backstepping and dynamic inversion control. Aerosp. Sci. Technol. 2020, 103, 105912. [Google Scholar] [CrossRef]
- Wang, X.; Chen, X.; Wen, L. Adaptive disturbance rejection control for automatic carrier landing system. Mathem. Prob. Eng. 2016, 7345056, 1–12. [Google Scholar] [CrossRef]
- Guan, Z.; Ma, Y.; Zheng, Z. Moving path following with prescribed performance and its application on automatic carrier landing. IEEE Trans. Aerosp. Elec. Syst. 2019, 56, 2576–2590. [Google Scholar] [CrossRef]
- Guan, Z.; Ma, Y.; Zheng, Z.; Guo, N. Prescribed performance control for automatic carrier landing with disturbance. Nonlin. Dyn. 2019, 94, 1335–1349. [Google Scholar] [CrossRef]
- Guan, Z.; Liu, H.; Zheng, Z.; Lungu, M.; Ma, Y. Fixed-time control for automatic carrier landing with disturbance. Aerosp. Sci. Technol. 2021, 108, 106403. [Google Scholar] [CrossRef]
- Basin, M. Finite-and fixed-time convergent algorithms: Design and convergence time estimation. Ann. Rev. Contr. 2019, 48, 209–221. [Google Scholar] [CrossRef]
- Li, C.; Liu, G.; Hong, G. A method of F-18/A carrier landing position prediction based on back propagation neural network. In Proceedings of the 7th IEEE International Conference on Mechanical and Aerospace Engineering (ICMAE), London, UK, 18–20 June 2016; pp. 507–511. [Google Scholar]
- Xue, Y.; Zhen, Z.; Yang, L.Q.; Wen, L.D. Adaptive fault-tolerant control for carrier-based UAV with actuator failures. Aerosp. Sci. Technol. 2020, 107, 106227. [Google Scholar] [CrossRef]
- Boskovic, J.; Redding, J. An autonomous carrier landing system for unmannned aerial vehicles. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, Chicago, IL, USA, 10–13 August 2009. [Google Scholar]
- Xia, G.; Dong, R.; Xu, J.; Zhu, Q. Linearized model of carrier-based aircraft dynamics in final-approach air condition. J. Aircr. 2016, 53, 33–47. [Google Scholar] [CrossRef]
- Yu Wu, Z.; Ni, J.; Qian, W.; Bu, X.; Liu, B. Composite prescribed performance control of small unmanned aerial vehicles using modified nonlinear disturbance observer. ISA Trans. 2021, 116, 30–45. [Google Scholar] [CrossRef] [PubMed]
- Tang, P.; Dai, Y.; Chen, J. Nonlinear Robust Control on Yaw Motion of a Variable-Speed Unmanned Aerial Helicopter under Multi-Source Disturbances. Aerospace 2022, 9, 42. [Google Scholar] [CrossRef]
- Chang, J.; Cieslak, J.; Guo, Z.; Henry, D. On the synthesis of a sliding-mode-observer-based adaptive fault-tolerant flight control scheme. ISA Trans. 2021, 111, 8–23. [Google Scholar] [CrossRef]
- Lee, S.; Lee, J.; Lee, S.; Choi, H.; Kim, Y.; Kim, S.; Suk, J. Sliding mode guidance and control for UAV carrier landing. IEEE Trans. Aerosp. Elec. Syst. 2018, 55, 951–966. [Google Scholar] [CrossRef]
- Kus, M. Autonomous Carrier Landing of a Fixed-Wing UAV with Airborne Deck Motion Estimation. Master’s Thesis, University of Texas at Arlington, Arlington, TX, USA, 2019. [Google Scholar]
- Duan, H.; Chen, L.; Zeng, Z. Automatic Landing for Carrier-based Aircraft under the Conditions of Deck Motion and Carrier Airwake Disturbances. IEEE Trans. Aerosp. Elec. Syst. 2022. [CrossRef]
- Su, Z.; Wang, H.; Yao, P.; Huang, Y.; Qin, Y. Back-stepping based anti-disturbance flight controller with preview methodology for autonomous aerial refueling. Aerosp. Sci. Technol. 2017, 61, 95–108. [Google Scholar] [CrossRef]
- Misra, G.; Gao, T.; Bai, X. Modeling and simulation of UAV carrier landings. AIAA Sci. Forum 2019, 1981. [Google Scholar] [CrossRef]
- Ma, Y.; Guan, Z.; Zheng, Z. Nonlinear control for automatic carrier landing with deck motion compensation. In Proceedings of the 37th IEEE Chinese Control Conference, Wuhan, China, 25–27 July 2018; pp. 9883–9888. [Google Scholar]
- Salazar, L.; Cobano, J.; Ollero, A. Small UAS-based wind feature identification system Part 1: Integration and Validation. Sensors 2016, 17, 8. [Google Scholar] [CrossRef]
- Brezoescu, C.A. Small Lightweight Aircraft Navigation in the Presence of Wind. Ph.D. Thesis, Université de Technologie de Compičgne, Compiègne, France, 2013. [Google Scholar]
- Che, J.; Chen, D. Automatic landing control using H-inf control and stable inversion. In Proceedings of the 40th Conference on Decision and Control, Orlando, FL, USA, 4–7 December 2001; pp. 241–246. [Google Scholar]
- Frost, W.; Bowles, R. Wind shear terms in the equations of aircraft motion. J. Aircraft 1984, 21, 866–872. [Google Scholar] [CrossRef]
- Napolitano, M.R. Aircraft Dynamics: From Modeling to Simulation; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Lungu, M. Auto-landing of fixed wing unmanned aerial vehicles using the backstepping control. ISA Trans. 2019, 95, 194–210. [Google Scholar] [CrossRef]
- Islam, S.; Bernstein, D. Recursive least squares for real-time implementation [lecture notes]. IEEE Control Syst. Magaz. 2019, 39, 82–85. [Google Scholar] [CrossRef]
- Han, J. From PID to active disturbance rejection control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Ran, M.; Li, J.; Xie, L. A new extended state observer for uncertain nonlinear systems. Automatica 2021, 131, 109772. [Google Scholar] [CrossRef]
- Witkowska, A.; Smierzchalski, R. Tuning of Parameters Backstepping Ship Course Controller by Genetic Algorithm. In Advances in Information Processing and Protection; Pejaś, J., Saeed, K., Eds.; Springer: Boston, MA, USA, 2007; pp. 159–168. [Google Scholar] [CrossRef]
- Wache, A.; Aschemann, H. Self-Tuning of Adaptive Backstepping Control for Reference Tracking. IFAC-PapersOnLine 2021, 54, 313–318. [Google Scholar] [CrossRef]
- Fossen, T.I. Guidance and Control of Ocean Vehicles. Doctors Thesis, University of Trondheim, Trondheim, Norway; John Wiley & Sons: Chichester, UK, 1999. [Google Scholar]
- Yuan, Y.; Duan, H.; Zeng, Z. Automatic Carrier Landing Control with External Disturbance and Input Constraint. IEEE Trans. Aerosp. Electron. Syst. 2022, 1–16. [Google Scholar] [CrossRef]
- Lungu, M. Control of double gimbal control moment gyro systems using the backstepping control method and a nonlinear disturbance observer. Acta Astron. 2021, 180, 639–649. [Google Scholar] [CrossRef]
- Jung, U.; Cho, M.; Woo, J.; Kim, C. Trajectory-Tracking Controller Design of Rotorcraft Using an Adaptive Incremental-Backstepping Approach. Aerospace 2021, 8, 248. [Google Scholar] [CrossRef]





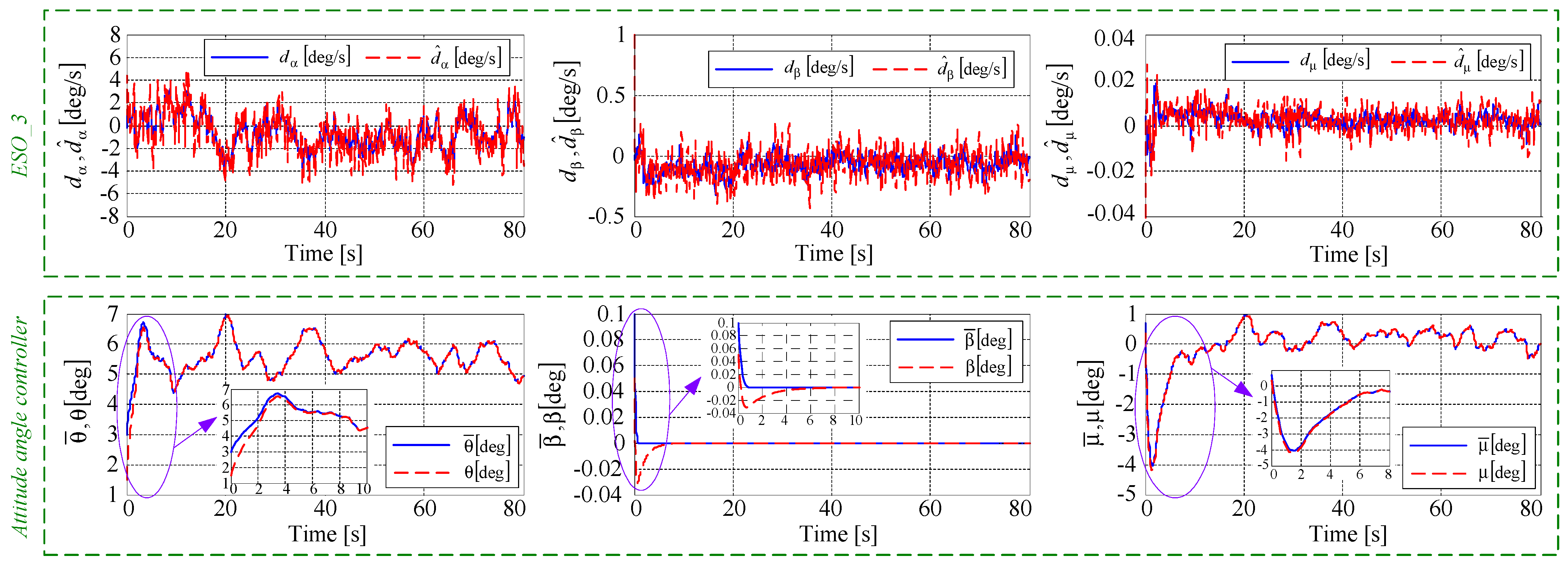
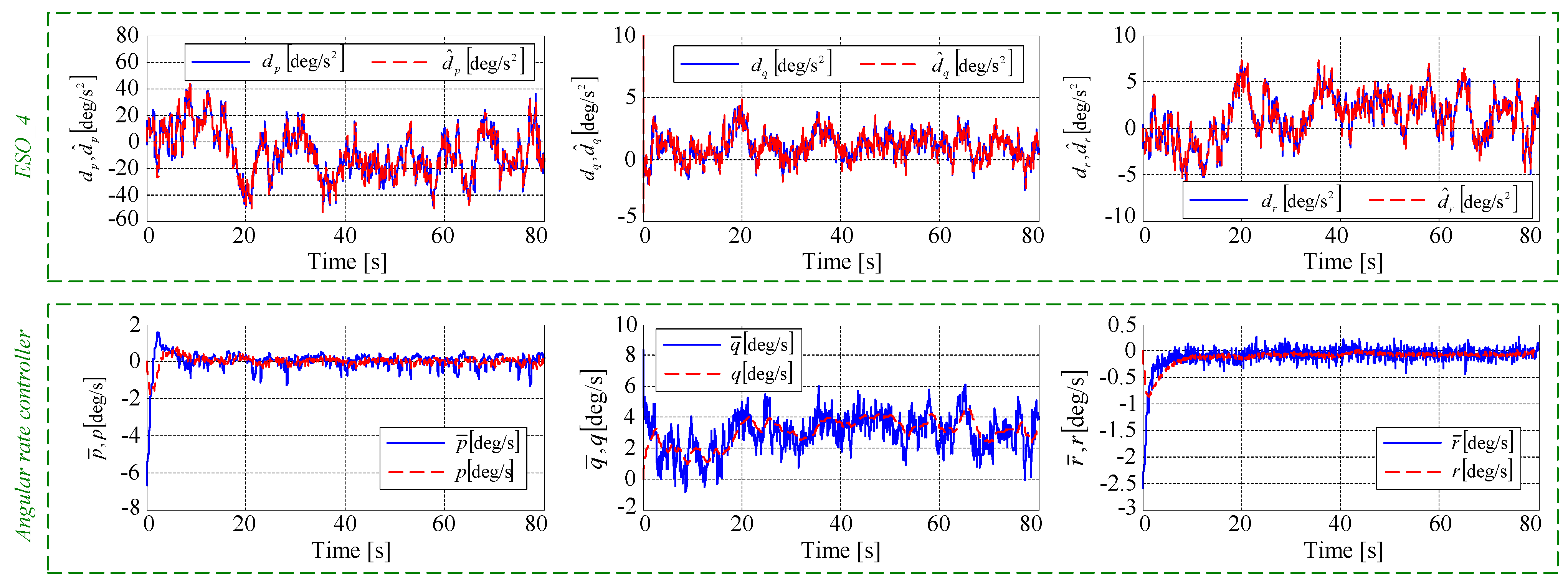

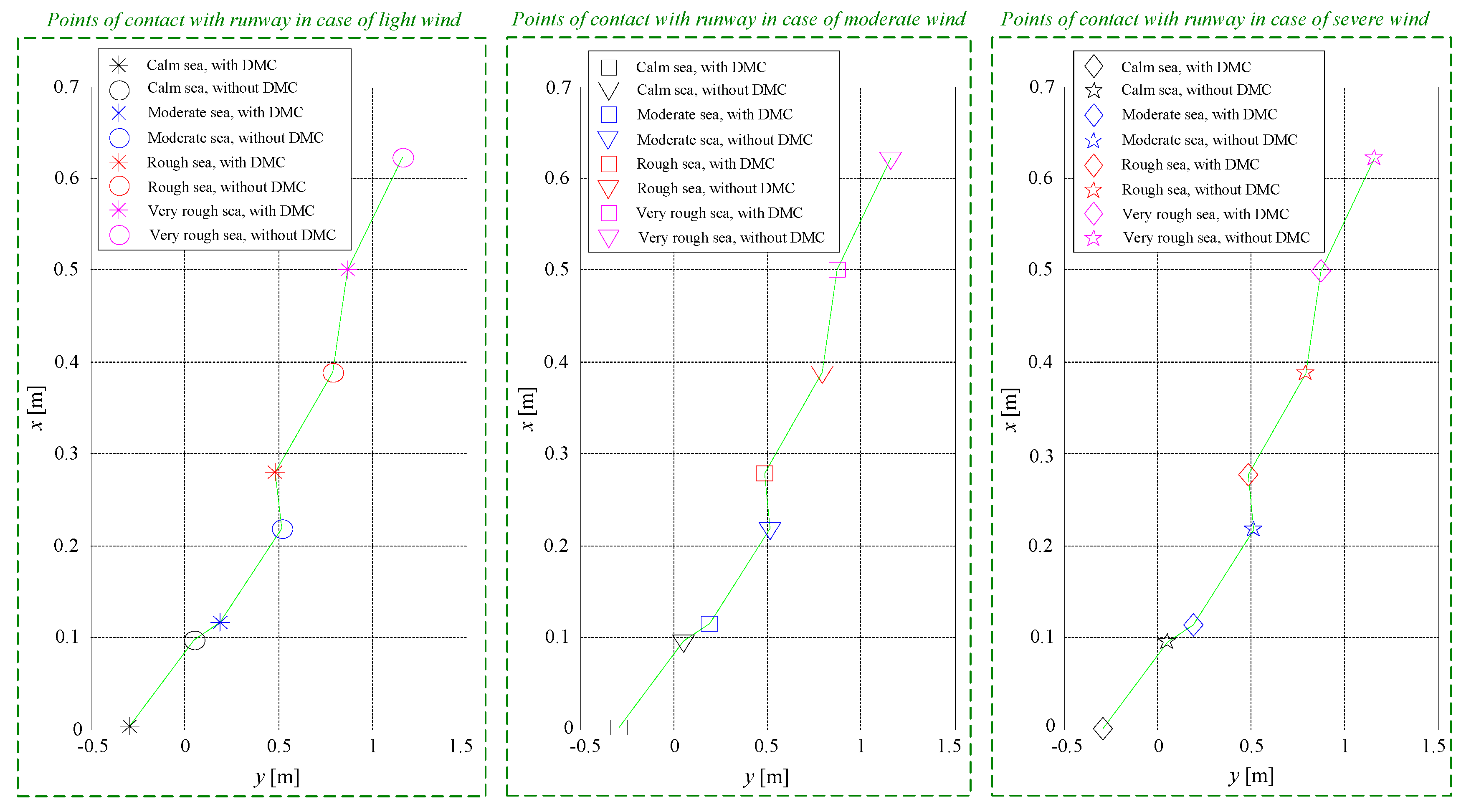
| Longitudinal Touchdown Error [m] | Without tracking differentiator-based DMC | With tracking differentiator-based DMC | ||||
| Light wind | Moderate wind | Severe wind | Light wind | Moderate wind | Severe wind | |
| Calm sea | 0.0975 m | 0.0964 m | 0.0951 m | 0.0028 m | 0.0018 m | 0.0007 m |
| Moderate sea | 0.2191 m | 0.2180 m | 0.2170 m | 0.1163 m | 0.1153 m | 0.1142 m |
| Rough sea | 0.3893 m | 0.3882 m | 0.3870 m | 0.2793 m | 0.2783 m | 0.2773 m |
| Very rough sea | 0.6233 m | 0.6223 m | 0.6211 m | 0.5011 m | 0.5002 m | 0.4990 m |
| Lateral Touchdown Error [m] | Without tracking differentiator-based DMC | With tracking differentiator-based DMC | ||||
| Light wind | Moderate wind | Severe wind | Light wind | Moderate wind | Severe wind | |
| Calm sea | 0.341 m | 0.341 m | 0.341 m | −0.2913 m | −0.2913 m | −0.2913 m |
| Moderate sea | 0.5151 m | 0.5151 m | 0.5151 m | 0.1930 m | 0.1930 m | 0.1930 m |
| Rough sea | 0.7915 m | 0.7915 m | 0.7915 m | 0.4833 m | 0.4832 m | 0.4832 m |
| Very rough sea | 1.1609 m | 1.1609 m | 1.1609 m | 0.8708 m | 0.8708 m | 0.8707 m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lungu, M.; Chen, M.; Vîlcică, D.-A. Backstepping- and Sliding Mode-Based Automatic Carrier Landing System with Deck Motion Estimation and Compensation. Aerospace 2022, 9, 644. https://doi.org/10.3390/aerospace9110644
Lungu M, Chen M, Vîlcică D-A. Backstepping- and Sliding Mode-Based Automatic Carrier Landing System with Deck Motion Estimation and Compensation. Aerospace. 2022; 9(11):644. https://doi.org/10.3390/aerospace9110644
Chicago/Turabian StyleLungu, Mihai, Mou Chen, and Dana-Aurelia Vîlcică (Dinu). 2022. "Backstepping- and Sliding Mode-Based Automatic Carrier Landing System with Deck Motion Estimation and Compensation" Aerospace 9, no. 11: 644. https://doi.org/10.3390/aerospace9110644
APA StyleLungu, M., Chen, M., & Vîlcică, D.-A. (2022). Backstepping- and Sliding Mode-Based Automatic Carrier Landing System with Deck Motion Estimation and Compensation. Aerospace, 9(11), 644. https://doi.org/10.3390/aerospace9110644








