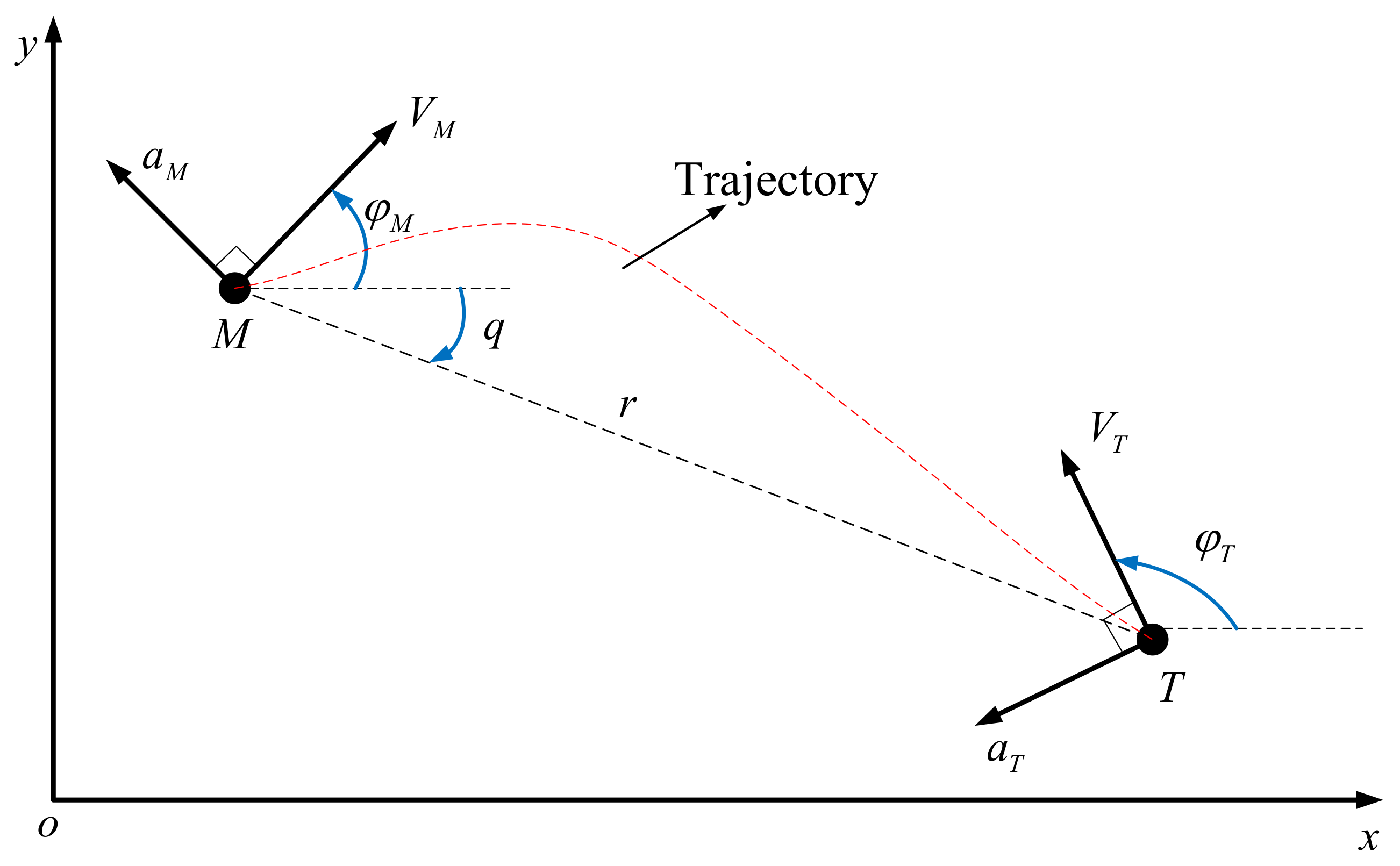
In this section, the proposed fractional-order control method is used as a guidance law for the interception of a missile with a target under an impact angle constraint. The planar missile–target engagement is shown in
Figure 1. In the inertial coordinate system, the kinematic equations of the missile and target are expressed as follows [
37,
38]:
where
r and
are the relative distance to the missile and the relative velocity of the target, respectively;
denotes the aspect angle of the target;
is the heading angle of the missile; and
and
are the velocities of the missile and target, respectively. We assume that
and
are constants.
and
denote the accelerations of the missile and target, respectively.
In this study, required data such as
q,
,
r,
, and
were available. Let
be the desired terminal LOS angle which is prespecified with a constant value. Let
be the LOS angle error, that is,
. Define
and
. The missile acceleration
can be regarded as a control variable, that is,
. Substituting these values into Equation (
67) yields
In the following subsection, the effectiveness of the proposed fractional-order sliding mode guidance law (
61) is verified through a series of simulations. First, simulations with different fractional orders in the three cases are presented to illustrate the influence of the fractional order on the guidance results. Simulations with
are given specifically for comparison with the fractional-order method. Subsequently, a comparison of the proposed guidance law and the fractional guidance law in Ref. [
15] exhibits the advantages of the proposed guidance law. Finally, simulations are performed with different desired terminal LOS angles to demonstrate the performance of the proposed method in satisfying the impact angle constraints.
3.1. Simulation Results with Different Fractional Orders
In this subsection, the simulations with the proposed guidance law (
61) under different fractional orders are described. The parameters of the proposed guidance law were as follows:
,
,
,
,
,
,
, and
. Additional constants and initial values are listed in
Table 1. The impact angle constraint was 20
. The maximum acceleration of the missile was set to 40
, where
was the acceleration owing to gravity.
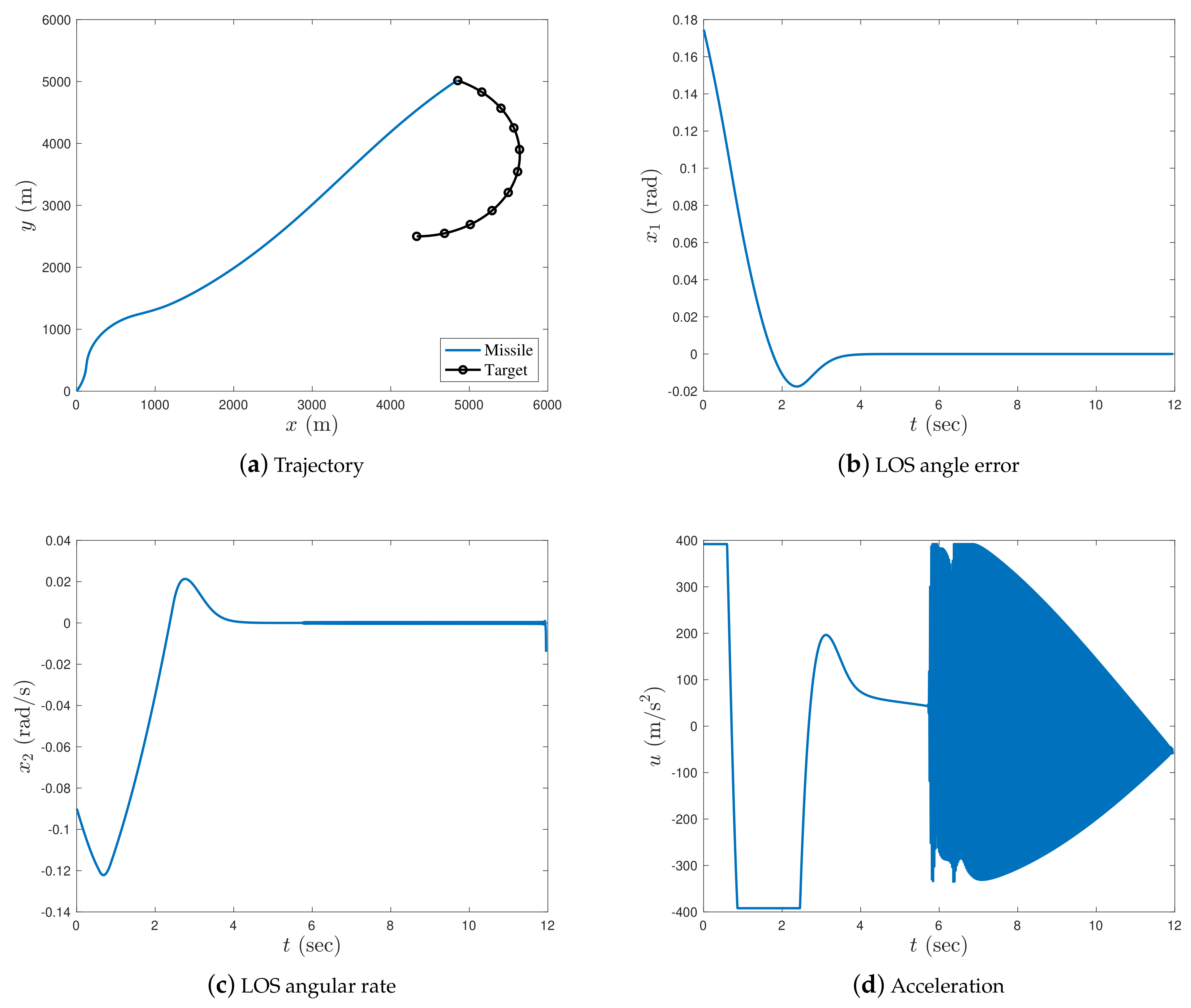
Figure 2 and
Figure 3 show the simulation results with the fractional differential (
) and fractional integral (
) guidance laws, respectively, in case 1.
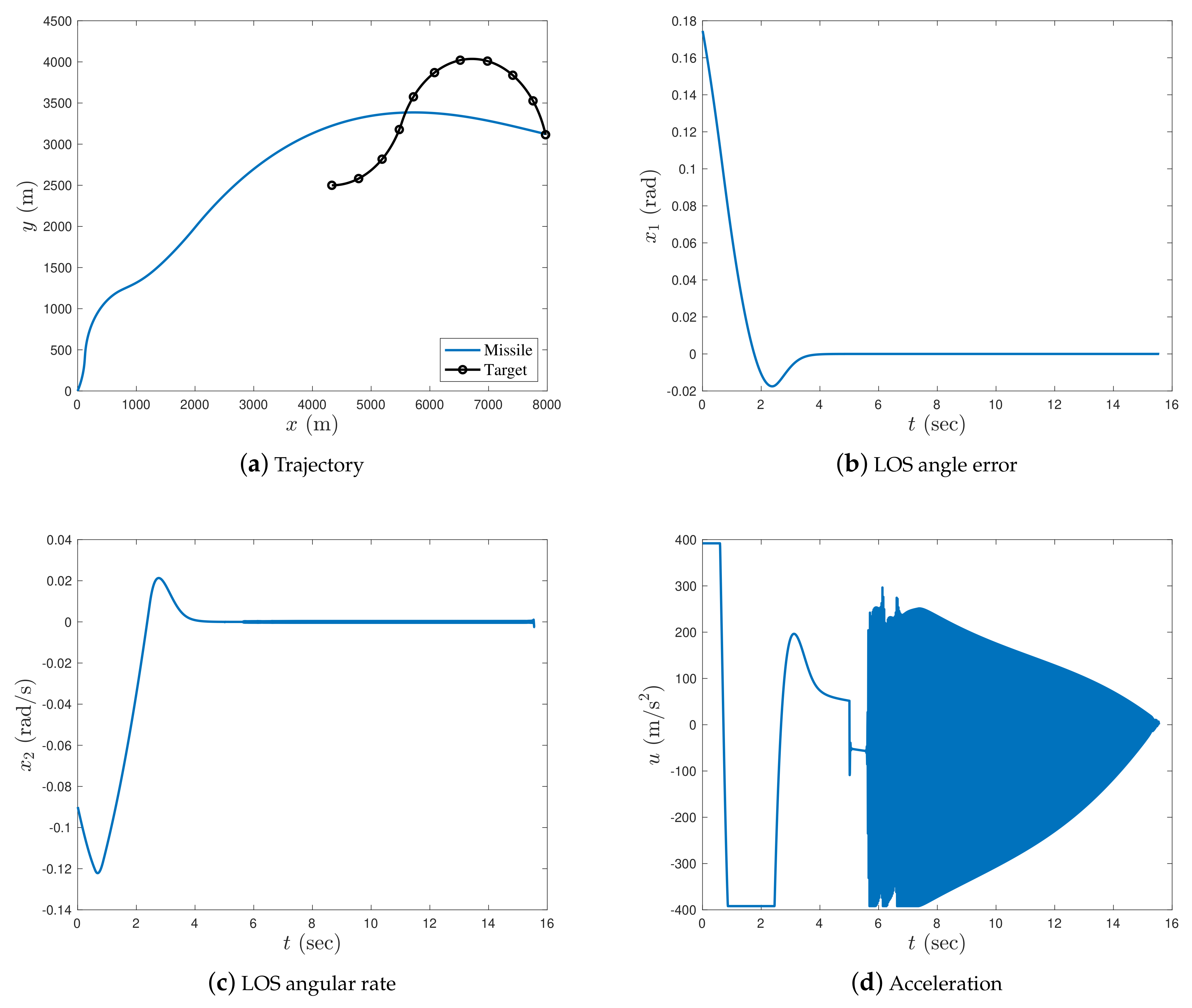
Figure 4 shows the simulation results with the integer (
) guidance law for case 1. Interception times, miss distances and LOS angle errors of the proposed guidance law under all chosen fractional orders in case 1 are shown in
Table 2.
Figure 5,
Figure 6 and
Figure 7 show the simulation results with fractional differential, fractional integral, and integer-order guidance laws, respectively, in case 2. Interception times, miss distances and LOS angle errors of the proposed guidance law under all selected fractional orders in case 2 are shown in
Table 3,
Figure 8 and
Figure 9 show the simulation results with
and
in case 3, and
Figure 10 depicts the simulation results with the integer-order guidance law in case 3. Interception times, miss distances, and the LOS angle errors of the proposed guidance law under all selected fractional orders in case 3 are shown in
Table 4.
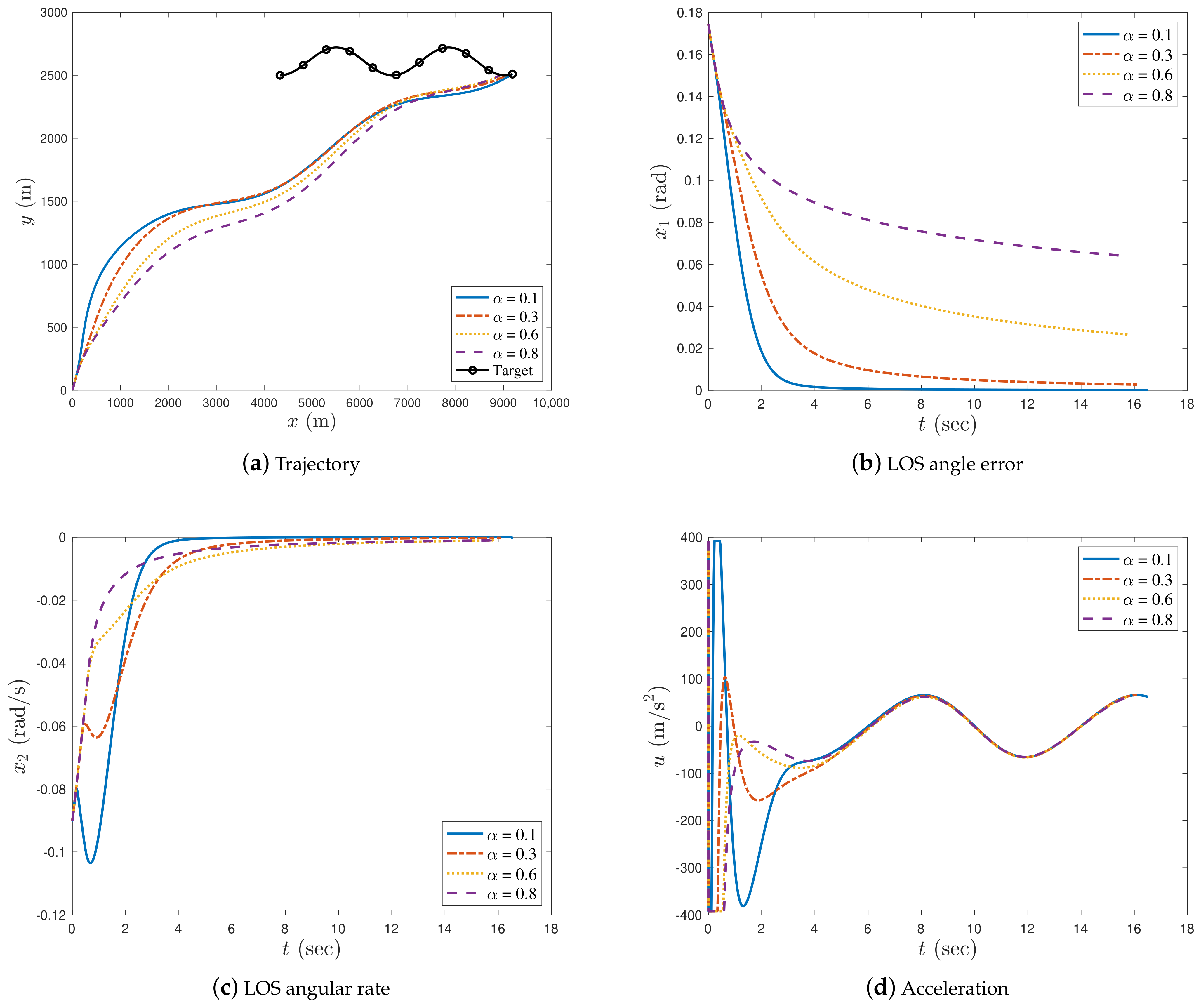
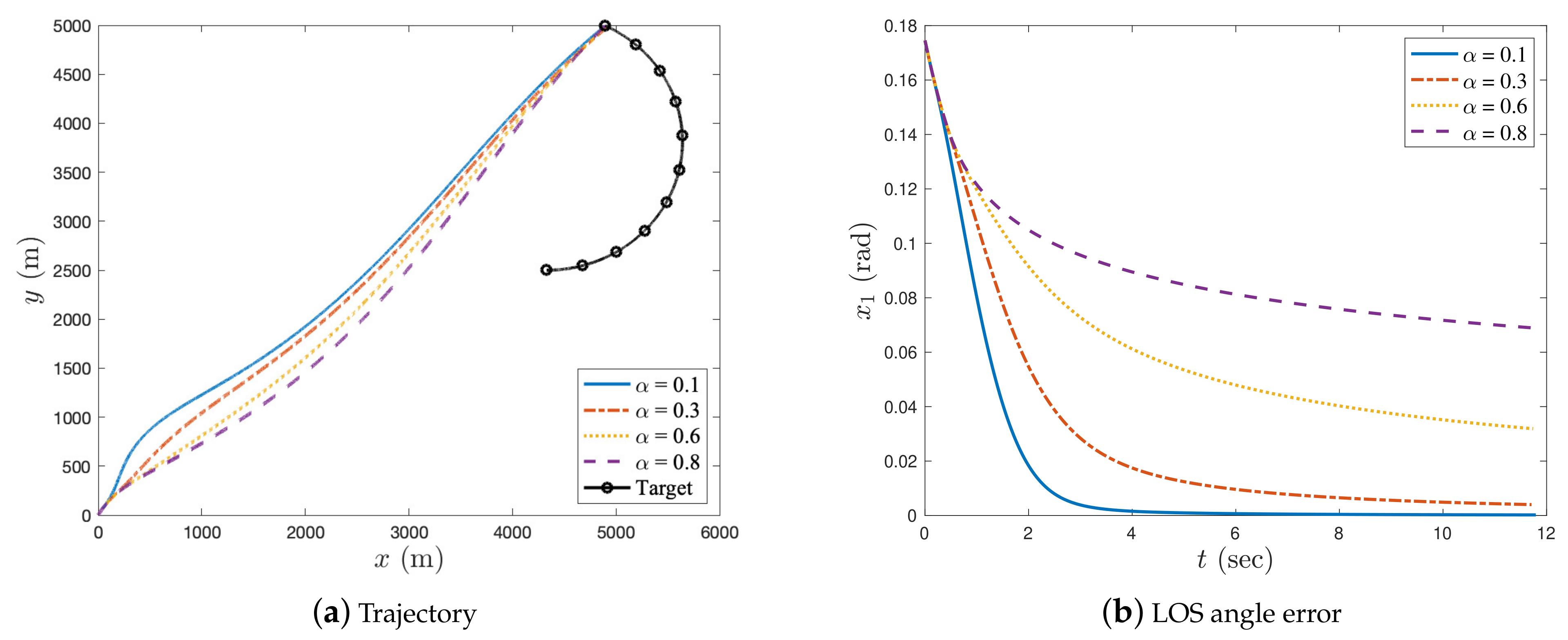
In case 1, as illustrated in
Figure 2a,
Figure 3a, and
Figure 4a, the missile successfully intercepted the manoeuvring target under all given fractional orders. As can be seen in
Figure 2b, when
, the LOS angle error increased with an increase in
α; therefore, the LOS angle error was asymptotically stable and the impact angle constraint of the missile could not be satisfied when
α was relatively large. Thus, we set
α sufficiently small to ensure the convergence of the LOS angle error during the interception process. When
, the LOS angle error asymptotically converged to zero, and the smaller the value of
α, the larger the oscillation of the LOS angle error (see
Figure 3b). In
Figure 4b, the LOS angle error converges to zero in finite time when
. From
Figure 2c,
Figure 3c, and
Figure 4c, it can be observed that the LOS angular rate of the missile converges to zero for all values of
α. In
Figure 2c and
Figure 3c, the LOS angular rate is asymptotically stable, whereas that in
Figure 4c is finite-time stable. In addition, it was observed that the closer the order of
α was to zero (either to the right or left), the faster the convergence speed of the missile’s LOS angular rate.
It can be noted from
Table 2 that the interception times taken by the proposed guidance law with
were similar, while the interception time was longer with the decrease in fractional order when
. It can also be observed that the miss distance and the LOS angle error were smaller when the fractional order of the proposed guidance law approached zero.
In
Figure 2d and
Figure 3d, the acceleration of the missile is smooth and steady for both the fractional differential and fractional integral guidance laws. However, as illustrated in
Figure 4d, the acceleration trajectory of the missile with the integer-order guidance law chattered after approximately 7 s. This is because the proposed guidance law contains a singular term when
.
Figure 2d and
Figure 3d show that the fractional-order differential and integral guidance laws were effective in eliminating the chattering caused by singular terms.
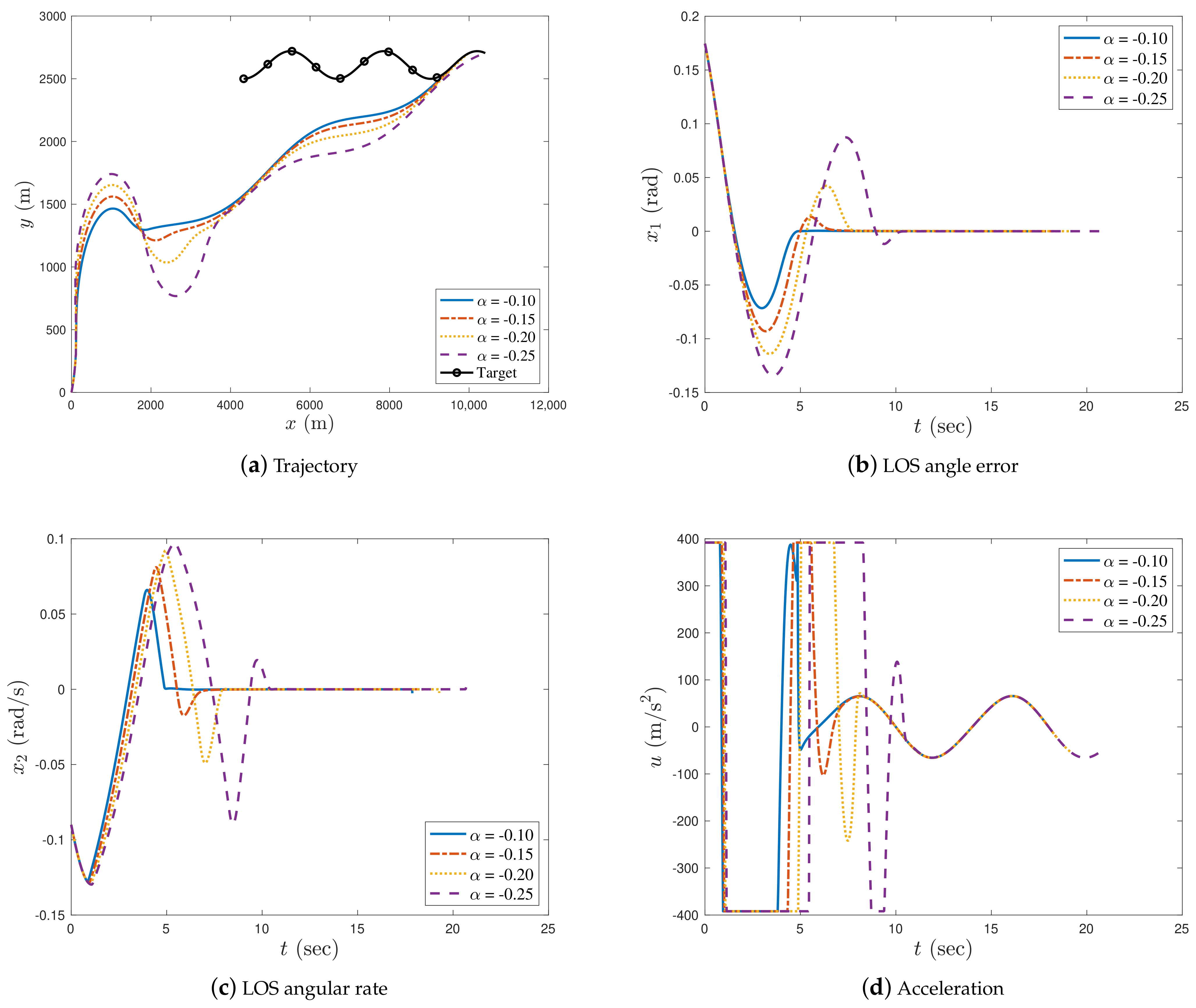
In case 2,
Figure 5,
Figure 6 and
Figure 7 show the simulation results under
,
, and
, respectively. As shown in
Figure 5a,
Figure 6a, and
Figure 7a, the missile achieved an accurate interception of the manoeuvring target for all different fractional orders. The impact time decreased when the fractional order approached zero (either from the left or right). From
Figure 5b,
Figure 6b, and
Figure 7b, it can be seen that the missile’s LOS angle error converged in the neighbourhood of zero when the fractional order was close to zero: the convergence time was shorter with smaller fractional orders. When
α was far from zero, the LOS angle error did not converge to zero during the interception process; therefore, the impact angle constraint was not satisfied. In
Figure 5c,
Figure 6c, and
Figure 7c, it can be seen that the missile’s LOS angular rate converged to zero during the interception process.
It can be noted from
Table 3 that the interception times taken by the proposed guidance law with
were similar, while the interception time was longer with the decrease in fractional order when
. It can also be observed that the miss distance and the LOS angle error were smaller when the fractional order of the proposed guidance law approached zero.
In
Figure 5d and
Figure 6d, as in case 1, the acceleration of the missile is smooth and steady for both
and
; however, the acceleration trajectory of the missile for
also chattered after approximately 7 s (see
Figure 7d) due to the proposed guidance law containing a singular term when
.
Figure 5d and
Figure 6d show that the fractional-order differential and integral guidance laws were effective in eliminating the chattering caused by the singular terms. In
Figure 6d, the acceleration of the missile with
had a small chatter at 12 s. Compared to the missile acceleration when
in
Figure 7d, the chattering is so small that it can be ignored. Thus, it can be concluded that the proposed fractional-order method is effective for eliminating chattering.
The simulation results for case 3 are shown in
Figure 8,
Figure 9 and
Figure 10 and
Table 4, from which it can be seen that the results were similar to those in cases 1 and 2; therefore, discussions on this case are omitted.
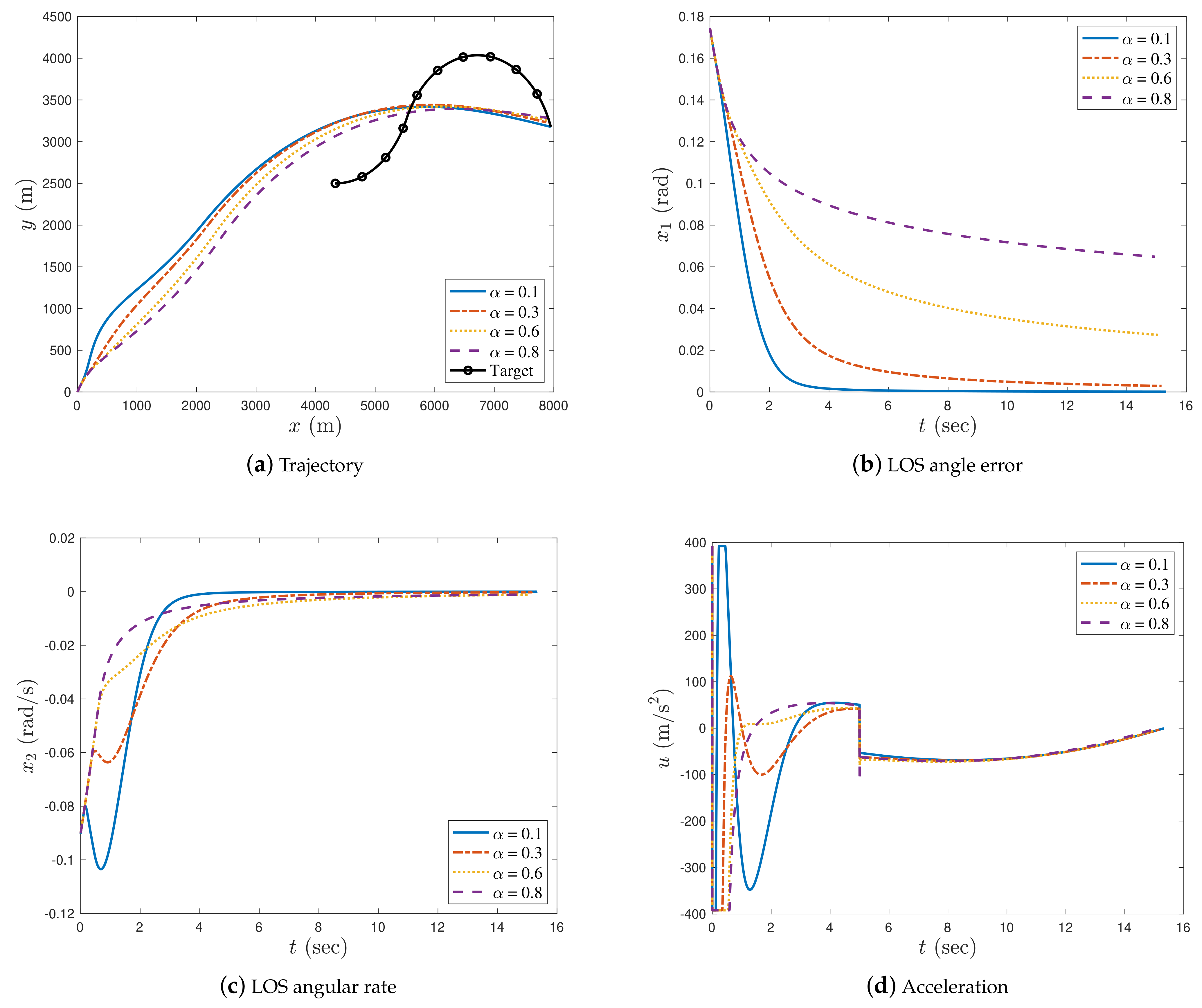
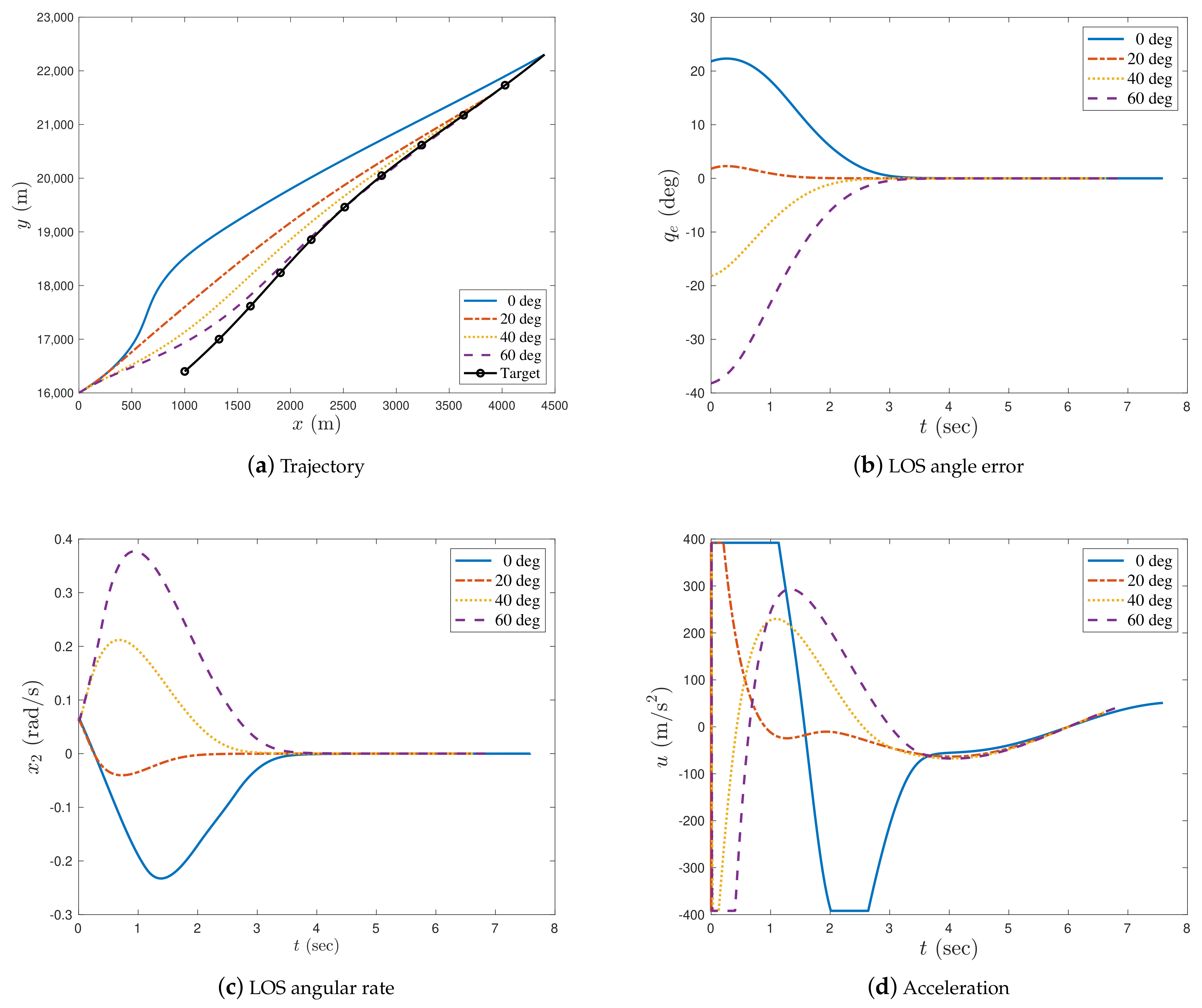
3.2. Simulations with Different Impact Angle Constraints
In this subsection, simulations with different desired terminal LOS angles but the same initial flight path angles as those of the previous subsection are described. These simulations were carried out to verify the proposed fractional-order guidance law with different impact angle constraints. The initial conditions of the missile and target are listed in
Table 5. The desired terminal LOS angles were 0
, 20
, 40
, and 60
. The target acceleration was chosen as
. The fractional order was set to
. Using the proposed guidance law, the trajectories of the missile and target, the missile LOS angle error, LOS angular rate, and acceleration were as shown in
Figure 11.
From
Figure 11a, we can observe that the missile successfully intercepted the manoeuvring target at different impact angles.
Figure 11b,c show that when
, or
, the LOS angle errors and LOS angular rates were asymptotically stable. From
Figure 11d we can observe that the accelerations of the missile were within reasonable bounds, and there was no chattering during the interception process with different terminal LOS angles. Therefore, the fractional guidance law is very effective in eliminating chattering. The only disadvantage is that when using fractional derivative or integral guidance laws, the LOS angle error and LOS angular rate converge to zero asymptotically rather than in finite time. However, the value of
α near zero can be adjusted continuously such that the LOS angle error and LOS angular rate can achieve satisfactory convergence.
3.3. Simulation for Comparison with Different Method
In this subsection, simulations for comparison between the guidance law proposed in this study and the guidance law in Ref. [
15] are presented.
The fractional-order sliding surface proposed by Sheng et al. [
15] is expressed as
where
and
α is non-zero. The corresponding fractional sliding mode-based guidance law is
where
P denotes a positive constant.
It is simple to prove that the sliding surface
converges to zero in finite time. Thus, there exists
such that for all
, the sliding surface satisfies
. Therefore, Equation (
70) can be represented as:
Without loss of generalisation, we assume
to obtain
Integrating both sides of Equation (
73) from
to
t yields:
where
C is a constant. Taking the fractional derivative of order
in the Caputo sense on both sides of Equation (
74) yields
We cannot explicitly determine the convergence of
. In Ref. [
15], the authors set
to be extremely small (
) so that the fractional-order term in Equation (
70) can be regarded as a small perturbation. Thus, only the stability of the system
must be examined. It is easy to see that the zero solution of system
is exponentially stable, and systems with small perturbations
are also stable.
In the simulations, the parameters of the proposed guidance law were
,
,
,
,
,
,
, and
, and the parameters of the guidance law in Ref. [
15] were
,
, and
. The terminal LOS angle was
. The target acceleration was chosen as
. The initial conditions and additional constants are listed in
Table 5. To investigate the efficiency of the proposed method, simulations were performed with
and
separately, and the results are shown in
Figure 12 and
Figure 13. The interception times, the miss distance, and the LOS angle errors are shown in
Table 6 and
Table 7.
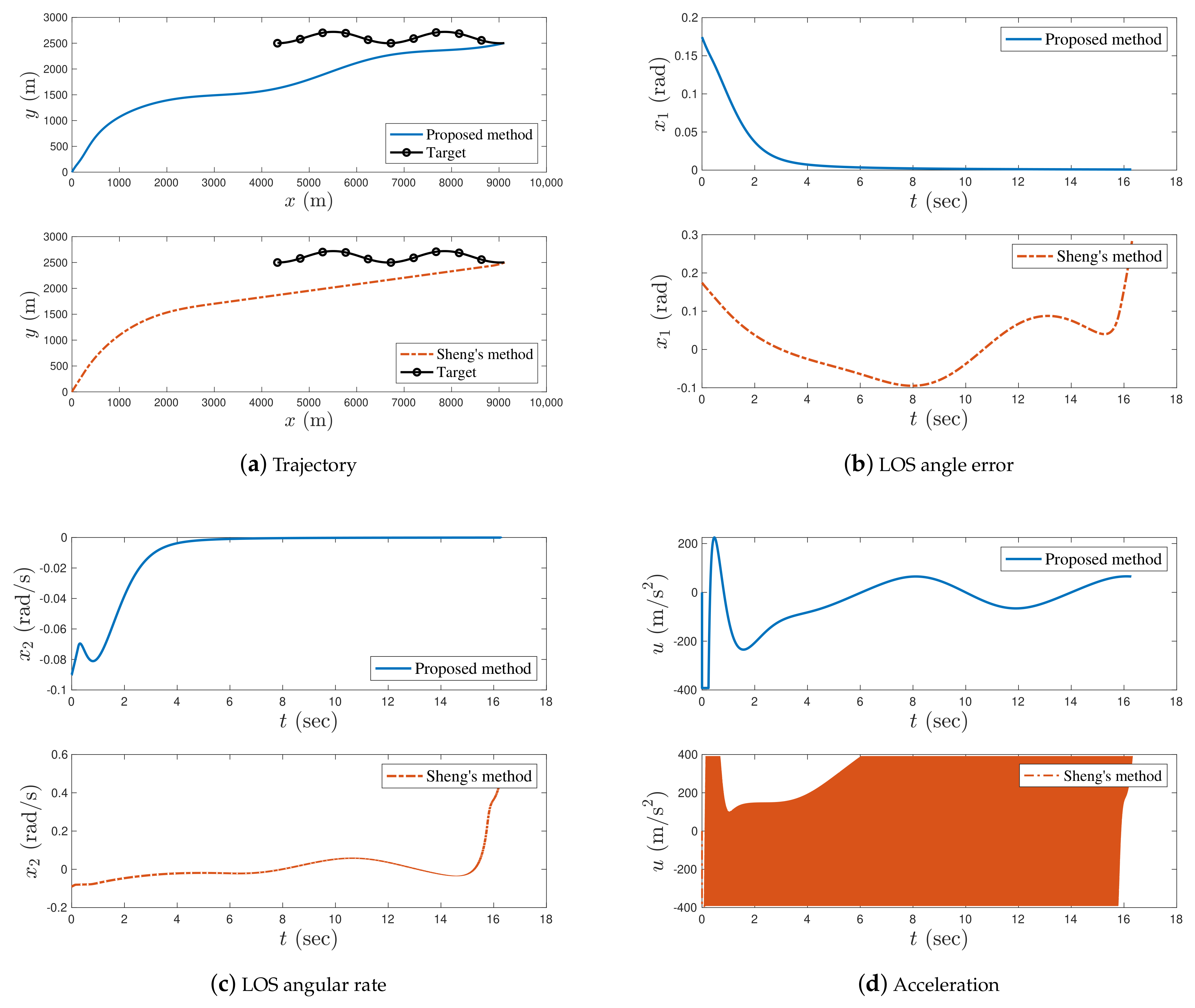
When
, the proposed fractional-order guidance law and the fractional-order guidance law from Ref. [
15] successfully intercepted a target (see
Figure 12a). The convergence speed of the LOS angle error and LOS angular rate with the proposed guidance law were faster than those with the guidance law from Ref. [
15]. As shown in
Figure 12d, the accelerations of the missiles with the proposed guidance law and guidance law in Ref. [
15] were all within a reasonable scope, but there was acceleration saturation when using the proposed method to achieve faster convergence.
From
Table 6, the missile distance and the LOS angle error of the proposed guidance law were smaller than those of Sheng’s method. Thus, the guidance precision of the proposed guidance law was significantly superior to that of Sheng’s method.
When
,
Figure 13a,c show that the LOS angle error and LOS angular rate with the proposed guidance law were asymptotically stable during the interception process, whereas the LOS angle error and LOS angular rate with the guidance law from Ref. [
15] were unstable. It can be observed in
Figure 13d that the acceleration of the missile with the proposed guidance law was within a reasonable scope without any chattering, whereas the acceleration of the missile with the guidance law in Ref. [
15] demonstrated high-frequency chattering in the acceleration bound. The simulation results indicated that the proposed guidance law had better robustness and a larger stability region than the guidance law in Ref. [
15].
From
Table 7, the missile distance of the proposed guidance law was smaller than that of Sheng’s method. It can be observed that the LOS angle error of Sheng’s method cannot ensure the terminal LOS angle constraint, while the LOS angle error of the proposed method was within a reasonable range. Thus, the guidance precision of the proposed guidance law is significantly superior to that of Sheng’s method.
The next set of simulations for the guidance laws based on the integer-order linear sliding mode (LSM) [
39], terminal sliding mode (TSM) [
39], and nonsingular fast terminal sliding mode (NFTSM) [
39] are shown for the comparison with the presented guidance law in this paper. All parameters of the LSM, TSM, and NFTSM guidance laws are the same as those chosen in the presented guidance law. The initial conditions of the missile and the target, the terminal LOS angle, and the target acceleration are the same as those of the previous simulation in this subsection. The fractional order of the proposed guidance law is set to 0.03. The trajectories of the missile and the target, the LOS angle, the LOS angular rate, and the missile acceleration for the four guidance laws are shown in
Figure 14.
As shown in
Figure 14a, it can be seen that the proposed fractional-order guidance law and the other three guidance laws based on integer-order sliding mode successfully intercepted a target. The convergence speed of the LOS angle error and LOS angular rate with the proposed guidance law were faster than those with the guidance laws based on integer-order sliding mode. As shown in
Figure 14d, the accelerations of the missiles with the proposed guidance law and guidance laws based on integer sliding mode were all within a reasonable scope, but there was acceleration saturation when using the proposed method to achieve faster convergence.
From
Table 8, it is clear that the interception time taken by the proposed guidance law and the NFTSM guidance law is similar. However, the LSM guidance law has a longer interception time than the other guidance laws. The miss distance of the proposed guidance law is smaller than the other guidance laws. However, the LOS angle error generated by the proposed guidance law is bigger than that of the other integer-order guidance laws. This is because the proposed guidance law can only ensure asymptotic convergence rather than the finite-time convergence.