Tuning of a Linear-Quadratic Stabilization System for an Anti-Aircraft Missile
Abstract
1. Introduction
2. Methods
2.1. Problem Formulation
2.2. Missile Airframe Dynamics
2.3. Stabilization System Design
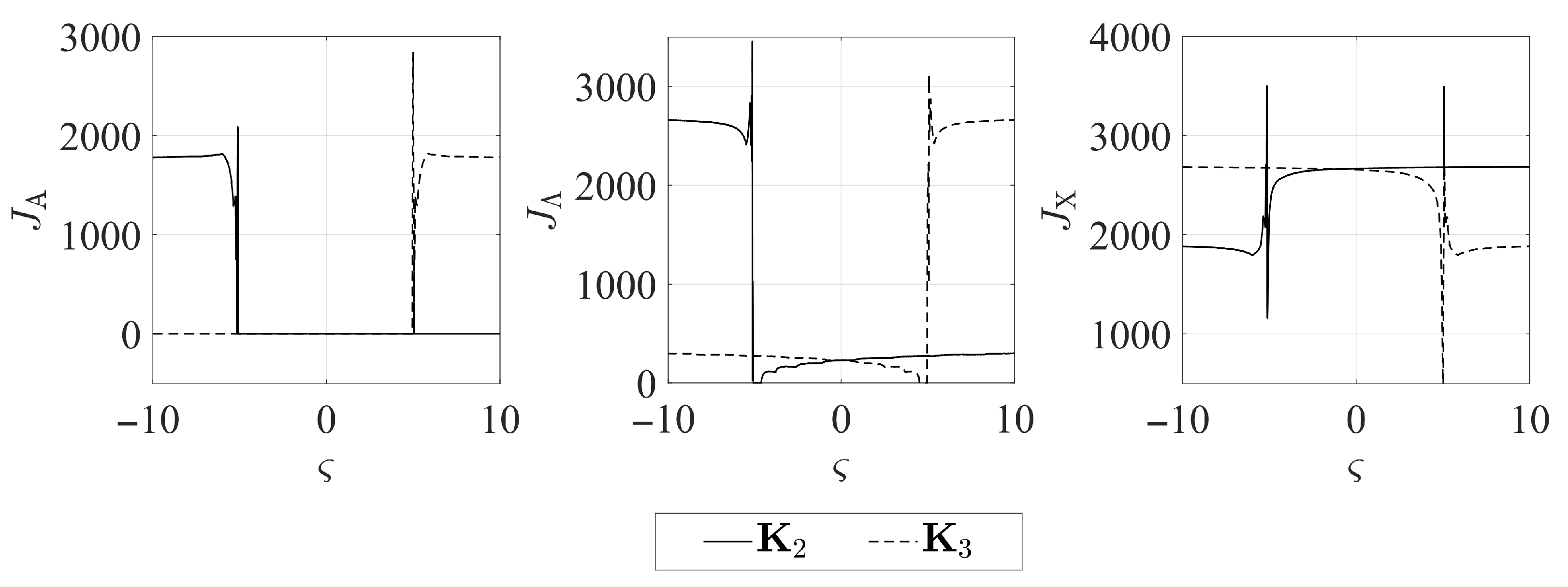
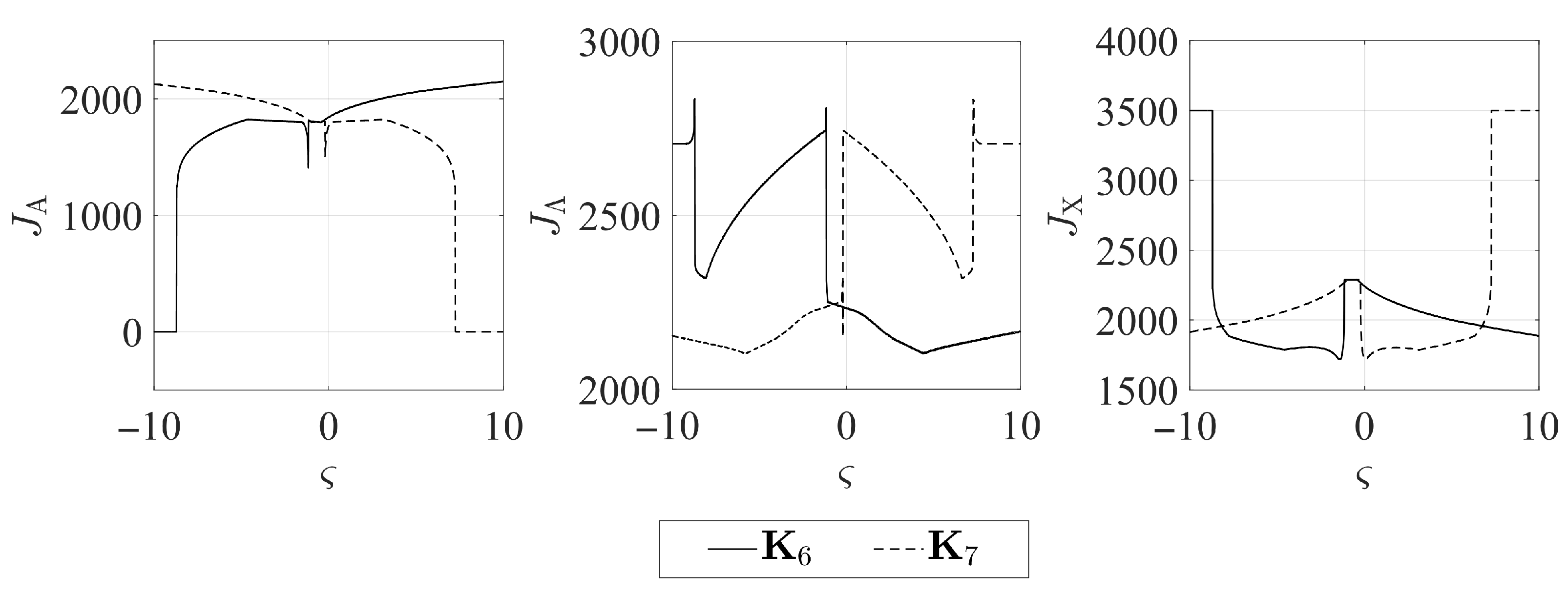
2.4. LQR Tuning Procedure
2.5. Numerical Simulation Issues
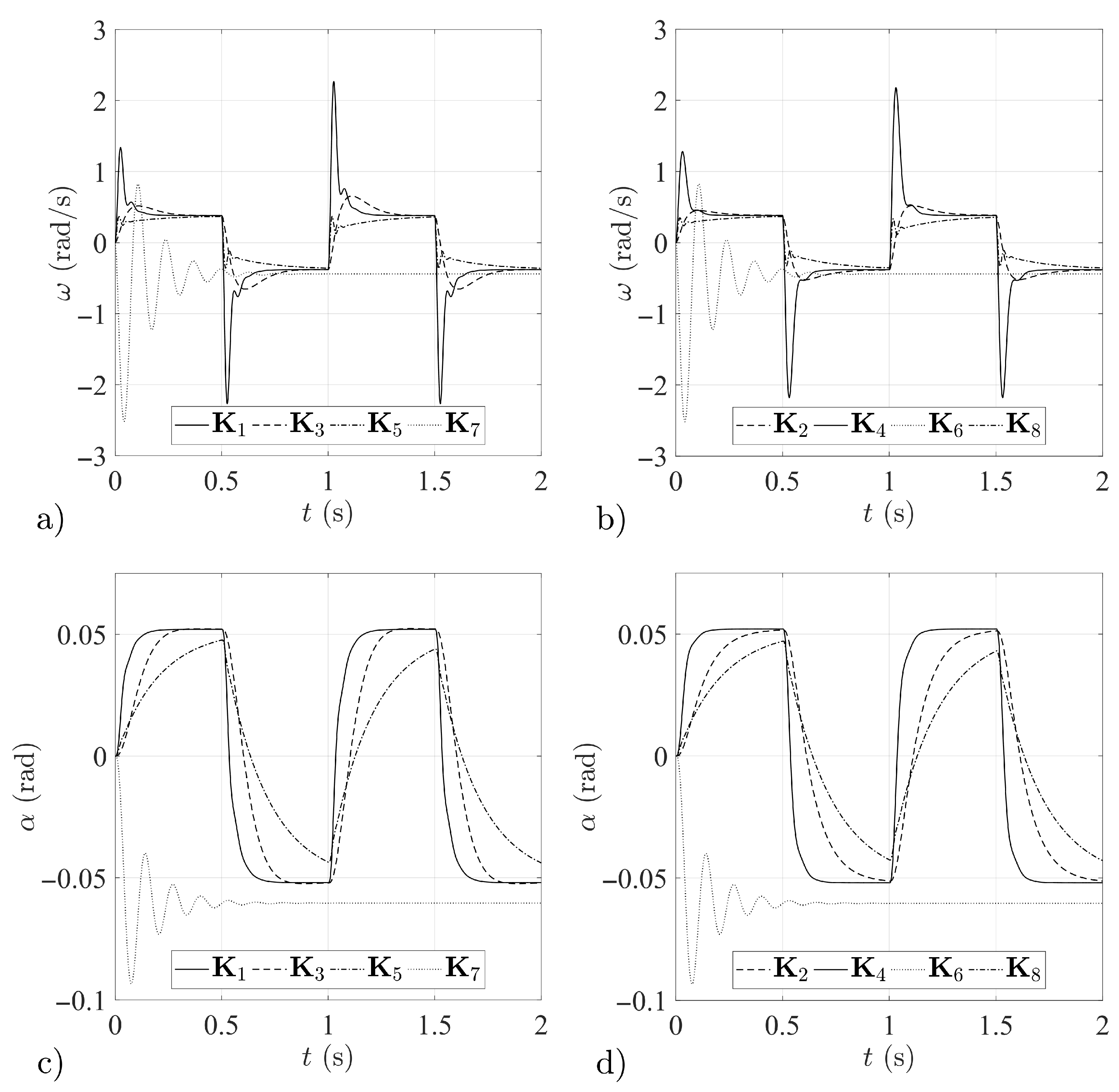
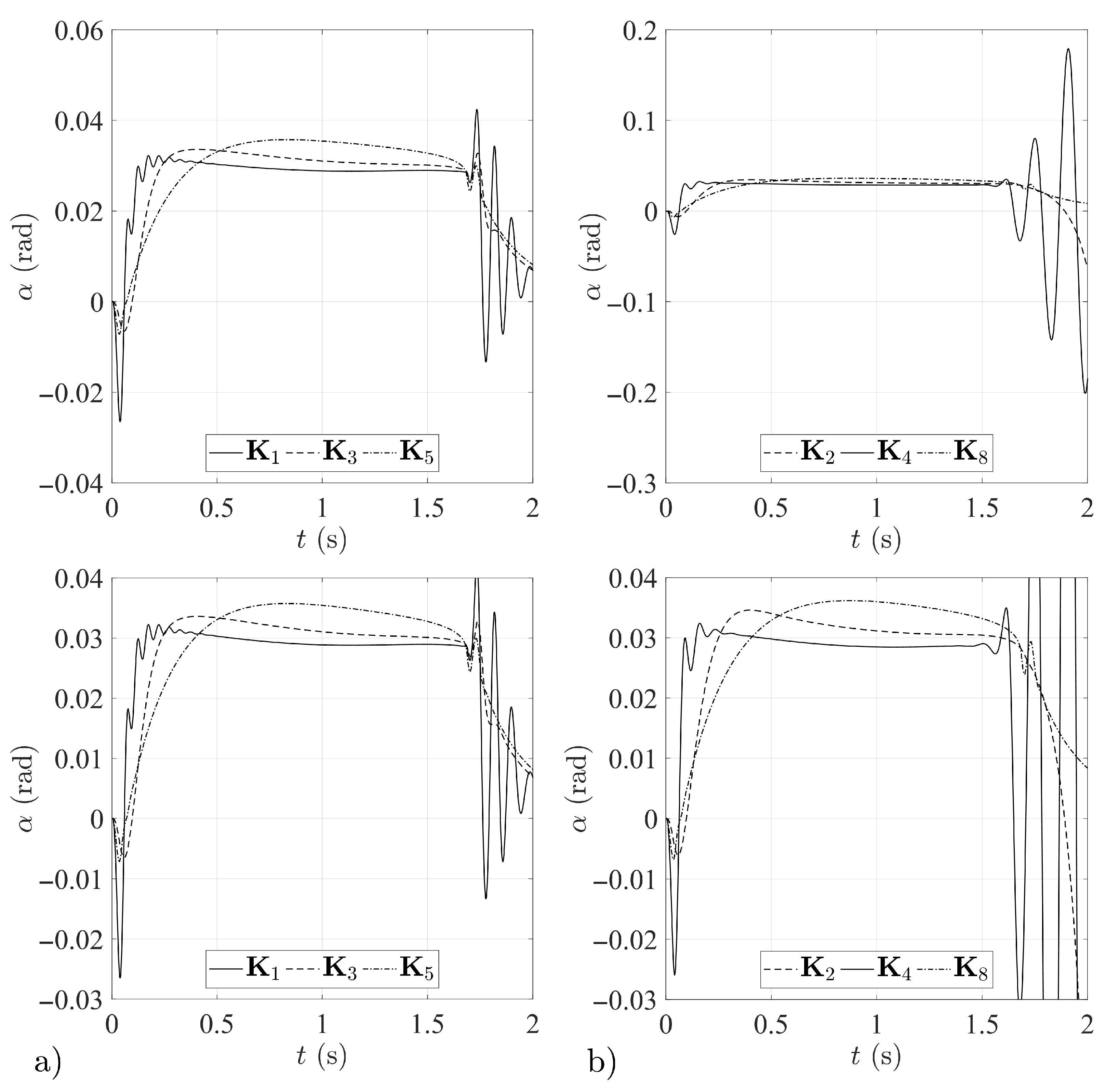
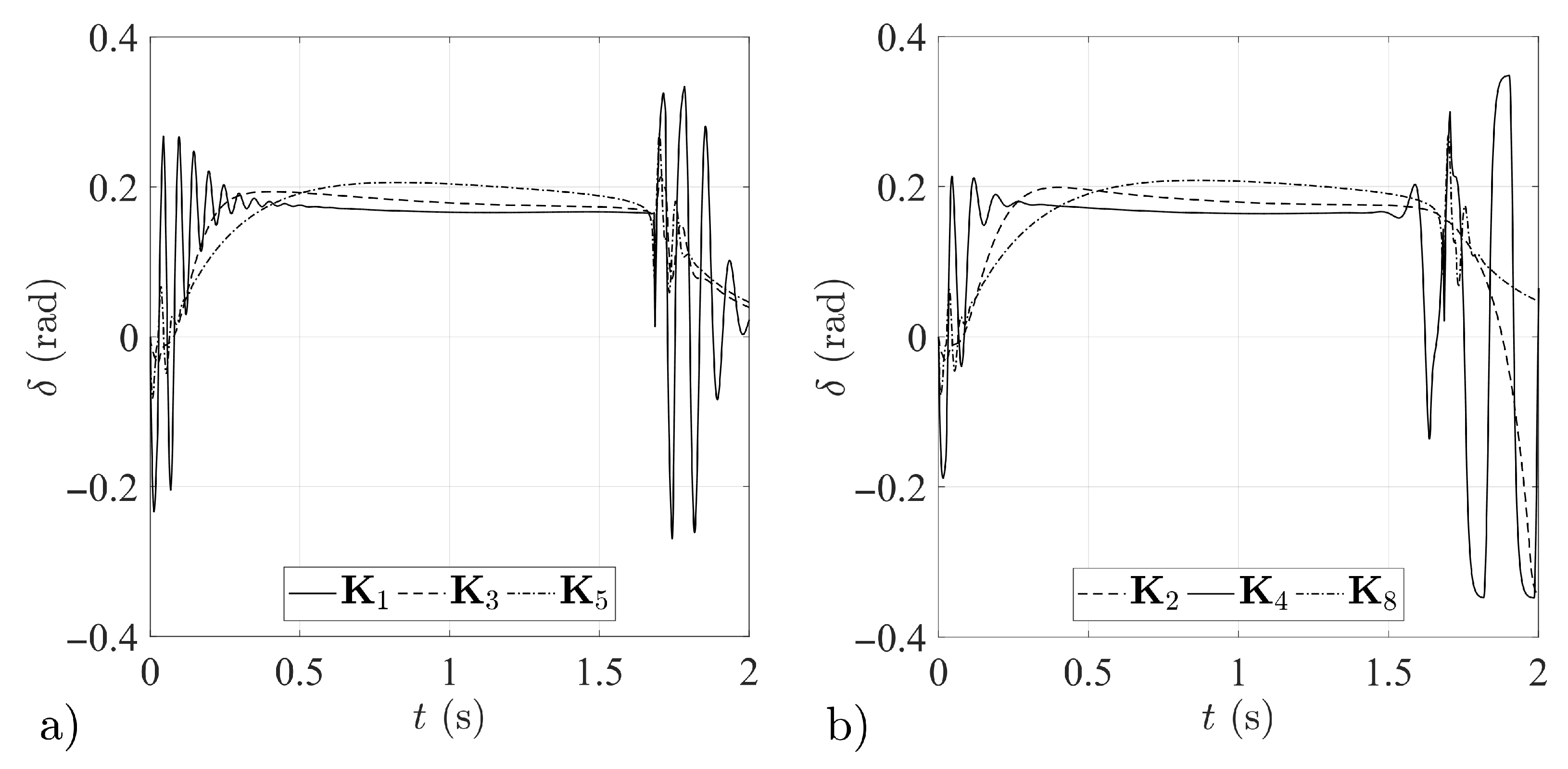
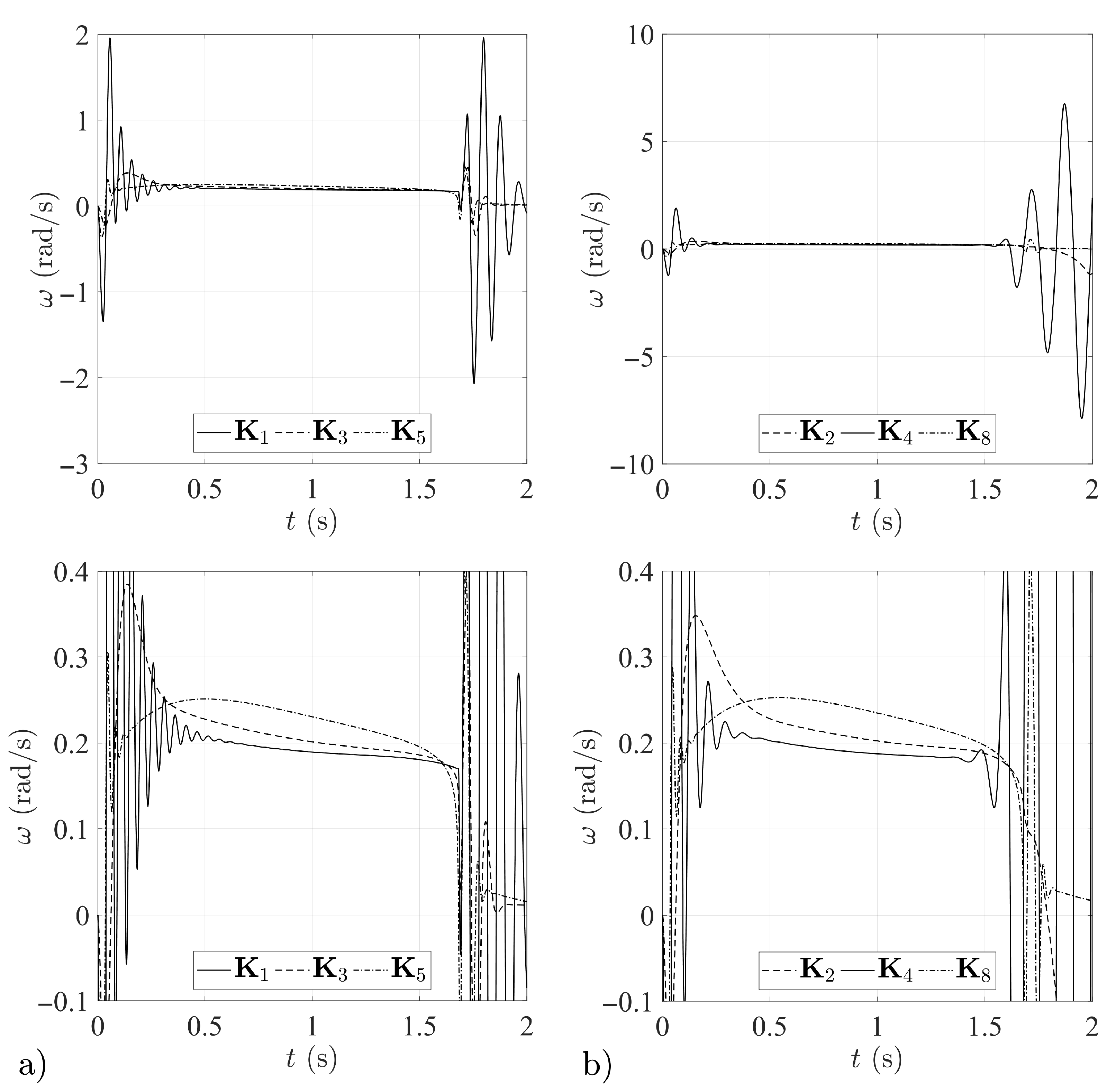
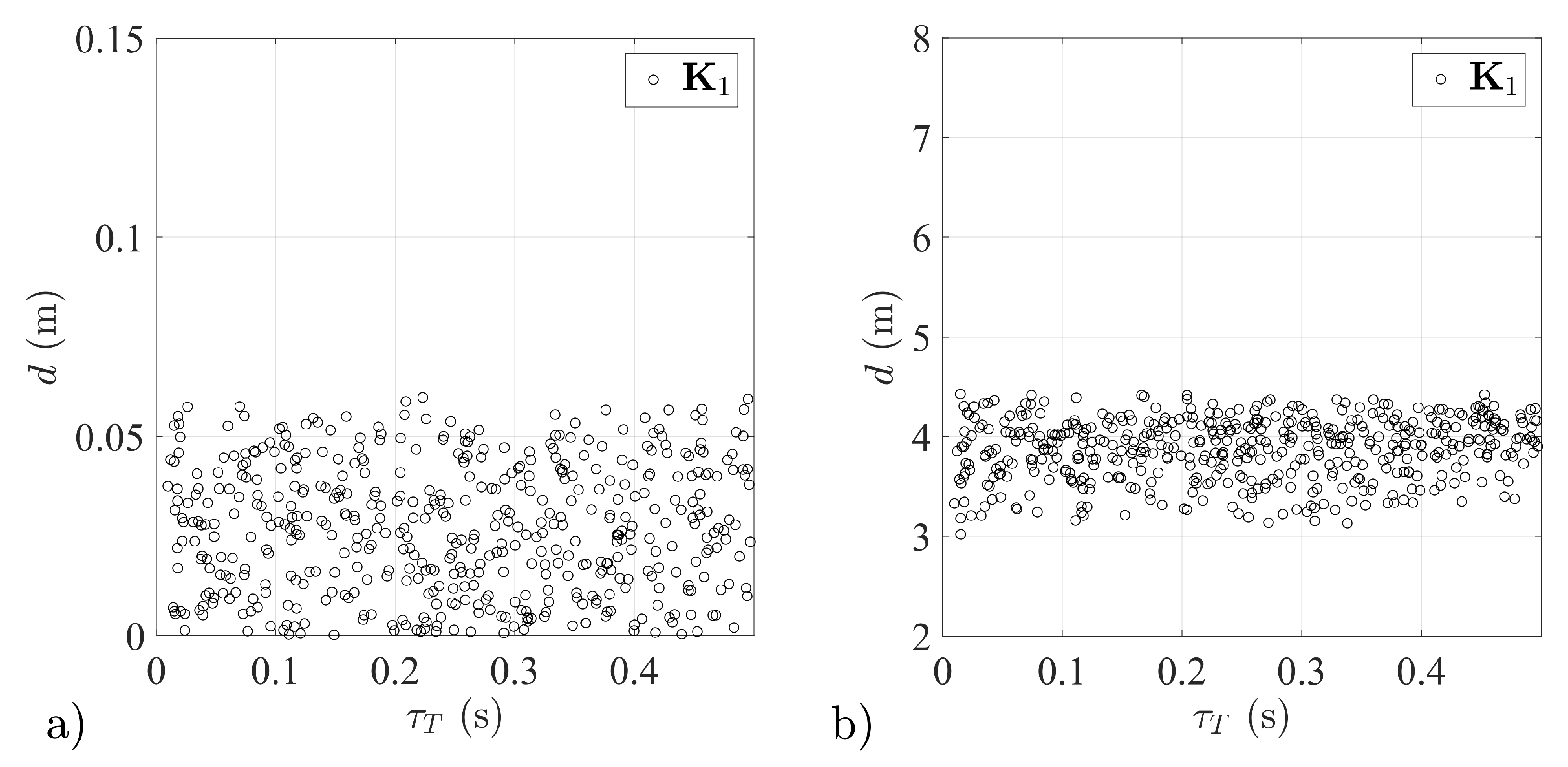
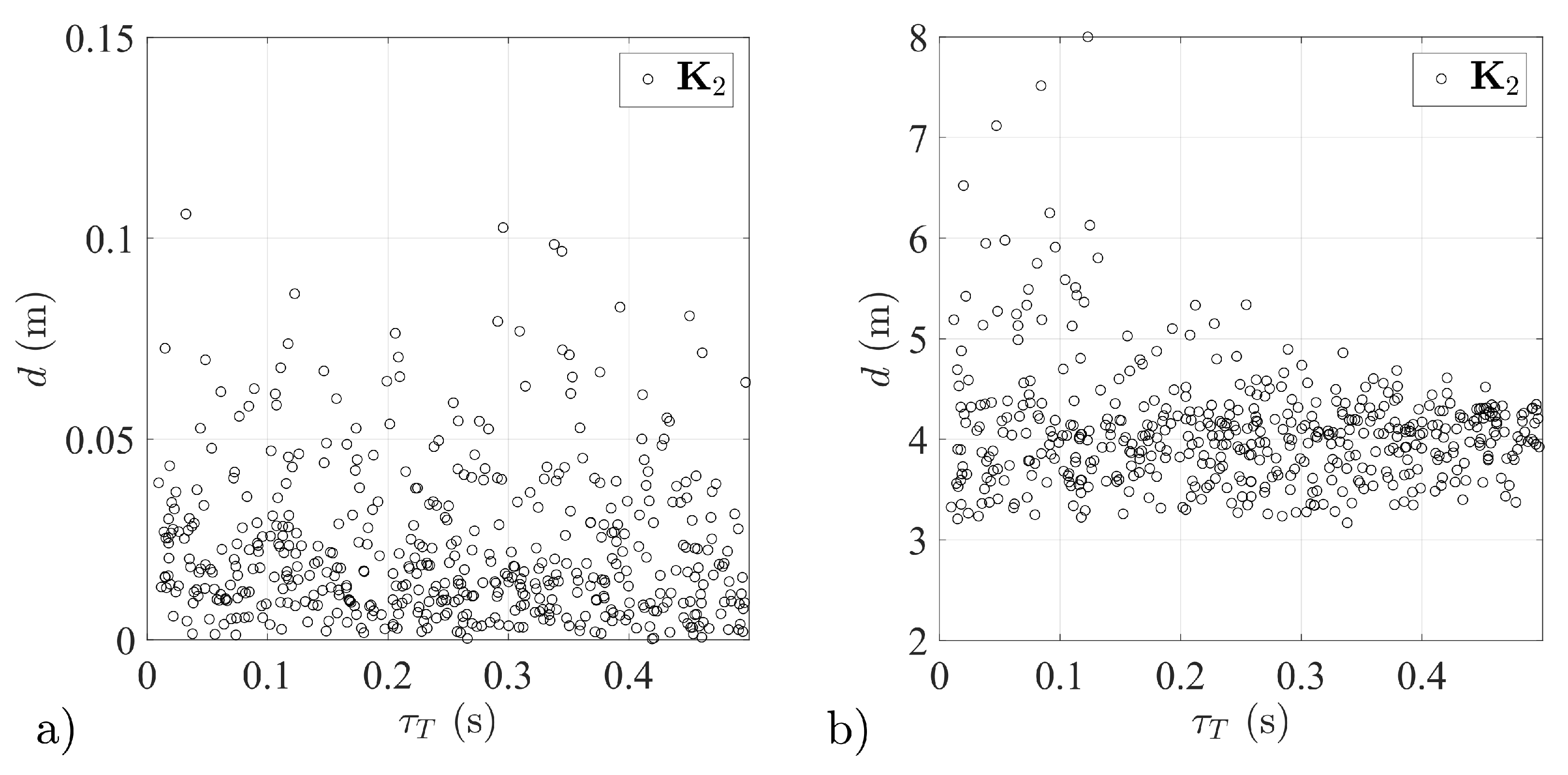
3. Results and Discussion
3.1. Sample Run Test
3.2. Monte Carlo Simulation Study
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Holloway, J.C.; Krstic, M. A Predictor Observer for Seeker Delay in the Missile Homing Loop. IFAC Pap. 2015, 48, 416–421. [Google Scholar] [CrossRef]
- Kaiwei, C.; Qunli, X.; Xiao, D.; Yuemin, Y. Influence of Seeker Disturbance Rejection and Radome Error on the Lyapunov Stability of Guidance Systems. Math. Probl. Eng. 2018, 2018, 1890426. [Google Scholar] [CrossRef]
- Lin, S.; Lin, D.; Wang, W. A Novel Online Estimation and Compensation Method for Strapdown Phased Array Seeker Disturbance Rejection Effect Using Extended State Kalman Filter. IEEE Access 2019, 7, 172330–172340. [Google Scholar] [CrossRef]
- Yunli, D.; Qunli, X.; Zhuomin, W. Effect of seeker disturbance rejection performance on the control system stability. In Proceedings of the 3rd International Symposium on Systems and Control in Aeronautics and Astronautics, Harbin, China, 8–10 June 2010. [Google Scholar] [CrossRef]
- Shixiang, L.; Tianyu, L.; Teng, S.; Qunli, X. Dynamic Modeling and Coupling Characteristic Analysis of Two-Axis Rate Gyro Seeker. Int. J. Aerosp. Eng. 2018, 2018, 8513684. [Google Scholar] [CrossRef]
- Grycewicz, H.; Mosiewicz, R.; Pietrasieński, J. Radio Control Systems, 1st ed.; Military University of Technology: Warsaw, Poland, 1984. (In Polish) [Google Scholar]
- Bużantowicz, W. A sliding mode controller design for a missile autopilot system. J. Theor. Appl. Mech. 2020, 58, 169–182. [Google Scholar] [CrossRef]
- Bużantowicz, W. A linear-quadratic stabilization system for a canard-controlled missile. In Proceedings of the 25th International Conference “Engineering Mechanics 2019”, Svratka, Czech Republic, 13–16 May 2019; pp. 73–76. [Google Scholar] [CrossRef]
- Åström, K.J.; Kumar, P.R. Control: A perspective. Automatica 2014, 50, 3–43. [Google Scholar] [CrossRef]
- Ogata, K. Modern Control Engineering, 5th ed.; Prentice Hall: New York, NY, USA, 2010. [Google Scholar]
- Alameri, S.; Lazic, D.; Ristanovic, M. A Comparative Study of PID, PID with Tracking, and FPID Controller for Missile Canard with an Optimized Genetic Tuning Method Using Simscape Modelling. Teh. Vjestn. 2018, 25 (Suppl. 2), 427–436. [Google Scholar] [CrossRef]
- Kada, B. A New Methodology to Design Sliding-PID Controllers: Application to Missile Flight Control System. IFAC Proc. Vol. 2012, 45, 673–678. [Google Scholar] [CrossRef]
- Kianfar, K.; Amiri, R.; Bozorgmehr, A. Designing the Non-Linear PID Controller for a Missile. In Proceedings of the 1st International Conference on Informatics and Computational Intelligence, Bandung, Indonesia, 12–14 December 2011; pp. 171–175. [Google Scholar] [CrossRef]
- Zhang, W.; Lei, J.; Shi, X.; Liang, G. Research on Robustness of Double PID Control of Supersonic Missiles. In Information Computing and Applications. ICICA 2011. Communications in Computer and Information Science; Liu, C., Chang, J., Yang, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 244. [Google Scholar] [CrossRef]
- Aboelela, M.A.S.; Ahmed, M.F.; Dorrah, H.T. Design of aerospace control systems using fractional PID controller. J. Adv. Res. 2012, 3, 225–232. [Google Scholar] [CrossRef]
- Ahmed, M.F.; Dorrah, H.T. Design of gain schedule fractional PID control for nonlinear thrust vector control missile with uncertainty. Automatika 2018, 59, 357–372. [Google Scholar] [CrossRef]
- Pan, B.; Fareed, U.; Qing, W.; Tian, S. A novel fractional order PID navigation guidance law by finite time stability approach. ISA Trans. 2019, 94, 80–92. [Google Scholar] [CrossRef]
- Çimen, T. A Generic Approach to Missile Autopilot Design using State-Dependent Nonlinear Control. IFAC Proc. Vol. 2011, 44, 9587–9600. [Google Scholar] [CrossRef]
- Menon, P.K.; Ohlmeyer, E.J. Integrated design of agile missile guidance and autopilot systems. Control Eng. Pract. 2001, 9, 1095–1106. [Google Scholar] [CrossRef]
- Chen, J.; Xiong, Z.; Liang, Z.; Bai, C.; Yi, K.; Ren, Z. Hypersonic Vehicles Profile-Following Based on LQR Design Using Time-Varying Weighting Matrices. In Advances in Some Hypersonic Vehicles Technologies, 1st ed.; Agarwal, R.K., Ed.; IntechOpen: London, UK, 2018; pp. 133–151. [Google Scholar] [CrossRef]
- Nocoń, Ł.; Koruba, Z. Modified linear-quadratic regulator used for controlling anti-tank guided missile in vertical plane. J. Theor. Appl. Mech. 2020, 58, 723–732. [Google Scholar] [CrossRef]
- Park, J.; Kim, Y.; Kim, J.H. Integrated Guidance and Control Using Model Predictive Control with Flight Path Angle Prediction against Pull-Up Maneuvering Target. Sensors 2020, 20, 3143. [Google Scholar] [CrossRef] [PubMed]
- Talole, S.E.; Godbole, A.A.; Kolhe, J.P.; Phadke, S.B. Robust Roll Autopilot Design for Tactical Missiles. J. Guid. Control Dyn. 2011, 34, 107–118. [Google Scholar] [CrossRef]
- Vincent, R.V.; Economou, J.; Wall, D.G.; Cleminson, J. H∞/LQR Optimal Control for a Supersonic Air-Breathing Missile of Asymmetric Configuration. IFAC Pap. 2019, 52, 214–218. [Google Scholar] [CrossRef]
- Devan, V.; Chandar, T.S. Cascaded LQR Control for Missile Roll Autopilot. In Proceedings of the 2018 International CET Conference on Control, Communication, and Computing (IC4), Trivandrum, India, 5–7 July 2018; pp. 45–50. [Google Scholar] [CrossRef]
- Wise, K.A. A Trade Study on Missile Autopilot Design Using Optimal Control Theory. In Proceedings of the AIAA Guidance, Navigation and Control Conference and Exhibit, Hilton Head, SD, USA, 20–23 August 2007. [Google Scholar] [CrossRef]
- Çimen, T. State-Dependent Riccati Equation (SDRE) Control: A Survey. IFAC Proc. Vol. 2008, 41, 3761–3775. [Google Scholar] [CrossRef]
- Franklin, G.F.; Powell, D.J.; Emami-Naeini, A. Feedback Control of Dynamic Systems, 6th ed.; Pearson Education: London, UK, 2011. [Google Scholar]
- Åström, K.J.; Murray, R.M. Feedback Systems: An Introduction for Scientists and Engineers, 1st ed.; Princeton University Press: Princeton, NJ, USA, 2012. [Google Scholar]
- Deng, X.; Sun, X.; Liu, R.; Wei, W. Optimal analysis of the weighted matrices in LQR based on the differential evolution algorithm. In Proceedings of the 29th Chinese Control and Decision Conference (CCDC), Chongqing, China, 28–30 May 2017; pp. 832–836. [Google Scholar] [CrossRef]
- Dong-Qing, F. Simulation Research on the Weighting Matrix of the Optimal Linear System. J. Zhengzhou Univ. Technol. 2000, 21, 11–14. [Google Scholar]
- Li, Y.; Liu, J.; Wang, Y. Design Approach of Weighting Matrices for LQR Based on Multi-objective Evolution Algorithm. In Proceedings of the 2008 IEEE International Conference on Information and Automation, Changsha, China, 20–23 June 2008; pp. 1188–1192. [Google Scholar] [CrossRef]
- Peng, Y.; Chen, Y.Q. Optimal design of the LQR controller based on the improved shuffled frog-leaping algorithm. CAAI Trans. Intell. Syst. 2014, 9, 480–484. [Google Scholar] [CrossRef]
- Siddiq, M.K.; Cheng, F.J.; Bo, Y.W. SDRE Based Integrated Roll, Yaw and Pitch Controller Design for 122 mm Artillery Rocket. Appl. Mech. Mater. 2013, 415, 200–208. [Google Scholar] [CrossRef]
- Abdelhamid, A.M.; El-Gaafary, A. Optimal Decentralized LQR Control to Enhance Multi-Area LFC System Stability. Math. Probl. Eng. 2018, 2018, 7232915. [Google Scholar] [CrossRef]
- Stefanescu, R.M.; Prioroc, C.L.; Stoica, A.M. Weighting matrices determination using pole placement for tracking maneuvers. UPB Sci. Bull. Ser. D Mech. Eng. 2013, 75, 31–40. [Google Scholar]
- Siouris, G.M. Missile Guidance and Control Systems, 1st ed.; Springer: New York, NY, USA, 2004. [Google Scholar]
- Zarchan, P. Tactical and Strategic Missile Guidance, 6th ed.; AIAA: Reston, VA, USA, 2012. [Google Scholar]
- Zaikang, Q.; Defu, L. Design of Guidance and Control Systems for Tactical Missiles, 1st ed.; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Brugarolas, P.B.; Fromion, V.; Safonov, M.G. Robust switching missile autopilot. In Proceedings of the American Control Conference, Philadelphia, PA, USA, 24–26 June 1998; Volume 6, pp. 3665–3669. [Google Scholar] [CrossRef]
- Idan, M.; Shima, T.; Golan, O.M. Integrated Sliding Mode Autopilot-Guidance for Dual Control Missiles. J. Guid. Control Dyn. 2007, 30, 1081–1089. [Google Scholar] [CrossRef]
- Lee, H.C.; Choi, J.W.; Song, T.L.; Song, C.H. Agile Missile Autopilot Design via Time-Varying Eigenvalue Assigment. In Proceedings of the 8th International Conference on Control, Automation, Robotics and Vision, Kunming, China, 6–9 December 2004; pp. 1832–1837. [Google Scholar] [CrossRef]
- McLain, T.W.; Beard, R.W. Nonlinear Robust Missile Autopilot Design Using Successive Galerkin Approximation. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, Portland, OR, USA, 9–11 August 1999; pp. 384–391. [Google Scholar] [CrossRef][Green Version]
- Shamma, J.S.; Cloutier, J.R. Gain-Sheduled Missile Autopilot Design Using Linear Parameter Varying Transformations. J. Guid. Control Dyn. 1993, 16, 256–263. [Google Scholar] [CrossRef]
- Shima, T.; Idan, M.; Golan, O.M. Sliding-Mode Control for Integrated Missile Autopilot Guidance. J. Guid. Control Dyn. 2006, 29, 250–260. [Google Scholar] [CrossRef]
- Zhao, Z.; Jun, H. A High Gain Integral Dynamic Compensation Method for Nonlinear Missile Control. IFAC Proc. Vol. 2011, 44, 2048–2053. [Google Scholar] [CrossRef]
- Cronvich, L.L. Missile Aerodynamics. Johns Hopkins APL Tech. Dig. 1983, 4, 175–186. [Google Scholar]
- Kurov, V.D.; Dolzhansky, J.M. Principles Behind the Solid-Propellant Rocket Design, 1st ed.; Publishing House of Ministry of National Defense: Warsaw, Poland, 1964. (In Polish) [Google Scholar]
- Moore, F. Approximate Methods for Weapon Aerodynamics, 1st ed.; Progress in Astronautics and Aeronautics; AIAA: Reston, VT, USA, 2000; Volume 186. [Google Scholar] [CrossRef]
- Ordaz, P.; Poznyak, A. The Furuta’s Pendulum Stabilization without the Use of a Mathematical Model: Attractive Ellipsoid Method with KL-adaptation. In Proceedings of the 51st IEEE Conference on Decision and Control (CDC), Maui, HI, USA, 10–13 December 2012; pp. 7285–7290. [Google Scholar] [CrossRef]
- Ordaz, P.; Poznyak, A. Adaptive-Robust Stabilization of the Furuta’s Pendulum via Attractive Ellipsoid Method. J. Dyn. Syst. Meas. Control 2016, 138, 021005. [Google Scholar] [CrossRef]
- Franklin, G.F.; Powell, D.J.; Workman, M.L. Digital Control of Dynamic Systems, 3rd ed.; Addison-Wesley: Menlo Park, CA, USA, 1997. [Google Scholar]
- Lin, C.L.; Chen, Y.Y. Design of Fuzzy Logic Guidance Law Against High-Speed Target. J. Guid. Control Dyn. 2000, 23, 17–25. [Google Scholar] [CrossRef]
- Bużantowicz, W. Dual-control missile guidance: A simulation study. J. Theor. Appl. Mech. 2018, 56, 727–739. [Google Scholar] [CrossRef]
- Bużantowicz, W. A Method of Visualizing Velocity and Acceleration Profiles of an Air Target. Probl. Mechatronics. Armament Aviat. Saf. Eng. 2018, 9, 9–20. [Google Scholar] [CrossRef]

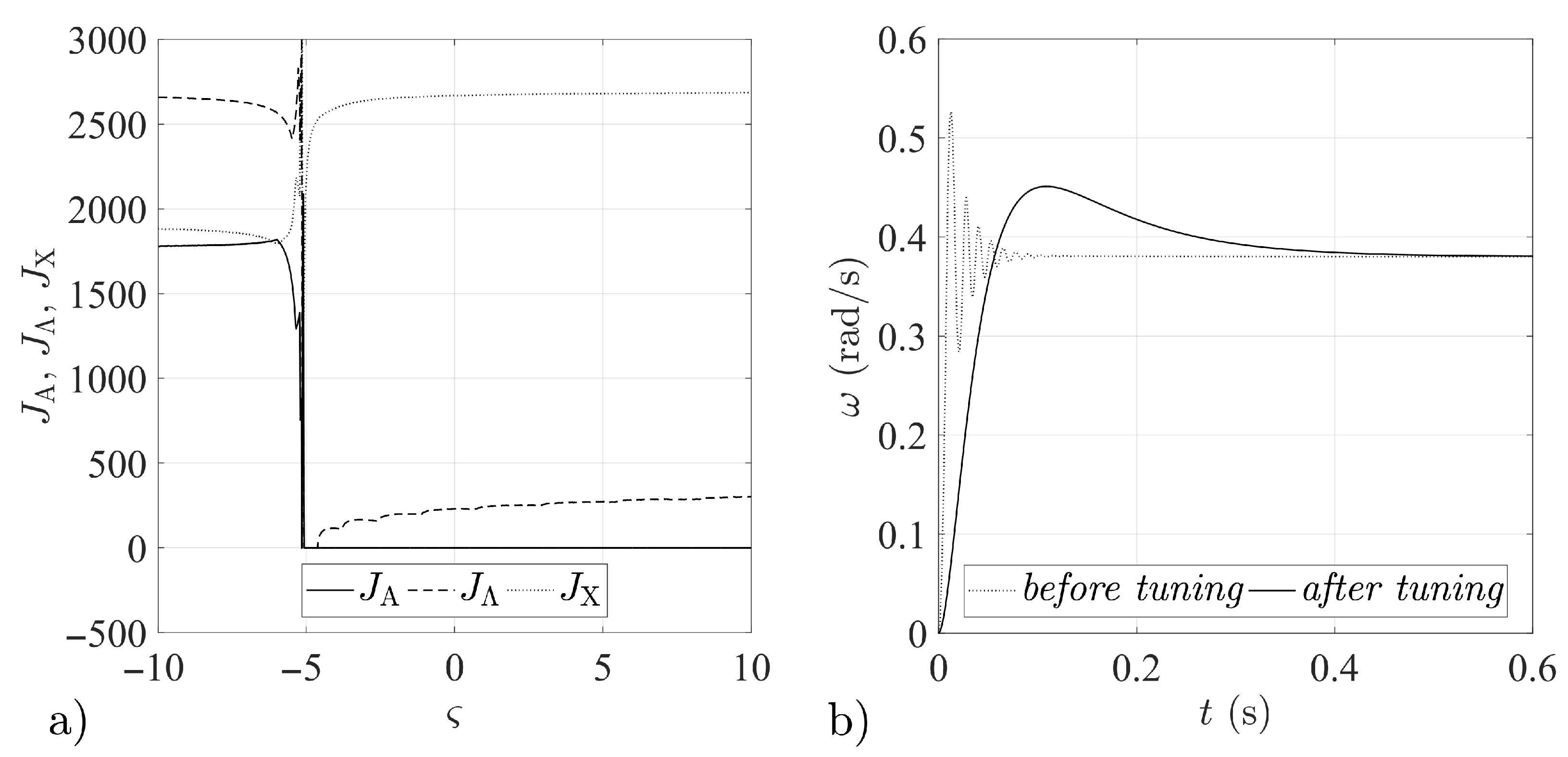
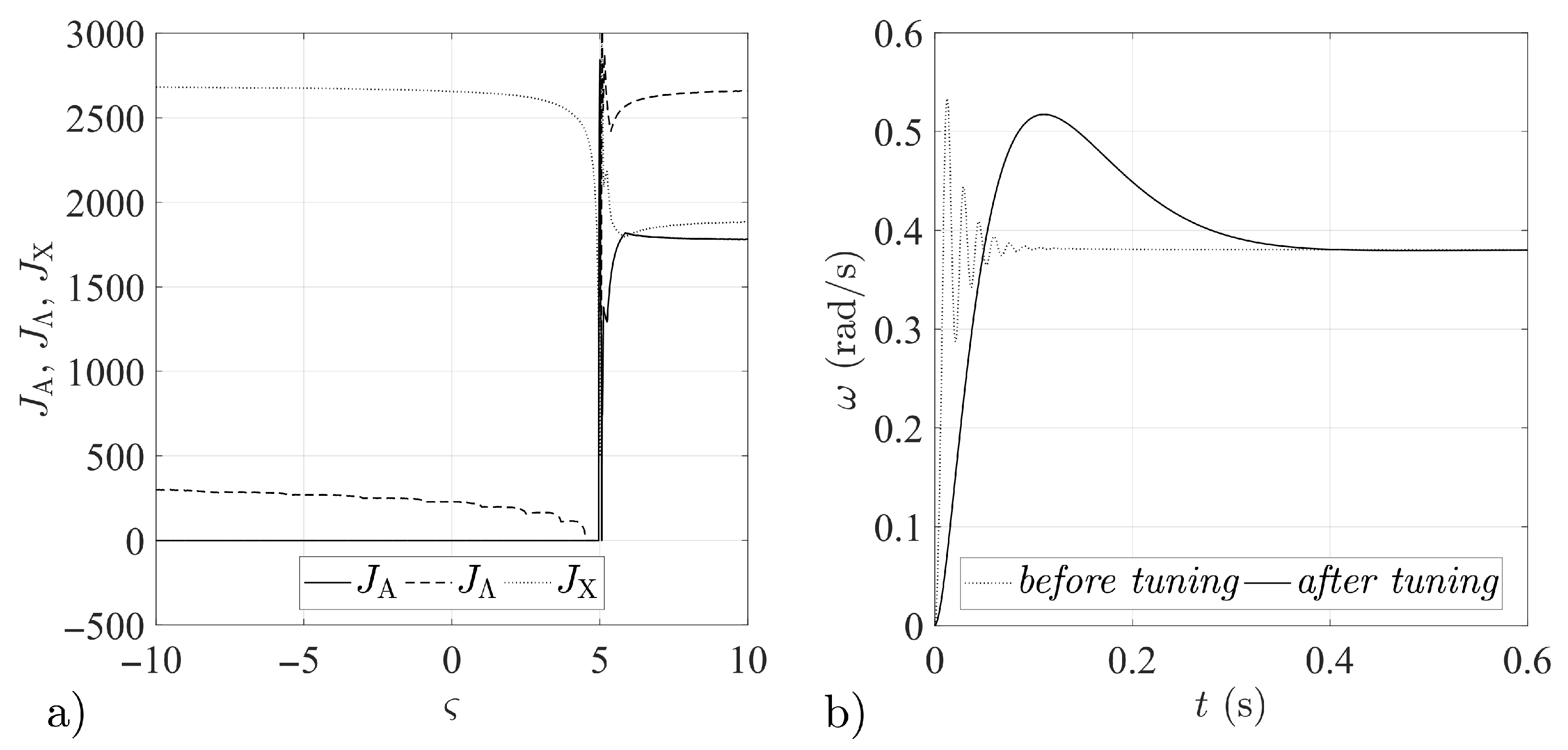

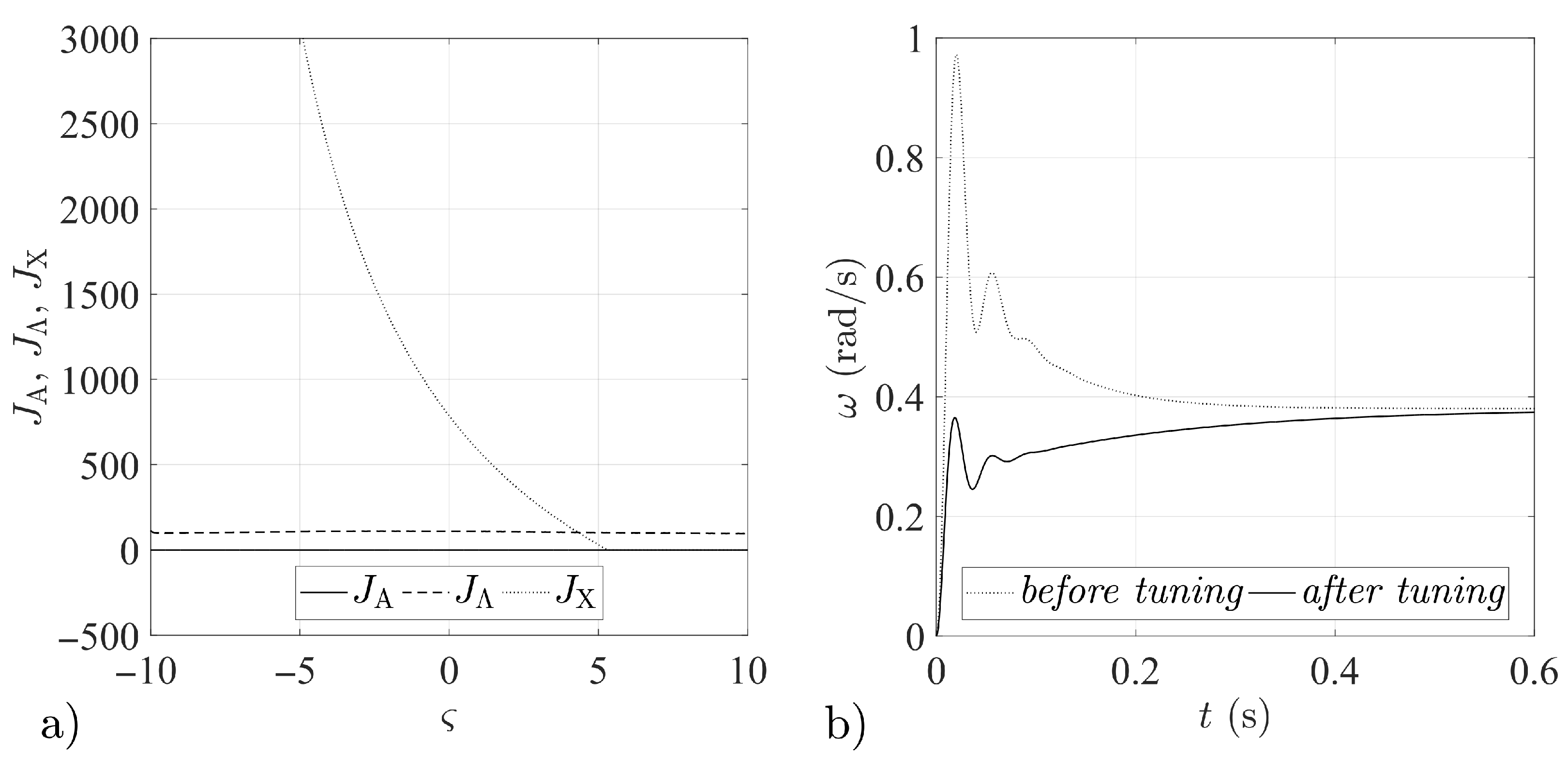


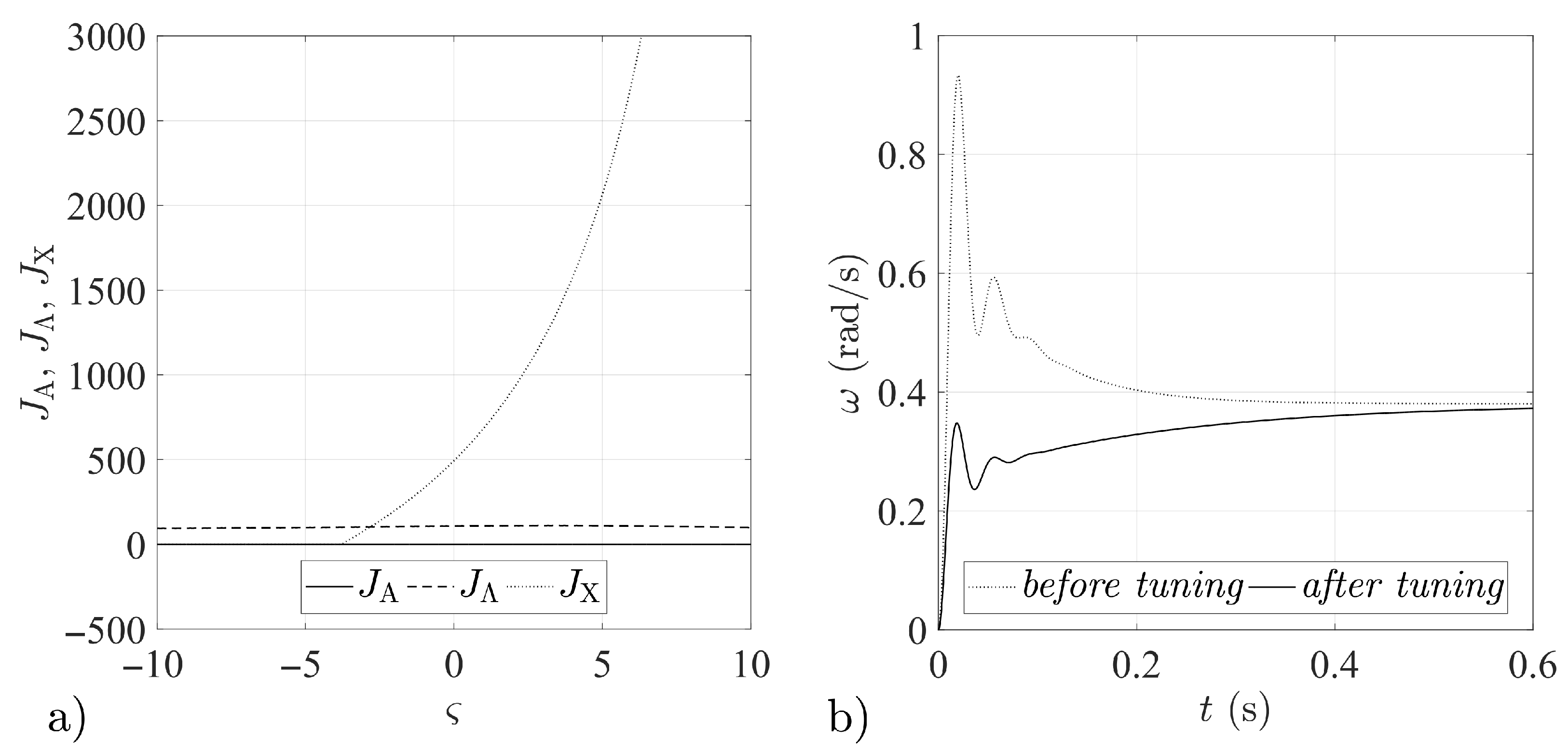




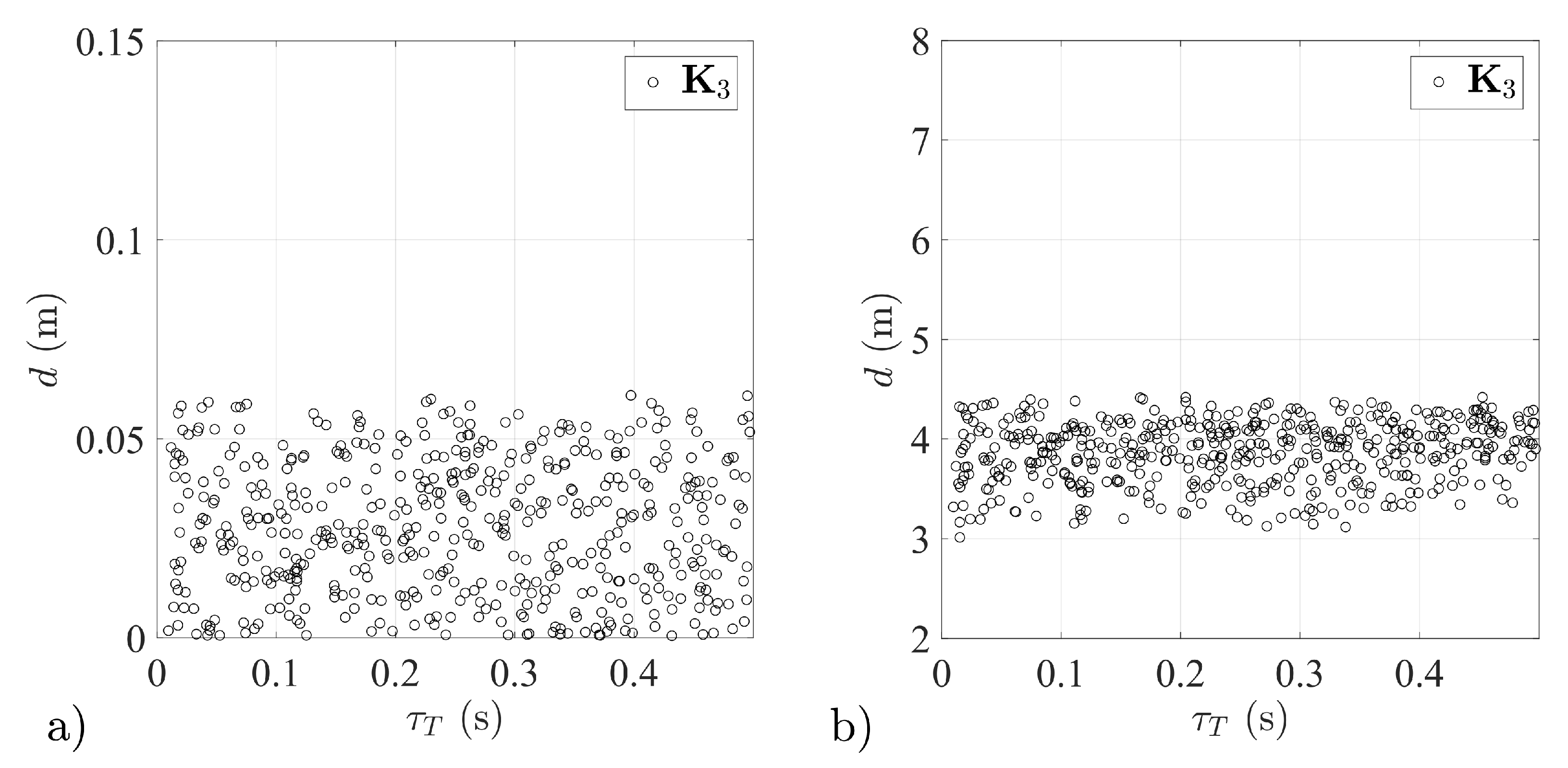

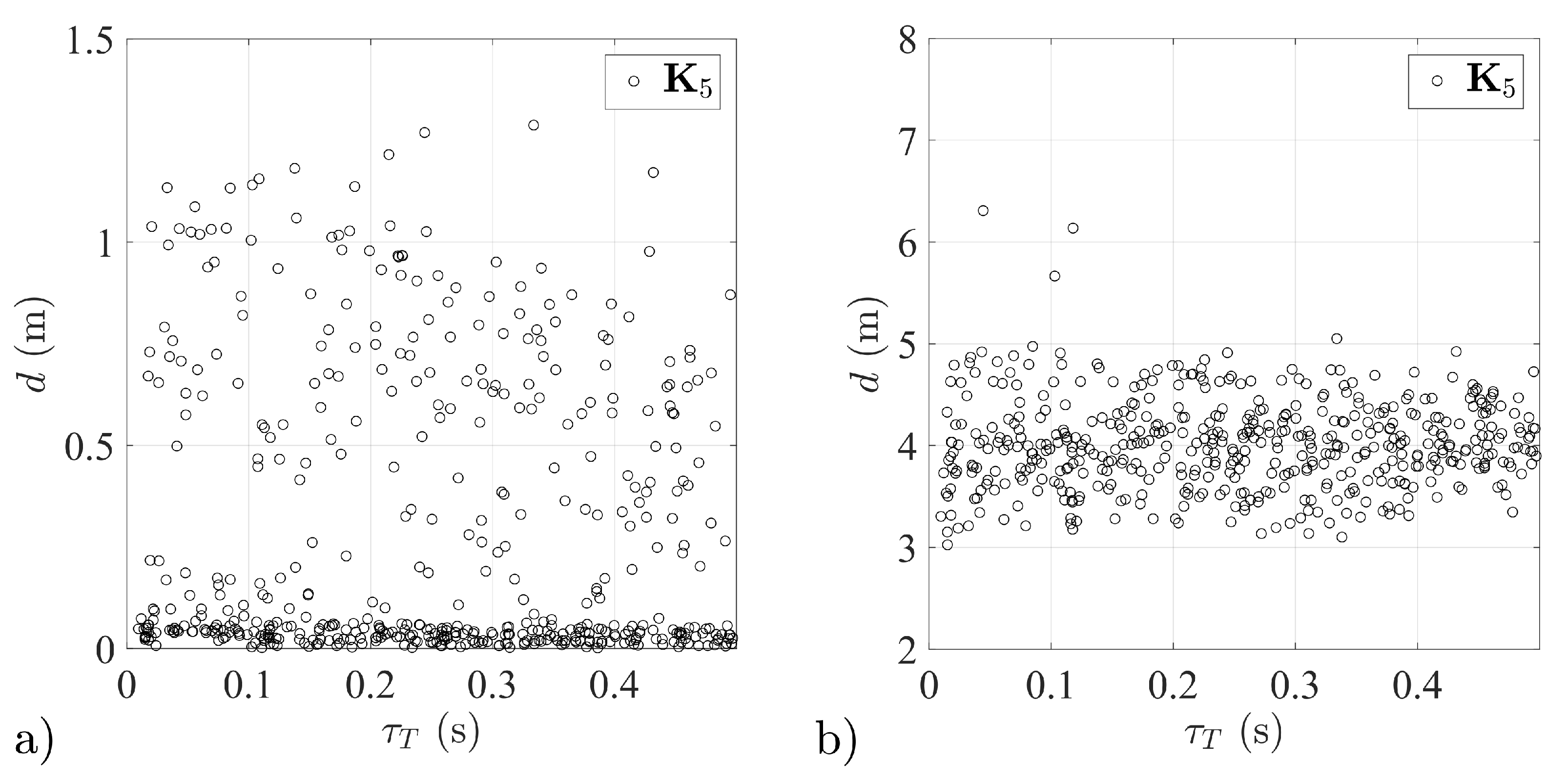

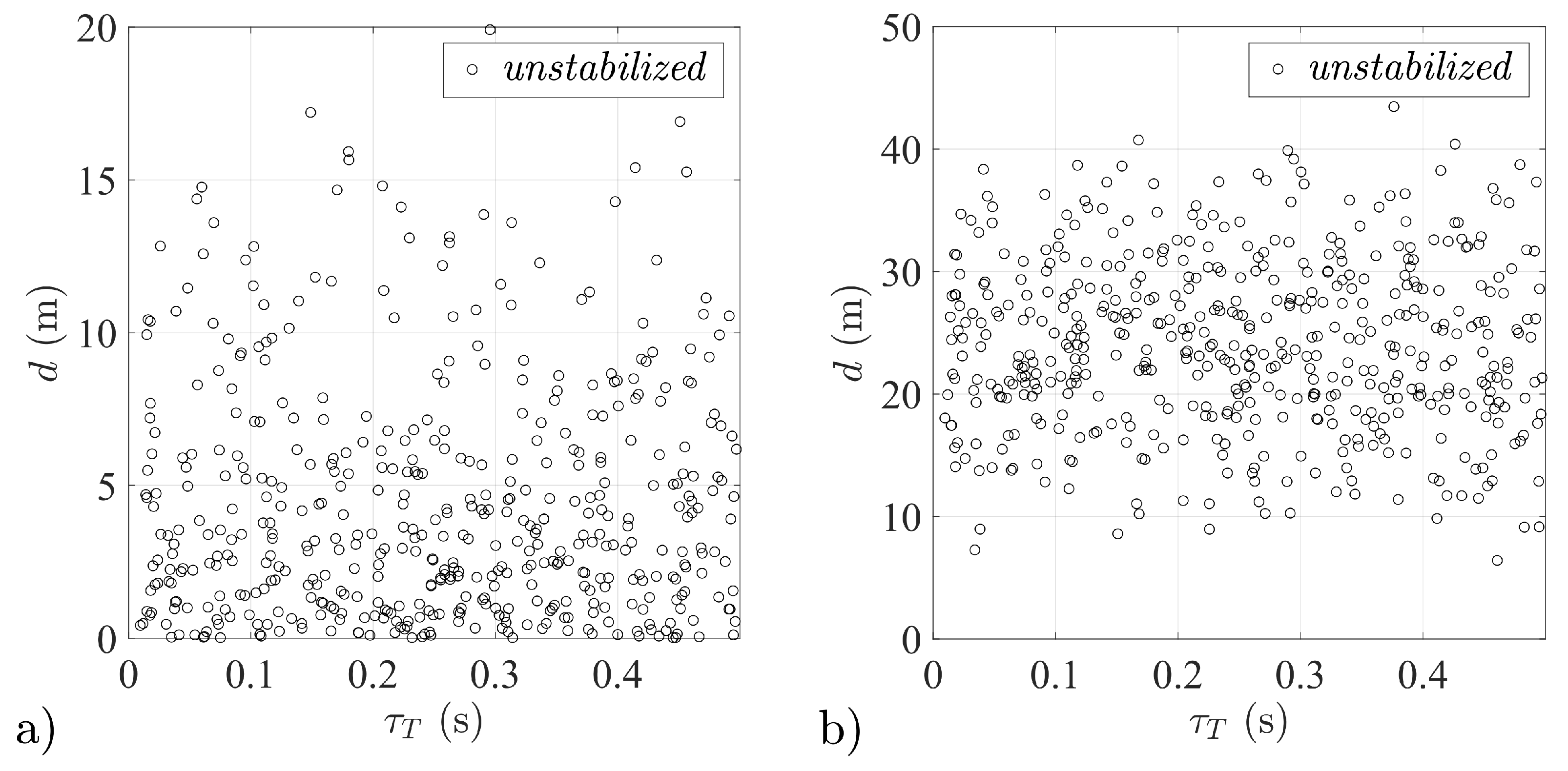
| Gain | Tuning Parameter | Axis of Symmetry | Tuning Parameter | Gain | ||
|---|---|---|---|---|---|---|
| Matrix | Matrix | |||||
| −5.060 | −5.015 | −0.045 | 5.015 | 4.970 | ||
| 0.940 | −0.310 | 1.250 | 0.310 | 1.560 | ||
| −9.190 | −8.455 | −0.735 | 8.455 | 7.720 | ||
| −6.210 | −7.000 | 0.790 | 7.000 | 7.790 |
| Missile | Gain | d | |||||
|---|---|---|---|---|---|---|---|
| Number | Matrix | (s) | (m) | (m/s) | (rad/s) | (rad) | (rad) |
| 1 | 1.68 | 3.51 | 163.28 | 0.170 | 0.029 | 0.4009 | |
| 2 | 1.68 | 3.51 | 161.05 | 0.136 | 0.029 | 0.3608 | |
| 3 | 1.68 | 3.51 | 164.26 | 0.154 | 0.029 | 0.3615 | |
| 4 | 1.68 | 4.22 | −102.01 | −0.459 | −0.032 | 0.4604 | |
| 5 | 1.68 | 3.51 | 159.16 | 0.090 | 0.029 | 0.3744 | |
| 6 | 1.61 | 242.53 | ― | ― | ― | 6.6262 | |
| 7 | 1.61 | 242.48 | ― | ― | ― | 6.6212 | |
| 8 | 1.68 | 3.51 | 155.68 | 0.067 | 0.029 | 0.3751 |
| Missile Number | Gain Matrix | |||
|---|---|---|---|---|
| (s) | (m) | (rad) | ||
| 1 | 1.5536 | 0.0269 | 0.8779 | |
| 2 | 1.5442 | 0.0324 | 0.8034 | |
| 3 | 1.5536 | 0.0259 | 0.8046 | |
| 4 | 1.5535 | 0.6617 | 1.0073 | |
| 5 | 1.5544 | 0.2885 | 0.8369 | |
| 8 | 1.5543 | 0.4090 | 0.8334 | |
| 9 | unstabilized | 1.5536 | 5.7028 | 6.3302 |
| Missile Number | Gain Matrix | |||
|---|---|---|---|---|
| (s) | (m) | (rad) | ||
| 1 | 1.5575 | 3.87 | 0.7433 | |
| 2 | 1.5580 | 4.10 | 0.6692 | |
| 3 | 1.5580 | 3.86 | 0.6705 | |
| 4 | 1.5576 | 5.44 | 0.8537 | |
| 5 | 1.5585 | 4.07 | 0.6946 | |
| 8 | 1.5553 | 4.17 | 0.6945 | |
| 9 | unstabilized | 1.5609 | 23.99 | 5.3195 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bużantowicz, W. Tuning of a Linear-Quadratic Stabilization System for an Anti-Aircraft Missile. Aerospace 2021, 8, 48. https://doi.org/10.3390/aerospace8020048
Bużantowicz W. Tuning of a Linear-Quadratic Stabilization System for an Anti-Aircraft Missile. Aerospace. 2021; 8(2):48. https://doi.org/10.3390/aerospace8020048
Chicago/Turabian StyleBużantowicz, Witold. 2021. "Tuning of a Linear-Quadratic Stabilization System for an Anti-Aircraft Missile" Aerospace 8, no. 2: 48. https://doi.org/10.3390/aerospace8020048
APA StyleBużantowicz, W. (2021). Tuning of a Linear-Quadratic Stabilization System for an Anti-Aircraft Missile. Aerospace, 8(2), 48. https://doi.org/10.3390/aerospace8020048






