1. Introduction
Ever since the early years of aviation, the design, analysis, and optimization of aircraft structures has been receiving ever-increasing attention from the scientific community and has, therefore, been subjected to extensive research studies. As a result, a wide variety of multi-fidelity computational tools, with application to a plethora of analysis disciplines, has emerged. During an aircraft design process, consisting mainly of the conceptual, preliminary and detailed design stages [
1], the various computational tools are designated to a corresponding design stage mainly based on the computational time, the modeling complexity and the ability to capture the physical phenomena present. Early on, low-fidelity models, associated with fast turnaround times, yet incapable of modeling higher order phenomena, are employed, along with empirical knowledge, to steer the design towards optimality. As the design knowledge on the current candidate configuration matures, higher fidelity tools are employed, aiming to replicate the relevant phenomena with greater accuracy, albeit at an elevated and often prohibitive computational cost. Throughout the stages, all relevant tools are coupled with optimization algorithms in order to gain further knowledge regarding the design space, as well as to obtain the corresponding optimized solution.
A key enabler to the mass reduction trend of aeronautical structures is the rise of composite materials in the aeronautics field. Lighter yet stiffer configurations have emerged with improved static and dynamic aeroelastic response through tailoring of their properties [
2]. One of the main challenges, nevertheless, remains the optimization of such structures, since the introduction of composite materials induces further computational and algorithmic complexity. On one hand, ply angles and thicknesses can be expressed as design variables, resulting into a more complex and irregular design space, but with facilitated algorithmic applicability. On the other hand, the use of lamination parameters for optimization purposes has been extensively studied over the past few years. Miki and Sugiyama [
3] was among the first to generate optimum designs of laminated composite plates for required in-plane and maximum bending stiffness, buckling strength and natural frequency utilizing lamination parameters. Fukunaga et al. [
4] explored the effect of bend-twist coupling on the fundamental frequency of symmetric laminated plates, indicating that this type of coupling reduces the fundamental frequencies. The optimal laminate configuration that maximizes the fundamental frequencies was also obtained. In his work, Liu et al. [
5] maximized the buckling load of composite materials panels using flexural parameters and compared its results with a stacking sequence optimization design generated via a genetic algorithm, indicating a close correlation between the two methods. In later works, efforts were directed towards closure of the up-to-then incomplete feasible design space of the lamination parameters [
6], and the aeroelastic tailoring of regular and variable stiffness composite materials wings [
7,
8], as well as the stiffness optimization subject to aeroelastic constraints [
9]. Macquart et al. [
10], as well as Bordogna et al. [
11], extended the capabilities of the state-of-the art lamination parameters optimization algorithms, introducing blending constraints in order to guarantee a certain degree of ply continuity inside a variable stiffness composite wing.
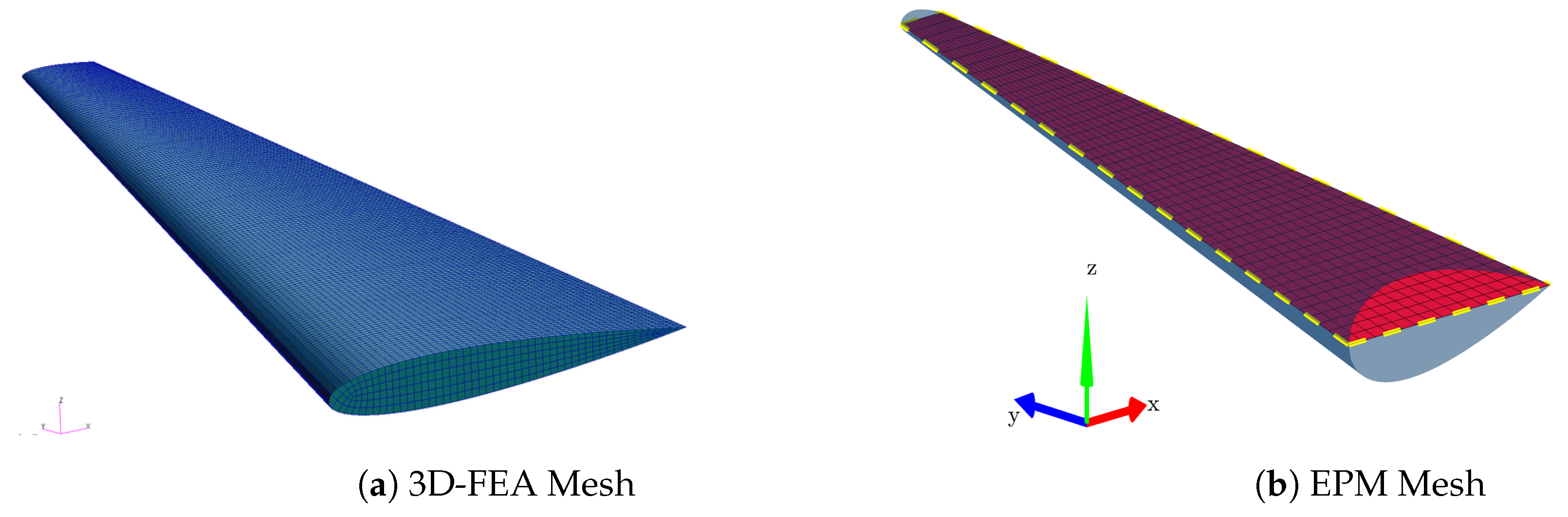
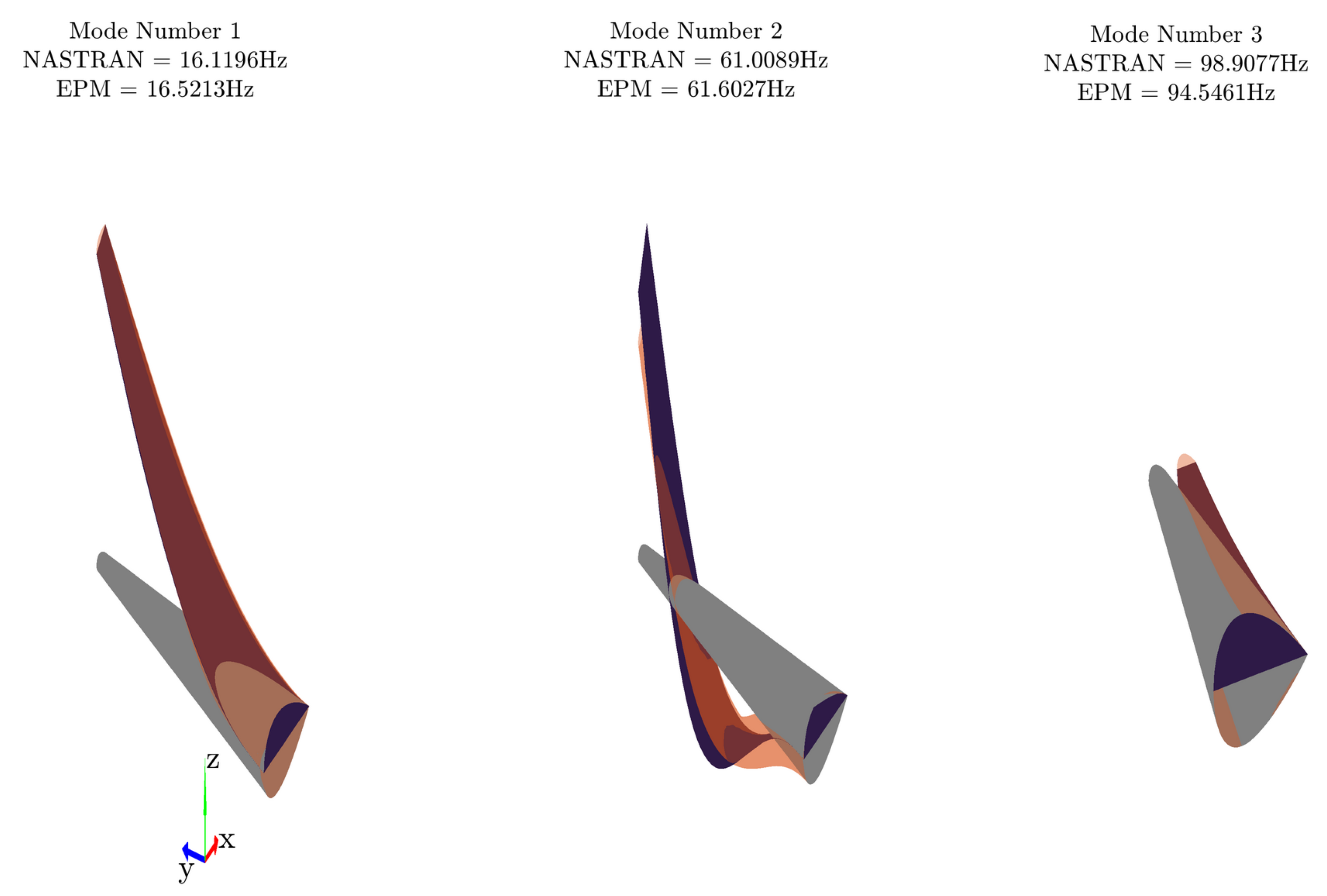
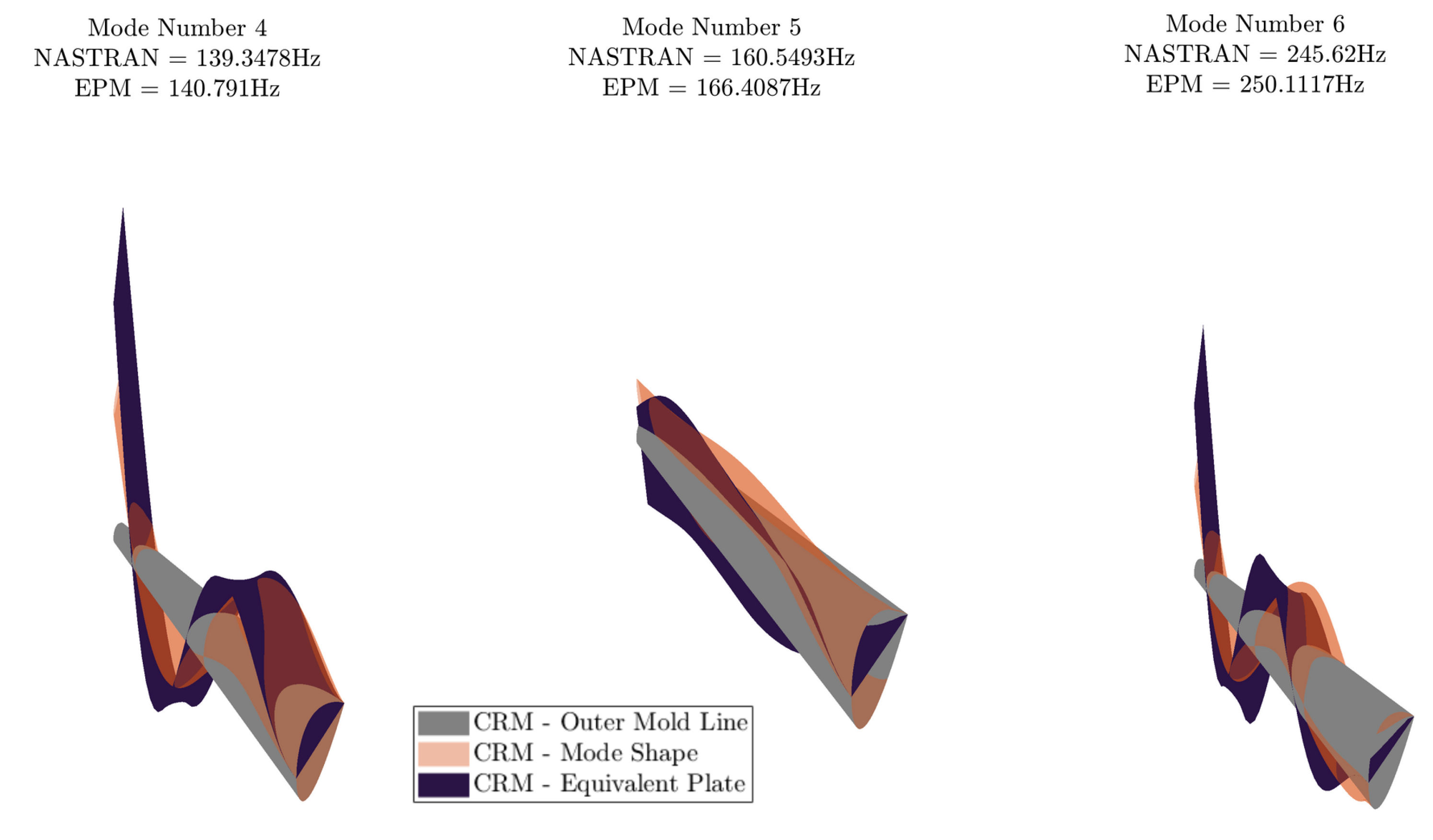
It becomes evident that various disciplines must be involved in the design optimization process of a modern airliner composite wing, the main contributors being the structural and aerodynamic analysis, since they significantly influence the performance and safety of wing structures. Additional disciplines may be included on a case by case basis. Regarding the structural representation, Finite Element Analysis (FEA) models are commonly used at the preliminary and detailed design stages, with reduced order, equivalent beam or plate models aiming to provide greater insight into the candidate configurations, as well as reduce the design space at an early stage of the design process. The equivalent plate methodology (EPM), often preferred due to its inherent facilitated coupling capabilities with concurrent aerodynamic and optimization codes, initially developed by Giles in a series of research papers [
12,
13,
14], was proven to be a robust and accurate enough numerical tool for the structural analysis of aircraft wings, as the comparison with a 3D FEA wing model showed. Later on, Livne et al. [
15] included the EPM in a multidisciplinary structural, aerodynamics and control analysis and optimization framework of a composite aircraft wing, and went on to also include transverse shear effects in the EPM formulation [
16]. The large deformation non-linear static and dynamic behavior of wings via the EPM formulation was also studied by Livne and Navarro [
17], with good correlation up to relatively large levels of loading being obtained. In their work, Kapania and Liu [
18] introduced Legendre polynomials in the EPM in order to avoid the numerical ill-conditioning problem often accompanied by the usage of simple polynomials as trial functions. The accuracy and efficiency of the method was demonstrated via a series of static, as well as free, vibration loading scenarios. Through a series of works, Krishnamurthy and Eldred [
19], Krishnamurthy and Tsai [
20], Krishnamurthy [
21] developed an optimization framework for obtaining an equivalent plate by minimizing an error function accounting for the displacements and frequencies between the two numerical models under consideration. The methodology was initially tested in plates with and without discrete damage and was later used to evaluate the static and dynamic structural response of a baseline aircraft wing. In each case, an optimum thickness and concentrated mass distribution for the equivalent plate model was obtained. A scaling down methodology was also developed and put into test via a series of numerical tests. The developed methodology indicated that the static response of the wing structure was accurately predicted, with the equivalent plate model being capable of reproducing the first five frequencies of the wing structure within five percent. Over the last few years, Na and Shin [
22], along with Henson and Wang [
23], further enhanced the current EPM capabilities to account for control surfaces, as well as for tow steered composite wing skins, respectively.
On the other hand, implementation of dedicated computational analysis tools for the relevant disciplines into optimization frameworks is of paramount importance. Pioneering research was conducted by Triplett [
24] and Love and Bohlman [
25], where one of the earliest multidisciplinary design and optimization tool, aeroelastic Tailoring and Structural Optimization (TSO), was developed. The Rayleigh-Ritz EPM for the structural model, coupled with the Doublet Lattice Method were combined to optimize the thickness distribution and laminate orientations of a fighter wing subject to strength and flutter velocity constraints. Haftka [
26] developed an automated procedure for the design and optimization of composite wings subject to strength and flutter constraints. A major conclusion drawn from this work was that the flutter speed may not be a continuous function of the structural stiffness; hence, in optimization under flutter constraint, the constraint should be formulated in terms of other, continuous parameters relative to the flutter phenomenon. Haftka [
27] also coupled a lifting-line theory-based aerodynamics model with a wingbox FEA model to perform aeroelastic analysis and optimization of a wing under stress and drag constraints. Weight versus drag trade-off studies for aluminium and composite wings were conducted. It was found that the composite wings were associated with lower mass and drag but their increased flexibility resulted in a larger variation in drag in the Pareto front. One of the earliest aerostructural optimization analysis was performed by Grossman et al. [
28], where, utilizing lifting-line aerodynamic models, along with beam equations for the structural representation, the optimum shape, and structural configuration of a sailplane wing was obtained. In a similar but more complex fashion, by means of numerical tools, Grossman et al. [
29] optimized a transport aircraft wing, while in parallel developing methodologies for the calculation of the sensitivity derivatives, as well as a sequential approximate optimization module.
While optimization procedures using low-fidelity components for the representation of the structural and aerodynamic problem have to date offered major gains in the overall performance of wing structures, they have inherent limitations. The limitations result from the main assumptions of such methods; for aerodynamics, non-linearities are typically neglected and simplified wetted geometries are used. On the structural side, the assumption of linear behavior, together with simplified FEA models, sees widespread use in optimization. Increasing the fidelity of the aerodynamic and structural solvers can influence the optimization results [
30] and represents a step beyond the current state-of-the-art [
31,
32]. For the coupled aeroelastic problem, linear approaches, both in terms of structure and aerodynamics represented in the frequency domain, are commonly used. Studying the dynamic aerostructural response with such formulations should be limited to linear or weakly non-linear cases due to the underlying assumptions. The dynamic response of wings that operate in the transonic region, as well as cases of boundary layer-shockwave interaction and flutter from flow separation (common in rotor blades), requires a high-fidelity representation of the aerodynamics to be accurately captured [
33,
34,
35]. Even when the aeroelastic behavior is correctly captured, the increased detail in the unsteady aerodynamic loads can influence the optimum result. In Reference [
36], different unsteady aerodynamic methods were used in a multi-fidelity aerostructural optimization of a helicopter rotor, leading to variation in the final optimum blade geometry. Even for the static aeroelastic case, differences due to varying fidelity of the aerodynamics solvers have indeed been pinpointed [
37]. Furthermore, the presence of structural non-linearities can additionally have a serious effect on the aeroelastic behavior of a wing structure [
38]. Such non-linearities are expected to become more common in the future due to the tendency to move to ever more efficient wings of very high-aspect ratio combined with highly flexible composite materials.
It becomes evident that the obtained optimized solution corresponds to a specific level of fidelity. Depending on the case, the assumptions in modeling each discipline can be justified. However, they could also represent an oversimplification of the actual physics of the problem and yield a sub-optimal design. The optimized solution can be in doubt due to the level of fidelity with which the various disciplines are approximated regardless of how tight the optimization constraints are set and how rigorous the optimization algorithm becomes. Thus, the fidelity of each component of the optimization framework must be carefully evaluated by taking into account the outstanding properties of each problem or by confirming the validity of the solution using higher fidelity analysis.
In the following brief overview, the emphasis will be mainly upon the impact of increasing the fidelity of the aerodynamic formulation. This is a conscious choice because solution and modeling procedures for even detailed 3D Shell-3D Beam Finite Element representations of the structure have become quite efficient. However, some examples covering the impact of high-fidelity structural modeling are also mentioned below.
Gains from the inclusion of high-fidelity analysis component to the optimization of future unconventional aircraft wing concepts have been reported in literature. In References [
39,
40], high-fidelity Computational Fluid Dynamics (CFD) was used to provide realistic predictions of engine-pylon-strut interference and viscous drag that was fed to the optimization procedure. Significant reduction in structural mass for a strut braced wing based upon the Common Research Model (CRM) wing planform was obtained by Variyar et al. [
41] by using a complete multi-fidelity optimization framework that encompassed both high-fidelity aerodynamics and structural dynamics. For a similar aircraft configuration, the accurate derivation of aerodynamic loads is stressed as a crucial parameter by Variyar et al. [
41] and a multi-fidelity approach even in conceptual stage deemed advantageous. Qian and Alonso [
42] have included a high-fidelity structural model in an aerostructural optimization procedure to discover gains in range and a reduction in structural mass. Smith et al. [
43] mention that including high-fidelity CFD and non-linear structural effects in the aeroelastic modeling of high aspect ratio wings can lead to important differences compared to linear methods. In this particular case, linear methods were found to lead to an overly conservative result for tip displacement and aerodynamic loads.
Unsurprisingly, most recently published studies regarding high-fidelity optimization frameworks deal with innovative wing configurations that are prone to aeroelastic instabilities (high-aspect ratio wings, transonic aircraft) and may also contain structural non-linearities. Quantifying the impact of higher-fidelity optimization to current state-of-the-art wings has not been as prominent in the literature. Liem et al. [
44] have shown that significant benefits from high-fidelity aerostructural optimization can also be realized for current state-of-the-art wings.
The decision on the required analysis capabilities that dictates the adequate level of fidelity has important ramifications from a computational-cost perspective. Further numerical analysis with high-fidelity tools is typically performed to assess the validity of the low-fidelity results and support the decision on the adequate level of fidelity. This approach has been used in industry and research centers [
45,
46,
47]. Multiple strategies to bridge the gap in computational cost and capabilities between high and low-fidelity potential flow solutions represented by the 3D Euler Equations and simple Vortex Lattice and Doublet Lattice Method (VLM and DLM) formulations, respectively have been presented in the literature. A practical approach is to employ medium-fidelity aerodynamics. This level of fidelity is represented by advanced 3D Panel Methods. These can allow for a more detailed geometrical modeling of aeronautical structures, including non-planar aerodynamic shapes. Furthermore, by employing advanced wake modeling techniques, reasonable approximations for re-circulation effects and induced drag can be achieved at a lower cost than high-fidelity solutions. Kennedy and Martins [
48] have employed a 3D Panel Method in a parallel aerostructural optimization framework and have additionally checked the validity of the obtained aerodynamic solutions against high-fidelity ones. Mieloszyk and Goetzendorf-Grabowski [
49] have used a 3D Panel method in conjunction with corrections for viscous effects to evaluate the flight dynamics stability constraints in the multidisciplinary optimization of a joined wing box configuration. Recently, a finite element beam model was coupled to 3D Panel Aerodynamics and employed in aeroelastic optimization by Conlan-Smith and Schousboe Andreasen [
50].
A natural path to reduce the computational cost associated with increased fidelity is to search for an optimized solution in smaller regions of the parameter space (thus requiring a smaller number of optimization iterations). This approach requires a priori some knowledge of the region in parameter space where the optimum is likely to be detected. In our multi-fidelity optimization framework, the low-fidelity part is entrusted with obtaining an initial optimized design with a high numerical certainty. Then, the high-fidelity modules explore further gains in performance by modeling the physics that the low-fidelity modules cannot. Overall, only a fraction of the iterations using high-fidelity are performed (in the range of 5–10%). Such an approach prevents a drastic increase in computational cost. The procedure of gradually moving to increasing level of fidelity but with a smaller number of solutions is typical of a multi-fidelity optimization framework [
51,
52].
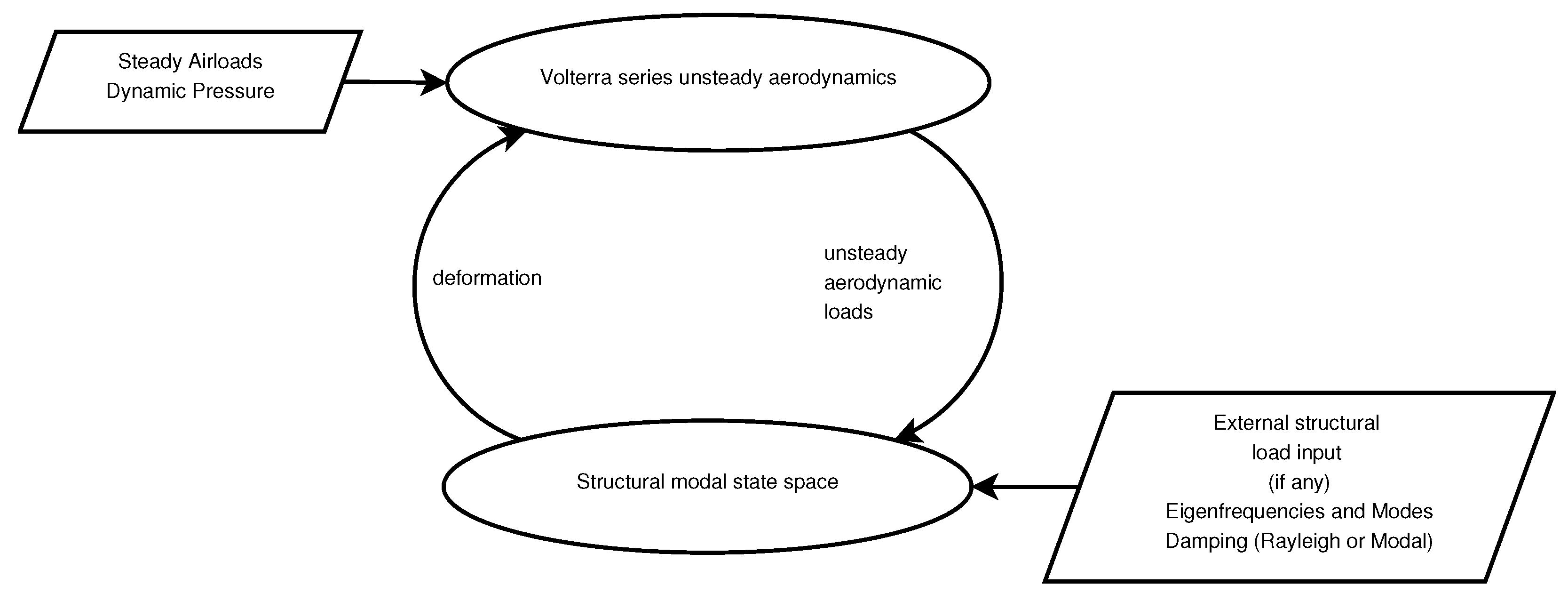
Still, a very high computational cost due to each high- fidelity solution is incurred, particularly so if a higher number of runs is needed. This cost is further exacerbated in the aeroelastic solutions. The cost of solving the unsteady aerodynamics with a high-fidelity method (typically a form of the Navier–Stokes equations with spatial approximation provided by the finite volume or element methods) tends to be higher than the structural solution. A combined computational cost of solving the structure and unsteady aerodynamics plus the interpolation of data between the two systems is then incurred. To mitigate this cost significantly, particularly for multiple runs around the same reference flow conditions, Order Reduction techniques can be used. Such techniques strive to approximate the physical behavior of the system on a greatly reduced (but physically meaningful) set of degrees of freedom. Most reduced order models are trained using samples from full solutions. The cost of obtaining those samples represents the main cost considering their use, since, afterward, they provide extremely rapid solution times. Several types of reduced order models (ROMs) have been used to treat aeroelasticity, including those based on (a) Mathematical decompositions, such as proper orthogonal decomposition (POD) [
53,
54], (b) Series approximations (for example, Volterra or Wiener) [
55], and (c) Machine Learning techniques [
56]. In our work, we integrate a ROM to the optimization framework that uses a Volterra series approximation for the unsteady aerodynamics coupled with mode superposition on the structural side. It serves as an intermediate step between low-fidelity aeroelastic solutions and the full coupled high-fidelity final run.
Although multiple analysis techniques of varying fidelity have been successfully used in optimization, the different computational cost that they incur combined with their setup complexity leads to a different combination of methods being adopted in each design stage of an aircraft structure. Specifically in industry, the current state-of-the-art is to include higher fidelity modules as design maturity increases (preliminary and detailed design stages), while exploiting the low computational cost of low-fidelity methods in the conceptual stage [
47,
57]. In the conceptual stage, efficient low-fidelity methods (panel method aerodynamics and equivalent beam or plate structural models) are commonly preferred due to the requirement to evaluate the feasibility of a vast number of design configurations.
Advances in computational capabilities combined with robust and optimized high-fidelity analysis tools has resulted in a trend to incorporate such methods already from the preliminary stage. In order to bridge the gap between capabilities and computational cost between high and low-fidelity methods, two of the most attractive methodologies consist of employing correction factors to the lower-fidelity tools [
37,
58], as well as constructing surrogate models. Furthermore, the construction of multi-fidelity optimization frameworks represents a highly active research topic. Such frameworks strive to combine the advantages of both worlds while mitigating the associated computational cost [
47].
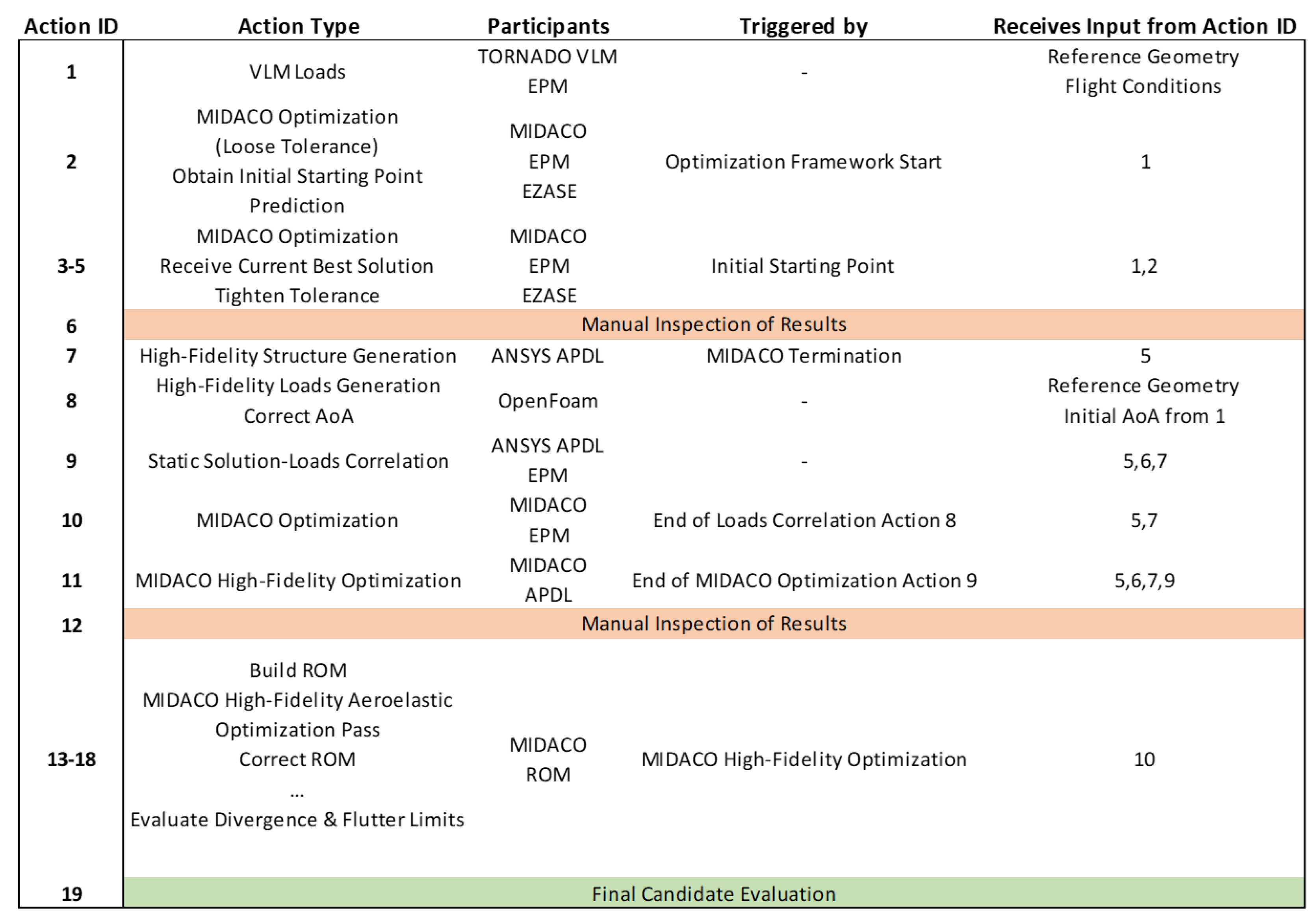
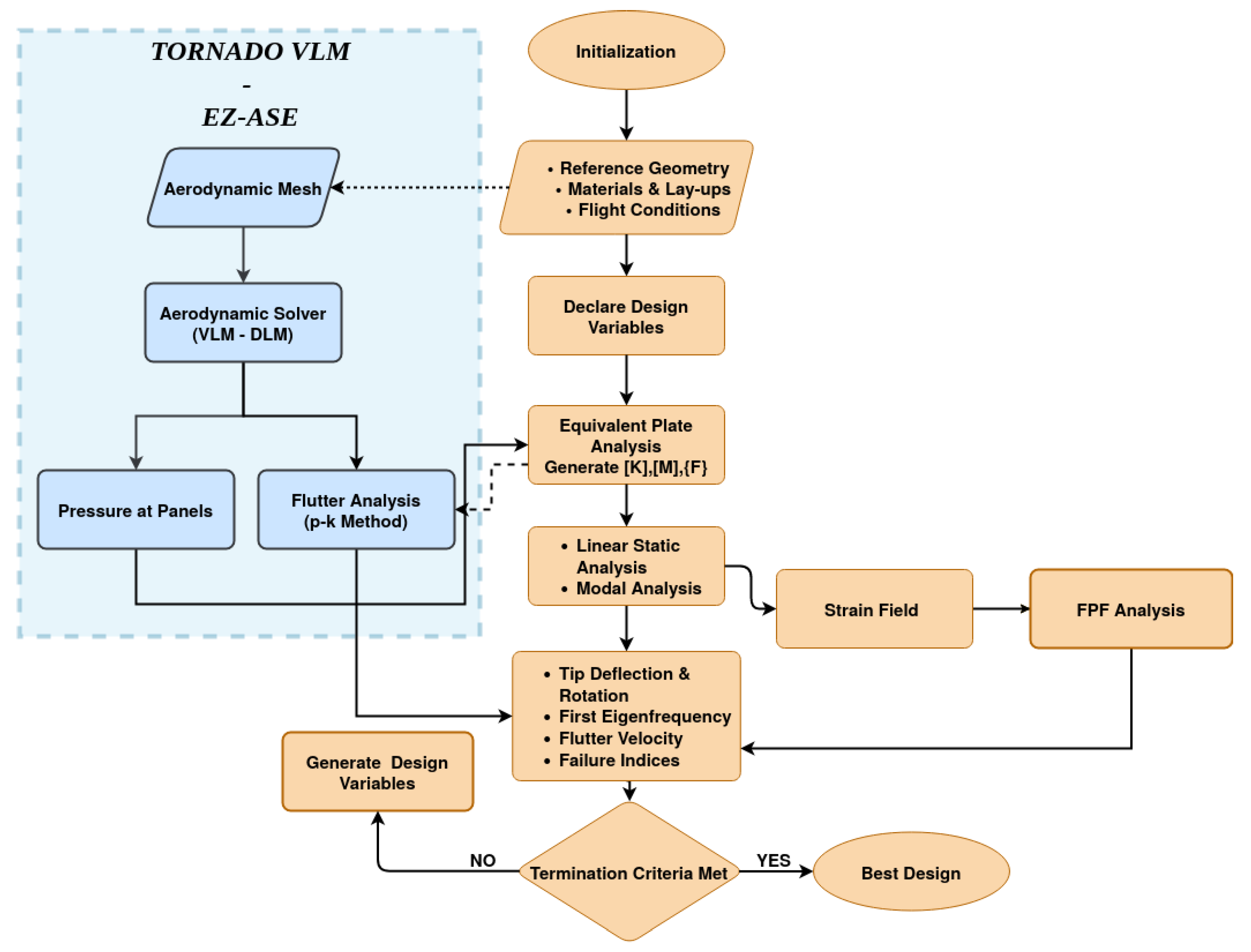
The primary research goal of the present study is to evaluate the gains in the structural optimization of a current state-of-the-art composite airliner wing by implementing a multi-fidelity optimization framework. Structural and aeroelastic constraints are used to formulate the optimization problem. The developed framework and the insight gained in the structural design and optimization of the composite wing model was used in the GRETEL project [
59]. As a case study, a modified, planar (untwisted) version of the Common Research Model (CRM) [
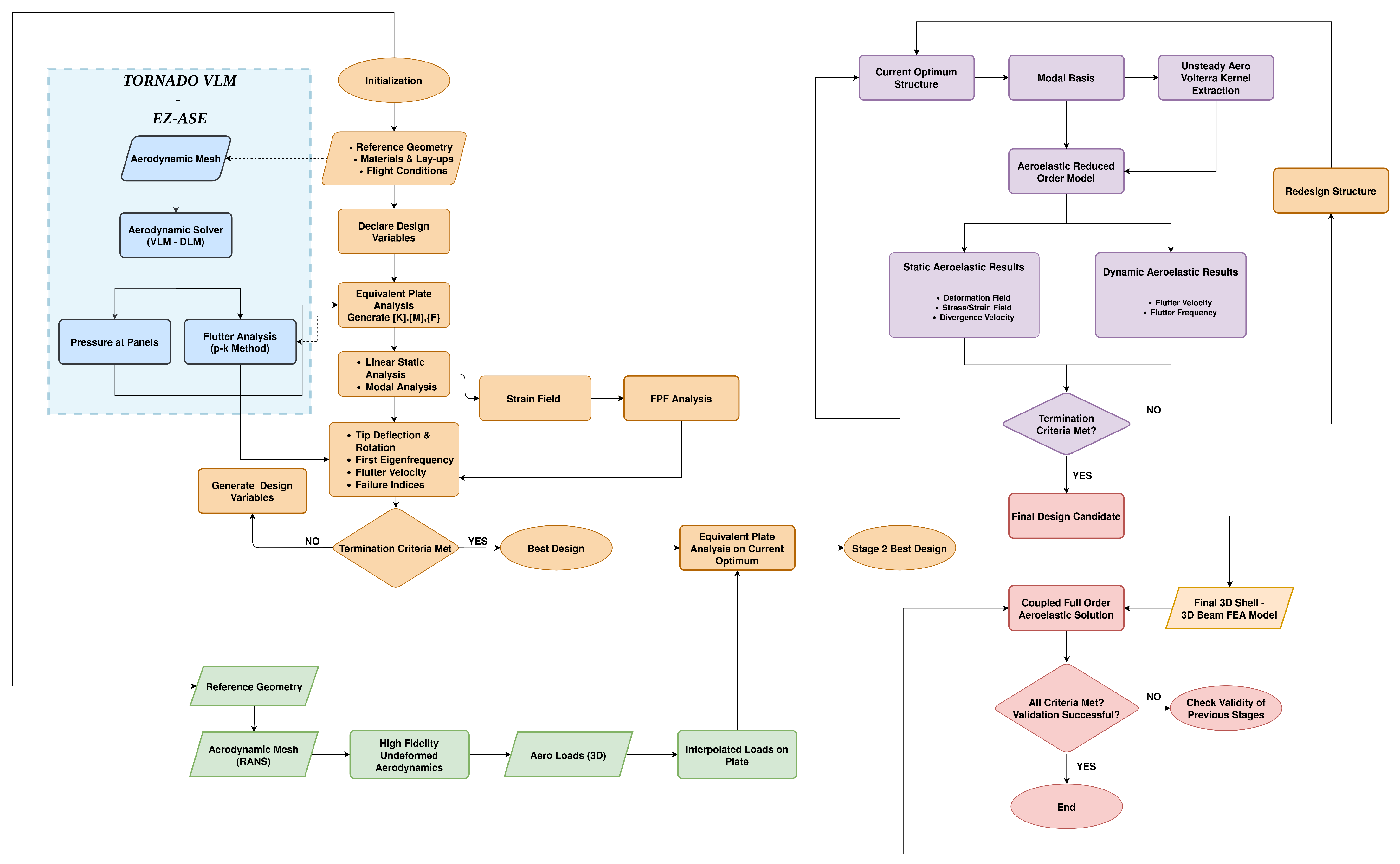
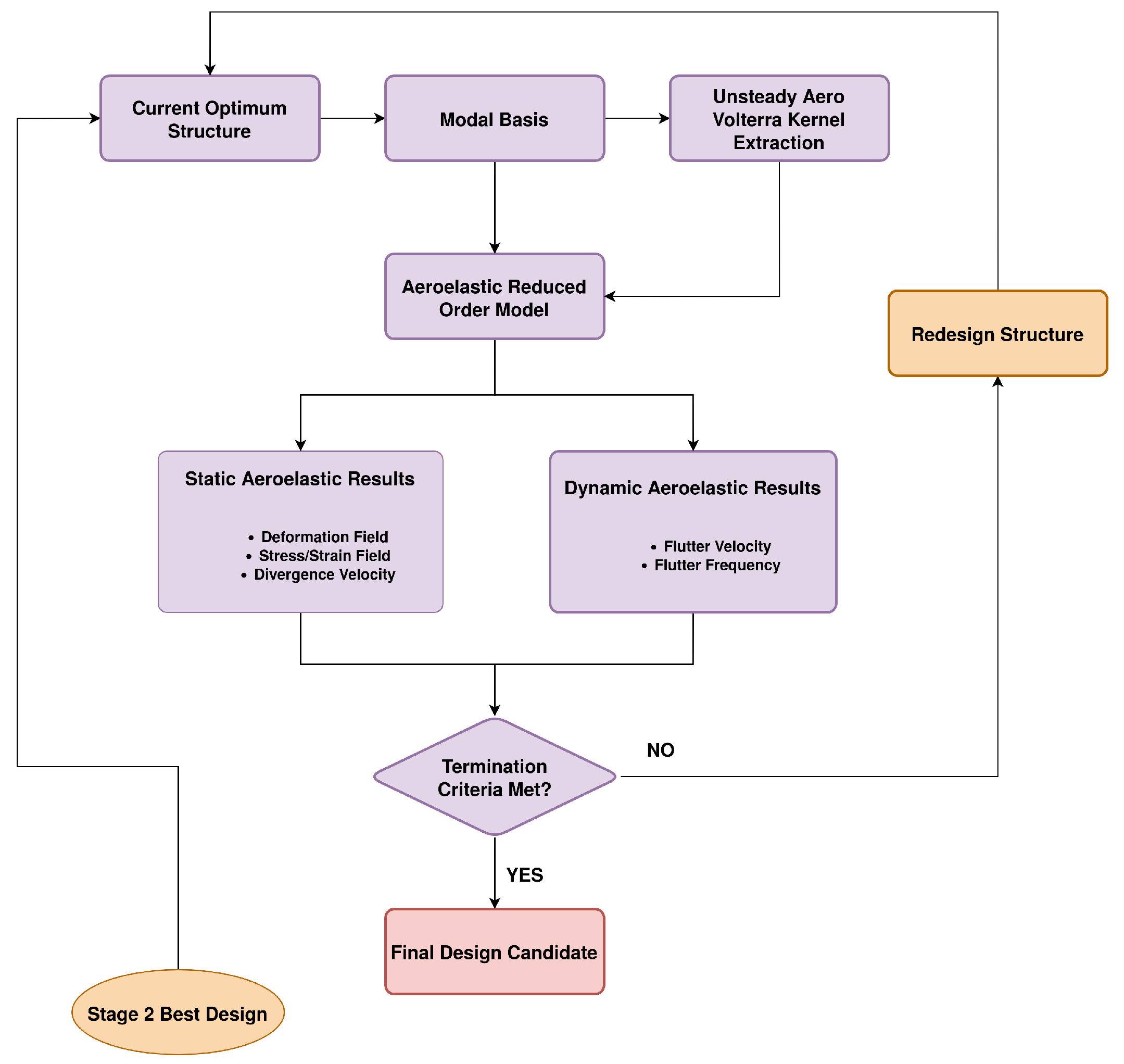
60] wing has been treated. The optimization framework is based on the Equivalent Plate Model (EPM) and the Mixed Integer Ant Colony Optimization (MIDACO) algorithm. At the first stage, an optimized configuration for the wing under low-fidelity aerodynamic loading is obtained. Higher fidelity aerodynamic solutions are then generated, and possible changes in the optimized solution are explored. Finally, the static aeroelastic response of the wing under consideration is also investigated. An overview of the performed actions of the developed optimization framework for the current case study is presented in
Appendix B, highlighting input-output relations.
Although multiple studies in the literature assess the gains of using multi-fidelity optimization frameworks for innovative wing configurations, the gains for the current-state-of-the-art wings are less prominently covered. The present study aims to contribute to closing this gap. We demonstrate that, through the use of this optimization tool, reasonable gains in structural mass of the test case wing were realized.