Estimation and Separation of Longitudinal Dynamic Stability Derivatives with Forced Oscillation Method Using Computational Fluid Dynamics
Abstract
:1. Introduction
2. Methodology
2.1. Moment Calculation
2.2. Forced Simple Harmonic Oscillation
2.2.1. Pitching Mode
2.2.2. Plunging Mode
2.2.3. Flapping Mode
3. Numerical Analysis
3.1. Computational Fluid Dynamics
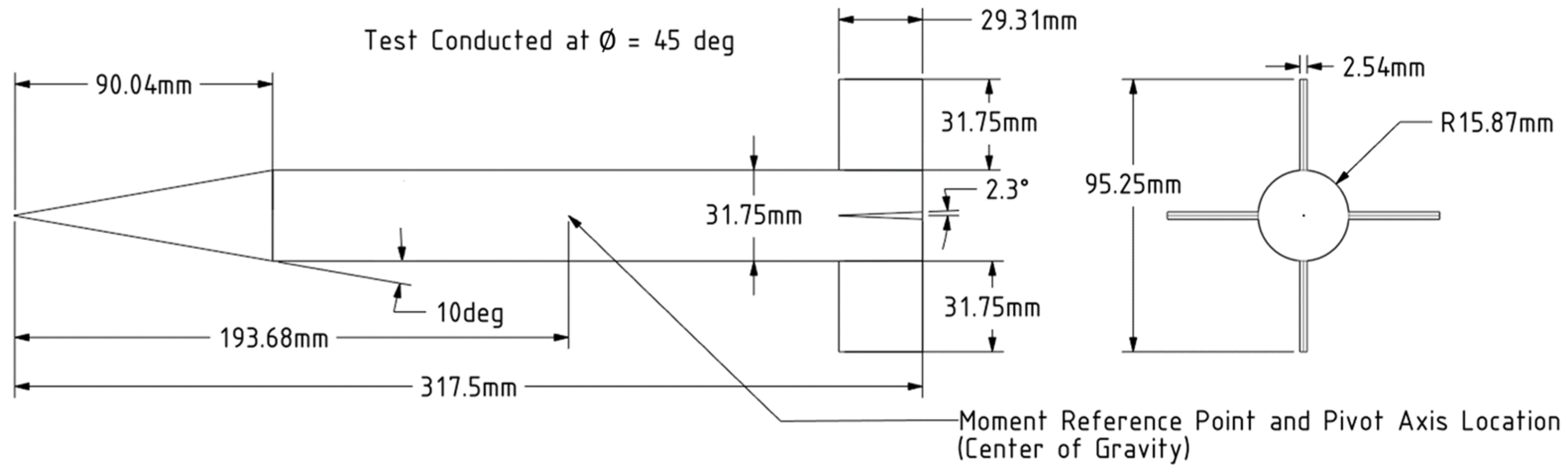
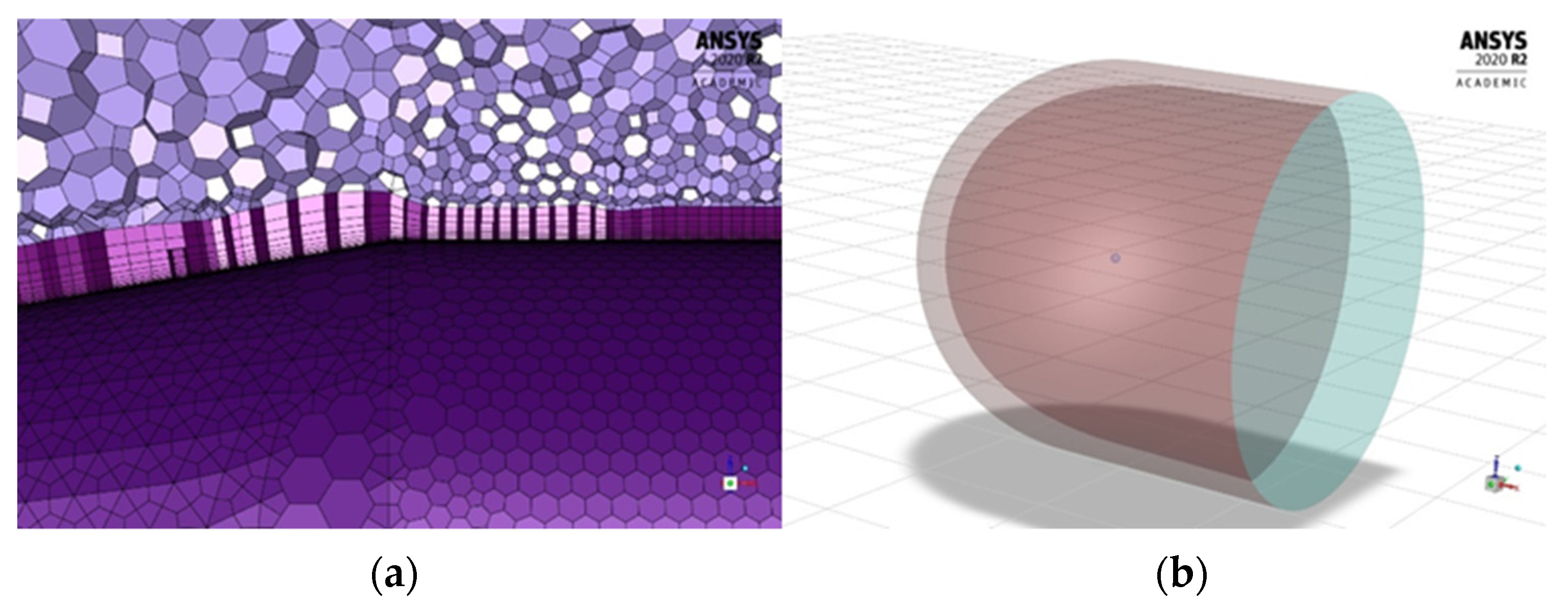
3.1.1. Geometry Configuration
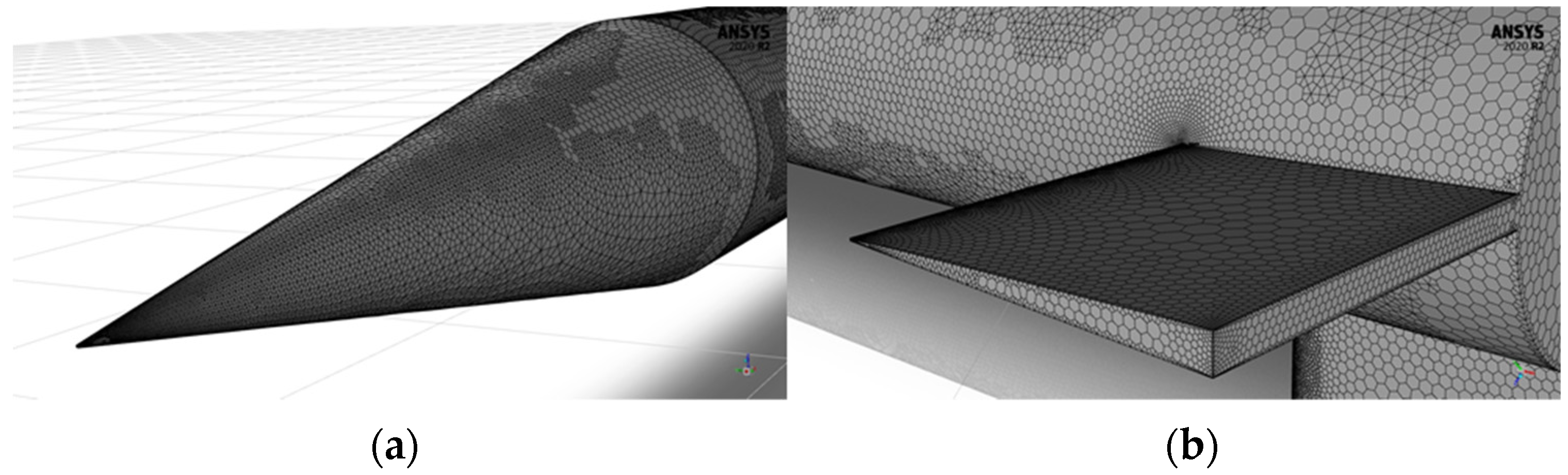
3.1.2. Mesh Configuration
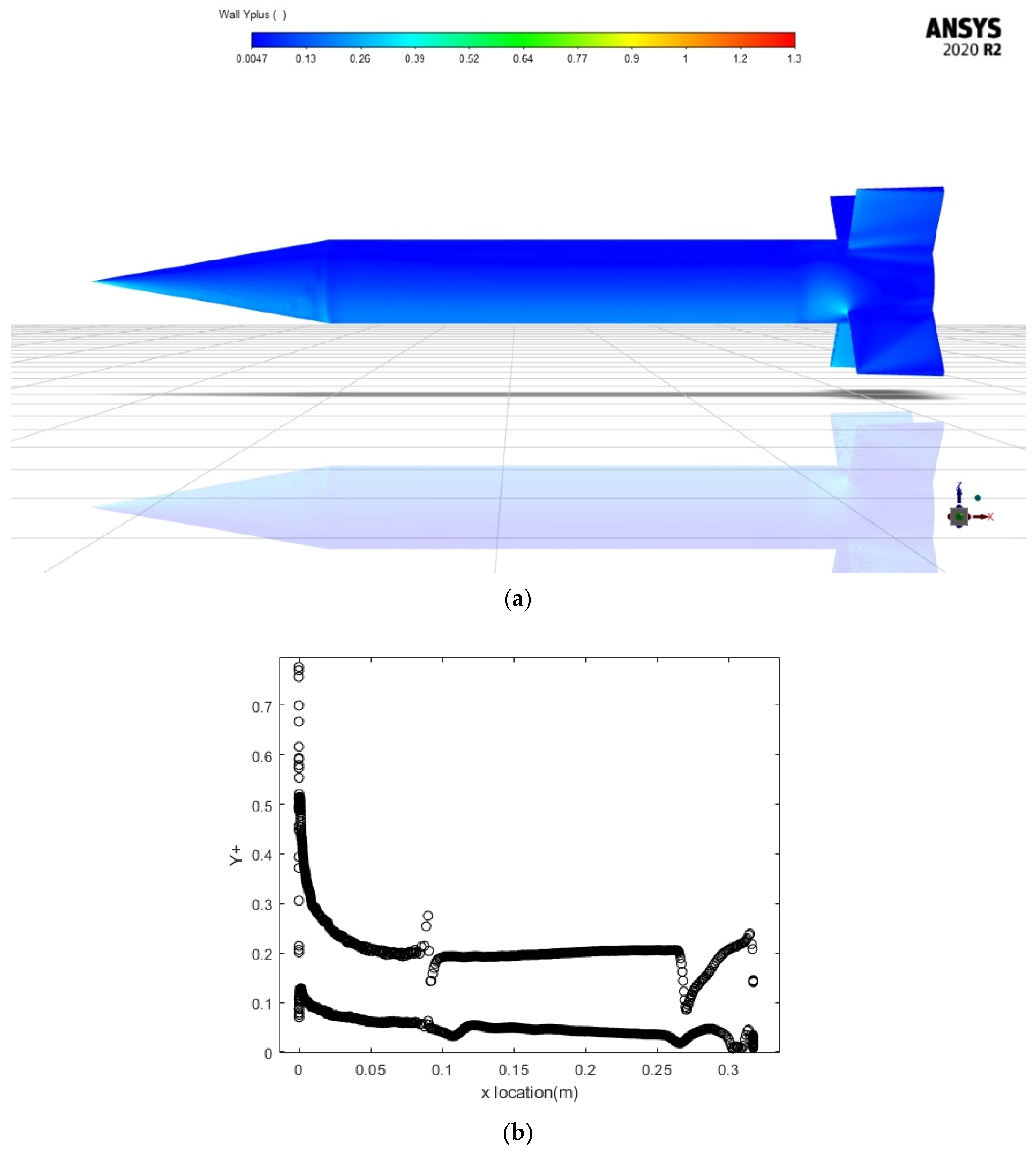
3.1.3. Mesh Convergence Study
3.1.4. Dynamic Mesh
3.2. Solver Setting
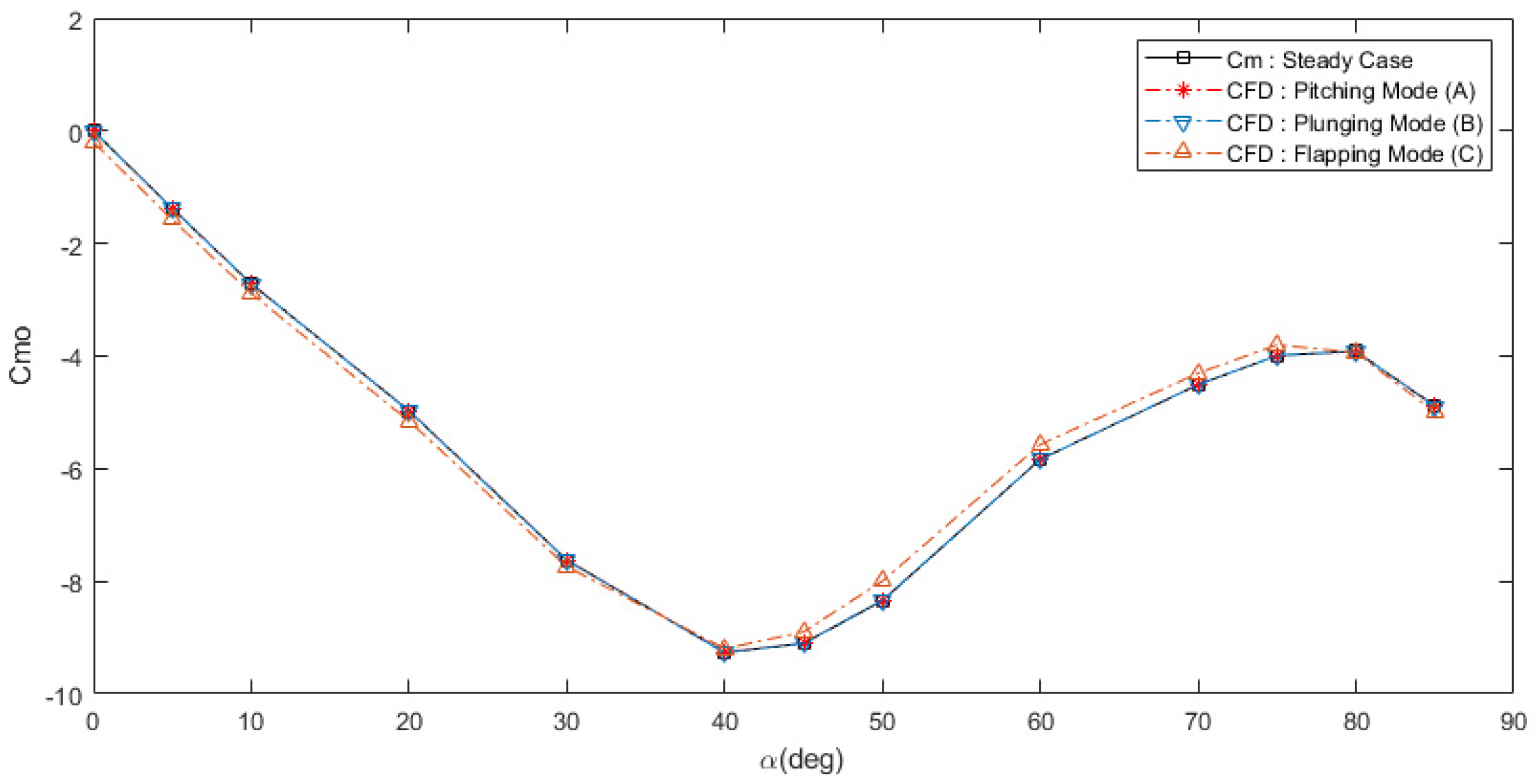
4. Validation and Results
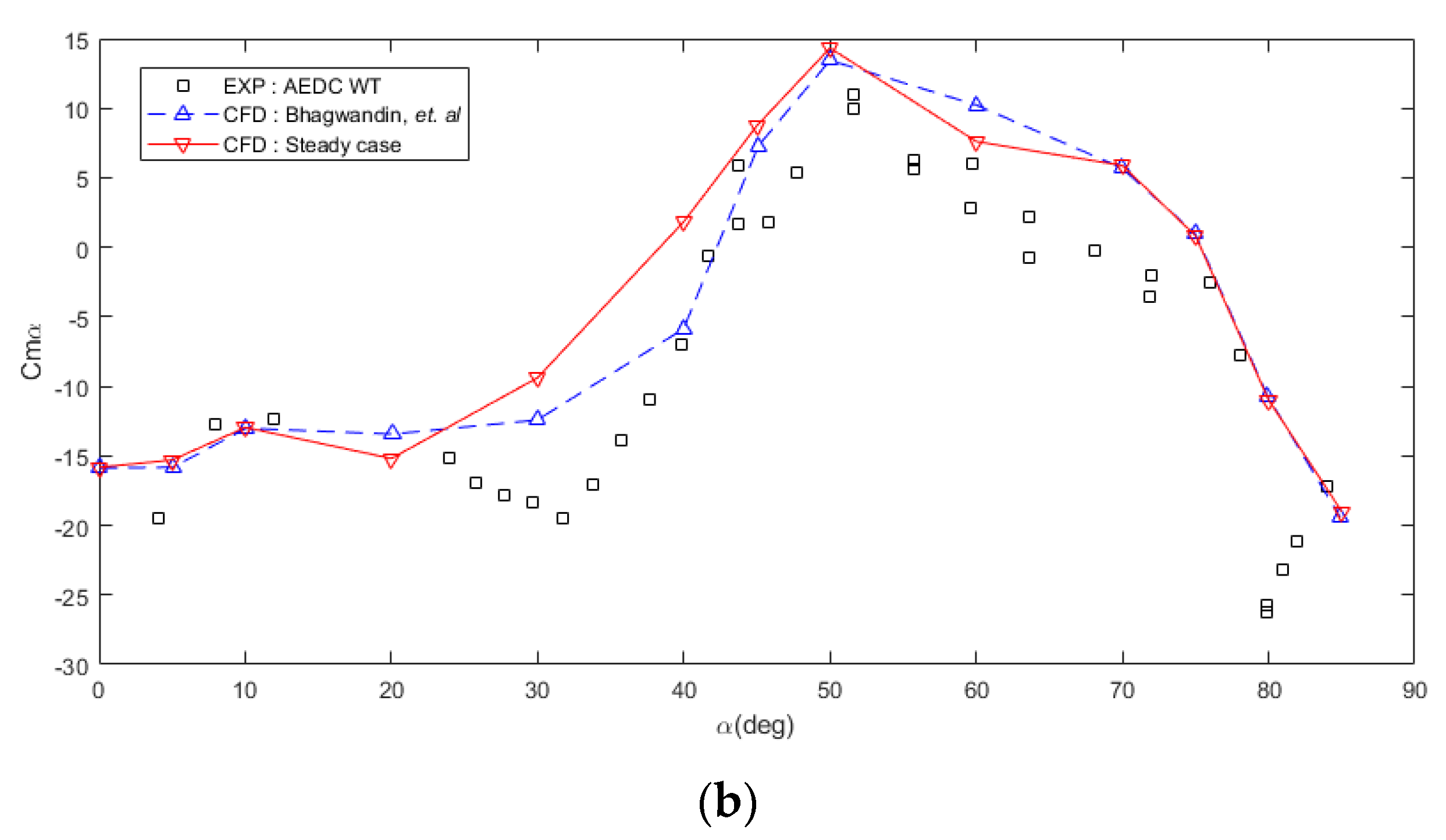
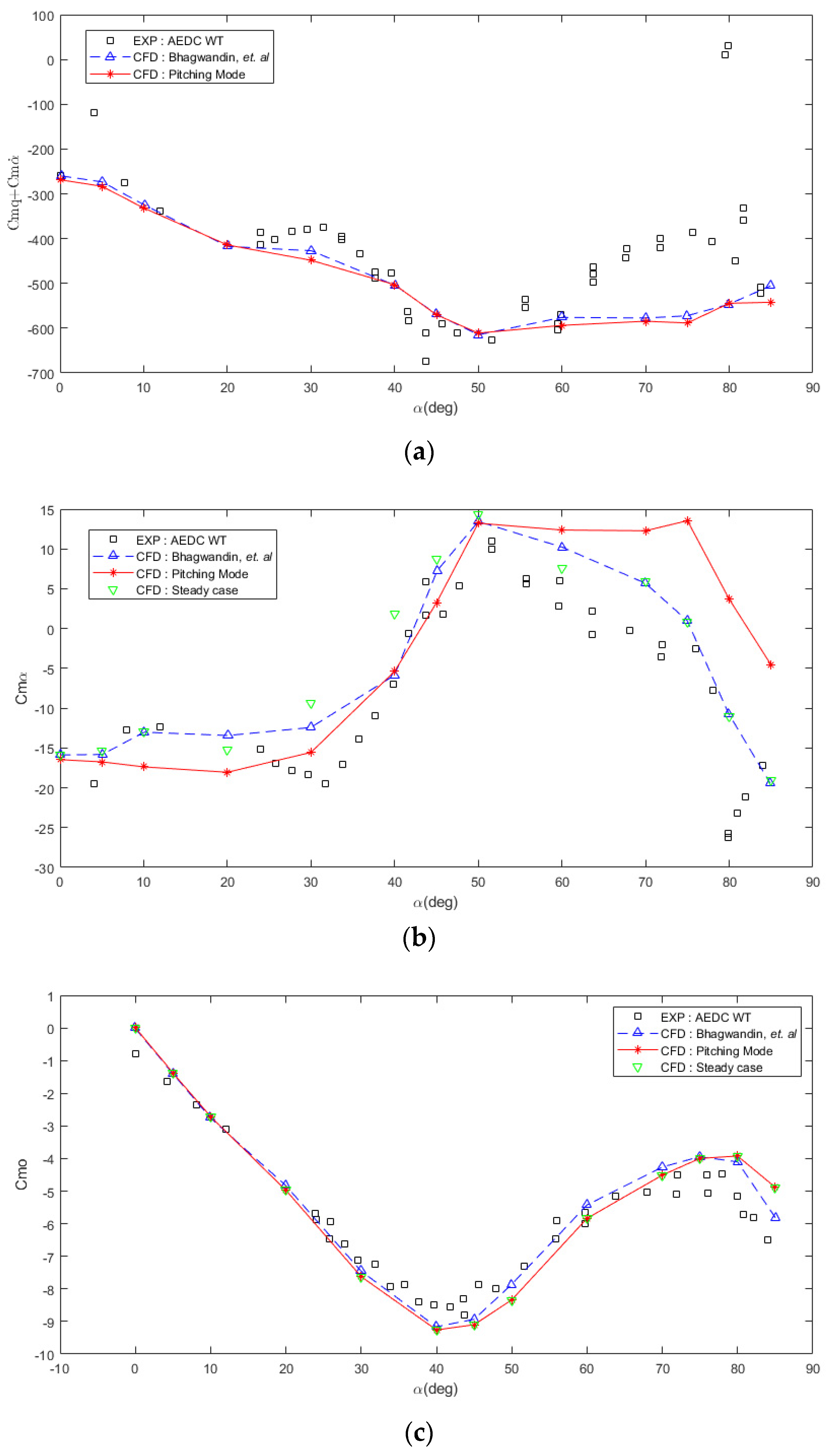
4.1. Computational Fluid Dynamics (CFD) Validation
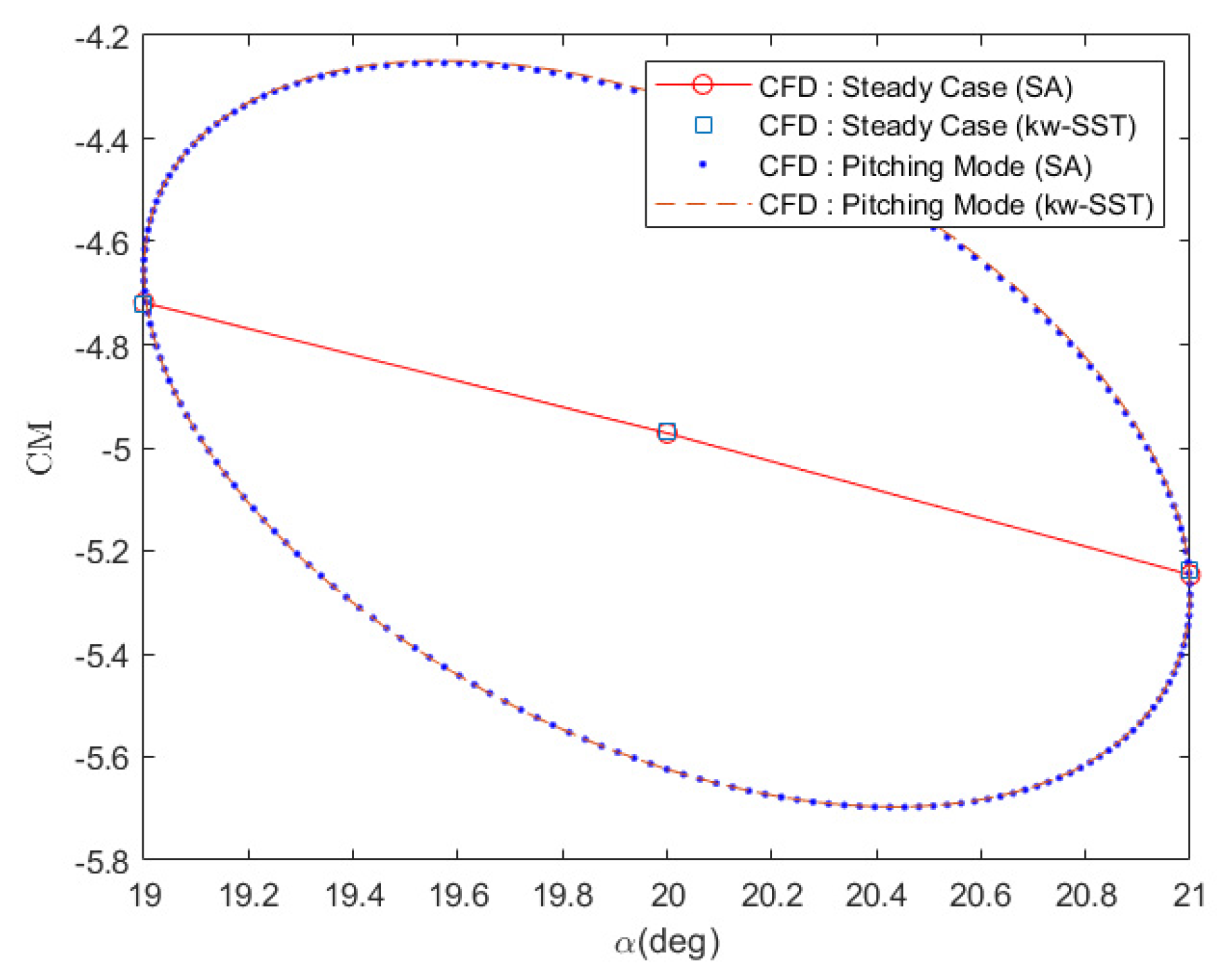
4.1.1. Steady Case Validation
4.1.2. Unsteady Case Data Filtering
4.1.3. Unsteady Case Validation
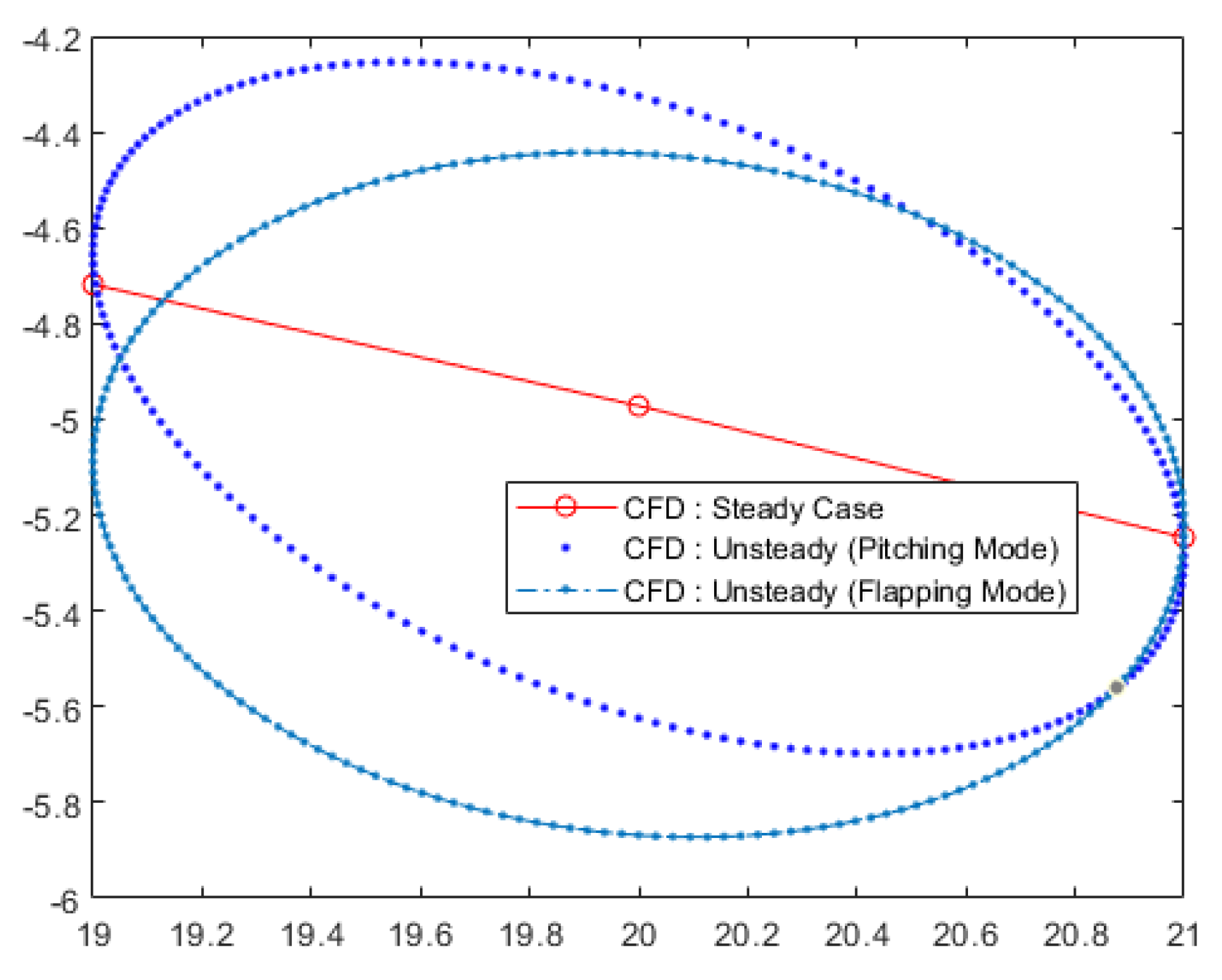
4.1.4. Steady vs. Unsteady Comparison
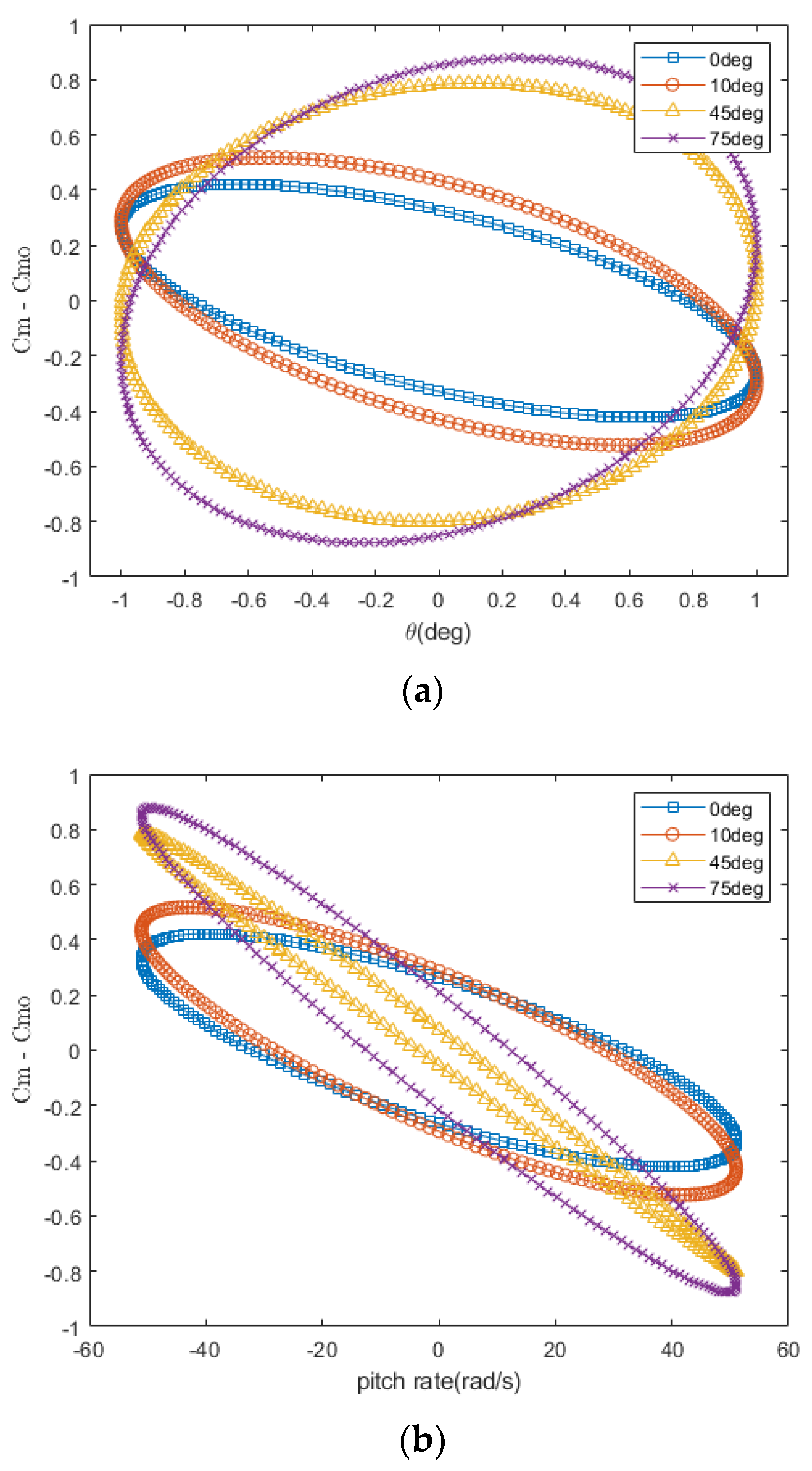
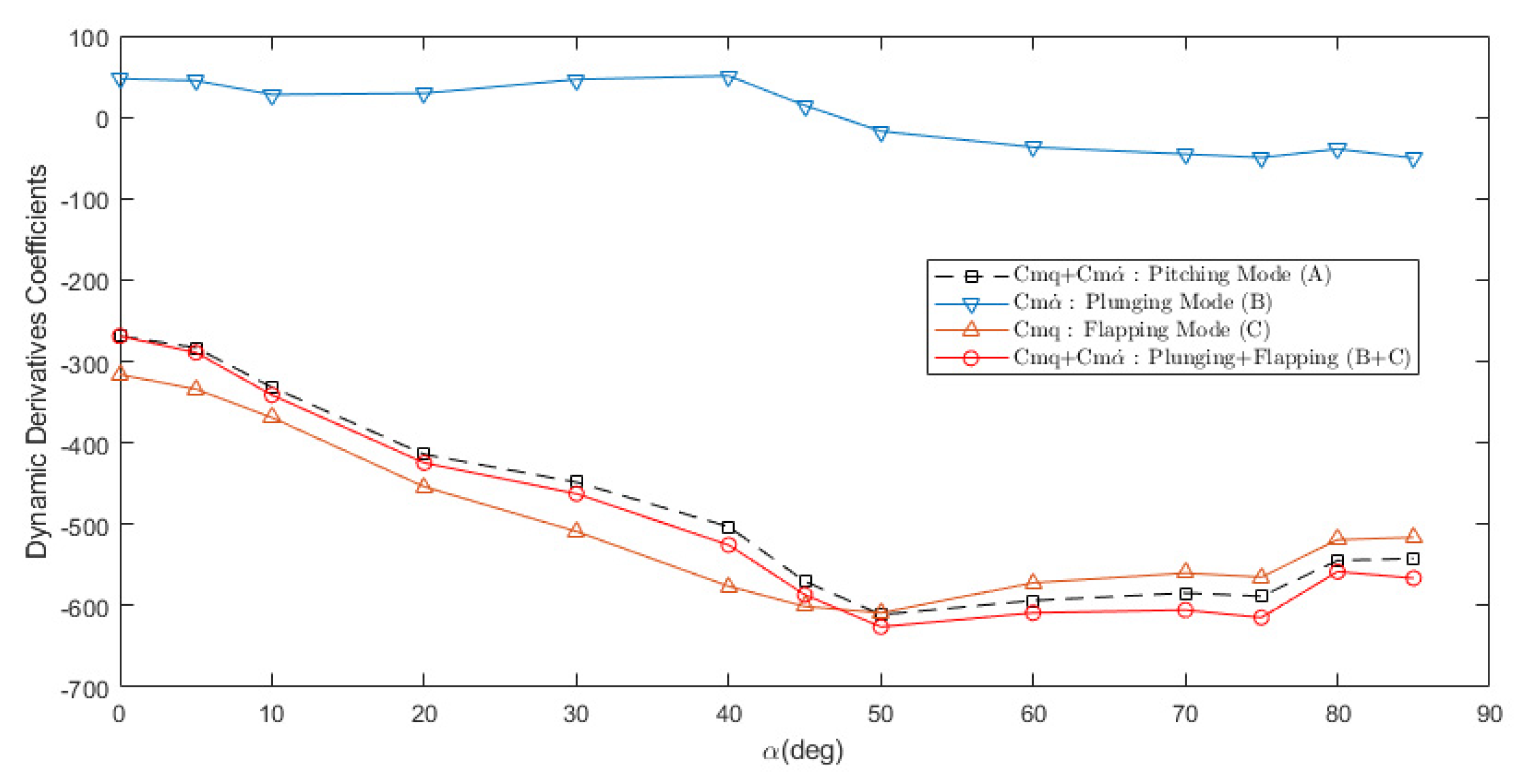
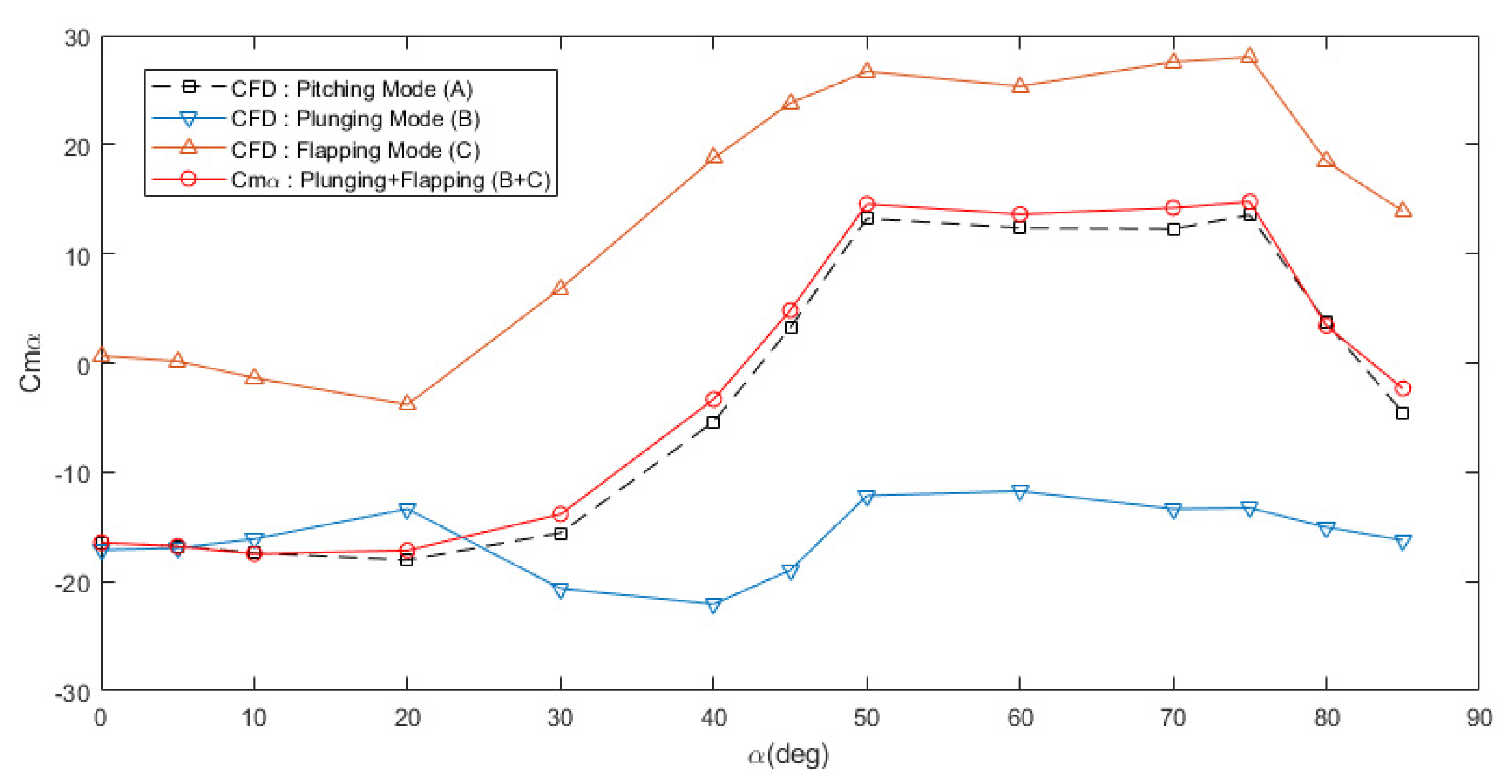
4.2. Coefficient Separation Method
Dynamic Derivative Coefficients
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Pitching moment coefficient | |
| Pitching moment coefficient in steady reference condition | |
| Pitching moment coefficient due to pitch rate | |
| Pitching moment coefficient due to change in angle of attack | |
| pitching moment coefficient due to change rate of the angle of attack | |
| Direct pitching moment coefficient damping derivatives | |
| l | Length reference (m) |
| d | Model diameter (m) |
| f | Dimensional frequency (Hz) |
| T | Period cycle |
| k | Reduced oscillation frequency |
| Pitch rate | |
| Freestream Mach number | |
| Reynolds number based on the model diameter | |
| Total Pressure, Pa | |
| Total Temperature, K | |
| Freestream dynamic pressure, Pa | |
| Freestream velocity, m/s | |
| Mean chord | |
| t | Time(s) |
| Δt | Time-step size(s) |
| N | Number of iteration |
| V | Velocity of the body relative to the fluid |
| Angle of attack (deg) | |
| Angle of attack change rate | |
| Angle of sideslip | |
| Density of the fluid | |
| Inclination angle measured normal to the horizontal plane to aircraft longitudinal axis | |
| Inclination angle oscillation amplitude | |
| Angular rate |
References
- Williams, J.E.; Vukelich, S.R. The USAF Stability and Control Digital Datcom; AFFDL-TR-79-3032; McDonnell Douglas Astronautics Co.: St. Louis, MO, USA, 1979. [Google Scholar]
- Dowell, E.H.; Bland, S.R.; Williams, M.H. Linear/nonlinear behavior in unsteady transonic aerodynamics. AIAA J. 1983, 21, 38–46. [Google Scholar] [CrossRef]
- Nguyen, L.T. Evaluation of Importance of Lateral Acceleration Derivatives in Extraction of Lateral-Directional Derivatives at High Angles of Attack; NASA TN D-7739; NASA: Washington, DC, USA, 1974.
- Davis, M.C.; White, J.T. X-43A Flight-Test-Determined Aerodynamic Force and Moment Characteristics at Mach 7.0. J. Spacecr. Rocket. 2008, 45, 472–484. [Google Scholar] [CrossRef] [Green Version]
- Greenwell, D.I. Frequency Effects on Dynamic Stability Derivatives Obtained from Small-Amplitude Oscillatory Testing. J. Aircr. 1998, 35, 776–783. [Google Scholar] [CrossRef]
- Kramer, B. Experimental evaluation of superposition techniques applied to dynamic aerodynamics. In Proceedings of the 40th AIAA Aerospace Sciences Meeting & Exhibit, Reno, NV, USA, 14–17 January 2002; p. 700. [Google Scholar]
- Da Ronch, A.; Vallespin, D.; Ghoreyshi, M.; Badcock, K.J. Evaluation of Dynamic Derivatives Using Computational Fluid Dynamics. AIAA J. 2012, 50, 470–484. [Google Scholar] [CrossRef]
- Chung, H.; Lee, H.; Lee, M. Estimation of Aircraft Longitudinal Dynamic Derivatives Using Dynamic Mesh Method. Available online: https://www.dbpia.co.kr/journal/publicationDetail?publicationId=PLCT00001276 (accessed on 23 March 2021).
- Lee, H.-R.; Gong, H.-J.; Kim, B.-S.; Lee, S.-S. Prediction of Pitch and Roll Dynamic Derivatives for Flight Vehicle using CFD. J. Korean Soc. Aeronaut. Space Sci. 2012, 40, 395–404. [Google Scholar] [CrossRef]
- Bhagwandin, V.; Sahu, J. Numerical Prediction of Pitch Damping Derivatives for a Finned Projectile at Angles of Attack. In Proceedings of the 50th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, American Institute of Aeronautics and Astronautics, Nashville, TN, USA, 9–12 January 2012; p. 691. [Google Scholar]
- Liu, X.; Liu, W.; Zhao, Y. Navier–Stokes predictions of dynamic stability derivatives for air-breathing hypersonic vehicle. Acta Astronaut. 2016, 118, 262–285. [Google Scholar] [CrossRef]
- Oktay, E.; Akay, H. CFD predictions of dynamic derivatives for missiles. In Proceedings of the 40th AIAA Aerospace Sciences Meeting & Exhibi, Reno, NV, USA, 14–17 January 2002; p. 276. [Google Scholar]
- Baigang, M.; Hao, Z.; Ban, W. Computational Investigation of simulation on the dynamic derivatives of flight vehicle. In Proceedings of the 29th Congress of the International Council of the Aeronautical Sciences, St. Petersburg, Russia, 7−12 September 2014; pp. 1–7. [Google Scholar]
- Sahu, J. Numerical Computations of Dynamic Derivatives of a Finned Projectile Using a Time-Accurate CFD Method. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference and Exhibit, Hilton Head, SC, USA, 20 August 2007; 2007; p. 6581. [Google Scholar]
- Bryan, G.H. Stability in Aviation: An Introduction to Dynamical Stability as Applied to the Motions of Aeroplanes; Macmillan and Company, Limited: London, UK, 1911. [Google Scholar]
- Theodorsen, T.; Garrick, I.E.; U.S.N.A.C. for Aeronautics. General Potential Theory of Arbitrary Wing Sections; US Government Printing Office: New York, NY, USA, 1933.
- Theodorsen, T.; Mutchler, W.H. General Theory of Aerodynamic Instability and the Mechanism of Flutter; Rept. 496; NACA: Washington, DC, USA, 1935. [Google Scholar]
- Alemdaroglu, N.; Iyigun, I.; Altun, M.; Uysal, H.; Quagliotti, F.; Guglieri, G. Determination of dynamic stability derivatives using forced oscillation technique. In Proceedings of the 40th AIAA Aerospace Sciences Meeting & Exhibit, Reno, NV, USA, 14–17 January 2002; p. 528. [Google Scholar]
- Guglieri, G.; Quagliotti, F.B. Dynamic stability derivatives evaluation in a low-speed wind tunnel. J. Aircr. 1993, 30, 421–423. [Google Scholar] [CrossRef]
- Hanff, E.S.; Orlik-Ruckemann, K.J. Wind-Tunnel Measurement of Dynamic Cross-Coupling Derivatives. J. Aircr. 1978, 15, 40–46. [Google Scholar] [CrossRef]
- Newman, D.M. A Technique for Measurement of Static and Dynamic Longitudinal Aerodynamic Derivatives Using the DSTO Water Tunnel; DSTO Defence Science and Technology Organisation: Victoria, Australia, 2011. [Google Scholar]
- Erm, L. An Experimental Investigation into the Feasibility of Measuring Static and Dynamic Aerodynamic Derivatives in a Water Tunnel. In Proceedings of the 30th AIAA Applied Aerodynamics Conference, New Orleans, LA, USA, 25–28 June 2012; p. 3119. [Google Scholar]
- Moelyadi, M.A.; Sachs, G. CFD based determination of dynamic stability derivatives in yaw for a bird. J. Bionic Eng. 2007, 4, 201–208. [Google Scholar] [CrossRef]
- Chung, H.-S.; Yang, K.-J.; Kwon, K.-B.; Lee, H.-K.; Kim, S.-T.; Lee, M.-S.; Reu, T. Estimation of Longitudinal Dynamic Stability Derivatives for a Tailless Aircraft Using Dynamic Mesh Method. J. Korean Soc. Aeronaut. Space Sci. 2015, 43, 232–242. [Google Scholar] [CrossRef]
- Mi, B.; Zhan, H. Review of Numerical Simulations on Aircraft Dynamic Stability Derivatives. Arch. Comput. Methods Eng. 2019, 27, 1515–1544. [Google Scholar] [CrossRef]
- Dupuis, A.D.; Hathaway, W. Aeroballistic Range Tests of the Basic Finner Reference Projectile at Supersonic Velocities; DREV-TM-9703; Defence Research Establishment: Valcartier, QC, Canada, 1997.
- Uselton, B.L.; Uselton, J.C. Test Mechanism for Measuring Pitch-Damping Derivatives of Missile Configurations at High Angles of Attack; AEDC-TR-75-43; Arnold Engineering Development Center Arnold AFB: Hillsboro, TN, USA, 1975. [Google Scholar]
- ANSYS Inc. 2009. Available online: https://www.afs.enea.it/project/neptunius/docs/fluent/html/udf/node4.htm (accessed on 23 March 2021).
- Ansys Inc. ANSYS Fluent. Available online: https://www.ansys.com/products/fluids/ansys-fluent (accessed on 21 January 2020).
- Araya, G. Turbulence Model Assessment in Compressible Flows around Complex Geometries with Unstructured Grids. Fluids 2019, 4, 81. [Google Scholar] [CrossRef] [Green Version]
- Schütte, A.; Marini, R.N. Computational Aerodynamic Sensitivity Studies for Generic Delta Wing Planforms. In New Results in Numerical and Experimental Fluid Mechanics XII. DGLR 2018. Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Dillmann, A., Heller, G., Krämer, E., Wagner, C., Tropea, C., Jakirlić, S., Eds.; Springer: Cham, Switzerland, 2020; Volume 142. [Google Scholar] [CrossRef] [Green Version]
- DeSpirito, J.; Silton, S.; Weinacht, P. Navier-Stokes predictions of dynamic stability derivatives: Evaluation of steady-state methods. J. Spacecr. Rocket. 2009, 46, 1142–1154. [Google Scholar] [CrossRef] [Green Version]
- Weinacht, P. Characterization of Small-Caliber Ammunition Performance Using a Virtual Wind Tunnel Approach. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference and Exhibit, Hilton Head, SC, USA, 20–23 August 2007; p. 6579. [Google Scholar]
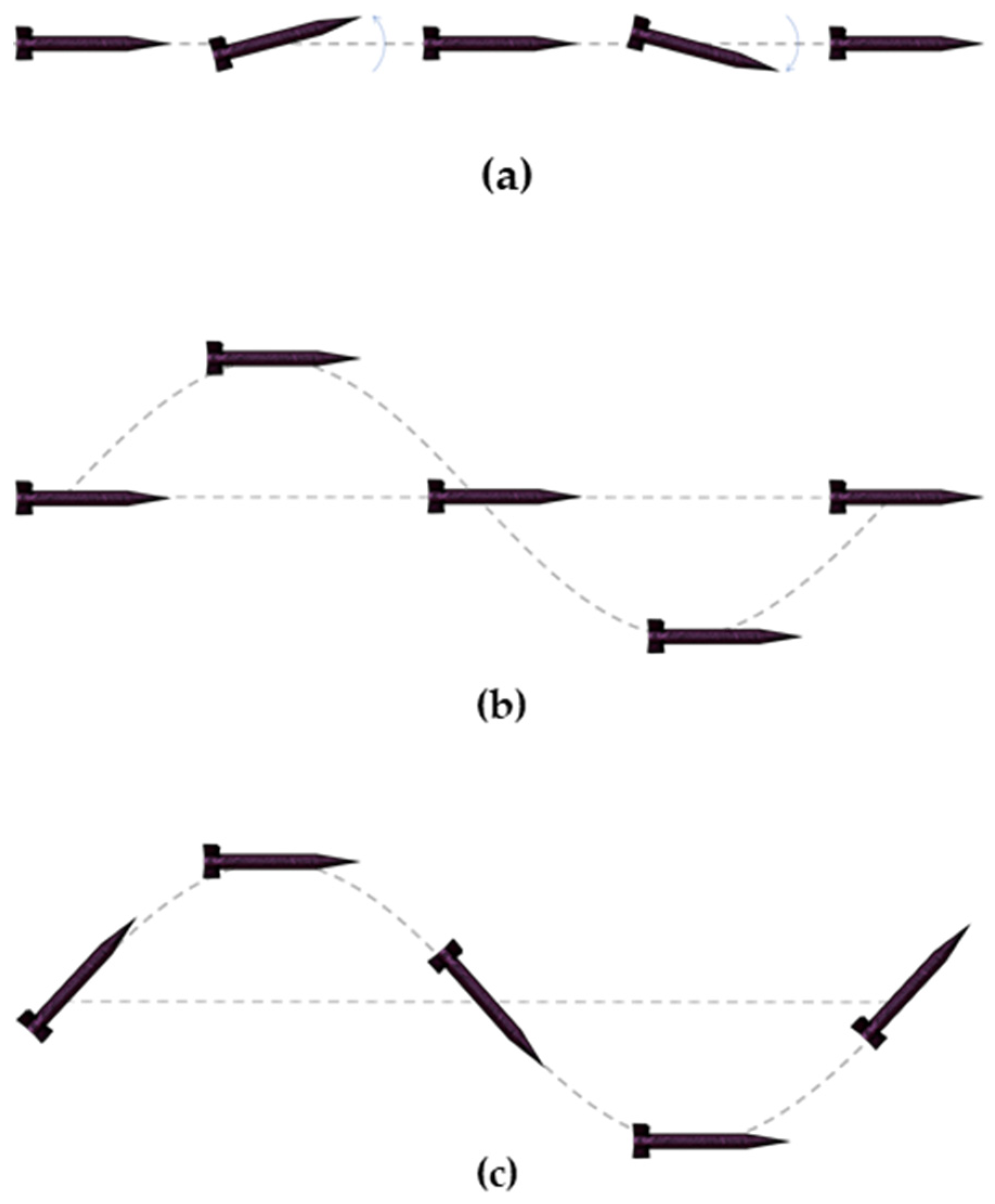






| Mode | Pitching | Plunging | Flapping |
|---|---|---|---|
| y-axis rotation (t) | - | * | |
| z-axis translation (t) | - | ||
| 0 |
| (Pa) | (K) | (Pa) | (m/s) | ||
|---|---|---|---|---|---|
| 0.086 × 106 | 1.96 | 22,753 | 306.11 | 8342.7 | 516.97 |
| α | (B) | (C) | Total (B + C) | (A) | Error |
|---|---|---|---|---|---|
| 0 | 47.231 | −316.149 | −268.918 | −268.700 | −0.08% |
| 10 | 27.708 | −369.019 | −341.311 | −331.720 | −2.89% |
| 45 | 14.321 | −600.932 | −586.611 | −570.105 | −2.90% |
| 75 | −49.649 | −564.955 | −614.604 | −588.397 | −4.45% |
| α | (B) | (C) | Total (B + C) | (A) | Error |
|---|---|---|---|---|---|
| 0 | −17.099 | 0.655 | −16.444 | −16.465 | 0.13% |
| 10 | −16.115 | −1.356 | −17.470 | −17.375 | −0.55% |
| 45 | −18.965 | 23.789 | 4.824 | 3.234 | −49.18% |
| 75 | −13.259 | 28.019 | 14.760 | 13.550 | −8.93% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Juliawan, N.; Chung, H.-S.; Lee, J.-W.; Kim, S. Estimation and Separation of Longitudinal Dynamic Stability Derivatives with Forced Oscillation Method Using Computational Fluid Dynamics. Aerospace 2021, 8, 354. https://doi.org/10.3390/aerospace8110354
Juliawan N, Chung H-S, Lee J-W, Kim S. Estimation and Separation of Longitudinal Dynamic Stability Derivatives with Forced Oscillation Method Using Computational Fluid Dynamics. Aerospace. 2021; 8(11):354. https://doi.org/10.3390/aerospace8110354
Chicago/Turabian StyleJuliawan, Nadhie, Hyoung-Seog Chung, Jae-Woo Lee, and Sangho Kim. 2021. "Estimation and Separation of Longitudinal Dynamic Stability Derivatives with Forced Oscillation Method Using Computational Fluid Dynamics" Aerospace 8, no. 11: 354. https://doi.org/10.3390/aerospace8110354
APA StyleJuliawan, N., Chung, H.-S., Lee, J.-W., & Kim, S. (2021). Estimation and Separation of Longitudinal Dynamic Stability Derivatives with Forced Oscillation Method Using Computational Fluid Dynamics. Aerospace, 8(11), 354. https://doi.org/10.3390/aerospace8110354







