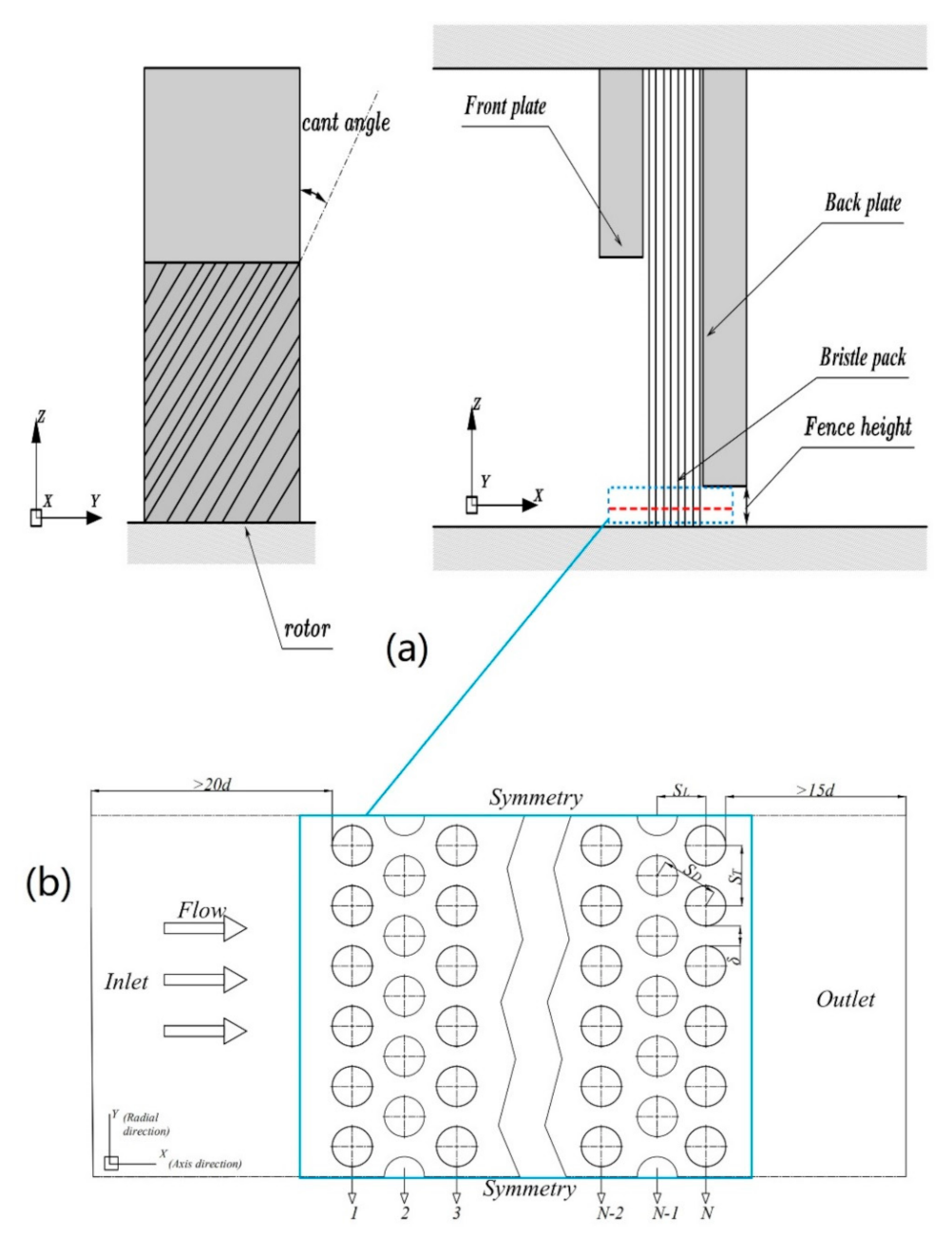
4.2. State of Working Fluid in the Model
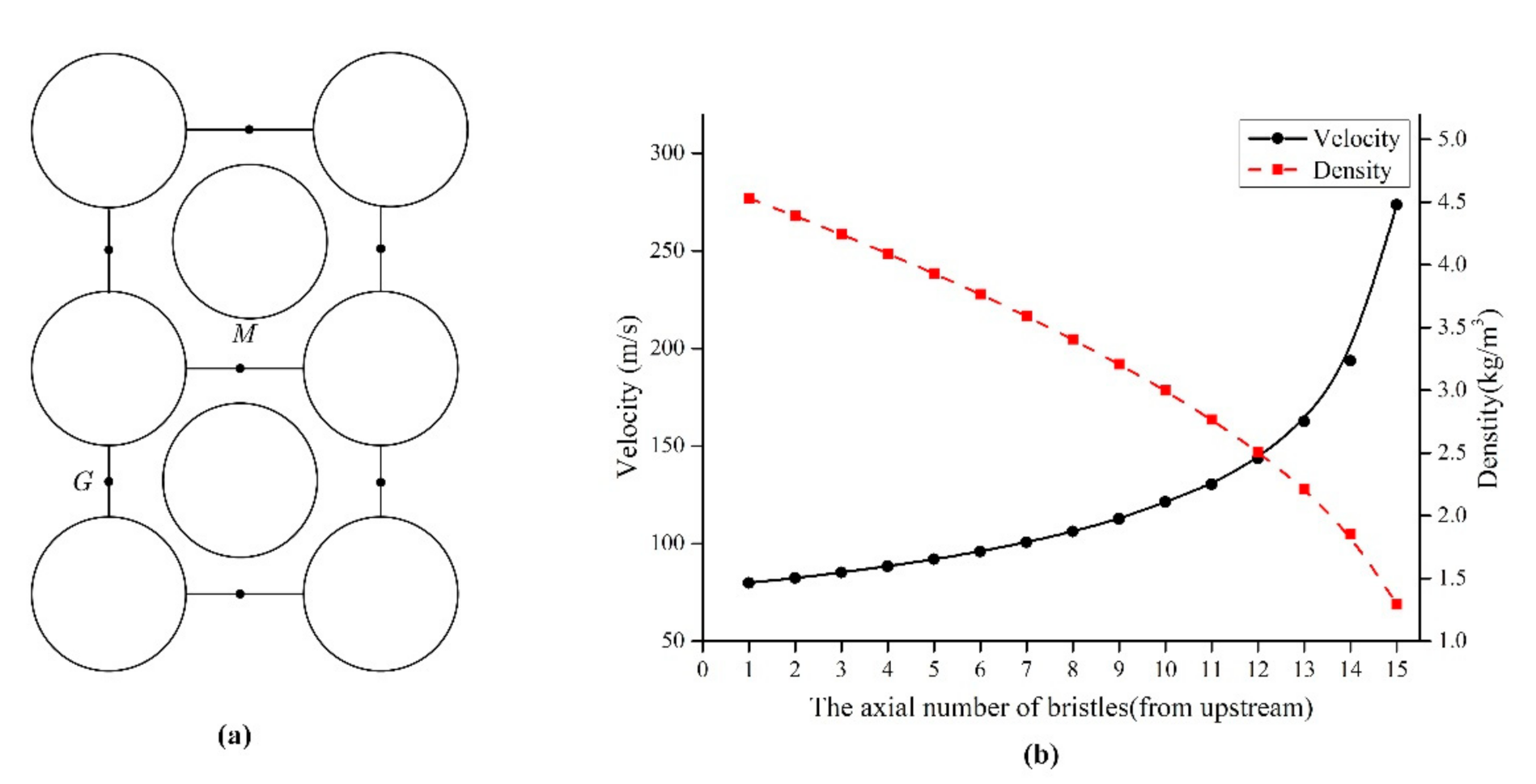
To study the velocity distribution and the density distribution of the gap, this paper extracts the simulation data of the midpoint of the gap centerline, as shown in points
G and
M in
Figure 4a, and the results of the velocity change and density change in the bristle gap are shown in
Figure 4b. The growth rate of velocity increases when the growth rate of density decreases. This change is described as index movement, which means that the flow is accelerated and expanded. According to conservation of mass, these finds can explain the reason for the accelerative flow which is found in Huang’s and Kang’s works [
8,
13]. Meanwhile, according to the polytropic process [
21], the relationship between
ρ and
P satisfies the following equation:
where
n is the polytropic exponent, and
c is the constant. To investigate the flow in the bristle pack, according to Equation (9), the case in which
SD/d = 1.10 and Δ
P = 0.3 MPa, the results from the numerical simulation can be fitted as follows:
P = 0.0788 ×
ρ1.057 (show in
Figure 5).
This result shows that the value of
n is greater than 1 but is less than the specific heat ratio (κ) of air of 1.402 [
21], and it indicates that the state variation is between the isothermal process and the isentropic process, as shown in
Table 3. However, the value is close to 1 as
δ decreases, and when the value is equal to 1, the state variation becomes an isothermal process. The cause may be the flow path narrow and the fluid resistance increases.
4.3. Dynamic Pressure of the Bristle Pack
According to Equation (10), the density and velocity of gas also determine the value of dynamic pressure (
D), as follows:
The dynamic pressure is an important physical quantity, since dimensionless parameterizations in the field of fluid mechanics are carried out by it, such as the pressure, drag, and lift of the cylinder surface. Furthermore, in the heat exchanger field,
Eu, represents the energy loss of fluid, which can also be obtained from the dynamic pressure. The dynamic pressure may also cause vibration. For brush seals, the chatter of bristles may cause the fatigue failure of the welding spot of the bristle root. For example, Liu [
11] studied the slippage of the upstream bristles with the dynamic head of the inlet swirl and found that the upstream bristles slipped when the dynamic head was bigger than 0.15 MPa. Since in this study the flow is compressible in the bristle pack, which is why the change of dynamic pressure is different from that of the heat exchangers.
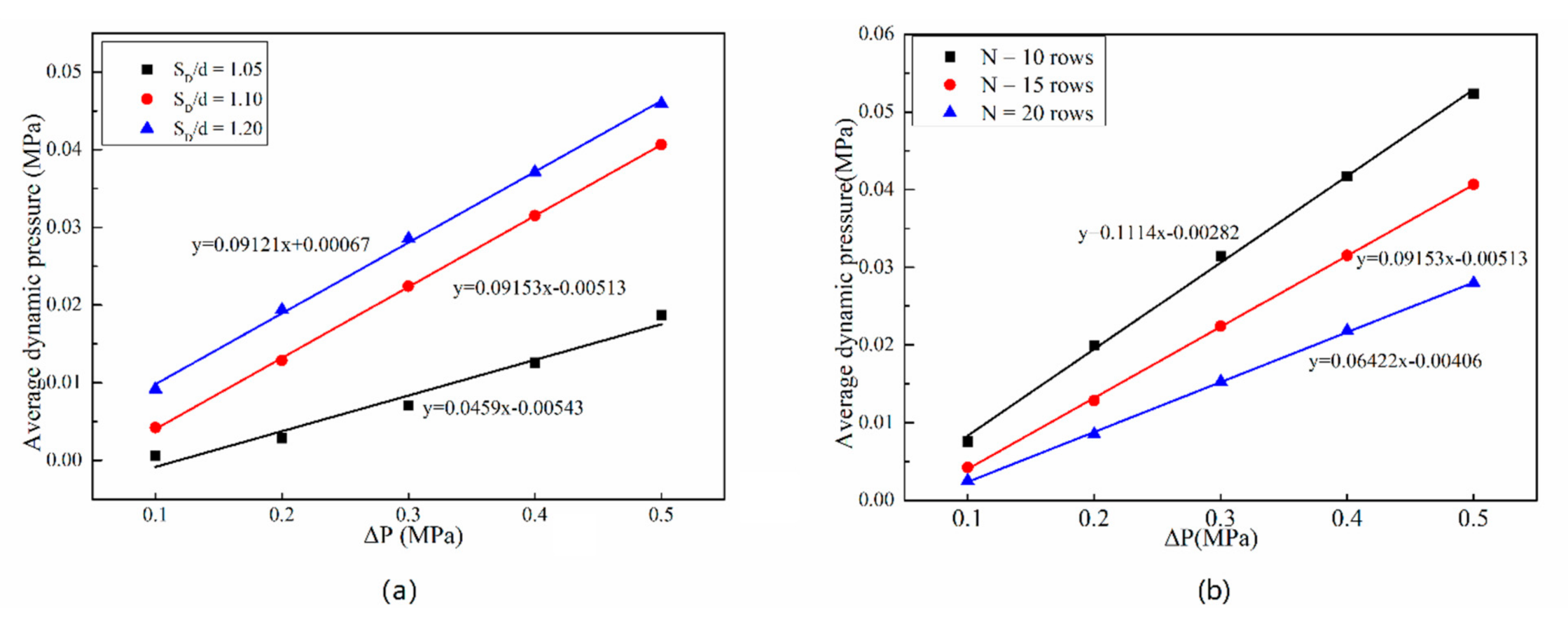
Figure 6a shows the dynamic pressure distribution of the bristle gap. The results show that the dynamic pressure increases as the row number increases. The differential pressure has a significant impact on dynamic pressure. As the Δ
P increases, the dynamic pressure increases in every row, and the growth rate increases obviously in the downstream, indicating high kinetic energy and the strong impact of fluid on the bristles. As shown in
Figure 6b, when the static pressure decreases, the dynamic pressure increases. This finding shows that the more pressure energy transforms into kinetic energy. Therefore, the mass flow rate will increase if the kinetic energy becomes larger.
The spacing of the bristles and the number of rows of the bristles are important parameters for designing brush seals [
22,
23]. Three different spacing and three different numbers of tube rows are used to investigate the impact of these parameters. As shown in
Figure 7a,
δ has a significant impact on the dynamic pressure, and the dynamic pressure increases as the Δ
P increases. Meanwhile, decreasing
δ can reduce the dynamic pressure, that is, a small
δ can effectively restrain the pressure energy transformation into kinetic energy. Curve fitting results show that the slope is 0.0459 when
SD/d = 1.05, which is smaller than that of with other gaps. Therefore, under
SD/d = 1.05, the fluid is blocked well.
The dynamic pressure distribution with different rows of bristles is shown in
Figure 7b. The growth rate with 20 rows is 0.06422, which is lower than that of 10 and 15 rows, thereby indicating that the increase in bristle rows can suppress the increase in dynamic pressure. As the axial number of bristles increases, the dynamic pressure decreases, suggesting that the mass flow rate will decrease.
4.4. Euler Number of Bristle Pack
In fluid mechanics, the Euler number expresses the relationship between the loss of pressure and dynamic pressure, and it reflects the magnitude of the loss of momentum during the flow process. Kang [
8] and Ma [
24] introduce the Euler number for the evaluation of the seal performance in their studies, but they ignore the change of the density term. In their studies, the calculation formula of Euler number is as follows:
where
Eu is the Euler number, Δ
P is the differential pressure between the upstream and downstream,
N is the number of rows in the X-direction, and
Vu and
Vexit are the velocities in the upstream and the gap of end row, respectively. As discussed in the previous section, the compressibility of the fluid can influence the dynamic pressure. Therefore, the equation needs to be amended to take this impact into account:
where
Du and
Pu are the dynamic pressure and the static pressure in the upstream, respectively; and
Dexit and
Pexit are the dynamic pressure and the static pressure in the gap of the end row, respectively.
For the tube bank model with different gaps, the area of the outlet is different, thereby potentially influencing the magnitude of the mass flux. Therefore, the mass flow rate can be compared using Equation (13) [
25]:
The parameters are given in Lee [
6].
NBcrf is the number of bristles per circumferential millimeter.
α is the cant angle of bristle (
Figure 1),
α = 45°;
FH is the fence height (
Figure 1),
FH = 1.075 mm;
dr is the rotor diameter,
dr = 180.05 mm;
Q is the total leakage flow rate of the seal;
m is the predicted flow rate of the tube bank model.
In accordance with Equation (12),
Eu is studied in a bristle pack with different gaps, and the results are shown in
Figure 8. In general,
Eu decreases as the pressure difference increases. The average value of
Eu for
SD/d = 1.05, 1.10 and 1.20 is 2.594, 0.562 and 0.373. Therefore, when
SD/d = 1.05,
Eu is 4.62 times larger than that of the
SD/d = 1.10 and 6.95 times larger than that of
SD/d = 1.20, respectively. This finding indicates that the ability to convert pressure drop to dynamic pressure head is weaker than that of the
SD/d = 1.10 and
SD/d = 1.20 cases. According to thermodynamics [
26], this phenomenon occurs because when 1 <
n <
κ shown in
Table 3, the gas is heated and expanded, which indicates that the aerodynamic drag is not the main reason for kinetic energy consumption. In addition, when
SD/d = 1.05,
n is the lowest and close to 1. This means that the gas under
SD/d = 1.05 condition is close to the isothermal process, and the pressure energy can transform more internal energy under the no-heat-exchange condition. This physical phenomenon is caused by the strong viscous resistance and interference drag in the small gap condition. As a result, the temperature of the
SD/d = 1.05 case is higher than that of the others. When
Eu decreases, the leakage rate increases, as shown in
Figure 8.
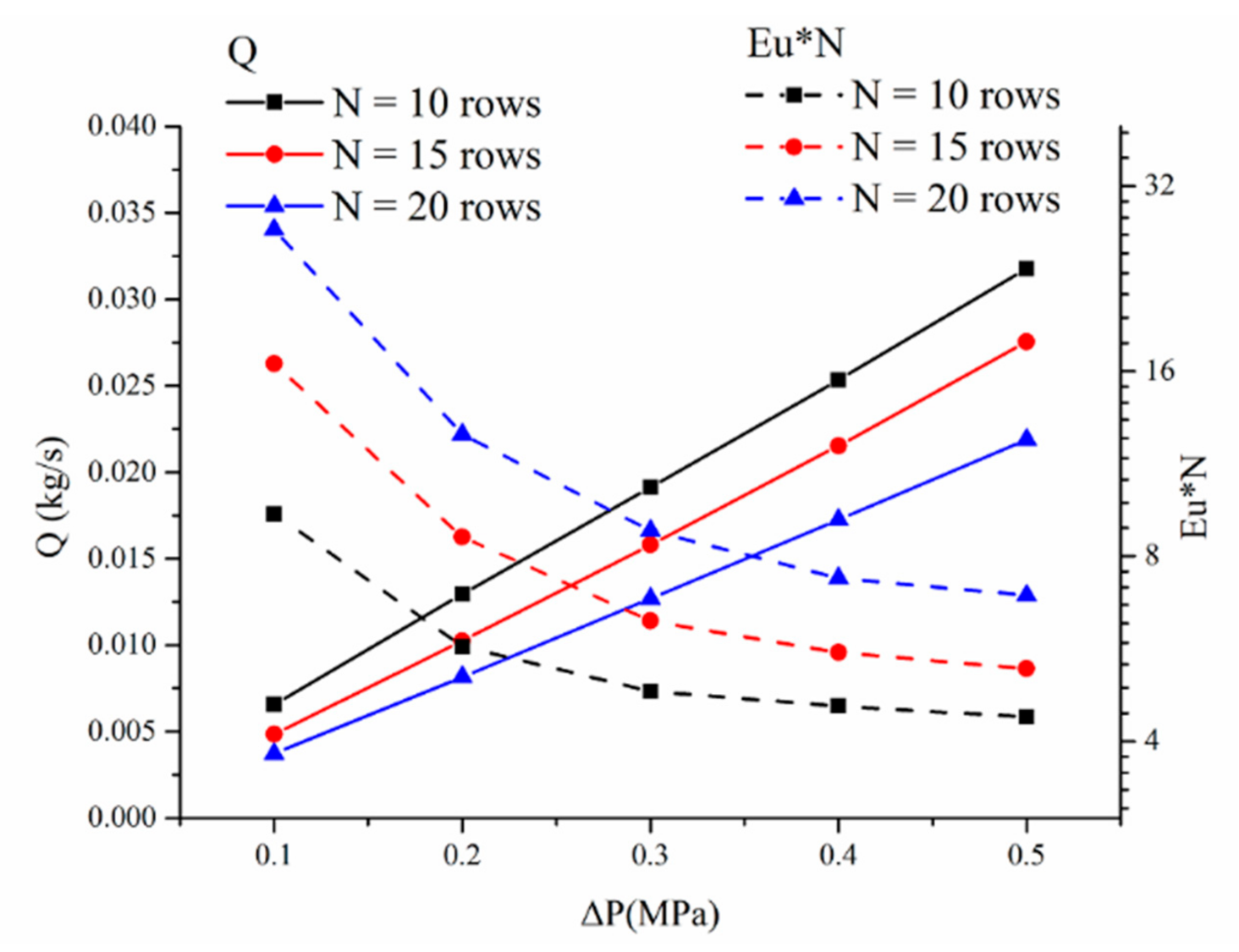
Given that it maybe has the same viscous resistance and interference drag to block the fluid in the same gap, the total pressure loss
Eu*N replaces
Eu in the study of the relationship between row number and fluid blocking ability. The results are given in
Figure 9, and it shows that
Eu increases with the increasing numbers of rows. The average values of
Eu*N of the 10-, 15-, and 20-row cases are 2.768, 8.432, and 12.581, respectively. The
Eu*N of the 20-row case is 1. 5 times that of the 15-row case and 4.5 times that of the 10-row case, indicating that the 20-row bristle model is better at blocking fluid than the other models. The cause is increasing friction loss due to increasing flow path. Furthermore, the leakage rate decreases as the rows increase, as shown in
Figure 9.
The gas flow can be retarded by increasing the number of bristle rows, and the magnitude of Eu at differential pressure from 0.1 to 0.2 MPa is
Eu20 >
Eu15 >
Eu10. However, when the differential pressure is 0.3 MPa, the
Eu values are sorted by magnitude changes to
Eu10 > Eu20 >
Eu15. The Eu value is
Eu10 >
Eu15 > Eu20 with the differential pressure increasing from 0.4 to 0.5MPa, as shown in
Table 4. This shows that increasing the number of rows at low differential pressure can have a positive impact on the fluid. However, when the differential pressure becomes large, the ability to block the gas by increasing the number of brush wire rows will be reduced.
The analyses in this section indicate that Eu and Q have a negative correlation, that is, leakage rate is related to the ability to convert pressure loss into kinetic energy. When the value of Eu is high, and the ability to convert pressure loss into kinetic energy is weak. More energy will be converted into internal energy, thereby reducing the leakage rate.
4.5. Pressure Distribution on the Bristle Surface
The flow around the circular cylinders is studied with the non-dimensional coefficient [
14]
CP. The deformation equation is as follows:
where
P0 is the stagnation pressure,
Pθ is the pressure on the surface of the cylinder, and
Vmax is the velocity in the gap between the bristles in the X-direction, which can be obtained due to the continuity equation as follows [
27]:
where
Vu is the velocity of the free stream. However, this equation is appropriate only for incompressible fluids because
ρ is ignored. The gas is the compressible fluid in the brush seal as discussed in the previous sections. Therefore, Equation (15) is not beneficial in comparing the pressure distribution on the surface of bristles. In this paper,
ρ is assumed to be homogeneous distributed in the bristle gap. According to the law of mass conservation, the equation of
Vmax can be written as follows:
According to Equations (14) and (16), the formula of
CP is then modified:
In addition, according to the conclusion of
Section 4.2, the Equation (17) can be rewritten as follows:
where
ρ∞ is the density of the inlet,
ρg is the density of the gap in each row, and
Pg is the pressure in the gap between bristles. In accordance with the flow direction,
θ is the angle of the pressure distribution on the surface, as shown in
Figure 10. Equation (17) is suitable for the dimensionless formula in numerical simulation because the local density value is easy to obtain. However, it is difficult to obtain the local density value in reality, so Equation (17) can be replaced by Equation (18).
In this paper, the 15 rows with
SD/d = 1.10 case is considered and Δ
P is 0.3 MPa. The first, 8th and 15th row are studied because the pressure distributions in the second to 14th row are similar. The
CP distributions for the chosen three rows are shown in
Figure 10, and the following results can be obtained,
The CP in the first and eighth rows of the bristles are symmetrical at about 180°. CPmin, the minimum pressure point, is located at 96° and the corresponding velocity is maximum. Then, an adverse pressure gradient appears, and the recirculation region is created at about 160°. In the region from 96° to 180°, the velocity decreases.
C*Pmin, the local minimum pressure point, is located at 41° in the cylinder surface of the eighth and 15th rows. This phenomenon occurs because the fluid is accelerated in the gap between the bristle and the front bristle [
14].
In the end row, the pressure distribution is asymmetric due to jet deflection. The jet deflection is relevant to the value of
ST/d. If
ST/d is smaller than 2, then the neighboring bristles have an impact on the fluid. This effect is called nearby interference [
14]. This jet deflection creates an aerodynamic force in the circumference direction, which leads to the oscillation in the end rows and the abrasion between the end row and the back plate. Meanwhile, the location of
CPmin in the first row and the eighth row is 96°. The location of
CPmin in the end row is 101°. The location of
CPmin in the end row moves backward 5° compared with the other rows.