Simplified 2D Skin Lattice Models for Multi-Axial Camber Morphing Wing Aircraft
Abstract
1. Introduction
2. Camber Morphing Wing Aircraft
2.1. Aircraft Model
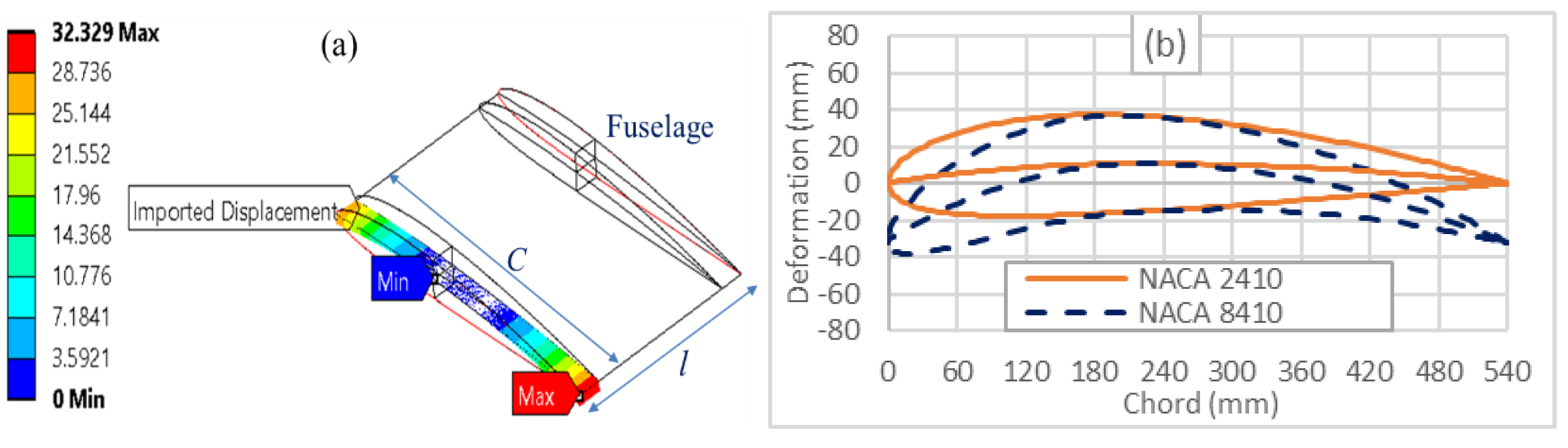
2.2. Wing Model
2.3. Camber Morphing Model
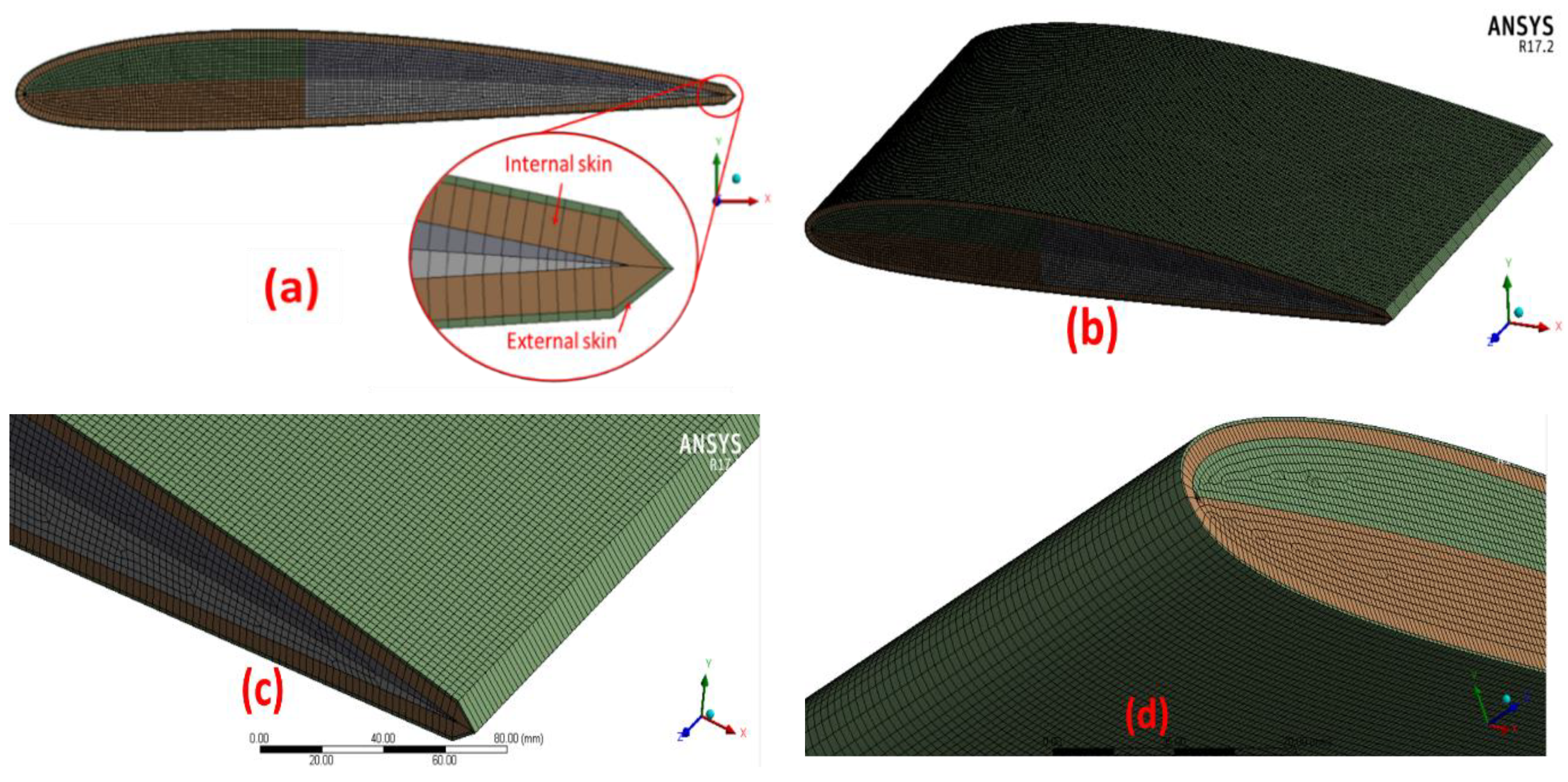
2.4. Internal and External Skin Models
3. Numerical Analysis
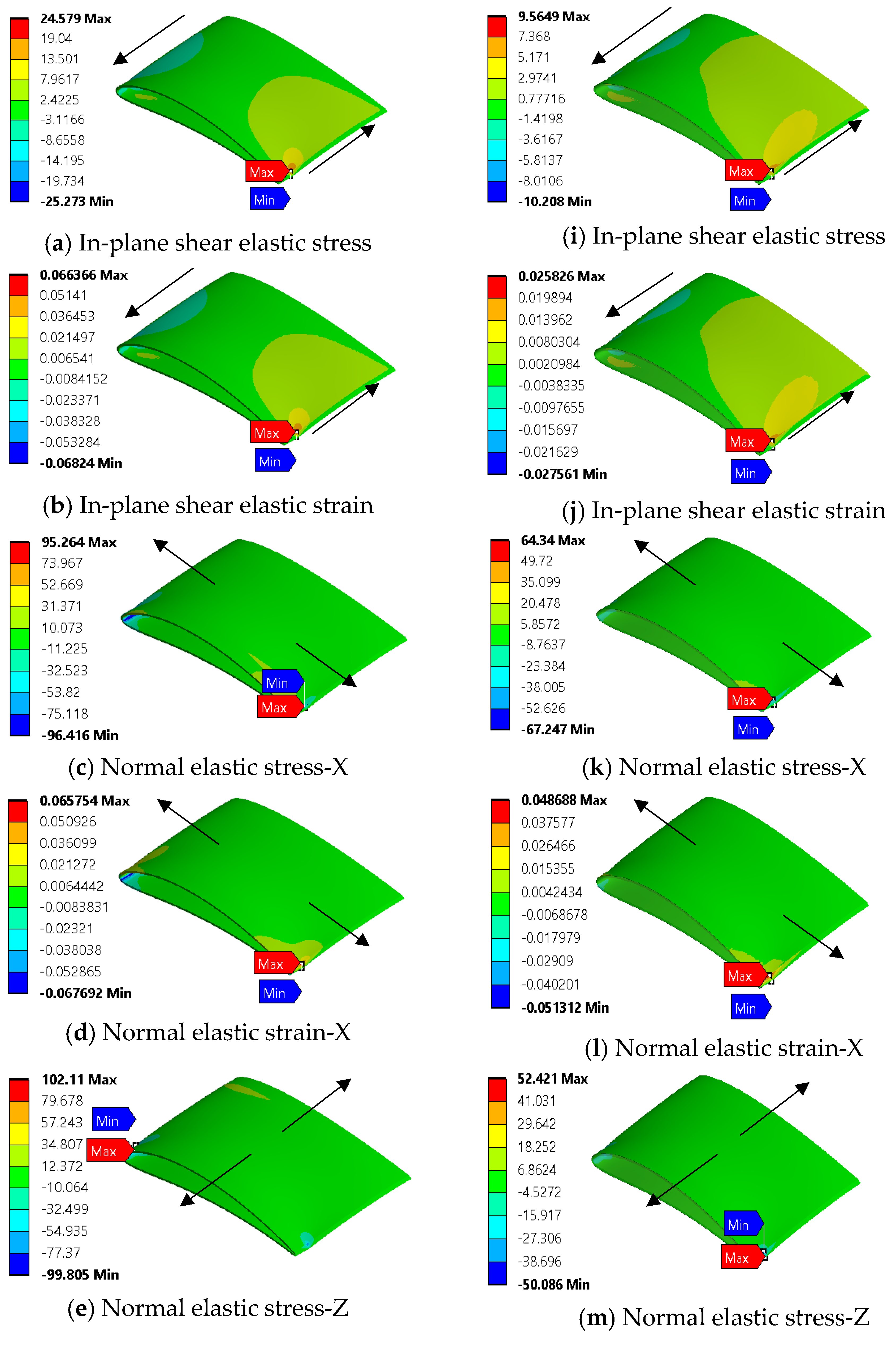
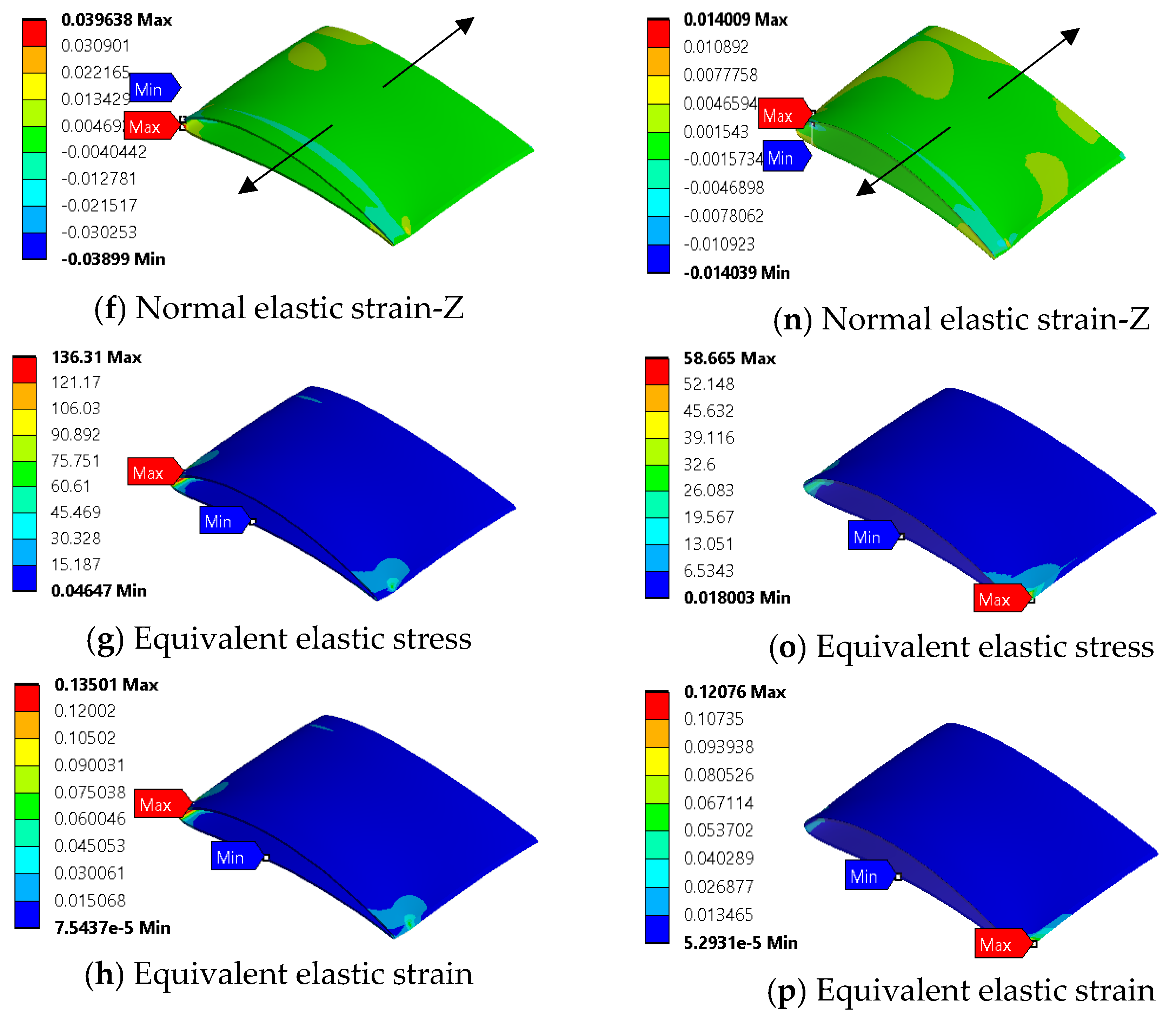
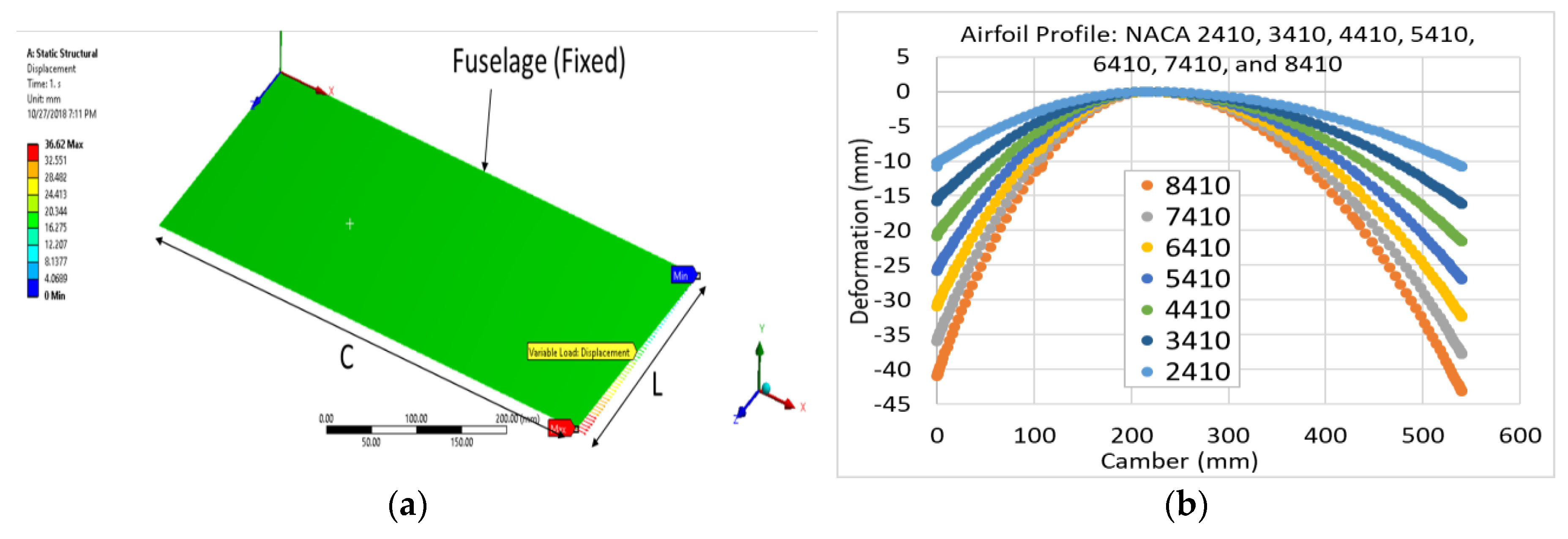
3.1. Wing Model Structural Analysis
3.2. 3D Wing Model Aerodynamic Analysis
3.3. 2D-Plate Bulk Material Structure
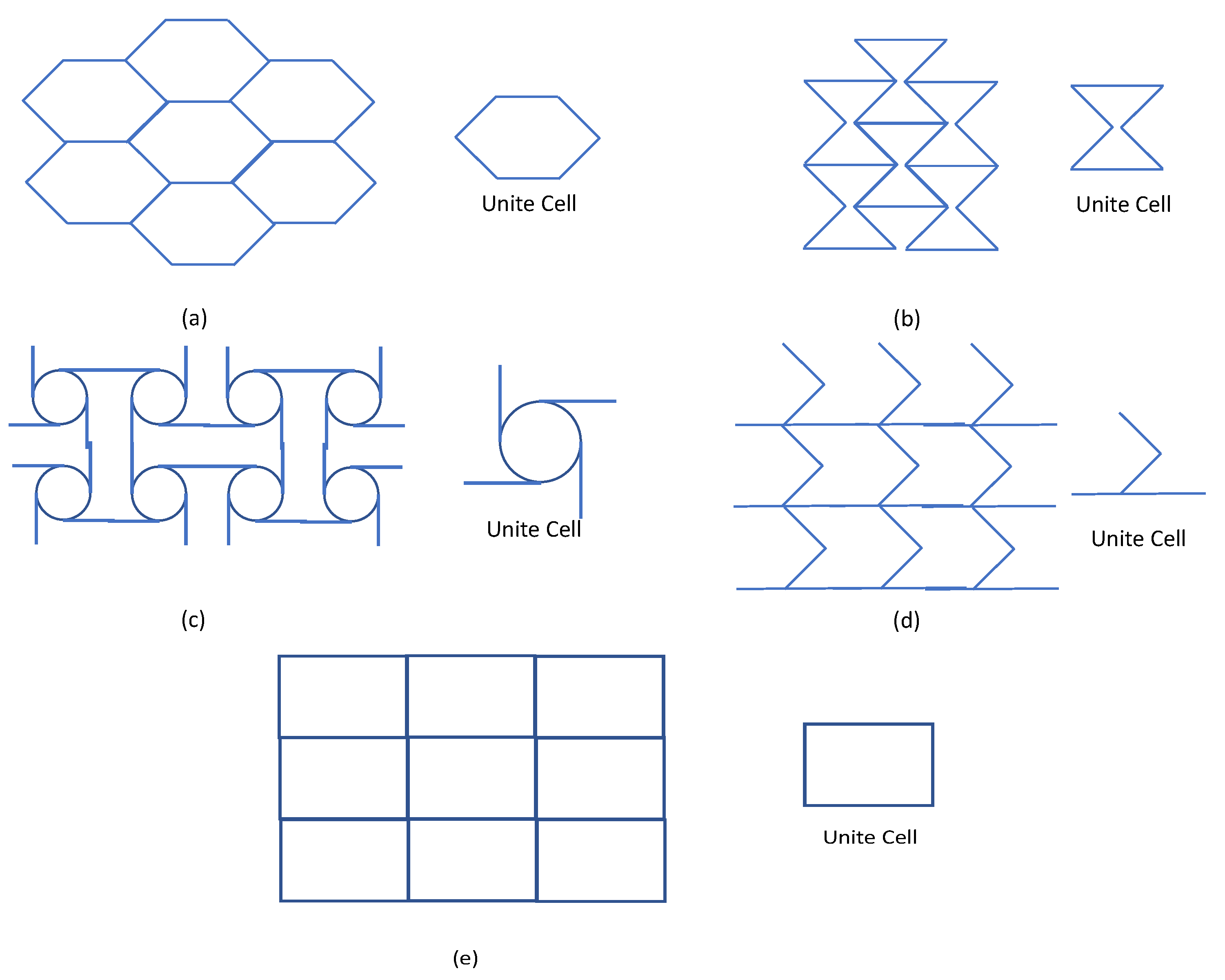
3.4. Lattice Structure for 2D-Plate Model
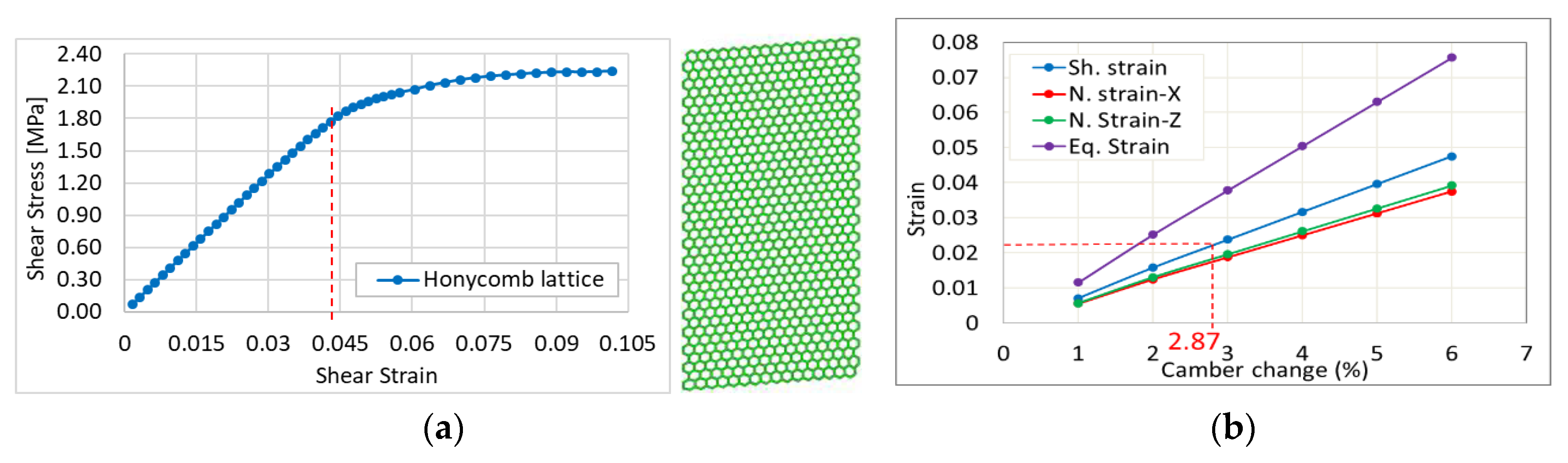
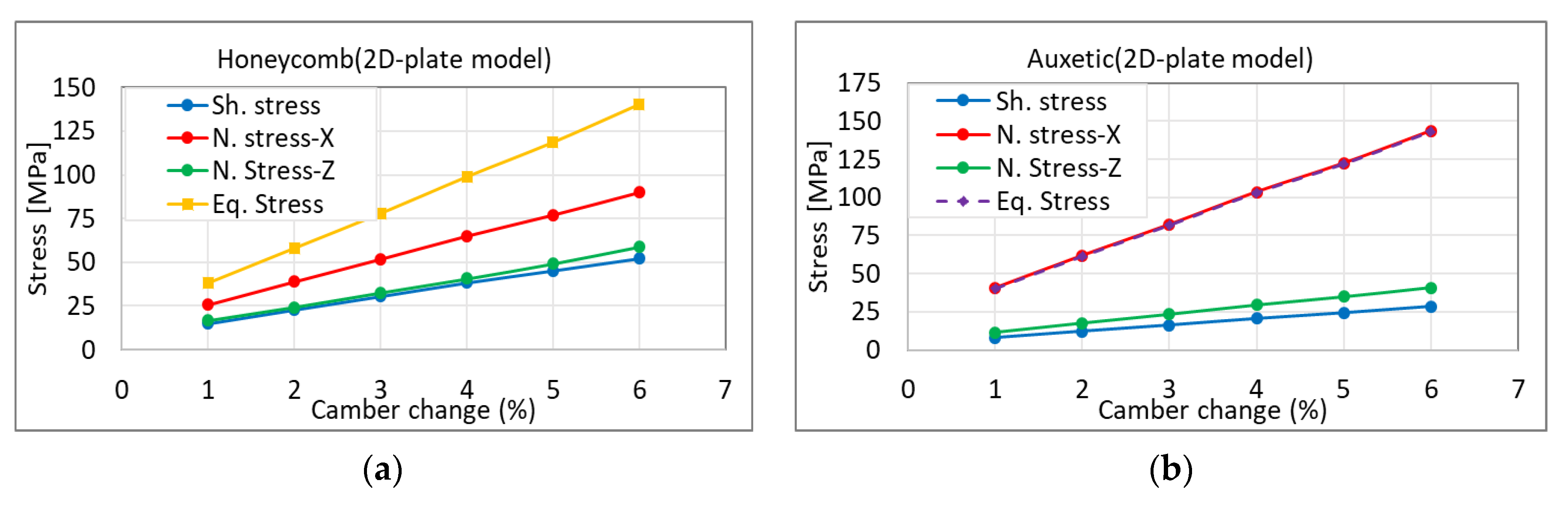
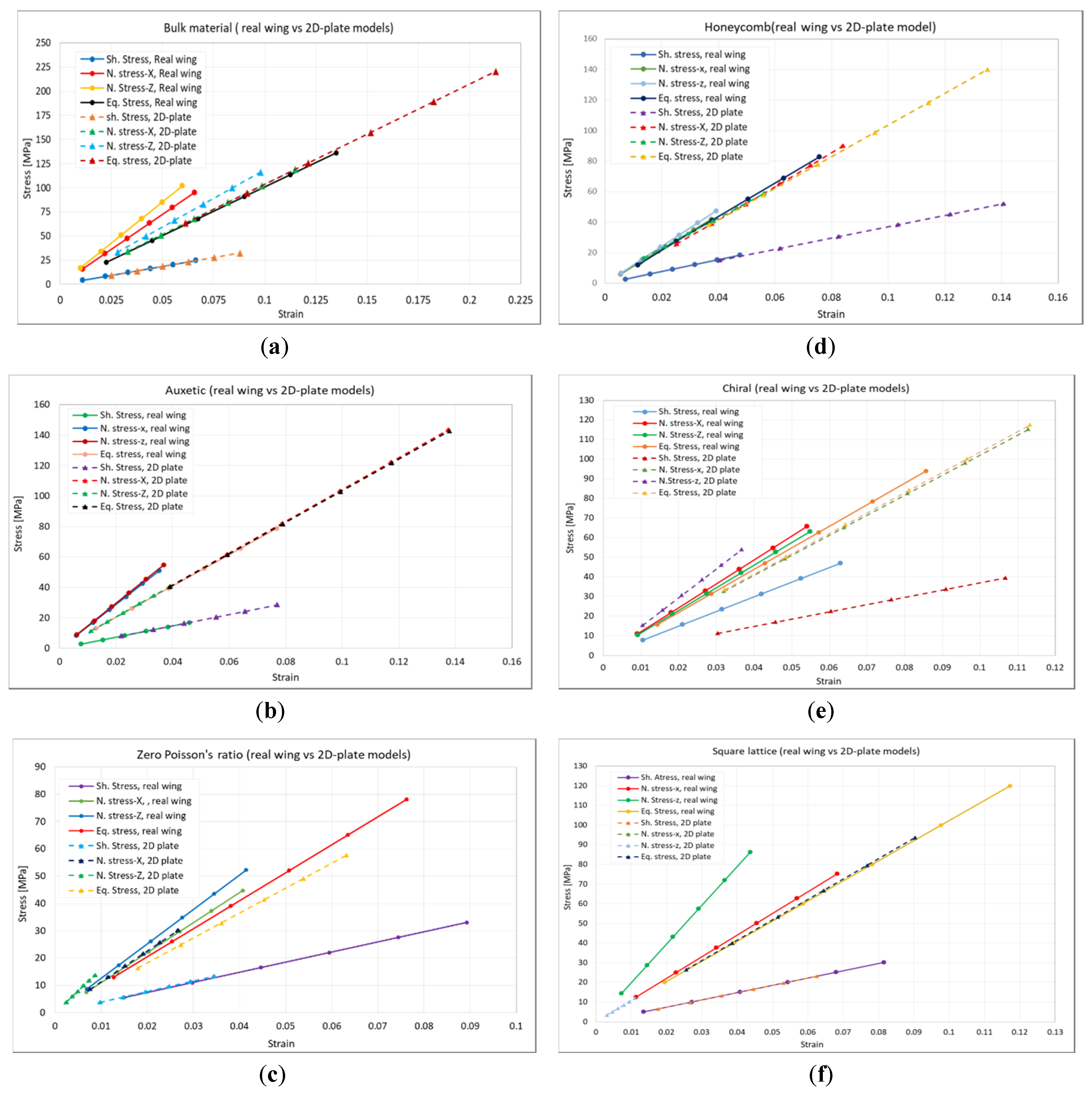
- Honeycomb lattice structure: Figure 12 demonstrates the honeycomb lattice 2D-plate model. The achieved result shows that the maximum elastic shear is 0.045, as shown in Figure 12a. If we consider a design safety factor to be two then, the desired elastic shear strain is 0.0225. This means that the expected morphing rate using this type of skin will be 2.87% (Figure 12b). In other words, if we use the honeycomb lattice as a wing cover, then we should not morph a wing more than 2.87%.
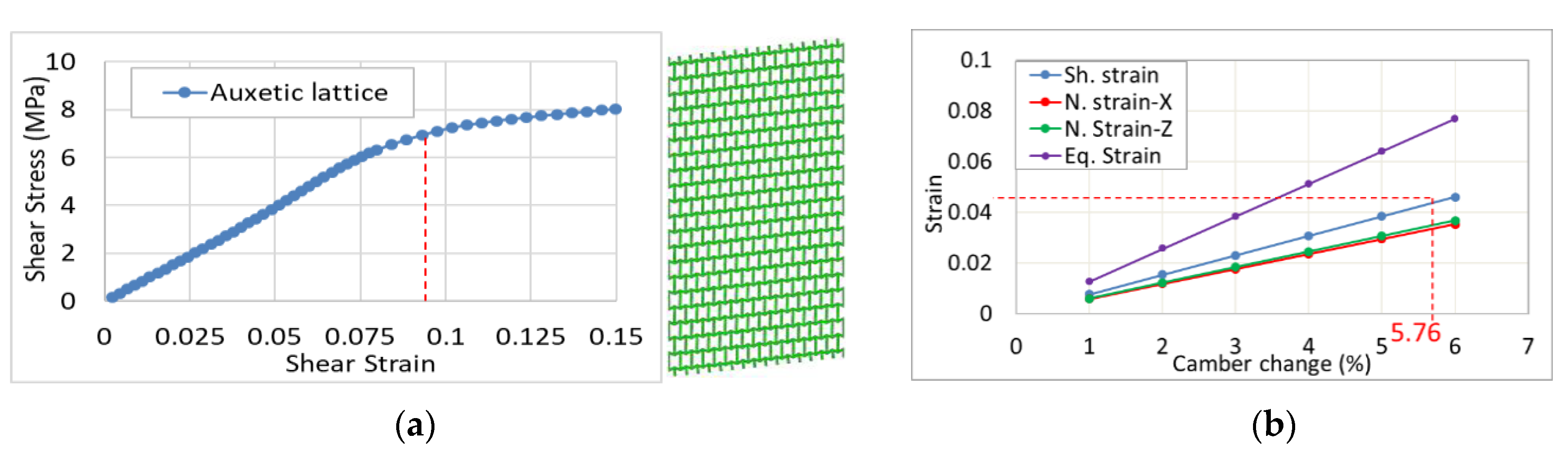
- Auxetic lattice structure: an auxetic 2D-plate model is shown in Figure 13. This model performs a more suitable shear elastic strain compared to other models. The achieved result shows that the maximum elastic shear is 0.09, as shown in Figure 13a. Thus, the desired elastic shear strain will be 0.045. This means that the expected morphing rate using this model will be 5.76% (Figure 13b).
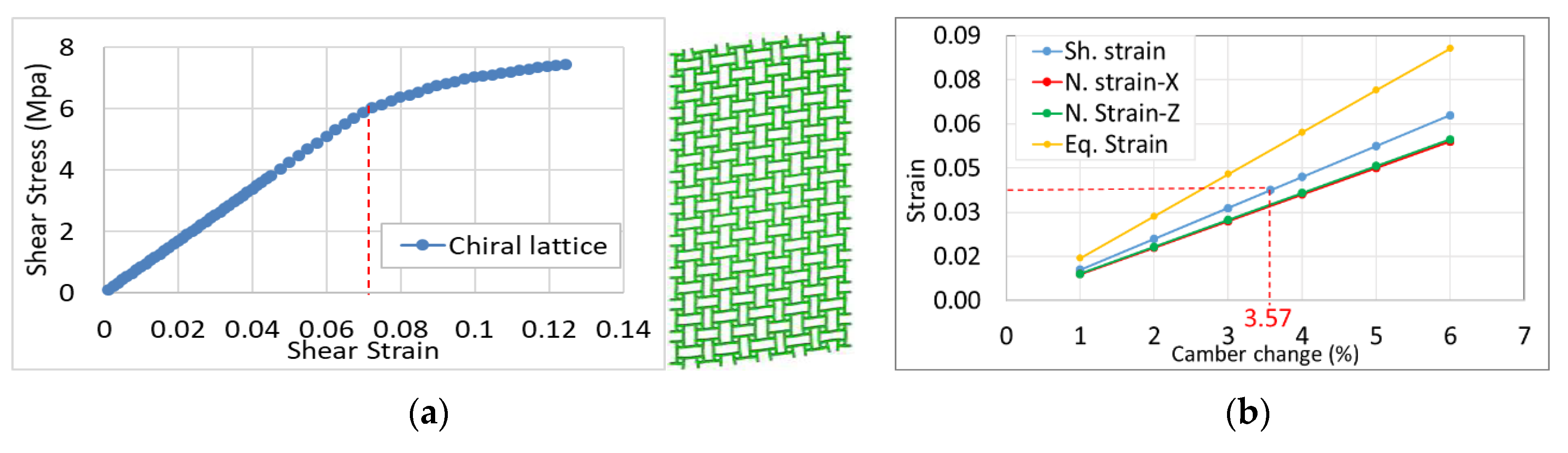
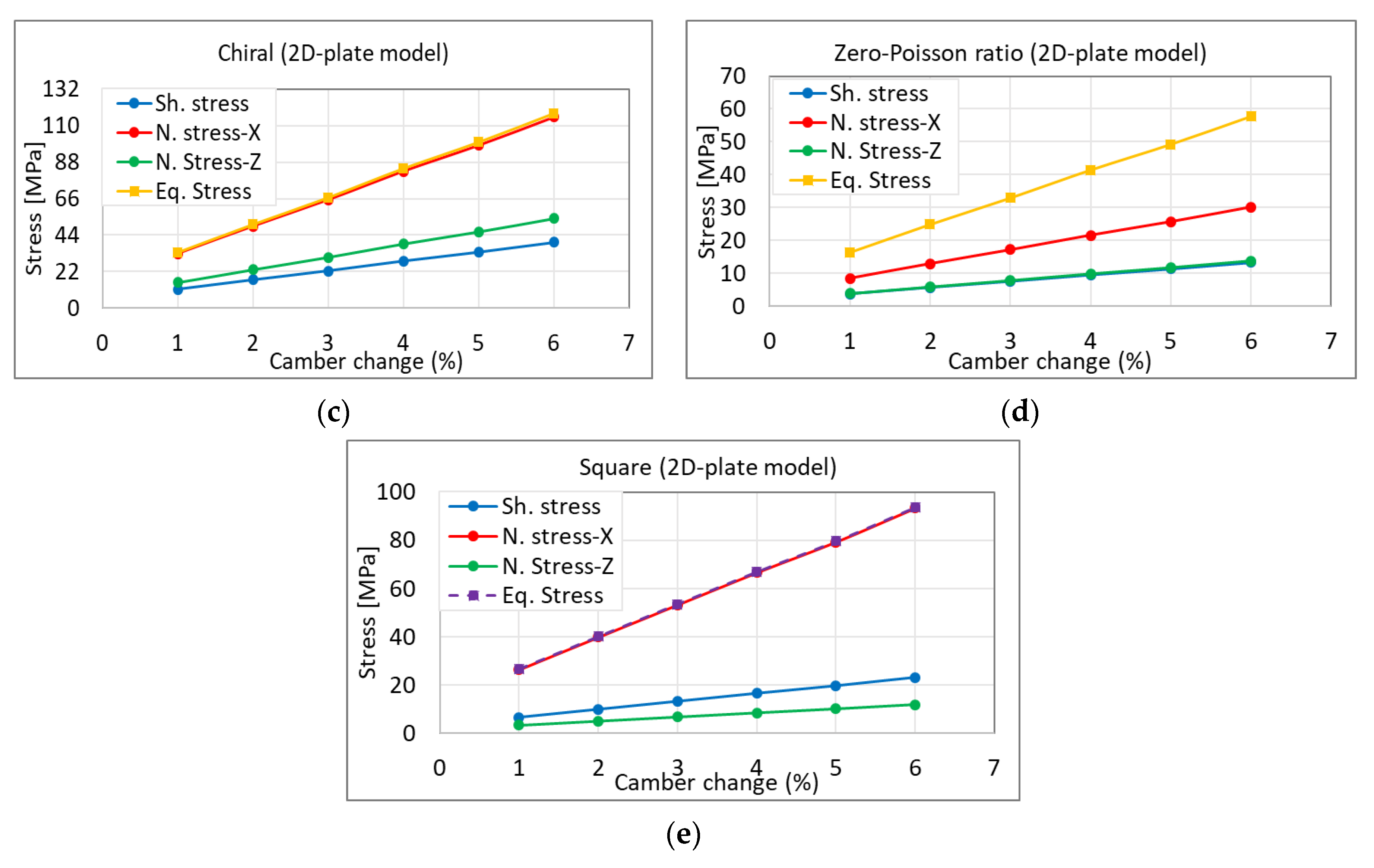
- Chiral lattice structure: a 2D-plate model for this model is shown in Figure 14. The maximum elastic shear strain is found to be 0.075. This means that the desired shear strain is 0.0375 when considering a 2 factor of safety. This result means that the maximum expected camber change using this type of structure will be 3.57% as shown in Figure 14b. The structure consumes a large amount of power to perform the morphing, as compared to other structures based on this result.
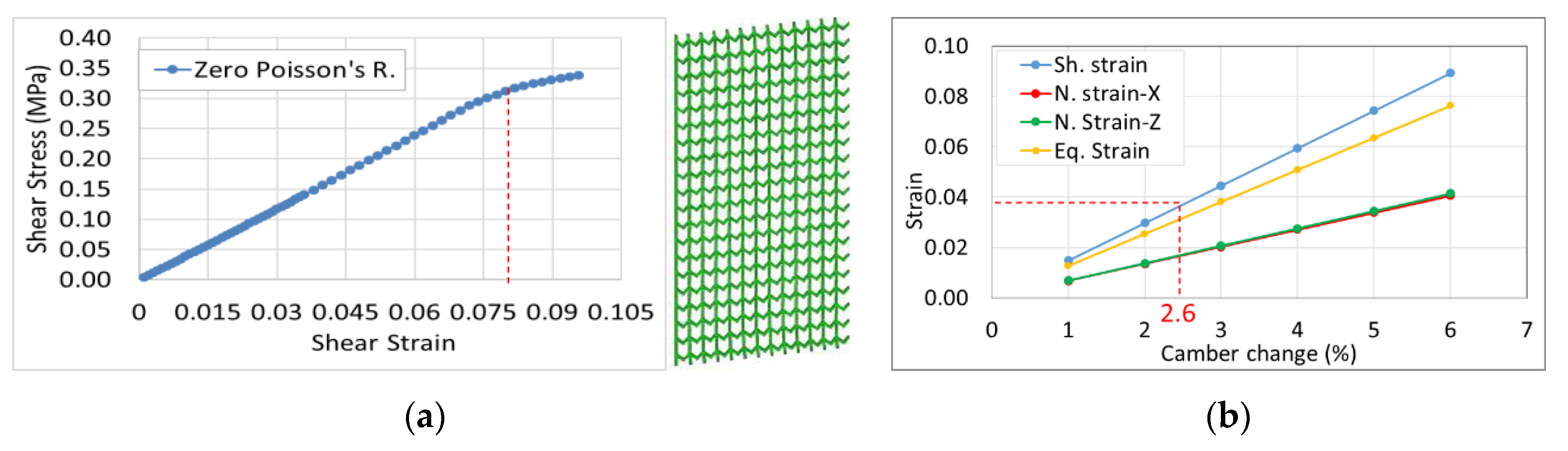
- Zero-Poisson ratio lattice: Figure 15 demonstrates the 2D-plate model for the zero-Poisson ratio lattice. The achieved result shows that the maximum elastic shear is 0.078 as shown in Figure 15a. Therefore, the desired elastic shear strain will be 0.039 which means that the expected morphing rate using this model will be 2.6% as shown in Figure 15b.
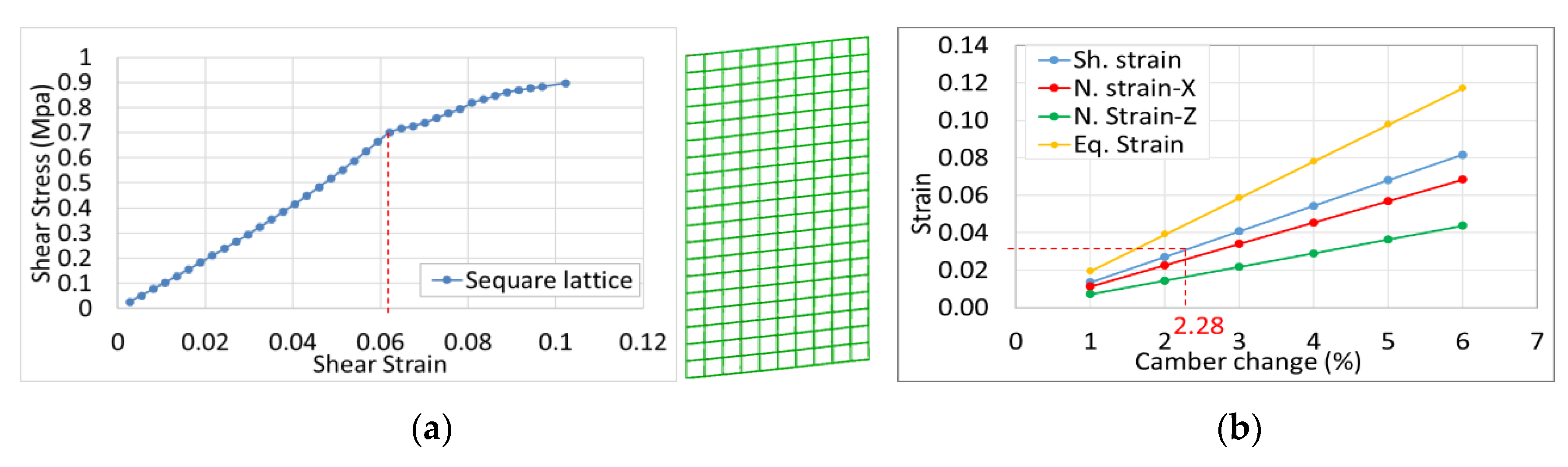
4. Comparison between 2D and 3D Models in Bulk and Lattice Structures
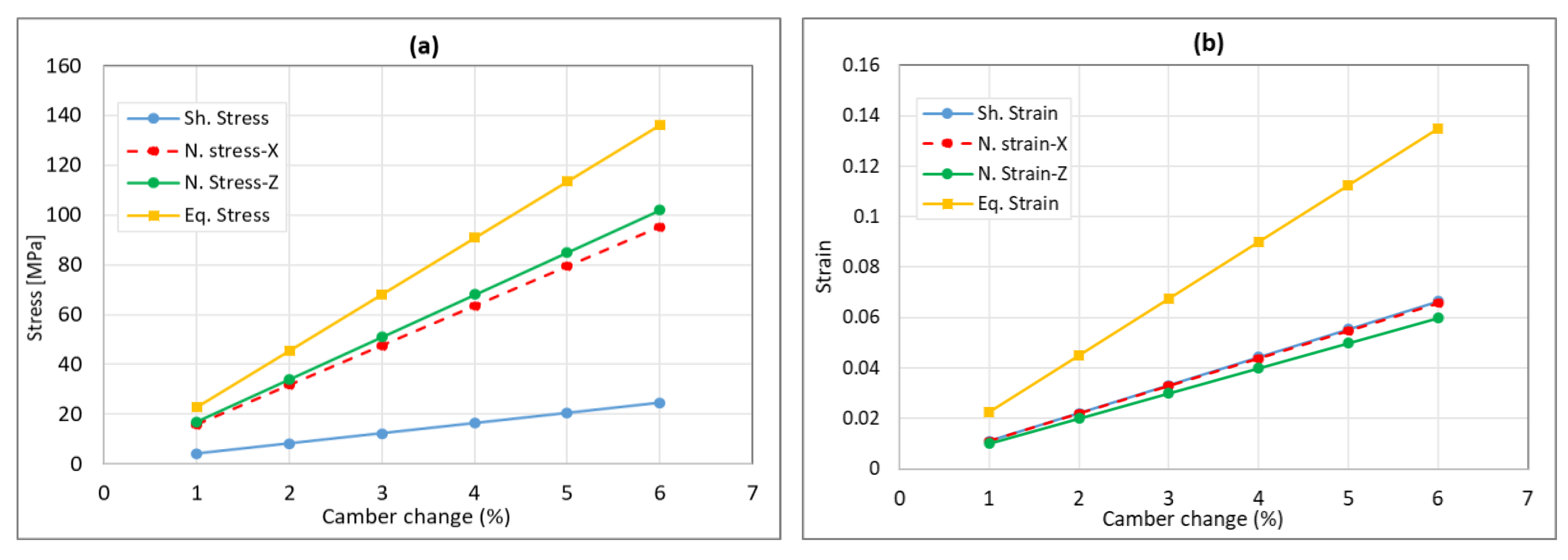
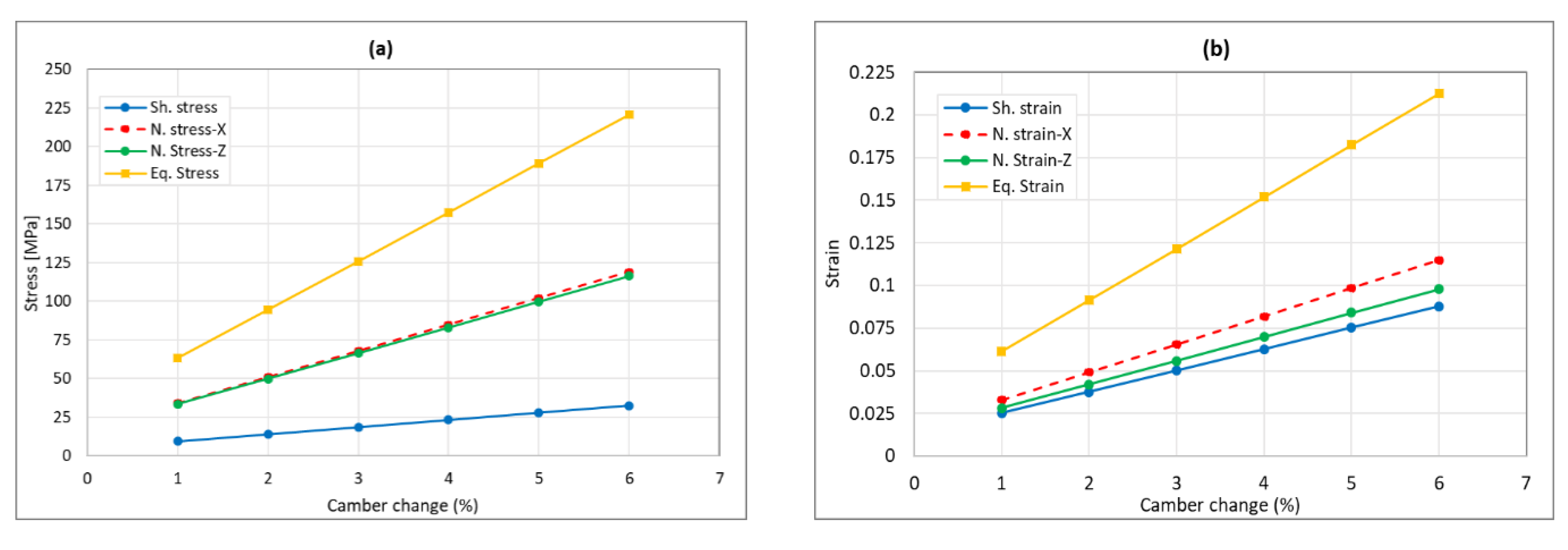
- Normal stress along x-direction (chord): the trendline for the 2D-plate model is y = 1036.9x or, mplate = 1036.9, while the wing is y = 1448.8x, or mwing = 1448.8. This means that the ratio, r, which is the result of dividing these trendlines with each other, will be 1.39. This translates when the normal strain-x of plate model is 0.0493, which is the case a 2% camber change, then the expected equivalent normal stress-x for 3D wing model will be r × mplae × x, or 1.39 × 1036.9 × 0.0493, which is 71.1 MPa for morphing a wing model.
- In-plane shear stress: the trendline for 2D-plate is the same for the wing, which is y = 370.36x or, mplate = 370.36. This means that the ratio r will be 1.0. This could be translated that when the in-plane shear strain of the plate model is 0.038, which is the case for a 2% camber change, then the expected equivalent in-plane shear stress for the wing model will be r × mplae × x, or 1.0 × 370.36 × 0.038, which is 14.1 MPa for morphing a wing model.
- Normal stress along z-direction (span): the trendline for 2D-plate model is y = 1187.3x or, mplate = 1187.3, while for the wing is y = 1706x, or mwing = 1706. This means that the ratio r will be 1.43. This could be translated that when the normal strain-z of the plate model is 0.042, which is the case for a 2% camber change, then the expected equivalent normal stress-z for the wing model will be r × mplae × x, or 1.43 × 1187.3 × 0.042, which is 102.5 MPa for morphing a wing model.
- Equivalent stress: the trendline for 2D-plate model is y = 1009.7x or, mplate = 1009.7, while for the wing is y = 1037x, or mwing = 1037. This means that the ratio r will be 1.03. This could be translated that when the equivalent strain of the plate model is 0.091, which is the case for a 2% camber change, then the expected equivalent stress for the wing model will be r × mplae × x, or 1.03 × 1009.7 × 0.091, which is 71.3 MPa for morphing a wing model.
5. Summary
6. Conclusions and Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Sun, J.; Guan, Q.; Liu, Y.; Leng, J. Morphing aircraft based on smart materials and structures: A state-of-the-art review. J. Intell. Mater. Syst. Struct. 2016, 27, 2289–2312. [Google Scholar] [CrossRef]
- Prisacariu, V.; Sandru, V.; Rău, C. Introduction morphing technology in unmanned aircraft vehicles (UAV). In Proceedings of the International Conference of Scientific Paper AFASES 2011, Brasov, Romania, 26–28 May 2011. [Google Scholar]
- Min, Z.; Kien, V.K.; Richard, L.J.Y. Aircraft morphing wing concepts with radical geometry change. IES J. Part A Civ. Struct. Eng. 2010, 3, 188–195. [Google Scholar] [CrossRef]
- Kammegne, M.J.T.; Botez, R.M.; Grigorie, L.T.; Mamou, M.; Mébarki, Y. Proportional fuzzy feed-forward architecture control validation by wind tunnel tests of a morphing wing. Chin. J. Aeronaut. 2017, 30, 561–576. [Google Scholar] [CrossRef]
- Barbarino, S.; Bilgen, O.; Ajaj, R.M.; Friswell, M.I.; Inman, D.J. A Review of Morphing Aircraft. J. Intell. Mater. Syst. Struct. 2011, 22, 823–877. [Google Scholar] [CrossRef]
- Ajaj, R.M.; Beaverstock, C.S.; Friswell, M.I. Morphing aircraft: The need for a new design philosophy. Aerosp. Sci. Technol. 2016, 49, 154–166. [Google Scholar] [CrossRef]
- De Gaspari, A.; Riccobene, L.; Ricci, S. Design, Manufacturing and Wind Tunnel Validation of a Morphing Compliant Wing. J. Aircr. 2018, 55, 2313–2326. [Google Scholar] [CrossRef]
- Cooper, J.E.; Chekkal, I.; Cheung, R.C.M.; Wales, C.; Allen, N.J.; Lawson, S.; Peace, A.J.; Cook, R.; Standen, P.; Hancock, S.D.; et al. Design of a Morphing Wingtip. J. Aircr. 2015, 52, 1394–1403. [Google Scholar] [CrossRef]
- Chekkal, I.; Cheung, R.; Wales, C.; Cooper, J.E.; Allen, N.; Lawson, S.; Peace, A.J.; Hancock, S.; Cook, R.; Standen, P.; et al. Design of a morphing wing tip. In Proceedings of the AIAA SciTech 22nd AIAA/ASME/AHS Adaptive Structures Conference, National Harbor, MD, USA, 13–17 January 2014. [Google Scholar]
- Li, D.; Zhao, S.; Da Ronch, A.; Xiang, J.; Drofelnik, J.; Li, Y.; Zhang, L.; Wu, Y.; Kintscher, M.; Monner, H.P.; et al. A review of modelling and analysis of morphing wings. Prog. Aerosp. Sci. 2018, 100, 46–62. [Google Scholar] [CrossRef]
- Thill, C.; Etches, J.; Bond, I.; Potter, K.; Weaver, P. Composite corrugated structures for morphing wing skin applications. Smart Mater. Struct. 2010, 19, 124009. [Google Scholar] [CrossRef]
- Vingliotti, A.; Pasini, D. Analysis and design of lattice materials for large cord and curvature variations in skin panels of morphing wings. Smart Mater. Struct. 2015, 24, 037006. [Google Scholar] [CrossRef]
- Kuder, I.K.; Arrieta, A.F.; Raither, W.E.; Ermanni, P. Variable stiffness material and structural concepts for morphing applications. Prog. Aerosp. Sci. 2013, 63, 33–55. [Google Scholar] [CrossRef]
- La, S.; Joe, W.Y.; Akbar, M.; Alsaidi, B. Surveys on Skin Design for Morphing Wing Aircraft: Status and Challenges. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, AIAA SciTech Forum, (AIAA), Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar] [CrossRef]
- Takahashi, H.; Yokozeki, T.; Hirano, Y. Development of variable camber wing with morphing leading and trailing sections using corrugated structures. J. Intell. Mater. Syst. Struct. 2016, 27, 2827–2836. [Google Scholar] [CrossRef]
- Bai, J.B.; Chen, D.; Xiong, J.J.; Shenoi, R.A. A corrugated flexible composite skin for morphing applications. J. Compos. Part B 2017, 131, 134–143. [Google Scholar] [CrossRef]
- Navaratne, R.; Dayyani, I.; Woods, B.; Friswell, M.I. Development and Testing of a Corrugated Skin for a Camber Morphing Aerofoil. In Proceedings of the 23rd AIAA/AHS Adaptive Structures Conference, AIAA SciTech Forum, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar] [CrossRef]
- Previtali, F.; Arrieta, A.F.; Ermanni, P. Double-walled corrugated structure for bending-stiff anisotropic morphing skins. J. Intell. Mater. Syst. Struct. 2015, 26, 599–613. [Google Scholar] [CrossRef]
- Previtali, F.; Molinari, G.; Arrieta, A.F.; Guillaume, M.; Ermanni, P. Design and experimental characterisation of a morphing wing with enhanced corrugated skin. J. Intell. Mater. Syst. Struct. 2016, 27, 278–292. [Google Scholar] [CrossRef]
- Sun, Y.; Pugno, N.M. In plane stiffness of multifunctional hierarchical honeycombs with negative Poisson’s ratio sub-structures. Compos. Struct. 2013, 106, 681–689. [Google Scholar] [CrossRef]
- Taylor, C.M.; Smith, C.W.; Miller, W.; Evans, K.E. The effects of hierarchy on the in-plane elastic properties of honeycombs. Int. J. Solids Struct. 2011, 48, 1330–1339. [Google Scholar] [CrossRef]
- Mousanezhad, D.; Haghpanah, B.; Ghosh, R.; Hamouda, A.M.; Nayeb-Hashemi, H.; Vaziri, A. Elastic properties of chiral, anti-chiral, and hierarchical honeycombs: A simple energy-based approach. Theor. Appl. Mech. 2016, 6, 81–96. [Google Scholar] [CrossRef]
- Bouakba, M.; Bezazi, A.; Scarpa, F. FE analysis of the in-plane mechanical properties of a novel Voronoi-type lattice with positive and negative Poisson’s ratio configurations. Int. J. Solids Struct. 2012, 49, 2450–2459. [Google Scholar] [CrossRef]
- Chen, Y.J.; Scarpa, F.; Liu, Y.J.; Leng, J.S. Elasticity of anti-tetrachiral anisotropic lattices. Int. J. Solids Struct. 2013, 50, 996–1004. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, L. Harnessing structural hierarchy to design stiff and lightweight phononic crystals. J. Extrem. Mech. 2016, 9, 91–96. [Google Scholar] [CrossRef]
- Chen, Y.; Yin, W.; Liu, Y.; Leng, J. Structural design and analysis of morphing skin embedded with pneumatic muscle fibers. Smart Mater. Struct. 2011, 20, 085033. [Google Scholar] [CrossRef]
- Chen, S.; Chen, Y.; Zhang, Z.; Liu, Y.; Leng, J. Experiment and analysis of morphing skin embedded with shape memory polymer composite tube. J. Intell. Mater. Syst. Struct. 2014, 25, 2052–2059. [Google Scholar] [CrossRef]
- Olympio, K.R.; Gandhi, F. Flexible Skins for Morphing Aircraft Using Cellular Honeycomb Cores. J. Intell. Mater. Syst. Struct. 2010, 21, 1719–1735. [Google Scholar] [CrossRef]
- Qiu, J.; Wang, C.; Huang, C.; Ji, H.; Xu, Z. Smart skin and actuators for morphing structures. In Proceedings of the 23rd International Congress of Theoretical and Applied Mechanics, Procedia IUTAM, Beijing, China, 19–24 August 2012; Volume 10, pp. 427–441. [Google Scholar] [CrossRef]
- Chen, Y.; Li, T.; Jia, Z.; Scarpa, F.; Yao, C.W.; Wang, L. 3D printed hierarchical honeycombs with shape integrity under large compressive deformations. J. Mater. Des. 2018, 137, 226–234. [Google Scholar] [CrossRef]
- Mitschke, H.; Robins, V.; Mecke, K.; Schröder-Turk, G.E. Finite auxetic deformations of plane tessellations. Proc. R. Soc. 2013, 469, 20120465. [Google Scholar] [CrossRef]
- Yang, L.; Harrysson, O.; West, H.; Cormier, D. Mechanical properties of 3D re-entrant honeycomb auxetic structures realized via additive manufacturing. Int. J. Solids Struct. 2015, 69, 475–490. [Google Scholar] [CrossRef]
- Chen, J.; Shen, X.; Li, J. Zero Poisson’s ratio flexible skin for potential two-dimensional wing morphing. J. Aerosp. Sci. Technol. 2015, 45, 228–241. [Google Scholar] [CrossRef]
- Olympio, K.R.; Gandhi, F. Zero Poisson’s Ratio Cellular Honeycombs for Flex Skins Undergoing One-Dimensional Morphing. J. Intell. Mater. Syst. Struct. 2010, 21, 1737–1753. [Google Scholar] [CrossRef]
- Ajdari, A.; Jahromi, B.H.; Papadopoulos, J.; Nayeb-Hashemi, H.; Vaziri, A. Hierarchical honeycombs with tailorable properties. Int. J. Solids Struct. 2012, 49, 1413–1419. [Google Scholar] [CrossRef]
- Jakubinek, M.; Ashrafi, B.; Martinez-Rubi, Y.; Laqua, K.; Palardy-Sim, M.; Roy, S.; Rahmat, M.; Sunesara, A.; Dénommée, S.; Simard, B. Multifunctional skin materials based on tailorable, carbon-nanotube-polyurethane composite sheets. In Proceedings of the 2018 AIAA SciTech Forum, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar] [CrossRef]
- Alsaidi, B.; Akbar, M.; La, S.; Joe, W.Y.; You, H.; Kim, S.; Yun, G. Modeling and Stress Analysis of Composite Skin Structure for Camber Morphing Wing. In Proceedings of the 2018 Multidisciplinary Analysis and Optimization Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar] [CrossRef]
- Wikipedia Open Source. Available online: https://en.wikipedia.org/wiki/AAI_RQ-7_Shadow (accessed on 18 October 2018).
- ANSYS. Fluent Theory Guide; Release 17.2; ANSYS Inc.: Cannonsburg, PA, USA, 2016; Available online: https://www.ansys.com/ (accessed on 10 April 2018).
- Tanaka, H.; Okada, H.; Shimasue, Y.; Liu, H. Flexible flapping wings with self-organized microwrinkles. Bioinspir. Biomim. 2015, 10, 046005. [Google Scholar] [CrossRef]



| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Wing span | 1.828 m | Wing chord | 0.54 m |
| Wing skin thickness | 4.6 kg/m2 | Spar location | 40% from leading edge |
| Morphing range | Fixed wing | Takeoff weight | 1452 N |
| Empty weight | 823.8 N | Gross weight | 1646.8 N |
| Parts | X Coordinate (mm) | Y Coordinate (mm) | Z Coordinate (mm) |
|---|---|---|---|
| Spar | 216 | 0–1828.78 | 0 |
| Fuselage (fixed end) | 0–540 | 0–40 | 0 |
| Actuator 1 | 0–540 | 298.13–338.13 | 0 |
| Actuator 2 | 0–540 | 596.26–636.26 | 0 |
| Actuator 3 | 0–540 | 894.39–934.39 | 0 |
| Actuator 4 | 0–540 | 1192.52–1232.52 | 0 |
| Actuator 5 | 0–540 | 1490.65–153.65 | 0 |
| Actuator 6 (free end) | 0–540 | 1788.78–1828.78 | 0 |
| Internal Skin | External Skin | ||||
|---|---|---|---|---|---|
| Parameters | Max. Val. | Min. Val. | Parameters | Max. Val. | Min. Val. |
| In-plane shear stress (MPa) | 24.6 | −25.3 | In-plane shear stress (MPa) | 9.6 | −10.2 |
| In-plane shear strain | 0.066 | −0.068 | In-plane shear strain | 0.029 | −0.028 |
| Normal stress-X (MPa) | 95 | −96 | Normal stress-X (MPa) | 64 | −67 |
| Normal strain-X | 0.066 | −0.067 | Normal strain-X | 0.049 | −0.051 |
| Normal stress-Z (MPa) | 102 | −100 | Normal stress-Z (MPa) | 52 | −50 |
| Normal strain-Z | 0.04 | −0.04 | Normal strain-Z | 0.01 | −0.01 |
| Equivalent Stress (MPa) | 136 | 0 | Equivalent Stress (MPa) | 58.6 | 0 |
| Equivalent Strain | 0.135 | 0 | Equivalent Strain | 0.121 | 0 |
| Parameters | Max. Val. | Min. Val. |
|---|---|---|
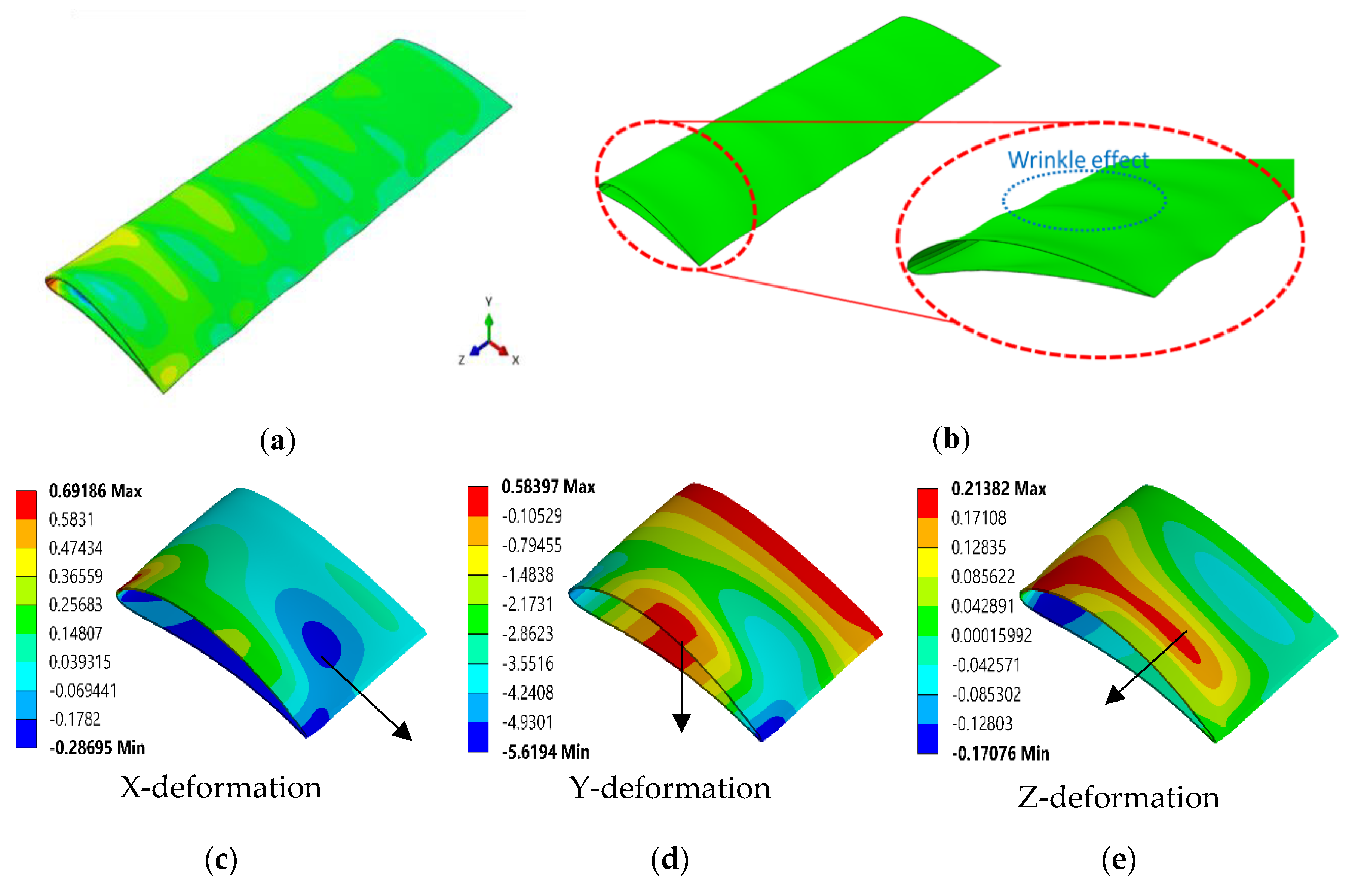
| Directional deformation-X (mm) | 0.692 | −0.287 |
| Directional deformation-Y (mm) | 0.584 | −5.619 |
| Directional deformation-Z (mm) | 0.214 | −0.171 |
| Type of Skin | Trendline: Plate Model | Trendline: Wing Model | Ratio (r) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Shear Stress | Normal Stress-x | Normal Stress-z | Eq. Stress | Shear Stress | Normal Stress-x | Normal Stress-z | Eq. Stress | r1 | r2 | r3 | r4 | |
| Bulk | y = 370.36x | y = 1036.9x | y = 1187.3x | y = 1009.7x | y = 370.36x | y = 1448.8x | y = 1706x | y = 1037x | 1.0 | 1.39 | 1.43 | 1.03 |
| Honeycomb | y = 370.36x | y = 1093.6x | y = 990.3x | y = 1037x | y = 389.52x | y = 1123.5x | y = 1211.5x | y = 1101.8x | 1.05 | 1.03 | 1.22 | 1.06 |
| Auxetic | y = 370.36x | y = 1044x | y = 1041.1x | y = 1036x | y = 370.36x | y = 1448.6x | y = 1487.3x | y = 1026.1x | 1.0 | 1.39 | 1.43 | 0.99 |
| Chiral | y = 370.36x | y = 1021x | y = 1469.2x | y = 1037x | y = 746.74x | y = 1217.3x | y = 1151.5x | y = 1097.8x | 2.01 | 1.19 | 0.78 | 1.06 |
| Zero-Poisson | y = 387.32x | y = 1128.6x | y = 1592.7x | y = 912.18x | y = 370.36x | y = 1099.4x | y = 1259.7x | y = 1024.7x | 0.96 | 0.97 | 0.79 | 1.12 |
| Square | y = 370.36x | y = 1035.8x | y = 1076.4x | y = 1036.7x | y = 370.36x | y = 1101x | y = 1972.2x | y = 1023.2x | 1.0 | 1.06 | 1.83 | 0.99 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsaidi, B.; Joe, W.Y.; Akbar, M. Simplified 2D Skin Lattice Models for Multi-Axial Camber Morphing Wing Aircraft. Aerospace 2019, 6, 90. https://doi.org/10.3390/aerospace6080090
Alsaidi B, Joe WY, Akbar M. Simplified 2D Skin Lattice Models for Multi-Axial Camber Morphing Wing Aircraft. Aerospace. 2019; 6(8):90. https://doi.org/10.3390/aerospace6080090
Chicago/Turabian StyleAlsaidi, Bashir, Woong Yeol Joe, and Muhammad Akbar. 2019. "Simplified 2D Skin Lattice Models for Multi-Axial Camber Morphing Wing Aircraft" Aerospace 6, no. 8: 90. https://doi.org/10.3390/aerospace6080090
APA StyleAlsaidi, B., Joe, W. Y., & Akbar, M. (2019). Simplified 2D Skin Lattice Models for Multi-Axial Camber Morphing Wing Aircraft. Aerospace, 6(8), 90. https://doi.org/10.3390/aerospace6080090





