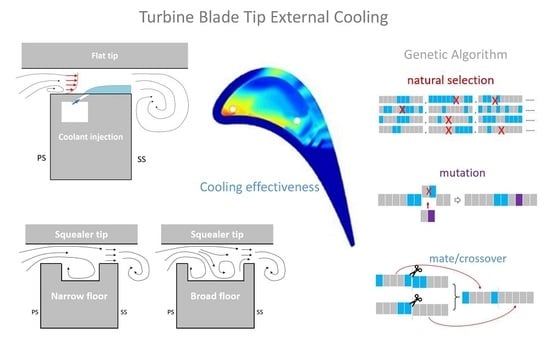
Turbine Blade Tip External Cooling Technologies
Abstract
1. Introduction
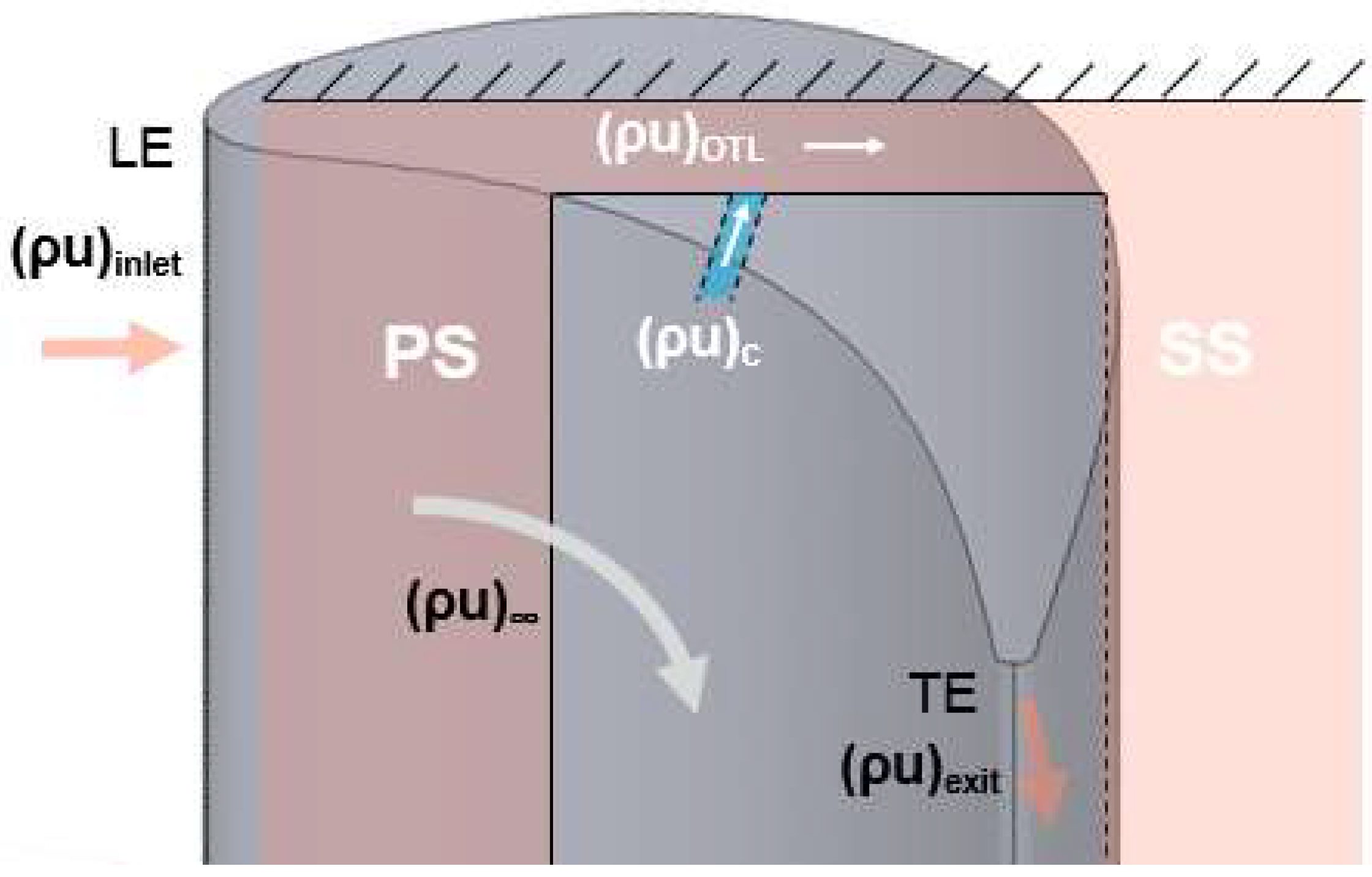
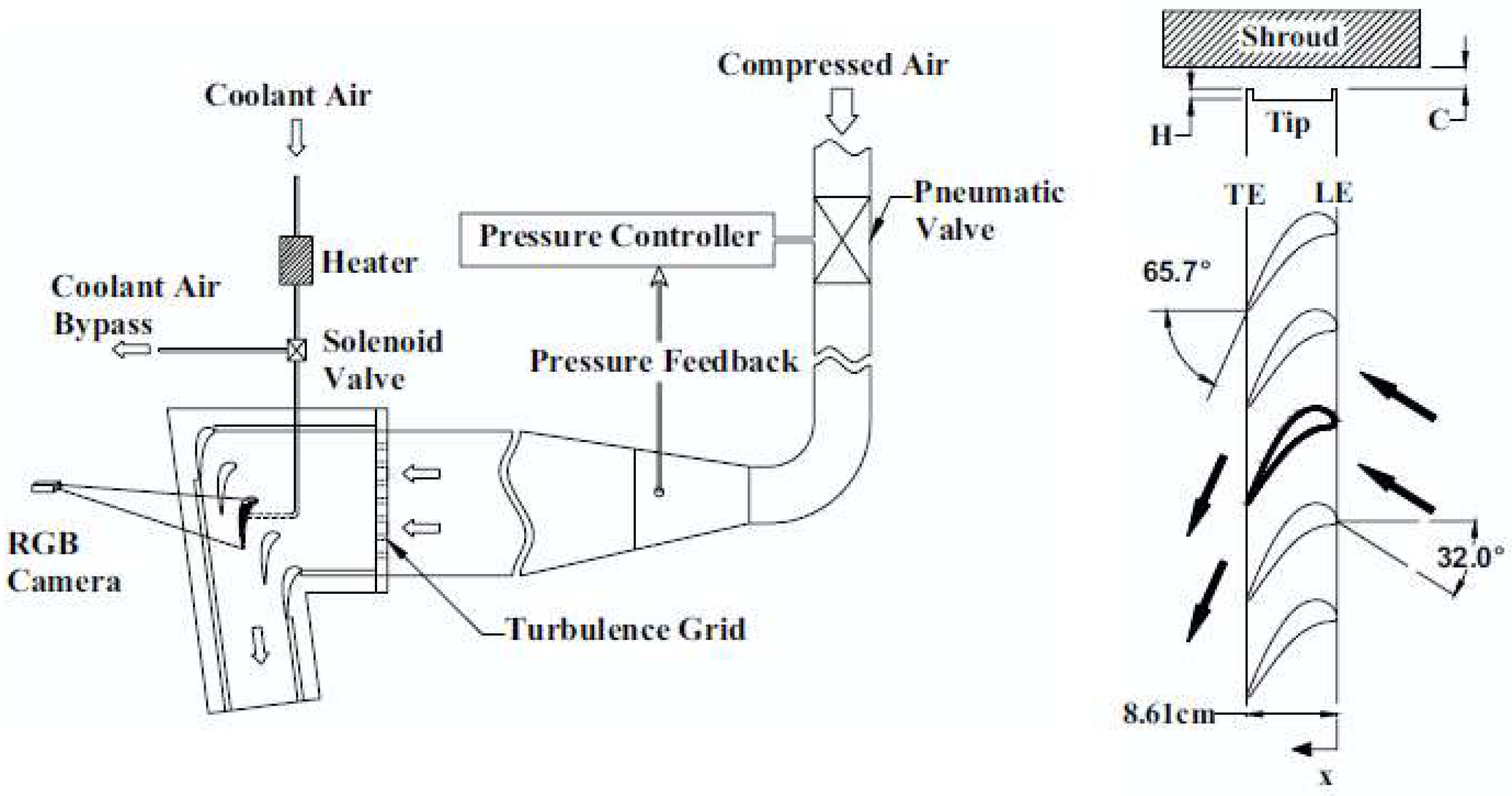
2. External Cooling on Flat Tip
2.1. Literature Review
2.2. Summary and Discussion
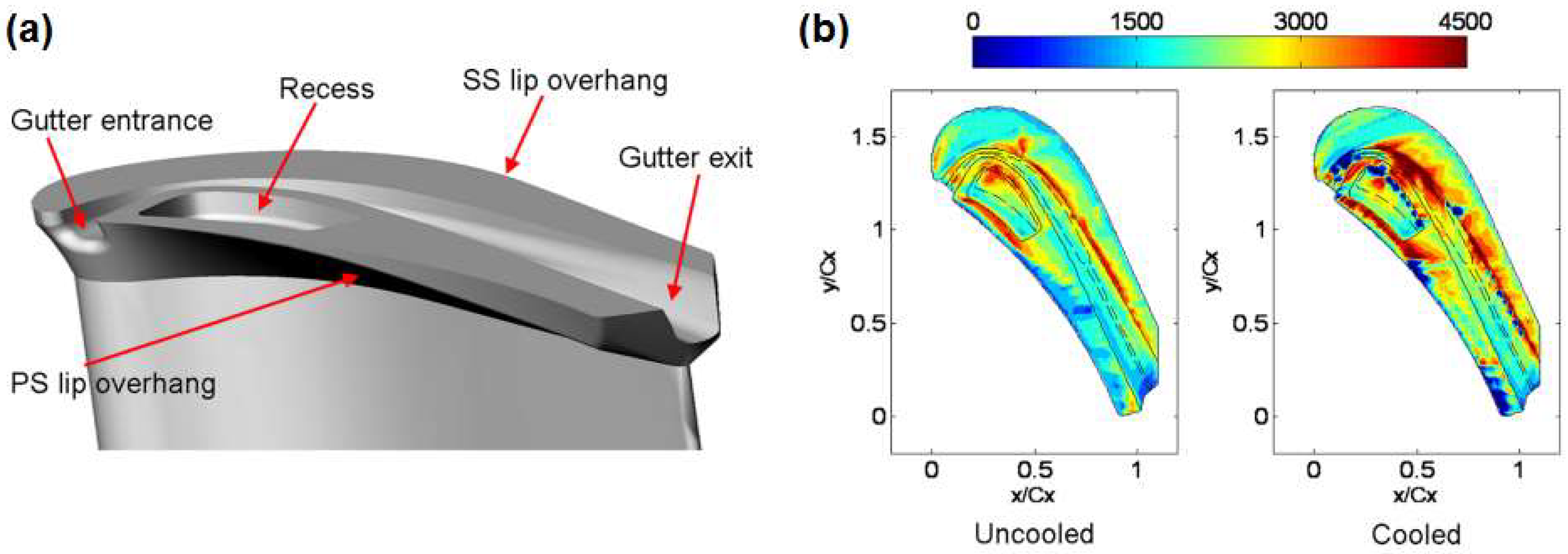
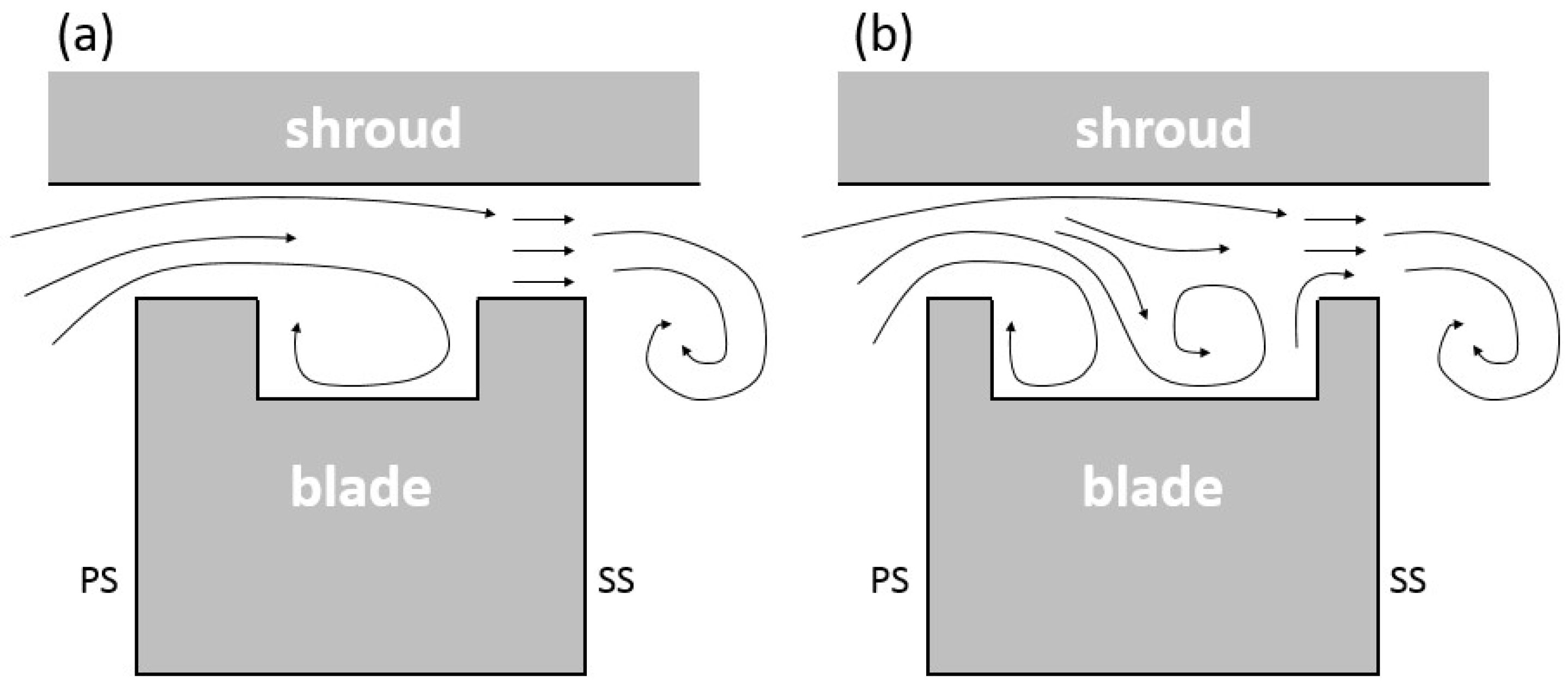
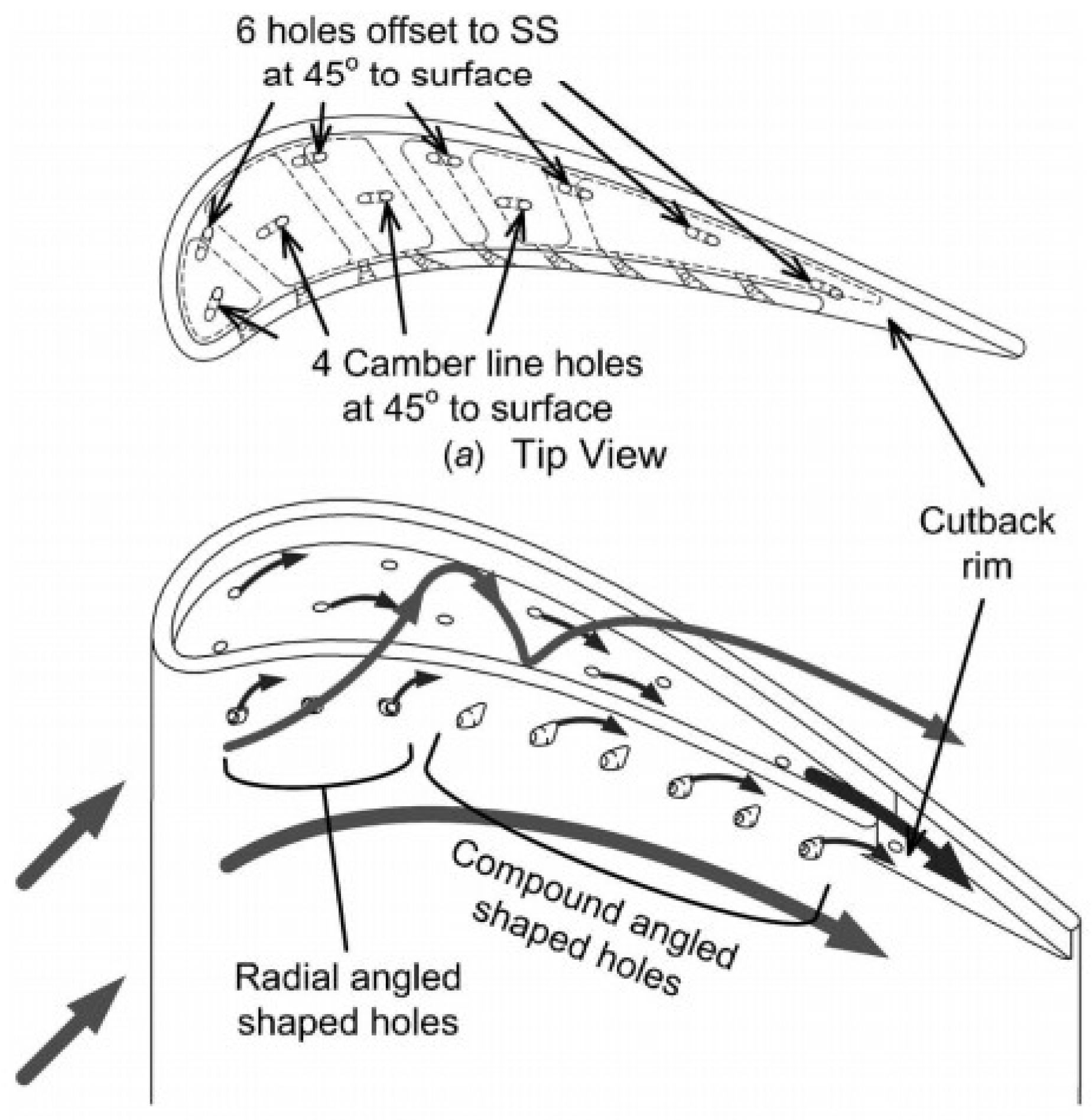
3. External Cooling on Squealer Tip
3.1. Literature Review
3.2. Summary and Discussion
- The rim on both the suction and pressure side are usually the most vulnerable parts to thermal damage;
- On the cavity floor, it is common to see the local high HTC near the leading edge region and the suction side region, which is mainly due to the OTL flow reattachment;
- Increase of cavity depth usually results in reduction of OTL flow rate, as well as reduction of cavity floor heat transfer;
- Comparing to the squealer tip, flat tip injection may perform better blocking effects to control the OTL. Better cooling performance may be achieved in flat tip without extra aerodynamic penalty.
4. New Techniques in Turbine Tip Cooling Design
4.1. Optimization Algorithms in Turbine Tip Cooling Optimization
4.2. Artificial Neural Network in Turbine Tip Cooling Performance Prediction
4.3. Summary and Discussions
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Alphabet | |
| Cx | axial chord [m] |
| d | flow channel width [m] |
| h | activation function in neuron network [-] |
| L | flow channel length [m] |
| M | cooling blowing ratio [-] |
| P | pressure [Pa] |
| Re | Reynolds number [-] |
| s | cascade pitch [m] |
| u | velocity [m/s] |
| x | axial distance from leading edge [m] |
| W | weight coefficient in neuron network [-] |
| Z | neuron note value [-] |
| Greek letters | |
| α | flow angle at leading and trailing edge [degree] |
| λ | reciprocal of Reynolds number [-] |
| μ | dynamic viscosity [kg/(m·s)] |
| ρ | density [kg/m3] |
| ψz | Zweifel coefficient [-] |
| Subscript | |
| 1 | cascade inlet |
| 2 | cascade exit |
| c | coolant flow condition |
| i | layer position of neuron network |
| j | note position in one neuron layer |
| k | note position in one neuron layer |
| LE | blade leading edge |
| m | total note number of each layer |
| OTL | over tip leakage flow condition |
| PS | pressure side |
| SS | suction side |
| s | static condition |
| TE | blade trailing edge |
| t | stagnation condition |
| ∞ | main flow condition |
References
- Denton, J.D. Loss Mechanisms in Turbomachines. ASME J. Turbomach. 1993, 115, 621–656. [Google Scholar] [CrossRef]
- Yaras, M.; Zhu, Y.; Sjolander, S.A. Flow Field in the Tip Gap of a Planar Cascade of Turbine Blades. J. Turbomach. 1989, 111, 276–283. [Google Scholar] [CrossRef]
- Heyes, F.J.G.; Hodson, H.P.; Dailey, G.M. The effect of blade tip geometry on the tip leakage flow in axial turbine cascades. ASME J. Turbomach. 1992, 114, 643–651. [Google Scholar] [CrossRef]
- Ameri, A.A. Heat Transfer and Flow on the Blade Tip of a Gas Turbine Equipped with a Mean Camberline Strip. ASME J. Turbomach. 2001, 123, 704–708. [Google Scholar] [CrossRef]
- Harvey, N.W.; Newman, D.A.; Haselbach, F.; Willer, L. An Investigation into a Novel Turbine Rotor Winglet: Part 1—Design and Model Rig Test Results. In Proceedings of the ASME Turbo Expo 2006: Power for Land, Sea, and Air, Barcelona, Spain, 8–11 May 2006; pp. 585–596. [Google Scholar]
- Schabowski, Z.; Hodson, H. The Reduction of Over Tip Leakage Loss in Unshrouded Axial Turbines Using Winglets and Squealers. ASME J. Turbomach. 2013, 136, 041001. [Google Scholar] [CrossRef]
- Bunker, R.S. A Review of Turbine Blade Tip Heat Transfer. In Proceedings of the Turbine 2000 International Symposium on Heat Transfer in Gas Turbine Systems, Izmir, Turkey, 13–18 August 2000. [Google Scholar]
- Mayle, R.E.; Metzger, D.E. Heat transfer at the tip of an unshrouded turbine blade. In Proceedings of the Seventh International Conference, Munich, Germany, 6–10 September 1982; Volume 3. [Google Scholar]
- Rezasoltani, M.; Lu, K.; Schobeiri, M.T.; Han, J.-C. A Combined Experimental and Numerical Study of the Turbine Blade Tip Film Cooling Effectiveness under Rotation Condition. ASME J. Turbomach. 2015, 137, 051009. [Google Scholar] [CrossRef]
- Thorpe, S.J.; Yoshino, S.; Thomas, G.A.; Ainsworth, R.W.; Harvey, N.W. Blade-Tip Heat Transfer in a Transonic Turbine. Proc. Inst. Mech. Eng. Part A 2005, 421–430. [Google Scholar] [CrossRef]
- Bunker, R.S.; Baily, J.C.; Ameri, A.A. Heat Transfer and Flow on the First Stage Blade Tip of a Power Generation Gas Turbine: Part l: Experimental Results. J. Turbomach. 1999, 122, 272–277. [Google Scholar]
- Ekkad, S.V.; Han, J. A Transient Liquid Crystal Thermography Technique for Gas Turbine Heat Transfer Measurements. Meas. Sci. Technol. 2000, 11, 957–968. [Google Scholar] [CrossRef]
- Xue, S.; Roy, A.; Ng, W.F.; Ekkad, S.V. A Novel Transient Technique to Determine Recovery Temperature, Heat Transfer Coefficient, and Film Cooling Effectiveness Simultaneously in a Transonic Turbine Cascade. ASME J. Therm. Sci. Eng. Appl. 2015, 7, 011016. [Google Scholar] [CrossRef]
- Arisi, A.; Phillips, J.; Ng, W.F.; Xue, S.; Moon, H.K.; Zhang, L. An Experimental and Numerical Study on the Aerothermal Characteristics of a Ribbed Transonic Squealer-Tip Turbine Blade With Purge Flow. ASME J. Turbomach. 2016, 138, 101007. [Google Scholar] [CrossRef]
- O’Dowd, D.O.; Zhang, Q.; He, L.; Ligrani, P.M.; Friedrichs, S. Comparison of Heat Transfer Measurement Techniques on a Transonic Turbine Blade Tip. ASME J. Turbomach. 2010, 133, 021028. [Google Scholar] [CrossRef]
- Mumic, F.; Eriksson, D.; Sunden, B. On Prediction of Tip Leakage Flow and Heat Transfer in Gas Turbines. In Proceedings of the ASME Turbo Expo 2004 Power for Land, Sea, and Air, Vienna, Austria, 14–17 June 2004. [Google Scholar]
- Mumic, F.; Eriksson, D.; Sunden, B. A Numerical Investigation of Tip Leakage Heat Transfer and Fluid Flow for a Gas Turbine Rotor Blade. In Proceedings of the 4th European Thermal Sciences Conference, Birmingham, UK, 29–31 March 2004. [Google Scholar]
- Yang, H.T.; Chen, H.C.; Han, J.C. Turbine Rotor with Various Tip Configurations Flow and Heat Transfer Prediction. AIAA J. Thermophys. Heat Transf. 2006, 22, 201–209. [Google Scholar] [CrossRef]
- Mischo, B.; Behr, T.; Abhari, R.S. Flow Physics and Profiling of Recessed Blade Tips—Impact on Performance and Heat Load. ASME J. Turbomach. 2008, 130, 021008. [Google Scholar] [CrossRef]
- Park, C.W.; Lau, S.C. Effect of Channel Orientation of Local Heat (Mass) Transfer Distributions in a Rotating Two- Pass Square Channel with Smooth Walls. ASME J. Heat Transf. 1998, 120, 624–632. [Google Scholar] [CrossRef]
- Park, C.W.; Kandis, M.; Lau, S.C. Heat/Mass Transfer Distribution in a Rotating Two-Pass Square Channel, Part I: Regional Heat Transfer, Smooth Channel. Int. J. Rotat. Mach. 1998, 4, 175–188. [Google Scholar] [CrossRef]
- Park, C.W.; Kukreja, R.T.; Lau, S.C. Heat/Mass Transfer Distribution in a Rotating Two-Pass Channel with Transverse Ribs. AIAA J. Thermophys. Heat Transf. 1998, 12, 80–86. [Google Scholar] [CrossRef]
- Park, C.W.; Kukreja, R.T.; Lau, S.C. Heat/Mass Transfer Distribution in a Rotating Two-Pass Channel with Angled Ribs. Int. J. Rotat. Mach. 1999, 5, 1–16. [Google Scholar] [CrossRef]
- Park, C.W.; Yoon, C.; Lau, S.C. Heat (Mass) Transfer in a Diagonally Oriented Rotating Two-Pass Channel with Rib- Roughened Walls. ASME J. Heat Transf. 2000, 122, 208–211. [Google Scholar] [CrossRef]
- Kukreja, R.T.; Park, C.W.; Lau, S.C. Heat/Mass Transfer Distribution in a Rotating Two-Pass Square Channel, Part II: Local Transfer Coefficient, Smooth Channel. Int. J. Rotat. Mach. 1998, 4, 1–15. [Google Scholar] [CrossRef]
- Lee, S.W.; Ahn, H.S.; Lau, S.C. Heat (Mass) Transfer Distribution in a Two-Pass Trapezoidal Channel with a 180-Deg Turn. ASME J. Heat Transf. 2007, 129, 1529–1537. [Google Scholar] [CrossRef]
- Mochizuki, S.; Murata, A.; Shibata, R.; Yang, J.W. Detailed Measurements of Local Heat Transfer Coefficients in Turbulent Flow through Smooth and Rib-Roughened Serpentine Passages with a 180° Sharp Bend. Int. J. Heat Mass Transf. 1999, 42, 1925–1934. [Google Scholar] [CrossRef]
- Al-Hadhrami, L.; Griffith, T.; Han, J.C. Heat Transfer in Two-Pass Rotating Rectangular Channels (AR = 2) with Five Different Orientations of 45 Deg V-Shaped Rib Turbulators. ASME J. Heat Transf. 2003, 125, 232–242. [Google Scholar] [CrossRef][Green Version]
- Kim, K.M.; Lee, D.H.; Cho, H.H. Detailed Measurement of Heat–Mass Transfer and Pressure Drop in a Rotating Two-Pass Duct with Transverse Ribs. Heat Mass Transf. 2007, 43, 801–815. [Google Scholar] [CrossRef]
- Kim, K.M.; Lee, D.H.; Rhee, D.H.; Cho, H.H. Local Heat/Mass Transfer Phenomena in Rotating Passage, Part 1: Smooth Passage. AIAA J. Thermophys. Heat Transf. 2006, 20, 188–198. [Google Scholar] [CrossRef]
- Kim, K.M.; Lee, D.H.; Rhee, D.H.; Cho, H.H. Local Heat/Mass Transfer Phenomena in Rotating Passage, Part 2: Angled Ribbed Passage. AIAA J. Thermophys. Heat Transf. 2006, 20, 199–210. [Google Scholar] [CrossRef]
- Kim, K.M.; Lee, D.H.; Cho, H.H. Rotational Effects on Pressure Drop in Smooth and Ribbed Two-Pass Ducts. AIAA J. Thermophys. Heat Transf. 2007, 21, 644–647. [Google Scholar] [CrossRef]
- Sunden, B.; Xie, G. Gas Turbine Blade Tip Heat Transfer and Cooling: A Literature Survey. Heat Transf. Eng. 2010, 31, 527–554. [Google Scholar] [CrossRef]
- Goldstein, R.J.; Eckert, E.R.G.; Burggraf, F. Effects of Hole Geometry and Density on Three Dimensional Film Cooling. Int. J. Heat Mass Transf. 1974, 17, 595–607. [Google Scholar] [CrossRef]
- Kim, Y.W.; Metzger, D.E. Heat Transfer and Effectiveness on Film Cooled Turbine Blade Tip Models. ASME J. Turbomach. 1995, 117, 13–21. [Google Scholar] [CrossRef]
- Ameri, A.A.; Rigby, D.L. A Numerical Analysis of Heat Transfer and Effectiveness on Film Cooled Turbine Blade Tip Models. In Proceedings of the 4th Air Breathing Engines, Florence, Italy, 5–10 September 1999. [Google Scholar]
- Acharya, S.; Yang, H.; Ekkad, S.V.; Prakash, C.; Bunker, R. Numerical Simulation of Film Cooling on the Tip of a Gas Turbine Blade. In Proceedings of the ASME TURBO EXPO, Amsterdam, The Netherlands, 3–6 June 2002. [Google Scholar]
- Christophel, J.R. Comparison of the Thermal Performance of Several Tip Cooling Designs for a Turbine Blade. Master’s Thesis, Faculty of Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2003. [Google Scholar]
- Christophel, J.R.; Couch, E.; Thole, K.A.; Cunha, F.J. Measured Adiabatic Effectiveness and Heat Transfer for Blowing from the Tip of a Turbine Blade. ASME J. Turbomach. 2005, 127, 251–262. [Google Scholar] [CrossRef]
- Christophel, J.R.; Thole, K.A.; Cunha, F.J. Cooling the Tip of a Turbine Blade Using Pressure Side Holes—Part I: Adiabatic Effectiveness Measurements. ASME J. Turbomach. 2005, 127, 270–277. [Google Scholar] [CrossRef]
- Christophel, J.R.; Thole, K.A.; Cunha, F.J. Cooling the Tip of a Turbine Blade Using Pressure Side Holes—Part II: Heat Transfer Measurements. ASME J. Turbomach. 2005, 127, 278–286. [Google Scholar] [CrossRef][Green Version]
- Hohlfeld, Ε.M.; Christophel, J.R.; Couch, E.L.; Thole, K.A. Predictions of Cooling from Dirt Purge Holes along the Tip of a Turbine Blade. Int. J. Turbo Jet Eng. 2005, 22, 139–152. [Google Scholar] [CrossRef]
- Nasir, H.; Ekkad, S.V.; Bunker, R.S. Effect of Tip and Pressure Side Coolant Injection on Heat Transfer Distributions for a Plane and Recessed Tip. ASME J. Turbomach. 2005, 129, 151–163. [Google Scholar] [CrossRef]
- Ahn, J.; Mhetras, S.; Han, J.-C. Film-Cooling Effectiveness on a Gas Turbine Blade Tip Using Pressure-Sensitive Paint. ASME J. Heat Transf. 2005, 127, 521–530. [Google Scholar] [CrossRef]
- Newton, P.J.; Lock, G.D.; Krishnababu, S.K.; Hodson, H.P.; Dawes, W.N.; Hannis, J.; Whitney, C. Aerothermal Investigations of Tip Leakage Flow in Axial Flow Turbines—Part III: TIP Cooling. ASME J. Turbomach. 2009, 131, 011008. [Google Scholar] [CrossRef]
- Rhee, D.; Cho, H.H. Local Heat/Mass Transfer Characteristics on a Rotating Blade with Flat Tip in Low-Speed Annular Cascade-Part I: Near-Tip Surface. ASME J. Turbomach. 2006, 128, 96–109. [Google Scholar] [CrossRef]
- Rhee, D.; Cho, H.H. Local Heat/Mass Transfer Characteristics on a Rotating Blade with Flat Tip in Low-Speed Annular Cascade-Part II: Tip and Shroud. In Proceedings of the ASME Turbo Expo 2005: Power for Land, Sea and Air, Reno-Tahoe, NV, USA, 6–9 June 2005. [Google Scholar]
- O’Dowd, D.O.; Zhang, Q.; He, L.; Cheong, B.C.Y.; Tibbott, I. Aerothermal Performance of a Cooled Winglet at Engine Representative Mach and Reynolds Numbers. ASME J. Turbomach. 2013, 135, 011041. [Google Scholar] [CrossRef]
- Kwak, J.S.; Han, J.-C. Heat Transfer Coefficient and Film-Cooling Effectiveness on the Squealer Tip of a Gas Turbine Blade. In Proceedings of the ASME TURBO EXPO, Amsterdam, The Netherlands, 3–6 June 2002. [Google Scholar]
- Yang, H.; Chen, H.-C.; Han, J.-C. Film-Cooling Prediction on Turbine Blade Tip with Various Film Hole Configurations. J. Thermophys. Heat Transf. 2006, 20, 558–568. [Google Scholar] [CrossRef]
- Mhetras, S.; Narzary, D.; Gao, Z.; Han, J.-C. Effect of a Cutback Squealer and Cavity Depth on Film-Cooling Effectiveness on a Gas Turbine Blade Tip. ASME J. Turbomach. 2008, 130, 021002. [Google Scholar] [CrossRef]
- Schlichting, H. Boundary-Layer Theory; McGraw-Hill, Inc.: New York, NY, USA, 1955. [Google Scholar]
- Horlock, J.H. Axial Flow Turbines: Fluid Mechanics and Thermodynamics; Butterworth: Woburn, MA, USA, 1966. [Google Scholar]
- Wang, Z.; Zhang, Q.; Liu, Y.; He, L. Impact of Cooling Injection on the Transonic Over-Tip Leakage Flow and Squealer Aerothermal Design Optimization. ASME J. Eng. Gas Turbines Power 2015, 137, 062603. [Google Scholar] [CrossRef]
- Gao, Z.; Narzary, D.; Mhetras, S.; Han, J.-C. Effect of Inlet Flow Angle on Gas Turbine Blade Tip Film Cooling. ASME J. Turbomach. 2009, 131, 031005. [Google Scholar] [CrossRef]
- Narzary, D.P. Experimental Study of Gas Turbine Blade Film Cooling and Heat Transfer. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2009. [Google Scholar]
- Ma, H.; Zhang, Q.; He, L.; Wang, Z.; Wang, L. Cooling Injection Effect on a Transonic Squealer Tip—Part I: Experimental Heat Transfer Results and CFD Validation. ASME J. Eng. Gas Turbines Power 2017, 139, 052506. [Google Scholar] [CrossRef]
- Ma, H.; Zhang, Q.; He, L.; Wang, Z.; Wang, L. Cooling Injection Effect on a Transonic Squealer Tip—Part 2: Analysis of Aerothermal Interaction Physics. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Korea, 13–17 June 2016. [Google Scholar]
- Park, J.S.; Lee, D.H.; Rhee, D.-H.; Kang, S.H.; Cho, H.H. Heat transfer and film cooling effectiveness on the squealer tip of a turbine blade. Energy 2014, 72, 331–343. [Google Scholar] [CrossRef]
- Tong, F.; Gou, W.; Li, L.; Liu, Q.; Yue, Z.; Xie, G. Investigation on heat transfer of a rotor blade tip with various film cooling holes arrangements and groove depths. Adv. Mech. Eng. 2015. [Google Scholar] [CrossRef]
- Wang, J.; Sundén, B.; Zeng, M.; Wang, Q. Film cooling effects on the tip flow characteristics of a gas turbine blade. Propuls. Power Res. 2015, 4, 9–22. [Google Scholar] [CrossRef]
- Tamunobere, O.; Acharya, S. Turbine Blade Tip Film Cooling with Blade Rotation—Part I: Tip and Pressure Side Coolant Injection. ASME J. Turbomach. 2016, 138, 091002. [Google Scholar] [CrossRef]
- Yan, X.; Huang, Y.; He, K. Investigations into heat transfer and film cooling effect on a squealer-winglet blade tip. Int. J. Heat Mass Transf. 2017, 115, 955–978. [Google Scholar] [CrossRef]
- Bucchieri, G.; Galbiati, M.; Coutandin, D.; Zecchi, S. Optimization techniques applied to the design of gas turbine blades cooling systems. In Proceedings of the ASME Turbo Expo 2006: Power for Land, Sea and Air, Barcelona, Spain, 8–11 May 2006. [Google Scholar]
- Deveci, K.; Maral, H.; Şenel, C.B.; Alpman, E.; Kavurmacıoğlu, L.; Camcı, C. Aerothermal Optimization of Squealer Geometry in Axial Flow Turbines Using Genetic Algorithm. J. Therm. Eng. 2018, 4, 1896–1911. [Google Scholar]
- Muller, S.D.; Walther, J.H.; Koumoutsakos, P.D. Evolution Strategies for Film Cooling Optimization. Technical Note. AIAA J. 2000, 39, 537–539. [Google Scholar] [CrossRef]
- Favaretto, C.F.F.; Funazaki, K. Application of Genetic Algorithms to Design of an Internal Turbine Cooling System. In Proceedings of the ASME Turbo Expo 2003, Atlanta, GA, USA, 16–19 June 2003. [Google Scholar]
- Dennis, B.H.; Egorov, I.N.; Dulikravich, G.S.; Yoshimura, S. Optimization of a Large Number of Coolant Passages Located Close to the Surface of a Turbine Blade. In Proceedings of the ASME Turbo Expo 2003, Atlanta, GA, USA, 16–19 June 2003. [Google Scholar]
- El-Ayoubi, C.; Ghaly, W.; Hassan, I. Optimization of Film Cooling Holes on the Suction Side of a High Pressure Turbine Blade. In Proceedings of the ASME Turbo Expo 2012, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Johnson, J.J. Genetic Algorithm Optimization of a Film Cooling Array on a Modern Turbine Inlet Vane. Ph.D. Thesis, Department of the Air Force, Air University, Montgomery, AL, USA, 2012. [Google Scholar]
- Yang, L.; Min, Z.; Parbat, S.N.; Chyu, M.K. Optimization of Hybrid-Linked Jet Impingement Cooling Channels Based on Response Surface Methodology and Genetic Algorithm. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar]
- Guo, H.; Ahdad, F.; Guo, W.; Yu, H. Heat Transfer Investigation on Center Housing Using Genetic Algorithms and Finite Element Method. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar]
- Maesschalck, C.D.; Paniagua, G. Turbine Tip Flow Control through Upstream Purge Cooling Optimization. In Proceedings of the 53rd AIAA/SAE/ASEE Joint Propulsion Conference, Atlanta, GA, USA, 10–12 July 2017. [Google Scholar]
- Kim, J.; Kang, Y.S.; Kim, D.; Lee, J.; Cha, B.J.; Cho, J. Optimization of a high pressure turbine blade tip cavity with conjugate heat transfer analysis. J. Mech. Sci. Technol. 2016, 30, 5529–5538. [Google Scholar] [CrossRef]
- Eryilmaz, I.; Inanli, S.; Gumusel, B.; Toprak, S.; Camci, C. Application of Artificial Neural Network for the Heat Transfer Investigation around a High-Pressure Gas Turbine Rotor Blade. In Proceedings of the ASME Turbo Expo 2011, Vancouver, BC, Canada, 6–10 June 2011. [Google Scholar]
- Ostanek, J.K. Improving Pin-Fin Heat Transfer Predictions Using Artificial Neural Networks. In Proceedings of the ASME Turbo Expo 2013, San Antonio, TX, USA, 3–7 June 2013. [Google Scholar]
- Dávalos, J.O.; García, J.C.; Urquiza, G.; Huicochea, A.; De Santiago, O. Prediction of Film Cooling Effectiveness on a Gas Turbine Blade Leading Edge Using ANN and CFD. Int. J. Turbo Jet Eng. 2016, 35. [Google Scholar] [CrossRef]
- Boccaletti, C.; Cerri, G.; Seyedan, B. A Neural Network Simulator of a Gas Turbine with a Waste Heat Recovery Section. In Proceedings of the ASME TURBOEXPO 2000, Munich, Germany, 8–11 May 2000. [Google Scholar]





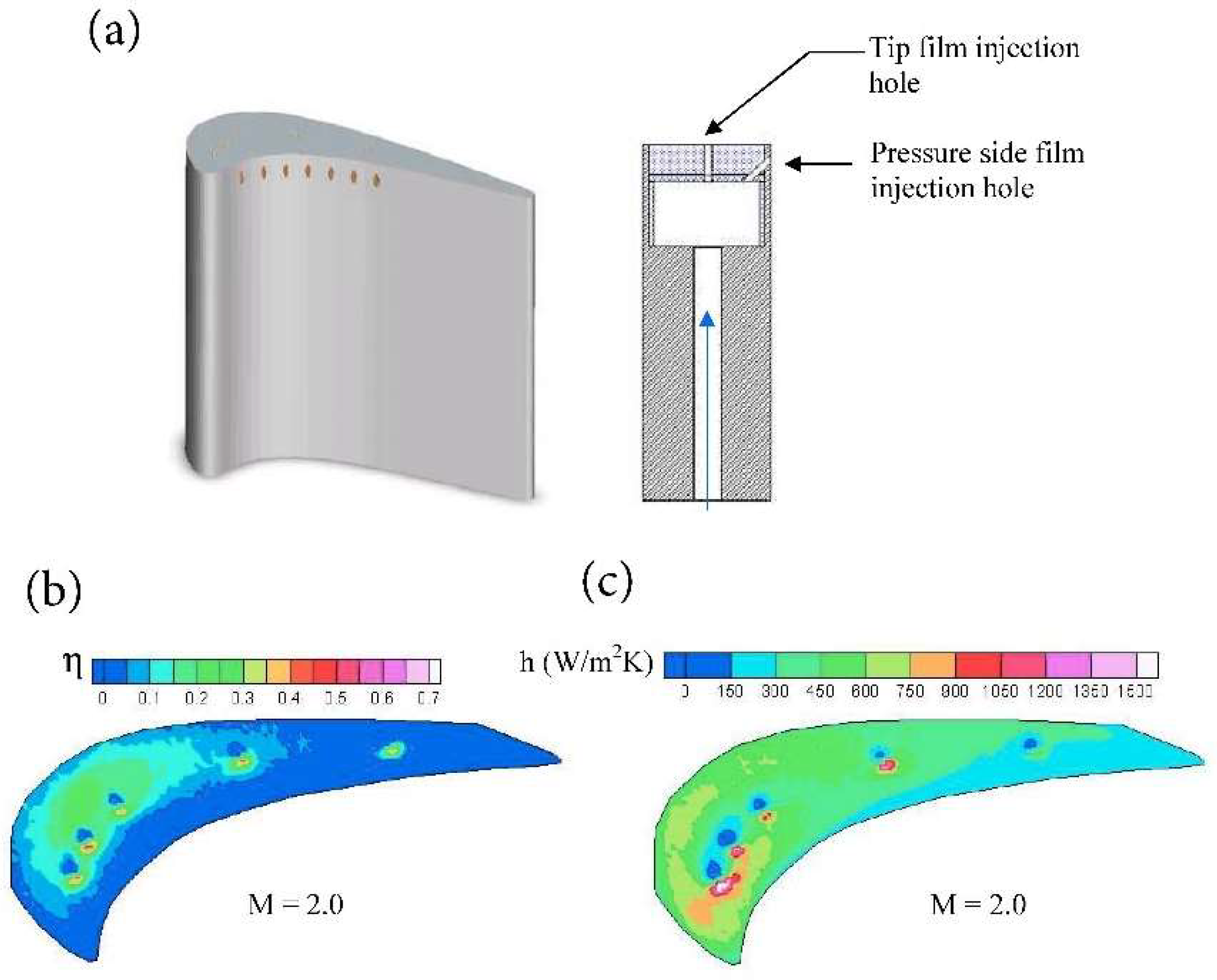
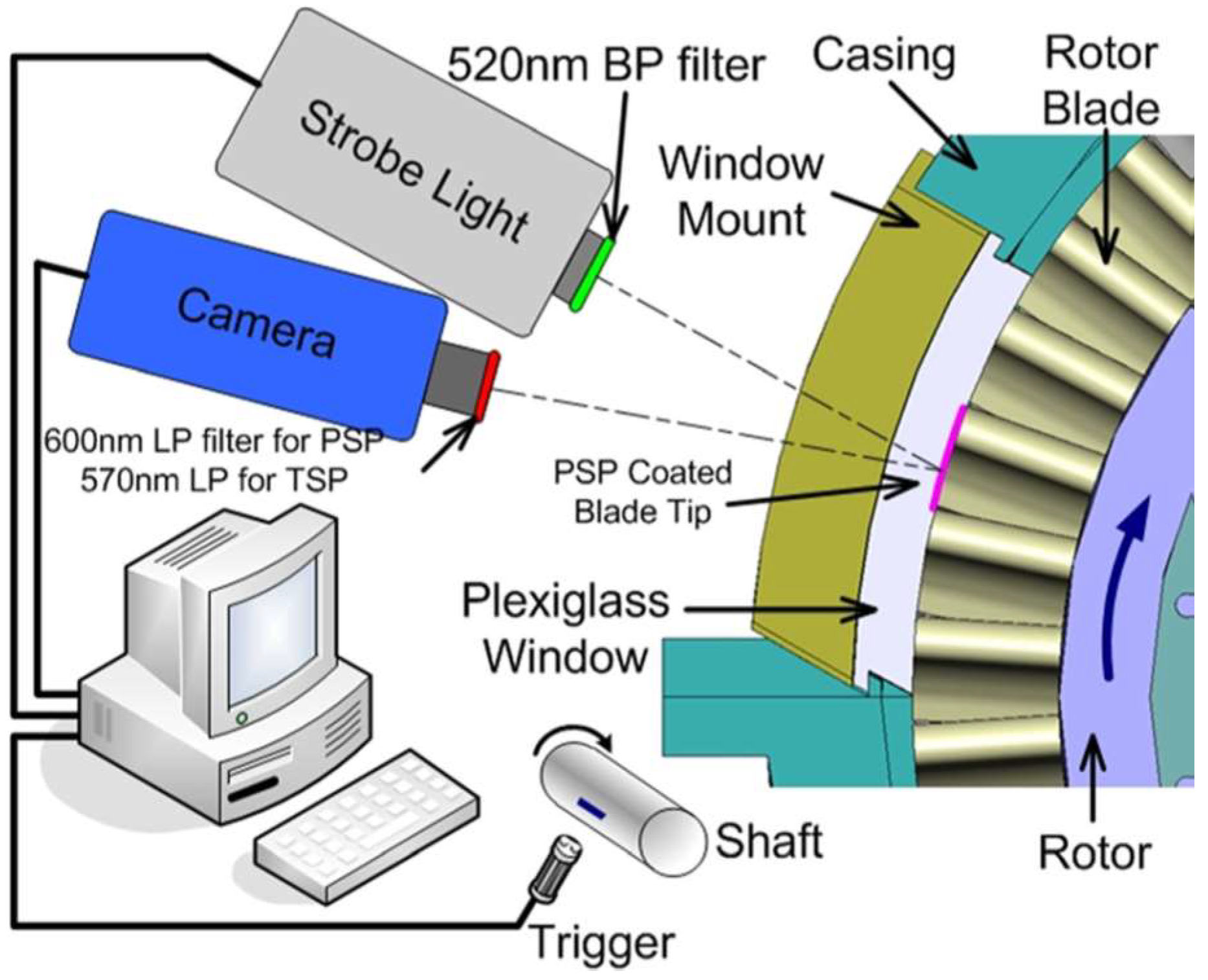
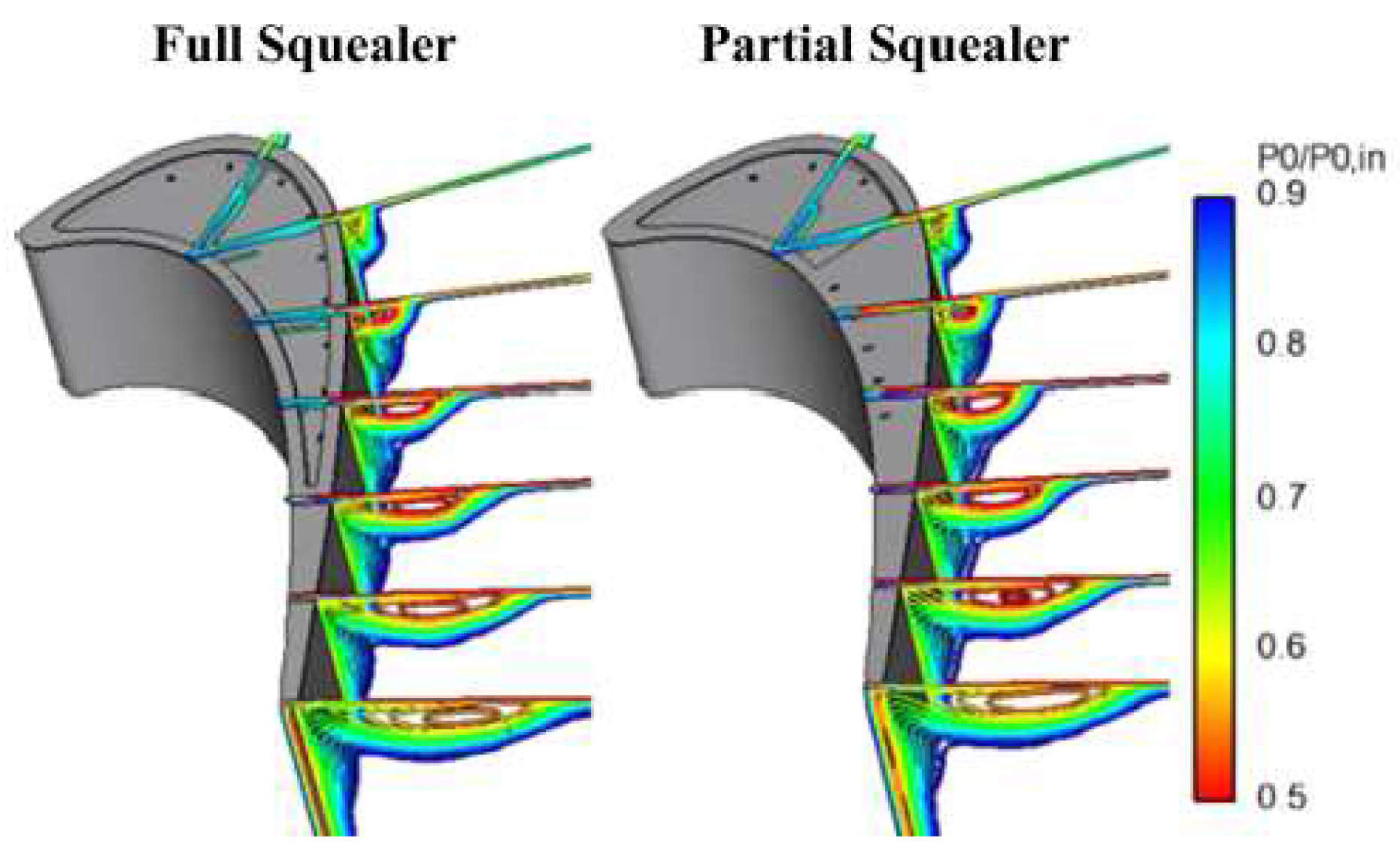
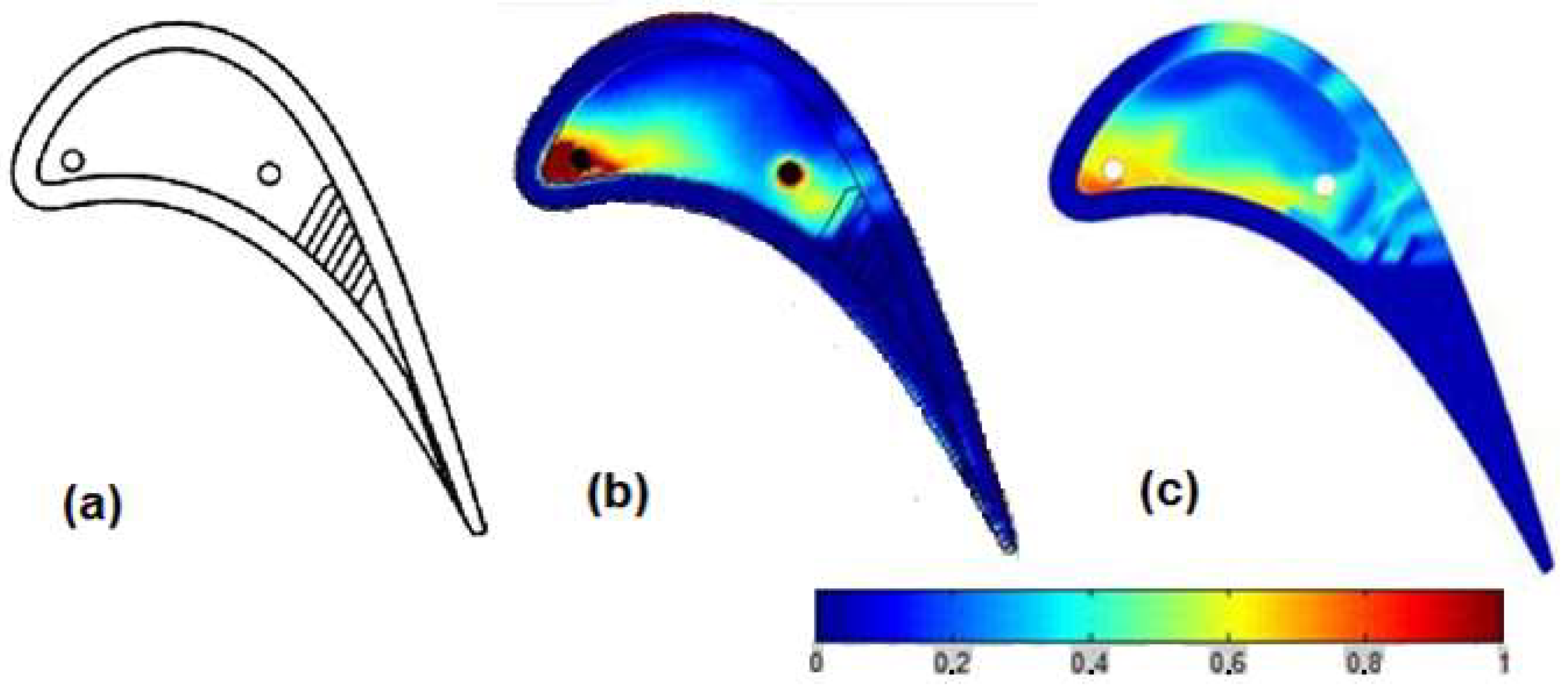
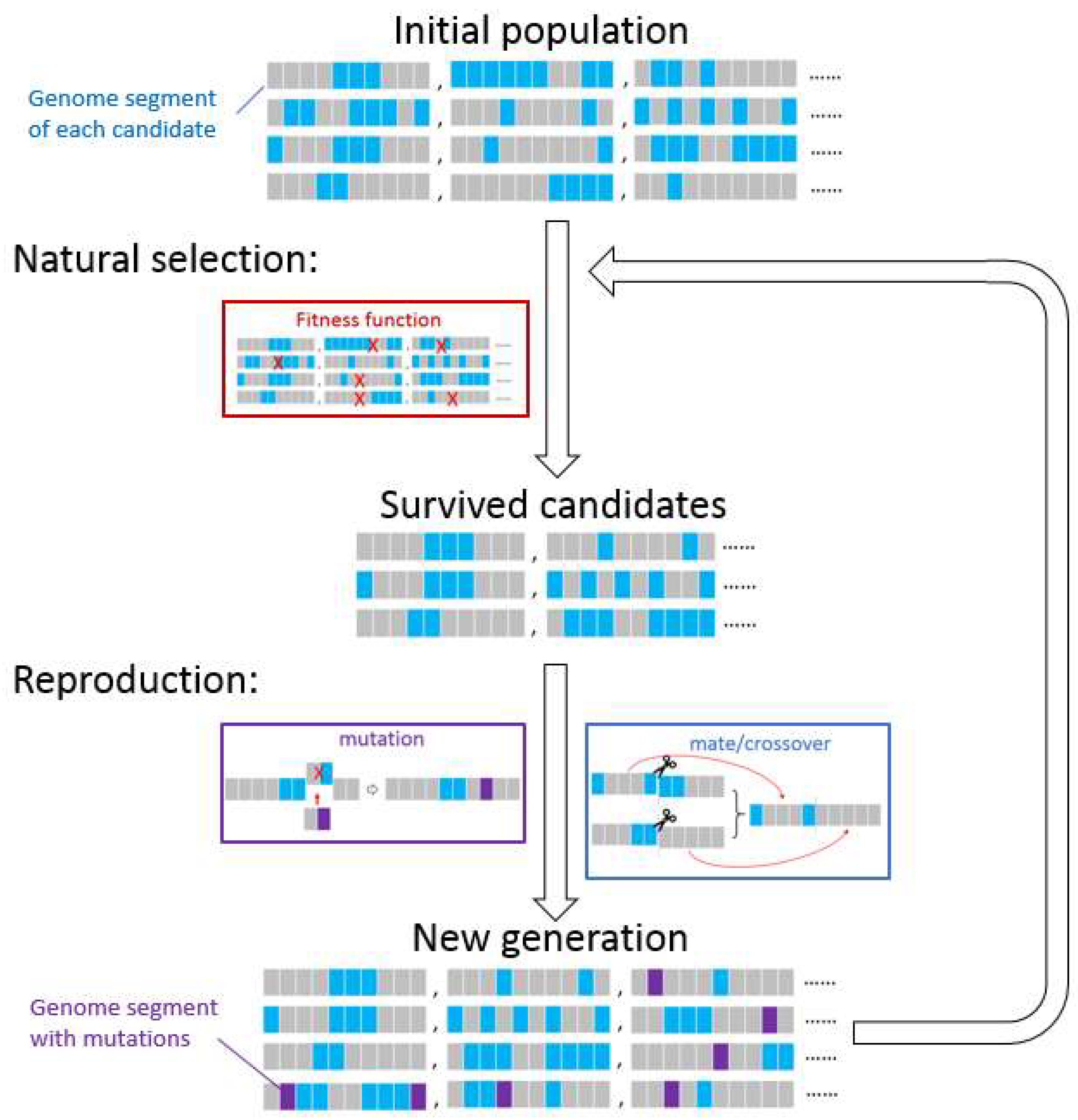
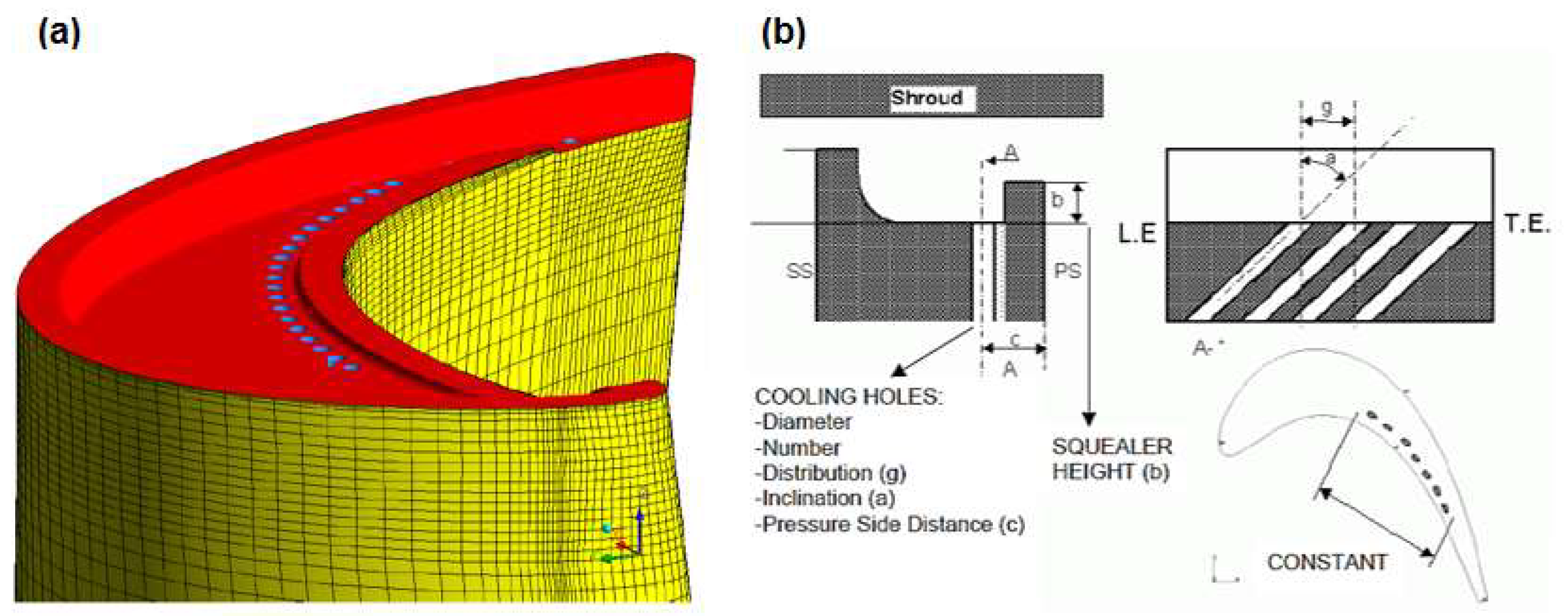

| Reference | Technique | Hole Number | Tip Clearance | Inject Location | M | Eta | HTC | NHFR | Note |
|---|---|---|---|---|---|---|---|---|---|
| Kwak 2002 [49] | Liquid crystal | 13 | 1.50% | Camber | 2 | 0.056 | * | ||
| 26 | 1.50% | Camber + PS_near tip | 2 | 0.069 | 534 | * | |||
| Acharya 2002 [37] | CFD | 8 | 1.0% | Tip near PS | 1.00 | 0.251 | * | ||
| 8 | 1.5% | Tip near PS | 1.00 | 0.311 | 876 | * | |||
| 8 | 2.5% | Tip near PS | 1.00 | 0.303 | * | ||||
| Christophel 2003 [38] | IR | 13 | 0.5% | LE(purge) + PS near tip | 0.58% | 0.618 | 1.10 | EDM holes | |
| 13 | 0.5% | LE(purge) + PS near tip | 0.68% | 0.699 | EDM holes | ||||
| 13 | 0.5% | LE(purge) + PS near tip | 1.00% | 0.679 | 1.28 | EDM holes | |||
| 13 | 1.6% | LE(purge) + PS near tip | 0.58% | 0.268 | 0.40 | EDM holes | |||
| 13 | 1.6% | LE(purge) + PS near tip | 0.68% | 0.299 | EDM holes | ||||
| 13 | 1.6% | LE(purge) + PS near tip | 1.00% | 0.498 | 0.85 | EDM holes | |||
| 13 | 0.5% | LE(purge) + PS near tip | 0.47% | 0.599 | Laser holes | ||||
| 13 | 0.5% | LE(purge) + PS near tip | 0.58% | 0.597 | 1.10 | Laser holes | |||
| 13 | 0.5% | LE(purge) + PS near tip | 0.68% | 0.649 | Laser holes | ||||
| 13 | 0.5% | LE(purge) + PS near tip | 1.00% | 0.708 | 1.51 | Laser holes | |||
| 13 | 1.6% | LE(purge) + PS near tip | 0.47% | 0.290 | Laser holes | ||||
| 13 | 1.6% | LE(purge) + PS near tip | 0.58% | 0.321 | 0.49 | Laser holes | |||
| 13 | 1.6% | LE(purge) + PS near tip | 0.68% | 0.349 | Laser holes | ||||
| 13 | 1.6% | LE(purge) + PS near tip | 1.00% | 0.487 | 0.88 | Laser holes | |||
| Nasir 2004 [43] | Liquid crystal | 5 | 1.0% | Camber | 1 | 0.045 | 620 | * | |
| 5 | 1.0% | Camber | 2 | 0.060 | 562 | * | |||
| 5 | 1.0% | Camber | 3 | 0.043 | 526 | * | |||
| 12 | 1.0% | Camber + PS near tip | 1 | 0.107 | 524 | * | |||
| 12 | 1.0% | Camber + PS near tip | 2 | 0.094 | 431 | * | |||
| 12 | 1.0% | Camber + PS near tip | 3 | 0.237 | 708 | * | |||
| 7 | 1.0% | PS near tip | 1 | 0.058 | 621 | * | |||
| 7 | 1.0% | PS near tip | 2 | 0.030 | 500 | * | |||
| 7 | 1.0% | PS near tip | 3 | 0.051 | 575 | * | |||
| Ahn 2005 [44] | PSP | 7 | 1.00% | Camber | 0.5 | 0.019 | |||
| 7 | 1.00% | Camber | 1 | 0.023 | |||||
| 7 | 1.00% | Camber | 2 | 0.022 | |||||
| 7 | 1.50% | Camber | 0.5 | 0.013 | |||||
| 7 | 1.50% | Camber | 1 | 0.021 | |||||
| 7 | 1.50% | Camber | 2 | 0.022 | |||||
| 7 | 2.50% | Camber | 0.5 | 0.006 | |||||
| 7 | 2.50% | Camber | 1 | 0.011 | |||||
| 7 | 2.50% | Camber | 2 | 0.026 | |||||
| 7 | 1.00% | PS near tip | 0.5 | 0.005 | |||||
| 7 | 1.00% | PS near tip | 1 | 0.050 | |||||
| 7 | 1.00% | PS near tip | 2 | 0.057 | |||||
| 7 | 1.50% | PS near tip | 0.5 | 0.004 | |||||
| 7 | 1.50% | PS near tip | 1 | 0.036 | |||||
| 7 | 1.50% | PS near tip | 2 | 0.031 | |||||
| 7 | 2.50% | PS near tip | 0.5 | 0.003 | |||||
| 7 | 2.50% | PS near tip | 1 | 0.020 | |||||
| 7 | 2.50% | PS near tip | 2 | 0.027 | |||||
| 14 | 1.00% | Camber + PS near tip | 0.5 | 0.012 | |||||
| 14 | 1.00% | Camber + PS near tip | 1 | 0.028 | |||||
| 14 | 1.00% | Camber + PS near tip | 2 | 0.062 | |||||
| 14 | 1.50% | Camber + PS near tip | 0.5 | 0.012 | |||||
| 14 | 1.50% | Camber + PS near tip | 1 | 0.020 | |||||
| 14 | 1.50% | Camber + PS near tip | 2 | 0.055 | |||||
| 14 | 2.50% | Camber + PS near tip | 0.5 | 0.019 | |||||
| 14 | 2.50% | Camber + PS near tip | 1 | 0.028 | |||||
| 14 | 2.50% | Camber + PS near tip | 2 | 0.050 | |||||
| Christophel 2005 [39] | IR | 2 | 0.5% | LE(purge) | 0.10% | 0.178 | 0.32 | purge holes | |
| 2 | 0.5% | LE(purge) | 0.19% | 0.382 | 0.71 | purge holes | |||
| 2 | 0.5% | LE(purge) | 0.29% | 0.431 | 0.86 | purge holes | |||
| 2 | 0.5% | LE(purge) | 0.38% | 0.448 | 1.16 | purge holes | |||
| 2 | 1.6% | LE(purge) | 0.10% | 0.074 | 0.11 | purge holes | |||
| 2 | 1.6% | LE(purge) | 0.19% | 0.099 | 0.08 | purge holes | |||
| 2 | 1.6% | LE(purge) | 0.29% | 0.198 | 0.26 | purge holes | |||
| 2 | 1.6% | LE(purge) | 0.38% | 0.300 | 0.51 | purge holes | |||
| Newton 2009 [45] | Liquid crystal | 11 | 4.4% C | Camber | 0.8 | 0.122 | 87 | 0.23 | * |
| 11 | 2.2% C | Tip near PS | 0.58 | 0.166 | 98 | 0.37 | * | ||
| 11 | 2.2% C | Tip near PS | 0.74 | 0.159 | 90 | 0.37 | * | ||
| 11 | 2.2% C | Tip near PS | 0.99 | 0.140 | 100 | 0.28 | * | ||
| Rezasoltani 2015 [9] | PSP | 7 | 1.50% | Camber | 1.25 | 0.035 | 2000 rpm | ||
| 8 | 1.50% | PS near tip | 1.25 | 0.089 | 2000 rpm | ||||
| 7 | 1.50% | Camber | 1.25 | 0.054 | 2550 rpm | ||||
| 8 | 1.50% | PS near tip | 1.25 | 0.059 | 2550 rpm | ||||
| 7 | 1.50% | Camber | 0.75 | 0.047 | 3000 rpm | ||||
| 7 | 1.50% | Camber | 1.25 | 0.650 | 3000 rpm | ||||
| 7 | 1.50% | Camber | 1.75 | 0.069 | 3000 rpm | ||||
| 8 | 1.50% | PS near tip | 0.75 | 0.024 | 3000 rpm | ||||
| 8 | 1.50% | PS near tip | 1.25 | 0.310 | 3000 rpm | ||||
| 8 | 1.50% | PS near tip | 1.75 | 0.040 | 3000 rpm | ||||
| Yang 2006 [50] | CFD | 7 | 1.50% | camber | 0.50 | 0.013 | * | ||
| 7 | 1.50% | camber | 1.00 | 0.016 | * | ||||
| 7 | 1.50% | camber | 2.00 | 0.002 | * | ||||
| 7 | 1.50% | Tip near PS | 0.50 | 0.045 | * | ||||
| 7 | 1.50% | Tip near PS | 1.00 | 0.071 | * | ||||
| 7 | 1.50% | Tip near PS | 2.00 | 0.041 | * | ||||
| 10 | 1.50% | camber + tip near PS | 0.25 | 0.028 | * | ||||
| 10 | 1.50% | camber + tip near PS | 0.50 | 0.056 | * | ||||
| 10 | 1.50% | camber + tip near PS | 1.00 | 0.070 | * | ||||
| Hohlfeld 2005 [42] | CFD | 2 | 0.54% | Tip near LE(purge) | 0.19% | 0.259 | |||
| 2 | 0.54% | Tip near LE(purge) | 0.29% | 0.363 | |||||
| 2 | 1.63% | Tip near LE(purge) | 0.05% | 0.057 | |||||
| 2 | 1.63% | Tip near LE(purge) | 0.10% | 0.045 | |||||
| 2 | 1.63% | Tip near LE(purge) | 0.19% | 0.019 | |||||
| 2 | 1.63% | Tip near LE(purge) | 0.29% | 0.025 | |||||
| 2 | 1.63% | Tip near LE(purge) | 0.38% | 0.046 |
| Reference | Technique | Hole Number | Tip Clearance | Cavity Depth | M | Inject Location | Eta | HTC | Note |
|---|---|---|---|---|---|---|---|---|---|
| Acharya 2002 [37] | CFD | 7 | 1.0% | 3.77% | 1.00 | Tip near PS | 0.234 | * | |
| 7 | 1.5% | 3.77% | 1.00 | Tip near PS | 0.171 | * | |||
| 7 | 2.5% | 3.77% | 1.00 | Tip near PS | 0.141 | * | |||
| 7 | 2.5% | 3.77% | 1.50 | Tip near PS | 0.228 | * | |||
| Kwak 2002 [49] | Liquid crystal | 13 | 1.50% | 4.22% | 1 | Camber | 0.072 | 416.786 | * |
| 13 | 1.50% | 4.22% | 2 | Camber | 0.083 | 397.001 | * | ||
| 26 | 1.50% | 4.22% | 1 | Camber + PS_near tip | 0.103 | 400.591 | * | ||
| 26 | 1.50% | 4.22% | 2 | Camber + PS_near tip | 0.113 | 375.995 | * | ||
| Tong 2014 [60] | CFD | 10 | 1.00% | 1.90% | PR = 3.57 | camber | 0.157 | 4644.18 | |
| 10 | 1.00% | 2.50% | PR = 3.65 | camber | 0.201 | 4552.06 | |||
| 10 | 1.00% | 3.10% | PR = 3.45 | camber | 0.138 | 4466.4 | |||
| Nasir 2004 [43] | Liquid crystal | 5 | 1.0% | 4.22% | 1 | Camber | 0.055 | 525.314 | * |
| 5 | 1.0% | 4.22% | 2 | Camber | 0.082 | 460.092 | * | ||
| 5 | 1.0% | 4.22% | 3 | Camber | 0.044 | 487.702 | * | ||
| 12 | 1.0% | 4.22% | 1 | Camber + PS near tip | 0.058 | 496.088 | * | ||
| 12 | 1.0% | 4.22% | 2 | Camber + PS near tip | 0.056 | 466.922 | * | ||
| 12 | 1.0% | 4.22% | 3 | Camber + PS near tip | 0.166 | 773.907 | * | ||
| 7 | 1.0% | 4.22% | 1 | PS near tip | 0.019 | 552.362 | * | ||
| 7 | 1.0% | 4.22% | 2 | PS near tip | 0.019 | 543.478 | * | ||
| 7 | 1.0% | 4.22% | 3 | PS near tip | 0.036 | 538.185 | * | ||
| Ahn 2005 [44] | PSP | 7 | 1.00% | 4.22% | 0.5 | Camber | 0.022 | ||
| 7 | 1.00% | 4.22% | 1 | Camber | 0.025 | ||||
| 7 | 1.00% | 4.22% | 2 | Camber | 0.051 | ||||
| 7 | 1.50% | 4.22% | 0.5 | Camber | 0.019 | ||||
| 7 | 1.50% | 4.22% | 1 | Camber | 0.028 | ||||
| 7 | 1.50% | 4.22% | 2 | Camber | 0.051 | ||||
| 7 | 2.50% | 4.22% | 0.5 | Camber | 0.023 | ||||
| 7 | 2.50% | 4.22% | 1 | Camber | 0.036 | ||||
| 7 | 2.50% | 4.22% | 2 | Camber | 0.039 | ||||
| 7 | 1.00% | 4.22% | 0.5 | PS near tip | 0.021 | ||||
| 7 | 1.00% | 4.22% | 1 | PS near tip | 0.026 | ||||
| 7 | 1.00% | 4.22% | 2 | PS near tip | 0.035 | ||||
| 7 | 1.50% | 4.22% | 0.5 | PS near tip | 0.020 | ||||
| 7 | 1.50% | 4.22% | 1 | PS near tip | 0.020 | ||||
| 7 | 1.50% | 4.22% | 2 | PS near tip | 0.021 | ||||
| 7 | 2.50% | 4.22% | 0.5 | PS near tip | 0.019 | ||||
| 7 | 2.50% | 4.22% | 1 | PS near tip | 0.021 | ||||
| 7 | 2.50% | 4.22% | 2 | PS near tip | 0.024 | ||||
| 14 | 1.00% | 4.22% | 0.5 | Camber + PS near tip | 0.033 | ||||
| 14 | 1.00% | 4.22% | 1 | Camber + PS near tip | 0.042 | ||||
| 14 | 1.00% | 4.22% | 2 | Camber + PS near tip | 0.060 | ||||
| 14 | 1.50% | 4.22% | 0.5 | Camber + PS near tip | 0.034 | ||||
| 14 | 1.50% | 4.22% | 1 | Camber + PS near tip | 0.048 | ||||
| 14 | 1.50% | 4.22% | 2 | Camber + PS near tip | 0.060 | ||||
| 14 | 2.50% | 4.22% | 0.5 | Camber + PS near tip | 0.039 | ||||
| 14 | 2.50% | 4.22% | 1 | Camber + PS near tip | 0.048 | ||||
| 14 | 2.50% | 4.22% | 2 | Camber + PS near tip | 0.066 | ||||
| Mhetras 2006 [51] | PSP | 9 | 1.50% | 2.10% | 0.50 | PS near tip | 0.015 | ||
| 9 | 1.50% | 2.10% | 1.00 | PS near tip | 0.027 | ||||
| 9 | 1.50% | 2.10% | 1.50 | PS near tip | 0.032 | ||||
| 9 | 1.50% | 2.10% | 2.00 | PS near tip | 0.038 | ||||
| 10 | 1.50% | 2.10% | 0.50 | Camber + tip near SS | 0.109 | ||||
| 10 | 1.50% | 2.10% | 1.00 | Camber + tip near SS | 0.147 | ||||
| 10 | 1.50% | 2.10% | 1.50 | Camber + tip near SS | 0.148 | ||||
| 10 | 1.50% | 2.10% | 2.00 | Camber + tip near SS | 0.153 | ||||
| 9 | 1.50% | 4.20% | 0.50 | PS near tip | 0.023 | ||||
| 9 | 1.50% | 4.20% | 1.00 | PS near tip | 0.027 | ||||
| 9 | 1.50% | 4.20% | 1.50 | PS near tip | 0.032 | ||||
| 9 | 1.50% | 4.20% | 2.00 | PS near tip | 0.047 | ||||
| 10 | 1.50% | 4.20% | 0.50 | Camber + tip near SS | 0.105 | ||||
| 10 | 1.50% | 4.20% | 1.00 | Camber + tip near SS | 0.133 | ||||
| 10 | 1.50% | 4.20% | 1.50 | Camber + tip near SS | 0.151 | ||||
| 10 | 1.50% | 4.20% | 2.00 | Camber + tip near SS | 0.163 | ||||
| Yang 2006 [50] | CFD | 7 | 1.50% | 3.77% | 0.50 | camber | 0.052 | ||
| 7 | 1.50% | 3.77% | 1.00 | camber | 0.075 | ||||
| 7 | 1.50% | 3.77% | 2.00 | camber | 0.045 | ||||
| 7 | 1.50% | 3.77% | 0.50 | camber + tip near LE | 0.127 | ||||
| 7 | 1.50% | 3.77% | 1.00 | camber + tip near LE | 0.173 | ||||
| 7 | 1.50% | 3.77% | 2.00 | camber + tip near LE | 0.190 | ||||
| 13 | 1.50% | 3.77% | 0.25 | Camber + tip near SS | 0.108 | ||||
| 13 | 1.50% | 3.77% | 0.50 | Camber + tip near SS | 0.167 | ||||
| 13 | 1.50% | 3.77% | 1.00 | Camber + tip near SS | 0.241 | ||||
| Gao 2009 [55] | PSP | 19 | 1.50% | 2.10% | 0.50 | Camber + tip near SS + PS near tip | 0.100 | Incidence −5 deg | |
| 19 | 1.50% | 2.10% | 1.00 | Camber + tip near SS + PS near tip | 0.135 | Incidence −5 deg | |||
| 19 | 1.50% | 2.10% | 1.50 | Camber + tip near SS + PS near tip | 0.132 | Incidence −5 deg | |||
| 19 | 1.50% | 2.10% | 2.00 | Camber + tip near SS + PS near tip | 0.140 | Incidence −5 deg | |||
| 19 | 1.50% | 2.10% | 0.50 | Camber + tip near SS + PS near tip | 0.115 | Incidence 0 deg | |||
| 19 | 1.50% | 2.10% | 1.00 | Camber + tip near SS + PS near tip | 0.135 | Incidence 0 deg | |||
| 19 | 1.50% | 2.10% | 1.50 | Camber + tip near SS + PS near tip | 0.132 | Incidence 0 deg | |||
| 19 | 1.50% | 2.10% | 2.00 | Camber + tip near SS + PS near tip | 0.136 | Incidence 0 deg | |||
| 19 | 1.50% | 2.10% | 0.50 | Camber + tip near SS + PS near tip | 0.110 | Incidence 5 deg | |||
| 19 | 1.50% | 2.10% | 1.00 | Camber + tip near SS + PS near tip | 0.150 | Incidence 5 deg | |||
| 19 | 1.50% | 2.10% | 1.50 | Camber + tip near SS + PS near tip | 0.171 | Incidence 5 deg | |||
| 19 | 1.50% | 2.10% | 2.00 | Camber + tip near SS + PS near tip | 0.171 | Incidence 5 deg | |||
| Nazary 2009 [56] | Liquid crystal | 33 | 0.87% | 1.81% | PR = 1.19 | (LE + PS + SS) near tip | 0.021 | * | |
| 33 | 0.87% | 1.81% | PR = 1.31 | (LE + PS + SS) near tip | 0.031 | * | |||
| 33 | 0.87% | 1.81% | PR = 1.48 | (LE + PS + SS) near tip | 0.043 | * | |||
| 33 | 0.87% | 1.81% | PR = 1.57 | (LE + PS + SS) near tip | 0.054 | * | |||
| 33 | 1.59% | 1.81% | PR = 1.17 | (LE + PS + SS) near tip | 0.008 | * | |||
| 33 | 1.59% | 1.81% | PR = 1.26 | (LE + PS + SS) near tip | 0.028 | * | |||
| 33 | 1.59% | 1.81% | PR = 1.39 | (LE + PS + SS) near tip | 0.028 | * | |||
| 33 | 1.59% | 1.81% | PR = 1.58 | (LE + PS + SS) near tip | 0.030 | * | |||
| 33 | 2.32% | 1.81% | PR = 1.16 | (LE + PS + SS) near tip | 0.016 | * | |||
| 33 | 2.32% | 1.81% | PR = 1.24 | (LE + PS + SS) near tip | 0.018 | * | |||
| 33 | 2.32% | 1.81% | PR = 1.39 | (LE + PS + SS) near tip | 0.028 | * | |||
| 33 | 2.32% | 1.81% | PR = 1.52 | (LE + PS + SS) near tip | 0.027 | * | |||
| 63 | 0.87% | 1.81% | 0.5 | (LE + PS + SS) near tip + rim hub | 0.007 | * | |||
| 63 | 0.87% | 1.81% | 1 | (LE + PS + SS) near tip + rim hub | 0.027 | * | |||
| 63 | 0.87% | 1.81% | 1.5 | (LE + PS + SS) near tip + rim hub | 0.042 | * | |||
| 63 | 0.87% | 1.81% | 2 | (LE + PS + SS) near tip + rim hub | 0.068 | * | |||
| 63 | 1.59% | 1.81% | 0.5 | (LE + PS + SS) near tip + rim hub | 0.011 | * | |||
| 63 | 1.59% | 1.81% | 1 | (LE + PS + SS) near tip + rim hub | 0.030 | * | |||
| 63 | 1.59% | 1.81% | 1.5 | (LE + PS + SS) near tip + rim hub | 0.046 | * | |||
| 63 | 1.59% | 1.81% | 2 | (LE + PS + SS) near tip + rim hub | 0.069 | * | |||
| 63 | 2.32% | 1.81% | 0.5 | (LE + PS + SS) near tip + rim hub | 0.017 | * | |||
| 63 | 2.32% | 1.81% | 1 | (LE + PS + SS) near tip + rim hub | 0.026 | * | |||
| 63 | 2.32% | 1.81% | 1.5 | (LE + PS + SS) near tip + rim hub | 0.053 | * | |||
| 63 | 2.32% | 1.81% | 2 | (LE + PS + SS) near tip + rim hub | 0.065 | * | |||
| Rezasoltani 2015 [9] | PSP | 7 | 1.50% | 3.15% | 1.25 | Camber | 0.097 | 2000 rpm | |
| 8 | 1.50% | 3.15% | 1.25 | PS near tip | 0.054 | 2000 rpm | |||
| 7 | 1.50% | 3.15% | 1.25 | Camber | 0.060 | 2550 rpm | |||
| 8 | 1.50% | 3.15% | 1.25 | PS near tip | 0.040 | 2550 rpm | |||
| 7 | 1.50% | 3.15% | 0.75 | Camber | 0.052 | 3000 rpm | |||
| 7 | 1.50% | 3.15% | 1.25 | Camber | 0.052 | 3000 rpm | |||
| 7 | 1.50% | 3.15% | 1.75 | Camber | 0.068 | 3000 rpm | |||
| 8 | 1.50% | 3.15% | 0.75 | PS near tip | 0.018 | 3000 rpm | |||
| 8 | 1.50% | 3.15% | 1.25 | PS near tip | 0.020 | 3000 rpm | |||
| 8 | 1.50% | 3.15% | 1.75 | PS near tip | 0.022 | 3000 rpm | |||
| Tamunobere 2016 [63] | Liquid crystal | 2 | 1.70% | NA | 1.5 | camber | 0.028 | 206.267 | * |
| 2 | 1.70% | NA | 3 | camber | 0.058 | 234.096 | * | ||
| 6 | 1.70% | NA | 1.5 | PS near tip | 0.021 | 220.824 | * | ||
| 6 | 1.70% | NA | 3 | PS near tip | 0.047 | 207.867 | * | ||
| 8 | 1.70% | NA | 1 | camber + PS near tip | 0.029 | 216.639 | * | ||
| 8 | 1.70% | NA | 1.5 | camber + PS near tip | 0.043 | 220.103 | * | ||
| 8 | 1.70% | NA | 2 | camber + PS near tip | 0.059 | 237.537 | * | ||
| 8 | 1.70% | NA | 3 | camber + PS near tip | 0.072 | 233.535 | * | ||
| 8 | 1.70% | NA | 4 | camber + PS near tip | 0.080 | 250.733 | * | ||
| Ma 2016 [58] | IR | 9 | 1.00% | NA | 0.45% | tip near PS | 0.050 | 1369.34 | * |
| 9 | 1.00% | NA | 0.45% | Camber | 0.042 | 1265.58 | * | ||
| 9 | 1.00% | NA | 0.45% | tip near SS | 0.042 | 1194.15 | * | ||
| Yan 2017 [63] | CFD | 13 | 1.00% | 4.16% | 1 | camber | 0.061 | squealer | |
| 13 | 1.00% | 4.16% | 1 | camber | 0.056 | SS rim only | |||
| 13 | 1.00% | 4.16% | 1 | camber | 0.076 | PS winglet | |||
| 13 | 1.50% | 4.16% | 1 | camber | 0.051 | squealer | |||
| 13 | 1.50% | 4.16% | 1 | camber | 0.061 | SS rim only | |||
| 13 | 1.50% | 4.16% | 1 | camber | 0.056 | PS winglet | |||
| 26 | 1.00% | 4.16% | 1 | Camber + PS near tip | 0.126 | squealer | |||
| 26 | 1.00% | 4.16% | 1 | Camber + PS near tip | 0.135 | SS rim only | |||
| 26 | 1.00% | 4.16% | 1 | Camber + PS near tip | 0.132 | PS winglet | |||
| 26 | 1.50% | 4.16% | 1 | Camber + PS near tip | 0.083 | squealer | |||
| 26 | 1.50% | 4.16% | 1 | Camber + PS near tip | 0.131 | SS rim only | |||
| 26 | 1.50% | 4.16% | 1 | Camber + PS near tip | 0.128 | PS winglet | |||
| Arisi 2015 [14] | IR | 2 | 1.00% | 1.50% | 1 | camber | 0.120 | 628.219 | Ma_exit 0.8; purge |
| 2 | 1.00% | 1.50% | 1 | camber | 0.124 | 702.173 | Ma_exit 1.0; purge |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, S.; Ng, W.F. Turbine Blade Tip External Cooling Technologies. Aerospace 2018, 5, 90. https://doi.org/10.3390/aerospace5030090
Xue S, Ng WF. Turbine Blade Tip External Cooling Technologies. Aerospace. 2018; 5(3):90. https://doi.org/10.3390/aerospace5030090
Chicago/Turabian StyleXue, Song, and Wing F. Ng. 2018. "Turbine Blade Tip External Cooling Technologies" Aerospace 5, no. 3: 90. https://doi.org/10.3390/aerospace5030090
APA StyleXue, S., & Ng, W. F. (2018). Turbine Blade Tip External Cooling Technologies. Aerospace, 5(3), 90. https://doi.org/10.3390/aerospace5030090