A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment
Abstract
1. Introduction
2. Aeroelastic Problem Formulation
Modal Solution Approach
3. Generalised Aerodynamic Load
Unsteady Modified Strip Theory
4. Added Aerodynamic States
Unsteady Air Load
5. Analytical Aeroelastic Analysis
6. Numerical Aeroelastic Analysis
7. Results and Discussion
7.1. Structural FEM and Aerodynamic DLM for Numerical Simulations
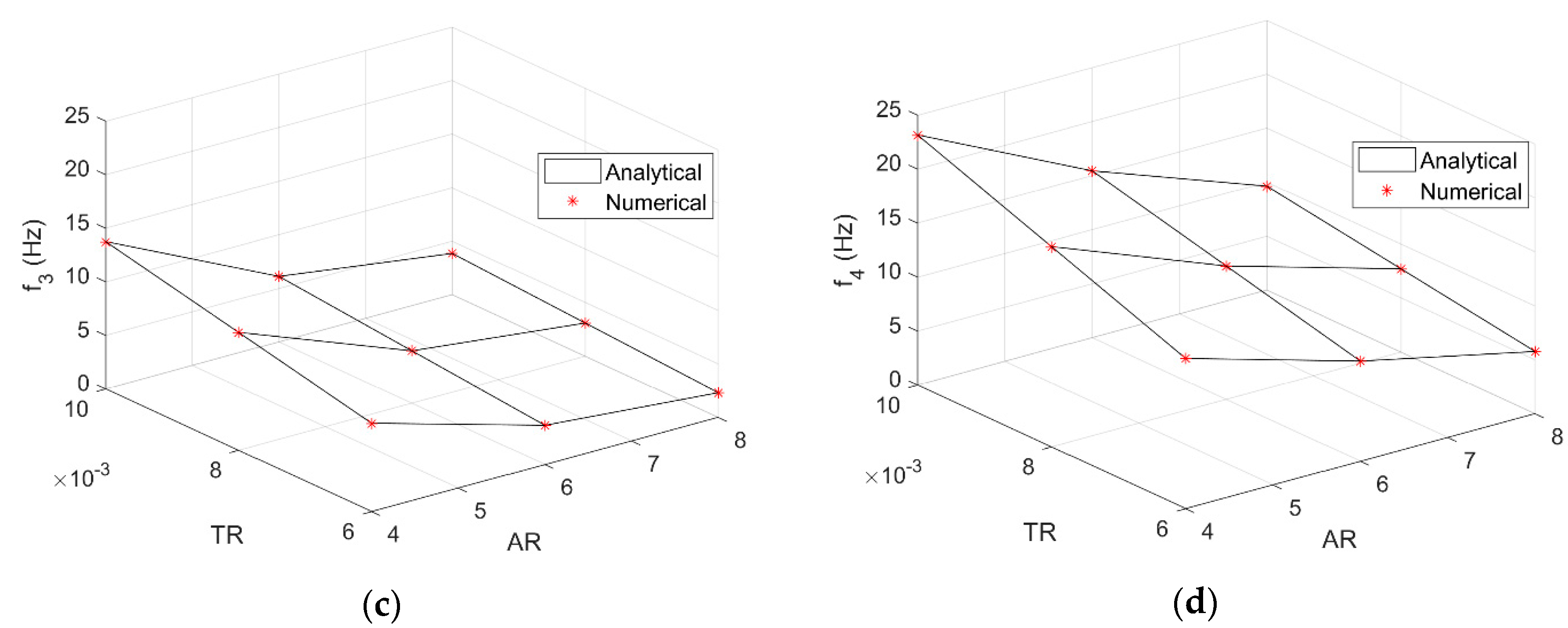
7.2. Natural Vibration Modes
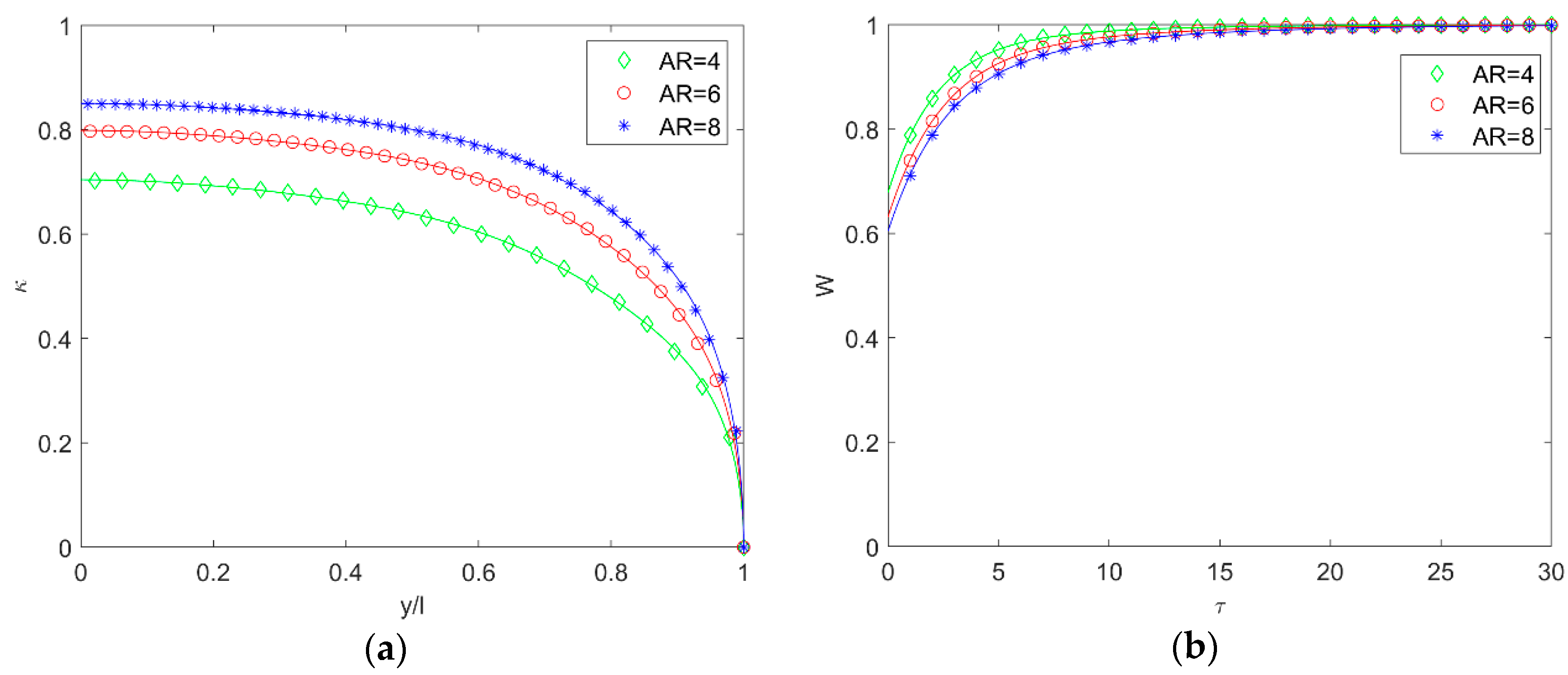
7.3. Steady and Unsteady Air Load
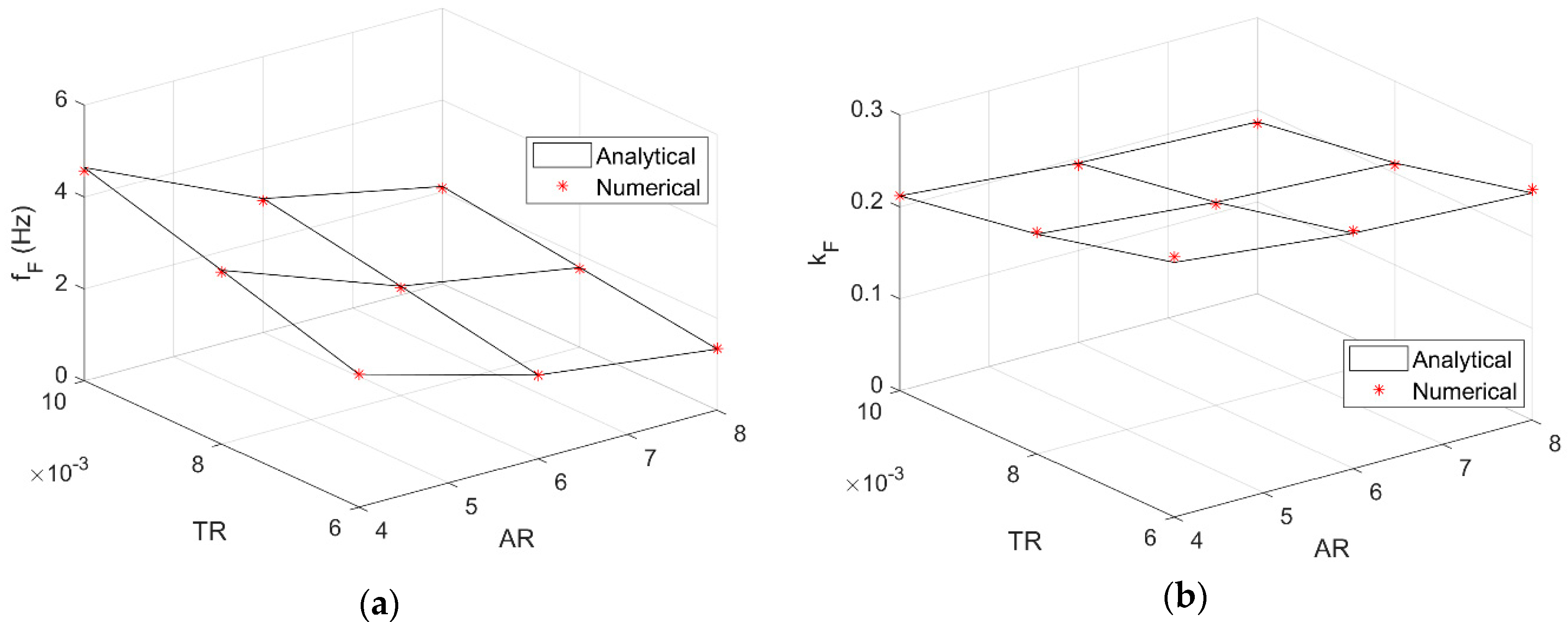
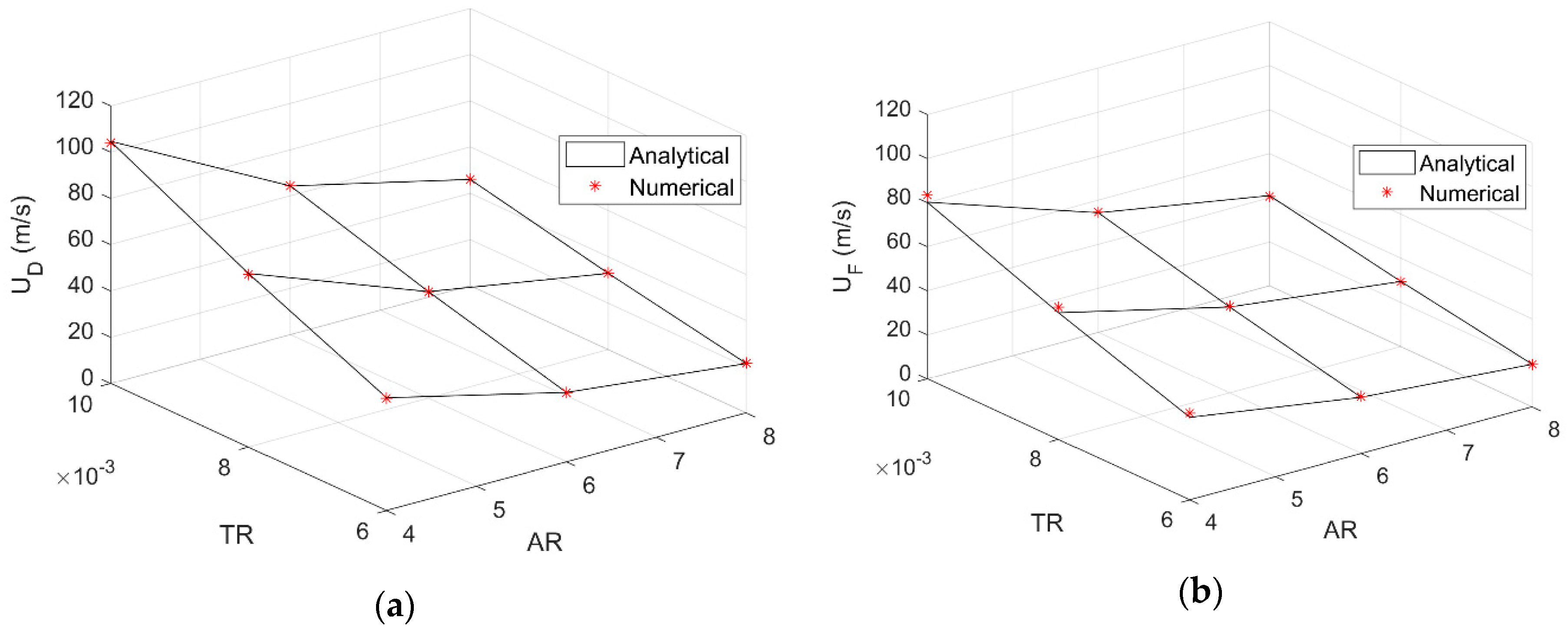
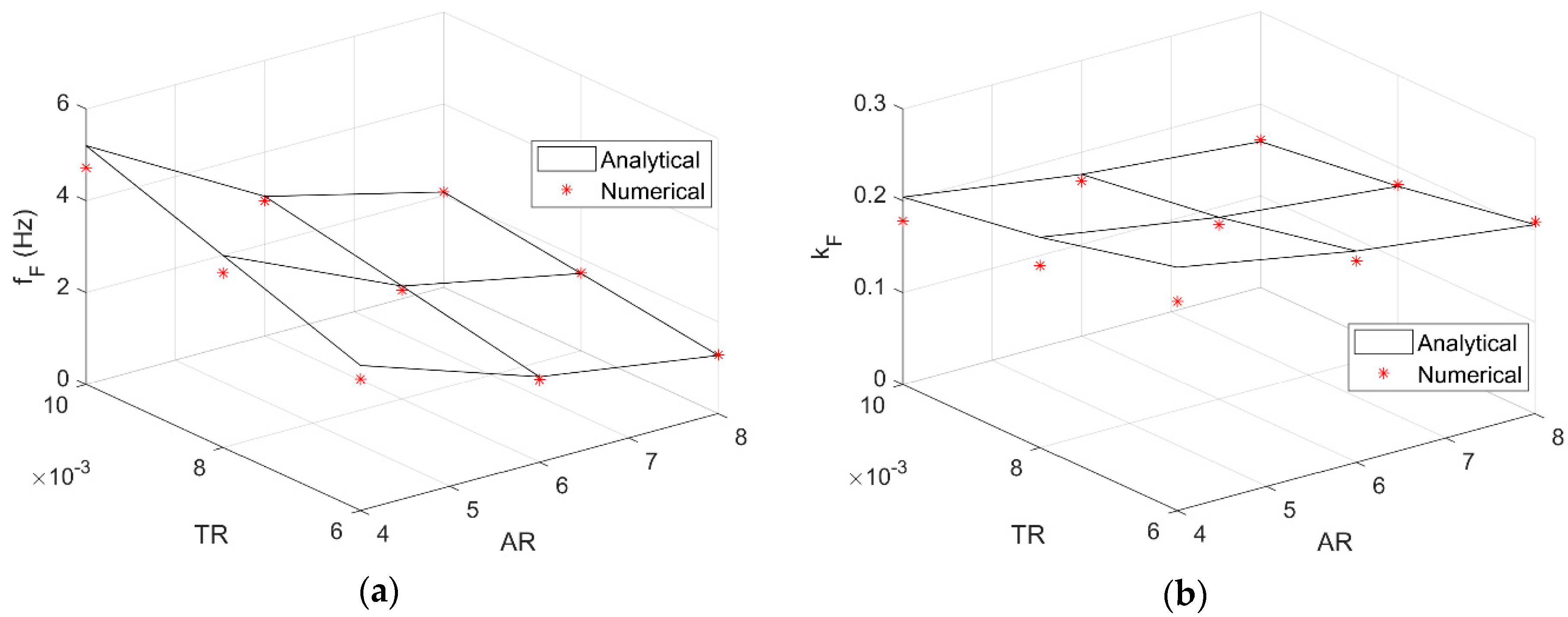
7.4. Divergence and Flutter Analysis
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| aerodynamic gain coefficient | |
| wing aspect ratio | |
| aerodynamic pole coefficient | |
| section chord | |
| section lift | |
| section lift derivative | |
| wing lift derivative | |
| generalised damping matrix | |
| elliptic integral of the second kind | |
| section Young’s elastic modulus | |
| angular frequency | |
| generalised aerodynamic load vector | |
| section shear elastic modulus | |
| section thickness | |
| section flexural area moments of inertia | |
| section torsional mass moments of inertia | |
| reduced frequency | |
| generalised stiffness matrix | |
| wing semi-span | |
| section aerodynamic force | |
| section mass | |
| section aerodynamic moment | |
| generalised mass matrix | |
| number of expansion terms | |
| time | |
| horizontal air speed | |
| vertical air speed | |
| section vertical displacement | |
| aerodynamic indicial-admittance function | |
| chordwise coordinate | |
| spanwise coordinate | |
| angle of attack | |
| section circulation | |
| flexural generalised coordinate | |
| section flexural displacement | |
| torsional generalised coordinate | |
| section torsional displacement | |
| aerodynamic load-scaling function | |
| eigenvalue | |
| section flexural mass moments of inertia | |
| section torsional mass moments of inertia | |
| Poisson ratio | |
| Oswald’s efficiency factor | |
| reference air density | |
| reduced time | |
| added aerodynamic state | |
| flexural assumed mode shape | |
| torsional assumed mode shapes | |
| generalised coordinates vector | |
| spanwise Glauert angle |
Appendix A. Lifting Line Models for Rectangular Straight Wings

Appendix A.1. Steady Lift Distribution
Appendix A.2. Unsteady Lift Development
References
- Cavagna, L.; Ricci, S.; Travaglini, L. NeoCASS: An Integrated Tool for Structural Sizing, Aeroelastic Analysis and MDO at Conceptual Design Level. Prog. Aerosp. Sci. 2011, 47, 621–635. [Google Scholar] [CrossRef]
- Alexandrov, N.M.; Hussaini, M.Y. Multidisciplinary Design Optimization: State of the Art; Proceedings in Applied Mathematics Series, 80; SIAM: Philadelphia, PA, USA, 1997. [Google Scholar]
- Martins, J.R.R.A.; Lambe, A.B. Multidisciplinary Design Optimization: A Survey of Architectures. AIAA J. 2013, 51, 2049–2075. [Google Scholar] [CrossRef]
- Vanderplaats, G.N. Numerical Optimization Techniques for Engineering Design: With Applications; Series in Mechanical Engineering; McGraw Hill: New York, NY, USA, 1984. [Google Scholar]
- Pike, E.C. Manual on Aeroelasticity; AGARD-R-578-71; AGARD: Neuilly sur Seine, France, 1971. [Google Scholar]
- Livne, E. The Future of Aircraft Aeroelasticity. J. Aircr. 2003, 40, 1066–1092. [Google Scholar] [CrossRef]
- Kesseler, E.; Guenov, M. Advances in Collaborative Civil Aeronautical Multidisciplinary Design Optimization; Progress in Astronautics and Aeronautics Series, 233; AIAA: Reston, VA, USA, 2010. [Google Scholar]
- Bungartz, H.J.; Schafer, M. Fluid-Structure Interaction: Modelling, Simulation, Optimization; Lecture Notes in Computational Science and Engineering, 53; Springer: Berlin, Germany, 2006. [Google Scholar]
- Dhatt, G.; Lefrancois, E.; Touzot, G. Finite Element Method; Numerical Methods Series; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Chung, T.J. Computational Fluid Dynamics; Cambridge Press: Cambridge, UK, 2002. [Google Scholar]
- Cavagna, L.; Quaranta, G.; Ghiringhelli, G.L.; Mantegazza, P. Efficient Application of CFD Aeroelastic Methods Using Commercial Software. In Proceedings of the 11th IFASD, Munich, Germany, 28 June–1 July 2005. [Google Scholar]
- Romanelli, G.; Serioli, E.; Mantegazza, P. A “Free” Approach to Computational Aeroelasticity; AIAA-2010-176; AIAA: Reston, VA, USA, 2010. [Google Scholar]
- Sucipto, T.; Berci, M.; Krier, J. Gust Response of a Flexible Typical Section via High- and (Tuned) Low-Fidelity Simulations. Comput. Struct. 2013, 122, 202–216. [Google Scholar] [CrossRef]
- Berci, M.; Mascetti, S.; Incognito, A.; Gaskell, P.H.; Toropov, V.V. Dynamic Response of Typical Section Using Variable-Fidelity Fluid Dynamics and Gust-Modeling Approaches—With Correction Methods. J. Aerosp. Eng. 2014, 27, 04014026. [Google Scholar] [CrossRef]
- Farhat, C.; Lesoinne, M.; Le Tallec, P. Load and Motion Transfer Algorithms for Fluid/Structure Interaction Problems with Non-Matching Discrete Interfaces: Momentum and Energy Conservation, Optimal Discretization and Application to Aeroelasticity. Comput. Methods Appl. Mech. Eng. 1998, 157, 95–114. [Google Scholar] [CrossRef]
- Cizmas, P.G.A.; Gargoloff, J.I. Mesh Generation and Deformation Algorithm for Aeroelasticity Simulations. J. Aircr. 2008, 45, 1062–1066. [Google Scholar] [CrossRef]
- Heil, M.; Hazel, A.L.; Boyle, J. Solvers for Large-Displacement Fluid–Structure Interaction Problems: Segregated Versus Monolithic Approaches. Comput. Mech. 2008, 43, 91–101. [Google Scholar] [CrossRef]
- Sheldon, J.P.; Miller, S.T.; Pitt, J.S. Methodology for Comparing Coupling Algorithms for Fluid-Structure Interaction Problems. World J. Mech. 2014, 4, 54. [Google Scholar] [CrossRef]
- Kloppel, T.; Popp, A.; Wall, W.A. Interface Treatment in Computational Fluid-Structure Interaction. In Proceedings of the 3rd ECCOMAS COMPDYN, Island of Corfu, Greece, 26–28 May 2011. [Google Scholar]
- Farhat, C.; Lakshminarayan, V. An ALE Formulation of Embedded Boundary Methods for Tracking Boundary Layers in Turbulent Fluid-Structure Interaction Problems. J. Comput. Phys. 2014, 263, 53–70. [Google Scholar] [CrossRef]
- Berci, M.; Toropov, V.V.; Hewson, R.W.; Gaskell, P.H. Multidisciplinary Multifidelity Optimisation of a Flexible Wing Aerofoil with Reference to a Small UAV. Struct. Multidiscip. Optim. 2014, 50, 683–699. [Google Scholar] [CrossRef]
- Quarteroni, A.; Rozza, G. Reduced Order Methods for Modeling and Computational Reduction; MS&A, 9; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Qu, Z.Q. Model Order Reduction Techniques with Applications in Finite Element Analysis; Springer: London, UK, 2004. [Google Scholar]
- Ghoreyshi, M.; Jirasek, A.; Cummings, R.M. Reduced Order Unsteady Aerodynamic Modeling for Stability and Control Analysis Using Computational Fluid Dynamics. Prog. Aerosp. Sci. 2014, 71, 167–217. [Google Scholar] [CrossRef]
- Gennaretti, M.; Mastroddi, F. Study of Reduced-Order Models for Gust-Response Analysis of Flexible Fixed Wings. J. Aircr. 2004, 41, 304–313. [Google Scholar] [CrossRef]
- Ripepi, M.; Verveld, M.J.; Karcher, N.W.; Franz, T.; Abu-Zurayk, M.; Görtz, S.; Kier, T.M. Reduced Order Models for Aerodynamic Applications, Loads and MDO; DLRK-2016-420057; DLR: Braunschweig, Germany, 2016. [Google Scholar]
- Berci, M. Semi-Analytical Static Aeroelastic Analysis and Response of Flexible Subsonic Wings. Appl. Math. Comput. 2015, 267, 148–169. [Google Scholar] [CrossRef]
- Sitaraman, J.; Baeder, J.D. Computational-Fluid-Dynamics-Based Enhanced Indicial Aerodynamic Models. J. Aircr. 2004, 41, 798–810. [Google Scholar] [CrossRef]
- Anderson, J.D. Fundamentals of Aerodynamics; Series in Aeronautical and Aerospace Engineering; McGraw-Hill: New York, NY, USA, 2007. [Google Scholar]
- Wagner, H. Uber die Entstenhung des Dynamischen Auftriebes von Tragflugeln. Z. Angew. Math. Mech. 1925, 5, 17–35. [Google Scholar] [CrossRef]
- Kussner, H.G. Zusammenfassender Beritch uber den Instationaren Auftrieb von Flugeln. Luftfahrtforsch 1936, 13, 410–424. [Google Scholar]
- Kayran, A. Küssner’s Function in the Sharp Edged Gust Problem—A Correction. J. Aircr. 2006, 43, 1596–1599. [Google Scholar] [CrossRef]
- Theodorsen, T. General Theory of Aerodynamic Instability and the Mechanism of Flutter; NACA 496; NACA: Washington, DC, USA, 1935. [Google Scholar]
- Von Karman, T.; Sears, W.R. Airfoil Theory for Non-Uniform Motion. J. Aeronaut. Sci. 1938, 5, 379–390. [Google Scholar] [CrossRef]
- Sears, W.R. Operational Methods in the Theory of Airfoils in Non-Uniform Motion. J. Frankl. Inst. 1940, 230, 95–111. [Google Scholar] [CrossRef]
- Katz, J.; Plotkin, A. Low Speed Aerodynamics; Cambridge Aerospace Series; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Diederich, F.W. Approximate Aerodynamic Influence Coefficients for Wings of Arbitrary Plan Form in Subsonic Flow; NACA TN 2092; NACA: Washington, DC, USA, 1950. [Google Scholar]
- Diederich, F.W.; Zlotnick, M. Calculated Spanwise Lift Distributions, Influence Functions and Influence Coefficients for Unswept Wings in Subsonic Flow; NACA 1228; NACA: Washington, DC, USA, 1955. [Google Scholar]
- Jones, R.T. The Unsteady Lift of a Wing of Finite Aspect Ratio; NACA 681; NACA: Washington, DC, USA, 1940. [Google Scholar]
- Jones, W.P. Aerodynamic Forces on Wings in Simple Harmonic Motion; ARC-RM-2026; HM Stationery Office: London, UK, 1945. [Google Scholar]
- Jones, W.P. Aerodynamic Forces on Wings in Non-Uniform Motion; ARC-RM-2117; HM Stationery Office: London, UK, 1945. [Google Scholar]
- Reissner, E. Effect of Finite Span on the Airload Distributions for Oscillating Wings—Part I: Aerodynamic Theory of Oscillating Wings of Finite Span; NACA TN-1194; Massachusetts Institute of Technology: Cambridge, MA, USA, 1947. [Google Scholar]
- Reissner, E.; Stevens, J.E. Effect of Finite Span on the Airload Distributions for Oscillating Wings—Part II: Methods of Calculation and Examples of Application; NACA TN-1195; NACA: Washington, DC, USA, 1947. [Google Scholar]
- Albano, E.; Rodden, W.P. A Doublet-Lattice Method for Calculating the Lift Distribution of Oscillating Surfaces in Subsonic Flows. AIAA J. 1969, 7, 279–285. [Google Scholar] [CrossRef]
- Rodden, W.P.; Harder, R.L.; Bellinger, E.D. Aeroelastic Addition to NASTRAN; NASA CR-3094; NASA: Washington, DC, USA, 1979.
- Quick Reference Guide. In MSC Nastran; MSC Software Corporation: Newport Beach, CA, USA, 2018.
- Holmes, R.B. A Course on Optimization and Best Approximation; Lecture Notes in Mathematics, 257; Springer: Berlin, Germany, 1972. [Google Scholar]
- Leishman, J.G. Principles of Helicopter Aerodynamics; Cambridge Aerospace Series; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Bisplinghoff, R.L.; Ashley, H. Principles of Aeroelasticity; Dover: Mineola, NY, USA, 2013. [Google Scholar]
- Reddy, J.N. Energy Principles and Variational Methods in Applied Mechanics; Wiley: Hoboken, NJ, USA, 2002. [Google Scholar]
- Hodges, D.H.; Pierce, G.A. Introduction to Structural Dynamics and Aeroelasticity; Cambridge Aerospace Series; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Bisplinghoff, R.L.; Ashley, H.; Halfman, R.L. Aeroelasticity; Dover: Mineola, NY, USA, 1996. [Google Scholar]
- Demasi, L.; Livne, E. Structural Ritz-Based Simple-Polynomial Nonlinear Equivalent Approach—An Assessment; AIAA-2005-2093; AIAA: Reston, VA, USA, 2005. [Google Scholar]
- Fung, Y.C. An Introduction to the Theory of Aeroelasticity; Dover: Mineola, NY, USA, 1993. [Google Scholar]
- Dowell, E.H. A Modern Course in Aeroelasticity; Solid Mechanics and Its Applications, 217; Springer: Berlin, Germany, 2015. [Google Scholar]
- Prandtl, L. Applications of Modern Hydrodynamics to Aeronautics; NACA TR-116; NACA: Washington, DC, USA, 1921. [Google Scholar]
- Helmbold, H.B. Der Unverwundene Ellipsenflügel als Tragende Fläche. In Jahrbuch der Deutschen Luftfahrtforschung; Deutsche Akademie der Luftfahrtforschung: Munich, Germany, 1942; Volume I, pp. 111–113. [Google Scholar]
- Diederich, F.W. A Plan-Form Parameter for Correlating Certain Aerodynamic Characteristics of Swept Wings; NACA TN 2335; NACA: Washington, DC, USA, 1951. [Google Scholar]
- Queijo, M.J.; Wells, W.R.; Keskar, D.A. Approximate Indicial Lift Function for Tapered, Swept Wings in Incompressible Flow; NASA TP-1241; NASA: Washington, DC, USA, 1978.
- Wells, W.R. An Approximate Analysis of Wing Unsteady Aerodynamics; AFFDL-TR-79-3046; University of Dayton Research Institute: Dayton, OH, USA, 1979. [Google Scholar]
- Jones, R.T. Classical Aerodynamic Theory; NASA RP 1050; NASA: Washington, DC, USA, 1979.
- Megson, T.H.G. Aircraft Structures for Engineering Students; Elsevier Aerospace Engineering Series; Elsevier: Oxford, UK, 2007. [Google Scholar]
- Yang, B. Strain, Stress and Structural Dynamics; Elsevier: London, UK, 2005. [Google Scholar]
- Amabili, M. Nonlinear Vibrations and Stability of Shells and Plates; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Berci, M.; Gaskell, P.H.; Hewson, R.W.; Toropov, V.V. A Semi-Analytical Model for the Combined Aeroelastic Behaviour and Gust Response of a Flexible Aerofoil. J. Fluids Struct. 2013, 37, 3–21. [Google Scholar] [CrossRef]
- Wright, J.R.; Cooper, J.E. Introduction to Aircraft Aeroelasticity and Loads; Aerospace Series; Wiley: Chichester, UK, 2015. [Google Scholar]
- Young, W.C.; Budynas, R.G. Roark’s Formulas for Stress and Strain; McGraw-Hill: New York, NY, USA, 2011. [Google Scholar]
- Han, S.M.; Benaroya, H.; Wei, T. Dynamics of Transversally Vibrating Beams Using Four Engineering Theories. J. Sound Vib. 1999, 225, 935–988. [Google Scholar] [CrossRef]
- Marzocca, P.; Librescu, L.; Silva, W.A. Aeroelastic Response and Flutter of Swept Aircraft Wings. AIAA J. 2002, 40, 801–812. [Google Scholar] [CrossRef]
- Craig, R.R.; Bampton, M.C.C. Coupling of Substructures for Dynamic Analyses. AIAA J. 1968, 6, 1313–1319. [Google Scholar]
- Allemang, R.J. The modal assurance criterion—Twenty years of use and abuse. Sound Vib. 2003, 37, 14–23. [Google Scholar]
- Drischler, J.A. Approximate Indicial Lift Function for Several Wings of Finite Span in Incompressible Flow as Obtained from Oscillatory Lift Coefficients; NACA TN-3639; NACA: Washington, DC, USA, 1956. [Google Scholar]
- Mateescu, D.; Seytre, J.F.; Berhe, A.M. Theoretical Solutions for Finite-Span Wings of Arbitrary Shapes Using Velocity Singularities. J. Aircr. 2003, 40, 450–460. [Google Scholar] [CrossRef]
- Lomax, H.; Heaslet, M.A.; Fuller, F.B.; Sluder, L. Two- and Three-Dimensional Unsteady Lift Problems in High-Speed Flight; NACA 1077; NACA: Washington, DC, USA, 1950. [Google Scholar]
- Gulcat, U. Fundamentals of Modern Unsteady Aerodynamics; Springer: Berlin, Germany, 2011. [Google Scholar]
- Glauert, H. The Elements of Aerofoil and Airscrew Theory; Cambridge Science Classics; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar]
- Quarteroni, A.; Sacco, R.; Saleri, F. Numerical Mathematics; Texts in Applied Mathematics, 37; Springer: Berlin, Germany, 2007. [Google Scholar]
- Abbott, I.H.; von Doenhoff, A.E. Theory of Wing Sections: Including a Summary of Aerofoil Data; Dover: New York, NY, USA, 1959. [Google Scholar]
- Peters, D.A.; Hsieh, M.C.A.; Torrero, A. A State-Space Airloads Theory for Flexible Airfoils. J. Am. Helicopter Soc. 2007, 52, 329–342. [Google Scholar] [CrossRef]
- Pettit, G.W. Model to Evaluate the Aerodynamic Energy Requirements of Active Materials in Morphing Wings. Master’s Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2001. [Google Scholar]
- Kutta, M.W. Auftriebskräfte in Strömenden Flüssigkeiten. Illustrierte Aeronautische Mitteilungen 1902, 6, 133–135. [Google Scholar]
- Joukowski, N.E. Sur les Tourbillons Adjionts. Traraux de la Section Physique de la Societé Imperiale des Amis des Sciences Naturales 1906, 13, 2. [Google Scholar]
- Nita, M.F. Contributions to Aircraft Preliminary Design and Optimization. Ph.D. Thesis, Politehnica University of Bucharest, Bucharest, Romania, 2012. [Google Scholar]
- Van Holten, T. Some Notes on Unsteady Lifting-Line Theory. J. Fluid Mech. 1976, 77, 561–579. [Google Scholar] [CrossRef]
- Sclavounos, P.D. An Unsteady Lifting-Line Theory. J. Eng. Math. 1987, 21, 201–226. [Google Scholar] [CrossRef]
- Guermond, J.L.; Sellier, A. A Unified Unsteady Lifting-Line Theory. J. Fluid Mech. 1991, 229, 427–451. [Google Scholar] [CrossRef]
- Wieseman, C.D. Methodology for Matching Experimental and Computational Aerodynamic Data; NASA TM-100592; NASA: Washington, DC, USA, 1988.
- Palacios, R.; Climent, H.; Karlsson, A.; Winzell, B. Assessment of Strategies for Correcting Linear Unsteady Aerodynamics Using CFD or Test Results. In Proceedings of the 9th IFASD, Madrid, Spain, 5–7 June 2001. [Google Scholar]
- Munk, M.M. Elements of the Wing Section Theory and of the Wing Theory; NACA 191; NACA: Washington, DC, USA, 1924. [Google Scholar]
- Lippisch, A. Method for the Determination of the Spanwise Lift Distribution; NACA TM 778; NACA: Washington, DC, USA, 1935. [Google Scholar]
- Pearson, H.A. Span Load Distribution for Tapered Wings with Partial-Span Flaps; NACA 585; NACA: Washington, DC, USA, 1937. [Google Scholar]
- DeYoung, J. Theoretical Additional Span Loading Characteristics of Wings with Arbitrary Sweep, Aspect Ratio, and Taper Ratio; NACA TN 1491; NACA: Washington, DC, USA, 1947. [Google Scholar]
- DeYoung, J. Theoretical Antisymmetric Span Loading for Wings of Arbitrary Plan Form at Subsonic Speeds; NACA TN 2140; NACA: Washington, DC, USA, 1950. [Google Scholar]
- Multhopp, H. Methods for Calculating the Lift Distribution of Wings (Subsonic Lifting-Surface Theory); ARC R&M 2884; Aeronautical Research Council: London, UK, 1955. [Google Scholar]
- Simpson, R.W. An Extension of Multhopp’s Lifting Surface Theory; Cranfield CoA Report 132; Cranfield University: Bedford, UK, 1960. [Google Scholar]
- Brebner, G.G.; Lemaire, D.A. The Calculation of the Spanwise Loading Sweptback Wings with Flaps or All-Moving Tips at Subsonic Speeds; ARC R&M 3487; Aeronautical Research Council: London, UK, 1967. [Google Scholar]
- Woodward, F.A. An Improved Method for the Aerodynamic Analysis of Wing-Body-Tail Configurations in Subsonic and Supersonic Flow; NASA CR-2228; NASA: Washington, DC, USA, 1973.
- Morino, L. A General Theory of Unsteady Compressible Potential Aerodynamics; NASA CR-2464; NASA: Washington, DC, USA, 1974.
- Blair, M. A Compilation for the Mathematics Leading to the Doublet Lattice Method; WL-TR-92-3028; USAF: Washington, DC, USA, 1992.
- Fossati, M. Evaluation of Aerodynamic Loads via Reduced-Order Methodology. AIAA J. 2015, 53, 2389–2405. [Google Scholar] [CrossRef]
- Thwapiah, G.; Campanile, L.F. Nonlinear Aeroelastic Behavior of Compliant Aerofoils. Smart Mater. Struct. 2010, 19, 253–262. [Google Scholar] [CrossRef]
- Cone, C.D. The Theory of Induced Lift and Minimum Induced Drag of Non-Planar Lifting Systems; NASA TR R-139; NASA: Washington, DC, USA, 1962.
- Forrester, A.J.; Keane, A.J. Engineering Design via Surrogate Modelling: A Practical Guide; Wiley: Chichester, UK, 2008. [Google Scholar]
- Berci, M.; Gaskell, P.H.; Hewson, R.W.; Toropov, V.V. Multifidelity Metamodel Building as a Route to Aeroelastic Optimization of Flexible Wings. J. Mech. Eng. Sci. 2011, 225, 2115–2137. [Google Scholar] [CrossRef]
- Tiffany, S.H.; Adams, W.M. Nonlinear Programming Extensions to Rational Function Approximation Methods for Unsteady Aerodynamic Forces; NASA-TP-2776; NASA: Washington, DC, USA, 1988.
- Garrick, L.E. On Some Reciprocal Relations in the Theory of Nonstationary Flows; NACA-629; NACA: Washington, DC, USA, 1938. [Google Scholar]
- Heaslet, M.A.; Spreiter, J.R. Reciprocity Relations in Aerodynamics; NACA-1119; NACA: Washington, DC, USA, 1953. [Google Scholar]
- Beddoes, T.S. Practical Computation of Unsteady Lift. Vertica 1984, 8, 55–71. [Google Scholar]
- Dowell, E.H. A Simple Method for Converting Frequency Domain Aerodynamics to the Time Domain; NASA-TM-81844; NASA: Washington, DC, USA, 1980.
- Leishman, J.G.; Nguyen, K.Q. State-Space Representation of Unsteady Airfoil Behavior. AIAA J. 1990, 28, 836–844. [Google Scholar] [CrossRef]
- Tobak, M. On the Use of the Indicial-Function Concept in the Analysis of Unsteady Motions of Wings and Wing-Tail Combinations; NACA 1188; NACA: Washington, DC, USA, 1954. [Google Scholar]
- Silva, W. Discrete-Time Linear and Nonlinear Aerodynamic Impulse Responses for Efficient Use of CFD Analyses. Ph.D. Thesis, College of William & Mary, Williamsburg, VA, USA, 1997. [Google Scholar]
- Ghoreyshi, M.; Jirásek, A.; Cummings, R.M. Computational Investigation into the Use of Response Functions for Aerodynamic-Load Modeling. AIAA J. 2012, 50, 1314–1327. [Google Scholar] [CrossRef]
- Rodden, W.P. Theoretical and Computational Aeroelasticity; Crest Pub.: Columbus, OH, USA, 2011. [Google Scholar]
- Van Zyl, L.H. Aeroelastic Divergence and Aerodynamic Lag Roots. J. Aircr. 2001, 38, 586–588. [Google Scholar] [CrossRef]
- Aeroelastic Analysis User’s Guide. In MSC Nastran; MSC Software Corporation: Newport Beach, CA, USA, 2018.
- Appa, K. Finite-Surface Spline. J. Aircr. 1989, 26, 495–496. [Google Scholar] [CrossRef]
- Harder, R.L.; Desmarais, R.N. Interpolation Using Surface Splines. J. Aircr. 1972, 9, 189–191. [Google Scholar] [CrossRef]
- Lanczos, C. An Iteration Method for the Solution of the Eigenvalue Problem of Linear Differential and Integral Operators; United States Governm. Press Office: Los Angeles, CA, USA, 1950; Volume 45.
- Bellinger, D.; Pototzky, T. A Study of Aerodynamic Matrix Numerical Condition. In Proceedings of the 3rd MSC Worldwide Aerospace Conference and Technology Showcase, Toulouse, France, 24–26 September 2001. [Google Scholar]
- Giesing, J.P.; Kalman, T.P.; Rodden, W.P. Correction Factor Techniques for Improving Aerodynamic Prediction Methods; NASA CR-144967; NASA: Washington, DC, USA, 1976.
- Lawrence, A.J.; Jackson, P. Comparison of Different Methods of Assessing the Free Oscillatory Characteristics of Aeroelastic Systems; ARC CP 1084; HM Stationery Office: London, UK, 1970. [Google Scholar]
- Goland, M. The Flutter of a uniform Cantilever Wing. J. Appl. Mech. 1945, 12, A197–A208. [Google Scholar]
- Goland, M.; Luke, Y.L. The Flutter of a Uniform Wing with Tip Weights. J. Appl. Mech. 1948, 15, 13–20. [Google Scholar]
- Wang, I. Component Modal Analysis of a Folding Wing. Ph.D. Thesis, Duke University, Durham, NC, USA, 2011. [Google Scholar]
- NASA. U.S. Standard Atmosphere; NASA TM-X-74335; NASA: Washington, DC, USA, 1976.
- Banerjee, J.R. Flutter sensitivity studies of high aspect ratio aircraft wings. WIT Trans. Built Environ. 1993, 2, 374–387. [Google Scholar]
- Sotoudeh, Z.; Hodges, D.H.; Chang, C.S. Validation Studies for Aeroelastic Trim and Stability Analysis of Highly Flexible Aircraft. J. Aircr. 2010, 47, 1240–1247. [Google Scholar] [CrossRef]
- Qin, Z. Vibration and Aeroelasticity of Advanced Aircraft Wings Modeled as Thin-Walled Beams—Dynamics, Stability and Control. Ph.D. Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2001. [Google Scholar]
- Palacios, R.; Epureanu, B. An Intrinsic Description of the Nonlinear Aeroelasticity of Very Flexible Wings; AIAA-2011-1917; AIAA: Reston, VA, USA, 2011. [Google Scholar]
- Dunning, P.D.; Stanford, B.K.; Kim, H.A.; Jutte, C.V. Aeroelastic Tailoring of a Plate Wing with Functionally Graded Materials. J. Fluids Struct. 2014, 51, 292–312. [Google Scholar] [CrossRef]
- Berci, M. Semi-Analytical Reduced-Order Models for the Unsteady Aerodynamic Loads of Subsonic Wings. In Proceedings of the 5th ECCOMAS COMPDYN, Crete, Greece, 25–27 May 2015. [Google Scholar]
- Hildebrand, F.B. A Least-Squares Procedure for the Solution of the Lifting-Line Integral Equation; NACA TN 925; NACA: Washington, DC, USA, 1944. [Google Scholar]
- Hoblit, F.M. Gust Loads on Aircraft: Concepts and Applications; AIAA Education Series; AIAA: Reston, VA, USA, 1988. [Google Scholar]
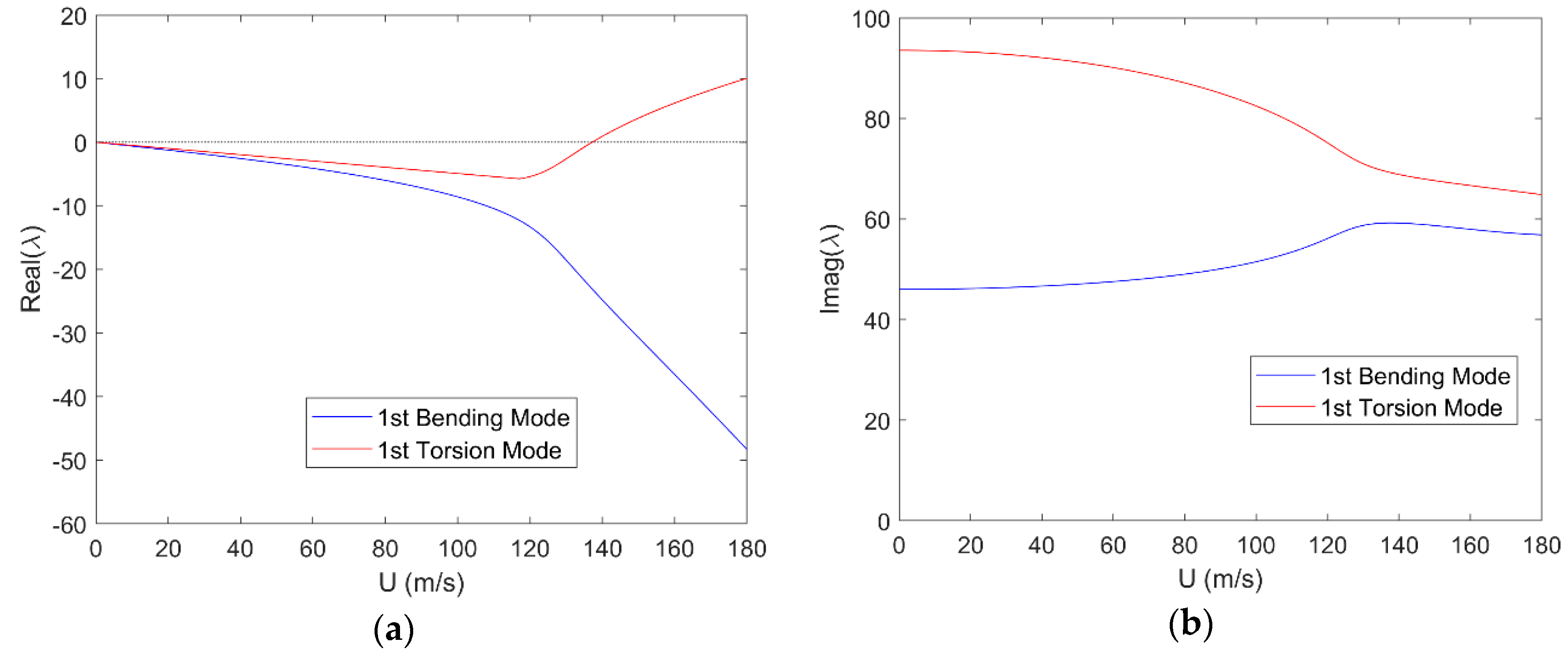
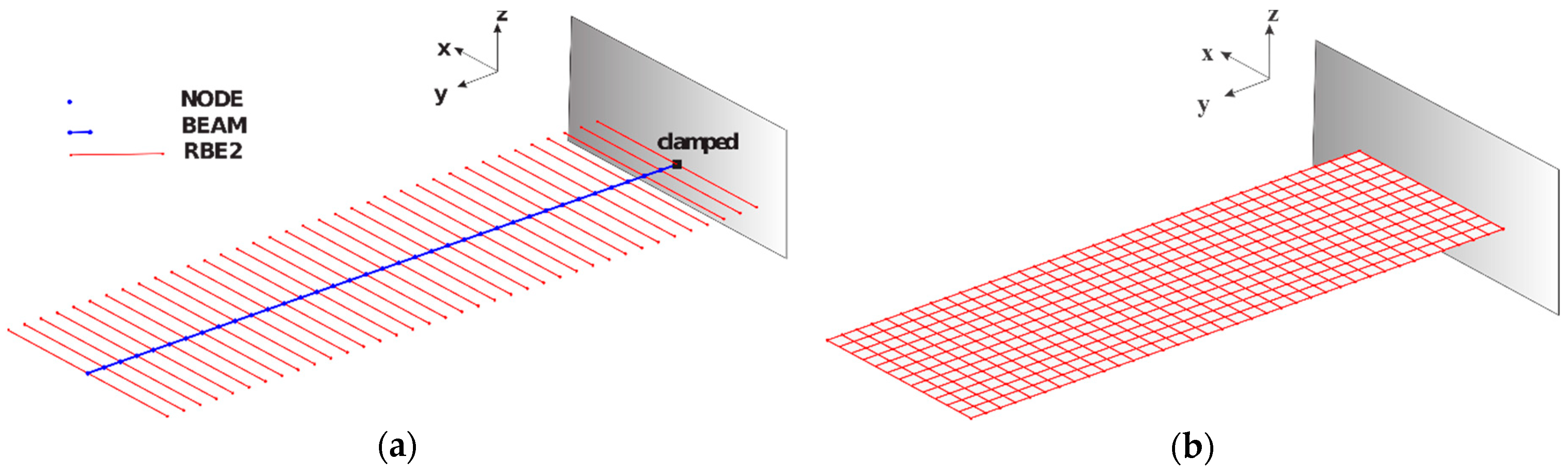
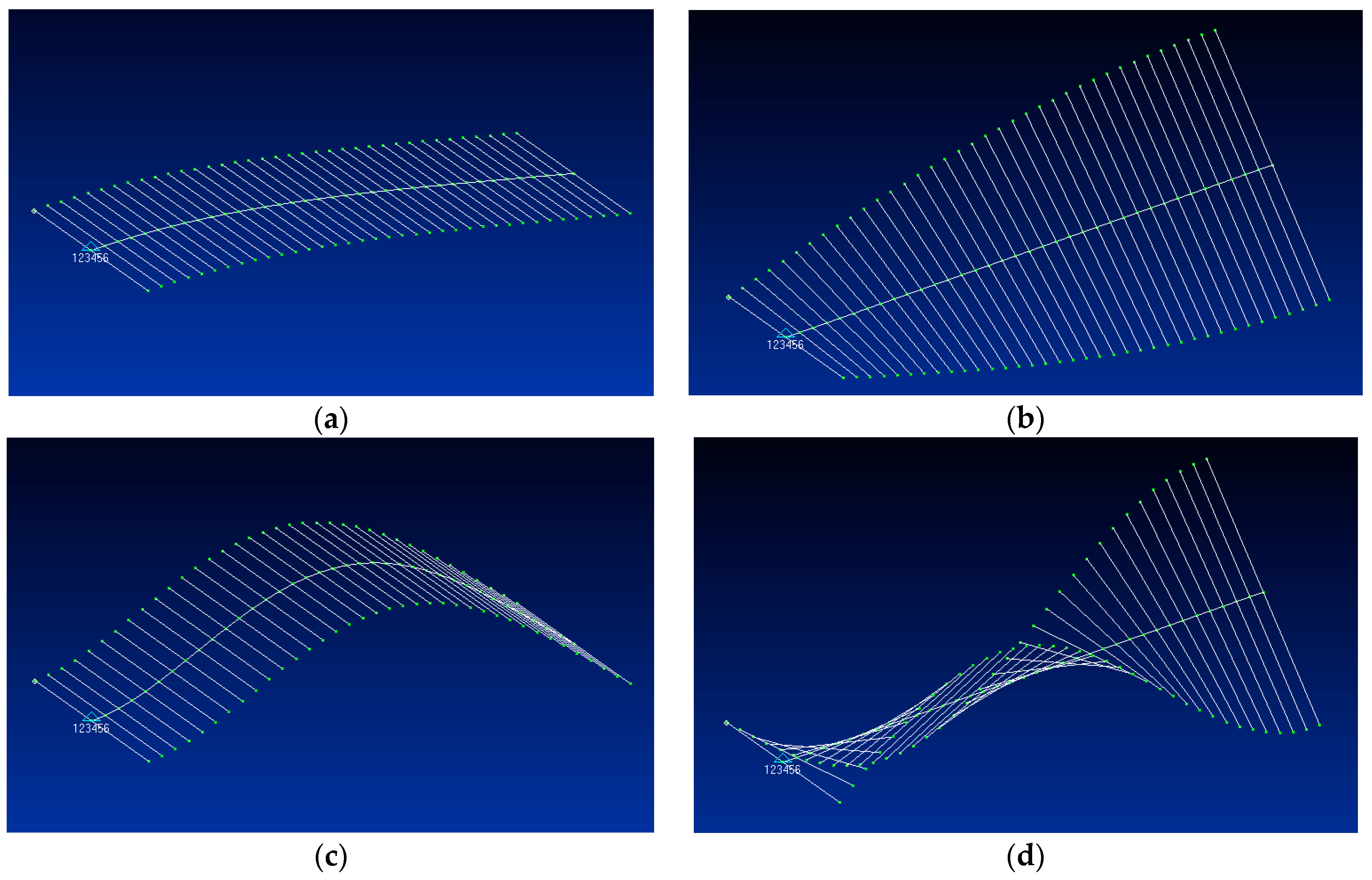
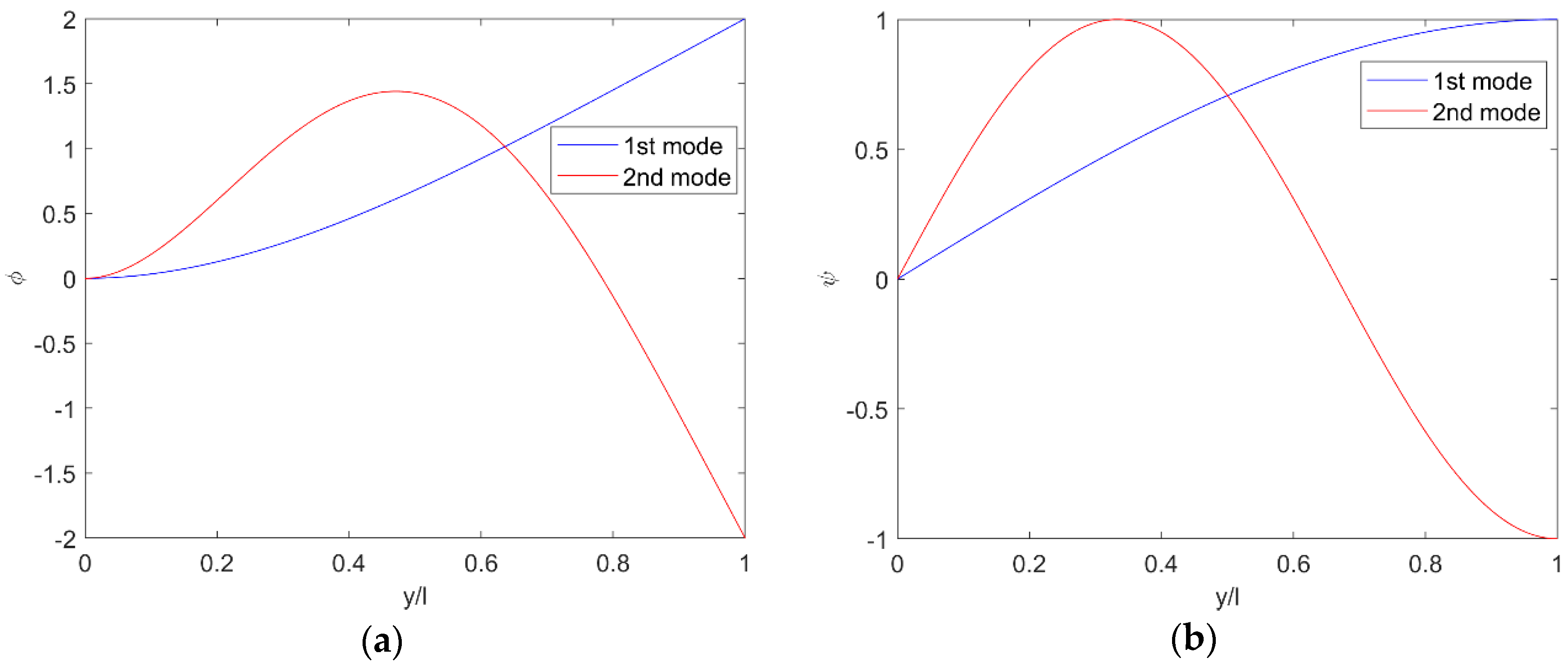


| AR | FEM: Nodes, Elements | DLM: Spanwise, Chordwise |
|---|---|---|
| 4 | 75, 24 | 24, 12 |
| 6 | 108, 36 | 36, 12 |
| 8 | 144, 48 | 48, 12 |
| AR | 1st Mode | 2nd Mode | 3rd Mode | 4th Mode |
|---|---|---|---|---|
| 4 | 1st Bending | 1st Torsion | 2nd Bending | 2nd Torsion |
| 6 | 1st Bending | 1st Torsion | 2nd Bending | 2nd Torsion |
| 8 | 1st Bending | 2nd Bending | 1st Torsion | 3rd Bending |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berci, M.; Cavallaro, R. A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment. Aerospace 2018, 5, 76. https://doi.org/10.3390/aerospace5030076
Berci M, Cavallaro R. A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment. Aerospace. 2018; 5(3):76. https://doi.org/10.3390/aerospace5030076
Chicago/Turabian StyleBerci, Marco, and Rauno Cavallaro. 2018. "A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment" Aerospace 5, no. 3: 76. https://doi.org/10.3390/aerospace5030076
APA StyleBerci, M., & Cavallaro, R. (2018). A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment. Aerospace, 5(3), 76. https://doi.org/10.3390/aerospace5030076






