Design of a Facility for Studying Shock-Cell Noise on Single and Coaxial Jets
Abstract
:1. Introduction
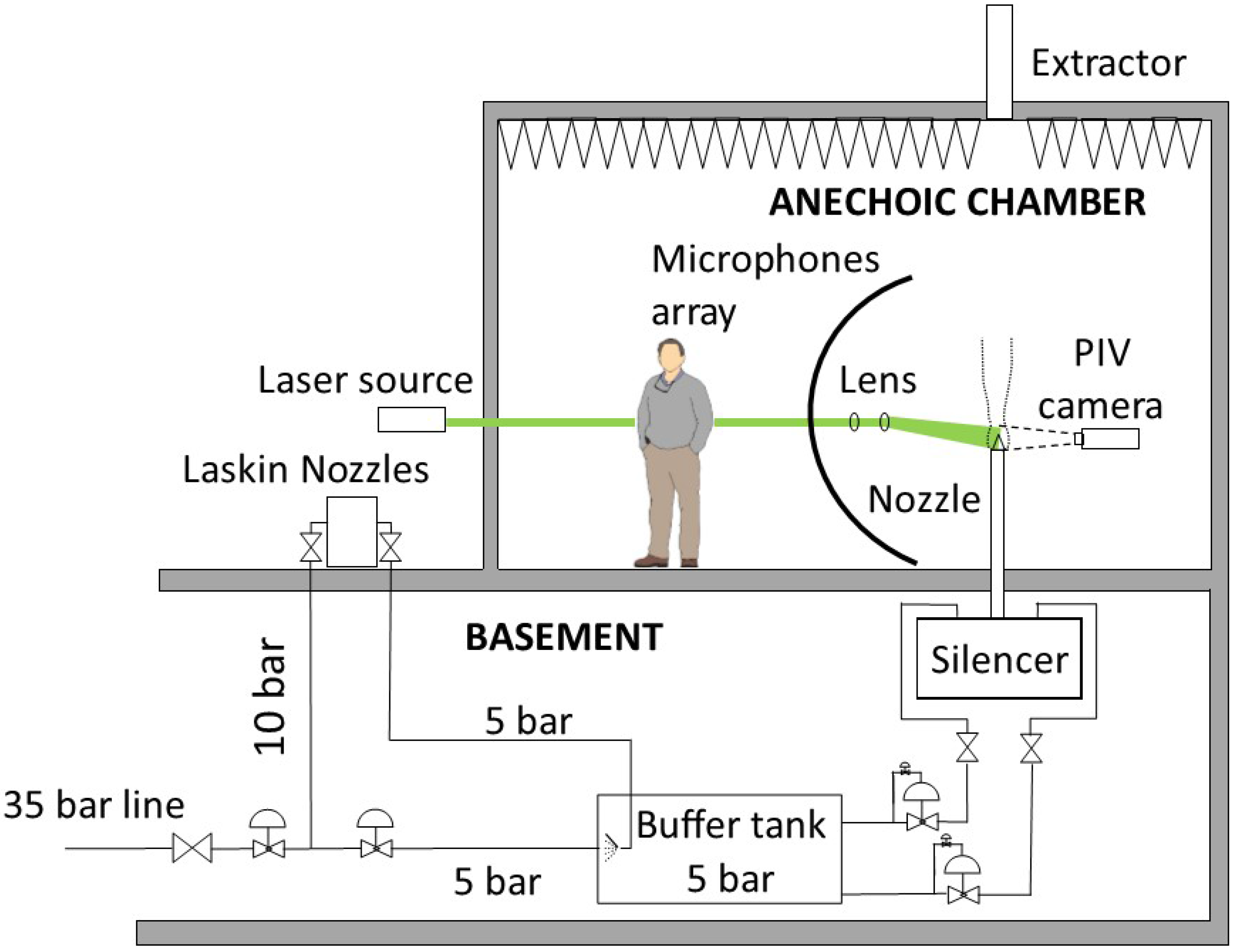
2. Facility Design Process
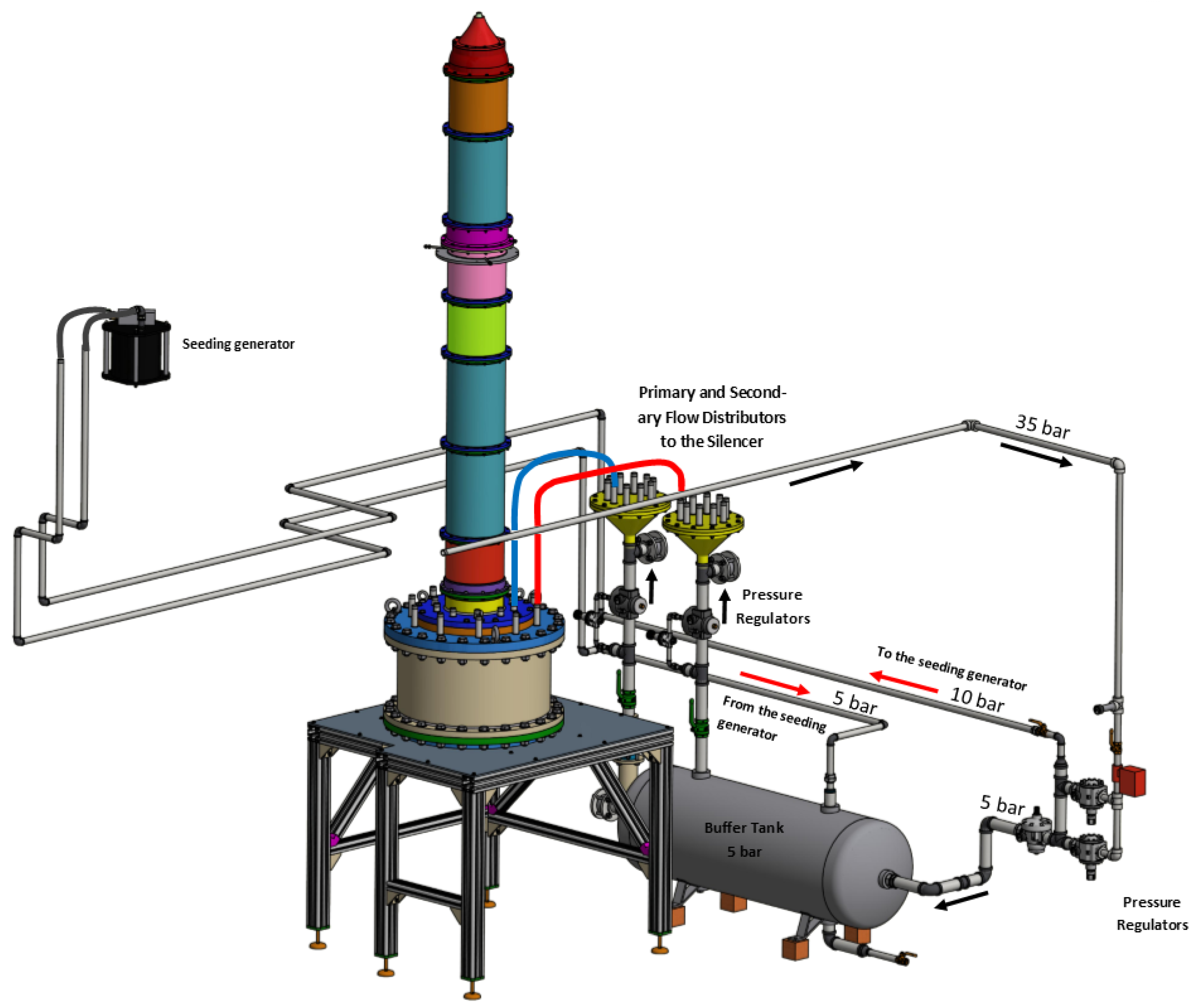
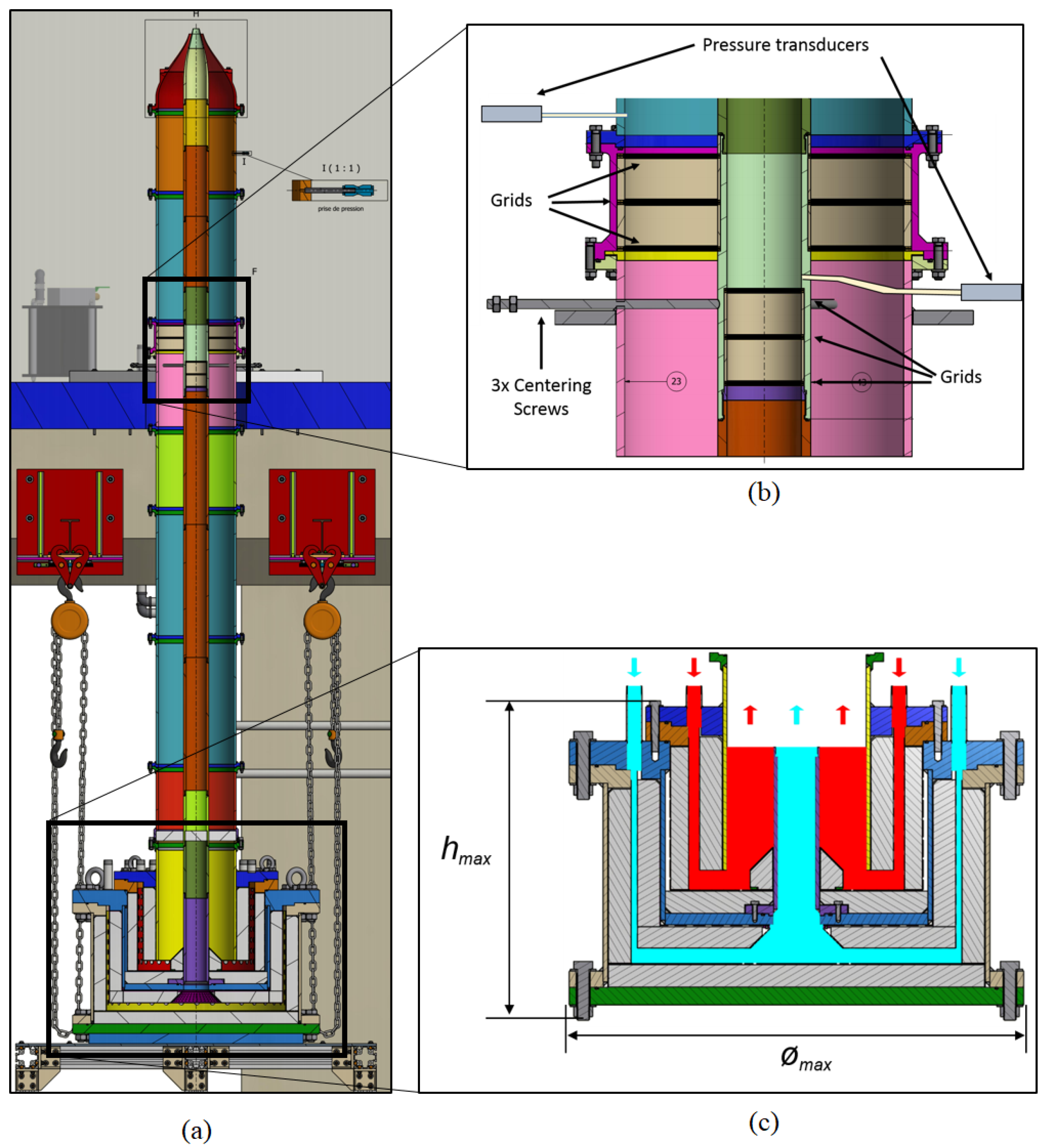
3. Facility Components
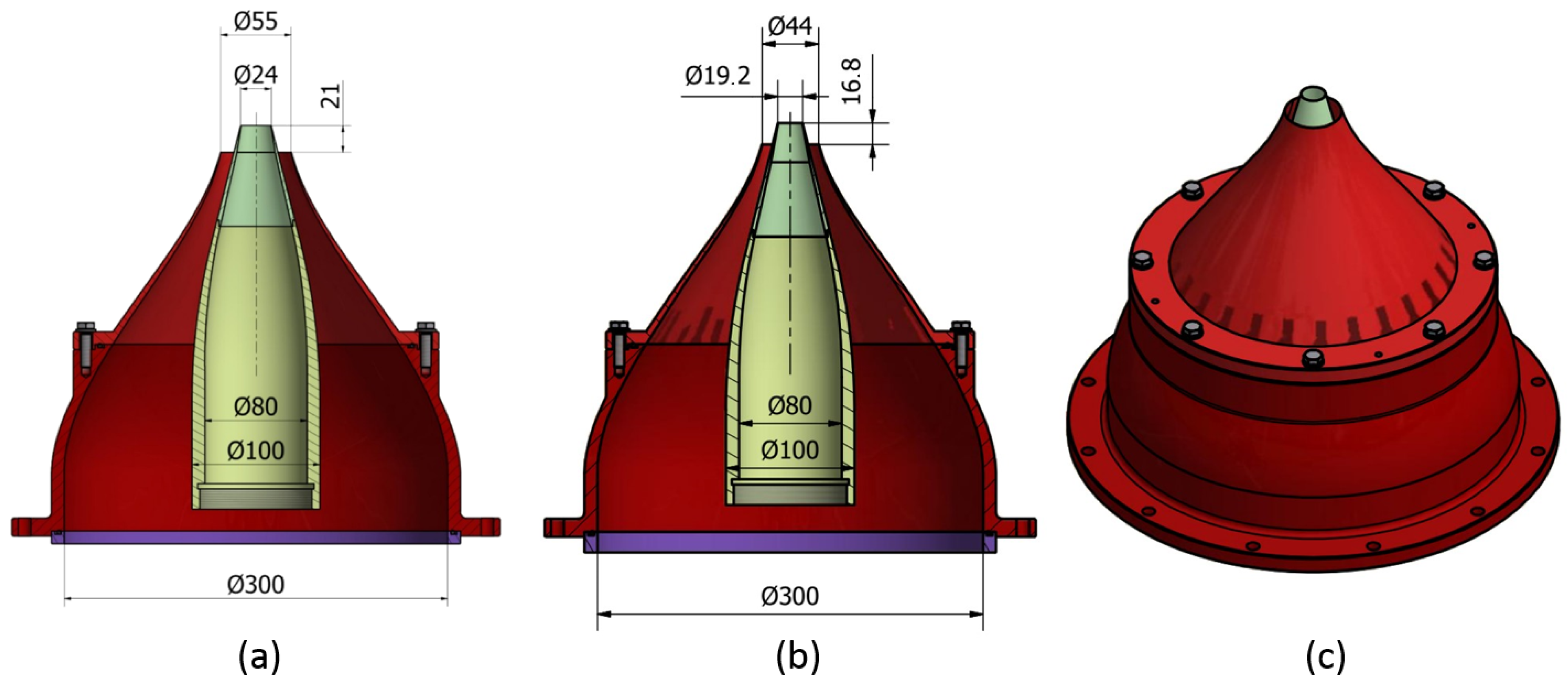
Coaxial Nozzles
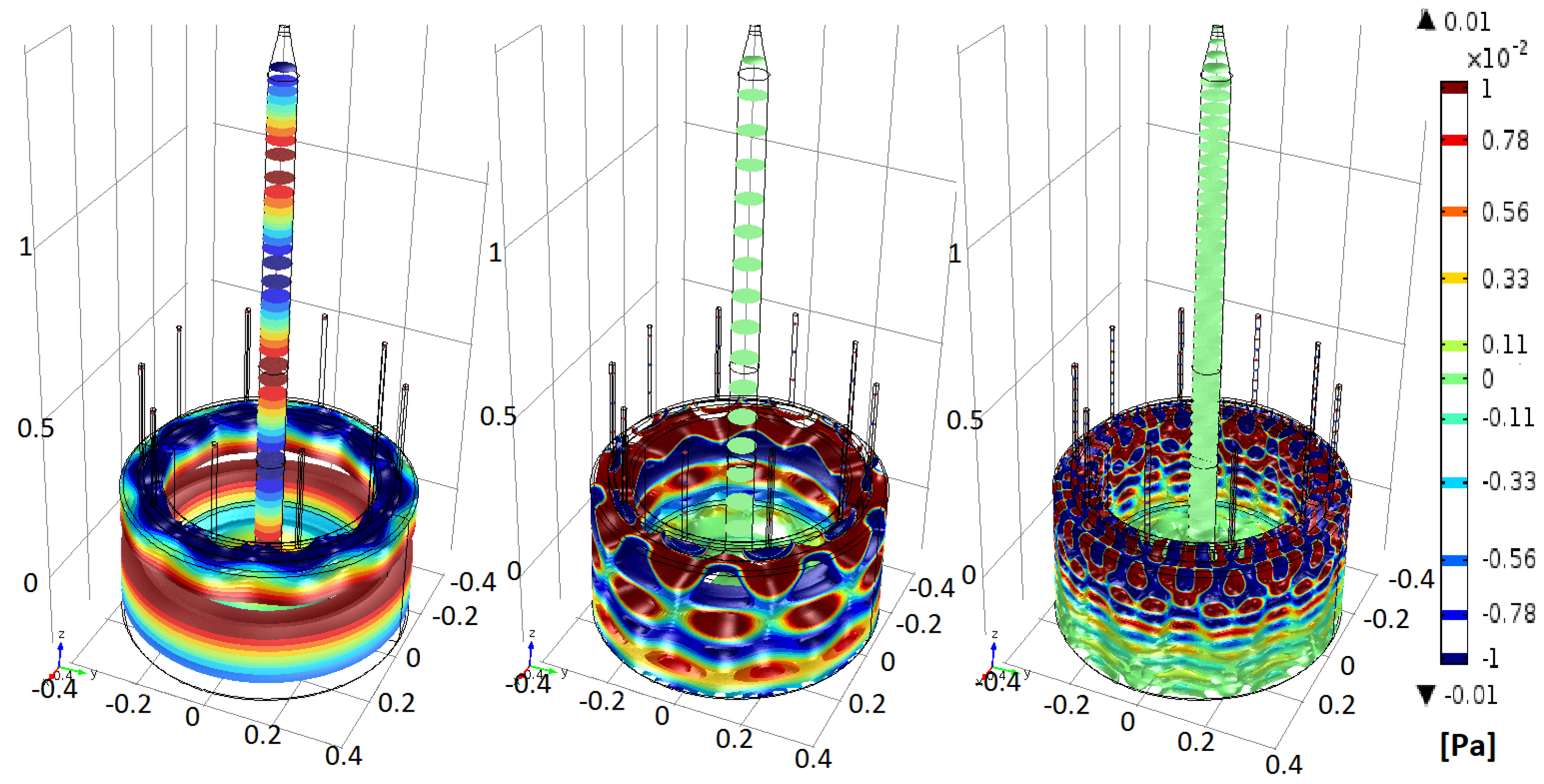
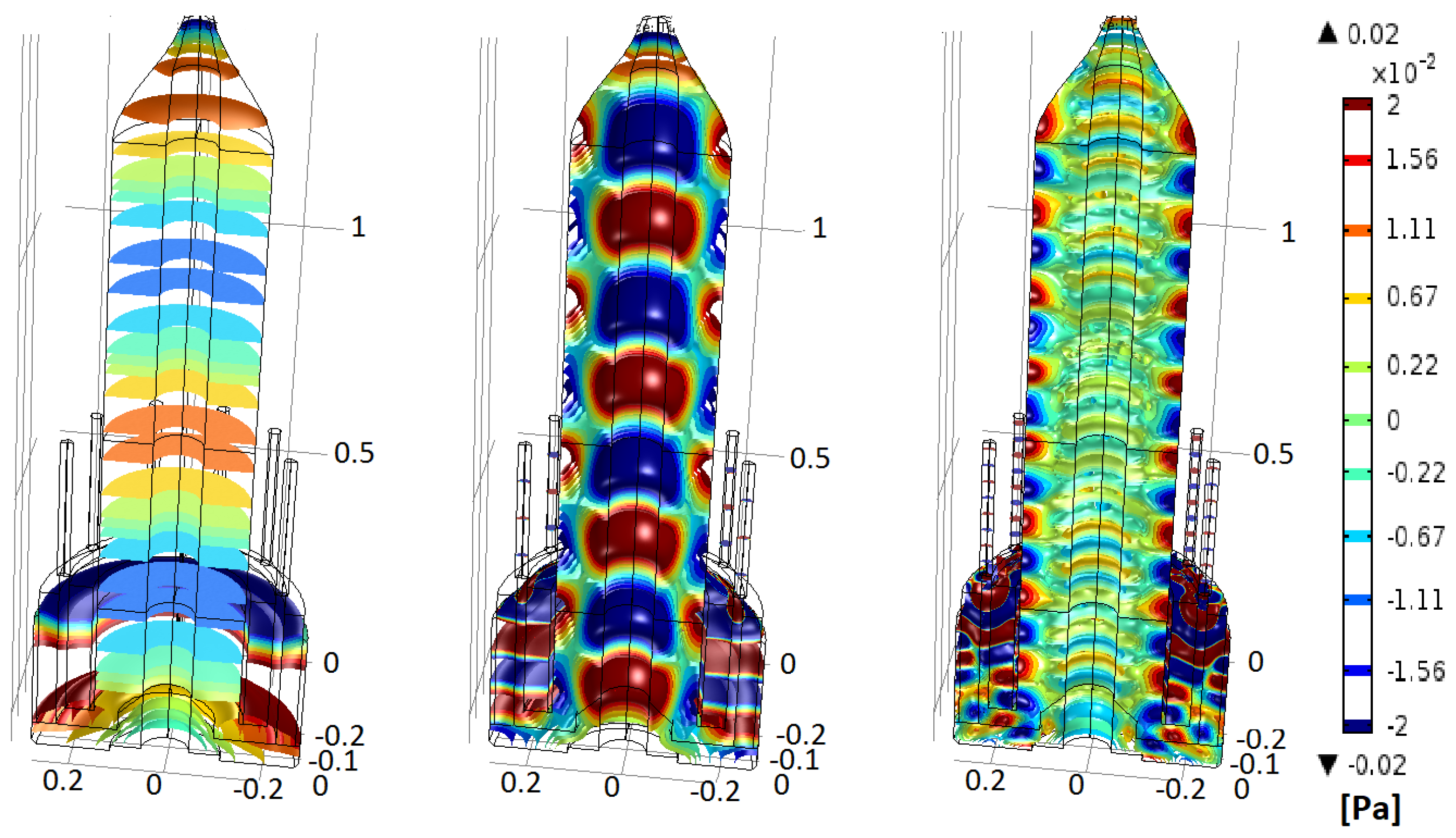
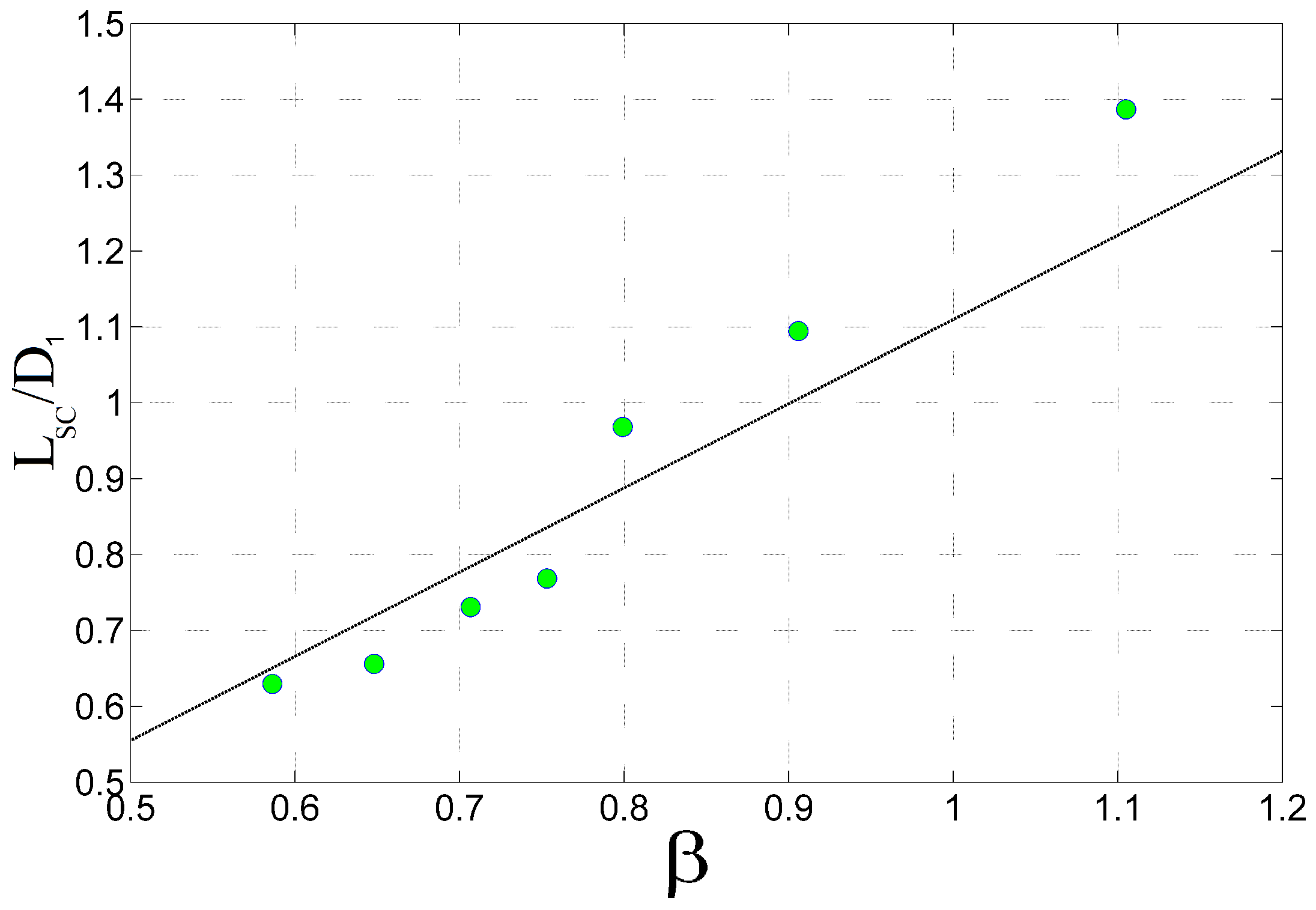
4. Silencer
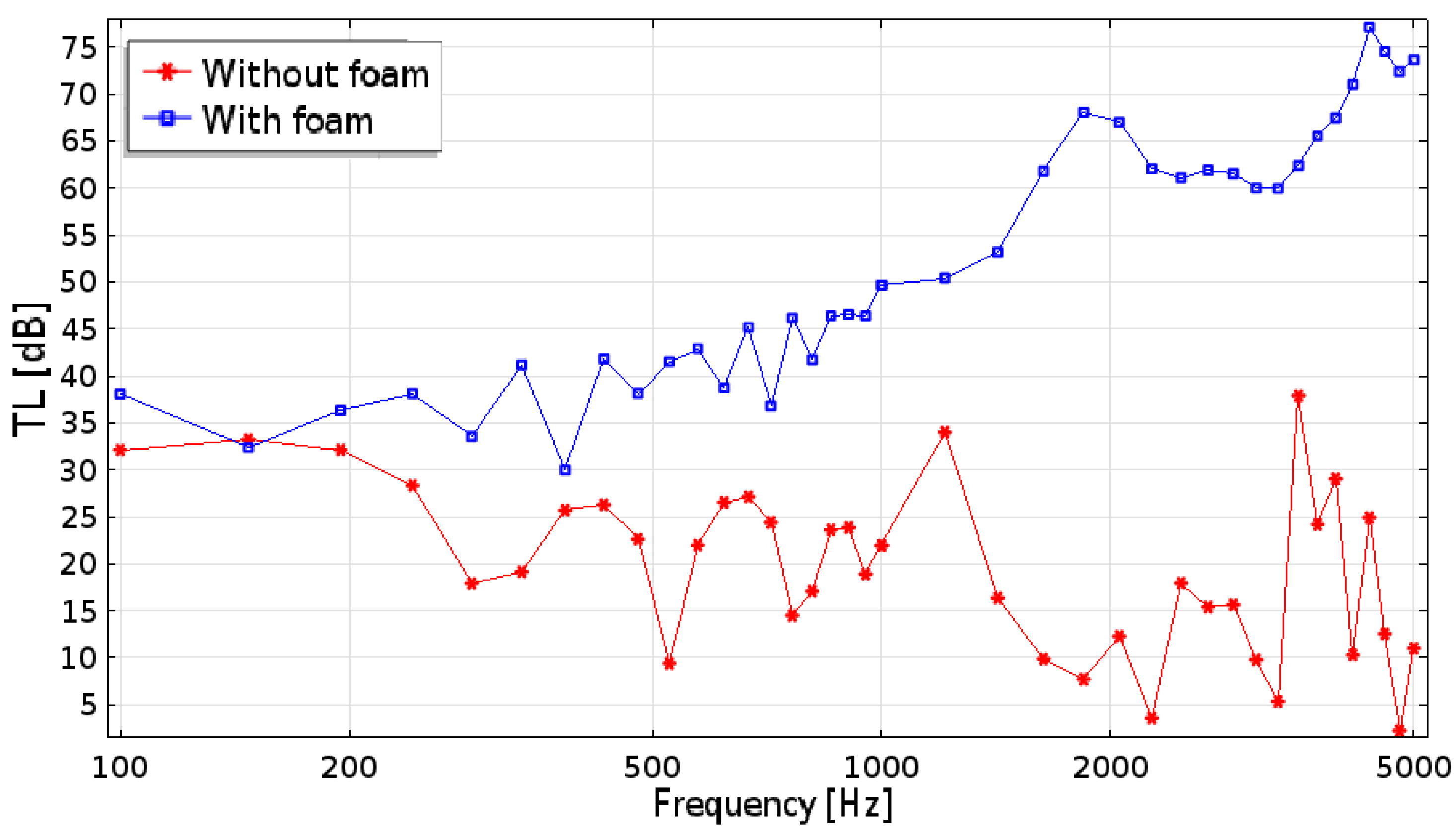
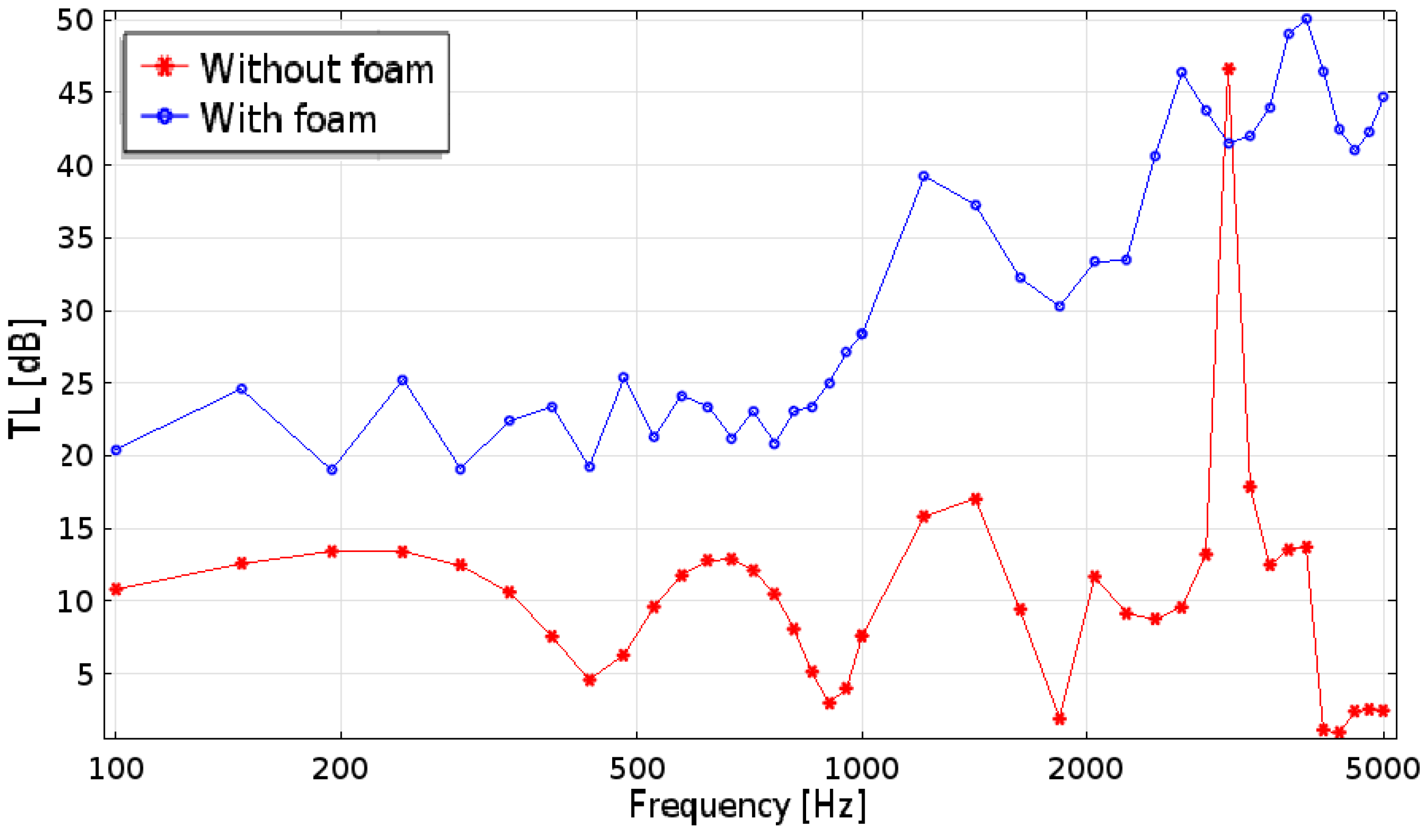
Acoustic Performance
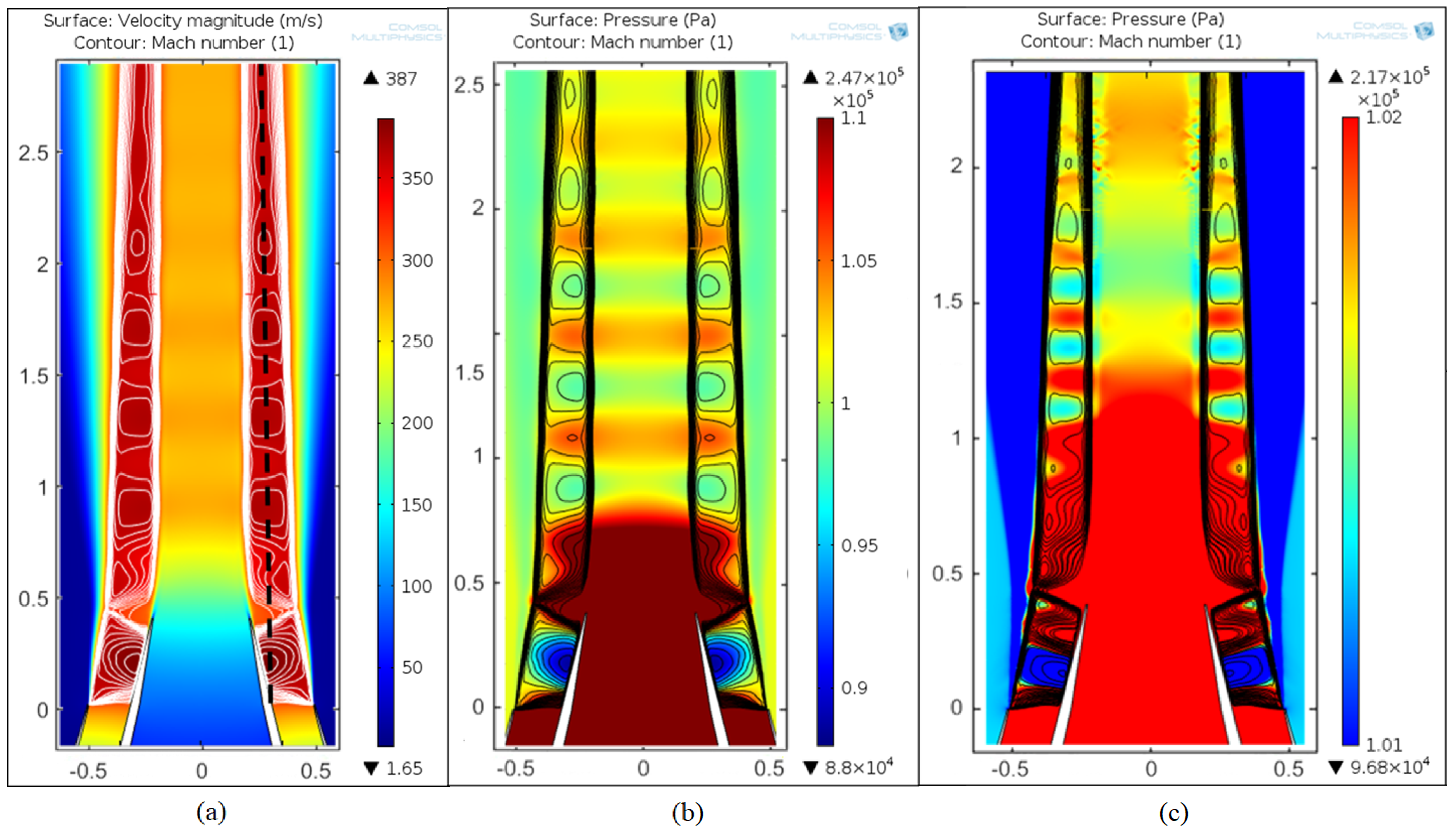
5. RANS Simulations of the Coaxial Jet
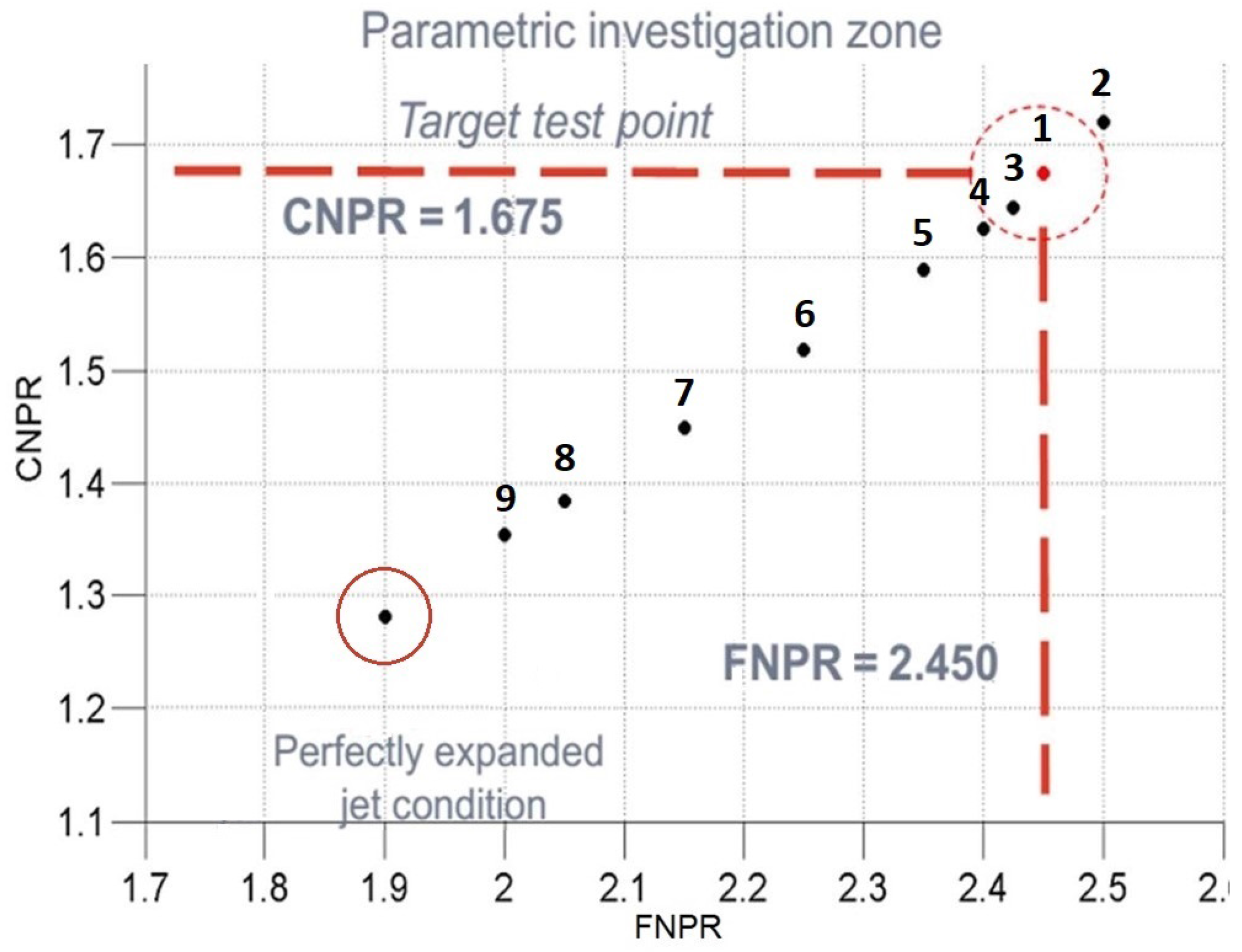
5.1. Test Conditions
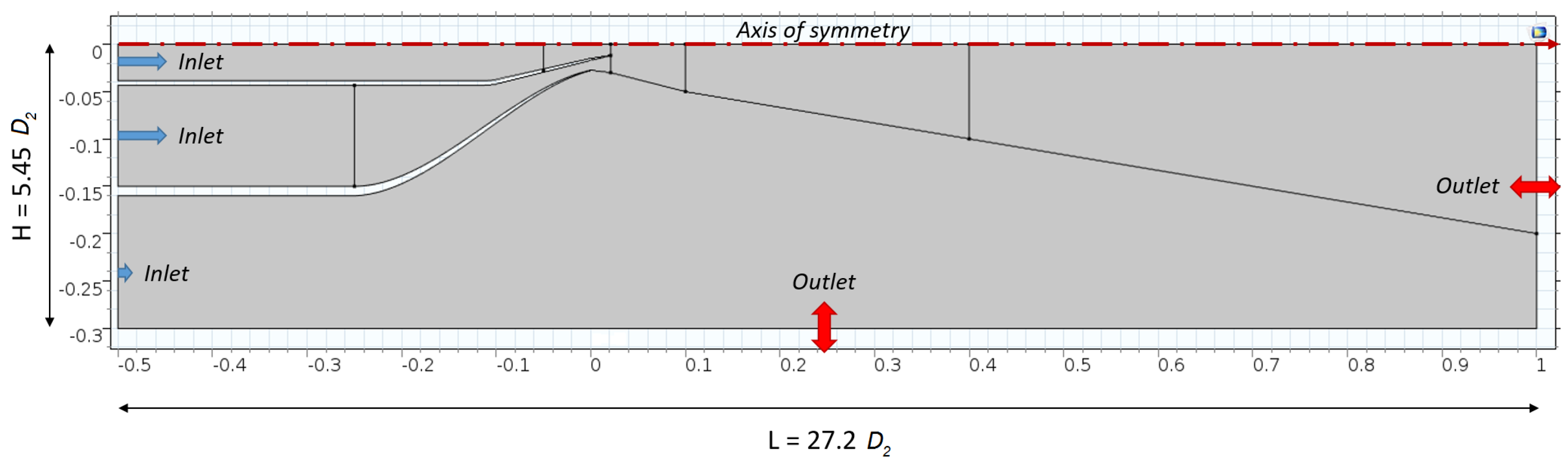
5.2. Fluid Dynamics Model
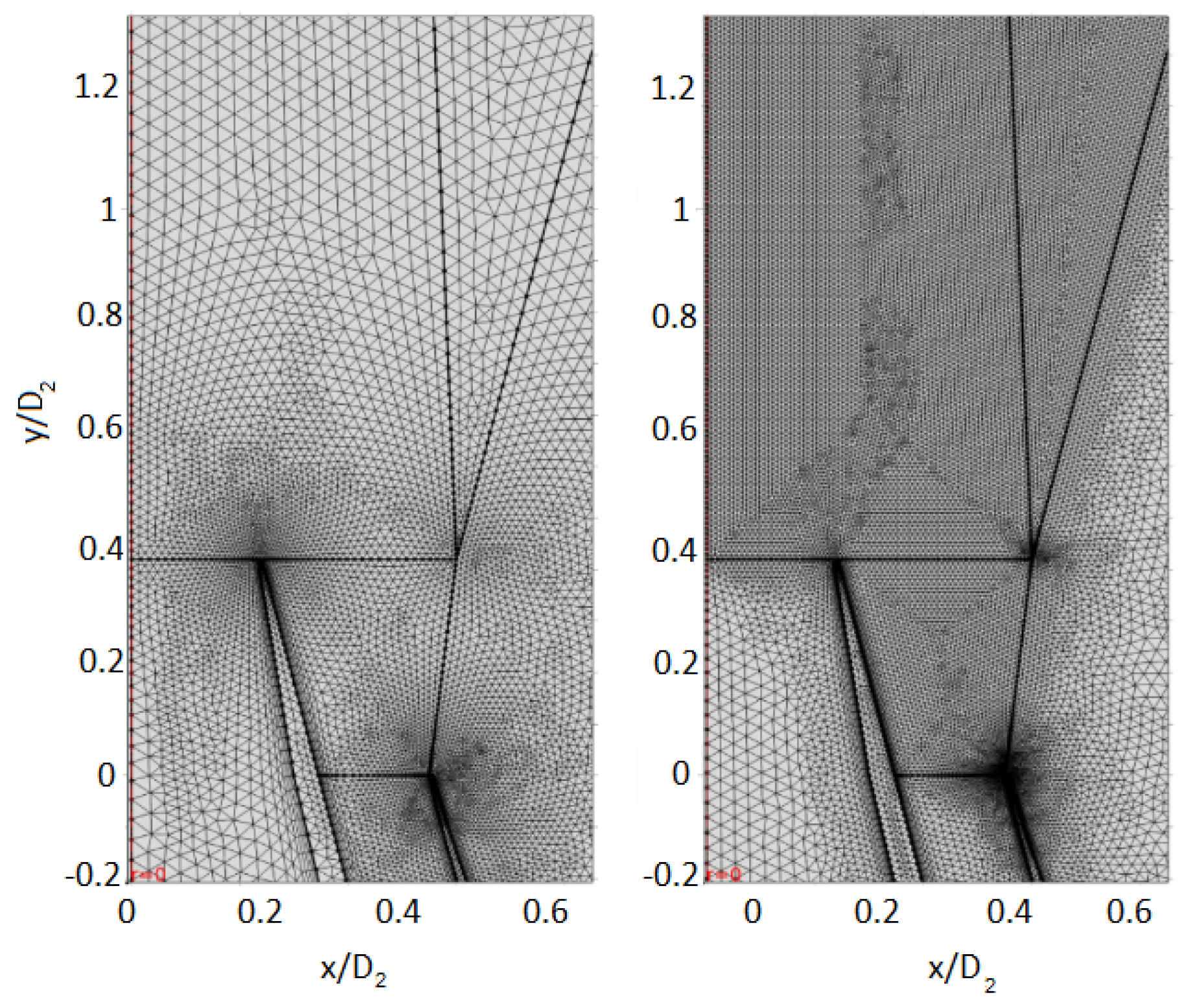
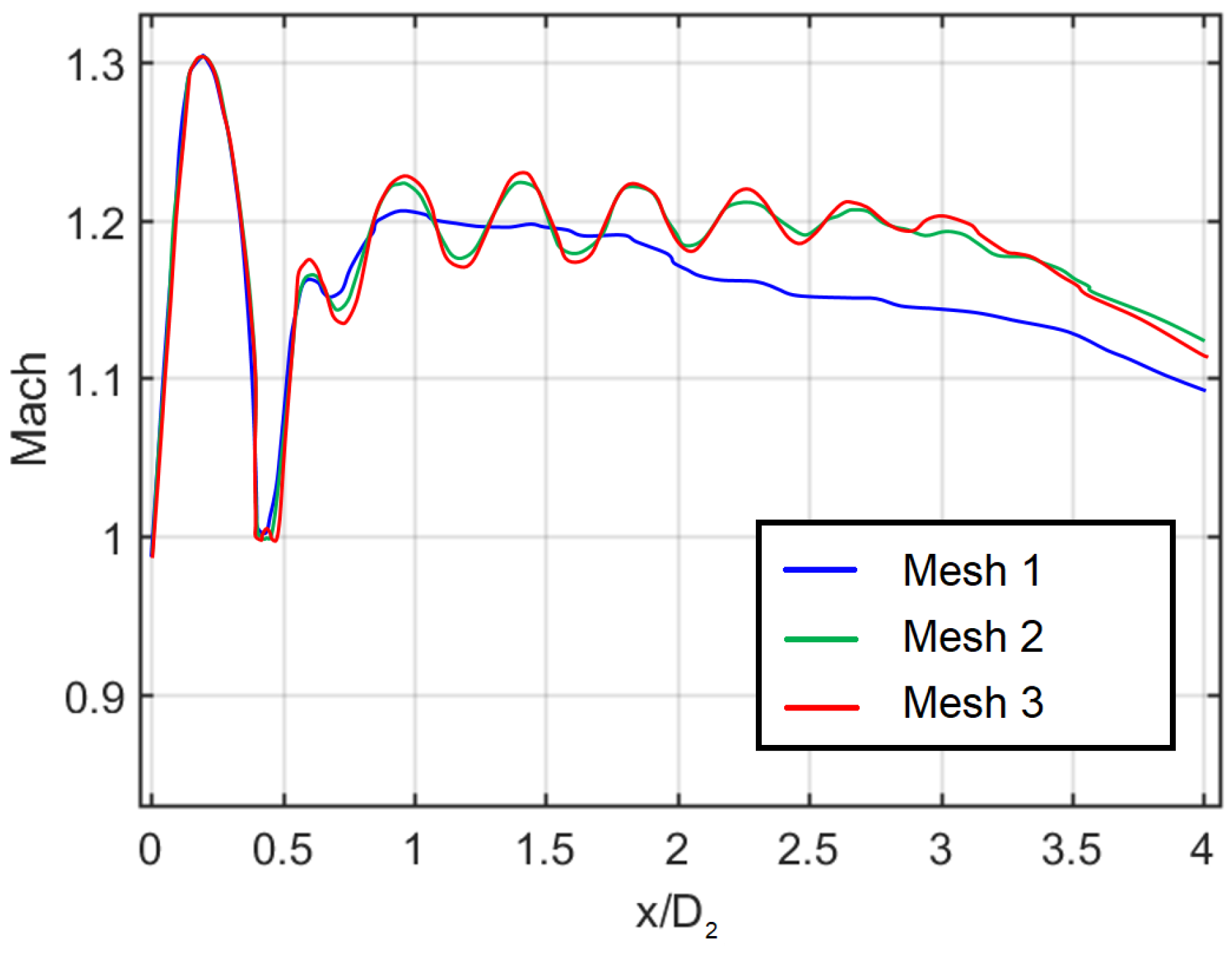
5.3. Mesh Sensitivity
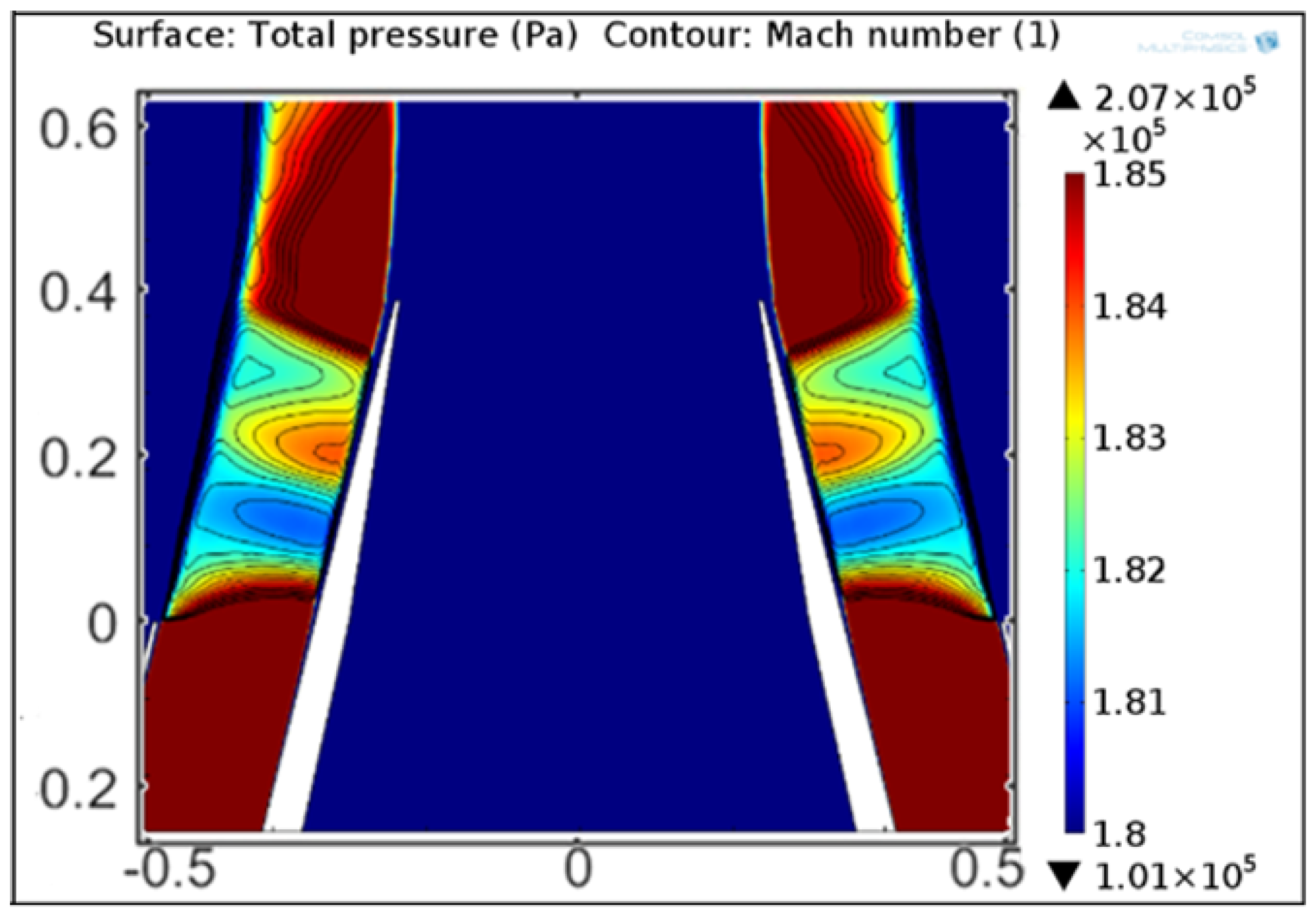
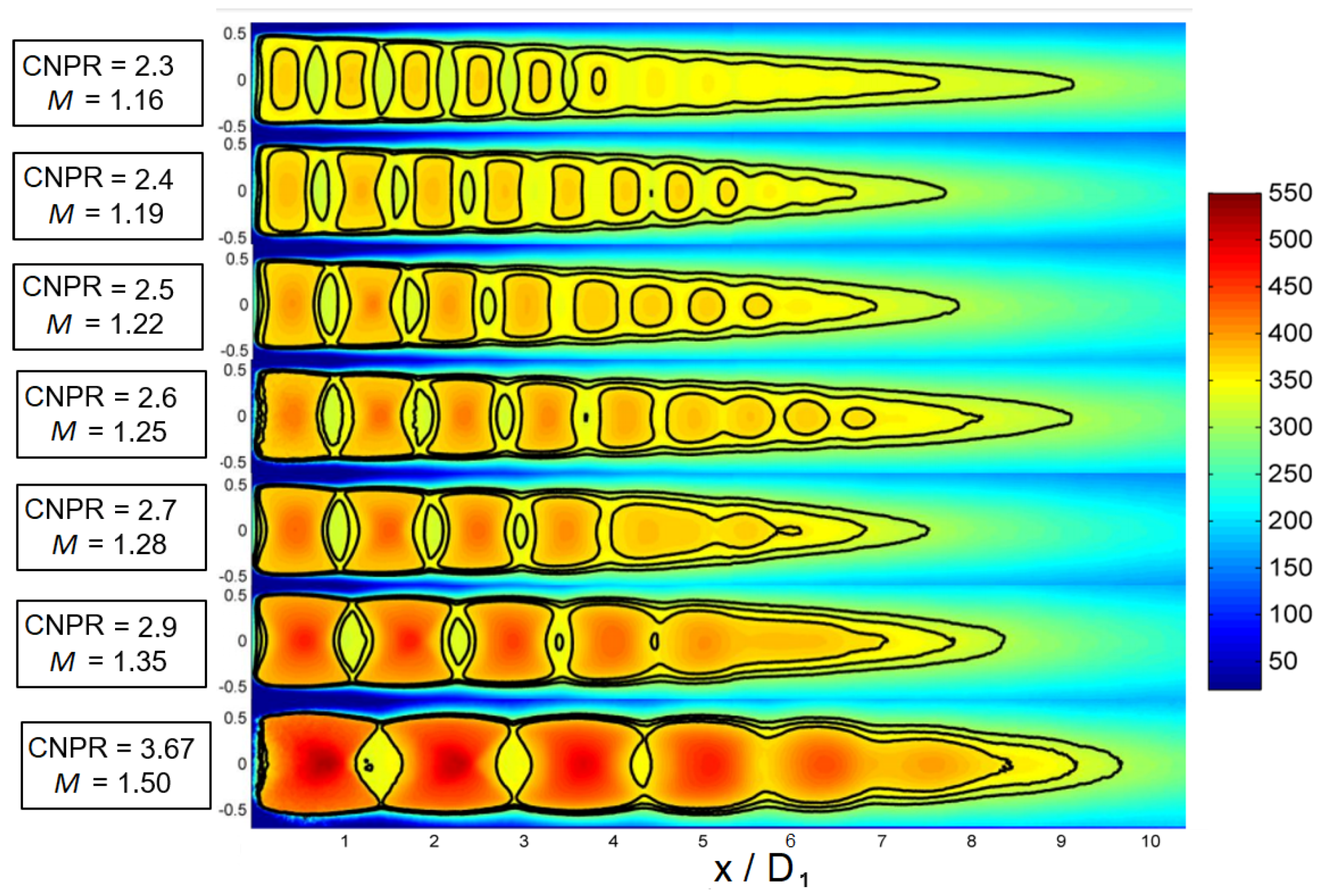
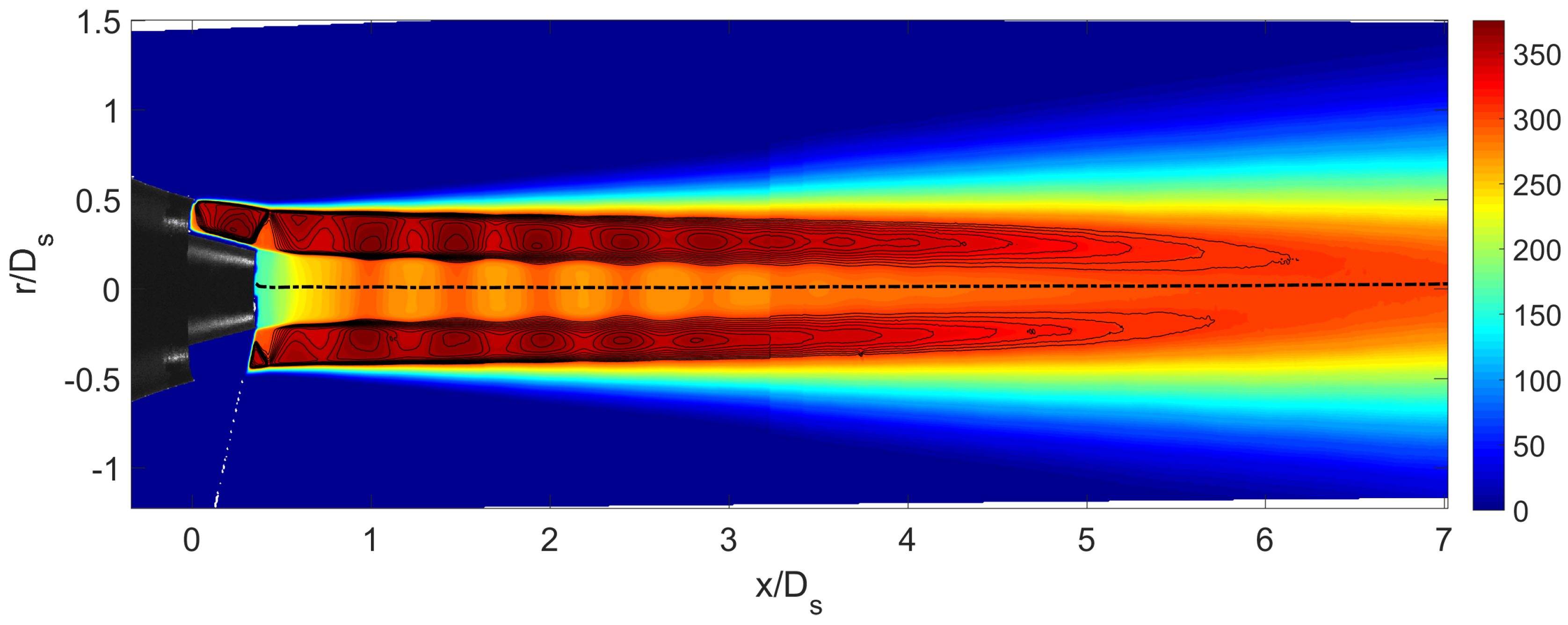
5.4. Results
6. Commissioning
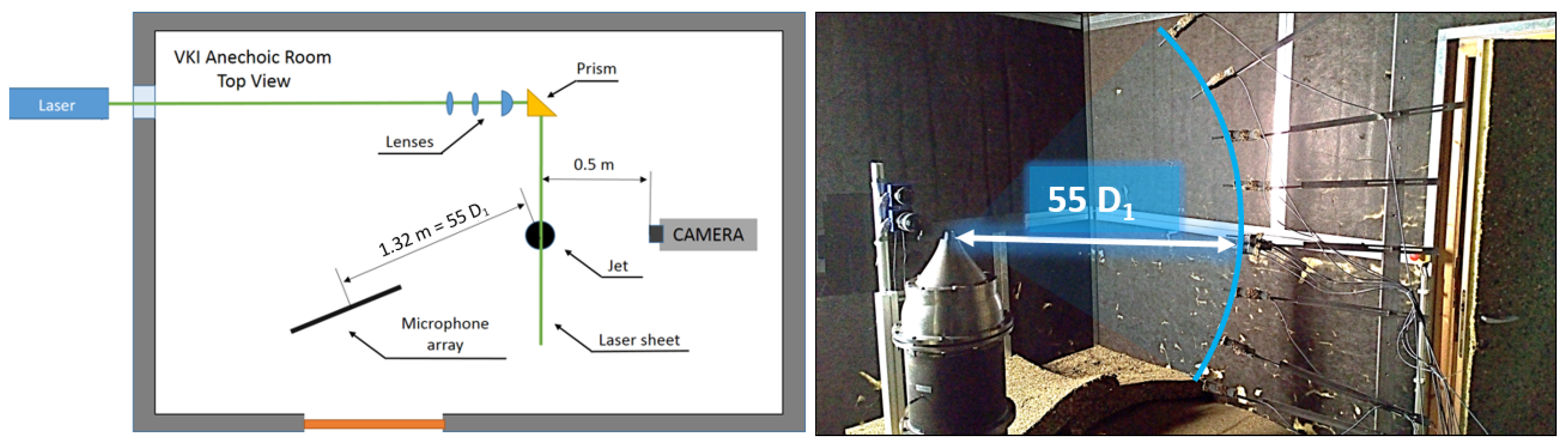
6.1. Experimental Setup
6.2. Operating Conditions
6.3. PIV Measurements
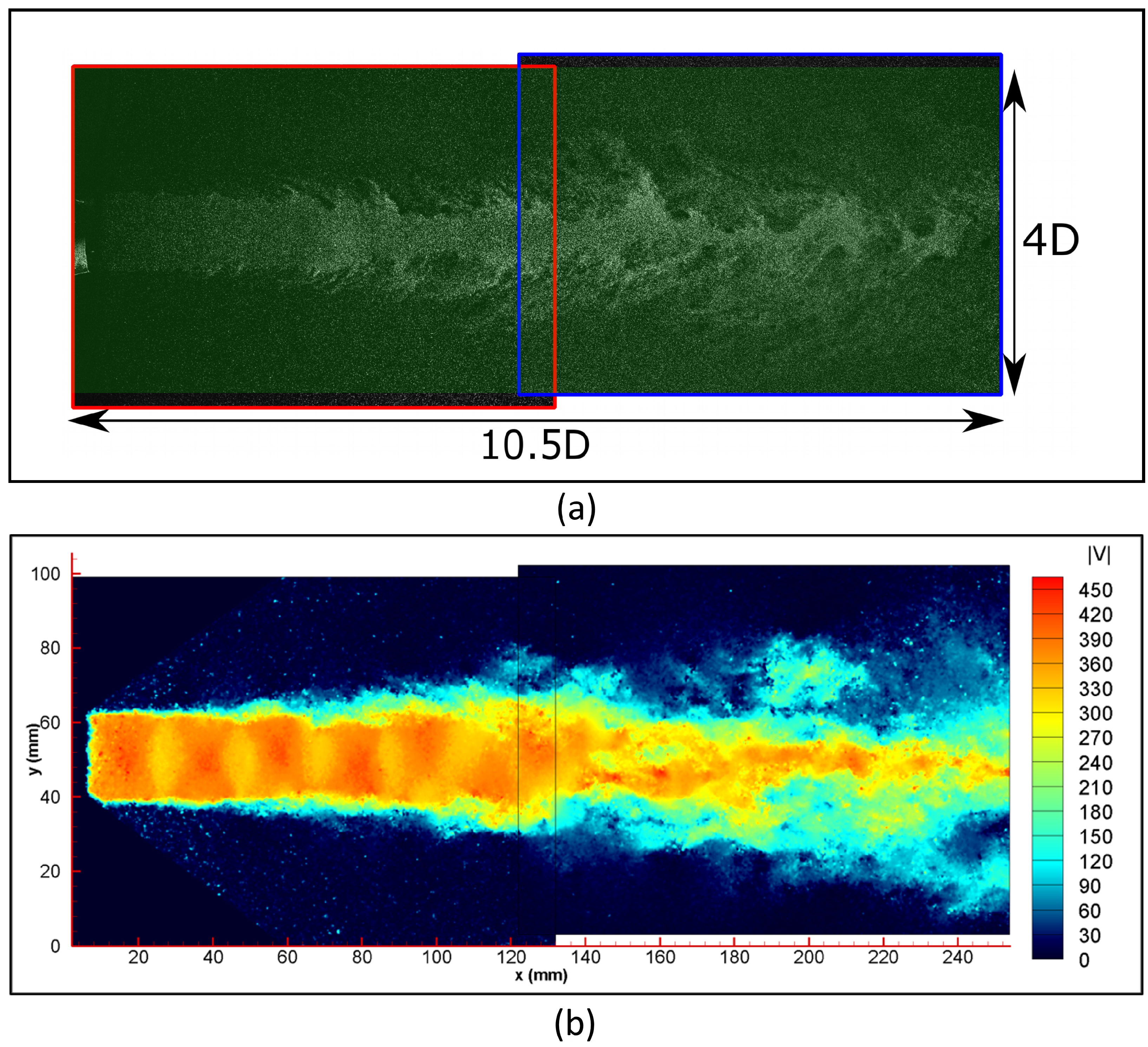
6.3.1. PIV Acquisition Procedure
6.3.2. PIV Equipment
6.3.3. Image Processing
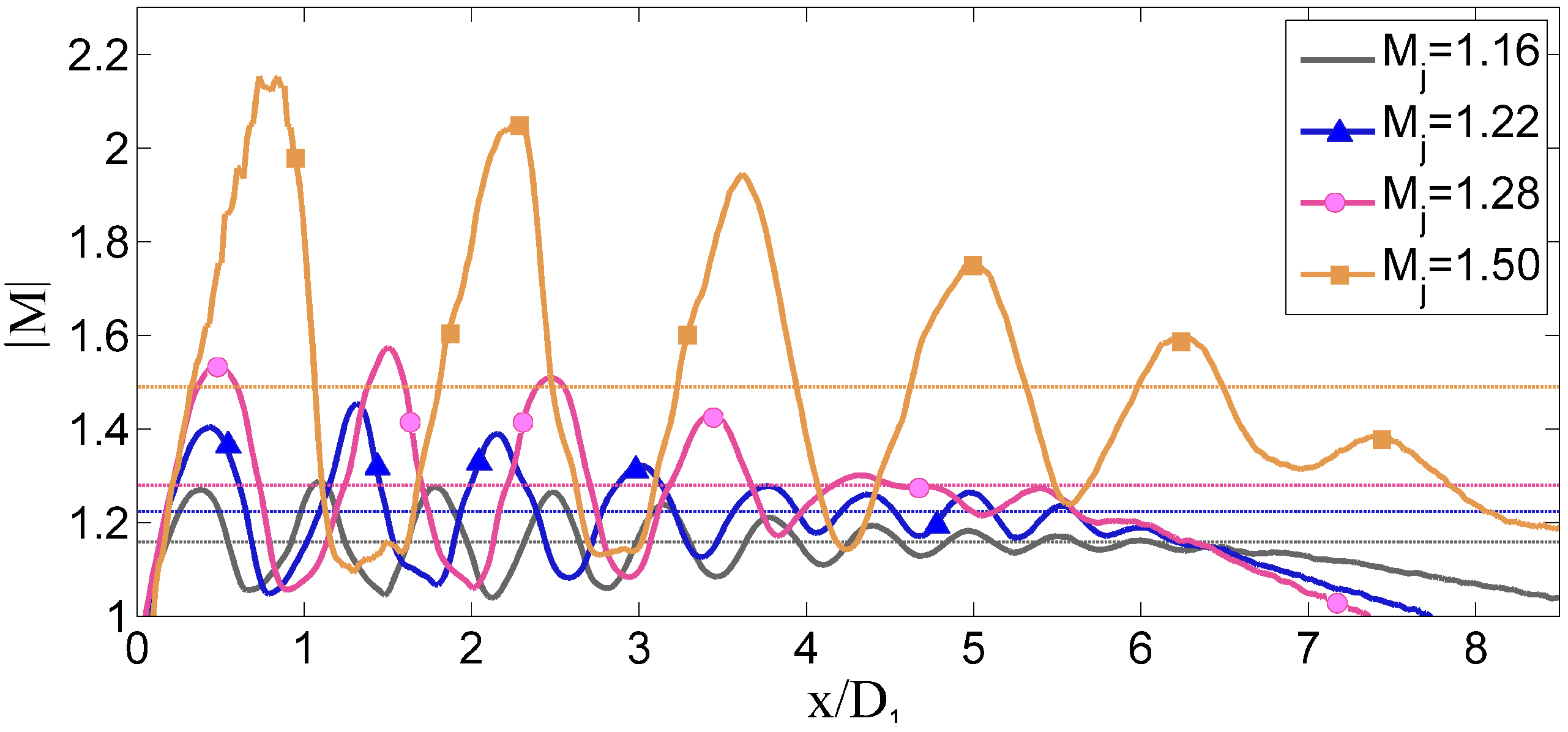
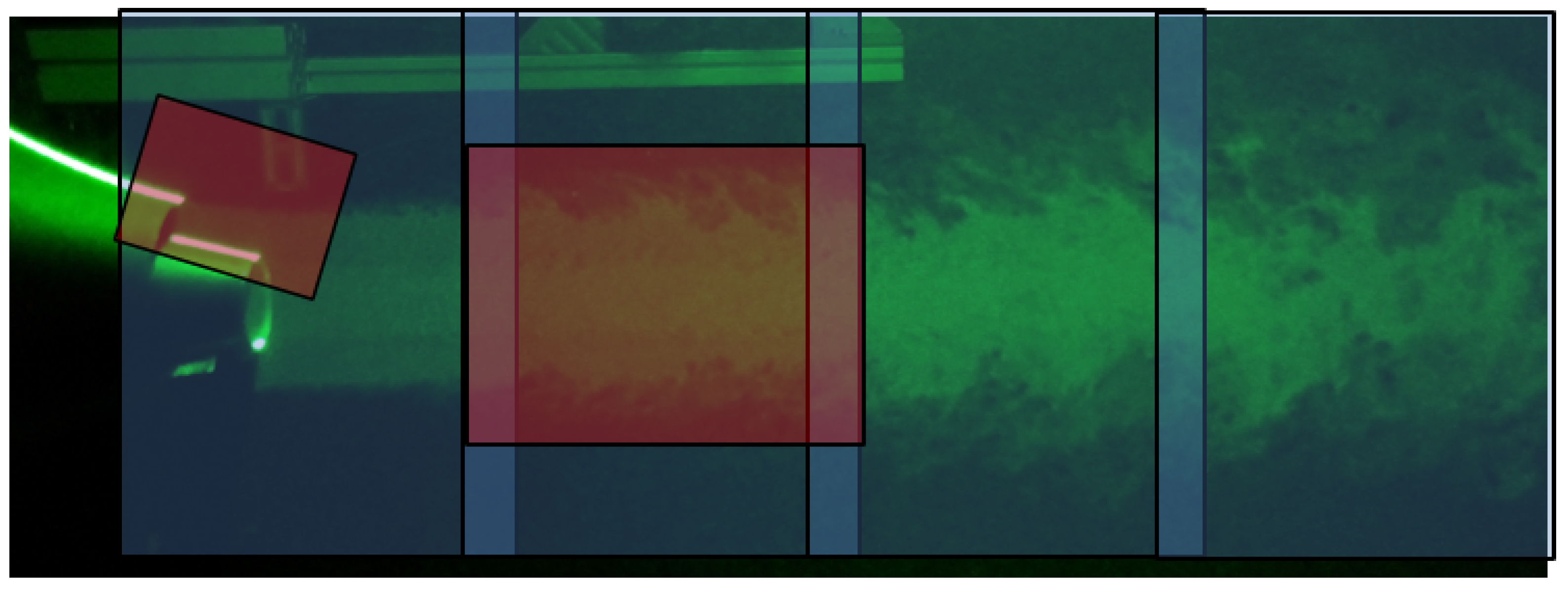
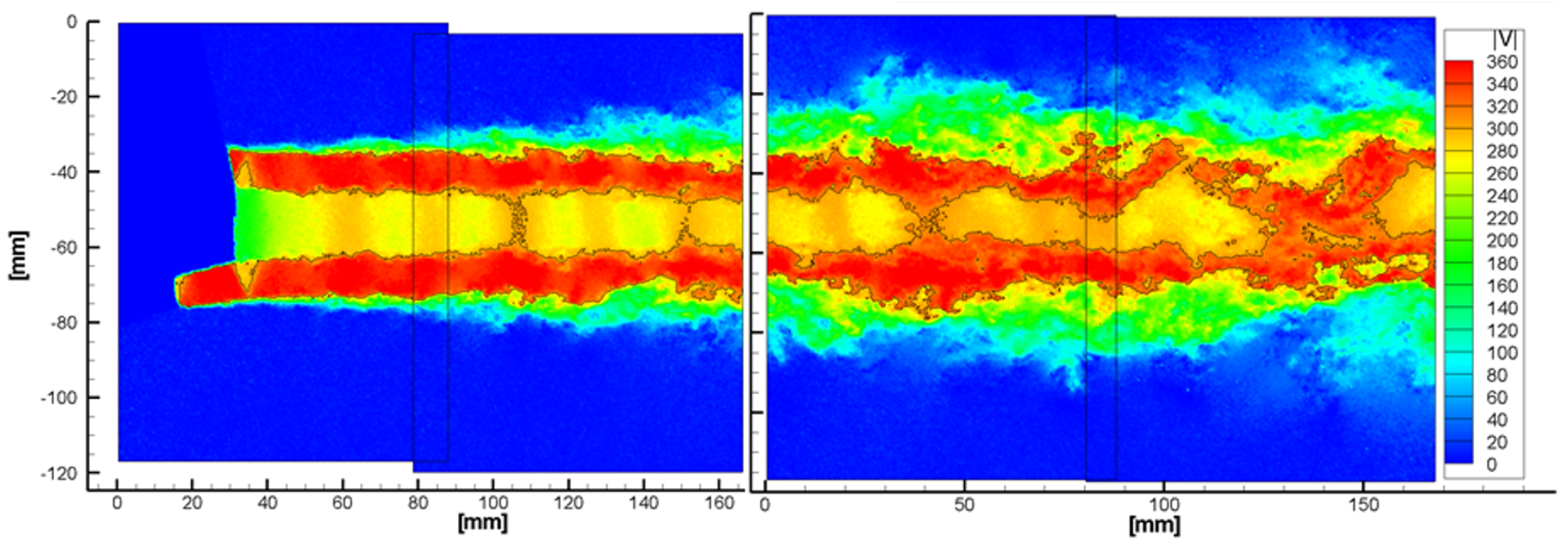
6.3.4. Results: PIV
6.4. Acoustic Measurements
6.4.1. Acquisition System
6.4.2. Data Processing
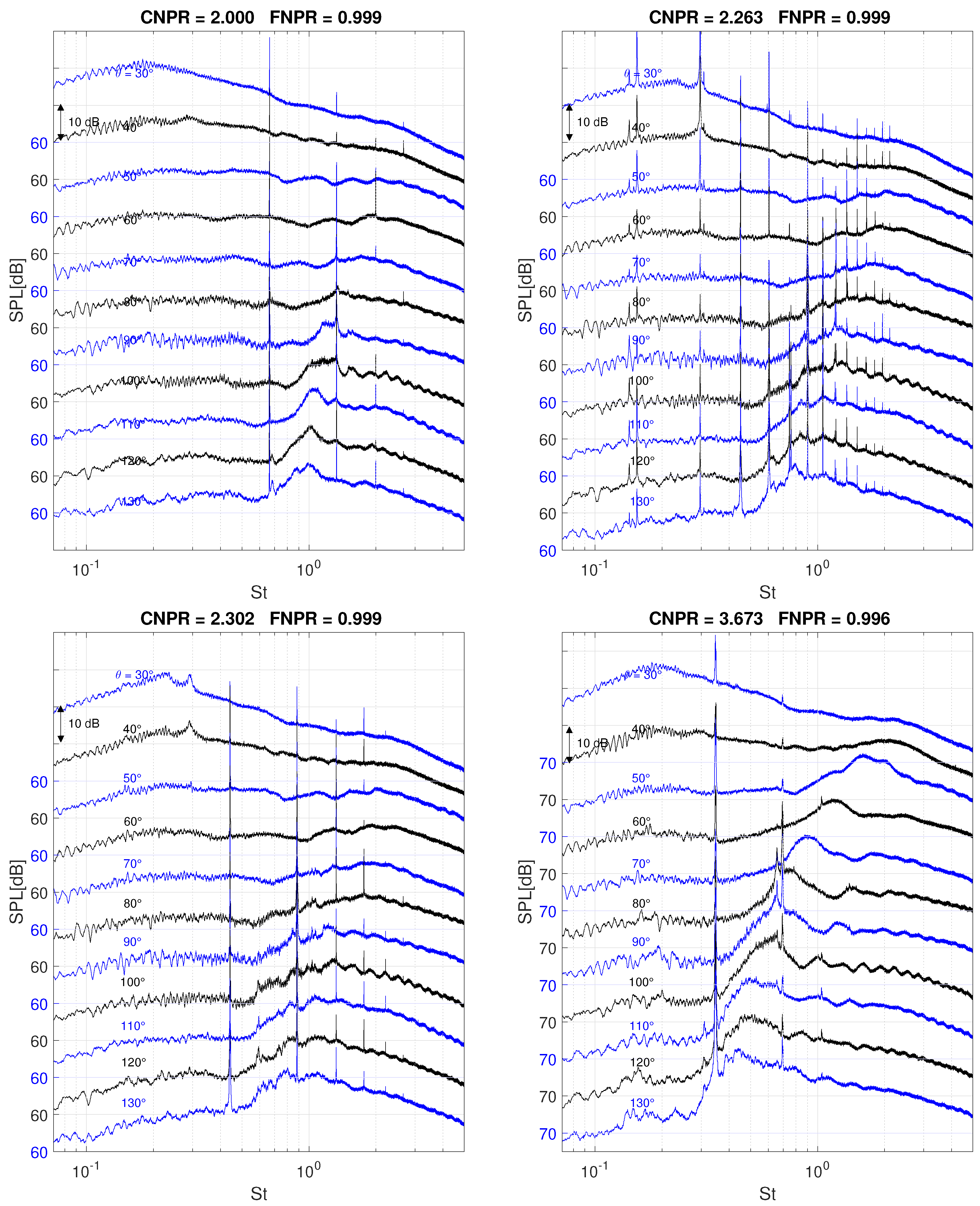
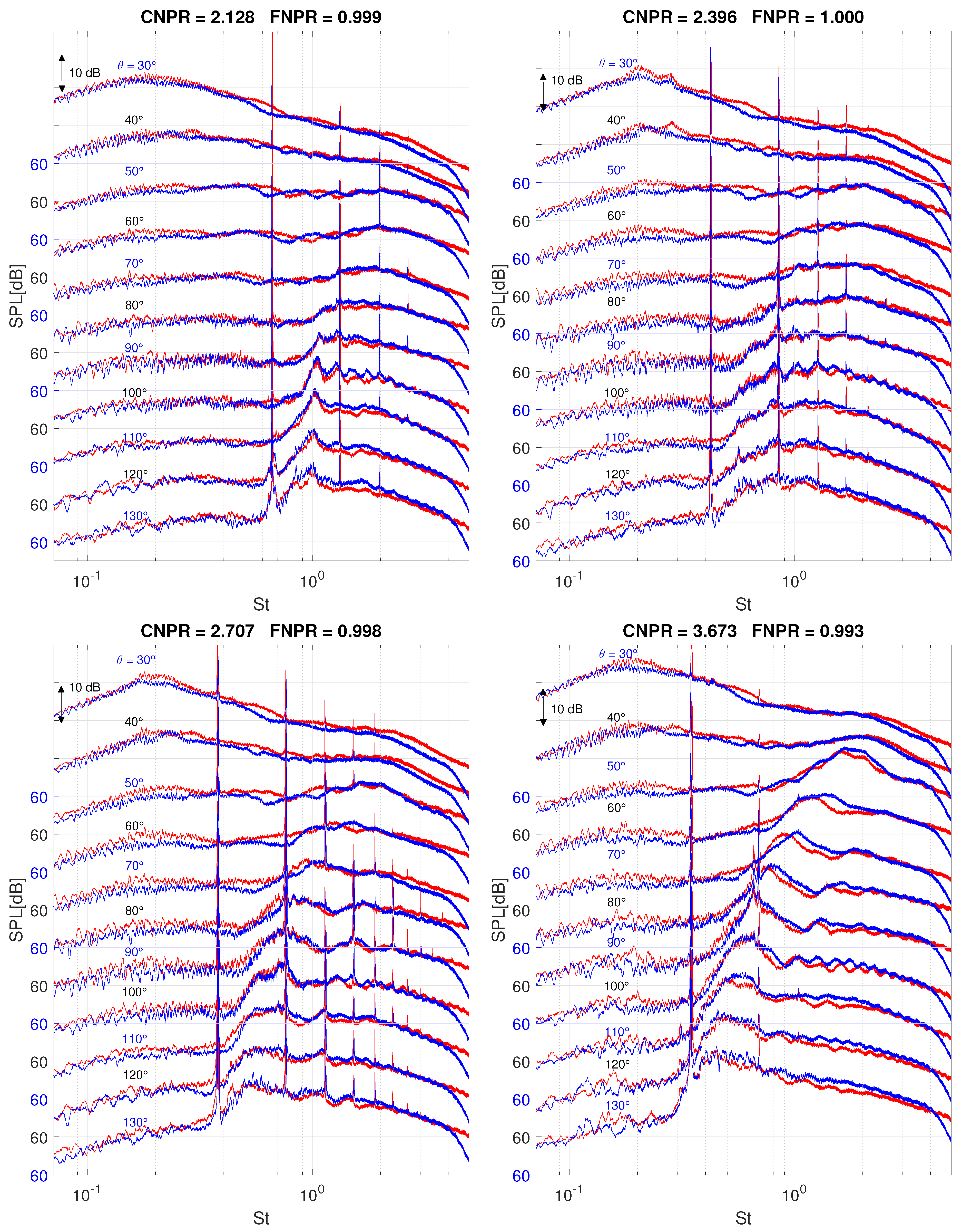
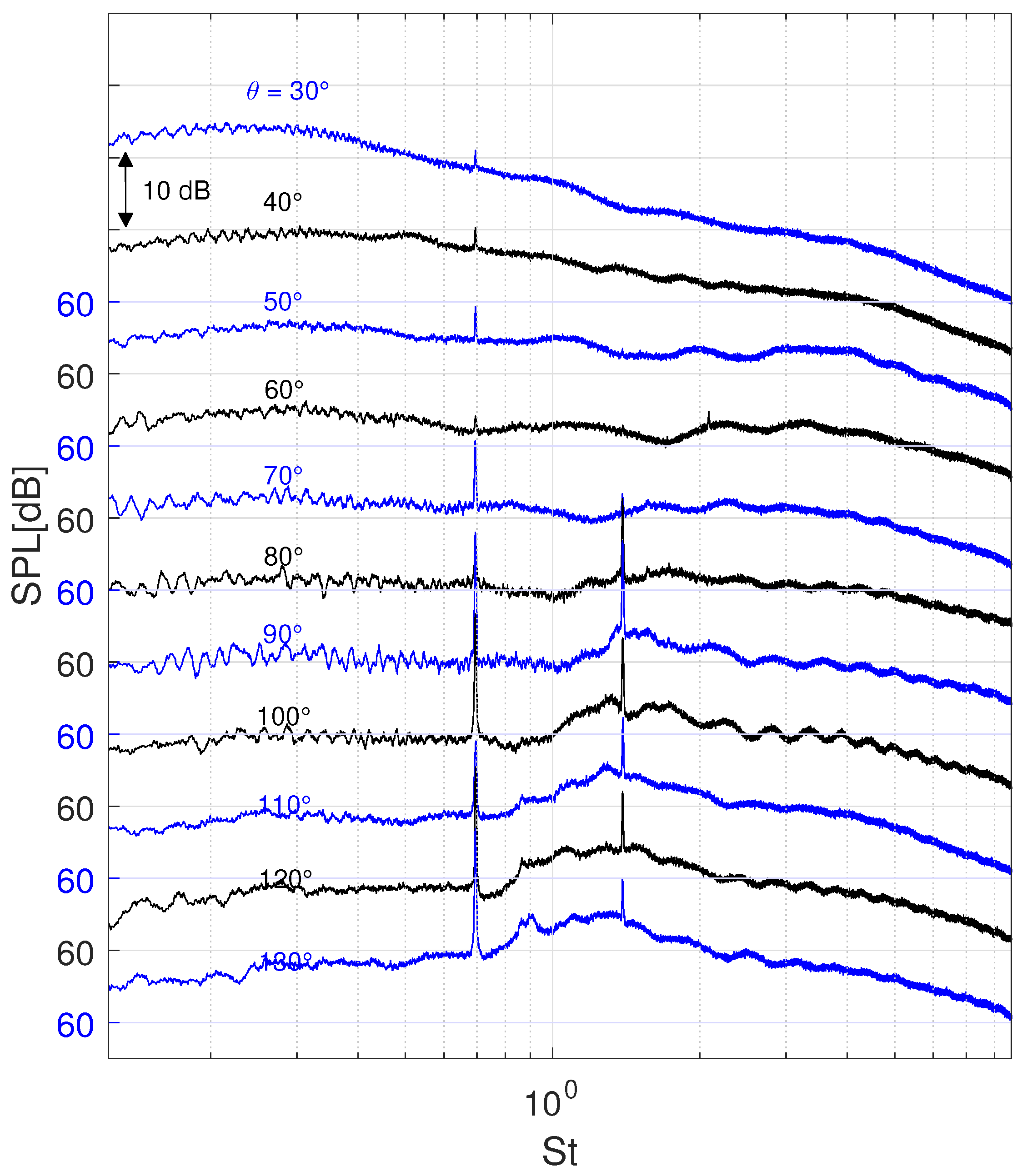
6.4.3. Results: Aeroacoustics
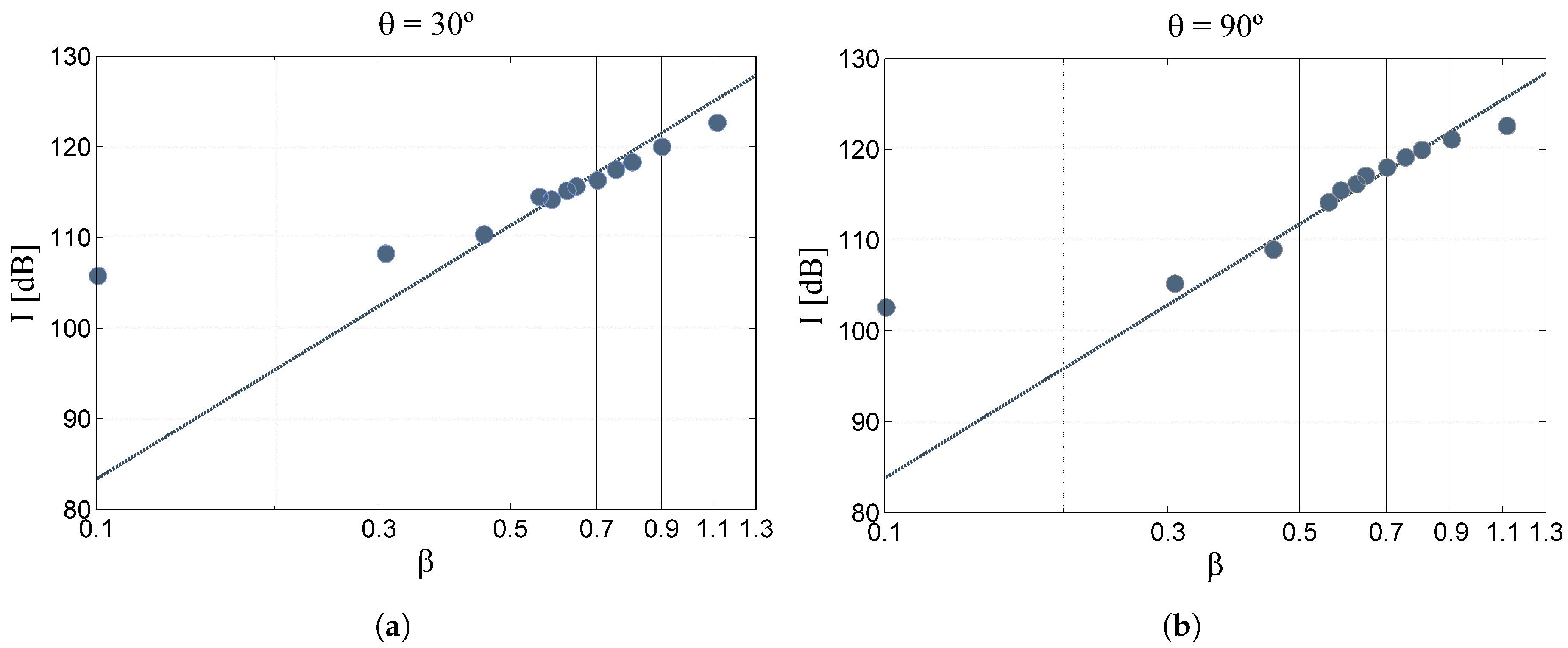
6.5. Comparison of the Sound Intensity Level
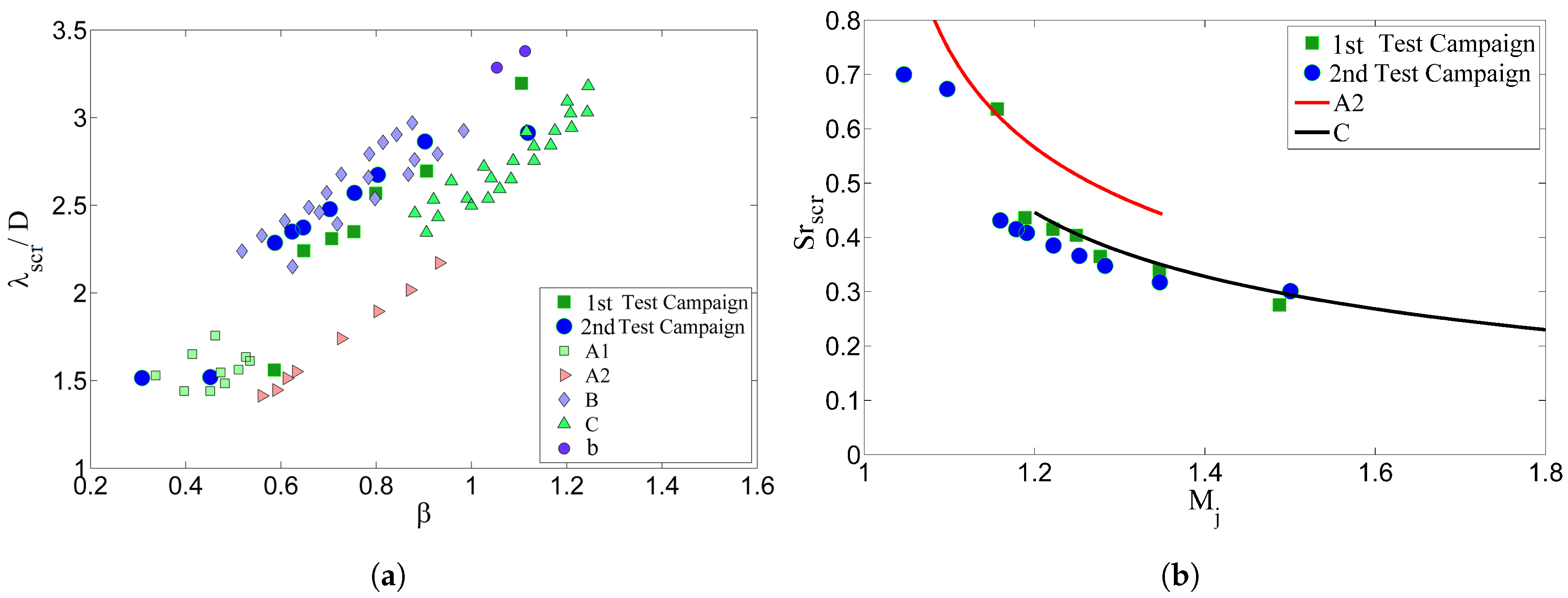
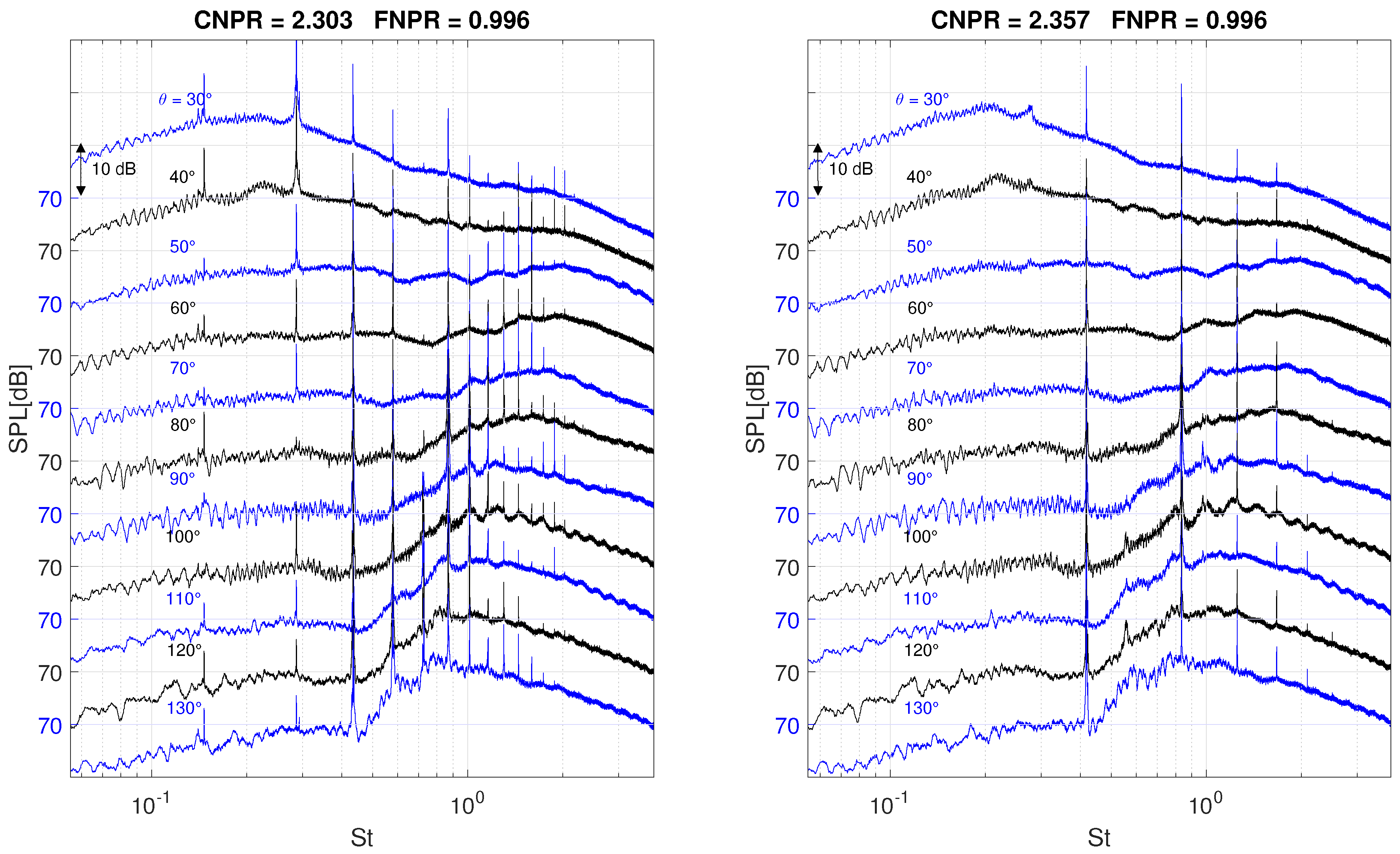
6.6. Nozzles Set Comparison
6.7. Preliminary Dual Stream Jet Results
7. Conclusions and Perspectives
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| A | Area, m |
| c | Speed of sound, m/s |
| D | Diameter, m |
| f | Frequency, Hz |
| Focal number | |
| h | Height, m |
| I | Sound intensity level, W/m |
| l | Length, m |
| L | Length, m |
| m | Mass, kg |
| Mass flow rate, kg/s | |
| M | Mach number |
| N | Number of samples |
| p | Pressure, Pa |
| P | Power, W |
| r | Radius, m |
| Strouhal number, | |
| t | Thickness, m |
| T | Temperature, K |
| Velocity vector, m/s | |
| Acronyms | |
| BBSAN | Broadband Shock-Associated Noise |
| BPR | By-Pass Ratio |
| CAA | Computational AeroAcoustics |
| CAD | Computer Aided Design |
| CFD | Computational Fluid Dynamics |
| CNPR | Core Nozzle Pressure Ratio |
| FAST | Free jet AeroacouSTic laboratory |
| FNPR | Fan Nozzle Pressure Ratio |
| FOV | Field of View |
| LES | Laser Extinction Spectroscopy |
| LES | Large Eddy Simulations |
| RANS | Reynolds Averaged Navier–Stokes |
| PIV | Particle Image Velocimetry |
| SIL | Sound Intensity Level |
| SPL | Sound Pressure Level |
| SWL | Sound Power Level |
| TL | Transmission loss |
| VKI | von Karman Institute |
| Subscripts | |
| 0 | Stagnation condition |
| 1 | Primary nozzle |
| 2 | Secondary nozzle |
| ∞ | Free stream condition |
| a | Ambient condition |
| Canister holding the acoustic absorbent material | |
| d | Design condition |
| Duct section between the nozzleand the silencer | |
| Equivalent | |
| Input | |
| Measured internally | |
| j | Fully expanded |
| Maximum | |
| Output | |
| Reference value | |
| s | Sampling |
| Shock-cell | |
| Screech | |
| Silencer | |
| Stainless steel wool | |
| Symbols | |
| Angle, ° | |
| Off-design factor | |
| Correction to the reactance | |
| Frequency resolution, Hz | |
| Time interval, s | |
| Wavelength, m | |
| Dynamic viscosity, Pa·s | |
| Area porosity | |
| Angle, ° | |
| Flow resistance |
References
- Powell, A. On the Mechanism of Choked Jet Noise. Proc. Phys. Soc. Sect. B 1953, 66, 1039. [Google Scholar]
- Tanna, H. An experimental study of jet noise part II: Shock associated noise. J. Sound Vib. 1977, 50, 429–444. [Google Scholar]
- Harper-Bourne, M.; Fisher, M. The noise from shock waves in supersonic jets. In Proceedings of the AGARD Conference on Noise Mechanisms, Brussels, Belgium, 19–21 September 1973. [Google Scholar]
- Tam, C.; Tanna, H. Shock associated noise of supersonic jets from convergent-divergent nozzles. J. Sound Vib. 1982, 81, 337–358. [Google Scholar]
- Norum, T.D.; Seiner, J.M. Broadband Shock Noise from Supersonic Jets. AIAA J. 1982, 20, 68–73. [Google Scholar]
- Norum, T.D.; Seiner, J.M. Measurements of Mean Static Pressure and Far Field Acoustics of Shock-Containing Supersonic Jets; NASA TM84521d; NASA Langley Research Center: Hampton, VA, USA, 1982.
- Tam, C.; Seiner, J.; Yu, J. Proposed relationship between broadband shock associated noise and screech tones. J. Sound Vib. 1986, 110, 309–321. [Google Scholar]
- Tam, C. Broadband shock-associated noise of moderately imperfectly expanded supersonic jets. J. Sound Vib. 1990, 140, 55–71. [Google Scholar]
- Colonius, T.; Lele, S.K. Computational aeroacoustics: Progress on nonlinear problems of sound generation. Prog. Aerosp. Sci. 2004, 40, 345–416. [Google Scholar]
- Lele, S.K.; Mendez, S.; Ryu, J.; Nichols, J.; Shoeybi, M.; Moin, P. Sources of high-speed jet noise: Analysis of LES data and modeling. Procedia Eng. 2010, 6, 84–93. [Google Scholar]
- Nichols, J.; Ham, F.; Lele, S.; Moin, P. Prediction of supersonic jet noise from complex nozzles. Cent. Turbul. Res. Annu. Res. Briefs 2011, 3–14. [Google Scholar]
- Shur, M.L.; Spalart, P.R.; Strelets, M.K. Noise Prediction for Underexpanded Jets in Static and Flight Conditions. AIAA J. 2011, 49, 2000–2017. [Google Scholar]
- Viswanathan, K. Parametric study of noise from dual-stream nozzles. J. Fluid Mech. 2004, 521, 36–68. [Google Scholar]
- Miller, S.; Morris, P. The Prediction of Broadband Shock-Associated Noise from Dualstream and Rectangular Jets Using RANS CFD. In Aeroacoustics Conferences; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2010. [Google Scholar]
- Guariglia, D. Shock-Cell Noise Investigation on a Subsonic/Supersonic Coaxial Jet. Ph.D. Thesis, Università degli Studi di Roma “La Sapienza”, Roma, Italy, 2017. [Google Scholar]
- COMSOL Multiphysics®5.0 Acoustic Module User Guide; COMSOL Inc.: Stockholm, Sweden, 2014.
- Givoli, D.; Neta, B. High-order non-reflecting boundary scheme for time-dependent waves. J. Comput. Phys. 2003, 186, 24–46. [Google Scholar]
- Tanna, H.; Brown, W.; Tam, C. Shock associated noise of inverted-profile coannular jets, part I: Experiments. J. Sound Vib. 1985, 98, 95–113. [Google Scholar]
- Tam, C.; Tanna, H. Shock associated noise of inverted-profile coannular jets, part II: Condition for minimum noise. J. Sound Vib. 1985, 98, 115–125. [Google Scholar]
- Tam, C.; Tanna, H. Shock associated noise of inverted-profile coannular jets, part III: Shock structure and noise characteristics. J. Sound Vib. 1985, 98, 127–145. [Google Scholar]
- Debiasi, M.; Papamoschou, D. Noise from imperfectly expanded supersonic coaxial jets. AIAA J. 2001, 39, 388–395. [Google Scholar]
- Bhat, T.; Ganz, U.; Guthrie, A. Acoustic and Flow-Field Characteristics of Shock-cell Noise from Dual Flow Nozzles. In Aeroacoustics Conferences; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2005. [Google Scholar]
- Rubio Carpio, A. Experimental Investigation on Shock-Cell Noise; Research Master Report; von Karman Institute for Fluid Dynamics: Rhode-Saint-Genèse, Belgium, 2016. [Google Scholar]
- PIVTEC–GmbH. Aerosol Generator PivPart45-M Series User Manual; PIVTEC–GmbH: Gottingen, Germany, 2013. [Google Scholar]
- Horváth, I. Development and Applications of the Light Extinction Spectroscopy Technique for Characterizing Small Particles. Ph.D. Thesis, Université Libre de Bruxelles, Bruxelles, Belgium, 2015. [Google Scholar]
- Mandon, J.B. Acoustic Dumping by Spray Curtains. Master’s Thesis, von Karman Institute, Rhode-Saint-Genèse, Belgium, 2016. [Google Scholar]
- André, B. Etude Experimentale de L’Effet du vol sur le Bruit de Choc de Jets Supersoniques Sous-Detendus. Ph.D. Thesis, Ecole Centrale de Lyon, Ecully, France, 2012. [Google Scholar]
- Horvath, I. Extreme PIV Applications: Simultaneous and Instantaneous Velocity and Concentration Measurements on Model and Real Scale Car Park Fire Scenarios. Ph.D. Thesis, Université libre de Bruxelles, Bruxelles, Belgium, 2012. [Google Scholar]
- Horvath, I. PIV Image Pre-Processing by Tucsok; Von Karman Institute: Rhode-Saint-Genèse, Belgium, 2011. [Google Scholar]
- Scarano, F.; Riethmuller, L.M. Iterative multigrid approach in PIV image processing with discrete window offset. Exp. Fluids 1999, 26, 513–523. [Google Scholar]
- Horvath, I. PIV Data Processing by Rabon; Von Karman Institute: Rhode-Saint-Genèse, Belgium, 2011. [Google Scholar]
- Stanislas, M.; Okamoto, K.; Kahler, C.J.; Westerweel, J.; Scarano, F. Main results of the third international PIV Challenge. Exp. Fluids 2008, 45, 27–71. [Google Scholar]
- André, B.; Castelain, T.; Bailly, C. Investigation of the mixing layer of underexpanded supersonic jets by particle image velocimetry. Int. J. Heat Fluid Flow 2014, 50, 188–200. [Google Scholar]
- Pérez Arroyo, C.; Daviller, G.; Boussuge, J.F.; Arieau, C. Large Eddy Simulation of Shock-Cell Noise From a Dual Stream Jet. In Proceedings of the 22nd AIAA/CEAS Conference, Lyon, France, 30 May–1 June 2016; American Institute of Aeronautics and Astronautics (AIAA): Reston, VA, USA, 2016. [Google Scholar]
- Pérez Arroyo, C. (CERFACS, Toulouse, France). Private communication, 2016. [Google Scholar]
- Savarese, A. Experimental Study and Modelling of Shock Cell Noise. Ph.D. Thesis, Ecole Nationale Superieure d’Ingegneurs de Poitiers, Poitiers, France, 2014. [Google Scholar]
- Savarese, A.; Jordan, P.; Girard, S.; Collin, E.; Porta, M.; Gervais, Y. Experimental study of shock-cell noise in underexpanded supersonic jets. In Proceedings of the 19th Aeroacoustics Conferences, Berlin, Germany, 27–29 May 2013; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2013. [Google Scholar]
- Harper-Bourne, M.; Fisher, M.J. The Noise from Shock waves in Supersonic Jets. In Proceedings of the No.131 of the AGARD Conference on Noise Mechanisms, Brussels, Belgium, 19–21 September 1973; pp. 11-1–11-14. [Google Scholar]
- Massey, K.C.; Ahuja, K.K. Screech Frequency Prediction in Light of Mode detection and Convection Speed Measurements for Heated Jets. In Proceedings of the 3rd AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 12–14 May 1997; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1997; pp. 315–324. [Google Scholar]
- Tam, C.K.W.; Parrish, S.A.; Viswanathan, K. Harmonics of Jet Screech Tones. AIAA J. 2014, 52, 2471–2479. [Google Scholar]
- Raman, G. Advances in understanding supersonic jet screech: Review and perspective. Prog. Aerosp. Sci. 1998, 34, 45–106. [Google Scholar]

| 0.98 m | 2.39 × 10 m | 35 m | 0.4 | ||||
| 0.76 m | 2.90 × 10 m | 166 kg/m | 0.25 | ||||
| ∼1200 kg | 0.192 m | 1.5 mm | 0 | ||||
| 11 bar | 0.174 m | 4 mm |
| Condition | FNPR | CNPR | ||
|---|---|---|---|---|
| 01 | 2.450 | 1.675 | 1.21 | 0.89 |
| 02 | 2.500 | 1.720 | 1.22 | 0.91 |
| 03 | 2.425 | 1.645 | 1.20 | 0.87 |
| 04 | 2.400 | 1.626 | 1.19 | 0.86 |
| 05 | 2.350 | 1.589 | 1.18 | 0.84 |
| 06 | 2.250 | 1.518 | 1.14 | 0.80 |
| 07 | 2.150 | 1.450 | 1.11 | 0.75 |
| 08 | 2.050 | 1.385 | 1.07 | 0.70 |
| 09 | 2.000 | 1.353 | 1.05 | 0.67 |
| Maximum Element Size in the Shock Region (m) | Total Number of Elements | |
|---|---|---|
| Mesh 1 | 0.002 | 3.4 × |
| Mesh 2 | 0.0005 | 2.7 × |
| Mesh 3 | 0.00025 | 6.0 × |
| CNPR | PIV & Nozzles 1 | Nozzles 1 | Nozzles 2 | (s) | |
|---|---|---|---|---|---|
| 1.8 | 0.96 | x | |||
| 1.9 | 1.0 | x | x | ||
| 2 | 1.05 | x | x | ||
| 2.13 | 1.1 | x | x | ||
| 2.26 | 1.15 | x | |||
| 2.30 | 1.16 | x | x | x | 1.5 |
| 2.36 | 1.18 | x | x | ||
| 2.40 | 1.19 | x | x | x | 1.5 |
| 2.46 | 1.21 | x | |||
| 2.50 | 1.22 | x | x | x | 1.2 |
| 2.60 | 1.25 | x | x | x | 1.2 |
| 2.70 | 1.28 | x | x | x | 1.2 |
| 2.96 | 1.35 | x | x | x | 1.2 |
| 3.67 | 1.50 | x | x | x | 1.0 |
| 4 | 1.56 | x |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guariglia, D.; Rubio Carpio, A.; Schram, C. Design of a Facility for Studying Shock-Cell Noise on Single and Coaxial Jets. Aerospace 2018, 5, 25. https://doi.org/10.3390/aerospace5010025
Guariglia D, Rubio Carpio A, Schram C. Design of a Facility for Studying Shock-Cell Noise on Single and Coaxial Jets. Aerospace. 2018; 5(1):25. https://doi.org/10.3390/aerospace5010025
Chicago/Turabian StyleGuariglia, Daniel, Alejandro Rubio Carpio, and Christophe Schram. 2018. "Design of a Facility for Studying Shock-Cell Noise on Single and Coaxial Jets" Aerospace 5, no. 1: 25. https://doi.org/10.3390/aerospace5010025
APA StyleGuariglia, D., Rubio Carpio, A., & Schram, C. (2018). Design of a Facility for Studying Shock-Cell Noise on Single and Coaxial Jets. Aerospace, 5(1), 25. https://doi.org/10.3390/aerospace5010025





