A Parameter Self-Tuning Rule Based on Spatial–Temporal Scale for Active Disturbance Rejection Control and Its Application in Flight Test Chamber Systems
Abstract
:1. Introduction
2. Preliminaries
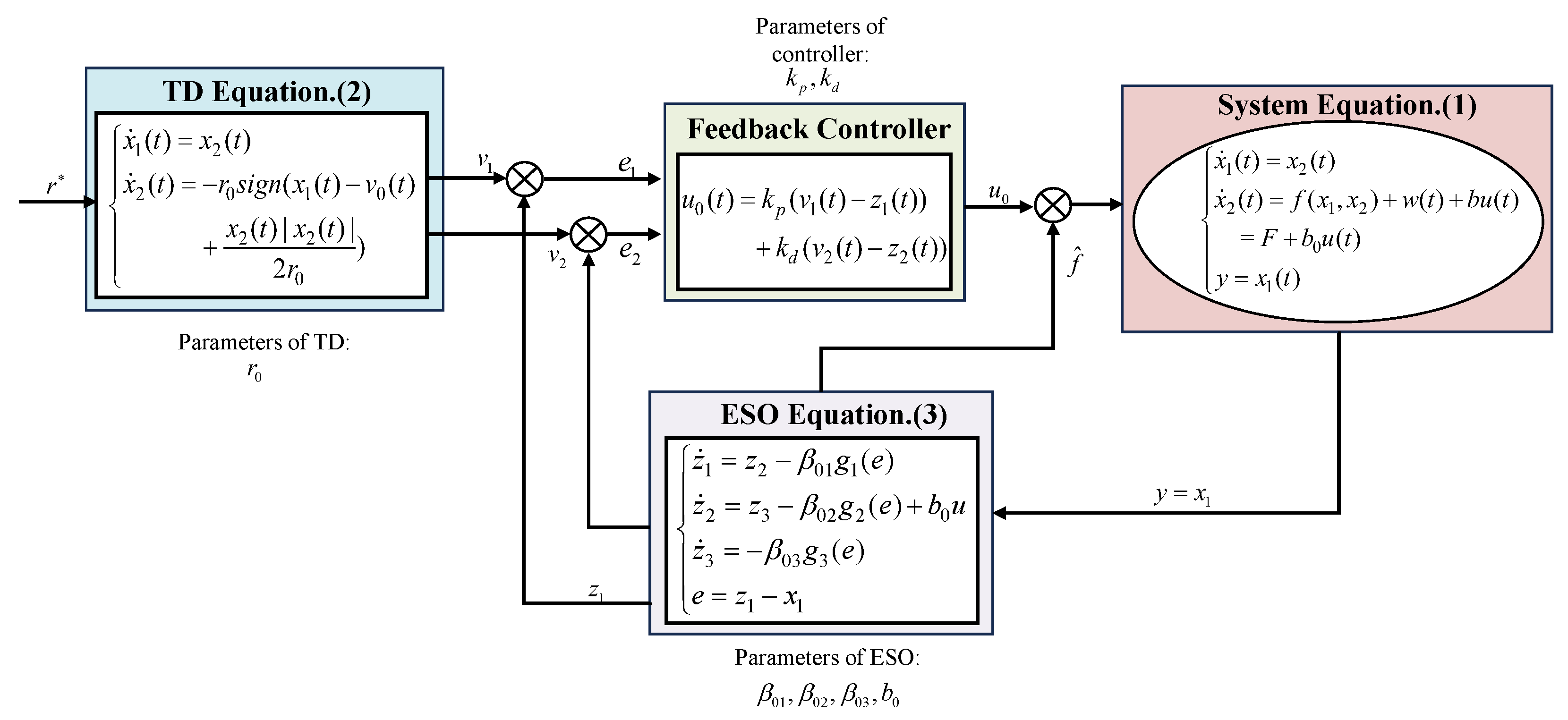
2.1. Brief Introduction of ADRC
2.2. System Spatial–Temporal Scale
3. Main Results: The Proposed ADRC Parameter Tuning Rule
3.1. Spatial–Temporal Scale Transformations of TD
3.2. Spatial–Temporal Scale Transformations of ESO and Feedback Controller
3.3. ADRC Parameter Tuning Rule
4. Simulation Results
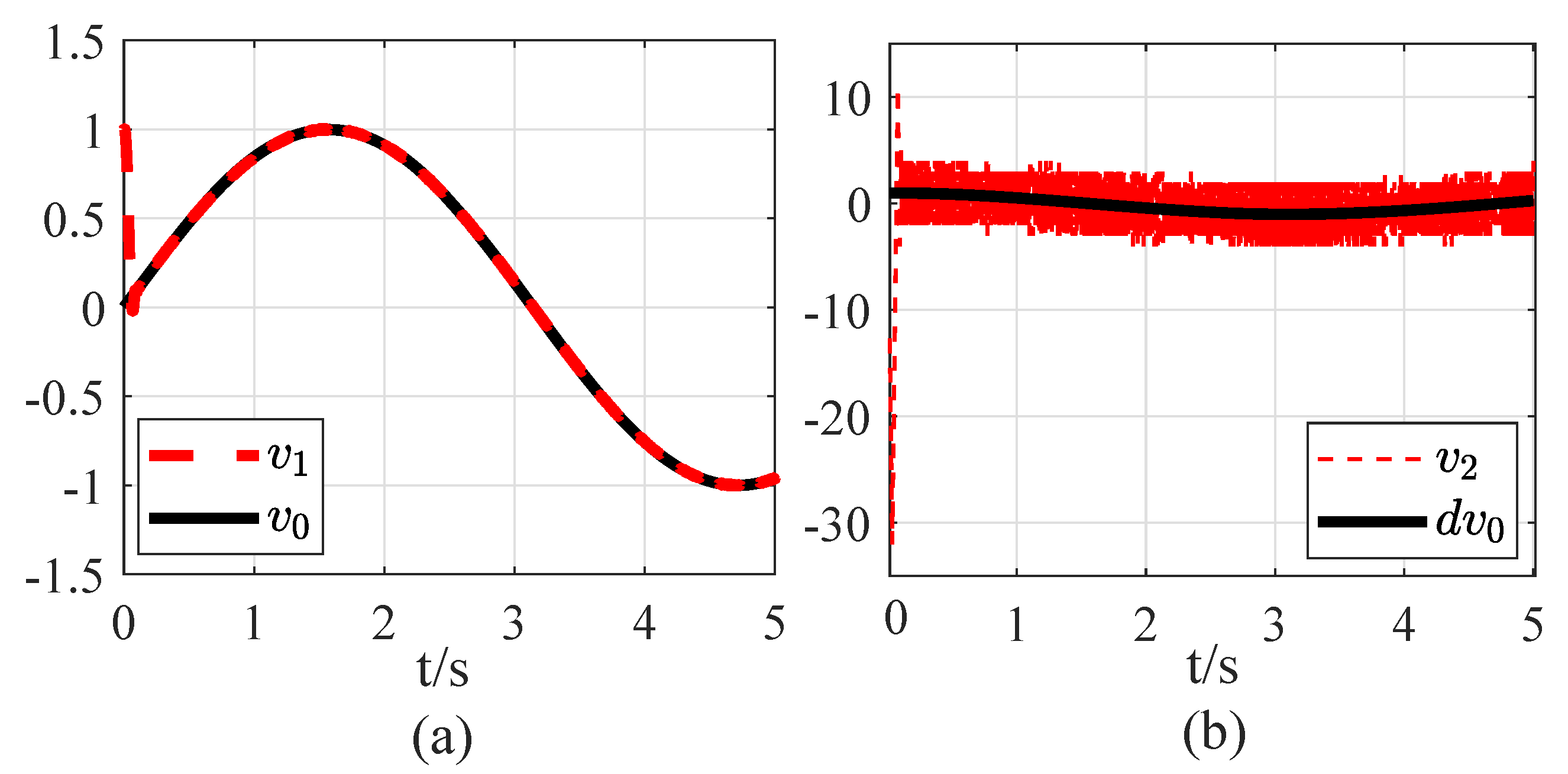
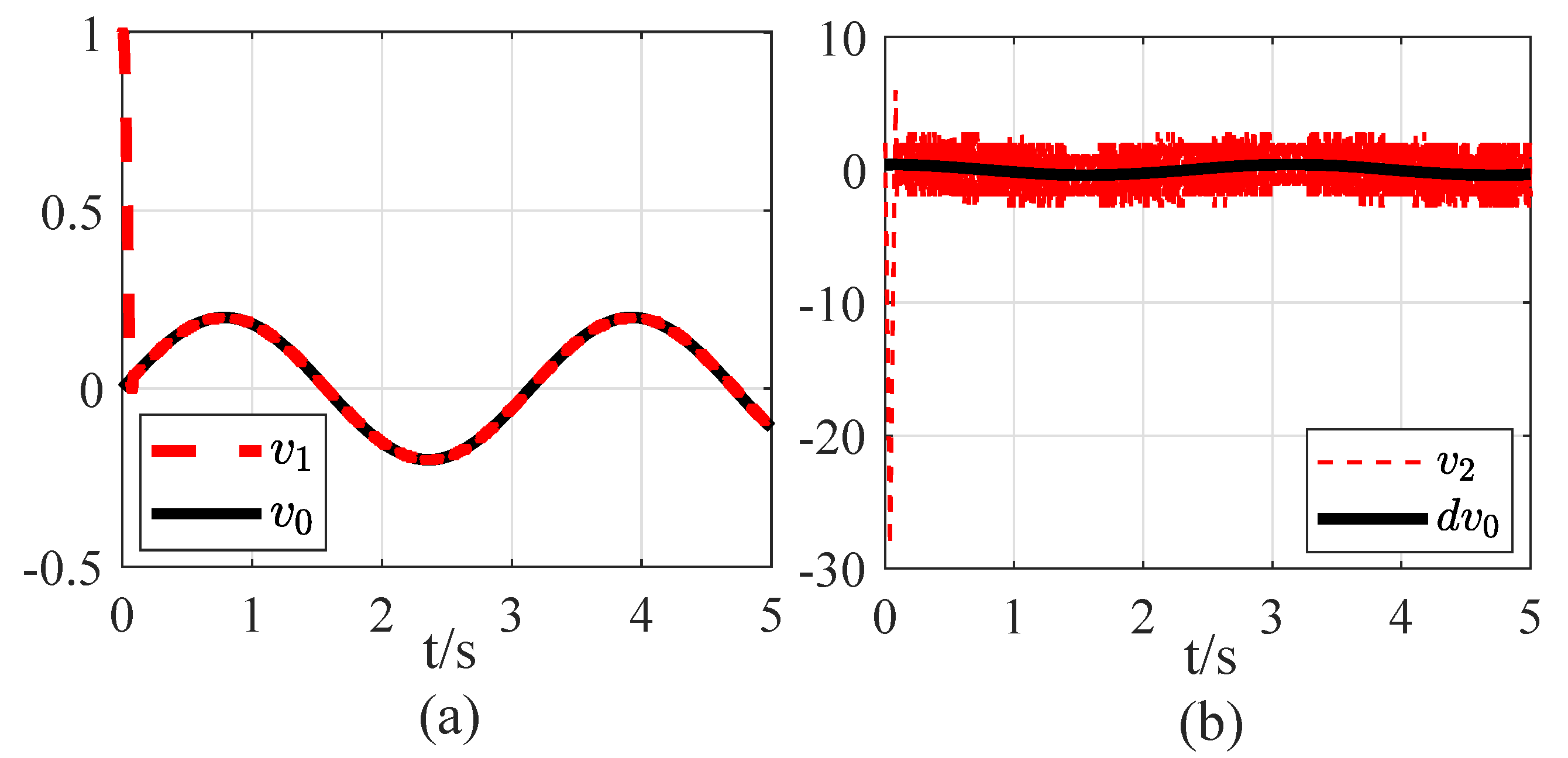
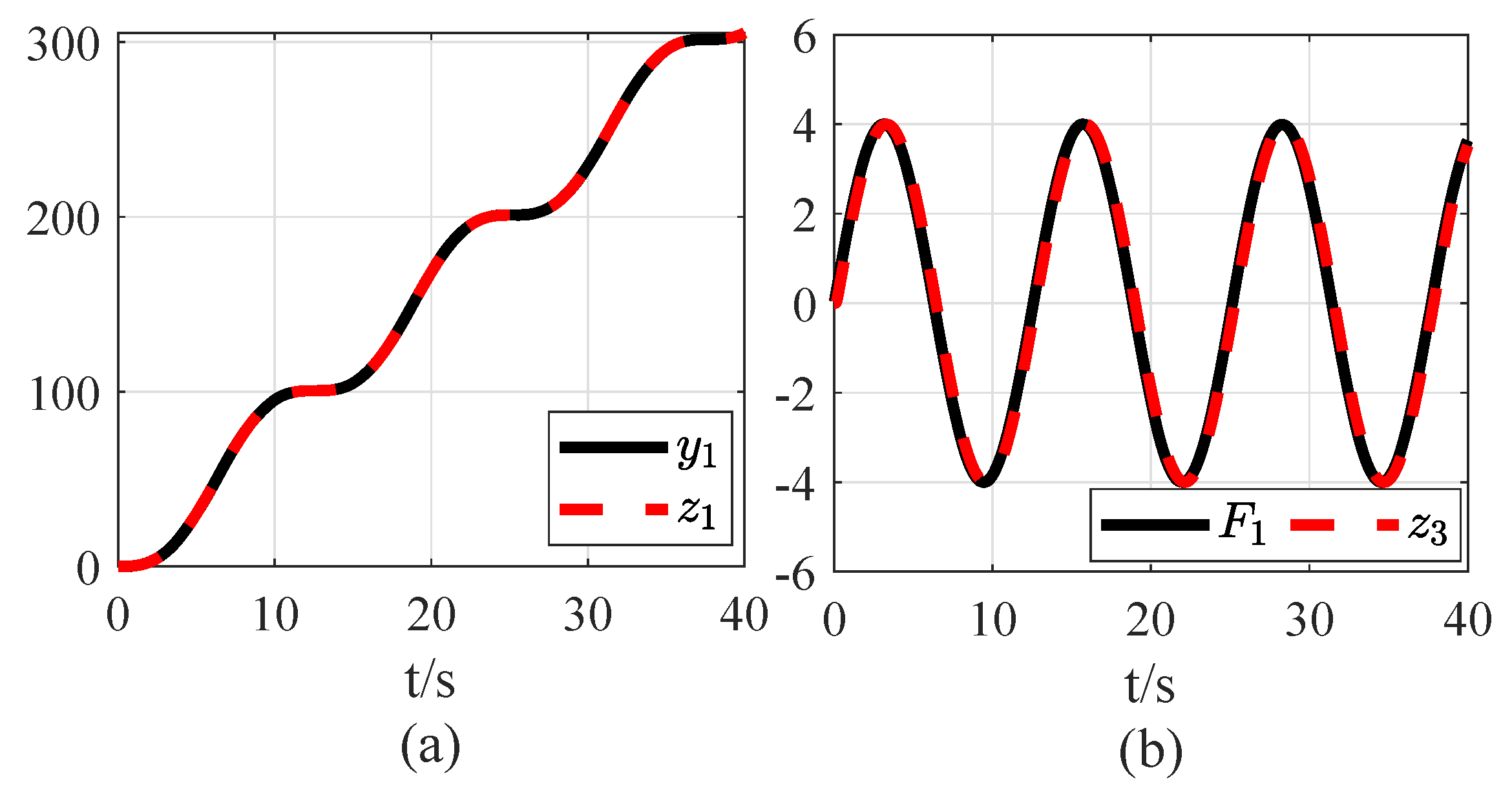
4.1. Signal Tracking and Derivative Acquisition of TD
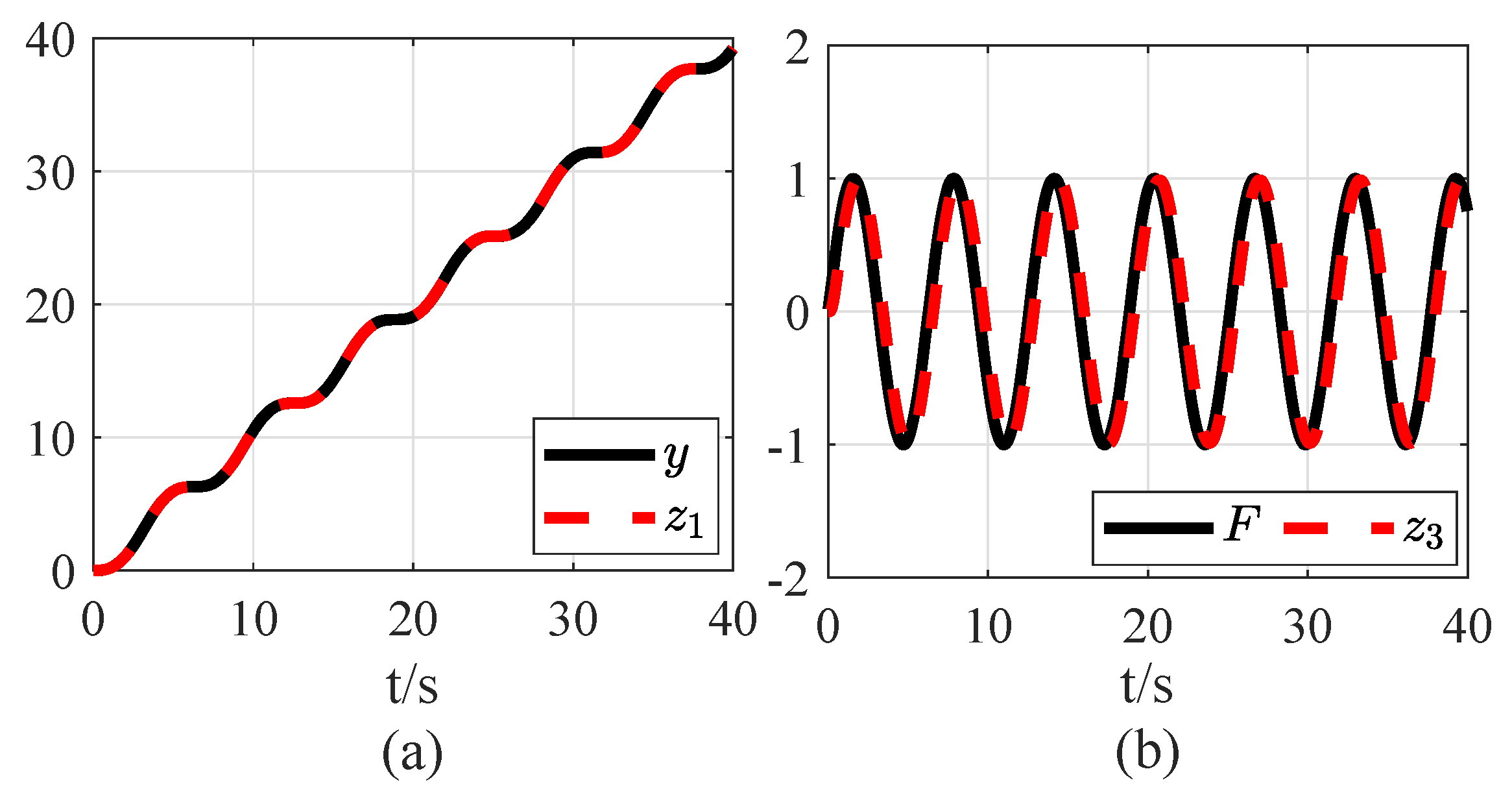
4.2. System States and Disturbance Estimation of ESO
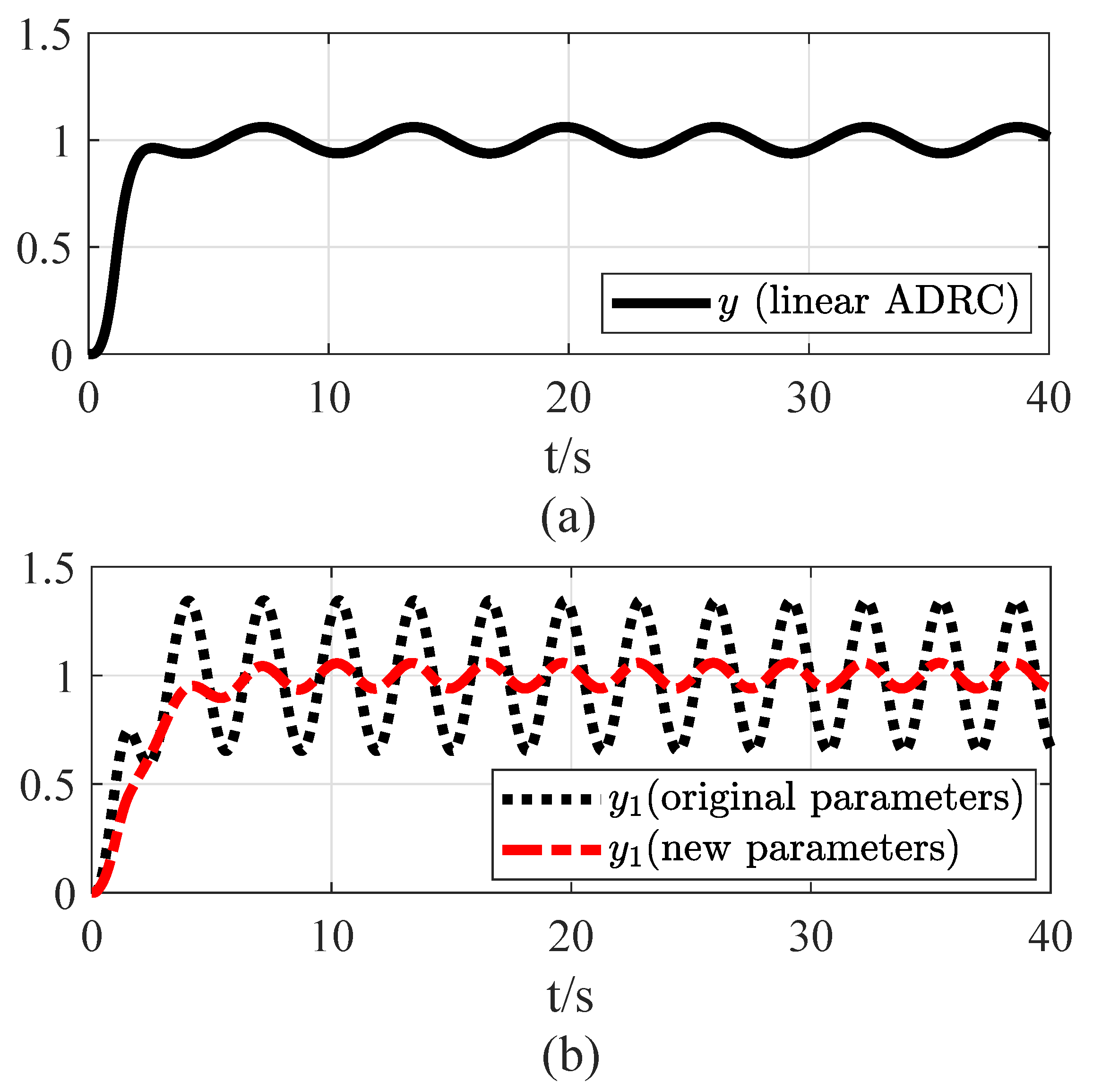
4.3. Control Performance of ADRC
5. Application
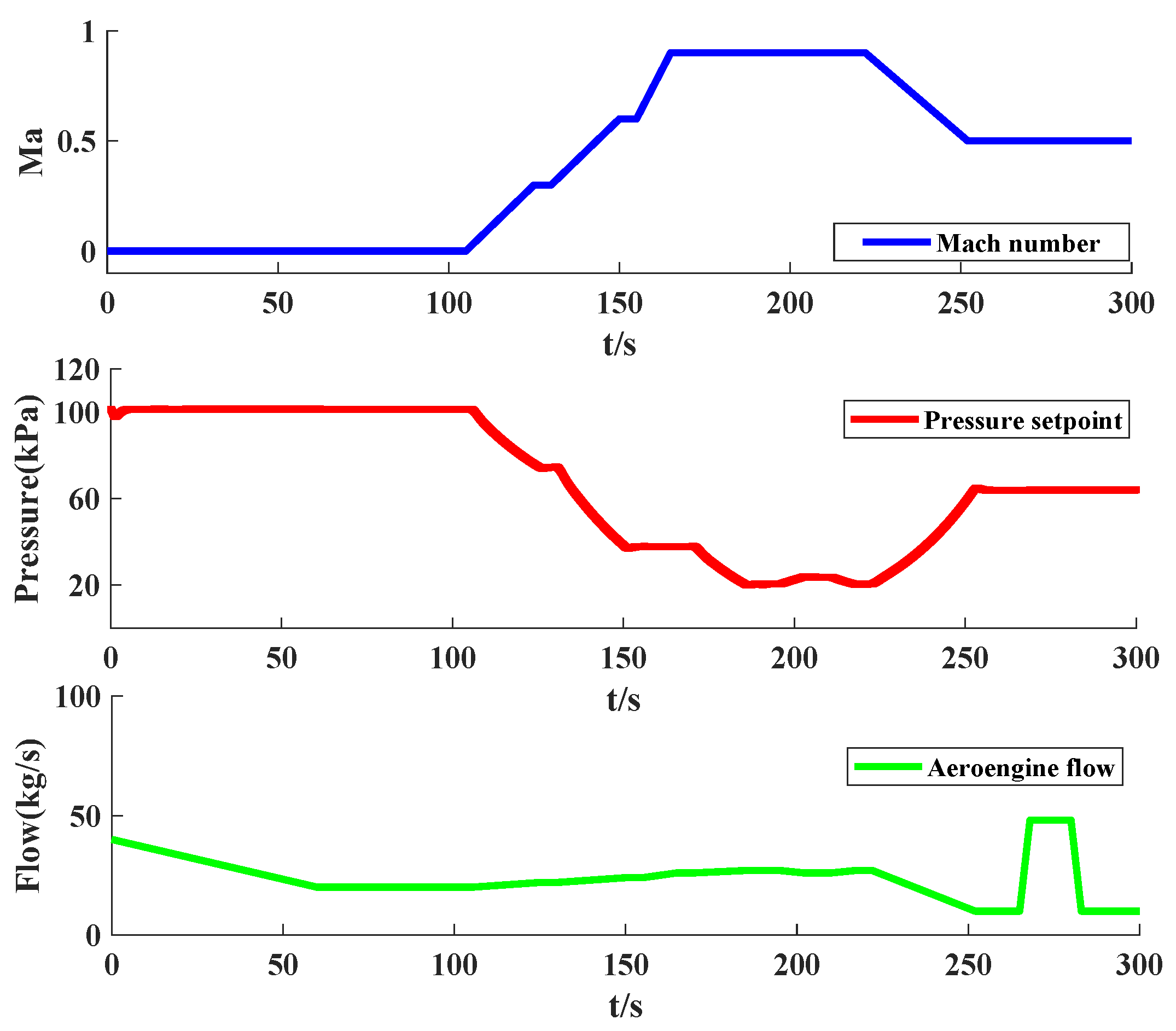
5.1. Introduction of the Flight Test Chamber System
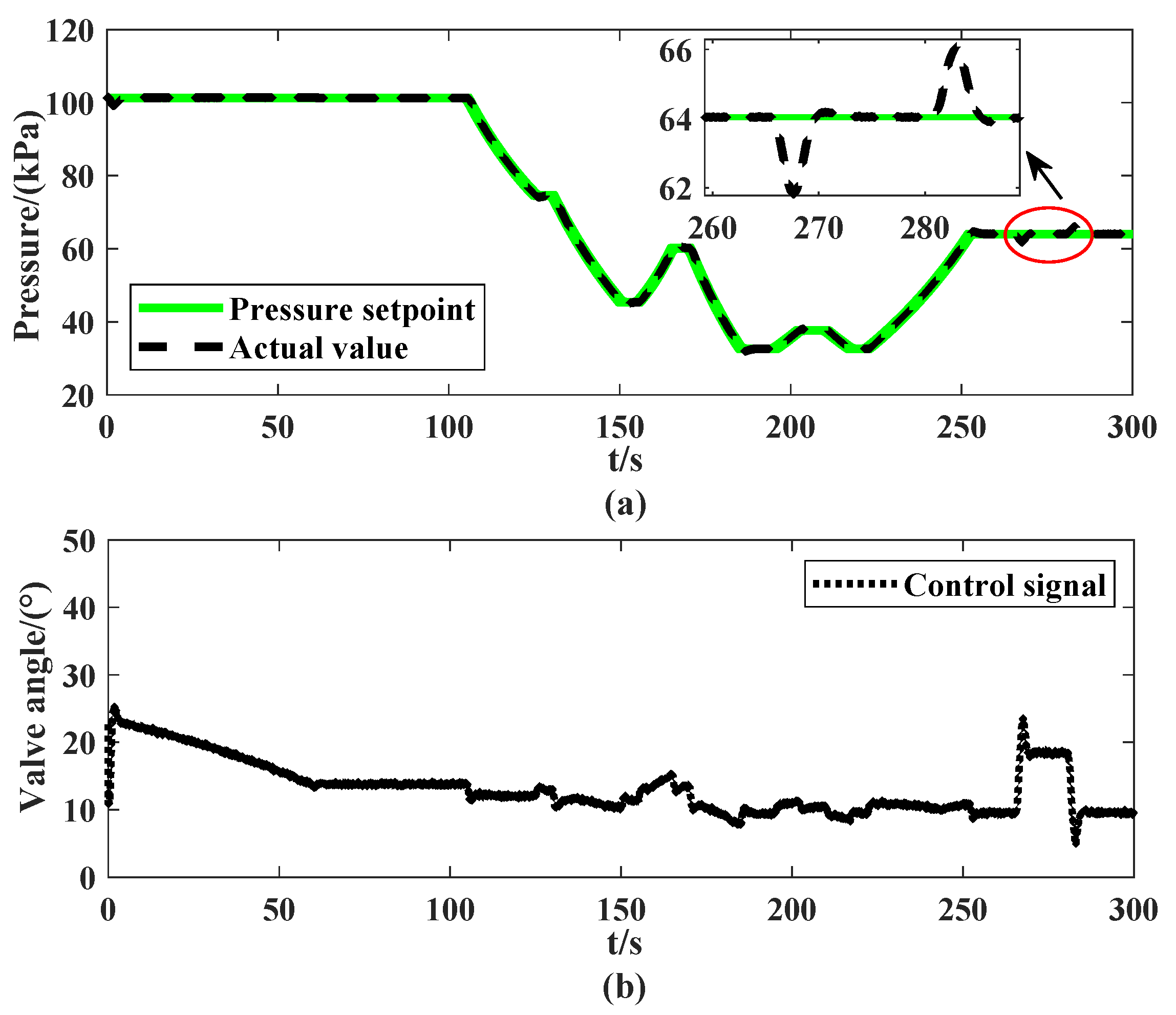
5.2. Test Results and Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Afkhami, S.; Fouladi, N.; Fard, M.P. Experimental and numerical investigation of transient starting of pre-evacuated exhaust diffuser in high altitude ground test. Aerosp. Sci. Technol. 2023, 133, 108111. [Google Scholar] [CrossRef]
- Zhu, M.; Wang, X.; Zhang, S.; Dan, Z.; Pei, X.; Miao, K.; Jiang, Z. PI gain scheduling control for flight environment simulation system of altitude ground test facilities based on LMI pole assignment. J. Propuls. Technol. 2019, 40, 2587–2597. [Google Scholar]
- Chen, W.; Yang, J.; Guo, L.; Li, S. Disturbance-observer-based control and related methods—An overview. IEEE Trans. Ind. Electron. 2015, 63, 1083–1095. [Google Scholar] [CrossRef]
- McKercher, R.G.; Khouli, F.; Wall, A.S.; Larose, G.L. Modelling and control of an urban air mobility vehicle subject to empirically-developed urban airflow disturbances. Aerospace 2024, 11, 220. [Google Scholar] [CrossRef]
- Li, S.; Yang, J.; Iwasaki, M.; Chen, W.H. Hierarchical disturbance/uncertainty estimation and attenuation for integrated modeling and motion control: Overview and perspectives. IEEE/ASME Trans. Mechatron. 2025, 25, 1–15. [Google Scholar] [CrossRef]
- Han, J. From PID to active disturbance rejection control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Gao, Z. Scaling and bandwidth-parameterization based controller tuning. In Proceedings of the ACC, Denver, CO, USA, 4–6 June 2003; pp. 4989–4996. [Google Scholar]
- Li, J.; Zhang, L.; Li, S.; Mao, Q.; Mao, Y. Active disturbance rejection control for piezoelectric smart structures: A review. Machines 2023, 11, 174. [Google Scholar] [CrossRef]
- Chen, S.; Chen, Z.; Zhao, Z.L. Parameter selection and performance analysis of linear disturbance observer based control for a class of nonlinear uncertain systems. IEEE Trans. Ind. Electron. 2021, 70, 11587–11597. [Google Scholar] [CrossRef]
- Guo, B.; Bacha, S.; Alamir, M.; Hably, A.; Boudinet, C. Generalized integrator-extended state observer with applications to grid-connected converters in the presence of disturbances. IEEE Trans. Control Syst. Technol. 2020, 29, 744–755. [Google Scholar] [CrossRef]
- Su, Z.g.; Sun, L.; Xue, W.; Lee, K.Y. A review on active disturbance rejection control of power generation systems: Fundamentals, tunings and practices. Control Eng. Pract. 2023, 141, 105716. [Google Scholar] [CrossRef]
- Li, S.; Lu, H.; Li, J.; Zheng, T.; He, Y. Fractional-order sliding mode controller based on ESO for a buck converter with mismatched disturbances: Design and experiments. IEEE Trans. Ind. Electron. 2025; in press. [Google Scholar] [CrossRef]
- Chen, S.; Xue, W.; Huang, Y.; Liu, P. On comparison between smith predictor and predictor observer based adrcs for nonlinear uncertain systems with output delay. In Proceedings of the ACC, Seattle, WA, USA, 24–26 May 2017; pp. 5083–5088. [Google Scholar]
- Hou, Q.; Zuo, Y.; Sun, J.; Lee, C.H.; Wang, Y.; Ding, S. Modified nonlinear active disturbance rejection control for PMSM speed regulation with frequency domain analysis. IEEE Trans. Power Electron. 2023, 38, 8126–8134. [Google Scholar] [CrossRef]
- Li, C.; Zhai, C.; Zhang, H.; Zheng, S. PSO-SMADRC for altitude test facility intake pressure environmental simulation system. Electron. Lett. 2024, 60, e13105. [Google Scholar]
- Wang, C.; Du, X.; Sun, X. Performance-seeking control of aero-propulsion system based on intelligent optimization and active disturbance rejection fusion controller. Aerospace 2023, 10, 151. [Google Scholar] [CrossRef]
- Xu, Z.; Zhang, H.; Zhai, C.; Qian, Q.; Wu, L. Fixed time active disturbance rejection compound decoupling control method for intake environment simulation system of altitude test facility. J. Propuls. Technol. 2024, 46, 239–252. [Google Scholar] [CrossRef]
- Li, C.; Zhang, H.; Gaoxi, X.; Zhai, C.; Dan, Z.; Wang, X. An efficient tracking differentiator based active disturbance rejection control for flight environment simulation system. Aerosp. Sci. Technol. 2024, 155, 109578. [Google Scholar] [CrossRef]
- Ran, M.; Li, J.; Xie, L. A new extended state observer for uncertain nonlinear systems. Automatica. 2021, 131, 109772. [Google Scholar] [CrossRef]
- Du, Y.; She, J.; Cao, W. Improving performance of disturbance rejection for nonlinear systems using improved equivalent-input-disturbance approach. IEEE Trans. Ind. Electron. 2023, 20, 941–952. [Google Scholar] [CrossRef]
- Zaragoza Prous, G.; Grustan-Gutierrez, E.; Felicetti, L. A decreasing horizon model predictive control for landing reusable launch vehicles. Aerospace 2025, 12, 111. [Google Scholar] [CrossRef]
- Chang, S.; Cao, J.; Pang, J.; Zhou, F.; Chen, W. Compensation control strategy for photoelectric stabilized platform based on disturbance observation. Aerosp. Sci. Technol. 2024, 145, 108909. [Google Scholar] [CrossRef]
- Li, J.; Xia, Y.; Qi, X.; Gao, Z. On the necessity, scheme, and basis of the linear–nonlinear switching in active disturbance rejection control. IEEE Trans. Ind. Electron. 2016, 64, 1425–1435. [Google Scholar] [CrossRef]
- Sun, L.; Xue, W.; Li, D.; Zhu, H.; Su, Z.g. Quantitative tuning of active disturbance rejection controller for FOPTD model with application to power plant control. IEEE Trans. Ind. Electron. 2021, 69, 805–815. [Google Scholar] [CrossRef]
- Yuan, D.; Ma, X.; Zeng, Q.; Qiu, X. Research on frequency-band characteristics and parameters configuration of linear active disturbance rejection control for second-order systems. Control Theory Appl. 2013, 30, 1630–1640. [Google Scholar]
- Liang, Q.; Wang, C.; Pan, J.; Wei, Y.; Yong, W. Parameter identification of b0 and parameter tuning law in linear active disturbance rejection control. Control Decis. 2015, 30, 1691–1695. [Google Scholar]
- Chen, Z.; Hao, Y.; Su, Z.; Sun, L. Data-driven iterative tuning based active disturbance rejection control for FOPTD model. ISA Trans. 2022, 128, 593–605. [Google Scholar] [CrossRef]
- Li, S.; Zhang, S.; Liu, Y.; Zhou, S. Parameter-tuning in active disturbance rejection controller using time scale. Control Theory Appl. 2012, 29, 125–129. [Google Scholar]
- Zhang, H.; Xie, Y.; Wang, J.; Dan, Z.; Guo, W. Parameter tuning algorithm for levant’s differentiator and its application in flight environment simulation control system. Control Theory Appl. 2023, 40, 1831–1838. [Google Scholar]
- Wang, J.; Zhang, H.; Dan, Z.; Zhang, S.; Xiao, G.; Zhai, C. A practical parameter tuning algorithm for super-twisting algorithm-based differentiator and its application in altitude ground test facility. Trans. Inst. Meas. Control 2024, 47, 610–620. [Google Scholar] [CrossRef]
- Ran, M.; Wang, Q.; Dong, C. Active disturbance rejection control for uncertain nonaffine-in-control nonlinear systems. IEEE Trans. Autom. Control 2016, 62, 5830–5836. [Google Scholar] [CrossRef]
- Liu, J.; Wang, X.; Liu, X.; Zhu, M.; Pei, X.; Dan, Z.; Zhang, S. μ-Synthesis-based robust L1 adaptive control for aeropropulsion system test facility. Aerosp. Sci. Technol. 2023, 140, 108457. [Google Scholar] [CrossRef]








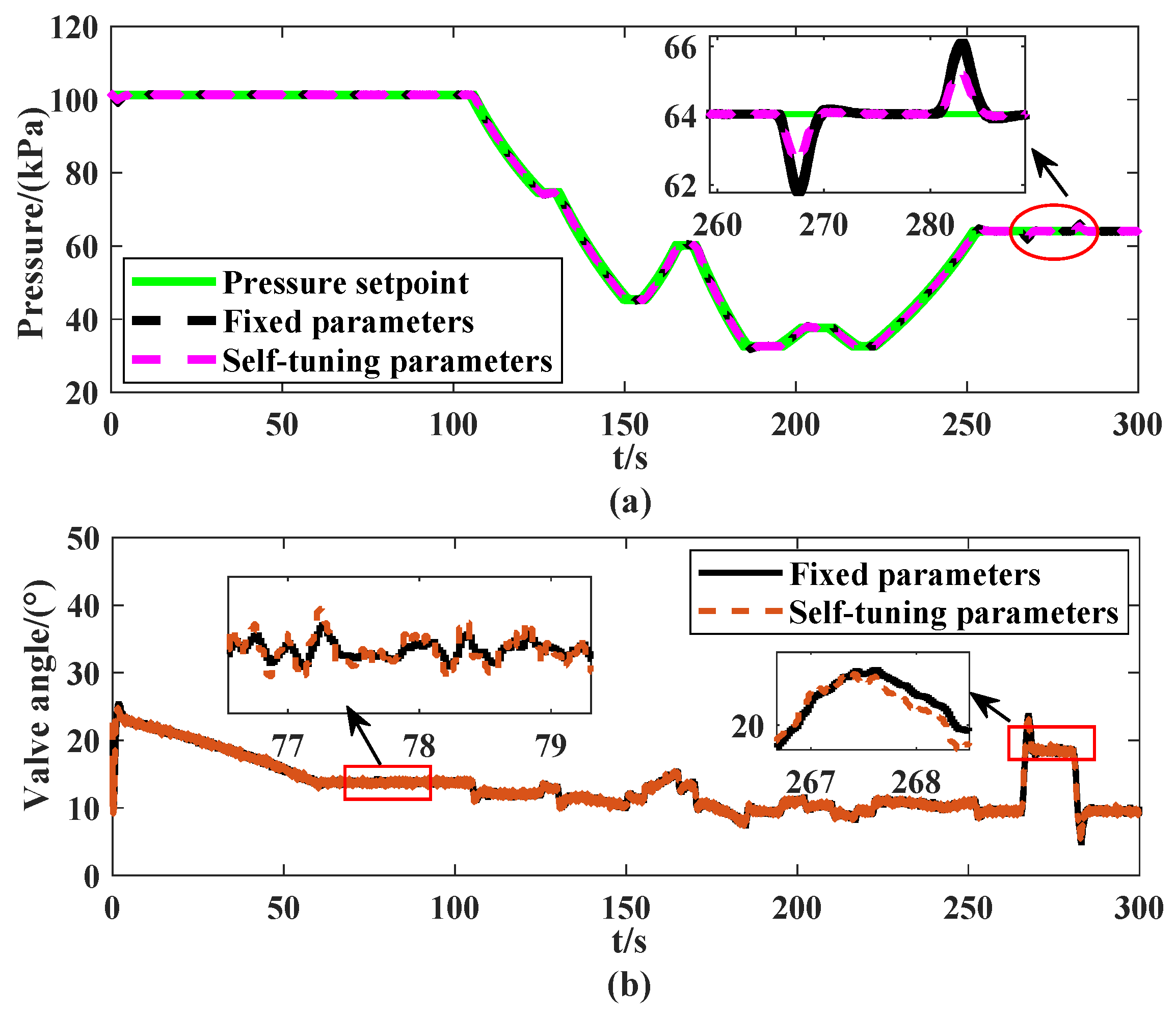
| Performance | Approach | 250∼300 s | Percentage Improvement |
|---|---|---|---|
| Maximum pressure error (kPa) | Fixed parameters | 2.1 | − |
| Self-tuning parameters | 0.9 | ||
| Maximum convergence time (s) | Fixed parameters | 8.0 | − |
| Self-tuning parameters | 4.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Z.; Zhang, H.; Xie, Y.; Zhai, C.; Wang, X.; Huang, F. A Parameter Self-Tuning Rule Based on Spatial–Temporal Scale for Active Disturbance Rejection Control and Its Application in Flight Test Chamber Systems. Aerospace 2025, 12, 465. https://doi.org/10.3390/aerospace12060465
Xu Z, Zhang H, Xie Y, Zhai C, Wang X, Huang F. A Parameter Self-Tuning Rule Based on Spatial–Temporal Scale for Active Disturbance Rejection Control and Its Application in Flight Test Chamber Systems. Aerospace. 2025; 12(6):465. https://doi.org/10.3390/aerospace12060465
Chicago/Turabian StyleXu, Zhuang, Hehong Zhang, Yunde Xie, Chao Zhai, Xin Wang, and Feng Huang. 2025. "A Parameter Self-Tuning Rule Based on Spatial–Temporal Scale for Active Disturbance Rejection Control and Its Application in Flight Test Chamber Systems" Aerospace 12, no. 6: 465. https://doi.org/10.3390/aerospace12060465
APA StyleXu, Z., Zhang, H., Xie, Y., Zhai, C., Wang, X., & Huang, F. (2025). A Parameter Self-Tuning Rule Based on Spatial–Temporal Scale for Active Disturbance Rejection Control and Its Application in Flight Test Chamber Systems. Aerospace, 12(6), 465. https://doi.org/10.3390/aerospace12060465






