Flexible Surface Reflector Antenna for Small Satellites
Abstract
1. Introduction
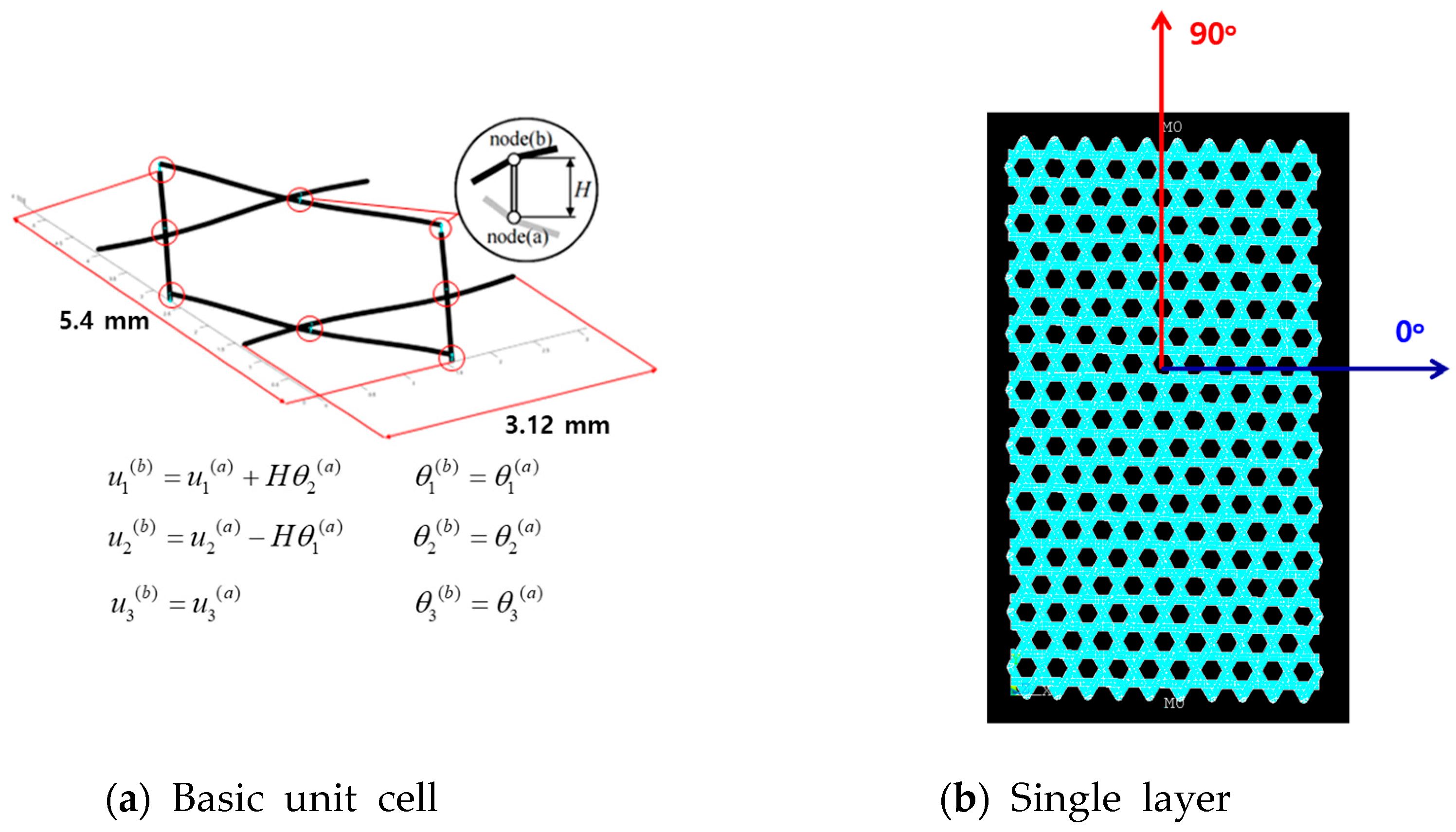
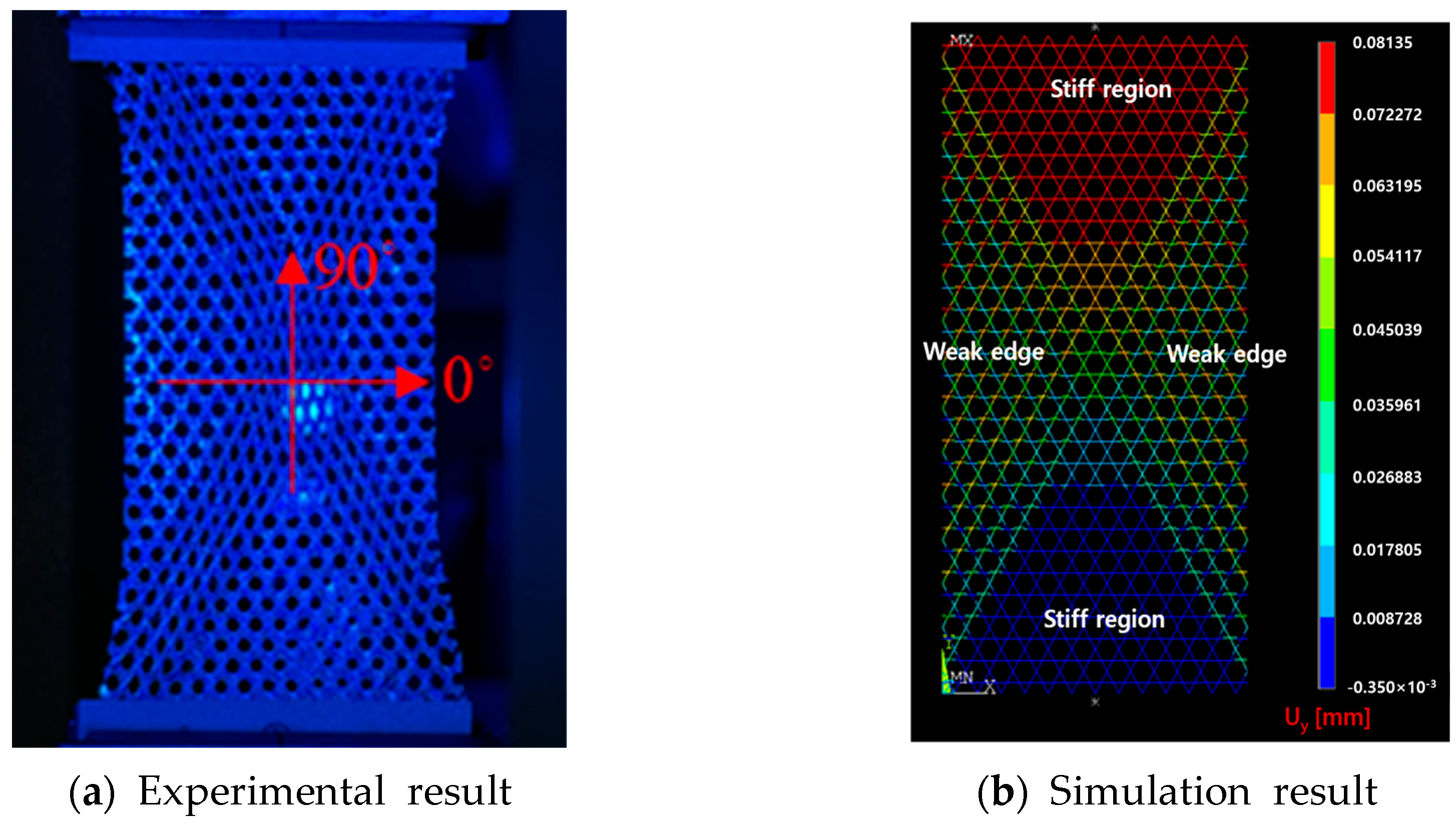
2. Mechanical and Electrical Properties of TWFS Composites
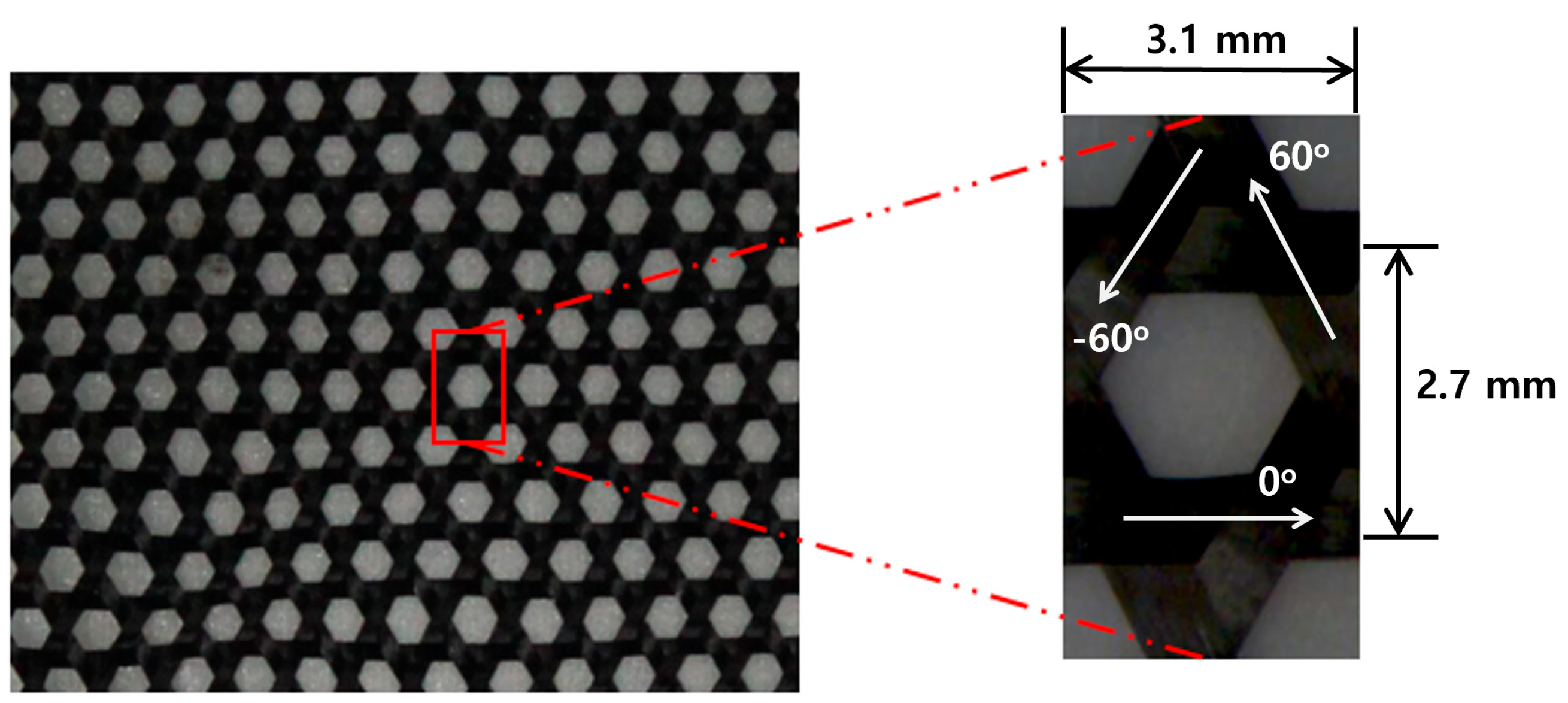
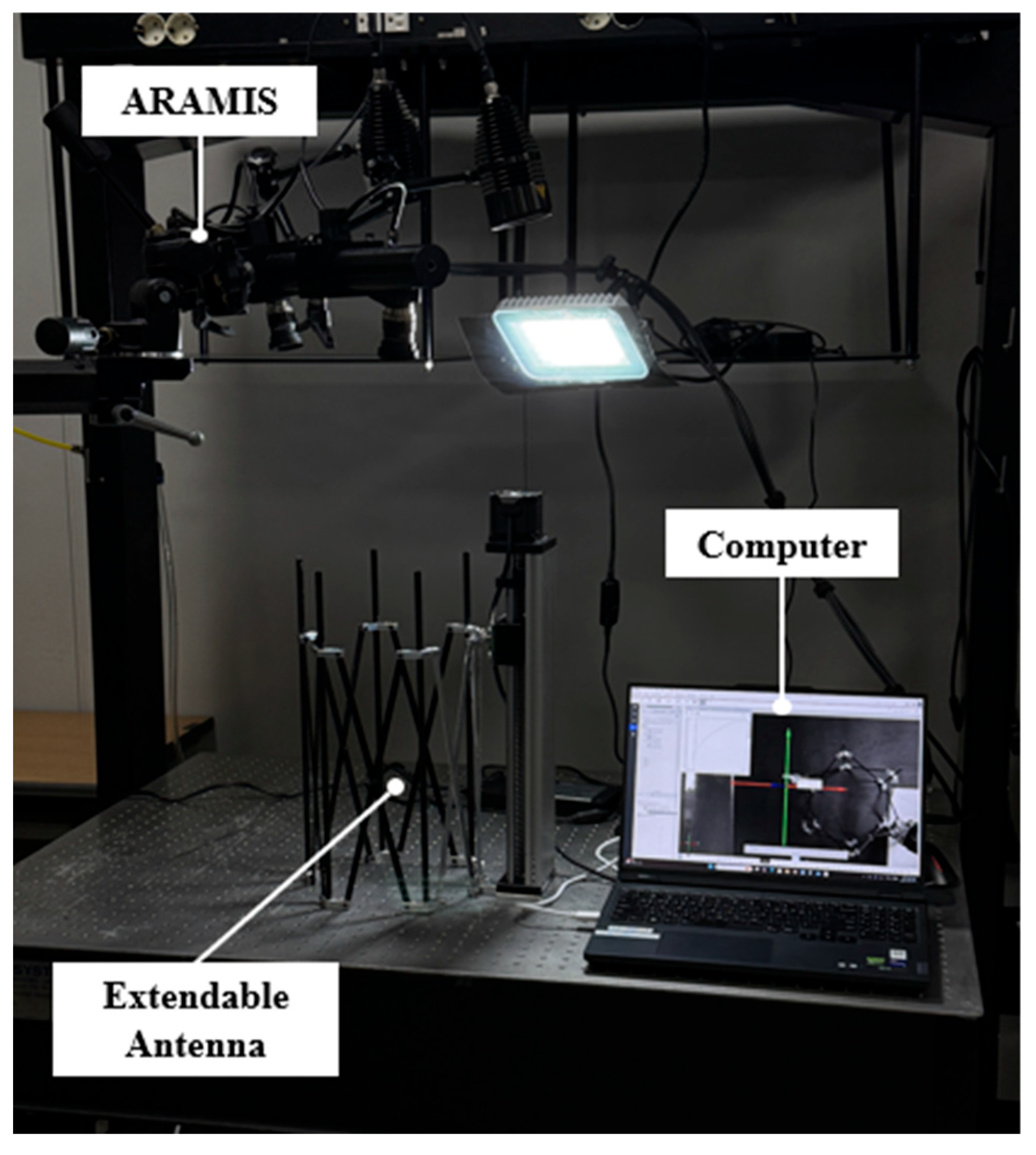
2.1. Fabrication of the TWFS Composite
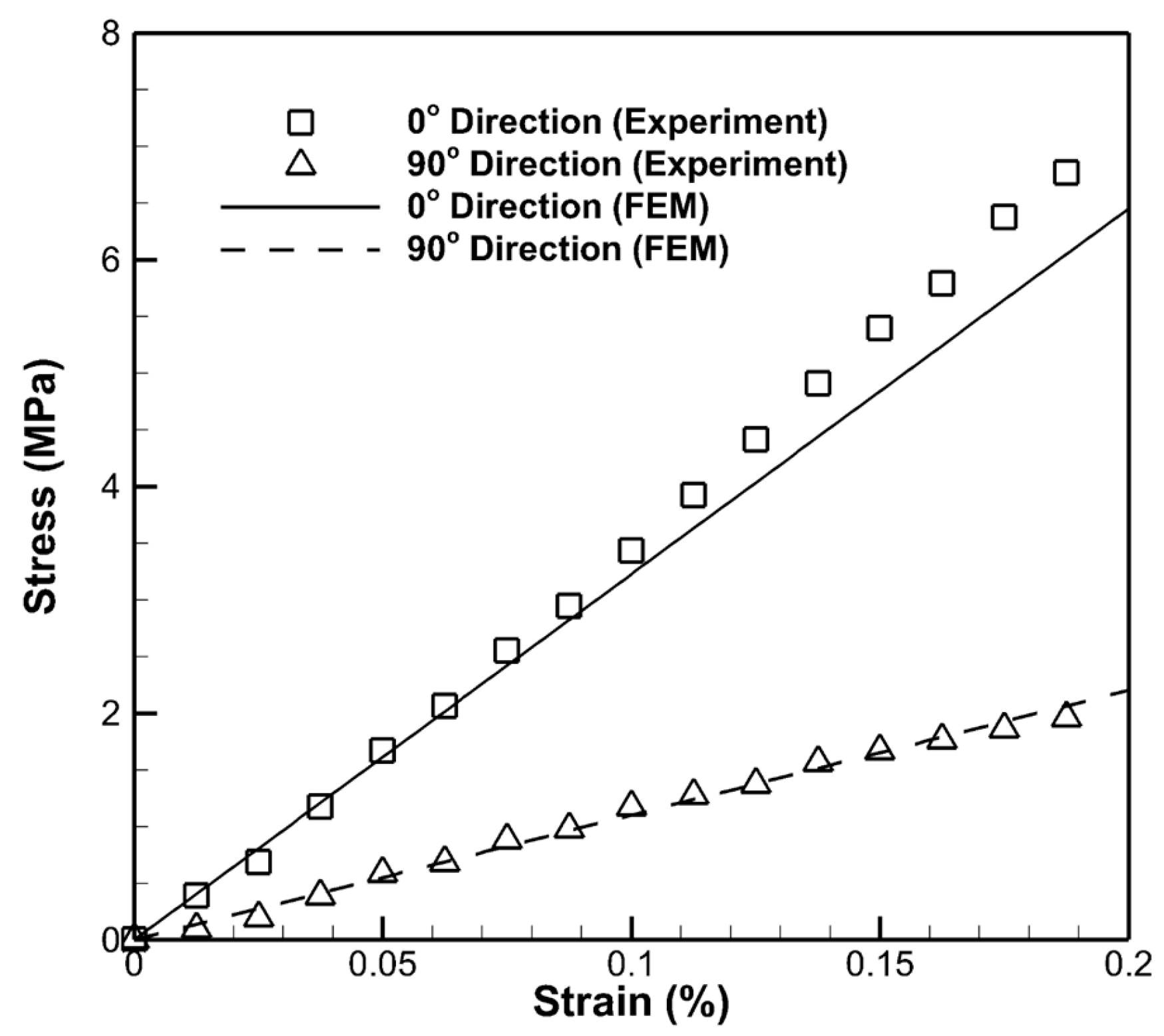
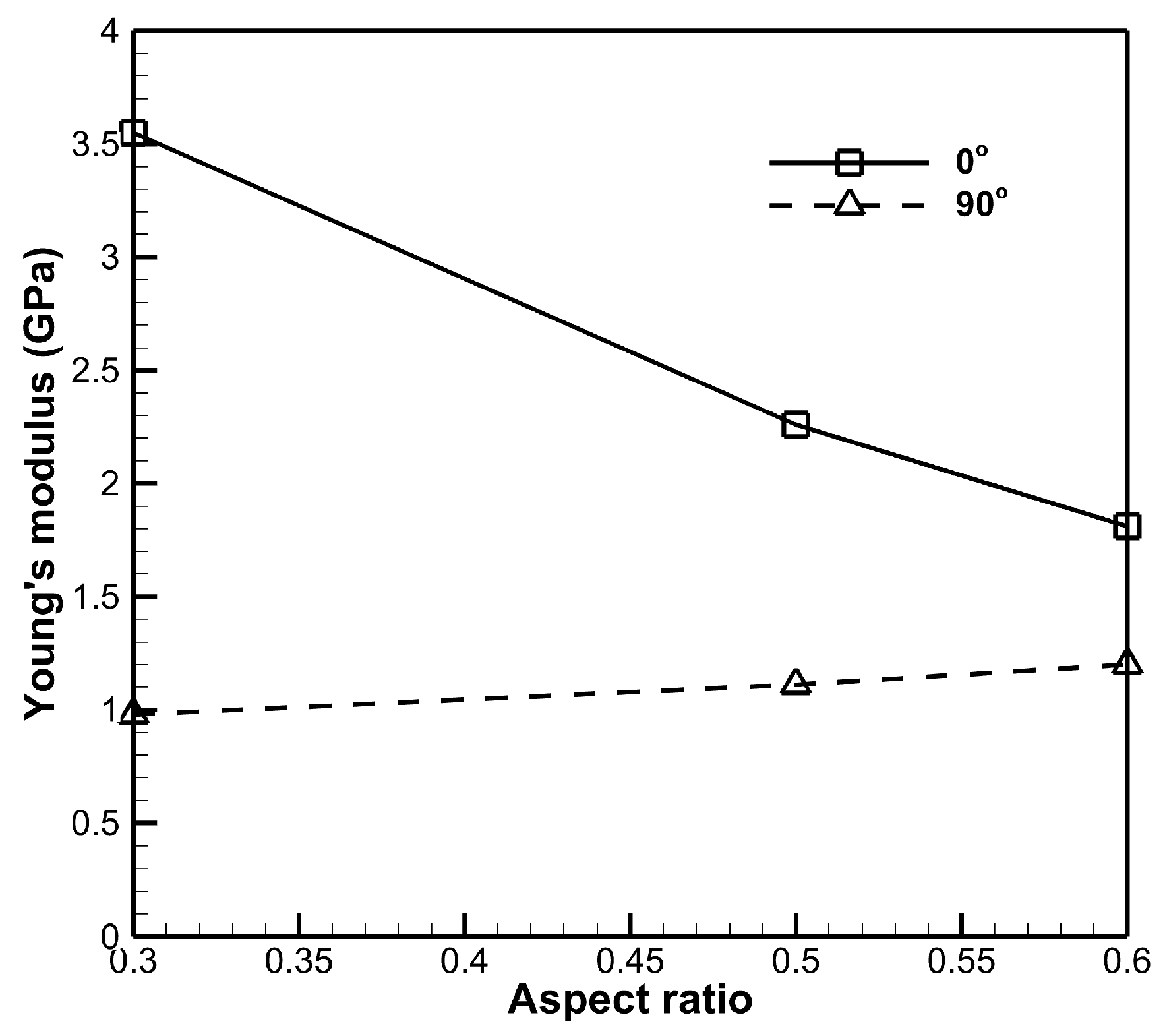
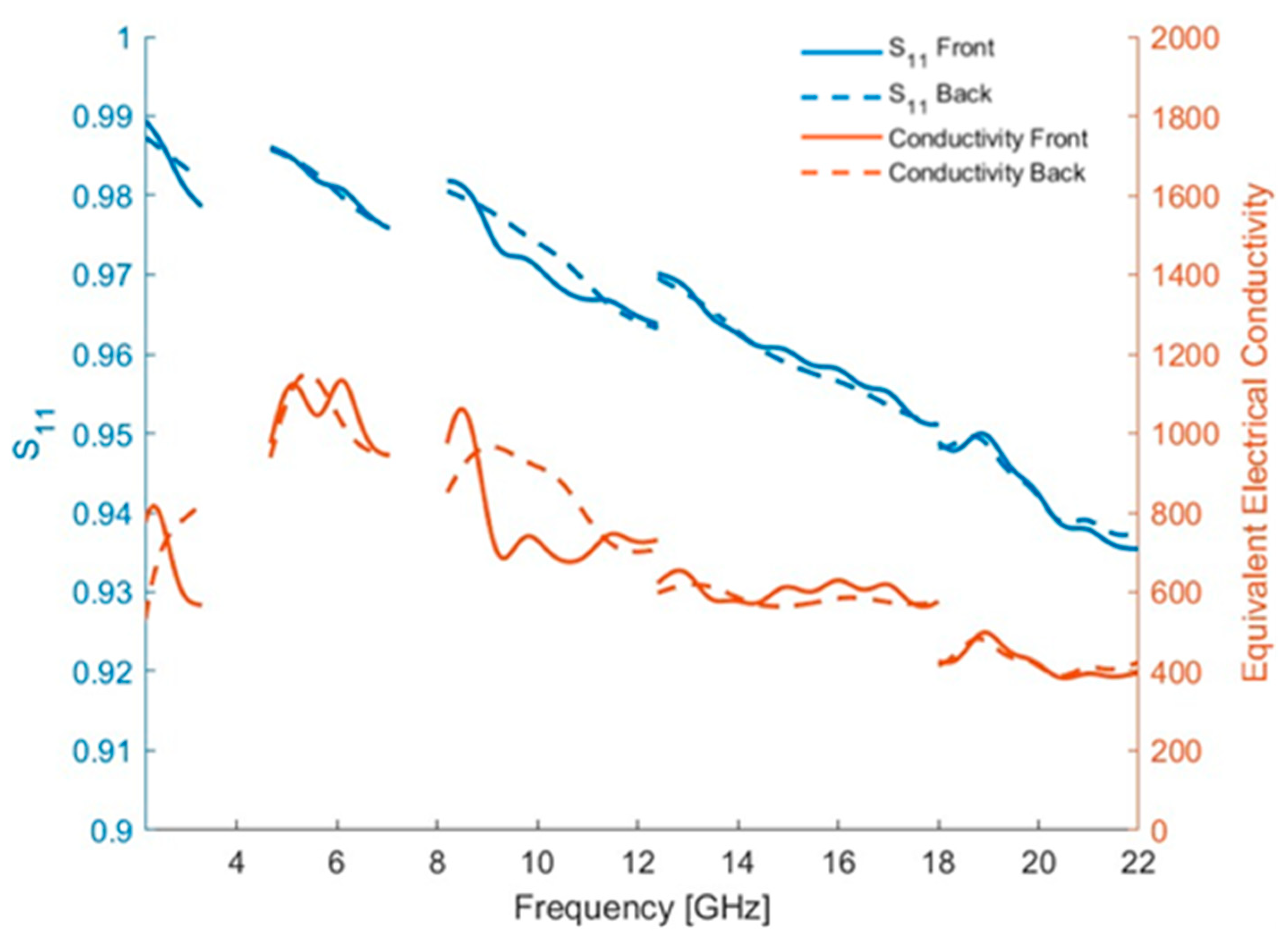
2.2. Electrical Properties of the TWFS
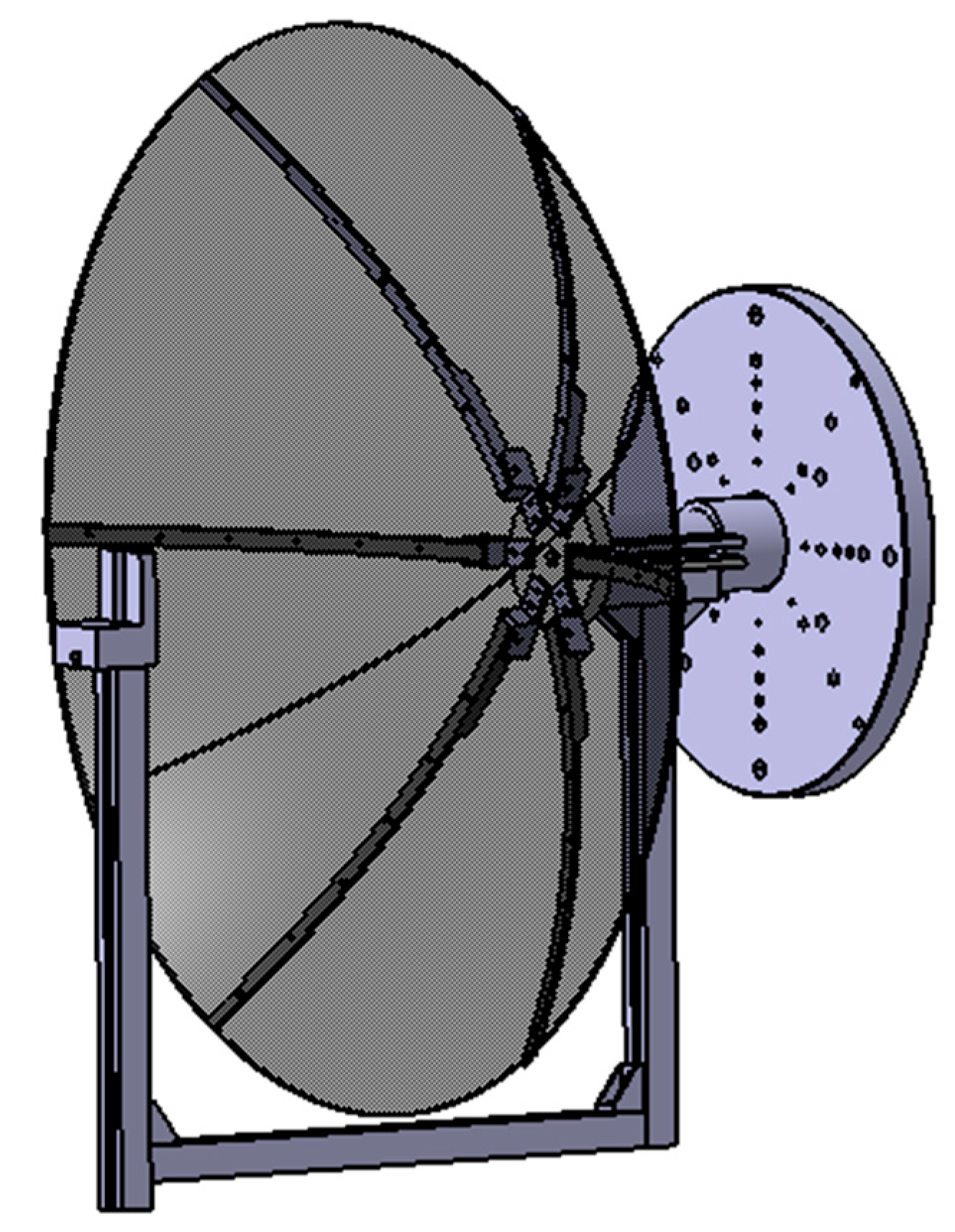
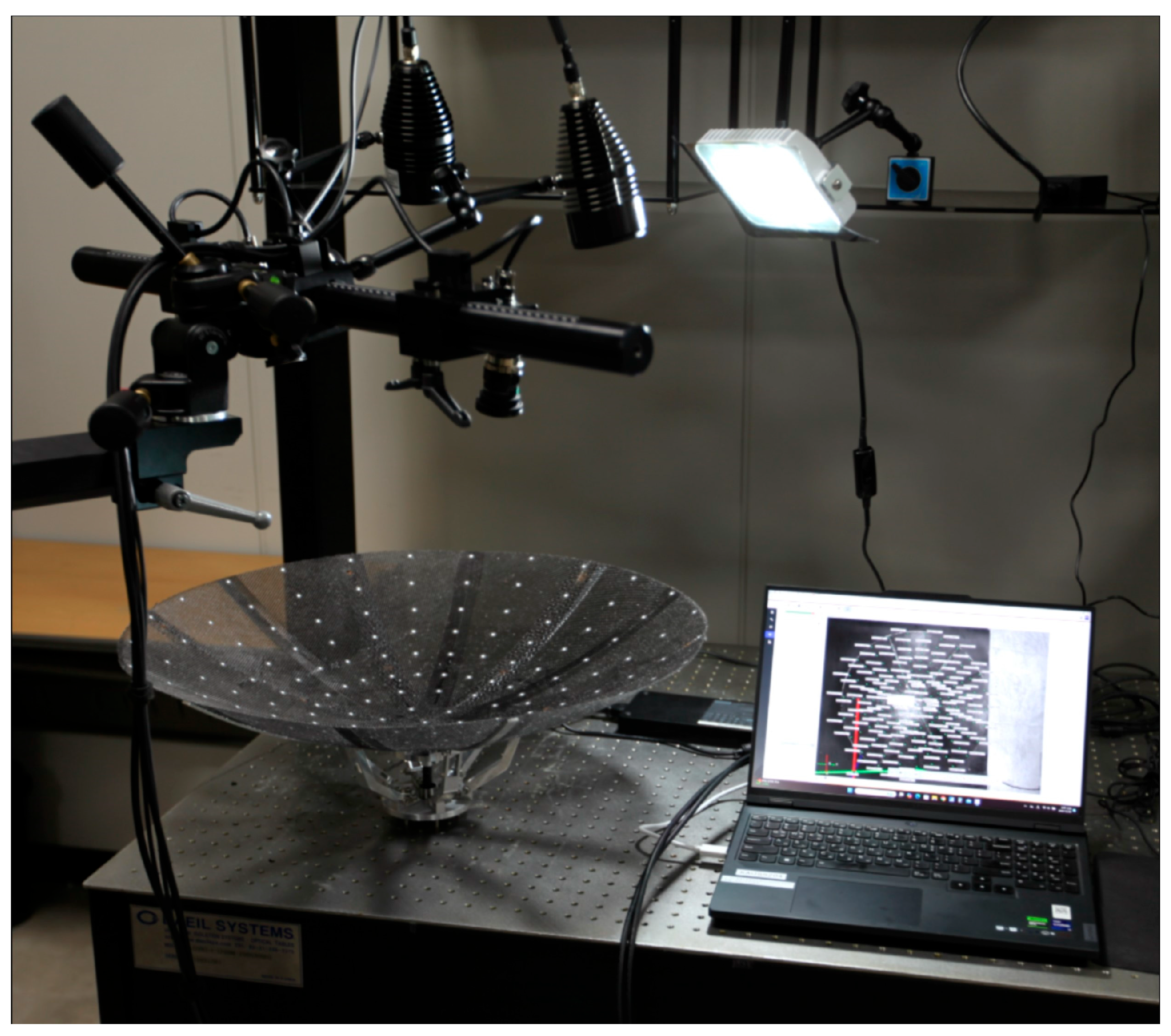
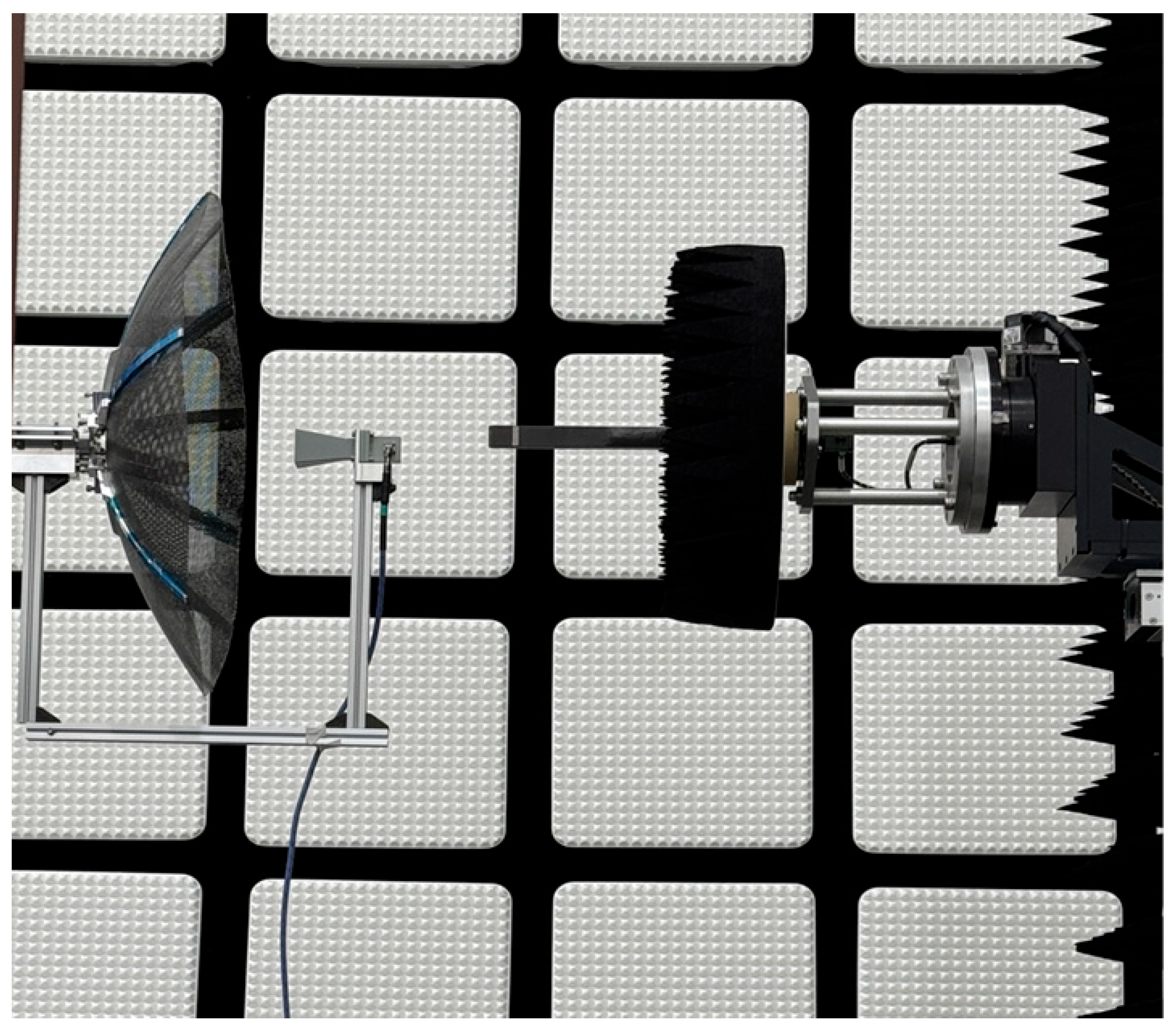
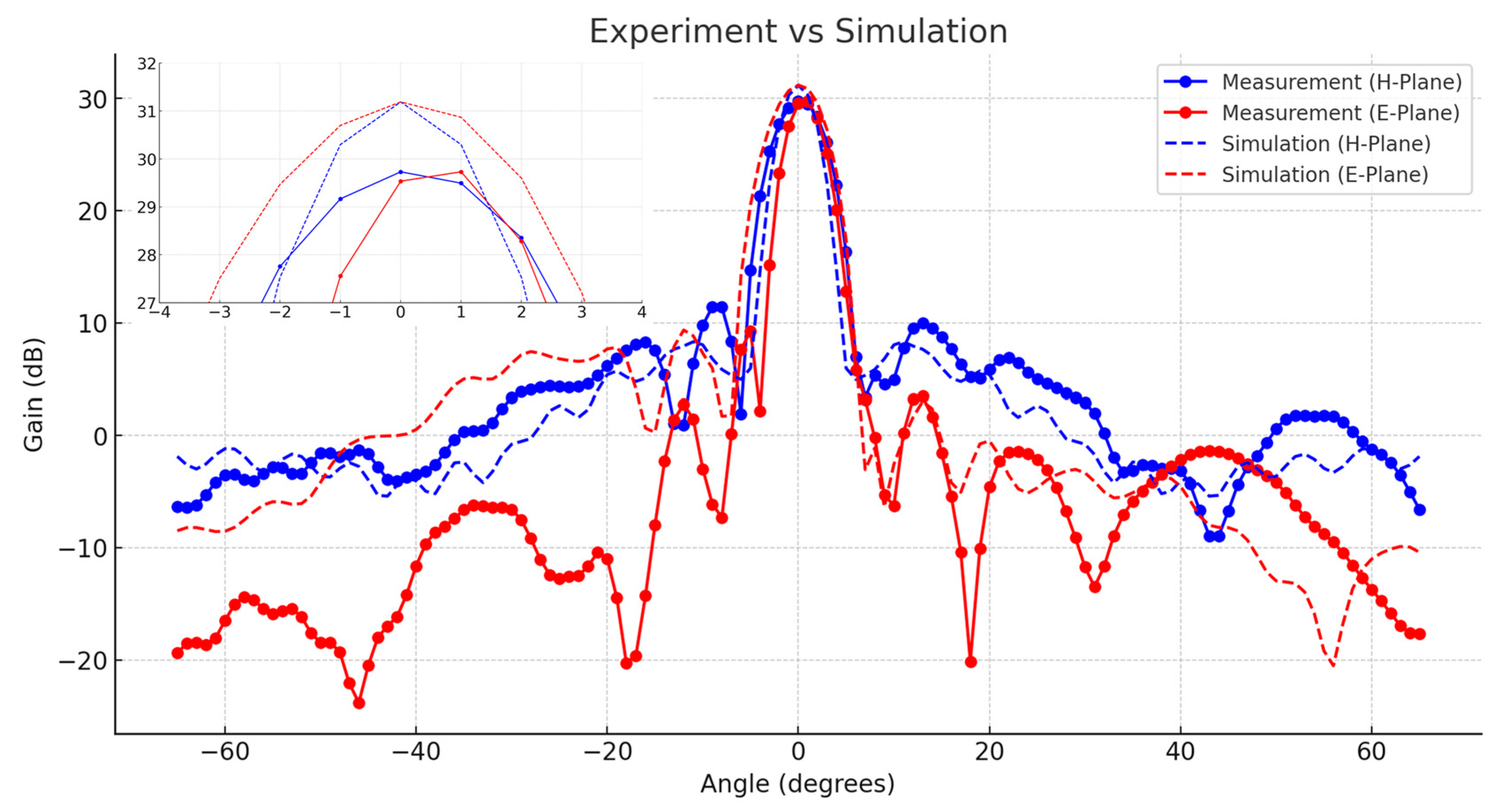
3. Performance of Reflector Antenna
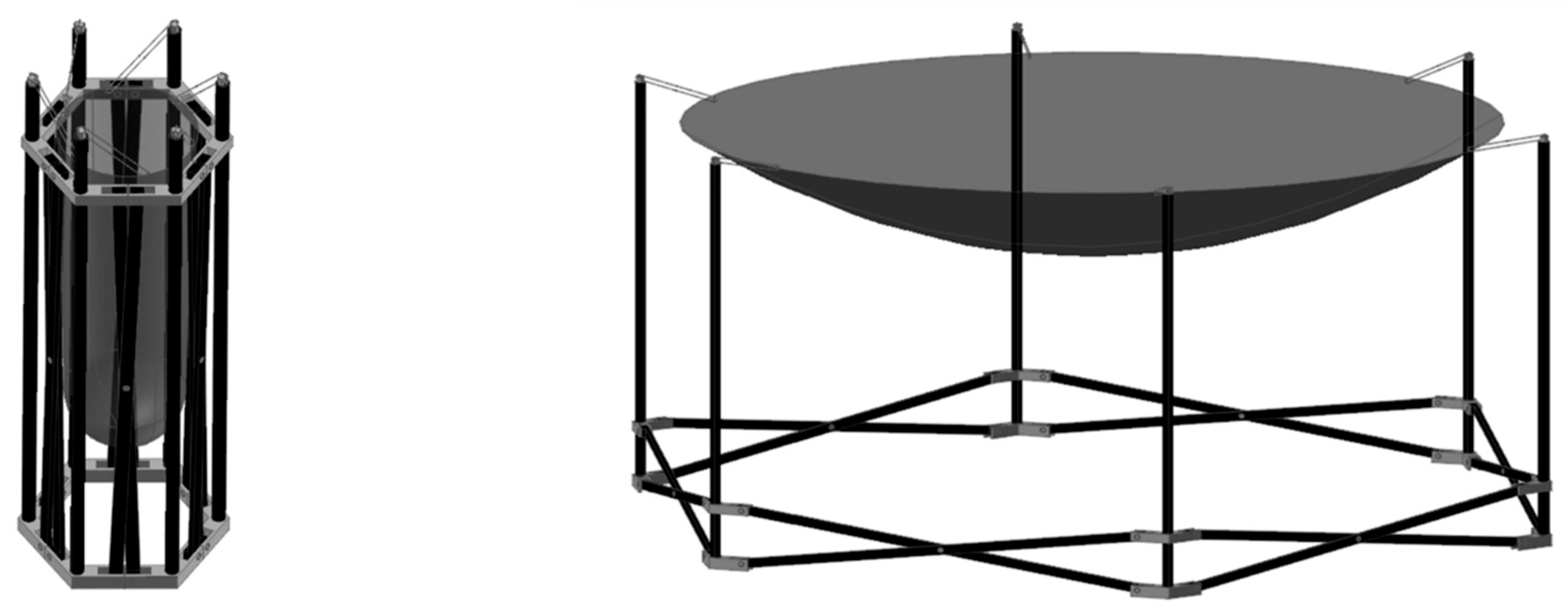
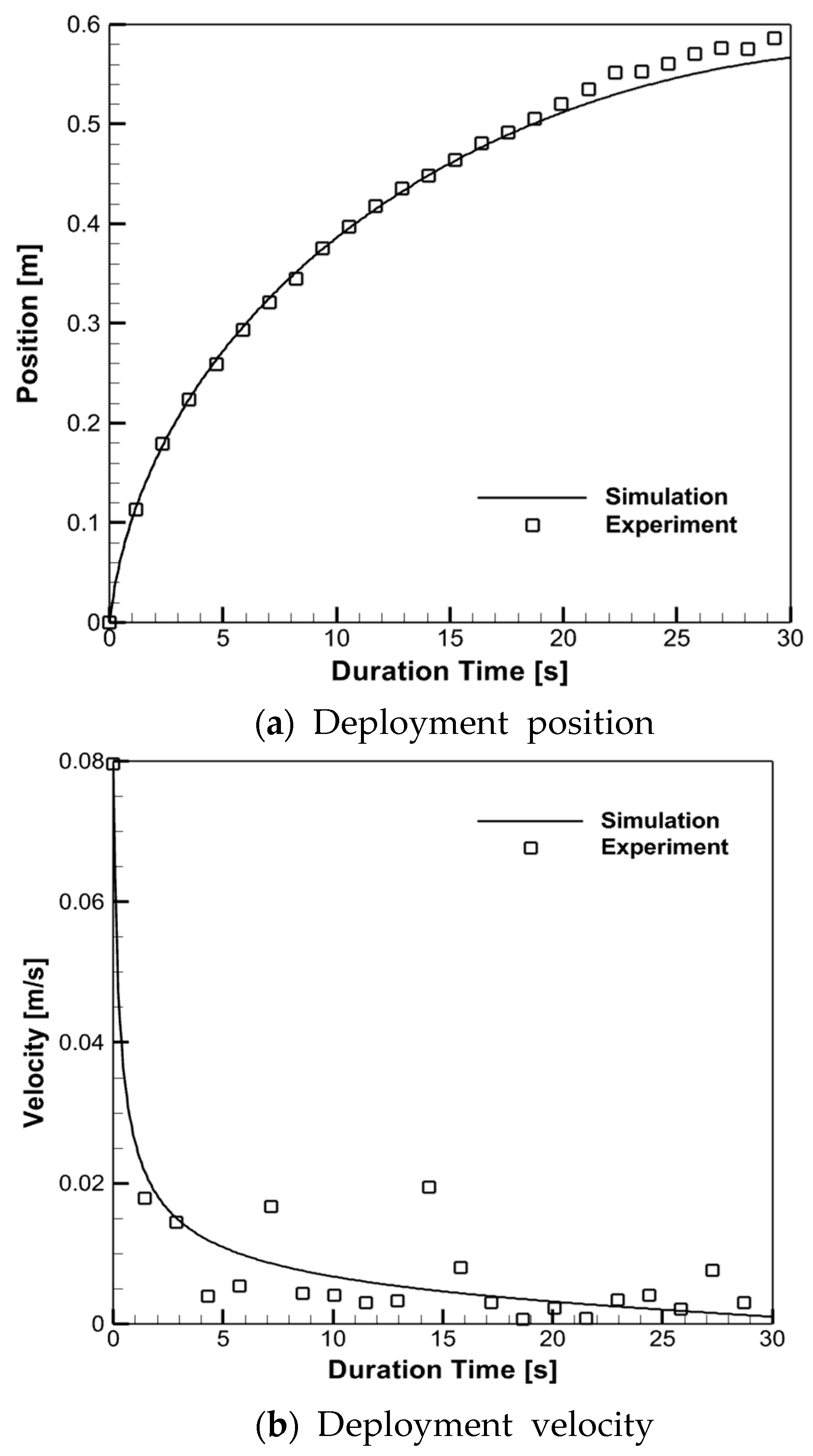
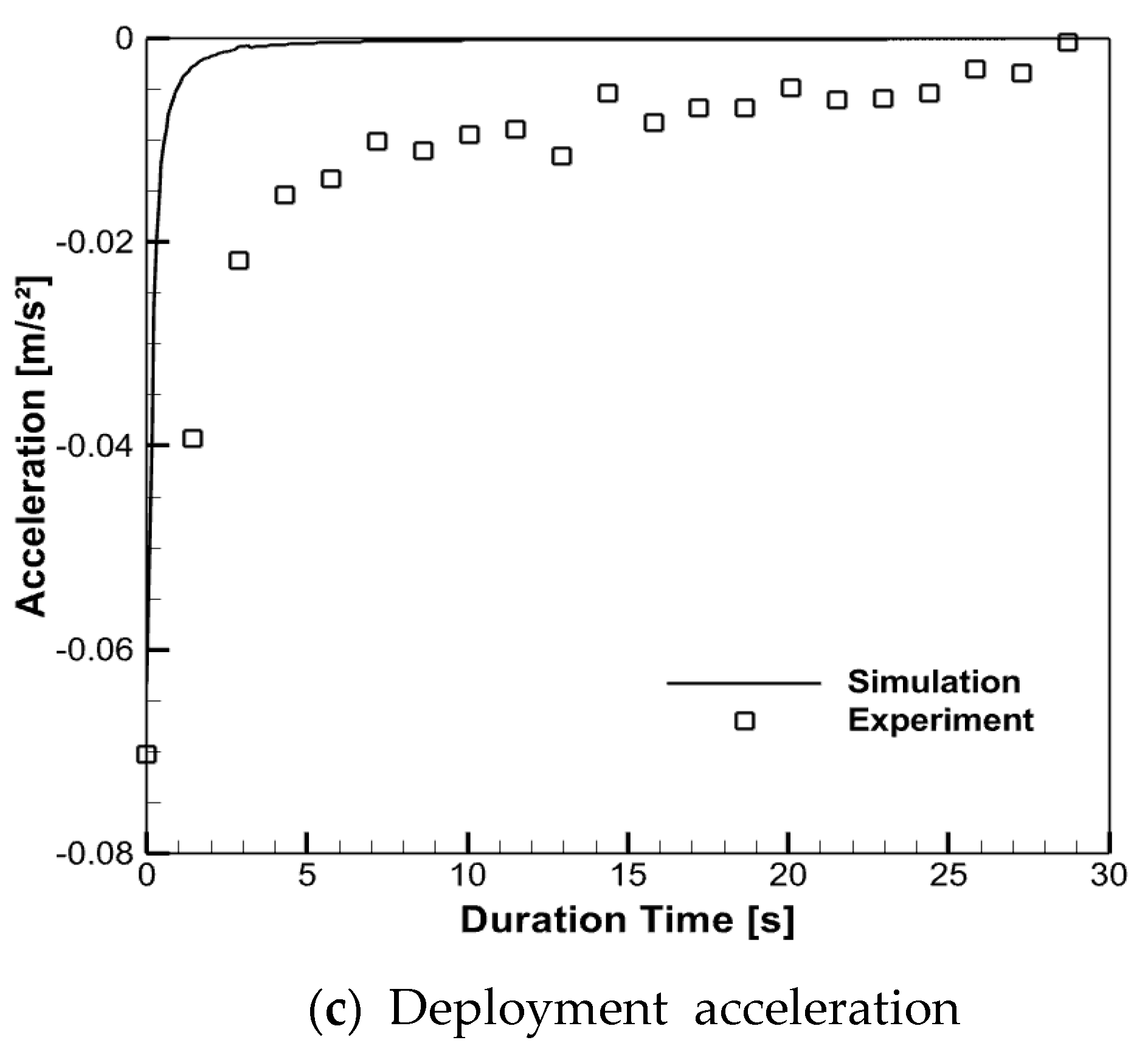
4. Deployment Mechanism
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rahmat-Samii, Y.; Manohar, V.; Kovitz, J.M. For Satellites, Think Small, Dream Big: A Review of Recent Antenna Developments for CubeSats. IEEE Antennas Propag. Mag. 2017, 59, 22–30. [Google Scholar] [CrossRef]
- Hedgepeth, J.M. Accuracy Potentials for Large Space Antenna Reflectors with Passive Structure. J. Spacecr. Rocket. 1982, 19, 211–217. [Google Scholar] [CrossRef]
- Peral, E.; Tanelli, S.; Statham, S.; Joshi, S.; Imken, T.; Price, D.; Sauder, J.; Chahat, N.; Williams, A. RainCube: The First Ever Radar Measurements from a CubeSat in Space. J. Appl. Remote Sens. 2019, 13, 032504. [Google Scholar] [CrossRef]
- Murphey, T.W.; William, F.; Bruce, D.; Juan, M. High Strain Composites. In Proceedings of the 2nd AIAA Spacecraft Structures Conference, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar]
- Datashvili, L.; Maghaldadze, N.; Baier, H.; Friemel, M.; Schmidt, L.D.R.; Luo, T. Enabling Technologies and Architectures at LSS GmbH for European Large Deployable and Reconfigurable Reflector Antennas. In Proceedings of the Deutscher Luft- Und Raumfahrtkongress, Munich, Germany, 23–25 September 2017. [Google Scholar]
- Datashvili, L. Multifunctional and Dimensionally Stable Flexible Fibre Composites for Space Applications. Acta Astronaut. 2010, 66, 1081–1086. [Google Scholar] [CrossRef]
- Tan, L.T.; Pellegrino, S. Ultra Thin Deployable Reflector Antennas. In Proceedings of the 45th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics & Materials Conference, Palm Springs, CA, USA, 19–22 April 2004. [Google Scholar]
- Dufour, L.; Datashvii, L.; Guinot, F.; Legay, H.; Goussetis, G. Origami Deployable Reflector Antenna for CubeSats. In Proceedings of the AIAA SciTech 2021 Forum, Virtual Event, 11–15 & 19–21 January 2021. [Google Scholar]
- Datashvili, L.; Baier, H.; da Rocha-Schmidt, L. Multi-scale Analysis of Structures Made of Triaxially Woven Fabric Composites with Stiff and Flexible Matrix Materials. In Proceedings of the 52nd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Denver, CO, USA, 4–7 April 2011. [Google Scholar]
- Kueh, A.; Pellegrino, S. Thermo-elastic behavior of single ply triaxial woven fabric composites. In Proceedings of the 47th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Newport, RI, USA, 1–4 May 2006. [Google Scholar]
- Kueh, A.; Soykasap, O.; Pellegrino, S. Thermo-Mechanical Behavior of Single-Ply Triaxial Weave Carbon Fiber Reinforced Plastic. In Proceedings of the European Conference on Spacecraft Structures, Materials and Mechanical Testing 2005 (ESA SP-581), Noordwijk, The Netherlands, 10–12 May 2005. [Google Scholar]
- ASTM D638; Standard test method for tensile properties of plastics. ASTM International: West Conshohocken, PA, USA, 2014.
- Aramis 3D Strain Measurement System. Available online: https://www.zeiss.com/metrology/en/home.html (accessed on 4 May 2025).
- Kueh, A.; Pellegrino, S. Triaxial Weave Fabric Composites, CUED/D-STRUCT/TR223. In European Space Agency Contractor Report; University of Cambridge: Cambridge, UK, 2007. [Google Scholar]
- Gao, J.; Chen, W.; Yu, B.; Fan, P.; Zhao, B.; Hu, J.; Zhang, D.; Fang, G.; Peng, F. A multi-scale method for predicting ABD stiffness matrix of single-ply weave-reinforced composite. Compos. Struct. 2019, 230, 111478. [Google Scholar] [CrossRef]
- Tian, W.; Qi, L.; Chao, X.; Liang, J.; Fu, M. Periodic boundary condition and its numerical implementation algorithm for the evaluation of effective mechanical properties of the composites with complicated micro-structures. Compos. Part B Eng. 2019, 162, 1–10. [Google Scholar] [CrossRef]
- Cheng, Q. Fiber-Reinforced Composites; Nova Science Publishers, Inc.: Hauppauge, NY, USA, 2011; pp. 612–615. [Google Scholar]
- Kueh, A. Size-influenced mechanical isotropy of singly-plied triaxially woven fabric composites. Compos. Part A Appl. Sci. Manuf. 2014, 57, 76–87. [Google Scholar] [CrossRef]
- Yoon, S.S.; Lee, J.W.; Lee, T.-K.; Roh, J.H. Insensitivity Characteristics in the Dual Polarization of Deployable CFRP Reflector Antennas for SAR. IEEE Trans. Antennas Propag. 2018, 66, 88–95. [Google Scholar] [CrossRef]
- Cherniavsky, A.G.; Gulyayev, V.I.; Gaidaichuk, V.V.; Fedoseev, A.I. Large Deployable Space Antennas Based on Usage of Polygonal Pantograph. J. Aerosp. Eng. 2005, 18, 139–145. [Google Scholar] [CrossRef]





| Property | Value |
|---|---|
| 97.79 GPa | |
| 6.34 GPa | |
| 1.64 MPa | |
| 2.27 MPa | |
| 0.37 | |
| 0.40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, D.-S.; Keum, D.-H.; Choi, J.-H.; Lee, M.-H.; Park, K.; Jung, H.-Y.; Kang, D.-S.; Yun, J.-H.; Lee, J.-W.; Roh, J.-H. Flexible Surface Reflector Antenna for Small Satellites. Aerospace 2025, 12, 414. https://doi.org/10.3390/aerospace12050414
Kang D-S, Keum D-H, Choi J-H, Lee M-H, Park K, Jung H-Y, Kang D-S, Yun J-H, Lee J-W, Roh J-H. Flexible Surface Reflector Antenna for Small Satellites. Aerospace. 2025; 12(5):414. https://doi.org/10.3390/aerospace12050414
Chicago/Turabian StyleKang, Dong-Seok, Dong-Hun Keum, Jun-Hyeong Choi, Min-Hyuk Lee, Kitae Park, Hwa-Young Jung, Deok-Soo Kang, Ji-Hyeon Yun, Jae-Wook Lee, and Jin-Ho Roh. 2025. "Flexible Surface Reflector Antenna for Small Satellites" Aerospace 12, no. 5: 414. https://doi.org/10.3390/aerospace12050414
APA StyleKang, D.-S., Keum, D.-H., Choi, J.-H., Lee, M.-H., Park, K., Jung, H.-Y., Kang, D.-S., Yun, J.-H., Lee, J.-W., & Roh, J.-H. (2025). Flexible Surface Reflector Antenna for Small Satellites. Aerospace, 12(5), 414. https://doi.org/10.3390/aerospace12050414






