Analysis of Aerodynamic Characteristics of Rotating Detonation Turbine Based on Proper Orthogonal Decomposition Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Two-Dimensional Numerical Model and Boundary Conditions
- (1)
- When > , the premixed gas is not injected into the combustor.
- (2)
- When < < , the premixed gas is injected into the combustor at subsonic speed.
- (3)
- When < < , the throat of the nozzle remains choked, with a normal shock wave downstream, and the premixed gas is injected into the combustor at subsonic speed.
- (4)
- When < , the premixed gas is injected into the combustor at supersonic speed.
2.2. Proper Orthogonal Decomposition Method
3. Results and Discussion
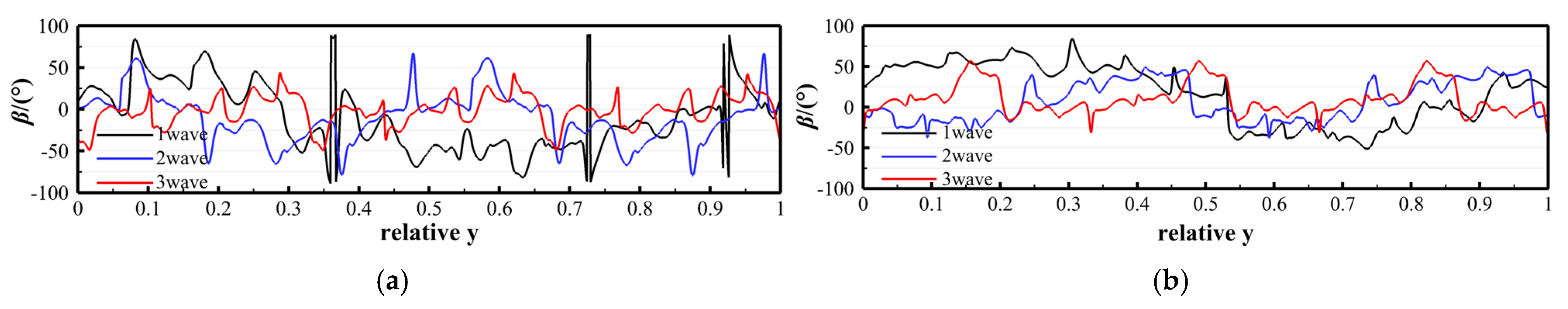
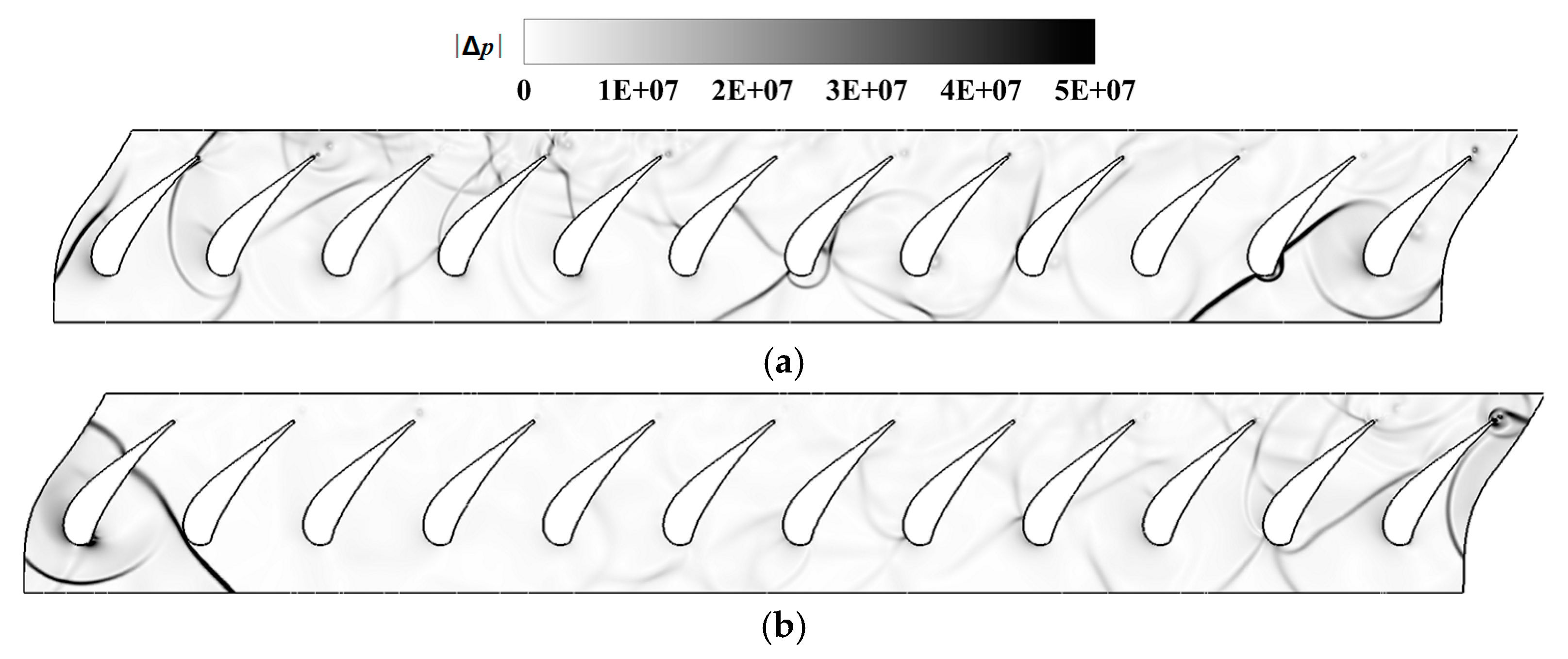
3.1. Turbine Flow Field Analysis
3.2. Analysis Based on POD
4. Conclusions
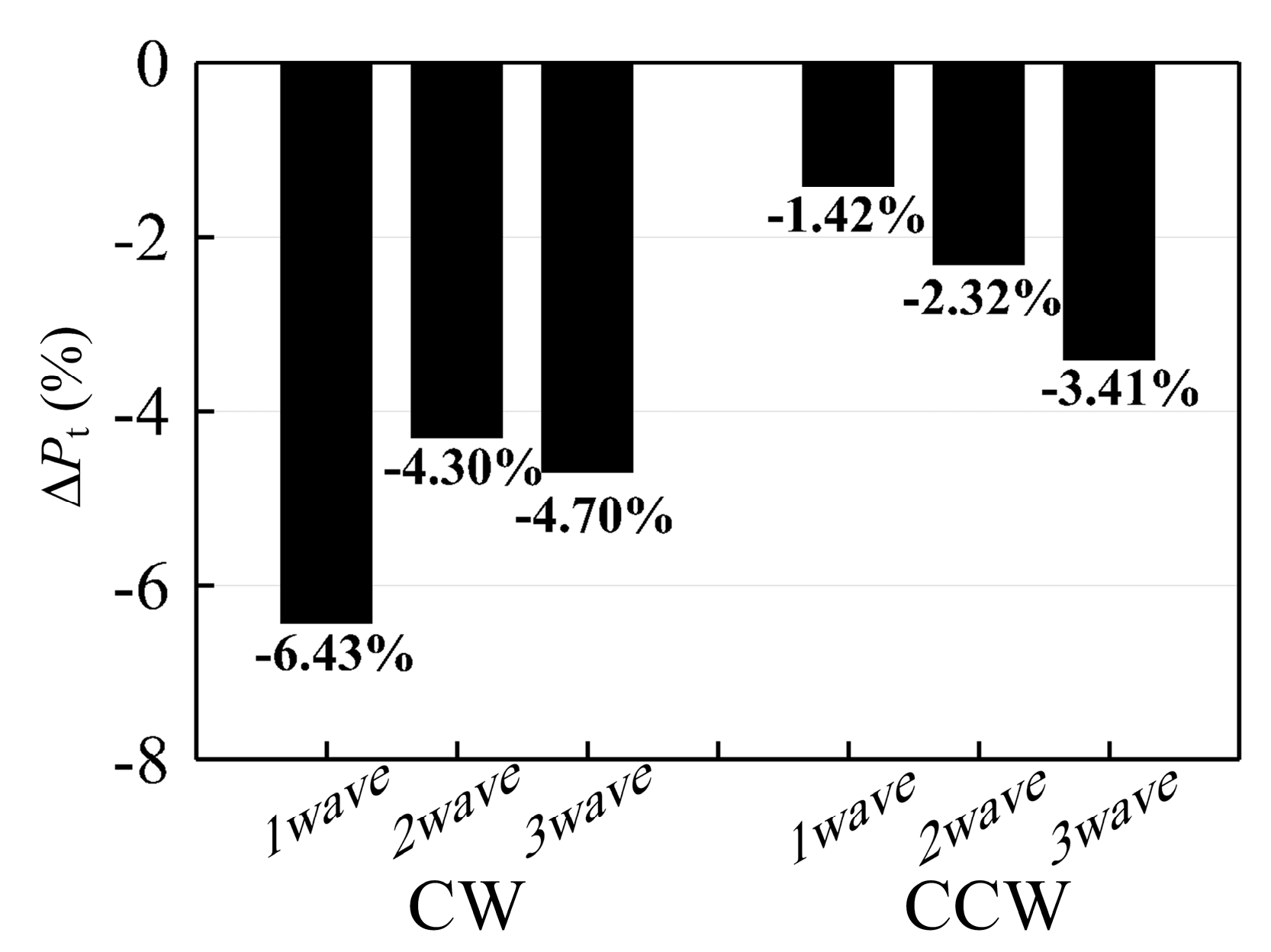
- In the turbine, rotating detonation combustion yields total pressure gain yet causes significant unsteady flow. Guide vanes somewhat suppress detonation-induced pressure fluctuations, but total pressure loss and airflow non-uniformity still exist, lowering rotor efficiency. More detonation waves reduce rotor inlet pressure gain but improve airflow uniformity and rotor efficiency. Using the rotor specific work gain–pulsation instability ratio as the performance metric, the turbine performs best in the double-wave counterclockwise mode (27.9% work gain, 5.0% instability, 86.4% efficiency) and worst in the single-wave clockwise mode (20.9% work gain, 11.8% instability, 84.0% efficiency).
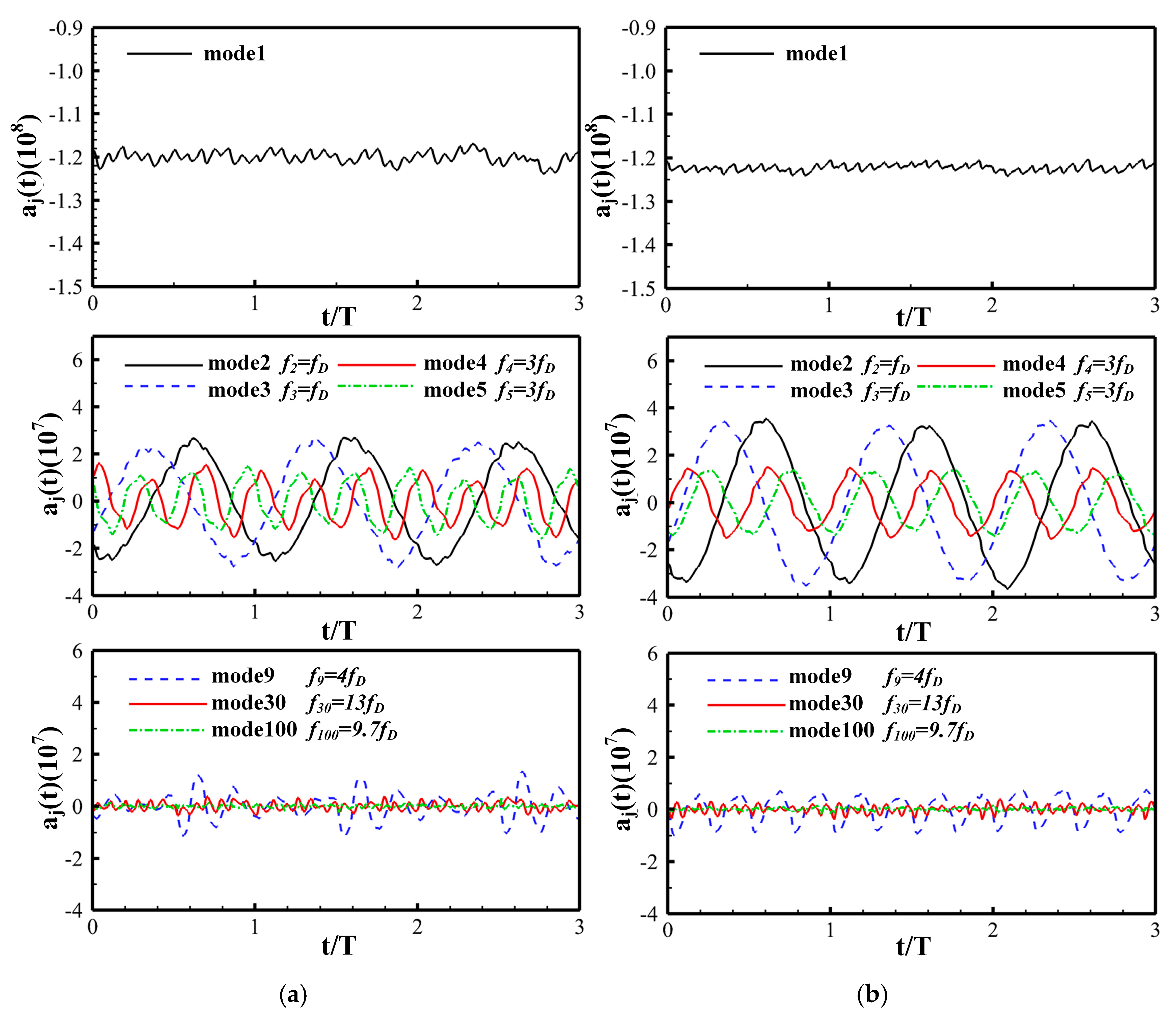
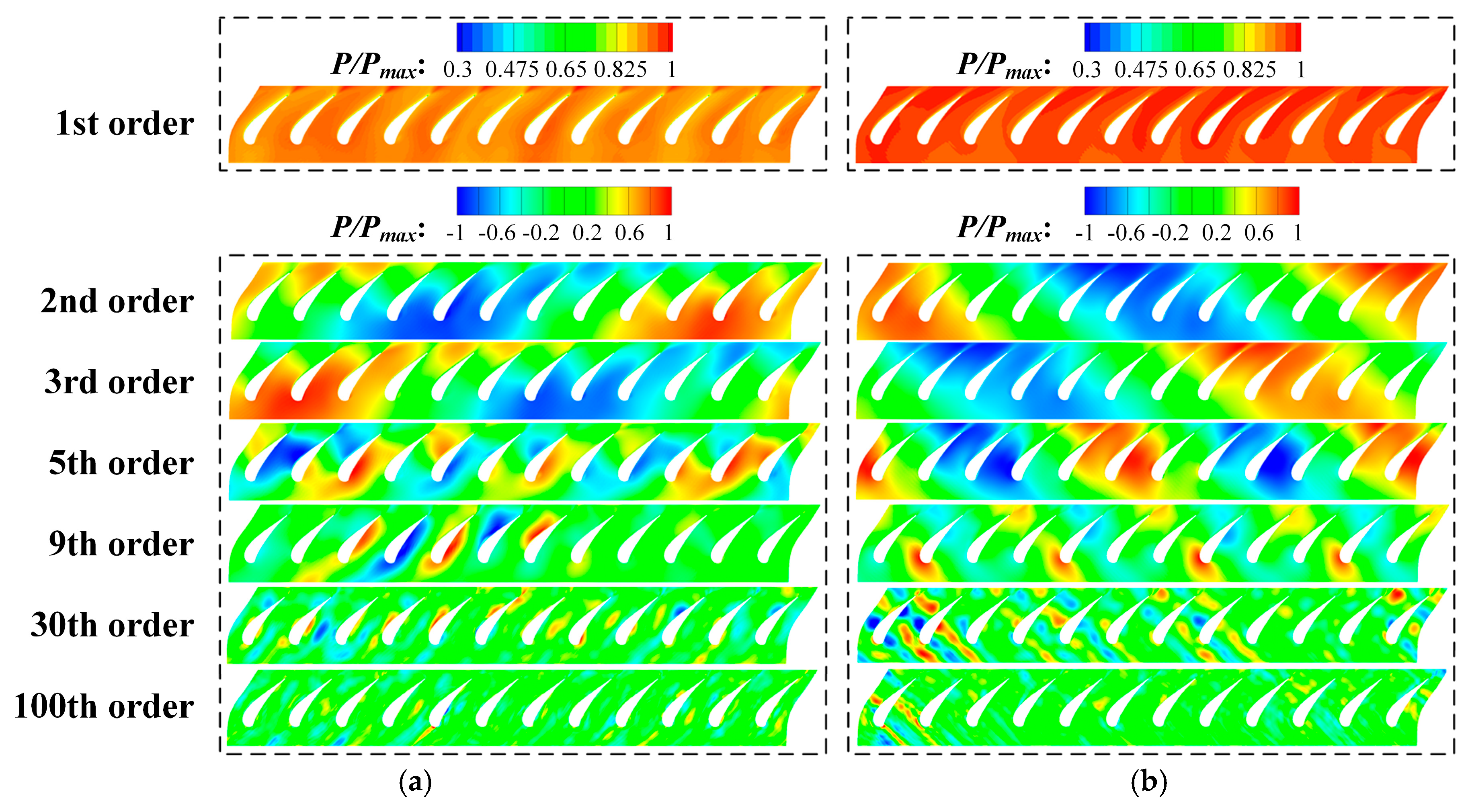
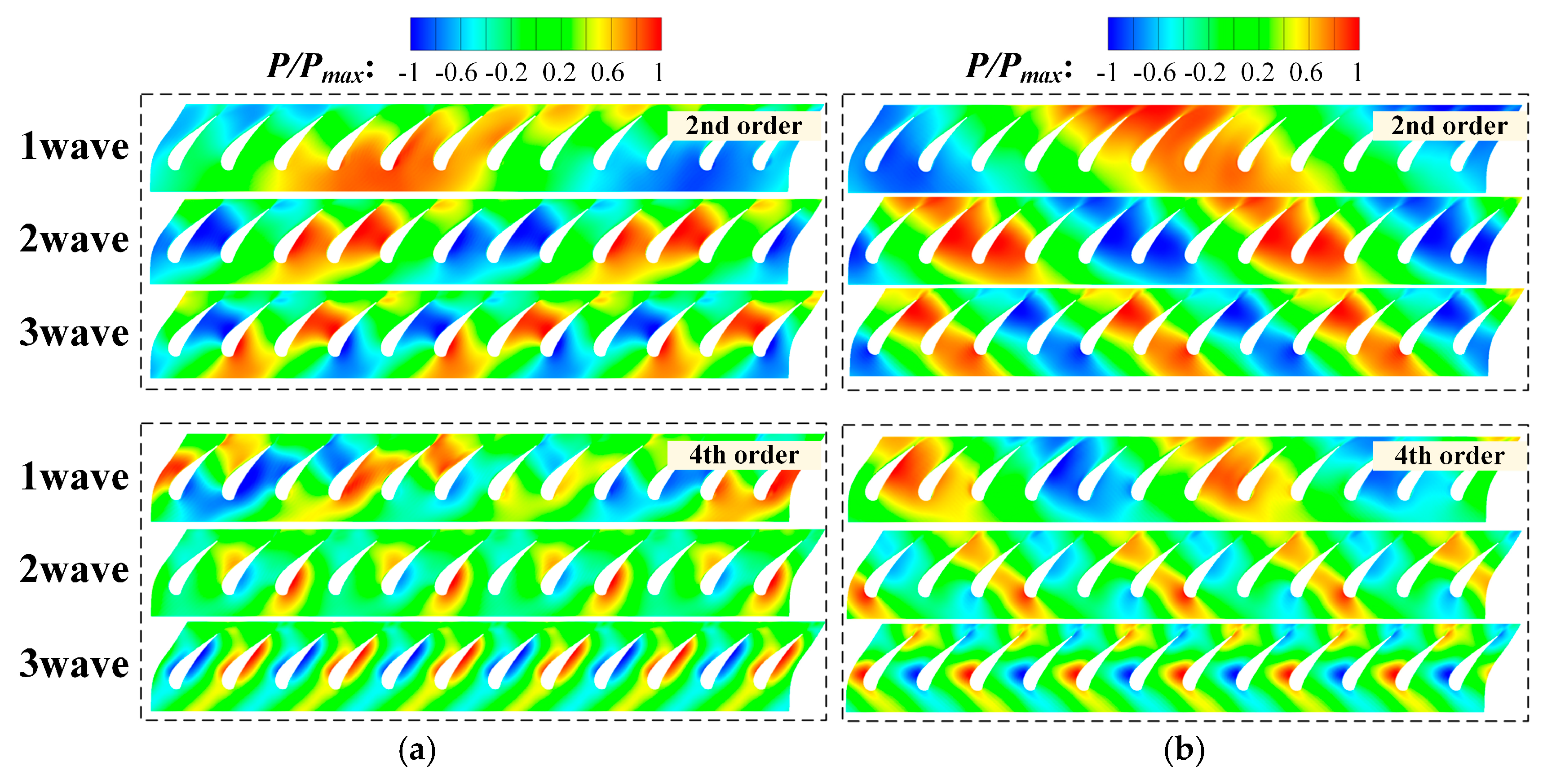
- POD modal analysis on the turbine guide vane region reveals distinct flow structures for each mode. The first-order mode shows time-averaged flow without clear wave systems, while other modes display non-uniform pressure distributions, indicating pulsating waves. Low-order modes beyond the first exhibit “pairing,” with adjacent modes sharing similar energy ratios and spatiotemporal structures but differing in phase. This suggests the guide vane channel’s wave system motion results from paired mode superposition.
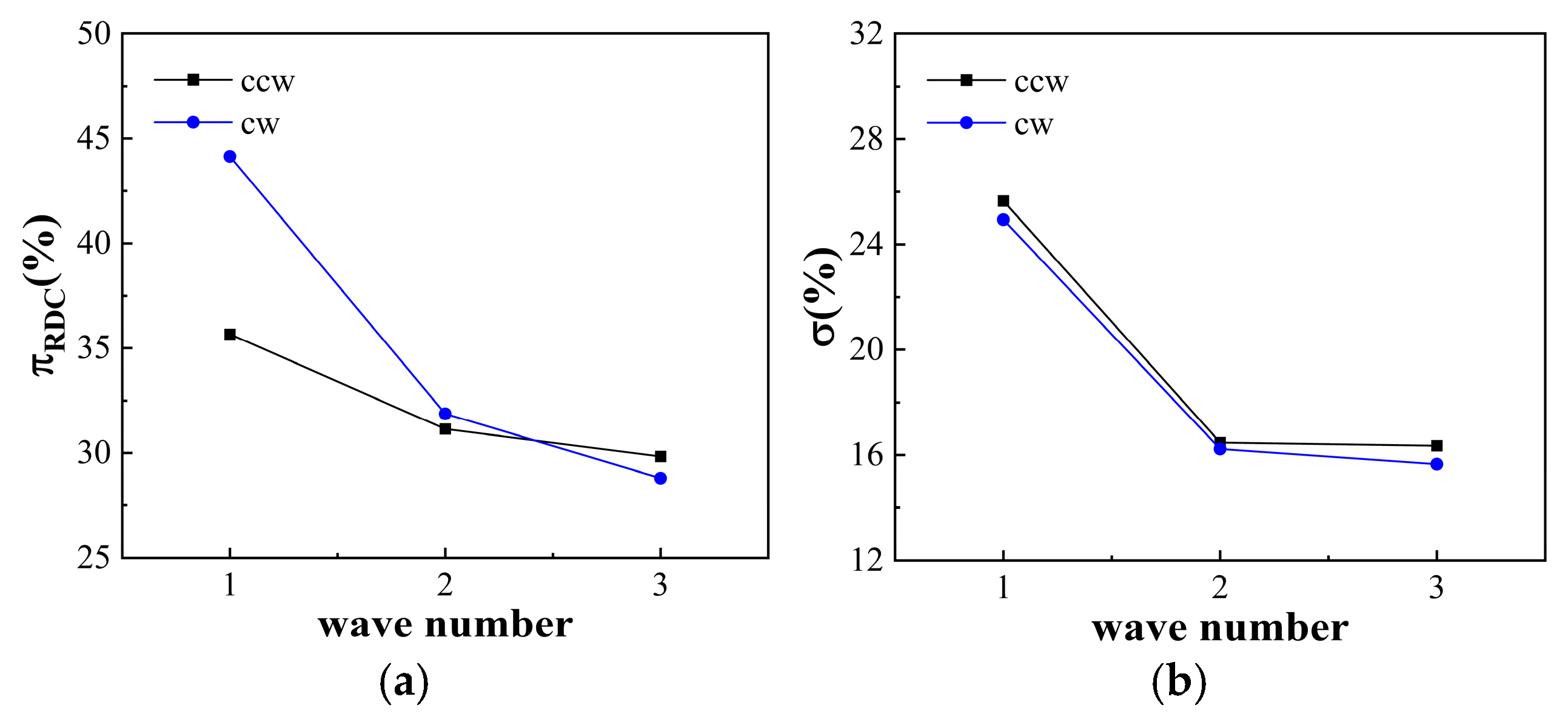
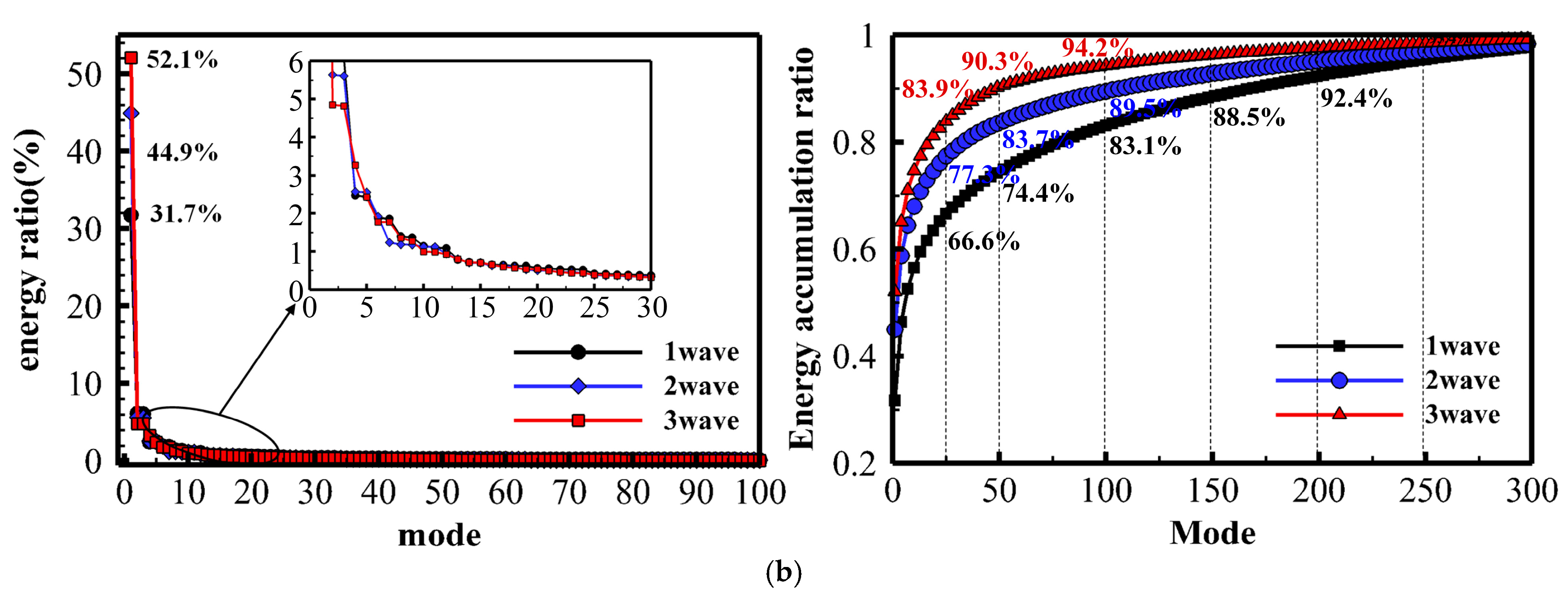
- In the rotating detonation turbine guide vanes flow field, wave propagation direction and wave front number influence the energy distribution and stability. As the number of detonation waves increases, the first-order mode energy proportion in the guide vanes flow field enhances, from 30.2% and 31.7% for single-wave to 51.2% and 52.1% for triple-wave. The cumulative proportion of the first 30-order energy also rises from 64.1% and 68.6% for single-wave to 85.4% and 85.6% for triple-wave, indicating decreased flow unsteadiness. The counterclockwise mode exhibits superior time-averaged flow dominance under identical wave numbers, attributed to its channel reflection wave system characteristics.
- In a rotating detonation turbine engine, the detonation chamber’s outflow is highly pulsatile, and the turbine components’ inflow is quasi-periodically unsteady, deviating from the design’s quasi-steady assumption and impacting the turbine’s work capability. Analysis shows that a higher first-order mode energy ratio and lower pulsating wave system mode energy ratio enhance rotor work efficiency and stability. Thus, enhancing the flow field’s average flow characteristics and increasing the first-order mode energy ratio can improve the rotating detonation turbine’s work performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| W | Width |
| x | X-coordinate |
| CW | Clockwise propagation |
| t | Time |
| RMS | Root mean square |
| L | Length |
| y | Y-coordinate |
| CCW | Counterclockwise propagation |
| n | Number |
| Greek | |
| Pre-exponential factor | |
| Activation energy (J/(kg·mol)) | |
| Reaction rate constant | |
| Temperature (K) | |
| Pressure (Pa) | |
| Pressure fluctuation component (Pa) | |
| Time-varying functions for temporal features | |
| Orthogonal basis functions for spatial features | |
| Total pressure gain | |
| Specific heat ratio | |
| Equivalence ratio | |
| Turbine specific work | |
| Position of an aerodynamic cross-section | |
| Temperature index | |
| Molar gas constant (J/(mol·K)) | |
| Area of an aerodynamic cross-section (m2) | |
| Total temperature (K) | |
| Total pressure (Pa) | |
| Time-averaged pressure component (Pa) | |
| Eigenvalue | |
| Detonation cycle | |
| Non-uniformity of total pressure | |
| Specific heat at constant pressure J/(kg·K) | |
| Mach number | |
| Turbine efficiency | |
| The relative flow angle (°) | |
| Subscript | |
| w | Wall |
| T | Turbine |
| 4 | Turbine inlet |
| RDC | Rotating detonation chamber |
| t | throat |
| 0 | Freestream |
| 5 | Turbine exit |
| MFA | The instantaneous mass-weighted average |
References
- Raman, V.; Prakash, S.; Gamba, M. Nonidealities in Rotating Detonation Engines. Annu. Rev. Fluid Mech. 2023, 55, 639–674. [Google Scholar] [CrossRef]
- Ma, J.Z.; Luan, M.-Y.; Xia, Z.-J.; Wang, J.-P.; Zhang, S.; Yao, S.; Wang, B. Recent Progress, Development Trends, and Consideration of Continuous Detonation Engines. AIAA J. 2020, 58, 4976–5035. [Google Scholar] [CrossRef]
- Zifei, J.; Tianqi, L.; Huiqiang, Z. Performance Analysis of Rotating Detonative Airbreathing Combined Cycle Engine. J. Rocket Propuls. 2021, 47, 86–92. [Google Scholar]
- Zifei, J.; Huiqiang, Z.; Qiaofeng, X.; Bing, W. Thermodynamic Process and Performance Analysis of the Continuous Rotating Detonation Turbine Engine. J. Tsinghua Univ. (Sci. Technol.) 2018, 58, 899–905. [Google Scholar]
- Sousa, J.; Paniagua, G.; Morata, E.C. Thermodynamic Analysis of a Gas Turbine Engine with a Rotating Detonation Combustor. Appl. Energy 2017, 195, 247–256. [Google Scholar] [CrossRef]
- Qi, L.; Dong, J.; Hong, W.; Wang, M.; Lu, T. Investigation of Rotating Detonation Gas Turbine Cycle under Design and Off-Design Conditions. Energy 2023, 264, 126212. [Google Scholar] [CrossRef]
- Naples, A.; Hoke, J.; Battelle, R.T.; Wagner, M.; Schauer, F.R. RDE Implementation into an Open-Loop T63 Gas Turbine Engine. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017; p. 1747. [Google Scholar]
- Naples, A.; Hoke, J.; Battelle, R.; Schauer, F. T63 Turbine Response to Rotating Detonation Combustor Exhaust Flow. J. Eng. Gas Turbines Power 2019, 141, 021029. [Google Scholar] [CrossRef]
- Wu, Y.; Weng, C.; Zheng, Q.; Wei, W.; Bai, Q. Experimental Research on the Performance of a Rotating Detonation Combustor with a Turbine Guide Vane. Energy 2021, 218, 119580. [Google Scholar] [CrossRef]
- Wei, W. Influence of Propagation Direction on Operation Performance of Rotating Detonation Combustor with Turbine Guide Vane. Def. Technol. 2021, 17, 1617–1624. [Google Scholar] [CrossRef]
- Wei, W.-L.; Weng, C.-S.; Wu, Y.-W.; Zheng, Q. Experimental Research on Influence of Turbine Guide Vane on Propagation Characteristics of Rotating Detonation Wave. Acta Phys. Sin. 2020, 69, 064703. [Google Scholar] [CrossRef]
- Zhou, S.; Ma, H.; Li, S.; Liu, D.; Yan, Y.; Zhou, C. Effects of a Turbine Guide Vane on Hydrogen-Air Rotating Detonation Wave Propagation Characteristics. Int. J. Hydrog. Energy 2017, 42, 20297–20305. [Google Scholar] [CrossRef]
- Li, Q.; Wu, Y.; Weng, C.; Wei, W.; Xu, G. Numerical Simulation of Interaction Between Rotating Detonation Wave and Turbine Plane Cascade. J. Propuls. Technol. 2022, 43, 294–304. [Google Scholar]
- Ji, B.; Li, J.; Wang, Z. Effect Mechanism of Pulsating Flow on Unsteady Aerodynamic Performance of Turbine. J. Aerosp. Power 2020, 35, 410–421. [Google Scholar]
- Lumley, J.L. The Structure of Inhomogeneous Turbulent Flows. Atmos. Turbul. Radio Wave Propag. 1967, 166–178. [Google Scholar]
- Torregrosa, A.J.; Broatch, A.; García-Tíscar, J.; Gomez-Soriano, J. Modal Decomposition of the Unsteady Flow Field in Compression-Ignited Combustion Chambers. Combust. Flame 2018, 188, 469–482. [Google Scholar] [CrossRef]
- Wang, L.; Gao, L.; Mao, X.; Guo, Y.; Yu, Y. Analysis of Tip Unsteady Flow Field in a Counter-Rotating Compressor Based on POD Method. J. Aerosp. Power 2025, 40, 20220896. [Google Scholar]
- Qiang, L.; Zhenbing, L.; Xiong, D.; Lin, W.; Yan, Z. Modal Analysis of Dual Syntehtic Jets Based on POD Method. Acta Aerodyn. Sin. 2020, 38, 1027–1033. [Google Scholar]
- Skilskyy, V.; Rossano, V.; De Stefano, G. CFD Analysis of Turbine Cascade Unsteady Aerodynamics Using a Hybrid POD Technique. In Proceedings of the International Conference on Computational Science and Its Applications, Hanoi, Vietnam, 1–4 July 2024; Springer: Berlin/Heidelberg, Germany, 2024; pp. 355–365. [Google Scholar]
- Zhang, C.; Lin, Z.; Dong, T. Numerical Study on the Interaction Characterization of Rotating Detonation Wave and Turbine Rotor Blades. Int. J. Hydrog. Energy 2022, 47, 6898–6910. [Google Scholar] [CrossRef]
- Asli, M.; Stathopoulos, P.; Paschereit, C.O. Aerodynamic Investigation of Guide Vane Configurations Downstream a Rotating Detonation Combustor. J. Eng. Gas Turbines Power 2021, 143, 061011. [Google Scholar] [CrossRef]
- Dolatabadi, A.M.; Eghbali, M.J.; Țurcanu, F.-E.; Mohamadpour, E.; Amini, R. Performance Evaluation of Condensing Flow Behavior Considering Magneto Hydrodynamics Field Effects. Appl. Therm. Eng. 2023, 219, 119472. [Google Scholar] [CrossRef]
- Dolatabadi, A.M.; Pour, M.S.; Ajarostaghi, S.S.M.; Poncet, S.; Hulme-Smith, C. Last Stage Stator Blade Profile Improvement for a Steam Turbine under a Non-Equilibrium Condensation Condition: A CFD and Cost-Saving Approach. Alex. Eng. J. 2023, 73, 27–46. [Google Scholar] [CrossRef]
- Luo, Z.; Huang, H.; Tan, H.; Liang, G.; Lv, J.; Wu, Y.; Li, L. On the Self-Similarity in an Annular Isolator under Rotating Feedback Pressure Perturbations. Aerospace 2023, 10, 188. [Google Scholar] [CrossRef]
- Li, Q.; Wu, Y.; Xia, Y.; Li, X.; Weng, C. Numerical Investigation on the Interactions between Rotating Detonation Wave Complex and Planar Turbine Cascade. Energy Sci. Eng. 2023, 11, 410–429. [Google Scholar] [CrossRef]
- Timko, L. Energy Efficient Engine High Pressure Turbine Component Test Performance Report (NASA CR-168289); National Aeronautics and Space Administration: Washington, DC, USA, 1984.
- Bykovskii, F.A.; Zhdan, S.A.; Vedernikov, E.F. Continuous Spin Detonation of Hydrogen-Oxygen Mixtures. 1. Annular Cylindrical Combustors. Combust. Explos. Shock Waves 2008, 44, 150–162. [Google Scholar] [CrossRef]
- Shen, D.; Cheng, M.; Wu, K.; Sheng, Z.; Wang, J. Effects of Supersonic Nozzle Guide Vanes on the Performance and Flow Structures of a Rotating Detonation Combustor. Acta Astronaut. 2022, 193, 90–99. [Google Scholar] [CrossRef]
- Zhou, S.; Ma, Y.; Liu, F.; Hu, N. Effects of a Straight Guide Vane on the Operating Characteristics of Rotating Detonation Combustor. Acta Astronaut. 2023, 203, 135–145. [Google Scholar] [CrossRef]
- Wu, D.; Zhou, R.; Liu, M.; Wang, J. Numerical Investigation of the Stability of Rotating Detonation Engines. Combust. Sci. Technol. 2014, 186, 1699–1715. [Google Scholar] [CrossRef]
- Bykovskii, F.A.; Vedernikov, E.F. Continuous Detonation of a Subsonic Flow of a Propellant. Combust. Explos. Shock Waves 2003, 39, 323–334. [Google Scholar] [CrossRef]
- Berkooz, G.; Holmes, P.; Lumley, J.L. The Proper Orthogonal Decomposition in the Analysis of Turbulent Flows. Annu. Rev. Fluid Mech. 1993, 25, 539–575. [Google Scholar] [CrossRef]
- SUN, A.; NIU, Q.; WANG, X. Spatiotemporal Reduced-Order Model of Supersonic Exhaust Plume Based on POD. J. Aerosp. Power 2024, 39, 279–289. [Google Scholar]
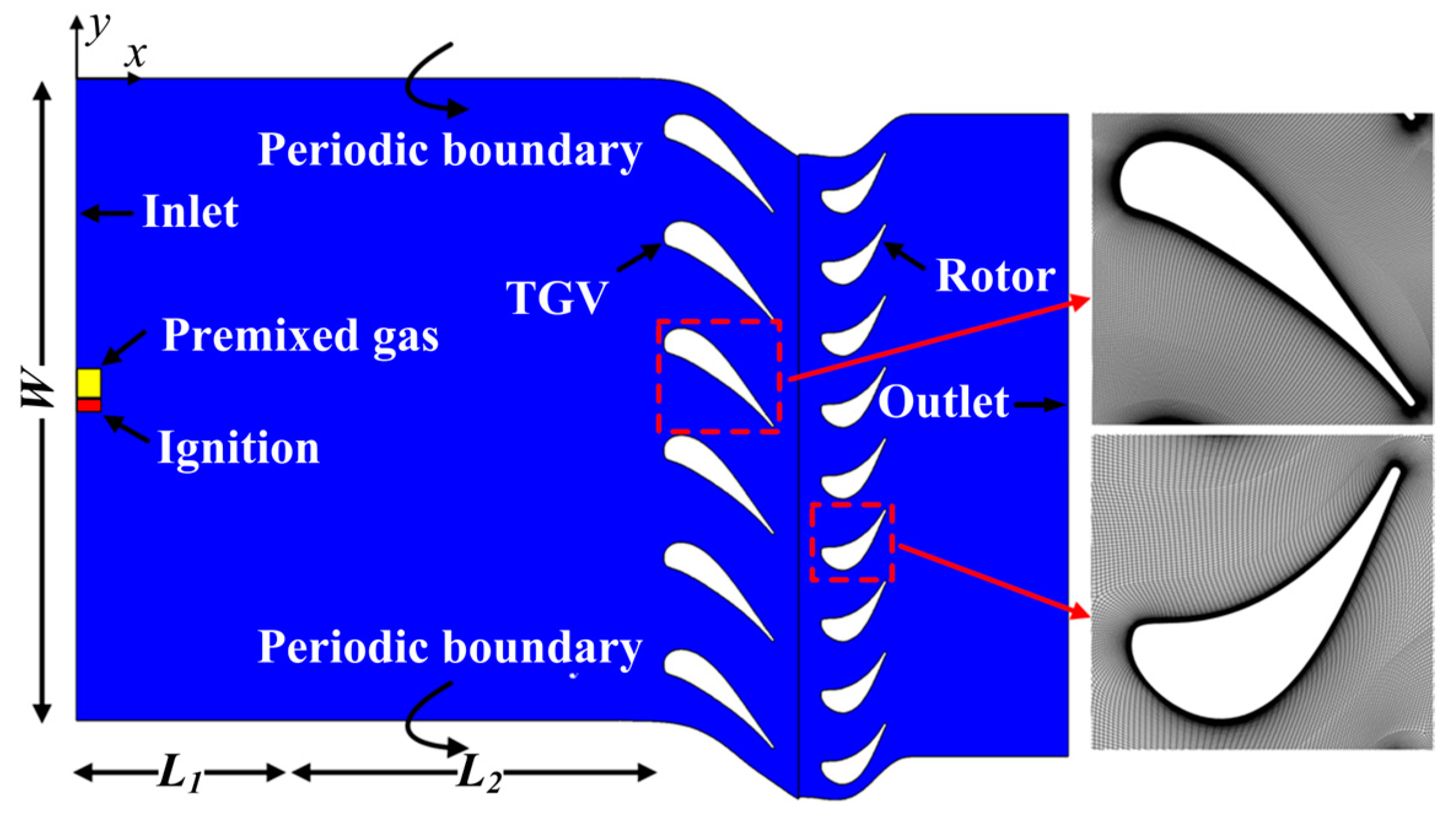
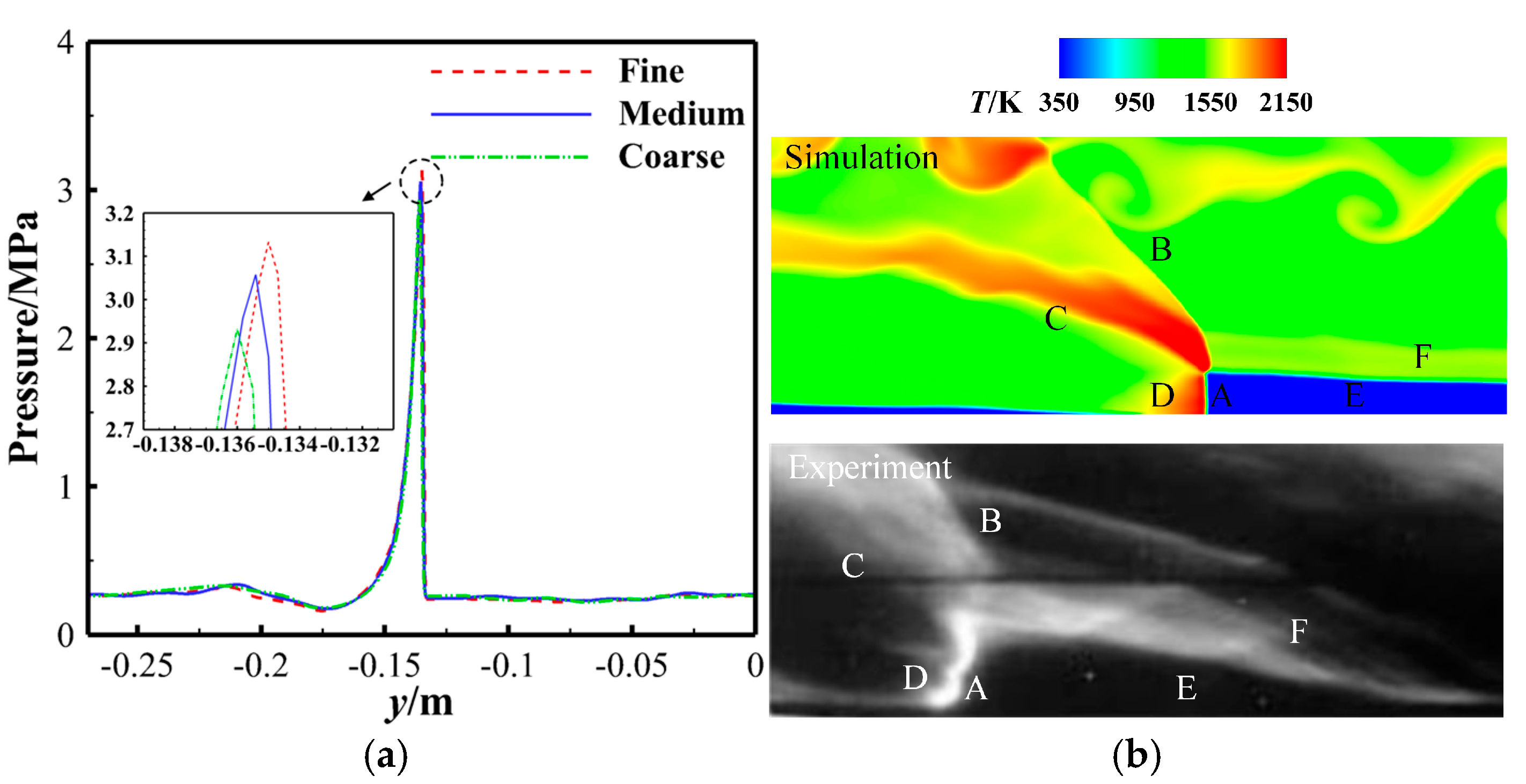
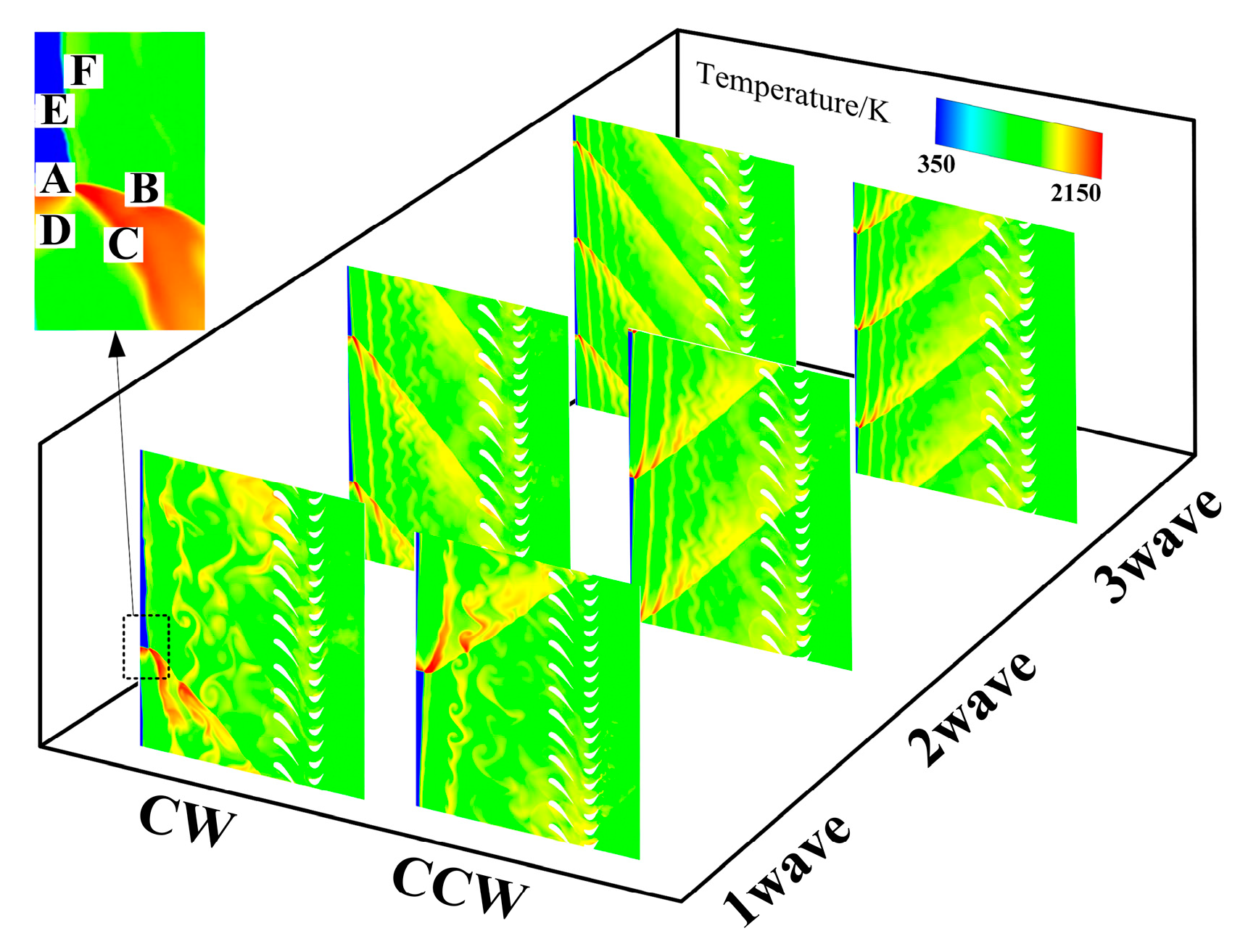
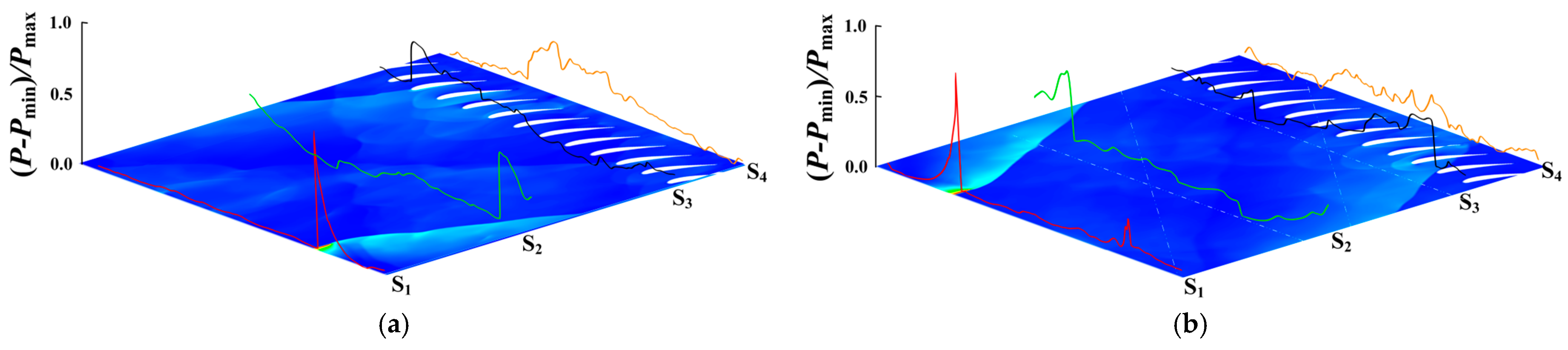

| Detonation Pressure/MPa | Detonation Temperature/K | Detonation Wave Velocity/(m/s) | |
|---|---|---|---|
| Numerical result | 3.15 | 2539 | 1664 |
| CJ theoretical value | 3.26 | 2630 | 1798 |
| Error | 3.37% | 3.46% | 7.45% |
| CW | CCW | |||||
|---|---|---|---|---|---|---|
| 1 Wave | 2 Wave | 3 Wave | 1 Wave | 2 Wave | 3 Wave | |
| Inlet total pressure gain (%) | 26.9 | 25.5 | 23.6 | 42.1 | 28.6 | 24.3 |
| Rotor efficiency | 0.840 | 0.887 | 0.890 | 0.758 | 0.864 | 0.875 |
| Specific work gain (%) | 20.9 | 27.1 | 27.5 | 29.1 | 27.9 | 27.8 |
| Instability (%) | 11.8 | 7.1 | 6.5 | 7.8 | 5.0 | 5.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ling, M.; Zhao, T.; Luo, W.; Zhu, J.; You, Y. Analysis of Aerodynamic Characteristics of Rotating Detonation Turbine Based on Proper Orthogonal Decomposition Method. Aerospace 2025, 12, 406. https://doi.org/10.3390/aerospace12050406
Ling M, Zhao T, Luo W, Zhu J, You Y. Analysis of Aerodynamic Characteristics of Rotating Detonation Turbine Based on Proper Orthogonal Decomposition Method. Aerospace. 2025; 12(5):406. https://doi.org/10.3390/aerospace12050406
Chicago/Turabian StyleLing, Meiting, Ting Zhao, Wenguo Luo, Jianfeng Zhu, and Yancheng You. 2025. "Analysis of Aerodynamic Characteristics of Rotating Detonation Turbine Based on Proper Orthogonal Decomposition Method" Aerospace 12, no. 5: 406. https://doi.org/10.3390/aerospace12050406
APA StyleLing, M., Zhao, T., Luo, W., Zhu, J., & You, Y. (2025). Analysis of Aerodynamic Characteristics of Rotating Detonation Turbine Based on Proper Orthogonal Decomposition Method. Aerospace, 12(5), 406. https://doi.org/10.3390/aerospace12050406







