Mechanics of Space Debris Removal: A Review
Abstract
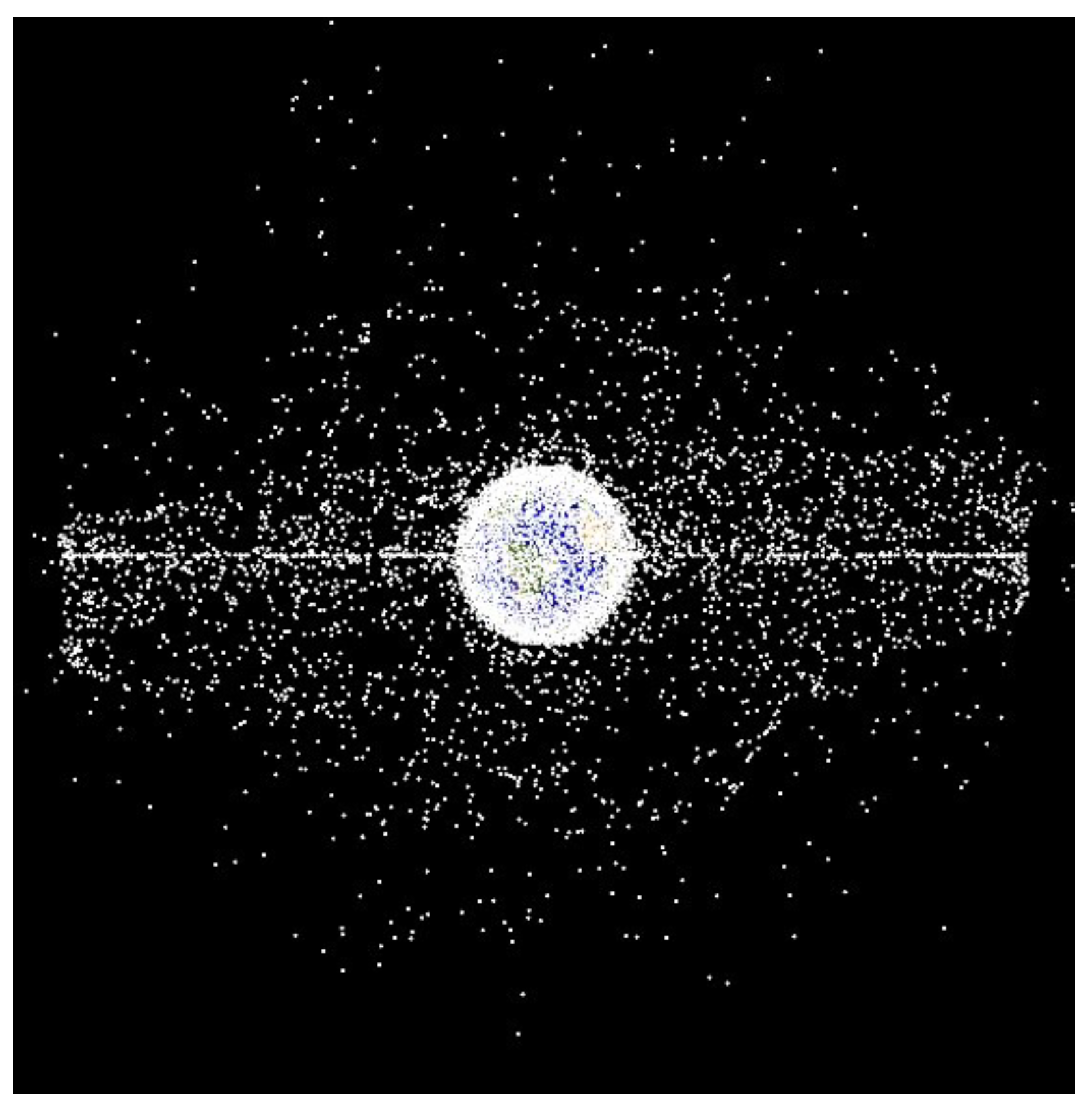
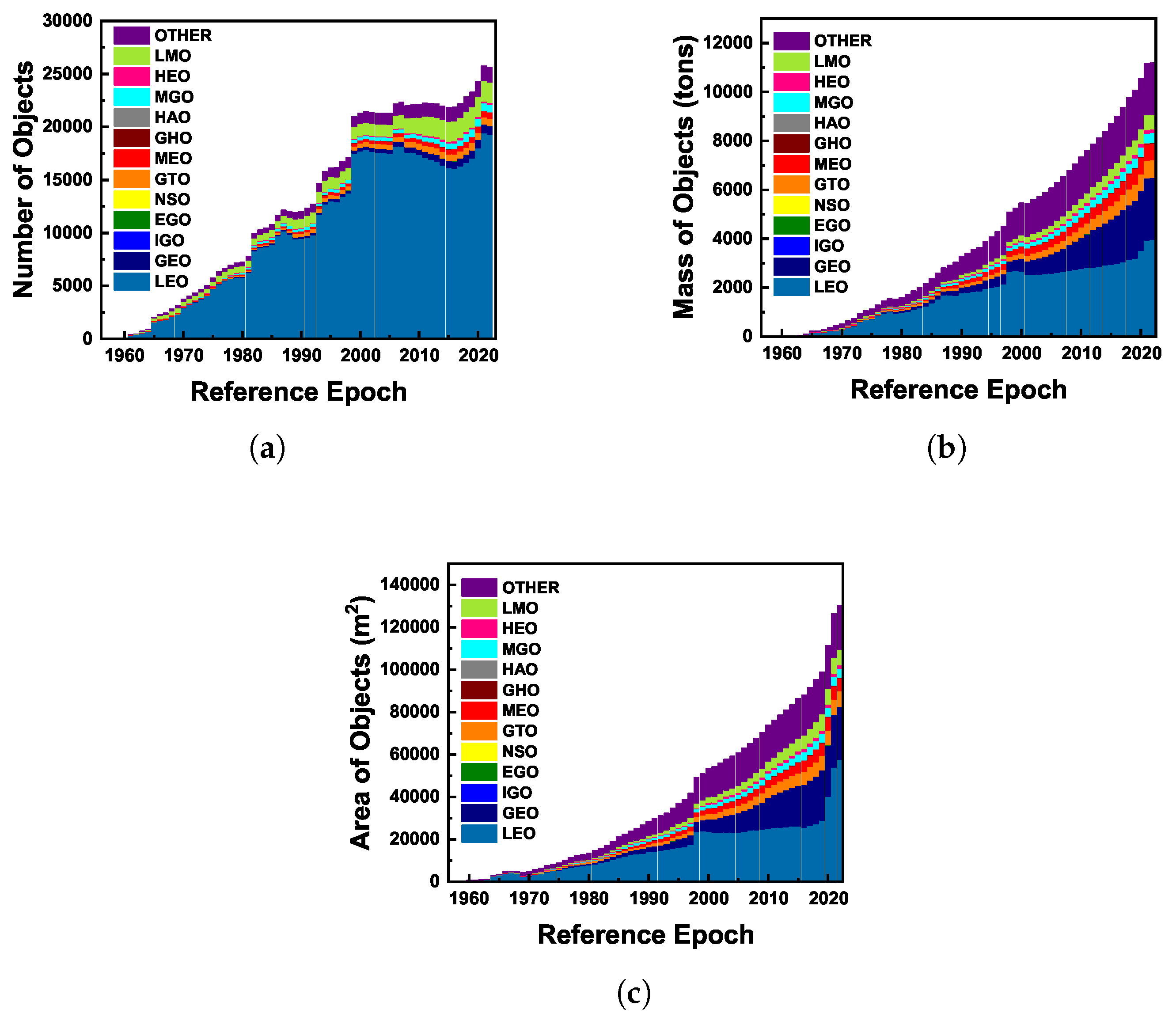
1. Introduction
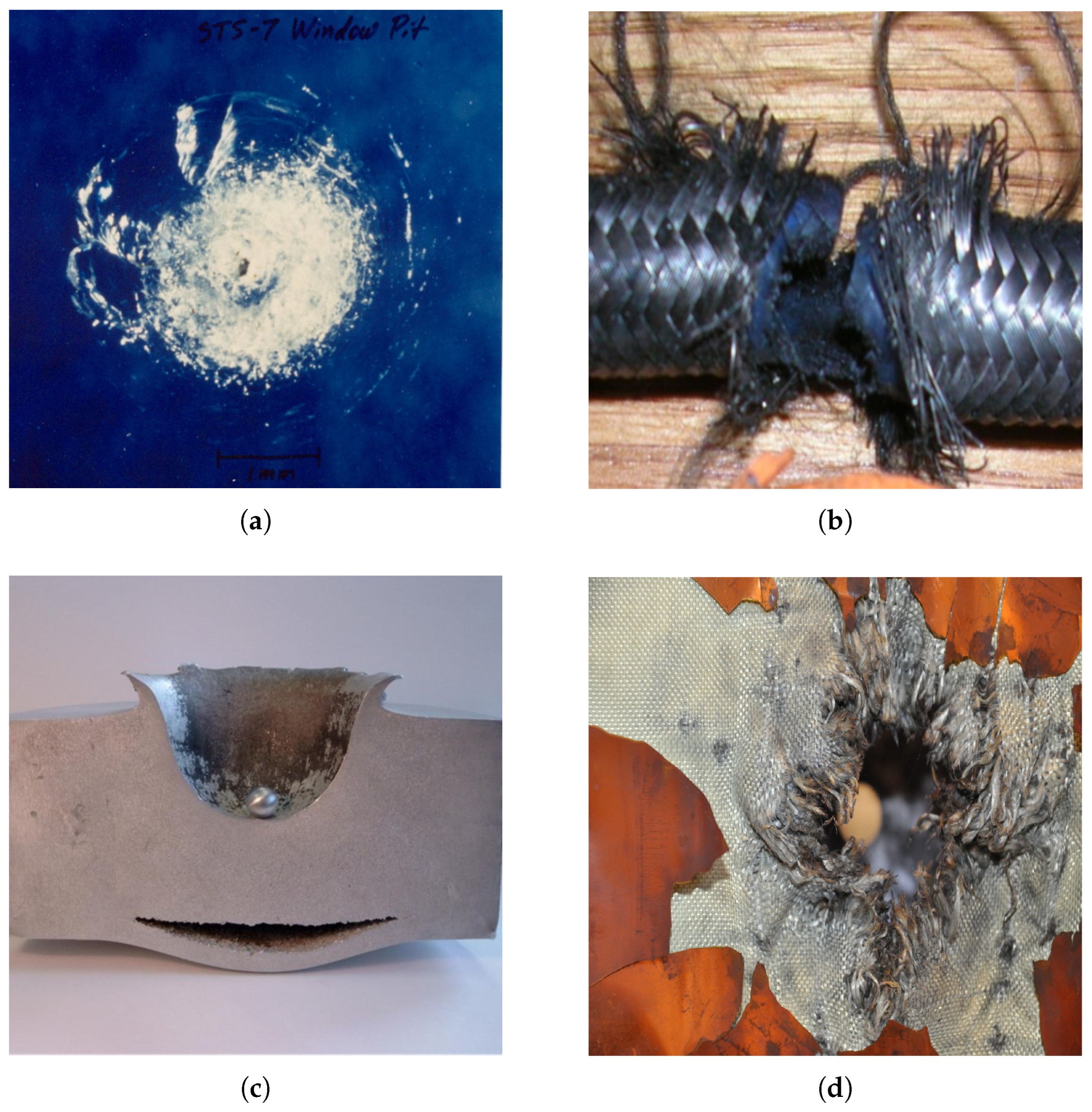
2. Current Status of Debris and Its Detection and Removal Methods
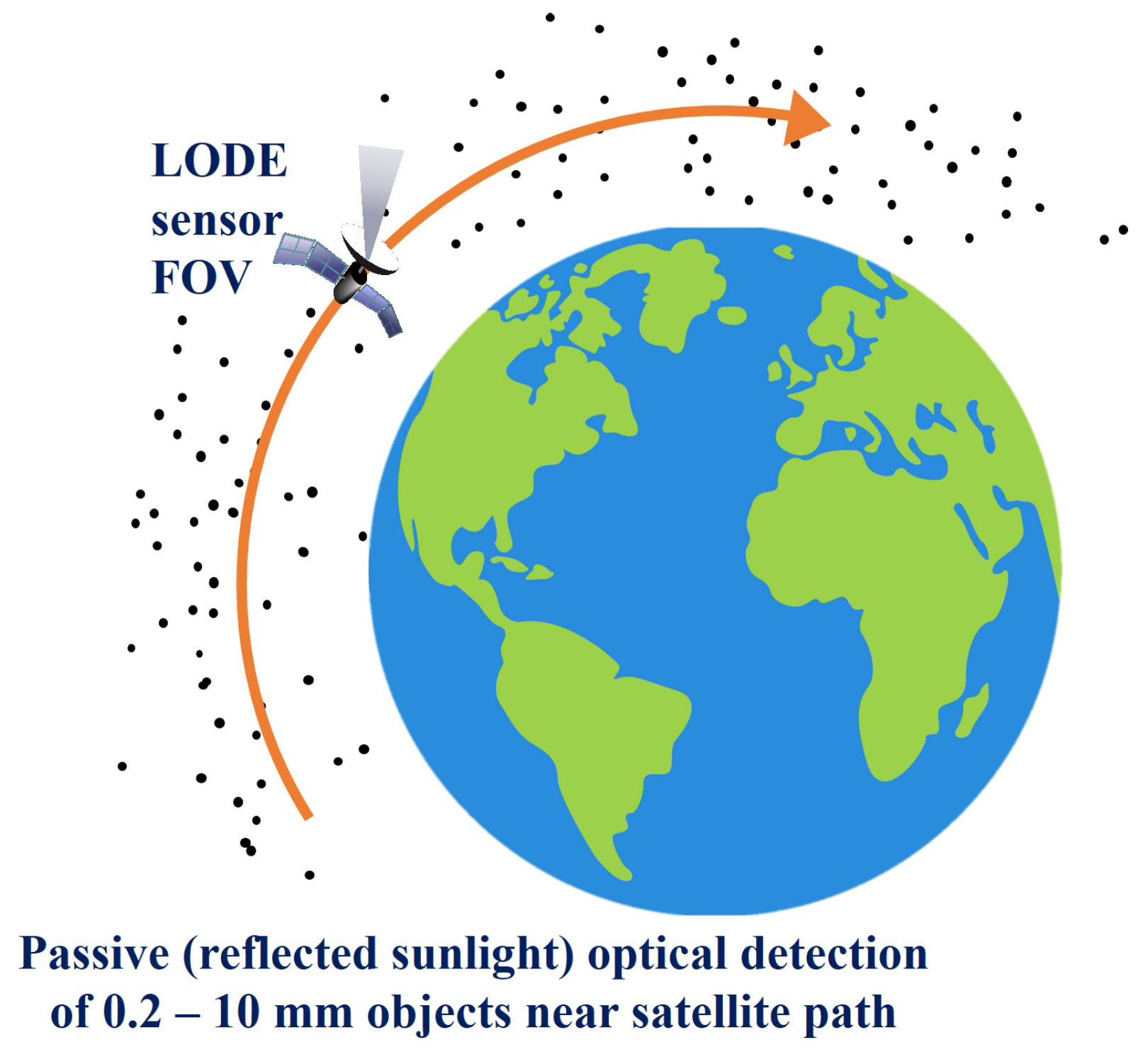
2.1. Optical Telescopes
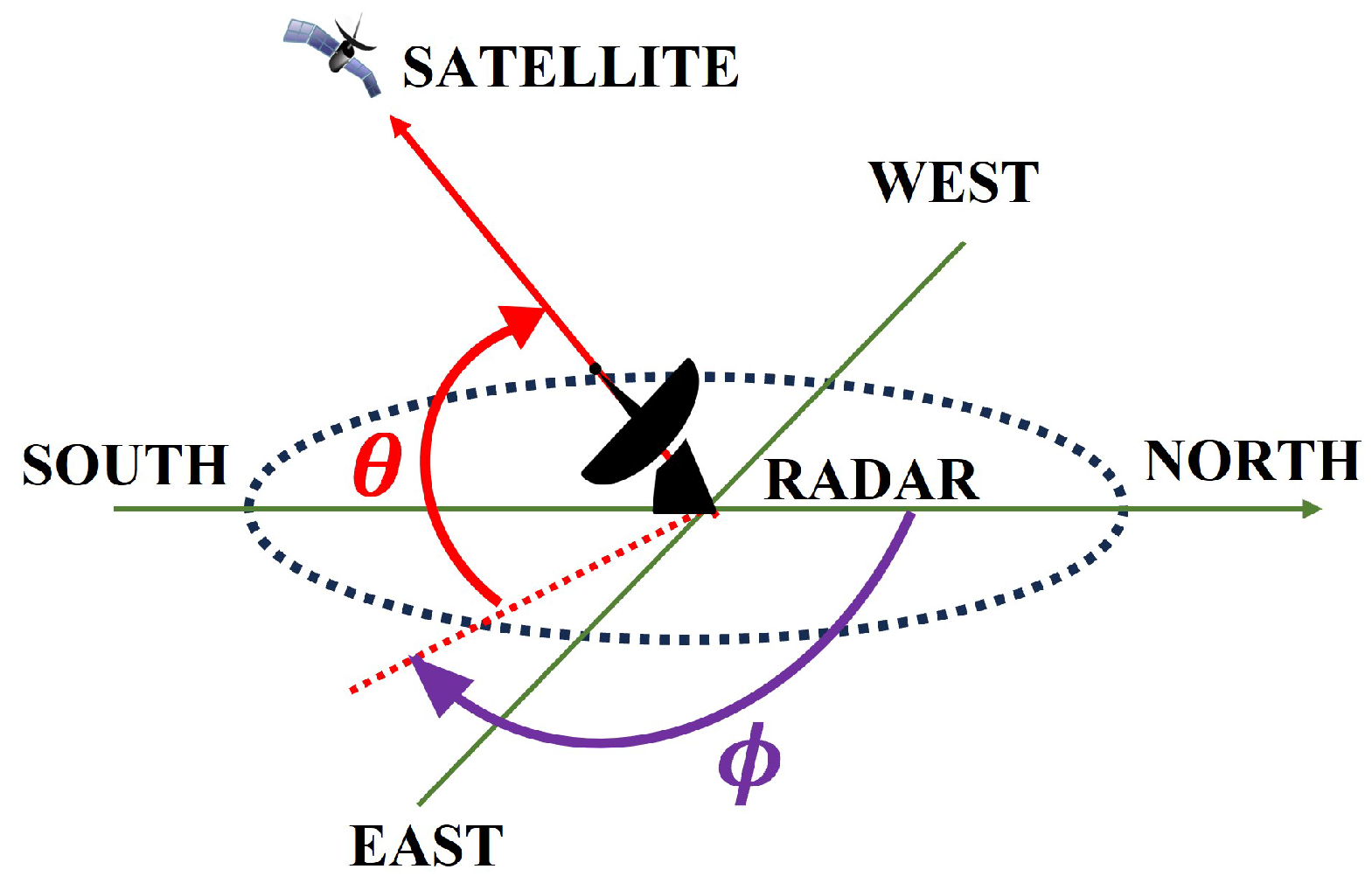
2.2. Radar
- In Equation (1), represents the radar cross-section, r is used as a representative distance between the radar and the target for RCS calculation and refers to the power of the radar signal scattered back to the radar, while represents the power of the radar signal incident upon the target. The RCS value is affected by variables such as frequency, polarization, target shape and composition. For simple targets, the RCS can be estimated using analytical approximations found in traditional radar references. However, for more complex scenarios, numerical solvers are necessary to accurately calculate the RCS [55,56,57,58].
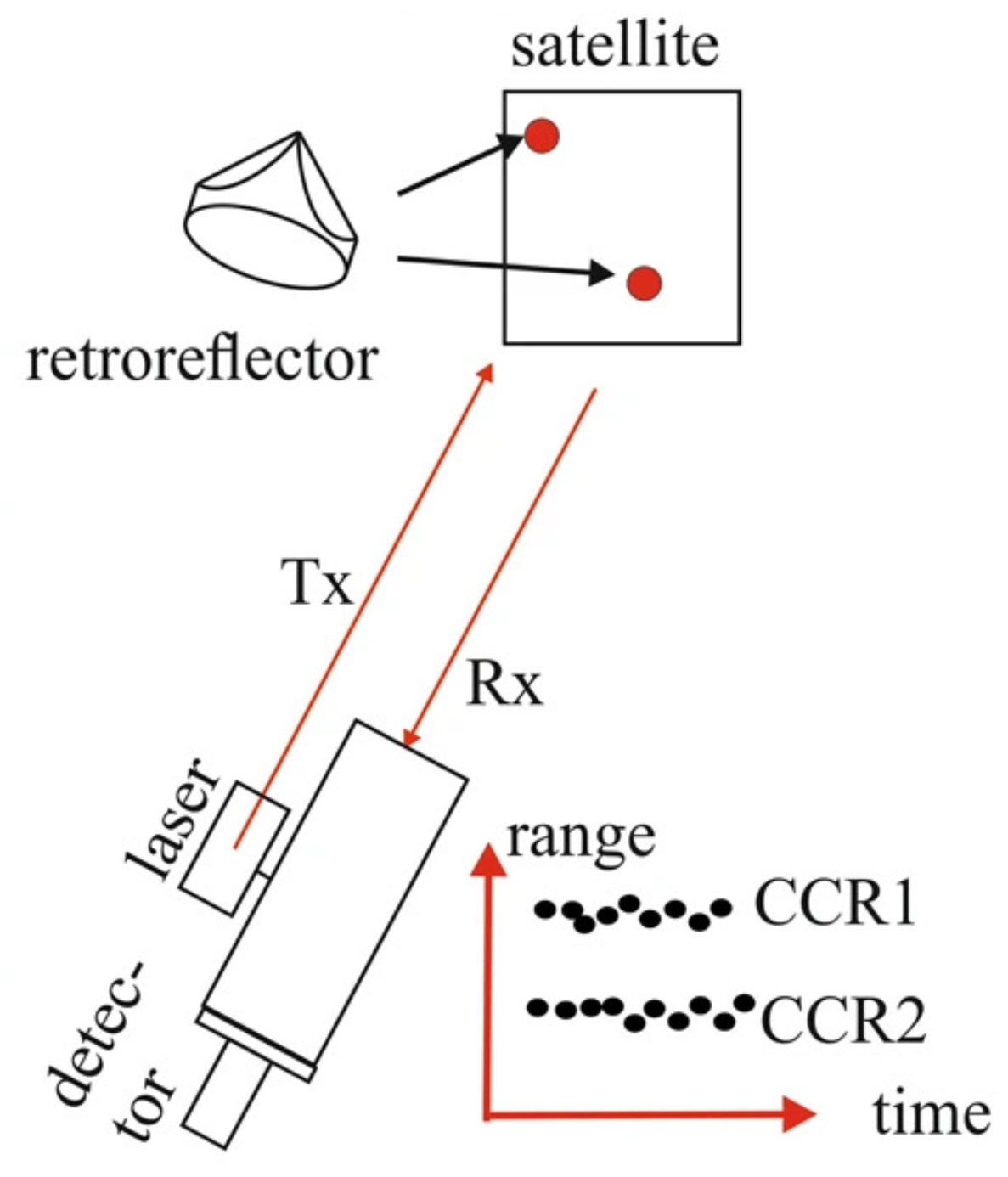
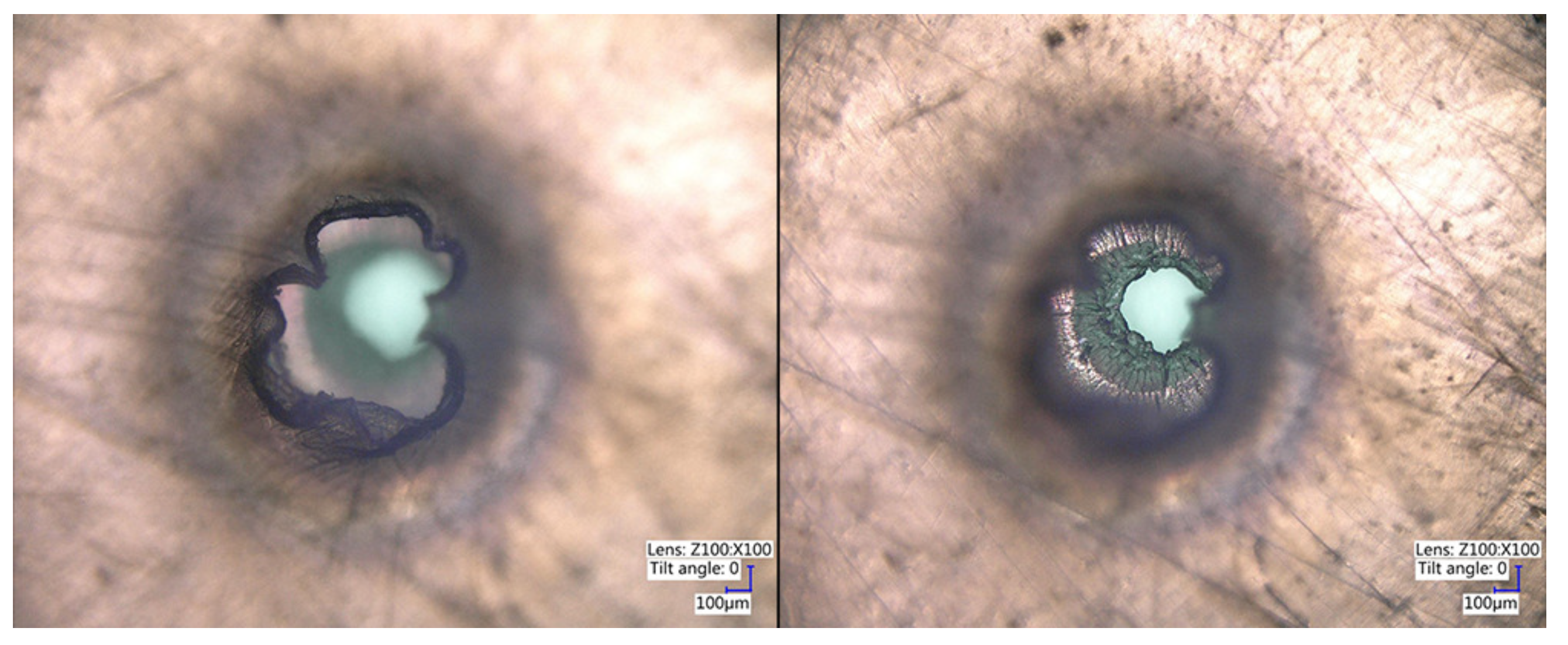
2.3. Debris Laser Ranging (DLR)
2.4. In Situ Sensors
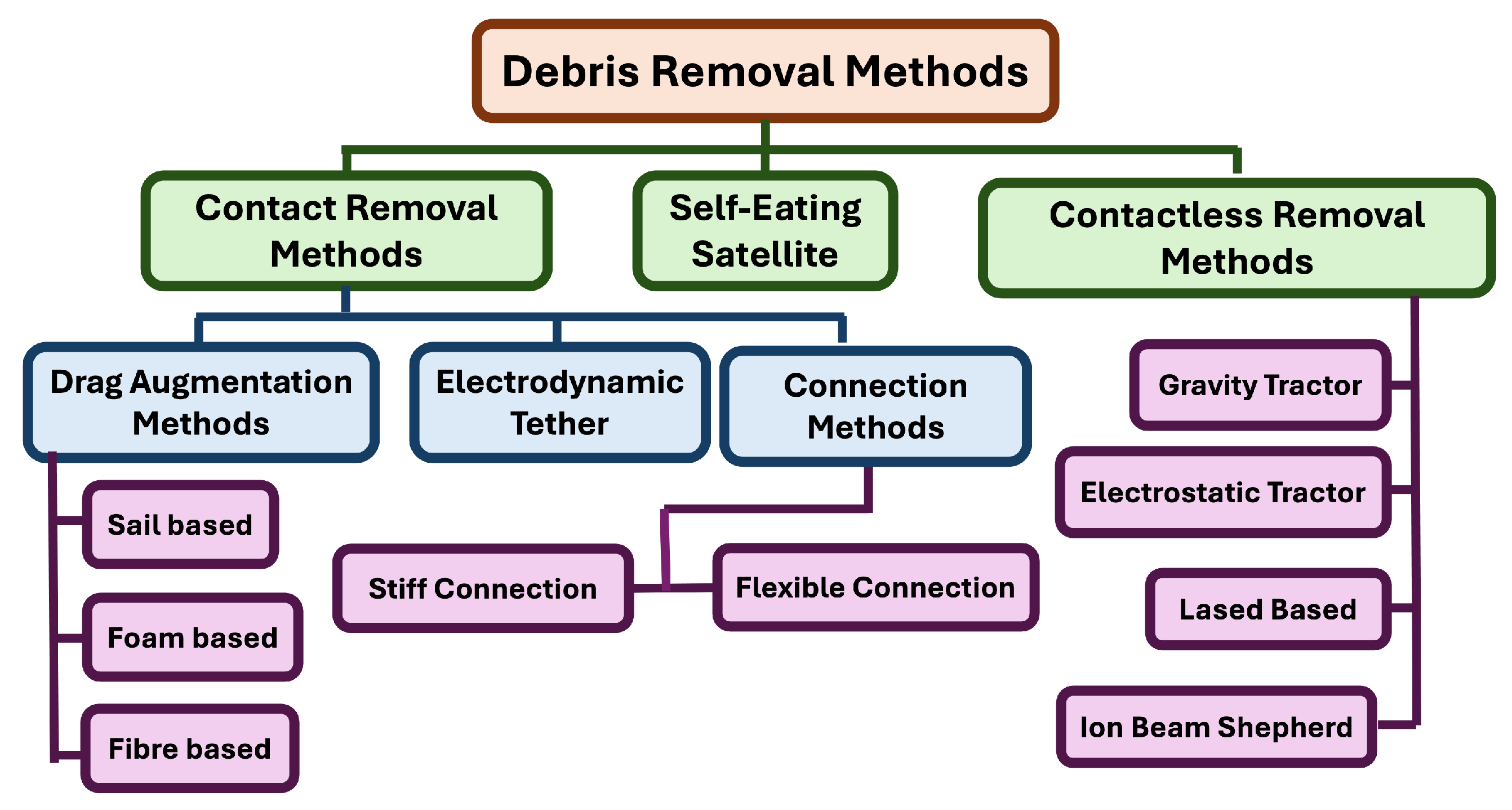
2.5. Contact-Less Debris Removal Methods
2.5.1. Gravity Tractor
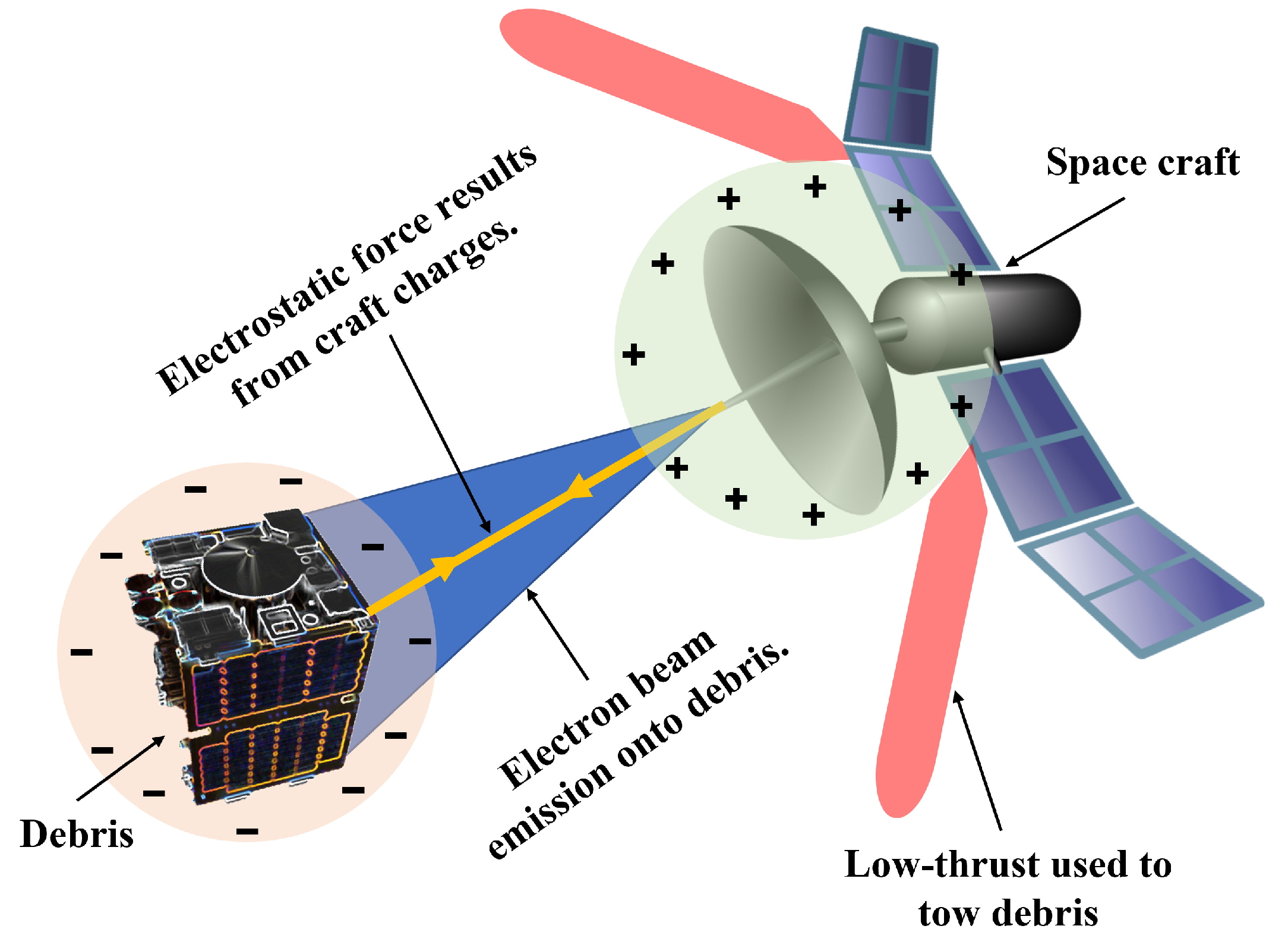
2.5.2. Electrostatic Tractor
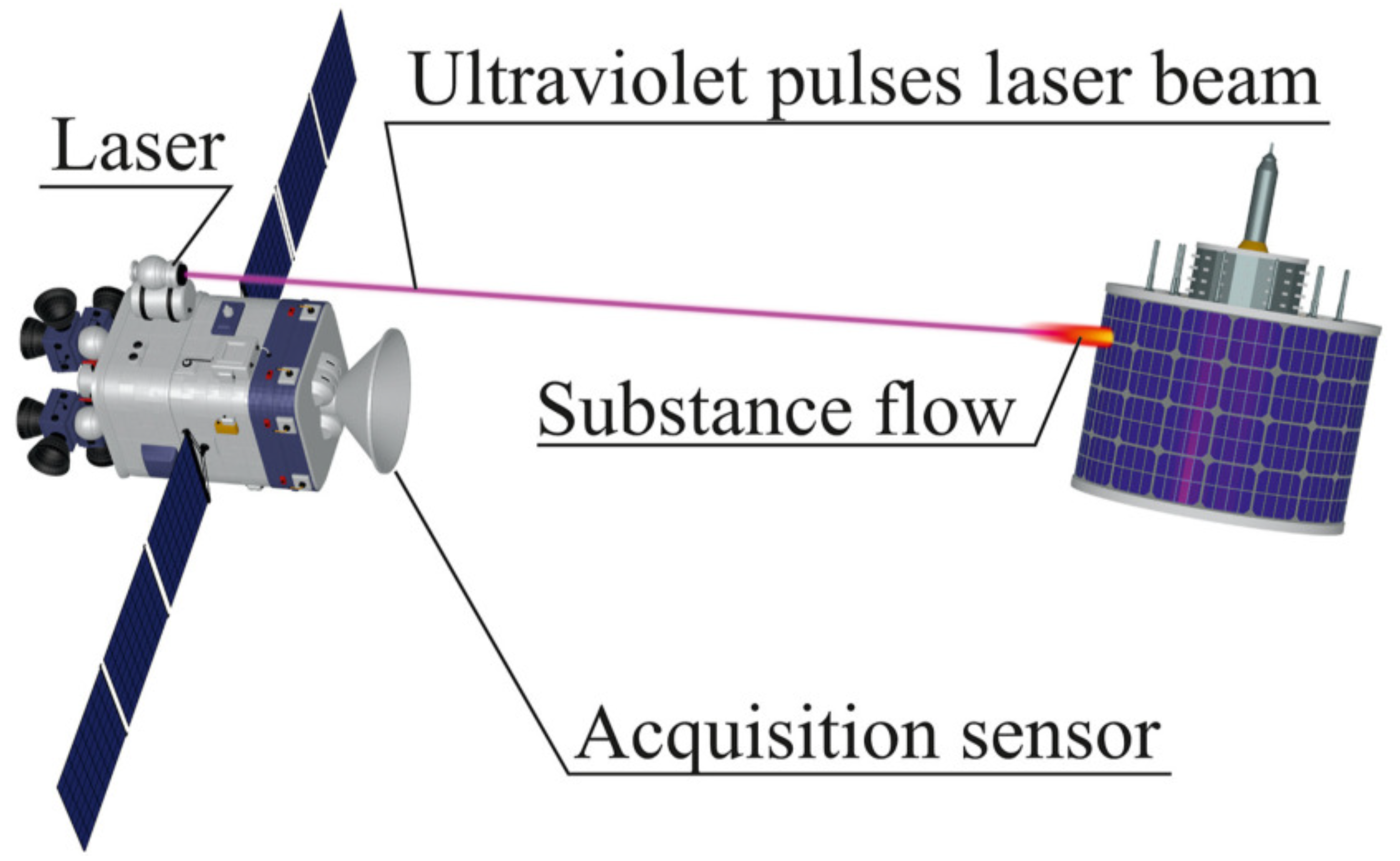
2.5.3. Laser-Based Method
Space-Based Lasers
Ground-Based Lasers
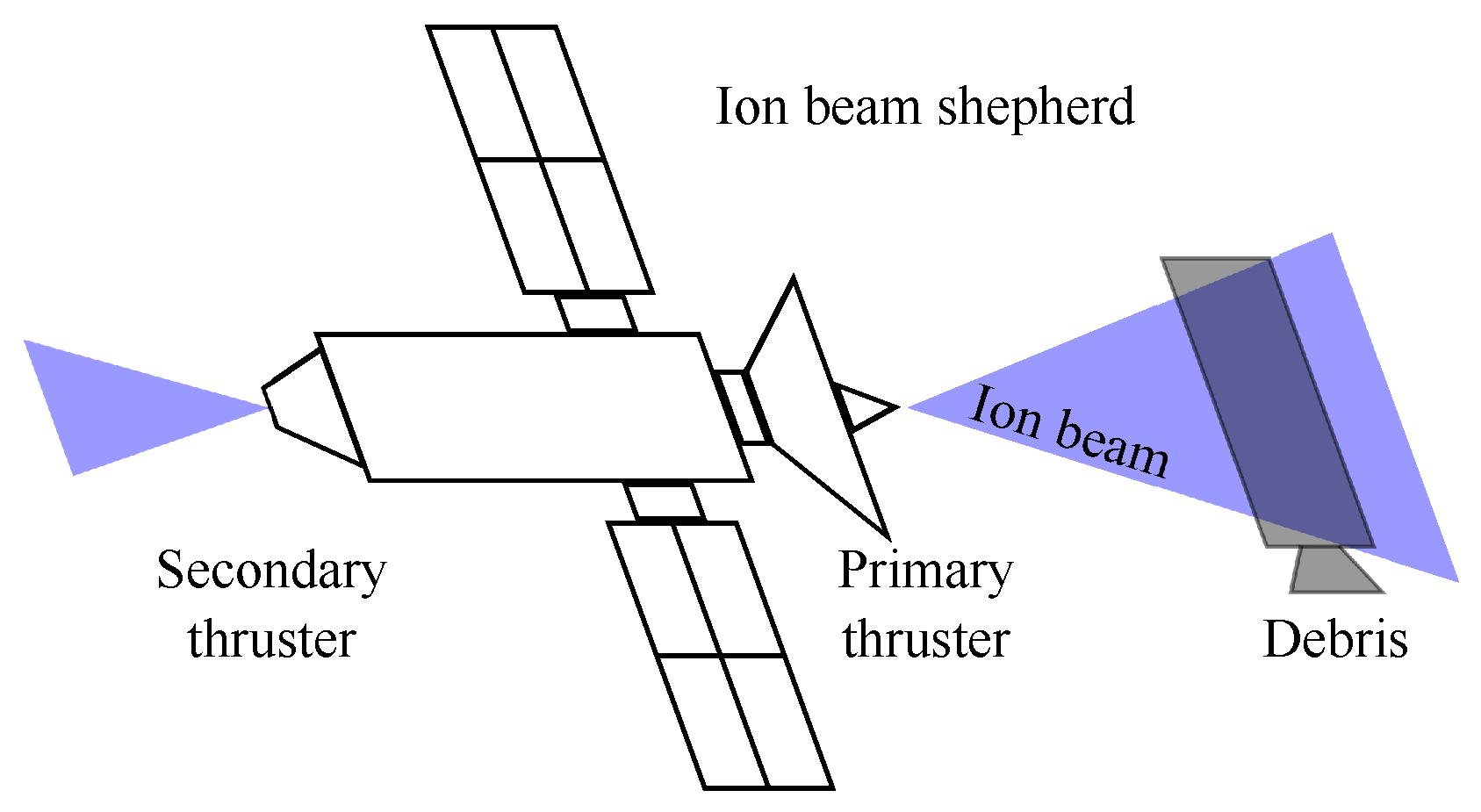
2.5.4. Ion Beam Shepherd (IBS)
2.6. Contact-Based Debris Removal Methods
2.6.1. Drag Augmentation Method
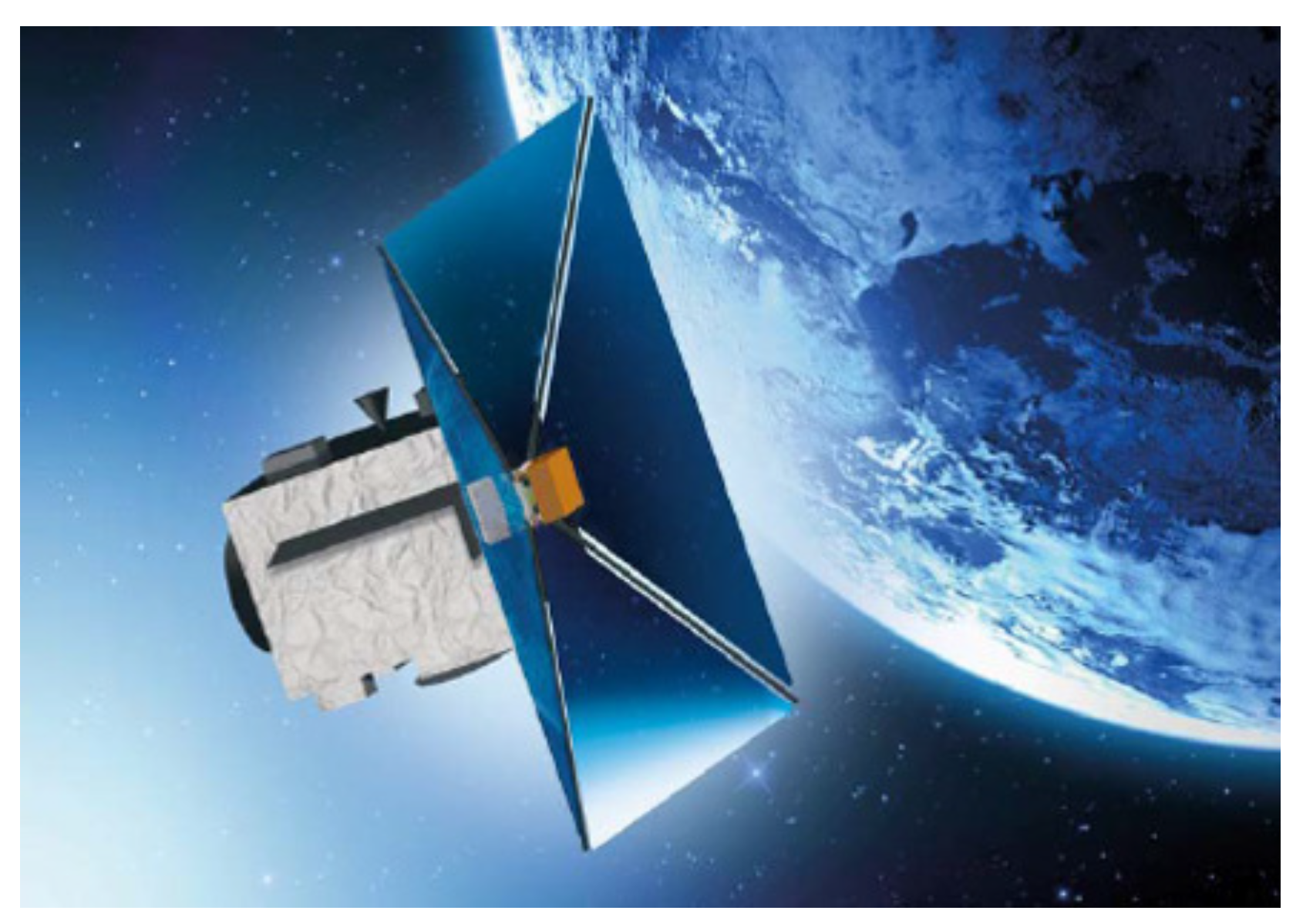
Sail-Based Method
Foam-Based Method
Fiber-Based Method
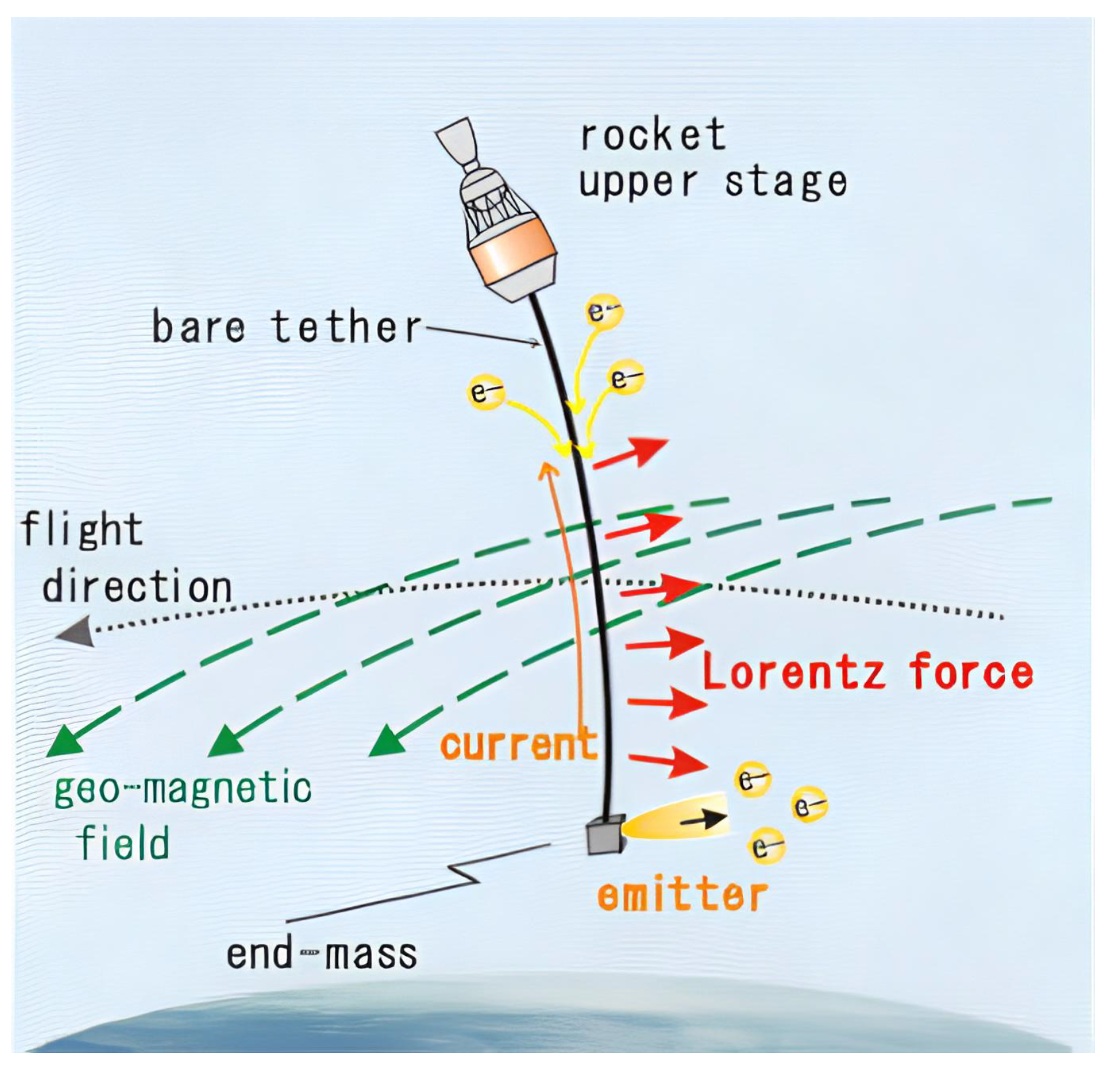
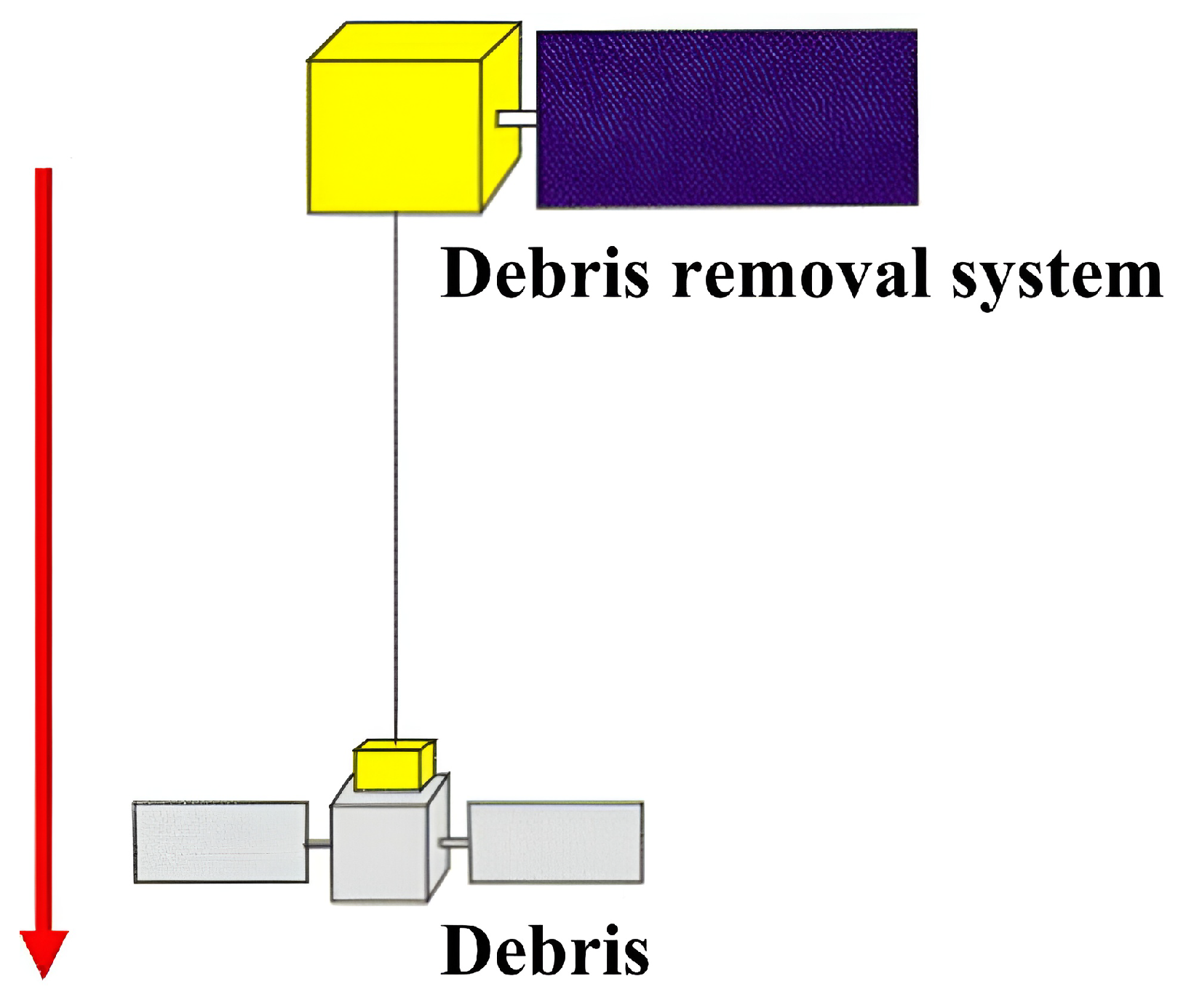
2.6.2. Electrodynamic Tethers (EDTs)
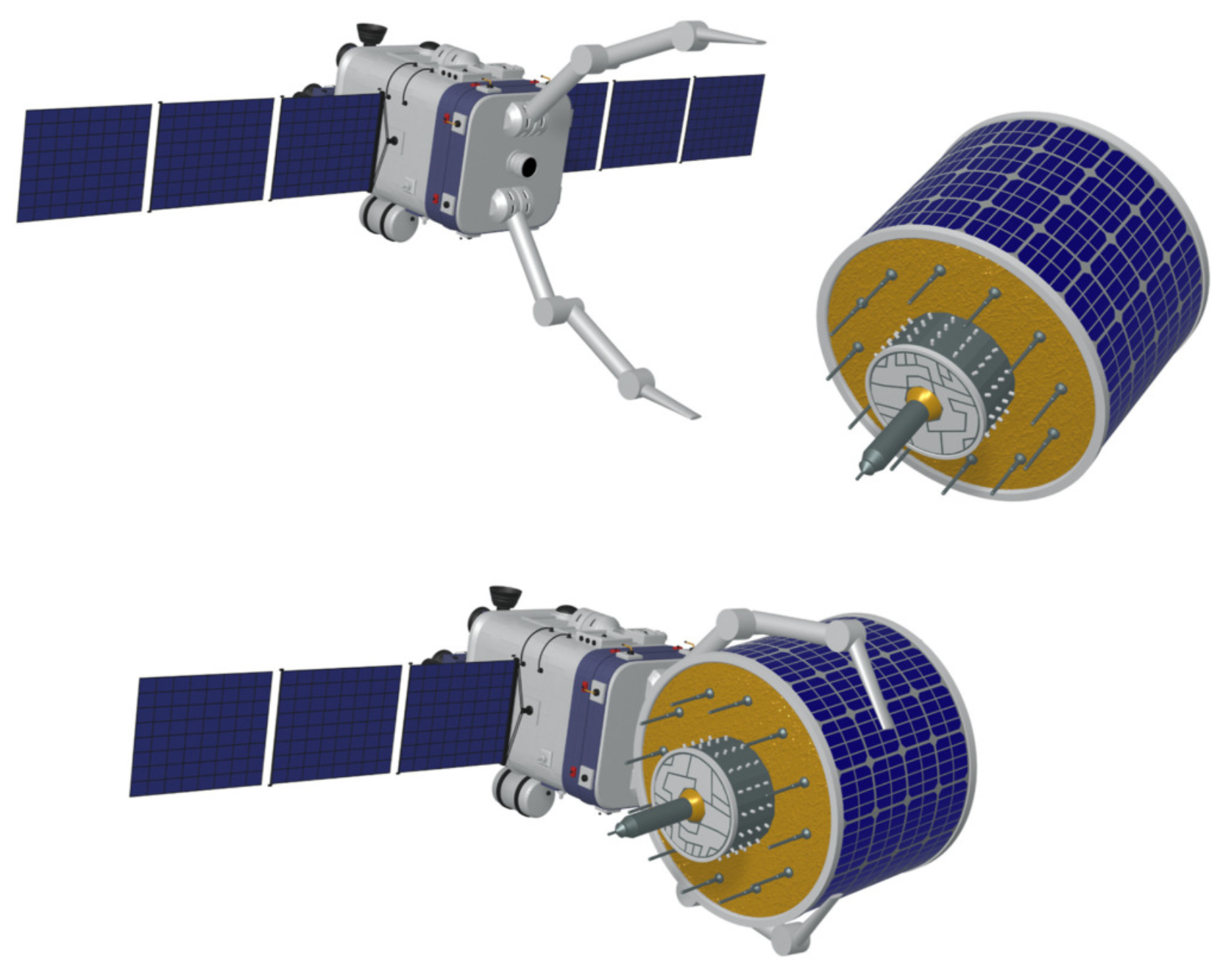
2.6.3. Stiff Connection-Based Methods
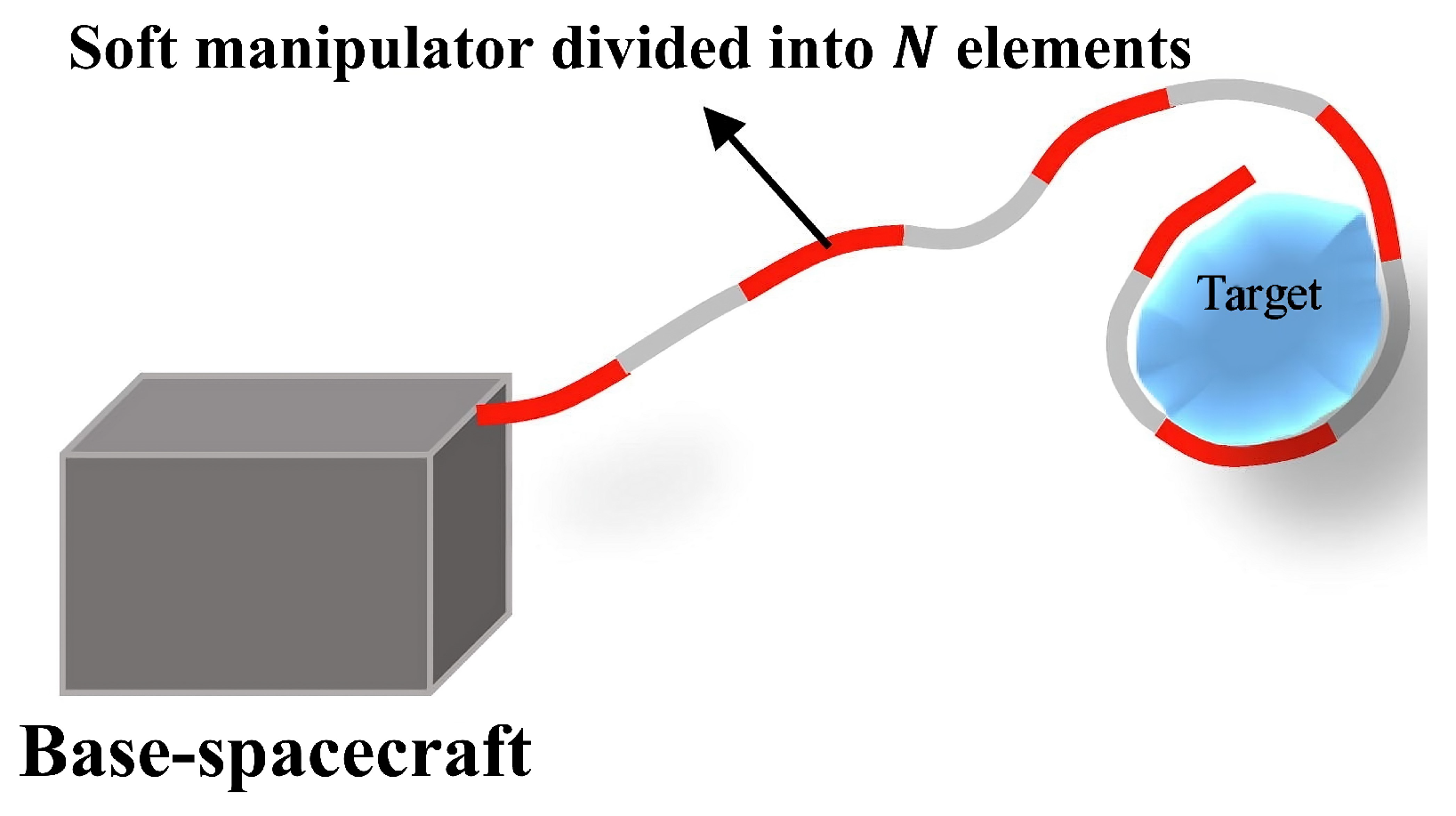
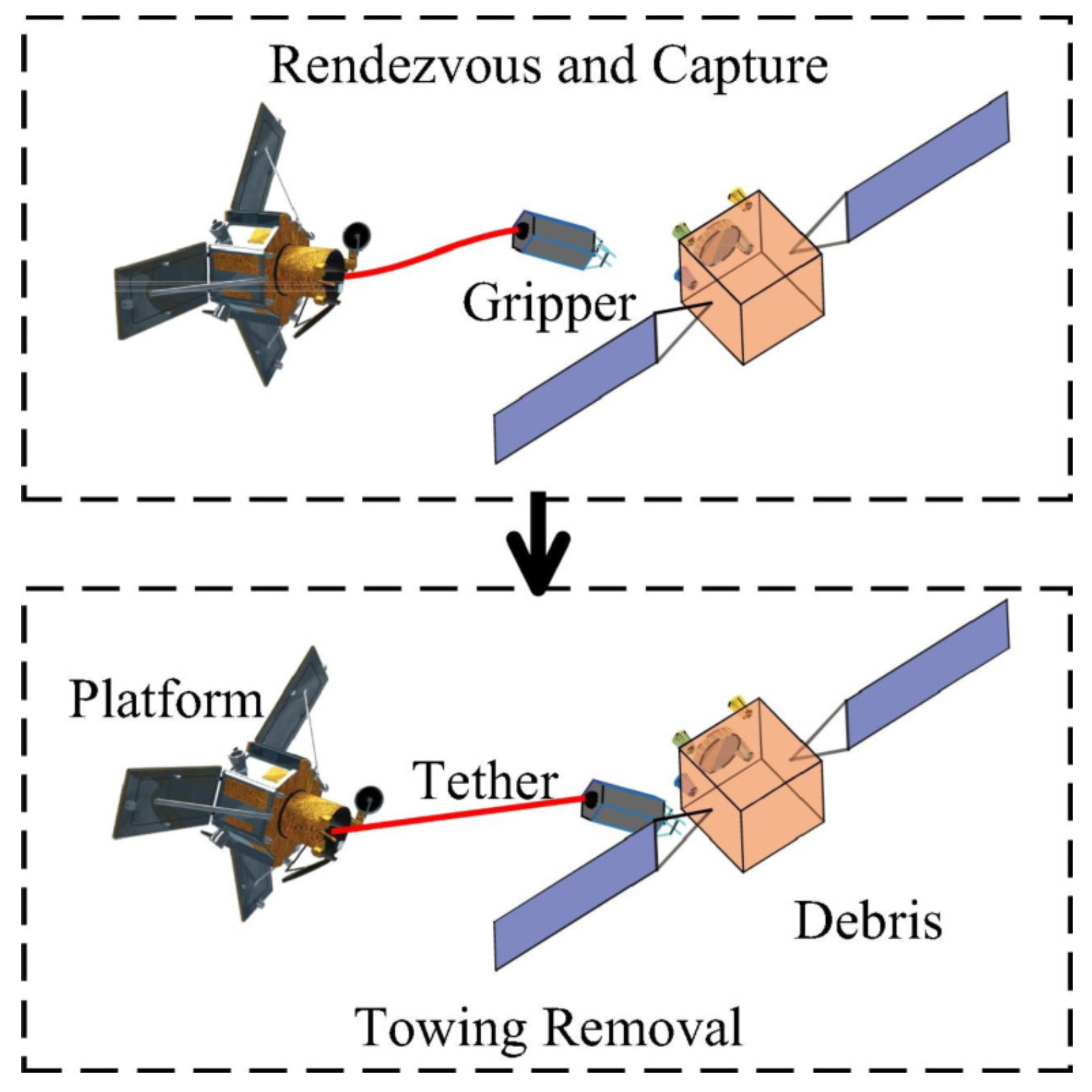
2.6.4. Flexible Connection Capturing Methods
2.7. Self-Eating Satellite
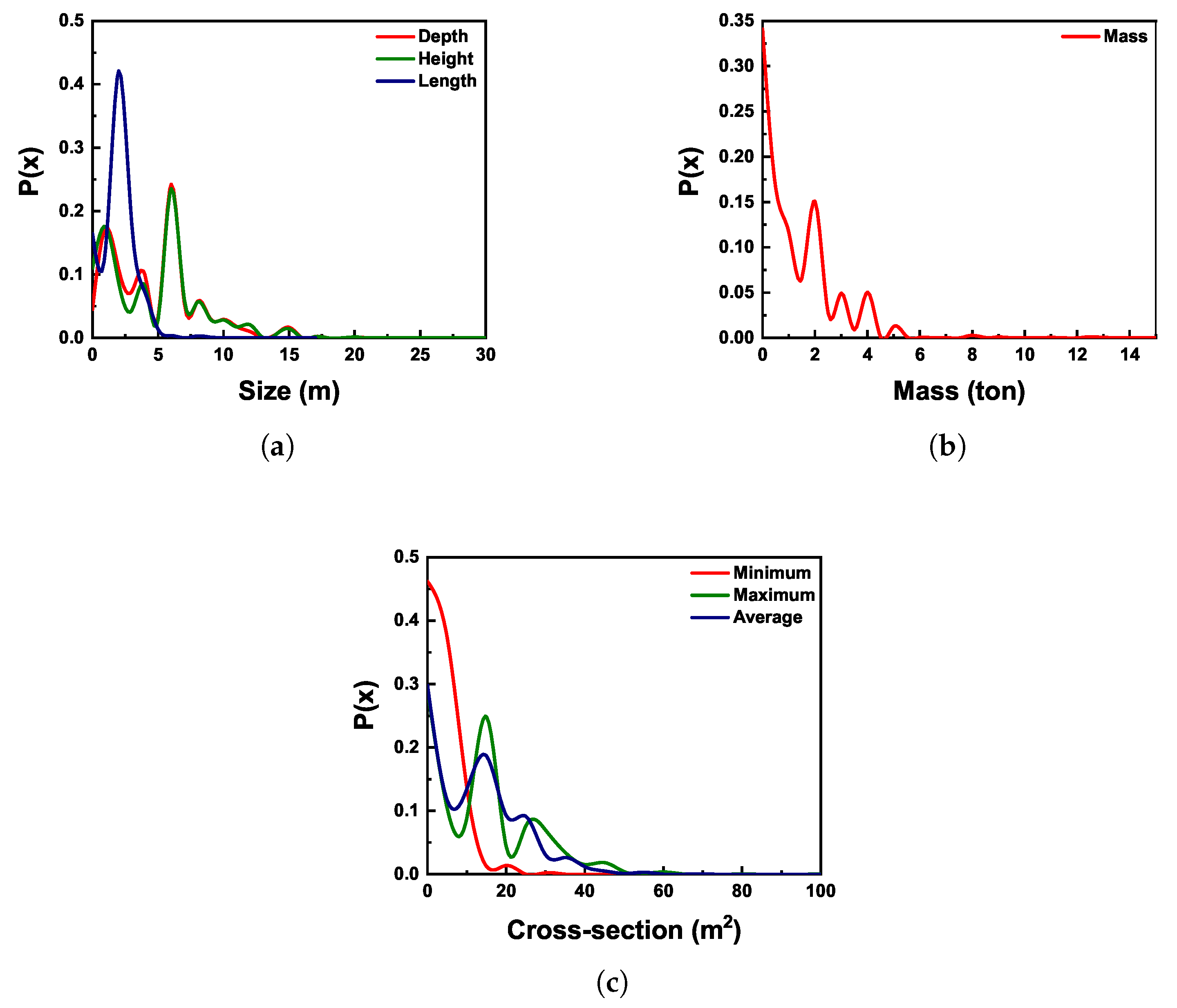
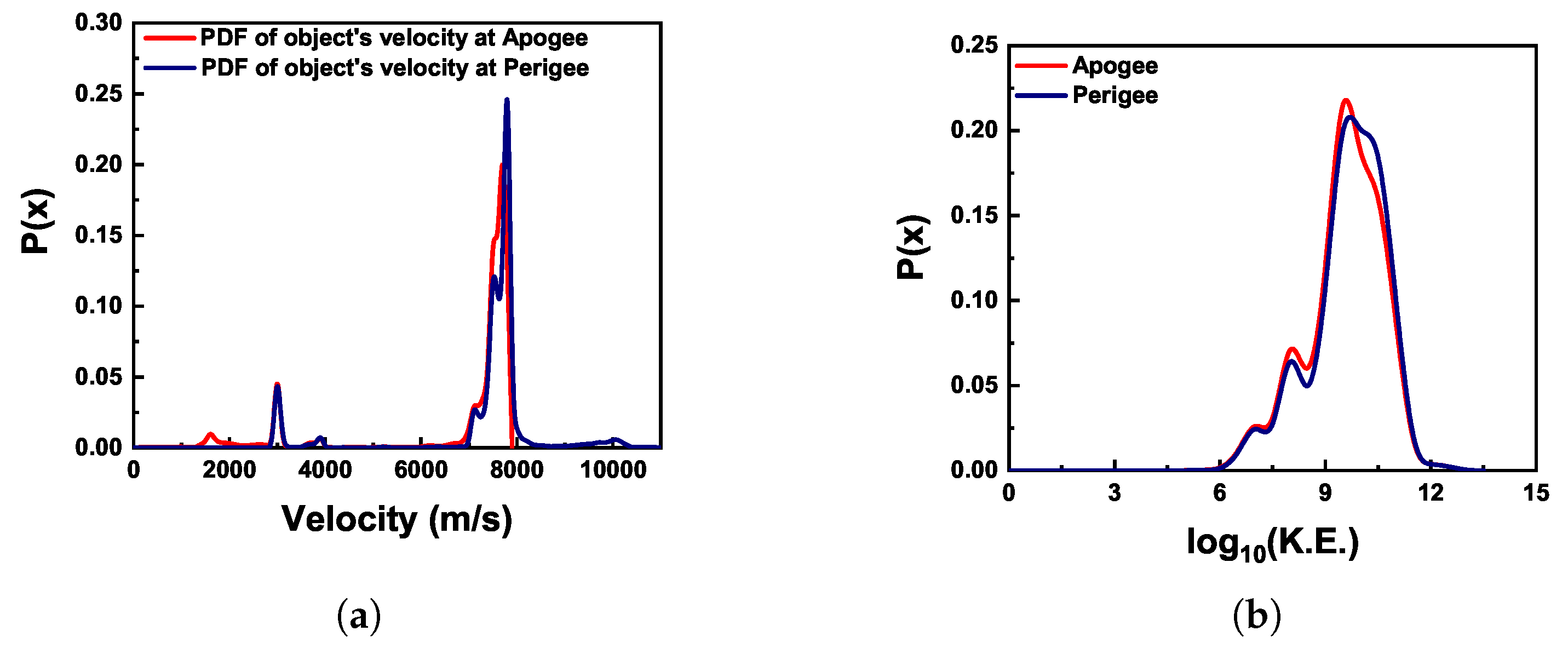
3. Design Parameters for Debris Removal Systems
4. Mitigation Strategies
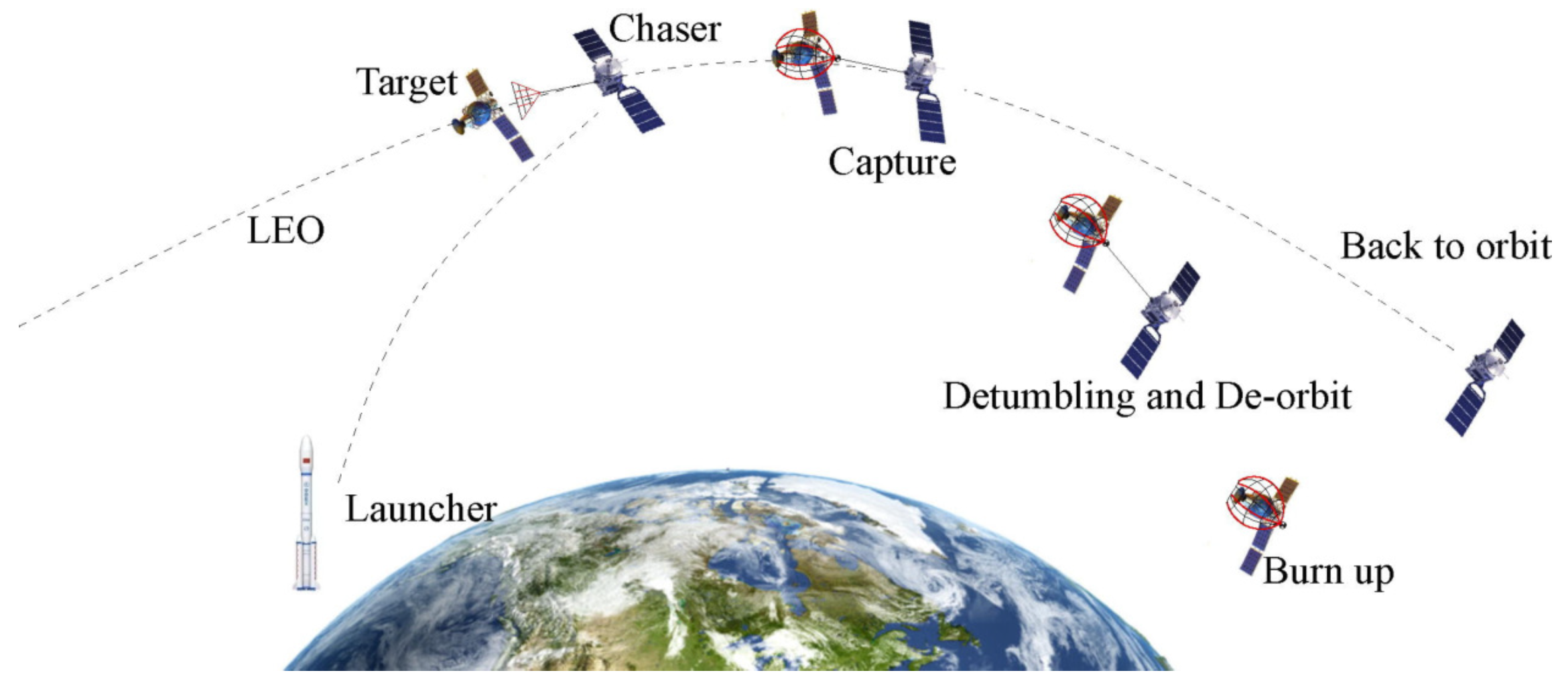
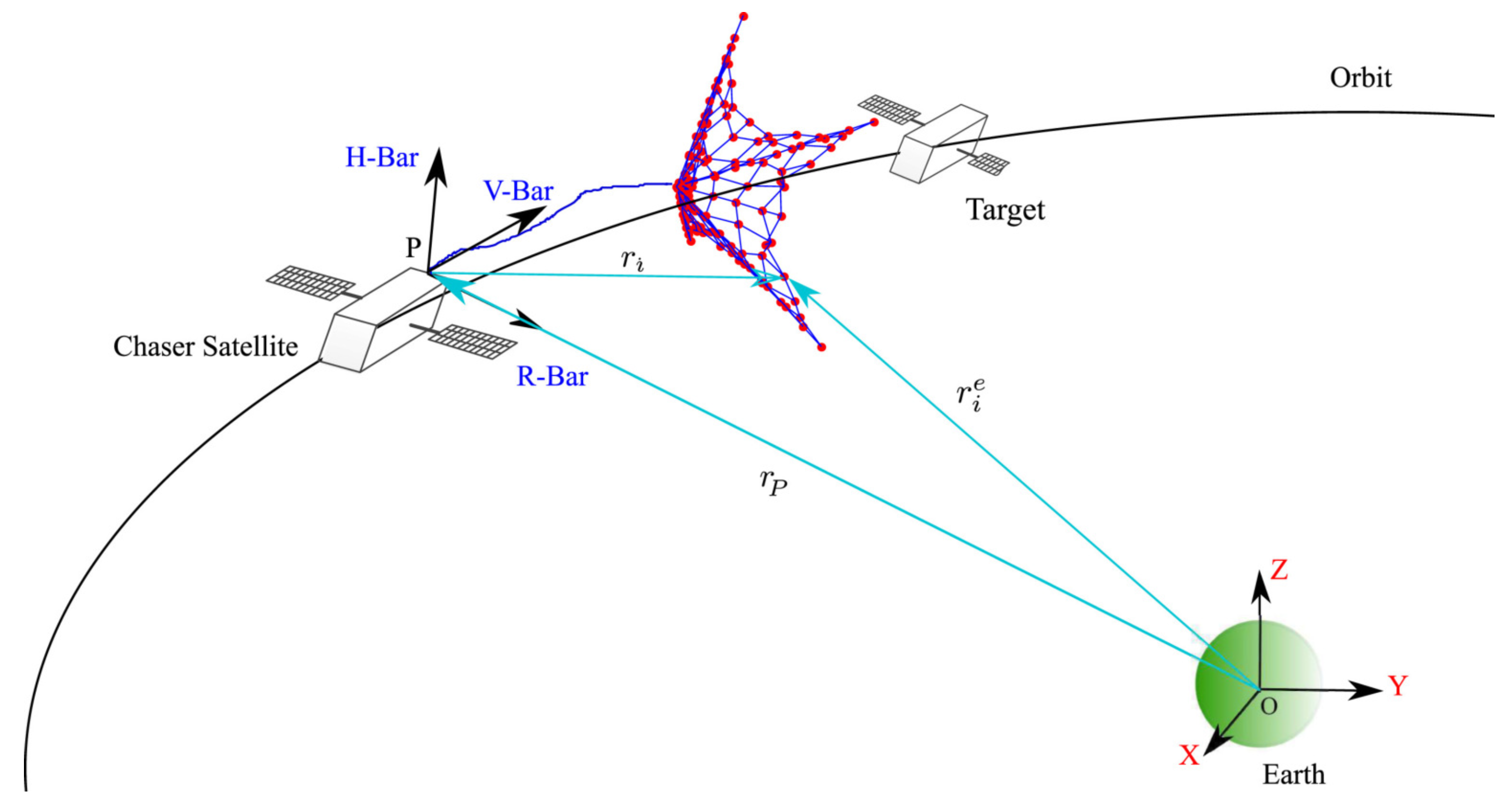
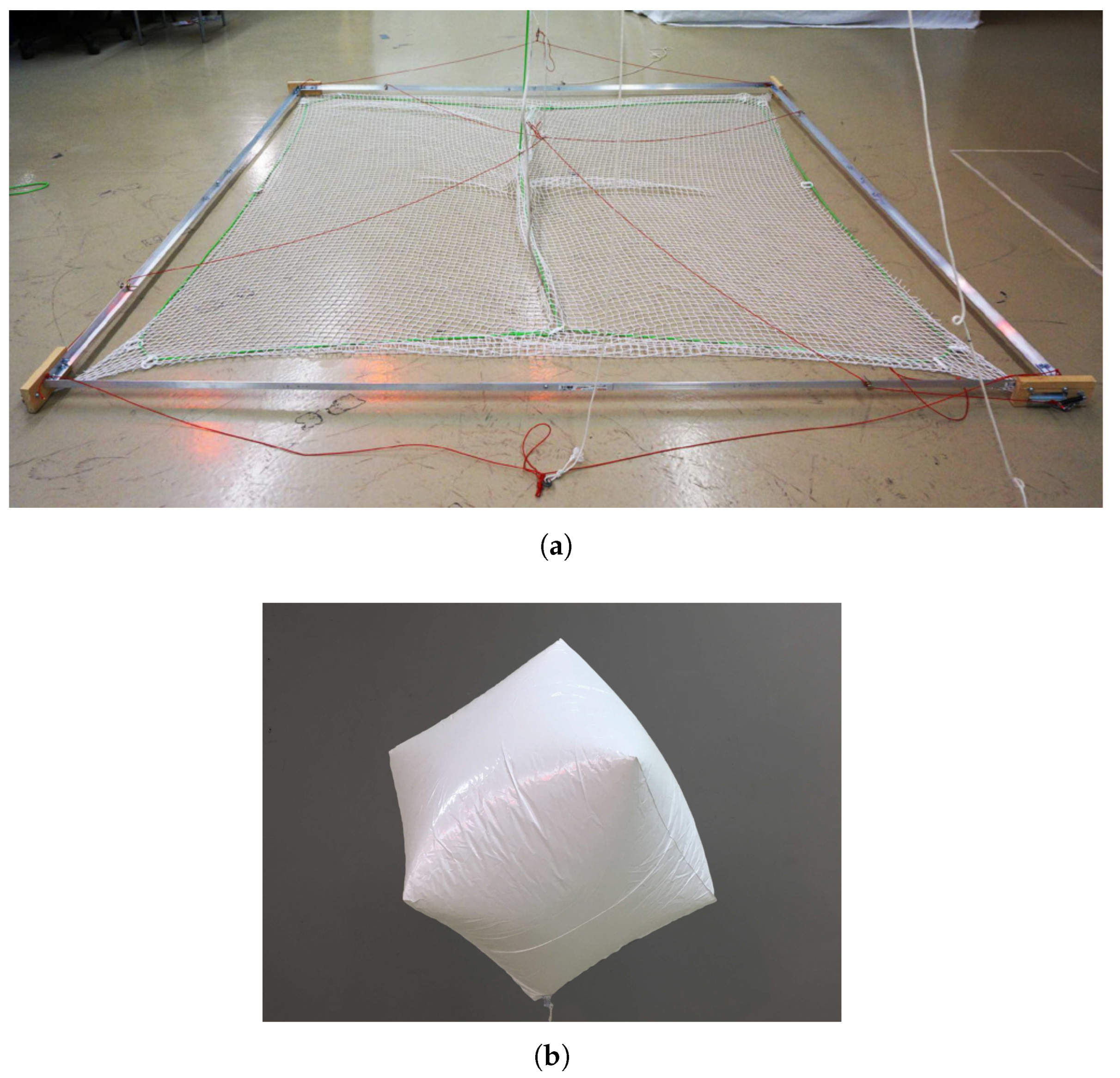
5. Net Capturing Systems: Modeling, Experiments and Space Missions
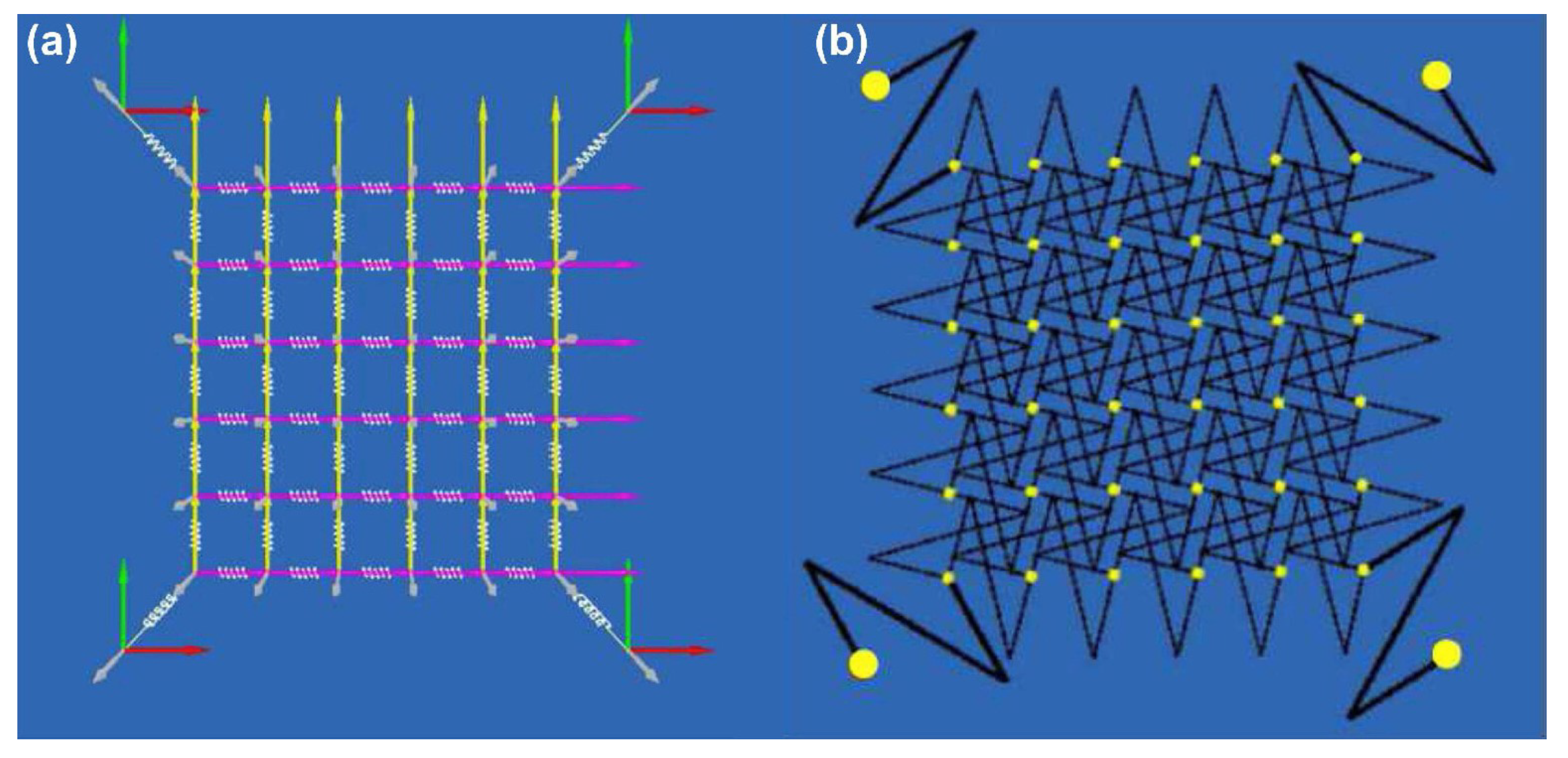
5.1. Modeling of Net Dynamics
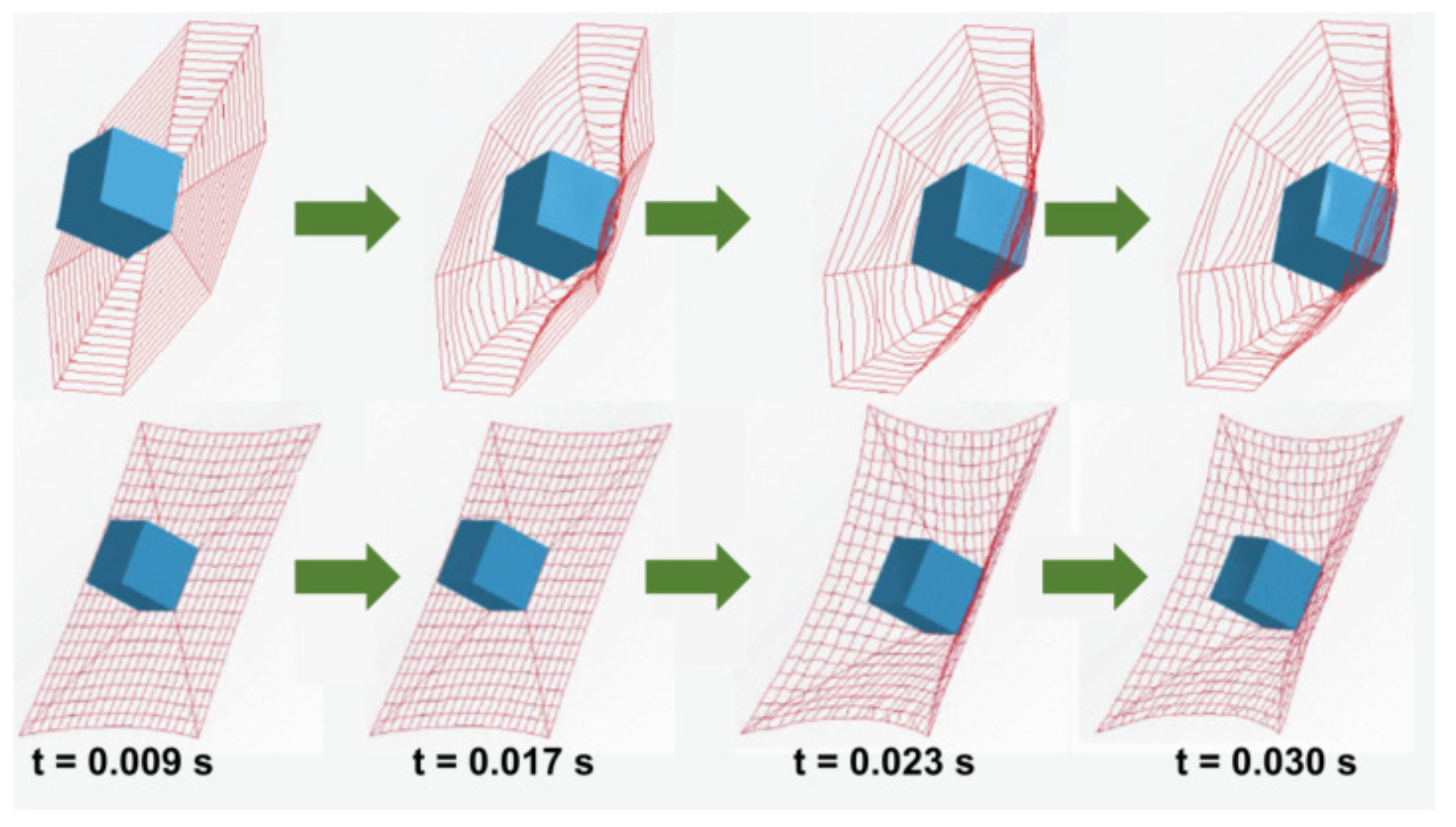
5.2. Debris-Net Contact Dynamics
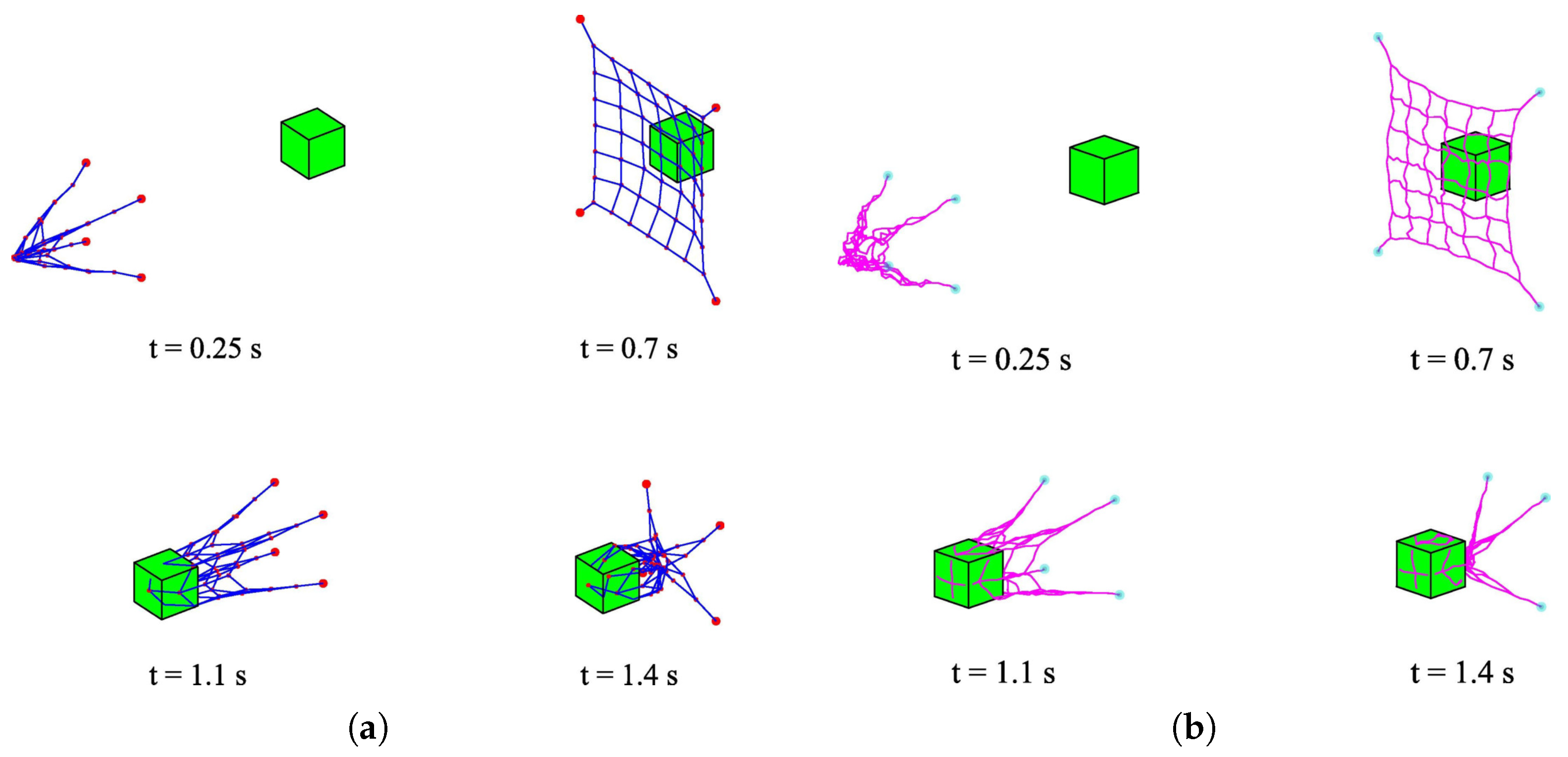
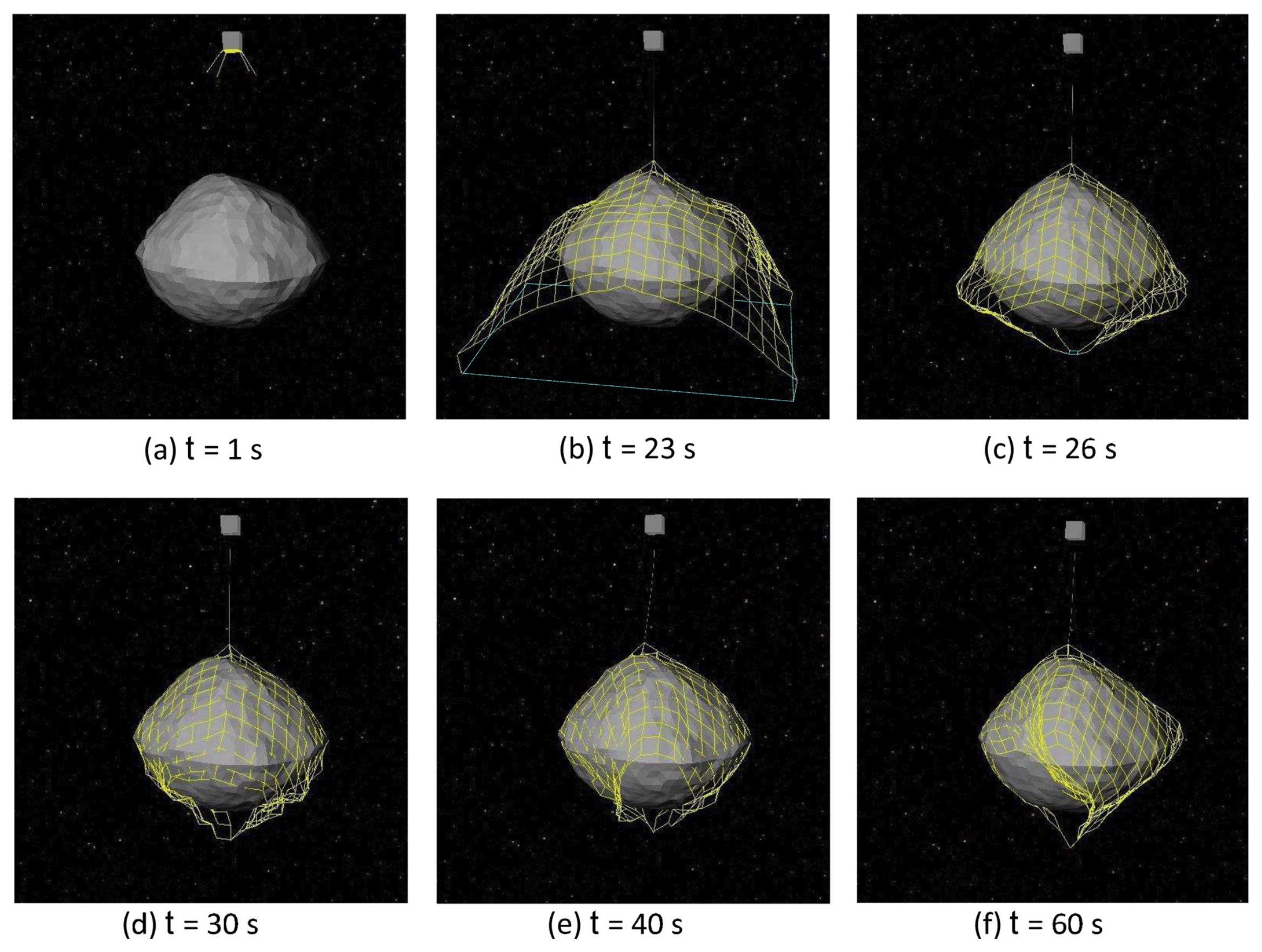
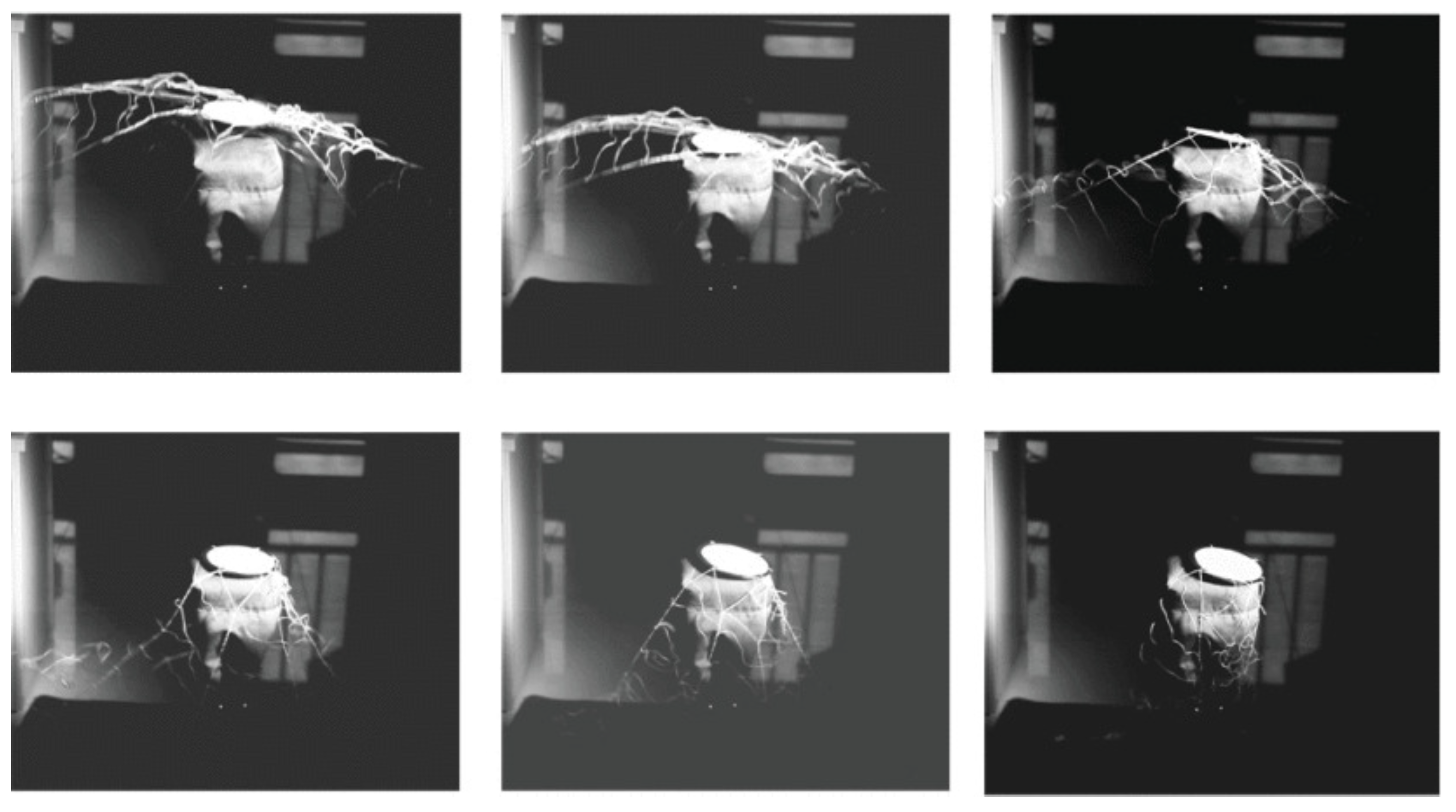
5.3. Numerical and Experimental Results for Space Debris Capture Systems
6. Preventive Steps
- Guideline 1: Limit debris released during normal operations.
- Guideline 2: Minimize the potential break-ups during operational phases.
- Guideline 3: Limit the probability of accidental collision in orbit.
- Guideline 4: Avoid intentional destruction and other harmful activities.
- Guideline 5: Minimize potential post-mission breakups resulting from stored energy.
- Guideline 6: Limit the long-term presence of spacecraft and launch vehicle orbital stages in the LEO region after the end of their mission.
- Guideline 7: Limit the long-term interference of spacecraft and launch vehicle orbital stages with the GEO region after the end of their mission.
- There is no unplanned physical contact between the payload and the vehicle or its components after payload separation.
- Energy sources such as chemical, pressure and kinetic energy do not result in the fragmentation of the vehicle or its components, creating new debris.
- Stored energy is removed by depleting residual fuel, venting any pressurized systems, and leaving batteries in a permanent discharge state.
- Avoidance of collisions with other large objects during routine operations.
- Post-mission disposal plans.
- Debris control strategies during routine operations, including explosion prevention and spacecraft shielding to avoid collisions with small debris.
7. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- ESA. ESA Space Debris User Portal, Space Environment Statistics. 2024. Available online: https://sdup.esoc.esa.int/discosweb/statistics/ (accessed on 20 September 2024).
- Leloglu, U.; Kocaoglan, E. Establishing space industry in developing countries: Opportunities and difficulties. Adv. Space Res. 2008, 42, 1879–1886. [Google Scholar] [CrossRef]
- Liou, J.C. USA space debris environment, operations, and measurement updates. Technical report, National Aeronautics and Space Administration, 2015. In Proceedings of the 52nd Session of the Scientific and Technical Subcommittee of the Committee on the Peaceful Uses of Outer Space, United Nations, Vienna, Austria, 2–13 February 2015. [Google Scholar]
- Schaub, H.; Jasper, L.E.Z.; Anderson, P.V.; McKnight, D.S. Cost and risk assessment for spacecraft operation decisions caused by the space debris environment. Acta Astronaut. 2015, 113, 66–79. [Google Scholar]
- Adilov, N.; Alexander, P.J.; Cunningham, B.M. An economic analysis of Earth orbit pollution. Environ. Resour. Econ. 2015, 60, 81–98. [Google Scholar] [CrossRef]
- Adilov, N.; Alexander, P.J.; Cunningham, B.M. The economics of orbital debris generation, accumulation, mitigation, and remediation. J. Space Saf. Eng. 2020, 7, 447–450. [Google Scholar]
- Adushkin, V.; Aksenov, O.Y.; Veniaminov, S.; Kozlov, S.; Tyurenkova, V. The small orbital debris population and its impact on space activities and ecological safety. Acta Astronaut. 2020, 176, 591–597. [Google Scholar]
- Haroun, F.; Ajibade, S.; Oladimeji, P.; Igbozurike, J.K. Toward the sustainability of outer space: Addressing the issue of space debris. New Space 2021, 9, 63–71. [Google Scholar]
- Air Command and Staff College. Chapter 6—Orbital Mechanics. In AU-18 Space Primer: Prepared by Air Command and Staff College Space Research Electives Seminar; Air University Press: Maxwell AFB, AL, USA, 2009. [Google Scholar]
- NASA. Chapter 5: Planetary Orbits; NASA: Washington, DC, USA. Available online: https://science.nasa.gov/learn/basics-of-space-flight/chapter5-1/ (accessed on 20 September 2024).
- ESA. Types of Orbit; ESA: Paris, France, 2020. [Google Scholar]
- Hall, L. The History of Space Debris. In Proceedings of the Space Traffic Management Conference, Daytona Beach, FL, USA, 5–6 November 2014. [Google Scholar]
- NASA. Impact, NASA Photo Gallery; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- Edelstein, K.S. Orbital impacts and the Space Shuttle windshield. In Proceedings of the Space Environmental, Legal, and Safety Issues, SPIE, Orlando, FL, USA, 17–21 April 1995; Volume 2483, pp. 52–61. [Google Scholar]
- Corsaro, R.; Liou, J.C.; Giovane, F. Spacecraft Damage-Detection System for Human Habitation Modules. In Proceedings of the 161st Acoustical Society of America Meeting, Seattle, WA, USA, 23–27 May 2011. [Google Scholar]
- ESA. ESA Test Impact, Hypervelocity Impact Sample; ESA: Paris, France, 2009. [Google Scholar]
- ESA. ESA Test Impact Analysis, ATV Shielding After Impact Test; ESA: Paris, France, 2014. [Google Scholar]
- Hammerstein, H.C.; Daire, S.A. Hazards of Space Debris. In Handbook of Life Support Systems for Spacecraft and Extraterrestrial Habitats; Springer International Publishing: Cham, Switzerland, 2020; pp. 1–23. [Google Scholar]
- ESA. ESA Space Insights, Impact Chip; ESA: Paris, France, 2016. [Google Scholar]
- Christiansen, E.; Hyde, J.; Bernhard, R. Space shuttle debris and meteoroid impacts. Adv. Space Res. 2004, 34, 1097–1103. [Google Scholar]
- Liou, J.-C.; Johnson, N.L. Risks in Space from Orbiting Debris. Science 2006, 311, 340–341. [Google Scholar]
- Belk, C.A. Meteoroids and Orbital Debris: Effects on Spacecraft; National Aeronautics and Space Administration, Marshall Space Flight Center: Huntsville, AL, USA, 1997; Volume 1408. [Google Scholar]
- Yano, H. Meteoroids and Space Debris Impacts on Telescopes in Space. Preserv. Astron. Wind. 1998, 139, 65. [Google Scholar]
- ESA. Space Safety, Active Debris Removal. Available online: https://www.esa.int/Space_Safety/Space_Debris/Active_debris_removal (accessed on 1 October 2024).
- Foust, J. SpaceNews, Upper Stages Top List of Most Dangerous Space Debris. 2020. Available online: https://spacenews.com/upper-stages-top-list-of-most-dangerous-space-debris/ (accessed on 13 October 2020).
- Pardini, C.; Anselmo, L. Assessment of the consequences of the Fengyun-1C breakup in low Earth orbit. Adv. Space Res. 2009, 44, 545–557. [Google Scholar]
- Jakhu, R.S. Iridium-Cosmos collision and its implications for space operations. In Yearbook on Space Policy 2008/2009: Setting New Trends; Springer: Vienna, Austria, 2010; pp. 254–275. [Google Scholar]
- Mejía-Kaiser, M. Collision Course: The 2009 Iridium-Cosmos Crash. In Proceedings of the 52th IISL Colloquium on the Law of Outer Space, Daejeon, Republic of Korea, 12–16 October 2009. [Google Scholar]
- Kessler, D.J.; Cour-Palais, B.G. Collision frequency of artificial satellites: The creation of a debris belt. J. Geophys. Res. Space Phys. 1978, 83, 2637–2646. [Google Scholar]
- NASA. Graphics, NASA’s Photo Gallery; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- Guerra, G.; Muresan, A.C.; Nordqvist, K.G.; Brissaud, A.; Naciri, N.; Luo, L. Active space debris removal system. INCAS Bull. 2017, 9, 97–116. [Google Scholar]
- ESA. ESA Annual Space Environment Report; Technical Report; European Space Agency: Paris, France, 2022. [Google Scholar]
- NASA. Orbital Debris Quarterly News; National Aeronautics and Space Administration (NASA): Washington, DC, USA, 2022; Volume 26.
- NASA. Orbital Debris Quarterly News; National Aeronautics and Space Administration (NASA): Washington, DC, USA, 2021; Volume 25.
- Mehrholz, D.; Leushacke, L.; Flury, W.; Jehn, R.; Klinkrad, H.; Landgraf, M. Detecting, tracking and imaging space debris. ESA Bull. 2002, 109, 128–134. [Google Scholar]
- Gruntman, M. Passive optical detection of submillimeter and millimeter size space debris in low Earth orbit. Acta Astronaut. 2014, 105, 156–170. [Google Scholar]
- Anttonen, A.; Kiviranta, M.; Höyhtyä, M. Space debris detection over intersatellite communication signals. Acta Astronaut. 2021, 187, 156–166. [Google Scholar]
- Ferro-Famil, L.; Pottier, E. Chapter 1: Synthetic Aperture Radar Imaging. In Microwave Remote Sensing of Land Surface; Elsevier: Amsterdam, The Netherlands, 2016; pp. 1–65. [Google Scholar]
- Zhang, M.; Wen, G.; Fan, C.; Guan, B.; Song, Q.; Liu, C.; Wang, S. Analysis of the Ranging Capability of a Space Debris Laser Ranging System Based on the Maximum Detection Distance Model. Remote Sens. 2024, 16, 727. [Google Scholar] [CrossRef]
- Zhang, Z.P.; Yang, F.M.; Zhang, H.F.; Wu, Z.B.; Chen, J.P.; Li, P.; Meng, W.D. The use of laser ranging to measure space debris. Res. Astron. Astrophys. 2012, 12, 212. [Google Scholar] [CrossRef]
- Zhang, H.; Long, M.; Deng, H.; Cheng, S.; Wu, Z.; Zhang, Z.; Zhang, A.; Sun, J. Developments of space debris laser ranging technology including the applications of picosecond lasers. Appl. Sci. 2021, 11, 10080. [Google Scholar] [CrossRef]
- Steindorfer, M.; Kirchner, G.; Koidl, F.; Wang, P.; Jilete, B.; Flohrer, T. Daylight space debris laser ranging. Nat. Commun. 2020, 11, 3735. [Google Scholar] [CrossRef]
- NASA. In Situ Measurements, Hubble Space Telescope, WFPC-2; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- NASA. Debris Measurements; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- Silha, J. Space Debris: Optical Measurements. In Reviews in Frontiers of Modern Astrophysics: From Space Debris to Cosmology; Springer International Publishing: Cham, Switzerland, 2020; pp. 1–21. [Google Scholar]
- Sun, R.Y.; Yu, S.X. Precise measurement of the light curves for space debris with wide field of view telescope. Astrophys. Space Sci. 2019, 364, 39. [Google Scholar]
- Lu, Y.; Zhao, C.Y. The Basic Shape Classification of Space Debris with Light Curves. Chin. Astron. Astrophys. 2021, 45, 190–208. [Google Scholar]
- Schildknecht, T. Optical surveys for space debris. Astron. Astrophys. Rev. 2007, 14, 41–111. [Google Scholar] [CrossRef]
- Luo, H.; Zheng, J.H.; Wang, W.; Cao, J.J.; Zhu, J.; Chen, G.P.; Zhang, Y.S.; Liu, C.S.; Mao, Y.D. FocusGEO II. A telescope with imaging mode based on image overlay for debris at Geosynchronous Earth Orbit. Adv. Space Res. 2022, 69, 2618–2628. [Google Scholar] [CrossRef]
- Woodgate, B.E.; Kimble, R.A.; Bowers, C.W.; Kraemer, S.; Kaiser, M.E.; Danks, A.C.; Grady, J.F.; Loiacono, J.J.; Brumfield, M.; Feinberg, L.; et al. The Space Telescope Imaging Spectrograph Design1. Publ. Astron. Soc. Pac. 1998, 110, 1183. [Google Scholar] [CrossRef]
- Green, J.C.; Froning, C.S.; Osterman, S.; Ebbets, D.; Heap, S.H.; Leitherer, C.; Linsky, J.L.; Savage, B.D.; Sembach, K.; Shull, J.M.; et al. The cosmic origins spectrograph. Astrophys. J. 2011, 744, 60. [Google Scholar] [CrossRef]
- NASA. Optical Measurements; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- Eaves, J.; Reedy, E. Principles of Modern Radar; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Cakaj, S.; Fischer, M.; Scholtz, A.L. Practical Horizon Plane for Low Earth Orbiting (LEO) Satellite Ground Stations. In Proceedings of the 8th WSEAS International Conference on Telecommunications and Informatics, Istanbul, Turkey, 30 May–1 June 2009; pp. 62–67. [Google Scholar]
- Li, H.J.; Kiang, Y.W. Chapter 10—Radar and Inverse Scattering. In The Electrical Engineering Handbook; Academic Press: Burlington, MA, USA, 2005; pp. 671–690. [Google Scholar]
- Emery, W.; Camps, A. Chapter 5—Radar. In Introduction to Satellite Remote Sensing; Elsevier: Amsterdam, The Netherlands, 2017; pp. 291–453. [Google Scholar]
- Skolnik, M.I. Introduction to Radar Systems; McGraw-hill: New York, NY, USA, 1980. [Google Scholar]
- Rihaczek, A.W. Principles of High-Resolution Radar; Artech House Publishers: Norwood, MA, USA, 1996. [Google Scholar]
- Smith, C.H.; Greene, B. The EOS space debris tracking system. In Proceedings of the Advanced Maui Optical and Space Surveillance Technologies Conference, Maui, HI, USA, 10–14 September 2006. [Google Scholar]
- Bartels, N.; Allenspacher, P.; Hampf, D.; Heidenreich, B.; Keil, D.; Schafer, E.; Riede, W. Space object identification via polarimetric satellite laser ranging. Commun. Eng. 2022, 1, 5. [Google Scholar] [CrossRef]
- Liou, J.C.; Burchell, M.; Corsaro, R.; Drolshagen, G.; Giovane, F.; Pisacane, V.; Stansbery, E. In situ measurement activities at the NASA Orbital Debris Program Office. In Proceedings of the European Conference on Space Debris, Darmstadt, Germany, 30 March–2 April 2009. Number JSC-CN-17516. [Google Scholar]
- Tsao, M.A.; Ngo, H.T.; Corsaro, R.D.; Anderson, C.R. An in situ measurement system for characterizing orbital debris. IEEE Trans. Instrum. Meas. 2016, 65, 2758–2772. [Google Scholar] [CrossRef]
- Furumoto, M.; Sahara, H. Statistical assessment of detection of changes in space debris environment utilizing in-situ measurements. Acta Astronaut. 2020, 177, 666–672. [Google Scholar] [CrossRef]
- Krisko, P.; Flegel, S.; Matney, M.J.; Jarkey, D.R.; Braun, V. ORDEM 3.0 and MASTER-2009 modeled debris population comparison. Acta Astronaut. 2015, 113, 204–211. [Google Scholar]
- Benvenuto, R.; Salvi, S.; Lavagna, M. Dynamics Analysis and GNC Design of Flexible Systems for Space Debris Active Removal. Acta Astronaut. 2015, 110, 247–265. [Google Scholar] [CrossRef]
- Nishida, S.I.; Kawamoto, S. Strategy for capturing of a tumbling space debris. Acta Astronaut. 2011, 68, 113–120. [Google Scholar]
- Botta, E.M.; Sharf, I.; Misra, A.K. Evaluation of net capture of space debris in multiple mission scenarios. In Proceedings of the 26th AAS/AIAA Space Flight Mechanics Meeting, Napa, CA, USA, 14–18 February 2016; pp. 16–254. [Google Scholar]
- Shan, M.; Shi, L. Post-capture control of a tumbling space debris via tether tension. Acta Astronaut. 2021, 180, 317–327. [Google Scholar]
- Wu, C.; Yue, S.; Shi, W.; Li, M.; Du, Z.; Liu, Z. Dynamic Simulation and Parameter Analysis of Harpoon Capturing Space Debris. Materials 2022, 15, 8859. [Google Scholar] [CrossRef]
- Ribeiro, J.; Pelicioni, L.C.; Caldas, I.; Lahoz, C.; Belderrain, M.C.N. Evolution of policies and technologies for space debris mitigation based on bibliometric and patent analyses. Space Policy 2018, 44, 40–56. [Google Scholar] [CrossRef]
- Guang, Z.; Zhang, J. Space Tether Net System for Debris Capture and Removal. In Proceedings of the 2012 4th International Conference on Intelligent Human-Machine Systems and Cybernetics, Nanchang, China, 26–27 August 2012; Volume 1, pp. 257–261. [Google Scholar]
- Mayorova, V.I.; Shcheglov, G.A.; Stognii, M.V. Analysis of the space debris objects nozzle capture dynamic processed by a telescopic robotic arm. Acta Astronaut. 2021, 187, 259–270. [Google Scholar] [CrossRef]
- Liu, E.; Yan, Y.; Yang, Y. Analysis and determination of capture area for space debris removal based on reachable domain. Adv. Space Res. 2021, 68, 1613–1626. [Google Scholar] [CrossRef]
- Nishida, S.; Uenaka, D.; Matsumoto, R.; Nakatani, S. Lightweight robot arm for capturing large space debris. J. Electr. Eng. 2018, 6, 271–280. [Google Scholar]
- Shan, M.; Guo, J.; Gill, E. Review and comparison of active space debris capturing and removal methods. Prog. Aerosp. Sci. 2016, 80, 18–32. [Google Scholar]
- Takahashi, K.; Charles, C.; Boswell, R.W.; Ando, A. Demonstrating a new technology for space debris removal using a bi-directional plasma thruster. Sci. Rep. 2018, 8, 14417. [Google Scholar]
- Phipps, C.R.; Baker, K.L.; Libby, S.B.; Liedahl, D.A.; Olivier, S.S.; Pleasance, L.D.; Rubenchik, A.; Trebes, J.E.; George, E.V.; Marcovici, B.; et al. Removing orbital debris with lasers. Adv. Space Res. 2012, 49, 1283–1300. [Google Scholar] [CrossRef]
- Bennett, T.; Schaub, H. Contactless electrostatic detumbling of axi-symmetric GEO objects with nominal pushing or pulling. Adv. Space Res. 2018, 62, 2977–2987. [Google Scholar] [CrossRef]
- Lu, E.T.; Love, S.G. Gravitational tractor for towing asteroids. Nature 2005, 438, 177–178. [Google Scholar] [CrossRef]
- Murdoch, N.; Izzo, D.; Bombardelli, C.; Carnelli, I.; Hilgers, A.; Rodgers, D. Electrostatic tractor for near Earth object deflection. In Proceedings of the 59th International Astronautical Congress, Glasgow, Scotland, 29 September–3 October 2008. [Google Scholar]
- Bengtson, M.; Wilson, K.; Hughes, J.; Schaub, H. Survey of the electrostatic tractor research for reorbiting passive GEO space objects. Astrodynamics 2018, 2, 291–305. [Google Scholar] [CrossRef]
- Schaub, H.; Sternovsky, Z. Active space debris charging for contactless electrostatic disposal maneuvers. Adv. Space Res. 2014, 53, 110–118. [Google Scholar] [CrossRef]
- Tsuno, K.; Wada, S.; Ogawa, T.; Saito, N.; Fukushima, T.; Ebisuzaki, T.; Nakamura, Y.; Sasoh, A. Laser ablation induced impulse study for removal of space debris mission using small satellite. Appl. Phys. A 2022, 128, 932. [Google Scholar] [CrossRef]
- Shen, S.; Jin, X.; Hao, C. Cleaning space debris with a space-based laser system. Chin. J. Aeronaut. 2014, 27, 805–811. [Google Scholar] [CrossRef]
- Phipps, C.R. L’ADROIT—A spaceborne ultraviolet laser system for space debris clearing. Acta Astronaut. 2014, 104, 243–255. [Google Scholar]
- Ledkov, A.; Aslanov, V. Review of contact and contactless active space debris removal approaches. Prog. Aerosp. Sci. 2022, 134, 100858. [Google Scholar] [CrossRef]
- Marcus, C.L.; Perez, A.D.; Zanetti, R. Guidance and Control for Laser-Based Non-Cooperative Target Detumbling. In Proceedings of the 46th Annual AAS Guidance, Navigation and Control (GN&C) Conference, Breckenridge, CO, USA, 1–7 February 2024. [Google Scholar]
- Anzaldua, A.; Barnhard, G.; Dunlop, D.; Phipps, C. A path to a commercial orbital debris cleanup, power-beaming, and communications utility, using technology development missions at the ISS. The Space Review. Available online: https://www.thespacereview.com/article/3363/1 (accessed on 6 November 2017).
- Walker, L.; Vasile, M. Space debris remediation using space-based lasers. Adv. Space Res. 2023, 72, 2786–2800. [Google Scholar]
- Yang, F.Y.; Nelson, B.; Aziz, J.; Carlino, R.; Perez, A.D.; Faber, N.; Foster, C.; Frost, C.; Henze, C.; Karacalioğlu, A.G.; et al. LightForce photon-pressure collision avoidance: Efficiency analysis in the current debris environment and long-term simulation perspective. Acta Astronaut. 2016, 126, 411–423. [Google Scholar] [CrossRef]
- Fang, Y. Influence rules of ground-based laser active removing centimeter-sized orbital debris in LEO. Optik 2018, 170, 210–219. [Google Scholar] [CrossRef]
- Urrutxua, H.; Bombardelli, C.; Hedo, J.M. A preliminary design procedure for an ion-beam shepherd mission. Aerosp. Sci. Technol. 2019, 88, 421–435. [Google Scholar] [CrossRef]
- Ledkov, A.S.; Aslanov, V.S. Active space debris removal by ion multi-beam shepherd spacecraft. Acta Astronaut. 2023, 205, 247–257. [Google Scholar] [CrossRef]
- Bombardelli, C.; Peláez, J. Ion Beam Shepherd for Contactless Debris Removal. J. Guid. Control Dyn. 2011, 34, 916–920. [Google Scholar] [CrossRef]
- Visagie, L.; Lappas, V.; Erb, S. Drag sails for space debris mitigation. Acta Astronaut. 2015, 109, 65–75. [Google Scholar] [CrossRef]
- Fernandez, J.M.; Visagie, L.; Schenk, M.; Stohlman, O.R.; Aglietti, G.S.; Lappas, V.J.; Erb, S. Design and development of a gossamer sail system for deorbiting in low earth orbit. Acta Astronaut. 2014, 103, 204–225. [Google Scholar] [CrossRef]
- Baba, M.H.; Manzoor, M.M.M.; Singh, A.; Kumar, R.; Thakur, A.K. Review analysis of problems associated with the various space debris removal methods. Mater. Today Proc. 2023, in press. [CrossRef]
- ESA. ADEO: Drag Sail Technology for Satellite Deorbiting, ADEO Artist Impression; ESA: Paris, France, 2020. [Google Scholar]
- Svotina, V.V.; Cherkasova, M.V. Space debris removal–Review of technologies and techniques. Flexible or virtual connection between space debris and service spacecraft. Acta Astronaut. 2023, 204, 840–853. [Google Scholar] [CrossRef]
- Gaspar, J.L.; Behun, V.; Mann, T.; Murphy, D.; Macy, B. Testing of a 20-meter solar sail system. In Proceedings of the Spacecraft Propulsion Subcommittee Joint Meeting, Monterey, CA, USA, 5–9 December 2005. [Google Scholar]
- Serfontein, Z.; Kingston, J.; Hobbs, S.; Holbrough, I.E.; Beck, J.C. Drag augmentation systems for space debris mitigation. Acta Astronaut. 2021, 188, 278–288. [Google Scholar] [CrossRef]
- Andrenucci, M.; Pergola, P.; Ruggiero, A.; Olympio, J.; Summerer, L. Active removal of space debris-expanding foam application for active debris removal. ESA Final Rep. 2011. Available online: https://www.esa.int/gsp/ACT/doc/ARI/ARI%20Study%20Report/ACT-RPT-MAD-ARI-10-6411-Pisa-Active_Removal_of_Space_Debris-Foam.pdf (accessed on 21 February 2011).
- Rostilov, T.; Ziborov, V. Experimental study of shock wave structure in syntactic foams under high-velocity impact. Acta Astronaut. 2021, 178, 900–907. [Google Scholar] [CrossRef]
- Yalçın, B.C.; Martinez, C.; Delisle, M.H.; Rodriguez, G.; Zheng, J.; Olivares-Mendez, M. ET-class: An energy transfer-based classification of space debris removal methods and missions. Front. Space Technol. 2022, 3, 792944. [Google Scholar]
- Wright, R.J. Orbital Debris Mitigation System and Method. U.S. Patent 8,567,725, 29 October 2013. [Google Scholar]
- Pardini, C.; Hanada, T.; Krisko, P.H.; Anselmo, L.; Hirayama, H. Are de-orbiting missions possible using electrodynamic tethers? Task review from the space debris perspective. Acta Astronaut. 2007, 60, 916–929. [Google Scholar]
- Yamagiwa, Y.; Kanbe, A.; Wakatsuki, M.; Tanaka, K.; Sumino, M.; Watanabe, T.; Sahara, H.; Fujii, H.A. Current Collection Experiment of Bare Electrodynamic Tether Using Sounding Rocket. Trans. Jpn. Soc. Aeronaut. Space Sci. Aerosp. Technol. Jpn. 2010, 8, 5–10. [Google Scholar]
- Kawamoto, S.; Makida, T.; Sasaki, F.; Okawa, Y.; Nishida, S. Precise numerical simulations of electrodynamic tethers for an active debris removal system. Acta Astronaut. 2006, 59, 139–148. [Google Scholar]
- Ohkawa, Y.; Kawamoto, S.; Higashide, M.; Iki, K.; Baba, M.; Kitamura, S.; Kibe, S. Electrodynamic tether propulsion for orbital debris deorbit. J. Space Technol. Sci. 2012, 26, 33–46. [Google Scholar]
- Olivieri, L.; Valmorbida, A.; Sarego, G.; Lungavia, E.; Vertuani, D.; Lorenzini, E.C. Test of tethered deorbiting of space debris. Adv. Astronaut. Sci. Technol. 2020, 3, 115–124. [Google Scholar]
- Fehse, W. Rendezvous with and capture/removal of non-cooperative bodies in orbit: The technical challenges. J. Space Saf. Eng. 2014, 1, 17–27. [Google Scholar]
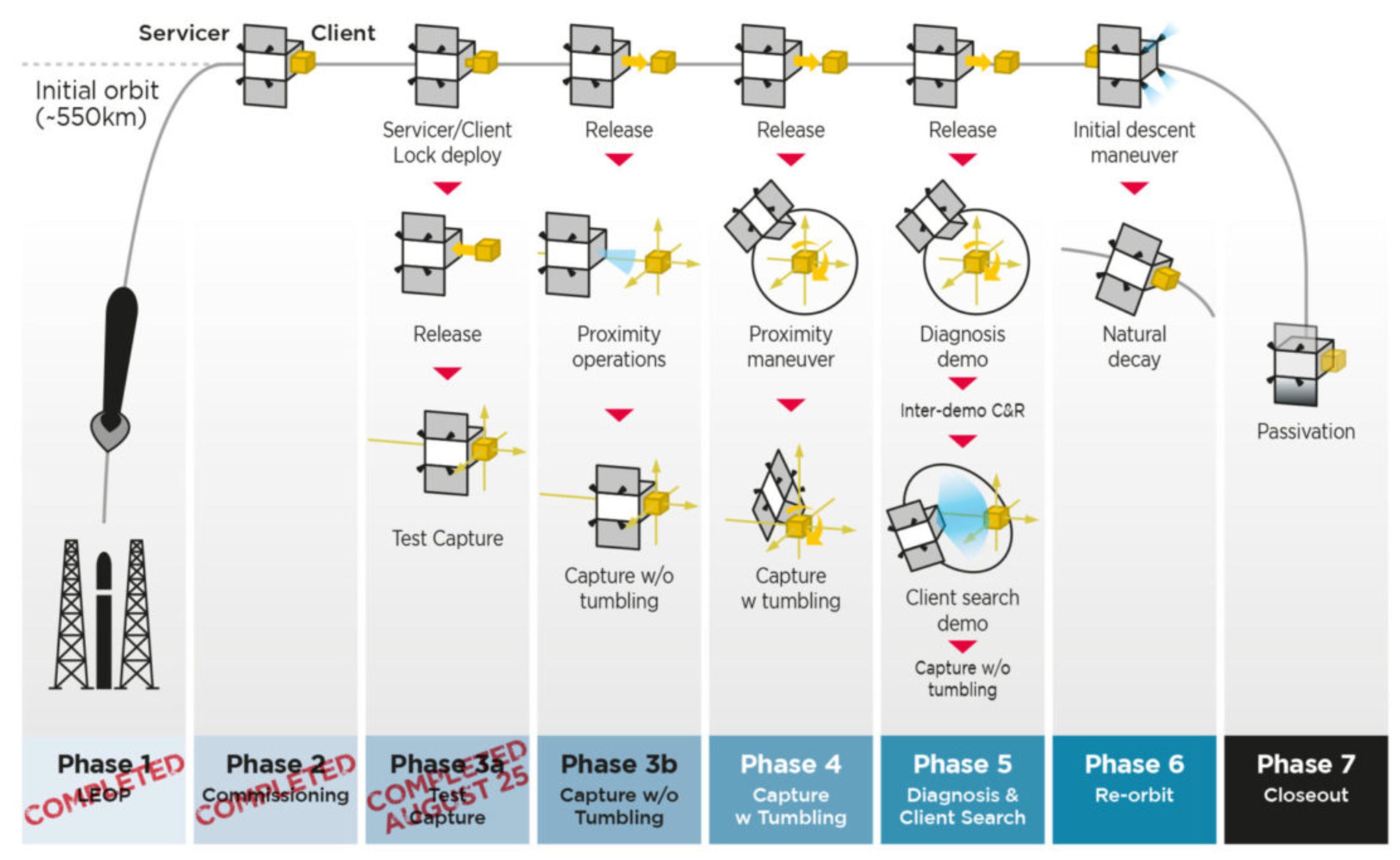
- Blackerby, C.; Okamoto, A.; Kobayashi, Y.; Fujimoto, K.; Seto, Y.; Fujita, S.; Iwai, T.; Okada, N.; Forshaw, J.; Auburn, J.; et al. The ELSA-d End-of-life Debris Removal Mission: Preparing for Launch. In Proceedings of the 70th International Astronautical Congress, Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Biesbroek, R.; Soares, T.; Hüsing, J.; Innocenti, L. The e.Deorbit CDF Study: A Design Study for the Safe Removal of a Large Space Debris. In Proceedings of the 6th European Conference on Space Debris, Darmstadt, Germany, 22–25 April 2013. [Google Scholar]
- Biesbroek, R.; Aziz, S.; Wolahan, A.; Cipolla, S.; Richard-Noca, M.; Piguet, L. The clearspace-1 mission: ESA and clearspace team up to remove debris. In Proceedings of the 8th European Conference on Space Debris (Virtual), Darmstadt, Germany, 20–23 April 2021; pp. 1–3. [Google Scholar]
- ESA. ClearSpace-1, The First Mission to Remove a Piece of Space Debris from Orbit, ClearSpace-1 Will Rendezvous with, Capture and Safely Bring Down a Satellite for a Safe Atmospheric Reentry. Available online: https://www.esa.int/Space_Safety/ClearSpace-1 (accessed on 1 October 2024).
- Han, D.; Dong, G.; Huang, P.; Ma, Z. Capture and detumbling control for active debris removal by a dual-arm space robot. Chin. J. Aeronaut. 2022, 35, 342–353. [Google Scholar] [CrossRef]
- Flores-Abad, A.; Ma, O.; Pham, K.; Ulrich, S. A review of space robotics technologies for on-orbit servicing. Prog. Aerosp. Sci. 2014, 68, 1–26. [Google Scholar]
- Zhu, A.; Ai, H.; Chen, L. FSTSMC Compliance Control for Dual-Arm Space Robot With SDBD Capture Satellite Operation. J. Comput. Nonlinear Dyn. 2023, 18, 061006. [Google Scholar]
- Fu, X.; Ai, H.; Chen, L. Integrated sliding mode control with input restriction, output feedback and repetitive learning for space robot with flexible-base, flexible-link and flexible-joint. Robotica 2023, 41, 370–391. [Google Scholar]
- Ai, H.; Zhu, A.; Wang, J.; Yu, X.; Chen, L. Buffer compliance control of space robots capturing a non-cooperative spacecraft based on reinforcement learning. Appl. Sci. 2021, 11, 5783. [Google Scholar] [CrossRef]
- Vyas, S.; Jankovic, M.; Kirchner, F. Momentum based classification for robotic active debris removal. J. Space Saf. Eng. 2022, 9, 649–655. [Google Scholar] [CrossRef]
- Nishida, S.; Kawamoto, S. Dynamical simulations for space debris capture. In Proceedings of the SICE Annual Conference 2011, Tokyo, Japan, 13–18 September 2011; IEEE: New York, NY, USA, 2011; pp. 2283–2286. [Google Scholar]
- Zhang, W.; Li, F.; Li, J.; Cheng, Q. Review of on-orbit robotic arm active debris capture removal methods. Aerospace 2022, 10, 13. [Google Scholar] [CrossRef]
- Aslanov, V.S.; Yudintsev, V.V. Docking of a space tug with upper stage debris object using deployable flexible beam. In Proceedings of the 68th International Astronautical Congress, IAC, Adelaide, Australia, 25–29 September 2017; pp. 1–7. [Google Scholar]
- Cheng, R.; Liu, Z.; Ma, Z.; Huang, P. Approach and maneuver for failed spacecraft de-tumbling via space teleoperation robot system. Acta Astronaut. 2021, 181, 384–395. [Google Scholar] [CrossRef]
- Xue, Z.; Zhang, X.; Liu, J. Trajectory planning of a dual-arm space robot for target capturing with minimizing base disturbance. Adv. Space Res. 2023, 72, 2091–2108. [Google Scholar]
- Wan, W.; Sun, C.; Yuan, J.; Hou, X.; Guo, Y.; Ou-yang, Y.; Li, Q.; Zhao, L.; Shi, H.; Han, D. Adaptive whole-arm grasping approach of tumbling space debris by two coordinated hyper-redundant manipulators. In Proceedings of the Intelligent Robotics and Applications: 12th International Conference, ICIRA 2019, Shenyang, China, 8–11 August 2019; Proceedings, Part I 12. Springer: Cham, Switzerland, 2019; pp. 450–461. [Google Scholar]
- Ellery, A. A robotics perspective on human spaceflight. Earth Moon Planets 1999, 87, 173–190. [Google Scholar]
- Poozhiyil, M.; Nair, M.H.; Rai, M.C.; Hall, A.; Meringolo, C.; Shilton, M.; Kay, S.; Forte, D.; Sweeting, M.; Antoniou, N.; et al. Active debris removal: A review and case study on LEOPARD Phase 0-A mission. Adv. Space Res. 2023, 72, 3386–3413. [Google Scholar]
- Nagaoka, K.; Kameoka, R.; Yoshida, K. Repeated impact-based capture of a spinning object by a dual-arm space robot. Front. Robot. AI 2018, 5, 115. [Google Scholar]
- Yan, L.; Xu, W.; Hu, Z.; Liang, B. Multi-objective configuration optimization for coordinated capture of dual-arm space robot. Acta Astronaut. 2020, 167, 189–200. [Google Scholar] [CrossRef]
- Soleymani, M.; Kiani, M. Planar soft space robotic manipulators: Dynamic modeling and control. Adv. Space Res. 2024, 74, 384–402. [Google Scholar]
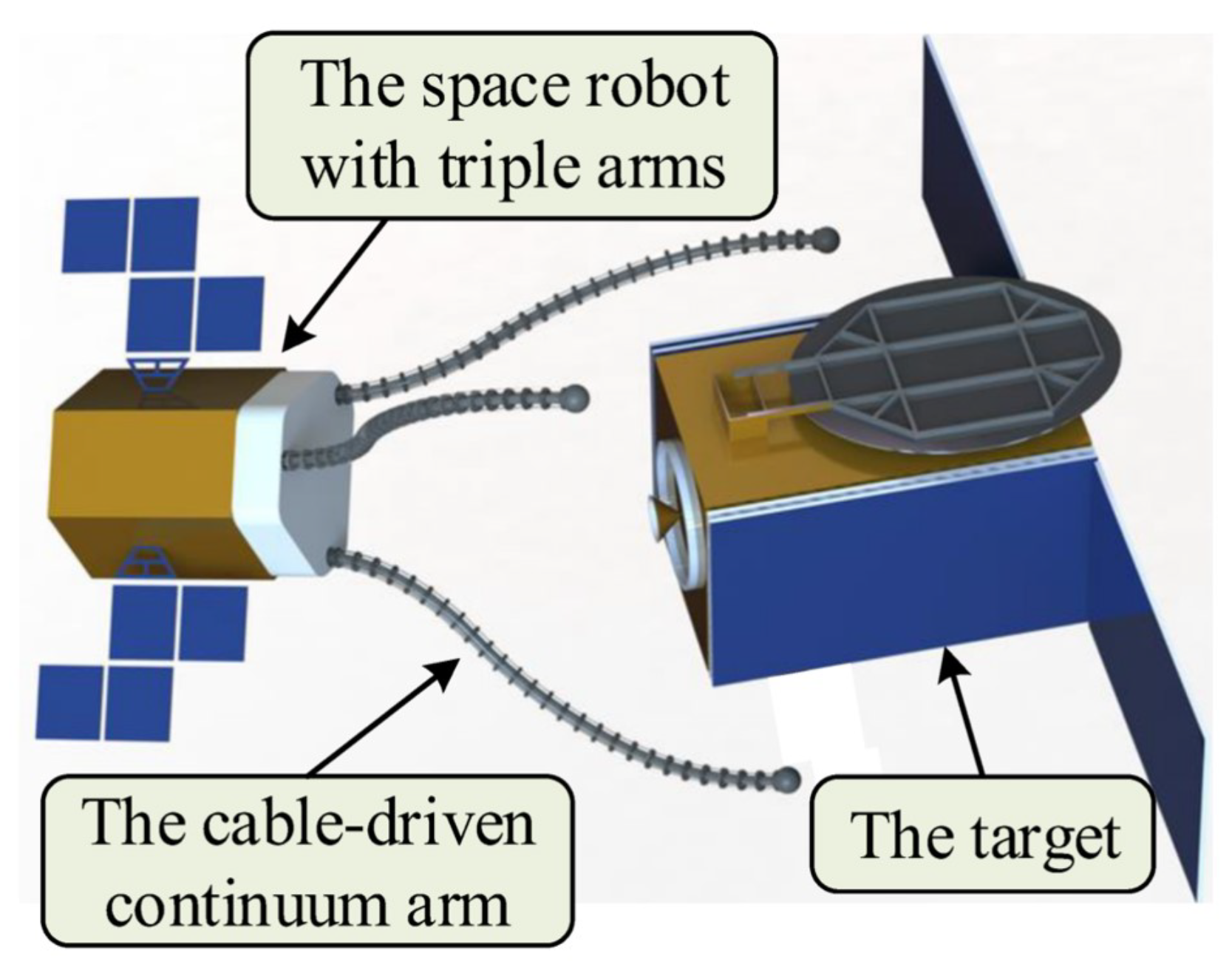
- Dai, Y.; Li, Z.; Chen, X.; Wang, X.; Yuan, H. A novel space robot with triple cable-driven continuum arms for space grasping. Micromachines 2023, 14, 416. [Google Scholar] [CrossRef]
- Meng, D.; Ouyang, X.; Tan, J.; Wang, X.; Liang, B. Kinematics modeling method of continuum space manipulator based on virtual discrete-jointed manipulator models. Acta Astronaut. 2023, 211, 257–267. [Google Scholar]
- Chen, X.; Zhang, X.; Huang, Y.; Cao, L.; Liu, J. A review of soft manipulator research, applications, and opportunities. J. Field Robot. 2022, 39, 281–311. [Google Scholar]
- McMahan, W.; Chitrakaran, V.; Csencsits, M.; Dawson, D.; Walker, I.D.; Jones, B.A.; Pritts, M.; Dienno, D.; Grissom, M.; Rahn, C.D. Field trials and testing of the OctArm continuum manipulator. In Proceedings of the 2006 IEEE International Conference on Robotics and Automation, ICRA 2006, Orlando, FL, USA, 15–19 May 2006; IEEE: New York, NY, USA, 2006; pp. 2336–2341. [Google Scholar]
- Agabiti, C.; Ménager, E.; Falotico, E. Whole-arm grasping strategy for soft arms to capture space debris. In Proceedings of the 2023 IEEE International Conference on Soft Robotics (RoboSoft), Singapore, 3–7 April 2023; IEEE: New York, NY, USA, 2023; pp. 1–6. [Google Scholar]
- Teeple, C.B.; Koutros, T.N.; Graule, M.A.; Wood, R.J. Multi-segment soft robotic fingers enable robust precision grasping. Int. J. Robot. Res. 2020, 39, 1647–1667. [Google Scholar]
- Chaudhary, B. Unconventional methods for space debris removal. In Proceedings of the Space Safety is No Accident: The 7th IAASS Conference, Friedrichshafen, Germany, 20–22 October 2014; Springer: Cham, Switzerland, 2015; pp. 49–58. [Google Scholar]
- Missel, J.; Mortari, D. Sling satellite for debris removal with aggie sweeper. Adv. Astronaut. Sci. 2011, 140, 60–64. [Google Scholar]
- Missel, J.; Mortari, D. Optimization of debris removal path for TAMU sweeper. Adv. Astronaut. Sci. 2012, 143, 935–945. [Google Scholar]
- Missel, J.; Mortari, D. Path optimization for Space Sweeper with Sling-Sat: A method of active space debris removal. Adv. Space Res. 2013, 52, 1339–1348. [Google Scholar] [CrossRef]
- Missel, J.; Mortari, D. Removing space debris through sequential captures and ejections. J. Guid. Control. Dyn. 2013, 36, 743–752. [Google Scholar]
- Busche, J.F.; Starke, G.; Knickmeier, S.; Dietzel, A. Controllable dry adhesion based on two-photon polymerization and replication molding for space debris removal. Micro Nano Eng. 2020, 7, 100052. [Google Scholar]
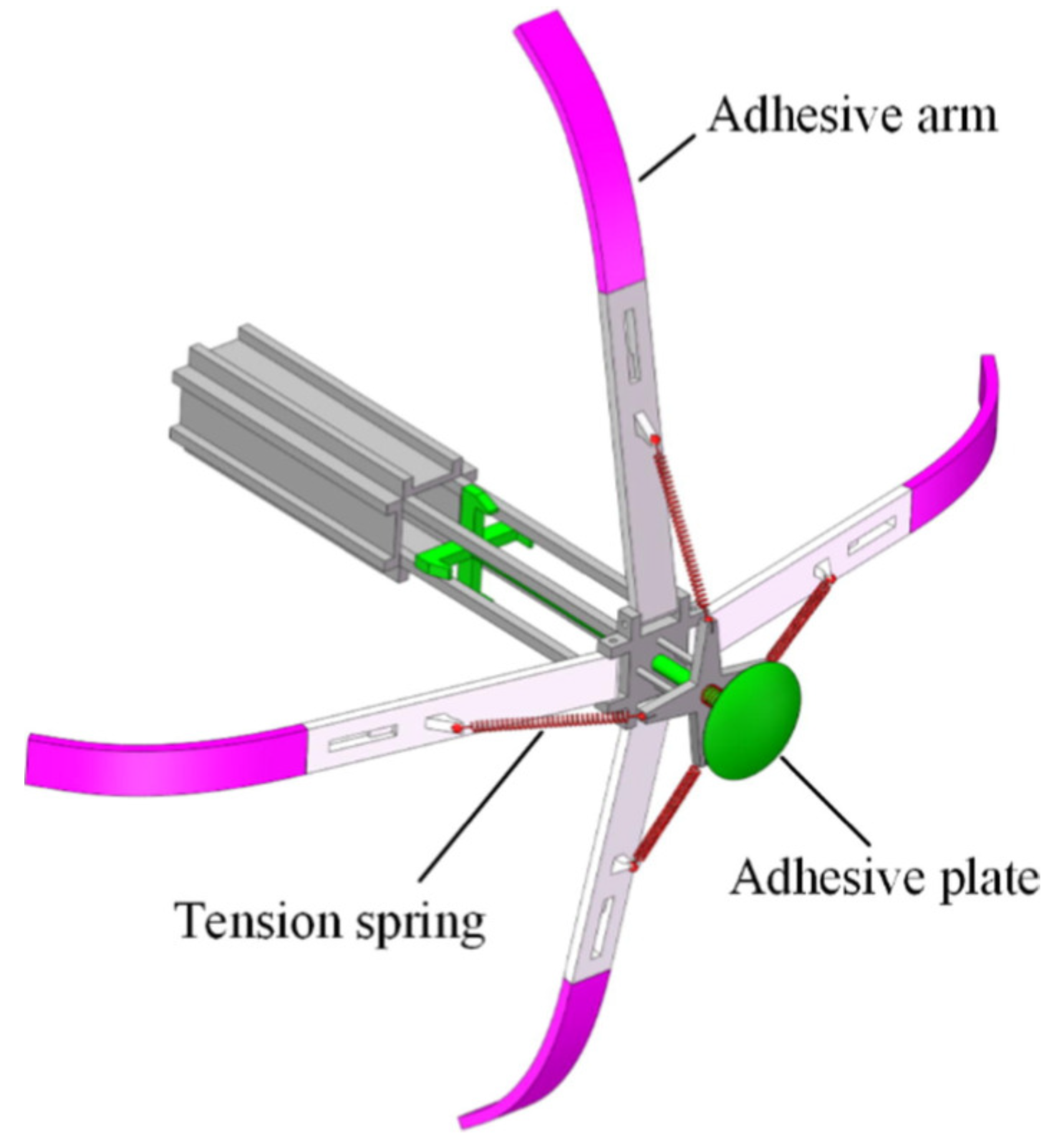
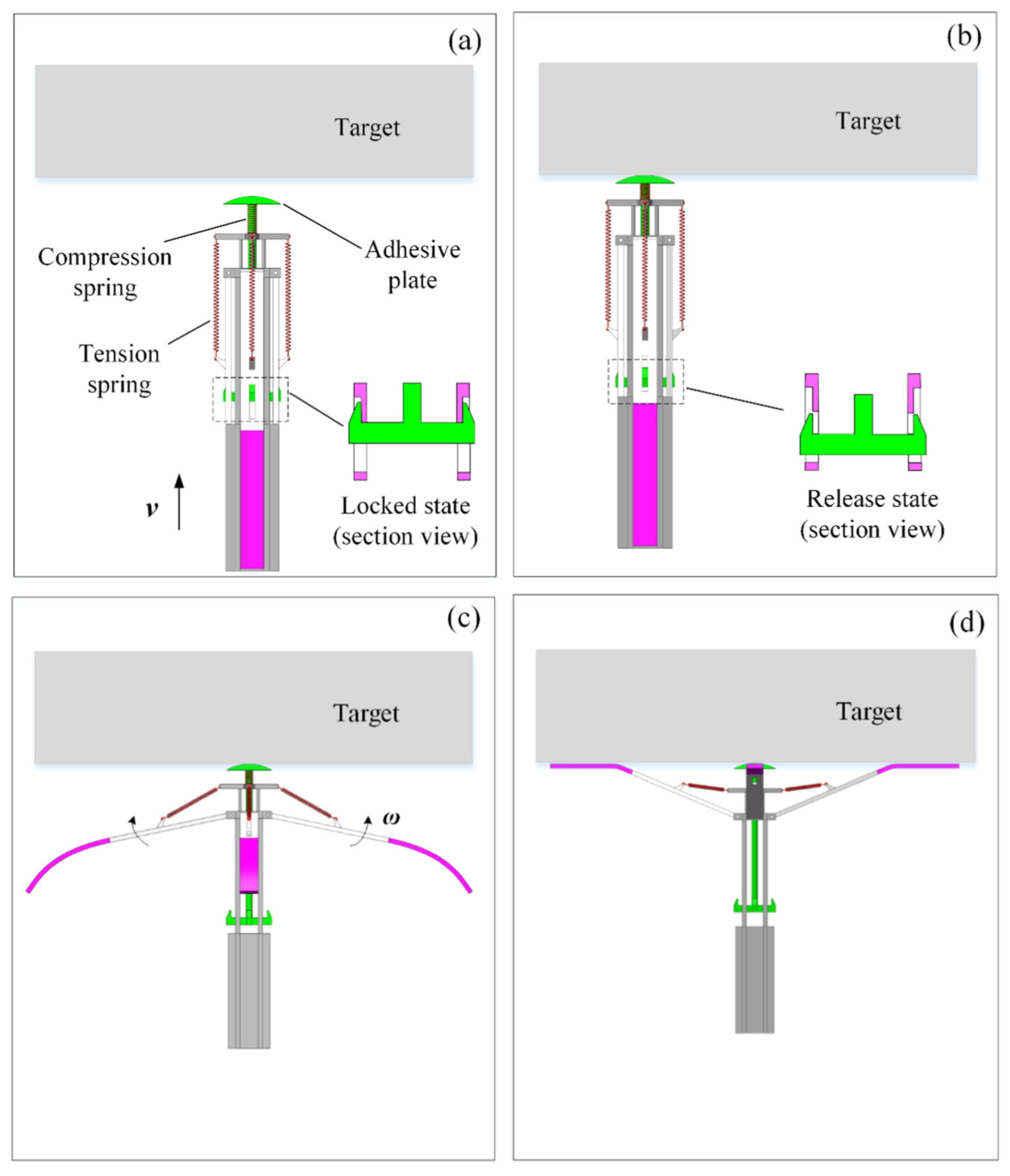
- Zhang, G.; Zhang, Q.; Feng, Z.; Chen, Q.; Yang, T. Dynamic modeling and simulation of a novel mechanism for adhesive capture of space debris. Adv. Space Res. 2021, 68, 3859–3874. [Google Scholar]
- Ben-Larbi, M.K.; Hensel, R.; Atzeni, G.; Arzt, E.; Stoll, E. Orbital debris removal using micropatterned dry adhesives: Review and recent advances. Prog. Aerosp. Sci. 2022, 134, 100850. [Google Scholar]
- Forshaw, J.L.; Aglietti, G.S.; Fellowes, S.; Salmon, T.; Retat, I.; Hall, A.; Chabot, T.; Pisseloup, A.; Tye, D.; Bernal, C.; et al. The active space debris removal mission RemoveDebris. Part 1: From concept to launch. Acta Astronaut. 2020, 168, 293–309. [Google Scholar] [CrossRef]
- Astroscale. Innovative Debris Removal, Astroscale ELSA-d Successfully Demonstrates Repeated Magnetic Capture; Astroscale: Tokyo, Japan, 2021. [Google Scholar]
- Shan, M.; Shi, L. Comparison of tethered post-capture system models for space debris removal. Aerospace 2022, 9, 33. [Google Scholar] [CrossRef]
- Huang, P.; Zhang, F.; Chen, L.; Meng, Z.; Zhang, Y.; Liu, Z.; Hu, Y. A review of space tether in new applications. Nonlinear Dyn. 2018, 94, 1–19. [Google Scholar]
- Jia, C.; Meng, Z.; Huang, P. Attitude control for tethered towing debris under actuators and dynamics uncertainty. Adv. Space Res. 2019, 64, 1286–1297. [Google Scholar]
- Wang, D.; Huang, P.; Cai, J.; Meng, Z. Coordinated control of tethered space robot using mobile tether attachment point in approaching phase. Adv. Space Res. 2014, 54, 1077–1091. [Google Scholar]
- Meng, Z.; Wang, B.; Huang, P.; Liu, Z. In-plane adaptive retrieval control for a noncooperative target by tethered space robots. Int. J. Adv. Robot. Syst. 2016, 13, 1729881416669485. [Google Scholar] [CrossRef]
- Bonnal, C.; Ruault, J.M.; Desjean, M.C. Active debris removal: Recent progress and current trends. Acta Astronaut. 2013, 85, 51–60. [Google Scholar]
- Lu, Y.; Huang, P.; Meng, Z.; Hu, Y.; Zhang, F.; Zhang, Y. Finite time attitude takeover control for combination via tethered space robot. Acta Astronaut. 2017, 136, 9–21. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, Z.; Zhang, Q.; Zeng, M.; Li, S. Dynamics and control of a tethered space-tug system using Takagi-Sugeno fuzzy methods. Aerosp. Sci. Technol. 2019, 87, 289–299. [Google Scholar] [CrossRef]
- Reed, J.; Barraclough, S. Development of harpoon system for capturing space debris. In Proceedings of the 6th European Conference on Space Debris, Darmstadt, Germany, 22–25 April 2013. [Google Scholar]
- Wayman, A.; Ratcliffe, A.; Barraclough, S.; Lurie, J.; Fernandez-Nunez, I.; Lupsa, M.; Aziz, S.; Wormnes, K.; Zwick, M.; Taylor, N.; et al. Design and testing of a full scale harpoon capture system. In Proceedings of the 7th European Space Debris Conference, Darmstadt, Germany, 18–21 April 2017. [Google Scholar]
- Lv, S.; Zhang, H.; Zhang, Y.; Ning, B.; Qi, R. Design of an integrated platform for active debris removal. Aerospace 2022, 9, 339. [Google Scholar] [CrossRef]
- Barnes, C.M.; Botta, E.M. A quality index for net-based capture of space debris. Acta Astronaut. 2020, 176, 455–463. [Google Scholar]
- Shan, M.; Guo, J.; Gill, E. Contact dynamic models of space debris capturing using a net. Acta Astronaut. 2019, 158, 198–205. [Google Scholar]
- Shan, M.; Guo, J.; Gill, E. Deployment dynamics of tethered-net for space debris removal. Acta Astronaut. 2017, 132, 293–302. [Google Scholar] [CrossRef]
- Shan, M.; Shi, L. Velocity-based detumbling strategy for a post-capture tethered net system. Adv. Space Res. 2022, 70, 1336–1350. [Google Scholar] [CrossRef]
- Trivailo, P.M.; Kojima, H. Dynamics of the net systems, capturing space debris. Trans. Jpn. Soc. Aeronaut. Space Sci. Aerosp. Technol. Jpn. 2016, 14, 57–66. [Google Scholar]
- Deghuria, A.; Debnath, R.; Varsha, G.; Raheja, K. Recent Advances in Space Debris Removal Techniques; A study. Preprints 2023. [Google Scholar] [CrossRef]
- Keidar, M.; Slotten, J. Self-Consuming Satellite. U.S. Patent 10,486,834, 26 November 2019. [Google Scholar]
- ESA. ESA DISCOS (Database and Information System Characterising Objects in Space); ESA: Paris, France, 2022. [Google Scholar]
- US Space Force. Current Catalog Files, LEO: Mean Motion > 11.25 and Eccentricity < 0.25. Accessed Data for Low Earth Orbit (LEO) Objects; US Space Force: Washington, DC, USA, 2022.
- U.S. Space Force. Databases Accessed on Space-Track.org. Accessed Databases Include Satellite Catalog Data; US Space Force: Washington, DC, USA, 2022.
- NASA. Debris Mitigation; Astromaterials Research and Exploration Science (ARES); NASA Orbital Debris Program Office: Houston, TX, USA.
- Ellery, A. Tutorial Review on Space Manipulators for Space Debris Mitigation. Robotics 2019, 8, 34. [Google Scholar] [CrossRef]
- Klinkrad, H.; Beltrami, P.; Hauptmann, S.; Martin, C.; Sdunnus, H.; Stokes, H.; Walker, R.; Wilkinson, J. The ESA Space Debris Mitigation Handbook 2002. Adv. Space Res. 2004, 34, 1251–1259. [Google Scholar]
- Council, N.R. Orbital Debris: A Technical Assessment; The National Academies Press: Washington, DC, USA, 1995. [Google Scholar]
- Hakima, H.; Bazzocchi, M.C. Low-thrust trajectory design for controlled deorbiting and reentry of space debris. In Proceedings of the 2021 IEEE Aerospace Conference (50100), Big Sky, MT, USA, 6–13 March 2021; IEEE: New York, NY, USA, 2021; pp. 1–10. [Google Scholar]
- Lidtke, A.A.; Lewis, H.G.; Armellin, R.; Urrutxua, H. Considering the collision probability of Active Debris Removal missions. Acta Astronaut. 2017, 131, 10–17. [Google Scholar]
- McKnight, B.S. Determination of breakup initial conditions. J. Spacecr. Rocket. 1991, 28, 470–477. [Google Scholar] [CrossRef]
- Yost, B.; Weston, S. State-of-the-Art Small Spacecraft Technology; Technical Report; NASA: Washington, DC, USA, 2024.
- Telaar, J.; Estable, S.; De Stefano, M.; Rackl, W.; Lampariello, R.; Ankersen, F.; Fernandez, J.G. Coupled control of chaser platform and robot arm for the e. deorbit mission. In Proceedings of the 10th International ESA conference on Guidance Navigation and Control Systems (GNC), Salzburg, Austria, 29 May–02 June 2017. [Google Scholar]
- Bischof, B. ROGER-Robotic geostationary orbit restorer. In Proceedings of the 54th International Astronautical Congress of the International Astronautical Federation, the International Academy of Astronautics, and the International Institute of Space Law, Bremen, Germany, 29 September–03 October 2003; p. IAA.5.2.08. [Google Scholar]
- Zinner, N.; Williamson, A.; Brenner, K.; Curran, J.; Isaak, A.; Knoch, M.; Leppek, A.; Lestishen, J. Junk hunter: Autonomous rendezvous, capture, and de-orbit of orbital debris. In Proceedings of the AIAA SPACE 2011 Conference and Exposition, Long Beach, CA, USA, 27–29 September 2011; p. 7292. [Google Scholar]
- Aglietti, G.S.; Taylor, B.; Fellowes, S.; Salmon, T.; Retat, I.; Hall, A.; Chabot, T.; Pisseloup, A.; Cox, C.; Zarkesh, A.; et al. The active space debris removal mission RemoveDebris. Part 2: In orbit operations. Acta Astronaut. 2020, 168, 310–322. [Google Scholar]
- Dupont, C.; Paris, C.; Missonnier, S.; Rommelaere, S.; Bonnal, C.; Directorate, C.L. Just-in-time Collision Avoidance mission: Reactive system for braking space debris. In Proceedings of the 8th European Conference for Aeronautics and Space Sciences, Madrid, Spain, 1–4 July 2019; 724. [Google Scholar]
- Jarry, A.; Bonnal, C.; Dupont, C.; Missonnier, S.; Lequette, L.; Masson, F. SRM plume: A candidate as space debris braking system for Just-In-Time Collision avoidance maneuver. Acta Astronaut. 2019, 158, 185–197. [Google Scholar]
- Bonnal, C.; McKnight, D.; Phipps, C.; Dupont, C.; Missonnier, S.; Lequette, L.; Merle, M.; Rommelaere, S. Just in time collision avoidance–A review. Acta Astronaut. 2020, 170, 637–651. [Google Scholar] [CrossRef]
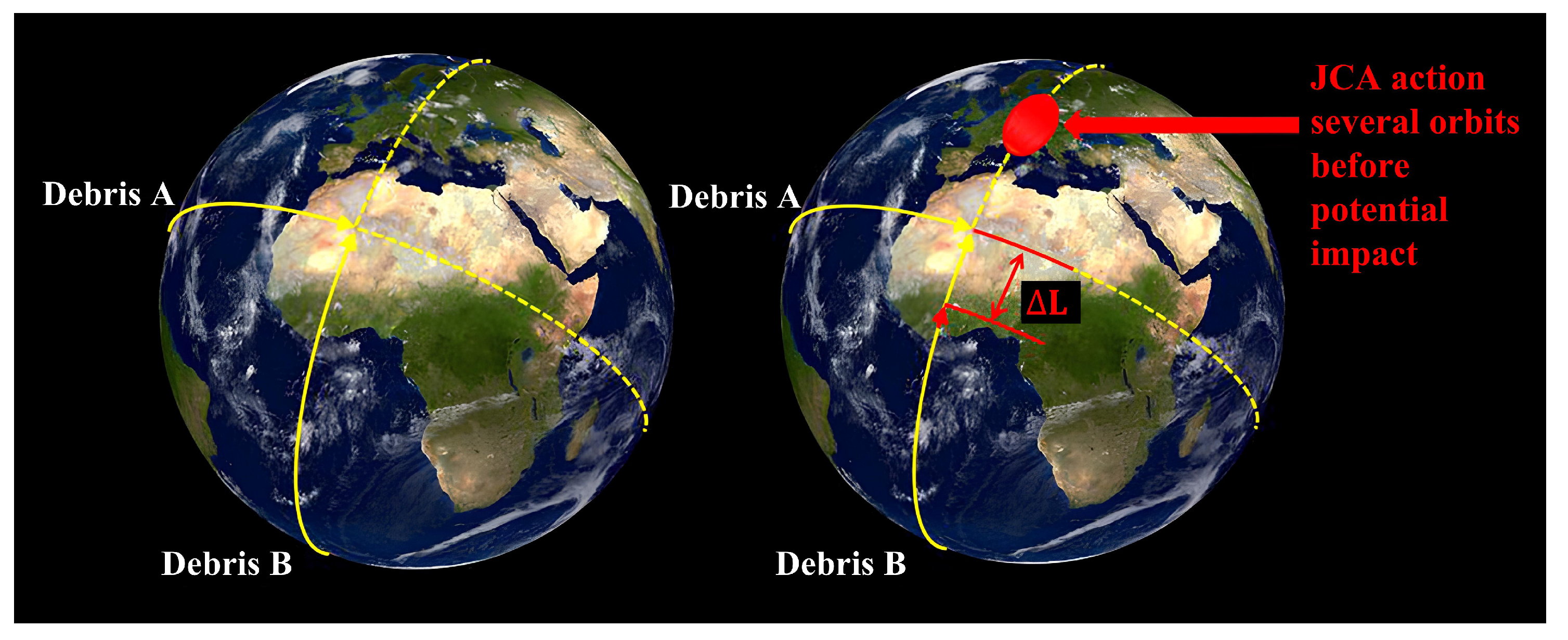
- Bonnal, C.; Dupont, C.; Missonnier, S.; Lequette, L.; Merle, M.; Rommelaere, S. Just-in-time Collision Avoidance (JCA) using a cloud of particles. In Proceedings of the First International Orbital Debris Conference, Sugar Land, TX, USA, 9–12 December 2019; p. 6062. [Google Scholar]
- Phipps, C.R.; Bonnal, C. A spaceborne, pulsed UV laser system for re-entering or nudging LEO debris, and re-orbiting GEO debris. Acta Astronaut. 2016, 118, 224–236. [Google Scholar]
- Klinkrad, H.; Johnson, N.L. Space debris environment remediation concepts. In Proceedings of the 5th European Conference on Space Debris, Darmstadt, Germany, 30 March–2 April 2009. [Google Scholar]
- Stadnyk, K.; Ulrich, S. Validating the Deployment of a Novel Tether Design for Net-Based Orbital Debris Removal Missions. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; p. 0719. [Google Scholar]
- Eringen, A.C. Mechanics of Continua; Krieger Pub Co.: Malabar, FL, USA, 1980. [Google Scholar]
- Ru, M.; Zhan, Y.; Cheng, B.; Zhang, Y. Capture Dynamics and Control of a Flexible Net for Space Debris Removal. Aerospace 2022, 9, 299. [Google Scholar] [CrossRef]
- Shan, M.; Guo, J.; Gill, E. Contact dynamics on net capturing of tumbling space debris. J. Guid. Control. Dyn. 2018, 41, 2063–2072. [Google Scholar] [CrossRef]
- Shabana, A. Dynamics of Multibody Systems; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Shan, M.; Guo, J.; Gill, E. An analysis of the flexibility modeling of a net for space debris removal. Adv. Space Res. 2020, 65, 1083–1094. [Google Scholar] [CrossRef]
- Kim, E.; Cho, M. Design of a planar multibody dynamic system with ANCF beam elements based on an element-wise stiffness evaluation procedure. Struct. Multidiscip. Optim. 2018, 58, 1095–1107. [Google Scholar] [CrossRef]
- Skrinjar, L.; Slavič, J.; Boltežar, M. A review of continuous contact-force models in multibody dynamics. Int. J. Mech. Sci. 2018, 145, 171–187. [Google Scholar] [CrossRef]
- Si, J.; Pang, Z.; Du, Z.; Cheng, C. Dynamics modeling and simulation of self-collision of tether-net for space debris removal. Adv. Space Res. 2019, 64, 1675–1687. [Google Scholar] [CrossRef]
- Botta, E.M.; Sharf, I.; Misra, A.K. Simulation of tether-nets for capture of space debris and small asteroids. Acta Astronaut. 2019, 155, 448–461. [Google Scholar] [CrossRef]
- Shan, M. Net Deployment and Contact Dynamics of Capturing Space Debris Objects. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2018. [Google Scholar]
- Botta, E.M.; Sharf, I.; Misra, A.K.; Teichmann, M. On the simulation of tether-nets for space debris capture with Vortex Dynamics. Acta Astronaut. 2016, 123, 91–102. [Google Scholar] [CrossRef]
- Jiménez, P.; Thomas, F.; Torras, C. 3D collision detection: A survey. Comput. Graph. 2001, 25, 269–285. [Google Scholar] [CrossRef]
- Mirtich, B.V. Impulse-Based Dynamic Simulation of Rigid Body Systems; University of California: Berkeley, CA, USA, 1996. [Google Scholar]
- Gilardi, G.; Sharf, I. Literature survey of contact dynamics modelling. Mech. Mach. Theory 2002, 37, 1213–1239. [Google Scholar] [CrossRef]
- Si, J.; Pang, Z.; Du, Z.; Fu, J. Dynamics modeling and simulation of a net closing mechanism for tether-net capture. Int. J. Aerosp. Eng. 2021, 2021, 8827141. [Google Scholar] [CrossRef]
- Xu, B.; Yang, Y.; Zhang, B.; Yan, Y.; Yi, Z. Bionic design and experimental study for the space flexible webs capture system. IEEE Access 2020, 8, 45411–45420. [Google Scholar] [CrossRef]
- Sharf, I.; Thomsen, B.; Botta, E.M.; Misra, A.K. Experiments and simulation of a net closing mechanism for tether-net capture of space debris. Acta Astronaut. 2017, 139, 332–343. [Google Scholar] [CrossRef]
- Gołębiowski, W.; Michalczyk, R.; Dyrek, M.; Battista, U.; Wormnes, K. Validated simulator for space debris removal with nets and other flexible tethers applications. Acta Astronaut. 2016, 129, 229–240. [Google Scholar]
- Inter-Agency Space Debris Coordination Committee (IADC). IADC Space Debris Mitigation Guidelines, Revision 3; Inter-Agency Space Debris Coordination Committee (IADC): Darmstadt, Germany, 2021.
- NASA, U.S. Government Orbital Debris Mitigation Standard Practices; NASA: Washington, DC, USA, 2000. [Google Scholar]
- Migaud, M.R. Protecting Earth’s Orbital Environment: Policy Tools for Combating Space Debris. Space Policy 2020, 52, 101361. [Google Scholar] [CrossRef]
- Federal Aviation Administration (FAA). Launch Activity and Orbital Debris Mitigation; Second Quarter 2002 Quarterly Launch Report; Federal Aviation Administration (FAA): Washington, DC, USA, 2002; pp. 8–13.
- Kensinger, K.; Duall, S.; Persaud, S. The United States Federal Communication Commission’s Regulations Concerning Mitigation of Orbital Debris. In Proceedings of the 4th European Conference on Space Debris, Darmstadt, Germany, 18–20 April 2005. [Google Scholar]























Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bigdeli, M.; Srivastava, R.; Scaraggi, M. Mechanics of Space Debris Removal: A Review. Aerospace 2025, 12, 277. https://doi.org/10.3390/aerospace12040277
Bigdeli M, Srivastava R, Scaraggi M. Mechanics of Space Debris Removal: A Review. Aerospace. 2025; 12(4):277. https://doi.org/10.3390/aerospace12040277
Chicago/Turabian StyleBigdeli, Mohammad, Rajat Srivastava, and Michele Scaraggi. 2025. "Mechanics of Space Debris Removal: A Review" Aerospace 12, no. 4: 277. https://doi.org/10.3390/aerospace12040277
APA StyleBigdeli, M., Srivastava, R., & Scaraggi, M. (2025). Mechanics of Space Debris Removal: A Review. Aerospace, 12(4), 277. https://doi.org/10.3390/aerospace12040277







