Investigation of the Thermal Vibration Behavior of an Orthogonal Woven Composite Nozzle Based on RVE Analysis
Abstract
1. Introduction
2. Simulation Model
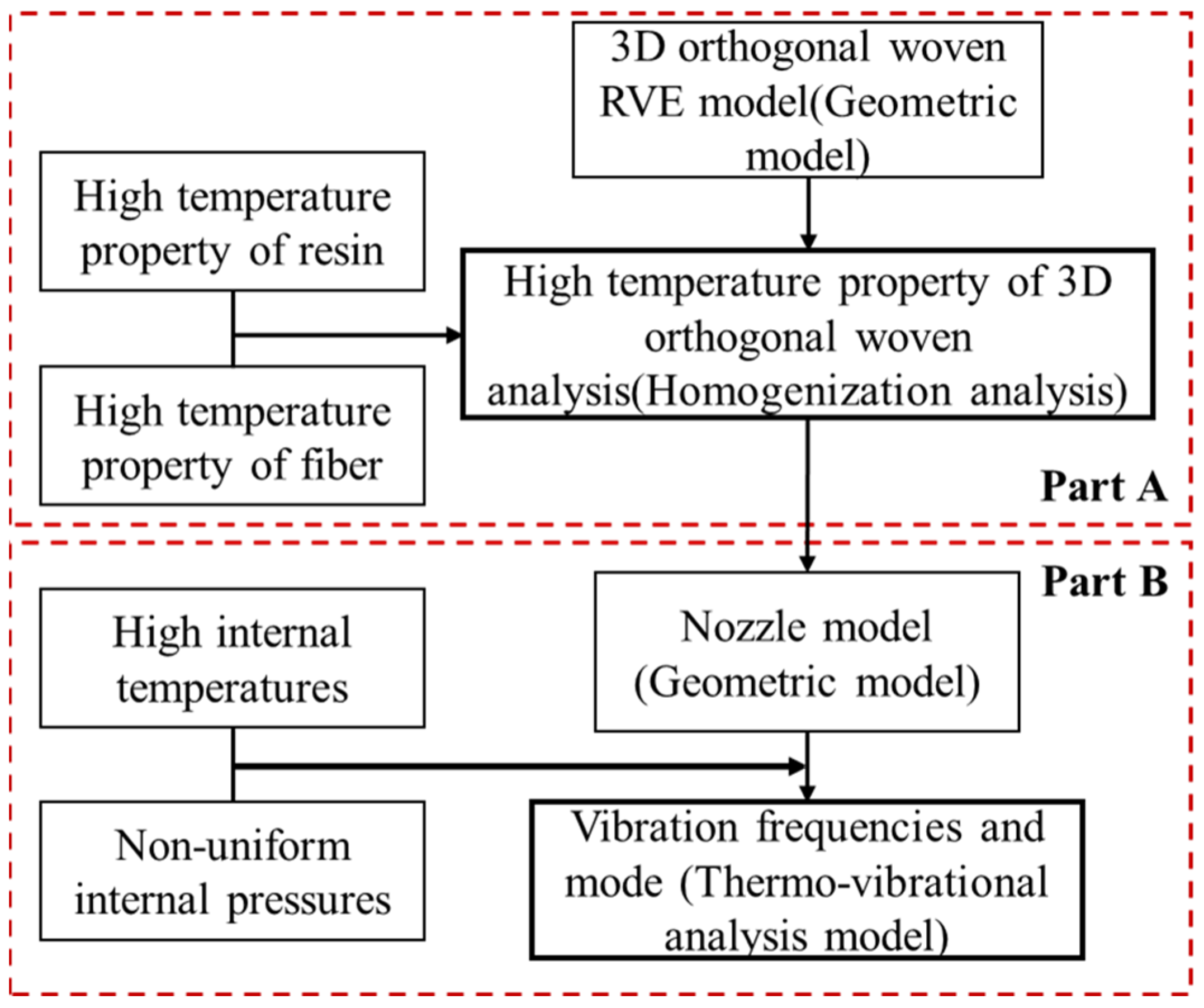
2.1. Thermo-Vibrational Coupling Analysis Process
2.2. Thermo-Vibrational Analysis Model of Composite Nozzle
2.3. RVE of 3D Orthogonal Woven Composites
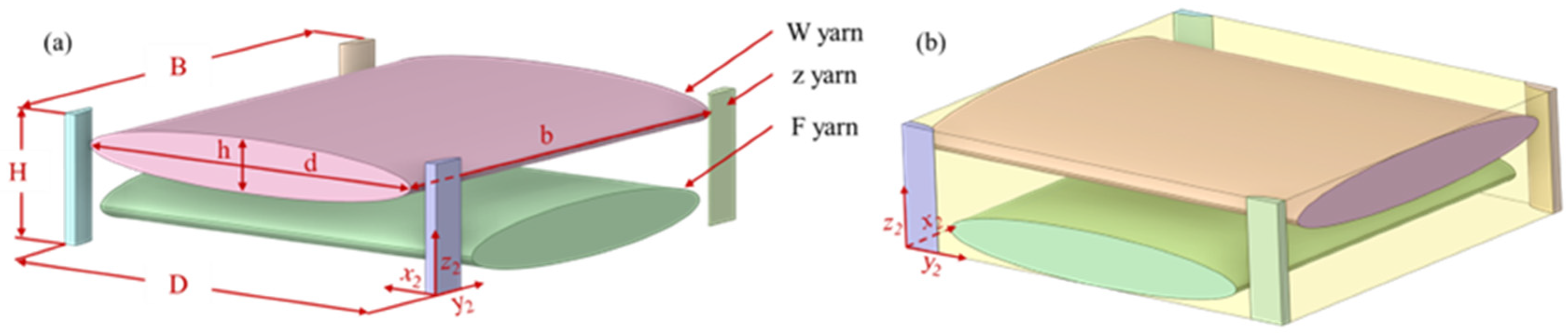
2.3.1. Geometric Model of RVE
2.3.2. RVE Simulation
3. Results
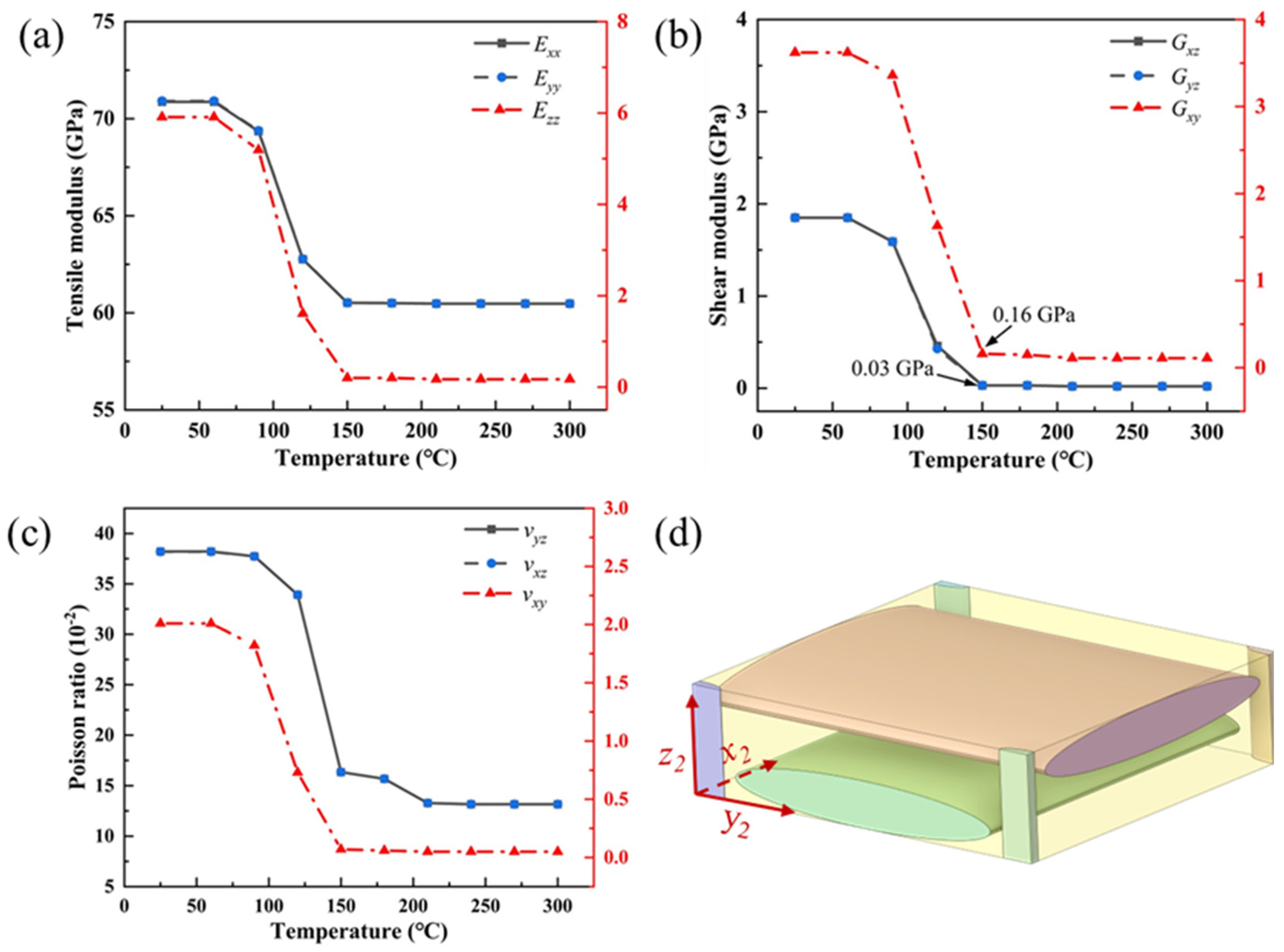
3.1. Material Properties Change with Temperature
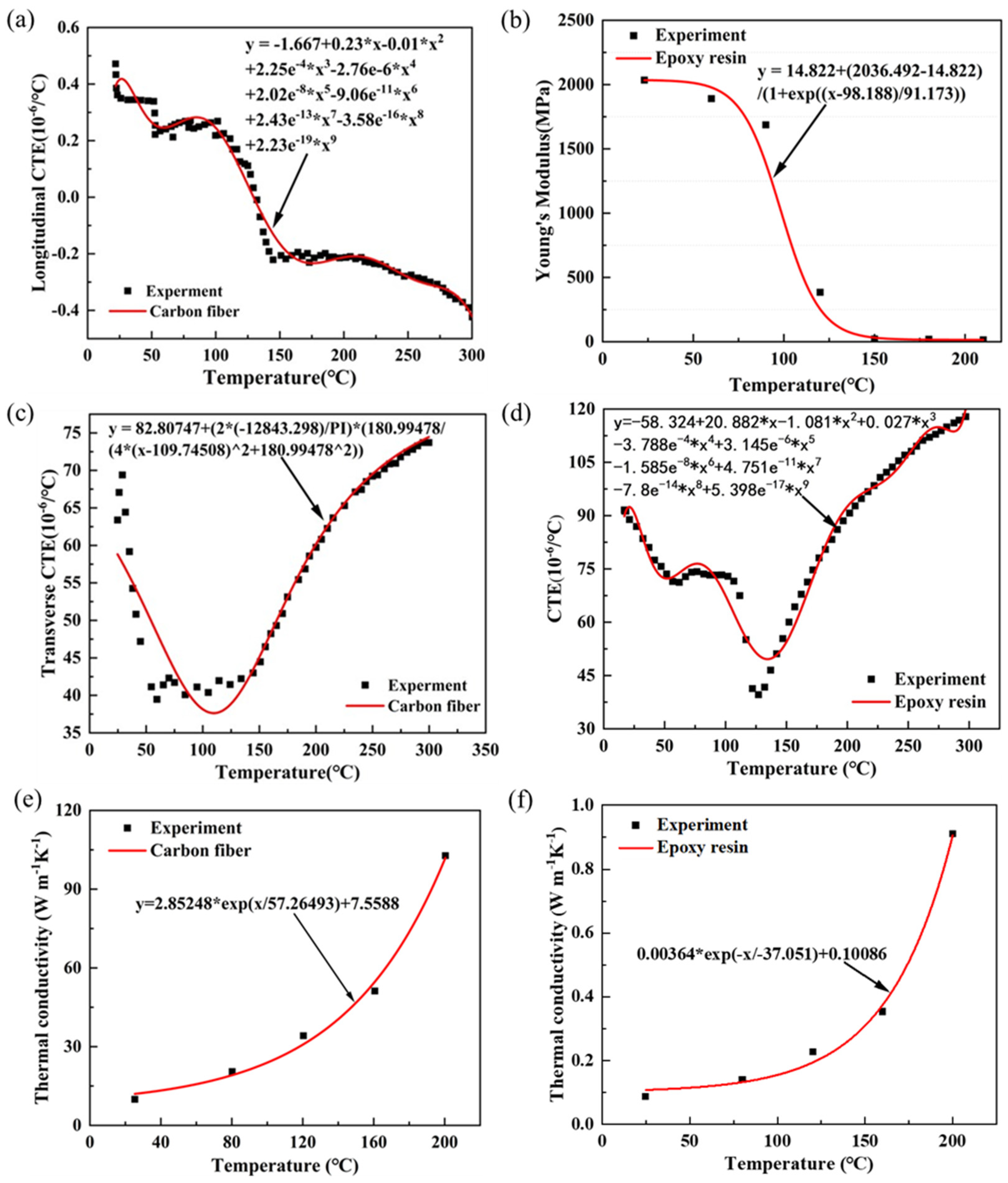
3.1.1. High-Temperature Properties of Fibers and Resins
3.1.2. RVE of the 3D Orthogonal Woven Composites at High Temperatures
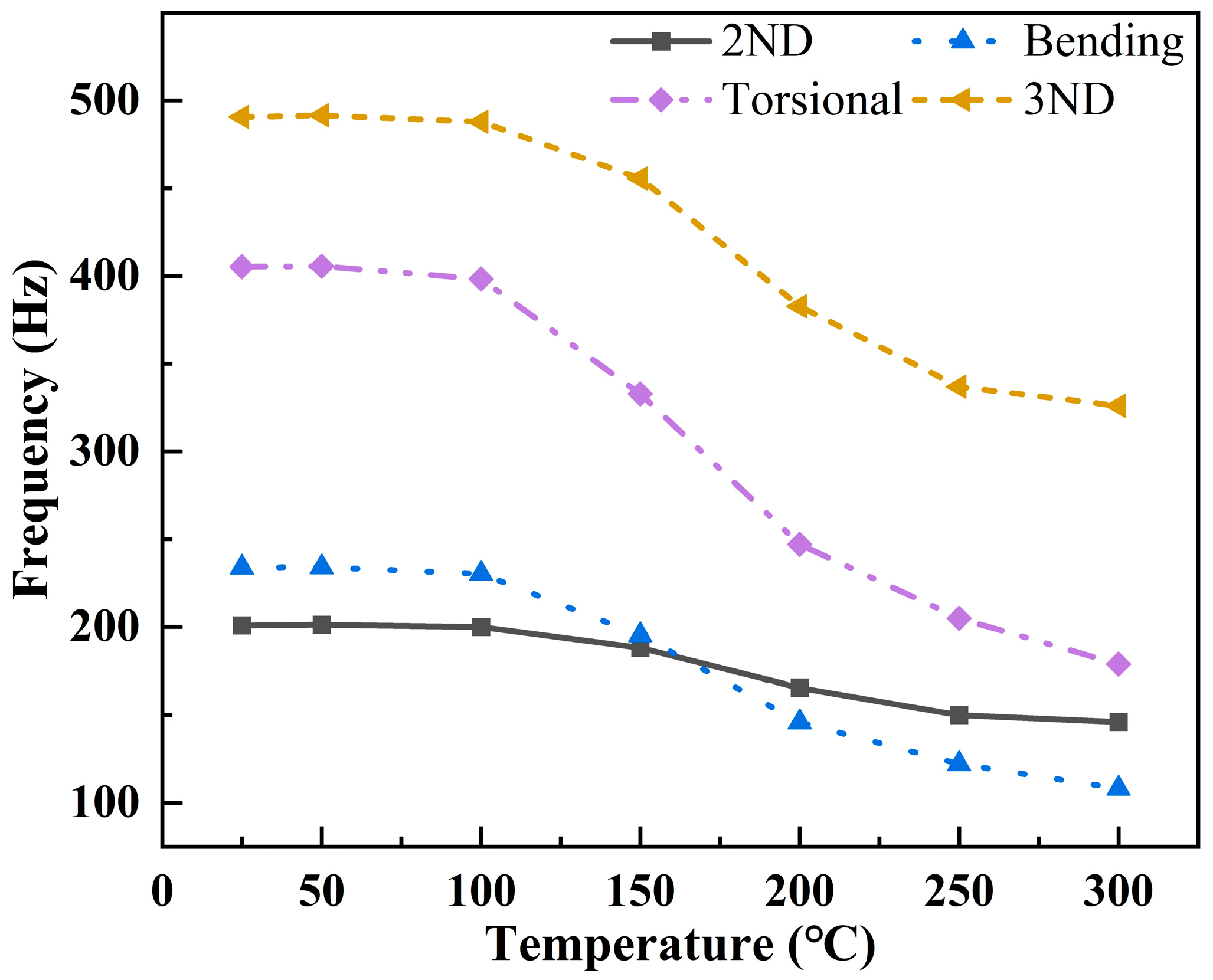
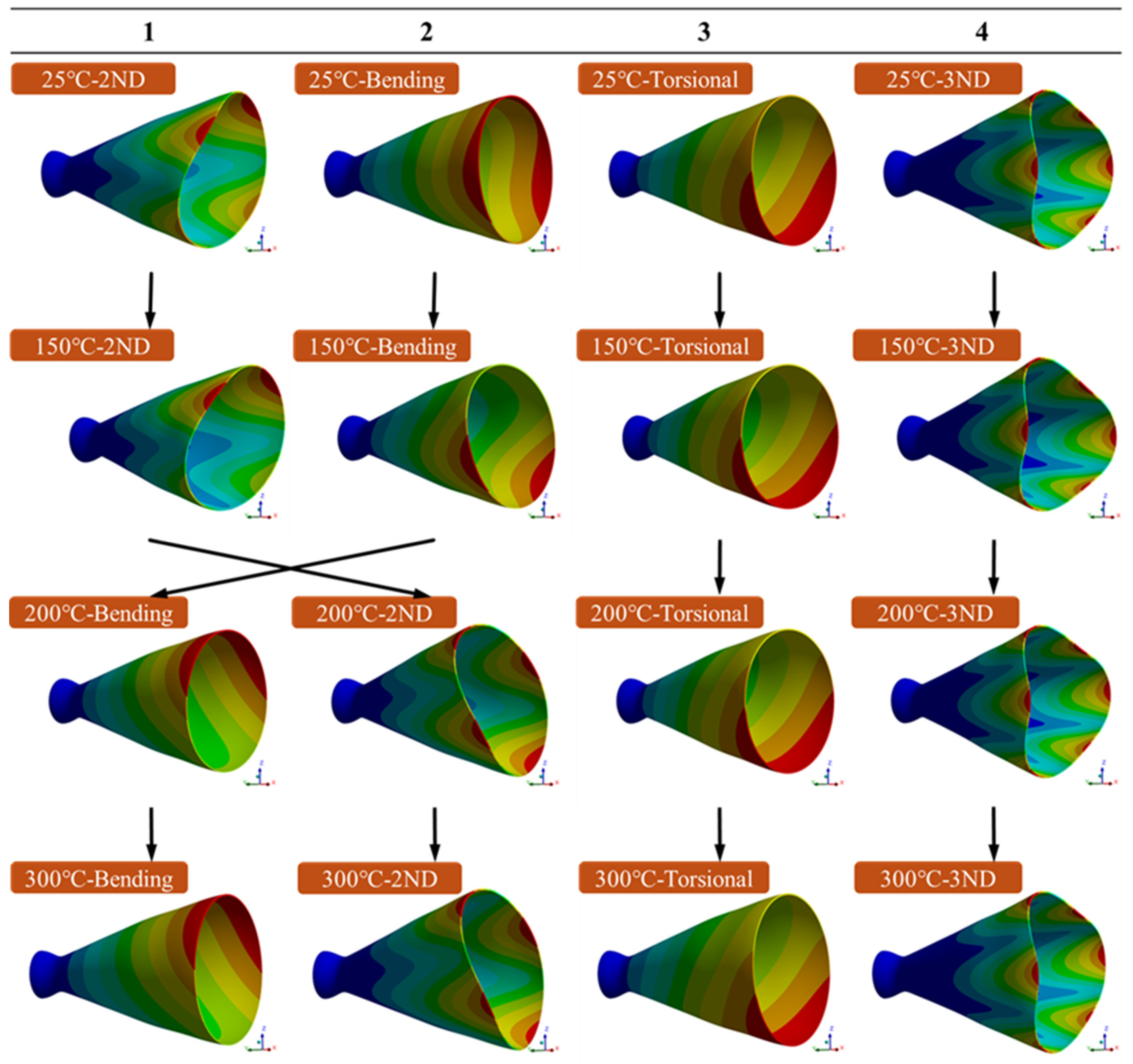
3.2. Modal Analysis of the Nozzle at High Temperature
3.3. Modal Analysis of the Nozzle with Non-Uniform Internal Pressure
4. Discussion
4.1. Material Properties from RVE Analysis at High Temperature
4.2. Vibration Behavior of the Composite Nozzle
4.3. Vibration Frequency and Modal Changes at High Temperatures
4.4. Vibration Frequency and Modal Changes with Non-Uniform Internal Pressures
5. Conclusions
- The rise in temperature of the composite nozzle‘s inner wall leads to material modulus loss and thermal stress intensification, causing a reduction in the frequencies of the first four mode orders. Under the dominant influence of thermal stress, the torsional mode, which is sensitive to shear modulus loss, exhibits a greater frequency drop. When the inner wall temperature reaches 300 °C, the 2ND and 3ND modes decrease by an average of 30.39%, while the bending and torsional modes drop by an average of 54.80%. The torsional modal frequency, in particular, drops from 405.26 Hz to 178.77 Hz, reflecting a 55.89% reduction compared to the frequency at 25 °C.
- The introduction of non-uniform internal pressure enhances the stiffness of the composite nozzle in the xz-plane, causing the frequencies of the 2ND and 3ND modes to increase by an average of 17.89% and 7.96%, respectively. However, under the dominant influence of thermal stress, the overall frequency of the first four mode orders still exhibits a downward trend. As the inner wall temperature of the nozzle rises to 300 °C, and the torsional modal frequency drops from 404.68 Hz to 177.01 Hz, reflecting a 56.26% decrease relative to its frequency at 25 °C. This trend aligns with the case without internal pressure. Meanwhile, the average frequency drop of the 2ND and 3ND modes is 27.6%.
- An inner wall temperature of 150 °C leads to a modal interchange between the bending mode and the 2ND mode due to the decreased shear modulus (i.e., decreased by 96.96% from 25 °C to 150 °C). Introducing non-uniform internal pressure loads on the inner wall of the composite nozzle reduces the modal shifting temperature to approximately 50 °C. It is likely because the combined effect of rising temperatures and decreasing modulus lowers the composite nozzle’s frequency, while non-uniform internal pressure increases the 2ND and 3ND modal frequencies.
- The RVE of the 3D orthogonal woven composite, constructed based on homogenization theory, shows that the material properties and shear modulus in the z-direction are primarily influenced by the performance of the epoxy resin. At a nozzle inner wall temperature of 300 °C, the 3D orthogonal woven composite experiences a loss of 98.27% in shear modulus and 97.12% in , consistent with the thermal response pattern of pure epoxy resin. Meanwhile, the properties in the x and y directions of the composite are predominantly governed by carbon fiber.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Young’s modulus of fiber in direction 1, GPa | Young’s modulus of resin in direction 1, GPa | ||
| Young’s modulus of fiber in direction 2 and 3, GPa | Young’s modulus of resin in direction 2 and 3, GPa | ||
| Shear modulus of fiber in 12-plane and 23-plane, GPa | Shear modulus of resin in 12-plane and 23-plane, GPa | ||
| Shear modulus of fiber in 23-plane, GPa | Shear modulus of resin in 23-plane, GPa | ||
| Poisson ratio of fiber in 12-plane and 13-plane | Poisson ratio of resin in 12-plane and 13-plane | ||
| Poisson ratio of fiber in 23-plane | Poisson ratio of resin in 23-plane | ||
| CTE of fiber in direction 1, 10−6/℃ | CTE of resin in direction 1, 10−6/℃ | ||
| CTE of fiber in direction 2 and direction 3, 10−6/℃ | CTE of resin in direction 2 and direction 3, 10−6/℃ | ||
| m−3 | m−3 | ||
| (m−1 k−1) | (m−1 k−1) | ||
| (m−1 k−1) | (m−1 k−1) | ||
| Young’s modulus of 3D orthogonal woven composite in direction x, GPa | (m−1 k−1) | ||
| Young’s modulus of 3D orthogonal woven composite in direction y, GPa | (m−1 k−1) | ||
| Young’s modulus of 3D orthogonal woven composite in direction z, GPa | (m−1 k−1) | ||
| Shear modulus of 3D orthogonal woven composite in xy-plane, GPa | CTE of 3D orthogonal woven composite in direction x, 10−6/℃ | ||
| Shear modulus of 3D orthogonal woven composite in xz-plane, GPa | CTE of 3D orthogonal woven composite in direction y, 10−6/℃ | ||
| Shear modulus of 3D orthogonal woven composite in yz-plane, GPa | CTE of 3D orthogonal woven composite in direction z, 10−6/℃ | ||
| Poisson ratio of 3D orthogonal woven composite in xy-plane | |||
| Poisson ratio of 3D orthogonal woven composite in xz-plane | |||
| Poisson ratio of 3D orthogonal woven composite in yz-plane |
References
- Gu, X.; Dong, L.; Li, T.; Yang, W. A Study on Influence of Flapping Dynamic Characteristics on Vibration Control of Active Rotor with Trailing-Edge Flaps. Aerospace 2023, 10, 776. [Google Scholar] [CrossRef]
- Xu, F.; Li, H.; Zhang, D. A Study on Dynamic Characteristics of Thin-Walled Cylindrical Cavities with a Large Aspect Ratio. Aerospace 2022, 9, 174. [Google Scholar] [CrossRef]
- Garelli, L.; Paz, R.R.; Storti, M.A. Fluid–Structure Interaction Study of the Start-up of a Rocket Engine Nozzle. Comput. Fluids 2010, 39, 1208–1218. [Google Scholar] [CrossRef]
- Wang, T.-S.; Zhao, X.; Zhang, S.; Chen, Y.-S. Development of an Aeroelastic Modeling Capability for Transient Nozzle Flow Analysis. J. Propul. Power 2014, 30, 1692–1700. [Google Scholar] [CrossRef]
- Zhang, J.A.; Shotorban, B.; Zhang, S. Numerical Experiment of Aeroelastic Stability for a Rocket Nozzle. J. Aerosp. Eng. 2017, 30, 04017041. [Google Scholar] [CrossRef]
- Yan, S.; Li, B.; Li, F.; Li, B. Finite Element Model Updating of Liquid Rocket Engine Nozzle Based on Modal Test Results Obtained from 3-D SLDV Technique. Aerosp. Sci. Technol. 2017, 69, 412–418. [Google Scholar] [CrossRef]
- Eitner, M.A.; Sirohi, J.; Tinney, C.E. Modal parameter estimation of a reduced-scale rocket nozzle using blind source separation. Meas. Sci. Technol. 2019, 30, 095401. [Google Scholar] [CrossRef]
- Chen, Y.; Jin, L.; Tang, X.; Huang, D.; Zhang, J. Dynamic Response of a Composite Fan Blade Excited Instantaneously by Multiple MFC Actuators. Aerospace 2022, 9, 301. [Google Scholar] [CrossRef]
- Guo, Y.; Guo, Y.; Zhang, Y.; Li, L.; Zhang, D.; Chen, S.; Eltaher, M.A. Thermally Induced Vibration of a Flexible Plate with Enhanced Active Constrained Layer Damping. Aerospace 2024, 11, 504. [Google Scholar] [CrossRef]
- Sha, Y.; Zhao, W.; Tang, X.; Zhao, F. Acoustic and Vibration Response and Fatigue Life Analysis of Thin-Walled Connection Structures under Heat Flow Conditions. Aerospace 2024, 11, 287. [Google Scholar] [CrossRef]
- Santos Silva, A.C.; Sebastian, C.M.; Lambros, J.; Patterson, E.A. High Temperature Modal Analysis of a Non-Uniformly Heated Rectangular Plate: Experiments and Simulations. J. Sound Vib. 2019, 443, 397–410. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, Y.; Xiao, D. Experimental Investigations of Thermal Modal Parameters for a C/SiC Structure under 1600 °C High Temperature Environment. Measurement 2020, 151, 107094. [Google Scholar] [CrossRef]
- Wu, D.; Wang, Y.; Shang, L.; Wang, H.; Pu, Y. Experimental and Computational Investigations of Thermal Modal Parameters for a Plate-Structure under 1200 °C High Temperature Environment. Measurement 2016, 94, 80–91. [Google Scholar] [CrossRef]
- Gao, Y.; Li, J. Effects of Braiding Angle on Modal Experimental Analysis of Three-Dimensional and Five-Directional Braided Composites. Compos. Part B Eng. 2012, 43, 2423–2428. [Google Scholar] [CrossRef]
- Pei, X.; Li, J.; Chen, K.; Ding, G. Vibration Modal Analysis of Three-Dimensional and Four Directional Braided Composites. Compos. Part B Eng. 2015, 69, 212–221. [Google Scholar] [CrossRef]
- Singh, D.B.; Singh, B.N. New Higher Order Shear Deformation Theories for Free Vibration and Buckling Analysis of Laminated and Braided Composite Plates. Int. J. Mech. Sci. 2017, 131–132, 265–277. [Google Scholar] [CrossRef]
- Huang, X.-R.; Zhu, H.; Li, D.; Jiang, L. Multi-Scale Sensitivity Analysis of Structural Vibration Behaviors of Three-Dimensional Braided Composites with Respect to Material Properties. Mech. Mater. 2020, 144, 103301. [Google Scholar] [CrossRef]
- Dan, L.; Yifeng, Z.; Senbiao, X.; Zheng, S. Static, Buckling, and Free-Vibration Analysis of Plain-Woven Composite Plate with Finite Thickness Using VAM-Based Equivalent Model. Thin-Walled Struct. 2021, 169, 108503. [Google Scholar]
- Yoo, J.-S.; Cho, I.-H.; Kim, C.-G. Thermoelastic Analysis of a Kick Motor Nozzle Incorporating Spatially Reinforced Composites. J. Spacecr. Rockets 2003, 40, 83–91. [Google Scholar] [CrossRef]
- Li, L.Y.; Wen, P.H.; Aliabadi, M.H. Meshfree Modeling and Homogenization of 3D Orthogonal Woven Composites. Compos. Sci. Technol. 2011, 71, 1777–1788. [Google Scholar] [CrossRef]
- Guo, J.; Wen, W.; Zhang, H.; Cui, H. Warp-Loaded Mechanical Performance of 3D Orthogonal Layer-to-Layer Woven Composite Perforated Structures with Different Apertures. Compos. Struct. 2021, 278, 114720. [Google Scholar] [CrossRef]
- Green, S.D.; Matveev, M.Y.; Long, A.C.; Ivanov, D. Mechanical Modelling of 3D Woven Composites Considering Realistic Unit Cell Geometry. Compos. Struct. 2014, 118, 284–293. [Google Scholar] [CrossRef]
- Dong, K.; Zhang, J.; Jin, L.; Gu, B.; Sun, B. Multi-Scale Finite Element Analyses on the Thermal Conductive Behaviors of 3D Braided Composites. Compos. Struct. 2016, 143, 9–22. [Google Scholar] [CrossRef]
- Dong, K.; Zhang, J.; Cao, M.; Wang, M.; Gu, B.; Sun, B. A Mesoscale Study of Thermal Expansion Behaviors of Epoxy Resin and Carbon Fiber/Epoxy Unidirectional Composites Based on Periodic Temperature and Displacement Boundary Conditions. Polym. Test. 2016, 55, 44–60. [Google Scholar] [CrossRef]
- Ruan, R.; Ye, L.; Feng, H.; Xu, L.-H.; Wang, Y. High Temperature Evolution of the Microstructure in the Radial Direction of PAN-Based Carbon Fibers and Its Relationship to Mechanical Properties. New Carbon Mater. 2020, 35, 295–306. [Google Scholar] [CrossRef]
- Ruan, C.; Lv, J.; Zu, L.; Liu, L.; Mei, H. Prediction of Thermo-Mechanical Properties of 8-Harness Satin-Woven C/C Composites by Asymptotic Homogenization. Materials 2024, 17, 1284. [Google Scholar] [CrossRef] [PubMed]
- Wei, X.; Chen, W.; Chen, L.; Wu, Q.; Xin, Y. Investigation of Temperature Effect on Thermo-Mechanical Property of Carbon Fiber/PEEK Composites. Rev. Adv. Mater. Sci. 2024, 63, 20240069. [Google Scholar] [CrossRef]
- Nema, A.; Mallineni, C.N.; Penumakala, P.K.; Adusumalli, R.; K, T.; Buragohain, M.K. Effect of Temperature on Flexural and Interlaminar Shear Strength Properties of Carbon-Epoxy Composites: Experiment and Modeling. Polym. Compos. 2024, 45, 9139–9155. [Google Scholar] [CrossRef]
- Pan, Z.; Gu, B.; Sun, B. Numerical Analyses of Thermo-Mechanical Behaviors of 3-D Rectangular Braided Composite under Different Temperatures. J. Text. Inst. 2015, 106, 173–186. [Google Scholar] [CrossRef]
- Bogdanovich, A.E. Multi-Scale Modeling, Stress and Failure Analyses of 3-D Woven Composites. J. Mater. Sci. 2006, 41, 6547–6590. [Google Scholar] [CrossRef]
- Mead, D.J. Vibration and Buckling of Flat Free–Free Plates under Non-Uniform in-Plane Thermal Stresses. J. Sound Vib. 2003, 260, 141–165. [Google Scholar] [CrossRef]
- Bai, Y.; Yu, K.; Zhao, J.; Zhao, R. Experimental and Simulation Investigation of Temperature Effects on Modal Characteristics of Composite Honeycomb Structure. Compos. Struct. 2018, 201, 816–827. [Google Scholar] [CrossRef]
- Burger, N.; Laachachi, A.; Ferriol, M.; Lutz, M.; Toniazzo, V.; Ruch, D. Review of Thermal Conductivity in Composites: Mechanisms, Parameters and Theory. Prog. Polym. Sci. 2016, 61, 1–28. [Google Scholar] [CrossRef]
- Kim, S.; Cho, H.; Joo, H.; Shin, S.; Kwak, J. Equivalent Structural Modeling Using Laminated Composite Shell Analysis for the Nozzle Component of a Launch Vehicle Engine. J. Aerosp. Eng. 2018, 31, 04018078. [Google Scholar] [CrossRef]
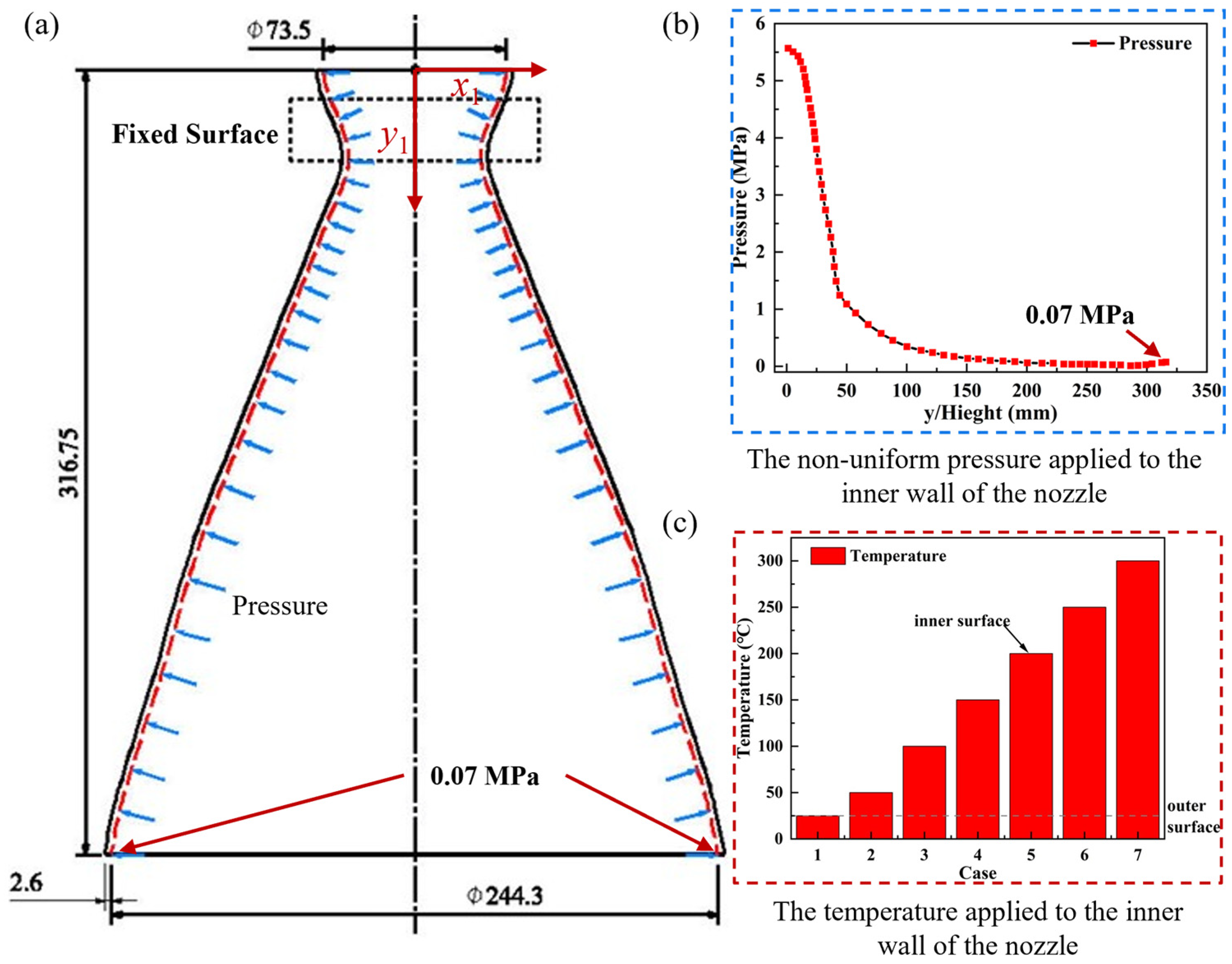



| Inner Wall Temperature | Outer Wall Temperature | |
|---|---|---|
| Case 1 | 25 °C | 25 °C |
| Case 2 | 50 °C | 25 °C |
| Case 3 | 100 °C | 25 °C |
| Case 4 | 150 °C | 25 °C |
| Case 5 | 200 °C | 25 °C |
| Case 6 | 250 °C | 25 °C |
| Case 7 | 300 °C | 25 °C |
| Unit mm | W Yarn | F Yarn | z Yarn | B 4 | D 5 | H 6 |
|---|---|---|---|---|---|---|
| d 1 | 4.33 | 4.17 | 0.91 | 5.02 | 5.48 | 1.45 |
| b 2 | 5.48 | 5.02 | 1.45 | |||
| h 3 | 0.62 | 0.64 | 0.29 |
| Properties | T700-12 k [24] | Properties | T700-12 k [23,24] |
|---|---|---|---|
| /GPa 1 | 230 | /10−6 °C−1 | −0.52 |
| /GPa | 14 | /10−6 °C−1 | 10.2 |
| /GPa | 9 | /kg m−3 | 1800 |
| /GPa | 5 | /(W m−1 k−1) 1 | 12.85 |
| 0.25 | /(W m−1 k−1) 2 | 1.45 | |
| 0.3 |
| Properties | Epoxy Resin [29] | Properties | Epoxy Resin [23] |
|---|---|---|---|
| /GPa | 2.04 | /10−6 °C−1 | 86.9 |
| /GPa | 2.04 | /10−6 °C−1 | 86.9 |
| /GPa | 0.77 | /kg m−3 | 1300 |
| /GPa | 0.77 | /(W m−1 k−1) | 0.18 |
| 0.33 | /(W m−1 k−1) | 0.18 | |
| 0.33 |
| Approach | /GPa | /GPa | /GPa | /GPa | ||
|---|---|---|---|---|---|---|
| Experimental [30] | 24.68 | 20.75 | ||||
| RVE | 24.21 | 24.39 | 2.59 | 2.27 | 0.101 | 0.43 |
| Error | 1.9% | 17.54% | 8.45% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Li, X.; Fan, C.; Song, W.; Chen, Y.; Jin, Y.; Han, X.; Zheng, J. Investigation of the Thermal Vibration Behavior of an Orthogonal Woven Composite Nozzle Based on RVE Analysis. Aerospace 2025, 12, 157. https://doi.org/10.3390/aerospace12020157
Wang L, Li X, Fan C, Song W, Chen Y, Jin Y, Han X, Zheng J. Investigation of the Thermal Vibration Behavior of an Orthogonal Woven Composite Nozzle Based on RVE Analysis. Aerospace. 2025; 12(2):157. https://doi.org/10.3390/aerospace12020157
Chicago/Turabian StyleWang, Lin, Xiaoniu Li, Congze Fan, Wenzhe Song, Yiwei Chen, Yufeng Jin, Xiaobo Han, and Jinghua Zheng. 2025. "Investigation of the Thermal Vibration Behavior of an Orthogonal Woven Composite Nozzle Based on RVE Analysis" Aerospace 12, no. 2: 157. https://doi.org/10.3390/aerospace12020157
APA StyleWang, L., Li, X., Fan, C., Song, W., Chen, Y., Jin, Y., Han, X., & Zheng, J. (2025). Investigation of the Thermal Vibration Behavior of an Orthogonal Woven Composite Nozzle Based on RVE Analysis. Aerospace, 12(2), 157. https://doi.org/10.3390/aerospace12020157





