Study of Fluid Flow Characteristics and Mechanical Properties of Aviation Fuel-Welded Pipelines via the Fluid–Solid Coupling Method
Abstract
1. Introduction
2. Methodology
2.1. Mathematical Model of Fluid–Solid Coupling
2.1.1. Solid State Control Equation
2.1.2. Fluid Control Equation
2.1.3. Fluid–Solid Coupling Control Equation
2.2. Numerical Simulations
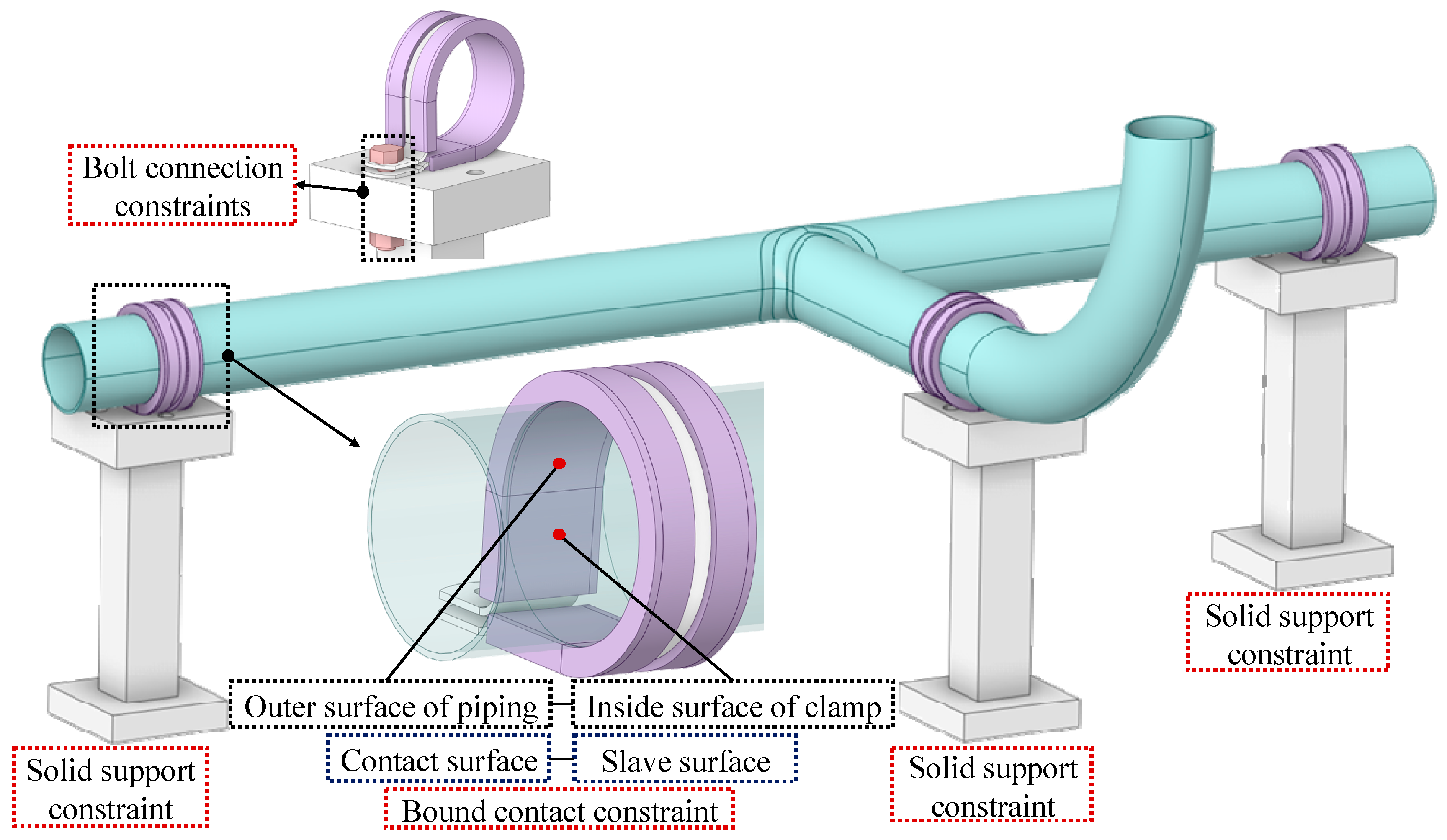
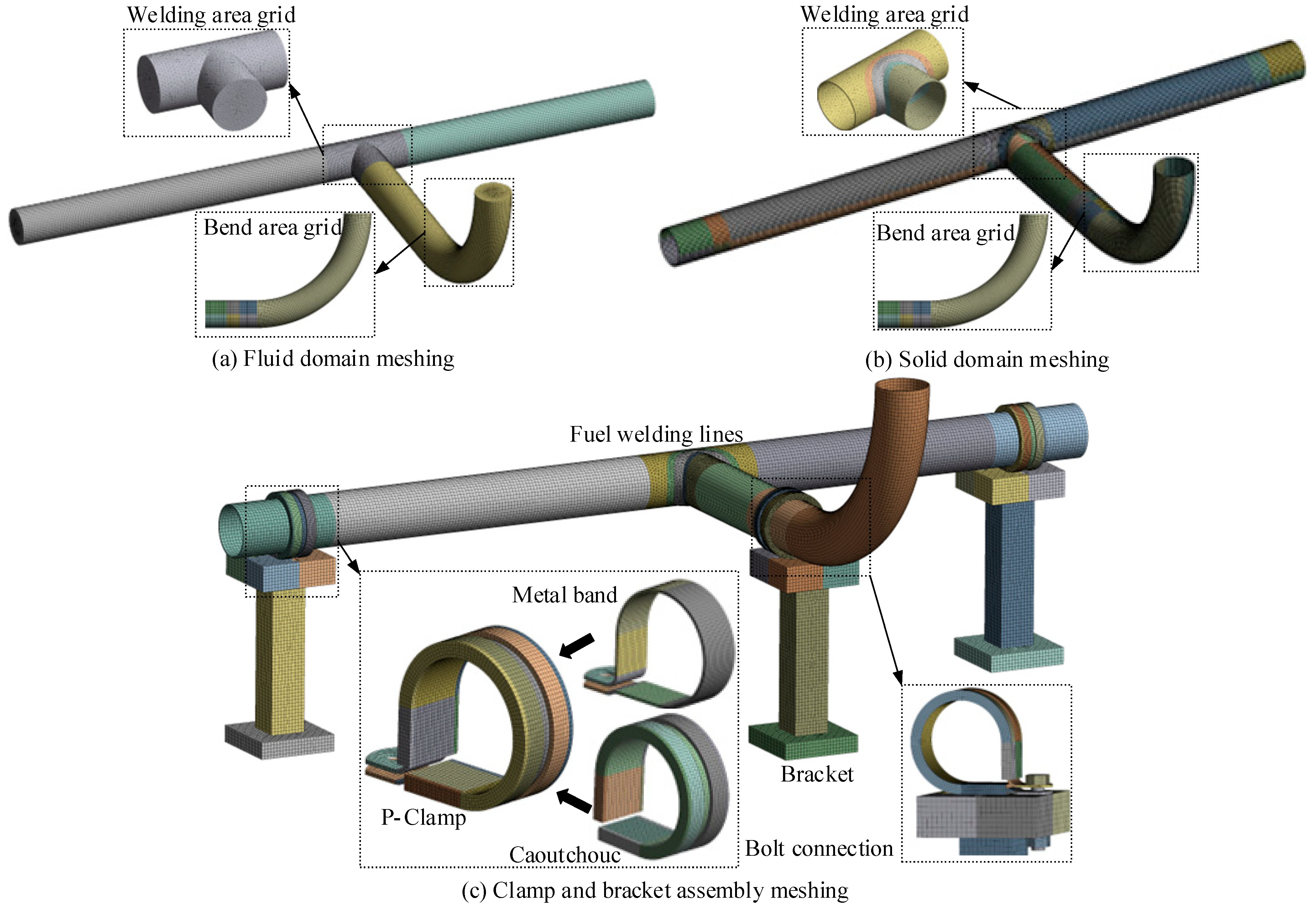
2.2.1. Physical Model, Boundary, and Initial Conditions
Welded Joint Parameters
Pipeline Physical Model
Boundary and Initial Condition Settings
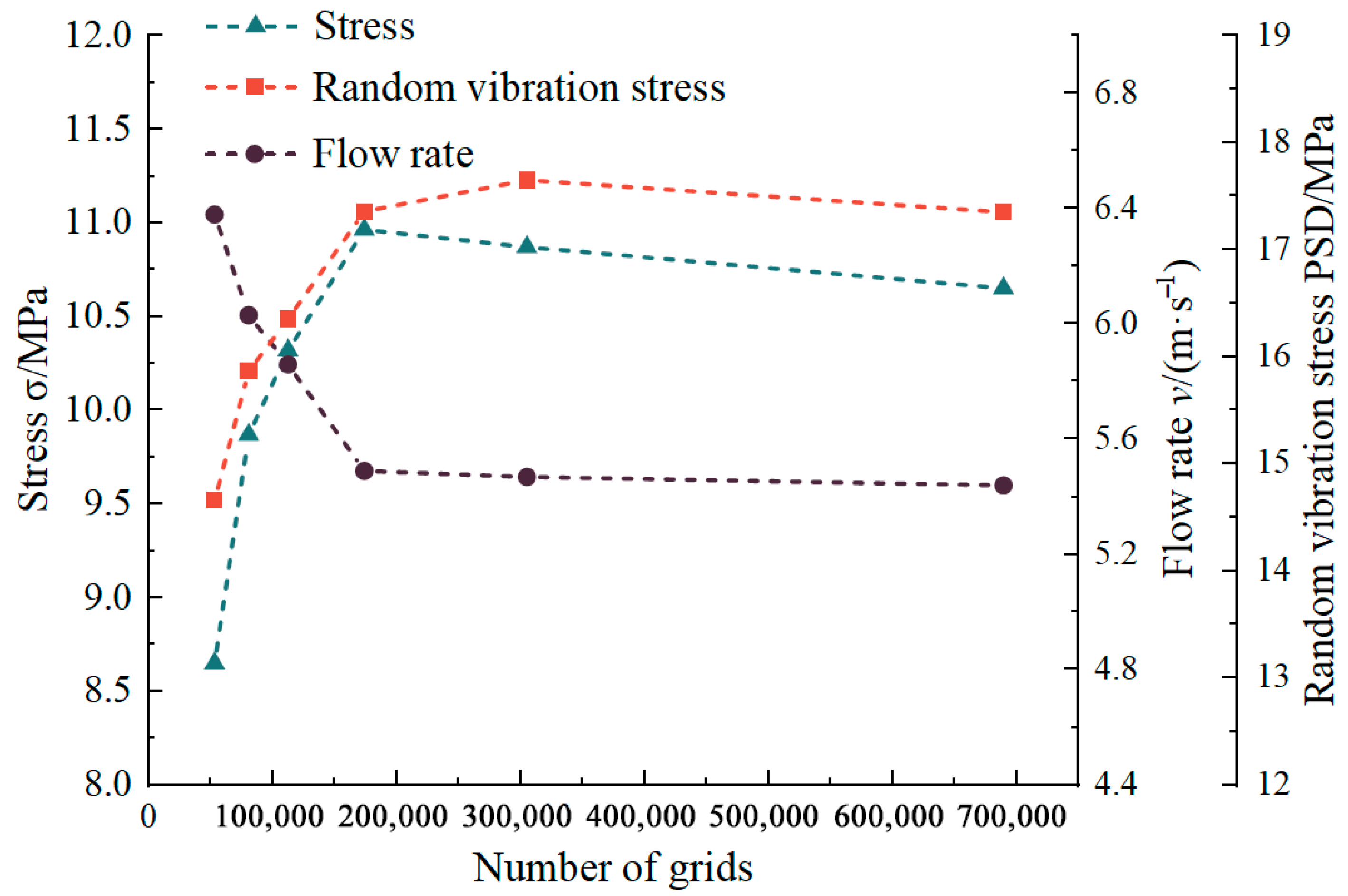
2.2.2. Grid Independence Verification
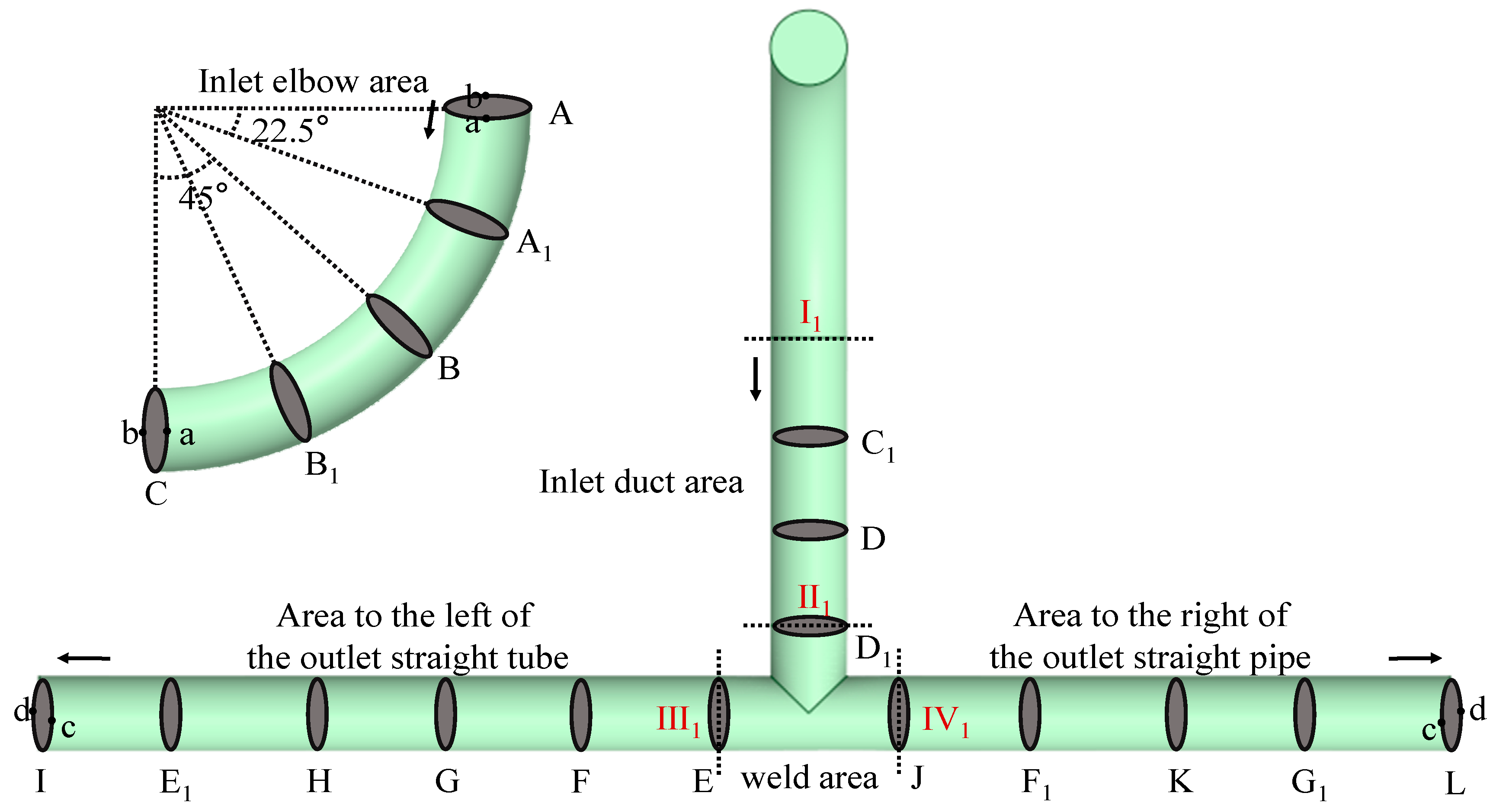
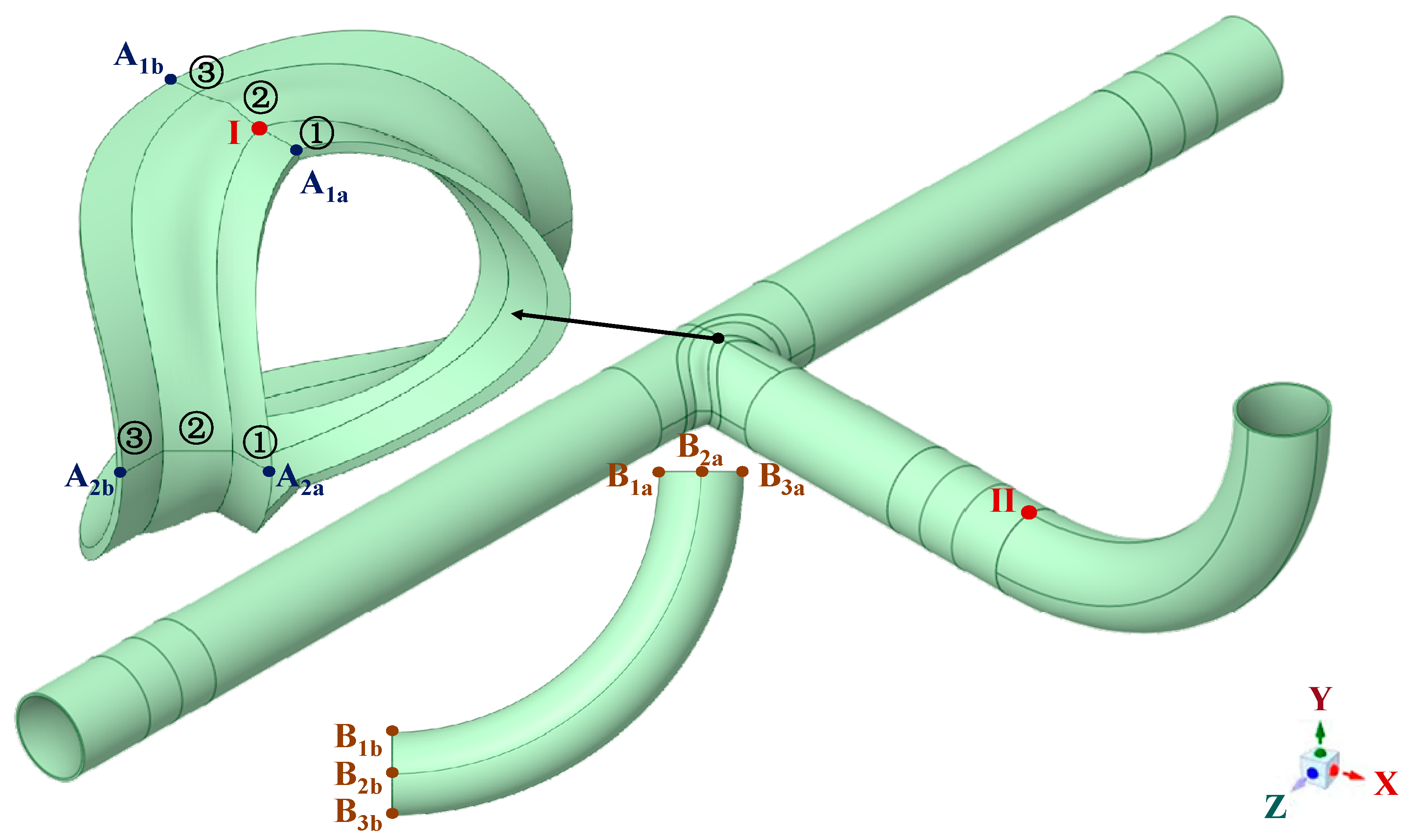
2.3. Monitoring Position Setting
2.3.1. Fluid Domain
2.3.2. Solid Domain
2.4. Pipeline Natural Frequency Analysis
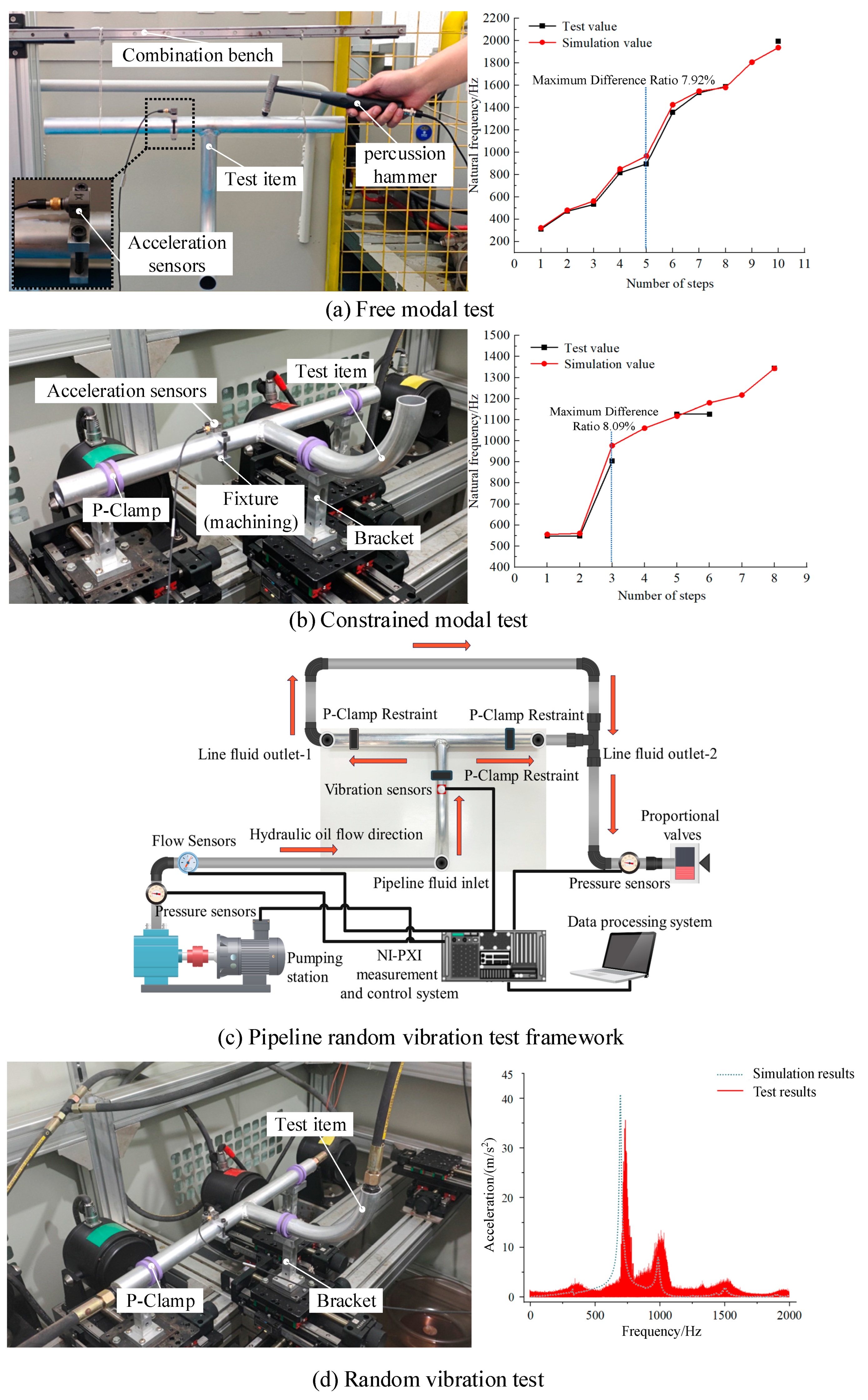
2.5. Validation and Analysis of the Numerical Model
3. Results and Discussion
3.1. Velocity Field Analysis with Different Fluid Properties
3.1.1. Fluid Pressure
3.1.2. Fluid Velocity
3.2. Pressure Field Analysis with Different Fluid Properties
3.3. Stress Analysis of Different Zones via Fluid Properties
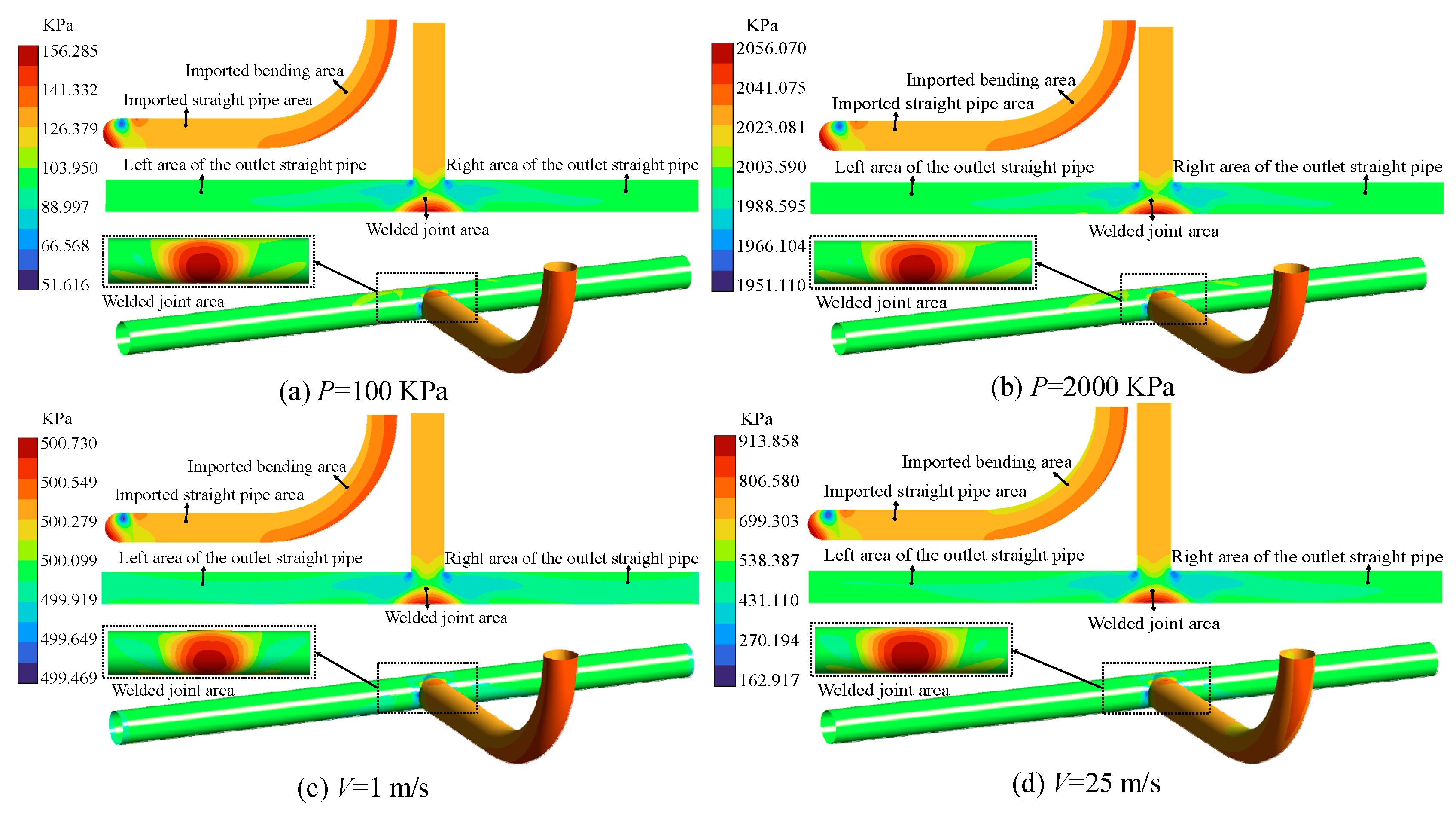
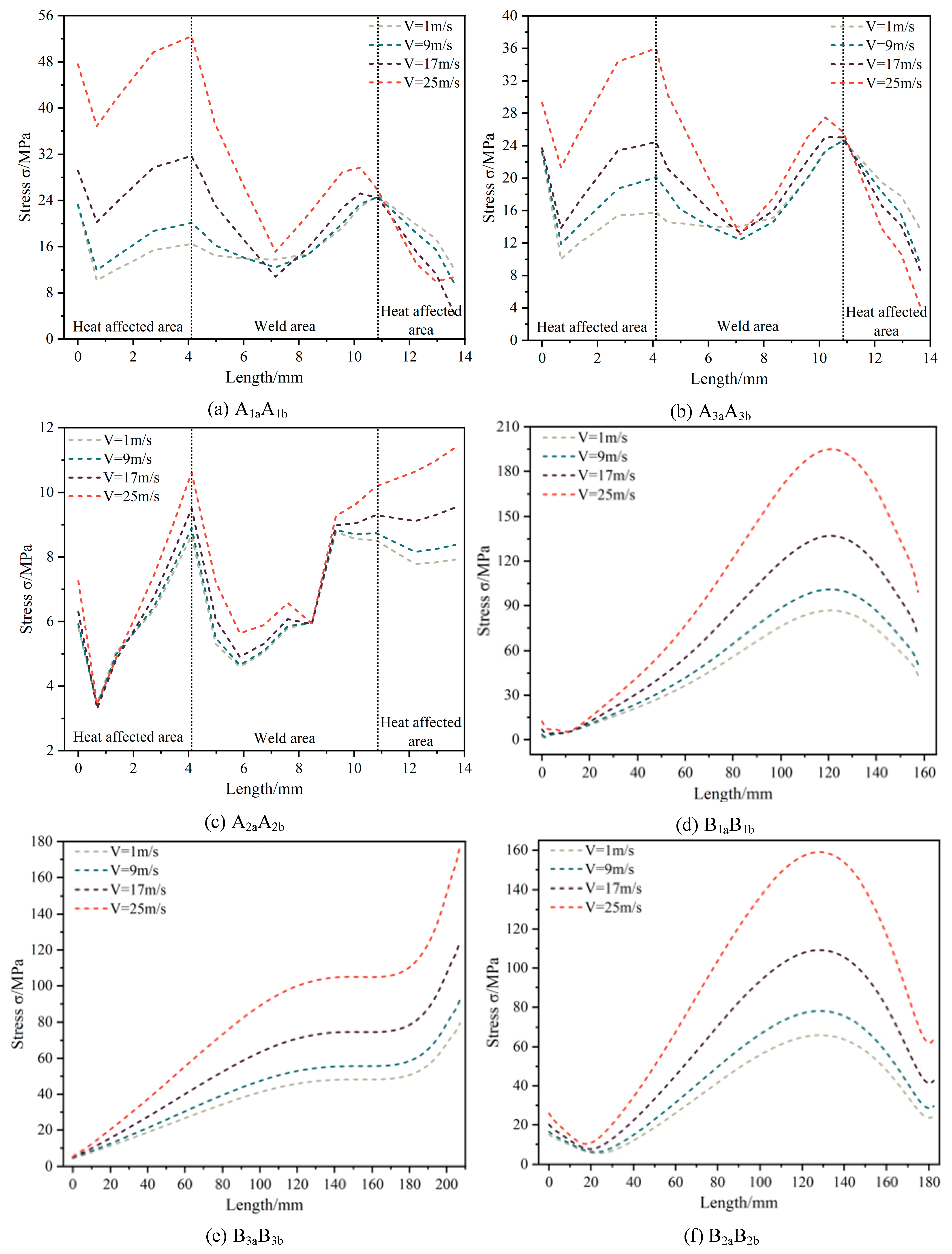
3.3.1. Stress Analysis of Fluid Pressure on Welded and Bent Pipe Areas
3.3.2. Stress Analysis of Fluid Velocity on Welded and Bent Pipe Areas
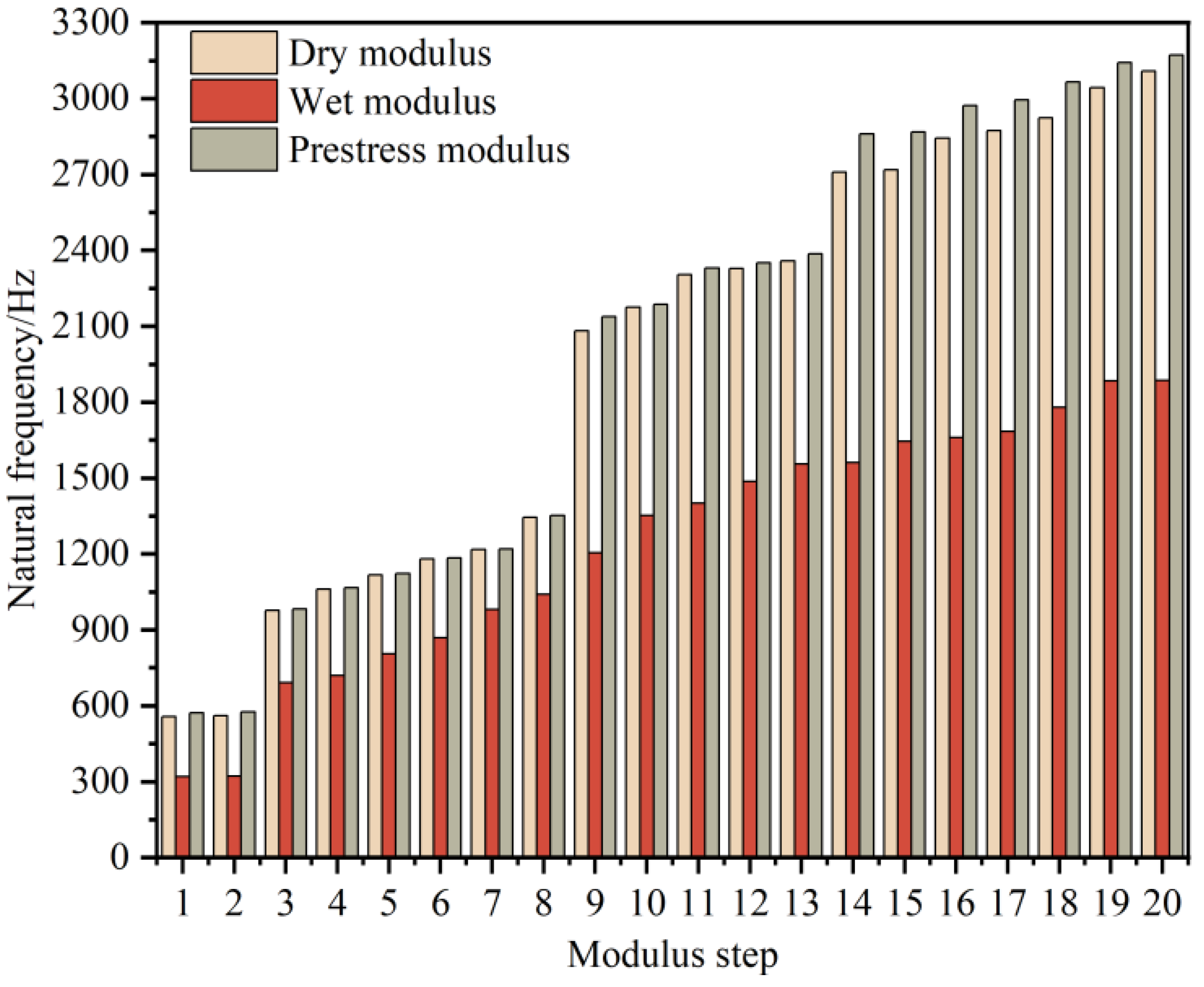
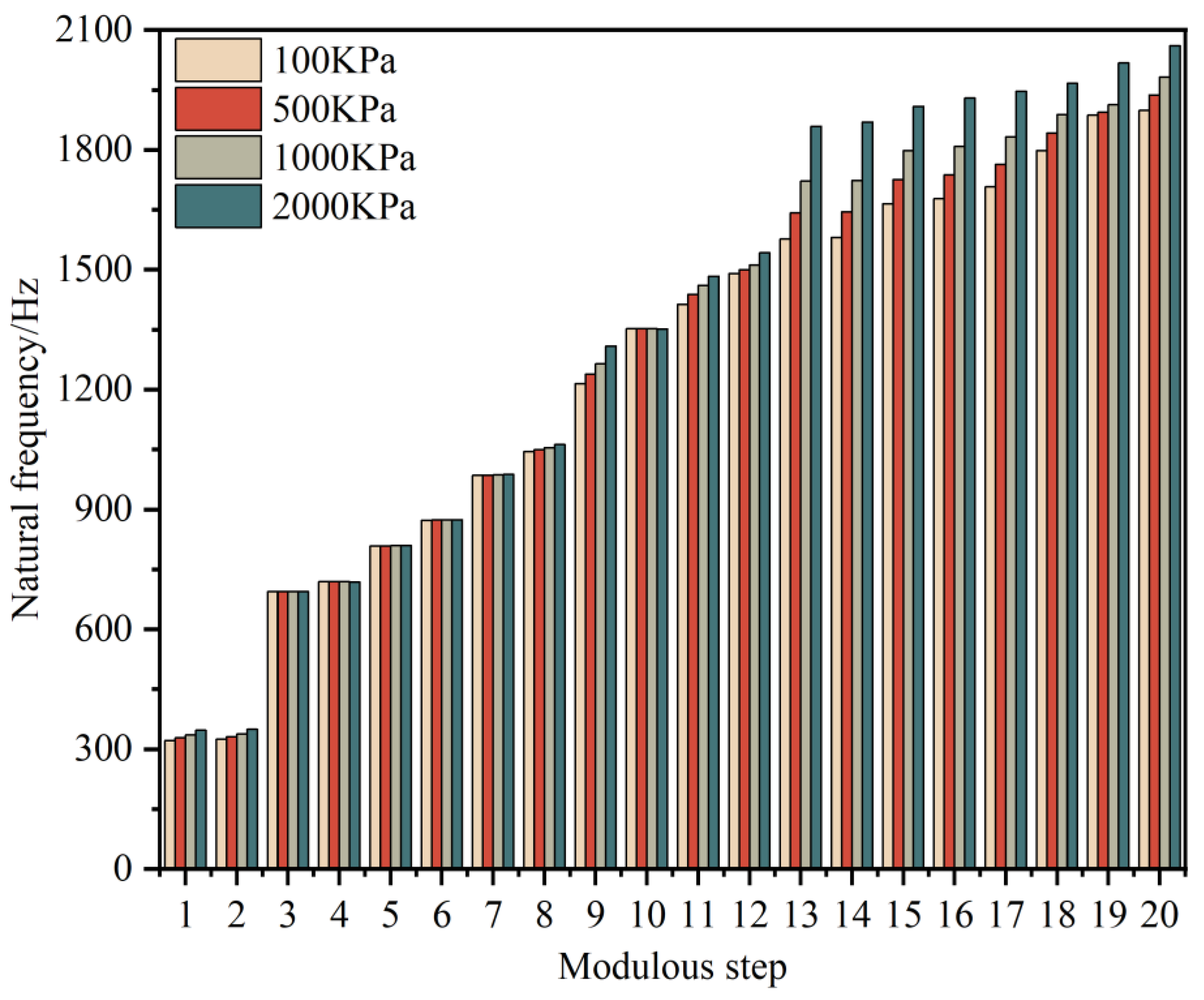
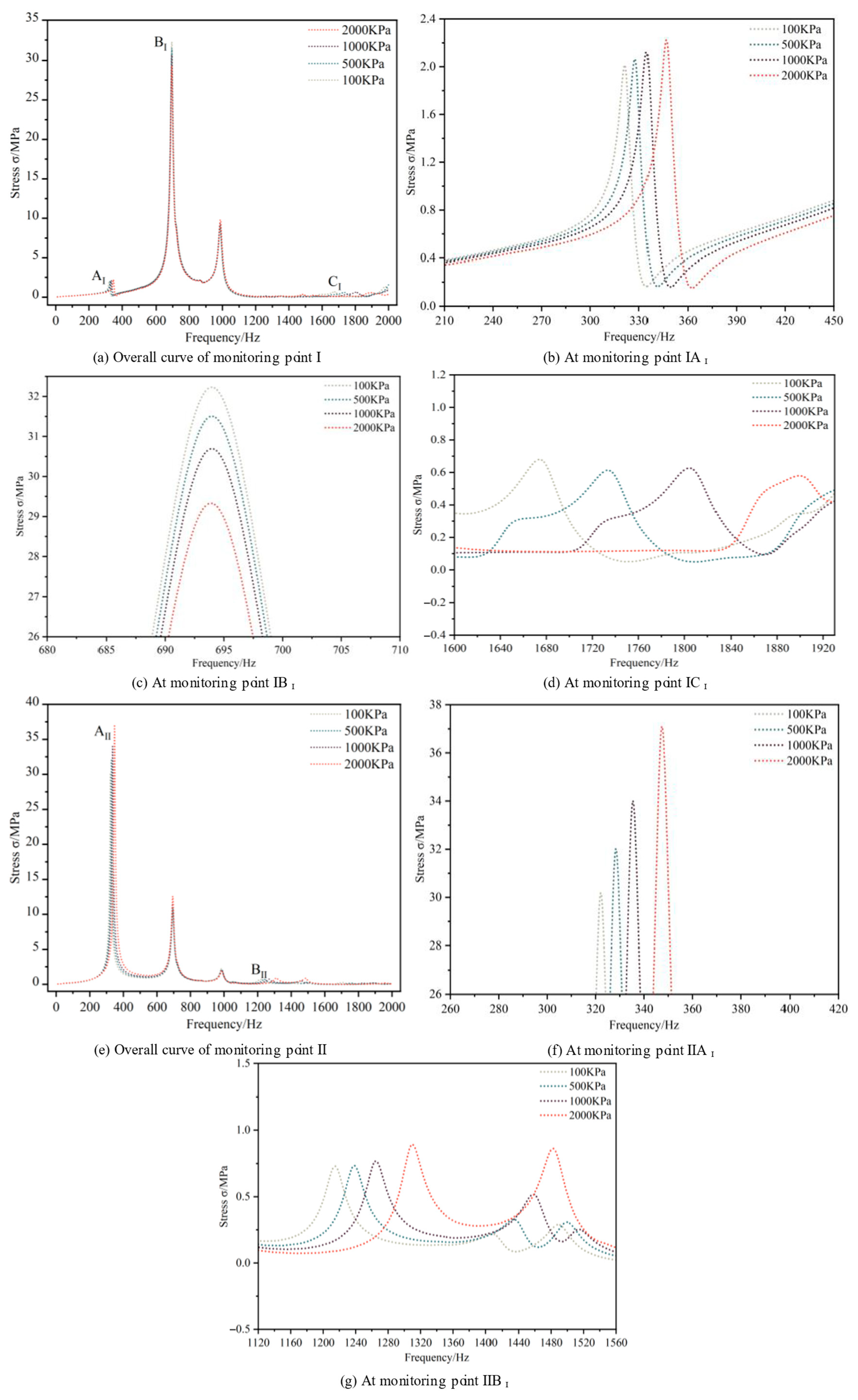
3.4. Modal Analysis of Pipelines with Different Fluid Characteristics
3.4.1. Fluid Pressure Influence
3.4.2. Fluid Velocity Influence
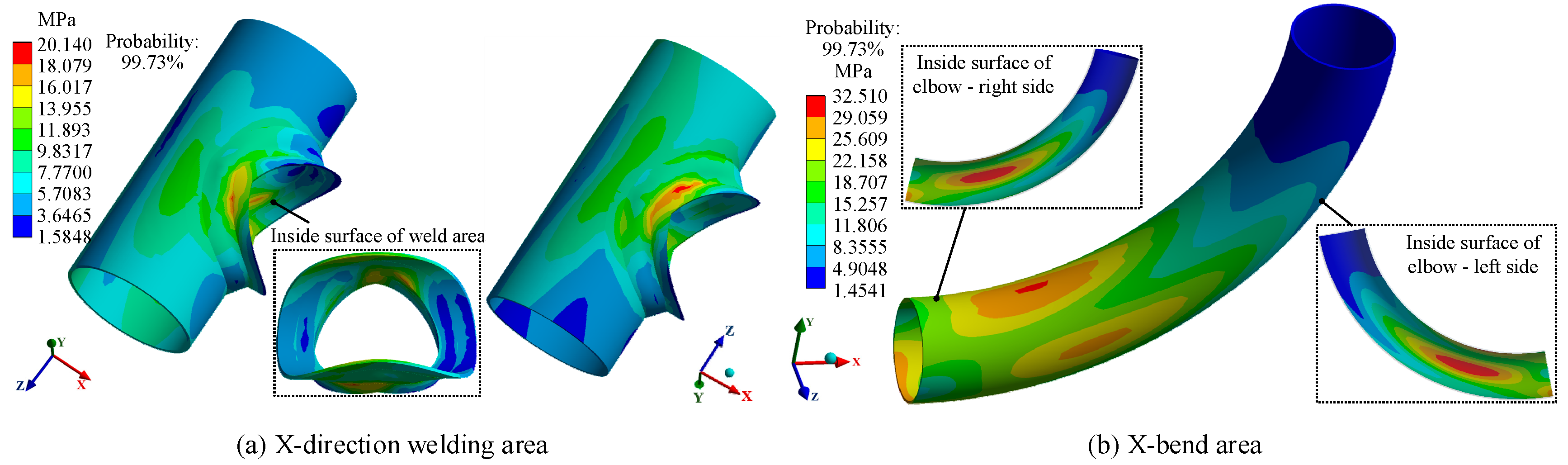
3.5. Impact Analysis of Random Vibration Characteristics of Pipelines
3.5.1. X-Axis Excitation Effect
3.5.2. Fluid Pressure Effect
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shalaby, H.M.; Riad, W.T.; Alhazza, A.A.; Behbehani, M.H. Failure analysis of fuel supply pipeline. Eng. Fail. Anal. 2006, 13, 789–796. [Google Scholar] [CrossRef]
- Lewis, P.R.; Hainsworth, S. Fuel line failure from stress corrosion cracking. Eng. Fail. Anal. 2006, 13, 946–962. [Google Scholar] [CrossRef]
- Viderščak, D.; Schauperl, Z.; Ormuž, K.; Šolić, S.; Nikšić, M. Failure analysis of high-pressure fuel injection lines. Eng. Fail. Anal. 2021, 121, 105176. [Google Scholar] [CrossRef]
- González, M.P.V.; Meije, A.G.; Muro, A.P.; García-Martínez, M.; Caballero, B.G. Failure analysis of a fuel control pressure tube from an aircraft engine. Eng. Fail. Anal. 2021, 126, 105452. [Google Scholar] [CrossRef]
- Al-Sahib, N.K.A.; Jameel, A.N.; Abdulateef, O.F. Investigation into the Vibration Characteristics and Stability of a Welded Pipe Conveying Fluid. Jordan J. Mech. Ind. Eng. 2010, 4, 378–387. [Google Scholar]
- Ji, W.; Ma, H.; Sun, W.; Cao, Y. Reduced-order modeling and vibration transfer analysis of a fluid-delivering branch pipeline that consider fluid–solid interactions. Front. Mech. Eng. 2024, 19, 10. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, M. Investigating the Mechanical Properties of Aluminum Alloy Ultra-Fine Grained Metals by Fluid-Solid Coupling Method. Sci. Adv. Mater. 2022, 14, 1605–1612. [Google Scholar] [CrossRef]
- Huang, W.; Garbatov, Y.; Soares, C.G. Fatigue reliability assessment of a complex welded structure subjected to multiple cracks. Eng. Struct. 2013, 56, 868–879. [Google Scholar] [CrossRef]
- Qiu, Y.; Shen, W.; Yan, R.; Xu, L.; Liu, E. Fatigue reliability evaluation of thin plate welded joints considering initial welding deformation. Ocean Eng. 2021, 236, 109440. [Google Scholar] [CrossRef]
- Dong, Y.; Garbatov, Y.; Soares, C.G. Strain-based fatigue reliability assessment of welded joints in ship structures. Mar. Struct. 2021, 75, 102878. [Google Scholar] [CrossRef]
- Zhang, S.; Shen, Y.; Qiu, H. The technology and welding joint properties of hybrid laser-tig welding on thick plate. Opt. Laser Technol. 2013, 48, 381–388. [Google Scholar]
- Li, J.; Liu, Y.; Gao, Y.; Jin, P.; Sun, Q.; Feng, J. Benefits of interfacial regulation with interlayers in laser welding Ti6Al4V/316L steel. Opt. Laser Technol. 2020, 125, 106007. [Google Scholar] [CrossRef]
- Yi, J.; Wang, G.; Li, S.-K.; Liu, Z.-W.; Gong, Y.-L. Effect of post-weld heat treatment on microstructure and mechanical properties of welded joints of 6061-T6 aluminum alloy. Trans. Nonferrous Met. Soc. China 2019, 29, 2035–2046. [Google Scholar] [CrossRef]
- Chu, Q.; Bai, R.; Jian, H.; Lei, Z.; Hu, N.; Yan, C. Microstructure, texture and mechanical properties of 6061 aluminum laser beam welded joints. Mater. Charact. 2018, 137, 269–276. [Google Scholar] [CrossRef]
- Ai, Y.; Lei, C.; Cheng, J.; Mei, J. Prediction of weld area based on image recognition and machine learning in laser oscillation welding of aluminum alloy. Opt. Lasers Eng. 2023, 160, 107258. [Google Scholar] [CrossRef]
- Lu, Y.; Zhu, S.; Zhao, Z.; Chen, T.; Zeng, J. Numerical simulation of residual stresses in aluminum alloy welded joints. J. Manuf. Process. 2020, 50, 380–393. [Google Scholar] [CrossRef]
- Wu, A.; Song, Z.; Nakata, K.; Liao, J.; Zhou, L. Interface and properties of the friction stir welded joints of titanium alloy Ti6Al4V with aluminum alloy 6061. Mater. Des. 2015, 71, 85–92. [Google Scholar] [CrossRef]
- Liu, F.; Sun, W.; Zhang, H.; Lyu, S. Dynamic modeling and stress reduction optimization of parallel pipelines based on pipe-solid element coupling. Int. J. Press. Vessel. Pip. 2024, 207, 105107. [Google Scholar] [CrossRef]
- Duan, J.; Gong, J.; Yao, H.; Deng, T.; Zhou, J. Numerical modeling for stratified gas–liquid flow and heat transfer in pipeline. Appl. Energy 2014, 115, 83–94. [Google Scholar] [CrossRef]
- Fu, H.; Wang, S.; Ling, K. Detection of two-point leakages in a pipeline based on lab investigation and numerical simulation. J. Pet. Sci. Eng. 2021, 204, 108747. [Google Scholar] [CrossRef]
- Figueiredo, A.B.; Baptista, R.M.; Rachid, F.B.d.F.; Bodstein, G.C.R. Numerical simulation of stratified-pattern two-phase flow in gas pipelines using a two-fluid model. Int. J. Multiph. Flow 2017, 88, 30–49. [Google Scholar] [CrossRef]
- Lazareff, M.; Moretti, R.; Errera, M.-P. Coupling methodology for thermal fluid-solid simulations through a full transient flight cycle. Int. J. Heat Mass Transf. 2023, 202, 123691. [Google Scholar] [CrossRef]
- Xu, Q.; Feng, J.; Zhang, S. Effects of different loads on structure stress of “L”-type large-diameter pipeline under burying and trench conditions based on fluid–structure–heat coupling. Int. J. Heat Mass Transf. 2017, 115, 387–397. [Google Scholar] [CrossRef]
- Xu, Q.; Feng, J.; Zhang, S. Combined effects of different temperature and pressure loads on the “L”-type large-diameter buried pipeline. Int. J. Heat Mass Transf. 2017, 111, 953–961. [Google Scholar] [CrossRef]
- Solomon, I.; Dundulis, G. Modeling of Pipe Whip Phenomenon Induced by Fast Transients Based on Fluid–Structure Interaction Method Using a Coupled 1D/3D Modeling Approach. Appl. Sci. 2023, 13, 10653. [Google Scholar] [CrossRef]
- Mohmmed, A.O.; Al-Kayiem, H.H.; Osman, A.B.; Sabir, O. One-way coupled fluid–structure interaction of gas–liquid slug flow in a horizontal pipe: Experiments and simulations. J. Fluids Struct. 2020, 97, 103083. [Google Scholar] [CrossRef]
- Talemi, R.; Cooreman, S.; Mahgerefteh, H.; Martynov, S.; Brown, S. A fully coupled fluid-structure interaction simulation of three-dimensional dynamic ductile fracture in a steel pipeline. Theor. Appl. Fract. Mech. 2019, 101, 224–235. [Google Scholar] [CrossRef]
- Jin, X.; Zhu, P.; Li, H.; Wang, Q. Research on Mechanical Properties of Locking Cap Lap Welds and Partitioned Seam Weld Model. J. Shanghai Jiaotong Univ. 2017, 51, 1297–1303. [Google Scholar]
- Chu, G. Laser Weld-seam Modeling for Finite Element Analysis during Tailor-welded Tube Hydroforming. Chin. J. Mech. Eng. 2012, 48, 38–43. [Google Scholar] [CrossRef]
- Jin, D.; Hou, C.; Shen, L. Effect of welding residual stress on the performance of CFST tubular joints. J. Constr. Steel Res. 2021, 184, 106827. [Google Scholar] [CrossRef]
- Chiocca, A.; Frendo, F.; Aiello, F.; Bertini, L. Influence of residual stresses on the fatigue life of welded joints. Numerical simulation and experimental tests. Int. J. Fatigue 2022, 162, 106901. [Google Scholar] [CrossRef]
- Ghafouri, M.; Ahola, A.; Ahn, J.; Björk, T. Welding-induced stresses and distortion in high-strength steel T-joints: Numerical and experimental study. J. Constr. Steel Res. 2022, 189, 107088. [Google Scholar] [CrossRef]
- Kim, H.; Lee, G.; Shin, S.; Yoo, H.; Cho, J.; Han, S.-W.; Rhie, C.; Kim, G. Strength Prediction FEM Model Development of Welded Steel Joint. Int. J. Precis. Eng. Manuf. 2022, 23, 1399–1409. [Google Scholar] [CrossRef]
- Paıdoussis, M.; Li, G. Pipes Conveying Fluid: A Model Dynamical Problem. J. Fluids Struct. 1993, 7, 137–204. [Google Scholar] [CrossRef]
- Bathe, K.; Zhang, H. Finite element developments for general fluid flows with structural interactions. Int. J. Numer. Methods Eng. 2004, 60, 213–232. [Google Scholar] [CrossRef]
- Zheng, Q.; Teng, Y. Vibration Simulation Analysis on Aeroengine External Pipelines Based on ANSYS. Open J. Acoust. Vib. 2022, 39, 1–5. [Google Scholar]
- Yu, T.; Zhang, Z.; Zhang, D.; Juan, M.; Jin, J. Vibration Analysis of Multi-Branch Hydraulic Pipeline System Considering Flu-id-Structure Interaction. Appl. Sci. 2022, 12, 12902. [Google Scholar] [CrossRef]
- Chmelko, V.; Harakaľ, M.; Žlábek, P.; Margetin, M.; Ďurka, R. Simulation of Stress Concentrations in Notches. Metals 2022, 12, 43. [Google Scholar] [CrossRef]
- Deng, J. Effect of Element and Mesh Density on the Results of Finite Element Analysis. Petro Chem. Equip. Technol. 2017, 38, 12–15. [Google Scholar]
- Sutton, E.; Juel, A.; Kowalski, A.; Fonte, C.P. Dynamics and friction losses of the flow of yield-stress fluids through 90° pipe bends. Chem. Eng. Sci. 2022, 251, 117484. [Google Scholar] [CrossRef]
- Paliwal, B.; Ramesh, K. An interacting micro-crack damage model for failure of brittle materials under compression. J. Mech. Phys. Solids 2008, 56, 896–923. [Google Scholar] [CrossRef]
- Shu, F.; Lv, Y.; Liu, Y.; Xu, F.; Sun, Z.; He, P.; Xu, B. Residual stress modeling of narrow gap welded joint of aluminum alloy by cold metal transferring procedure. Constr. Build. Mater. 2014, 54, 224–235. [Google Scholar] [CrossRef]
- Rinaldi, S.; Prabhakar, S.; Vengallatore, S.; Païdoussis, M.P. Dynamics of microscale pipes containing internal fluid flow: Damping, frequency shift, and stability. J. Sound Vib. 2010, 329, 1081–1088. [Google Scholar] [CrossRef]
- Lee, P.J.; Duan, H.-F.; Ghidaoui, M.; Karney, B. Frequency domain analysis of pipe fluid transient behaviour. J. Hydraul. Res. 2013, 51, 609–622. [Google Scholar] [CrossRef]
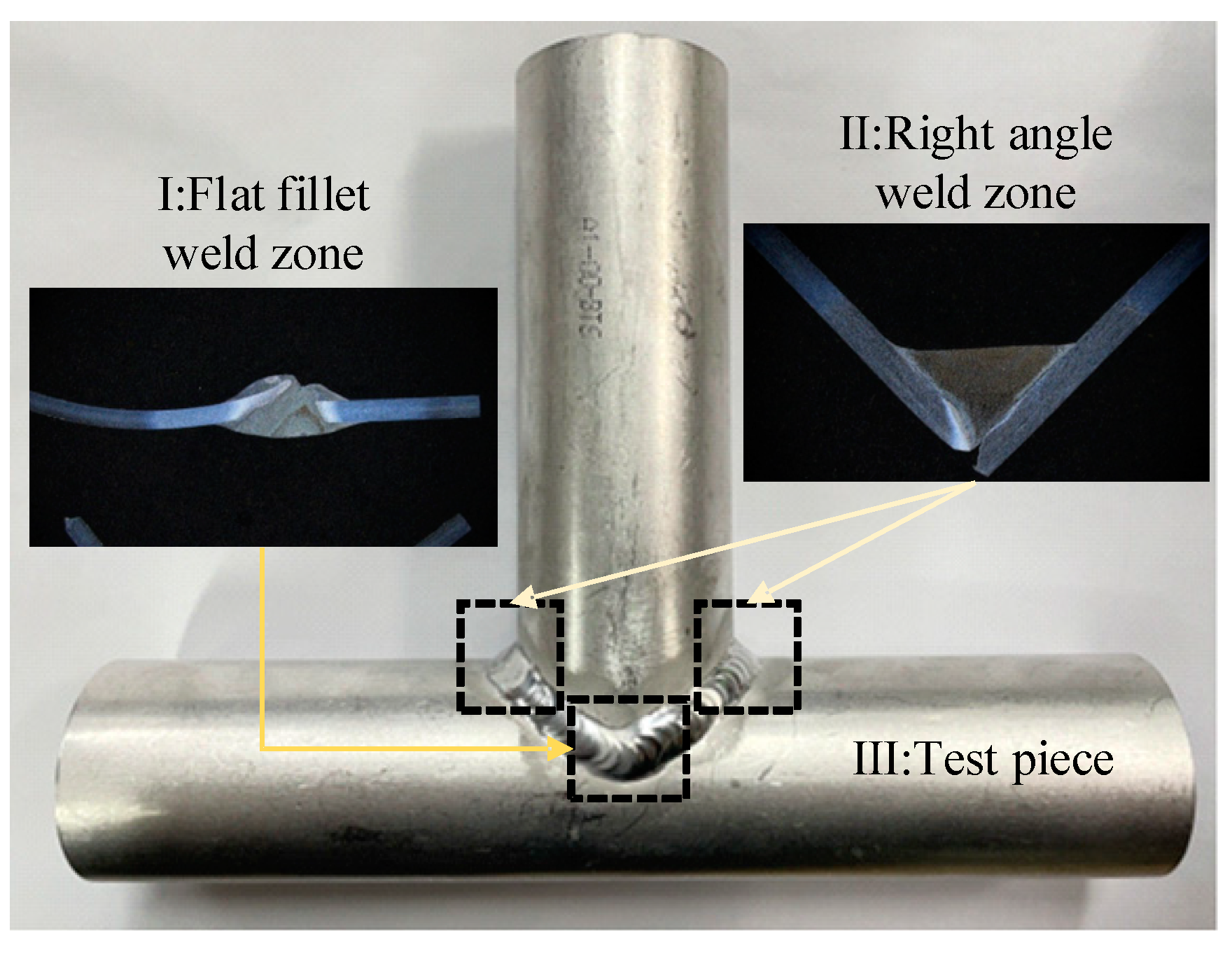
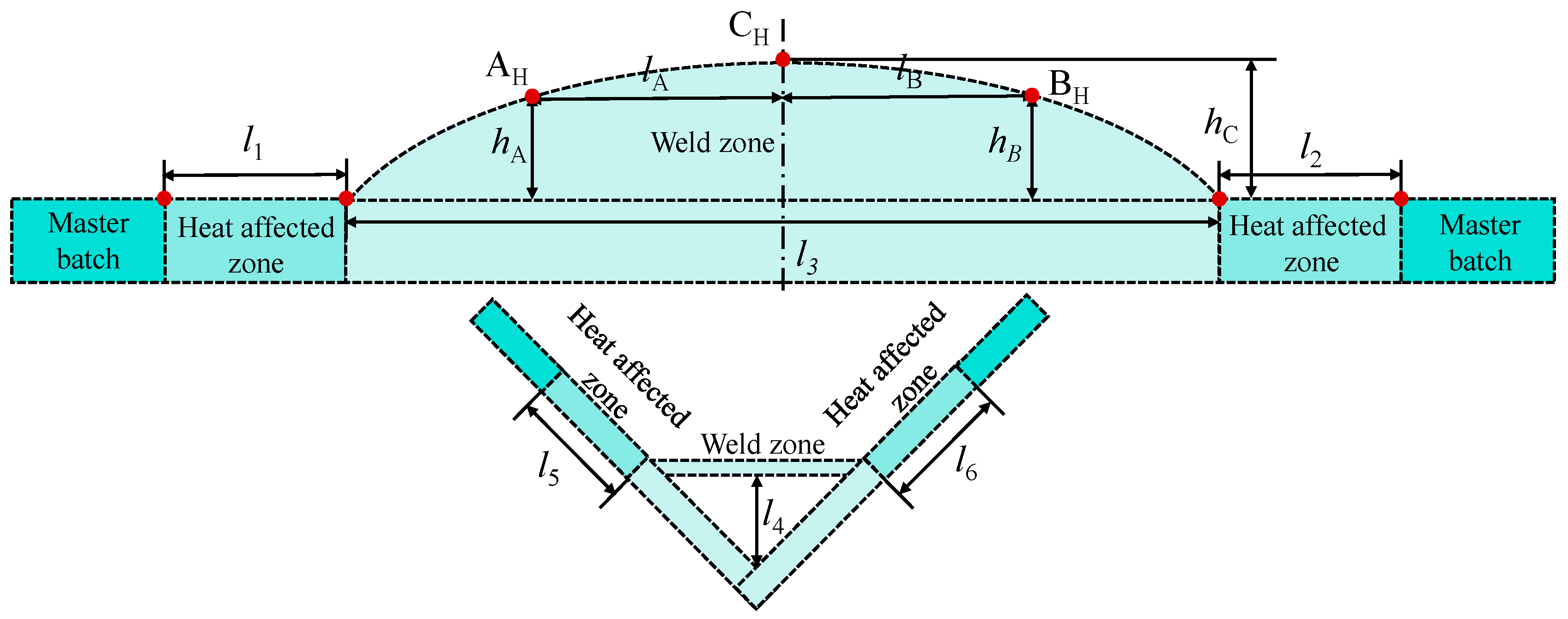
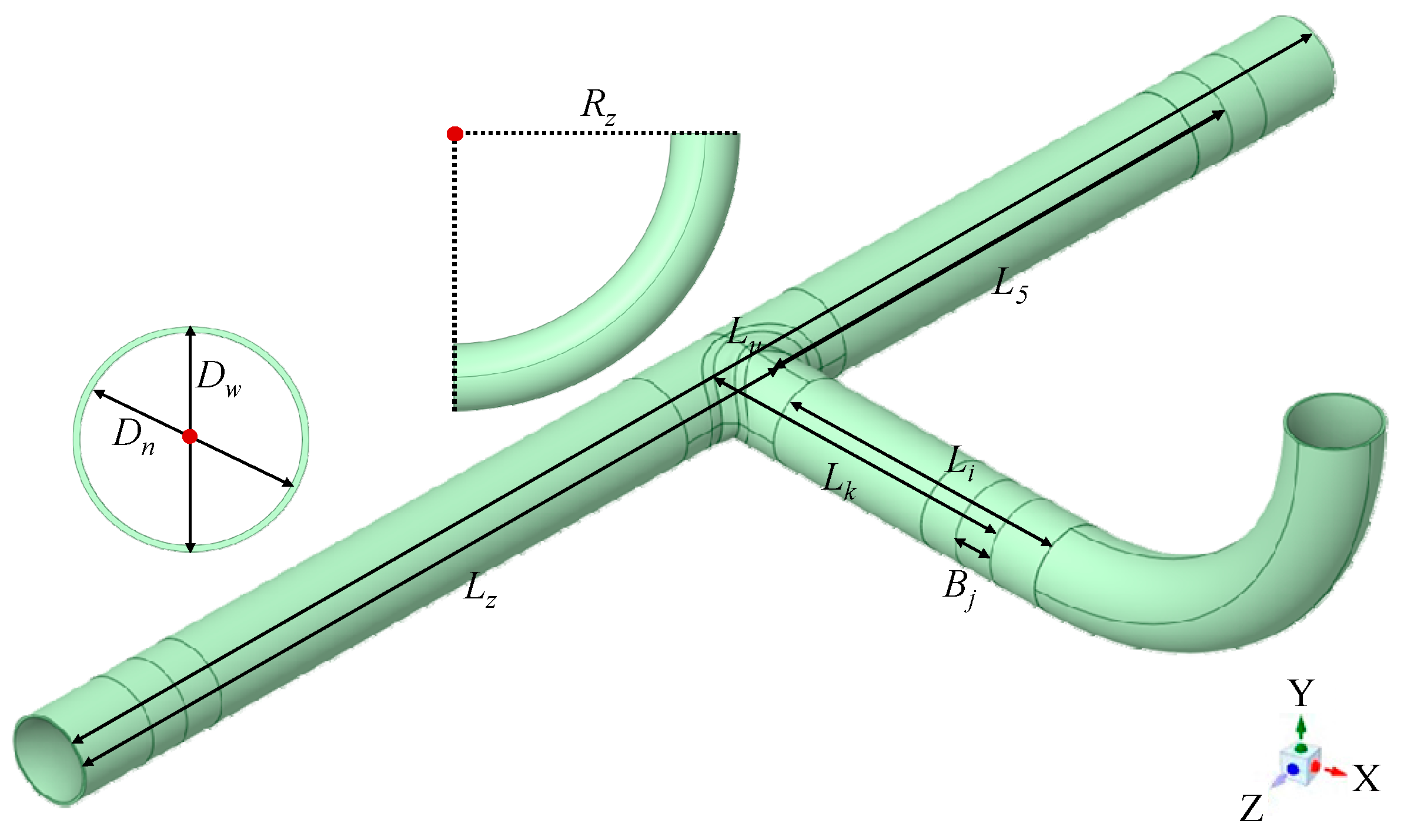
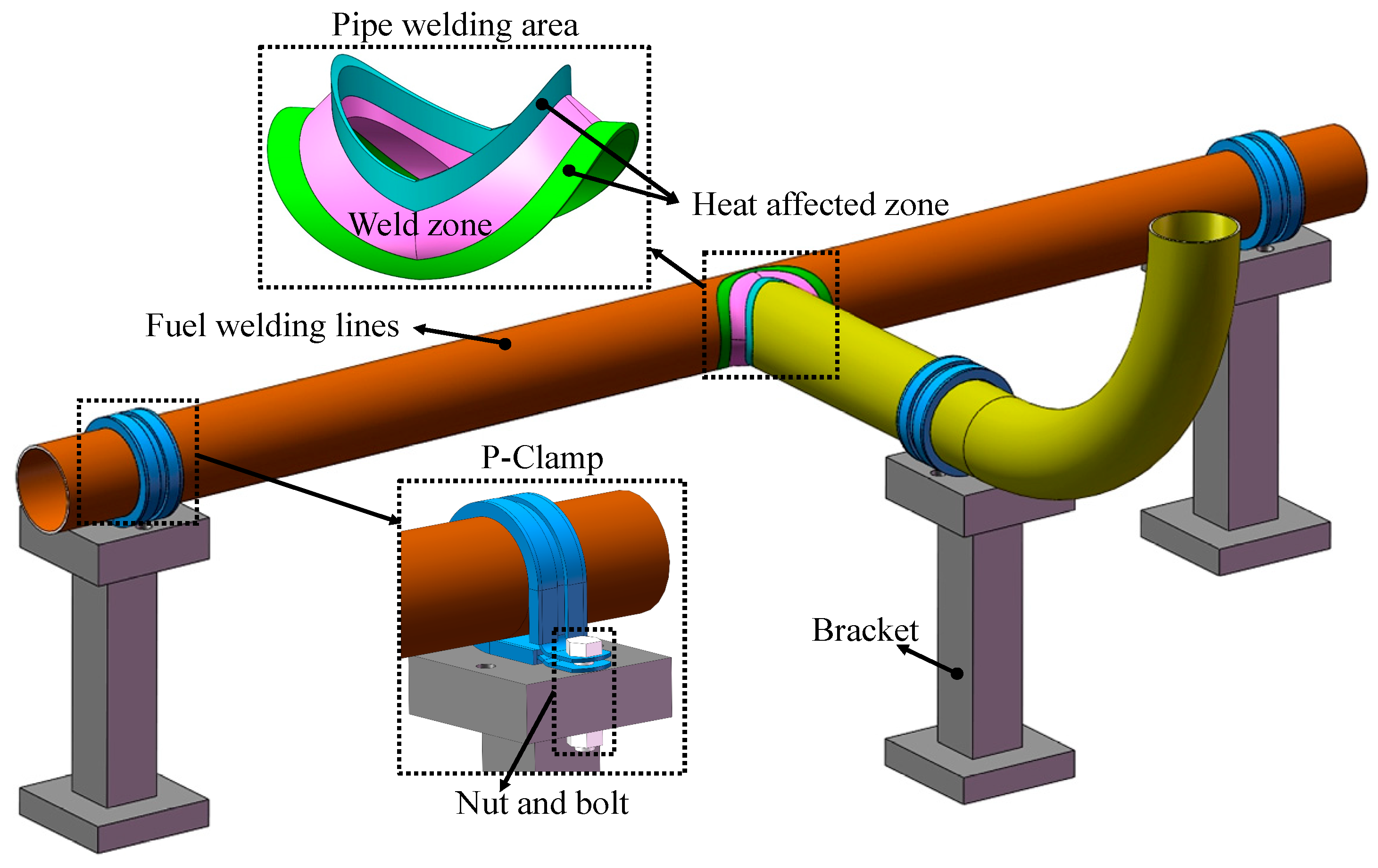






| Position | Parameters | Size/mm |
|---|---|---|
| Weld thickness | l4 | 2.60 |
| HAZ of right-angle weld branch pipe | l5 | 7.81 |
| HAZ of right-angle weld supervisor | l6 | 8.02 |
| Outside residual height | hC | 0.72 |
| Outside width | l3 | 6.61 |
| Height of point A | hA | 0.20 |
| Distance of point A from the center line | LA | 1.76 |
| Height of point B | hB | 0.31 |
| Distance of point B from the center line | lB | 3.15 |
| Height of HAZ of flat fillet weld branch pipe | l1 | 4.74 |
| Height of HAZ of flat fillet weld supervisor | l2 | 6.06 |
| Name | Density/(kg/m3) | E/GPa | υ |
|---|---|---|---|
| BMZ | 2.713 × 103 | 75 | 0.35 |
| WZ | 2.713 × 103 | 81.8 | 0.32 |
| HAZ | 2.713 × 103 | 74 | 0.29 |
| Parameter Symbol | Parameter Meaning | Design Data/mm |
|---|---|---|
| Dw | Outside diameter of main and branch lines | 31.75 |
| Dn | Inside diameter of main and branch lines | 29.97 |
| Lu | Length of main line | 550 |
| Lz | Vertical distance between the end face of the main line and the axis of the branch line | 350 |
| Li | Straight length of branch line | 150 |
| Rz | Bending radius of branch line | 116 |
| Bj | Width of clamp to line contact | 15 |
| Lk | Distance of branch pipe clamp end face from main line end face | 30 |
| Ld | Distance of straight pipe clamp end face from Branch line elbow end face | 20 |
| Name | Unit Quality | Aspect Ratio | Orthogonal Quality | Deviation |
|---|---|---|---|---|
| Solid domain | 0.648 | 2.438 | 0.876 | 0.438 |
| Fluid domain | 0.793 | 1.977 | 0.915 | 0.151 |
| Clamp | 0.876 | 1.646 | 0.953 | 0.003 |
| Nuts and bolts | 0.928 | 1.379 | 0.702 | 0.219 |
| Brackets | 0.825 | 1.883 | 0.991 | 0.244 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, C.; Di, M.; Gong, H.; Zhang, J.; Sun, S.; Ye, K.; Li, B.; Quan, L. Study of Fluid Flow Characteristics and Mechanical Properties of Aviation Fuel-Welded Pipelines via the Fluid–Solid Coupling Method. Aerospace 2025, 12, 60. https://doi.org/10.3390/aerospace12010060
Guo C, Di M, Gong H, Zhang J, Sun S, Ye K, Li B, Quan L. Study of Fluid Flow Characteristics and Mechanical Properties of Aviation Fuel-Welded Pipelines via the Fluid–Solid Coupling Method. Aerospace. 2025; 12(1):60. https://doi.org/10.3390/aerospace12010060
Chicago/Turabian StyleGuo, Changhong, Mengran Di, Hanwen Gong, Jin Zhang, Shibo Sun, Kehua Ye, Bin Li, and Lingxiao Quan. 2025. "Study of Fluid Flow Characteristics and Mechanical Properties of Aviation Fuel-Welded Pipelines via the Fluid–Solid Coupling Method" Aerospace 12, no. 1: 60. https://doi.org/10.3390/aerospace12010060
APA StyleGuo, C., Di, M., Gong, H., Zhang, J., Sun, S., Ye, K., Li, B., & Quan, L. (2025). Study of Fluid Flow Characteristics and Mechanical Properties of Aviation Fuel-Welded Pipelines via the Fluid–Solid Coupling Method. Aerospace, 12(1), 60. https://doi.org/10.3390/aerospace12010060





