Vibration and Fault Analysis of a Rotor System of a Twin-Spool Turbo-Jet Engine in Ground Test

Abstract

According to the characteristics of the rotor system in an aero-engine and the vibrational test requirements of the aero-engine ground test, suitable vibration measurement sensors and test positions were selected. The vibration signals at the casings for the compressor and turbine of a twin-spool turbo-jet engine were collected under the states of maximum power and afterburning respectively, and the power spectrum analysis was carried out to determine the positions and causes of vibration. Furthermore, methods and preventive measures for eliminating vibration have been proposed. The results indicated that the main rotor vibration excited by mass imbalance in the twin-spool turbo-jet engine was significant. Rotor spindle misalignment or rotor radial stiffness unevenness also induced the vibration. The aerodynamic pulse vibration formed by the rotor blades of the first stage of the low pressure compressor was large, and rub induced vibration fault may occur at the turbine rotor seals. Based on the power spectrum analysis technology, the rotor system faults information including the type, position, and the degree can be quickly identified, and useful attempts and explorations have been made to reduce the vibration faults of the twin-spool turbo-jet engine.

1. Introduction

As the core component of aircraft, an aero-engine is affected by the harsh conditions such as temperature, pressure, and speed during operation. The turbine blades of the aero-engine may be damaged due to long-term high temperatures during operation. Bearings may be damaged by fatigue due to long-term use. The fuel system may leak due to aging or damaged seals. As a consequence, in order to ensure flight safety and maintain aero-engine performance, regular maintenance of the engine must be carried out, which not only consumes a good deal of time, energy, and financial resources, but also often causes missed failure detection and results in flight accidents [1]. For this reason, if the engine fault can be found and eliminated early when the ground test and maintenance of the engine is carried out, the flight accidents, especially major destructive accidents, can be avoided. During the operation of the engine, with the occurrence of faults, there are changes in physical parameters such as vibration, noise, temperature, pressure. Aviation engine fault diagnosis technology is used to judge and identify the working state and fault of the engine based on the changes of these parameters, and make early predictions to prevent problems before they occur and enable the engine to achieve good power, economy, and emission performance, thus the uneconomical and unreasonable phenomena caused by “excessive maintenance” will be avoided. Scholars all over the world have conducted extensive and deep explorations on this [2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19].

Zhang Y et al. built the fuel system fault tree, obtained the fault characteristics and fault modes, and analyzed the reasons and influence factors of the fault, which improved the reliability of the engine fuel system [2].

Daponte P et al. proposed a system called “Drones Bench” to test a light vertical take off and landing (VTOL) remotely piloted aircraft system (RPAS). This system can be used to identify fault through measuring the thrust force module, power consumption, and attitude stability of an RPAS [3].

For wind power applications, the generator rotating speed may change with the operation condition. Pezzani C M et al. proposed a resampling technique based on sequential tracking for spectral analysis of mechanical vibration, and successfully detected bearing faults in permanent magnet synchronous generators in wind turbines [4].

Ma X et al. established a dynamic model of a twin-spool aero-engine, analyzed the vibration response caused by friction, and discussed the influence of rotational speed and speed ratio on the frictional vibration. Research results showed that when friction occurs, the combined frequency components in spectrum can be used as diagnostic frequencies for friction faults in twin-spool aircraft engines [6].

Based on Isograph software and fault tree analysis, Yang F et al. drew the vibration fault tree and studied the abnormal vibration fault of a compressor in the component test of an aero-engine [7].

Dhamande LS et al. carried out the vibration measurement in the conditions of different speed and load, and extracted new composite fault features by continuous and discrete wavelet transform of vibration signals [11].

Zvonarev et al. eliminated the influence of the initial phase of the engine, and used the instantaneous value of rotor oscillation phase to diagnose defects and faults that cause changes in rotor stiffness and damping parameters, thereby identifying the state of the turbo-jet engine rotor [15].

Lu Y et al. proposed a fault diagnosis method for aircraft engines based on vibration signals from host measurement points. By analyzing the vibration signals, preliminary analysis and judgment were made on the general location of engine faults, structural fault forms, bearing failures, and other issues [18].

In conclusion, the monitoring and diagnosis method based on vibration test and signal analysis was an effective method for early fault prediction of the aero-engine rotor system. However, the above studies rarely involved the vibration detection and fault analysis during the ground test of a twin-spool turbo-jet engine. In addition, the researchers focused on how to find out the misalignment and rubbing-induced faults, and rarely considered how to eliminate the faults. During the ground test, the vibration detection and analysis of the twin-spool turbo-jet engine is an important step to ensure the performance and quality of the engine. The difficulties in vibration analysis of the engine during ground test mainly include a weak signal and noise interference. These difficulties require researchers to develop a vibration measurement method that can accurately capture and analyze weak signals and establish a standardized vibration measurement system. At the same time, appropriate signal processing technology is also needed to extract vibration characteristics, so as to effectively diagnose and prevent faults. Therefore, in this paper, based on the ground test of a twin-spool turbo-jet engine, real-time vibration signal in the time-domain was acquired, the rotor system vibration energy of the twin-spool turbo-jet engine was analyzed in the frequency domain, and the position and degree of the vibration source were identified, which laid the foundation for subsequent vibration reduction control research.

2. Vibration Test System

2.1. Main Structure of the Twin-Spool Turbo-Jet Engine

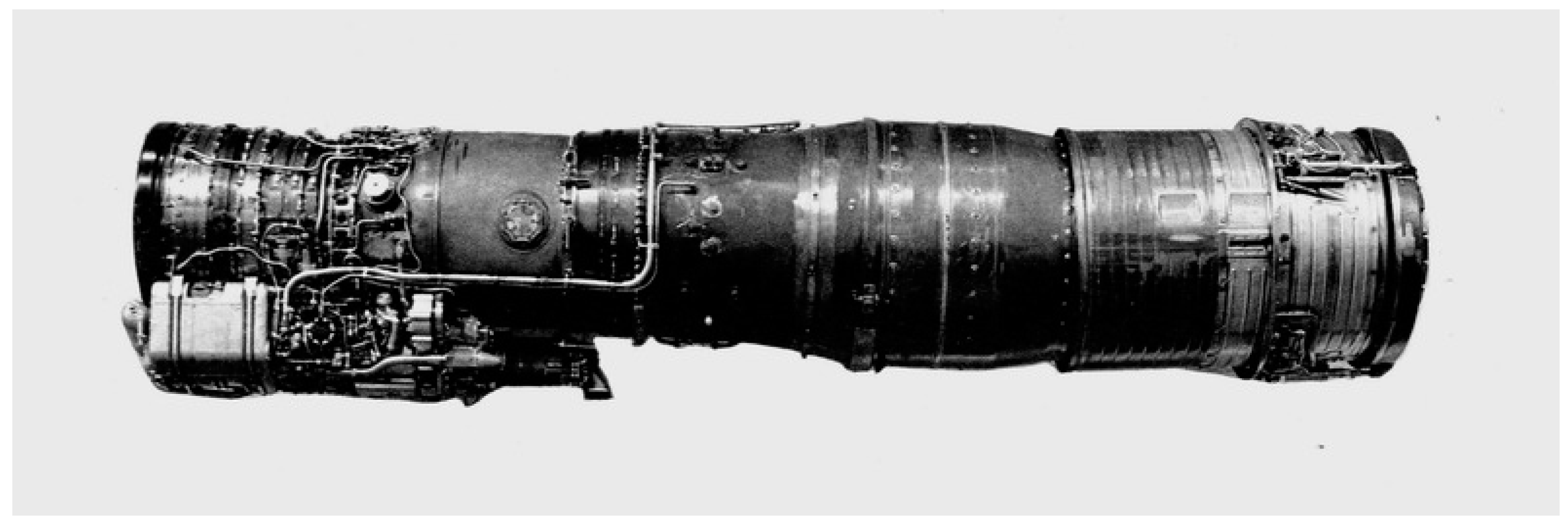
Figure 1 illustrates the twin-spool turbo-jet engine with an afterburner tested in this paper. The twin-spool turbo-jet engine mainly consists of an eight stage axial flow compressor, an annular combustion chamber, a two stage axial flow turbine, and an afterburner. The compressor consists of a row of guide vanes, a three stage low pressure compressor, and a five stage high pressure compressor. The main combustion chamber is located between the compressor and the turbine. The turbine consists of a row of nozzle guide vanes, a single stage high pressure turbine, and a single stage low pressure turbine. The three stage low pressure compressor rotor is splined with the low pressure turbine rotor to form the low pressure rotor system of the aero-engine. The five stage high pressure compressor rotor is splined with the high pressure turbine rotor to form the high pressure rotor system of the aero-engine [20].

2.2. Vibration Test Method of the Twin-Spool Turbo-Jet Engine

In order to monitor the operating status of the turbo-jet engine, it is necessary to pick up its vibration signal, effectively analyze the vibration signal through appropriate tools to extract relevant vibration characteristic information, and lay the foundation for fault diagnosis and analysis.

2.2.1. Vibration Evaluation Parameters

According to theoretical analysis and long-term test results, vibration overload coefficient (K) and double amplitude (S) are specified as the vibration evaluation parameters of a turbo-jet engine.

The vibration overload coefficient K is defined as the ratio of the vibration acceleration at the gravity center of the aero-engine to gravitational acceleration during operation, which represents the vibration level of the whole aero-engine:

$$K={\displaystyle \frac{a}{g}}={\displaystyle \frac{{(2\pi f)}^{2}A}{g}}\approx {\displaystyle \frac{S{N}_S}{500}}$$

(1)

In which a is the acceleration amplitude of the rotor, $a=A\times {\omega }^2$, A is the amplitude, S represents the double amplitude (the peak-to-peak value), S = 2A, f is the rotation frequency of the aero-engine rotor, that is the fundamental frequency of the rotor,

$$f={\displaystyle \frac{\omega }{2\pi }}=N_S$$

(1)

According to the vibration overload coefficient K based on gravitational acceleration g, the evaluation standard of the vibration amplitude is simplified, and it becomes possible to compare the vibration intensity of various types of aero-engines. In addition, a standard basis for the design of the aero-engine structure is provided.

(2)

K value is mostly measured with vibration speed sensors in the aero-engine test. The relationship between the output value of the vibrometer and the double amplitude is corrected based on the vibration calibration table, and the calibration curve is given. The low-pass filter of the vibrometer is dependent on the aero-engine rotational speed and the selected resonance frequency.

(3)

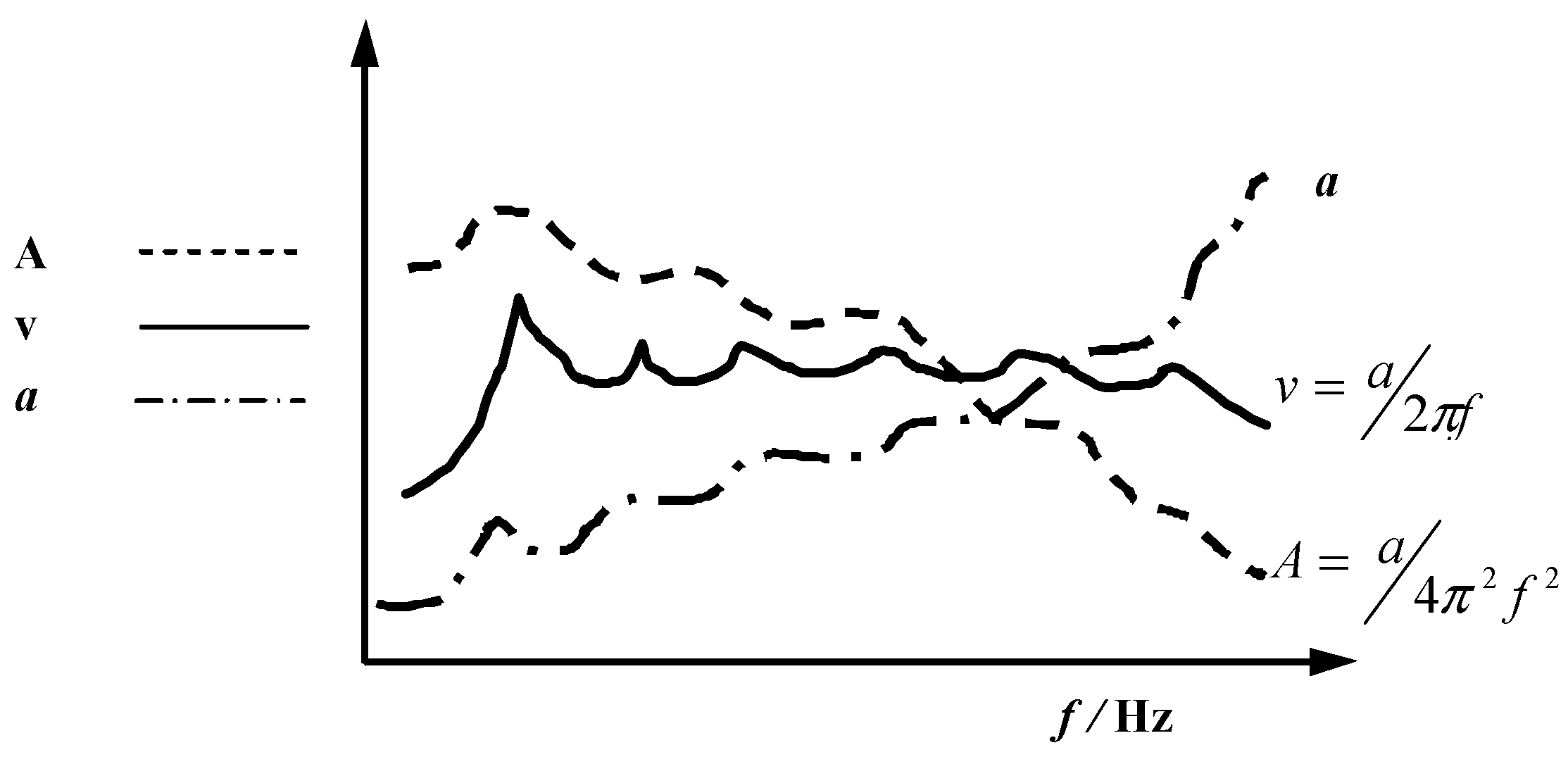
Differential and integral operations can be used in the frequency domain to perform operational conversions on double amplitude (S), velocity (v), and acceleration (a). Figure 2 illustrates the calculus relationship of the vibration amplitude (A), velocity (v), and acceleration sensor output signals (a).

It can be seen from Figure 2 that the displacement sensor is more sensitive in the low-frequency range and the acceleration sensor is more sensitive in the high-frequency range. The speed sensor is selected to maintain the sensitivity unchanged over a relatively wide frequency band.

2.2.2. Requirements Related to Vibration Measurement

According to the testing and installation conditions of the turbo-jet engine and the frequency range of the measured parameters, four vibration measurement points at the twin-spool turbo-jet engine are selected. Due to the influence of the rigidity of the test bench, the vibration overload coefficient measured by the same engine installed on a different engine test bench may be different. Therefore, the standard prototype of the same type of engine is used to carry out the bench calibration. In addition, due to the limitation of the installation position of the vibration sensor and the structure of support settings, the vibration threshold of the same engine is also different; it is necessary to obey the rules of the test technology. Moreover, the vibration sensors are also calibrated before measuring.

According to the technical specification of the turbo-jet engine, the maximum allowable vibration overload coefficients at the horizontal and vertical directions of the compressor casing and turbine casing cannot exceed certain values under various operating conditions. Therefore, four vibration measuring points were located respectively at the horizontal positions and the vertical positions of the compressor casing and the turbine casing.

3. Power Spectrum Analysis

In addition to performing various processing on signals to eliminate or reduce the effects of noise and interference, it is also necessary to preprocess the vibration signal and extract feature information to recognize the operating state and diagnose faults effectively. After years of theoretical research and experimental verification, some characteristics related to the equipment operating state or certain faults have been obtained and confirmed. Therefore, the purpose of signal analysis and processing for fault diagnosis is to remove false information, extract feature information related to the operating status or fault of the equipment, and make it prominent through various analysis and processing methods, thereby improving the real-time performance of status monitoring and the accuracy of fault identification.

The signal power of the unit frequency band is called the power spectrum density function, referred to as the power spectrum, and the power spectrum is usually calculated using Welch’s method of periodograms. Power spectrum includes self power spectrum and cross spectrum analysis. The self power spectrum and the amplitude spectrum provide the same amount of information, but under the same conditions, the self power spectrum is clearer than the amplitude spectrum. The self power spectrum can be obtained by performing Fourier transform on the autocorrelation function of the signal, or it can be calculated from the amplitude spectrum.

In the frequency domain, frequency components can be directly processed, which avoided calculus operations in the time domain and simplified the calculation process. In the vibration analysis of aircraft engine rotor systems, power spectrum analysis can help identify the vibration characteristics of the rotor system. For the purpose of improving the efficiency and accuracy of power spectrum analysis, the following issues must be considered.

(1)

In order to prevent spectral aliasing, the signal before transmitting to the computer needs to filter out frequency components higher than half of the sampling frequency to ensure that the sampled signal reflects the characteristics of the original signal accurately by low-pass filtering.

(2)

It is essential to choose a suitable window function in order to minimize spectrum leakage.

(3)

The relevant inspection and calibration must be performed before the system is used.

4. Characteristic Frequency

4.1. Characteristic Frequency under Maximum Power State

The vibration characteristic frequencies of the rotors under the state of maximum power are shown in

Table 1. Vibrational characteristic frequencies of the rotors under the maximum power state.

	Fundamental Frequency /Hz	1/2 Octave /Hz	Second Harmonic Frequency/Hz	Third Harmonic Frequency/Hz	Forth Order Harmonic Frequency/Hz
High	f2M = 190.0	${\displaystyle \frac{1}{2}}{f}_{2M}=95$	2 f2M = 380	3 f2M = 570	4 f2M = 760
Low	f1M = 186.5	${\displaystyle \frac{1}{2}}{f}_{1M}=93.3$	2 f1M = 373	3 f1M = 559.5	4 f1M = 746

. Among them, f_1M represents the fundamental frequency of the low pressure rotor, while f_2M represents the fundamental frequency of the high pressure rotor. In addition, the fundamental frequency of the starting generator gear is 141.4 Hz under the state of maximum power.

Under the state of maximum power, the rotation speed of the low pressure rotor is 11,190 rpm, and the first stage of the compressor has 24 blades. Therefore, the first stage compressor blade passing frequency is 4476 Hz. Further, the second harmonic frequency of the intermediate bearing roller is 616.6 Hz.

4.2. Characteristic Frequency under Afterburning State

Table 2. Vibrational characteristic frequencies of the rotors under the afterburning state.

	Fundamental Frequency /Hz	1/2 Octave /Hz	Second Harmonic Frequency/Hz	Third Harmonic Frequency/Hz	Forth Order Harmonic Frequency/Hz
High	f2A = 190.4	${\displaystyle \frac{1}{2}}{f}_{2A}=92.5$	2 f2A = 380.8	3 f2A = 571.2	4 f2A = 761.6
Low	f1A = 187.7	${\displaystyle \frac{1}{2}}{f}_{1A}=93.8$	2 f1A = 375.4	3 f1A = 563.1	4 f1A = 750.8

illustrates the vibrational characteristic frequencies of the rotors under the state of afterburning. Among them, f_1A represents the fundamental frequency of the low pressure rotor, while f_2A represents the fundamental frequency of the high pressure rotor. In addition, the fundamental frequency of the starting generator gear is 141.7 Hz under the state of afterburning.

5. Vibration Power Spectrum Analysis

5.1. Maximum Power State

5.1.1. Vibration Power Spectrum Analysis at Compressor Casing under the State of Maximum Power

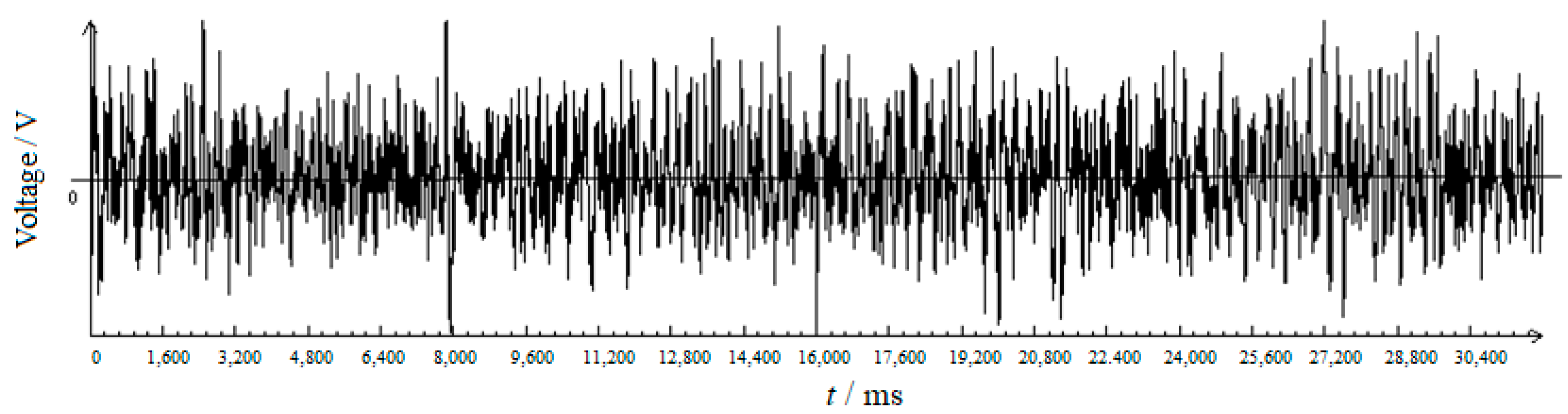
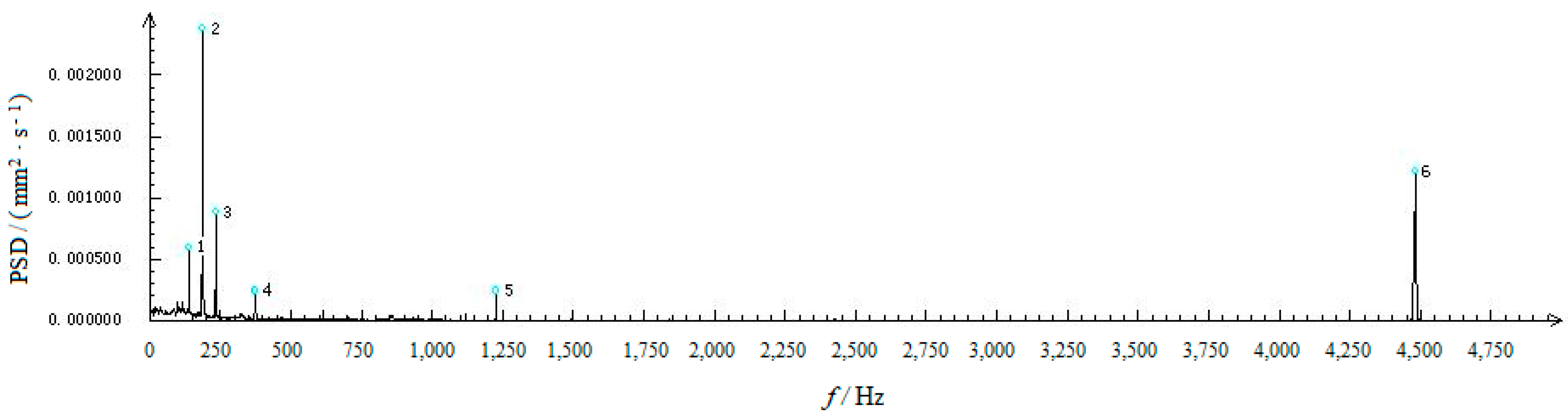
The vibration waveform measured at the casing for the turbo-jet engine compressor is shown in Figure 3, and Figure 4 shows the corresponding power spectrum.

As seen in Figure 4, a peak value appeared in the power spectrum at 141.8 Hz, and the fundamental frequency of the starting generator gear under the state of maximum power was 141.4 Hz, reflecting the starting generator gear imbalance. The second spike appeared in the power spectrum at 189.9 Hz near the fundamental frequency of the low pressure rotor (186.5 Hz), which indicated the severity of the low pressure rotor imbalance. Another peak value appeared in the power spectrum at 374.3Hz near the second harmonic frequency of the low pressure rotor (373 Hz), which represented low pressure rotor misalignment or radial stiffness unevenness. The fifth peak value was obtained in the power spectrum at 1227.6 Hz near the second harmonic frequency of the middle bearing roller (the fundamental frequency 616.6 Hz), which represented the second harmonic vibration induced by rotor misalignment. The sixth peak value appeared at 4481.7Hz in the power spectrum, which was equivalent to the pneumatic pulse vibration formed by the twenty-four rotor blades of the first stage compressor under the excitation of the low pressure rotor rotation frequency (4476 Hz).

5.1.2. Vibration Power Spectrum Analysis at Turbine Casing under the State of Maximum Power

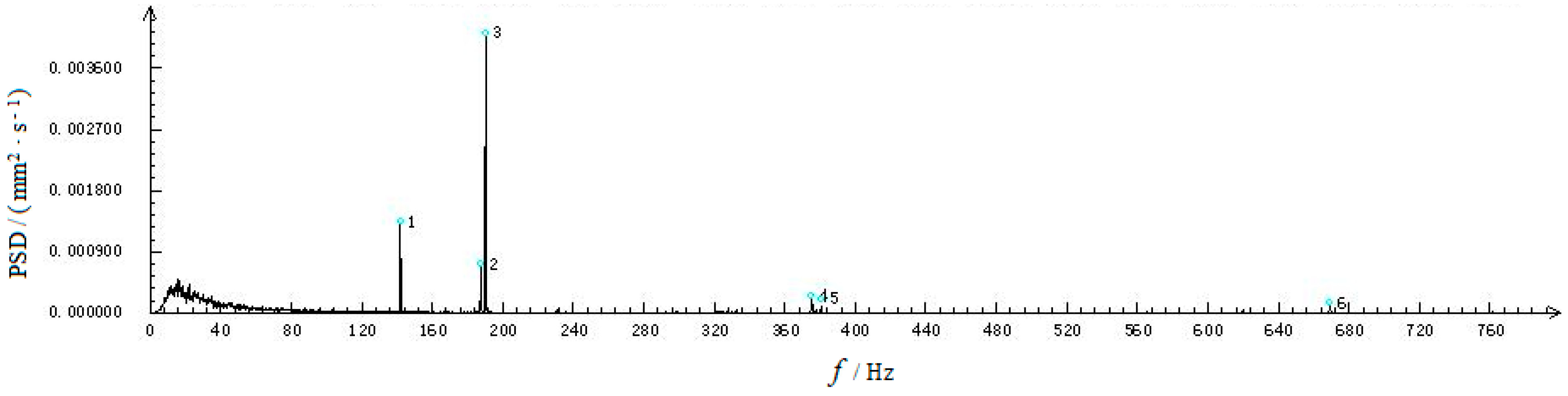
Figure 5 illustrates the vibration waveform collected at the casing for the turbo-jet engine turbine under the state of maximum power, and Figure 6 shows the power spectrum of Figure 5.

As seen in Figure 6, the first value peak appeared in the power spectrum at 141.8 Hz, and the fundamental frequency of the starting generator gear at the state of maximum power was 141.4 Hz, reflecting the severity of the starting generator gear imbalance. The second spike appeared in the power spectrum at 187.1 Hz near the fundamental frequency of the low pressure rotor (186.5 Hz), which represented the severity of the low pressure rotor imbalance. The fourth spike appeared in the power spectrum at 374.8 Hz near the second harmonic frequency of the low pressure rotor (373 Hz), which represented the low pressure rotor misalignment or radial stiffness unevenness. The fifth spike appeared in the power spectrum at 377.8 Hz near the second harmonic frequency of the high pressure rotor (380 Hz), which reflected the severity of high pressure rotor misalignment.

Around the sixth, seventh, and eighth spikes, there were continuous frequencies of approximately aperiodic signals, with a center frequency close to the fourth harmonic frequency of the high pressure rotor (760 Hz), which did not happen at the casing for the turbo-jet engine compressor. This was due to the dynamic and static rubbing faults at the sealed teeth of the turbine rotor experienced, which were caused by the misalignment of the rotor or the runout of the turbine end face. This phenomenon occurs occasionally under the states of maximum power and the afterburning of the twin-spool turbo-jet engine.

5.2. Afterburning State

5.2.1. Vibration Power Spectrum Analysis at Compressor Casing under the State of Afterburning

The vibration waveform measured at the casing for the twin-spool turbo-jet engine compressor is shown in Figure 7, and Figure 8 illustrates the corresponding power spectrum.

As seen in Figure 8, the first spike appeared in the power spectrum at 141.9 Hz, and the fundamental frequency of the starting generator gear under the state of afterburning was 141.7 Hz, reflecting the starting generator gear imbalance. The second peak value was obtained in the power spectrum at 187.7 Hz near the fundamental frequency of the low pressure rotor (187.7 Hz), which represented the severity of the low pressure rotor imbalance. The third spike appeared in the power spectrum at 190.4 Hz near the fundamental frequency of the high pressure rotor (190.4 Hz), which represented the severity of high pressure rotor imbalance. The fourth spike appeared in the power spectrum at 375.4 Hz near the second harmonic frequency of the low pressure rotor (375.4 Hz), which represented low pressure rotor misalignment or radial stiffness unevenness. The fifth spike appeared in the power spectrum at 380.9 Hz near the second harmonic frequency of the high pressure rotor (380.8 Hz), which represented the severity of high pressure rotor misalignment.

5.2.2. Vibration Power Spectrum Analysis of the Turbine Casing under the State of Afterburning

The vibration waveform collected at the casing for the twin-spool turbo-jet engine turbine is shown in Figure 9, and Figure 10 shows the corresponding power spectrum of Figure 9.

As seen in Figure 10, the first spike appeared in the power spectrum at 141.9 Hz, and the fundamental frequency of the starting generator gear at the state of afterburning was 141.7 Hz, reflecting the severity of the starting generator gear imbalance. The second peak value was obtained in the power spectrum at 187.7 Hz near the fundamental frequency of the low pressure rotor (187.7 Hz), which represented the severity of the low pressure rotor imbalance. The third spike appeared in the power spectrum at 190.4 Hz near the fundamental frequency of the high pressure rotor (190.4 Hz), which represented the severity of high pressure rotor imbalance. The fifth spike appeared in the power spectrum at 376.8 Hz near the second harmonic frequency of the low pressure rotor (375.4 Hz), which represented low pressure rotor misalignment or radial stiffness unevenness. The sixth spike appeared in the power spectrum at 380.9 Hz near the second harmonic frequency of the high pressure rotor (380.8 Hz), which represented the severity of high pressure rotor misalignment.

Around seventh, eighth, and ninth spikes, there were continuous frequencies of approximately aperiodic signals, with a center frequency close to the fourth harmonic frequency of the high pressure rotor (761.6 Hz), which did not happen at the casing for the turbo-jet engine compressor, similar to that of maximum power state.

6. Conclusions

During the test process of an aero-engine, although some vibration components are relatively large, they are stable and do not change with time, which is not harmful to the engine operation. However, some smaller frequency components, especially the components that grow rapidly, often contain symptoms of failure. In particular, it should be noted that some frequency components that do not exist in the original spectrum or are relatively weak suddenly appear and soar, which may damage the normal working state of the engine in a short time. Based on the power spectrum analysis of the low-frequency bands at the casings for the compressor and turbine of a twin-spool turbo-jet engine under the states of maximum power and afterburning, there were some important results that were achieved, as follows.

Firstly, the main rotor vibration excited by mass imbalance always dominated, and it was essential to increase the dynamic balance accuracy and enhance the performance and precision of the engine to reduce the vibration caused by mass imbalance. Usually, if the remaining imbalance of the compressor and turbine rotor is reduced by half, the vibration amplitude would decrease by about 5%. The faults caused by rotor imbalance are easy to identify and correct, but cannot be completely eliminated.

Secondly, due to the varying degrees of deformation of the rotor when subjected to radial forces, such as main shaft misalignment or uneven stiffness distribution in the radial direction, the second harmonic vibration component was significant. For rigid couplings and gear couplings, the radial excitation frequency was mainly dominated by the fundamental frequency and the second or fourth harmonic frequency, accompanied by high-frequency doubling. By using higher precision main bearings, it is possible to reduce bearing roundness errors and roundness harmonics, thereby reducing the influence of second harmonic components.

Thirdly, the twenty-four rotor blades of the first stage of the compressor were excited by the fundamental frequency of the low pressure rotor, resulting in a quantity of pneumatic pulse vibration.

Finally, there was obvious vibration near the fourth harmonic frequency at the casing for the turbo-jet engine turbine, but it was not apparent at the casing for the turbo-jet engine compressor, which may result from the friction faults between the static and dynamic components at the sealed teeth of the turbine rotor caused by the rotor misalignment or the runout of the turbine end face. In this case, friction faults can be reduced by aligning the rotor and stator correctly in the axial and radial directions and optimizing the position of the sealing teeth.

Vibration analysis of the areo-engine is an important field of aero-engine condition monitoring, but the simple data-driven vibration analysis method is suitable for experienced researchers. In recent years, convolutional neural networks (CNNs) have made a series of breakthrough research results in various signal and information processing fields, which greatly improves the accuracy of fault detection methods [21]. Ultrasonic guided wave (UGW) testing realizes large-scale nondestructive testing of large structures because of its advantages of high propagation efficiency and low energy consumption [22]. In the follow-up research, based on the above research results, deep learning and ultrasonic guided wave technology will be further explored to realize the vibration optimization design of aero-engine under the condition of high background noise, so as to reduce the overall vibration level of the aero-engine and ensure flight safety.