3.1. The Results of the Effect of Turbulence Intensity on Cascades
Total pressure loss coefficient (Yp) is widely regarded as a key parameter for reflecting the energy consumption and flow characteristics inside a turbine. In turbine research, reducing turbine losses is a goal that is pursued. The total pressure loss coefficient is defined in Formula (2). As shown in
Figure 7, with the increase in turbulence intensity, the total pressure loss of the blade row significantly increases. When turbulence intensity increases from 1% to 26%, the total pressure loss increases by 14.6%. Under high turbulence intensity, the increase in loss is progressive. An anomaly occurs at low turbulence intensity, where the total pressure loss peaks at Tu = 2%, but at Tu = 4%, 6%, and 8%, the total pressure loss coefficients are significantly lower than at Tu = 2%, decreasing by 6.7%, 2.6%, and 0.8%, respectively. Given the above circumstances, this paper selects several special operating conditions at low turbulence intensity for investigation.
In the cascade passage, due to the influence of turbulence intensity, variations not only exist in secondary flow loss but also in profile loss across different spanwise and circumferential regions.
Figure 8 and
Figure 9 depict the distribution of total pressure losses downstream of the cascade. These figures clearly demonstrate that areas with high losses are predominantly concentrated in the boundary layer along the suction surface, as well as in the secondary flow and corner regions. Through analysis of the spanwise distribution curve of circumferential average total pressure loss, it is evident that blade profile loss decreases with increased turbulence intensity. However, secondary flow losses exhibit a noticeable increase. Comparing these findings, it is apparent that as turbulence intensity increases, the high-loss region associated with secondary flow moves closer to the blade. This trend becomes less pronounced when Tu > 2%, indicating that turbulence intensity also influences the spanwise migration of the secondary flow.
The near-endwall flow structure exhibits significant differences under varying inlet turbulence intensities.
Figure 10 compares the total pressure loss distribution downstream of the cascade at different axial positions for turbulence intensities of 1% and 6%. It is evident that with higher turbulence intensity, the high-loss region downstream of the cascade becomes more extensive. Specifically,
Figure 10c shows that at an axial position of Cax = 140% in the near-end zone, there is only one high-loss region under low turbulence intensity (Tu = 1%). However, under higher turbulence intensity (Tu = 6%), the high-loss region expands significantly, presenting two closely spaced high-loss cores corresponding to the counter vortex and passage vortex. The development of endwall secondary flow has always been a focal point in turbine aerodynamic research.
Figure 11 uses the flood of secondary kinetic energy (C
SKE) to illustrate the distribution of secondary kinetic energy intensity downstream of the turbine cascade. Regions with higher secondary kinetic energy closely coincide with areas of higher total pressure loss, and the intensity of secondary kinetic energy exhibits a positive correlation with turbulence intensity. As the distance from the cascade exit increases, the intensity of secondary kinetic energy gradually decreases.
Figure 12 displays the distribution of vorticity downstream of the cascade. The counter-rotating vortex and passage vortex are precisely located in regions of high total pressure loss. With an increase in turbulence intensity, the vorticity intensity also increases, causing the distance between the cores of the two vortices to expand. This results in the two high-loss cores observed in
Figure 10f. Under low turbulence intensity, due to the lower vorticity intensity, the high total pressure loss core is positioned exactly in the middle region between the two vortices.
Ref. [
26] provides a detailed definition of the coefficient of secondary turbulent kinetic energy (C
SKE).
The SKE is defined as
where
,
, and
are the velocity components in the spanwise, pitchwise, and axial directions, respectively.
and
and are the secondary (SEC) velocities. φ is the mass averaged exit flow angle in a plane. The non-dimensional secondary kinetic energy is defined as
where
is the mass averaged velocity at the inlet.
The streamwise vorticity coefficient is defined as:
where
,
, and
are the vorticity of spanwise, pitchwise, and axial directions.
3.2. Separation and Transition of Suction Surface Boundary Layer in Different Tu
Boundary layer separation on the surfaces of turbine cascades has a detrimental impact on turbine efficiency. Therefore, accurately determining the position of boundary layer separation is crucial before exploring methods to prevent or mitigate separation. Researchers have utilized different approaches to identify boundary layer separation, including analyzing the constant region (highlighted in the red box area in
Figure 13a of the cascade suction surface load (Cp) distribution and determining the position where the blade surface friction coefficient (Cf) becomes negative (as shown in
Figure 14)) [
22,
23,
24,
27]. In this section, these two methods are combined with the velocity distribution of the suction surface flow field to precisely capture the transition characteristics of boundary layer separation on the suction surface, considering variations in inlet turbulence intensity.
Figure 13a illustrates the distribution of the blade surface static pressure coefficient (Cp) along the axial direction (z) at the 50% spanwise position for five different levels of incoming turbulence intensity. Remarkably, the load distributions on the blade surface exhibit high consistency across the different turbulence intensities, indicating that the main flow structures within the flow passage are not significantly influenced by variations in incoming turbulence intensity.
As shown in
Figure 13, it is evident that at the 50% spanwise position, the load distribution on the blade surface fluctuates significantly at x/Cax = 80%. The red line (Tu = 1%) and black line (Tu = 2%) exhibit the most significant changes, followed by the green line (Tu = 4%). Taking the black line as an example, its load distribution curve displays a distinct gradient decline, forming a so-called “constant pressure platform” [
28]. This phenomenon is attributed to boundary layer separation on the blade surface, where the boundary layer exhibits weak backflow in the separation region. If separation is severe and large-scale, it significantly impacts the flow efficiency. Many researchers utilize the size and length of separation bubbles to define the extent of separation regions.
Figure 13 (right) also reveals that the separation bubbles formed by the incoming flow corresponding to the black line are larger than those formed by the red line. This indicates that turbulence intensity of the incoming flow influences the development of the boundary layer on the blade surface. As turbulence intensity increases, the boundary layer separation bubble becomes smaller. When Tu = 4%, the separation bubbles practically disappear, indicating smoother development of the boundary layer under this condition. When Tu > 4%, the separation bubble completely vanishes.
After boundary layer separation occurs, low-energy fluid accumulates and backflows on the surface of the cascade. As a result, the friction coefficient (Cf) of the cascade surface in the boundary layer separation region becomes negative.
Figure 14 illustrates the distribution of friction coefficient along the blade surface under different incoming flow conditions with varying turbulence intensities. The transition from positive to negative friction coefficient indicates boundary layer separation, while the transition from negative to positive signifies the reattachment of separated flow. Furthermore, it can be observed that when the incoming turbulence intensity is 1%, the boundary layer separates at approximately 80.7% of the axial chord (x/Cax = 0.807), and the separated flow reattaches at 88.6% of the axial chord (x/Cax = 0.886). Similarly, for an incoming turbulence intensity of 2%, the boundary layer separates at around 81.1% of the axial chord (x/Cax = 0.811), and reattachment occurs at 86.7% of the axial chord (x/Cax = 0.867). Conversely, at turbulence intensities of 6%, 8%, and 10%, no boundary layer separation is observed.
In summary, higher turbulence intensity in the incoming flow reduces the likelihood of boundary layer separation. Conversely, lower turbulence intensity makes it easier for the boundary layer to separate, resulting in a larger separation area. These findings indicate that in the analysis of
Figure 13, the sudden flattening of the static pressure coefficient gradient and the formation of a “constant pressure platform” effectively reflect the degree of boundary layer separation and the size of the separation area formed.
It is evident from
Figure 14 that each curve of the friction coefficient (Cf) follows a similar three-stage pattern: a descending stage, a sudden rise with a steep gradient, and a subsequent gradual decline. The wall shear stress is often used to assess the flow conditions near the wall. Many researchers define the region where shear stress increases sharply as the boundary layer transition zone. In
Figure 14, the points marked with red five-pointed stars can be approximately regarded as the start points of boundary layer transition. As for the end points of transition, it can be identified as the extreme point where the Cf curve rises sharply and the gradient reaches zero at the onset of transition. It is evident that as the incoming turbulence intensity increases, boundary layer transition occurs earlier. This is because higher turbulence intensity corresponds to a significant increase in turbulent kinetic energy. When the laminar boundary layer develops to a certain extent, it becomes more influenced by the strong momentum of the mainstream flow, which promotes early boundary layer transition. This has a positive impact on the flow structure within the passage. Overall, these observations contribute to a better understanding of the flow behavior and provide insights into the promotion of flow structure.
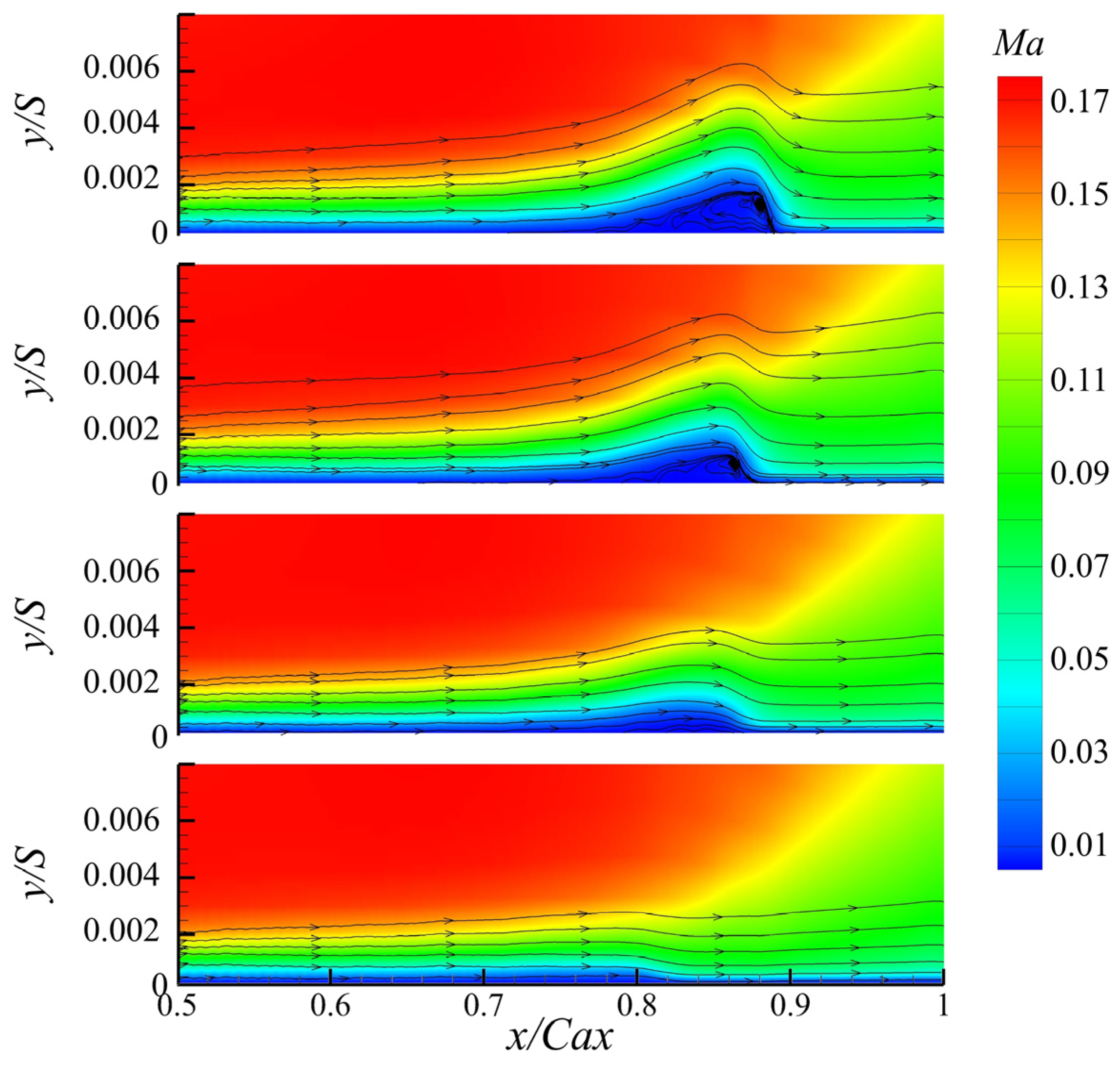
The development of the boundary layer is closely associated with the flow structure on the blade surface. To effectively visualize the evolution of the boundary layer near the separation region on the suction surface, this paper presents a comparative analysis of the velocity along the wall of the suction surface for four different turbulence levels, as illustrated in
Figure 15. This figure clearly demonstrates the changes in separation bubble size, the axial location of the separation starting point, and the reattachment point of the boundary layer.
At a turbulence level of 1% in the incoming flow, the separation bubble attains its maximum thickness and length. The boundary layer initiates thickening from approximately 0.7 times the axial chord length, resulting in the accumulation of low-energy fluid on the wall area and the formation of separation bubbles characterized by backflow. This substantially raises the extent to which the boundary layer is lifted. The presence of low-energy fluid within the boundary layer has a direct impact on the flow passage, causing it to narrow and influencing the mainstream flow. The separation phenomenon concludes at an axial chord length of 0.94, with the boundary layer reattaching to the blade surface. At this point, the previously adhered layer of low-energy fluid on the blade surface becomes significantly thinner, transitioning into a turbulent boundary layer. This transition occurs due to the intense momentum exchange between the boundary layer and the mainstream flow following the lifting of the boundary layer, facilitating the transfer of energy from the mainstream to the low-energy fluid and resulting in the formation of a turbulent boundary layer.
However, the accumulation of low-energy fluid in the separation bubble region causes a constriction in the flow passage, adversely affecting the mainstream flow and leading to increased energy loss. This hampers the achievement of an optimal flow state within the cascade. Therefore, it is imperative to minimize such occurrences in order to maintain a favorable flow structure.
To validate the transition of the boundary layer near the separation bubble region,
Figure 15 offers a visual representation, while
Figure 16 presents velocity profiles at five axial positions for four different turbulence levels. At x/Cax = 0.78, the velocity patterns clearly indicate a laminar boundary layer across all turbulence levels. However, at x/Cax = 0.81, the velocity profile curve for Tu = 6.0% gradually shifts towards a turbulent boundary layer, exhibiting a fuller profile. This suggests the initiation of boundary layer transition. By x/Cax = 0.84, the velocity pattern of the boundary layer with Tu = 6.0% has completely transitioned into a turbulent boundary layer, consistent with the earlier analysis in
Figure 14, where the rise in the Cf curve gradient was identified as the transition starting point. Similarly, at x/Cax = 0.87, the velocity pattern of the boundary layer with Tu = 4.0% starts to show a saturation trend, indicating the onset of transition. Finally, at x/Cax = 0.90, the velocity pattern of the boundary layer with Tu = 4.0% has fully transformed into a turbulent boundary layer, resembling the behavior observed for Tu = 6.0%. This further validates the proposed transition starting and ending points and affirms the accuracy of this study.
In conclusion, the precision of the boundary layer transition analysis put forth in this study is reinforced by examining the distribution of velocity profiles. Additionally, the analysis presented in
Figure 16 establishes the starting points of the transition, aligning with previous research findings.
3.3. Flow Characteristics in the Blade Endwall in Different Tu
Different inlet turbulence intensities result in a thickening of the boundary layer upstream of the cascade leading edge. As shown in
Figure 17, when the turbulence intensity increases from 1% to 6%, the boundary layer thickness more than triples.
Figure 18 compares the turbulent kinetic energy coefficients under different turbulence intensities. The turbulence intensity increased from 1% to 4%, and the peak of C
SKE increased by more than three times. The turbulence intensity increased from 1% to 4%, and the peak of C
SKE increased by more than three times. The significant increase in C
SKE is concentrated in the downstream area of 50% x/Cax, which is also the location where secondary flow is rapidly developing.
Different turbulence intensities have varying effects on the migration characteristics of secondary flow and the pressure distribution on the cascade surface. To study these patterns more accurately,
Figure 19 presents the load coefficient distribution at three positions (10% span, 30% span, and 50% span) on the high-pressure turbine cascade under different turbulence intensities. A significant change in load distribution is observed at the 10% span position compared to the other positions (as shown by the black dashed line in
Figure 19). Specifically, the load factor at the leading edge of the blade decreases, while the load on the cascade surface near the trailing edge increases. This indicates a decrease in the pressure difference between the suction and pressure surfaces at the leading edge, resulting in increased pressure on the suction surface while maintaining the pressure on the pressure surface unchanged. Conversely, the suction surface pressure decreases at the trailing edge. Importantly, these phenomena become more pronounced with increasing turbulence intensity. This is because turbulence and vortices are generated as the airflow passes through the cascade passage, and these disturbances accumulate at the trailing edge of the blade, forming larger vortex structures. These structures impede airflow into the trailing edge, creating a lower static pressure area at the root of the blade. With higher turbulence intensity, the vortex structure becomes more distinct, resulting in even lower static pressure and increased load coefficient at the trailing edge of the cascade.
Figure 20 illustrates the static pressure distribution on the suction surface and endwall of the cascade. The large bending curvature of the cascade leads to the formation of a low-pressure zone in the flow passage. This region is typically located at the throat of the cascade and is influenced by a significant velocity gradient. As the fluid passes through the throat, it gradually slows down, leading to an increase in pressure. Near the trailing edge, a reverse pressure gradient forms, often causing an elevated risk of boundary layer separation. This phenomenon contributes to the formation of separation bubbles, as mentioned earlier.
Observing and analyzing fluid flow trajectories on the turbine blade’s surface provides valuable information about secondary flow, centrifugal force effects, and energy dissipation. Such knowledge plays a crucial role in turbine design optimization and performance analysis.
Figure 21 presents the distribution of limit streamlines on the endwall and suction surface to demonstrate flow trajectories on the blade surface and the influence of turbulence intensity on the development of separation bubbles and secondary vortex in the endwall. The limit streamline on the suction surface describes three-dimensional flow separation in the cascade passage, showing the migration path of the horseshoe vortex suction surface branch (S1), passage vortex (S2), and corner vortex (S3) from the endwall to the blade center. The image provides intuitive information on the size and position of separation bubbles on the suction surface. It can be observed that the spanwise length of separation bubbles accounts for approximately 40% of the cascade’s span under low turbulence intensity.
Figure 22 shows the change in the ratio of radial velocity to axial velocity, which indicates that the velocity of secondary migration from the suction surface to the center of the blade is proportional to turbulence intensity.
However, due to the closed separation bubbles on the suction surface at low turbulence intensities, the migration of S1 to the center of the blade is impeded. Consequently, the distance between S1 and S2 is relatively small at the trailing edge of the cascade. However, as the turbulence intensity increases and the separation bubble disappears, S1 loses its blocking effect, causing S1 and S2 to approach each other and then gradually separate as they migrate to the center of the blade. This has an impact on the subsequent development of the trailing filament vortex, and the follow-up
Figure 21 proves this conclusion. Therefore, the separation state of the boundary layer on the suction surface is closely related to the secondary flow in the endwall.
To further investigate the influence of inlet turbulence on the energy loss in the endwall of a high-pressure turbine cascade,
Figure 23 presents the results of static entropy at different axial positions within the cascade passage, and provides a three-dimensional streamline distribution in the endwall. The static entropy distribution shows that higher turbulence intensity results in greater energy loss. Conversely, in the high-loss region (red region), high turbulence intensity inhibits boundary layer separation, resulting in a reduction in the area of profile loss region (as shown by the black rectangle in
Figure 23). Generally, higher turbulence intensity can decrease profile loss caused by separation but introduces higher secondary flow loss.
The strip streamlines in the
Figure 24 demonstrate the trajectory of the passage vortex’s formation. The passage vortex originates from the suction surface branch of the horseshoe vortex at the cascade’s leading edge. As the mainstream enters the cascade passage, the flow passage gradually narrows, and the fluid begins to accelerate. Under the shear stress of the endwall, the streamlines near the endwall interweave, indicating the emergence of numerous vortex mechanisms. With increased turbulence intensity in the flow field, the vortex system becomes more prominent, migrates along the suction surface towards the cascade center, and gradually separates from the cascade surface.
It is very important to clarify the development process of secondary flow to reduce the loss caused by secondary flow. Vortex identification adopts the Q-criterion method. The Q-criterion vortex identification method is currently recognized and widely accepted as a method for identifying turbulent vortex structures [
29].
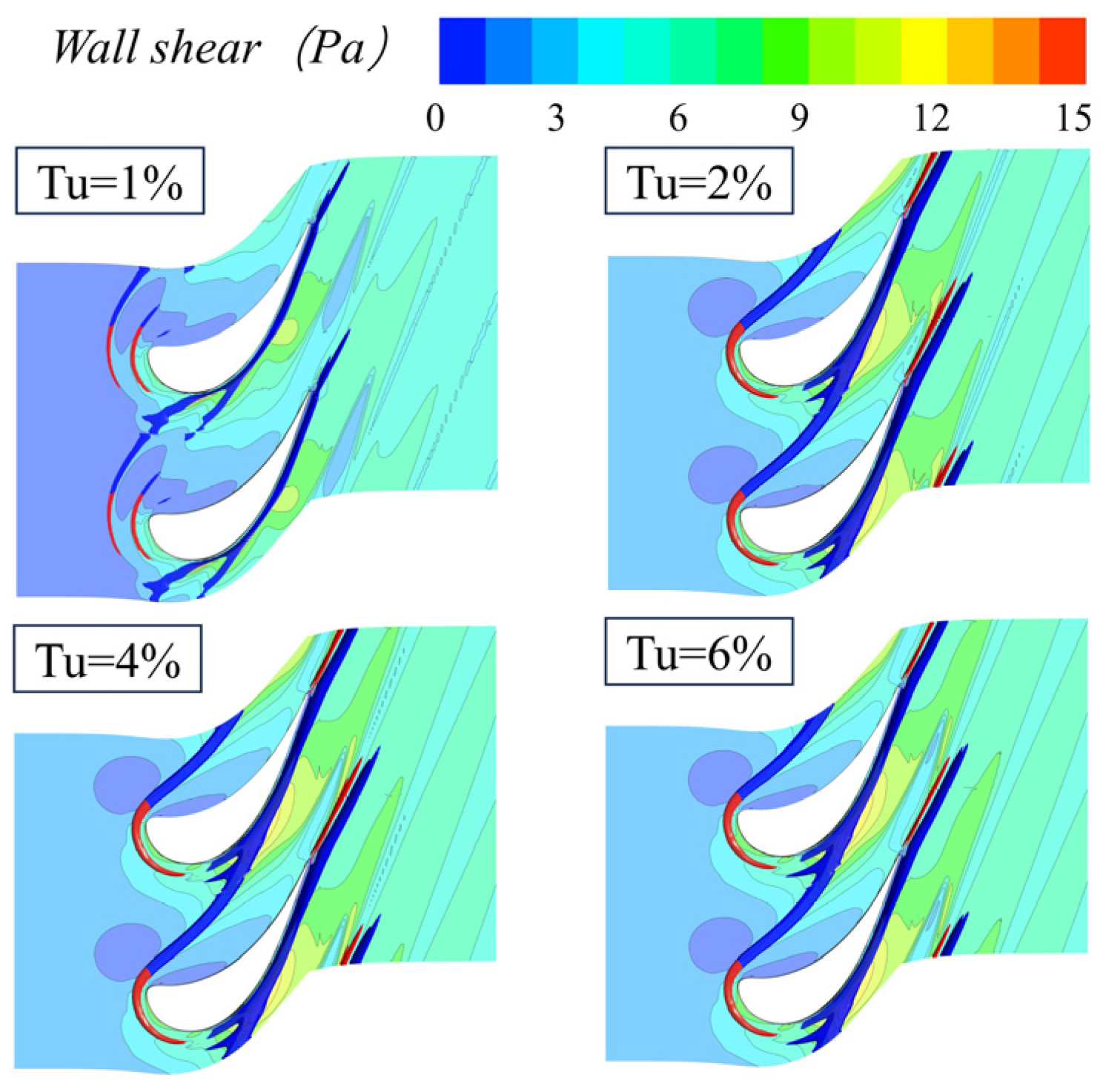
Figure 24 clearly shows that as the turbulence intensity increases, the identifiable vortex system becomes more obvious. When Tu = 1%, we can observe two tiny horseshoe vortices. However, these horseshoe vortices are so weak along the suction surface that they almost disappear when they migrate to the suction surface, which echoes the phenomenon that the proportion of low shear stress zone at the leading edge of the cascade is large. Usually, the high shear stress region of the endwall mainly occurs in the region affected by strong interference and strong secondary flow, which is related to the characteristics of higher turbulence intensity. The higher turbulence intensity will result in the formation of a stronger shear stress zone. After the passage vortex rises off, especially in the first half of the passage, the shear stress coefficient rises sharply. It can be observed that with the increase in turbulence intensity, the strong shear stress region extends to the region near the trailing edge, which means that the loss of the endwall boundary layer in this region also increases.
In order to further reveal the development characteristics of vortex structure in the passage of high-pressure turbine cascade under different turbulence intensities,
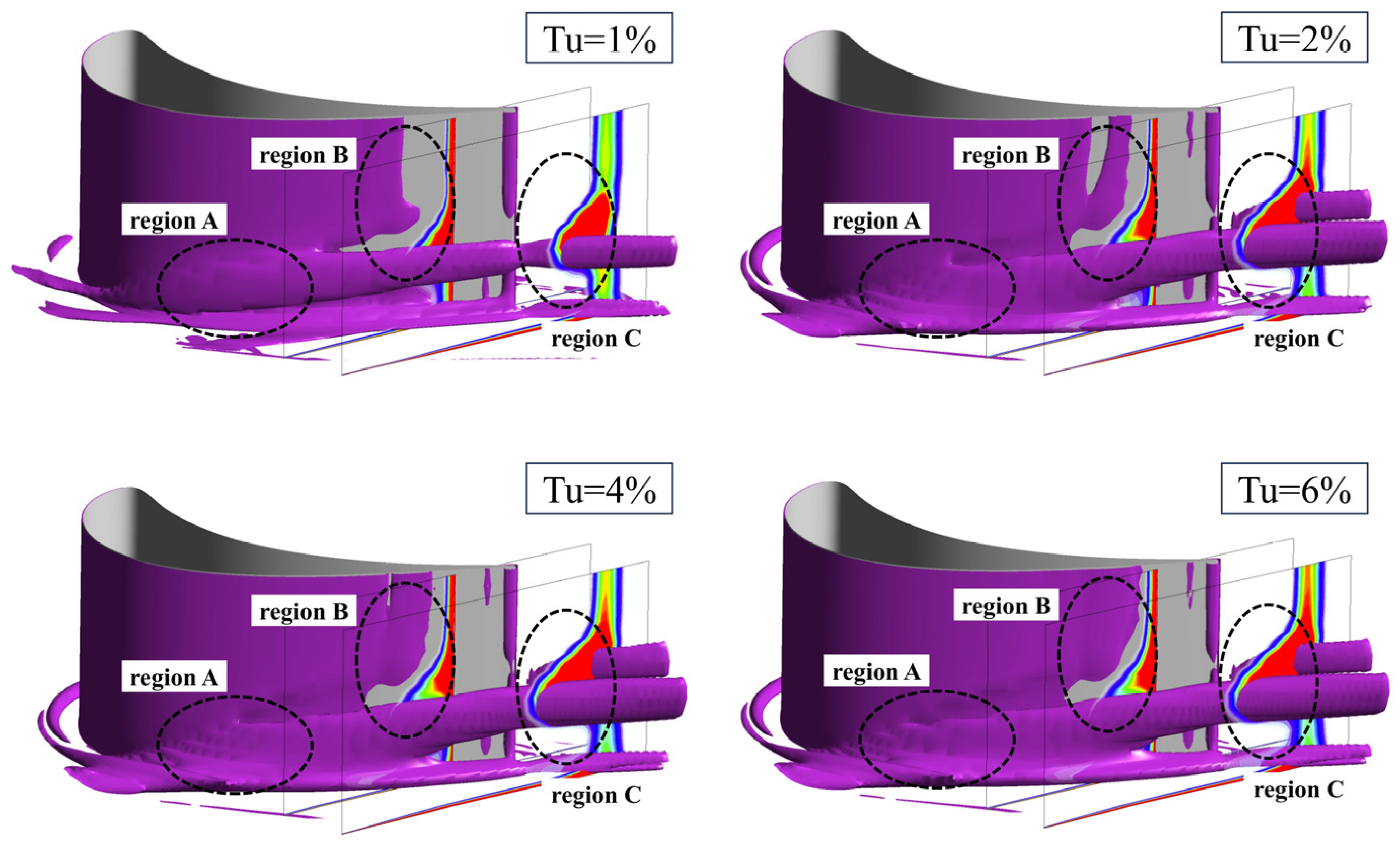
Figure 25 shows the vortex iso-surface map identified by Q-criterion and the total pressure loss distribution perpendicular to the axial direction near the trailing edge of the cascade. Many researchers extensively use the Q-criterion for vortex identification in the study of turbomachinery [
30,
31]. It can be seen from the whole vortex system structure that the counter vortex developed from the pressure surfaces of adjacent blades is mixed with the angular vortex. Due to the insufficient driving force of the pressure surface branch of horseshoe vortex, the low-energy fluid in the endwall boundary layer has a small rolling amplitude. In contrast, the passage vortex develops from the horseshoe vortex along the suction surface, and develops rapidly due to the large transverse pressure gradient. Eventually, the passage vortex strengthens and begins to approach the mid-blade position in the A region. The above characteristics are the common characteristics of vortex system development in the flow passage.
In order to study the variation in different losses in the cascade passages in a turbine, including secondary loss (SEC), suction surface profile loss (SBL), free flow loss (FS), and endwall loss (EW), the main positions and methods of these losses are determined in
Figure 26 according to the distribution relationship of the total pressure loss nephogram.
Figure 27 shows the proportion of these losses at different axial positions (Cax = 0%, Cax = 33.3%, Cax = 66.6%, Cax = 100%) under Tu = 1% and Tu = 6% conditions.
By comparison, it can be found that the secondary flow loss caused by the vortex system increases gradually with the mainstream from leading edge to trailing edge of cascade, and the secondary flow loss is greater under high turbulence. This is closely related to the structural strength of the generated vortex system, which shows that the secondary flow loss is mainly contributed by the vortex system. The proportion of free flow loss and endwall profile loss decreases along the axial direction, not because their true losses decrease, but because the strong secondary flow leads to an increase in the proportion of loss at the same axial position, which makes the relative proportion of free flow loss and endwall profile loss decrease. It is worth noting that the performance of profile loss on suction surface is better at high turbulence than at low turbulence. This is because the high turbulence intensity inhibits the boundary layer separation, which is consistent with the previous analysis results.