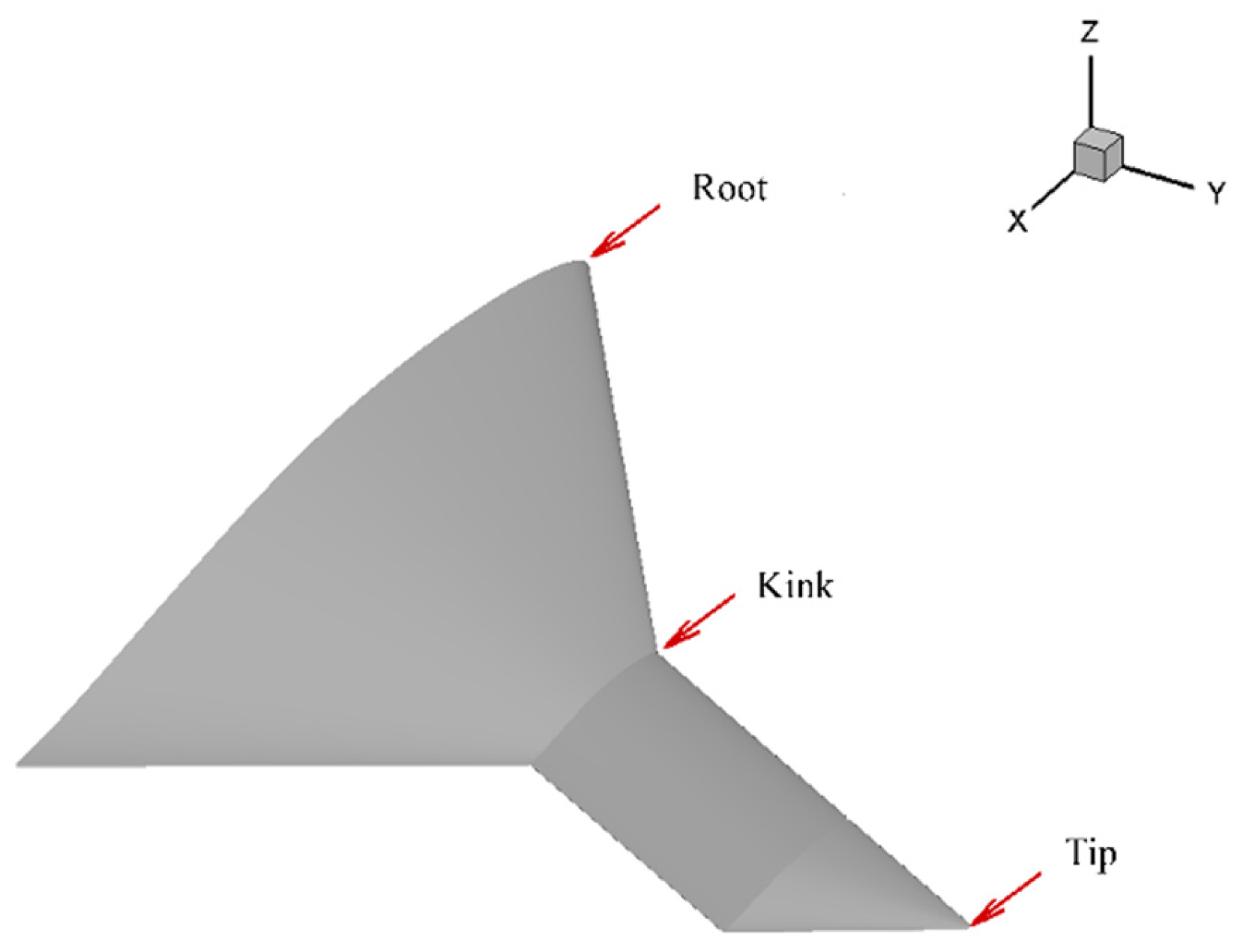
Figure 1.
Spanwise zoning of flying wing.
Figure 1.
Spanwise zoning of flying wing.
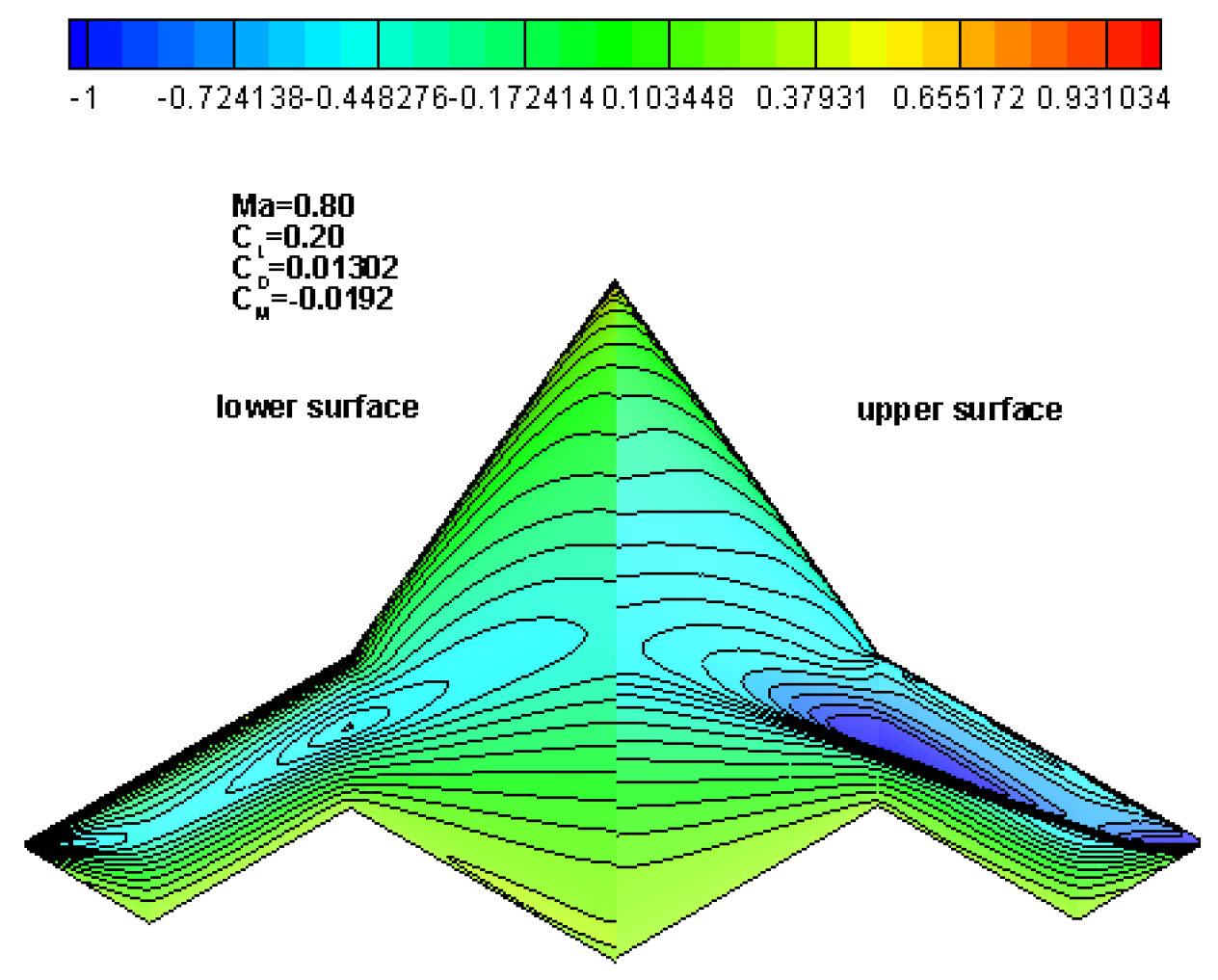
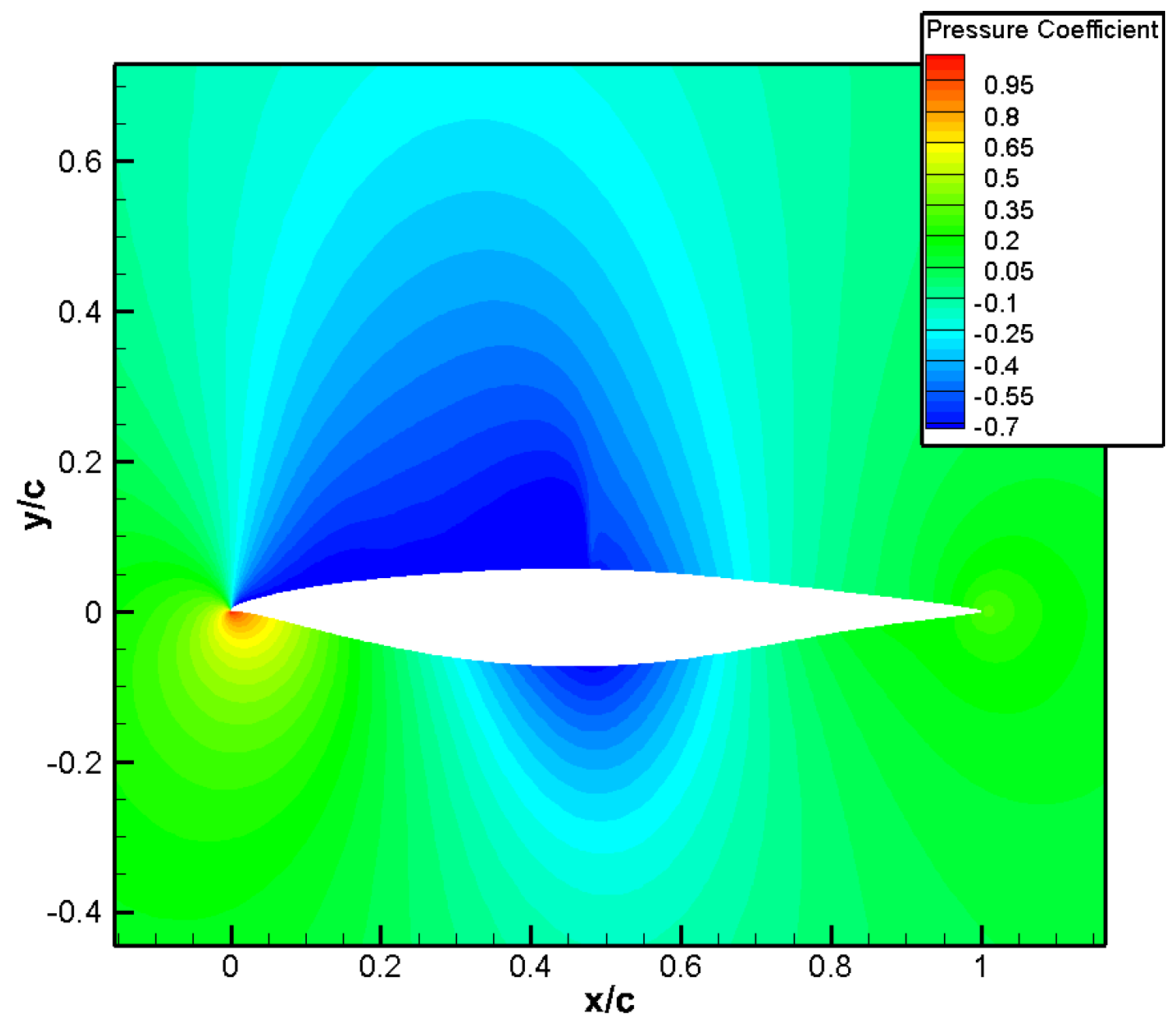
Figure 2.
Pressure distribution contour map.
Figure 2.
Pressure distribution contour map.
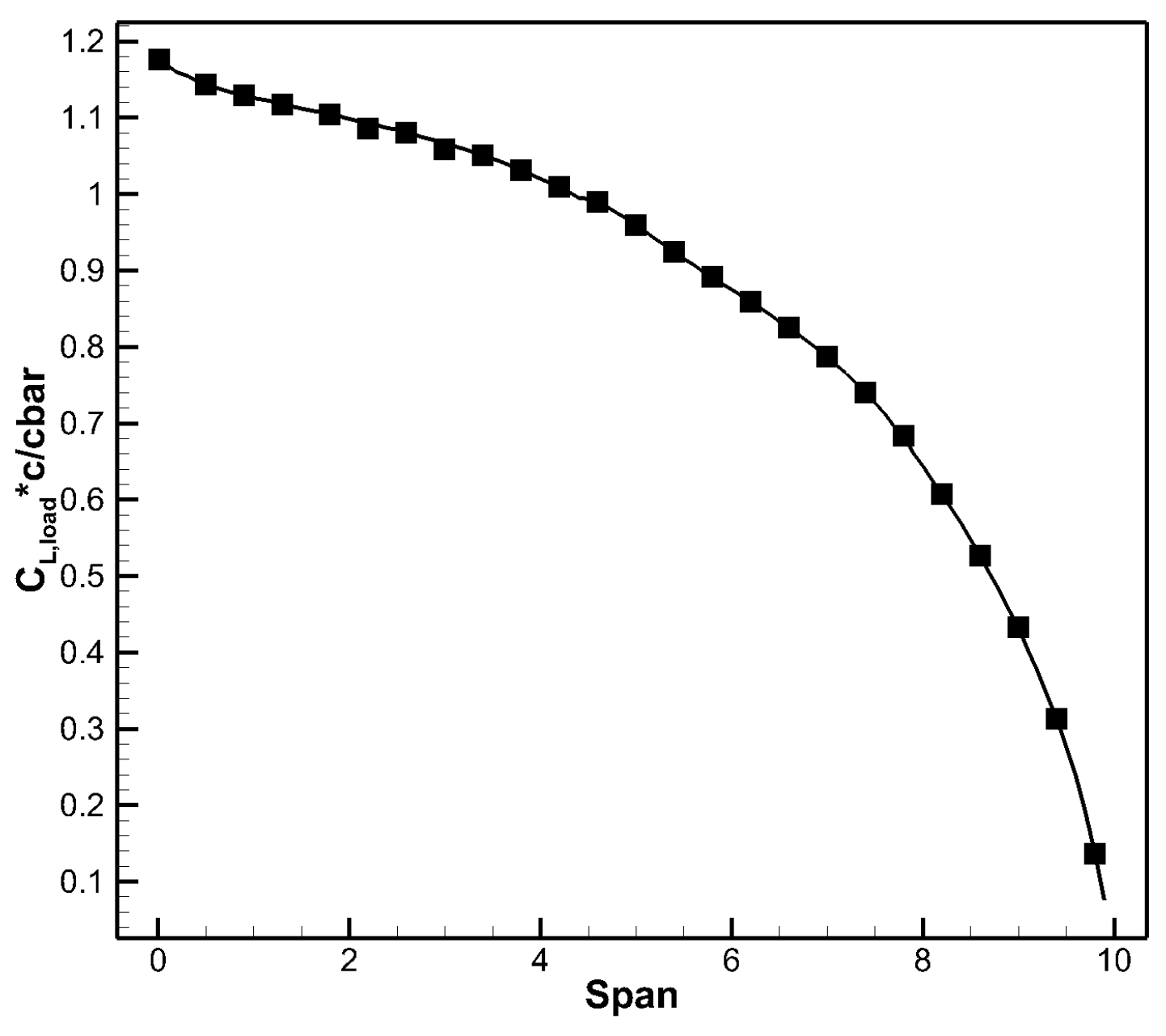
Figure 3.
Spanwise lift coefficient distribution.
Figure 3.
Spanwise lift coefficient distribution.
Figure 4.
Spanwise circulation distribution.
Figure 4.
Spanwise circulation distribution.
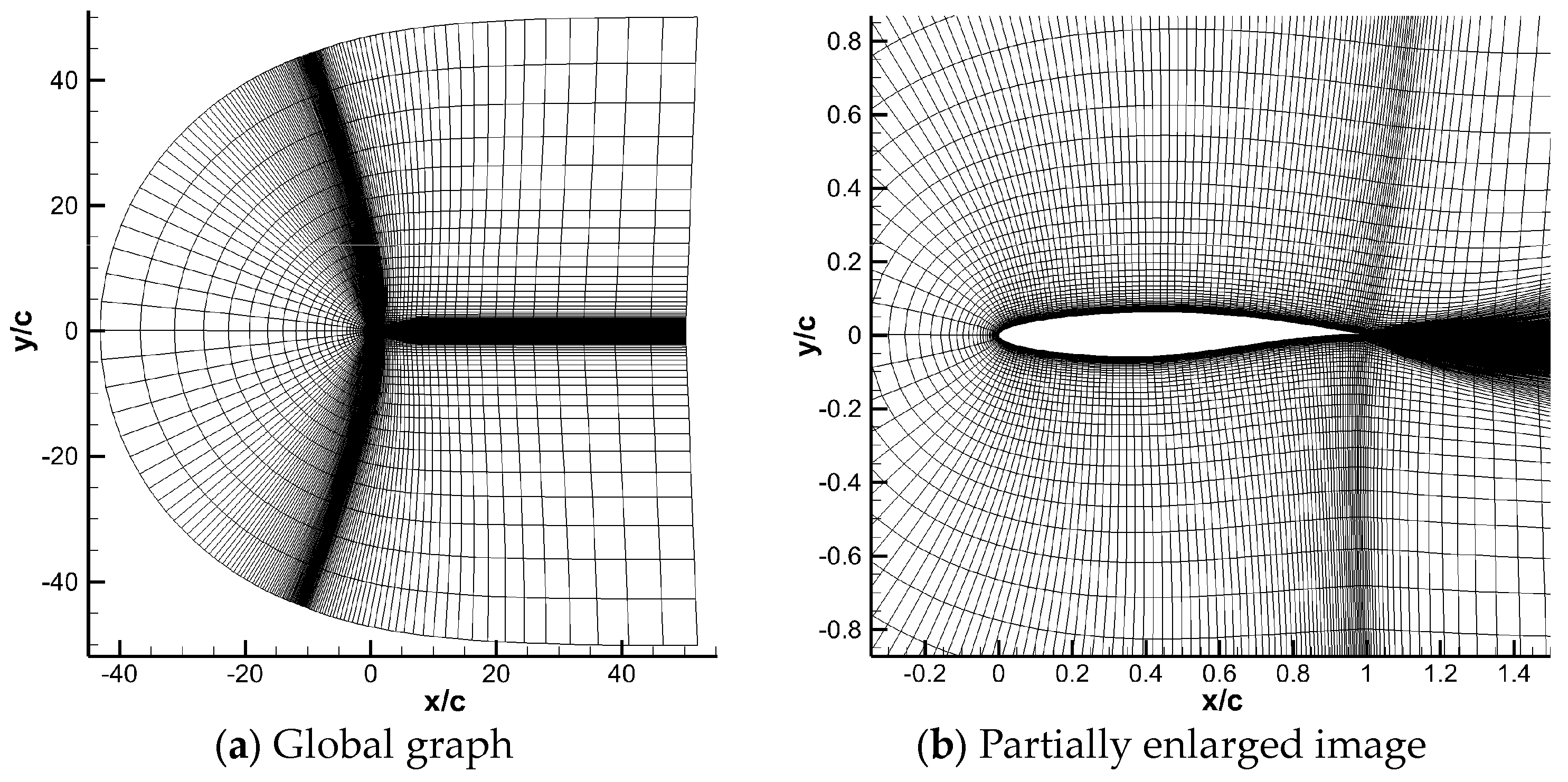
Figure 5.
RAE2822 computational grid.
Figure 5.
RAE2822 computational grid.
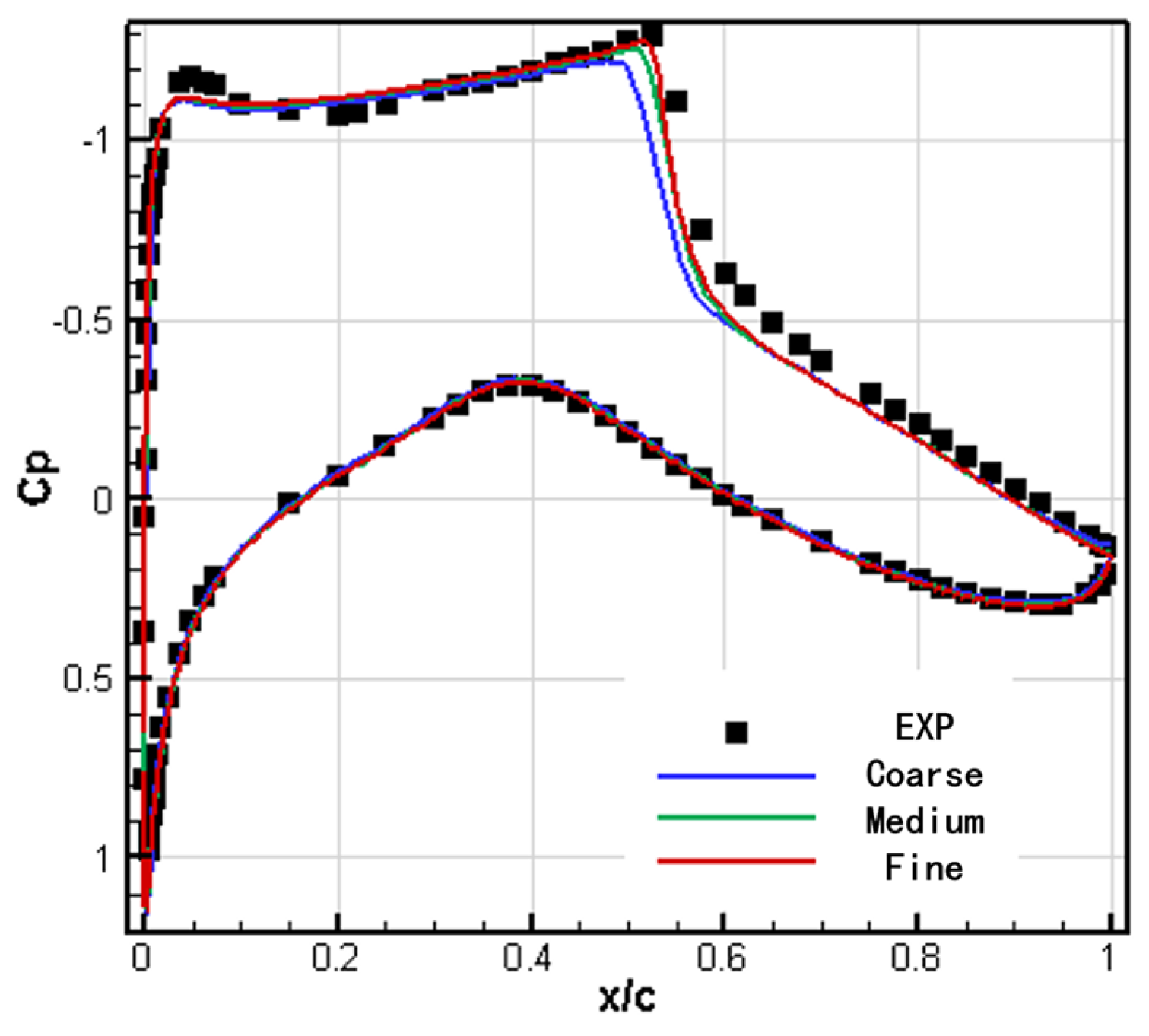
Figure 6.
Comparison of pressure distribution on airfoils.
Figure 6.
Comparison of pressure distribution on airfoils.
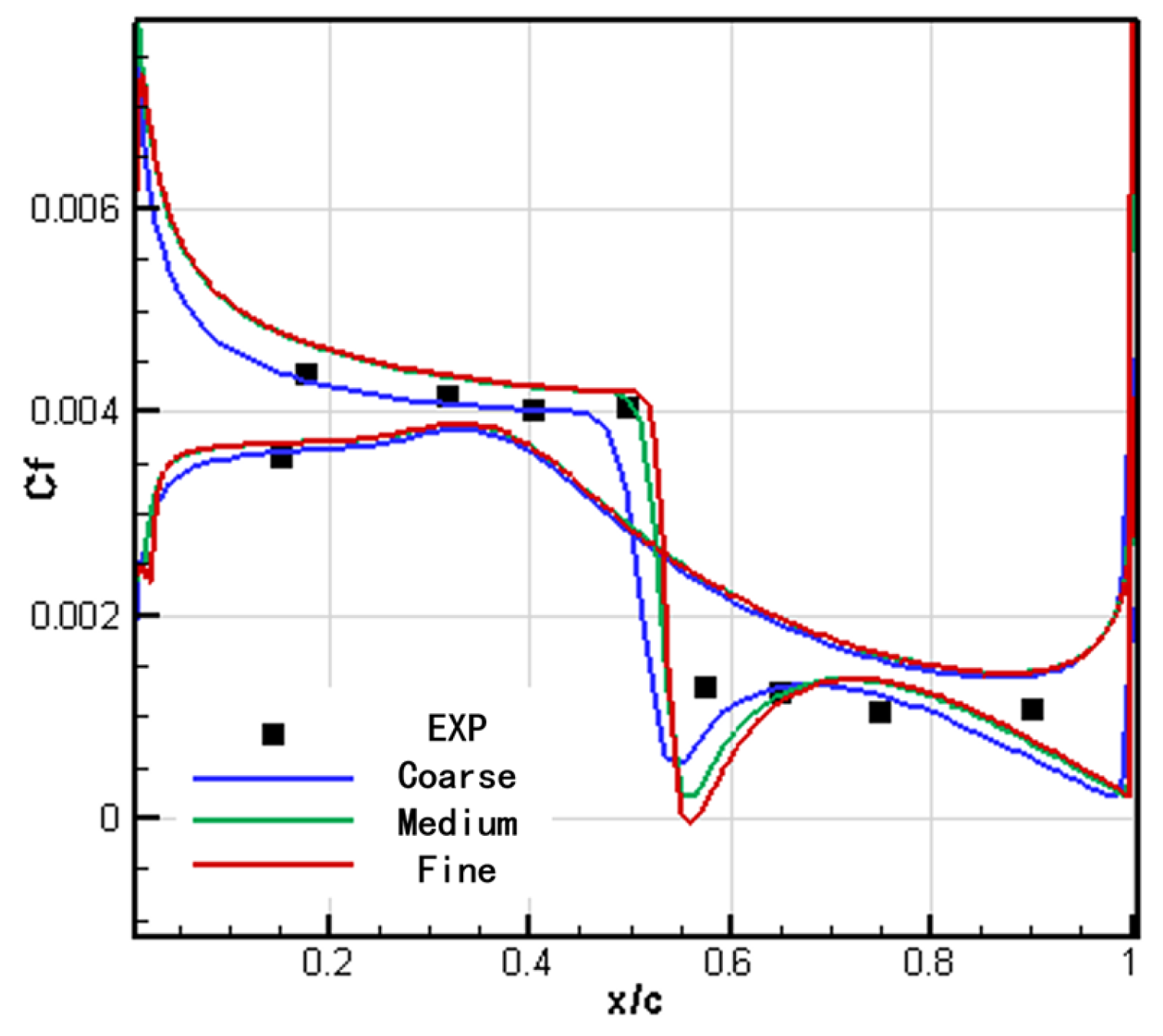
Figure 7.
Comparison of wing friction distribution.
Figure 7.
Comparison of wing friction distribution.
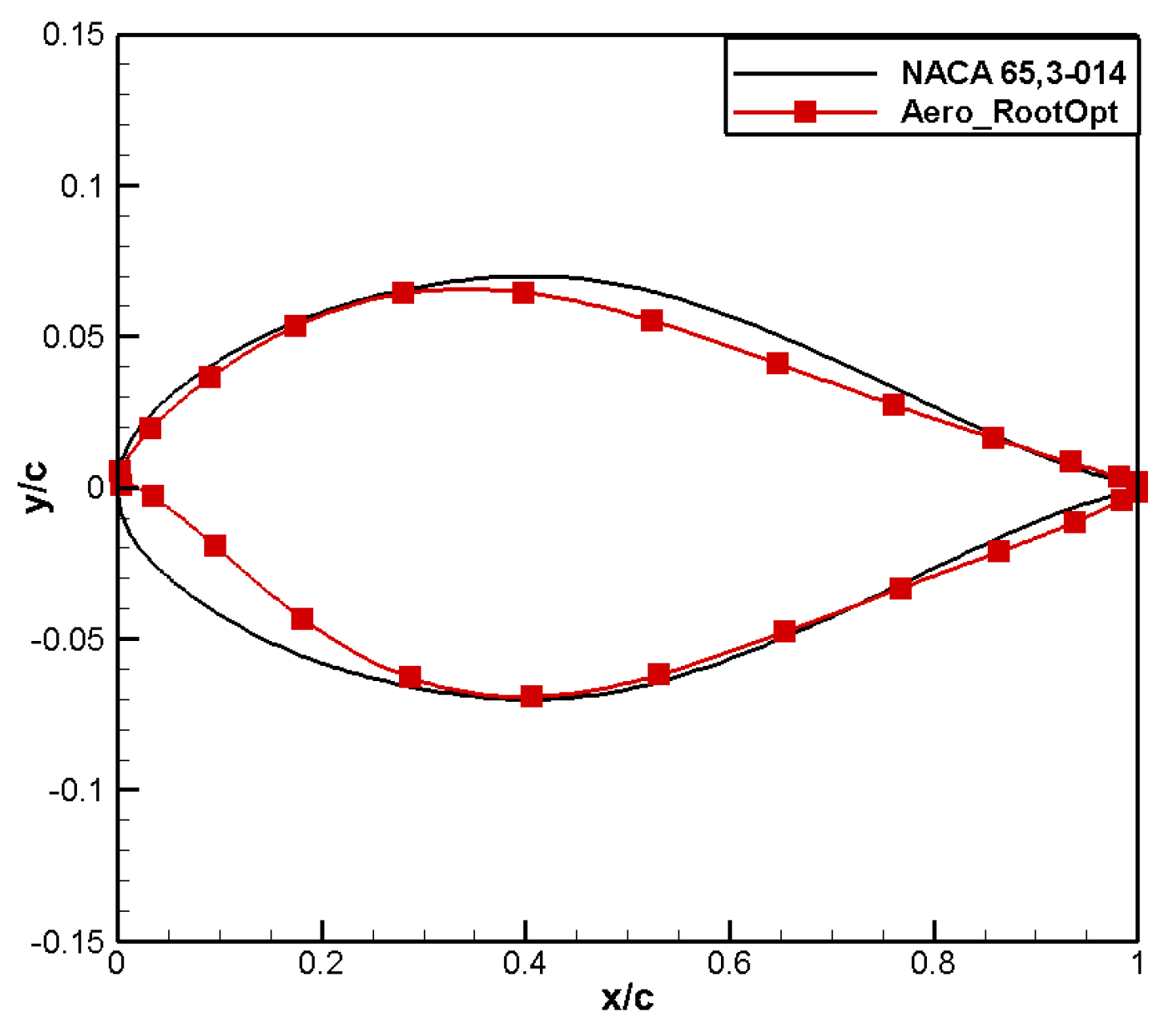
Figure 8.
Comparison of airfoil shape for aero root airfoil.
Figure 8.
Comparison of airfoil shape for aero root airfoil.
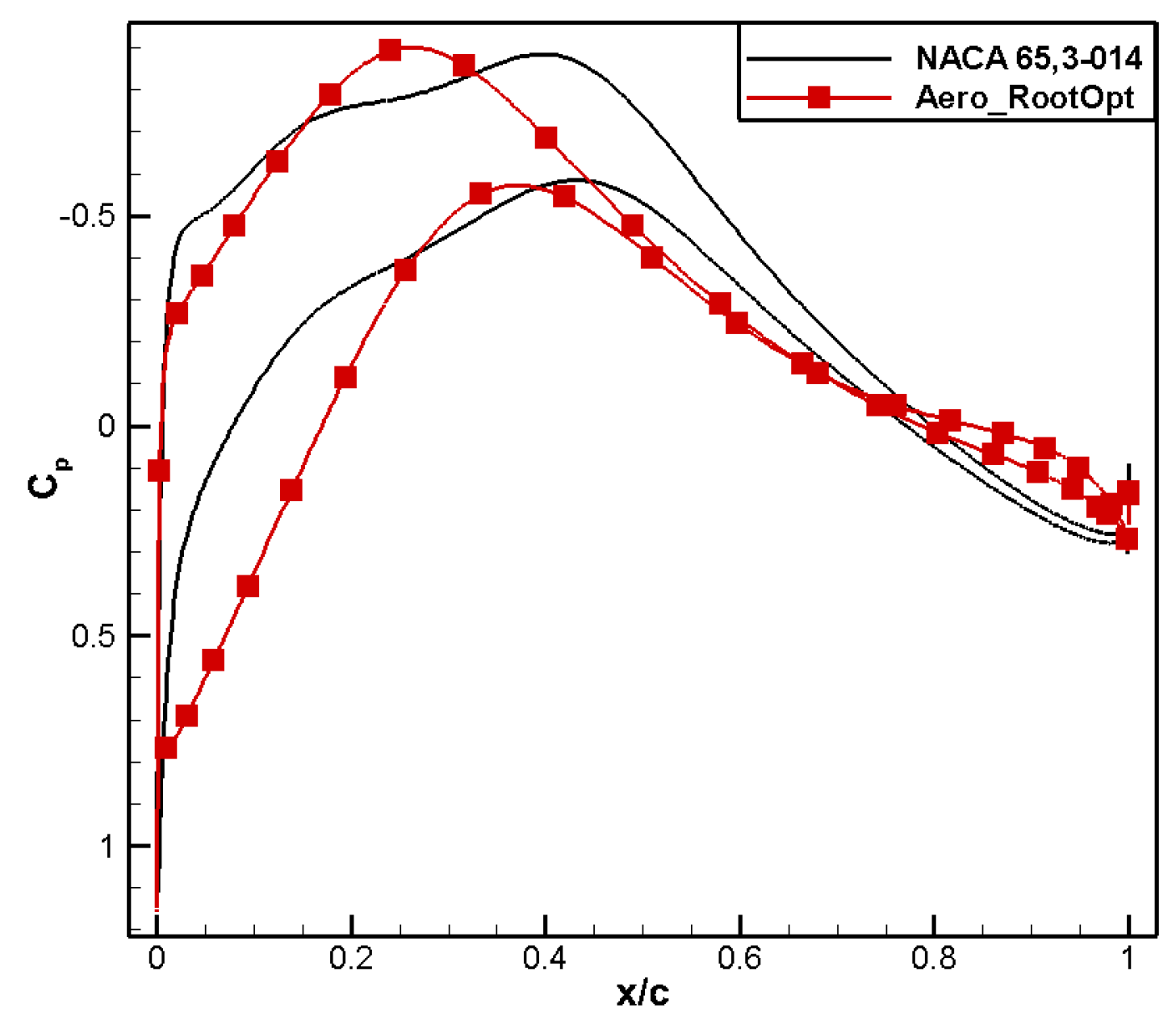
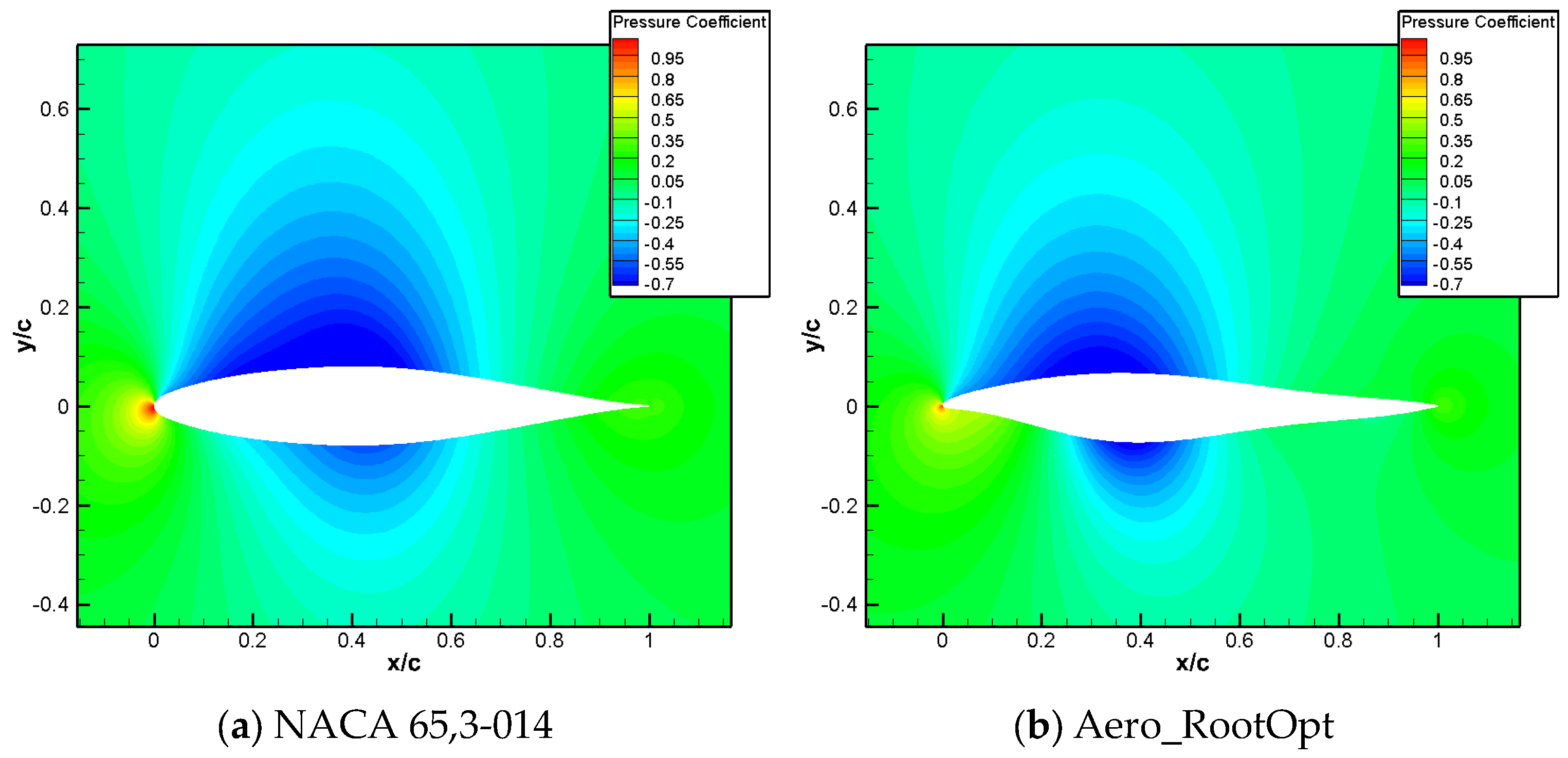
Figure 9.
Comparison of pressure coefficient distribution for aero root airfoil.
Figure 9.
Comparison of pressure coefficient distribution for aero root airfoil.
Figure 10.
Comparison of pressure distribution for aero root airfoil.
Figure 10.
Comparison of pressure distribution for aero root airfoil.
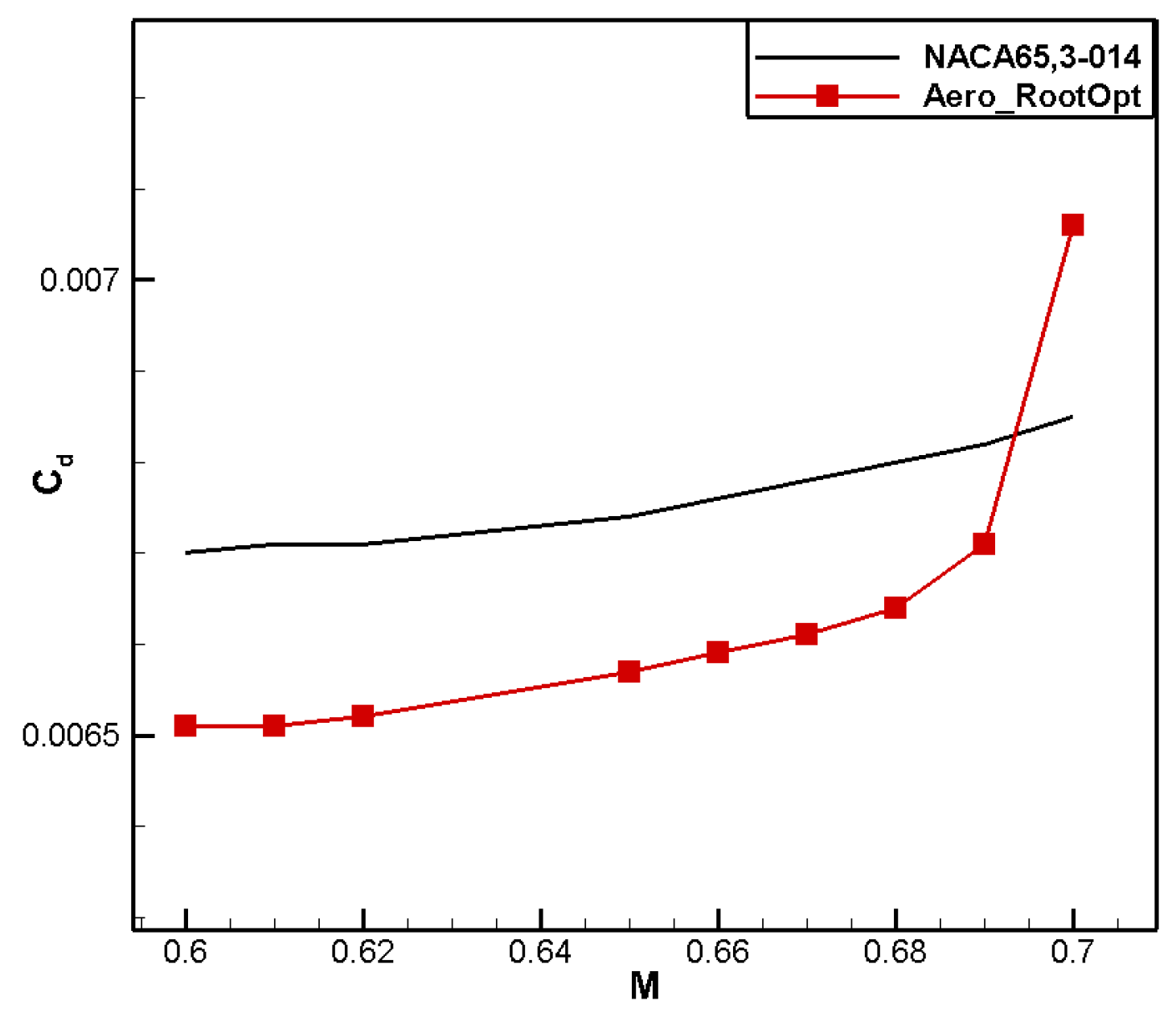
Figure 11.
Comparison of drag characteristics for aero root airfoil.
Figure 11.
Comparison of drag characteristics for aero root airfoil.
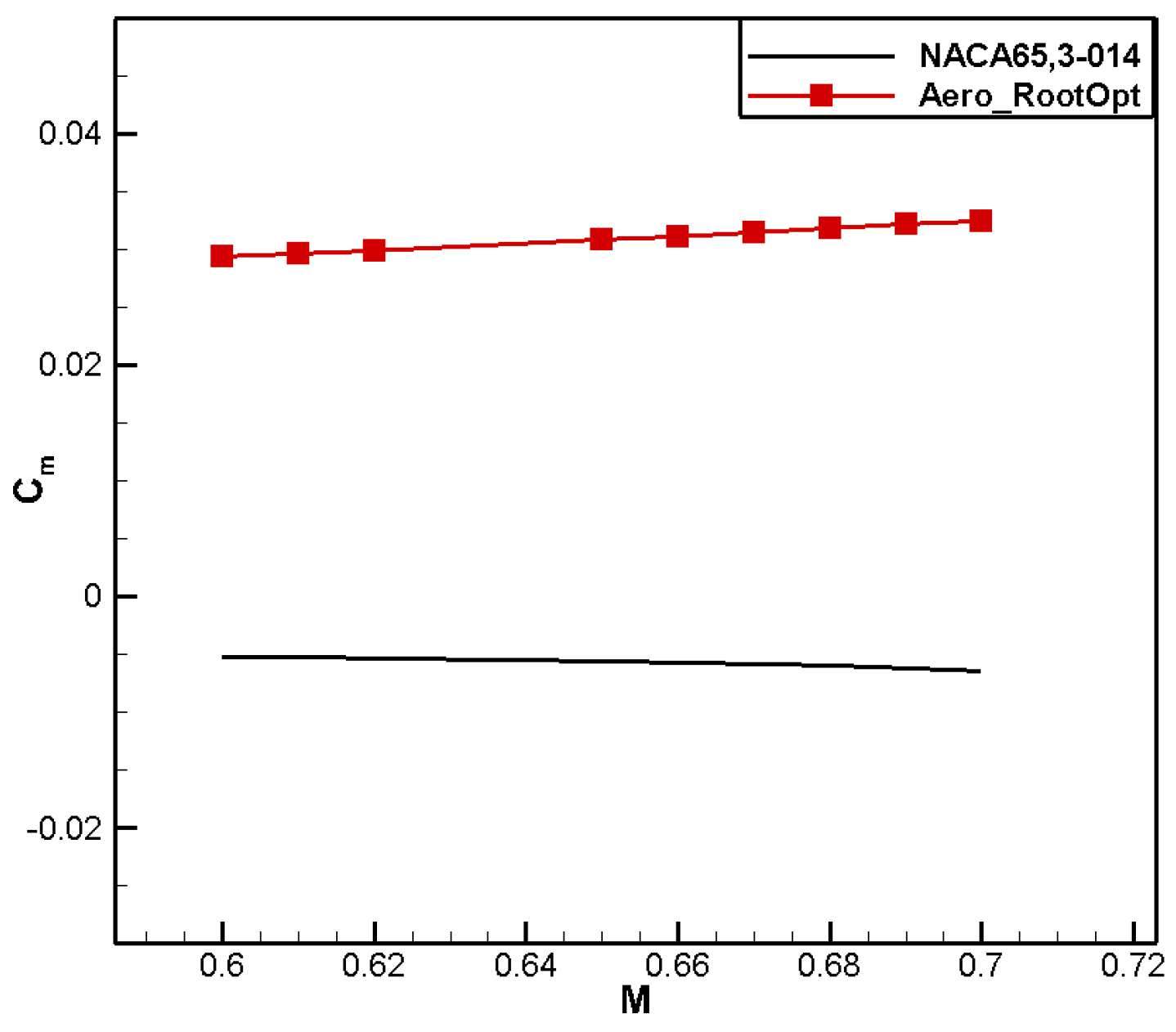
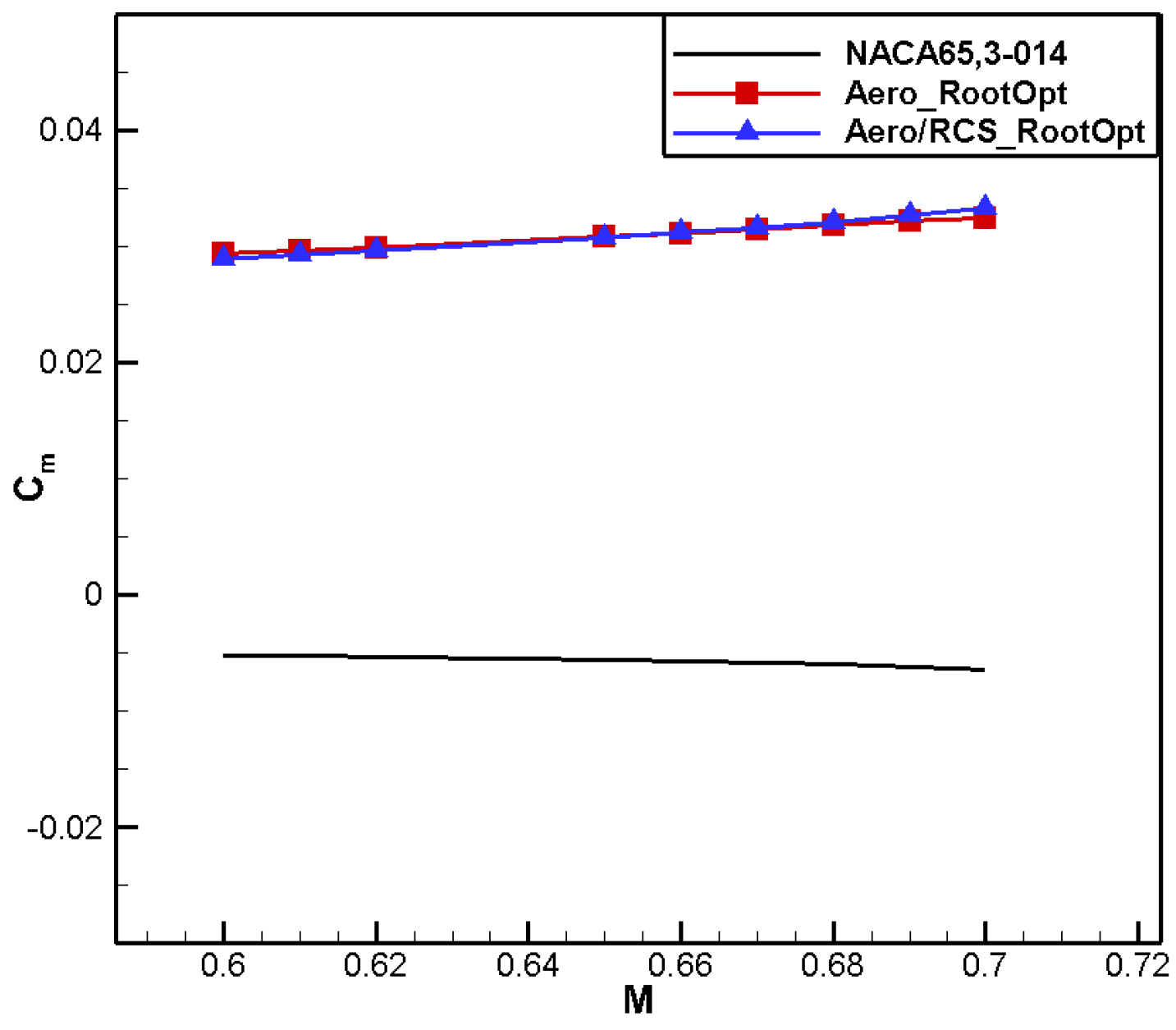
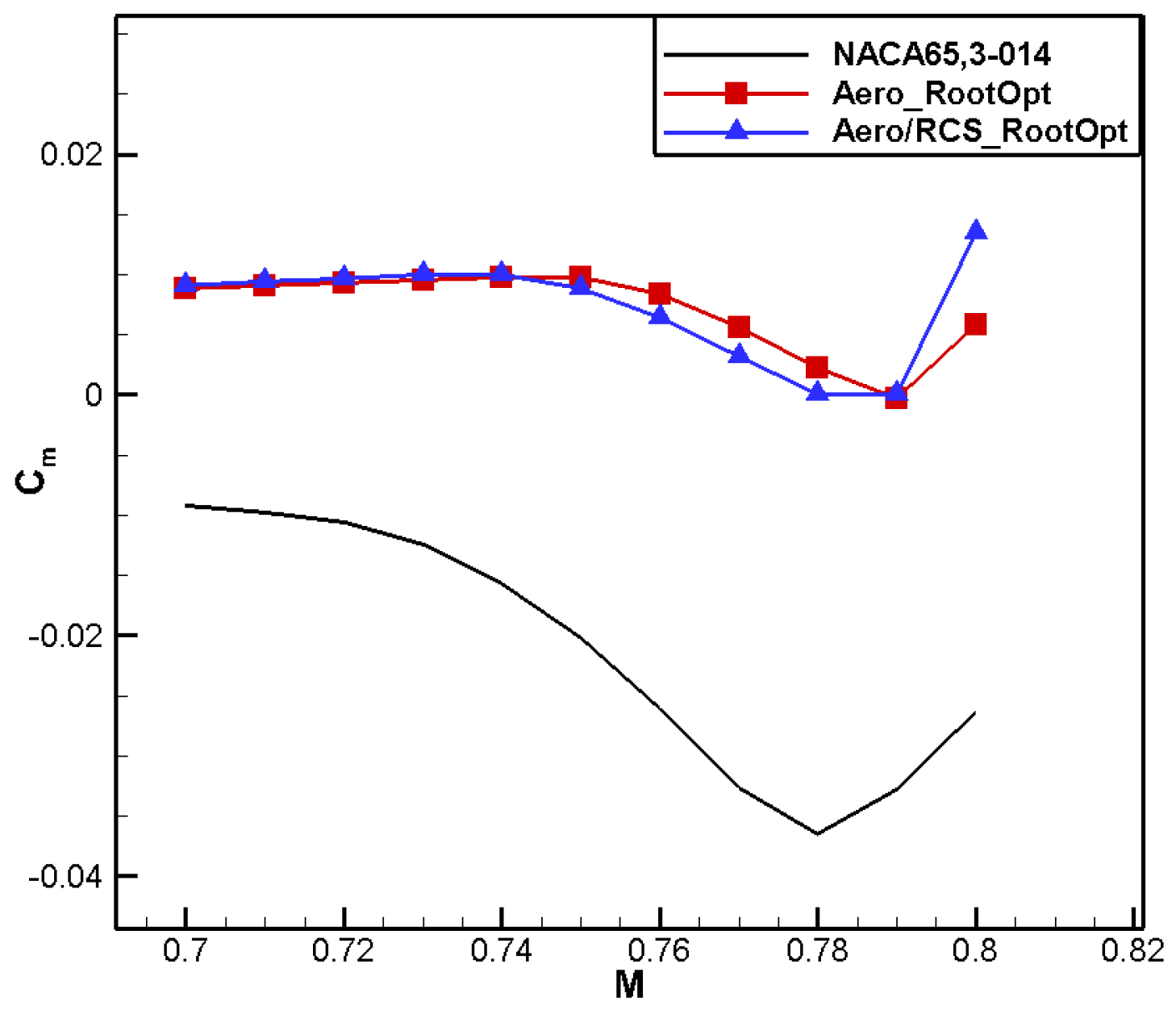
Figure 12.
Comparison of moment characteristics for aero root airfoil.
Figure 12.
Comparison of moment characteristics for aero root airfoil.
Figure 13.
Pressure distribution of BaseModel (left) and AeroOpt1 (right).
Figure 13.
Pressure distribution of BaseModel (left) and AeroOpt1 (right).
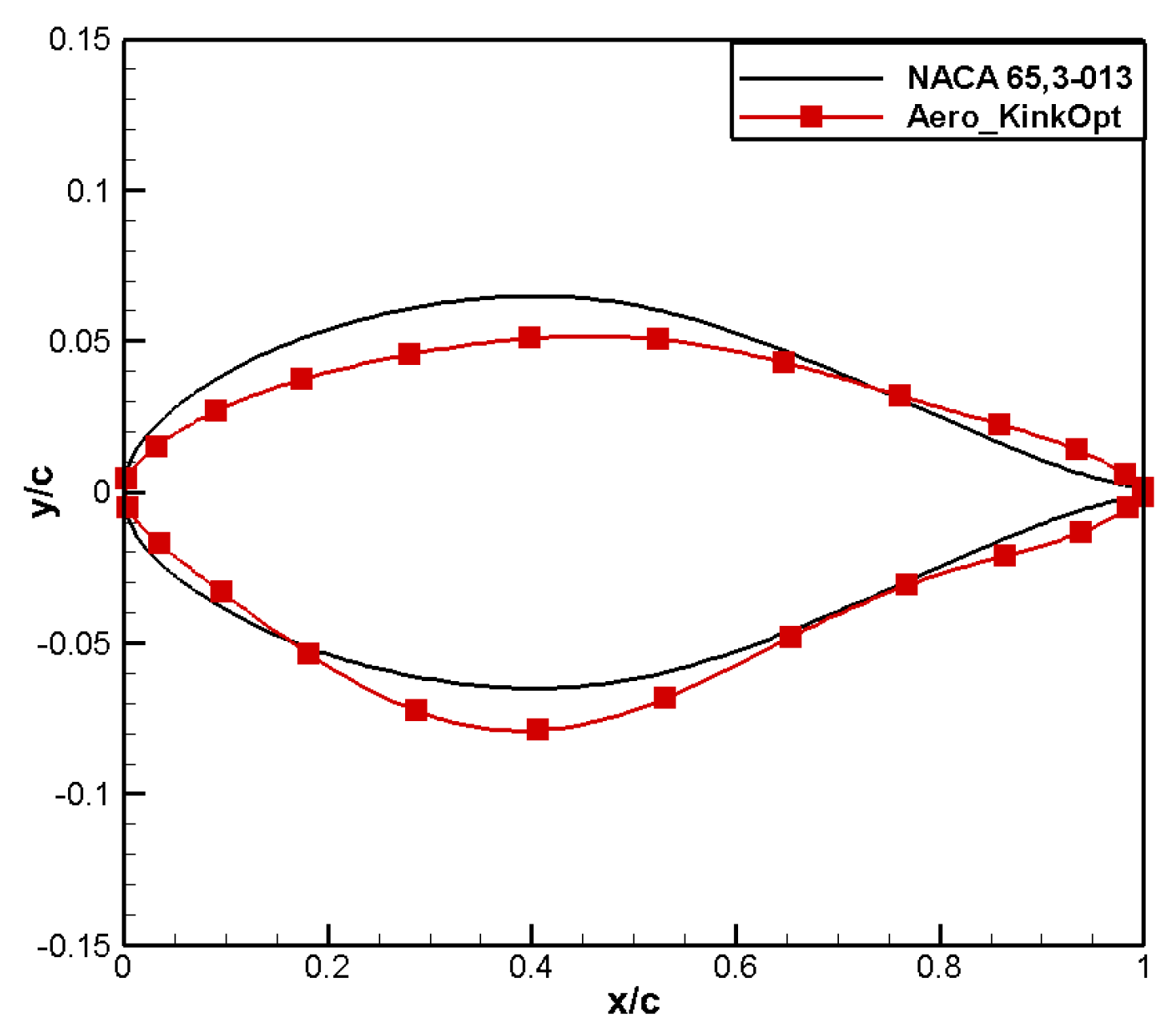
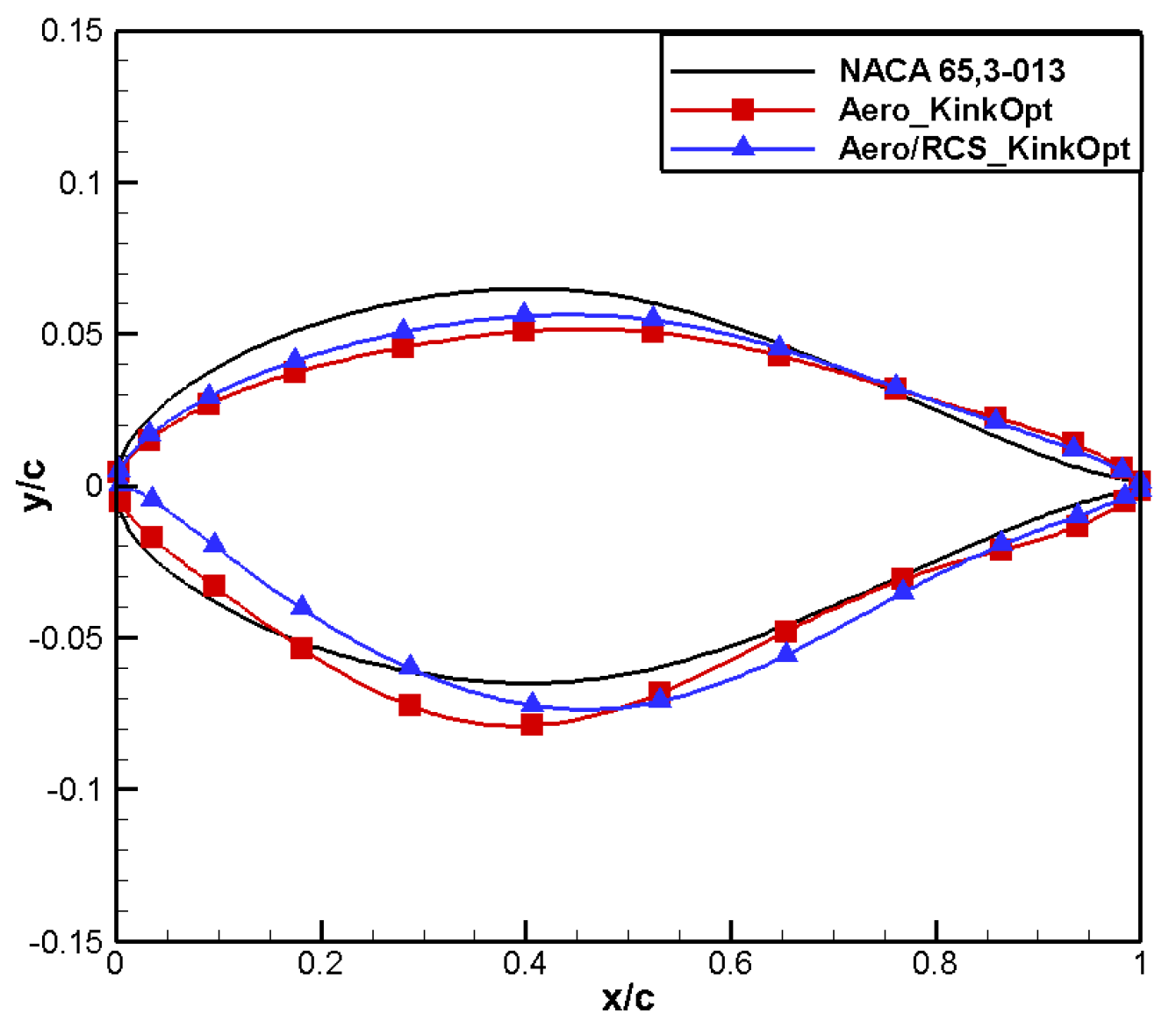
Figure 14.
Comparison of airfoil shape for aero kink airfoil.
Figure 14.
Comparison of airfoil shape for aero kink airfoil.
Figure 15.
Comparison of pressure distribution for aero kink airfoil.
Figure 15.
Comparison of pressure distribution for aero kink airfoil.
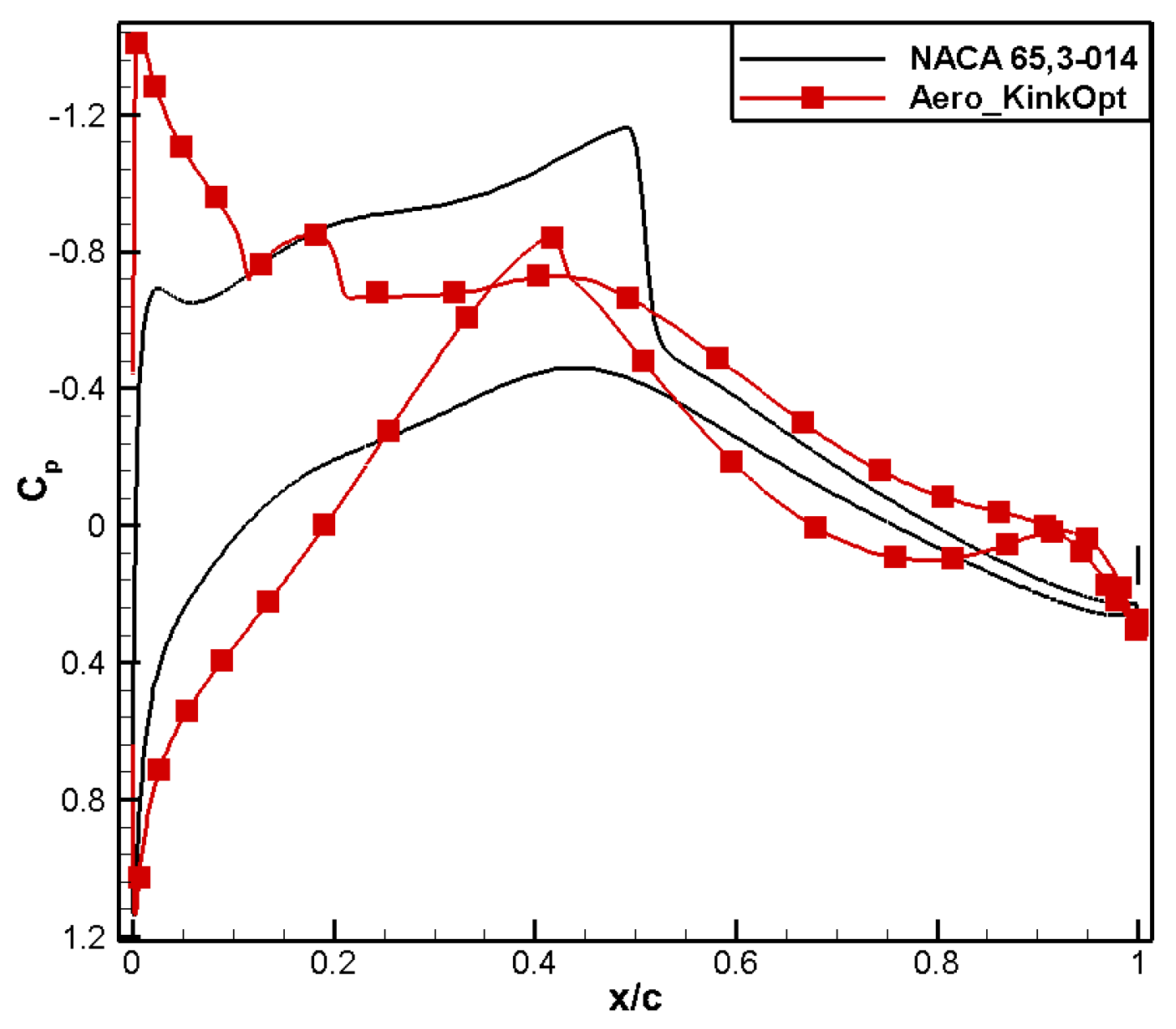
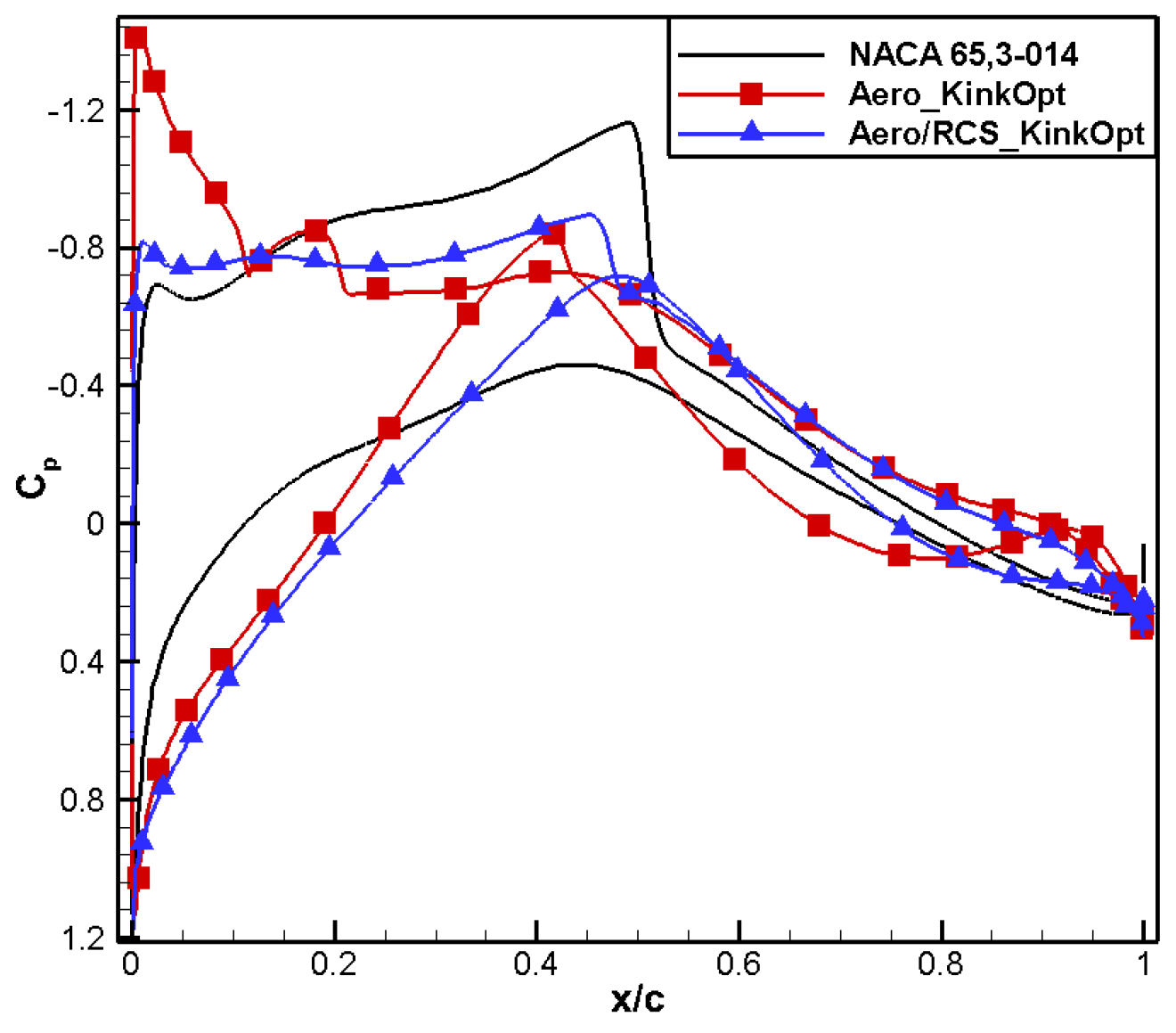
Figure 16.
Comparison of pressure distribution for aero kink airfoil.
Figure 16.
Comparison of pressure distribution for aero kink airfoil.
Figure 17.
Comparison of drag characteristics for aero kink airfoil.
Figure 17.
Comparison of drag characteristics for aero kink airfoil.
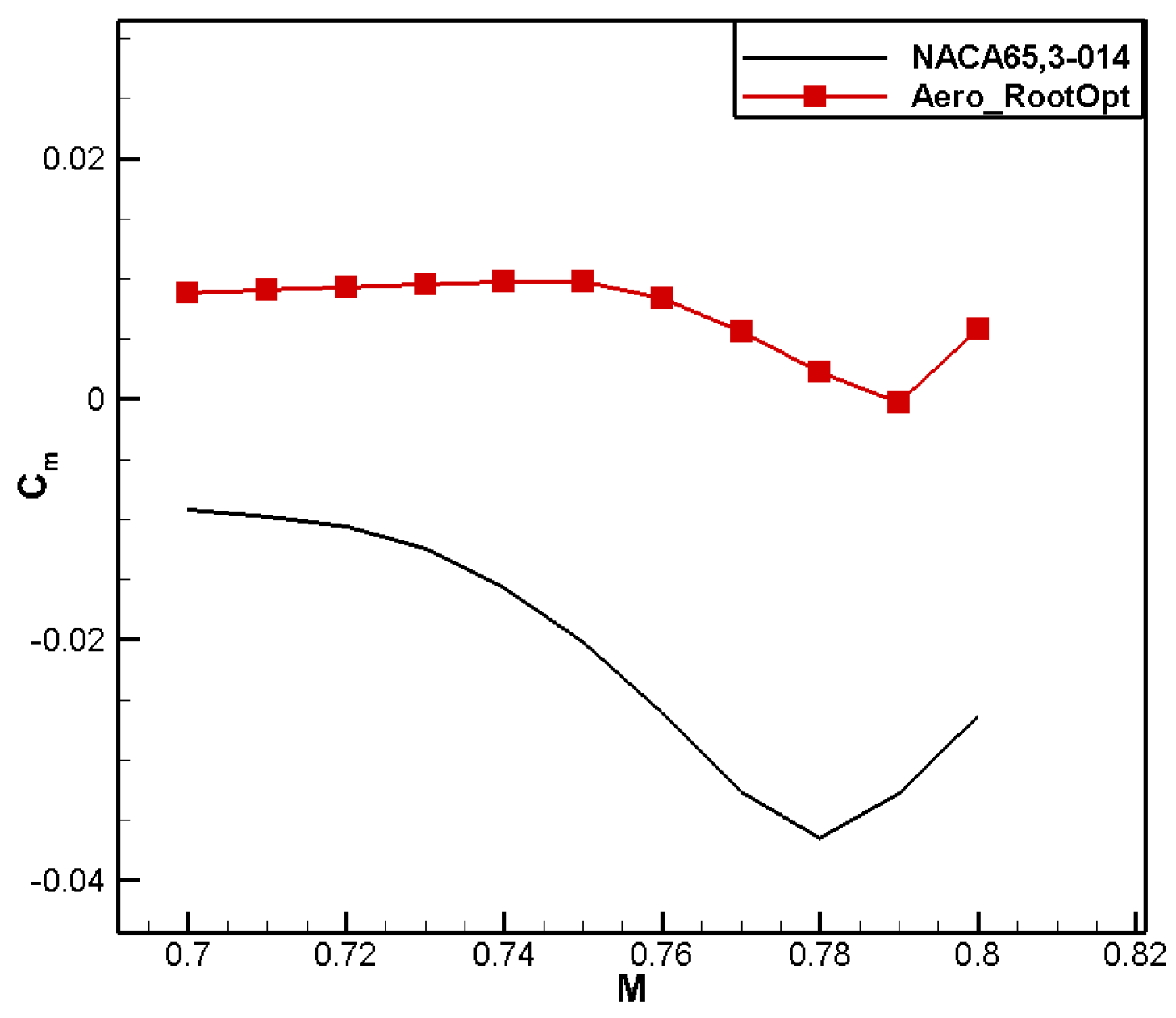
Figure 18.
Comparison of moment characteristics for aero kink airfoil.
Figure 18.
Comparison of moment characteristics for aero kink airfoil.
Figure 19.
Pressure distribution for aeroOpt1 (left) and AeroOpt2 (right).
Figure 19.
Pressure distribution for aeroOpt1 (left) and AeroOpt2 (right).
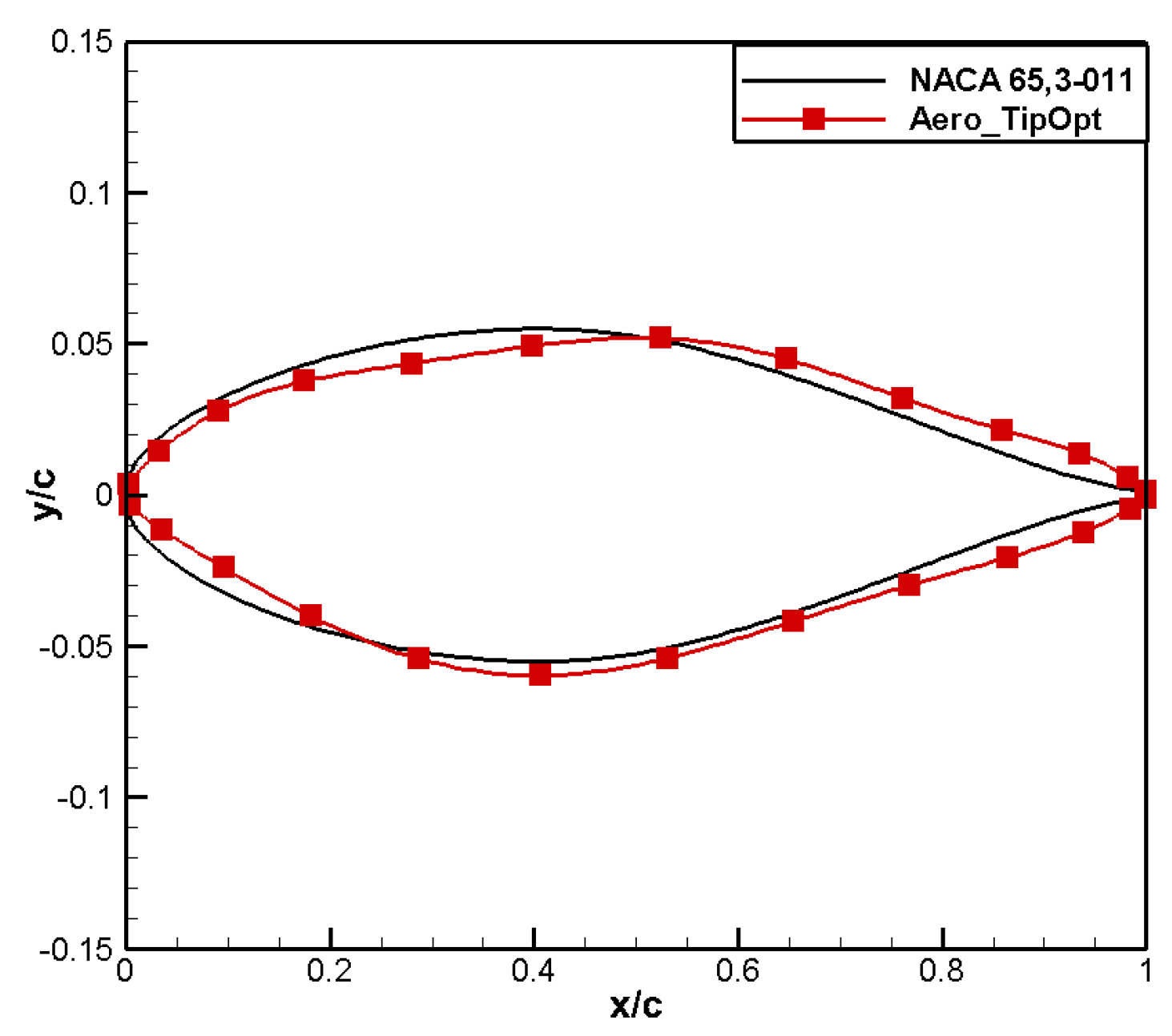
Figure 20.
Comparison of airfoil shape for aero tip airfoil.
Figure 20.
Comparison of airfoil shape for aero tip airfoil.
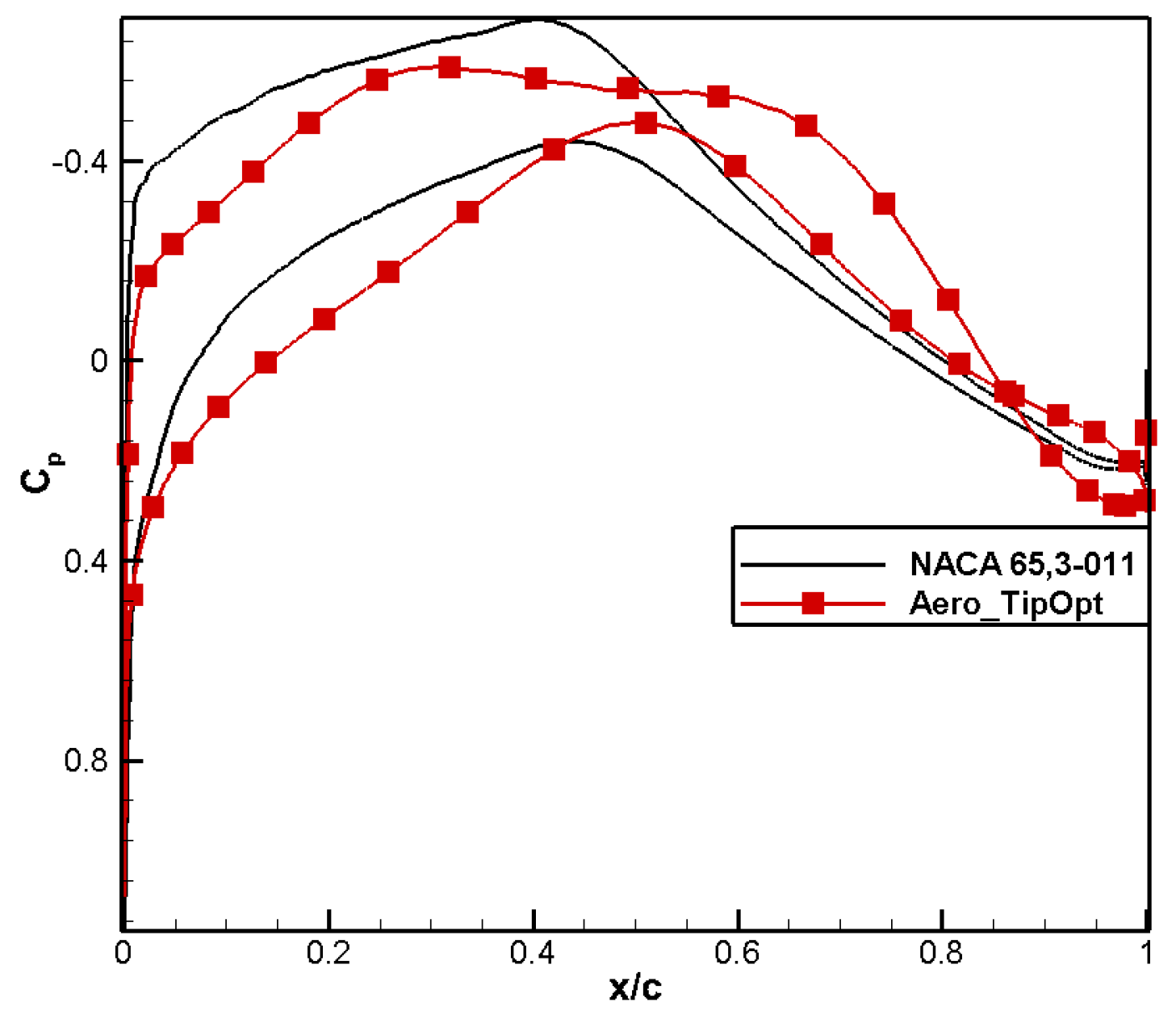
Figure 21.
Comparison of pressure distribution for aero tip airfoil.
Figure 21.
Comparison of pressure distribution for aero tip airfoil.
Figure 22.
Comparison of pressure distribution for aero tip airfoil.
Figure 22.
Comparison of pressure distribution for aero tip airfoil.
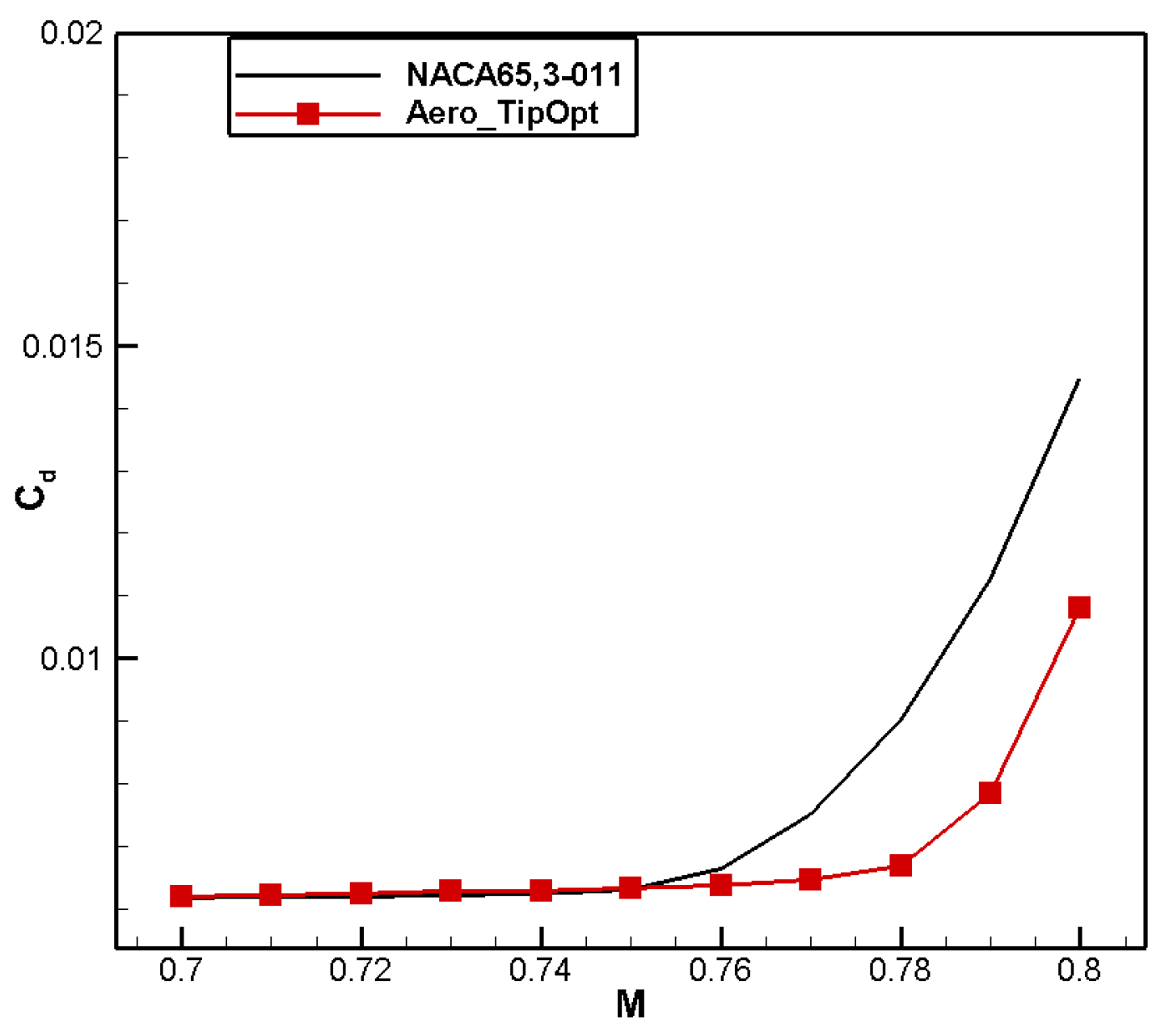
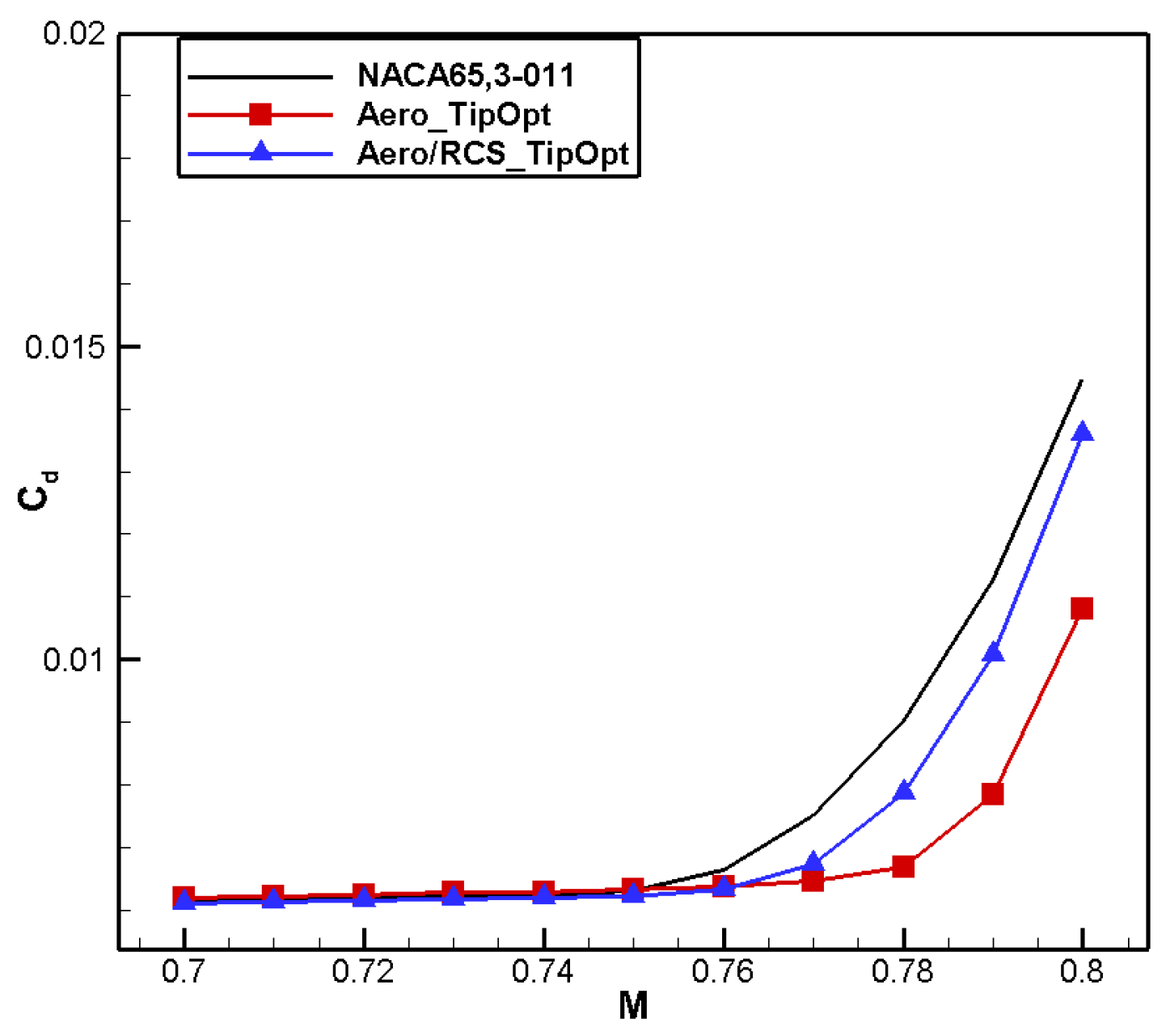
Figure 23.
Comparison of drag characteristics for aero tip airfoil.
Figure 23.
Comparison of drag characteristics for aero tip airfoil.
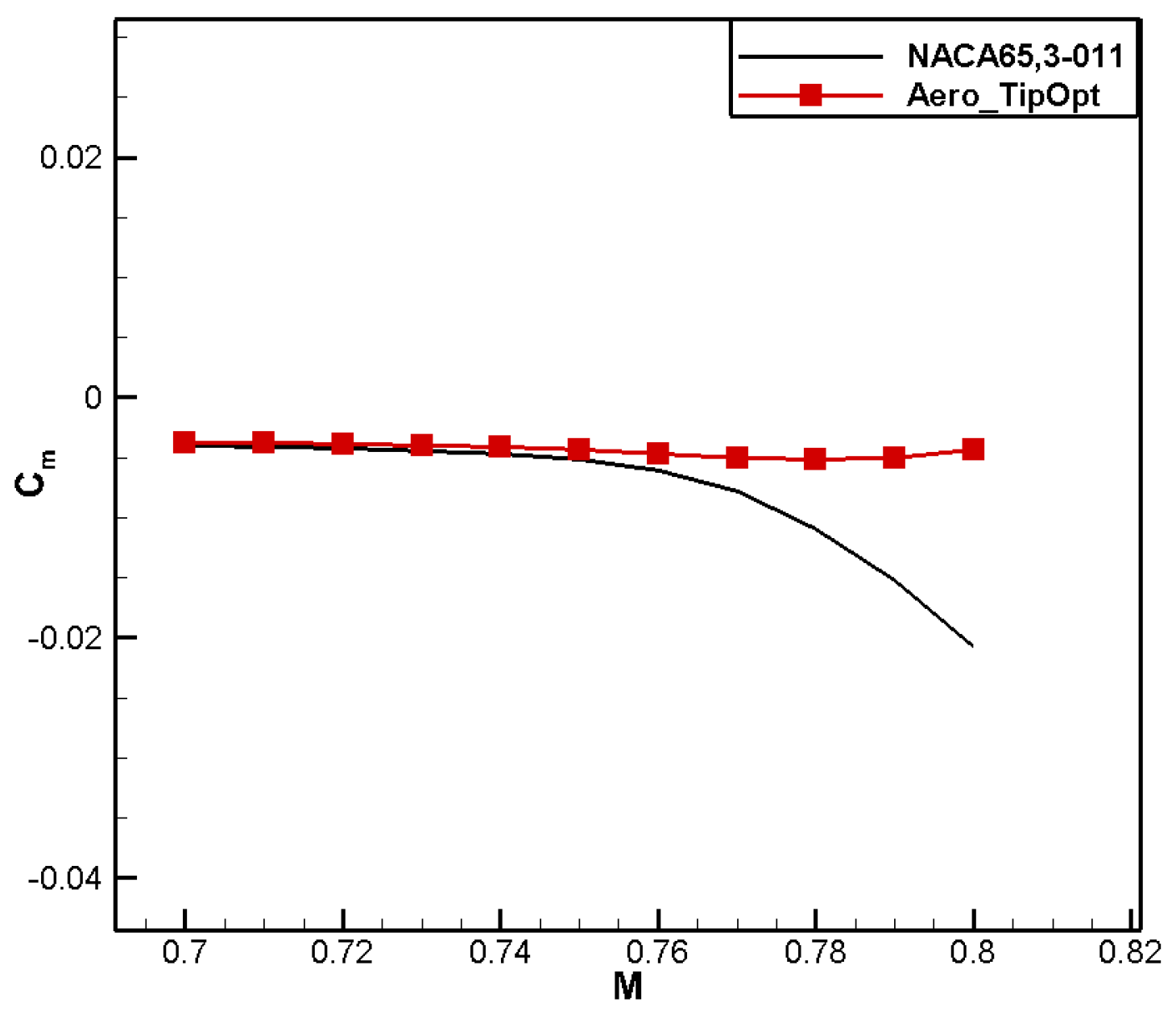
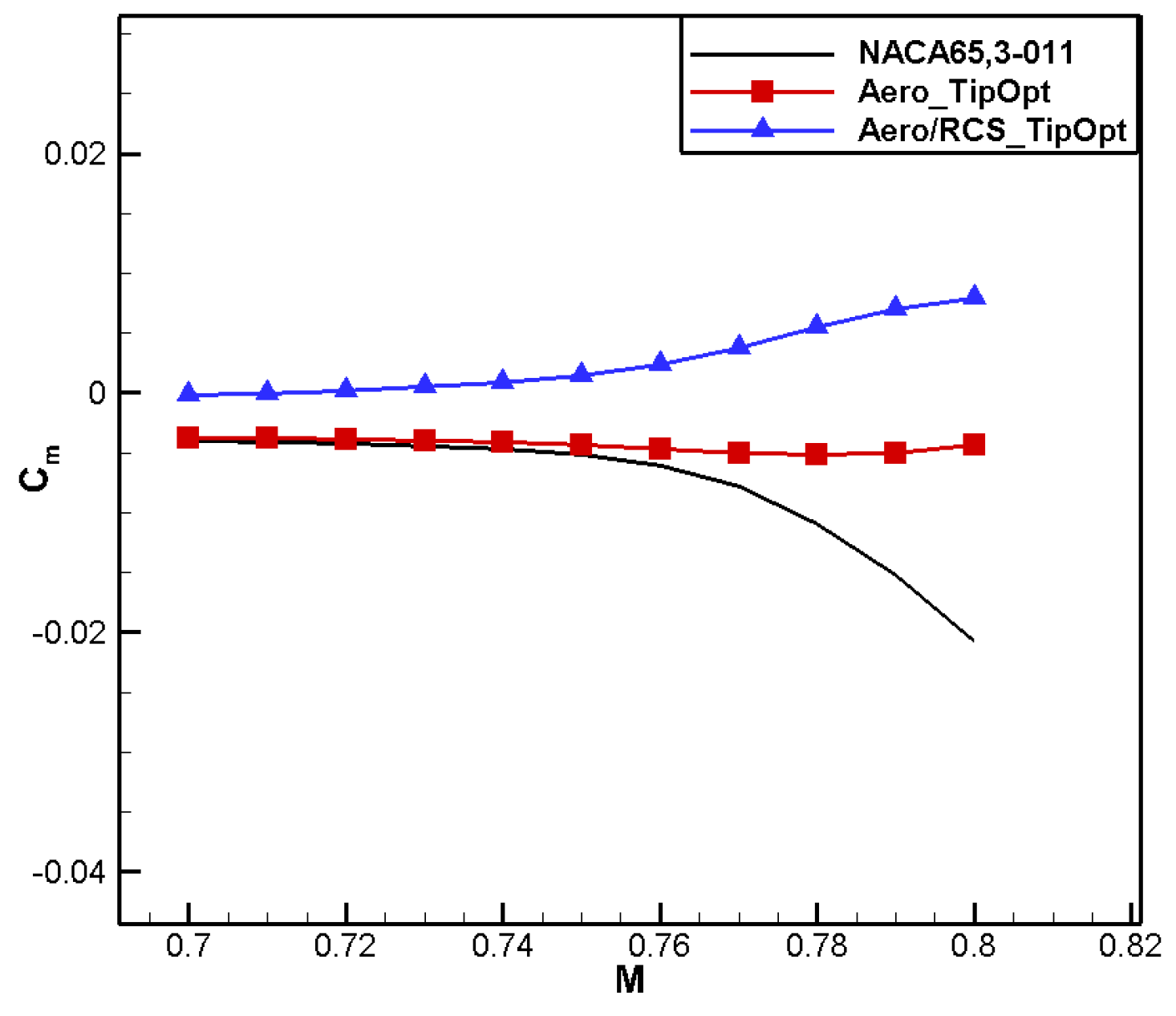
Figure 24.
Comparison of moment characteristics for aero tip airfoil.
Figure 24.
Comparison of moment characteristics for aero tip airfoil.
Figure 25.
Pressure distribution for aeroOpt2 (left) and AeroOpt3 (right).
Figure 25.
Pressure distribution for aeroOpt2 (left) and AeroOpt3 (right).
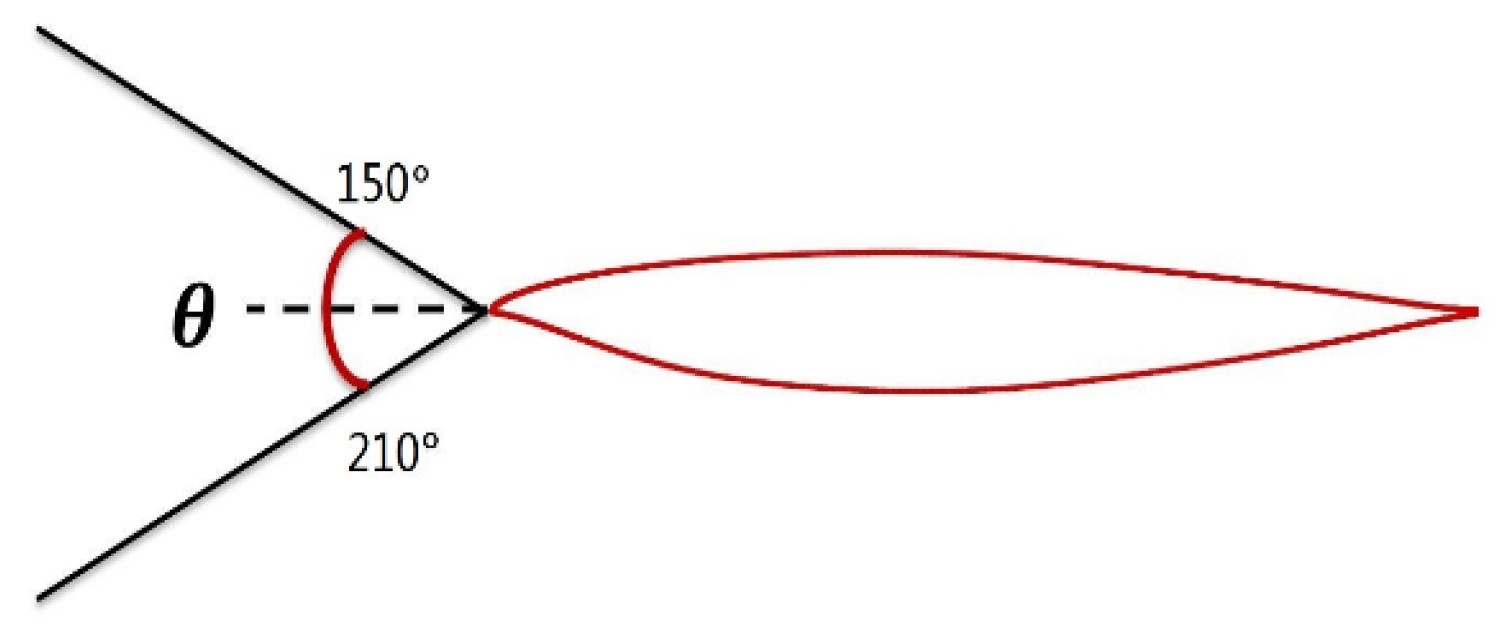
Figure 26.
Sketch of incident angle.
Figure 26.
Sketch of incident angle.
Figure 27.
Comparison of airfoil shape for aero/stealth root airfoil.
Figure 27.
Comparison of airfoil shape for aero/stealth root airfoil.
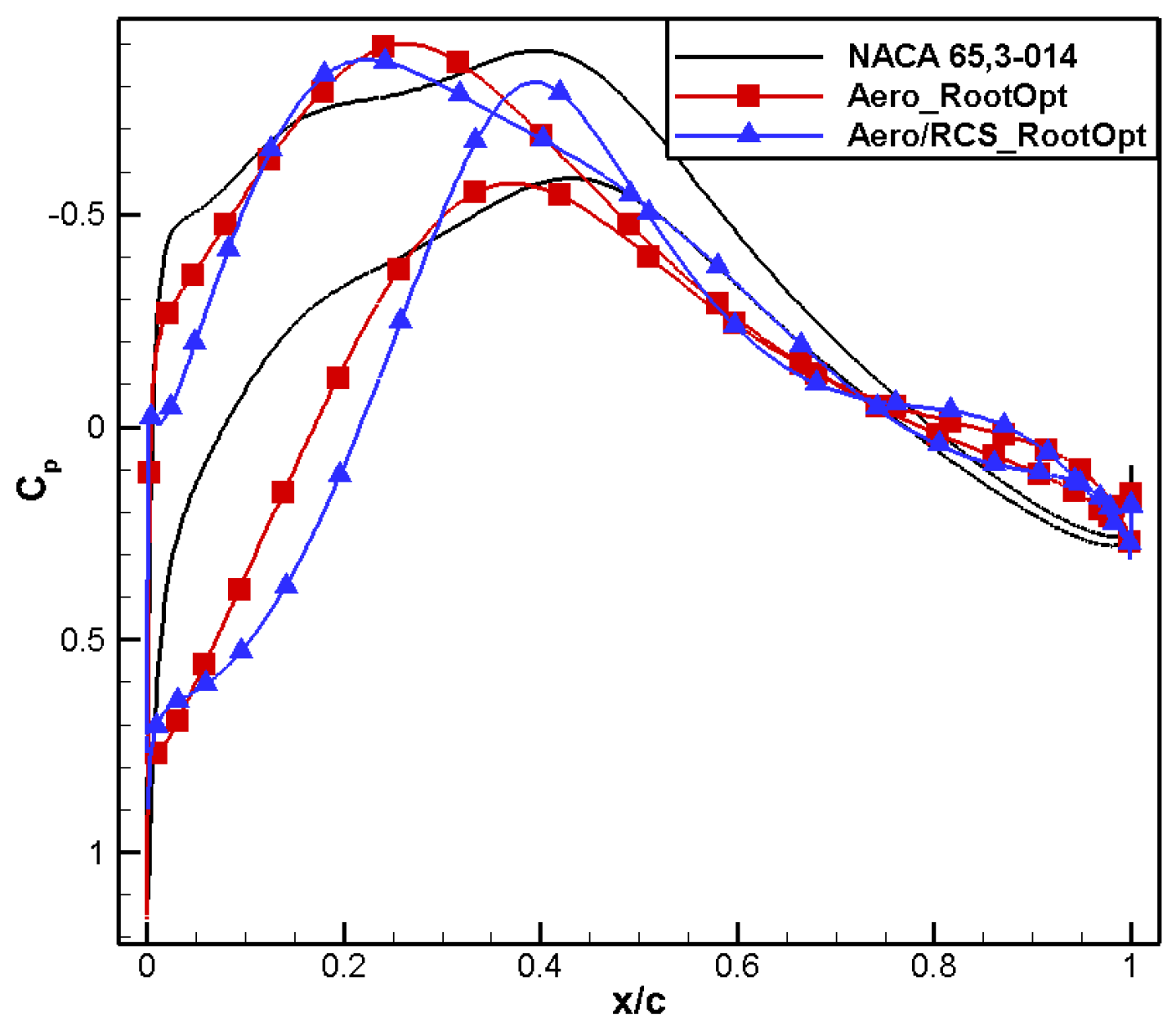
Figure 28.
Comparison of pressure coefficient distribution for aero/stealth root airfoil.
Figure 28.
Comparison of pressure coefficient distribution for aero/stealth root airfoil.
Figure 29.
Pressure distribution of optimized airfoil for aero/stealth root airfoil.
Figure 29.
Pressure distribution of optimized airfoil for aero/stealth root airfoil.
Figure 30.
Comparison of drag characteristics for aero/stealth root airfoil.
Figure 30.
Comparison of drag characteristics for aero/stealth root airfoil.
Figure 31.
Comparison of moment characteristics for aero/stealth root airfoil.
Figure 31.
Comparison of moment characteristics for aero/stealth root airfoil.
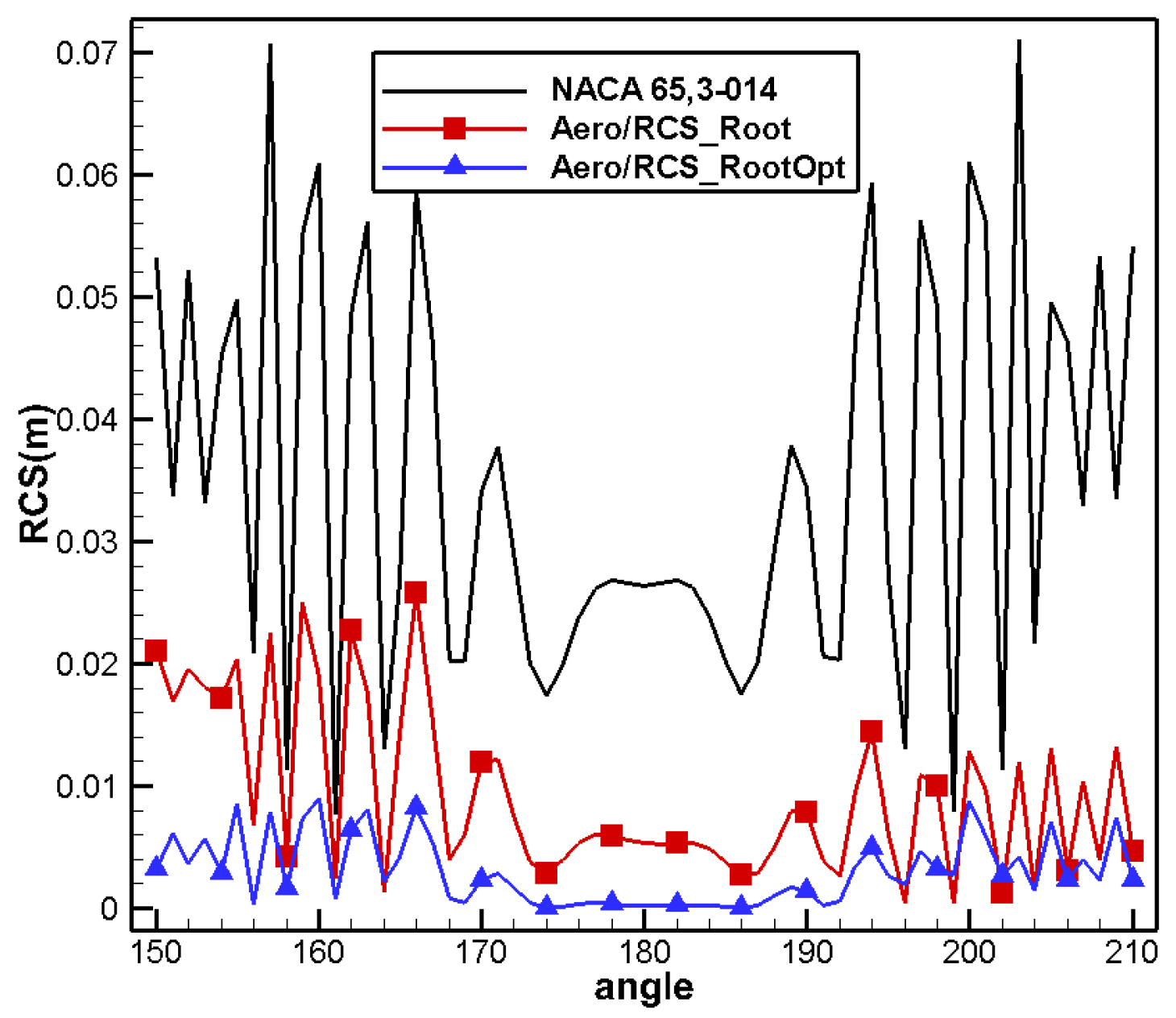
Figure 32.
Comparison of frontal RCS for aero/stealth root airfoil.
Figure 32.
Comparison of frontal RCS for aero/stealth root airfoil.
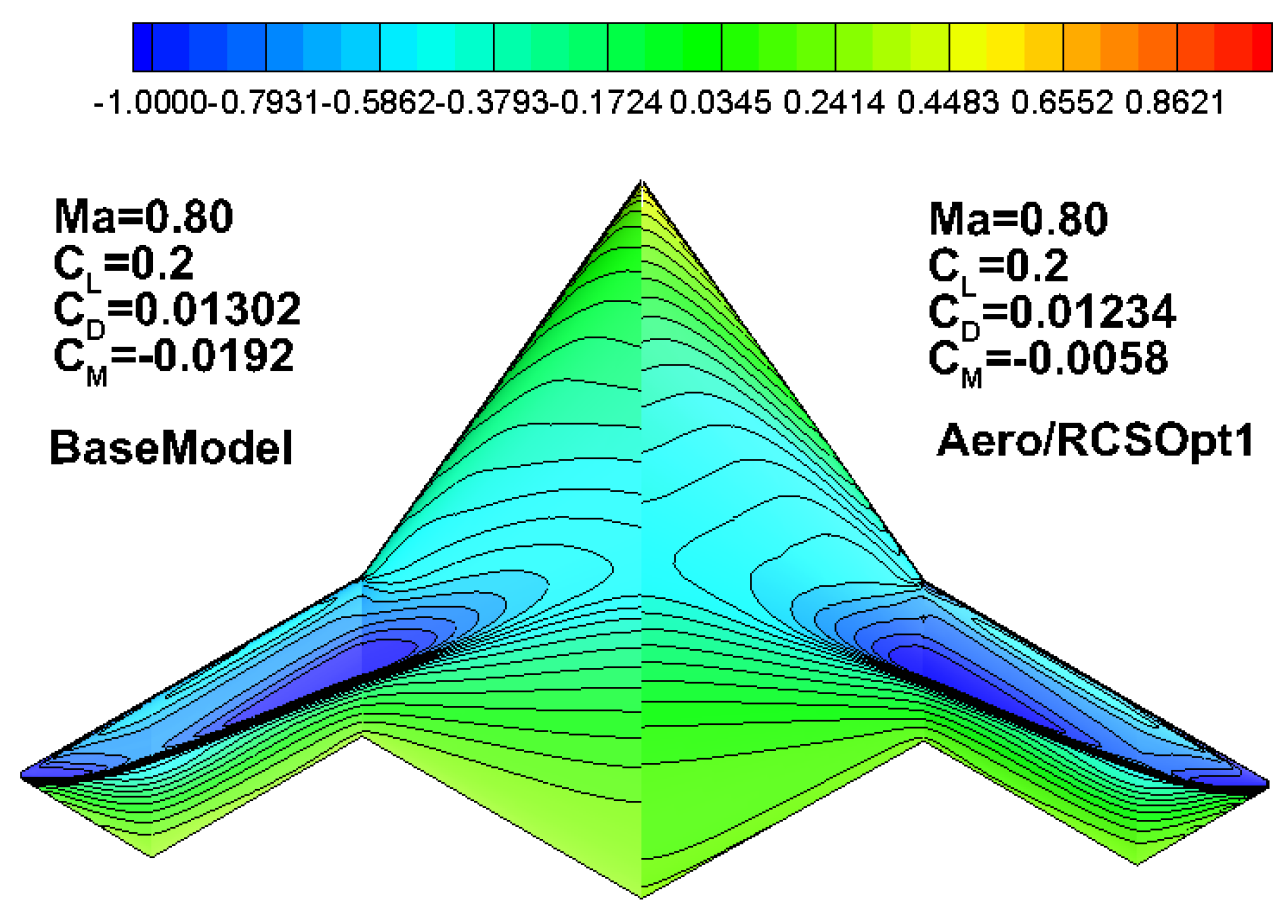
Figure 33.
Pressure distribution of BaseModel (left) and Aero/RCSOpt1 (right).
Figure 33.
Pressure distribution of BaseModel (left) and Aero/RCSOpt1 (right).
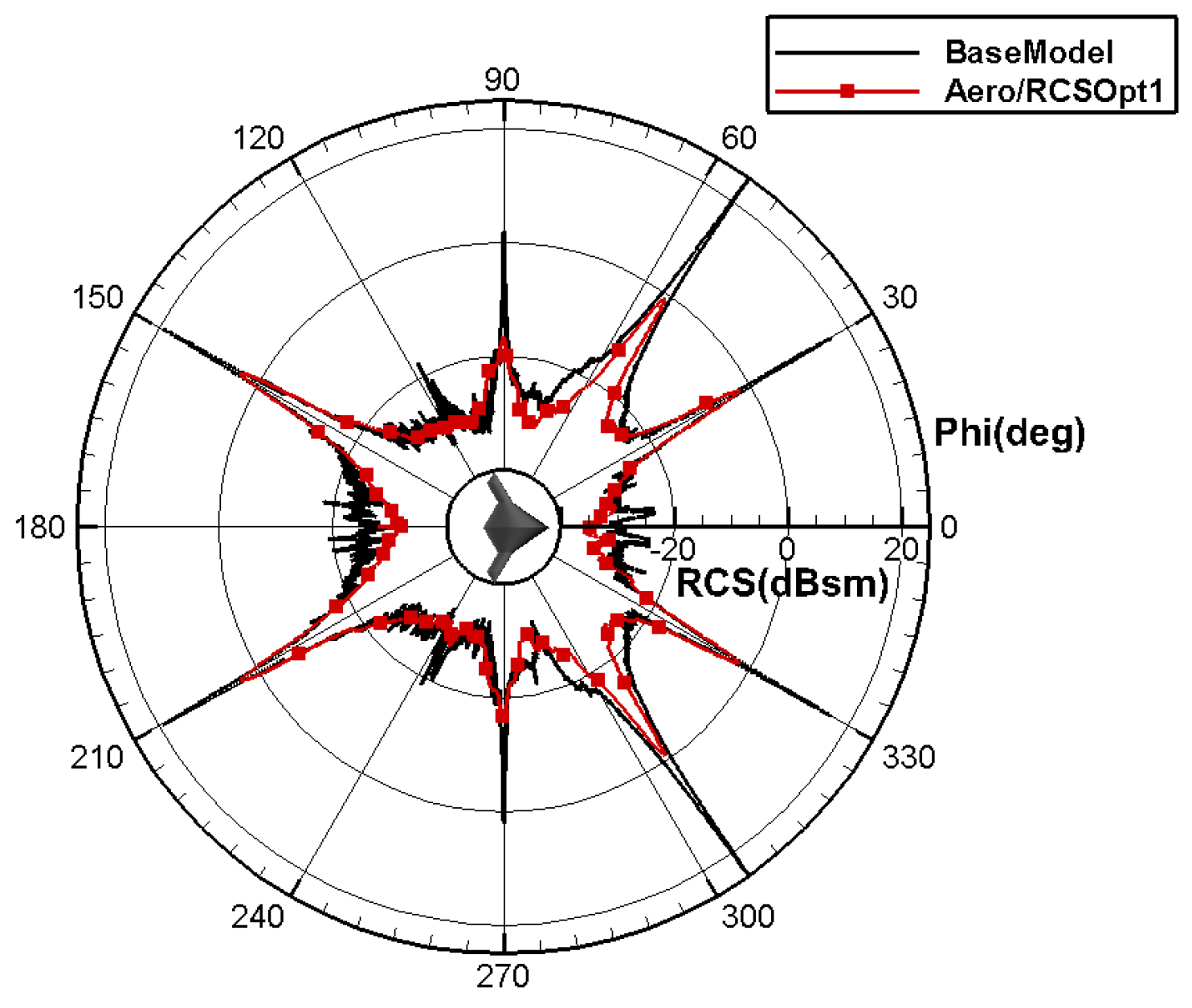
Figure 34.
Comparison of RCS in the pitch direction for layout with aero/stealth root airfoil.
Figure 34.
Comparison of RCS in the pitch direction for layout with aero/stealth root airfoil.
Figure 35.
Comparison of RCS in the yaw direction for layout with aero/stealth root airfoil.
Figure 35.
Comparison of RCS in the yaw direction for layout with aero/stealth root airfoil.
Figure 36.
Comparison of airfoil shape for aero/stealth kink airfoil.
Figure 36.
Comparison of airfoil shape for aero/stealth kink airfoil.
Figure 37.
Comparison of pressure distribution for aero/stealth kink airfoil.
Figure 37.
Comparison of pressure distribution for aero/stealth kink airfoil.
Figure 38.
Pressure distribution for aero/stealth kink airfoil.
Figure 38.
Pressure distribution for aero/stealth kink airfoil.
Figure 39.
Comparison of drag characteristics for aero/stealth kink airfoil.
Figure 39.
Comparison of drag characteristics for aero/stealth kink airfoil.
Figure 40.
Comparison of moment characteristics for aero/stealth kink airfoil.
Figure 40.
Comparison of moment characteristics for aero/stealth kink airfoil.
Figure 41.
Frontal RCS for aero/stealth kink airfoil.
Figure 41.
Frontal RCS for aero/stealth kink airfoil.
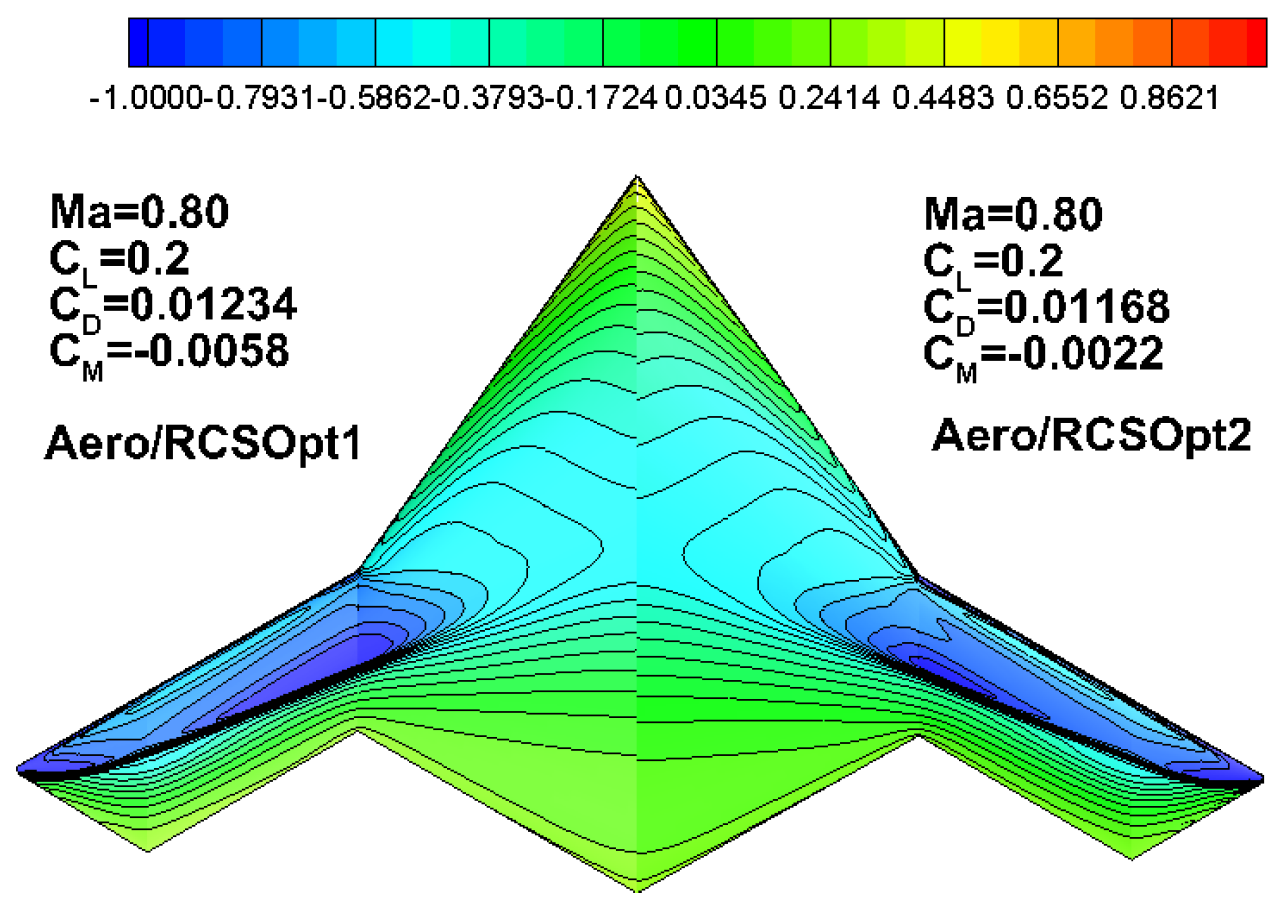
Figure 42.
Pressure distribution for aero/RCSOpt1 (left) and Aero/RCSOpt2 (right).
Figure 42.
Pressure distribution for aero/RCSOpt1 (left) and Aero/RCSOpt2 (right).
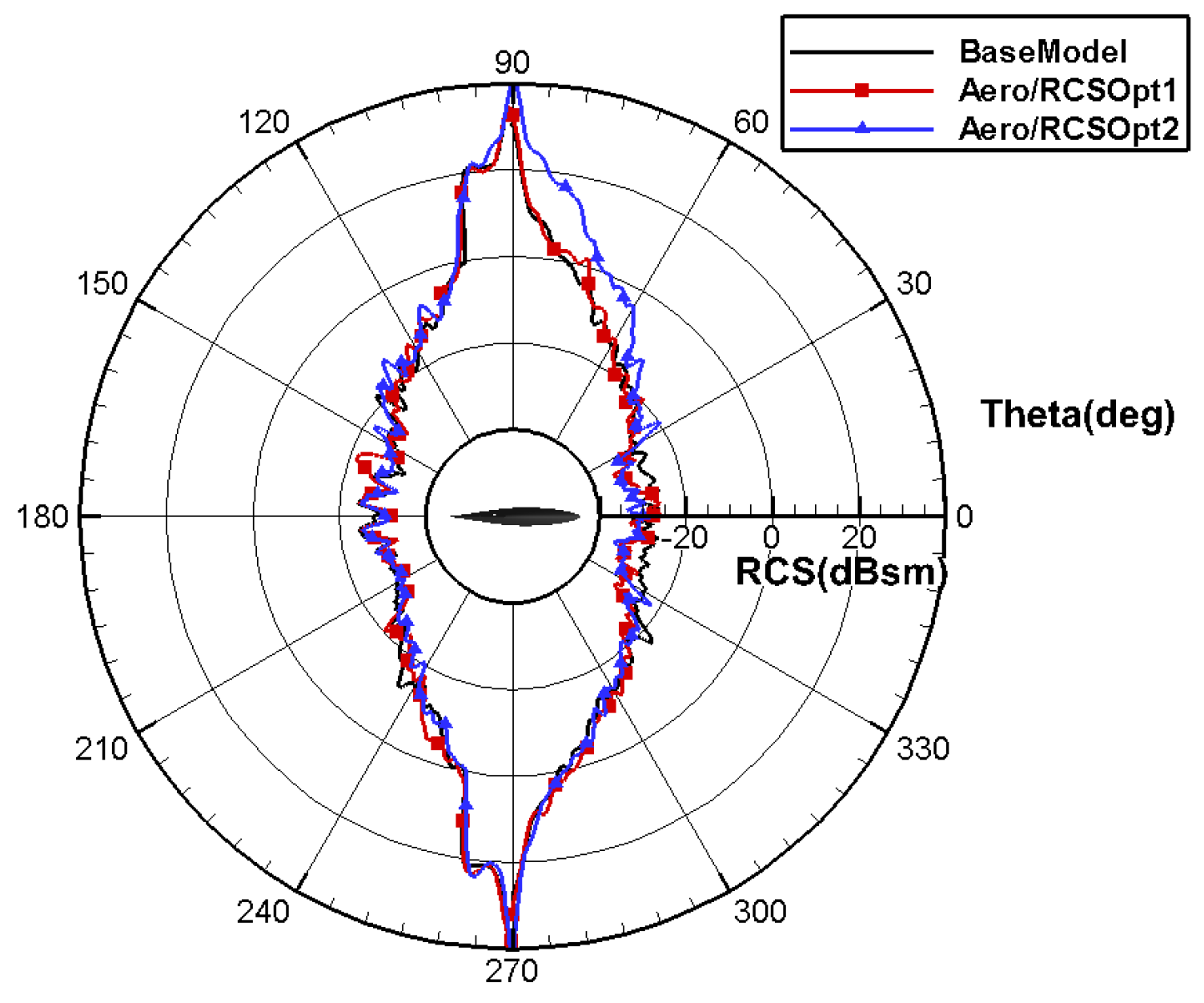
Figure 43.
Comparison of RCS in the pitch direction for layout with aero/stealth root and kink airfoil.
Figure 43.
Comparison of RCS in the pitch direction for layout with aero/stealth root and kink airfoil.
Figure 44.
Comparison of RCS in the yaw direction for layout with aero/stealth root and kink airfoil.
Figure 44.
Comparison of RCS in the yaw direction for layout with aero/stealth root and kink airfoil.
Figure 45.
Comparison of airfoil shape for aero/stealth tip airfoil.
Figure 45.
Comparison of airfoil shape for aero/stealth tip airfoil.
Figure 46.
Comparison of pressure distribution for aero/stealth tip airfoil.
Figure 46.
Comparison of pressure distribution for aero/stealth tip airfoil.
Figure 47.
Pressure distribution for aero/stealth tip airfoil.
Figure 47.
Pressure distribution for aero/stealth tip airfoil.
Figure 48.
Comparison of drag characteristics for aero/stealth tip airfoil.
Figure 48.
Comparison of drag characteristics for aero/stealth tip airfoil.
Figure 49.
Comparison of moment characteristics for aero/stealth tip airfoil.
Figure 49.
Comparison of moment characteristics for aero/stealth tip airfoil.
Figure 50.
Frontal RCS for aero/stealth tip airfoil.
Figure 50.
Frontal RCS for aero/stealth tip airfoil.
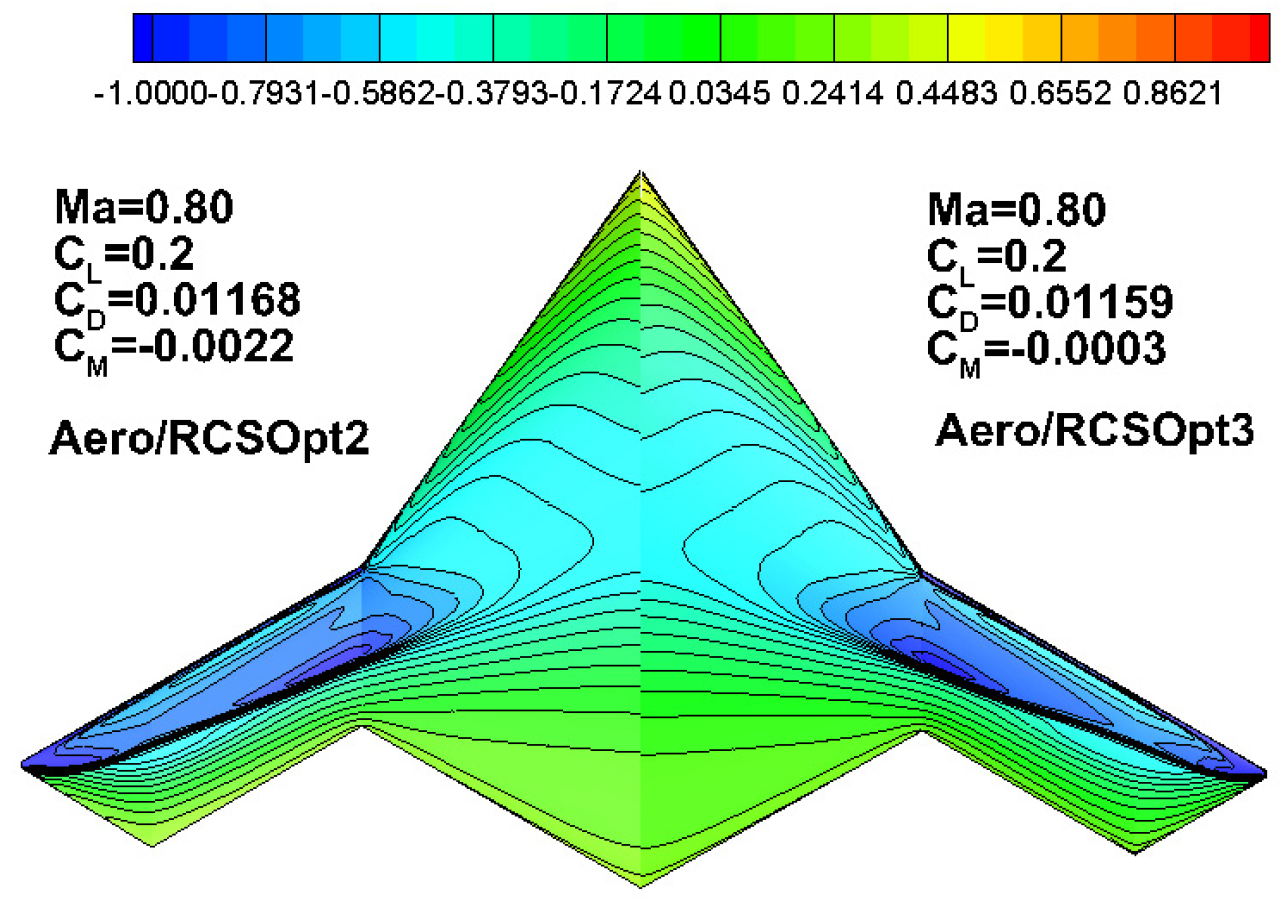
Figure 51.
Pressure distribution for aero/RCSOpt2 (left) and Aero/RCSOpt3 (right).
Figure 51.
Pressure distribution for aero/RCSOpt2 (left) and Aero/RCSOpt3 (right).
Figure 52.
Comparison of RCS in the pitch direction for layout with aero/stealth root, kink and tip airfoil.
Figure 52.
Comparison of RCS in the pitch direction for layout with aero/stealth root, kink and tip airfoil.
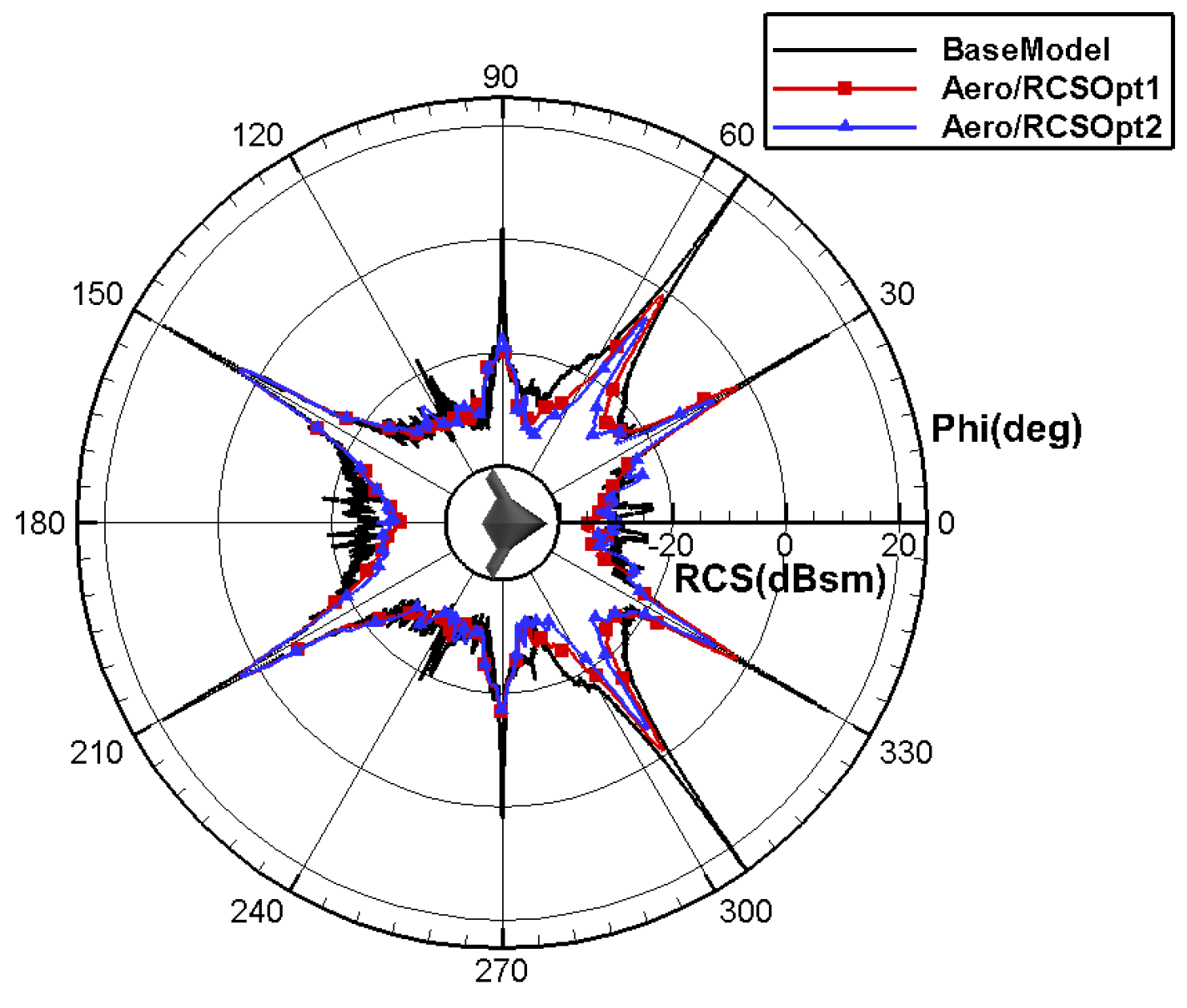
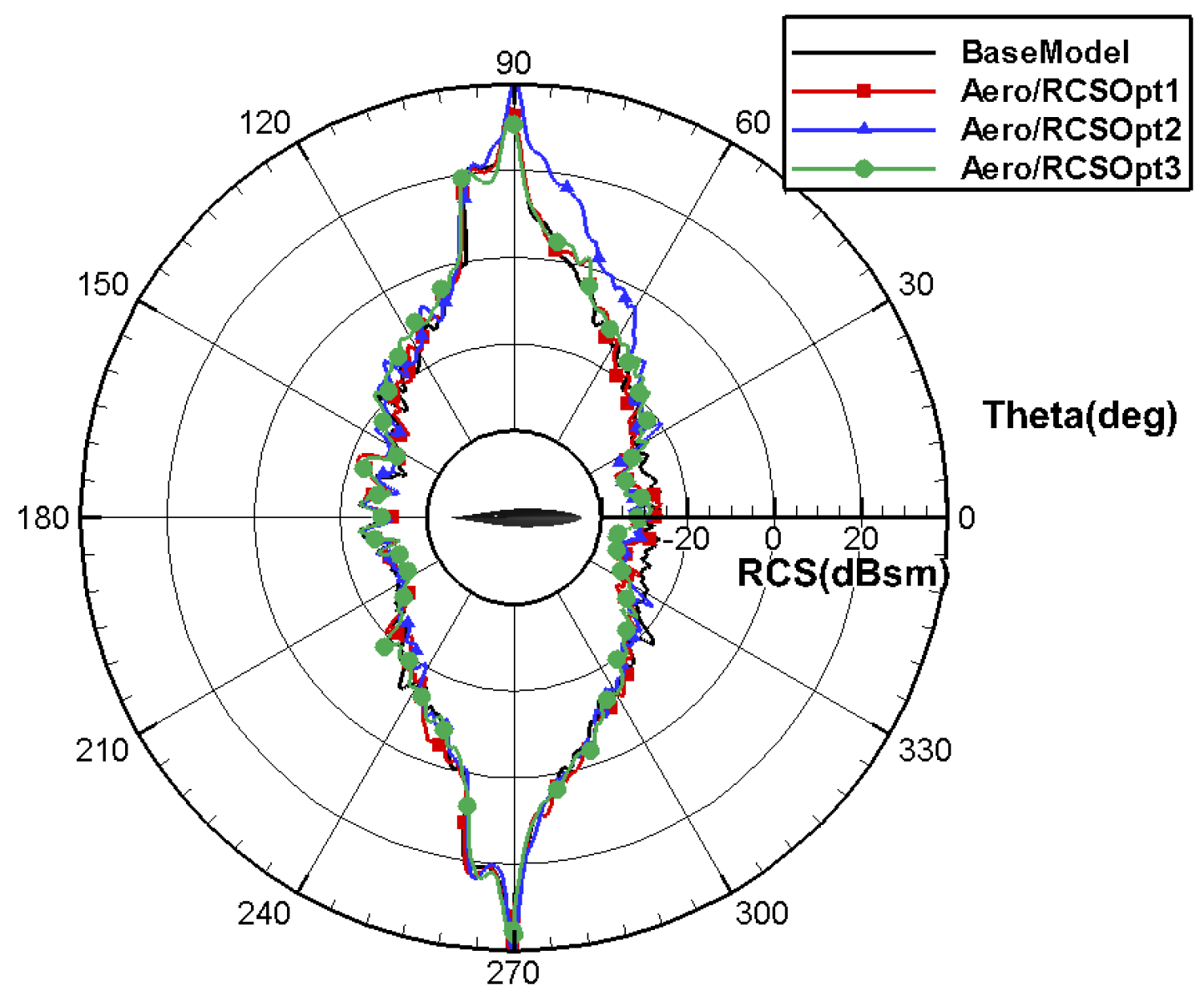
Figure 53.
Comparison of RCS in the yaw direction for layout with aero/stealth root, kink and tip airfoil.
Figure 53.
Comparison of RCS in the yaw direction for layout with aero/stealth root, kink and tip airfoil.
Table 1.
Layout parameters of the baseline aircraft.
Table 1.
Layout parameters of the baseline aircraft.
| Geometric Parameter | Value |
|---|
| Span (m) | 18.93 |
| Reference wing area (m2) | 87.42 |
| Reference center (m) | (6.17, 0, 0) |
| Mean aerodynamic chord (m) | 6.57 |
Table 2.
Computation states.
Table 2.
Computation states.
| Parameter | Value |
|---|
| Cruise Mach number | 0.8 |
| Cruise lift coefficient | 0.2 |
| Reynolds number (unit chord length) | |
| Incident wave frequency (GHz) | 9 |
| Polarization mode | Vertical Polarization |
Table 3.
Computational results for X-47B layout.
Table 3.
Computational results for X-47B layout.
| | Aerodynamic | Stealth |
|---|
| Parameter | CL | CD | CM | Yaw (−60°~60°) | Pitch (−30°~30°) |
| BaseModel | 0.20 | 0.01302 | −0.0192 | −27.6898 | 4.9591 |
Table 4.
RAE2822 airfoil calculation grid parameters.
Table 4.
RAE2822 airfoil calculation grid parameters.
| Far Field | Mesh Size | Normal First Layer Distance | Normal Growth Rate | Leading-Edge Grid Spacing | Trailing-Edge Grid Spacing |
|---|
| 50 | 265 × 101 | 1 × 10−6 | 1.173 | 0.005 | 0.005 |
| 50 | 501 × 201 | 1 × 10−6 | 1.079 | 0.001 | 0.001 |
| 50 | 1001 × 401 | 1 × 10−6 | 1.037 | 0.0005 | 0.0005 |
Table 5.
Comparison of numerical calculation and wind tunnel test results for RAE2822 airfoil.
Table 5.
Comparison of numerical calculation and wind tunnel test results for RAE2822 airfoil.
| | Cl | Cd |
|---|
| EXP | 0.803 | 0.0168 |
| Coarse | 0.731 | 0.0174 |
| Medium | 0.763 | 0.0171 |
| Fine | 0.771 | 0.0170 |
Table 6.
Comparison for aerodynamic results of root airfoil.
Table 6.
Comparison for aerodynamic results of root airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA |
|---|
| NACA 65,3-014 | 0.25 | 0.0067 | - | −0.0067 | - | 1.47° |
| Aero_RootOpt | 0.25 | 0.0065 | −0.0002 | 0.0308 | 0.0375 | 2.13° |
Table 7.
Computational results for layout with aero root airfoil.
Table 7.
Computational results for layout with aero root airfoil.
| Configuration | | Station | | CL | CD | Delta CD | CM | Delta CM |
|---|
| | Root | Kink | Tip | | | | | |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
Table 8.
Comparison for aerodynamic results for aero kink airfoil.
Table 8.
Comparison for aerodynamic results for aero kink airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA |
|---|
| NACA 65,3-013 | 0.40 | 0.0085 | - | −0.0125 | - | 1.98° |
| Aero_KinkOpt | 0.40 | 0.0069 | −0.0016 | 0.0100 | 0.00225 | 2.94° |
Table 9.
Computational results for layout with aero root and kink airfoil.
Table 9.
Computational results for layout with aero root and kink airfoil.
| Configuration | | Station | | CL | CD | Delta CD | CM | Delta CM |
|---|
| | Root | Kink | Tip | | | | | |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
| AeroOpt2 | Aero_RootOpt | Aero_KinkOpt | NACA 65,3-011 | 0.20 | 0.01138 | −0.00164 | −0.0026 | 0.0166 |
Table 10.
Comparison for aerodynamic results for aero tip airfoil.
Table 10.
Comparison for aerodynamic results for aero tip airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA |
|---|
| NACA 65,3-011 | 0.20 | 0.0062 | - | −0.0044 | - | 1.05° |
| Aero_TipOpt | 0.20 | 0.0061 | −0.0001 | −0.0021 | 0.0023 | 0.98° |
Table 11.
Computational results for layout with aero root, kink and tip airfoil.
Table 11.
Computational results for layout with aero root, kink and tip airfoil.
| Configuration | | Station | | CL | CD | Delta CD | CM | Delta CM |
|---|
| | Root | Kink | Tip | | | | | |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
| AeroOpt2 | Aero_RootOpt | Aero_KinkOpt | NACA 65,3-011 | 0.20 | 0.01138 | −0.00164 | −0.0026 | 0.0166 |
| AeroOpt3 | Aero_RootOpt | Aero_KinkOpt | Aero_TipOpt | 0.20 | 0.01129 | −0.00173 | −0.0009 | 0.0183 |
Table 12.
Comparison for aerodynamic/stealth results for aero/stealth root airfoil.
Table 12.
Comparison for aerodynamic/stealth results for aero/stealth root airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA | RCS (m) | Delta RCS (m) |
|---|
| NACA 65,3-014 | 0.25 | 0.0067 | - | −0.0067 | - | 1.47° | 0.0345 | - |
| Aero_RootOpt | 0.25 | 0.0065 | −0.0002 | 0.0308 | 0.0375 | 2.13° | 0.0092 | −0.0253 |
| Aero/RCS_RootOpt | 0.25 | 0.0066 | −0.0001 | 0.0307 | 0.0374 | 2.07° | 0.0029 | −0.0316 |
Table 13.
Airfoil plan.
| Configuration | | Station | |
|---|
| | Root | Kink | Tip |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
| Aero/RCSOpt1 | Aero/RCS_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
Table 14.
Aerodynamic computational results for layout with aero/stealth root airfoil.
Table 14.
Aerodynamic computational results for layout with aero/stealth root airfoil.
| Configuration | CL | CD | Delta CD | CM | Delta CM |
|---|
| BaseModel | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
| Aero/RCSOpt1 | 0.20 | 0.01234 | −0.00068 | −0.0058 | 0.0134 |
Table 15.
Stealth computational results for layout with aero/stealth root airfoil.
Table 15.
Stealth computational results for layout with aero/stealth root airfoil.
| Configuration | Average RCS in Pitch
Direction () dBsm | Delta Average RCS in Pitch Direction () dBsm | Average RCS in Yaw Direction () dBsm | Delta Average RCS in Yaw Direction () dBsm |
|---|
| BaseModel | −27.6898 | - | 4.9591 | - |
| AeroOpt1 | −29.8037 | 2.1139 | 1.0780 | 3.8811 |
| Aero/RCSOpt1 | −30.1186 | 2.4288 | −2.9281 | 7.8872 |
Table 16.
Comparison for aerodynamic/stealth results for aero/stealth kink airfoil.
Table 16.
Comparison for aerodynamic/stealth results for aero/stealth kink airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA | RCS (m) | Delta RCS (m) |
|---|
| NACA 65,3-013 | 0.40 | 0.0085 | - | −0.0125 | - | 1.98° | 0.0299 | - |
| Aero_KinkOpt | 0.40 | 0.0069 | −0.0016 | 0.0100 | 0.0225 | 2.94° | 0.0086 | −0.0213 |
| Aero/RCS_KinkOpt | 0.40 | 0.0070 | −0.0015 | 0.0100 | 0.0225 | 2.51° | 0.0040 | −0.0259 |
Table 17.
Airfoil plan.
| Configuration | | Station | |
|---|
| | Root | Kink | Tip |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
| Aero/RCSOpt1 | Aero/RCS_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
| AeroOpt2 | Aero_RootOpt | Aero_KinkOpt | NACA 65,3-011 |
| Aero/RCSOpt2 | Aero/RCS_RootOpt | Aero/RCS_KinkOpt | NACA 65,3-011 |
Table 18.
Aerodynamic computational results for layout with aero/stealth root and kink airfoil.
Table 18.
Aerodynamic computational results for layout with aero/stealth root and kink airfoil.
| Configuration | CL | CD | Delta CD | CM | Delta CM |
|---|
| BaseModel | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
| Aero/RCSOpt1 | 0.20 | 0.01234 | −0.00068 | −0.0058 | 0.0134 |
| AeroOpt2 | 0.20 | 0.01138 | −0.00164 | −0.0026 | 0.0166 |
| Aero/RCSOpt2 | 0.20 | 0.01168 | −0.00134 | −0.0022 | 0.0170 |
Table 19.
Stealth computational results for layout with aero/stealth root and kink airfoil.
Table 19.
Stealth computational results for layout with aero/stealth root and kink airfoil.
| Configuration | Average RCS in Pitch Direction () dBsm | Delta Average RCS in Pitch Direction () dBsm | Average RCS in Yaw Direction () dBsm | Delta Average RCS in Yaw Direction () dBsm |
|---|
| BaseModel | −27.6898 | - | 4.9591 | - |
| AeroOpt1 | −29.8037 | 2.1139 | 1.0780 | 3.8811 |
| Aero/RCSOpt1 | −30.1186 | 2.4288 | −2.9281 | 7.8872 |
| AeroOpt2 | −30.3734 | 2.6836 | 0.3756 | 4.5835 |
| Aero/RCSOpt2 | −31.7116 | 4.0218 | −4.7836 | 9.7427 |
Table 20.
Comparison for aerodynamic/stealth results for aero/stealth tip airfoil.
Table 20.
Comparison for aerodynamic/stealth results for aero/stealth tip airfoil.
| Configuration | Cl | Cd | Delta Cd | Cm | Delta Cm | AOA | RCS (m) | Delta RCS (m) |
|---|
| NACA 65,3-011 | 0.20 | 0.0062 | - | −0.0044 | - | 1.05° | 0.0219 | - |
| Aero_TipOpt | 0.20 | 0.0061 | 0.0001 | −0.0021 | 0.0023 | 0.98° | 0.0070 | 0.0149 |
| Aero/RCS_TipOpt | 0.20 | 0.0061 | 0.0001 | 0.0017 | 0.0061 | 1.12° | 0.0032 | 0.0187 |
Table 21.
Airfoil plan.
| Configuration | | Station | |
|---|
| | Root | Kink | Tip |
|---|
| BaseModel | NACA 65,3-014 | NACA 65,3-013 | NACA 65,3-011 |
| AeroOpt1 | Aero_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
| Aero/RCSOpt1 | Aero/RCS_RootOpt | NACA 65,3-013 | NACA 65,3-011 |
| AeroOpt2 | Aero_RootOpt | Aero_KinkOpt | NACA 65,3-011 |
| Aero/RCSOpt2 | Aero/RCS_RootOpt | Aero/RCS_KinkOpt | NACA 65,3-011 |
| AeroOpt3 | Aero_RootOpt | Aero_KinkOpt | Aero_TipOpt |
| Aero/RCSOpt3 | Aero/RCS_RootOpt | Aero/RCS_KinkOpt | Aero/RCS_TipOpt |
Table 22.
Aerodynamic computational results for layout with aero/stealth root, kink and tip airfoil.
Table 22.
Aerodynamic computational results for layout with aero/stealth root, kink and tip airfoil.
| Configuration | CL | CD | Delta CD | CM | Delta CM |
|---|
| BaseModel | 0.20 | 0.01302 | - | −0.0192 | - |
| AeroOpt1 | 0.20 | 0.01220 | −0.00082 | −0.0058 | 0.0134 |
| Aero/RCSOpt1 | 0.20 | 0.01234 | −0.00068 | −0.0058 | 0.0134 |
| AeroOpt2 | 0.20 | 0.01138 | −0.00164 | −0.0026 | 0.0166 |
| Aero/RCSOpt2 | 0.20 | 0.01168 | −0.00134 | −0.0022 | 0.0170 |
| AeroOpt3 | 0.20 | 0.01129 | −0.00173 | −0.0009 | 0.0183 |
| Aero/RCSOpt3 | 0.20 | 0.01159 | −0.00143 | −0.0003 | 0.0189 |
Table 23.
Stealth computational results for layout with aero/stealth root, kink and tip airfoil.
Table 23.
Stealth computational results for layout with aero/stealth root, kink and tip airfoil.
| Configuration | Average RCS in Pitch
Direction () dBsm | Delta Average RCS in Pitch Direction () dBsm | Average RCS in Yaw Direction () dBsm | Delta Average RCS in Yaw Direction () dBsm |
|---|
| BaseModel | −27.6898 | - | 4.9591 | - |
| AeroOpt1 | −29.8037 | 2.1139 | 1.0780 | 3.8811 |
| Aero/RCSOpt1 | −30.1186 | 2.4288 | −2.9281 | 7.8872 |
| AeroOpt2 | −30.3734 | 2.6836 | 0.3756 | 4.5835 |
| Aero/RCSOpt2 | −31.7116 | 4.0218 | −4.7836 | 9.7427 |
| AeroOpt3 | −30.6619 | 2.9721 | 0.0545 | 4.9046 |
| Aero/RCSOpt3 | −32.5061 | 4.8163 | −7.6436 | 12.3951 |