Numerical Simulation of Gas Flow Coupled with Burning Surface Regression Based on Immersed Boundary Method and Face Offsetting Method
Abstract
1. Introduction
2. Governing Equations and Discrete Methods of Euler Flow
- (1)
- The combustion of propellant is completed instantaneously on the burning surface, without considering the physical and chemical reaction processes such as the phase transition and combustion. The newly generated gas is injected into the combustion chamber along the local burning surface, and it is evenly mixed with the main gas stream after injection. Moreover, there is no chemical reaction during the flow process. The chemical composition and thermodynamic parameters of the newly generated gas are exactly the same as those of the main gas stream.
- (2)
- The gas in the motor is a pure gas phase and an ideal gas with a constant specific heat capacity, and the friction between the gas and the channel surface is ignored.
- (3)
- The gas flow in the motor is adiabatic, and there is no heat and work exchange with the external environment.
- (4)
- The influence of gravity and other forces on the gas flow is ignored.
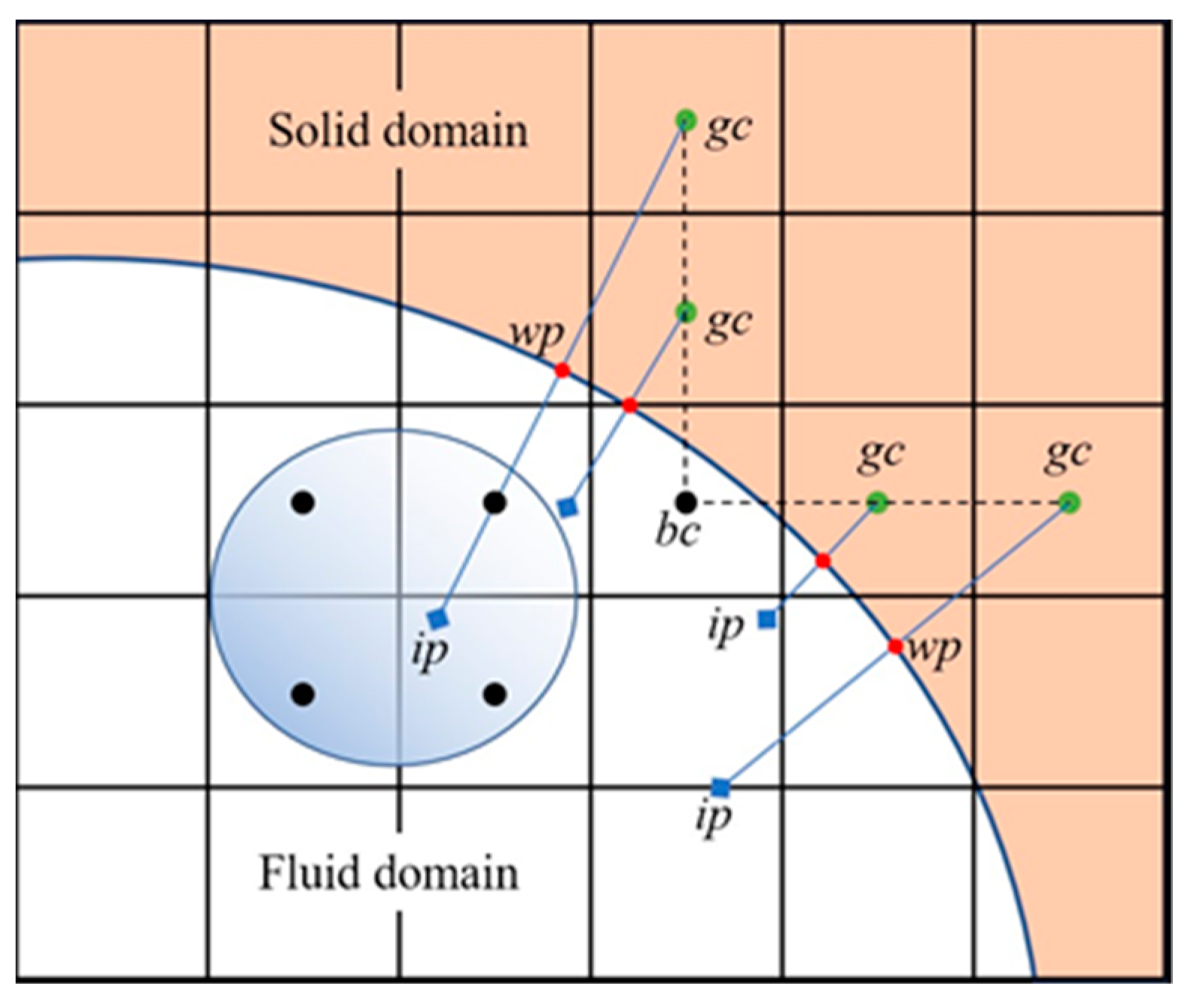
3. Application of Immersed Boundary Method
3.1. Slip Wall
3.2. Mass Flow Inlet
3.3. Pressure Outlet
3.4. Rotational Periodic Boundary
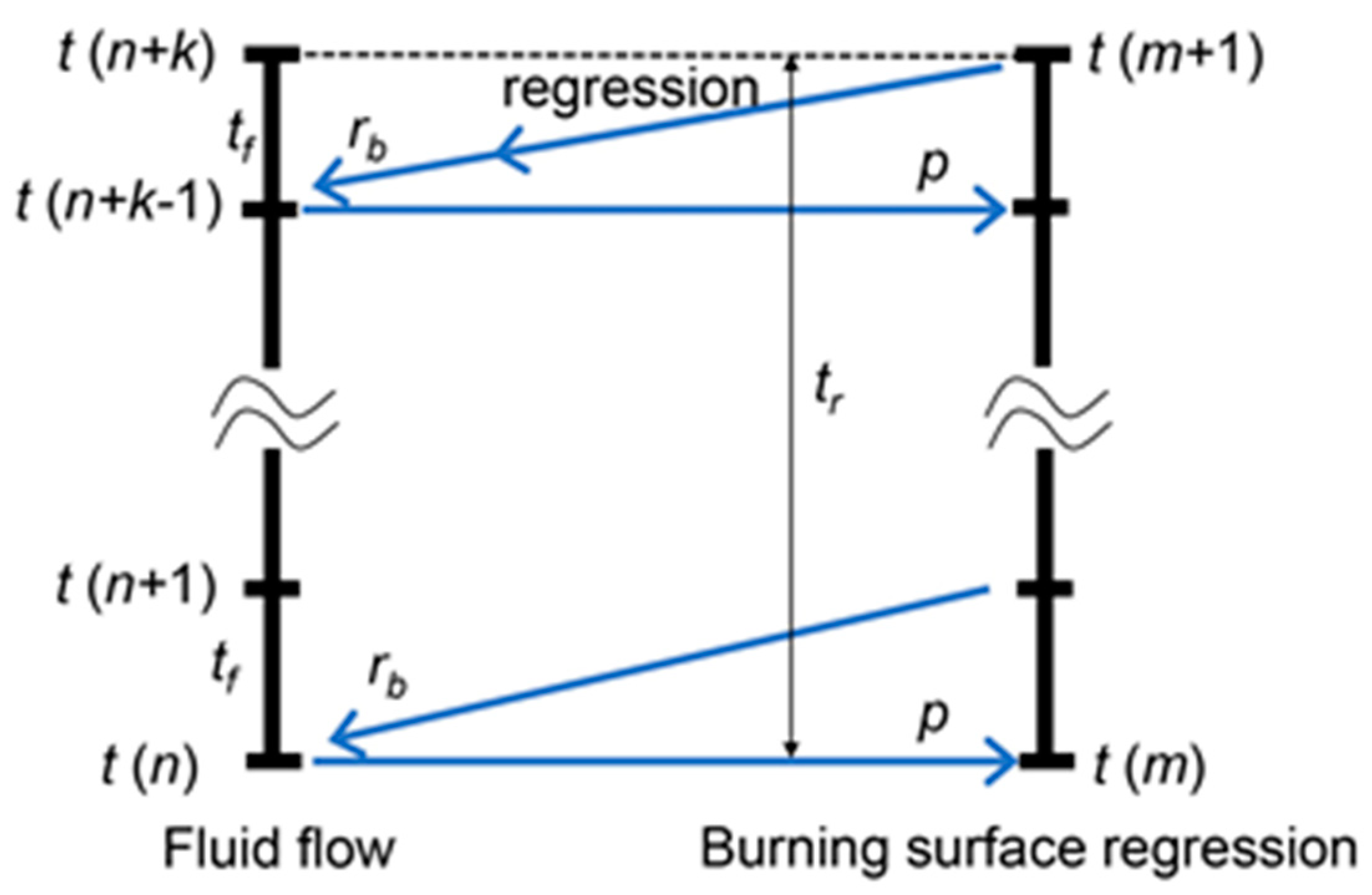
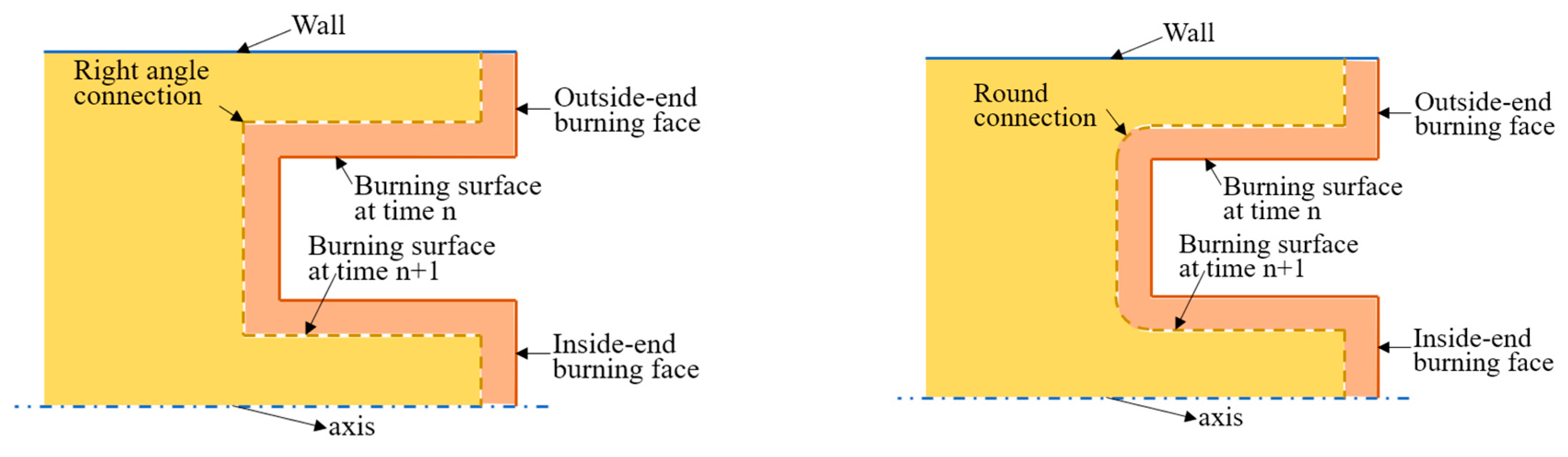
4. Application of Face Offsetting Method
5. Coupling Calculation Method
6. Numerical Results and Discussion
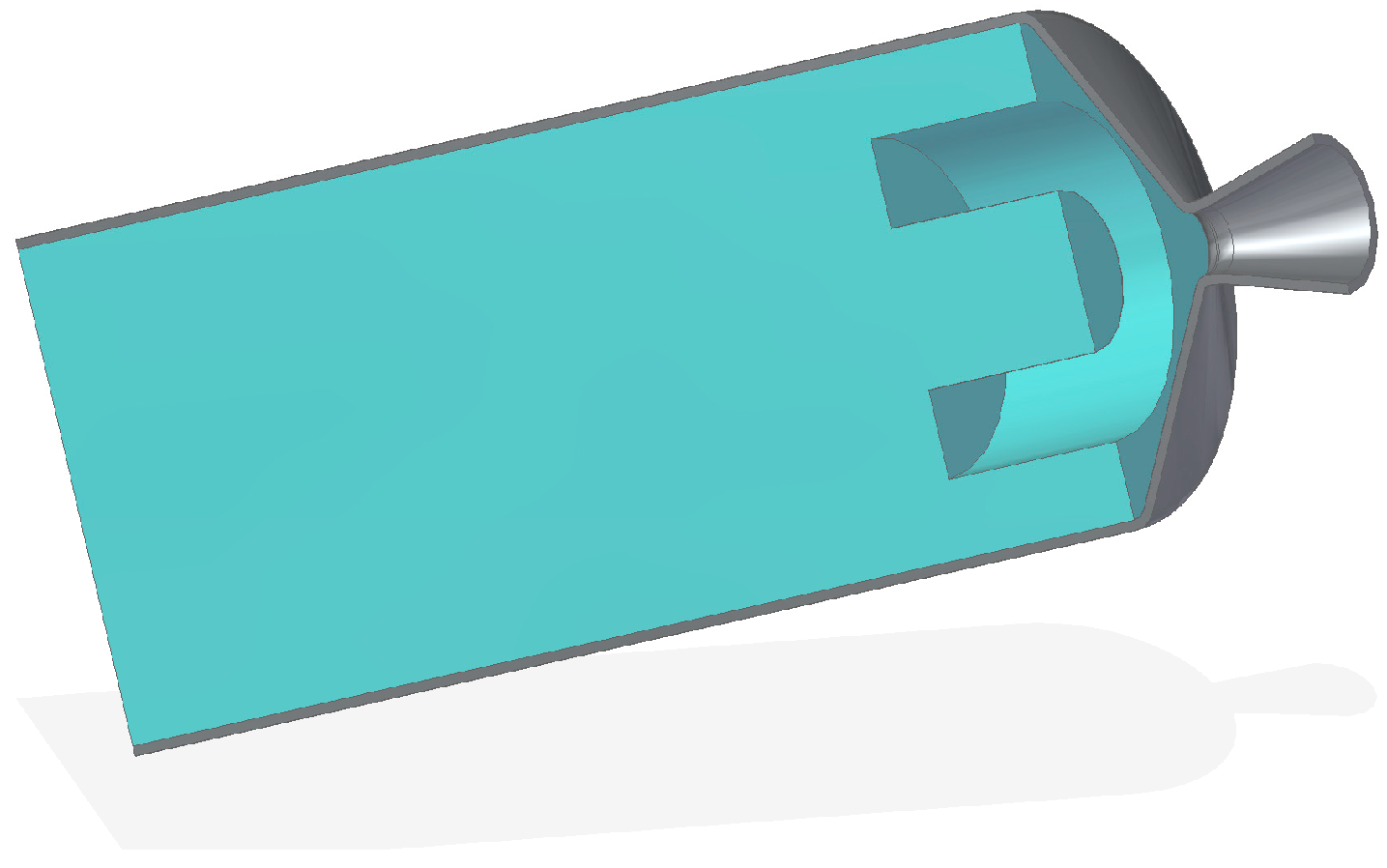
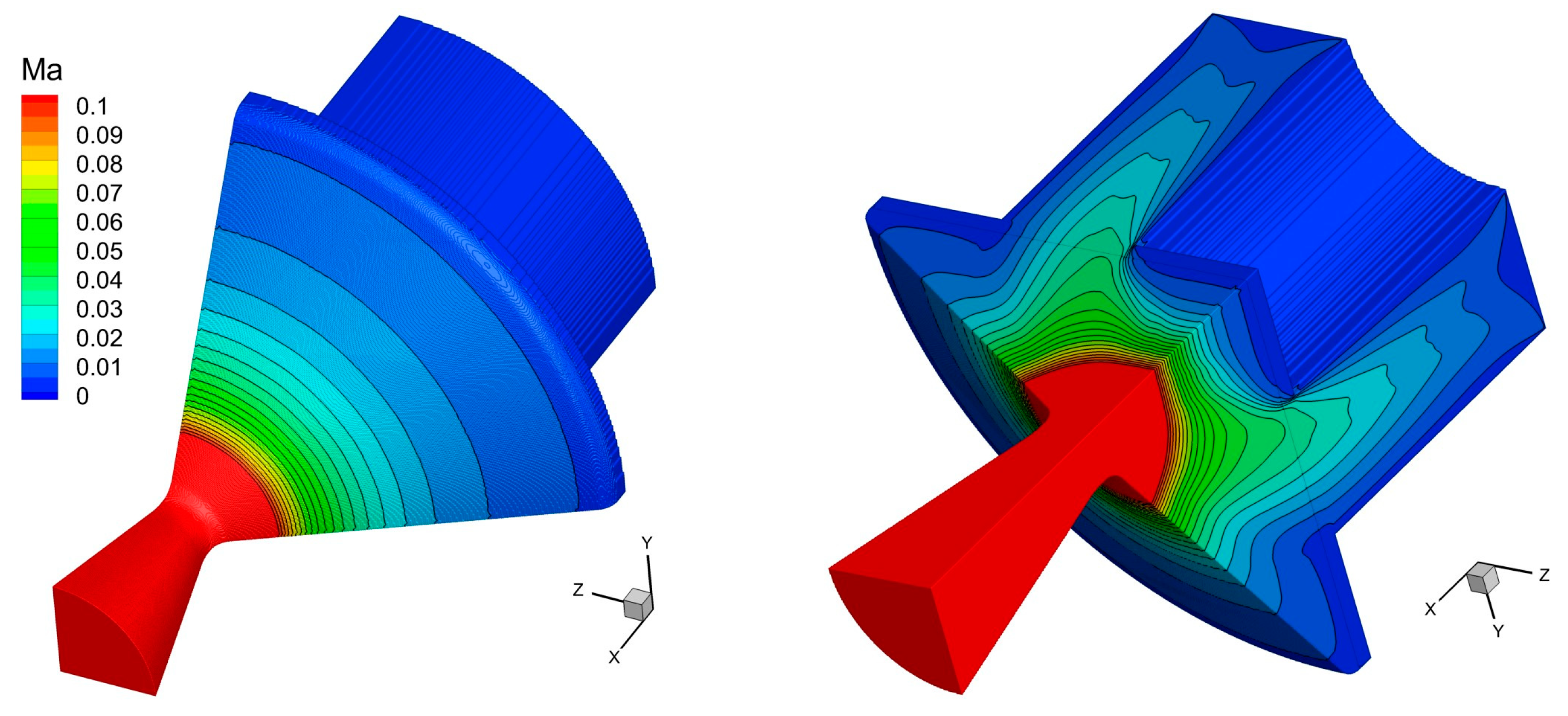
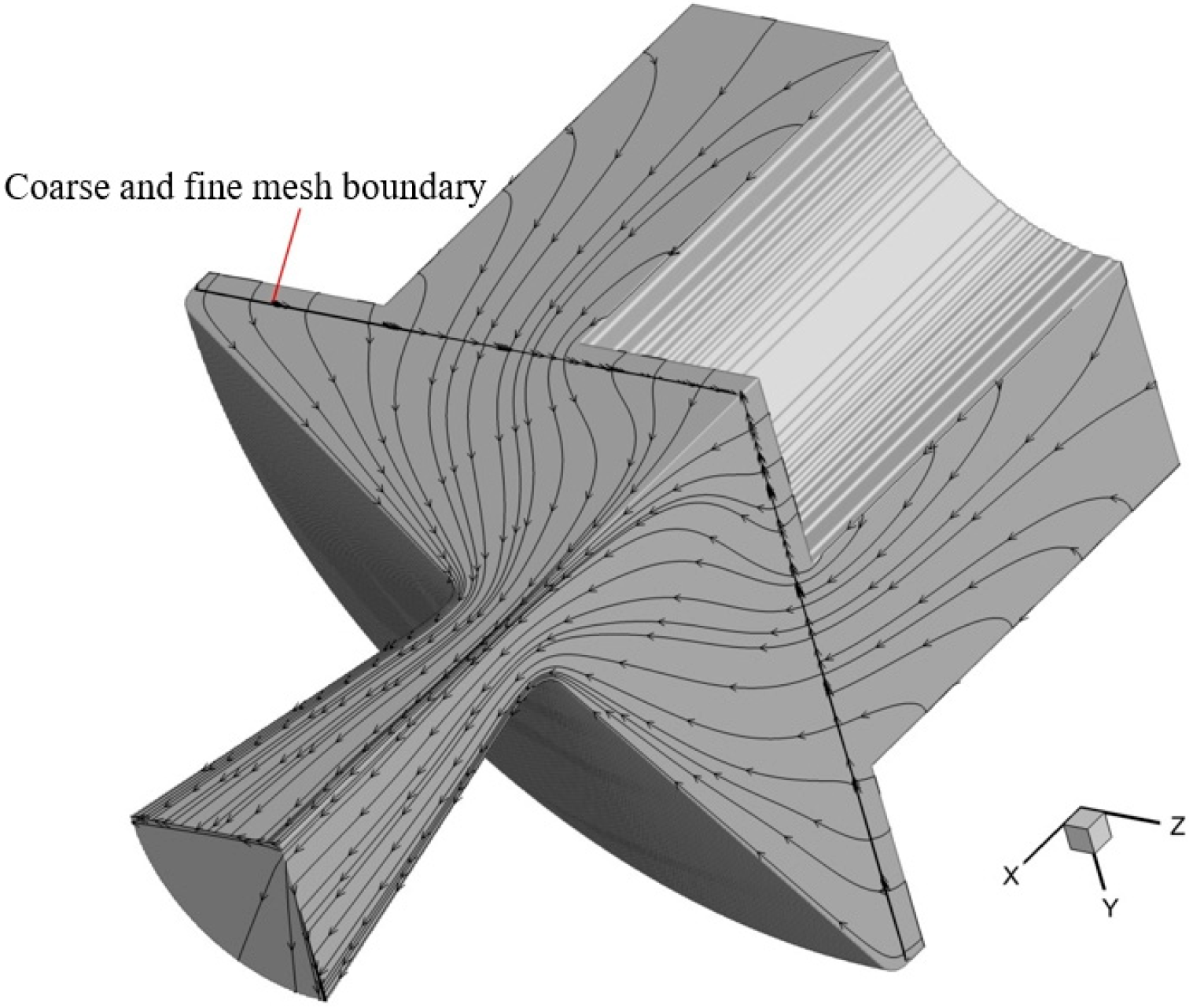
6.1. Numerical Simulation and Result Analysis of Three-Dimensional Internal Flow Field of Motor with End-Slotted and End-Burning Propellant
6.1.1. Quasi-Static Flow
6.1.2. Transient Flow Coupled with Burning Surface Regression
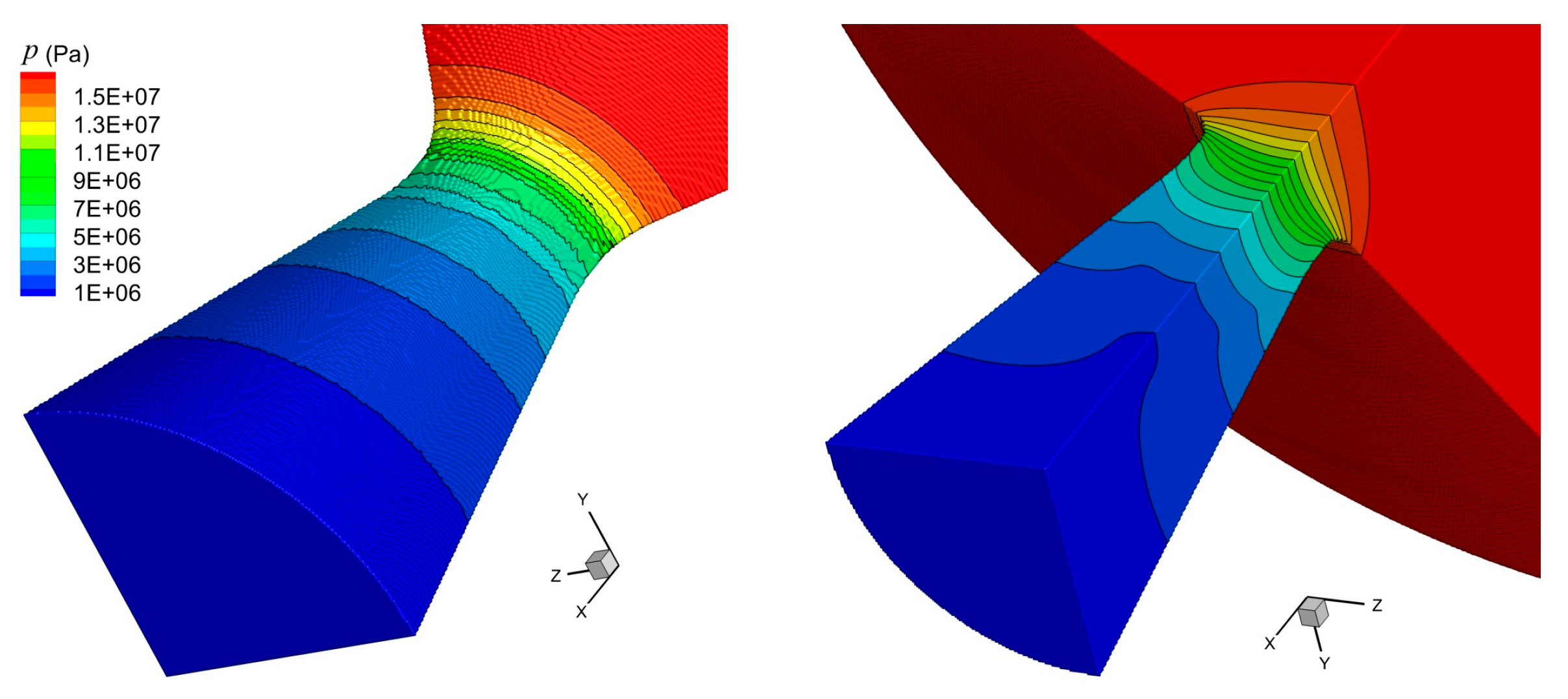
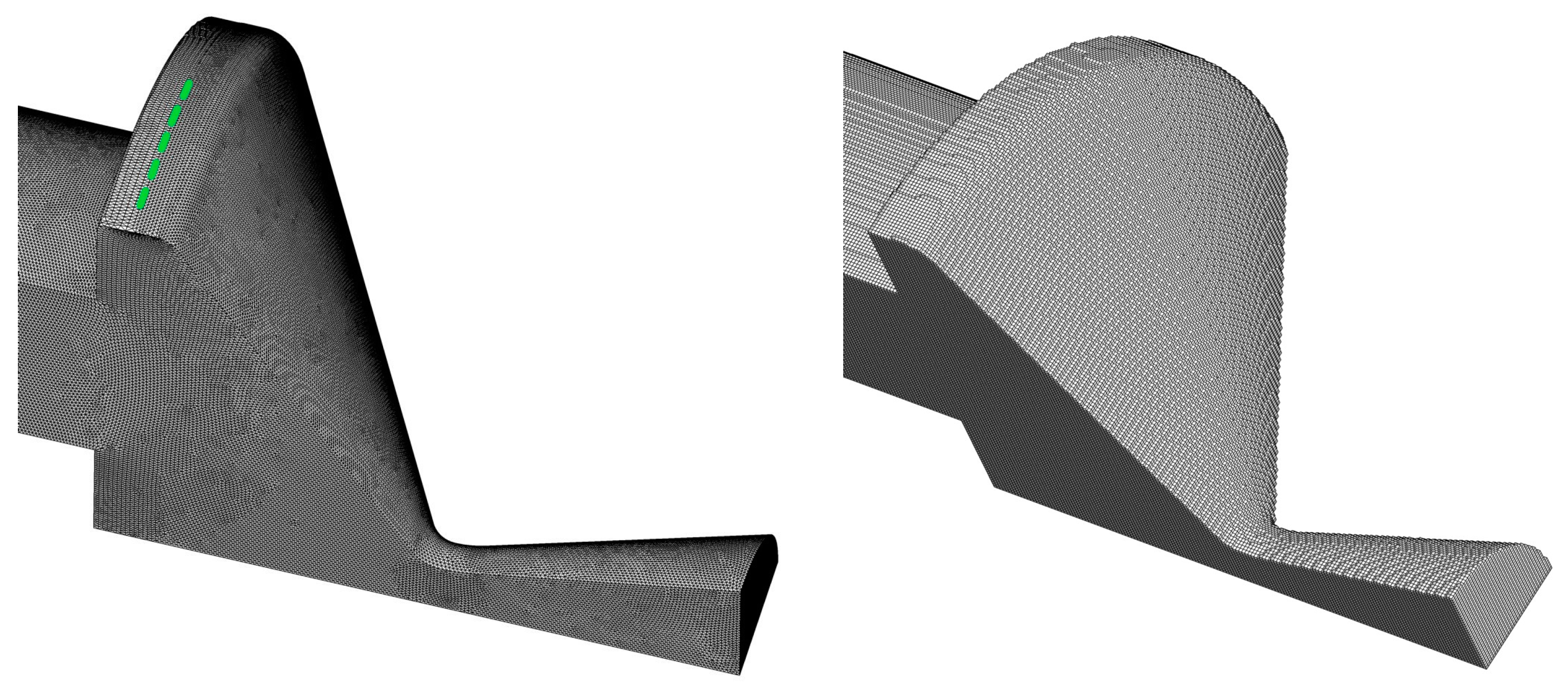
6.2. Numerical Simulation and Experimental Verification of Three-Dimensional Internal Flow Field of Erosive Burning Motor
7. Conclusions
- (1)
- The immersed boundary method and face offsetting method are combined to simulate the three-dimensional internal flow field in the motor in the case of the parallel burning surface regression with the erosive burning. The coupling problem of gas flow with non-parallel burning surface regression with erosive burning is solved. By comparing and analyzing the calculation results of the axisymmetric two-dimensional model and the experimental results in the reference, the rationality of the result obtained by the three-dimensional immersed boundary method coupled with the face offsetting method is verified.
- (2)
- For the end-burning propellant, the intersection point between the propellant end surface and the shell wall is set to remain fixed so that the nearby surface grid can remain smooth during the movement. Although the combustion surface area fluctuation can result in the same frequency fluctuation of combustion chamber pressure, the amplitude of fluctuation is small; moreover, the frequency is low and can be identified. Generally, it does not interfere with the pressure fluctuation caused by vortex shedding.
- (3)
- The simulation result of three-dimensional gas flow coupled with the burning surface regression is reasonable and reliable, but the accuracy of the result is slightly low due to the large grid size. The follow-up work focuses on the distributed and parallel improvement of the existing calculation program so as to achieve the application of a fine enough grid for more accurate results.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guoy, D.; Wilmarth, T.; Alexander, P.J.; Jiao, X.; Campbell, M.; Shaffer, E.; Fiedler, R.; Cochran, W. Parallel mesh adaptation for highly evolving geometries with application to solid propellant rockets. In Proceedings of the 16th International Meshing Roundtable; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- He, Z.; Gao, Y.; Gu, X.; Wang, G. The Study of Dynamic Grids Application to Calculate the Inner Flow Field of Solid Rocket Motor. J. Proj. Rocket. Missiles Guid. 2008, 28, 164–166. (In Chinese) [Google Scholar]
- Tang, B. Numerical Simulation of Erosive Burning Unsteady Process in Solid Rocket Motor. Ph.D. Thesis, Nanjing University of Science and Technology, Nanjing, China, 2014. (In Chinese). [Google Scholar]
- Song, D.; Zhou, C. Numerical simulation of Transient Internal Flowfields of Solid Rocket Motor. J. Proj. Rocket. Missiles Guid. 2010, 30, 147–149. (In Chinese) [Google Scholar]
- Wei, T. Study on Combustion Chamber Flow Field of Solid Fuel Ramjet. Ph.D. Thesis, Nanjing University of Science and Technology, Nanjing, China, 2012. (In Chinese). [Google Scholar]
- Su, T. Analysis and Numerical Simulation of a Solid Rocket Booster Flow Field. Ph.D. Thesis, Nanjing University of Science and Technology, Nanjing, China, 2016. (In Chinese). [Google Scholar]
- Gueyffier, D.; Roux, F.X.; Fabignon, Y.; Chaineray, G.; Lupoglazoff, N.; Vuillot, F.; Hijlkema, J.; Alauzet, F. Accurate Computation of Grain Burning Coupled with Flow Simulation in Rocket Chamber. J. Propuls. Power 2015, 31, 1761–1776. [Google Scholar] [CrossRef]
- Li, Q.; He, G.Q.; Liu, P.J.; Li, J. Coupled simulation of fluid flow and propellant burning surface regression in a solid rocket motor. Comput. Fluids 2014, 93, 146–152. [Google Scholar] [CrossRef]
- Zhou, W.; Xie, F.; Li, S. Coupled Simulation of Internal Flow Field and Burning Surface Regression in a Single-Pulse Solid Attitude Control Motor. J. Propuls. Technol. 2019, 40, 107–114. (In Chinese) [Google Scholar]
- Han, W. Simulation of the Transient Internal Flow of SRM with the Grain Surface Regression Based on Level-Set Method. Ph.D. Thesis, Harbin Engineering University, Harbin, China, 2016. (In Chinese). [Google Scholar]
- Wang, G.; Li, D.; Han, W.; Zhang, Y. Simulation of Transient Internal Flow Field of Solid Rocket Motor Based on Level-set Method. Acta Armament. 2017, 38, 1520–1531. (In Chinese) [Google Scholar]
- Zhang, Y. Simulation of Working Process of SRM with Complex Grain Based on Porous Media Model. Ph.D. Thesis, Harbin Engineering University, Harbin, China, 2018. (In Chinese). [Google Scholar]
- Mittal, R.; Iaccarino, G. Immersed boundary methods. Annu. Rev. Fluid Mech. 2005, 37, 239–261. [Google Scholar] [CrossRef]
- Sotiropoulos, F.; Yang, X. Immersed boundary methods for simulating fluid–structure interaction. Prog. Aerosp. Sci. 2014, 65, 1–21. [Google Scholar] [CrossRef]
- Tran, P.H.; Plourde, F. Computing compressible internal flows by means of an Immersed Boundary Method. Comput. Fluids 2014, 97, 21–30. [Google Scholar] [CrossRef]
- Tran, P.H.; Plourde, F. Application of immersed boundary technique in SRM simulations: Toward new insights. In Proceedings of the AIAA Computational Fluid Dynamics Conference, San Diego, CA, USA, 24–27 June 2013. [Google Scholar]
- Hirose, T.; Ogawa, S.; Sasaki, D.; Fukushima, Y.; Obayashi, S. Application of Immersed Boundary Method with Wall Injection for Solid Rocket Motor Internal Flow. In Proceedings of the AIAA Modeling and Simulation Technologies Conference, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar]
- Lin, Q.; Jiang, K.; Wang, H.; Wang, J. Application of immersed boundary method to the simulation of transient flow in solid rocket motors. Aerosp. Sci. Technol. 2021, 119, 107228. [Google Scholar] [CrossRef]
- Lin, Q.; Wang, J.; Jiang, K.; Wang, H. Application of immersed boundary method to the simulation of three-dimensional flow in solid rocket motors. AIP Adv. 2023, 13, 065016. [Google Scholar]
- Yuan, C. Numerical Simulation of SRM Grain Regression and Flow Field Based on Immersed Boundary Method. Ph.D. Thesis, Harbin Engineering University, Harbin, China, 2018. (In Chinese). [Google Scholar]
- Osher, S.; Sethian, J. Fronts propagating with curvature-dependent speed: Algorithms based on Hamilton–Jacobi formulations. J. Comput. Phys. 1988, 79, 12–49. [Google Scholar] [CrossRef]
- Osher, S.; Fedkiw, R. Level Set Methods and Dynamic Implicit Surfaces; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Sethian, J. Level Set Methods and Fast Marching Methods; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Puckett, E.G.; Almgren, A.S.; Bell, J.B.; Marcus, D.L.; Rider, W.J. A High-Order Projection Method for Tracking Fluid Interfaces in Variable Density Incompressible Flows. J. Comput. Phys. 1997, 130, 269–282. [Google Scholar] [CrossRef]
- Rudman, M. Volume-tracking methods for interfacial flow calculations. Int. J. Numer. Methods Fluids 1997, 24, 671–691. [Google Scholar] [CrossRef]
- Willcox, M.A.; Brewster, M.Q.; Tang, K.C.; Stewart, D.C. Solid Propellant Grain Design and Burnback Simulation Using a Minimum Distance Function. J. Propuls. Power 2007, 23, 465–475. [Google Scholar] [CrossRef]
- Jiao, X. Face offsetting: A unified approach for explicit moving interfaces. J. Comput. Phys. 2007, 220, 612–625. [Google Scholar] [CrossRef]
- Qin, F. Algorithm Research on Burning Surface of Complex Charge for Solid Rocket Motor. Ph.D. Thesis, Northwestern Polytechnical University, Xi’an, China, 2003. (In Chinese). [Google Scholar]
- Wei, R.; Bao, F.; Liu, Y.; Hui, W. Combined Acceleration Methods for Solid Rocket Motor Grain Burnback Simulation Based on the Level Set Method. Int. J. Aerosp. Eng. 2018, 2018, 4827810. [Google Scholar]
- Oh, S.H.; Lee, H.J.; Roh, T.S. Development of a hybrid method in a 3-D numerical burn-back analysis for solid propellant grains. Aerosp. Sci. Technol. 2020, 106, 106103. [Google Scholar] [CrossRef]
- Ren, P.; Wang, H.; Zhou, G.; Li, J.; Cai, Q.; Yu, J.; Yuan, Y. Solid rocket motor propellant grain burnback simulation based on fast minimum distance function calculation and improved marching tetrahedron method. Chin. J. Aeronaut. 2021, 34, 208–224. [Google Scholar] [CrossRef]
- Dunlap, R.; Hermsen, R.W.; Willouchby, P.G. Flowfield in the Combustion Chamber of a Solid Propellant Rocket Motor. AIAA J. 1974, 12, 1440–1442. [Google Scholar] [CrossRef]
- Wu, X.; Chen, J.; Wang, D. Principles of Solid Rocket Engines; Ordnance Industry Press: Beijing, China, 2015. (In Chinese) [Google Scholar]
- Toro, E.F.; Spruce, M.; Speares, W. Restoration of the contact surface in the HLL Riemann solver. Shock Waves 1994, 4, 25–34. [Google Scholar] [CrossRef]
- Toro, E.F. Riemann Solvers and Numerical Methods for Fluid Dynamics: A Practical Introduction; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Harten, A.; Lax, P.D.; Van Leer, B. On upstream differencing and Godunov-type scheme for hyperbolic conservation laws. SIAM Rev. 1983, 25, 35–61. [Google Scholar] [CrossRef]
- Batten, P.; Leschziner, M.A.; Goldberg, U.C. Average-state Jacobians and implicit methods for compressible viscous and turbulent flows. J. Comput. Phys. 1997, 137, 38–78. [Google Scholar] [CrossRef]
- Ghias, R.; Mittal, R.; Dong, H. A sharp interface immersed boundary method for compressible viscous flows. J. Comput. Phys. 2007, 225, 528–553. [Google Scholar] [CrossRef]
- Mittal, R.; Dong, H.; Bozkurttas, M.; Najjar, F.; Vargas, A.; von Loebbecke, A. A versatile sharp interface immersed boundary method for incompressible flows with complex boundaries. J. Comput. Phys. 2008, 227, 4825–4852. [Google Scholar] [CrossRef] [PubMed]
- Dadone, A.; Grossman, B. Ghost-Cell Method for Inviscid Two-Dimensional Flows on Cartesian Grids. Comput. Fluids 2007, 36, 1513–1528. [Google Scholar] [CrossRef]
- Matsumura, Y.; Jackson, T.L. Numerical simulation of fluid flow through random packs of cylinders using immersed boundary method. Phys. Fluids 2014, 26, 043602. [Google Scholar] [CrossRef]
- Xiao, W.; Mao, C.; Jin, T.; Luo, K.; Fan, J. Fully resolved simulation of a shockwave interacting with randomly clustered particles via a ghost-cell immersed boundary method. Phys. Fluids 2020, 32, 066105. [Google Scholar] [CrossRef]
- Mortimer, F.; Fairweather, M. Assessment of behavioral modification techniques through immersed boundary method simulation of binary particle interactions in isotropic turbulence. Comput. Fluid 2021, 33, 073307. [Google Scholar] [CrossRef]
- Cai, S.G.; Mozaffari, S.; Jacob, J.; Sagaut, P. Application of immersed boundary based turbulence wall modeling to the Ahmed body aerodynamics. Phys. Fluids 2022, 34, 095106. [Google Scholar] [CrossRef]
- Li, C.; Bale, R.; Wang, W.; Tsubokura, M. A sharp interface immersed boundary method for thin-walled geometries in viscous compressible flows. Int. J. Mech. Sci. 2023, 253, 108401. [Google Scholar] [CrossRef]
- Wang, X.; Deiterding, R.; Liang, J.; Cai, X.; Zhao, W. A second-order-accurate immersed boundary ghost-cell method with hybrid reconstruction for compressible flow simulations. Comput. Fluids 2022, 237, 105314. [Google Scholar] [CrossRef]
- Choung, H.; Saravanan, V.; Lee, S.; Cho, H. Nonlinear weighting process in ghost-cell immersed boundary methods for compressible flow. J. Comput. Phys. 2021, 433, 110198. [Google Scholar] [CrossRef]
- Jiao, X. Volume and Feature Preservation in Surface Mesh Optimization. In Proceedings of the 15th International Meshing Roundtable; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Song, Y. Study on Burning Law of Single Chamber Dual Thrust of Grain for Solid Rocket Motor. Master’s Thesis, Shenyang Ligong University, Shenyang, China, 2017. (In Chinese). [Google Scholar]
- Hasegawa, H.; Tokudome, S.I.; Hanzawa, M.; Kohno, M. Erosive Burning of Aluminized Composite Propellants: X-ray Absorption Measurement, Correlation, and Application. J. Propuls. Power 2006, 22, 975–983. [Google Scholar] [CrossRef]

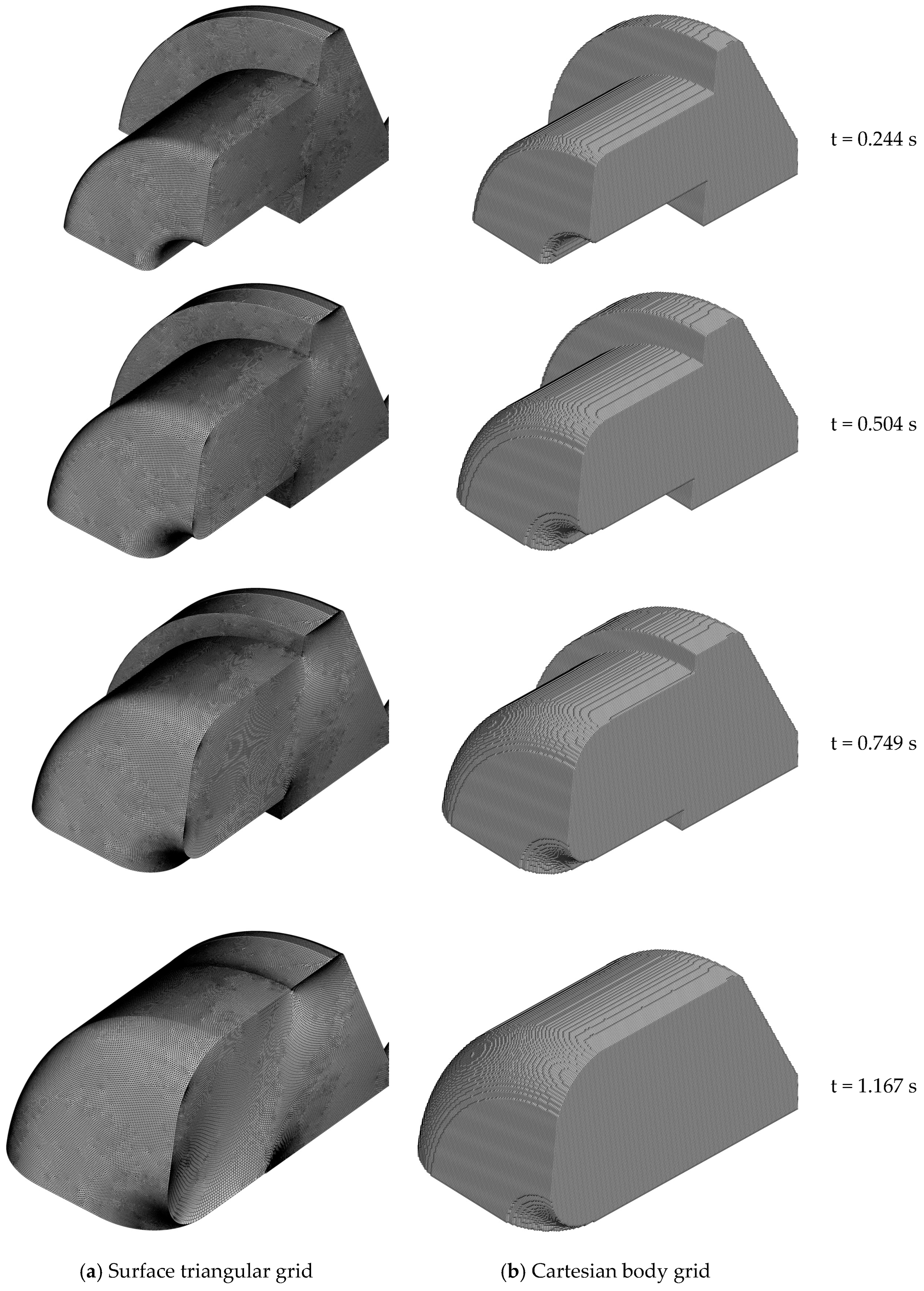
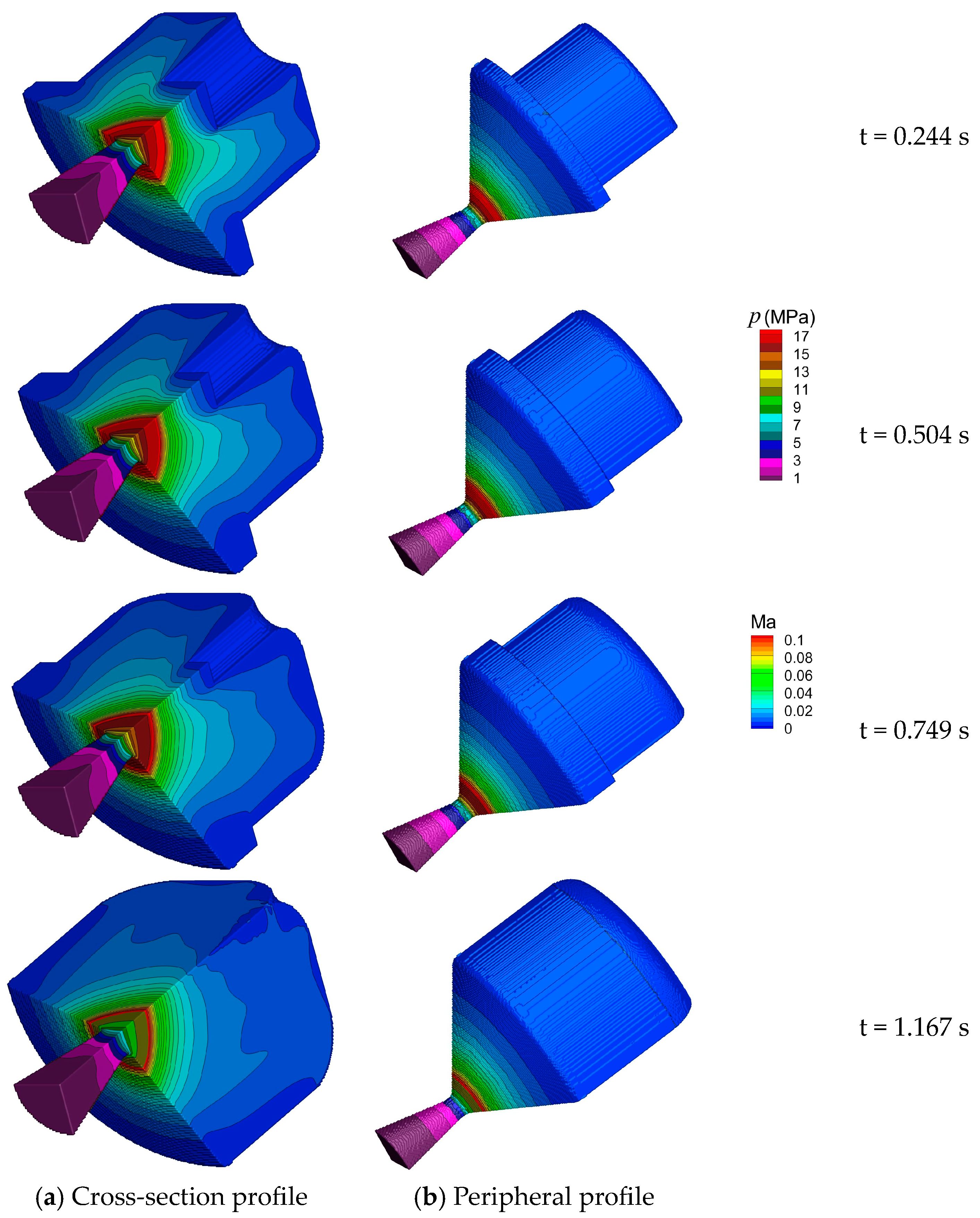
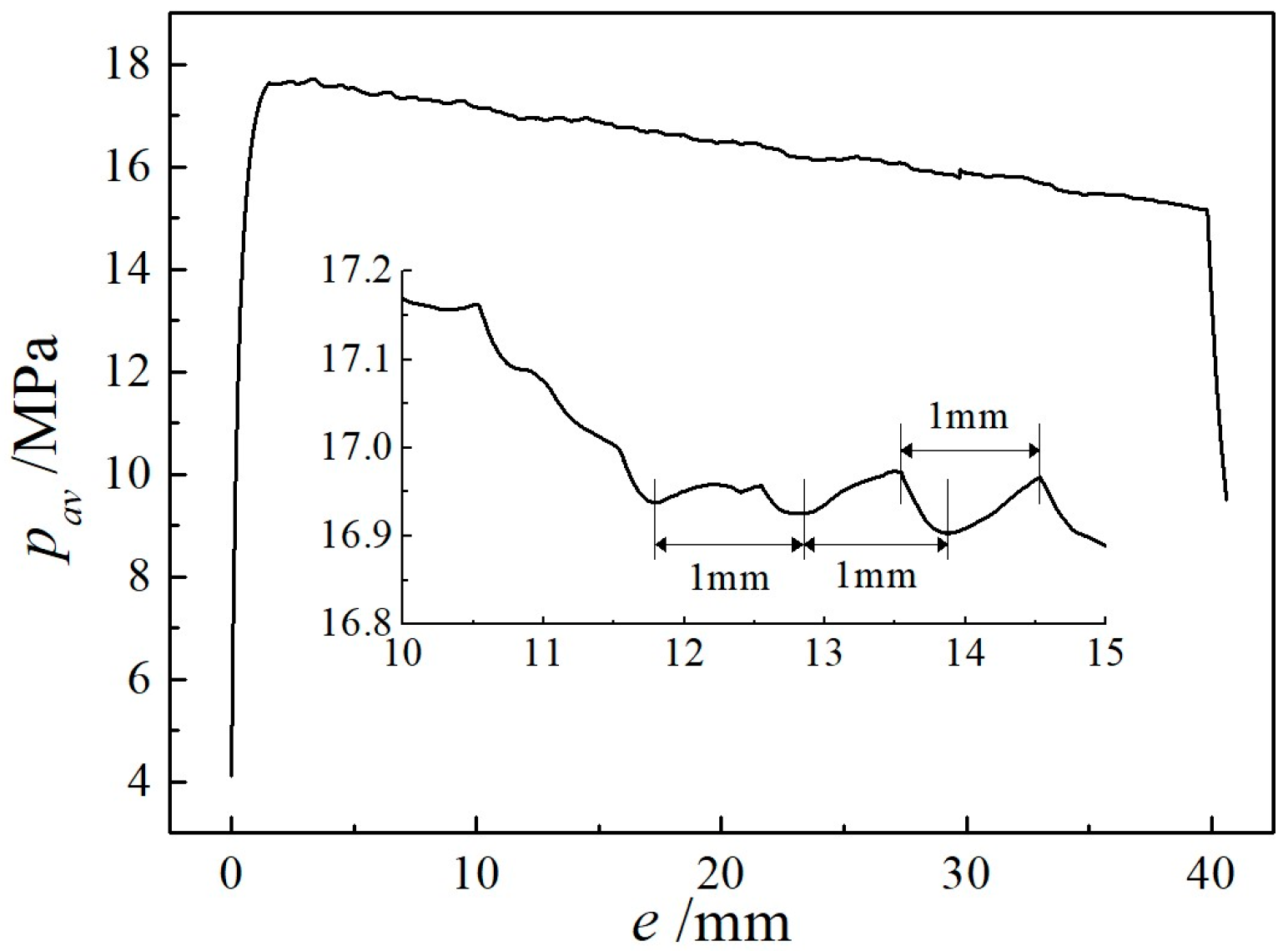
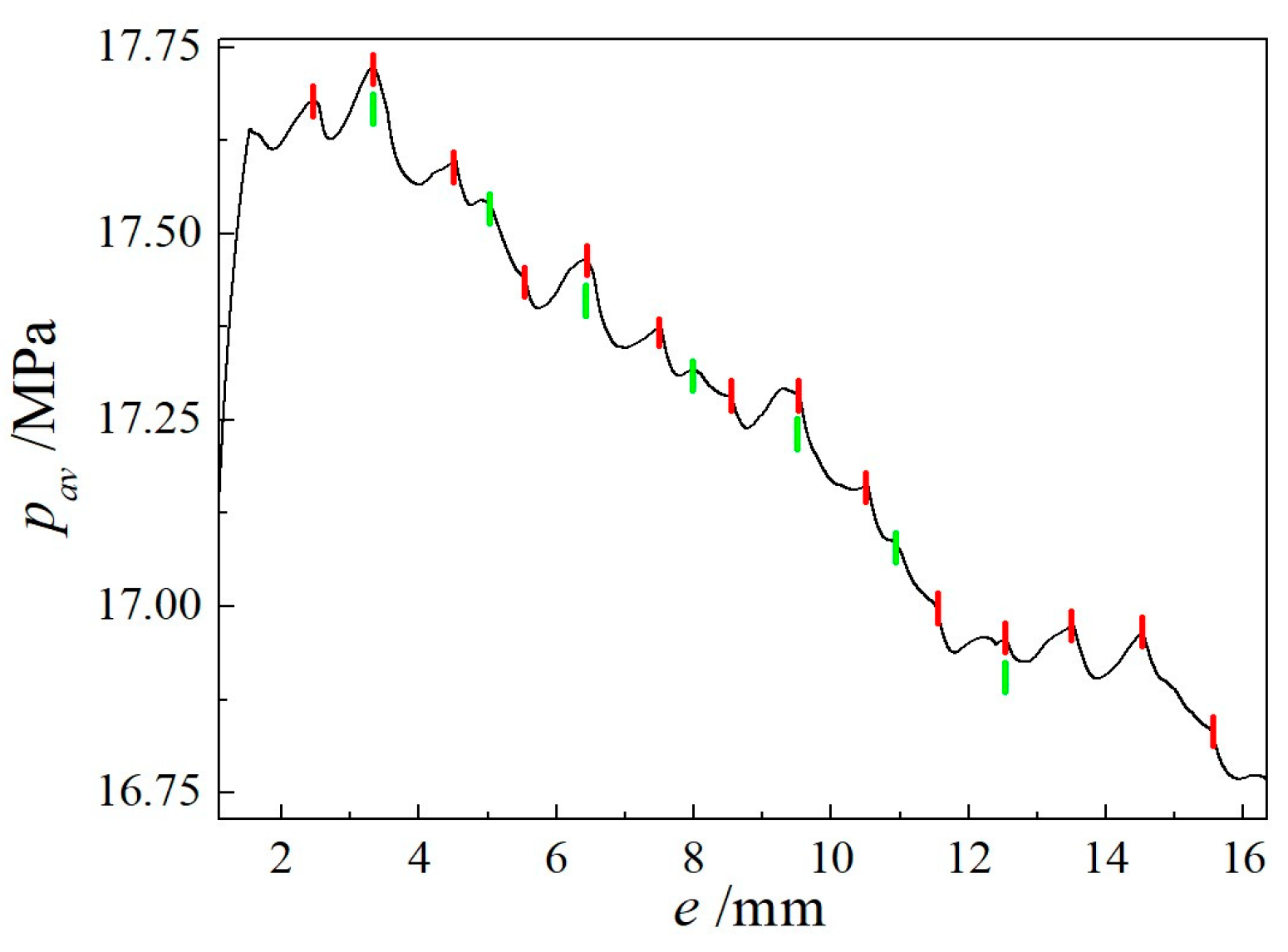
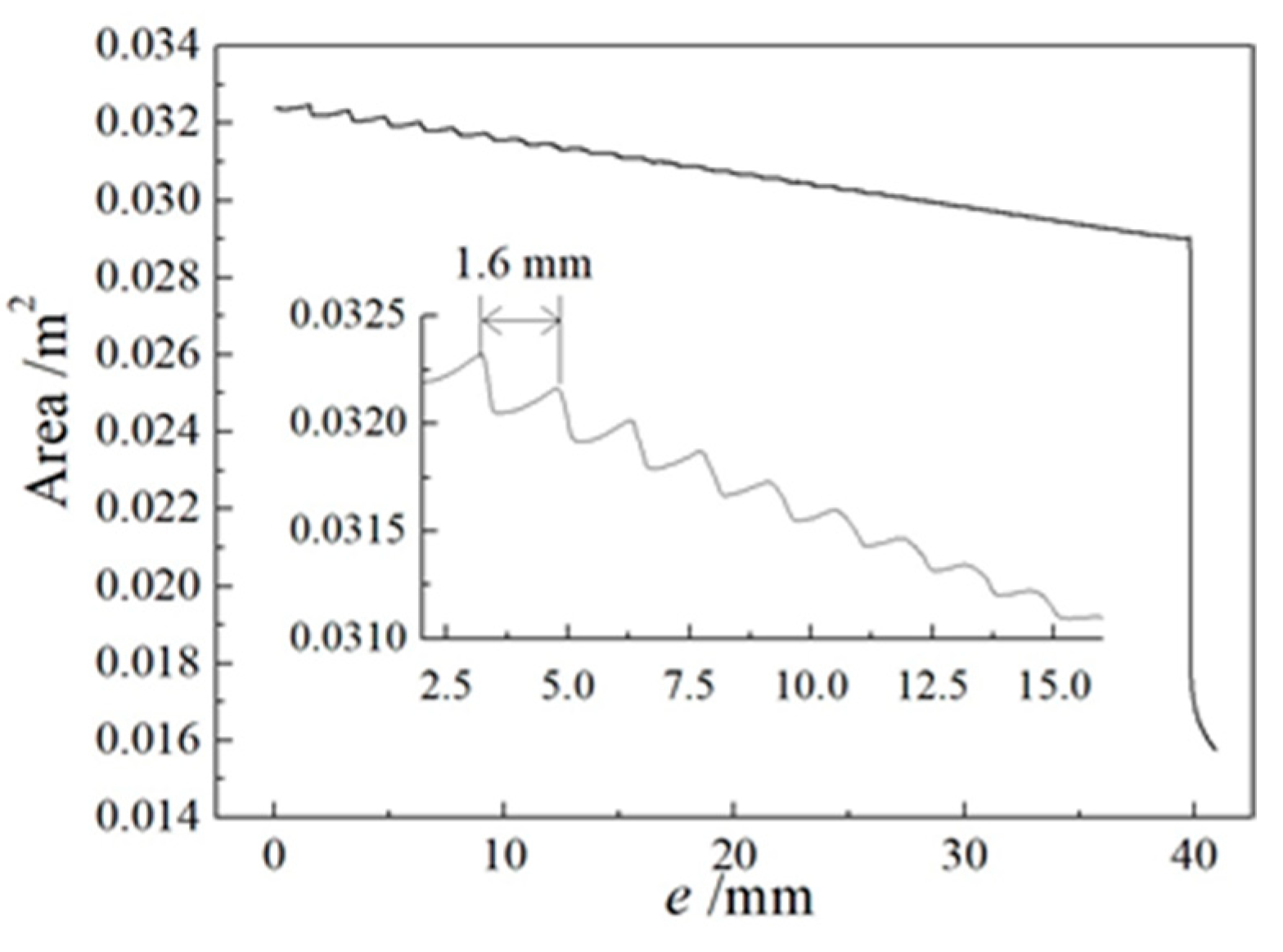
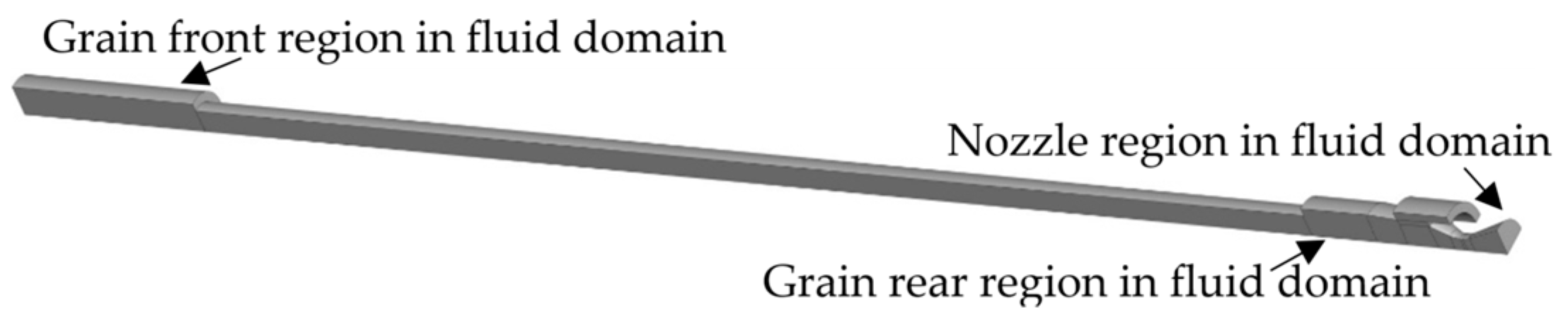
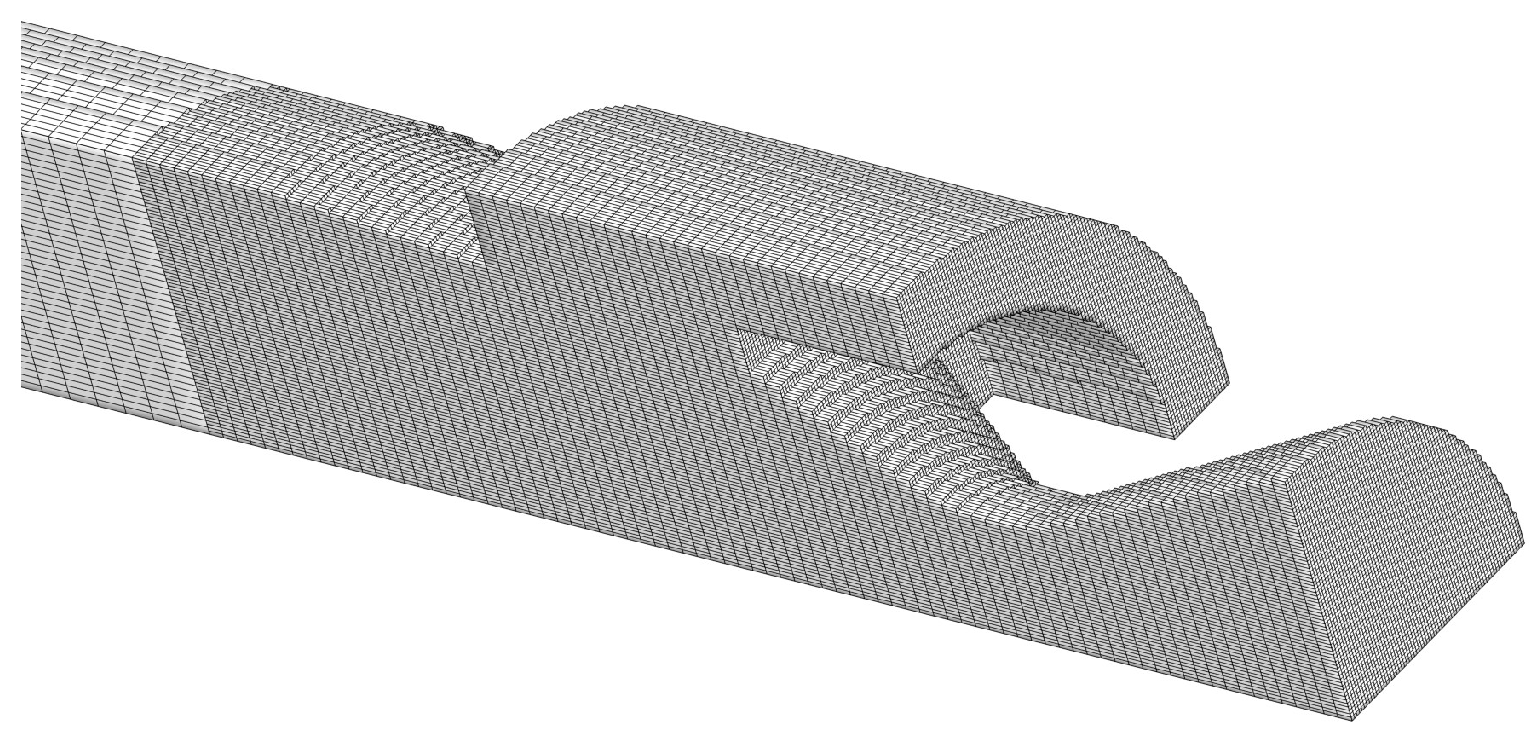

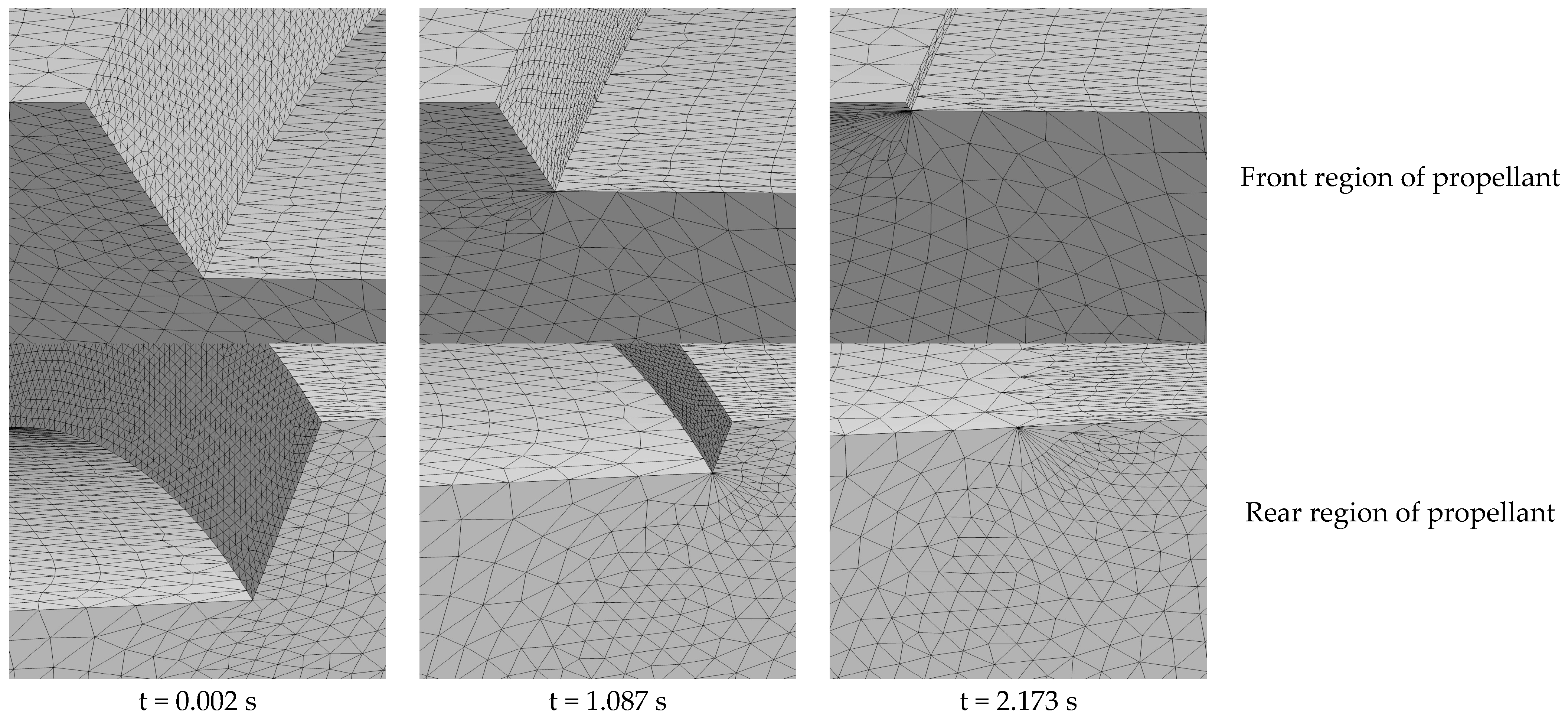
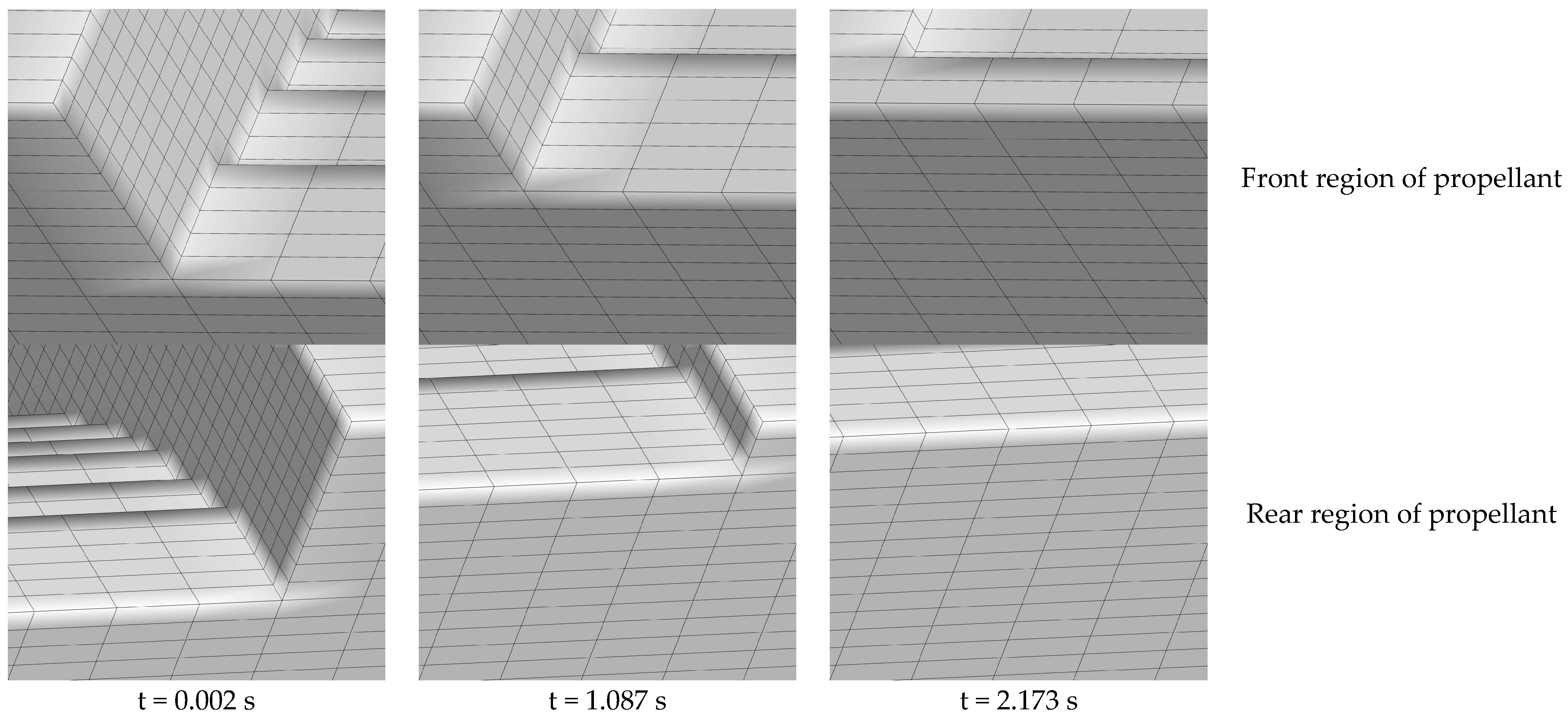
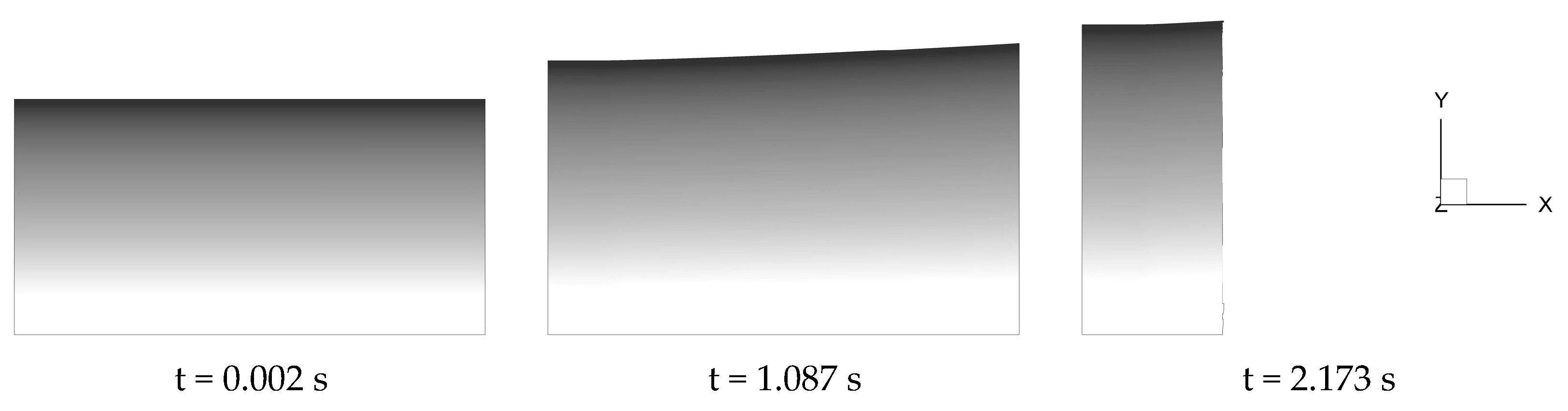
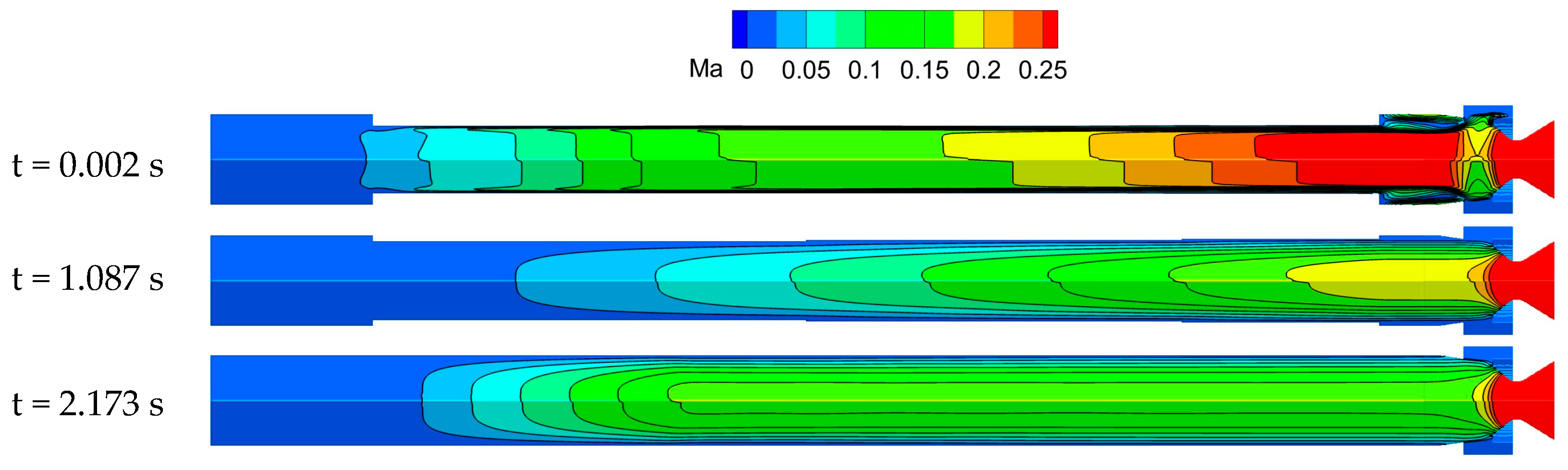
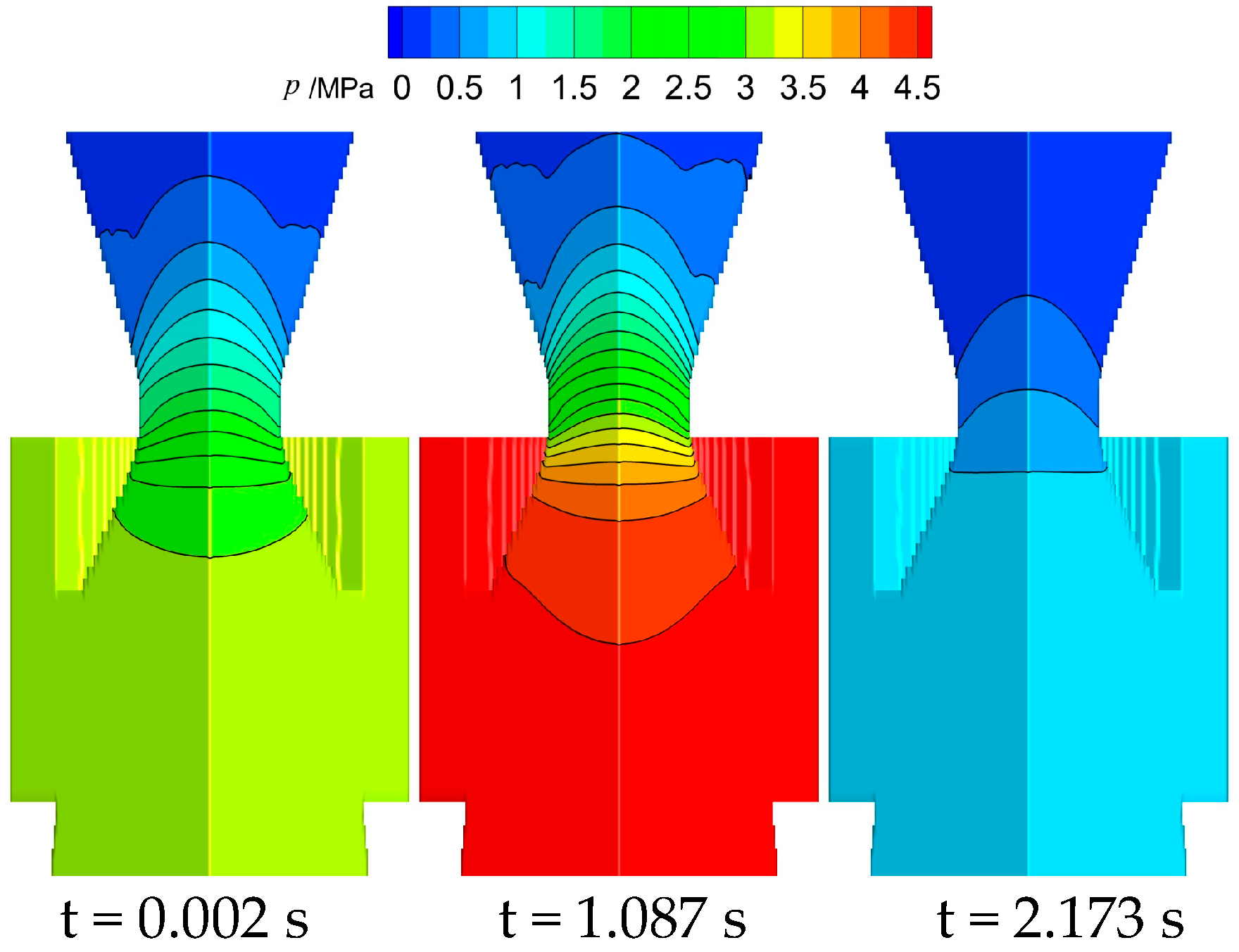
| Items | Value |
|---|---|
| Initial value | p = 4.0 MPa, ρ = 4.13 kg/m3, u = 0 m/s, v = 0 m/s |
| Propellant parameters | Cp = 1965.55 J/kg/K, T0 = 3532 K, ϒ = 1.162 ρp = 1800 kg/m3, a = 0.015 m/s/MPan, n = 0.3 |
| Grids | (MPa) | ||
|---|---|---|---|
| 2D | 3D | Difference | |
| Uniform 1 mm mesh | 17.32 | 17.88 | 3.2% |
| Uniform 0.5 mm mesh | 16.66 | 16.96 | 1.8% |
| Nozzle surface mesh fine 0.25 mm | 16.45 | 16.58 | 0.8% |
| Items | Value |
|---|---|
| Initial value condition | p = 3.0 MPa, ρ = 3.01 kg/m3, u = 0 m/s, v = 0 m/s |
| Propellant parameters | Cp = 2050.2 J/kg/K, T0 = 3041 K, ϒ = 1.19 ρp = 1700 kg/m3, a = 0.0049 m/s/MPan, n = 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, D.; Lin, Q.; Wang, H.; Tao, R. Numerical Simulation of Gas Flow Coupled with Burning Surface Regression Based on Immersed Boundary Method and Face Offsetting Method. Aerospace 2024, 11, 550. https://doi.org/10.3390/aerospace11070550
Su D, Lin Q, Wang H, Tao R. Numerical Simulation of Gas Flow Coupled with Burning Surface Regression Based on Immersed Boundary Method and Face Offsetting Method. Aerospace. 2024; 11(7):550. https://doi.org/10.3390/aerospace11070550
Chicago/Turabian StyleSu, Dongjian, Qingyu Lin, Hao Wang, and Ruyi Tao. 2024. "Numerical Simulation of Gas Flow Coupled with Burning Surface Regression Based on Immersed Boundary Method and Face Offsetting Method" Aerospace 11, no. 7: 550. https://doi.org/10.3390/aerospace11070550
APA StyleSu, D., Lin, Q., Wang, H., & Tao, R. (2024). Numerical Simulation of Gas Flow Coupled with Burning Surface Regression Based on Immersed Boundary Method and Face Offsetting Method. Aerospace, 11(7), 550. https://doi.org/10.3390/aerospace11070550






