Study on the Active Control of the Dynamic Stall of Rotor Airfoils Based on Plasma Excitation
Abstract
1. Introduction
2. Numerical Computation Methods
2.1. Flow Field Control Equations
2.2. Turbulence Model
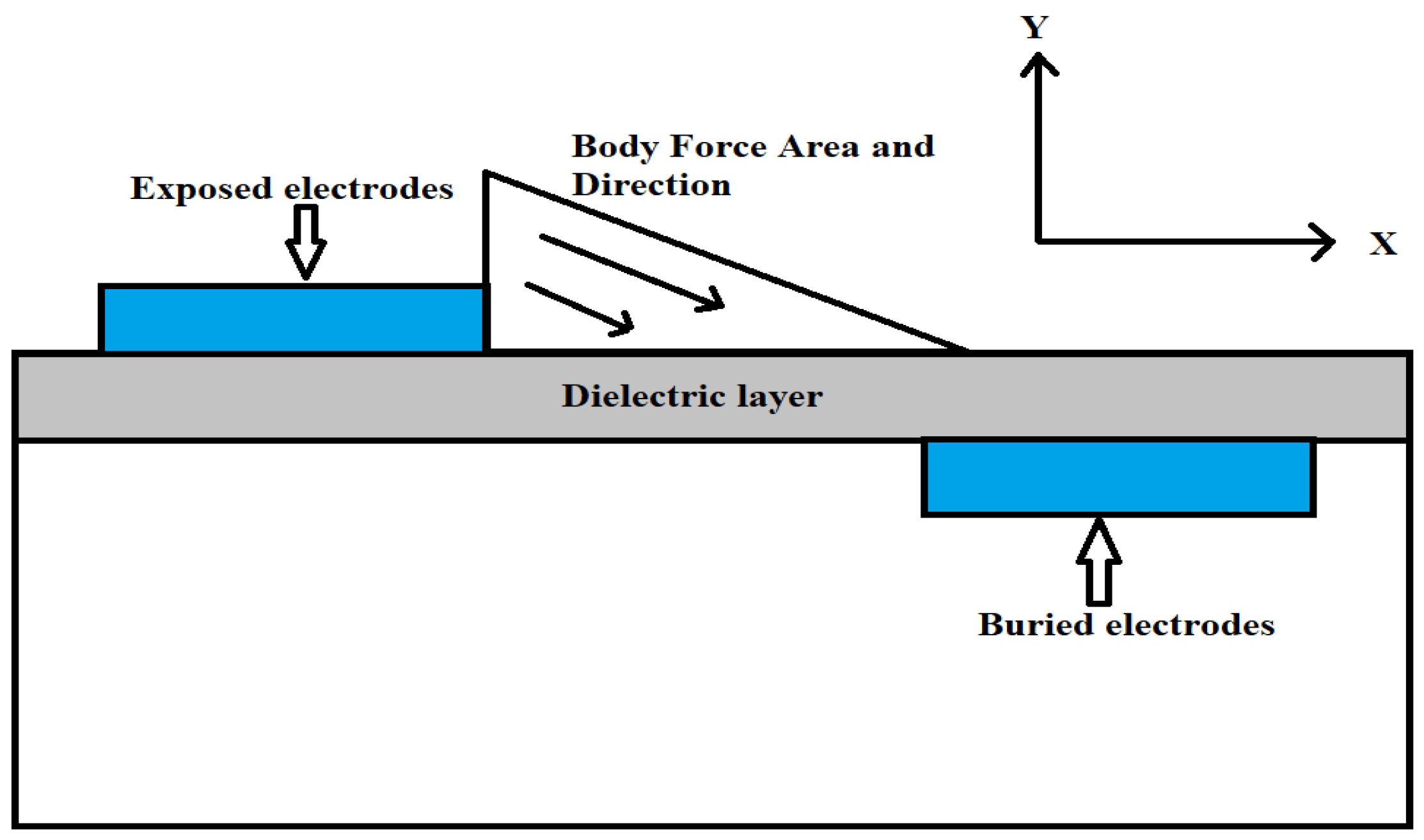
2.3. Mathematical Model of AC DBD Plasma
3. Grid Generation and Numerical Method Validation
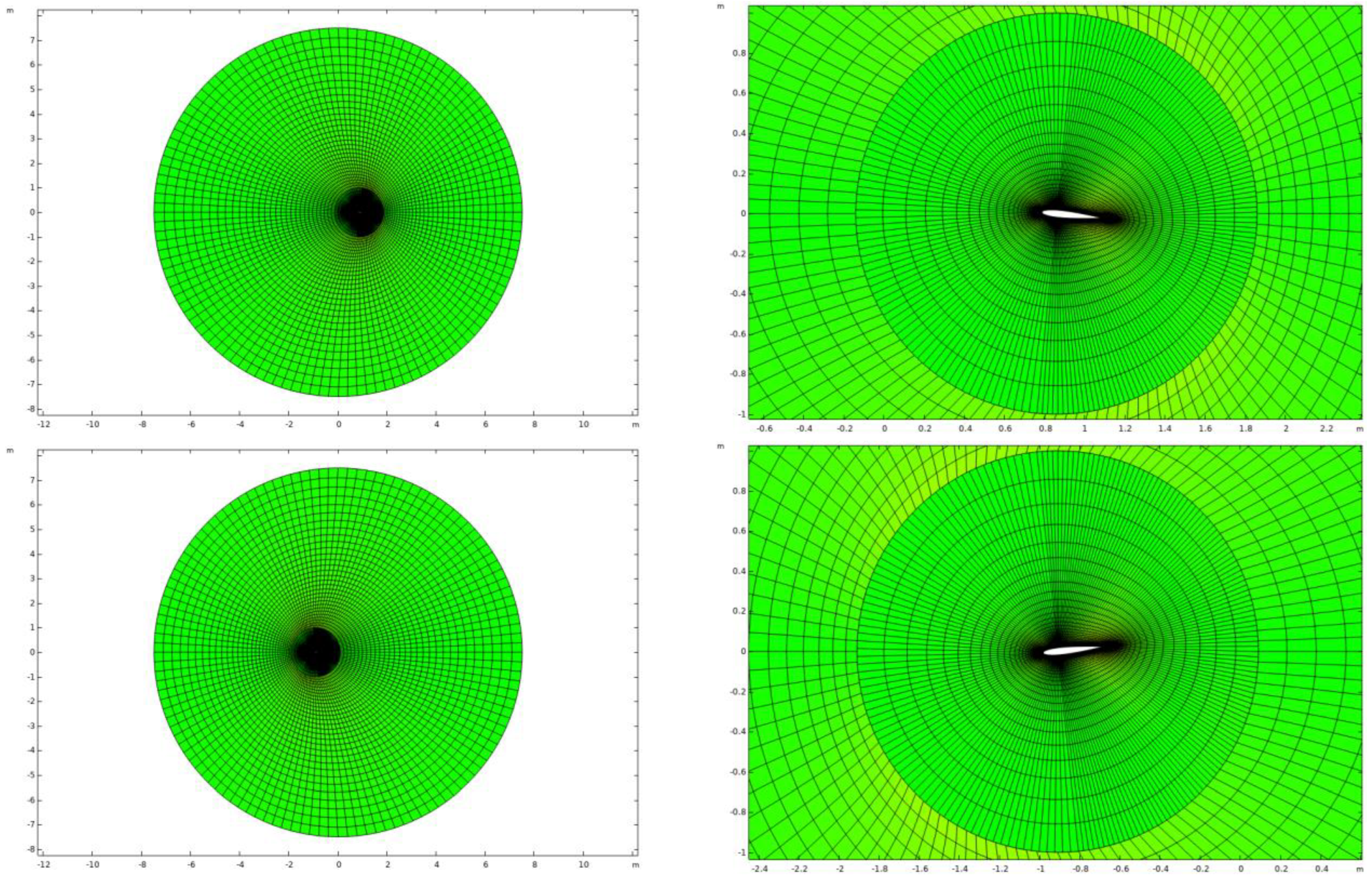
3.1. Grid Generation
3.2. Validation of the Numerical Simulation Methods
3.3. Validation of the AC DBD Plasma Mathematical Model
4. Results and Discussion
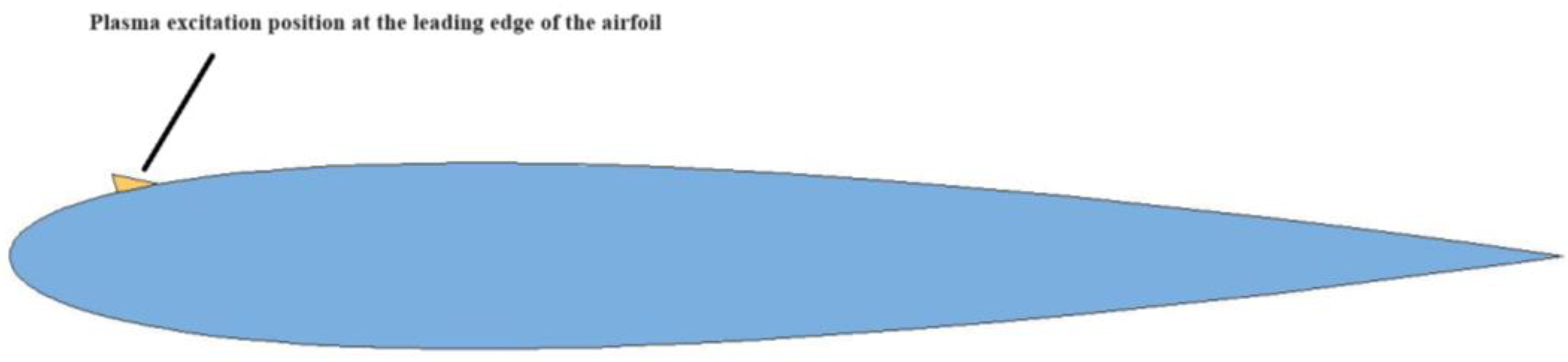
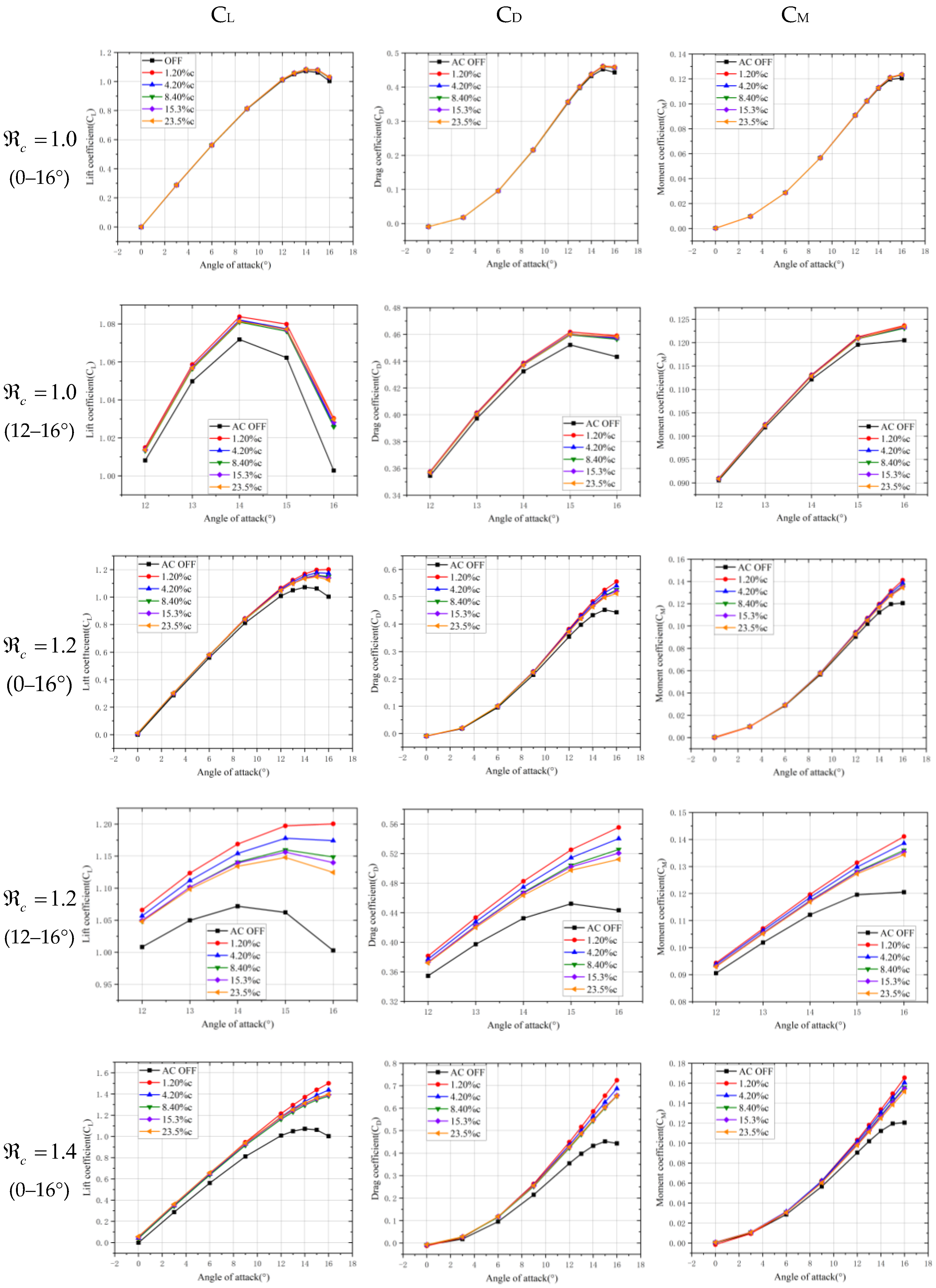
4.1. Impact of the AC DBD Plasma Excitation on the Static Stall Characteristics of Airfoils
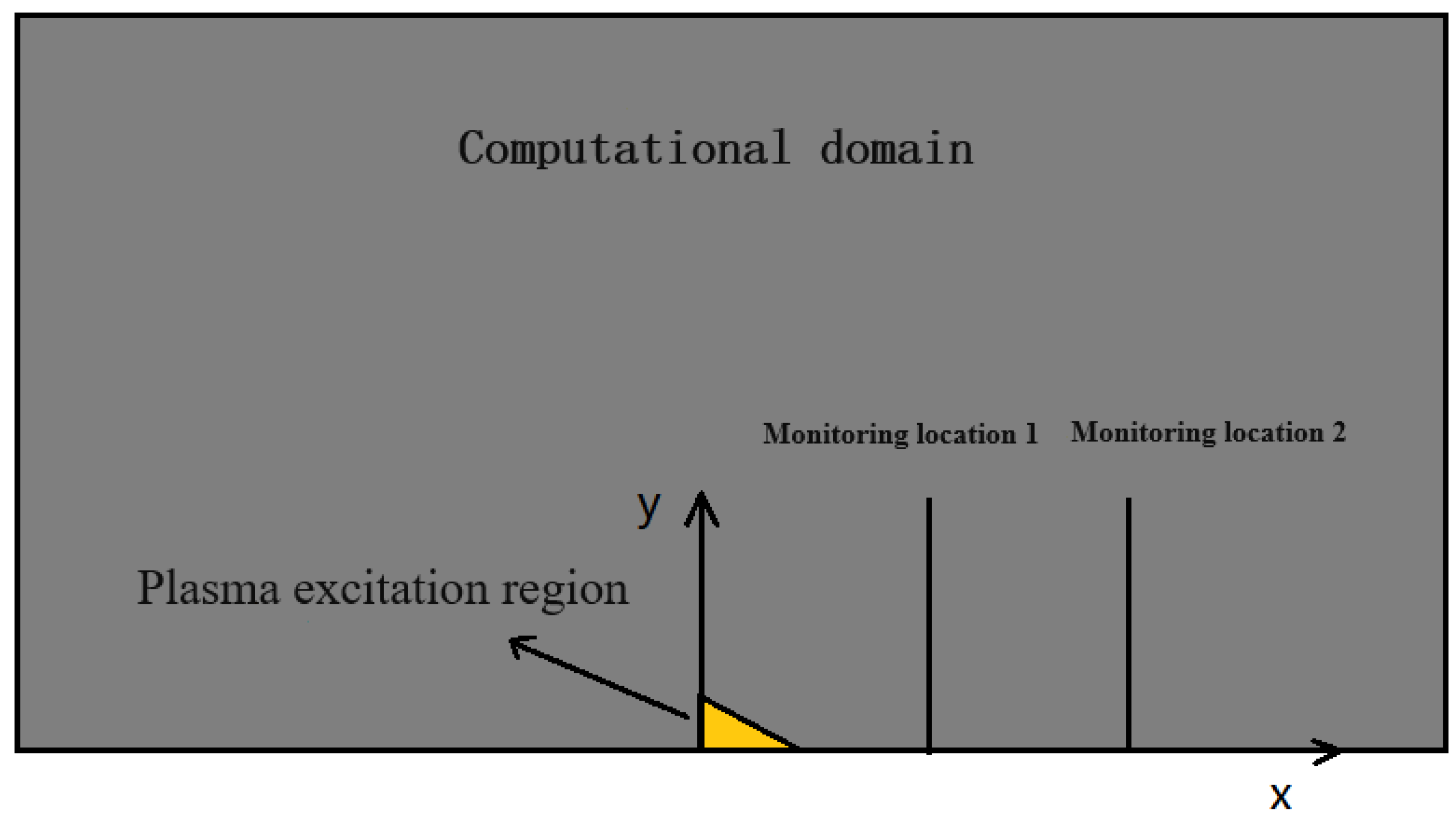
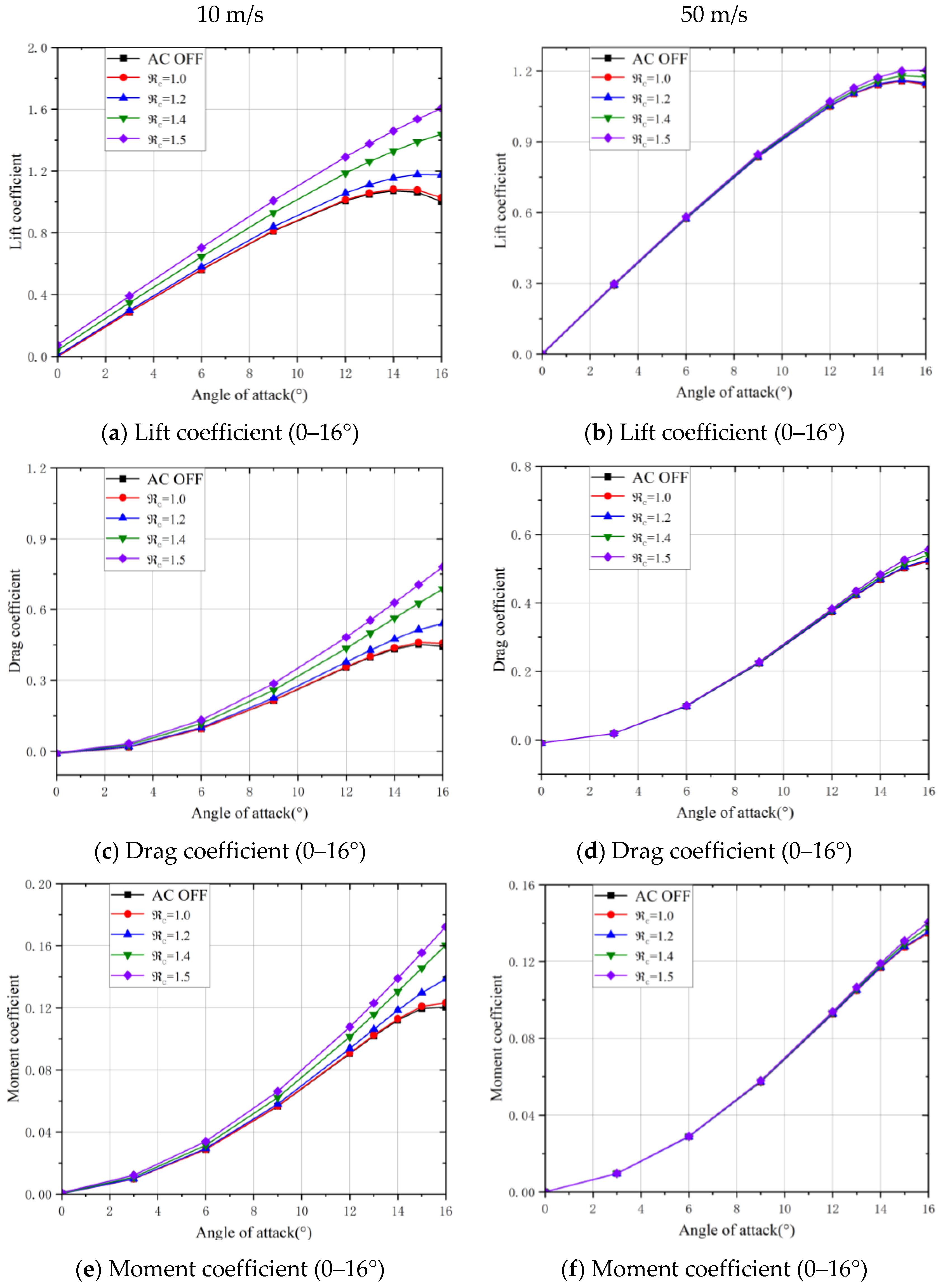
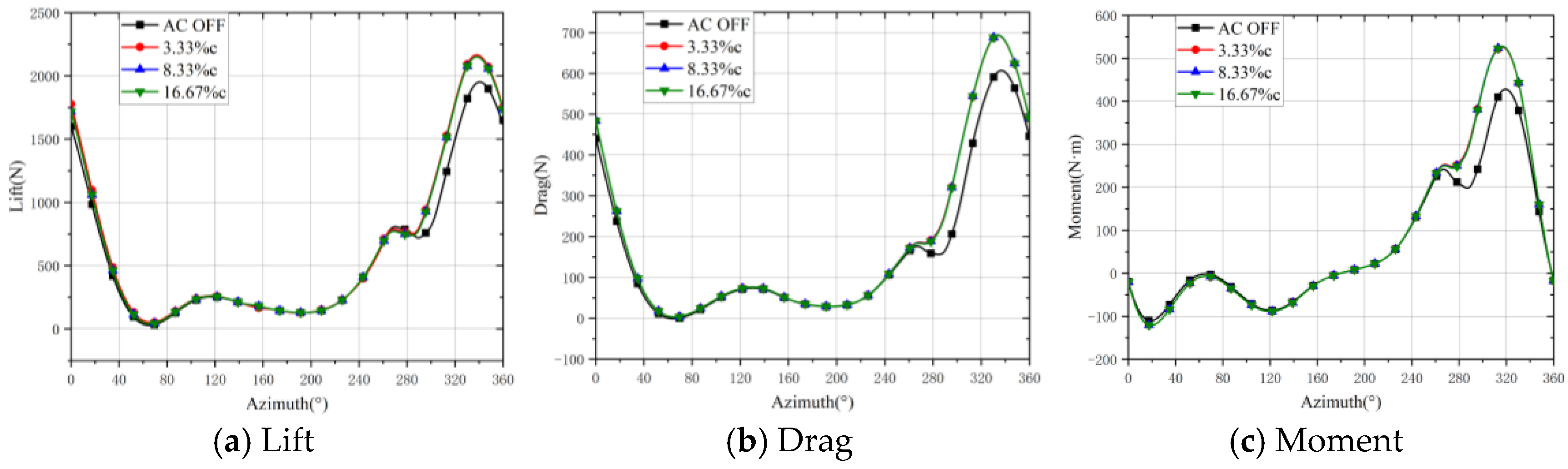
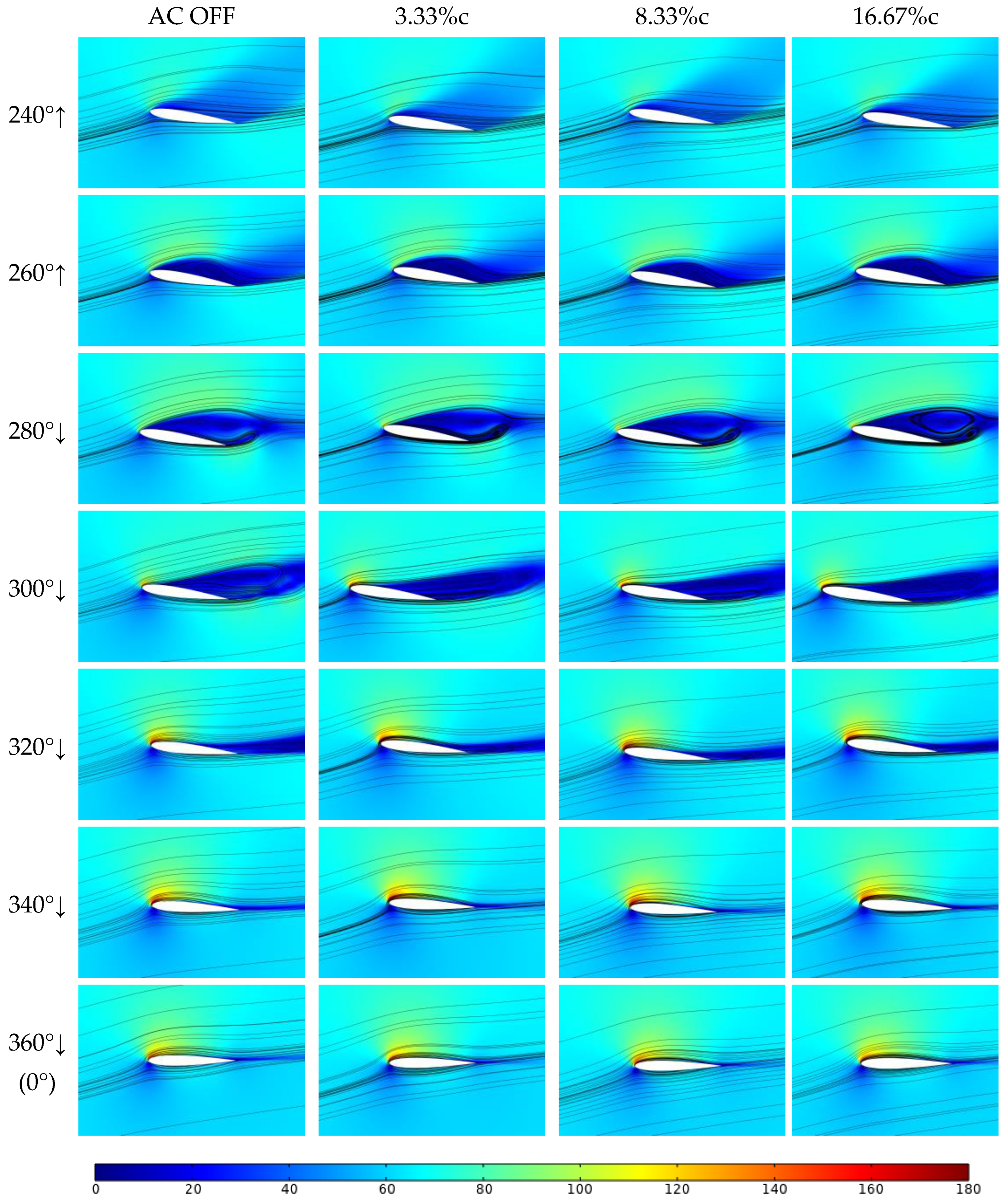
4.2. Impact of the Plasma Excitation Intensity on the Dynamic Stall Characteristics of Airfoils in Constant Incoming Velocity States
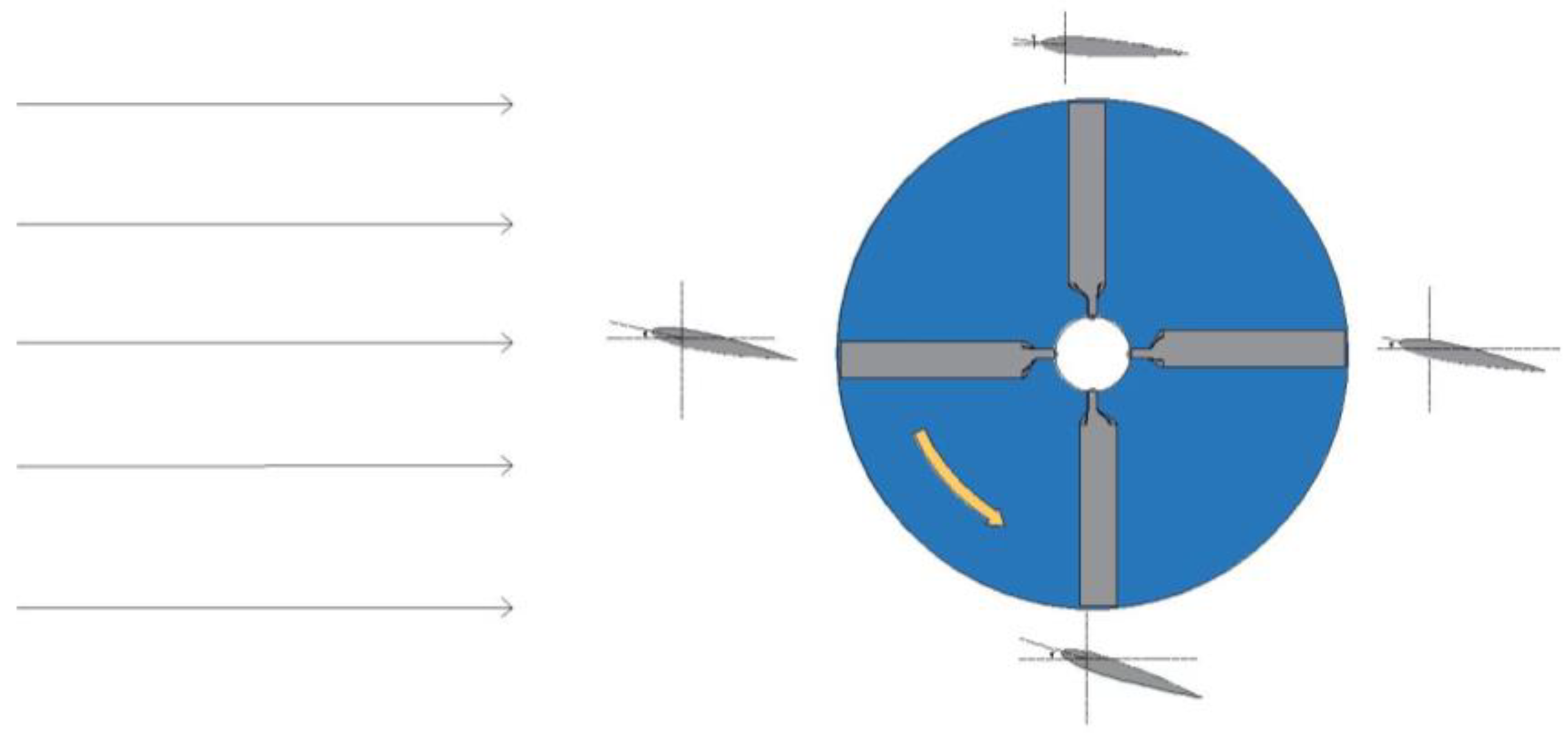
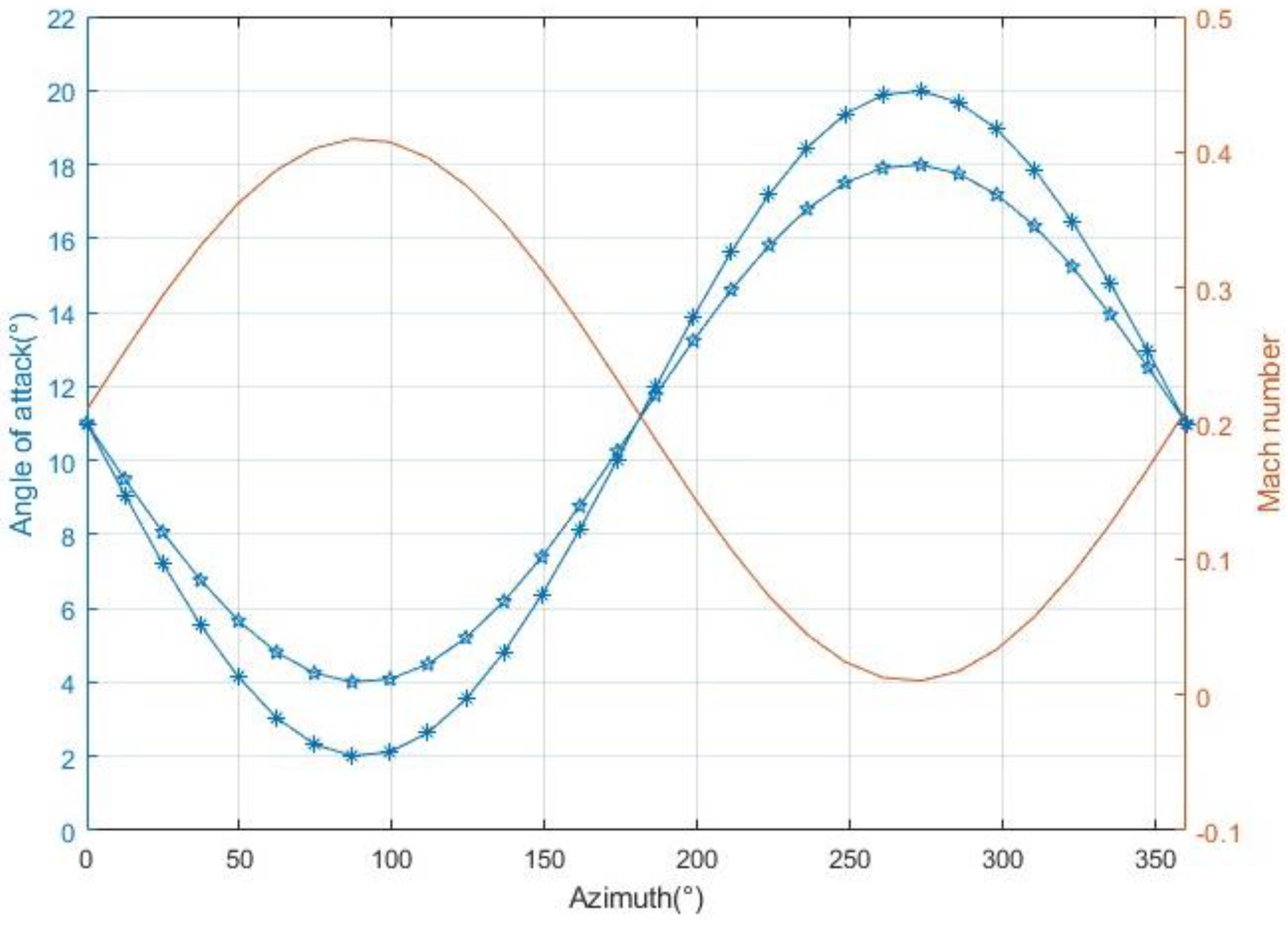
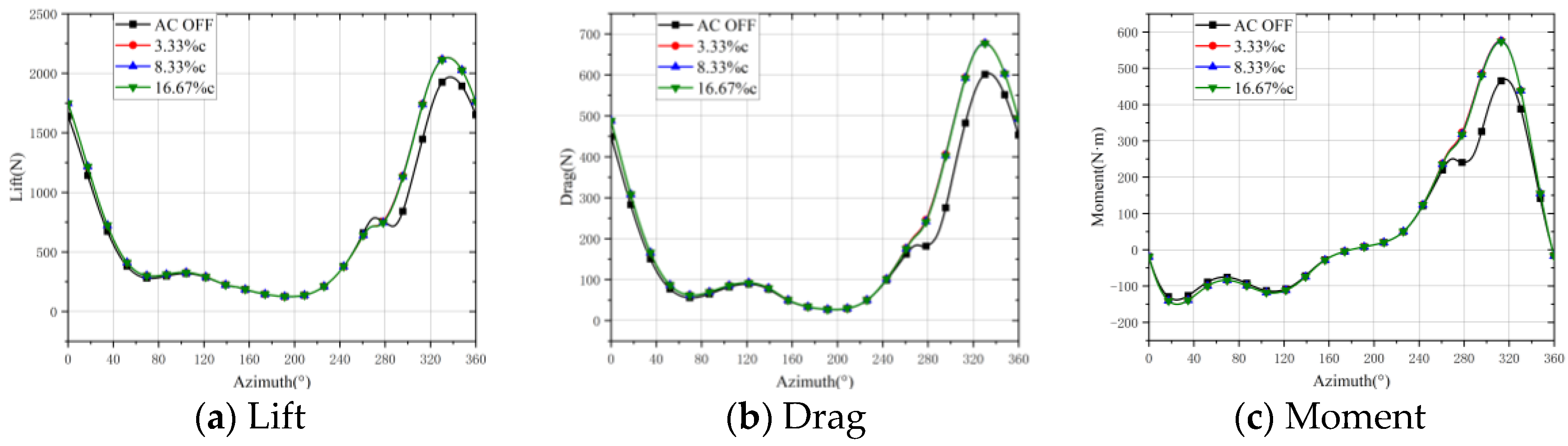
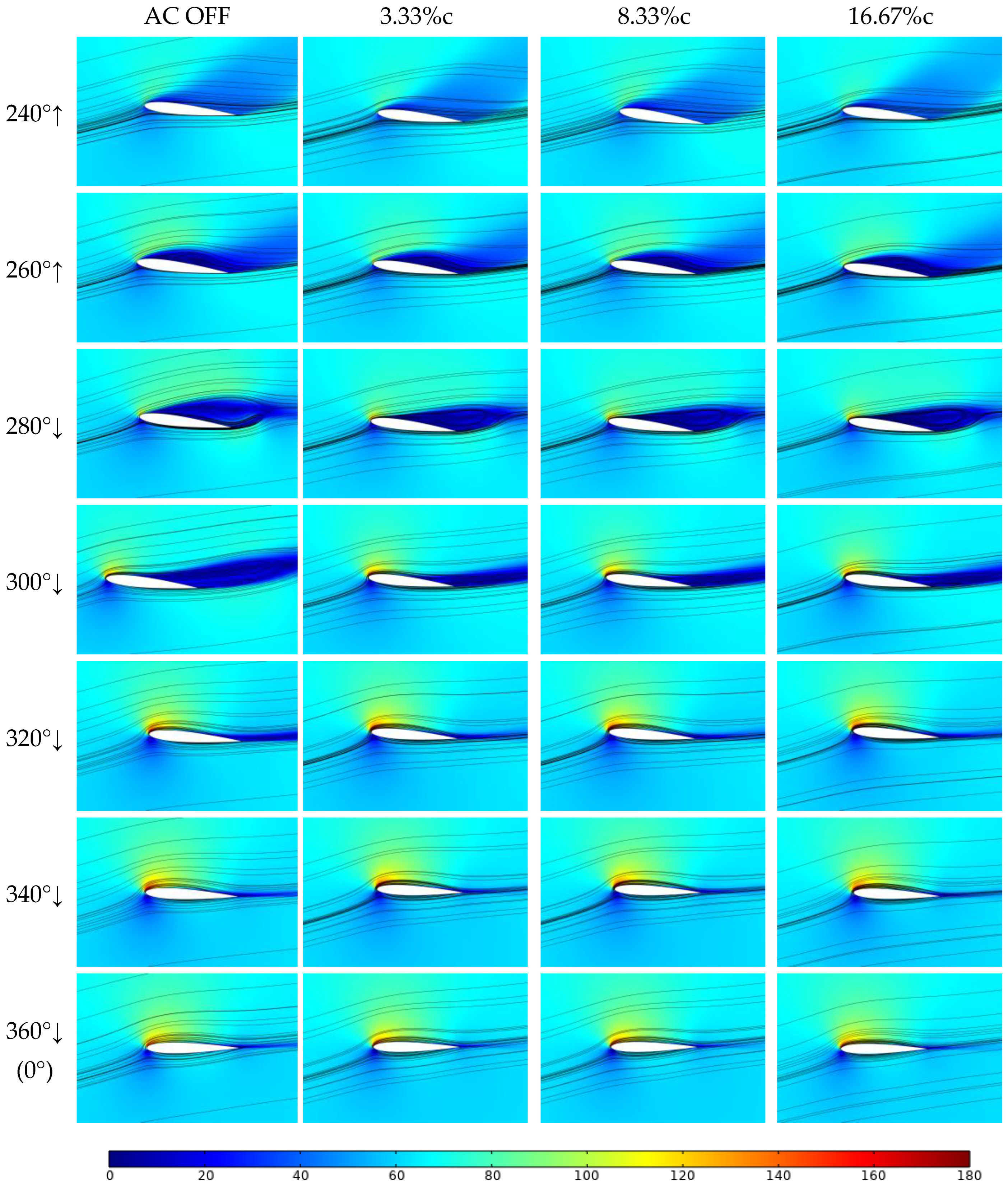
4.3. Impact of the Plasma Excitation on the Dynamic Stall Characteristics of Airfoils in a Variable Incoming Flow Condition
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gardner, A.D.; Jones, A.R.; Mulleners, K.; Naughton, J.W.; Smith, M.J. Review of rotating wing dynamic stall: Experiments and flow control. Prog. Aerosp. Sci. 2023, 137, 100887. [Google Scholar] [CrossRef]
- Wei, B.; Gao, Y.; Hu, S. Experimental and numerical study on dynamic stall under a large Reynolds number. Adv. Aerodyn. 2023, 5, 16. [Google Scholar] [CrossRef]
- Benton, S.I.; Visbal, M.R. The onset of dynamic stall at a high, transitional Reynolds number. J. Fluid Mech. 2019, 861, 860–885. [Google Scholar] [CrossRef]
- Corke, T.C.; Thomas, F.O. Dynamic stall in pitching airfoils: Aerodynamic damping and compressibility effects. Annu. Rev. Fluid Mech. 2015, 47, 479–505. [Google Scholar] [CrossRef]
- Choudhry, A.; Leknys, R.; Arjomandi, M.; Kelso, R. An insight into the dynamic stall lift characteristics. Exp. Therm. Fluid Sci. 2014, 58, 188–208. [Google Scholar] [CrossRef]
- Gupta, R.; Ansell, P.J. Unsteady Flow Physics of Airfoil Dynamic Stall. AIAA J. 2019, 57, 165–175. [Google Scholar] [CrossRef]
- Ham, N.D. Aerodynamic loading on a two-dimensional airfoil during dynamic stall. AIAA J. 1968, 6, 1927–1934. [Google Scholar] [CrossRef]
- McCroskey, W.J.; McAlister, K.W.; Carr, L.W.; Pucci, S.L. An Experimental Study of Dynamic Stall on Advanced Airfoil Sections. In Volume 1. Summary of the Experiment; NTRS: Washington, DC, USA, 1982. [Google Scholar]
- Mcalister, K.W.; Pucci, S.L.; McCroskey, W.J.; Carr, L.W. An Experimental Study of Dynamic Stall on Advanced Airfoil Sections. In Volume 2. Pressure and Force Data; NASA TM-84245; NTRS: Washington, DC, USA, 1982. [Google Scholar]
- Kaufmann, K.; Merz, C.B.; Gardner, A.D. Dynamic Stall Simulations on a Pitching Finite Wing. J. Aircr. 2017, 54, 1303–1316. [Google Scholar] [CrossRef]
- Gompertz, K.; Jensen, C.D.; Gregory, J.W.; Bons, J.P. Compressible Dynamic Stall Mechanisms Due to Airfoil Pitching and Freestream Mach Oscillations; The Vertical Flight Society: Fairfax, WV, USA, 2012. [Google Scholar]
- Hird, K.; Frankhouser, M.W.; Gregory, J.W.; Bons, J.P. Compressible Dynamic Stall of an SSC-A09 Airfoil Subjected to Coupled Pitch and Freestream Mach Oscillations; The Vertical Flight Society: Fairfax, WV, USA, 2014. [Google Scholar]
- Hird, K.; Frankhouser, M.W.; Naigle, S.; Gregory, J.W.; Bons, J.P. Study of an SSC-A09 Airfoil in Compressible Dynamic Stall with Freestream Mach Oscillations; The Vertical Flight Society: Fairfax, WV, USA, 2015. [Google Scholar]
- Benton, S.I.; Visbal, M.R. Extending the Reynolds number range of high frequency control of dynamic stall. AIAA J. 2019, 57, 2675–2681. [Google Scholar] [CrossRef]
- Zha, G.C.; Carroll, B.F.; Paxton, C.D.; Conley, C.A.; Wells, A. High-Performance Airfoil Using Coflow Jet Flow Control. AIAA J. 2005, 45, 2087–2090. [Google Scholar] [CrossRef]
- Patel, M.P.; Ng, T.T.; Vasudevan, S.; Corke, T.C.; He, C. Plasma Actuators for Hingeless Aerodynamic Control of an Unmanned Air Vehicle. J. Aircr. 2007, 44, 1264–1274. [Google Scholar] [CrossRef]
- Post, M.L.; Corke, T.C. Separation control using plasma actuators: Dynamic stall vortex control on oscillating airfoil. AIAA J. 2006, 44, 3125–3135. [Google Scholar] [CrossRef]
- Mitsuo, K.; Watanabe, S.; Atobe, T.; Kato, H.; Tanaka, M.; Uchida, T. Lift Enhancement of a Pitching Airfoil in Dynamic Stall by DBD Plasma Actuators; AIAA-2013–1119; AIAA: Reston, VA, USA, 2013. [Google Scholar]
- Li, G.Q.; Zhang, W.G.; Jiang, Y.B.; Pengyu, Y. Experimental investigation of dynamic stall flow control for wind turbine airfoils using a plasma actuator. Energy 2019, 185, 90–101. [Google Scholar]
- Sha, J.; Shi, Z.-W.; Chen, Z.; Yao, Z.-Y. Dynamic Stall Control of NACA0012 Airfoil Using AC-DBD Plasma Actuators. Phys. Gases 2021, 6, 50–61. [Google Scholar] [CrossRef]
- Shyy, W.; Jayaraman, B.; Andersson, A. Modeling of glow discharge-induced fluid dynamic. J. Appl. Phys. 2002, 92, 6343. [Google Scholar] [CrossRef]
- Debiasi, M.; Li, J.M. Experimental study of a DBD-plasma driven channel flow. In Proceedings of the 49th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2011. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, W.; Guo, K.; Li, Y. Study on the Active Control of the Dynamic Stall of Rotor Airfoils Based on Plasma Excitation. Aerospace 2024, 11, 474. https://doi.org/10.3390/aerospace11060474
Kong W, Guo K, Li Y. Study on the Active Control of the Dynamic Stall of Rotor Airfoils Based on Plasma Excitation. Aerospace. 2024; 11(6):474. https://doi.org/10.3390/aerospace11060474
Chicago/Turabian StyleKong, Weihong, Keyi Guo, and You Li. 2024. "Study on the Active Control of the Dynamic Stall of Rotor Airfoils Based on Plasma Excitation" Aerospace 11, no. 6: 474. https://doi.org/10.3390/aerospace11060474
APA StyleKong, W., Guo, K., & Li, Y. (2024). Study on the Active Control of the Dynamic Stall of Rotor Airfoils Based on Plasma Excitation. Aerospace, 11(6), 474. https://doi.org/10.3390/aerospace11060474




