Implementation and Verification of a Micro-Jet-Vane System of a Solid Rocket Motor for a Micro-Nano Satellite
Abstract
1. Introduction
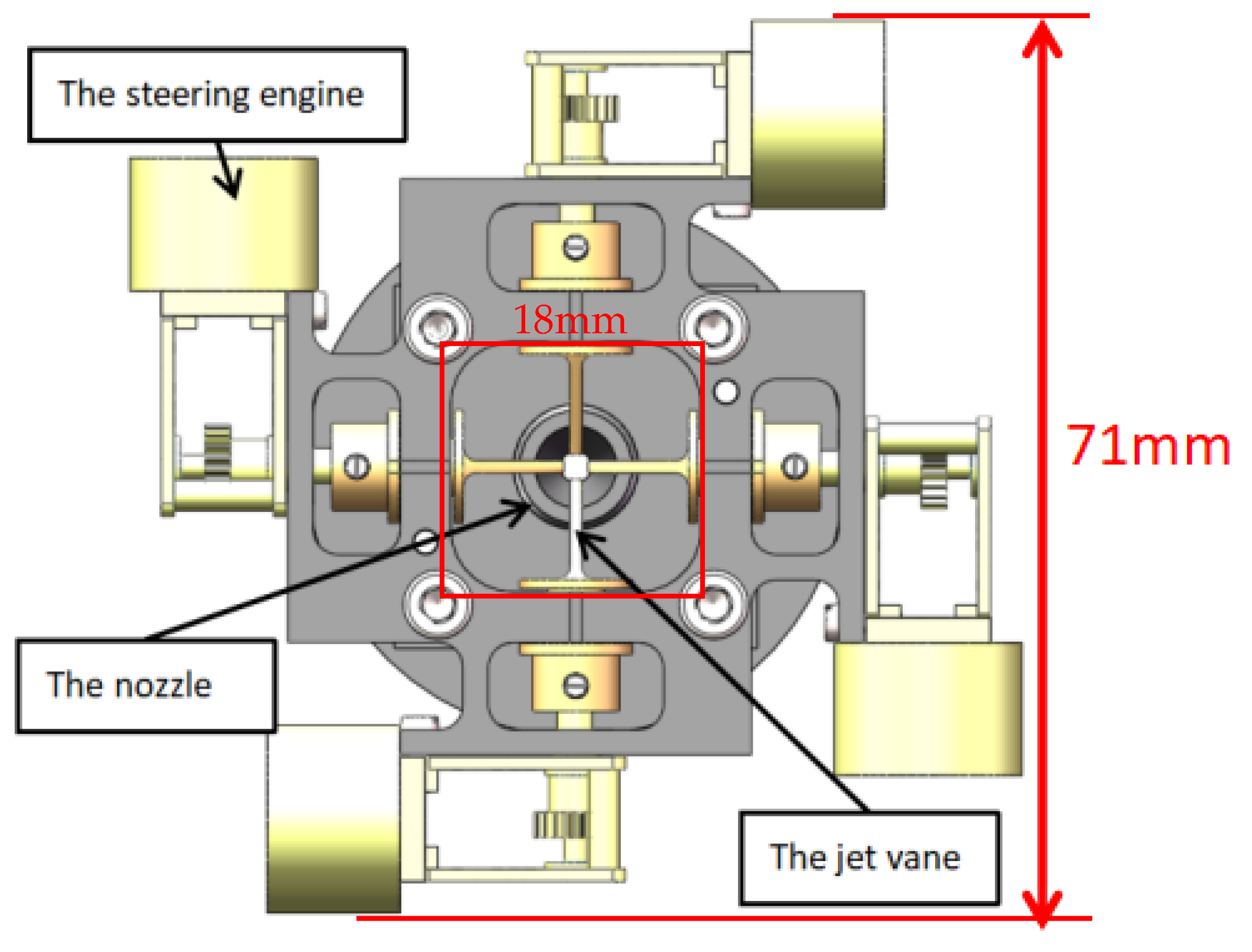
2. Structure Design of Jet Vanes
2.1. Parameters of the Solid Rocket Motor
2.2. Theoretical Calculation of the Jet Vane
2.3. Design of the Jet Vane
3. Numerical Simulation of Jet Vanes Using CFD
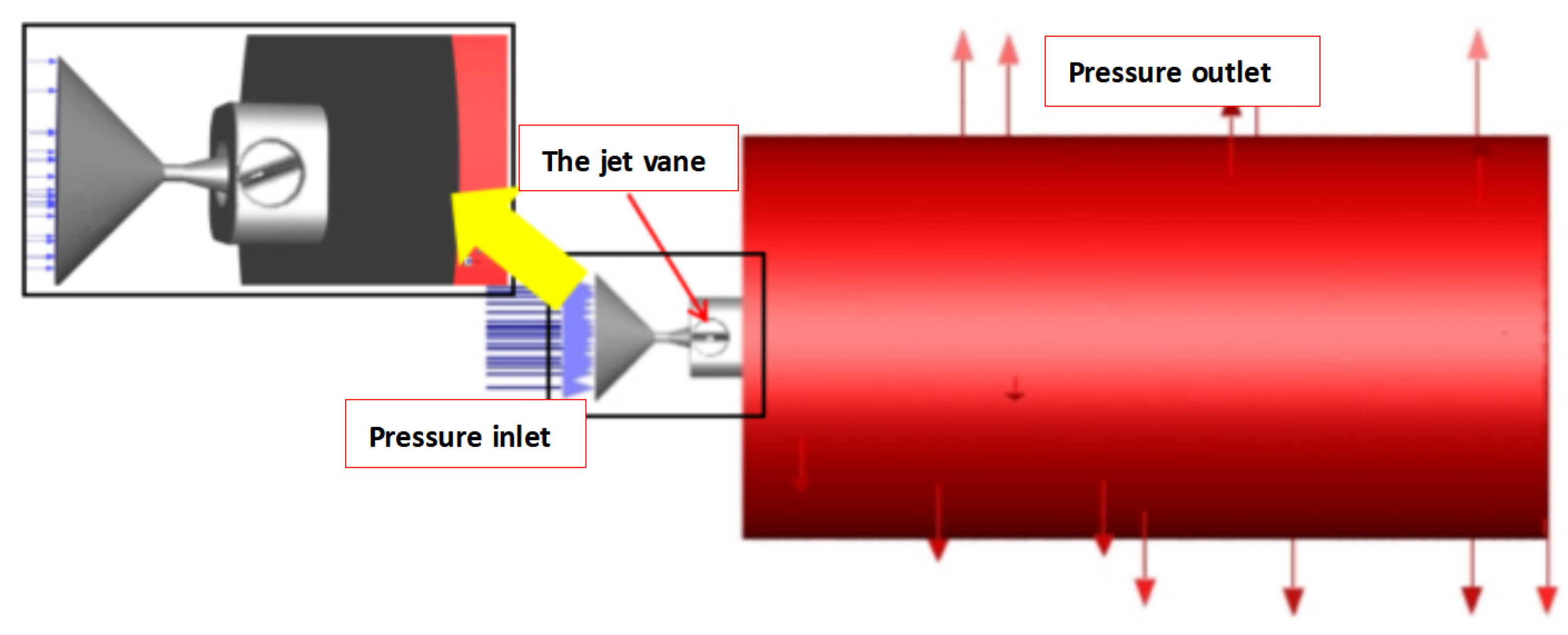
3.1. Physical Model
3.2. Mathematical Models
3.3. Simulation Conditions for Motor Jet-Ablation Test
- (A)
- Inlet: The pressure-inlet boundary is adopted at the Inlet boundary. The total pressure is set to 8 MPa and the total temperature is set to 1380 K.
- (B)
- Wall: The adiabatic solid wall boundary is adopted without considering the exchange of energy between the fluid domain and the solid wall.
- (C)
- Outlet: Because the tests are carried out in an atmospheric environment, the outlet boundary is set as the pressure-outlet boundary, with a pressure of 101,325 Pa and an ambient temperature of 323 K.
3.4. Grid Independence Verification
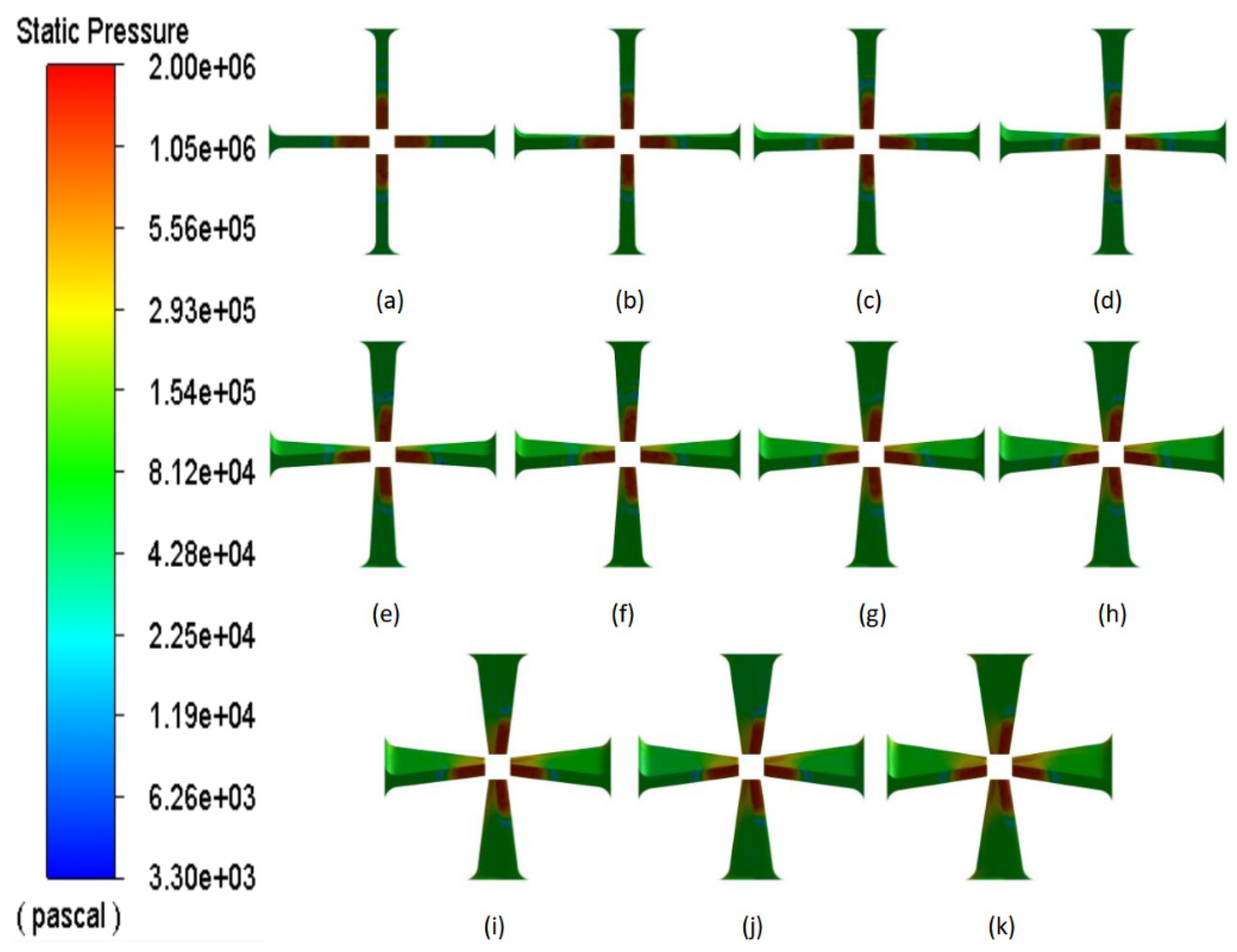
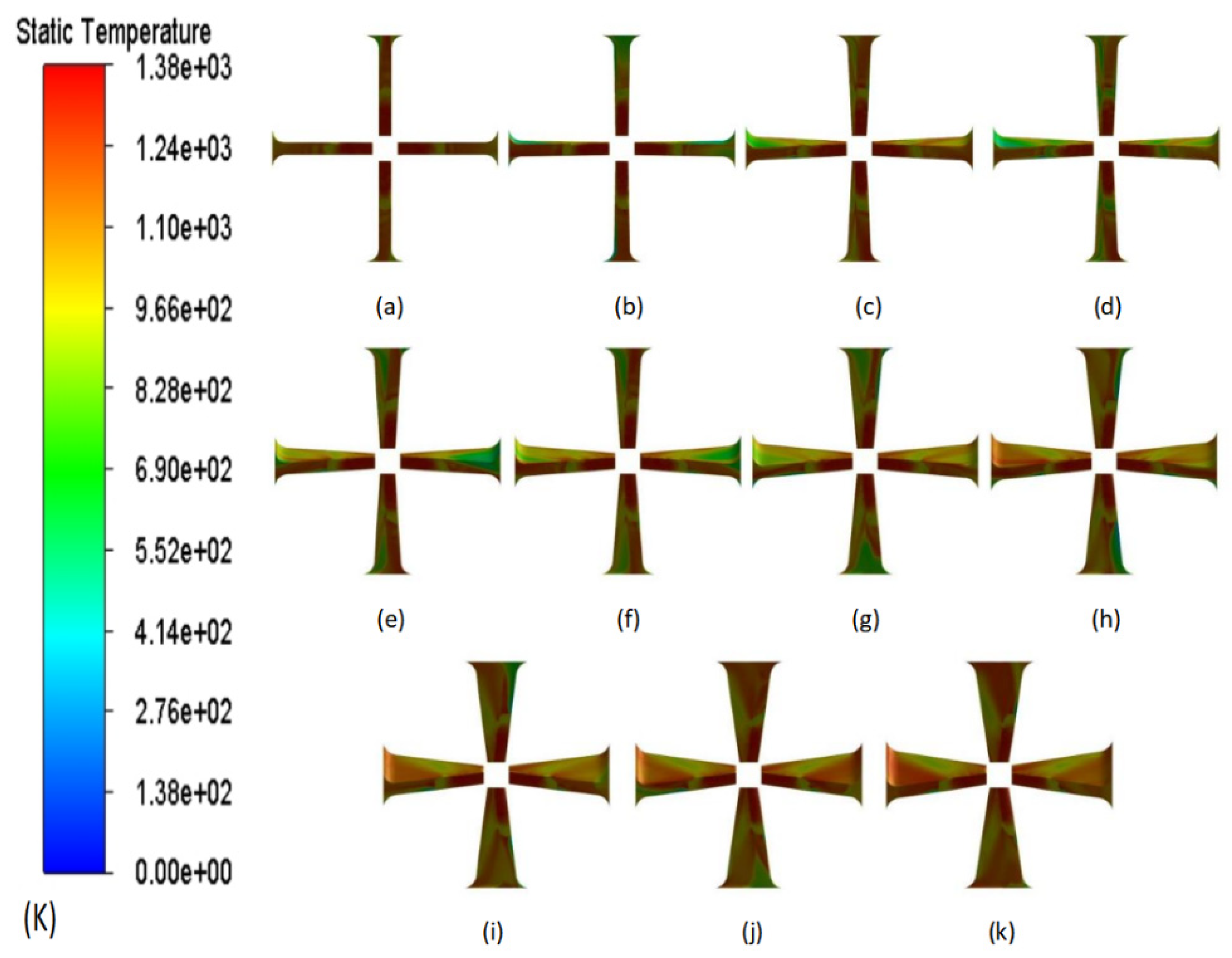
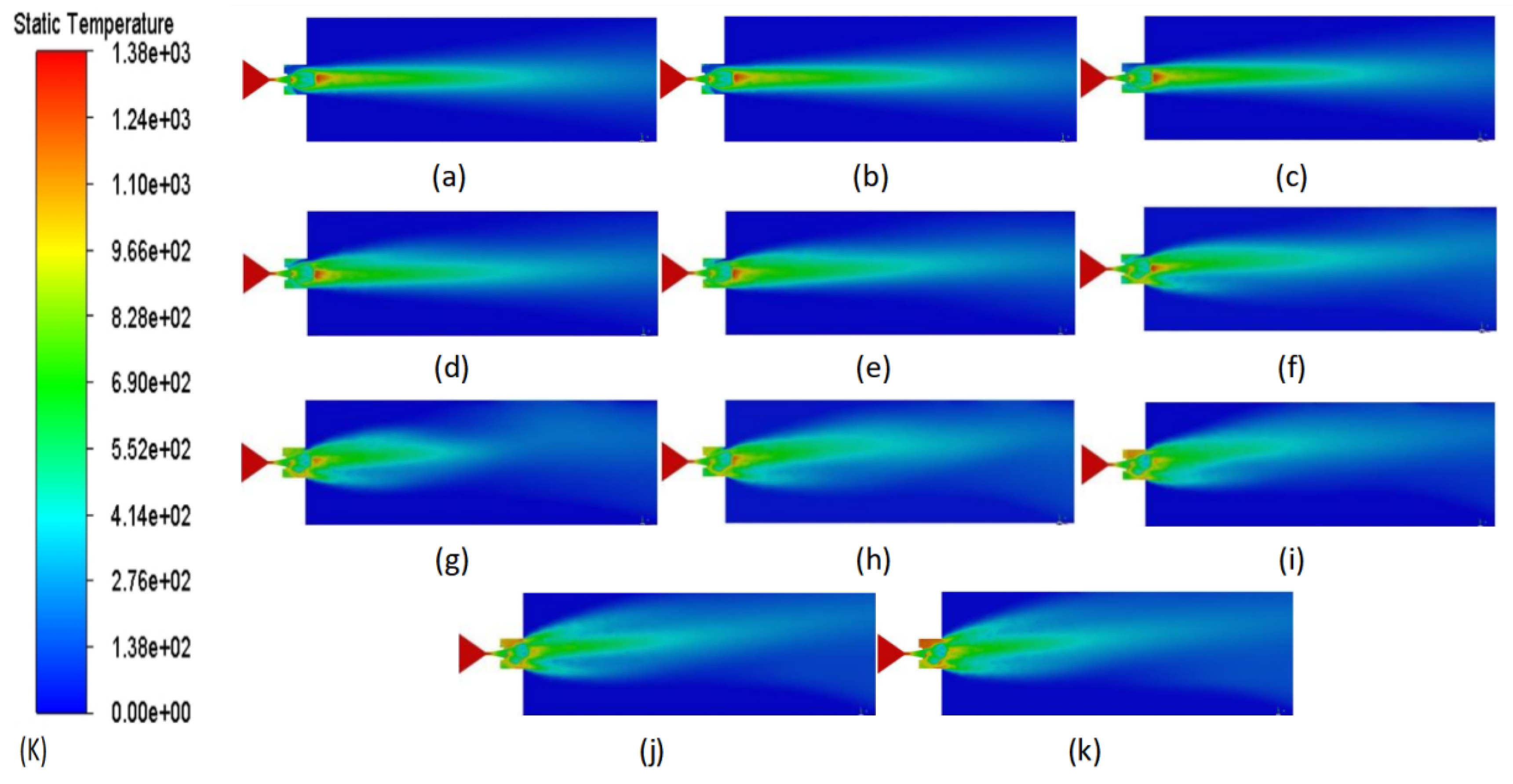
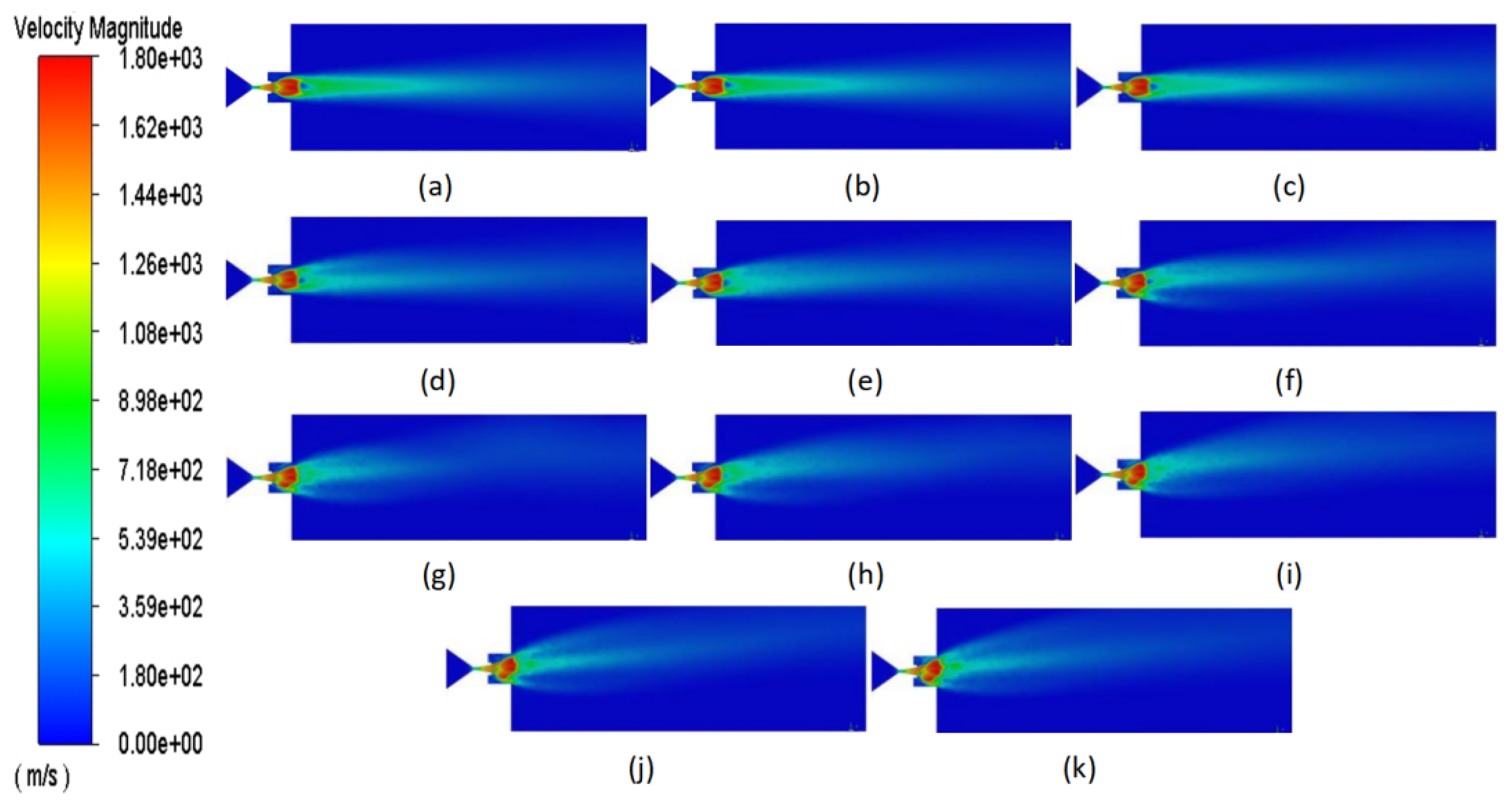
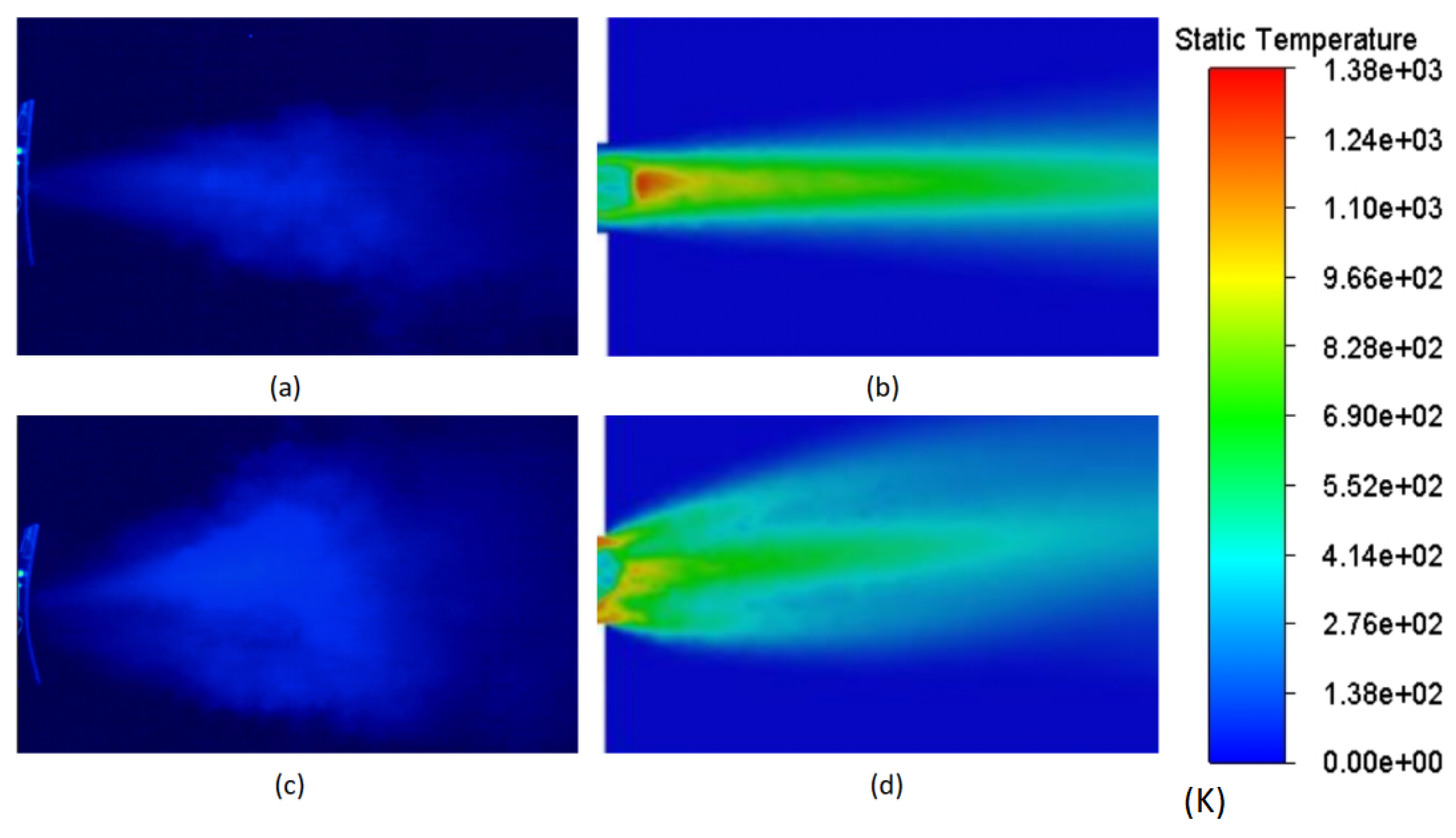
3.5. Simulation Results and Analysis
4. Jet Vane Ablation Test for Motor Jets
4.1. Initial Material Selection for Jet Vanes
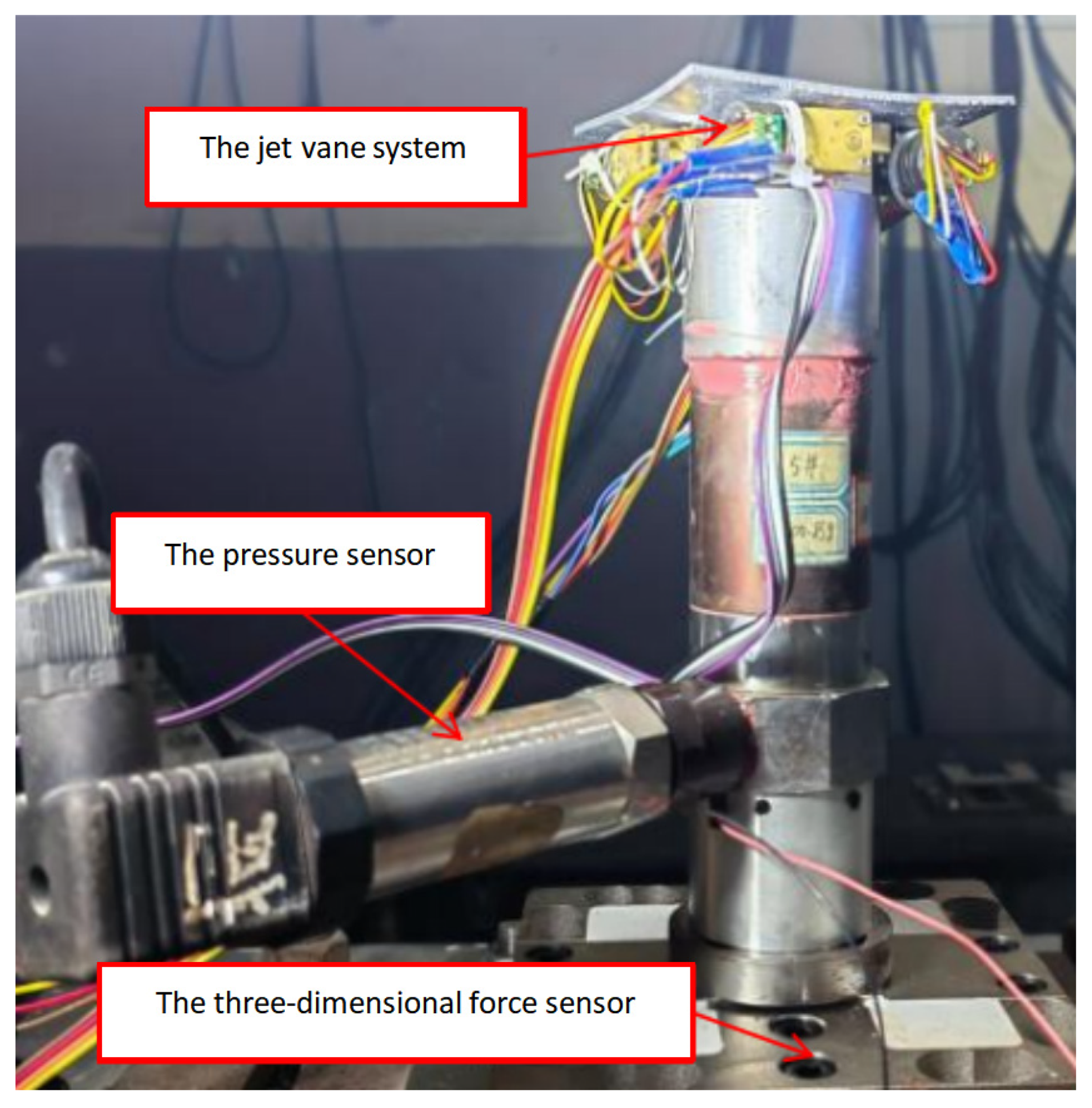
4.2. Introduction of the Test Equipment
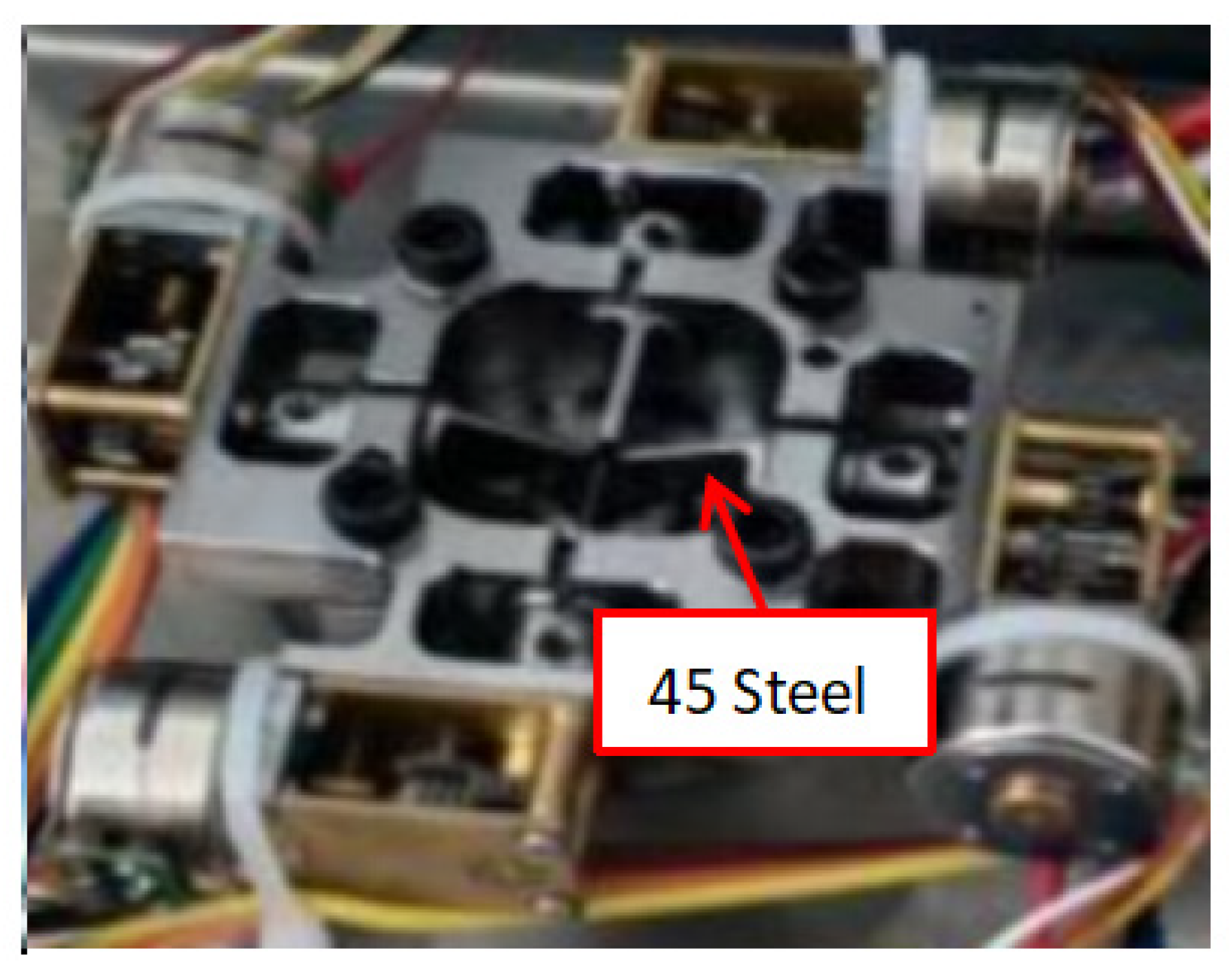
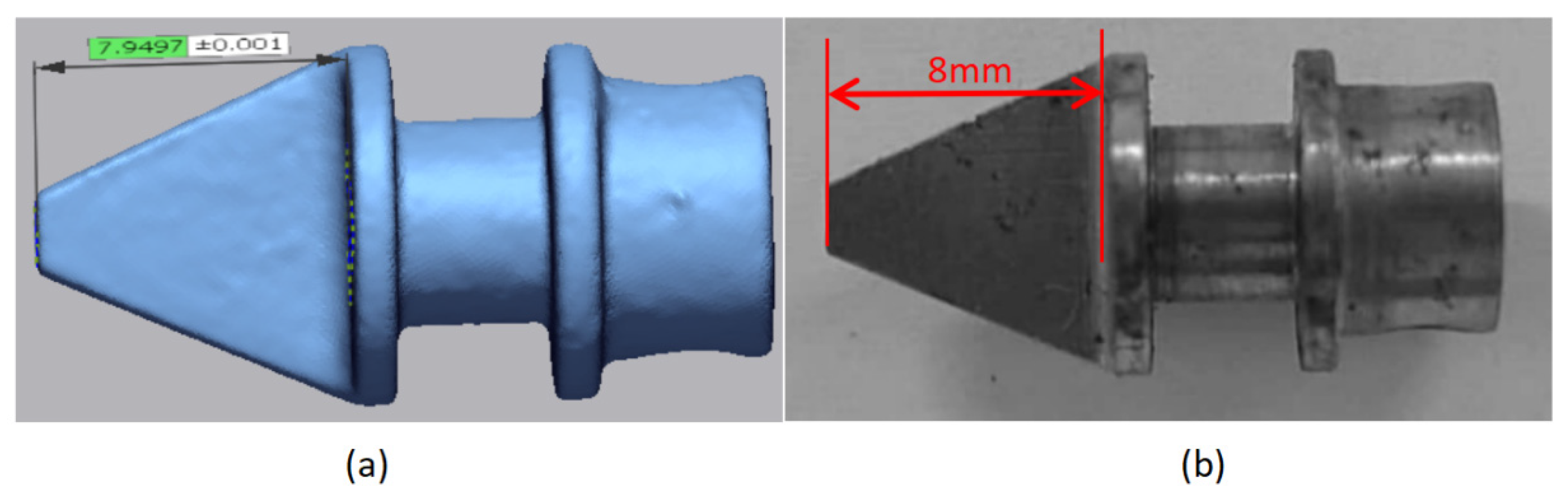
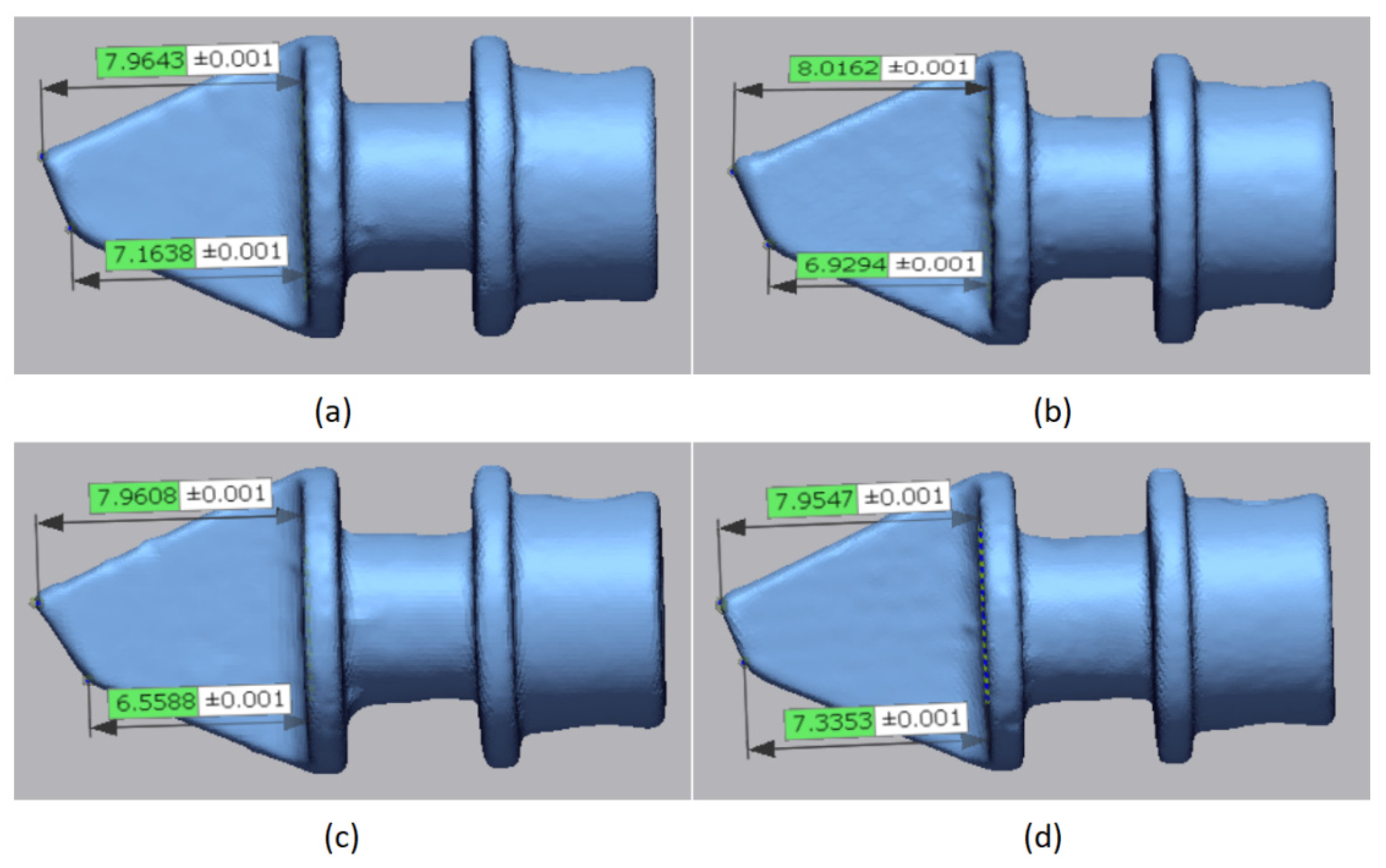
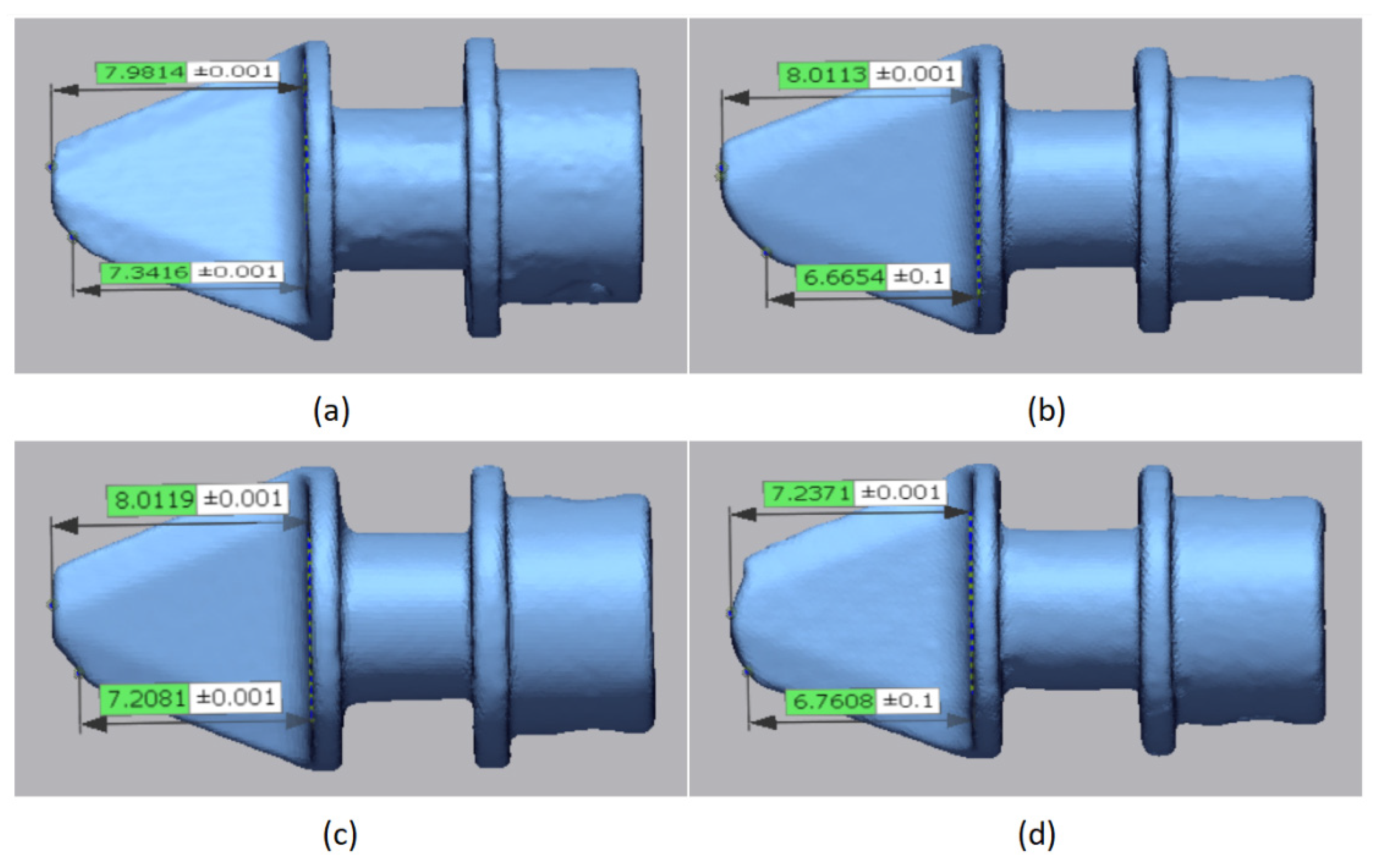
4.3. Ablation Analysis of 45 Steel Jet Vanes
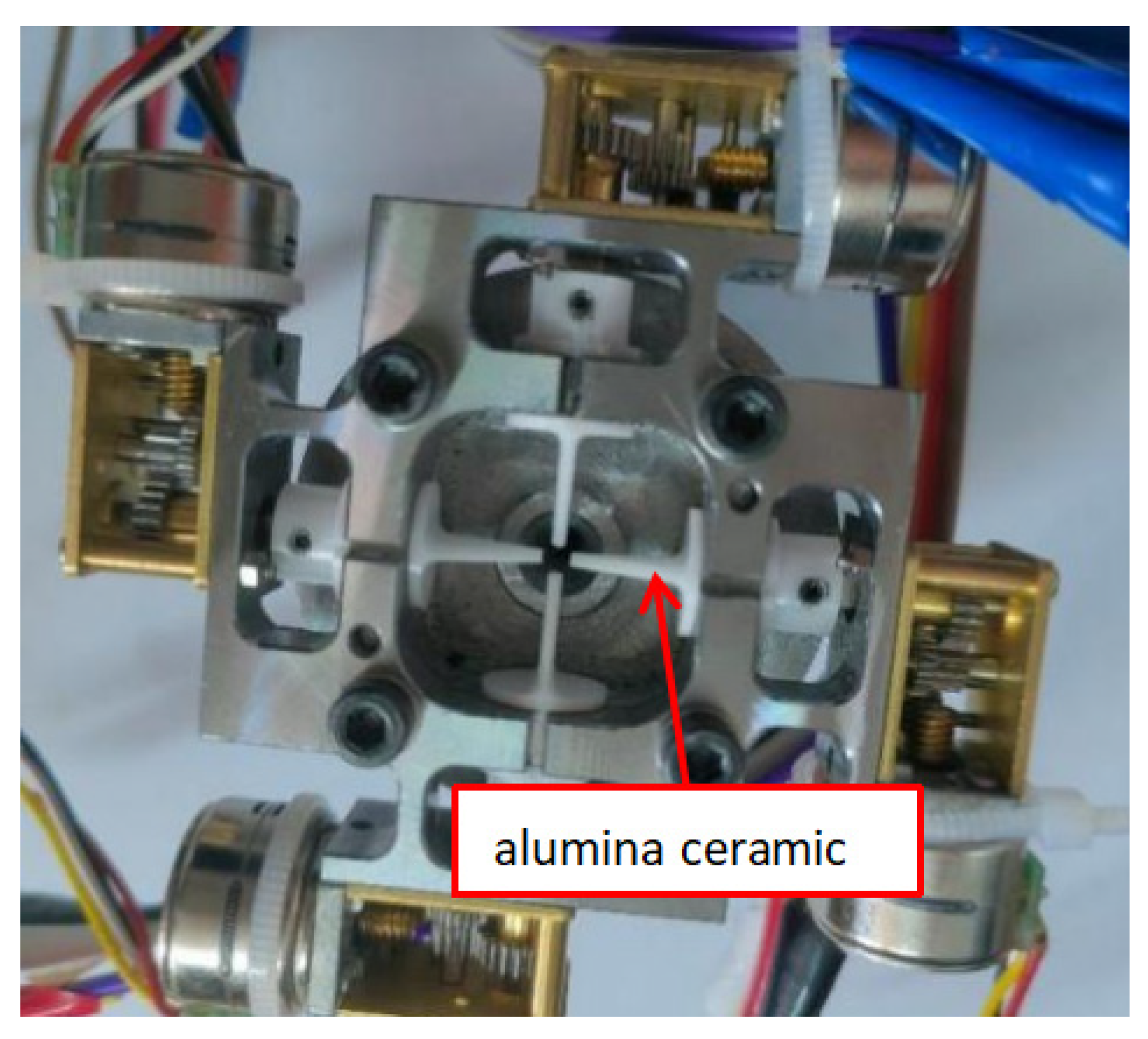
4.4. Ablation Analysis of Alumina-Ceramic Jet Vanes
4.5. Ablation Analysis of Tungsten–Molybdenum-Alloy Jet Vanes
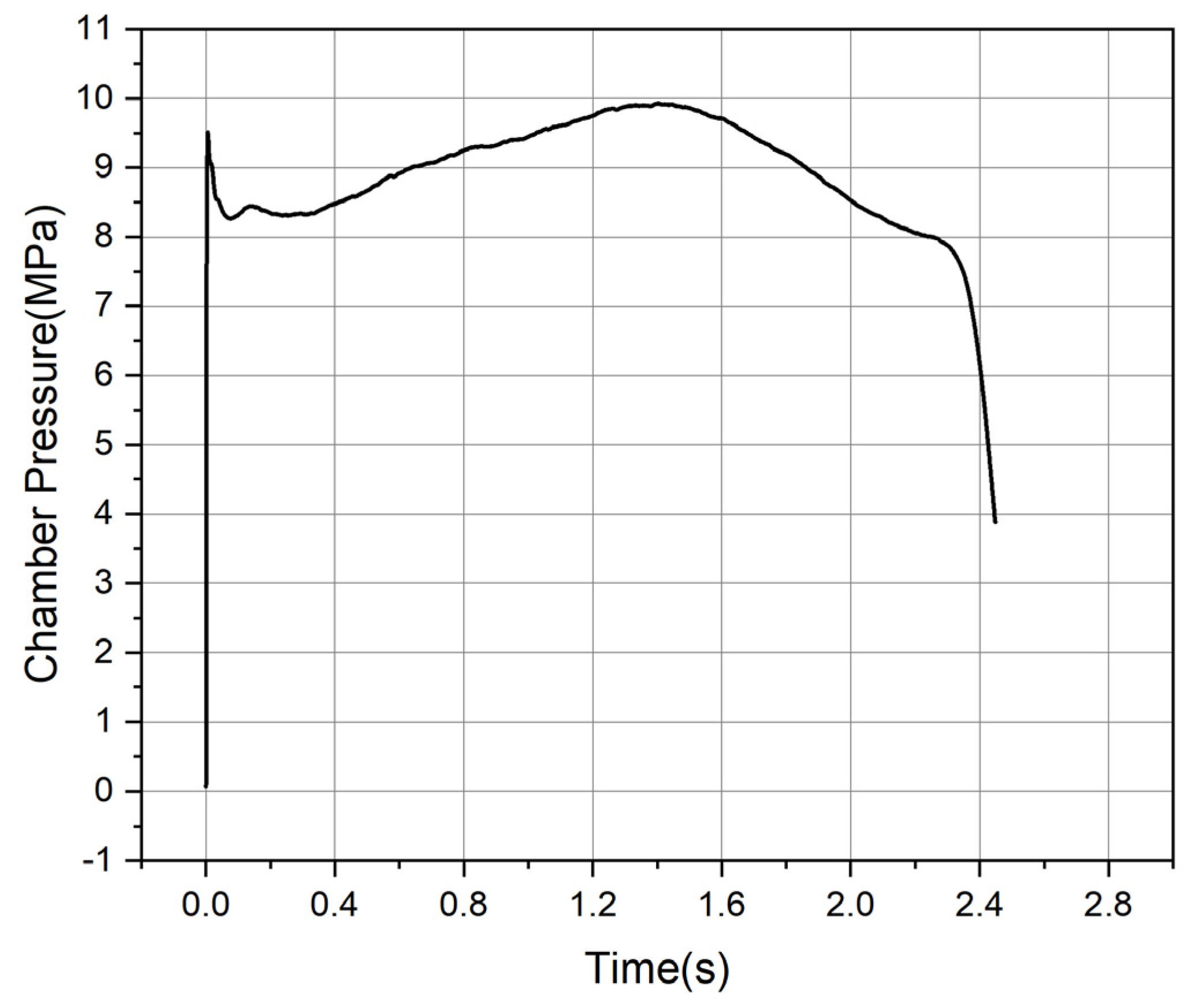
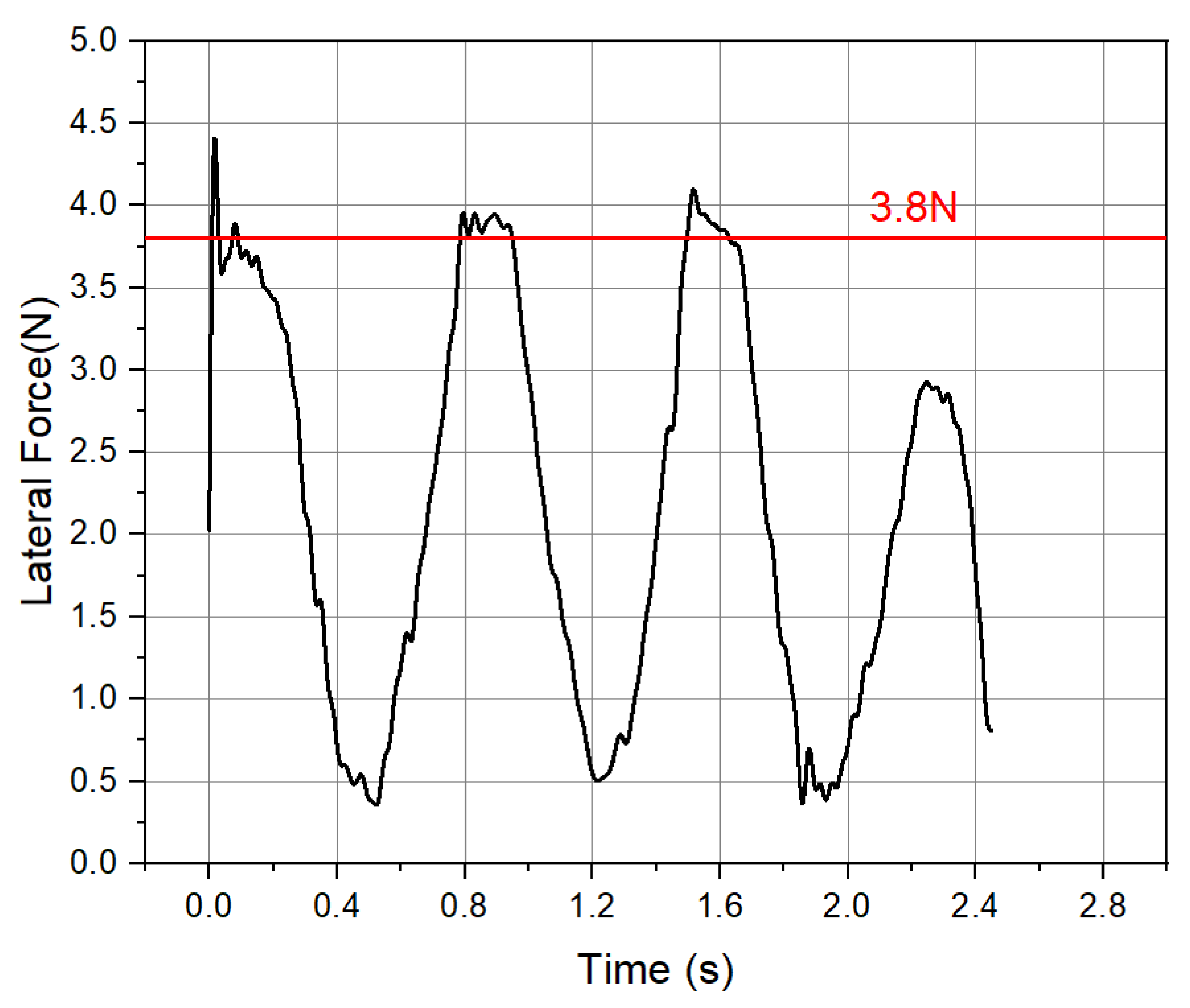
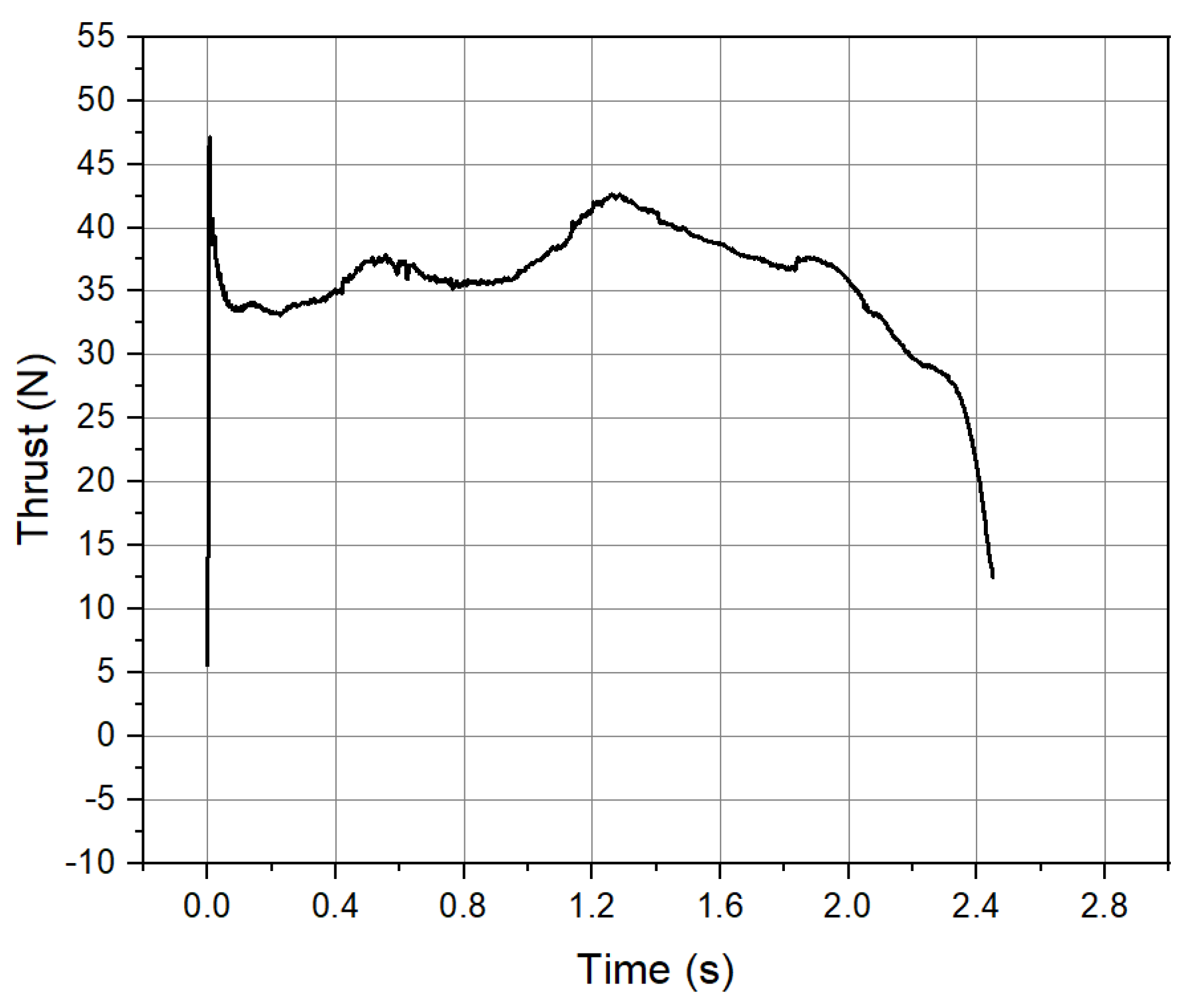
5. Comparative Analysis of Experiment and Simulation
6. Conclusions
- (1)
- As a refractory metal, the tungsten–molybdenum-alloy jet vanes exhibited no significant ablation during the experiment, indicating that tungsten–molybdenum alloy is an ideal material for micro-jet vanes. In contrast, the 45 steel jet vanes showed extensive ablation. Additionally, the alumina-ceramic jet vanes experienced fragmentation and ablation due to defects in the alumina ceramics, suggesting that 45 steel and alumina ceramics are not suitable materials for micro-jet vanes.
- (2)
- Meanwhile, the deflection of the gas flow in the test matches the temperature contour. When the deflection of the jet vanes is 20°, the peak lateral force obtained from the test is approximately 3.8 N, whereas the calculated lateral force from numerical simulation is 3.76 N, with an error of less than 1%. The fact that the simulated lateral force values matched those obtained from experiments demonstrates the accuracy of the simulation results.
- (3)
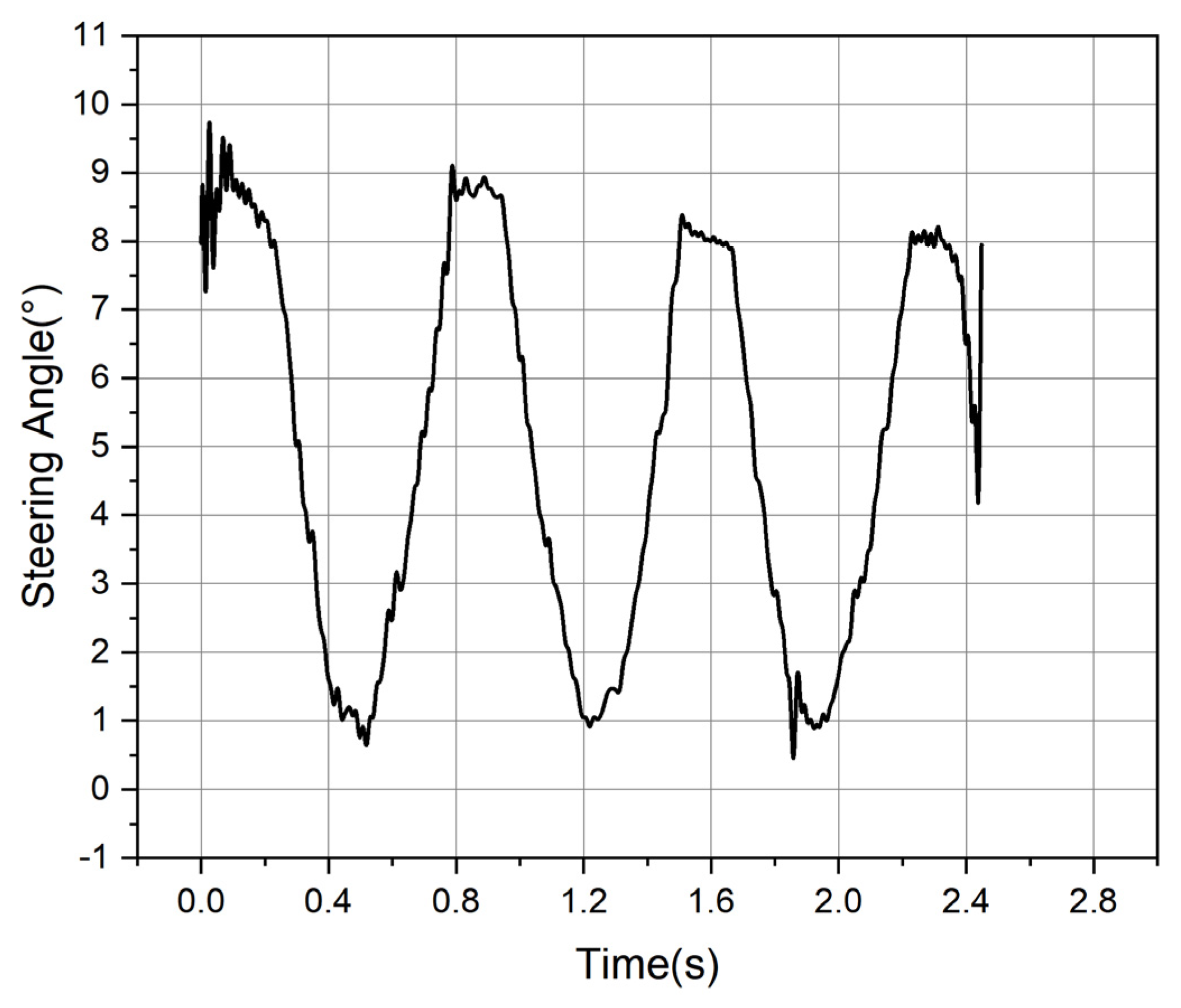
- The steering angle is the vector angle between lateral force and thrust, representing the steering capability facilitated by the jet vanes. The peak steering angle generated by the jet vanes is approximately 8°; thus, this system of jet vanes can achieve a strong steering capability and ensure rapid vector maneuvering of the micro-nano satellite at large angles and across a wide range.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, X.; Zhang, R.; Xie, X.; Yu, Y. Discussion on Micro-nano Satellites Industry Development. Spacecr. Eng. 2018, 27, 117–121. [Google Scholar]
- Liu, X.; Kang, X.; He, W.; Xu, M.; Guo, D.; Hang, G.; Li, L. Key technologies and prospect of ionic liquid electrospray thruster. J. Astronaut. 2019, 40, 977–986. [Google Scholar]
- Ketsdever, A.D.; Micci, M.M. Micropropulsion for Small Spacecraft; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2000. [Google Scholar]
- Zhang, W.; Peng, P.; Shen, Z.; Shi, W.; Xu, Y. Design and Practice of Exquisite Micro-nano Satellite. Spacecr. Eng. 2018, 27, 19–26. [Google Scholar]
- Yu, W.; Zhong, X.; Li, X.; Liu, J. Prospects of Picosat Development. Piezoelectrics Acoustooptics 2004, 26, 289–292. [Google Scholar]
- Levchenko, I.; Bazaka, K.; Ding, Y.; Raitses, Y.; Mazouffre, S.; Henning, T.; Klar, P.J.; Shinohara, S.; Schein, J.; Garrigues, L.; et al. Space Micropro-pulsion Systems for Cubesats and Small Satellites: From Proximate Targets to Furthermost Frontiers. Appl. Phys. Rev. 2018, 5, 011104. [Google Scholar] [CrossRef]
- Leomanni, M.; Garulli, A.; Giannitrapani, A.; Scortecci, F. Propul-sion Options for Very Low Earth Orbit Microsatellites. Acta Astronaut. 2017, 133, 444–454. [Google Scholar] [CrossRef]
- Janson, S.W.; Helvajian, H.; Robinson, E.Y. The concept of ‘nanosatellite’ for revolutionary low-cost space systems. In Proceedings of the 44th International Astronautics Federation Conference, Graz, Austria, 16–22 October 1993. [Google Scholar]
- Youngner, D.; Thai Lu, S.; Choueiri, E.; Neidert, J.; Black, R., III; Graham, K.; Fahey, D.; Lucus, R.; Zhu, X. MEMS Mega-pixel Micro-thruster Arrays for Small Satellite Station keeping. In Proceedings of the 14th AIAA/USU Small Satellite Conference, Logan, UT, USA, 21–25 August 2000; Paper SSC00-X-2. Utah State University: Logan, UT, USA, 2000. [Google Scholar]
- Rossi, C. Micropropulsion for Space—A Survey of MEMS-Based Micro Thrusters and their Solid Propellant Technology. Sens. Update 2002, 10, 257–292. [Google Scholar] [CrossRef]
- Mueller, J.; Ziemer, J.; Hofer, R.; Wirz, R.; O’Donnell, T. Asurvey of micro-thrust propulsion options for micro spacecraft and formation flying missions. In Proceedings of the 5th Annual CubeSat Developers Workshop, San Luis Obispo, CA, USA, 9 April 2008. [Google Scholar]
- Bonin, G.; Roth, N.; Armitage, S.; Newman, J.; Risi, B.; Zee, R.E. CanX-4 and CanX-5 Precision Formation Flight: Mission Accomplished! In Proceedings of the Small Satellite Conference, Logan, UT, USA, 8–13 August 2015. [Google Scholar]
- Zhang, Z.Y.; Deng, H.Y.; Liao, W.-H.; Zhang, X.; Zhao, L.; Bi, Y.-F. Review on Hybrid Propulsion Technology of Micro/Nano Satellite. J. Astronaut. 2022, 43, 282–292. [Google Scholar]
- Chen, M.; Liu, X.; Zhou, H.; Chen, C.; Jia, H. Research and development of micro electric propulsion technology for micro/nano satellites. J. Solid Rocket. Technol. 2021, 44, 188–206. [Google Scholar]
- Wu, J.-J.; Hu, Z.-J.; Zhang, Y.; He, Z.-C.; Ou, Y.; Zheng, P.; Zhao, Y.-Z.; Li, Y.-Q. Research Progress of Space Micro-Propulsion Technology Based on Solid-Propellant. J. Propuls. Technol. 2023, 44, 20–38. [Google Scholar] [CrossRef]
- Sathiyanathan, K.; Lee, R.; Chesser, H.; Dubois, C.; Stowe, R.; Farinaccio, R.; Ringuette, S. Solid propellant microthruster design for nanosatellite applications. J. Propuls. Power 2011, 27, 1288–1294. [Google Scholar] [CrossRef]
- Mueller, J. Thruster options for microspacecraft: A review and evaluation of state-of-the-art and emerging technologies. Prog. Astronaut. Aeronaut. 2000, 187, 45–138. [Google Scholar]
- Duan, D. Application and Analysis of Thrust Vectoring Technology in Air-to-Air Missile. Aerodyn. Missile J. 2012, 4, 84–87. [Google Scholar]
- Xie, Y.Q.; Li, S.; Zhou, X.F. The Application Analysis of Thrust Vector Control Systems of Air to Air Missile. Sci. Technol. Eng. 2009, 9, 6109–6113. [Google Scholar]
- Liu, J.; Zeng, W.; Tang, J.; Fu, B.; Tan, T.; Wang, Q. Development status and Research Trend of Vertical Launching Multi-purpose Missile. J. Proj. Rocket. Missiles Guid. 2019, 39, 172–177. [Google Scholar] [CrossRef]
- Luo, L.; Zhou, Y.; Zhang, Y.; Jiu, X.; Liu, J.; Zhu, X.; Wu, Y. Research status and development trend of toughening and toughening technology for molybdenum based materials. Trans. Nonferrous Met. Soc. China 2019, 29, 525–537. [Google Scholar]
- Peterson, J.H.; Meiners, K.E.; Ripleylotee, M. Fabrication of Copper Infiltrated Tungsten Jet Vanes. J. Met. 1981, 33, A8. [Google Scholar]
- Sogutcu, B.; Sumer, B. Expemental and Numerical Investigation of a Jet Vane of Thrust Vector Control system. In Proceedings of the AIAA Propilsion and Energy 2019 Forum, Indianapolis, IN, USA, 19−22 August 2019. [Google Scholar]
- Djugum, R.; Sharp, K. The Fabrication and Performance of High Temperature Carbon-Carbon Composites Containing Tantalum Filler. J. Aust. Ceram. Soc. 2015, 51, 103–109. [Google Scholar]
- Liu, L.; Li, K.; Li, H.; Shen, X. Numerical Analysis on Thermostructure of C/C Composites Jet Vane. Mech. Sci. Technol. 2011, 30, 793–796+803. [Google Scholar]
- Bansard, S.; Plouvier, S. Experimental Simulation of Ther-mo-Mechanical Ablation of Carbon/Phenolic Composite under the Impact of Liquid Alumina Particles. High Temp. Mater. Process. 2005, 9, 431–441. [Google Scholar] [CrossRef]
- Xue, H.; Chen, X.; Zheng, J. Numerical research on flow-thermal coupling of carbon-phenolic jet-vane based on pyrolysis kinetics. J. Solid Rocket. Technol. 2015, 38, 503–509. [Google Scholar]
- Xue, H.; Chen, X.; Zheng, J. Ablation performance of carbon-phenolic jet vanes. J. Solid Rocket. Technol. 2017, 40, 706–713. [Google Scholar]
- Yan, G.; Deng, X.; Lin, J. The Research of High-thermal-conductive Aluminum Nitride Substrate in Airport Power Electronics. Print. Circuit Inf. 2017, 25, 32–37. [Google Scholar] [CrossRef]
- Upadhya, K.; Yang, J.M.; Hoffman, W.P. Materials for Ultrahigh Temperature Structural Applications. Am. Cermic Bull. 1997, 72, 51–56. [Google Scholar]
- Liu, J.; Lyu, Z.; Zhou, Y.; Huang, S.; Chen, H.; Xu, N. Research Progress of Advanced Ceramic Materials for Thermal Barrier Coatings. Surf. Technol. 2022, 51, 42–52. [Google Scholar] [CrossRef]
- Liu, G.-C.; Sun, M.; Hua, L.; Zhou, L.-Y.; Liu, Y.; Xia, H.-W. Research progress on properties and formation mechanism of high-entropy ceramic materials. Chin. J. Nonferrous Met. 2023, 33, 1902–1913. [Google Scholar] [CrossRef]
- Bai, H.; Shi, Z.; Xue, H.; Cai, H. Numerical simulation and Test on AlN ceramic Jet Vane. J. Aerosp. Power 2022, 1–13. [Google Scholar] [CrossRef]
- Kendall, R.P.; Votta, L.G.; Post, D.E.; Moyer, E.T.; Morton, S.A. Verification and Validation in CREATE Multiphysics HPC Software Applications. Comput. Sci. Mot. 2017, 19, 18–26. [Google Scholar] [CrossRef]
- Menter, F.R. Zonal two equation k-ω turbulence models for aerodynamic flows. In Proceedings of the AIAA-93-2906, Orlando, FL, USA, 6–9 July 1993. [Google Scholar]











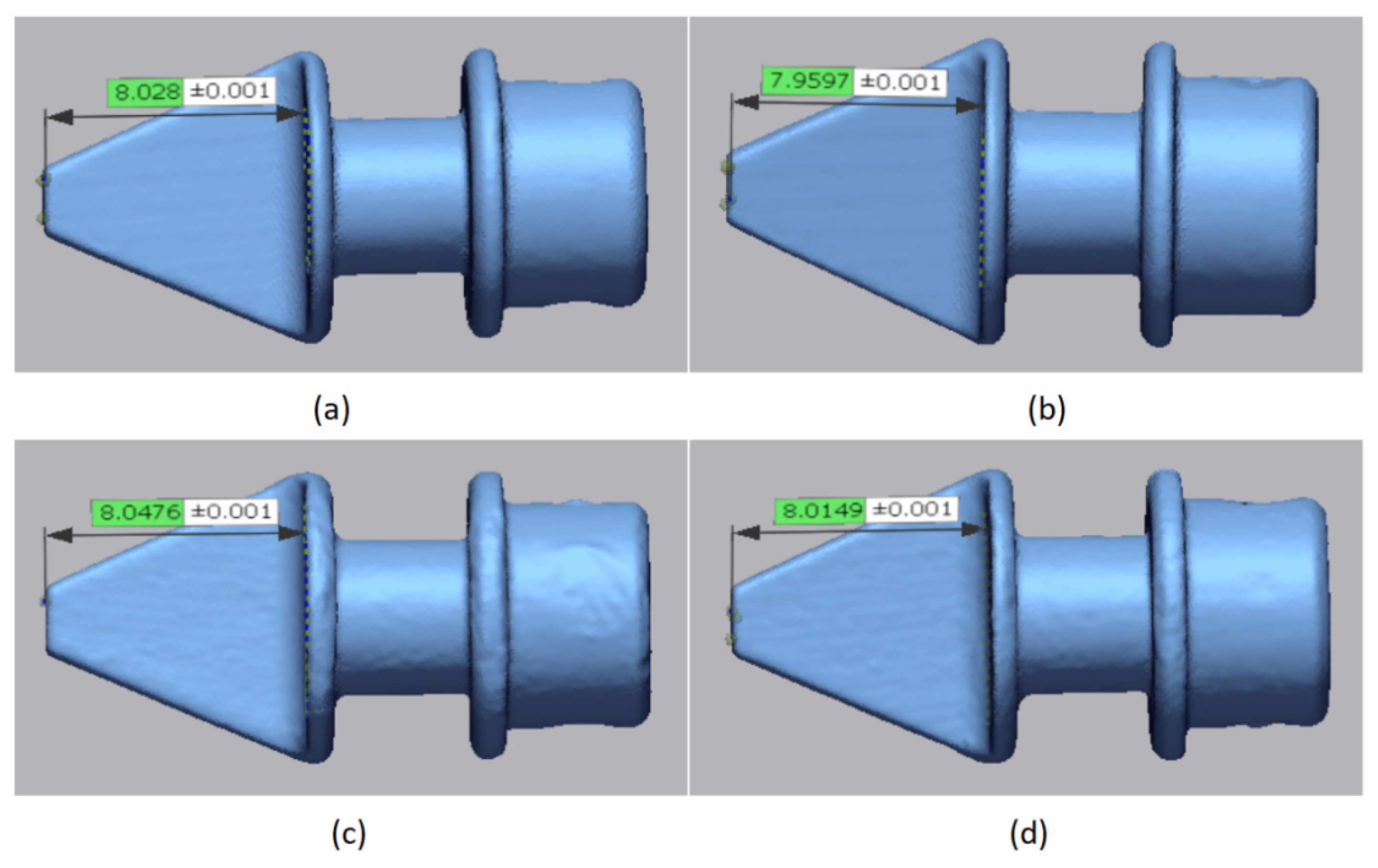
| Parameters of the Solid Rocket Motor | Values |
|---|---|
| Total gas temperature Tc/(K) | 1380 |
| Total pressure Pc/(MPa) | 8 |
| Specific heat ratio of gas k | 1.2 |
| Throat diameter Dt/(mm) | 2.5 |
| Pressure at the nozzle outlet Pe/(MPa) | 0.2 |
| Temperature at the nozzle outlet Te/(K) | 946.6 |
| Gas density at the nozzle outlet /(kg/m3) | 0.68 |
| Gas velocity at the nozzle outlet Ve/(m/s) | 1605.6 |
| Mach number at the nozzle exit Mae | 2.81 |
| Expansion ratio of nozzle | 7 |
| Thrust Fc/(N) | 50 |
| Parameters of the Gas | Values |
|---|---|
| Total gas temperature Tc/(K) | 1380 |
| Molecular weight/(g/mol) | 26.885 |
| Specific heat capacity/(J/(Kg·K)) | 2975.3 |
| Size of the Grid | Number of Grids | Lateral Force (N) |
|---|---|---|
| 0.2 mm | 9,115,316 | 3.79 |
| 0.3 mm | 3,249,412 | 3.77 |
| 0.4 mm | 1,767,302 | 3.78 |
| 0.5 mm | 1,354,466 | 3.76 |
| 0.6 mm | 911,809 | 3.02 |
| 0.7 mm | 760,415 | 3.21 |
| 0.8 mm | 689,252 | 3.04 |
| 0.9 mm | 641,845 | 3.23 |
| 1 mm | 615,419 | 3.01 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Feng, W.; Tan, Y.; Liu, Y.; Hui, W. Implementation and Verification of a Micro-Jet-Vane System of a Solid Rocket Motor for a Micro-Nano Satellite. Aerospace 2024, 11, 384. https://doi.org/10.3390/aerospace11050384
Zhang G, Feng W, Tan Y, Liu Y, Hui W. Implementation and Verification of a Micro-Jet-Vane System of a Solid Rocket Motor for a Micro-Nano Satellite. Aerospace. 2024; 11(5):384. https://doi.org/10.3390/aerospace11050384
Chicago/Turabian StyleZhang, Gang, Wen Feng, Youwen Tan, Yang Liu, and Weihua Hui. 2024. "Implementation and Verification of a Micro-Jet-Vane System of a Solid Rocket Motor for a Micro-Nano Satellite" Aerospace 11, no. 5: 384. https://doi.org/10.3390/aerospace11050384
APA StyleZhang, G., Feng, W., Tan, Y., Liu, Y., & Hui, W. (2024). Implementation and Verification of a Micro-Jet-Vane System of a Solid Rocket Motor for a Micro-Nano Satellite. Aerospace, 11(5), 384. https://doi.org/10.3390/aerospace11050384






