Design of Stress Release Support Mechanism for Large-Size Body-Mounted Radiator
Abstract
1. Introduction
2. Stress Release and Restraint
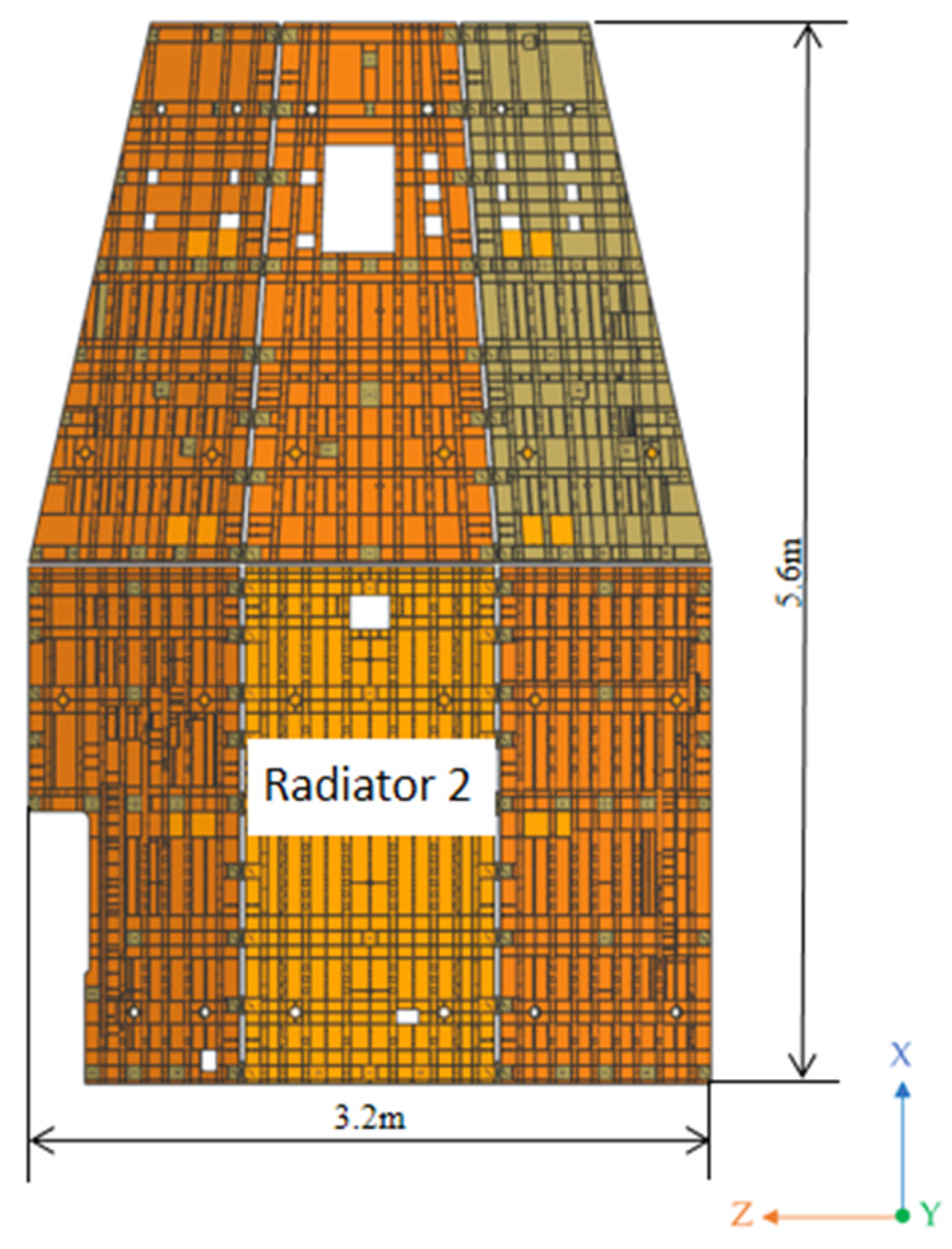
2.1. Layout Design and Release Principle of Stress Release Mechanism
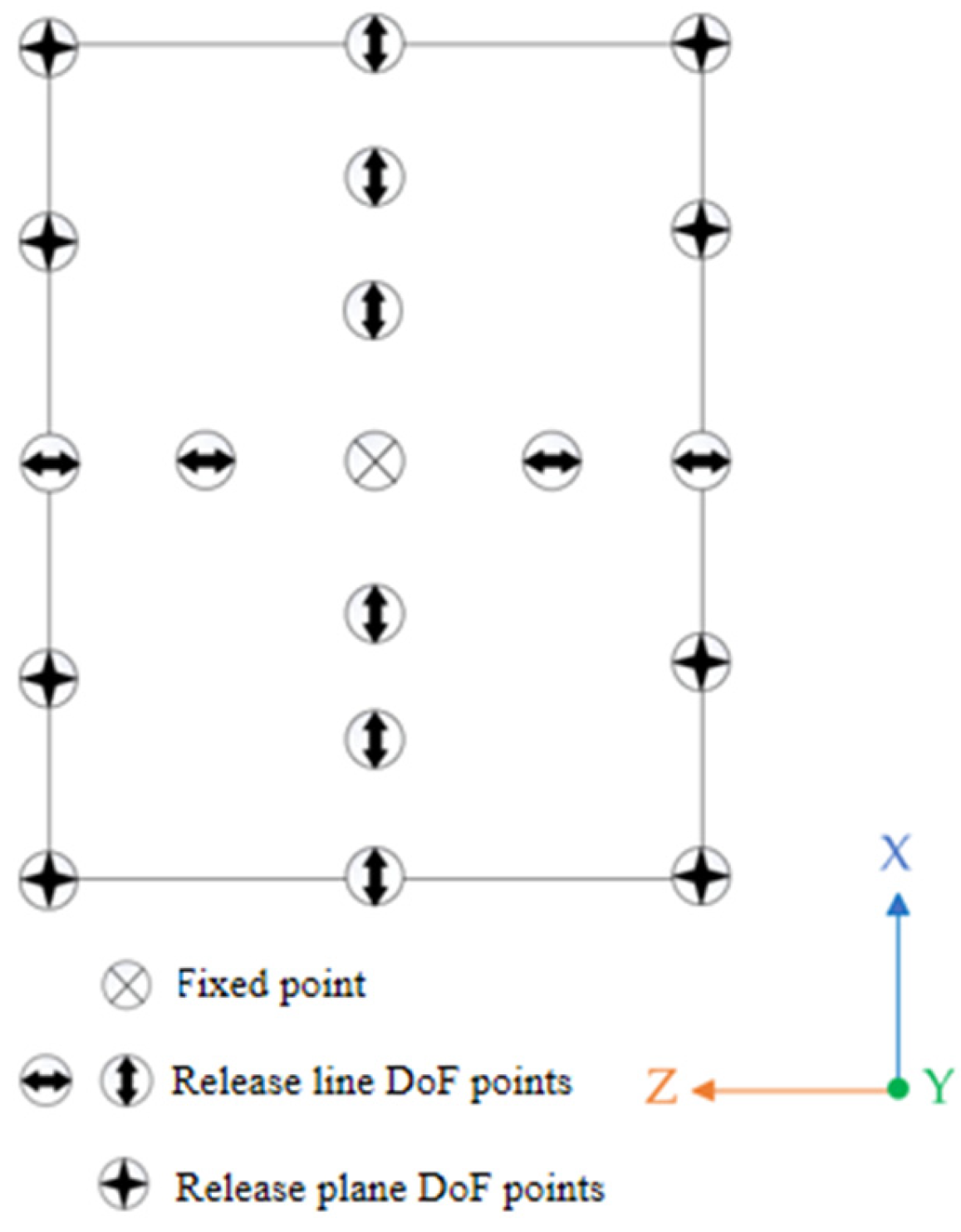
2.2. Degree-of-Freedom Analysis
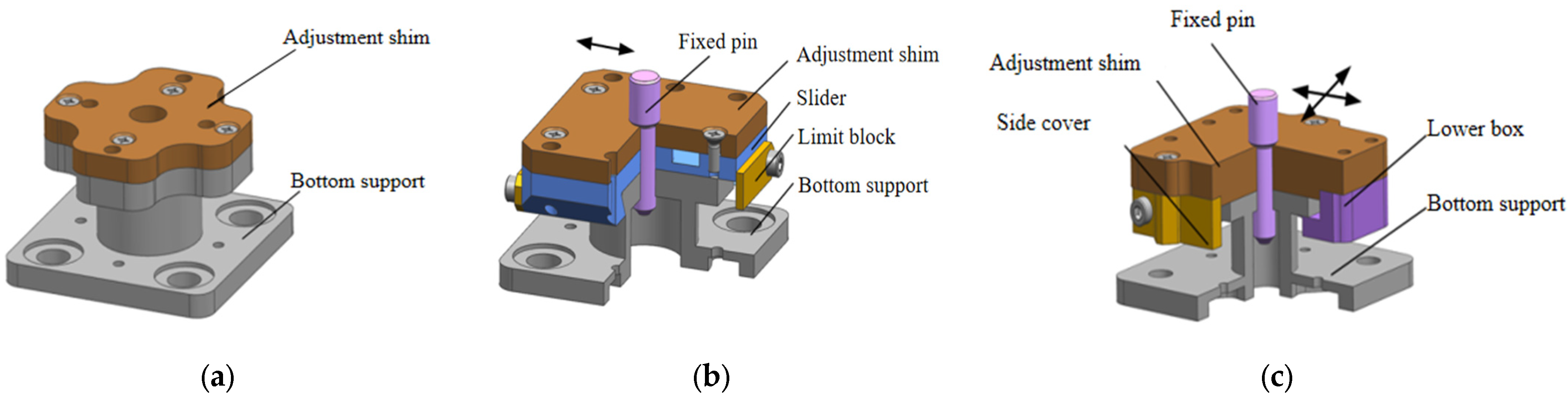
3. Design of Stress Relief Mechanism
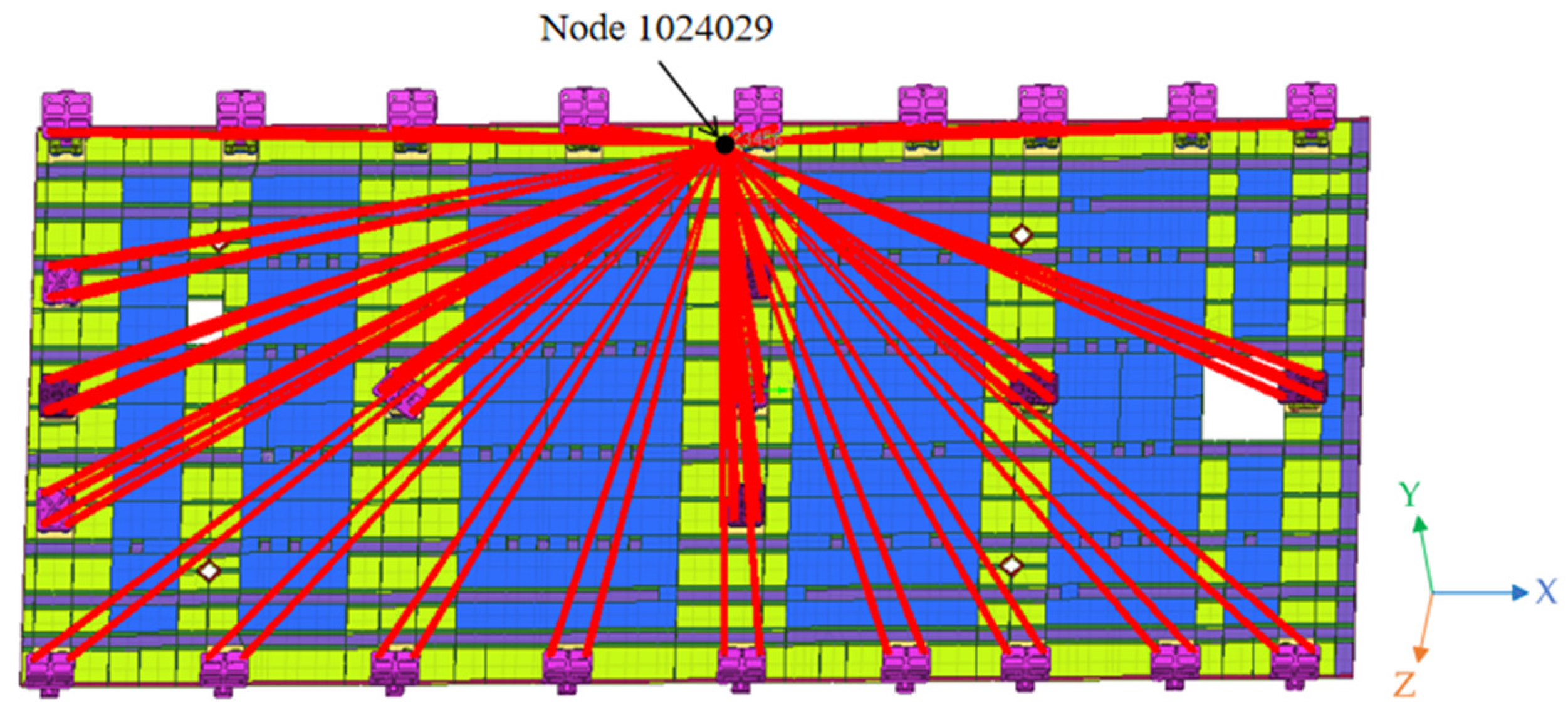
4. Finite Element Simulation Analysis
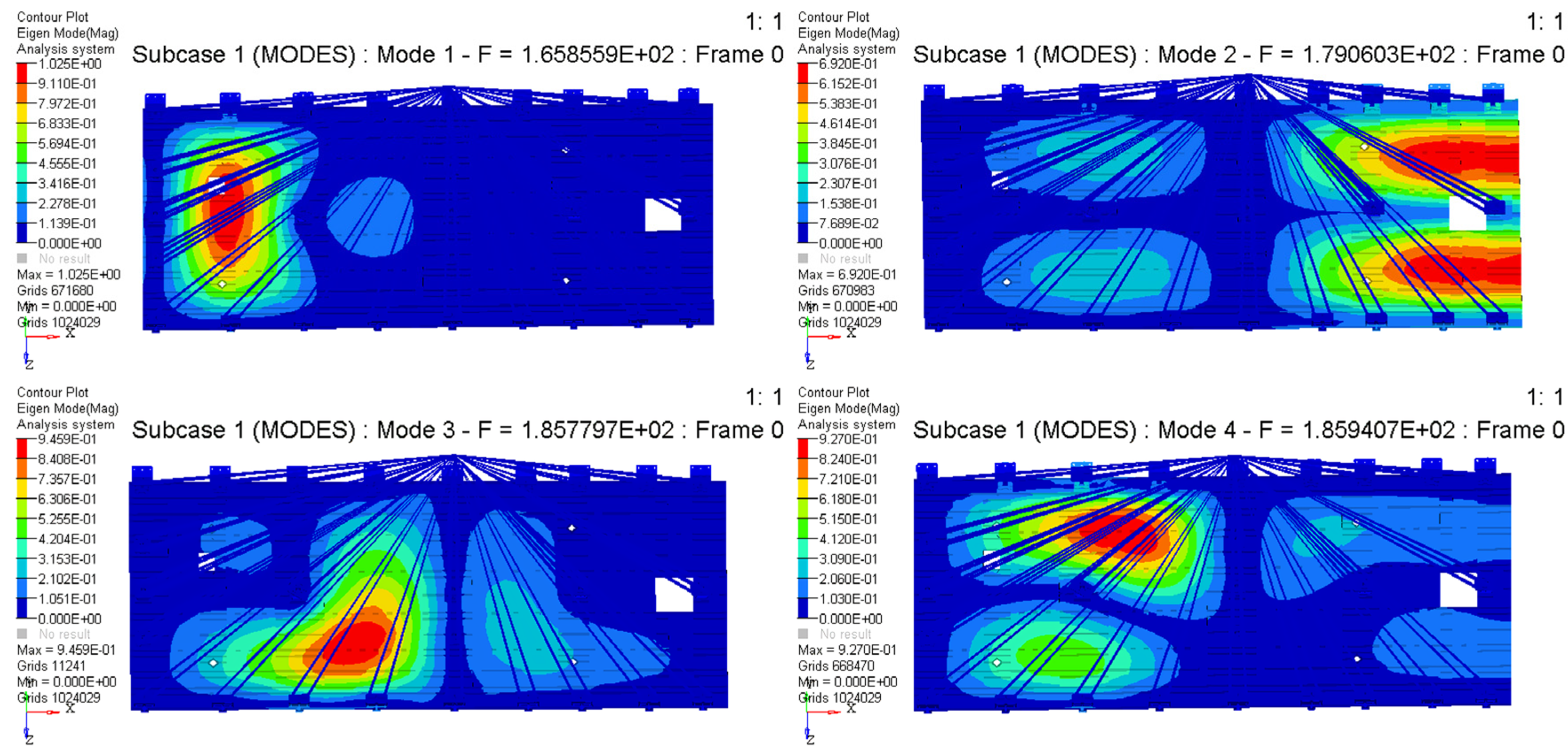
4.1. Modal Analysis
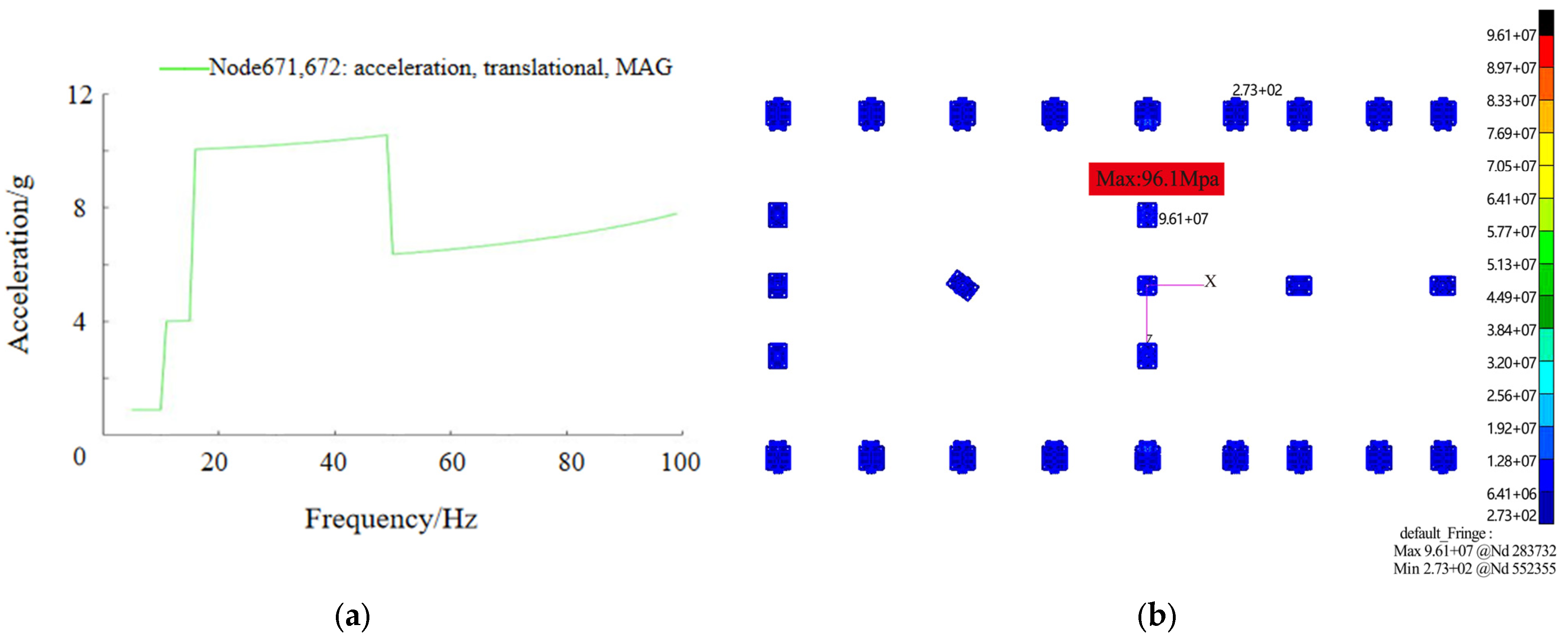
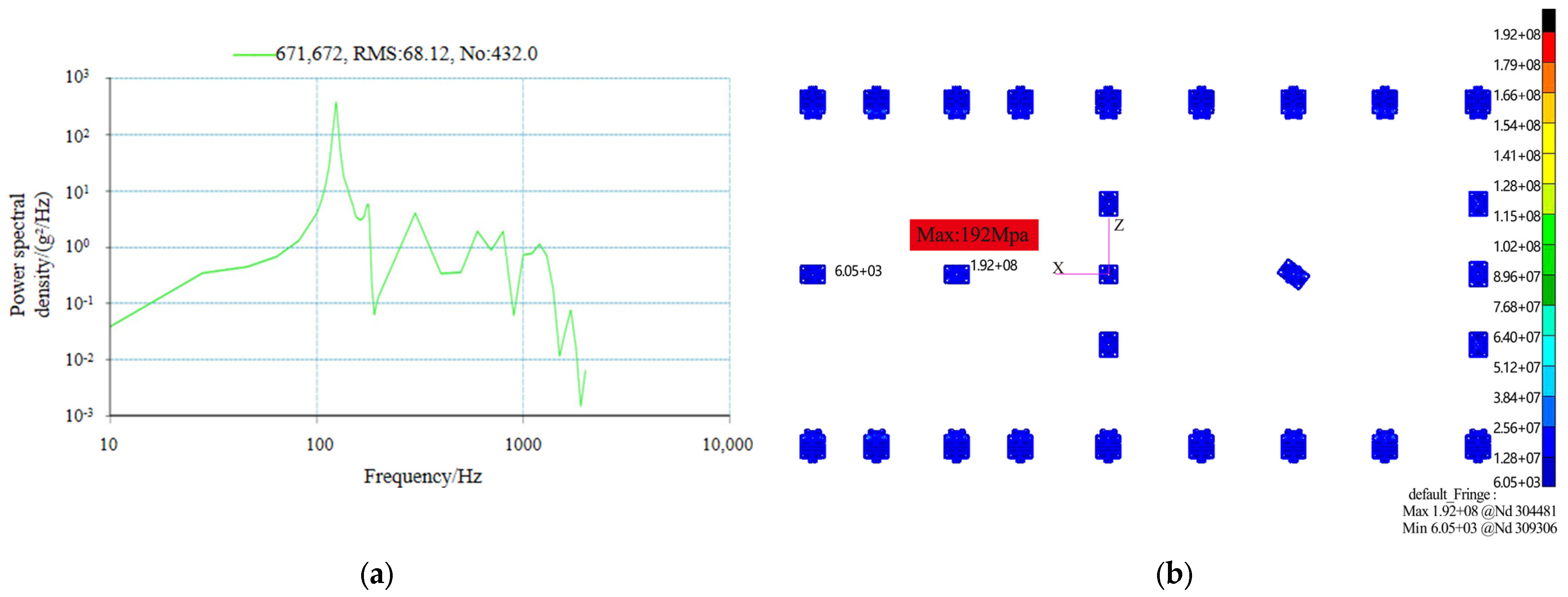
4.2. Frequency Response Analysis
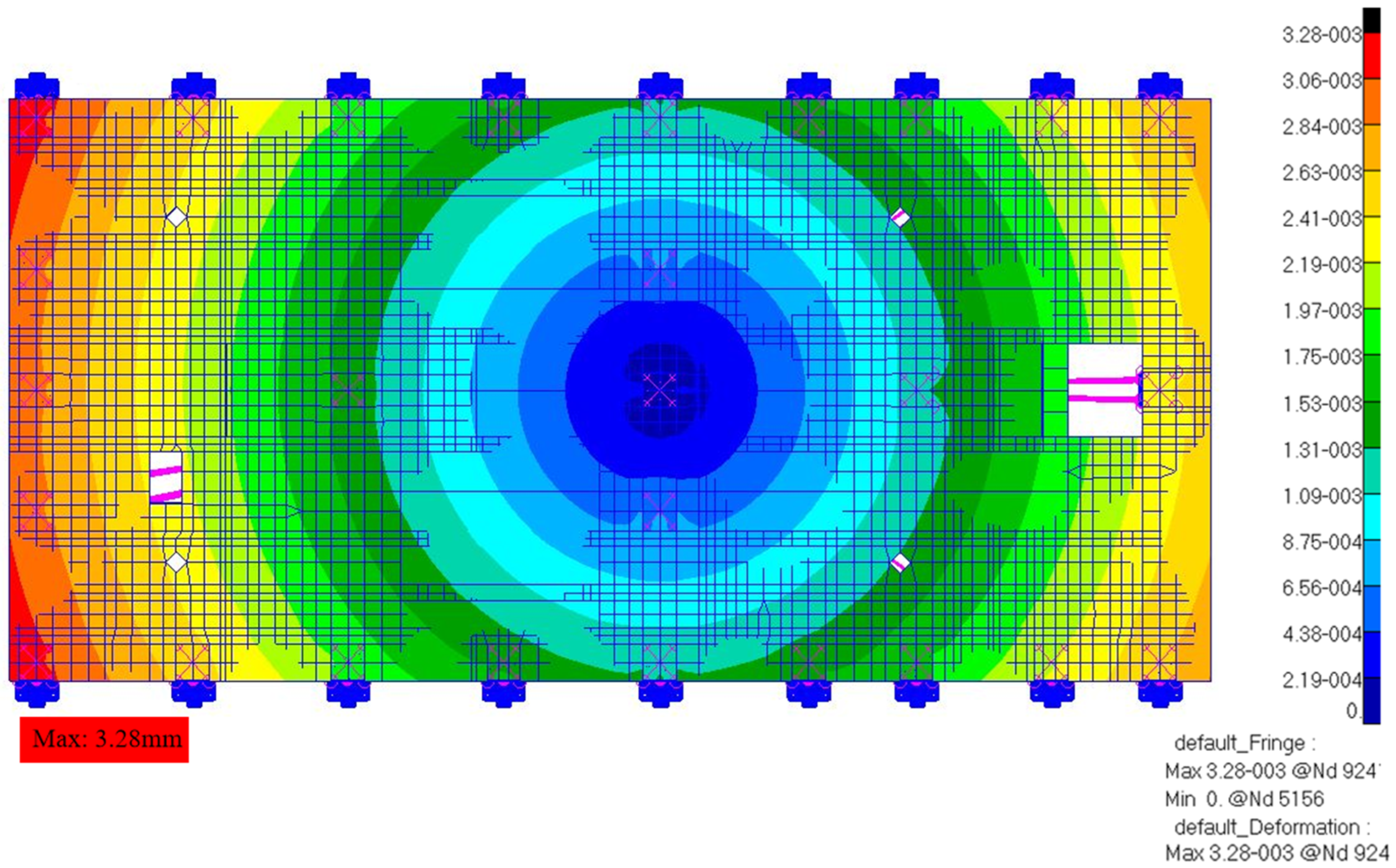
4.3. Thermal Strain Analysis
5. Experiment
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sebag, J.; Gressler, W.J.; Liang, M.; Axelrod, T.; Claver, C.; Andrew, J. Calibration systems for LSST. In Ground-Based & Airborne Telescopes V; International Society for Optics and Photonics: Washington, DC, USA, 2014; p. 91453L. [Google Scholar]
- Su, D.; Wang, Y. The Tracking Motion of the Large Sky Area Multi-object Fibre Spectroscopic Telescope (LAMOST). J. Acta Astrophys. -CA Sin. 1997, 17, 315–322. [Google Scholar]
- SPACE. Chinese Astronomers Say Their New Space Telescope Will Outdo Hubble [EB/OL]. (2023-10-04) [2023-11-15]. Available online: https://www.space.com/china-space-telescope-xuntian (accessed on 1 February 2024).
- Swift, W.L.; Dolan, F.X.; Zagarola, M.V. The NI-CMOS Cooling SYSTEM5 Years of Successful On-Orbit Operation. In Aip Conference; American Institute of Physics: College Park, MD, USA, 2008. [Google Scholar]
- Nowak, M.D.; Cofie, E.; Davila, P.S.; Eegholm, B.H.; Young, P.J. Cryo-genic performance of a high precision photogrammetry system for verification of the James W-ebb Space Telescope Integrated Science Instrument Module and associated ground support equipment structural alignment requirements. In Optical System Alignment, Tolerancing, & Verification IV; International Society for Optics and Photonics: Washington, DC, USA, 2010. [Google Scholar]
- Kim, H.K.; Choi, S.; Park, S.O.; Lee, K.H. Node-base-d spacecraft radiator design optimization. J. Adv. Space Res. 2015, 55, 1445–1469. [Google Scholar] [CrossRef]
- Wang, K.J.; Dong, J.H.; Ming, X.; Zhang, H.H.; Zhang, L.H.; Sun, L.J. The Flexible Structure Design in the Whiffletree Structure. J. Chang. Univ. Sci. Technol. (Nat. Sci. Ed.) 2015, 38, 12–17. [Google Scholar]
- Zhang, A.; Zhang, T.; Li, N.; Wang, J.; Yang, S. Design and Verification of Main Supporting Structure for H-XMT Satellite Telescope. J. Spacecr. Eng. 2018, 27, 139–142. [Google Scholar]
- Shao, M.Q.; Zhang, L.; Li, L.; Wei, L.; Jia, X.Z. Design and Test of Flexible Supporting Structure for Ultra-light Mirror. J. Guangzi Xuebao/Acta Photonica Sin. 2019, 48, 1222001. [Google Scholar] [CrossRef]
- Dong, W.; Sun, L.; Du, Z. Stiffness research on a high-precision, large-workspace parallel mechanism with compliant joints. J. Precis. Eng. 2008, 32, 222–231. [Google Scholar] [CrossRef]
- Dong, W.; Sun, L.N.; Du, Z.J. Design of a precision compliant parallel positioner driven by dual piezoelectric actuators. J. Sens. Actuators A Phys. 2007, 135, 250–256. [Google Scholar] [CrossRef]
- Shi, X.D.; Li, J.L.; Wang, L.W. Design of Large Displacement and High Precision Linear Stage Based on Laminated Flexure Hinge and Ultrasonic Motor. J. Manuf. Technol. Mach. Tool 2007, 2, 104–106. [Google Scholar]
- Tan, K.; Zong, G.H.; Bi, S.S. Multi-leaf configuration of expanded -motion-range flexure hinges. J. Dual-Use Technol. Prod. 2007, 38–42. [Google Scholar] [CrossRef]
- Zhao, S.B.; Bi, S.S.; Zong, G.H. New Large-deflection Flexure Pivot Based on Curved Flexure Element. J. Mech. Eng. 2009, 45, 8–12. [Google Scholar] [CrossRef]
- Yuan, Y. Research on the On-Orbit Integrated Optical Load Support Technology; University of Chinese Academy of Sciences: Changchun, China, 2021. [Google Scholar]
- Liu, X.; Huang, H.; Zheng, Y. Vibration time domain waveform control of Hexapod multi-degree-of freedom micro excitation system. J. Aerosp. Control. Appl. 2020, 46, 56–63. [Google Scholar]
- Shen, Z.X.; Hu, G.K. Study on Thermally Induced Vibrations in Large-scale Spacecraft Structures. J. Manned Spacefl. 2016, 22, 117–125. [Google Scholar]
- Liu, X.; Liang, X.G. Optimization Design and Analysis of Heat Transfer for Space Radiator. J. Astronaut. 2016, 37, 605. [Google Scholar]
- Wu, Q.W. Optimum design on the primary mirror central supported in a space remote-sensing camera. J. Opt. Technol. 1998, 24, 73–75. [Google Scholar]
- Zhang, J.Q.; Dong, D.Y.; Yan, C.X. Primary support of high resolution space imaging spectrometer. J. Opt. Precis. Eng. 2009, 17, 2450–2455. [Google Scholar]
- Wang, K.J.; Dong, J.H.; Zhou, P.W.; Wang, X.Y.; Jiang, P. Back support structure design of mirror of space remote sensor. J. Infrared Laser Eng. 2023, 48, 11–25. [Google Scholar]
- Jia, X.H.; Zhang, D.W. Design and forward kinematics of 3-DOF precision positioning stage. In Proceedings of the 2009 International Conference on Mechatronics and Automation, Changchun, China, 9–12 August 2009; pp. 3324–3329. [Google Scholar]
- Wang, K.J.; Dong, J.H.; Li, W. Finite element analysis of overall structure of space camera. J. OME Inf. 2010, 17, 81–87. [Google Scholar]
- Li, Z.X. Analysis and test on the response of primary mirror flexure under random vibration. J. Infrared Laser Eng. 2014, 43, 100–107. [Google Scholar]
- Liu, Q. Research on the Bottom Support Structure of the Extra-Wide Coverage Remote Senor; University of Chinese Academy of Sciences: Changchun, China, 2013. [Google Scholar]





| Node Number | Element Number | |
|---|---|---|
| 1D (Rigids) | 3399 | 189 |
| 2D (Shell) | 6744 | 7471 |
| 3D (Solid) | 106286 | 59552 |
| Material | ρ (10−9 t/mm3) | E (GPa) | μ |
|---|---|---|---|
| 7075 | 2.8 | 70 | 0.33 |
| TC4 | 4.44 | 107 | 0.33 |
| Polyimide | 2.3 | 200 | 0.4 |
| Model Order | 1st | 2nd | 3rd | 4th | 5th |
|---|---|---|---|---|---|
| Simulation results (Hz) | 165.9 | 179.1 | 185.8 | 185.9 | 204.5 |
| Direction | X | Y, Z | ||
|---|---|---|---|---|
| parameters | Freq. Frequency (Hz) | magnitude | Freq. Frequency (Hz) | magnitude |
| 10–15 | 8.83 mm | 5–10 | 14.89 mm | |
| 15–30 | 8 g | 10–20 | 6.0 g | |
| 30–100 | 5.5 g | 20–70 | 12.75 g | |
| — | — | 70–100 | 4 g | |
| Scan freq. | 2 oct/min | |||
| Direction | X | Y, Z | ||
|---|---|---|---|---|
| parameters | Freq. (Hz) | Magnitude | Freq. (Hz) | Magnitude |
| 10–20 | 9 dB/Oct | 10–20 | 9 dB/Oct | |
| 20–200 | 0.125 g2/Hz | 20–200 | 0.3 g2/Hz | |
| 200–2000 | −3 dB/Oct | 200–2000 | −3 dB/Oct | |
| Total RMS accelerations | 8.99 g | 13.9 g | ||
| Testing time/min | 2 min | |||
| Vibration Direction | Sine Vibration | Random Vibration | ||||
|---|---|---|---|---|---|---|
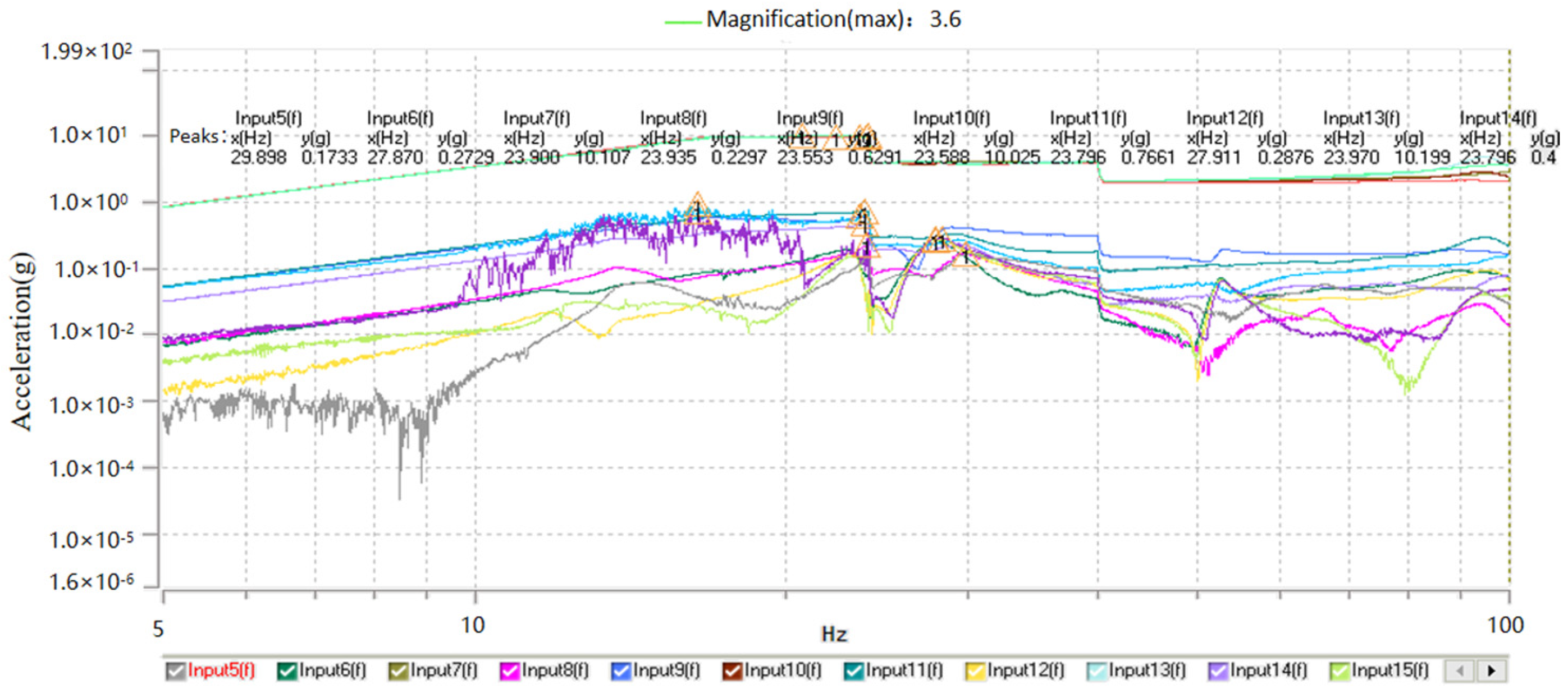
| Max Acceleration Response/g | Multiple | Stress/Mpa | Root Square ACC Response/g | Multiple | Stress/Mpa | |
| X | 7.82 | 1.3 | 96.1 | 14.43 | 1.6 | 126 |
| Y | 7.28 | 3.6 | 34.9 | 62.38 | 4.5 | 192 |
| Z | 2.50 | 1.3 | 30.0 | 18.63 | 1.3 | 176 |
| Frequency | 1st | 2nd | 3rd | 4th | 5th |
|---|---|---|---|---|---|
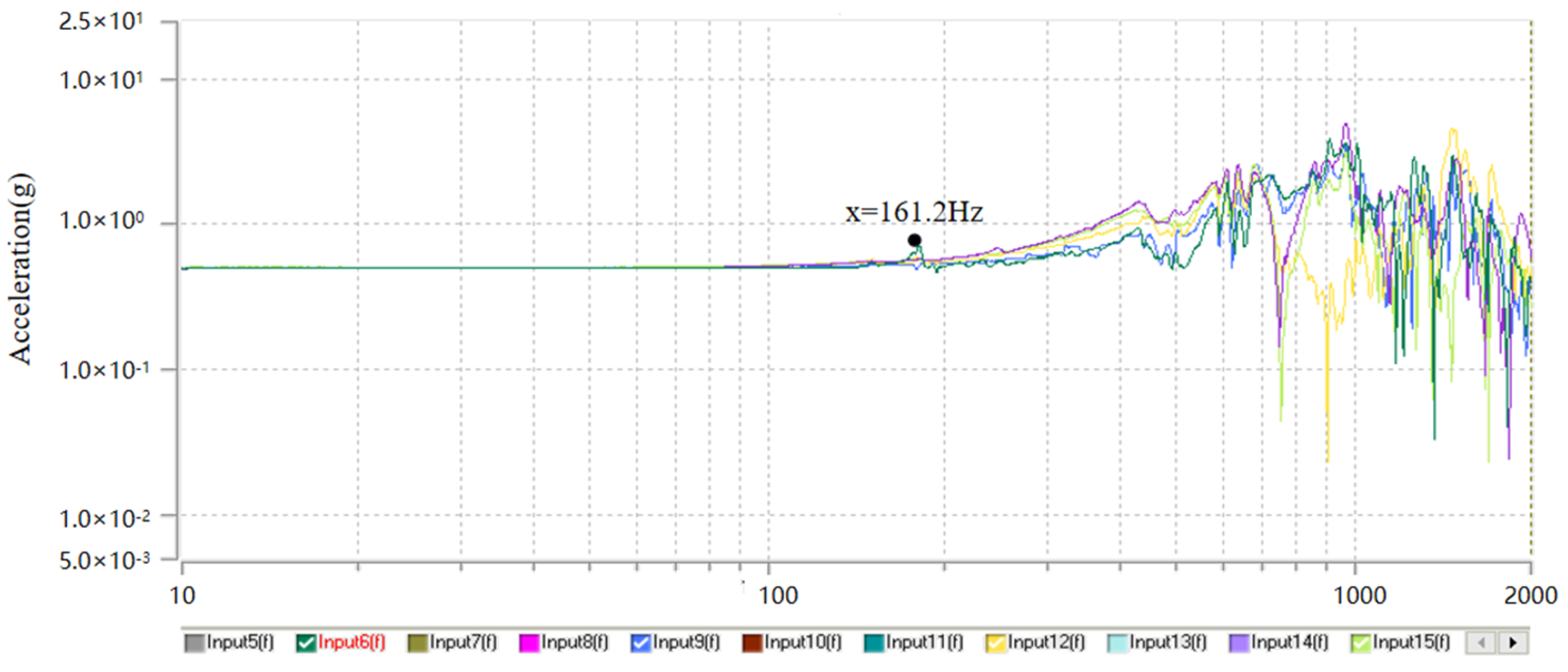
| Test results (Hz) | 161.2 | 173.5 | 181.7 | 184.4 | 209.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, X.; Han, K.; Lu, Z.; Zhang, S.; Guo, L. Design of Stress Release Support Mechanism for Large-Size Body-Mounted Radiator. Aerospace 2024, 11, 202. https://doi.org/10.3390/aerospace11030202
Huang X, Han K, Lu Z, Zhang S, Guo L. Design of Stress Release Support Mechanism for Large-Size Body-Mounted Radiator. Aerospace. 2024; 11(3):202. https://doi.org/10.3390/aerospace11030202
Chicago/Turabian StyleHuang, Xingxing, Kang Han, Zhenyu Lu, Shuncheng Zhang, and Liang Guo. 2024. "Design of Stress Release Support Mechanism for Large-Size Body-Mounted Radiator" Aerospace 11, no. 3: 202. https://doi.org/10.3390/aerospace11030202
APA StyleHuang, X., Han, K., Lu, Z., Zhang, S., & Guo, L. (2024). Design of Stress Release Support Mechanism for Large-Size Body-Mounted Radiator. Aerospace, 11(3), 202. https://doi.org/10.3390/aerospace11030202





