Analysis of Energy and Thermal Performance of High-Altitude Airship under Variable Attitude
Abstract
1. Introduction
2. Methodology
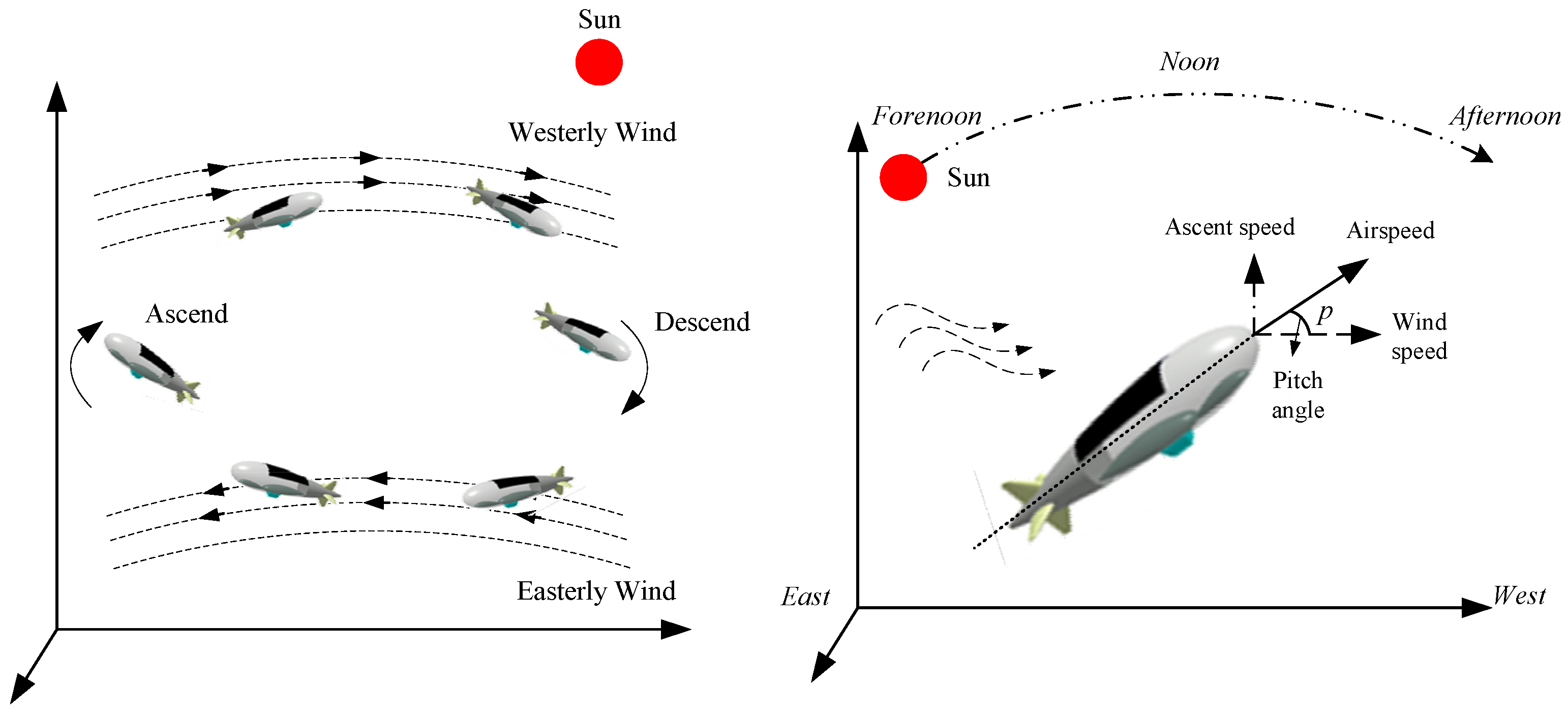
2.1. Working Mode Description
- The turbulent wind in the stratosphere is ignored, and it is assumed that the airship always flies stably.
- The yaw angle is always consistent with the direction of the wind during the flight of airships.
- The airship hull is airtight and has sufficient stiffness, so the shape and volume changes can be ignored.
- Since the wind in the vertical direction is small, the influence of the wind in the vertical direction is ignored.
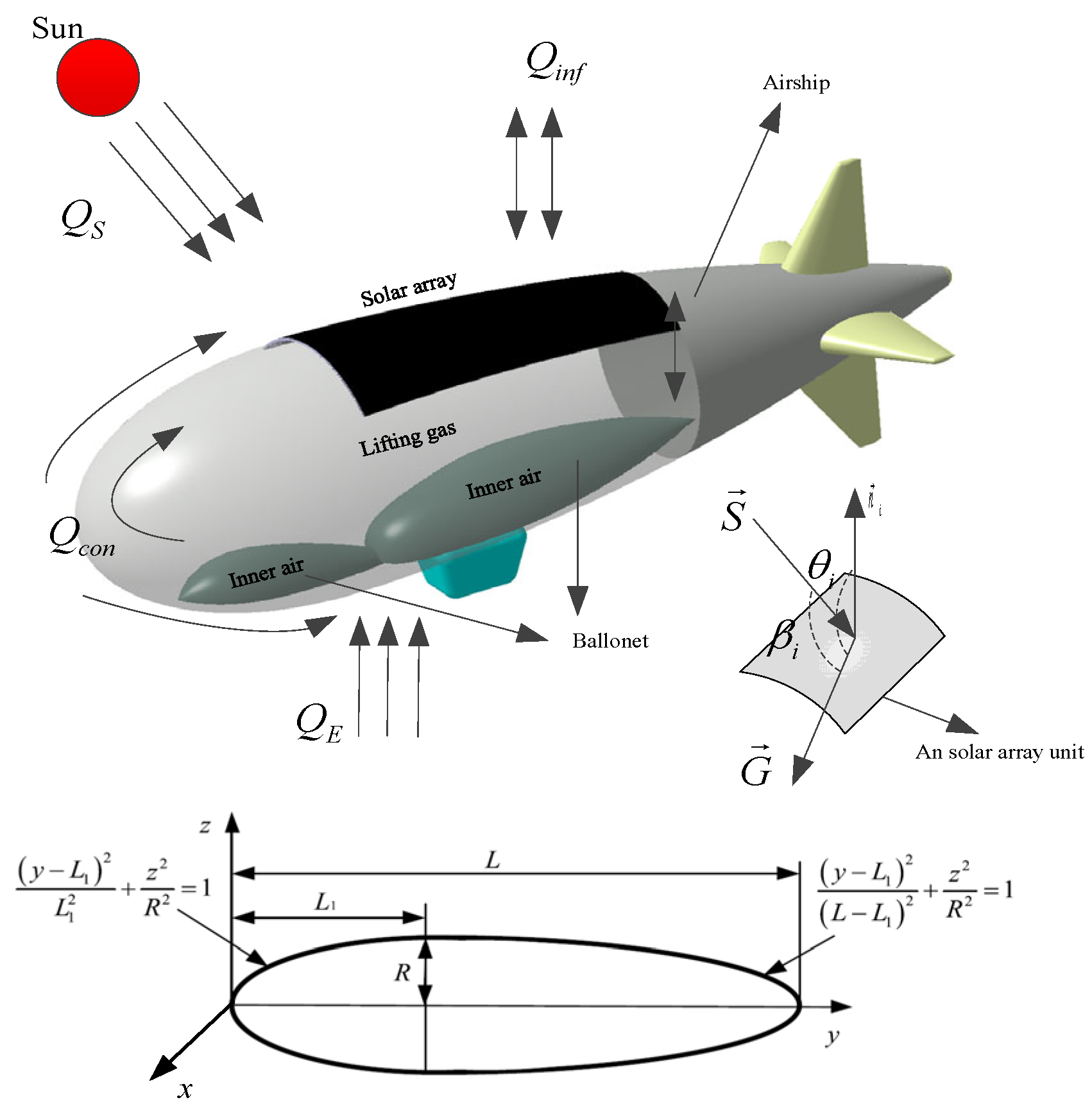
2.2. Solar Radiation Model
2.3. Thermal Model of the Airship’s Solar Cells
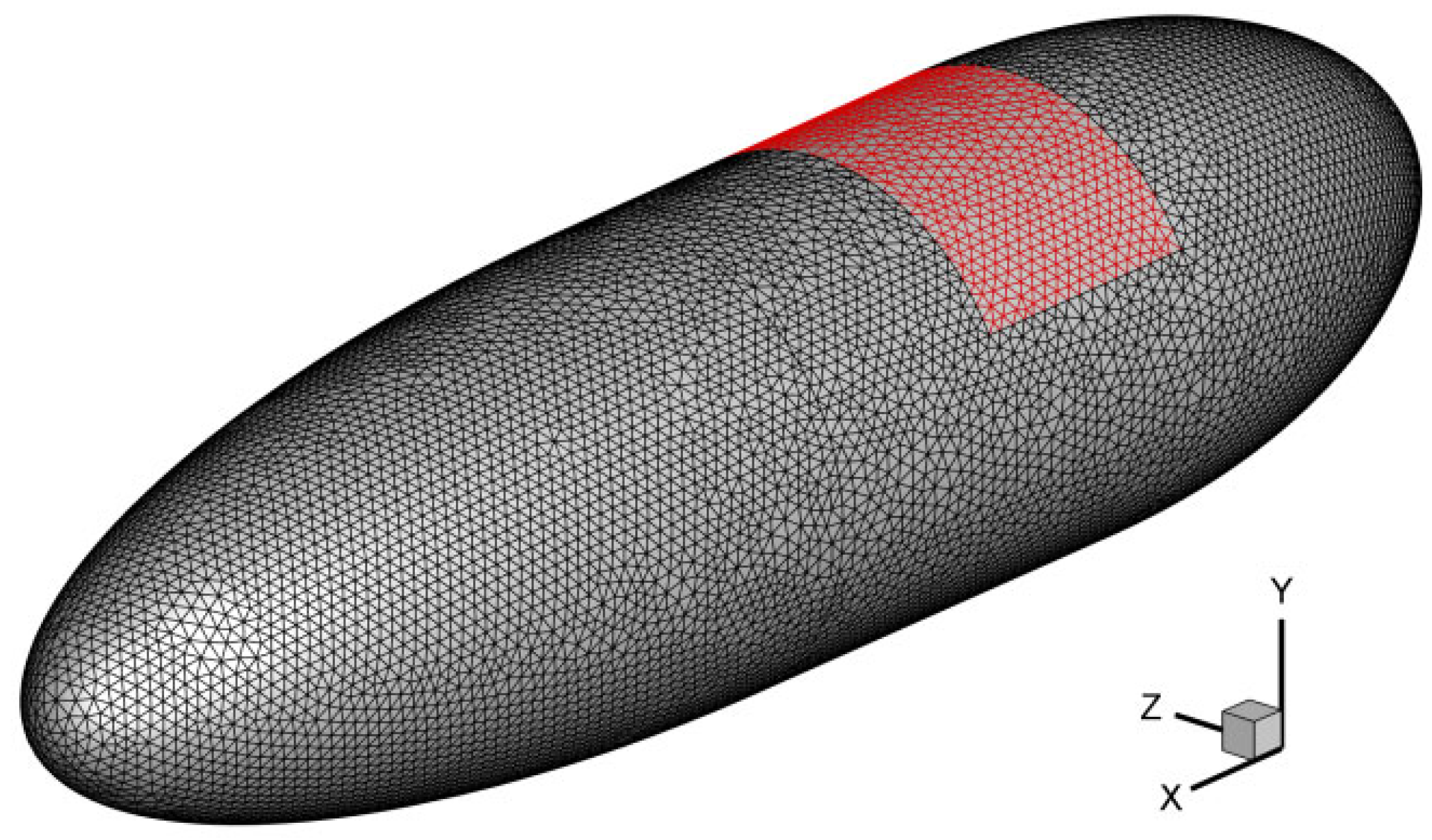
2.4. CFD Simulation Method
- (1)
- Mass and Momentum Conservation Equations:
- (2)
- Energy Conservation Equations:
- The initial velocity of the lifting gas inside the airship is zero.
- The initial temperatures of the solar panel, envelope, and lifting gas are all set to 300 K.
- The initial temperature of the atmosphere is set at 216.5 K.
2.5. Optimization Model of Pitch Angle
3. Result and Discussion
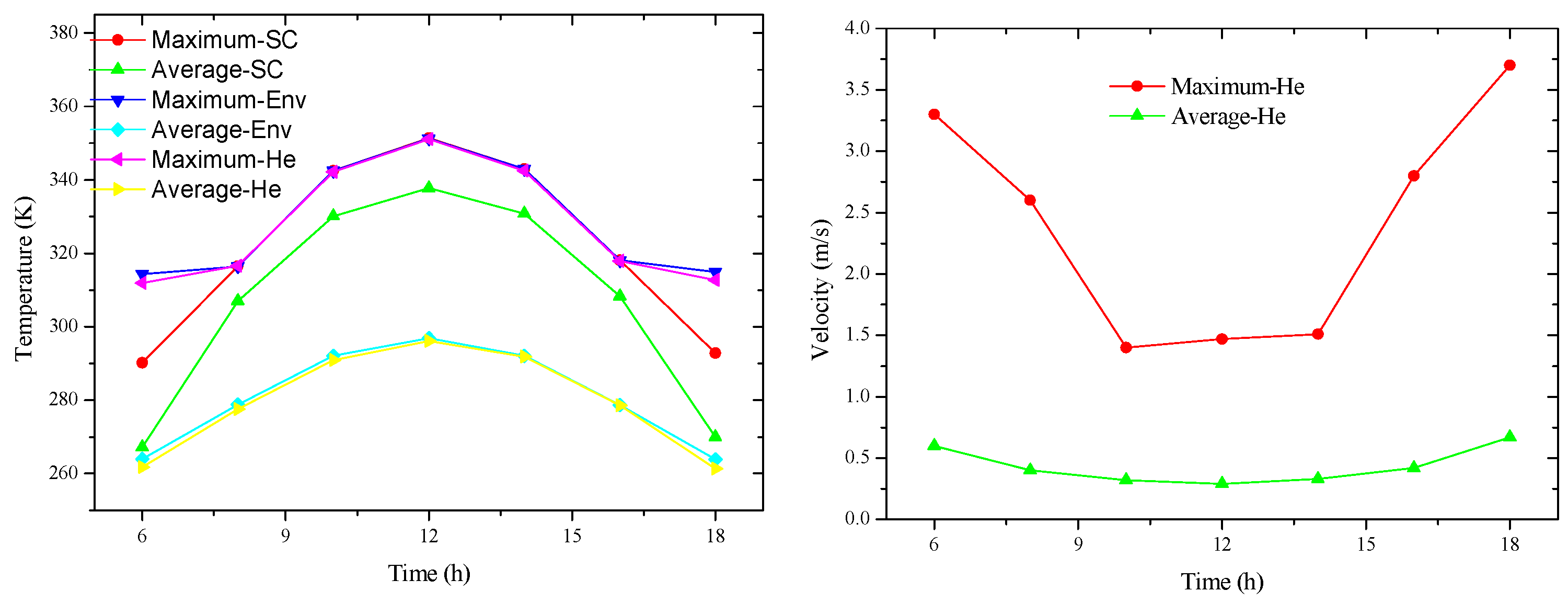
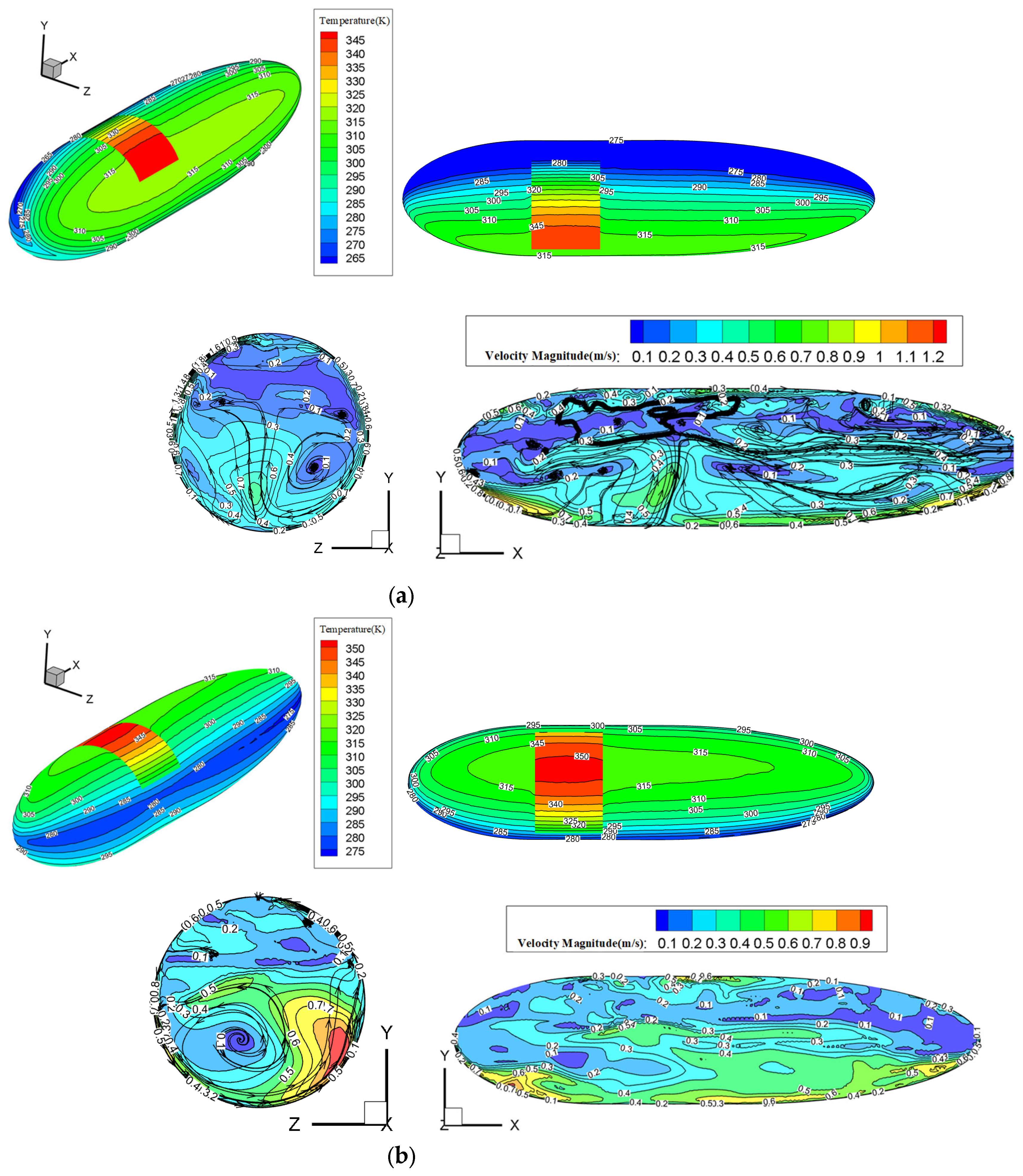
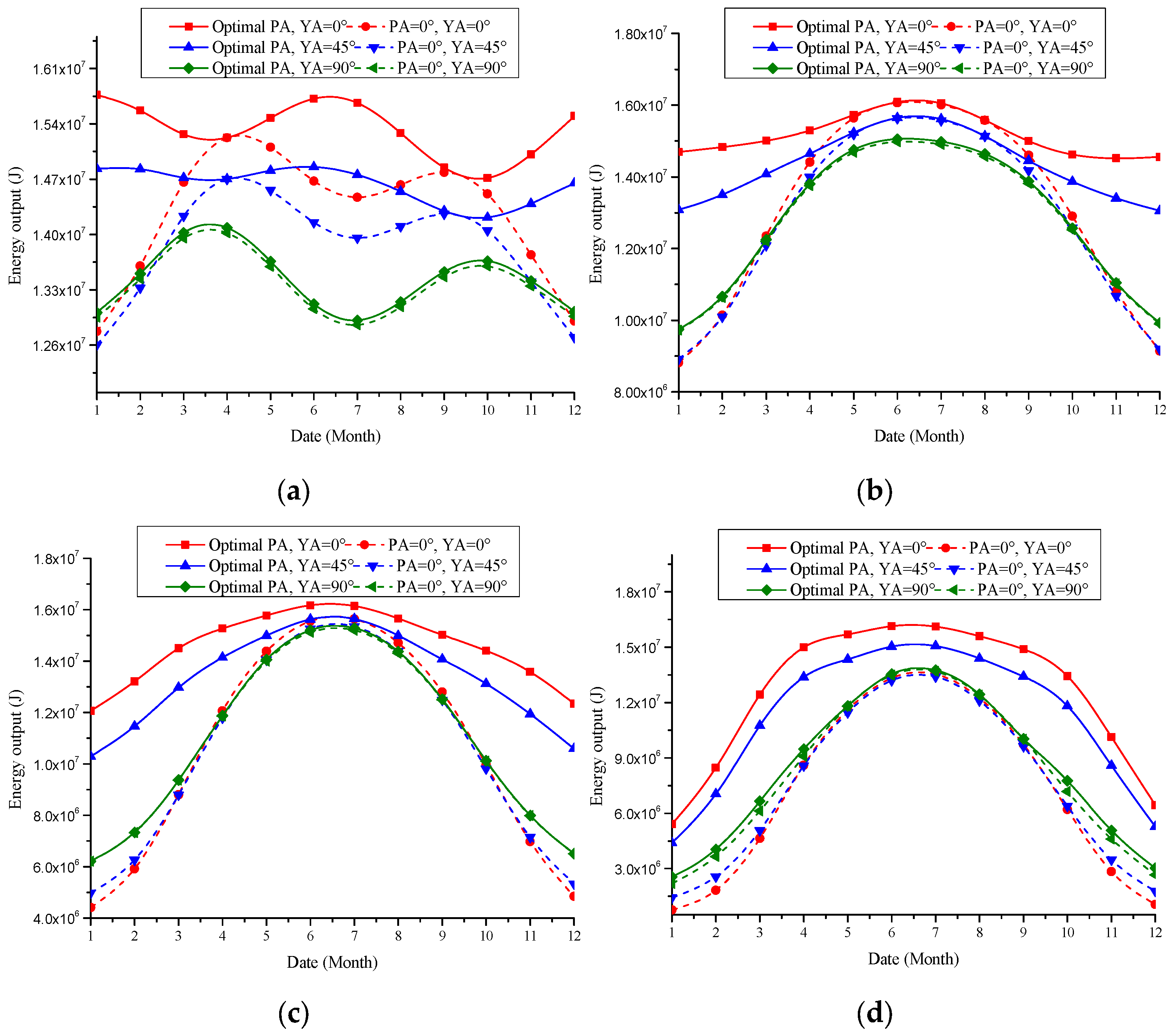
3.1. Effect of Airship Attitude on the Thermal Performance
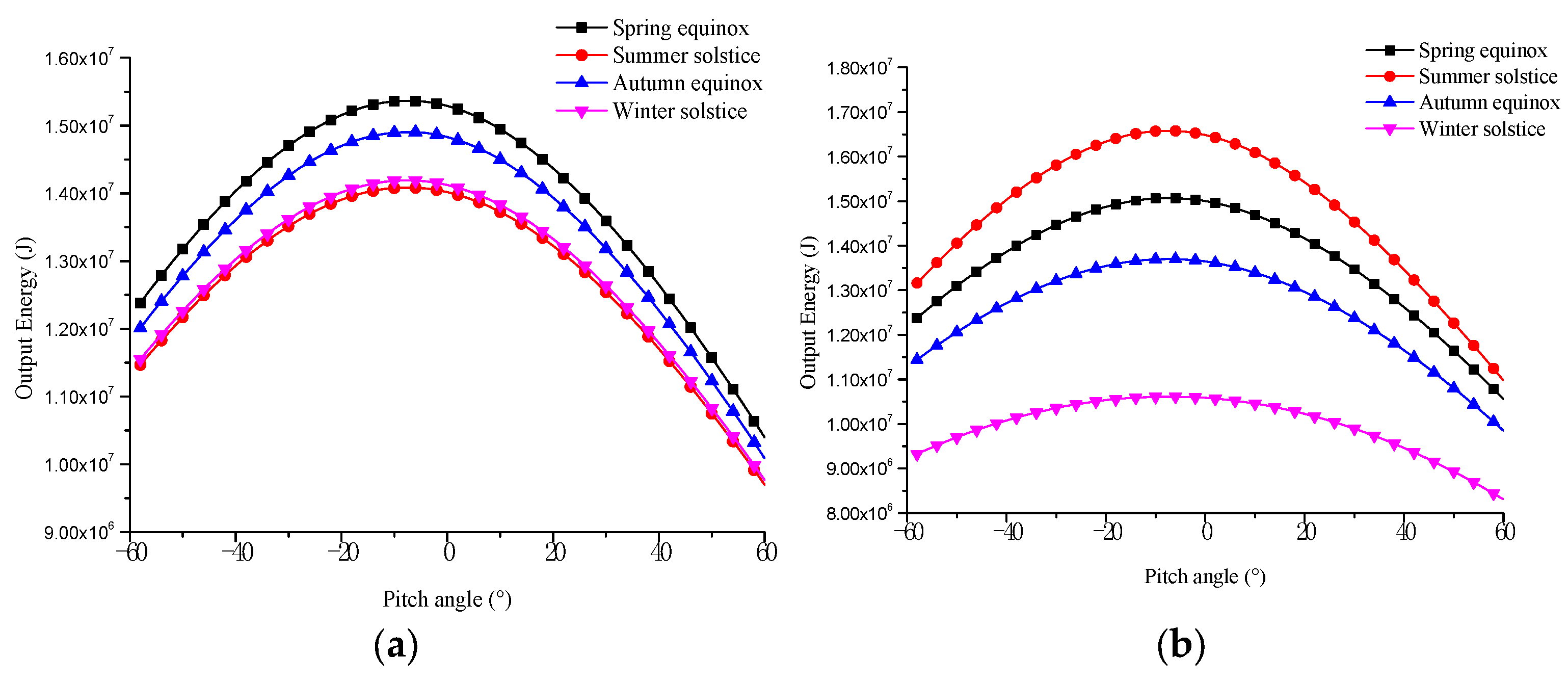
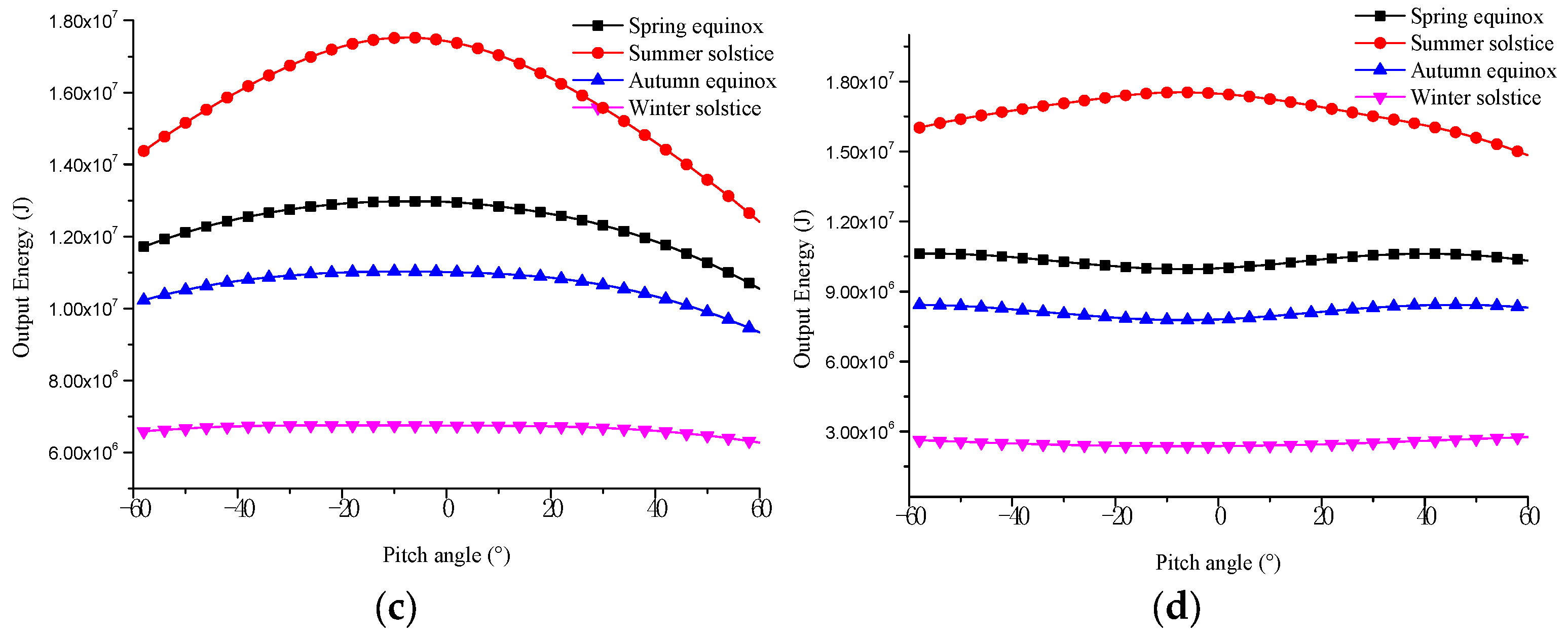
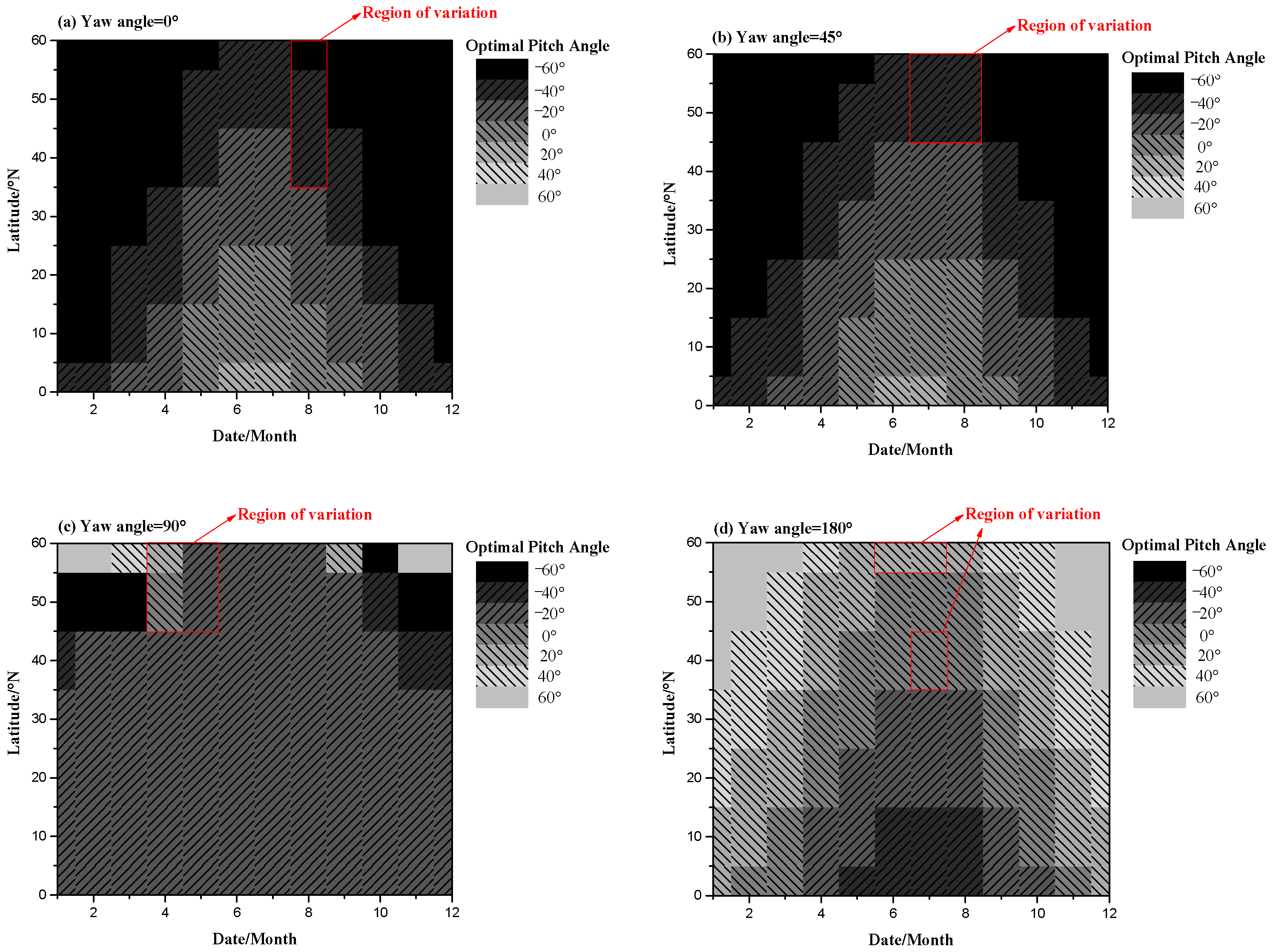
3.2. Optimal Pitch Angles
3.2.1. Disregarding Thermal Effects
3.2.2. Incorporating Thermal Effects
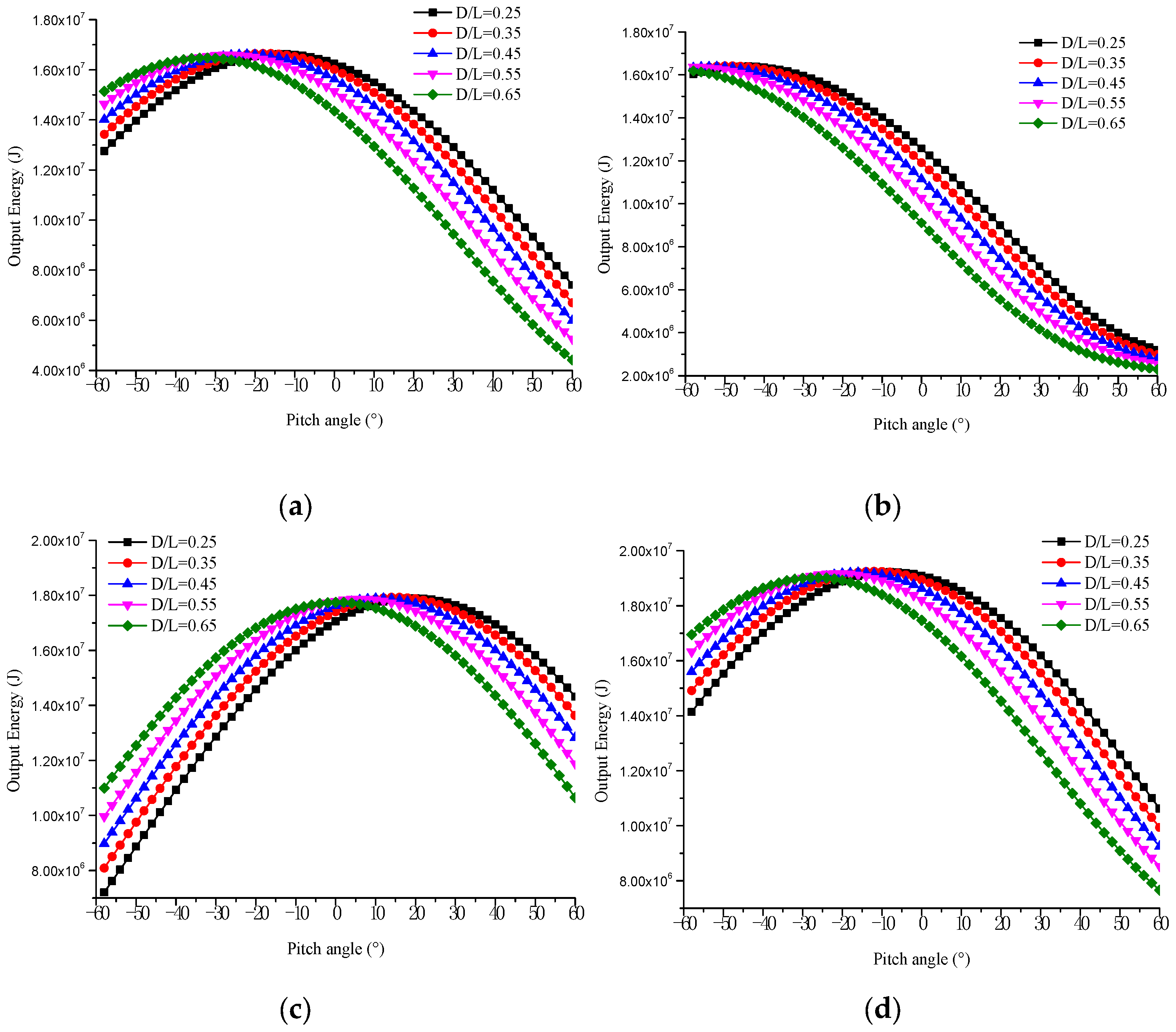
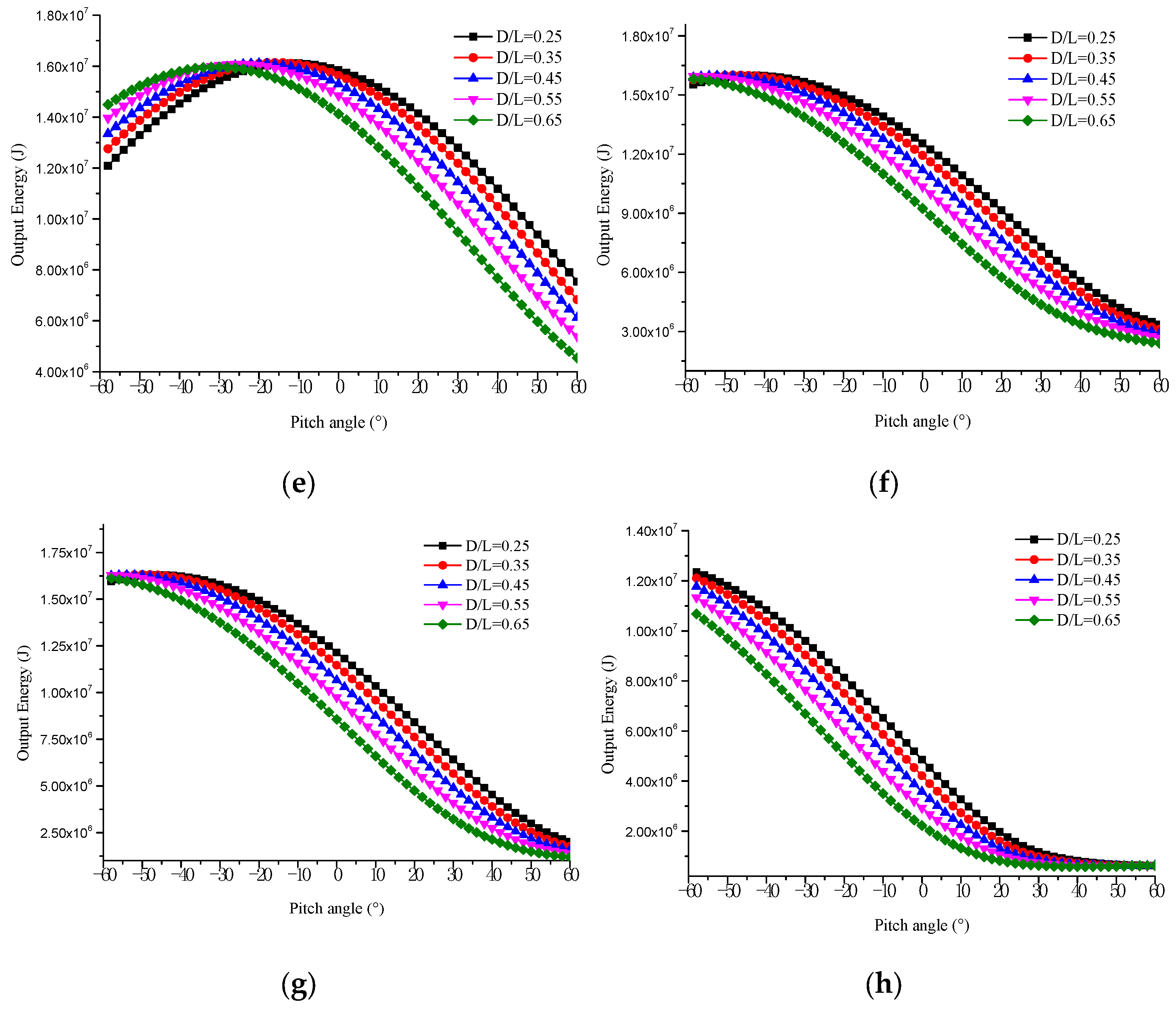
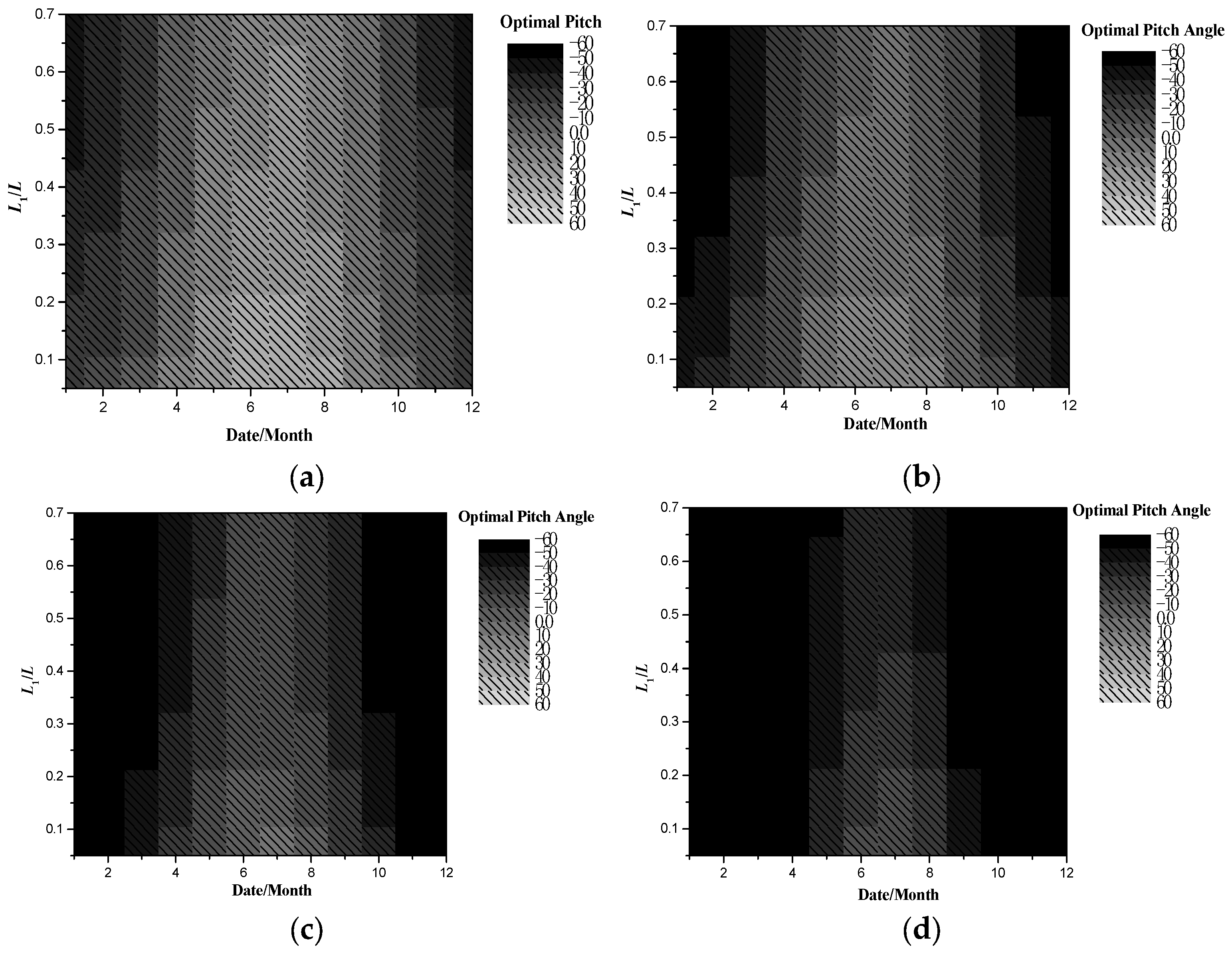
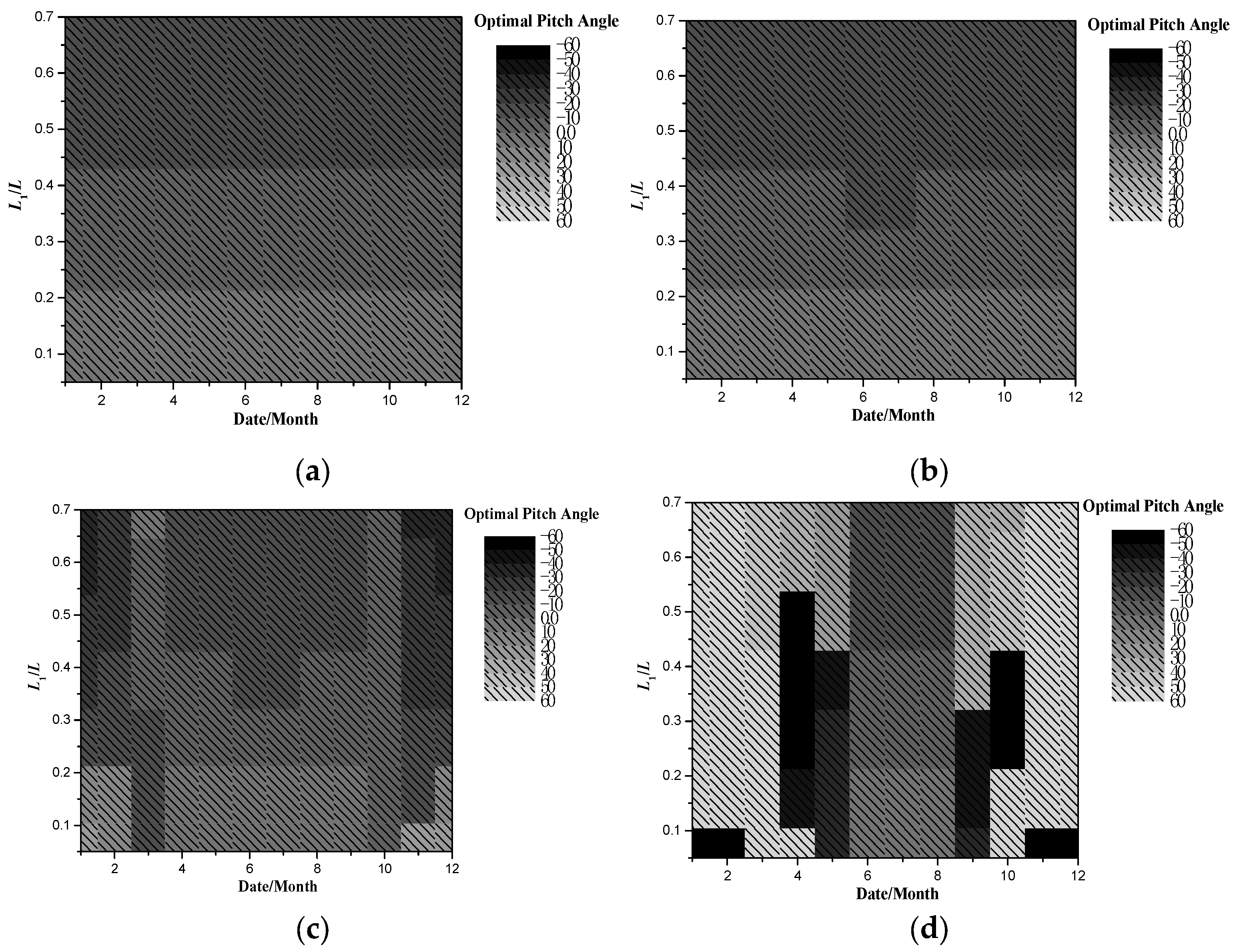
3.3. Influence Analysis of Airship Geometry Parameters
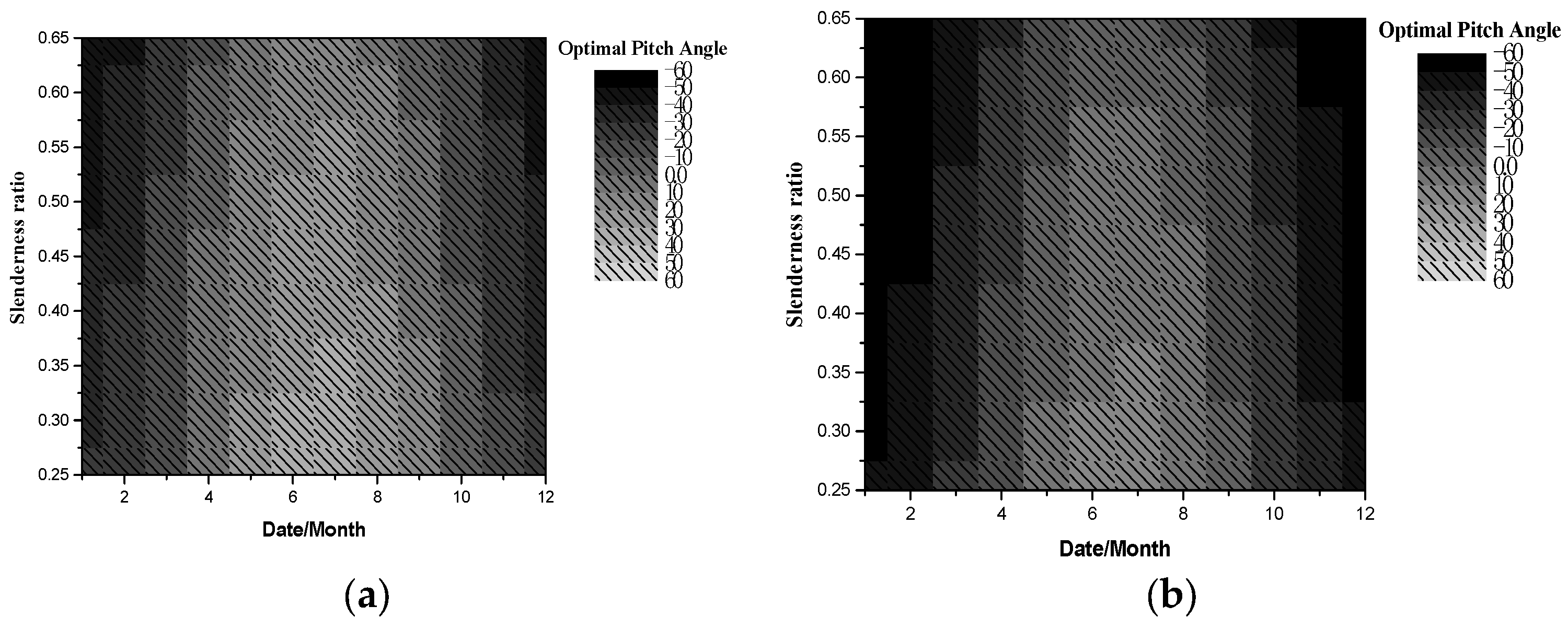
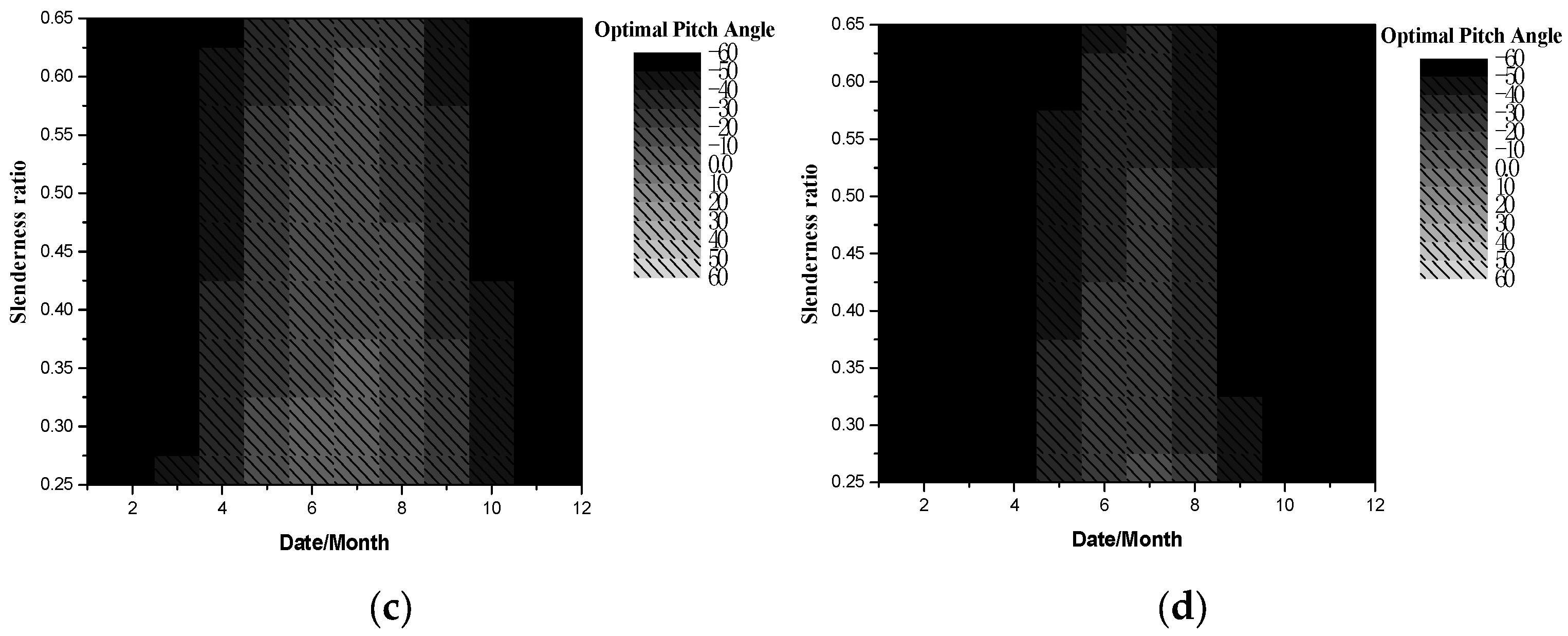
3.3.1. Slenderness Ratio
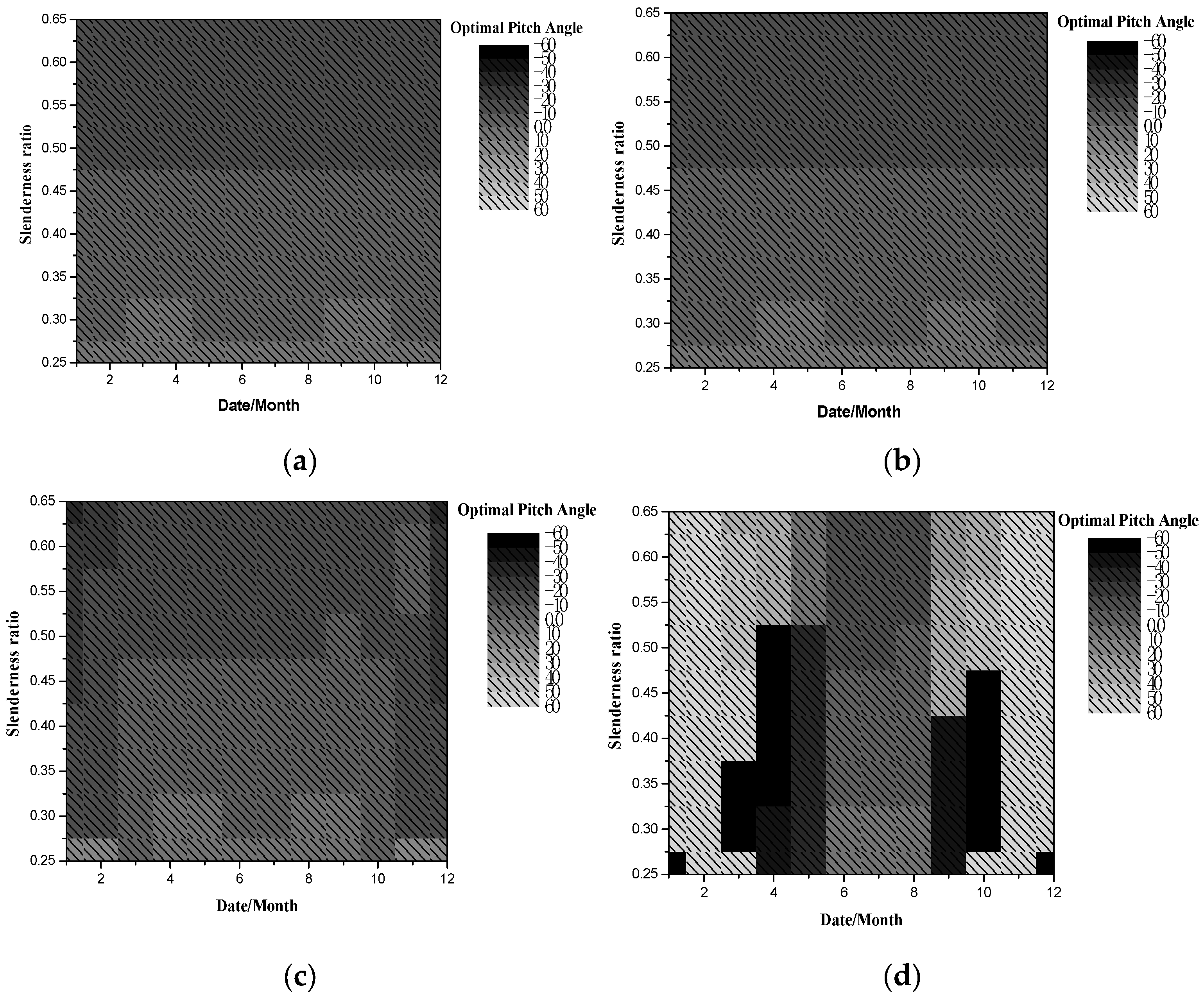
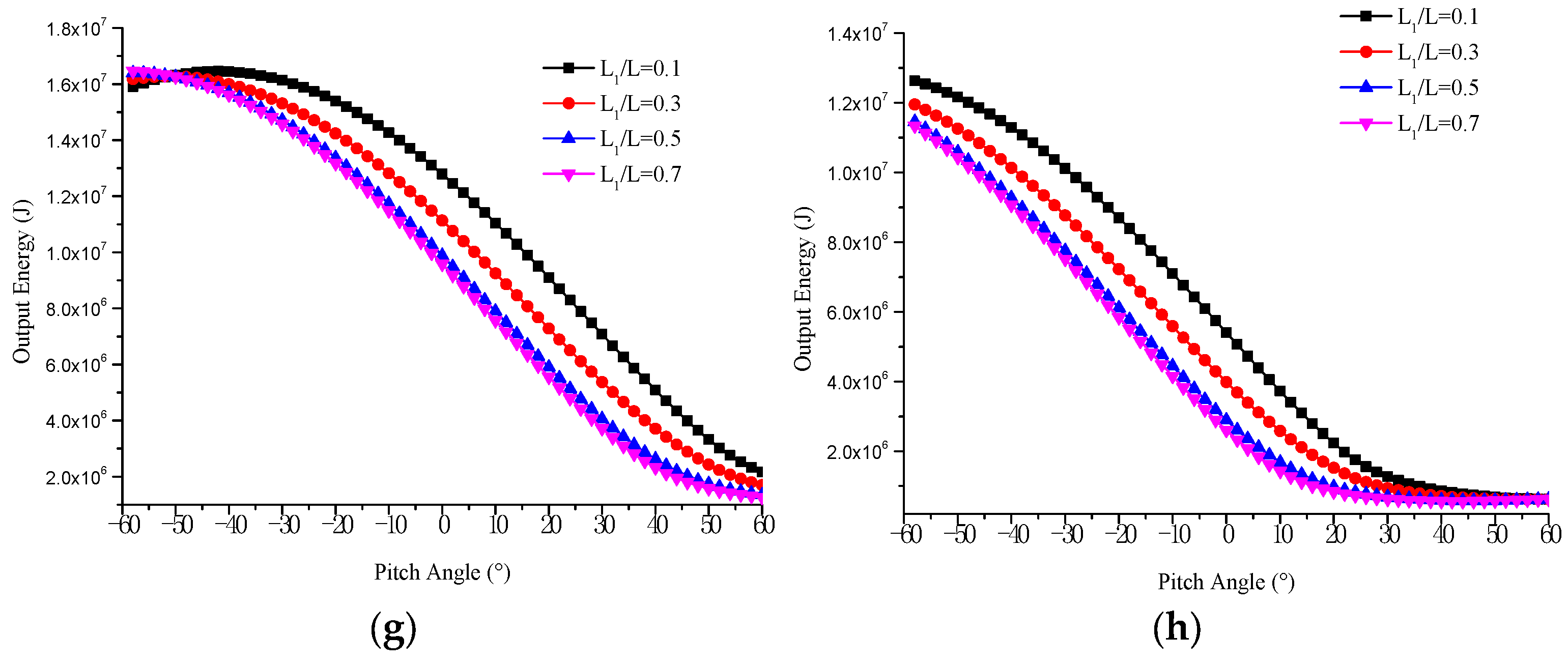
3.3.2. The Ratio of Forebody Length to Total Length (L1/L)
4. Conclusions
- (1)
- The pitch angle change will offset the maximum power output point in a day, so the output power may not be at its maximum when the noon solar radiation is at its maximum. This may change the strategy of selecting the solar array system to power the airship directly.
- (2)
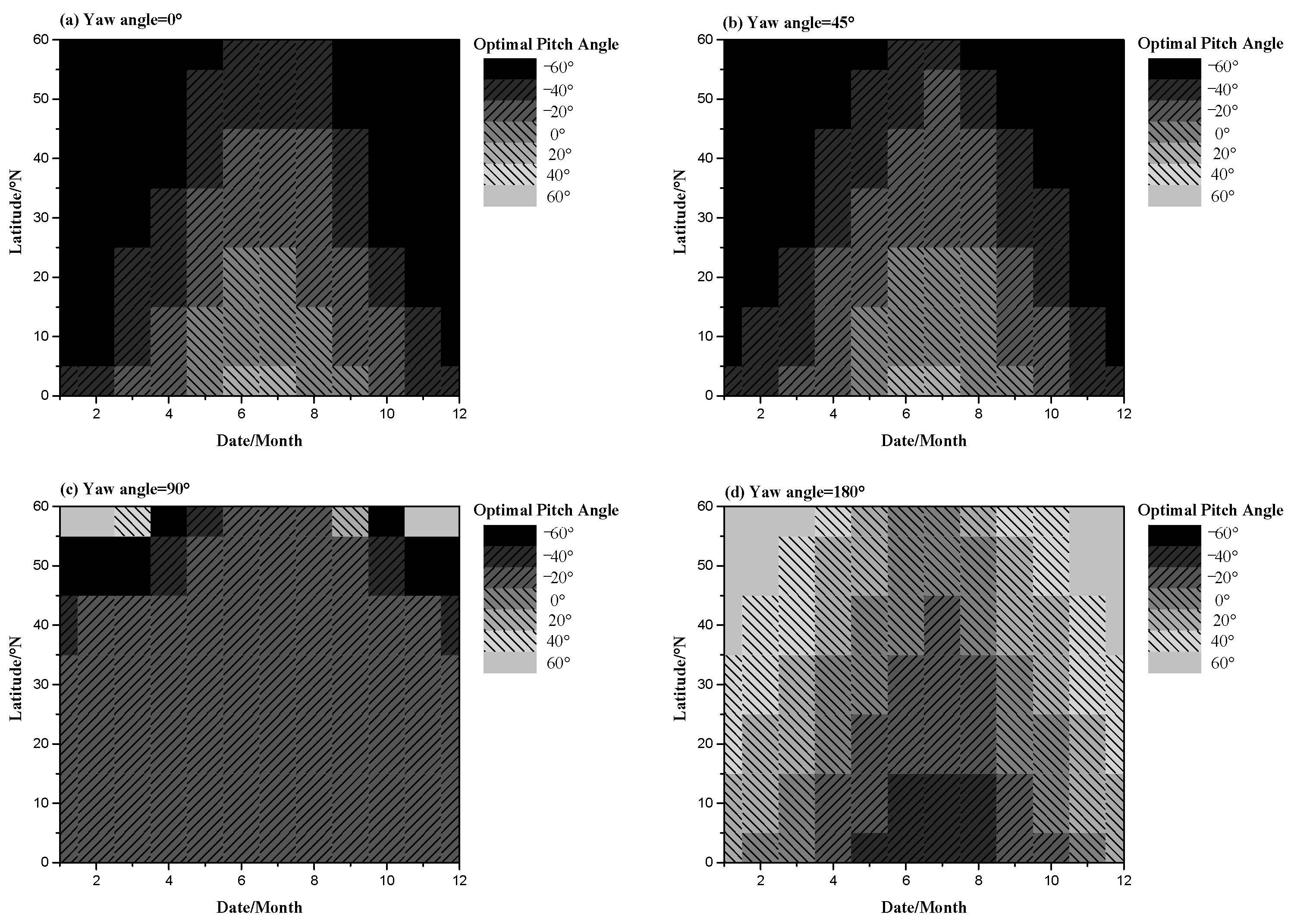
- In low-latitude summer, the solution of the optimal pitch angle needs to consider the thermal effect, while on other dates and latitudes the optimal pitch angle is almost fixed.
- (3)
- The optimal pitch angle of the airship varies significantly with yaw angles. When the yaw angle is 90° or 270° (heading west or east), the optimal pitch angle can be considered 0° at any latitude and date. The optimal pitch angle varies significantly with latitude and date at other yaw angles.
- (4)
- When the yaw angle of the airship is different, the shape parameters affect the optimal pitch angle differently. When the yaw angle is 0° or 180° (heading south or north), the influence is the greatest, and when the airship faces 90° (heading west or east), the effect is the least.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shi, H.; Song, B.; Yao, Q. Study of the Solar Power System of Stratospheric Airships. Chin. Space Sci. Technol. 2009, 1, 004. [Google Scholar]
- Manikandan, M.; Pant, R.S. Research and advancements in hybrid airships—A review. Prog. Aerosp. Sci. 2021, 100741. [Google Scholar] [CrossRef]
- Schmidt, D.K.; Stevens, J.; Roney, J. Near-Space Station-Keeping Performance of a Large High-Altitude Notional Airship. J. Aircr. 2007, 44, 611–615. [Google Scholar] [CrossRef]
- Wang, J.; Meng, X.; Li, C. Recovery trajectory optimization of the solar-powered stratospheric airship for the station-keeping mission. Acta Astronaut. 2021, 178, 159–177. [Google Scholar] [CrossRef]
- Shi, H.; Geng, S.; Qian, X. Thermodynamics analysis of a stratospheric airship with hovering capability. Appl. Therm. Eng. 2019, 146, 600–607. [Google Scholar] [CrossRef]
- Wang, J.; Meng, X.; Li, C.; Qiu, W. Analysis of long-endurance station-keeping flight scenarios for stratospheric airships in the presence of thermal effects. Adv. Space Res. 2021, 67, 4121–4141. [Google Scholar] [CrossRef]
- Shan, C.; Lv, M.; Sun, K.; Gao, J. Analysis of energy system configuration and energy balance for stratospheric airship based on position energy storage strategy. Aerosp. Sci. Technol. 2020, 101, 105844. [Google Scholar] [CrossRef]
- Du, H.; Li, J.; Zhu, W.; Qu, Z.; Zhang, L.; Lv, M. Flight performance simulation and station-keeping endurance analysis for stratospheric super-pressure balloon in real wind field. Aerosp. Sci. Technol. 2019, 86, 1–10. [Google Scholar] [CrossRef]
- Siyu, L.; Kangwen, S.; Jian, G.; Haoquan, L. Receiving energy analysis and optimal design of crystalline silicon solar cell array on solar airship. Energy 2023, 282, 128988. [Google Scholar] [CrossRef]
- Lv, M.; Li, J.; Du, H.; Zhu, W.; Meng, J. Solar array layout optimization for stratospheric airships using numerical method. Energy Convers. Manag. 2017, 135, 160–169. [Google Scholar] [CrossRef]
- Shan, C.; Sun, K.; Ji, X.; Cheng, D. A reconfiguration method for photovoltaic array of stratospheric airship based on multilevel optimization algorithm. Appl. Energy 2023, 352, 121881. [Google Scholar] [CrossRef]
- Tang, J.; Xie, W.; Zhou, P.; Yang, H.; Zhang, T.; Wang, Q. Multidisciplinary Optimization and Analysis of Stratospheric Airships Powered by Solar Arrays. Aerospace 2023, 10, 43. [Google Scholar] [CrossRef]
- Dai, Q.; Cao, L.; Zhang, G.; Fang, X. Thermal performance analysis of solar array for solar powered stratospheric airship. Appl. Therm. Eng. 2020, 171, 115077. [Google Scholar] [CrossRef]
- Liu, Q.; Yang, Y.; Wang, Q.; Cui, Y.; Cai, J. Icing performance of stratospheric airship in ascending process. Adv. Space Res. 2019, 64, 2405–2416. [Google Scholar] [CrossRef]
- Shi, H.; Song, B.; Yao, Q.; Cao, X. Thermal performance of stratospheric airships during ascent and descent. J. Thermophys. Heat Transf. 2009, 23, 816–821. [Google Scholar] [CrossRef]
- Yang, X.W.; Yang, X.X.; Deng, X.L. Horizontal trajectory control of stratospheric airships in wind field using Q-learning algorithm. Aerosp. Sci. Technol. 2020, 106, 106100. [Google Scholar] [CrossRef]
- Yang, X.; Liu, D. Renewable power system simulation and endurance analysis for stratospheric airships. Renew. Energy 2017, 113, 1070–1076. [Google Scholar] [CrossRef]
- Wu, J.; Fang, X.; Wang, Z.; Hou, Z.; Ma, Z.; Zhang, H.; Dai, Q.; Xu, Y. Thermal modeling of stratospheric airships. Prog. Aerosp. Sci. 2015, 75, 26–37. [Google Scholar] [CrossRef]
- Przenzak, E.; Szubel, M.; Filipowicz, M. The numerical model of the high temperature receiver for concentrated solar radiation. Energy Convers. Manag. 2016, 125, 97–106. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, Z.; Pan, H.; Salman, W.; Yuan, Y.; Liu, Y. A portable high-efficiency electromagnetic energy harvesting system using supercapacitors for renewable energy applications in railroads. Energy Convers. Manag. 2016, 118, 287–294. [Google Scholar] [CrossRef]
- Ran, H.; Thomas, R.; Mavris, D. A Comprehensive Global Model of Broadband Direct Solar Radiation for Solar Cell Simulation. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007. [Google Scholar]
- Dai, Q.; Fang, X.; Li, X.; Tian, L. Performance simulation of high altitude scientific balloons. Adv. Space Res. 2012, 49, 1045–1052. [Google Scholar] [CrossRef]
- Li, X.; Fang, X.; Dai, Q. Research on thermal characteristics of photovoltaic array of stratospheric airship. J. Aircr. 2011, 48, 1380–1386. [Google Scholar] [CrossRef]
- Du, H.; Li, J.; Zhu, W.; Yao, Z.; Cui, E.; Lv, M. Thermal performance analysis and comparison of stratospheric airships with rotatable and fixed photovoltaic array. Energy Convers. Manag. 2018, 158, 373–386. [Google Scholar] [CrossRef]
- Long, Y.; Wang, L.; Cappelleri, D.J. Modeling and global trajectory tracking control for an over-actuated MAV. Adv. Robot. 2014, 28, 145–155. [Google Scholar] [CrossRef]
- Incropera, F.P. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 1985; pp. 139–162. [Google Scholar]
- Xiong, J.; Bai, J.B.; Chen, L. Simplified analytical model for predicting the temperature of balloon on high-altitude. Int. J. Therm. Sci. 2014, 76, 82–89. [Google Scholar] [CrossRef]
- Hughes, D.W. Atmospheric Physics. Nature 1976, 221, 981. [Google Scholar] [CrossRef]
- Colozza, A.J.; Dolce, J. Convective Array Cooling for a Solar Powered Aircraft. NASA/CR-2003-212084. Available online: https://ntrs.nasa.gov/citations/20030015690 (accessed on 7 September 2013).
- Zhu, W.; Xu, Y.; Du, H.; Li, J. Thermal performance of high-altitude solar powered scientific balloon. Renew. Energy 2019, 135, 1078–1096. [Google Scholar] [CrossRef]
- Lu, G.Y.; Wong, D.W. An adaptive inverse-distance weighting spatial interpolation technique. Comput. Geosci. 2008, 34, 1044–1055. [Google Scholar] [CrossRef]
- Jeong, D.I.; St-Hilaire, A.; Gratton, Y.; Bélanger, C.; Saad, C. A guideline to select an estimation model of daily global solar radiation between geostatistical interpolation and stochastic simulation approaches. Renew. Energy 2017, 103, 70–80. [Google Scholar] [CrossRef]
- Chung, T.J. Computational Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2002; p. 1036. [Google Scholar]
- Meng, J.; Yao, Z.; Du, H.; Lv, M. Thermal protection method of the solar array for stratospheric airships. Appl. Therm. Eng. 2017, 111, 802–810. [Google Scholar] [CrossRef]
- Liu, Q.; Yang, Y.; Cui, Y.; Cai, J. Thermal performance of stratospheric airship with photovoltaic array. Adv. Space Res. 2017, 59, 1486–1501. [Google Scholar] [CrossRef]



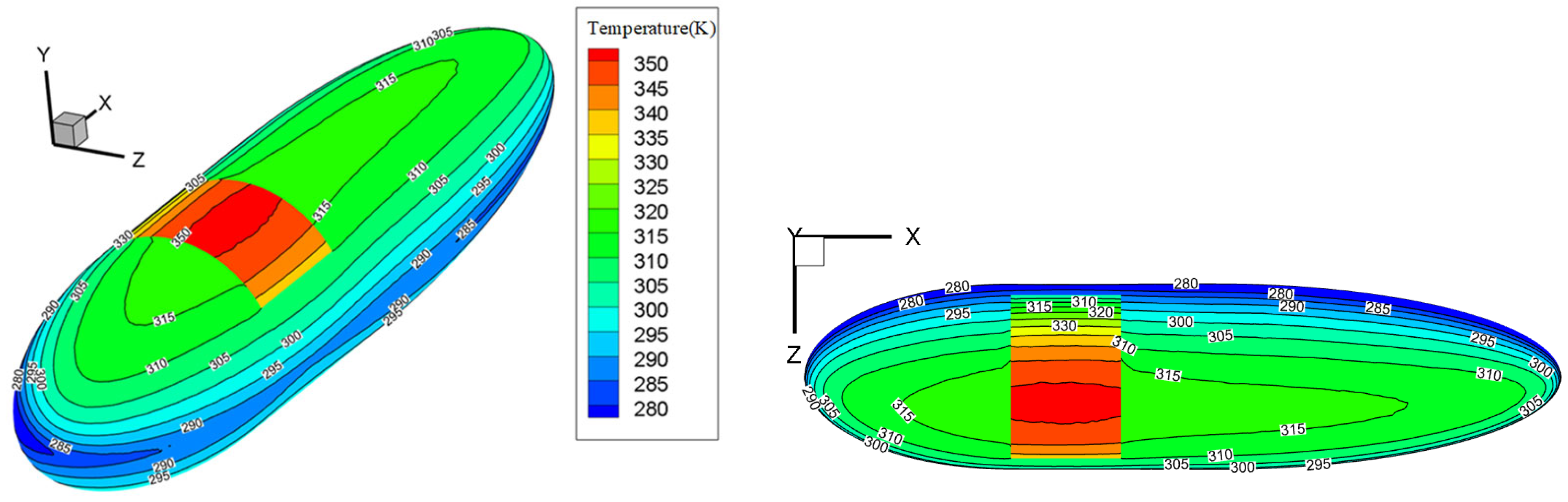

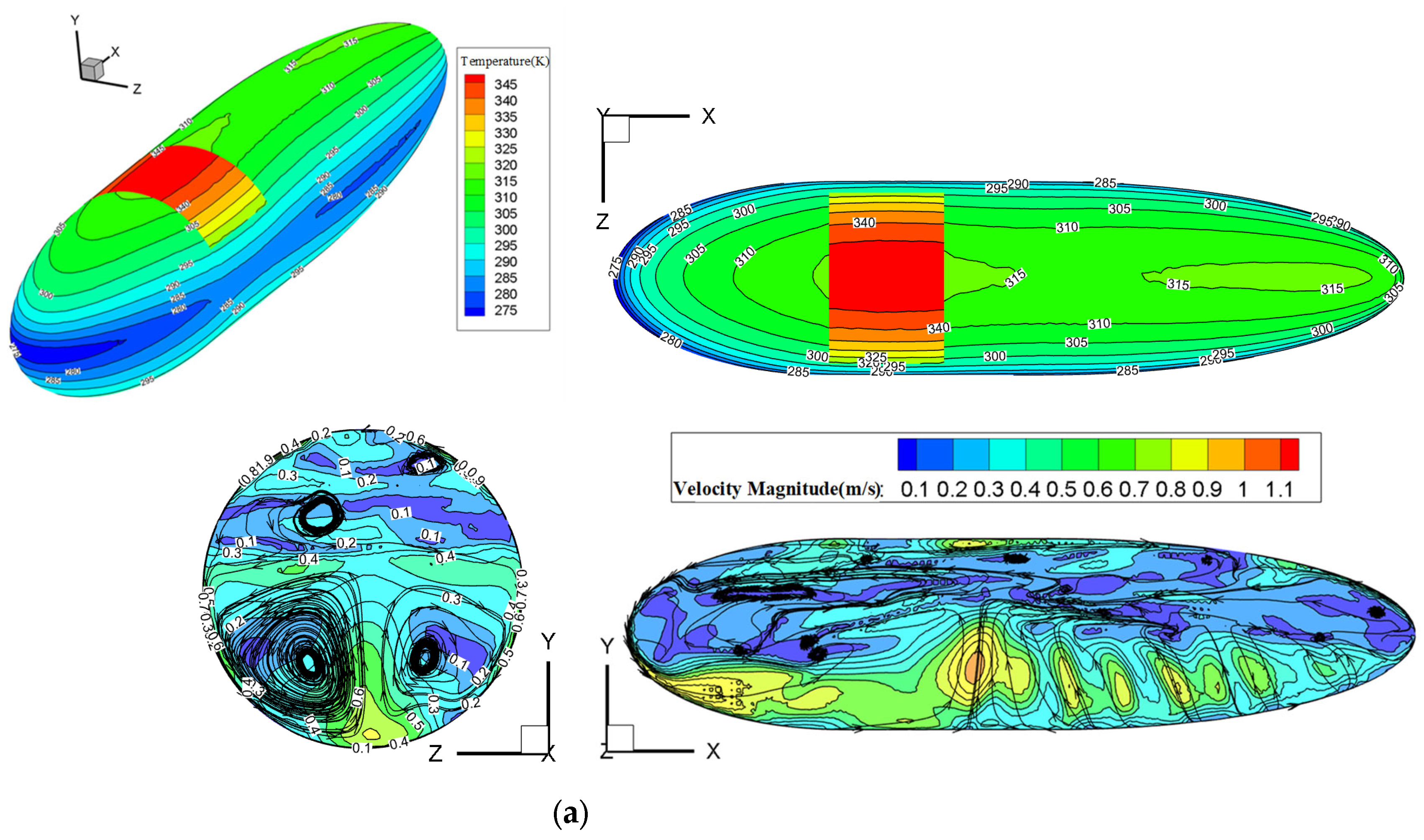
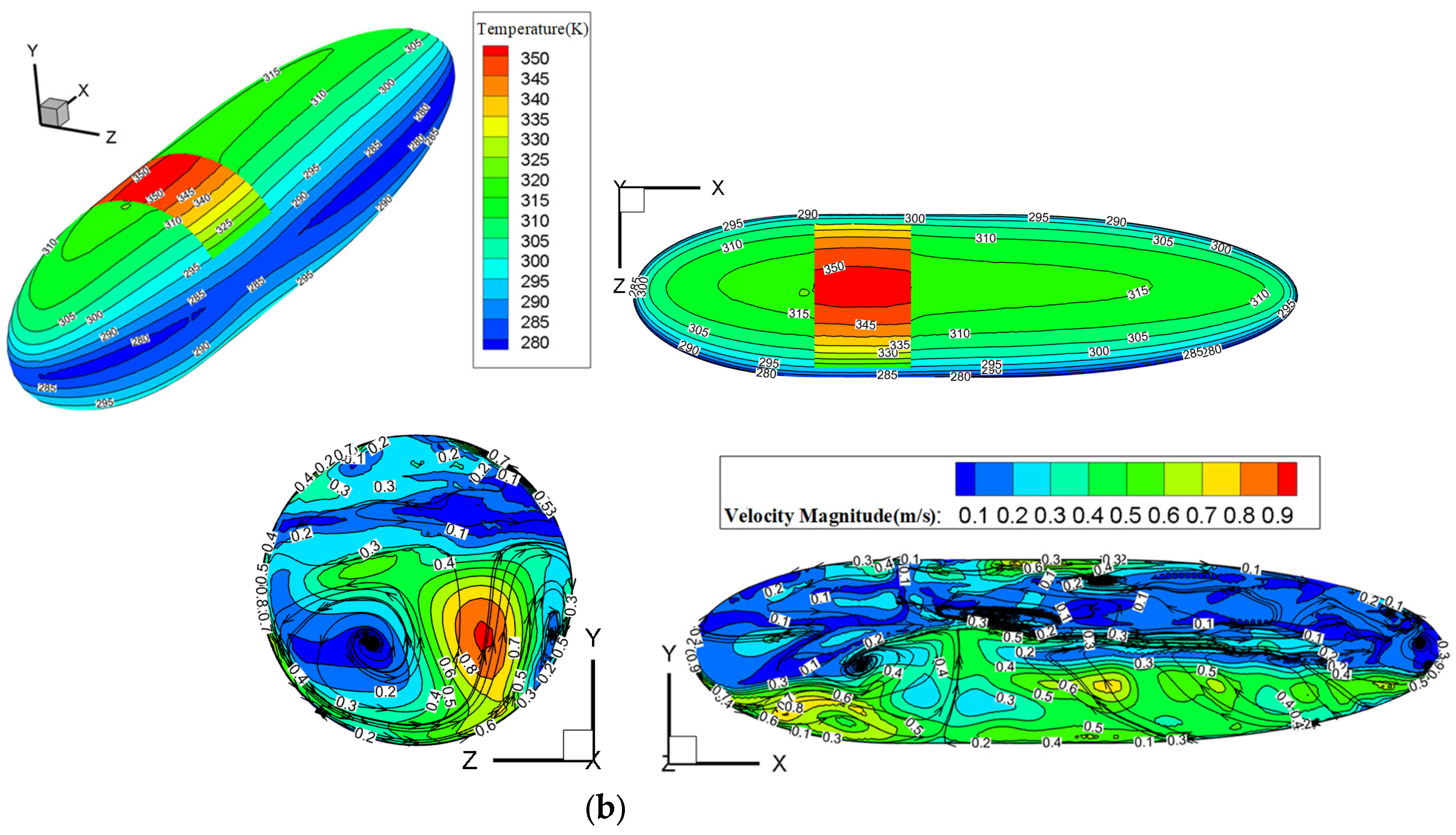
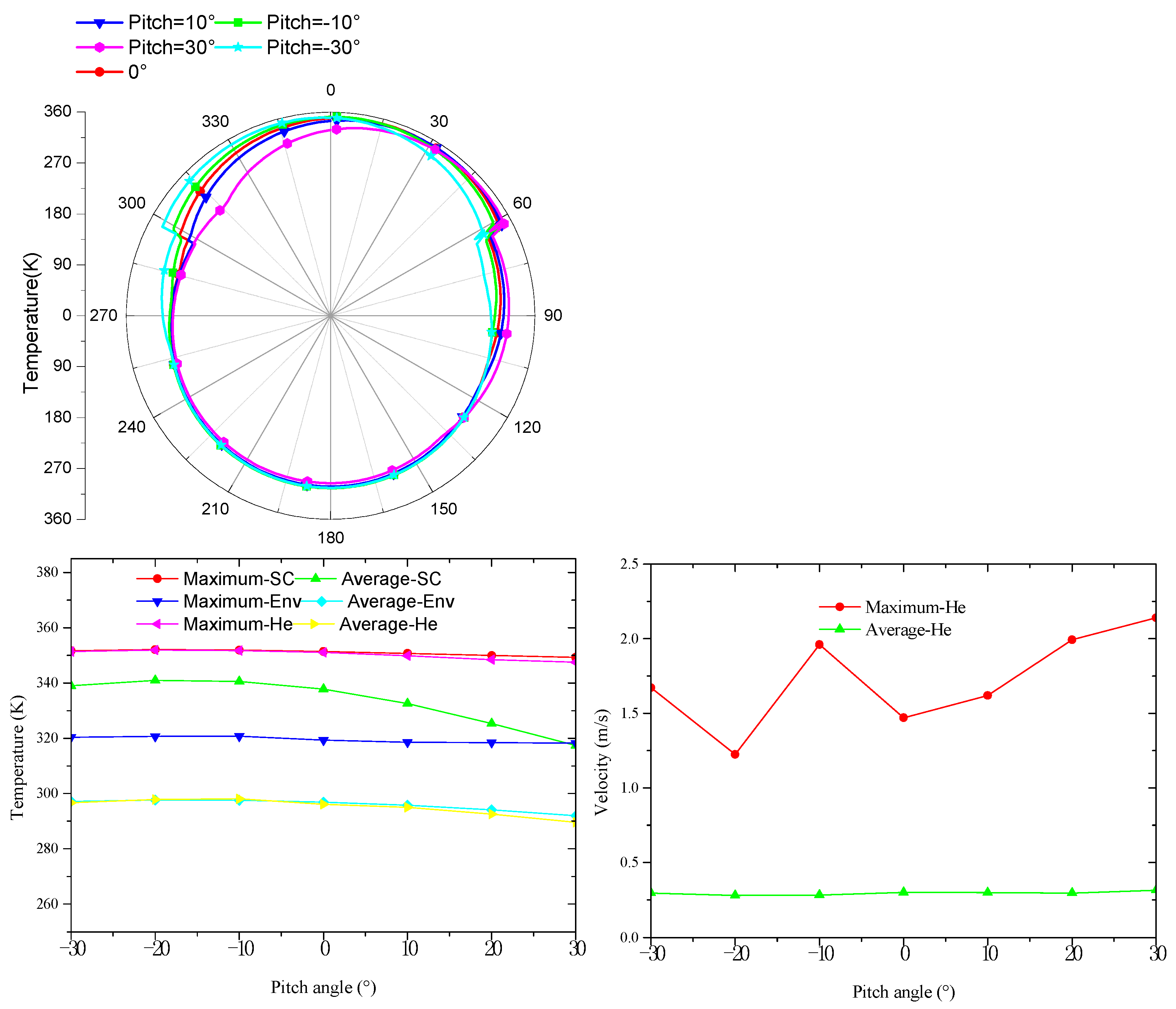




| Airship | Solar Panel | Environment | |||
|---|---|---|---|---|---|
| Absorptivity of envelope | 0.33 | Absorptivity | 0.93 | Environment temperature, K | 216.5 |
| Emissivity of envelope | 0.8 | Emissivity | 0.9 | Initial temperature, K | 220 |
| Thickness | 0.2 mm | Thickness | 0.2 mm | Location | 40° N, 116° E |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Length, m | 220 | Volume, m3 | 380,000 |
| Operating altitude, m | 19,000~23,000 | The central angle of the solar array, , ° | 120 |
| Operating latitude, ° | 0~60N | The leading edge of the solar array, yL, m | 30 |
| Operating pitch angle range, ° | −60~60 | Solar panel area, m2 | 8000 |
| Diameter, m | 100 | Slenderness ratio, 2R/L | 0.45 |
| Area, m2 | 33,000 | The ratio of forebody length to the total length, L1/L | 0.36 |
| Parameter | Optimization Variable: Pitch Angle | Constraints | ||
|---|---|---|---|---|
| Dates | Latitudes | Yaw Angle | ||
| Minimum | −60° | 1/1 | 0° N | 0° |
| Maximum | −60° | 12/31 | 60° N | 180° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Fu, H.; Zhu, W.; Yang, K.; Xu, Y. Analysis of Energy and Thermal Performance of High-Altitude Airship under Variable Attitude. Aerospace 2024, 11, 109. https://doi.org/10.3390/aerospace11020109
Zhang B, Fu H, Zhu W, Yang K, Xu Y. Analysis of Energy and Thermal Performance of High-Altitude Airship under Variable Attitude. Aerospace. 2024; 11(2):109. https://doi.org/10.3390/aerospace11020109
Chicago/Turabian StyleZhang, Bangchu, Hao Fu, Weiyu Zhu, Kuijian Yang, and Yuanming Xu. 2024. "Analysis of Energy and Thermal Performance of High-Altitude Airship under Variable Attitude" Aerospace 11, no. 2: 109. https://doi.org/10.3390/aerospace11020109
APA StyleZhang, B., Fu, H., Zhu, W., Yang, K., & Xu, Y. (2024). Analysis of Energy and Thermal Performance of High-Altitude Airship under Variable Attitude. Aerospace, 11(2), 109. https://doi.org/10.3390/aerospace11020109







