Transient Energy Growth in a Free Cylindrical Liquid Jet
Abstract
1. Introduction
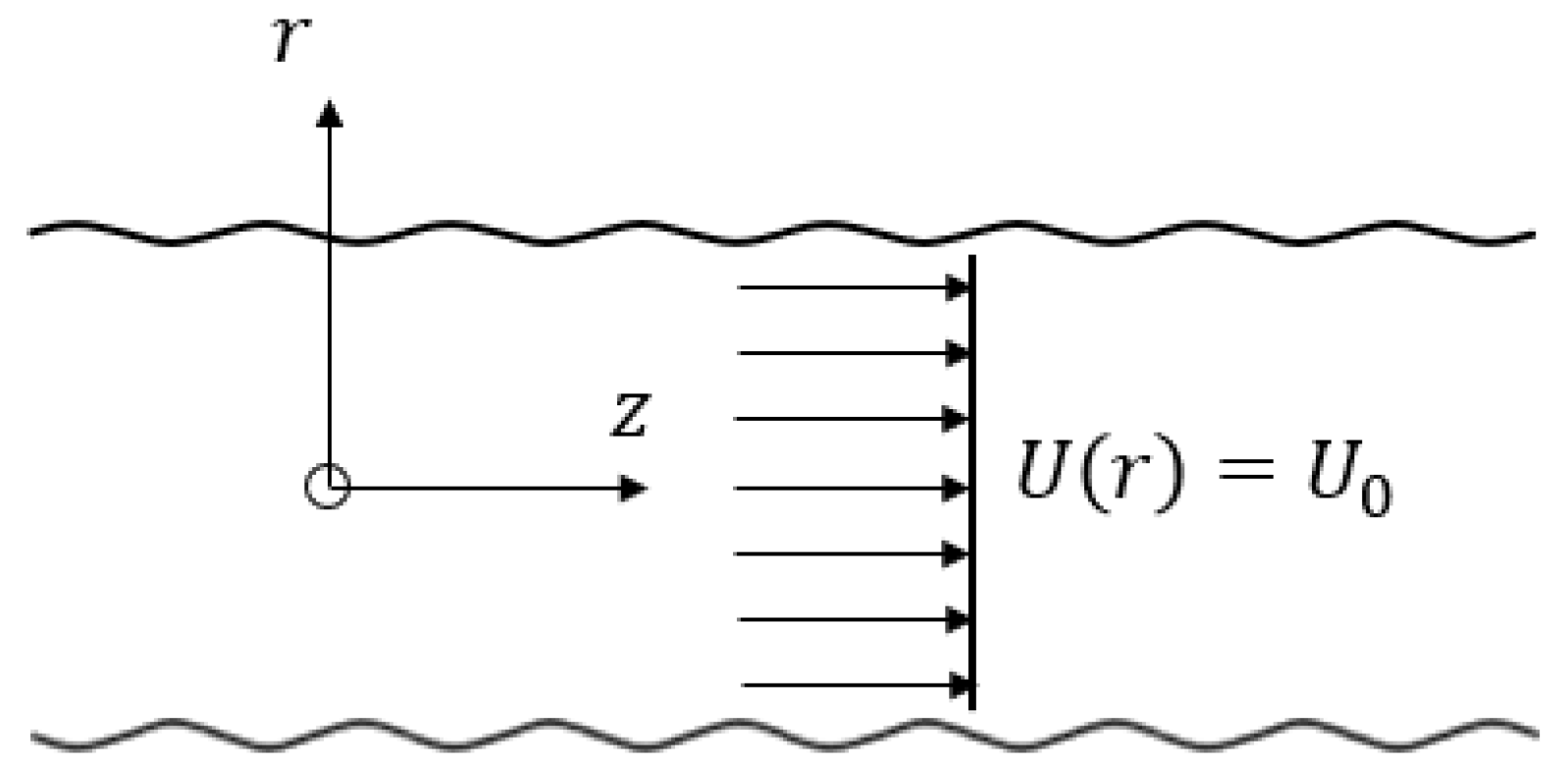
2. Method
2.1. Governing Equations
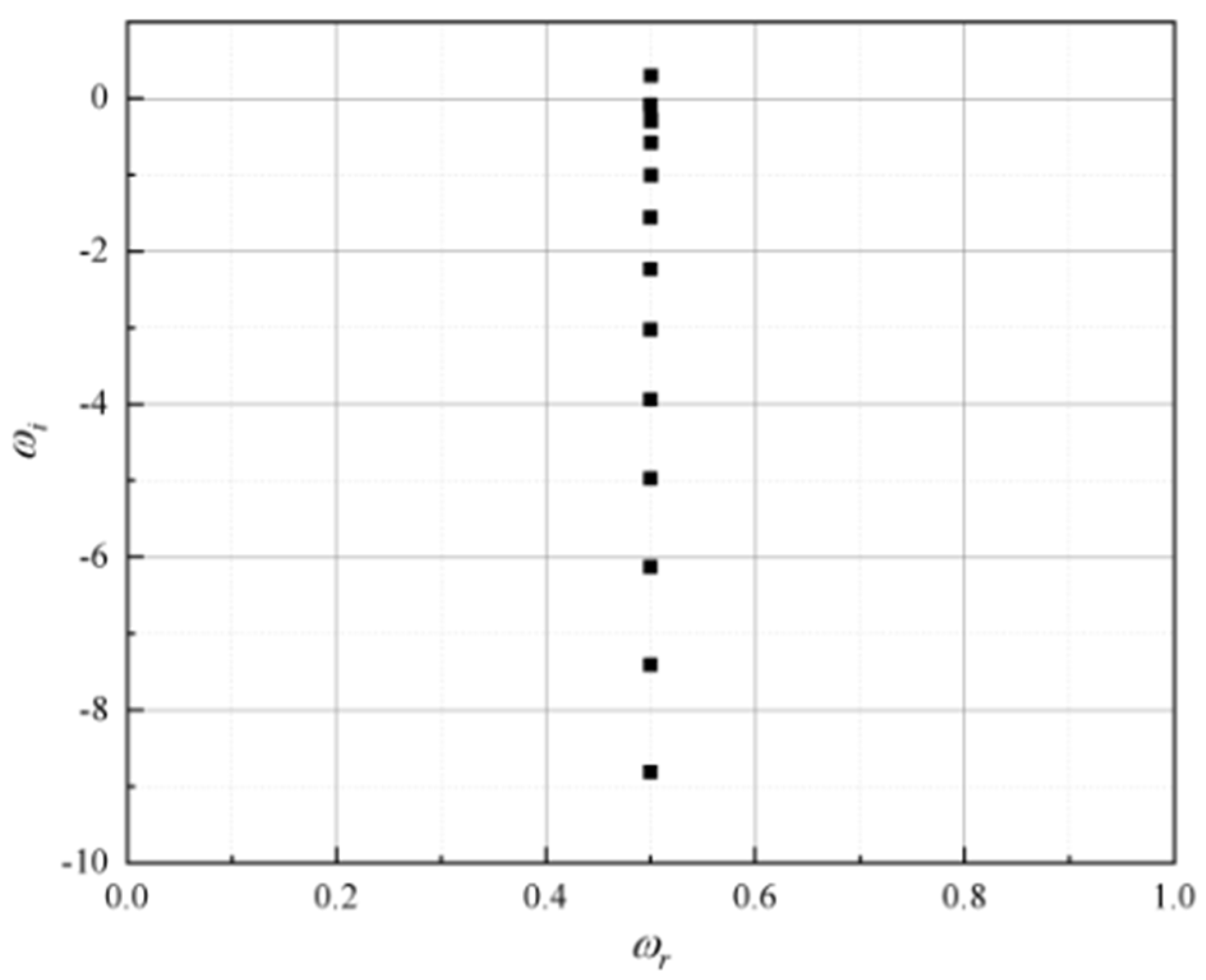
2.2. Modal Analysis
2.3. Non-Modal Analysis
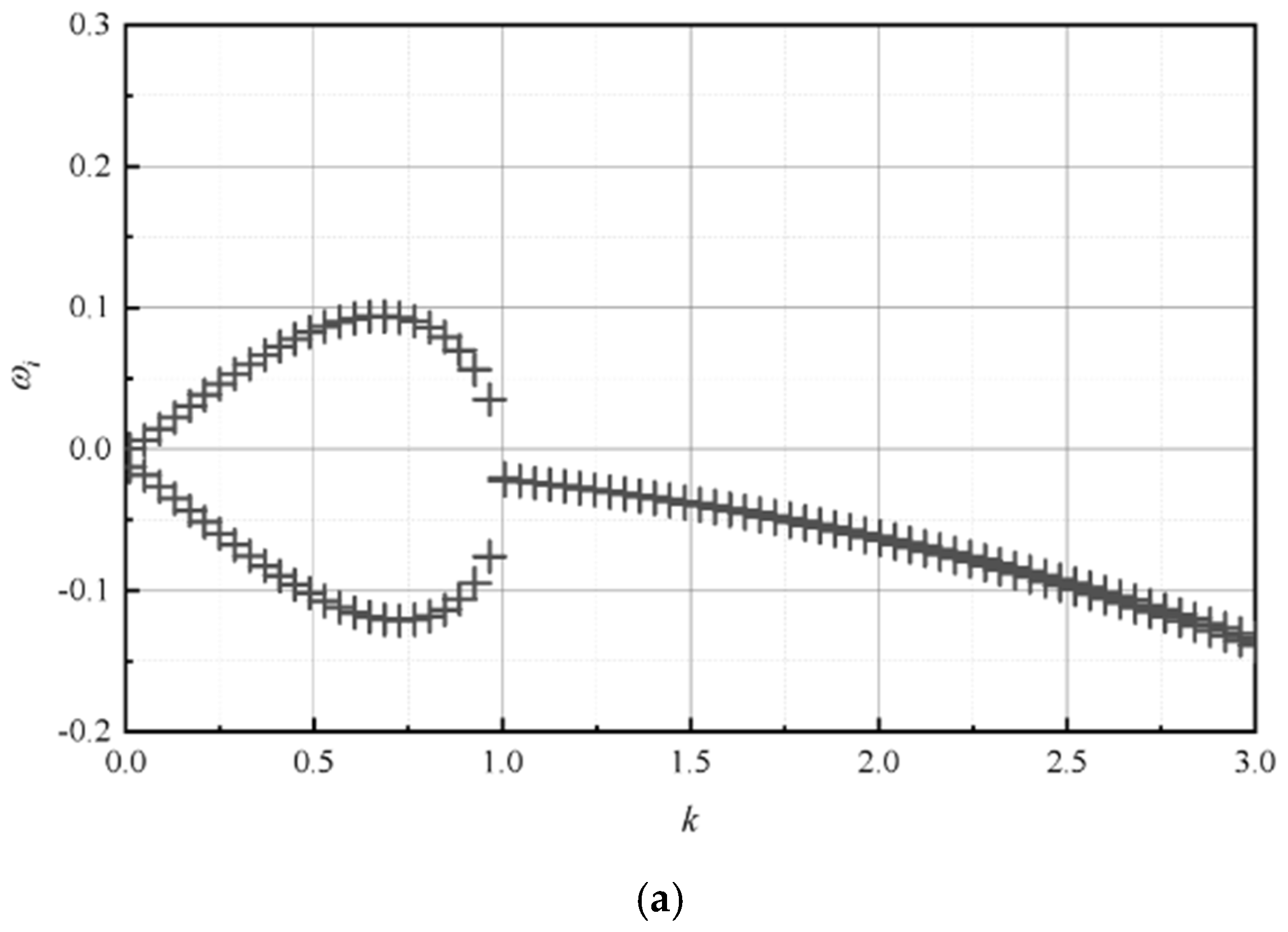
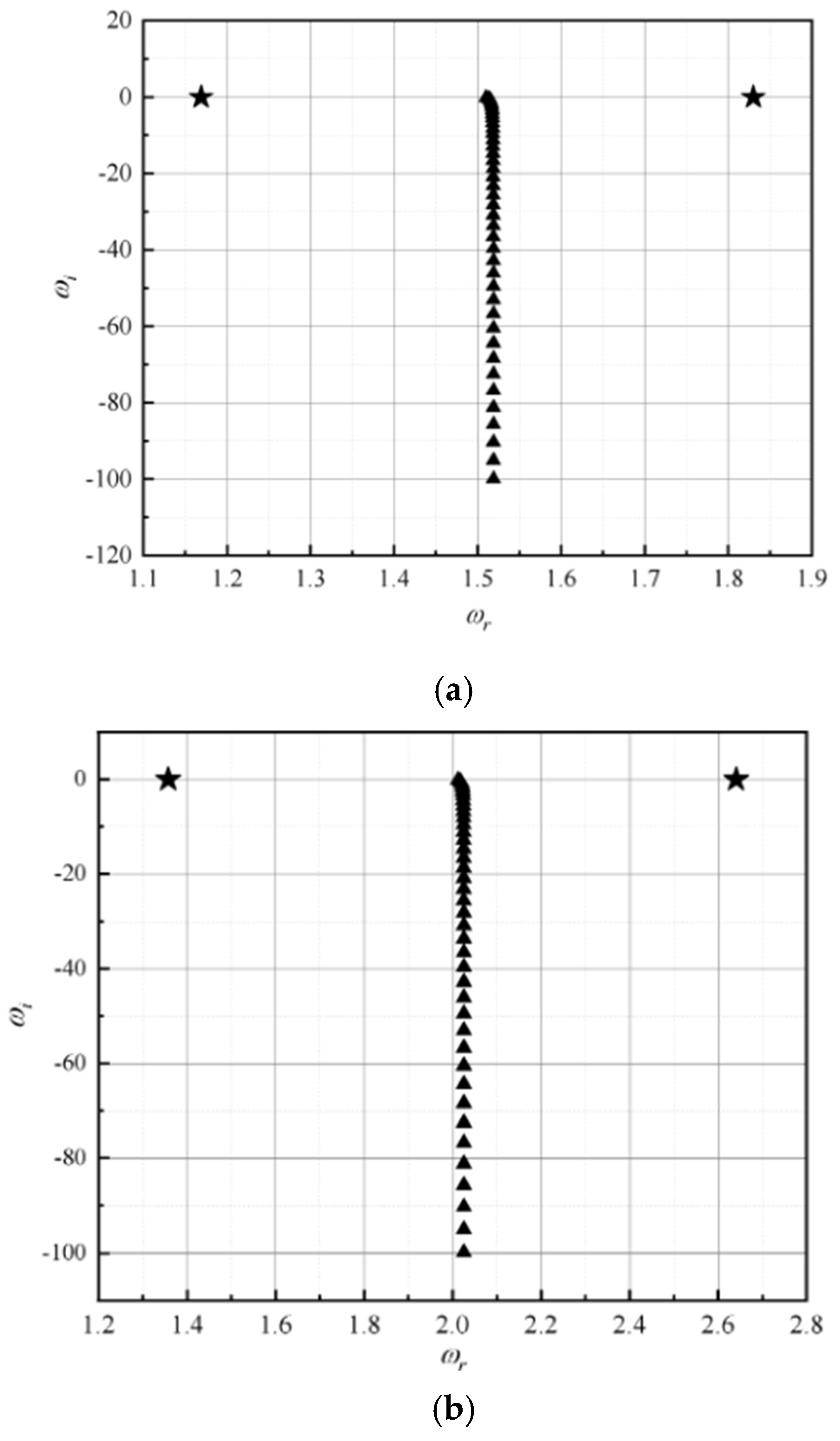
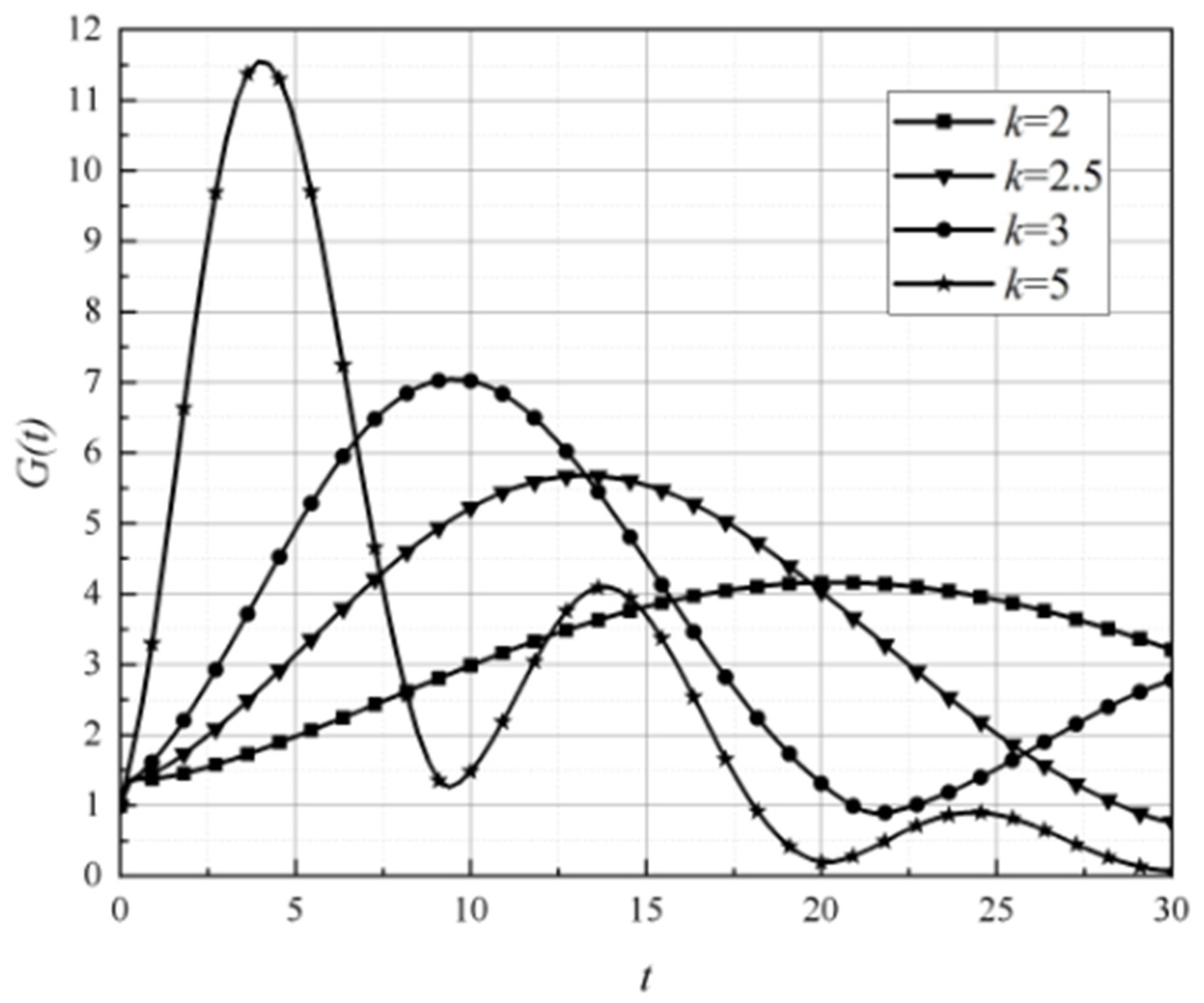
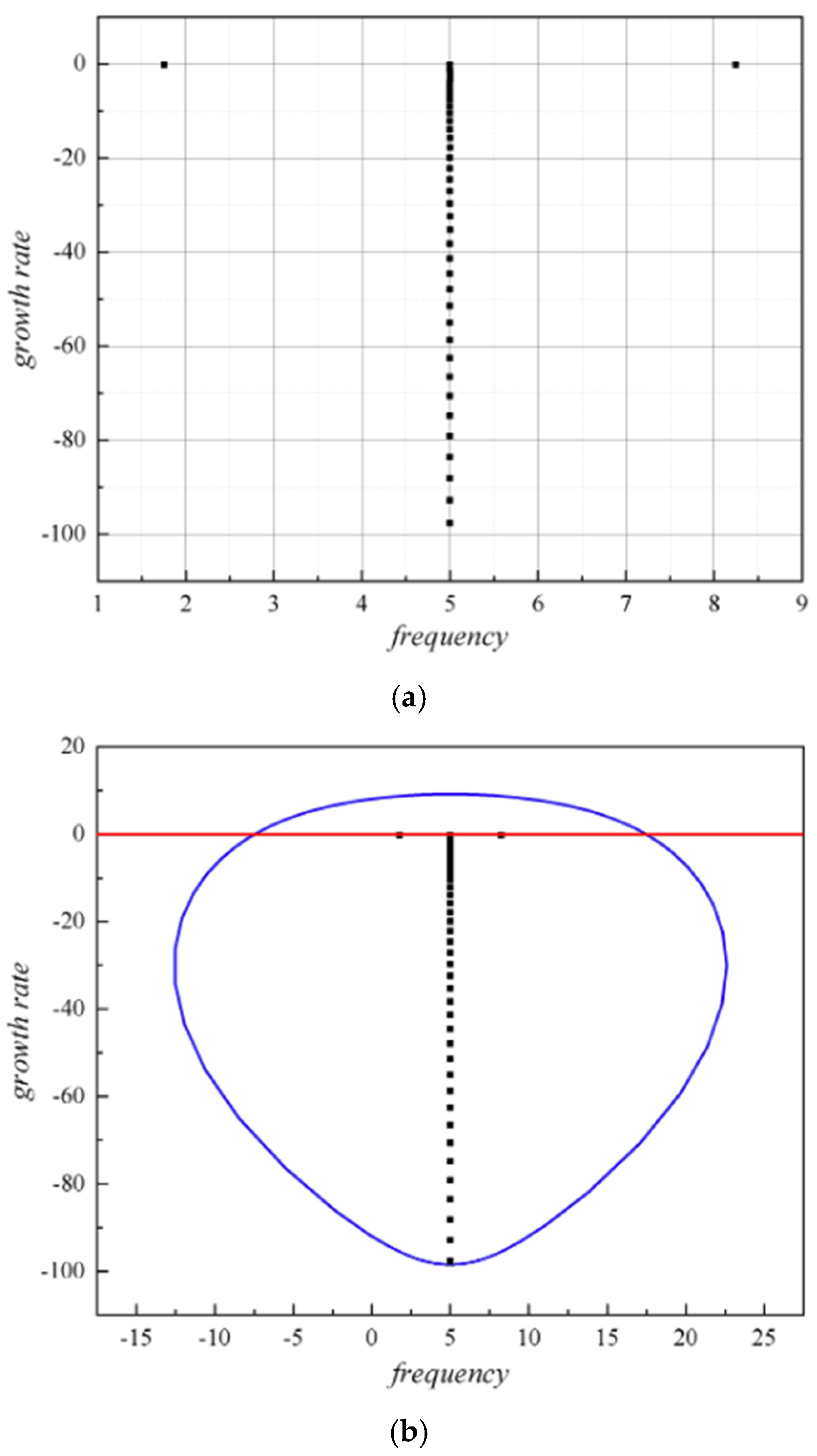
3. Results
3.1. Optimal Growth
3.2. Optimal Initial Condition
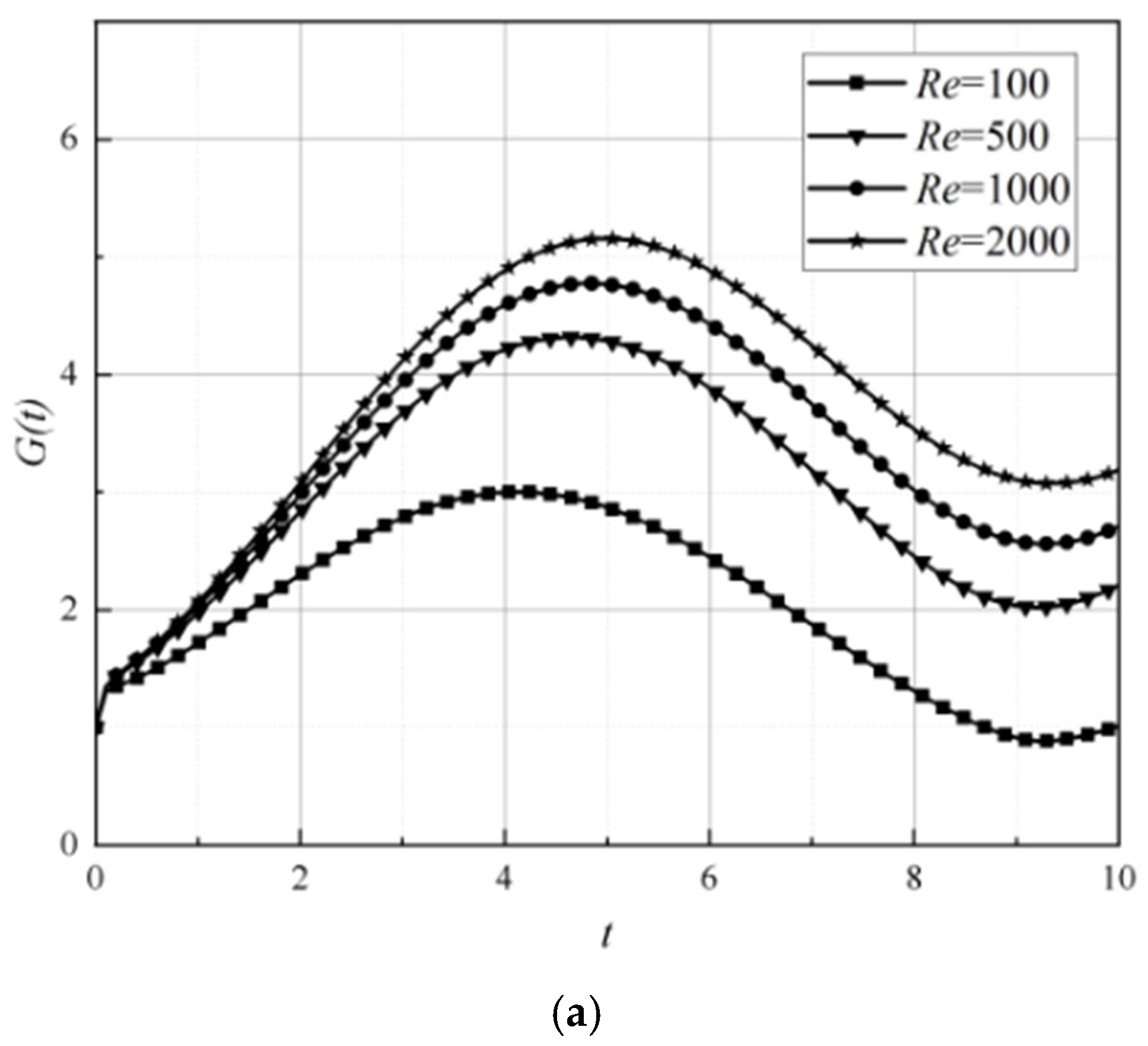
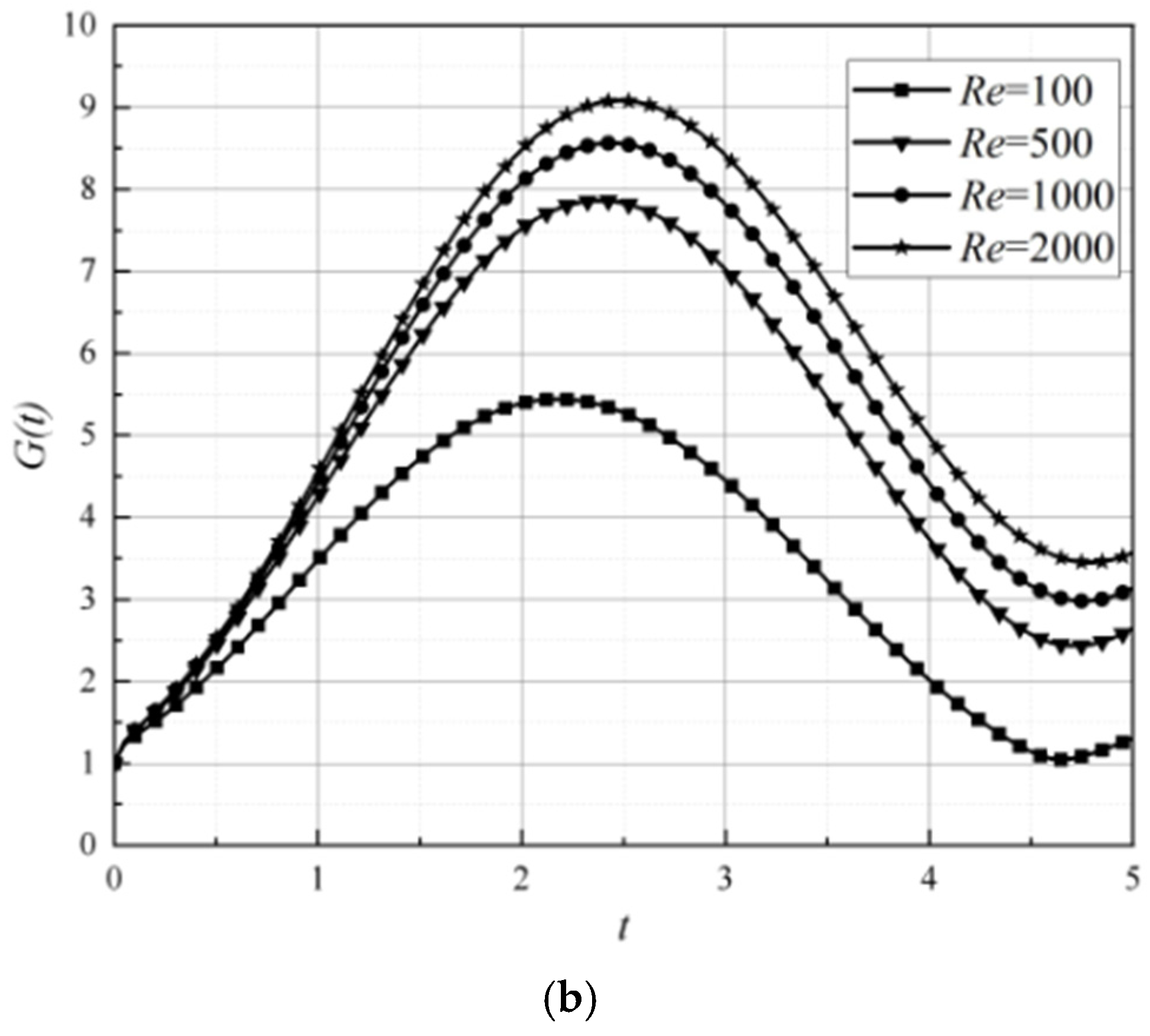
3.3. The Influence of Reynolds Number
3.4. The Influence of Weber Number
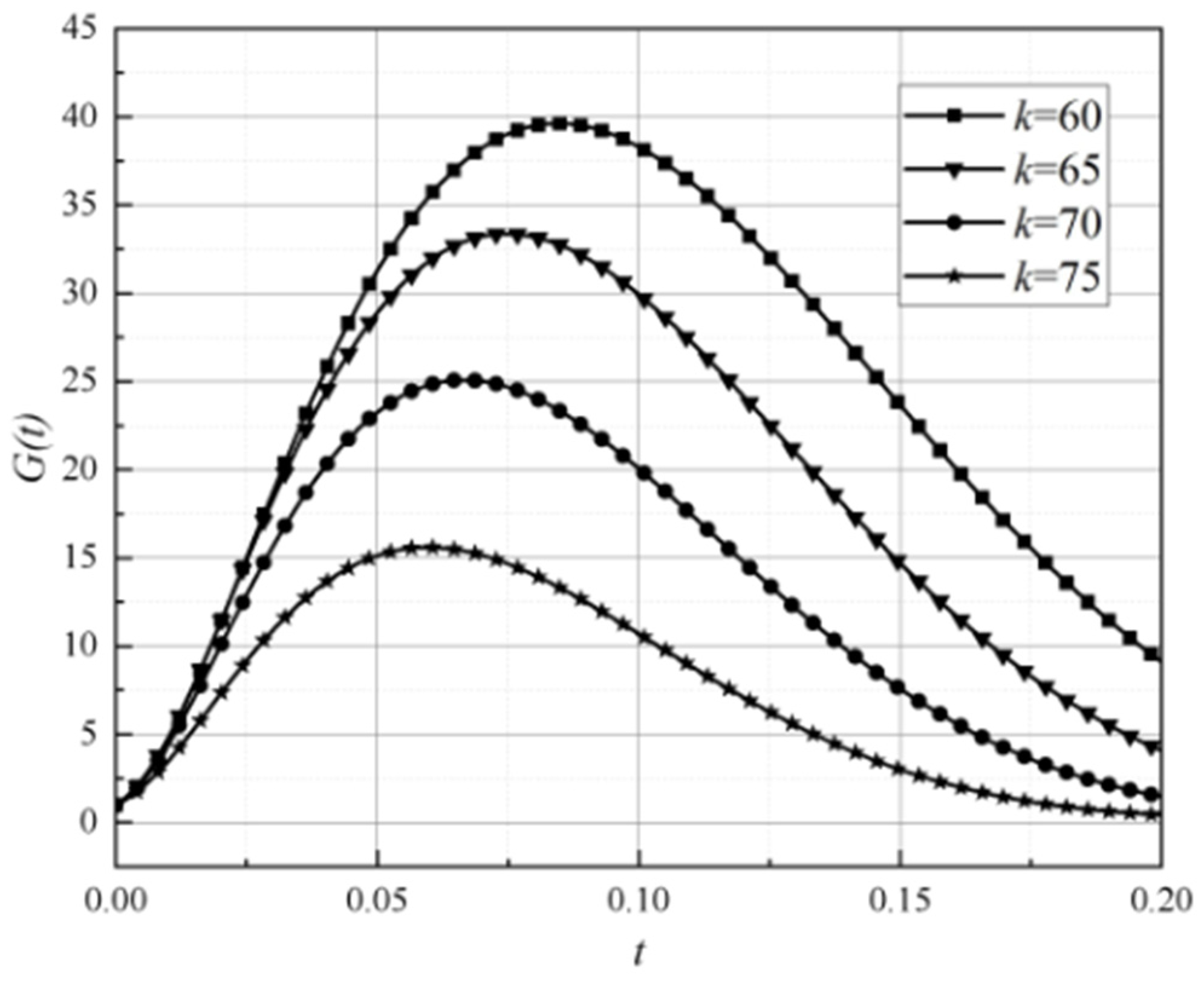
3.5. Numerical Range
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rayleigh, L. On the instability of jets. Proc. London Math. Soc. 1878, s1, 4–13. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Hydrodynamic and Hydromagnetic Stability; Oxford University Press: Oxford, UK, 1961. [Google Scholar]
- Keller, J.B.; Rubinow, S.I.; Tu, Y.O. Spatial instability of a jet. Phys. Fluids 1973, 16, 2052. [Google Scholar] [CrossRef]
- Lin, S.P.; Ibrahim, E.A. Instability of a viscous liquid jet surrounded by a viscous gas in a vertical pipe. J. Fluid Mech. 1990, 218, 641. [Google Scholar] [CrossRef]
- Yang, L.; Tong, M.; Fu, Q. Linear stability analysis of a three-dimensional viscoelastic liquid jet surrounded by a swirling gas stream. J. Non-Newton. Fluid Mech. 2013, 191, 1–13. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, Z. Instability of a viscoelastic liquid jet with axisymmetric and asymmetric disturbances. Int. J. Multiph. Flow 2008, 34, 42–60. [Google Scholar] [CrossRef]
- Xie, L.; Yang, L.; Ye, H. Instability of gas-surrounded Rayleigh viscous jets: Weakly nonlinear analysis and numerical simulation. Phys. Fluids 2017, 29, 074101. [Google Scholar] [CrossRef]
- Ye, H.; Peng, J.; Yang, L. Instability of eccentric compound threads. Phys. Fluids 2017, 29, 082110. [Google Scholar] [CrossRef]
- Li, S.; Yang, R.; Mu, K.; Luo, X.; Si, T. Thermal effects on the instability of coaxial liquid jets in the core of a gas stream. Phys. Fluids 2019, 31, 032106. [Google Scholar] [CrossRef]
- Schmid, P.J. Nonmodal Stability Theory. Ann. Rev. Fluid Mech. 2007, 39, 129–162. [Google Scholar] [CrossRef]
- Orszag, S.A. Accurate solution of the Orr-Sommerfeld equation. J. Fluid Mech. 1971, 50, 689–703. [Google Scholar] [CrossRef]
- Herron, I.H. Observations on the role of the vorticity in the stability of wall bounded flows. Stud. Appl. Math. 1991, 85, 269–286. [Google Scholar] [CrossRef]
- Busse, F.H. Bounds on the transport of mass and momentum by turbulent flow between parallel plates. Z. Angew. Math. Phys. 1969, 20, 1–14. [Google Scholar] [CrossRef]
- Joseph, D.D.; Carmi, S. Stability of Poiseuille flow in pipes, annuli and channels. Q. Appl. Math. 1969, 26, 575–599. [Google Scholar] [CrossRef]
- Joseph, D.D. Nonlinear stability of the Boussinesq equations by the method of energy. Arch. Rat. Mech. Anal. 1966, 22, 163–184. [Google Scholar] [CrossRef]
- Drazin, P.G.; Reid, W.H. Hydrodynamic Stability; Cambridge University Press: Cambridge, UK, 1981. [Google Scholar]
- Herbert, T. Secondary instability of boundary layers. Ann. Rev. Fluid Mech. 1988, 20, 487–526. [Google Scholar] [CrossRef]
- Patel, V.C.; Head, M.R. Some observations on skin friction and velocity profiles in fully developed pipe and channel flows. J. Fluid Mech. 1969, 38, 181–201. [Google Scholar] [CrossRef]
- Lundbladh, A.; Johansson, A.V. Direct simulation of turbulent spots in plane Couette flow. J. Fluid Mech. 1991, 229, 499–516. [Google Scholar] [CrossRef]
- Tillmark, N.; Alfredsson, H. Experiments on transition in plane Couette flow. J. Fluid Mech. 1992, 235, 89–102. [Google Scholar] [CrossRef]
- Farrell, B.F. Optimal excitation of perturbations in viscous shear flow. Phys. Fluids 1988, 31, 2093–2102. [Google Scholar] [CrossRef]
- Gustavsson, L.H. Excitation of direct resonances in plane Poiseuille flow. Stud. Appl. Math. 1986, 75, 227–248. [Google Scholar] [CrossRef]
- Gustavsson, L.H. Energy growth of three-dimensional disturbances in plane Poiseuille flow. J. Fluid Mech. 1991, 224, 241–260. [Google Scholar] [CrossRef]
- Trepethen, L.N. Pseudospectra of matrices. In Numerical Analysis 1991; Griffiths, D.F., Watson, G.A., Eds.; Longman: Harlow, UK, 1992. [Google Scholar]
- Treethen, L.N.; Trefethen, A.E.; Reddy, S.C.; Driscoll, T.A. A New Direction in Hydrodynamic Stability: Beyond Eigenvalues; Tech. Rep. CTC 92TR115; Cornell Theory Center, Cornell University: Ithaca, NY, USA, 1992. [Google Scholar]
- Trefethen, L.N.; Trefethen, A.N.; Reddy, S.C.; Driscoll, T.A. Hydrodynamic Stability Without Eigenvalues. Science 1993, 261, 578–584. [Google Scholar] [CrossRef] [PubMed]
- Reddy, S.C.; Henningson, D.S. Energy growth in viscous channel flows. J. Fluid Mech. 1993, 252, 209–238. [Google Scholar] [CrossRef]
- Schmid, P.J.; Henningson, D.S. Stability and Transition in Shear Flows; Springer: New York, NY, USA, 2001. [Google Scholar]
- Schmid, P.J.; Henningson, D.S. Optimal energy density growth in Hagen–Poiseuille flow. J. Fluid Mech. 1994, 277, 197–225. [Google Scholar] [CrossRef]
- Schmid, P.J.; Brandt, L. Analysis of Fluid Systems: Stability, Receptivity, Sensitivity. Appl. Mech. Rev. 2014, 66, 024803. [Google Scholar] [CrossRef]
- De Luca, L.; Costa, M.; Caramiello, C. Energy growth of initial perturbations in two-dimensional gravitational jets. Phys. Fluids 2002, 14, 289–299. [Google Scholar] [CrossRef]
- Yecko, P.; Zaleski, S. Transient growth in two-phase mixing layers. J. Fluid Mech. 2005, 17, 43–52. [Google Scholar] [CrossRef]
- Li, F.; Yin, X.-Y.; Yin, X.-Z. Transient growth in a two-fluid channel flow under normal electric field. Phys. Fluids 2009, 21, 094105. [Google Scholar] [CrossRef]
- Liu, R.; Liu, Q.S. Non-modal instability in plane Couette flow of a power-law fluid. J. Fluid Mech. 2011, 676, 145–171. [Google Scholar] [CrossRef]
- Hu, K.X.; Zheng, S.; Chen, Q.S. Transient growth in thermocapillary liquid layers. Phys. Rev. Fluids 2020, 5, 014001. [Google Scholar] [CrossRef]
- Chaudhary, I.; Garg, P.; Subramanian, G.; Shankar, V. Linear instability of viscoelastic pipe flow. J. Fluid Mech. 2021, 908, A11. [Google Scholar] [CrossRef]
- Trefethen, L.N.; Embree, M. Spectra and Pseudospectra; Princeton University Press: Princeton, NJ, USA, 2005. [Google Scholar]
- Andersson, P.; Berggren, M.; Henningson, D.S. Optimal Disturbances and Bypass Transition in Boundary Layers. Phys. Fluids 1999, 11, 134–150. [Google Scholar] [CrossRef]
- Horn, R.A.; Johnson, C.R. Topics in Matrix Analysis; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, D.; Fu, Q.; Yang, L. Transient Energy Growth in a Free Cylindrical Liquid Jet. Aerospace 2024, 11, 985. https://doi.org/10.3390/aerospace11120985
Huang D, Fu Q, Yang L. Transient Energy Growth in a Free Cylindrical Liquid Jet. Aerospace. 2024; 11(12):985. https://doi.org/10.3390/aerospace11120985
Chicago/Turabian StyleHuang, Dongqi, Qingfei Fu, and Lijun Yang. 2024. "Transient Energy Growth in a Free Cylindrical Liquid Jet" Aerospace 11, no. 12: 985. https://doi.org/10.3390/aerospace11120985
APA StyleHuang, D., Fu, Q., & Yang, L. (2024). Transient Energy Growth in a Free Cylindrical Liquid Jet. Aerospace, 11(12), 985. https://doi.org/10.3390/aerospace11120985






