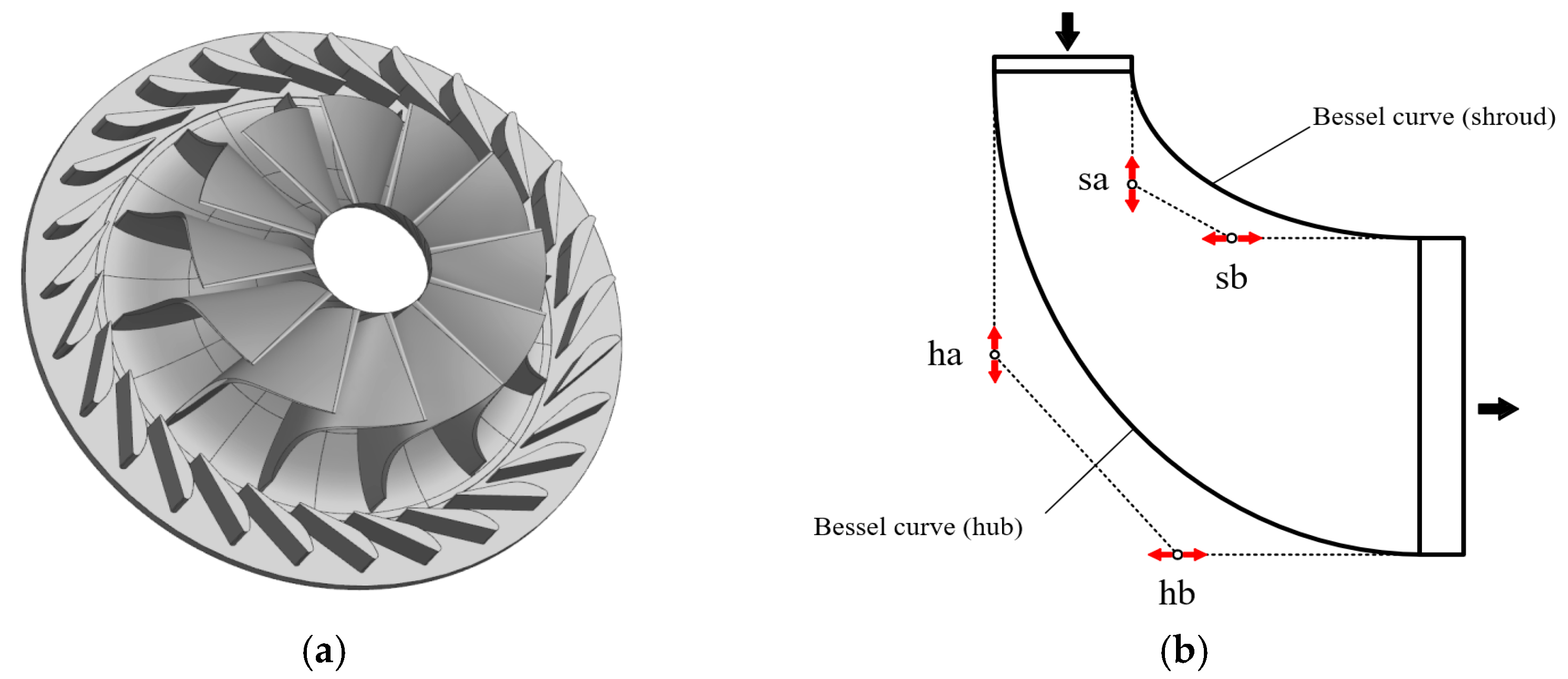
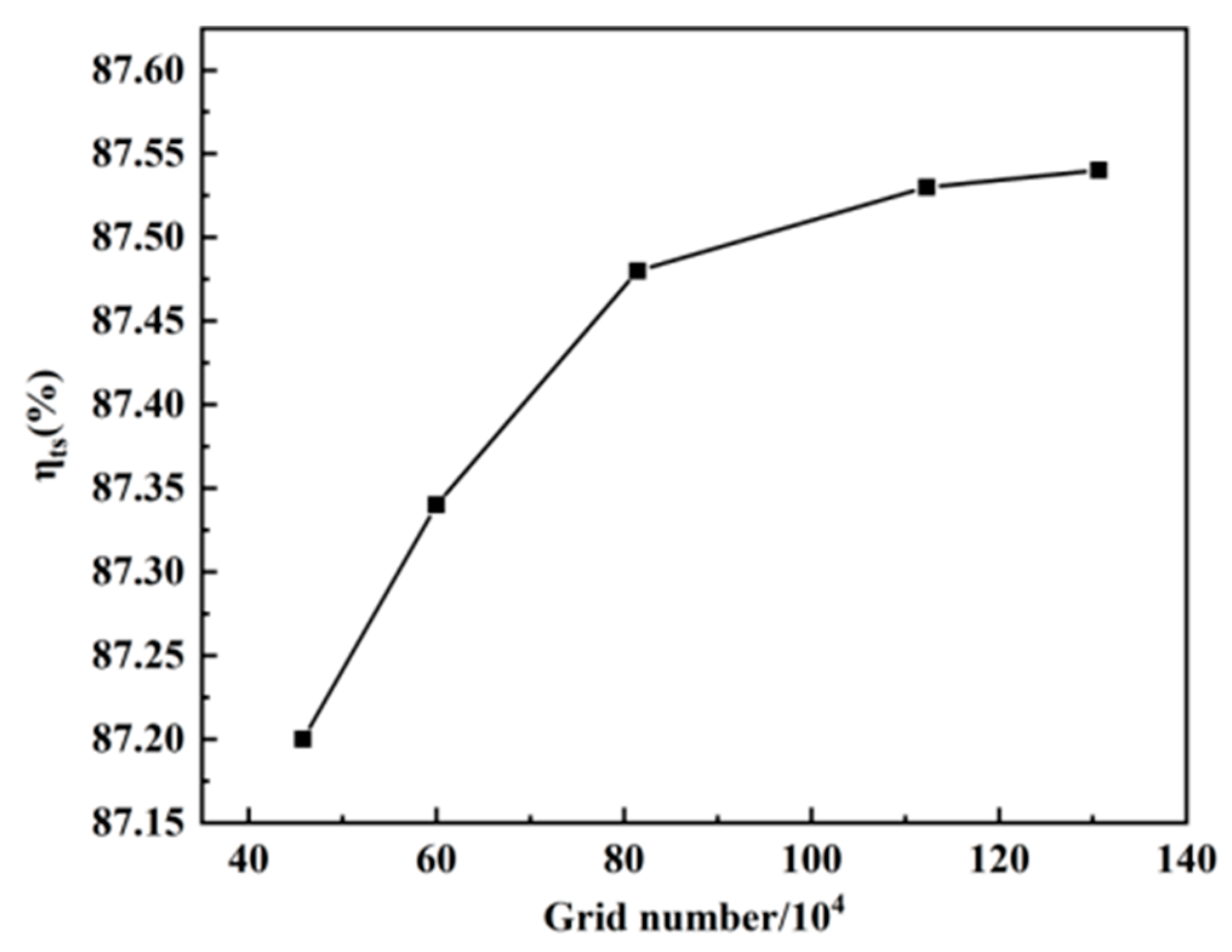
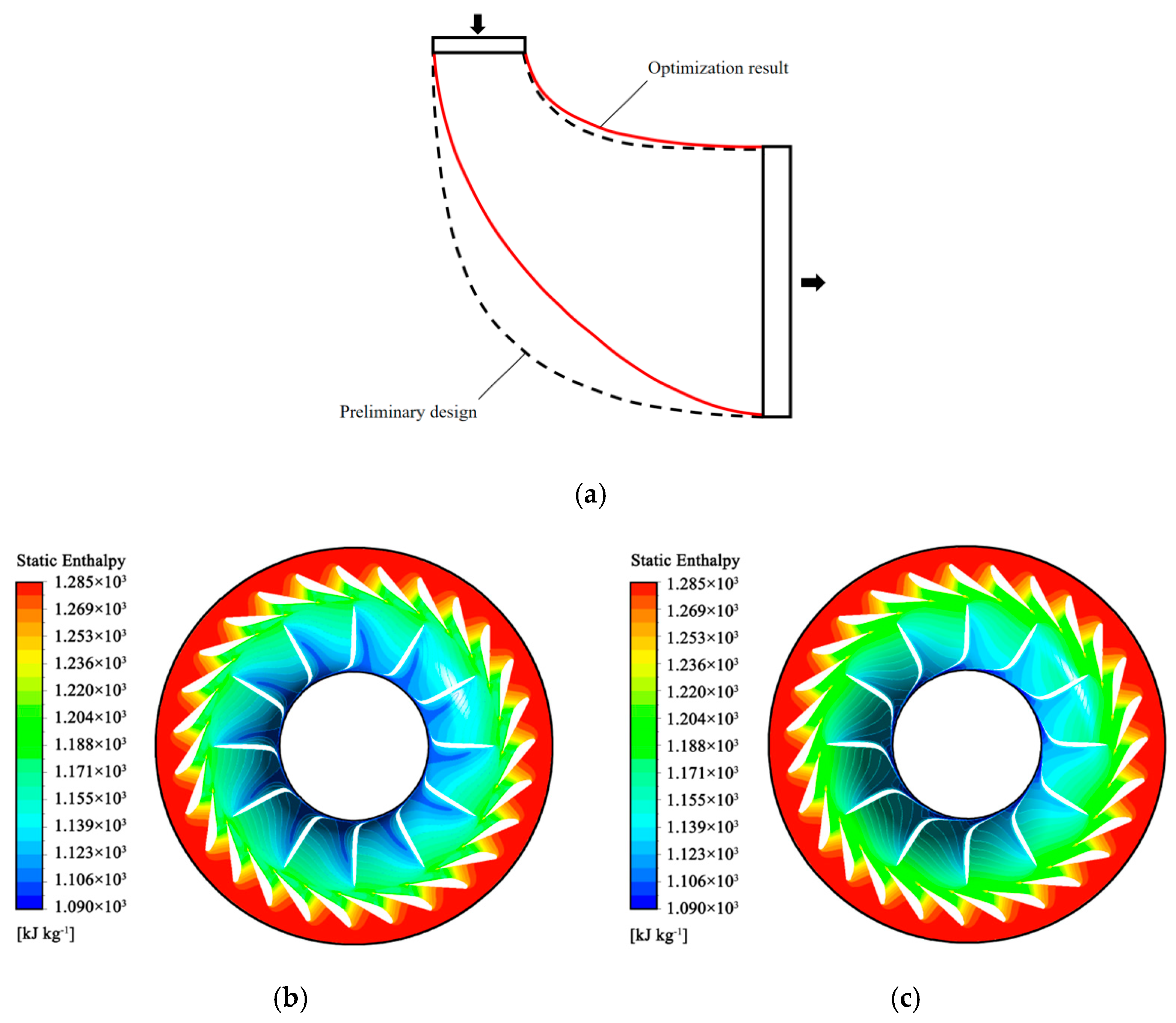
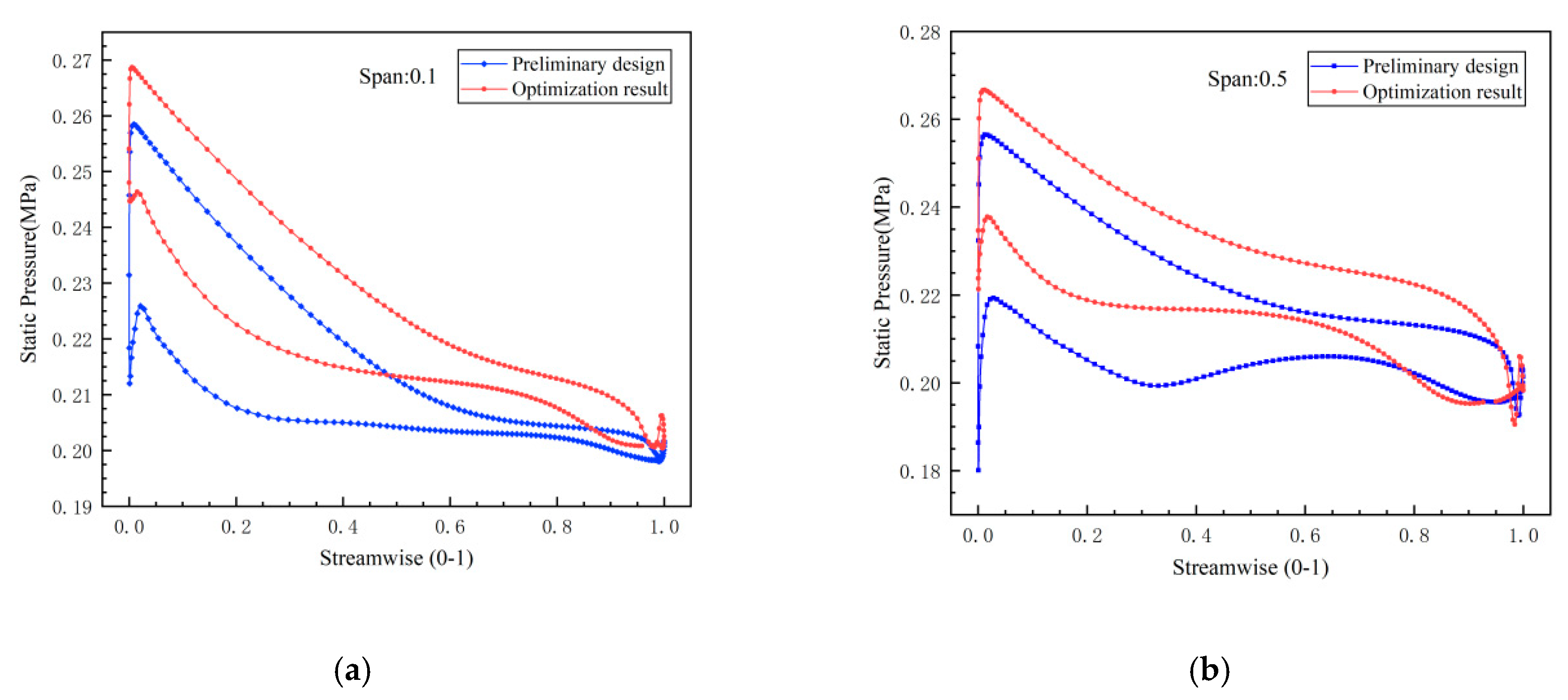
1. Introduction
The utilization of hydrogen as a fuel has garnered significant attention due to its high calorific value and low emissions, making it a cleaner alternative to conventional fossil fuels [
1]. Hydrogen combustion produces only water vapor, eliminating waste residues and carbon dioxide emissions, posing hydrogen energy as a focal point in the current and future energy landscape [
2]. Hydrogen energy is a hot topic in the long-term development trend of future energy [
3]. As a vital component in converting heat energy into electricity, turbines are crucial in energy efficiency. Radial turbines, in particular, are known for their higher efficiency and compact size than axial flow turbines, making them suitable for distributed energy systems. Because of their more minor power, they are widely used in distributed energy [
4]. As the demand for cleaner energy sources grows, there is an increasing shift towards using hydrogen in both aero engines and gas turbines. Research shows that hydrogen-fueled gas turbines, when coupled with solar hydrogen production technology, can achieve 100% renewable energy-based systems [
5]. Studies also indicate a significant improvement in thermal efficiency for hydrogen turbines compared to traditional natural gas turbines, with fossil fuel savings increasing from 37.5% to 41.5% [
6,
7]. These findings underscore the importance of research on hydrogen-fueled gas turbines for enhancing thermal efficiency, energy savings, and emissions reductions.
Extensive efforts have been made to design efficient and effective radial turbines. Previous studies have utilized large datasets to develop one-dimensional design programs, initial impeller design methods, and parametric profiles for subsonic and supersonic radial turbine blades. Classman et al. [
8], according to a large amount of experimental data, wrote the one-dimensional design program of the radial turbine and verified it by experiment. Whitfield et al. [
9] developed a unary dimensionless initial design method for impellers, which can reasonably determine impellers’ geometrical and thermal parameters. Reichert et al. [
10] designed parametric subsonic and supersonic radial turbine stationary blade profiles and conducted two-dimensional numerical calculations. The results showed that the ratio of the thickness of the trailing edge to the width of the throat greatly influenced the blade profile loss. These studies highlight the critical influence of geometrical and thermal parameters on turbine performance.
Previous research has involved the optimization of radial turbines through various approaches. Jankowski et al. [
11] conducted a comprehensive study on the multi-objective optimization of an Organic Rankine Cycle (ORC) power plant. They focused on the one-dimensional design of a radial-inflow turbine (RIT) with backswept rotor blades. The study aimed to determine the optimal parameters affecting the turbine geometry, specifically the nozzle pitch to chord ratio and the blade angle at the rotor inlet. By employing the Non-dominated Sorting Genetic Algorithm-II (NSGA-II) and Technique for Order Preference by Similarity to Ideal Solution (TOPSIS), they identified the optimal design point. Witanowski et al. [
12] employed a hybrid approach to optimize the shape of a single-stage radial inflow turbine (RIT) for a 10 kW Organic Rankine Cycle (ORC) unit. They utilized a combination of deterministic and stochastic methods, including Principal Component Analysis (PCA), to enhance the turbine’s efficiency. The study compared the performance of eight different algorithms, including three deterministic, one stochastic, and four hybrid algorithms. Uusitalo and Zocca [
13] investigated the design and numerical analysis of supersonic radial-inflow turbines for transcritical Organic Rankine Cycle (ORC) processes. They focused on the impact of fluid and turbine inlet conditions on the resulting turbine geometry, rotational speed, efficiency, and loss distribution. Ebaid et al. [
4] optimized the impeller geometry parameters globally and designed the radial turbine with a diameter of 16.91 cm, a rotational speed of 6 × 10
4 rpm, and an output power of 60 kW. Hasenjager et al. [
14] used B-spline curves for the aerodynamic optimization of a single-stage, ultra-low-spreading ratio transonic nozzle for a high-pressure axial flow turbine and showed that the two objective variables, total pressure loss at nozzle and uniformity of flow field at the outlet of the vane, were in a reciprocal relationship during the optimization process. Kim et al. [
15] optimized blade modeling through numerical calculation and improved the efficiency of a supercritical carbon dioxide turbine. Carrillo et al. [
16] successfully designed a micro radial turbine. They used Fortran language to write a one-dimensional design program to predict turbine performance and calculate the turbine’s detailed parameters and used numerical simulation to analyze the internal fluid flow of the turbine. Vilim et al. [
17], through experimental data, verified the one-dimensional design theory of a radial turbine. They found that the mathematical model differed significantly from the experimental results. Thus, they analyzed the result and optimized the loss model. Xi et al. [
18] developed a radial turbine thermal design program for small gas turbines based on the investigation of radial turbine reaction degree and speed ratio selection method and compared the results of the one-dimensional program with the three-dimensional CFD numerical simulation. Li et al. [
19,
20] used the NURBS curve parametric expression and geometric profile, combined with CFD numerical experiments, to study the aerodynamic optimization of radial turbines with organic working fluid. After aerodynamic optimization, the meridian flow profile of the impeller and the flow channel changed more evenly and smoothly. The efficiency of the turbine stage was improved. Zhang et al. [
21] completed the aerodynamic design and numerical analysis of a 15 MW supercritical carbon dioxide (CO
2) axial flow turbine and a 1.5 MW radial turbine. The actual physical properties of carbon dioxide were from the Refprop database. Nithesh K et al. [
22] used R134a as the working fluid for a one-dimensional radial turbine design for ocean thermal energy generation. They used three-dimensional numerical simulations to analyze and predict the working performance of the turbine. Qi J et al. [
23] proposed a one-dimensional design program for a supercritical carbon dioxide radial turbine. They studied the internal flow laws of radial turbines with powers ranging from 0.1 MW to 0.2 MW. Futral et al. [
24] proposed a calculation program to predict turbine performance based on the given turbine geometry, efficiency, and pressure ratio and verified its feasibility through experiments. Zhou et al. [
25] proposed a design method for a CO
2 radial turbine and designed a turbine with a power of 1.5 MW. They used ANSYS-CFX 2022 R1 commercial software to perform three-dimensional numerical simulations to validate the one-dimensional design results. There was little difference between the simulation results and one-dimensional design results, indicating the rationality of the design method.
In addition, various optimization algorithms have been applied to the design and optimization of turbines. Panic et al. [
26] developed a novel multi-objective optimization framework for the design of axial compressors. They employed a combination of genetic algorithms and computational fluid dynamics (CFD) simulations to optimize the compressor’s aerodynamic performance. The study focused on minimizing the total pressure loss and maximizing the isentropic efficiency. Mueller L et al. [
27] aimed for the total-to-static efficiency and the moment of inertia of the radial turbine impeller and used differential evolution algorithms, artificial neural networks, and other methods to provide recommendations for turbine optimization. Wang [
28] used particle swarm optimization to design a supercritical carbon dioxide radial turbine and conducted a three-dimensional numerical simulation to verify the design. By comparing four different flow path schemes, she selected the best one and successfully improved the turbine efficiency. Wang [
29] designed a supercritical carbon dioxide radial turbine with a power of 70 MW. She analyzed the effect of blade inlet and outlet angle on efficiency and optimized the blade profile.
Obviously, in view of the literature survey, it can be summarized that most previous research has involved radial turbines focusing on organic cycles [
19,
20], micro-turbines [
16,
30], or supercritical carbon dioxide systems [
10,
15]. With the extensive utilization of hydrogen energy, the demand for hydrogen turbines is increasing, but research on hydrogen fuel radial turbines is still scarce. With respect to the aerodynamic performance difference resulting from the increasing water content in the working fluid, it is necessary to redesign turbines with a hydrogen combustion system.
Despite the progress in turbine design, there remains a gap in understanding the impact of hydrogen combustion products, particularly the increased water content, on the aerodynamic performance of turbines. This study addresses this gap by designing a radial turbine using the differential evolution (DE) algorithm to optimize its aerodynamic performance. This research also investigates the effects of varying water content in the combustion products on turbine efficiency and performance metrics. To bridge the knowledge gap, this paper presents a detailed analysis of flow losses, enthalpy drop, Mach number, and power output in radial turbines operating with hydrogen combustion products. The study utilizes an orthogonal experiment and analysis of means (ANOM) to refine the turbine design and achieve optimal performance. By examining the effects of water content variations, this research aims to provide valuable insights into optimizing hydrogen-fueled radial turbines, enhancing their efficiency and contributing to sustainable energy solutions.