Comparison Between Numerical and Experimental Methodologies for Total Enthalpy Determination in Scirocco PWT
Abstract
1. Introduction
2. Facility Operating Conditions
3. Enthalpy Measurement and Rebuilding
3.1. Mass-Averaged Enthalpy Measurement
3.1.1. Sonic Throat Method
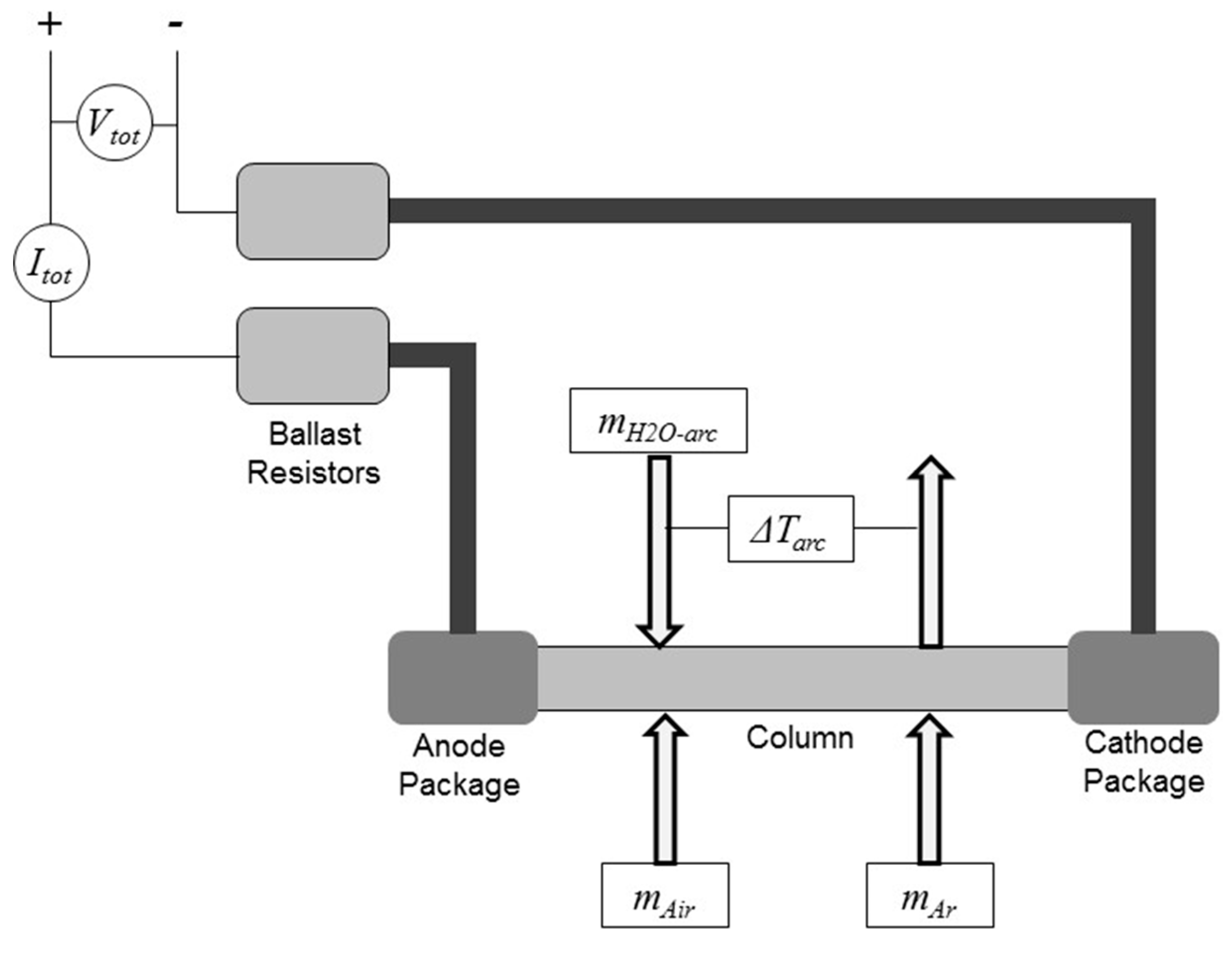
3.1.2. Energy Balance Method
- is the voltage of the Power Supply System between the anode and cathode bar, V;
- is the electrical current of the Power Supply System of the anode bar, A;
- is the water flow rate of the arc heater cooling system, (m3/h);
- is the delta temperature of the water flow of the arc heater cooling system, (K);
- is the air mass-flow rate of the arc heater complex, (kg/s);
- is the argon mass-flow rate of the arc heater complex, (kg/s).
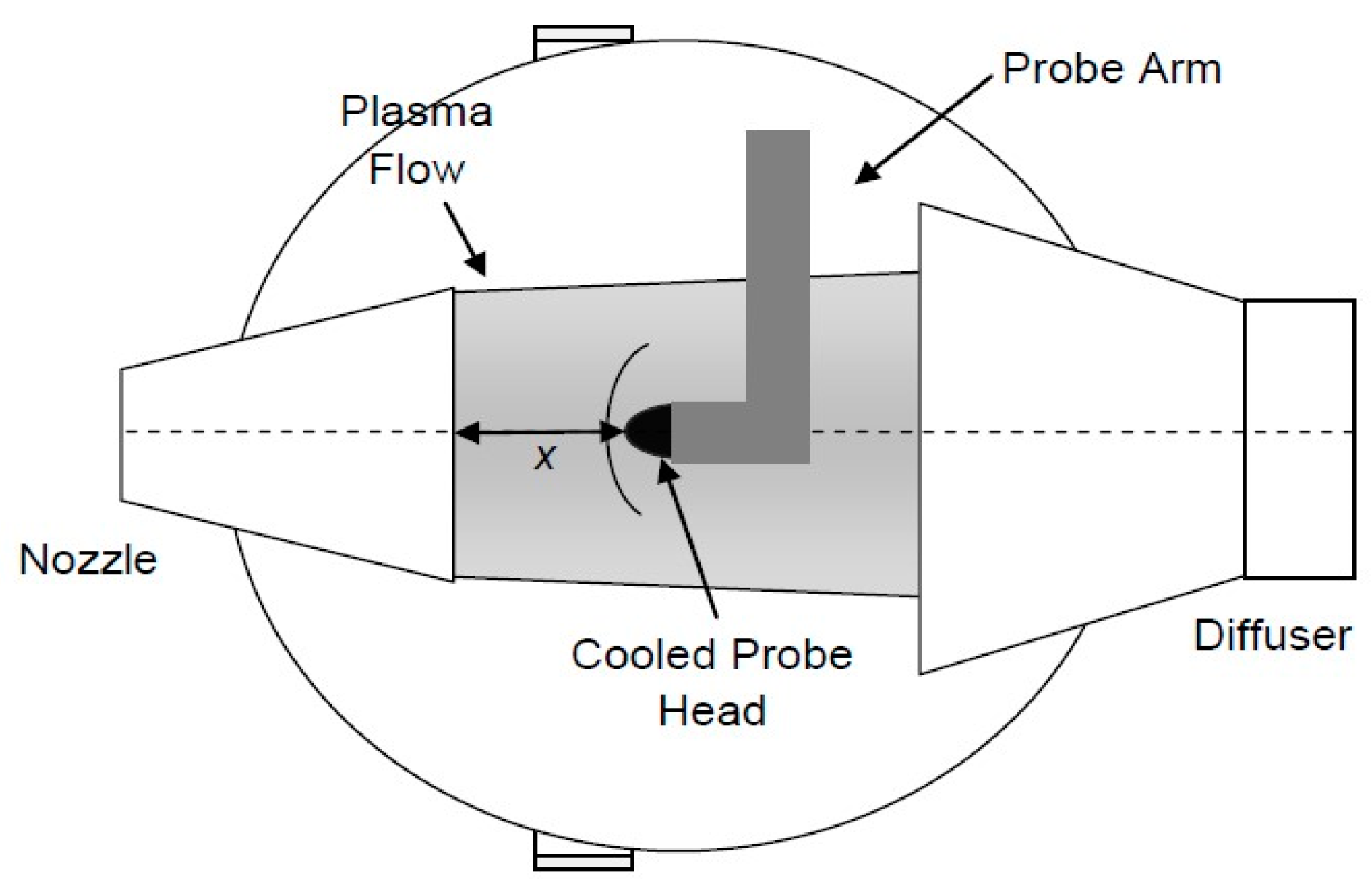
3.2. Centerline Enthalpy Measurement—Heat Transfer Method
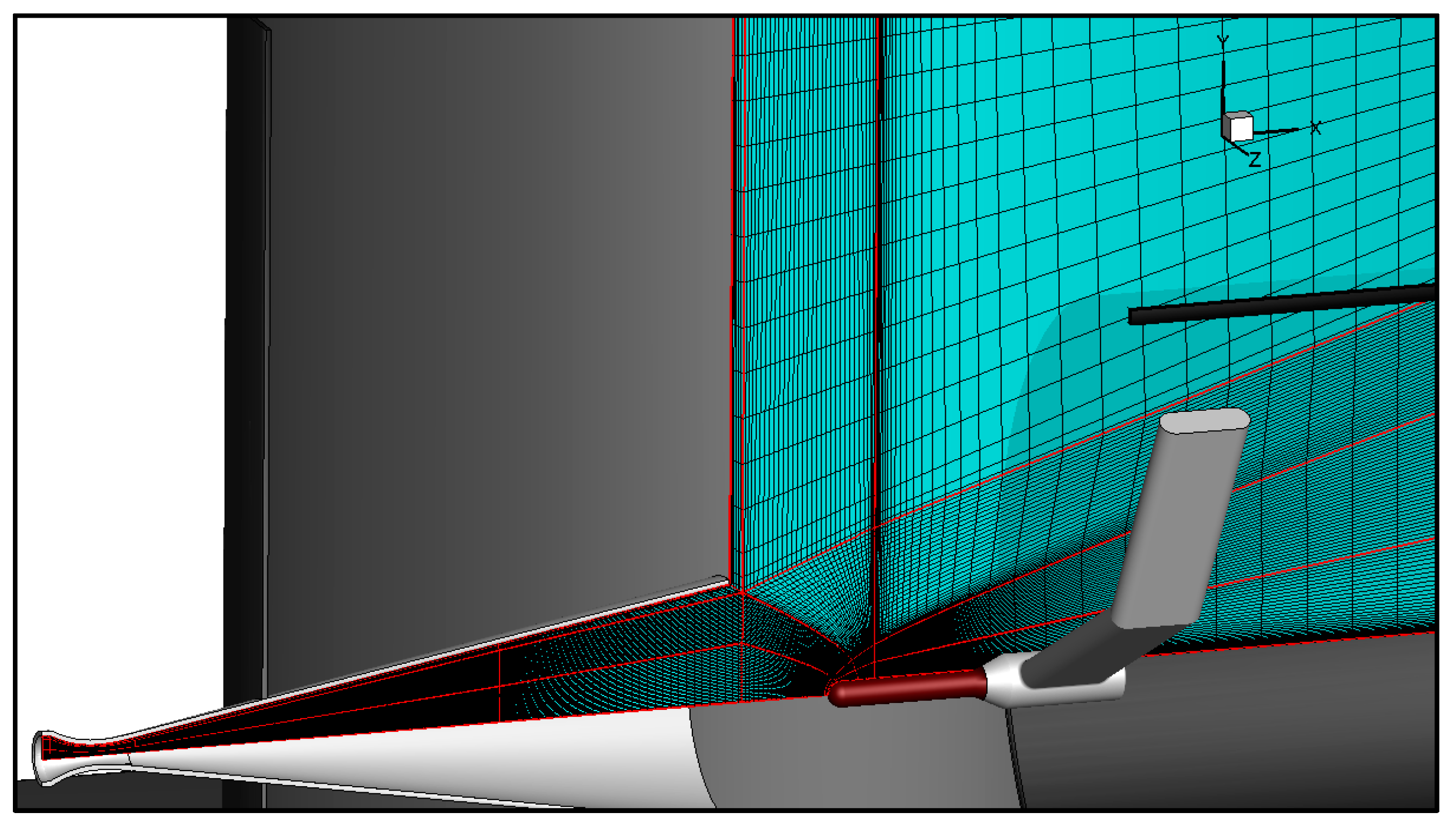
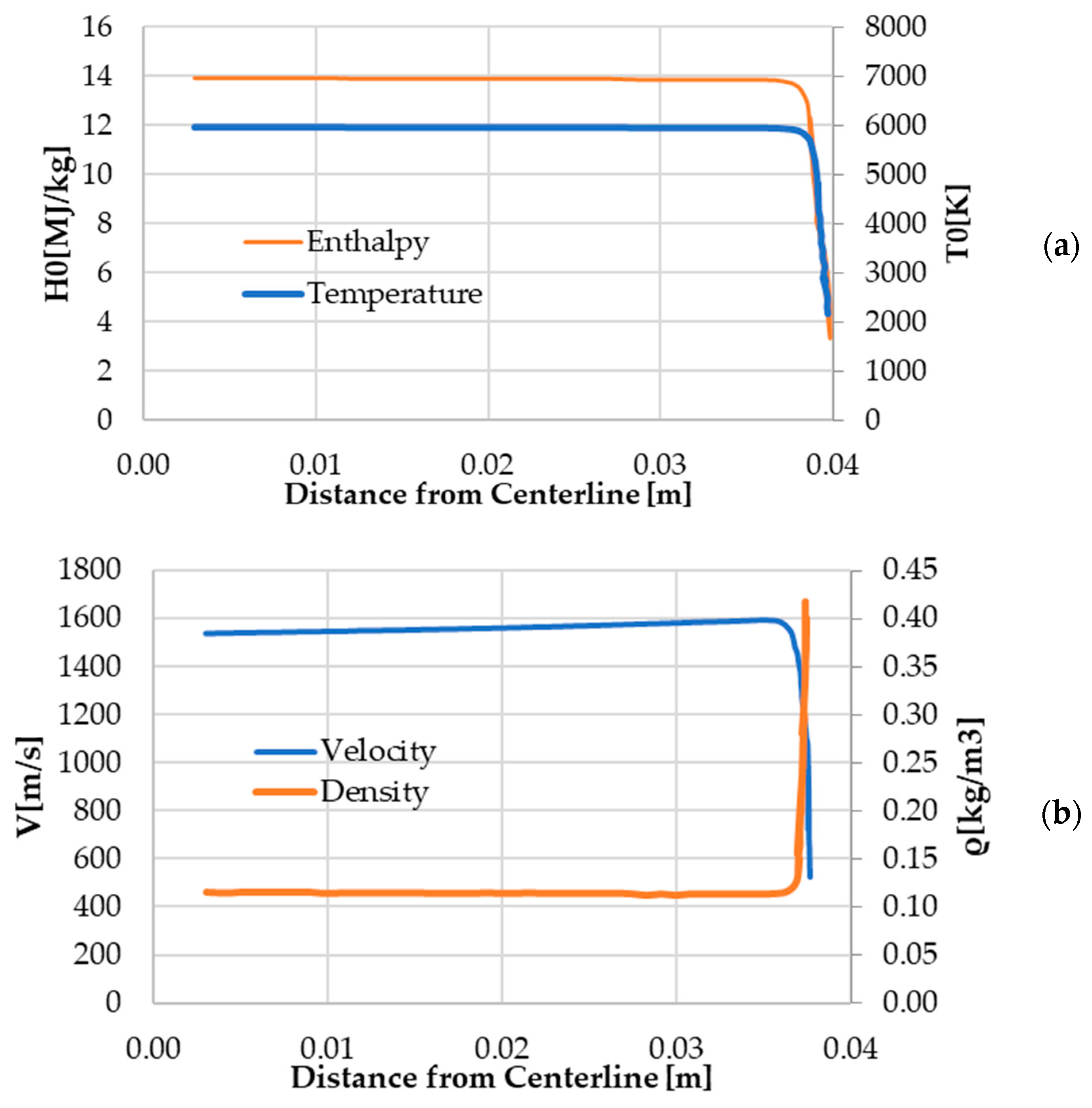
3.3. Centerline Enthalpy Measurement—CFD Rebuilding
- The 2D-axi RANS approach (CIRA NExT solver);
- The time marching to steady-state solution strategy;
- The 2° order Upwind Flux Difference Splitting convective scheme;
- The 5-species air in thermal and chemical non-equilibrium as a working gas model;
- The fixed temperature (T = 370 K) nozzle wall boundary condition;
- The fixed temperature (T = 370 K) fully catalytic wall boundary condition for the calibration probe.
4. Results and Discussion
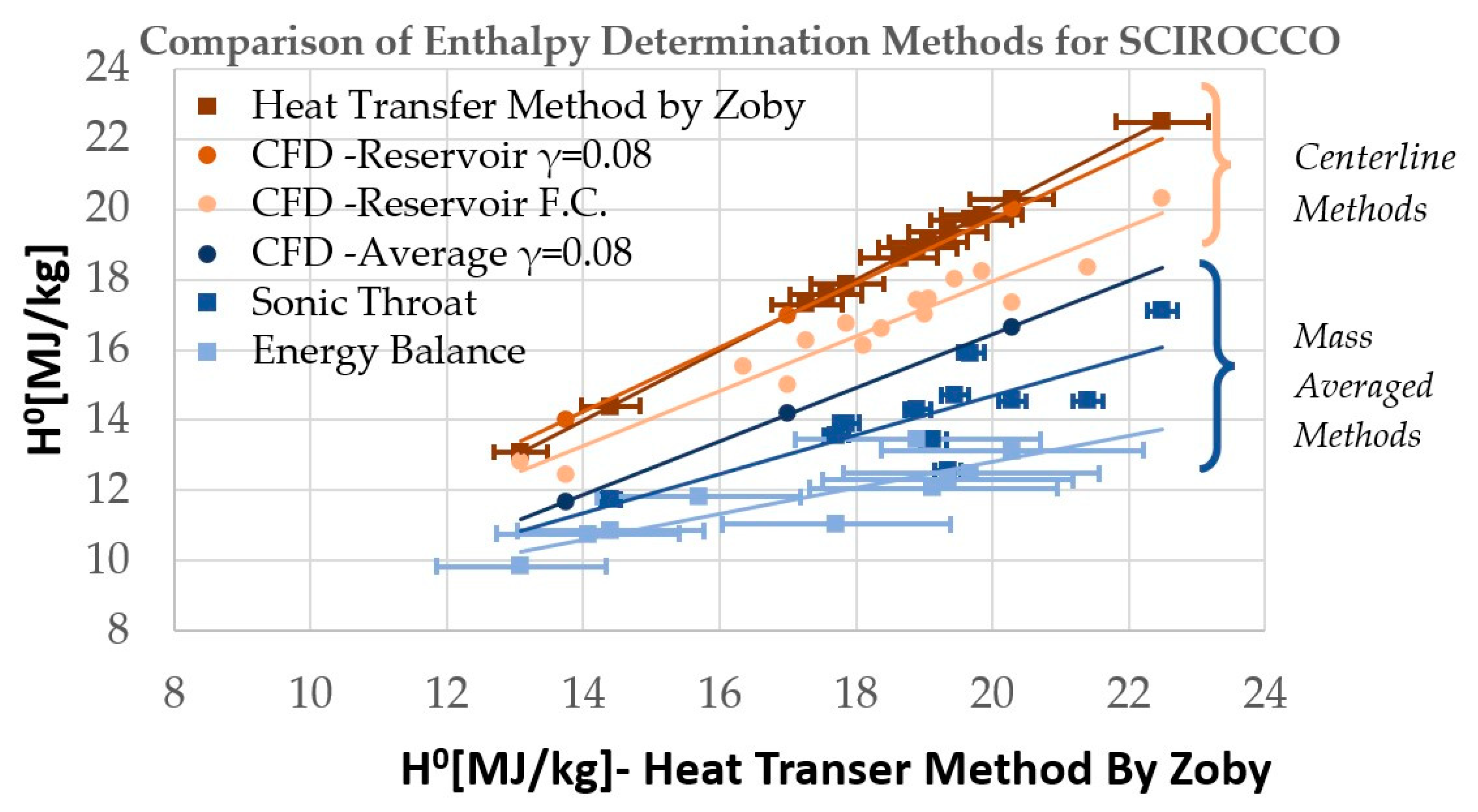
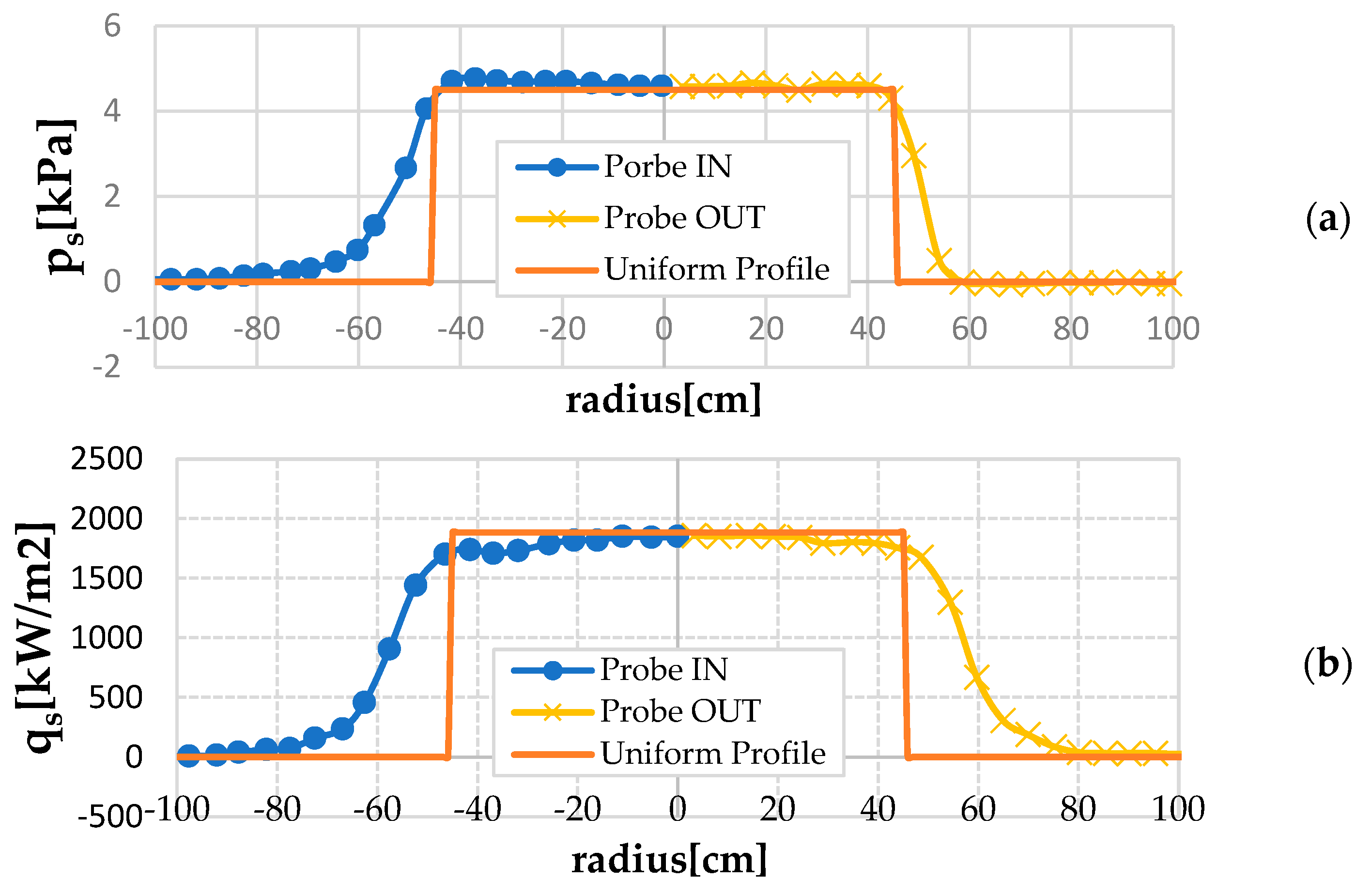
4.1. Mass Averaged Enthalpy Measurement and Profile Uniformity Characterization
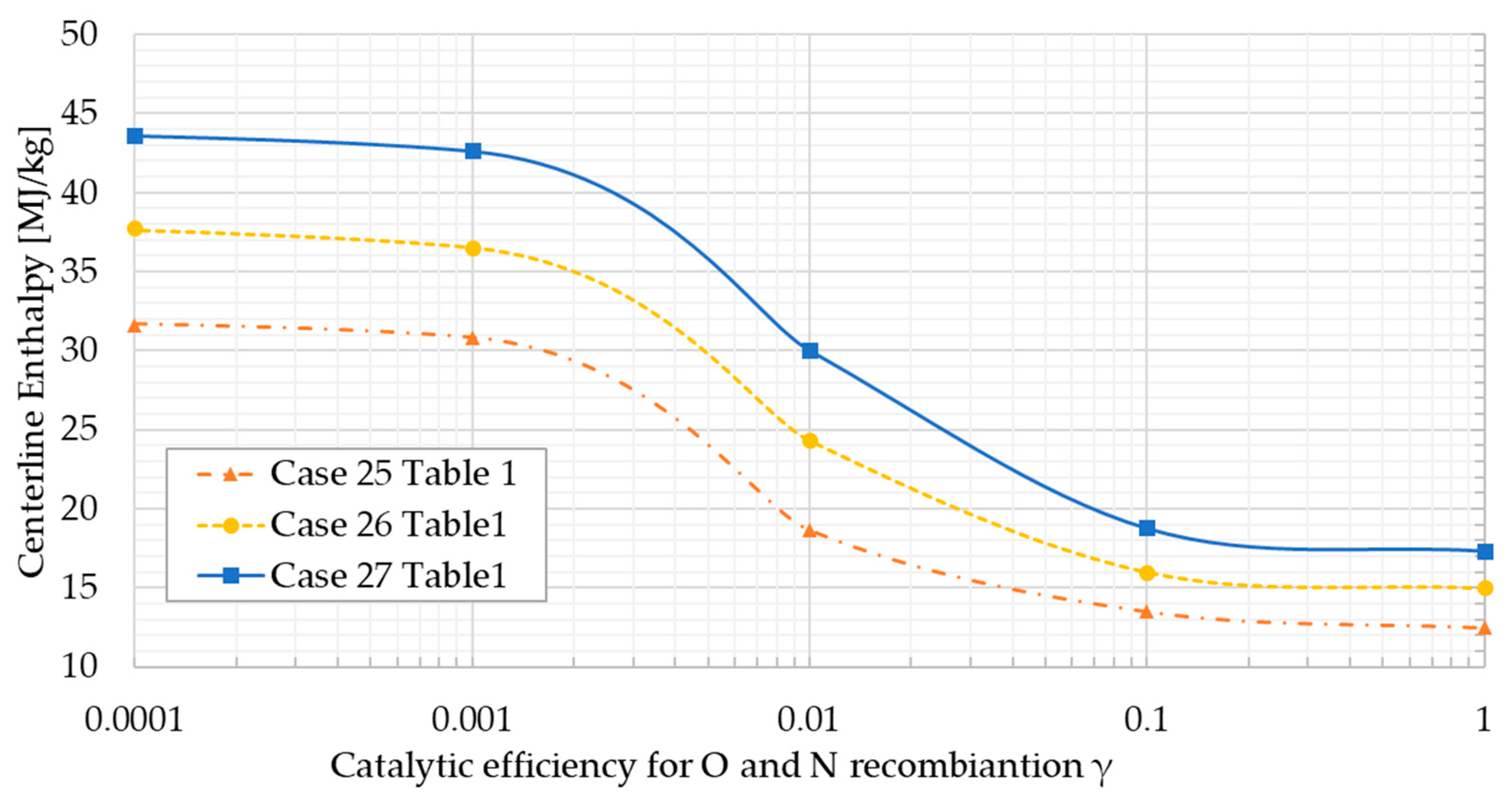
4.2. Centerline Enthalpy Measurement and Surface–Catalytic Recombination Coefficient Estimation
5. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| air | Air |
| ARC | Ames Research Center |
| arc | Electrical arc column |
| ar: | Argon |
| CFDs | Computational fluid dynamics |
| CuO | Cupric oxide (black copper oxide) |
| Cu2O | Cuprous oxide (red copper oxide) |
| D | Diameter of standard probe |
| Di | Diffusion coefficient of the i-th species |
| EB | Energy balance |
| FC | Fully catalytic |
| γ | Catalytic efficiency |
| γN | Catalytic efficiency for the N + N → N2 reaction |
| γO | Catalytic efficiency for the O + O → O2 reaction |
| H⁰ | Reservoir enthalpy |
| hi | Enthalpy of the i-th species |
| IHF | The Interaction Heating Facility |
| k | Mixture thermal conductivity |
| kv,i | Vibrational conductivity of bi-atomic species |
| LIF | Laser-Induced Fluorescence |
| ṁ | Flow rate |
| Ns | Number of chemical species |
| Nvib | Number of chemical species with vibrational energy mode |
| P⁰ | Reservoir pressure |
| ps | Probe stagnation pressure |
| PWT | Plasma Wind Tunnel |
| q | Total heat flux |
| qc | Convective (roto-translational) heat flux |
| qd | Diffusive (chemical) heat flux |
| qs | Probe stagnation wall heat flux |
| qv | Vibrational heat flux |
| RA | Specific gas constant for species A |
| ρ | Density |
| ρw | Wall density |
| ST | Sonic throat |
| T | Temperature |
| Tw: | Wall temperature |
| Tvib,i | Vibrational temperature of the i-th vibrational species |
| TPS | Thermal Protection Systems |
| U | Uncertainty |
| v | Velocity |
| x | Distance of probe from the nozzle exit section |
| Yi | Mass fraction of the i-th species |
| YA,w | Mass fraction of species A at the wall |
| |MA| | Total flux of atoms impinging the surface |
| |MA↓| | Flux of atoms that recombine at the surface |
References
- Guida, D.; Smoraldi, A.; Schettino, A. Design of a High Enthalpy Hypersonic Nozzle for ‘Ghibli’ Plasma Wind Tunnel. In Proceedings of the ICAS 2024 AIAA, Firenze, Italy, 9–13 September 2024. [Google Scholar]
- Fletcher, D.G. Measurement Requirements for Improved Modeling of Arcjet Facility Flows. In Proceedings of the RTO AVT Course on “Measurement Techniques for High Enthalpy and Plasma Flows”, Rhode-Saint-Genèse, Belgium, 25–29 October 1999. RTO EN-8. [Google Scholar]
- Hightower, T.M.; Balboni, J.A.; Donald, C.L.M.; Anderson, K.F.; Martinez, E.R. Enthalpy by Energy Balance for Aerodynamic Heating Facility at NASA Ames Research Center Arc Jet Complex. In Proceedings of the 48th International Instrumentation Symposium, ISA, San Diego, CA, USA, 5–9 May 2002. [Google Scholar]
- Winovich, W. On the Equilibrium Sonic-Flow Method for Evaluating Electric Arc Air-Heater Performances; NASA TN D-2132; National Aeronautics and Space Administration: Washington, DC, USA, 1964.
- Loehle, S. Comparison of Heat Flux Gages for High Enthalpy Flows—NASA Ames and IRS. In Proceedings of the 46th AIAA Thermophysics Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar] [CrossRef]
- Driver, D.; Philippidi, D.; Terrazas-Salinas, I. Uncertainty Analysis of Coaxial Thermocouple Calorimeters Used in Arc Jets; NASA Ames Research Center: Moffett Field, CA, USA, 2018; p. 94035.
- ASTM Standard E 457-08; Standard Test Method for Measuring Heat Transfer Rate Using a Thermal Capacitance (Slug) Calorimeter. ASTM: West Conshohocken, PA, USA, 2008.
- Terrazas-Salinas, I.; Carballo, J.; Driver, D.; Balboni, J. Comparison of Heat Transfer Measurement Devices in Arc Jet Flows with Shear; Session TP/HT-21: Entry, Descent, and Landing; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar] [CrossRef]
- Anderson, L.A.; Sheldahl, R.E. Experiments with Two Flow-Swallowing Enthalpy Probes in High-Energy Supersonic Streams. AIAA J. 1971, 9, 1804–1810. [Google Scholar] [CrossRef]
- Baronets, P.N.; Bykove, N.G.; Gordeev, A.N.; Pershin, I.S.; Yakushin, M.I. Experimental characterization of induction plasmatron for simulation of entry into Martian atmosphere. In Aerothermodynamics for Space Vehicles; Harris, R.A., Ed.; ESA SP-426; European Space Agency (ESA): Paris, France, 1998; p. 421. [Google Scholar]
- Grey, J.; Jacobs, P.F.; Sherman, M.P. Calorimetric Probe for the Measurement of Extremely High Temperatures. Rev. Sci. Instrum. 1962, 33, 738–741. [Google Scholar] [CrossRef]
- Zhang, N.; Sun, F.; Zhu, L.; Planche, M.P.; Liao, H.; Dong, C.; Coddet, C. Measurement of Specific Enthalpy Under Very Low Pressure Plasma Spray Condition. J. Therm. Spray Technol. 2012, 21, 489–495. [Google Scholar] [CrossRef]
- Fasoulas, S.; Stockle, T.; Auweter-Kurtz, M. Measurement of Specific Enthalpy in Plasma Wind Tunnels Using a Mass Injection Probe. In Proceedings of the 32nd AIAA Thermophysics Conference, Atlanta, GA, USA, 23–25 June 1997. [Google Scholar]
- Laux, T.; Feigl, M.; Auweter-Kurtz, M.; Stockle, T. Estimation of the Surface Catalycity of PVD Coatings by Simultaneous Heat Flux and LIF Measurements in High Enthalpy Air Flows. In Proceedings of the 34th Thermophysics Conference, Denver, CO, USA, 19–22 June 2000. [Google Scholar]
- Park, C.; Raiche, G.A., II; Driver, D.M.; Olejniczak, J.; Terrazas-Salinas, I.; Hightower, T.M.; Saka, T. Comparison of Enthalpy Determination Methods for Arc-Jet Facility. J. Thermophys. Heat Transf. 2006, 20, 672–679. [Google Scholar] [CrossRef]
- Grinstead, J.H.; Driver, D.M.; Raiche, G.A., II. Radial Profiles of Arc-Jet Flow Properties Measured with Laser-Induced Fluorescence of Atomic Nitrogen. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, AIAA Paper 2003-0400, Reno, NV, USA, 6–9 January 2003. [Google Scholar]
- Park, C. Stagnation-Point Radiation for Apollo 4. J. Thermophys. Heat Transf. 2004, 18, 349–357. [Google Scholar] [CrossRef]
- Grau, T.; Stöckle, T.; Fasoulas, S.; Messerschmid, E. Comparison of Numerical Results with Experimental Investigations Obtained by Newly Developed Probes in a Plasma Wind Tunnel. In Aerothermodynamics for Space Vehicles; Harris, R.A., Ed.; ESA SP-426; European Space Agency (ESA): Paris, France, 1999; p. 445. [Google Scholar]
- Park, C. Review of Chemical-Kinetic Problems of Future NASA Missions I—Earth Entries. J. Thermophys. Heat Transf. 1993, 7, 385–398. [Google Scholar] [CrossRef]
- Park, C.; Lee, S.-H. Validation of Multi-Temperature Nozzle Flow Code NOZNT. In Proceedings of the 28th Thermophysics Conference, AIAA 93-2862, Orlando, FL, USA, 6–9 July 1993. [Google Scholar]
- Goulard, R. On Catalytic Recombination Rates in Hypersonic Stagnation Heat Transfer. J. Jet Propuls. 1958, 28, 737–745. [Google Scholar] [CrossRef]
- Winkler, E.L.; Sheldahl, R.E. Influence of Calorimeter Surface Treatment on Heat-Transfer Measurements in Arc-Heated Test Streams. AIAA J. 1966, 4, 715–716. [Google Scholar]
- Viladegut, A.; Chazot, O. Empirical Modeling of Copper Catalysis for Enthalpy Determination in Plasma Facilities. AIAA J. 2019, 57, 2512–2520. [Google Scholar] [CrossRef]
- Cheung, T.M.; Park, G.; Schrijer, F.F.J. Oxygen and Nitrogen Surface Catalytic Recombination on Copper Oxide in Tertiary Gas Mixtures. In Proceedings of the 2015 World Congress on Aeronautics, N/Ao, Bio, Robotics and Energy, Incheon, Republic of Korea, 25–28 August 2015. [Google Scholar]
- Cinquegrana, D.; Votta, R.; Purpura, C.; Trifoni, E. Continuum breakdown and surface catalysis effects in NASA arc jet testing at SCIROCCO. Aerosp. Sci. Technol. 2019, 88, 258–272. [Google Scholar] [CrossRef]
- Purpura, C.; De Filippis, F.; Barrera, P.; Mandanici, D.D. Experimental characterization of the CIRA plasma wind tunnel SCIROCCO test section. Acta Astronaut. 2008, 62, 2–10. [Google Scholar] [CrossRef]
- Medtherm Corp. Coaxial Surface Thermocouple Probe, Bulletin 500; Medtherm Corp.: Huntsville, AL, USA, 2000. [Google Scholar]
- Zoby, E.V. Empirical Stagnation-Point Heat-Transfer Relation in Several Gas Mixtures at High Enthalpy Levels; NASA TN D-4799; NASA: Washington, DC, USA, 1968.
- Ranuzzi, G.; Cutrone, L. Numerical Simulation of LRE and HRE Reacting Flowfields. In Proceedings of the 67th International Astronautical Congress, Guadalajara, Mexico, 26–30 September 2016; p. 34544. [Google Scholar]
- Pandolfi, M.; Borrelli, S. An Upwind Formulation for Hypersonic Non-equilibrium Flows. In Modern Research Topics in Aerospace Propulsion; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Flament, C. Chemical and Vibrational Nonequilibrium Nozzle Flow Calculation by an Implicit Upwind Method. In Proceedings of the 8th GAMM Conference on Numerical Methods in Fluid Mechanics, Delft, The Netherlands, 27–29 September 1989. [Google Scholar]
- Millikan, R.C.; White, D.R. Systematics of Vibrational Relaxation. J. Chem. Phys. 1963, 39, 3209–3213. [Google Scholar] [CrossRef]
- Park, C. Two-Temperature Interpretation of Dissociation Rate Data for N2 and O2. In Proceedings of the Aerospace Sciences Meetings, American Institute of Aeronautics and Astronautics, Reno, NV, USA, 11–14 January 1988. [Google Scholar] [CrossRef]
- Yun, K.; Mason, E. Collision Integrals for the Transport Properties of Dissociating Air at High Temperatures. Phys. Fluids 1962, 5, 380–386. [Google Scholar] [CrossRef]
- Cutrone, L.; Mastellone, A.; Ranuzzi, G.; Schettino, A.; Matrone, A. User Manual of CAST v 2.1. Tech. Rep. CIRA/CAST/DT-86: User’s Manual CAST V.2—REV.1; CIRA: Capua, Italy, 2009. [Google Scholar]
- Kang, S.-W.; Jones, W.L.; Dunn, M.G. Theoretical and Measured Electron-Density Distributions at High Altitudes. AIAA J. 1973, 11, 141–149. [Google Scholar] [CrossRef]
- Scott, C.D. Wall Boundary Equations with Slip and Catalysis for Multicomponent Nonequilibrium Gas Flows; NASA TM X-58111; NASA: Washington, DC, USA, 1973.
- Savino, L.; Martucci, A.; Del Vecchio, A.; De Cesare, M. A Novel Physics Methodology Based on Compact Emission Spectroscopy in the VNIR (0.4–0.9 μm) Ranges for Plasma Shock Layer and Material Temperature Determinations. Infrared Phys. Technol. 2020, 108, 103353. [Google Scholar] [CrossRef]
- Cipullo, A.; Savino, L.; Marenna, E.; De Filippis, F. Thermodynamic State Investigation of Hypersonic Air Plasma Flow Produced by an Arc-Jet Facility. Aerosp. Sci. Technol. 2012, 23, 358–362. [Google Scholar] [CrossRef]
- Savino, L.; Cinquegrana, D.; French, A.; De Cesare, M. Resolved Optical Emission Spectroscopy as Accurate Physics Methodology for Plasma Freestream Temperature Characterization. J. Quant. Spectrosc. Radiat. Transf. 2022, 291, 108323. [Google Scholar] [CrossRef]
- Driver, D.M.; Sepka, S. Side Arm Reactor Study of Copper Catalysis. In Proceedings of the 45th AIAA Thermophysics Conference, AIAA 2015-2666, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef]
- Vasil’evskii, S.A.; Gordeev, A.N.; Kolesnikov, A.F.; Chaplygin, A.V. Thermal Effect of Surface Catalysis in Subsonic Dissociated-Air Jets. Fluid Dyn. 2020, 55, 708–720. [Google Scholar] [CrossRef]
- Pope, R.B. Stagnation-Point Convective Heat Transfer in Frozen Boundary Layers. In Proceedings of the 6th Aerospace Sciences Meeting, AIAA Paper No. 68-15, New York, NY, USA, 22–24 January1968. [Google Scholar]
- Nawaz, A.; Driver, D.; Terrazas-Salinas, I.; Sepka, S. Surface Catalysis and Oxidation on Stagnation Point Heat Flux Measurements in High Enthalpy Arc Jets. In Proceedings of the 44th AIAA Thermophysics Conference, AIAA, San Diego, CA, USA, 24–27 June 2013. [Google Scholar]
- Kidd, C.T. High Heat-Flux Measurements and Experimental Calibrations/Characterizations; NASA CP 3061; NASA Langley Research Center: Hampton, VA, USA, 1993; pp. 317–335.
- Kidd, C.T.; Nelson, C.G.; Scott, W.T. Extraneous Thermoelectric EMF Effects Resulting from the Press-Fit Installation of Coaxial Surface Thermocouples in Metal Models. In Proceedings of the 40th International Instrumentation Symposium, Baltimore, MD, USA, 1–5 May 1994; Instrument Society of America: Research Triangle Park, NC, USA, 1994; pp. 317–335. [Google Scholar]
- Brune, J.A.; Morrow, C.C. Uncertainty Analysis of Slug Calorimeters in the NASA Hy-METS Arc-Jet Facility. J. Thermophys. Heat Transf. 2024, 38, 232–249. [Google Scholar] [CrossRef]


| [kW/m2] | V [kV] | [kPa] | [kg/s] | [kg/s] | [A] | Energy Balance [MJ/kg] | Sonic Throat [MJ/kg] | Heat Transfer [MJ/kg] | Stagnation [MJ/kg] | |
|---|---|---|---|---|---|---|---|---|---|---|
| Test Case | Experimental | CFD | ||||||||
| Instrumentation Data | Mass Averaged | Centerline | Full Cat. | |||||||
| 1 | 1478 | 7.4 | 3.41 | 0.64 | 0.03 | 2289 | 10.85 | 11.75 | 14.40 | N/A |
| 2 | 2226 | 7.2 | 4.14 | 0.68 | 0.03 | 4045 | 12.48 | 15.92 | 19.69 | N/A |
| 3 | 2639 | 6.8 | 4.46 | 0.68 | 0.03 | 5135 | N/A | 18.35 | 22.49 | 20.30 |
| 4 | 2063 | 7.4 | 3.86 | 0.69 | 0.03 | 3681 | 13.83 | 14.20 | 18.90 | 17.43 |
| 5 | 2178 | 7.4 | 3.96 | 0.68 | 0.03 | 3970 | N/A | 14.71 | N/A | 18.00 |
| 6 | 2107 | 6.4 | 5.00 | 0.90 | 0.03 | 3483 | 12.07 | 13.45 | N/A | N/A |
| 7 | 2107 | 6.5 | 5.00 | 0.90 | 0.03 | 3483 | 12.30 | 12.56 | N/A | N/A |
| 8 | 1440 | 5.3 | 3.73 | 0.62 | 0.03 | 2132 | 10.77 | N/A | N/A | N/A |
| 9 | 1910 | 5.3 | 4.90 | 0.74 | 0.03 | 3012 | 11.03 | 13.57 | N/A | N/A |
| 10 | 2270 | 6.6 | 6.20 | 0.96 | 0.03 | 3636 | 13.14 | 14.54 | N/A | N/A |
| 11 | 2420 | 6.6 | 6.00 | 0.96 | 0.03 | 4060 | 11.24 | 14.54 | N/A | 18.34 |
| 12 | 1227 | 6.6 | 2.79 | 0.48 | 0.04 | 1836 | 9.84 | N/A | 13.08 | 12.81 |
| 13 | 1411 | 6.6 | 3.00 | 0.48 | 0.04 | 2224 | N/A | N/A | N/A | 14.00 |
| 14 | 1752 | 6.2 | 3.24 | 0.48 | 0.04 | 3032 | 11.82 | N/A | N/A | N/A |
| 15 | 1878 | 7.1 | 3.78 | 0.60 | 0.04 | 3204 | N/A | N/A | 17.28 | 16.28 |
| 16 | 1920 | 7.1 | 3.70 | 0.62 | 0.04 | 3340 | N/A | 13.92 | 17.87 | 16.74 |
| 17 | 1743 | 6.8 | 4.04 | 0.62 | 0.04 | 2790 | N/A | N/A | N/A | N/A |
| 18 | 1850 | 6.8 | 4.10 | 0.62 | 0.04 | 3047 | N/A | N/A | N/A | 15.55 |
| 19 | 1940 | 6.7 | 4.14 | 0.62 | 0.04 | 3268 | N/A | N/A | N/A | 16.12 |
| 20 | 2012 | 6.7 | 4.15 | 0.62 | 0.04 | 3453 | N/A | N/A | N/A | 16.59 |
| 21 | 2072 | 6.6 | 4.18 | 0.62 | 0.04 | 3607 | N/A | N/A | N/A | 17.04 |
| 22 | 2164 | 6.6 | 4.13 | 0.62 | 0.04 | 3873 | N/A | N/A | 19.06 | 17.46 |
| 23 | 2181 | 7 | 3.87 | 0.61 | 0.04 | 4009 | N/A | N/A | 19.84 | 18.24 |
| 24 | 2232 | 6.9 | 3.88 | 0.61 | 0.04 | 4152 | N/A | N/A | 20.28 | N/A |
| 25 | 1414 | 6.8 | 3.98 | 0.84 | 0.04 | 3793 | N/A | N/A | N/A | 12.46 |
| 26 | 1860 | 6.8 | 4.50 | 0.84 | 0.04 | 2601 | N/A | N/A | N/A | 14.99 |
| 27 | 2300 | 6.7 | 4.90 | 0.84 | 0.04 | 1802 | N/A | N/A | N/A | 17.33 |
| Quantity | Sensor | Maker/Model | Uncertain | Range | unit |
|---|---|---|---|---|---|
| Voltage | Voltage Divider | N/A | ±1.20% rdg | 0–30,000 | V |
| Electrical current | Hall Effect Sensor | CTL-10000Y03/CTA212H-24, Ohio Semitronics, Hilliard, OH, USA | ±0.10% FS | 0–10,000 | A |
| Air mass flow rate | Coriolis Force Sensor | CMF200, Micromotion, St. Louis, MO, USA | ±0.10% rdg | 0–12.1 | kg/s |
| Argon mass flow rate | Coriolis Force Sensor | CMF205, Micromotion, St. Louis, MO, USA | ±0.18% rdg | 0.001–0.1 | kg/s |
| Water flow rate (arc heater) | Orifice Plate | Venturi tube +ABB 600T, ABB, Zurich, Switzerland | ±0.035% FS | 0–2500 | m3/h |
| Differential temperature (arc heater) | Thermopile | ROSEMOUNT model 3144 D111Q4 Emerson Rosemount, Chanhassen, MN, USA | ±0.318 °C | 0–20 | °C |
| Stagnation pressure | Absolute Pressure Transducer | Validyne P55A, Validyne Engineering Corp, Northridge, CA, USA | ±0.25% rdg | 0–106 | Pa |
| Gas | ||
|---|---|---|
| lbm | g | |
| Air | 0.0461 | 0.1235 |
| Argon | 0.0651 | 0.1744 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smoraldi, A.; Cutrone, L. Comparison Between Numerical and Experimental Methodologies for Total Enthalpy Determination in Scirocco PWT. Aerospace 2024, 11, 1023. https://doi.org/10.3390/aerospace11121023
Smoraldi A, Cutrone L. Comparison Between Numerical and Experimental Methodologies for Total Enthalpy Determination in Scirocco PWT. Aerospace. 2024; 11(12):1023. https://doi.org/10.3390/aerospace11121023
Chicago/Turabian StyleSmoraldi, Antonio, and Luigi Cutrone. 2024. "Comparison Between Numerical and Experimental Methodologies for Total Enthalpy Determination in Scirocco PWT" Aerospace 11, no. 12: 1023. https://doi.org/10.3390/aerospace11121023
APA StyleSmoraldi, A., & Cutrone, L. (2024). Comparison Between Numerical and Experimental Methodologies for Total Enthalpy Determination in Scirocco PWT. Aerospace, 11(12), 1023. https://doi.org/10.3390/aerospace11121023






